Perceptions of Lecturers and Engineering Students of Sophism and Paradox: The Case of Differential Equations
Abstract
1. Introduction
2. Theoretical Background and Relevant Literature
2.1. Problem-Based Learning
2.2. Puzzle-Based Learning
2.3. Students’ Attitudes toward and Perceptions of Mathematics and PzBL
2.4. Teaching and Learning of Differential Equations
3. Methods
3.1. Data Collection
3.2. The Instruments
3.2.1. The Questionnaire
3.2.2. Interviews
3.3. Data Analysis
3.4. Reliability and Validity
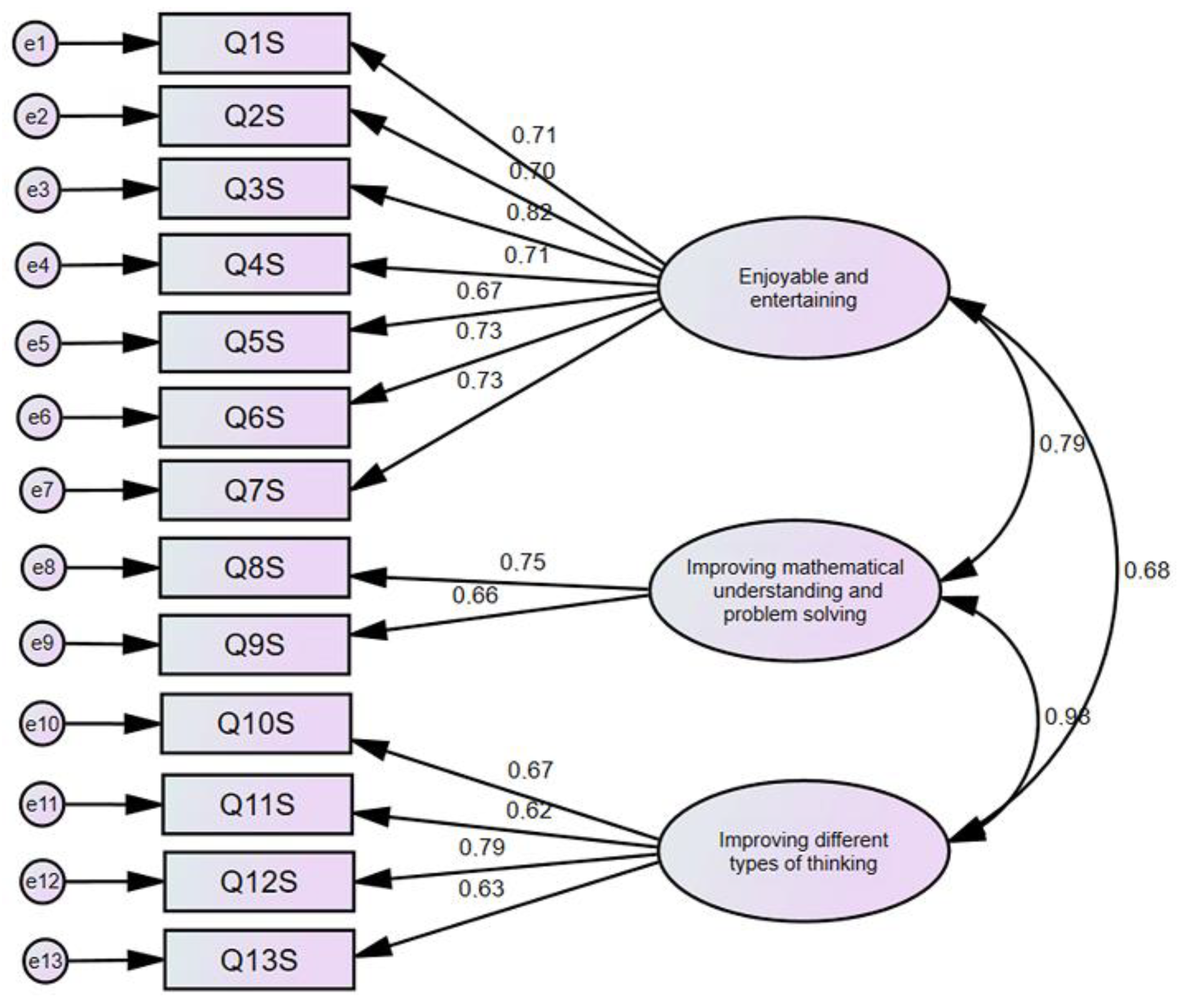
4. Results
4.1. The Questionnaire Results
4.1.1. Enjoyable and Entertaining Activities
4.1.2. Improving Mathematical Understanding and Problem-Solving Skills
4.1.3. Improving Different Types of Thinking
4.2. The Interview Results
Advantages and Disadvantages of SoPa Tasks
Many lecturers only focus on routine problems and how they can be solved. It is like you are on the road, and you just look straight ahead without paying attention to your surroundings. In my opinion, these types of tasks are like roadside which can help us to show students how fascinating it is that the concepts are related to each other… (T3).
Depending on the characteristics of students, some are interested in solving sophism and paradox, and some are not. Those who want to master the topic are interested in solving them, and those who just focus on passing the course are not interested (H2).
Students need to evaluate all information and reasoning given in the task to verify or refute the reasoning in the task. In my opinion, engaging in these tasks can motivate students to follow the DEs topics with more interest. Additionally, sophism and paradox tasks are very useful for evaluating dissertations and articles. For example, sometimes we could find invalid reasoning in a published article, while the reasoning seems apparently true in the first read… (T16).
4.3. How SoPa Could Be Included in the Teaching of DEs
SoPa tasks can be used in classrooms along with routine problems. They lead to deep mathematical understanding and more attention to detail. Solving SoPa tasks helps students to develop their critical thinking, and they will learn not to accept anything without reason (M3).
I believe these problems should be included from the primary level in order to help students develop their creative thinking and mathematical understanding (L2).
It is better that first, the lecturer solves a few examples of SoPa tasks in the lecture to help students become familiar with such tasks. Then, these types of tasks can be given to students to solve in the lecture to increase students’ participation. The lecturer should manage the lecture environment in a way that students feel safe to share their thoughts… I prefer to use these tasks in the lecture to have better control over students’ thinking processes (T1).
SoPa tasks should be given to students as homework assignments in order to give students enough time to think about how they can solve them; then, students could share their solutions in tutorials.
4.4. Using SoPa Tasks in Assessments
SoPa tasks are not suitable for assessments because solving them requires creativity and considering the problem from different angles. Only students who learned the lessons deeply are capable of solving them. Consequently, many students will fail to solve such problems and become disappointed about learning mathematics (L4).
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
For example, a DE | |
| (A1) | |
| is exact because If we factor out in (A1) and then eliminate it, we have, respectively, | |
| and | |
| . | (A2) |
| The DE (A2) is still exact because Consider now a DE | |
| . | (A3) |
| Equation (A3) is not exact because | |
| If we factor out in (A3) and eliminate it, we have, respectively, | |
| and | |
| . | (A4) |
| This new DE (A4) is also not exact because | |
Thus, factoring out and eliminating a common factor does not impact the exactness of a DE.
| |
| (A5) | |
| can be solved with an integrating factor. Reza and Ali separately solved Equation (A5) for him. Based on their responses, Ehsan concluded that this DE has two integrating factors and both functions defined implicitly by equations and are general solutions. Is this possible? Justify your answer. Reza’s solution: | |
| Now, we multiply the DE by the integrating factor, and the new DE | |
| (A6) | |
| is exact because We can solve (A6) using the standard method: | |
| Differentiation with respect to yields | |
| and we set . Therefore, and is the general solution of the given DE. Ali’s solution: | |
| Multiply (A5) by the integrating factor, then the new DE | |
| (A7) | |
| is exact because We solve (A7) using the standard method: | |
| Differentiate the result with respect to | |
| and we set . Therefore, and is the general solution of the given DE. | |
References
- Winberg, C.; Adendorff, H.; Bozalek, V.; Conana, H.; Pallitt, N.; Wolff, K.; Olsson, T.; Roxå, T. Learning to teach STEM disciplines in higher education: A critical review of the literature. Teach. High. Educ. 2019, 24, 930–947. [Google Scholar] [CrossRef]
- Falkner, N.; Sooriamurthi, R.; Michalewicz, Z. Teaching Puzzle-based Learning: Development of Transferable Skills. J. Teach. Math. Comput. Sci. 2012, 10, 245–268. [Google Scholar] [CrossRef]
- Michalewicz, Z.; Michalewicz, M. Puzzle-Based Learning; Hybrid Publishers: Melbourne, VIC, Australia, 2008. [Google Scholar]
- Freeman, S.; Eddy, S.; McDonough, M.; Smith, M.; Okoroafor, N.; Jordt, H.; Wenderoth, M. Active learning increases student performance in science, engineering, and mathematics. Proc. Natl. Acad. Sci. USA 2014, 111, 8410–8415. [Google Scholar] [CrossRef]
- Lugosi, E.; Uribe, G. Active learning strategies with positive effects on students’ achievements in undergraduate mathematics education. Int. J. Math. Educ. Sci. Technol. 2022, 53, 403–424. [Google Scholar] [CrossRef]
- Lambros, A. Problem-Based Learning in K-8 Classrooms: A Teacher’s Guide to Implementation; Corvin Press, Inc.: Thousand Oaks, CA, USA, 2002. [Google Scholar]
- Klymchuk, S. Puzzle-based learning in engineering mathematics: Students’ attitudes. Int. J. Math. Educ. Sci. Technol. 2017, 48, 1106–1119. [Google Scholar] [CrossRef]
- Thomas, C.; Badger, M.; Ventura-Medina, E.; Sangwin, C. Puzzle-based learning of mathematics in engineering. Eng. Educ. 2013, 8, 122–134. [Google Scholar] [CrossRef]
- Arslan, S. Traditional instruction of differential equations and conceptual learning. Teach. Math. Its Appl. Int. J. IMA 2010, 29, 94–107. [Google Scholar] [CrossRef]
- Rasmussen, C.L. New directions in differential equations: A framework for interpreting students’ understandings and difficulties. J. Math. Behav. 2001, 20, 55–87. [Google Scholar] [CrossRef]
- Klymchuk, S.; Staples, S.G. Paradoxes and Sophisms in Calculus; MAA: Washington, DC, USA, 2013; Volume 45. [Google Scholar]
- Yew, E.H.; Goh, K. Problem-based learning: An overview of its process and impact on learning. Health Prof. Educ. 2016, 2, 75–79. [Google Scholar] [CrossRef]
- Barrows, H.S. Problem-based learning in medicine and beyond: A brief overview. New Dir. Teach. Learn. 1996, 68, 3–12. [Google Scholar] [CrossRef]
- Hmelo-Silver, C.E. Problem-based learning: What and how do students learn? Educ. Psychol. Rev. 2004, 16, 235–266. [Google Scholar] [CrossRef]
- Capon, N.; Kuhn, D. What’s so good about problem-based learning? Cogn. Instr. 2004, 22, 61–79. [Google Scholar] [CrossRef]
- Dochy, F.; Segers, M.; Van den Bossche, P.; Gijbels, D. Effects of problem-based learning: A meta-analysis. Learn. Instr. 2003, 13, 533–568. [Google Scholar] [CrossRef]
- Michalewicz, Z.; Falkner, N.; Sooriamurthi, R. Puzzle-based learning: An introduction to critical thinking and problem solving. Decis. Line 2011, 42, 6–9. [Google Scholar]
- Radmehr, F.; Vos, P. Issues and challenges for 21st century assessment in mathematics education. In Science and Mathematics Education for 21st Century Citizens: Challenges and Ways Forwards; Leite, L., Oldham, E., Afonso, A.S., Viseu, F., Dourado, L., Martinho, H., Eds.; Nova Science Publishers: New York, NY, USA, 2020; pp. 437–462. [Google Scholar]
- Falkner, N.; Sooriamurthi, R.; Michalewicz, Z. Puzzle-Based Learning for Engineering and Computer Science. IEEE Comput. 2010, 43, 20–28. [Google Scholar] [CrossRef]
- Parhami, B. A puzzle-based seminar for computer engineering freshmen. Comput. Sci. Educ. 2008, 18, 261–277. [Google Scholar] [CrossRef]
- Ramalingam, D.; Anderson, P.; Duckworth, D.; Scoular, C.; Heard, J. Creative Thinking: Definition and Structure; The Australian Council for Educational Research: Camberwell, VIC, Australia, 2020. [Google Scholar]
- Maričića, S.; Špijunović, K. Developing Critical Thinking in Elementary Mathematics Education through a Suitable Selection of Content and Overall Student Performance. Procedia—Soc. Behav. Sci. 2020, 180, 653–659. [Google Scholar] [CrossRef]
- Aydin, I.E. Attitudes toward online communications in open and distance learning. Turk. Online J. Distance Educ. 2012, 13, 333–346. [Google Scholar]
- Pickens, J. Attitudes and perceptions. Organ. Behav. Health Care 2005, 4, 43–76. [Google Scholar]
- Nedaei, M.; Radmehr, F.; Drake, M. Exploring engineering undergraduate students’ attitudes toward mathematical problem posing. J. Prof. Issues Eng. Educ. Pract. 2019, 145, 04019009. [Google Scholar] [CrossRef]
- Sarouphim, K.M.; Chartouny, M. Mathematics education in Lebanon: Gender differences in attitudes and achievement. Educ. Stud. Math. 2017, 94, 55–68. [Google Scholar] [CrossRef]
- Byers, T.; Imms, W.; Hartnell-Young, E. Comparative analysis of the impact of traditional versus innovative learning environment on student attitudes and learning outcomes. Stud. Educ. Eval. 2018, 58, 167–177. [Google Scholar] [CrossRef]
- Ellis, J.; Kelton, M.L.; Rasmussen, C. Student perceptions of pedagogy and associated persistence in calculus. ZDM 2014, 46, 661–673. [Google Scholar] [CrossRef]
- Attard, C. Engagement with Mathematics: What Does It Mean and What Does It Look Like? Aust. Prim. Math. Classr. 2012, 17, 9–13. [Google Scholar]
- Flegg, J.; Mallet, D.; Lupton, M. Students’ perceptions of the relevance of mathematics in engineering. Int. J. Math. Educ. Sci. Technol. 2012, 43, 717–732. [Google Scholar] [CrossRef]
- Perdigones Borderias, A.; Gallego Vazquez, E.; Garcia Garcia, M.N.; Fernandez Alvarez, P.; Perez Martin, E.; Cerro Carrascosa, J.D. Physics and mathematics in the engineering curriculum: Correlation with applied subjects. Int. J. Eng. Educ. 2014, 30, 1509–1521. [Google Scholar]
- Hamzeh, E. Lebanese Middle School Students’ Attitudes toward Mathematics as a Subject and toward Mathematics Teachers. Unpublished. Master’s Thesis, Lebanese American University, Beirut, Lebanon, 2009. [Google Scholar]
- Klingler, K.L. Mathematic Strategies for Teaching Problem Solving: The Influence of Teaching Mathematical Problem Solving Strategies on Students’ Attitudes in Middle School. Un-published. Master’s Thesis, Central Florida University, Orlando, FL, USA, 2012. [Google Scholar]
- Merrick, K.E. An empirical evaluation of puzzle-based learning as an interest approach for teaching introductory computer science. IEEE Trans. Educ. 2010, 53, 677–680. [Google Scholar] [CrossRef]
- Czocher, J.A. How can emphasizing mathematical modeling principles benefit students in a traditionally taught differential equations course? J. Math. Behav. 2017, 45, 78–94. [Google Scholar] [CrossRef]
- Keene, K.A. A characterization of dynamic reasoning: Reasoning with time as parameter. J. Math. Behav. 2007, 26, 230–246. [Google Scholar] [CrossRef]
- Kwon, O.N.; Rasmussen, C.; Allen, K. Students’ retention of mathematical knowledge and skills in differential equations. Sch. Sci. Math. 2005, 105, 227–239. [Google Scholar] [CrossRef]
- Beier, J.C.; Gevertz, J.L.; Howard, K.E. Building context with tumor growth modeling projects in differential equations. PRIMUS 2015, 25, 297–325. [Google Scholar] [CrossRef]
- Maat, S.M.; Zakaria, E. Exploring Students’ Understanding of Ordinary Differential Equations Using Computer Algebraic System (CAS). Turk. Online J. Educ. Technol.-TOJET 2011, 10, 123–128. [Google Scholar]
- Creswell, J. Research Design: Qualitative, Quantitative, and Mixed Methods Approaches, 4th ed.; SAGE Publication Inc.: Thousand Oaks, CA, USA, 2014. [Google Scholar]
- Reiter, B. Theory and methodology of exploratory social science research. Int. J. Sci. Res. Methodol. 2017, 5, 129–150. [Google Scholar]
- Badger, M.; Sangwin, C.; Ventura-Medina, E.; Thomas, C. A Guide to Puzzle-Based Learning in STEM Subjects. Available online: https://www.maths.ed.ac.uk/~csangwin/Publications/GuideToPuzzleBasedLearningInSTEM.pdf (accessed on 19 March 2023).
- McDonald, J.H. Handbook of Biological Statistics; Sparky House Publishing: Baltimore, MD, USA, 2009; Volume 2, pp. 6–59. [Google Scholar]
- Kennedy, B.L.; Thornberg, R. Deduction, Induction, and Abduction. In The SAGE Handbook of Qualitative Data Collection; Flick, U., Ed.; SAGE Publications Ltd.: Thousand Oaks, CA, USA, 2018; pp. 49–64. [Google Scholar]
- Vaismoradi, M.; Turunen, H.; Bondas, T. Content analysis and thematic analysis: Implications for conducting a qualitative descriptive study. Nurs. Health Sci. 2013, 15, 398–405. [Google Scholar] [CrossRef] [PubMed]
- Bolarinwa, O.A. Principles and methods of validity and reliability testing of questionnaires used in social and health science researches. Niger. Postgrad. Med. J. 2015, 22, 195–201. [Google Scholar] [CrossRef]
- Lei, P.W.; Wu, Q. Introduction to structural equation modeling: Issues and practical considerations. Educ. Meas. Issues Pract. 2007, 26, 33–43. [Google Scholar] [CrossRef]
- Hu, L.T.; Bentler, P.M. Cutoff criteria for fit indexes in covariance structure analysis: Conventional criteria versus new alternatives. Struct. Equ. Model. A Multidiscip. J. 1999, 6, 1–55. [Google Scholar] [CrossRef]
- Browne, M.W.; Cudeck, R. Alternative ways of assessing model fit. In Testing Structural Equation Models; Bollen, K.A., Long, J.S., Eds.; Sage: Newbury Park, CA, USA, 1993; pp. 136–162. [Google Scholar]
- Kilic, A.F.; Doğan, N. Comparison of confirmatory factor analysis estimation methods on mixed-format data. Int. J. Assess. Tools Educ. 2021, 8, 21–37. [Google Scholar] [CrossRef]
- Boudreau, M.C.; Gefen, D.; Straub, D.W. Validation in information systems research: A state-of-the-art assessment. MIS Q. 2001, 25, 1–16. [Google Scholar] [CrossRef]
- Tracy, S.J. Qualitative quality: Eight “big-tent” criteria for excellent qualitative research. Qual. Inq. 2010, 16, 837–851. [Google Scholar] [CrossRef]
- Rezvanifard, F.; Radmehr, F.; Rogovchenko, Y. Advancing engineering students’ conceptual understanding through puzzle-based learning: A case study with exact differential equations. Math. Its Appl. Int. J. IMA 2022, 1–24. [Google Scholar] [CrossRef]
- Ramirez, G.; Shaw, S.T.; Maloney, E.A. Math anxiety: Past research, promising interventions, and a new interpretation framework. Educ. Psychol. 2018, 53, 145–164. [Google Scholar] [CrossRef]
- Parhami, B. Motivating computer engineering freshmen through mathematical and logical puzzles. IEEE Trans. Educ. 2009, 52, 360–364, Appendix: The sophism and paradox. [Google Scholar] [CrossRef]

| Student Label | Gender | Performance in Solving the SoPa Tasks | Perception of SoPa Tasks |
|---|---|---|---|
| H1 | Female | High | P |
| H2 | Male | High | P |
| H3 | Male | High | P |
| H4 | Male | High | P |
| M1 | Female | Medium | P |
| M2 | Female | Medium | N |
| M3 | Female | Medium | N |
| M4 | Male | Medium | P |
| M5 | Male | Medium | N |
| L1 | Female | Low | P |
| L2 | Female | Low | N |
| L3 | Female | Low | P |
| L4 | Male | Low | N |
| Lecturer Code | Qualification | Years of Teaching DEs | Gender |
|---|---|---|---|
| T1 | PhD in applied mathematics—optimization | 20 | Male |
| T2 | PhD in applied mathematics—numerical analysis | 19 | Male |
| T3 | PhD in applied mathematics—numerical analysis | 19 | Male |
| T4 | PhD in statistics | 15 | Female |
| T5 | PhD in applied mathematics—numerical analysis | 15 | Male |
| T6 | PhD in pure mathematics—group theory | 10 | Male |
| T7 | PhD in applied mathematics—differential equations | 10 | Female |
| T8 | PhD in applied mathematics—numerical analysis | 10 | Male |
| T9 | PhD in applied mathematics—differential equations | 8 | Female |
| T10 | PhD in applied mathematics—numerical analysis | 7 | Female |
| T11 | PhD in pure mathematics—algebraic graphs and combinatorics | 6 | Male |
| T12 | PhD in applied mathematics—control and optimization | 5 | Male |
| T13 | PhD in applied mathematics—dynamic systems and geometric theories | 5 | Male |
| T14 | PhD in applied mathematics—control and optimization | 3 | Male |
| T15 | PhD in pure mathematics—algebraic graphs and combinatorics | 3 | Female |
| T16 | PhD in applied mathematics—optimization | 2 | Male |
| T17 | PhD in applied mathematics—numerical analysis | 2 | Female |
| Themes | Items |
|---|---|
| Enjoyable and entertaining activities | 1. The use of sophism/paradox tasks in the teaching of DEs makes the teaching entertaining and enjoyable. |
| 2. Sophism/paradox tasks are enjoyable and entertaining activities. | |
| 3. Students can learn DEs in an entertaining way by solving sophism/paradox tasks. | |
| 4. Students’ curiosity can be increased by solving sophism/paradox tasks. | |
| 5. The use of sophism/paradox tasks in teaching DEs increases students’ participation in the classroom. | |
| 6. The moment of discovering the correct solution to a sophism/paradox task is very enjoyable. | |
| 7. Solving sophism/paradox tasks increases students’ motivation to learn DEs. | |
| Improving mathematical understanding and problem-solving skills | 8. Engaging in solving sophism/paradox tasks improves students’ problem-solving skills. |
| 9. The use of sophism/paradox tasks in teaching DEs improves students’ conceptual understanding of DEs. | |
| Improving different types of thinking | 10. To solve a sophism/paradox task, students should consider the problem from different angles. |
| 11. Engaging in solving sophism/paradox tasks improves students’ critical thinking skills. | |
| 12. Engaging in solving sophism/paradox tasks improves students’ creative thinking skills. | |
| 13. Engaging in solving sophism/paradox tasks leads students to analyze other DEs problems from different angles as well. |
| Chi-Square | CFI | RMSEA | |
|---|---|---|---|
| Sophism | 93.835 | 0.9 | 0.06 |
| Paradox | 105.200 | 0.9 | 0.07 |
| Themes | Items | Type | S/L * | Strongly Disagree | Disagree | Nor Agree or Disagree | Agree | Strongly Agree | p-Value So vs. Pa (S) | p-Value So vs. Pa (L) | p-Value Sophism (L vs. S) | p-Value Paradox (L vs. S) | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| N | % | N | % | N | % | N | % | N | % | ||||||||
| Enjoyable and entertaining activities | 1 | Sophism | S | 14 | 10.4 | 14 | 10.4 | 27 | 20.1 | 46 | 34.3 | 33 | 24.6 | 0.525 | 0.524 | 0.086 | 0.942 |
| L | 0 | 0 | 1 | 5.9 | 1 | 5.9 | 12 | 70.6 | 3 | 17.6 | |||||||
| Paradox | S | 8 | 6 | 8 | 6 | 36 | 26.9 | 50 | 37.3 | 32 | 23.9 | ||||||
| L | 1 | 5.9 | 1 | 5.9 | 4 | 23.5 | 8 | 47.1 | 3 | 17.6 | |||||||
| 2 | Sophism | S | 11 | 8.2 | 26 | 19.4 | 22 | 16.4 | 48 | 35.8 | 27 | 20.1 | 0.093 | 0.950 | 0.391 | 0.114 | |
| L | 0 | 0 | 2 | 11.8 | 2 | 11.8 | 6 | 35.3 | 7 | 41.2 | |||||||
| Paradox | S | 6 | 4.5 | 13 | 9.7 | 30 | 22.4 | 58 | 43.3 | 27 | 20.1 | ||||||
| L | 1 | 5.9 | 2 | 11.8 | 2 | 11.8 | 4 | 23.5 | 8 | 47.1 | |||||||
| 3 | Sophism | S | 16 | 11.9 | 11 | 8.2 | 31 | 23.1 | 29 | 21.6 | 47 | 35.1 | 0.026 ** | 1.000 | 0.343 | 0.189 | |
| L | 1 | 5.9 | 1 | 5.9 | 1 | 5.9 | 6 | 35.3 | 8 | 47.1 | |||||||
| Paradox | S | 4 | 3 | 15 | 11.2 | 25 | 18.7 | 42 | 31.3 | 48 | 35.8 | ||||||
| L | 1 | 5.9 | 1 | 5.9 | 0 | 0 | 7 | 41.2 | 8 | 47.1 | |||||||
| 4 | Sophism | S | 9 | 6.7 | 21 | 15.7 | 28 | 20.9 | 33 | 24.6 | 43 | 32.1 | 0.140 | 0.642 | 0.046 ** | 0.737 | |
| L | 0 | 0 | 0 | 0 | 2 | 11.8 | 10 | 58.8 | 5 | 29.4 | |||||||
| Paradox | S | 4 | 3 | 13 | 9.7 | 27 | 20.1 | 49 | 36.6 | 41 | 30.6 | ||||||
| L | 0 | 0 | 0 | 0 | 3 | 17.6 | 7 | 41.2 | 7 | 41.2 | |||||||
| 5 | Sophism | S | 9 | 6.7 | 12 | 9 | 22 | 16.4 | 27 | 20.1 | 64 | 47.8 | 0.862 | 0.925 | 0.066 | 0.257 | |
| L | 0 | 0 | 0 | 0 | 5 | 29.4 | 7 | 41.2 | 4 | 23.5 | |||||||
| Paradox | S | 7 | 5.2 | 11 | 8.2 | 17 | 12.7 | 31 | 23.1 | 68 | 50.7 | ||||||
| L | 1 | 5.9 | 1 | 5.9 | 5 | 29.4 | 5 | 29.4 | 5 | 29.4 | |||||||
| 6 | Sophism | S | 11 | 8.2 | 12 | 9 | 36 | 26.9 | 39 | 29.1 | 36 | 26.9 | 0.795 | 0.733 | 0.641 | 0.374 | |
| L | 1 | 5.9 | 1 | 5.9 | 6 | 35.3 | 7 | 51.2 | 2 | 11.8 | |||||||
| Paradox | S | 8 | 6 | 8 | 6 | 37 | 27.6 | 45 | 33.6 | 36 | 26.9 | ||||||
| L | 0 | 0 | 3 | 17.6 | 4 | 23.5 | 7 | 41.2 | 3 | 17.6 | |||||||
| 7 | Sophism | S | 11 | 8.2 | 12 | 9 | 33 | 24.6 | 42 | 31.3 | 36 | 26.9 | 0.624 | 1.000 | 0.465 | 0.887 | |
| L | 0 | 0 | 1 | 5.9 | 2 | 11.8 | 7 | 41.2 | 7 | 41.2 | |||||||
| Paradox | S | 7 | 5.2 | 9 | 6.7 | 29 | 21.6 | 52 | 38.8 | 37 | 27.6 | ||||||
| L | 0 | 0 | 1 | 5.9 | 3 | 17.6 | 6 | 35.3 | 7 | 41.2 | |||||||
| Improving mathematical understanding and problem-solving skills | 8 | Sophism | S | 10 | 7.5 | 6 | 4.5 | 28 | 20.9 | 43 | 32.1 | 47 | 35.1 | 0.168 | 1.000 | 0.579 | 0.335 |
| L | 1 | 5.9 | 1 | 5.9 | 1 | 5.9 | 7 | 41.2 | 7 | 41.2 | |||||||
| Paradox | S | 2 | 1.5 | 9 | 6.7 | 27 | 20.1 | 42 | 31.3 | 54 | 40.3 | ||||||
| L | 1 | 5.9 | 1 | 5.9 | 1 | 5.9 | 7 | 41.2 | 7 | 41.2 | |||||||
| 9 | Sophism | S | 6 | 4.5 | 15 | 11.2 | 28 | 20.9 | 37 | 27.6 | 48 | 35.8 | 0.700 | 0.895 | 0.807 | 0.921 | |
| L | 0 | 0 | 1 | 5.9 | 3 | 17.6 | 4 | 23.5 | 9 | 52.9 | |||||||
| Paradox | S | 7 | 5.2 | 9 | 6.7 | 25 | 18.7 | 43 | 32.1 | 50 | 37.3 | ||||||
| L | 0 | 0 | 1 | 5.9 | 2 | 11.8 | 6 | 35.3 | 8 | 47.1 | |||||||
| Improving different types of thinking | 10 | Sophism | S | 6 | 4.5 | 11 | 8.2 | 28 | 20.9 | 39 | 29.1 | 50 | 37.3 | 0.757 | 0.706 | 0.669 | 0.060 |
| L | 0 | 0 | 1 | 5.9 | 2 | 11.8 | 8 | 47.1 | 6 | 35.3 | |||||||
| Paradox | S | 4 | 3 | 7 | 5.2 | 32 | 23.9 | 44 | 32.8 | 47 | 35.1 | ||||||
| L | 0 | 0 | 2 | 11.8 | 0 | 0 | 9 | 52.9 | 6 | 35.3 | |||||||
| 11 | Sophism | S | 8 | 6 | 7 | 5.2 | 22 | 16.4 | 33 | 24.6 | 64 | 47.8 | 0.140 | 0.484 | 0.068 | 0.245 | |
| L | 0 | 0 | 0 | 0 | 1 | 5.9 | 1 | 5.9 | 15 | 88.2 | |||||||
| Paradox | S | 3 | 2.2 | 16 | 11.9 | 27 | 20.1 | 33 | 24.6 | 55 | 41 | ||||||
| L | 0 | 0 | 0 | 0 | 2 | 11.8 | 3 | 17.6 | 12 | 70.6 | |||||||
| 12 | Sophism | S | 7 | 5.2 | 9 | 6.7 | 19 | 14.2 | 49 | 36.6 | 50 | 37.3 | 0.988 | 0.884 | 0.883 | 0.903 | |
| L | 0 | 0 | 0 | 0 | 2 | 11.8 | 8 | 47.1 | 7 | 51.2 | |||||||
| Paradox | S | 5 | 3.7 | 9 | 6.7 | 19 | 14.2 | 51 | 38.1 | 50 | 37.3 | ||||||
| L | 0 | 0 | 1 | 5.9 | 2 | 11.8 | 9 | 52.9 | 5 | 29.4 | |||||||
| 13 | Sophism | S | 7 | 5.2 | 11 | 8.2 | 20 | 14.9 | 44 | 32.8 | 52 | 38.8 | 0.990 | 1.000 | 0.526 | 0.495 | |
| L | 0 | 0 | 0 | 0 | 4 | 23.5 | 4 | 23.5 | 9 | 52.9 | |||||||
| Paradox | S | 7 | 5.2 | 9 | 6.7 | 19 | 14.2 | 47 | 35.1 | 52 | 38.8 | ||||||
| L | 0 | 0 | 0 | 0 | 4 | 23.5 | 4 | 23.5 | 9 | 52.9 | |||||||
| Themes | Sub-Themes | S/L | So | Pa | A Sample Response |
|---|---|---|---|---|---|
| Enjoyable and entertaining activities | Entertaining and enjoyable | S | 1 | 2 | “Solving sophism and paradox tasks are enjoyable because students can come up with a correct solution themselves related to their current knowledge. Additionally, it is a nice break during a lecture” (L1). |
| L | 14 | 14 | “Solving sophism motivate students, even the lazy ones…when students are asked to find a mistake, everyone is automatically interested in finding the invalid reasoning. It creates a competitive and enjoyable atmosphere in the lecture” (T16). | ||
| Engaging students’ minds | S | 3 | 3 | “Paradoxes and sophisms challenge students’ mathematical knowledge and encourage them to improve their mathematical understanding” (M2). | |
| L | 8 | 7 | “Sophisms and paradoxes are very interesting problems. The nature of these problems arouses students’ curiosity and engage students to find the correct solution” (T3). | ||
| Increasing students’ participation | S | 0 | 0 | ||
| L | 2 | 2 | “Using sophisms and paradoxes in the classroom increases the interaction between the lecturer and students” (T5). | ||
| Increasing students’ motivation to learn mathematics and solve mathematical problems | S | 1 | 0 | “Sophism break the monotony of classwork and might increase students’ interest in solving problems” (M1). | |
| L | 3 | 3 | “Some students found DEs lectures boring. These problems can motivate students to learn DEs and participate in classroom discussions” (T8). | ||
| Improving mathematical understanding and problem-solving skills | Improving students’ mathematical understanding | S | 11 | 7 | “Sophisms and paradoxes help students to become better problem-solvers… These tasks promote deep mathematical understanding” (L3). |
| L | 11 | 11 | “Sophism and paradox tasks are beneficial to use in teaching. If a student can refute a false statement, he/she has good knowledge of the topic. To do so, students need to consider different theorems simultaneously. This helps them to develop a meaningful understanding of DEs concepts” (T5). | ||
| Increasing students’ ability to solve real-world problems | S | 3 | 2 | “In the real world, sometimes engineers need to pay close attention to details, find an error in a system, or design a new model. All of these could be improved by solving sophisms” (H1). | |
| L | 7 | 8 | “These tasks can help students to solve real-world problems as prepare them to make decisions based on logic. They learn not to make decisions based on the appearance of the problem” (T9). | ||
| Improving students’ problem-solving skills | S | 1 | 2 | “By solving sophisms and paradoxes, students become familiar with new strategies and skills that can be used for solving mathematical problems; therefore, their problem-solving skills can be improved” (H3). | |
| L | 10 | 10 | “They are effective in increasing students’ problem-solving skills. Students can learn DEs conceptually since they should examine the problems from different perspectives. These tasks enable students to develop new skills and strategies to solve other mathematical problems” (T13). | ||
| Increasing the opportunities for sustainable mathematical learning | S | 3 | 1 | “To solve sophisms, students need to find relationships between different concepts. They find a solution themselves that makes the learning more sustainable for them” (M5). | |
| L | 0 | 0 | |||
| Reducing students’ mathematical misunderstanding | S | 2 | 2 | “Students might identify their misunderstandings by solving sophisms and paradoxes” (L1). | |
| L | 2 | 2 | “Students realize their misunderstandings by solving sophism and paradox tasks because they examine the reasoning in the task several times and their accuracy would be increased” (T17). | ||
| Improving different types of thinking | Improving creativity | S | 1 | 2 | “Solving a paradox requires creativity. We need to identify relationships between different mathematical concepts to find a suitable approach” (M4). |
| L | 9 | 9 | “Sophisms should be used in the classroom to cultivate thinking of engineers who play an important role in society. It could increase creativity …” (T10). | ||
| Improving critical thinking skills | S | 6 | 8 | “To solve paradoxes and sophisms correctly, students need to critique them. They need to consider all possibilities and different aspects of the given problem” (H2). | |
| L | 16 | 15 | “Sophism and paradox tasks improve students’ critical thinking. They need to give a reason for their judgment. I believe these tasks provide an opportunity for students to discover the relationships between mathematical concept(s)” (T1). | ||
| Improving lateral thinking (thinking outside the box) | S | 2 | 0 | “Sophisms motivate students to look at the problems from different angles and use different approaches to solve them” (L1). | |
| L | 8 | 8 | “Sophisms and paradoxes challenge the mind, relate to various mathematical remarks, and require reasoning. Students should scrutinize the problem and look at the problem from different angles to evaluate the reasoning in the task” (T6). |
| Themes | S\L | So | Pa | A Sample Response |
|---|---|---|---|---|
| Possibility of creating a mathematical misunderstanding or distracting students from learning mathematics | S | 4 | 1 | “If a student could not identify the wrong argument in a sophism, it could create a mathematical misunderstanding for the student” (L1). |
| L | 5 | 4 | “If lecturers and students pay too much attention to sophism and paradox tasks, students may think that each task that they engage with has a trick and distract them from learning mathematics” (T8). | |
| Lack of experience in solving SoPa tasks | S | 2 | 2 | “The teaching in our class is based on routine problems. Students do not have enough experience solving paradoxes, so there is a high possibility that students do not perform well in solving paradox tasks” (M2). |
| L | 6 | 6 | “Students do not have enough experience in solving sophism and paradox tasks. Therefore, students’ grades and their motivation to learn may decrease” (T15). | |
| Time-consuming activities | S | 0 | 1 | “Finding the starting point for solving paradox tasks takes too much time” (M3). |
| L | 9 | 9 | “Using these tasks is time-consuming. It can be used as long as we have the time to deal with these tasks in the classroom because it requires more discussion in the classroom” (T6). | |
| Not appropriate for engineering students | S | 1 | 0 | “Sophisms are not appropriate for engineering students because in the problems we encounter in engineering, students can solve the problems with routine algorithms. …. I prefer to solve routine problems because I do not like challenging questions” (L4). |
| L | 0 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rezvanifard, F.; Radmehr, F.; Drake, M. Perceptions of Lecturers and Engineering Students of Sophism and Paradox: The Case of Differential Equations. Educ. Sci. 2023, 13, 354. https://doi.org/10.3390/educsci13040354
Rezvanifard F, Radmehr F, Drake M. Perceptions of Lecturers and Engineering Students of Sophism and Paradox: The Case of Differential Equations. Education Sciences. 2023; 13(4):354. https://doi.org/10.3390/educsci13040354
Chicago/Turabian StyleRezvanifard, Faezeh, Farzad Radmehr, and Michael Drake. 2023. "Perceptions of Lecturers and Engineering Students of Sophism and Paradox: The Case of Differential Equations" Education Sciences 13, no. 4: 354. https://doi.org/10.3390/educsci13040354
APA StyleRezvanifard, F., Radmehr, F., & Drake, M. (2023). Perceptions of Lecturers and Engineering Students of Sophism and Paradox: The Case of Differential Equations. Education Sciences, 13(4), 354. https://doi.org/10.3390/educsci13040354







