Modeling Cylindrical Inhomogeneity of Finite Length with Steigmann–Ogden Interface
Abstract
1. Introduction
2. Energy-Equivalent Short Cylindrical Fiber with Steigmann–Ogden Surface Model
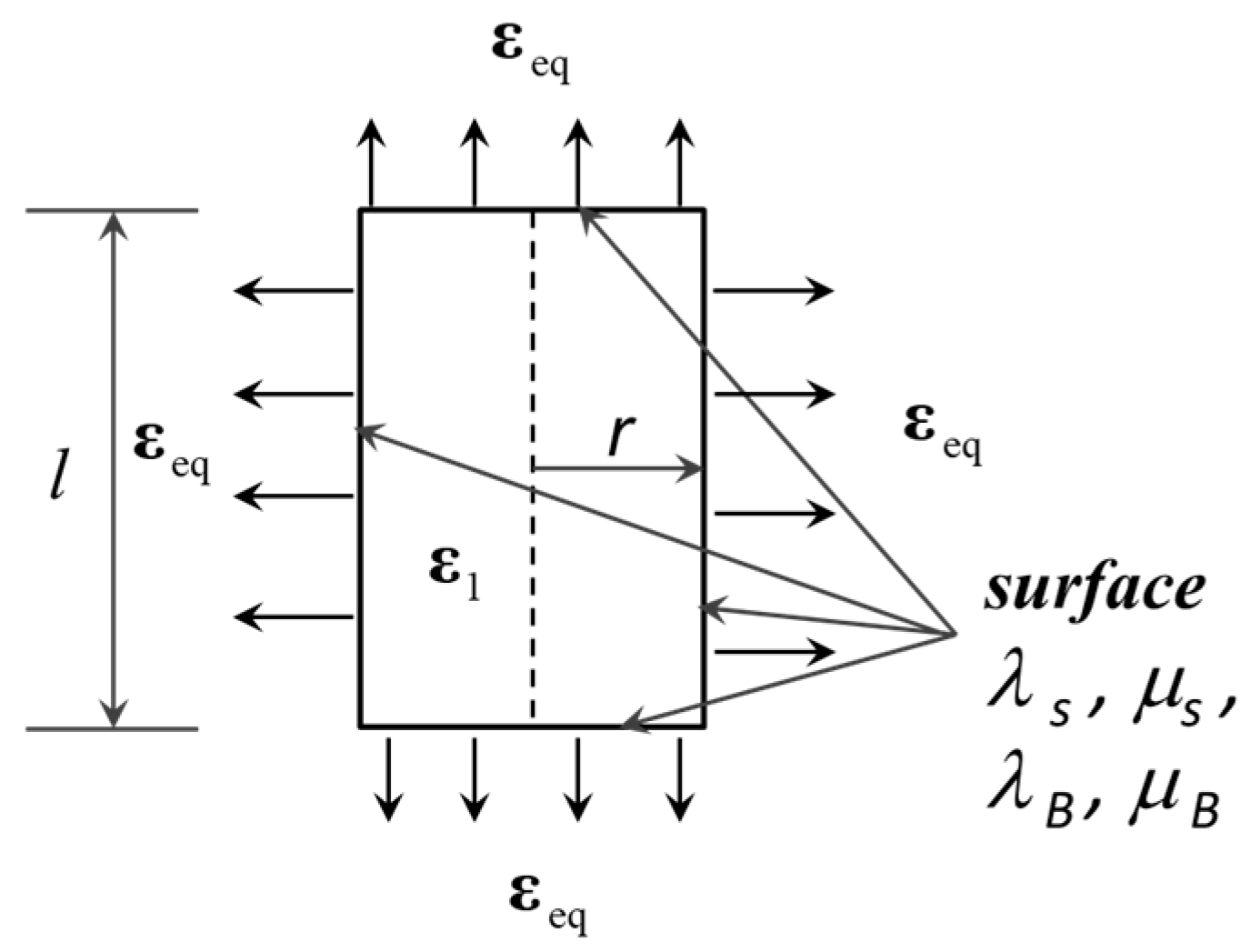
2.1. General Considerations
2.2. Steigmann–Ogden Surface Model and Associated Elastic Energy
2.3. Contribution of the Surface Bending to the Energy of Equivalent Cylinder
2.3.1. Evaluation of the Surface Energy Related to the Bending
2.3.2. Constitutive Tensor of the Energy-Equivalent Cylinder
3. Comparison with the Existing Results for the Cylinder of Infinite Length with Gurtin–Murdoch and Steigmann–Ogden Interfaces
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Components of Curvature Tensors for Cylinder
| 1 | 1 | ||
| 1 | 2 | 0 | 0 |
| 2 | 2 | 0 | 0 |
| 2 | 1 | 0 | 0 |
Appendix B. Properties of the Energy-Equivalent Cylinder of Finite Length Accounting for Surface Bending
References
- Benveniste, Y.; Miloh, T. Imperfect soft and stiff interfaces in two-dimensional elasticity. Mech. Mater. 2001, 33, 309–323. [Google Scholar] [CrossRef]
- Dong, C.Y.; Zhang, G.L. Boundary element analysis of three dimensional nanoscale inhomogeneities. Int. J. Solids Struct. 2013, 50, 201–208. [Google Scholar] [CrossRef]
- Dong, H.; Wang, J.; Rubin, M. Cosserat interphase models for elasticity with application to the interphase bonding a spherical inclusion to an infinite matrix. Int. J. Solid. Struct. 2014, 51, 462–477. [Google Scholar] [CrossRef][Green Version]
- Hashin, Z. Thermoelastic properties of fiber composites with imperfect interface. Mech. Mater. 1990, 8, 333–348. [Google Scholar] [CrossRef]
- Hashin, Z. Thermoelastic properties of particulate composites with imperfect interface. J. Mech. Phys. Solids 1991, 39, 745–762. [Google Scholar] [CrossRef]
- He, L.H.; Li, Z.R. Impact of surface stress on stress concentration. Int. J. Solids Struct. 2006, 43, 6208–6219. [Google Scholar] [CrossRef]
- Huang, Z.P.; Wang, J. A theory of hyperelasticity of multi-phase media with surface/interface effect. Acta Mech. 2006, 182, 195–210. [Google Scholar] [CrossRef]
- Jasiuk, I.; Kouider, M.W. The effect of an inhomogeneous interphase on the elastic constants of transversely isotropic composites. Mech. Mat. 1993, 15, 53–63. [Google Scholar] [CrossRef]
- Serpilli, M.; Rizzoni, R.; Lebon, F.; Dumont, S. An asymptotic derivation of a general imperfect interface law for linear multiphysics composites. Int. J. Solids Struct. 2019, 180–181, 97–107. [Google Scholar] [CrossRef]
- Zhang, W.X.; Wang, T. Effect of surface energy on the yield strength of nanoporous materials. Appl. Phys. Lett. 2007, 90, 063104. [Google Scholar] [CrossRef]
- Rubin, M.; Benveniste, Y.A. Cosserat shell model for interphases in elastic media. J. Mech. Phys. Solids 2014, 52, 1023–1052. [Google Scholar] [CrossRef]
- Gurtin, M.E.; Murdoch, A.I. A continuum theory of elastic material surfaces. Arch. Ration. Mech. Anal. 1975, 57, 291–323. [Google Scholar] [CrossRef]
- Gurtin, M.E.; Murdoch, A.I. Surface stress in solids. Int. J. Solids Struct. 1978, 14, 431–440. [Google Scholar] [CrossRef]
- Brisard, S.; Dormieux, L.; Kondo, D. Hashin–Shtrikman bounds on the bulk modulus of a nanocomposite with spherical inhomogeneities and interface effects. Comput. Mater. Sci. 2010, 48, 589–596. [Google Scholar] [CrossRef]
- Chen, T.; Dvorak, G.J. Fibrous nano-composites with interface stresses: Hill’s and Levin’s connection for effective moduli. Appl. Phys. Lett. 2006, 88, 211912. [Google Scholar] [CrossRef]
- Chen, T.; Dvorak, G.J.; Yu, C.C. Size-dependent elastic properties of unidirectional nano-composites with interface stresses. Acta Mech. 2007, 188, 39–54. [Google Scholar] [CrossRef]
- Duan, H.L.; Wang, J.; Huang, Z.P.; Karihaloo, B.L. Size-dependent effective elastic constants of solids containing nanoinhomogeneities with interface stress. J. Mech. Phys. Solids 2005, 53, 1574–1596. [Google Scholar] [CrossRef]
- Lim, C.W.; Li, Z.R.; He, L.H. Size-dependent, non-uniform elastic field inside a nano-scale spherical inclusion due to interface stress. Int. J. Solids Struct. 2006, 43, 5055–5065. [Google Scholar] [CrossRef]
- Nazarenko, L.; Stolarski, H. Energy-based definition of equivalent inhomogeneity for various interphase models and analysis of effective properties of particulate composites. Comp. Part B. 2016, 94, 82–94. [Google Scholar] [CrossRef]
- Altenbach, H.; Eremeyev, V.A. On the shell theory on the nanoscale with surface stresses. Int. J. Eng. Sci. 2011, 49, 1294–1301. [Google Scholar] [CrossRef]
- Miller, R.E.; Shenoy, V.B. Size-dependent elastic properties of nanosized structural elements. Nanotechnology 2000, 11, 139–147. [Google Scholar] [CrossRef]
- Steigmann, D.J.; Ogden, R.W. Plane deformations of elastic solids with intrinsic boundary elasticity. Proc. R. Soc. Lond. A 1997, 453, 853–877. [Google Scholar] [CrossRef]
- Steigmann, D.J.; Ogden, R.W. Elastic surface-substrate interactions. Proc. R. Soc. Lond. A 1999, 455, 437–474. [Google Scholar] [CrossRef]
- Chhapadia, P.; Mohammadi, P.; Sharma, P. Curvature-dependent surface energy and implications for nanostructures. J. Mech. Phys. Solids 2011, 59, 2103–2115. [Google Scholar] [CrossRef]
- Mohammadi, P.; Sharma, P. Atomistic elucidation of surface roughness on curvature-dependent surface energy, surface stress, and elasticity. Appl. Phys. Latter 2012, 100, 133110. [Google Scholar] [CrossRef]
- Eremeyev, V.A.; Wiczenbach, T. On Effective Bending Stiffness of a Laminate Nanoplate Considering Steigmann–Ogden Surface Elasticity. Appl. Sci. 2020, 10, 7402. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Seppecher, P. Edge contact forces and quasi-balanced power. Meccanica 1997, 32, 33–52. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Seppecher, P.; Madeo, A. How contact interactions may depend on the shape of Cauchy cuts in Nth gradient continua: Approach “à la d’Alembert”. Z. Angew. Math. Phys. 2012, 63, 1119–1141. [Google Scholar] [CrossRef]
- Javili, A.; Dell’Isola, F.; Steinmann, P. Geometrically nonlinear higher-gradient elasticity with energetic boundaries. J. Mech. Phys. Solids 2013, 61, 2381–2401. [Google Scholar] [CrossRef]
- Javili, A.; Ottosen, N.S.; Ristinmaa, M.; Mosler, J. Aspects of interface elasticity theory. Math. Mech. Solids 2018, 23, 1004–1024. [Google Scholar] [CrossRef]
- Mindlin, R.D. Micro-structure in linear elasticity. Arch. Ration. Mech. Anal. 1964, 16, 51–78. [Google Scholar] [CrossRef]
- Mindlin, R.D. Second gradient of strain and surface-tension in linear elasticity. Int. J. Solids Struct. 1965, 1, 417–438. [Google Scholar] [CrossRef]
- Toupin, R.A. Elastic materials with couple-stresses. Arch. Ration. Mech. Anal. 1962, 11, 385–414. [Google Scholar] [CrossRef]
- Eremeyev, V.A.; Lebedev, L.P. Mathematical study of boundary-value problems within the framework of Steigmann–Ogden model of surface elasticity. Contin. Mech. Therm. 2016, 28, 407–422. [Google Scholar] [CrossRef]
- Eremeev, V. On dynamic boundary conditions within the linear Steigmann-Ogden model of surface elasticity and strain gradient elasticity. In Dynamical Processes in Generalized Continua and Structures. Advanced Structured Materials; Altenbach, H., Belyaev, A., Eremeyev, V., Krivtsov, A., Porubov, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2019; Volume 103. [Google Scholar]
- Zemlyanova, A.Y.; Mogilevskaya, S.G. Circular inhomogeneity with Steigmann–Ogden interface: Local fields, neutrality, and Maxwell’s type approximation formula. Int. J. Solids Struct. 2018, 135, 85–98. [Google Scholar] [CrossRef]
- Gao, X.; Huang, Z.; Qu, J.; Fang, D. A curvature-dependent interfacial energy-based interface stress theory and its applications to nanostructured materials: (I) general theory. J. Mech. Phys. Solids 2014, 66, 59–77. [Google Scholar] [CrossRef]
- Gao, X.; Huang, Z.; Fang, D. Curvature-dependent interfacial energy and its effects on the elastic properties of nanomaterials. Int. J. Solid. Struct. 2017, 113, 100–107. [Google Scholar] [CrossRef]
- Nazarenko, L.; Stolarski, H.; Altenbach, H. Effective properties of random nano-materials including Steigmann–Ogden interface model of surface. Comput. Mech. under review.
- Zemlyanova, A.Y.; Mogilevskaya, S.G. On spherical inhomogeneity with Steigmann–Ogden interface. J. Appl. Mech. 2018, 85, 121009. [Google Scholar] [CrossRef]
- Nazarenko, L.; Bargmann, S.; Stolarski, H. Energy-equivalent inhomogeneity approach to analysis of effective properties of nano-materials with stochastic structure. Int. J. Solids Struct. 2015, 59, 183–197. [Google Scholar] [CrossRef]
- Nazarenko, L.; Stolarski, H.; Altenbach, H. Effective properties of short-fiber composites with Gurtin-Murdoch model of interphase. Int. J. Solids Struct. 2016, 97–98, 75–88. [Google Scholar] [CrossRef]
- Nazarenko, L.; Bargmann, S.; Stolarski, H. Closed-form formulas for the effective properties of random particulate nanocomposites with complete Gurtin–Murdoch model of material surfaces. Contin. Mech. Thermodyn. 2017, 29, 77–96. [Google Scholar] [CrossRef]
- Itskov, M. Tensor Algebra and Tensor Analysis for Engineers; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Hill, R. Elastic properties of reinforced solids: Some theoretical principles. J. Mech. Phys. Solids 1963, 11, 357–372. [Google Scholar] [CrossRef]
- Milton, G.W. The Theory of Composites; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Christensen, R.M.; Lo, K.M. Solution for effective shear properties in three phase sphere and cylinder models. J. Mech. Phys. Solids 1979, 27, 315–330. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nazarenko, L.; Stolarski, H.; Altenbach, H. Modeling Cylindrical Inhomogeneity of Finite Length with Steigmann–Ogden Interface. Technologies 2020, 8, 78. https://doi.org/10.3390/technologies8040078
Nazarenko L, Stolarski H, Altenbach H. Modeling Cylindrical Inhomogeneity of Finite Length with Steigmann–Ogden Interface. Technologies. 2020; 8(4):78. https://doi.org/10.3390/technologies8040078
Chicago/Turabian StyleNazarenko, Lidiia, Henryk Stolarski, and Holm Altenbach. 2020. "Modeling Cylindrical Inhomogeneity of Finite Length with Steigmann–Ogden Interface" Technologies 8, no. 4: 78. https://doi.org/10.3390/technologies8040078
APA StyleNazarenko, L., Stolarski, H., & Altenbach, H. (2020). Modeling Cylindrical Inhomogeneity of Finite Length with Steigmann–Ogden Interface. Technologies, 8(4), 78. https://doi.org/10.3390/technologies8040078






