Rapid Evaluation of the Decay Time of a Plasma Brake-Based CubeSat
Abstract
1. Introduction
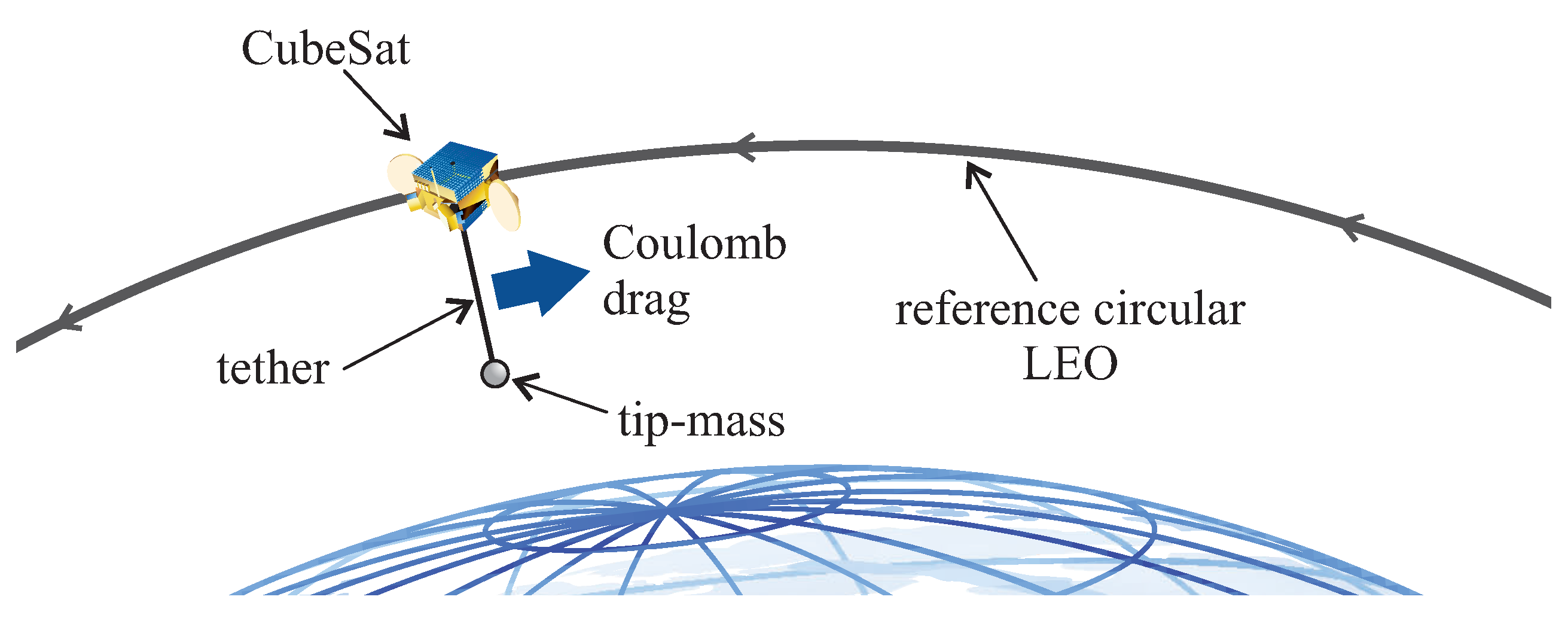
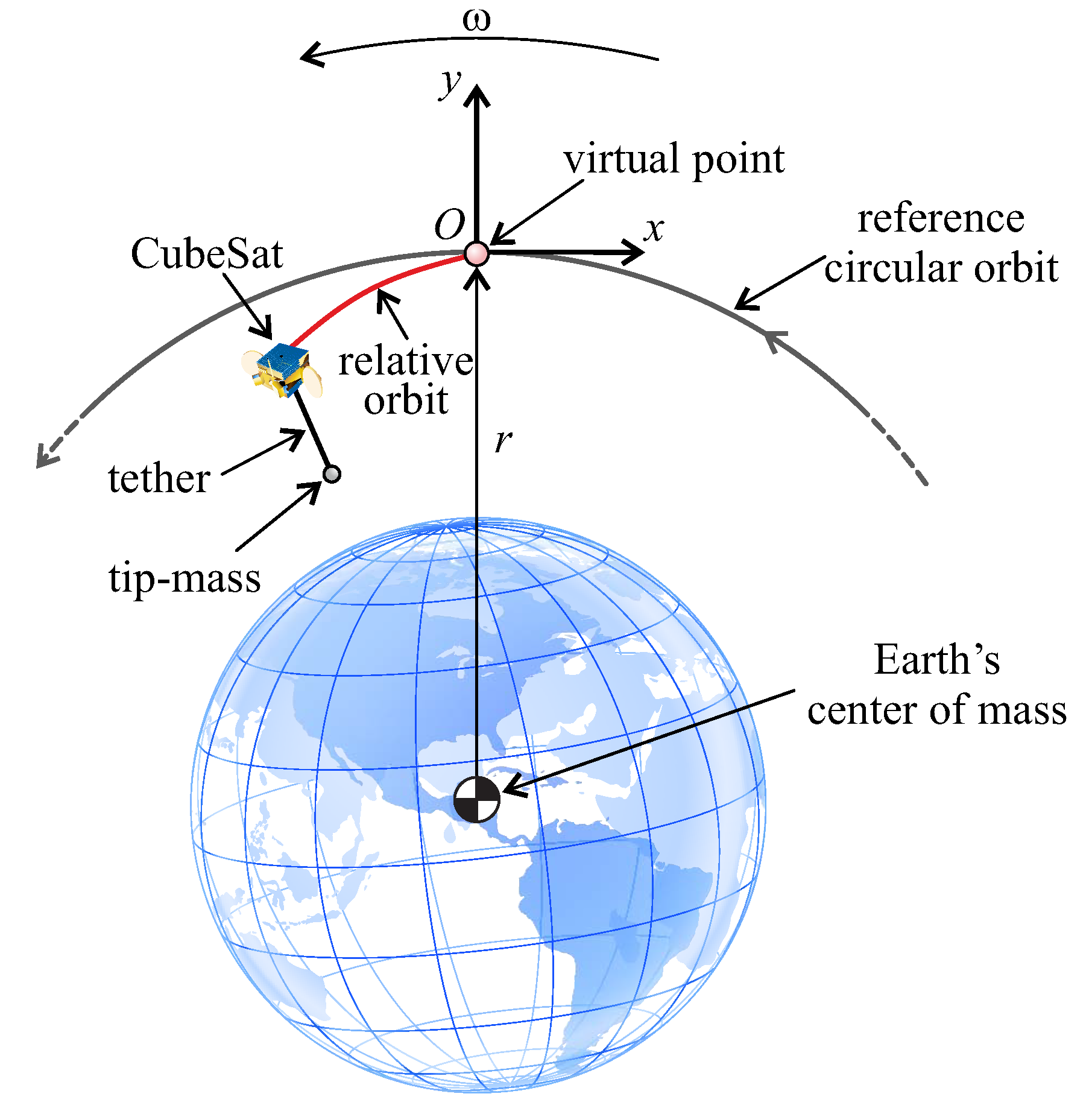
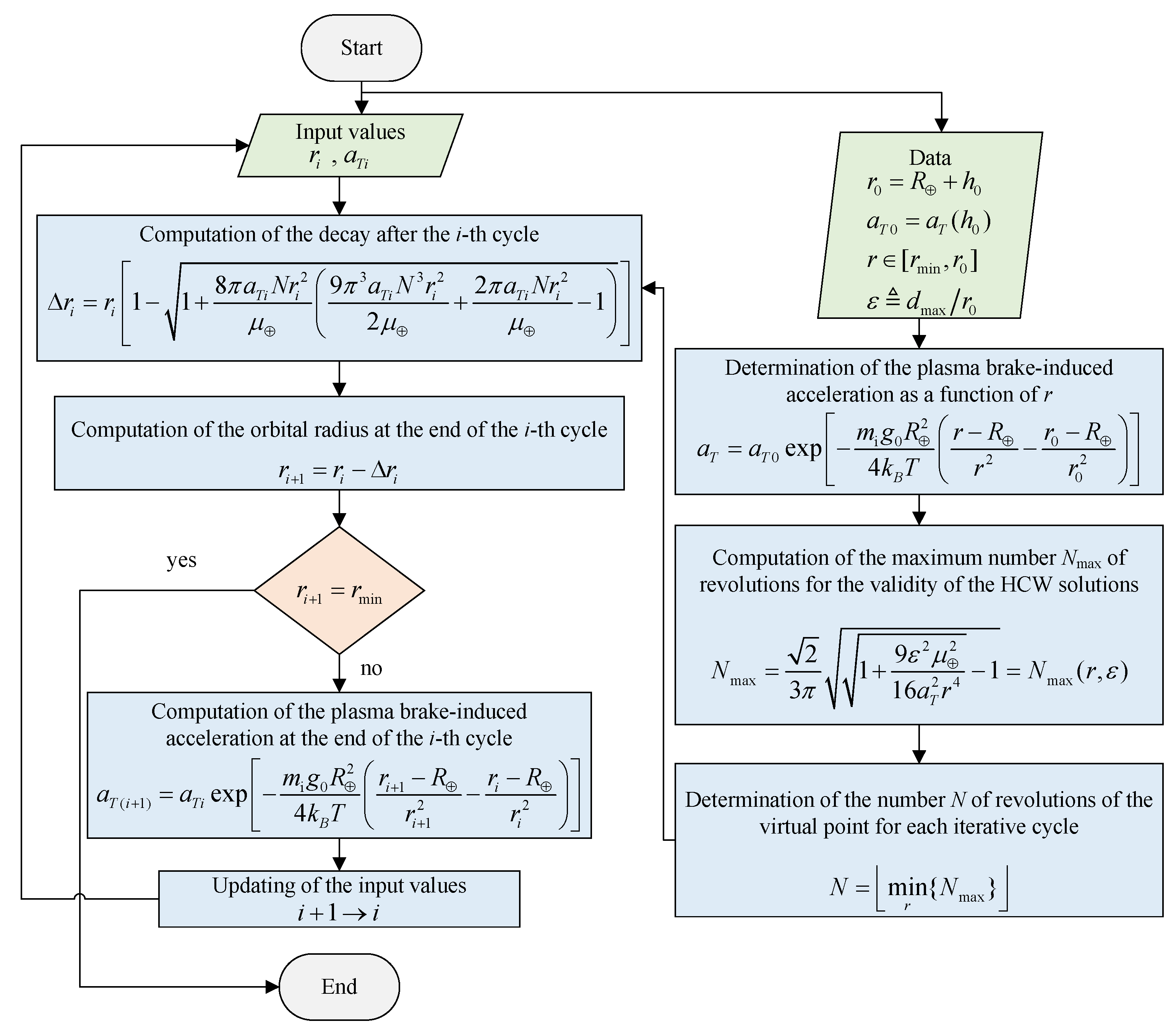
2. Mathematical Model
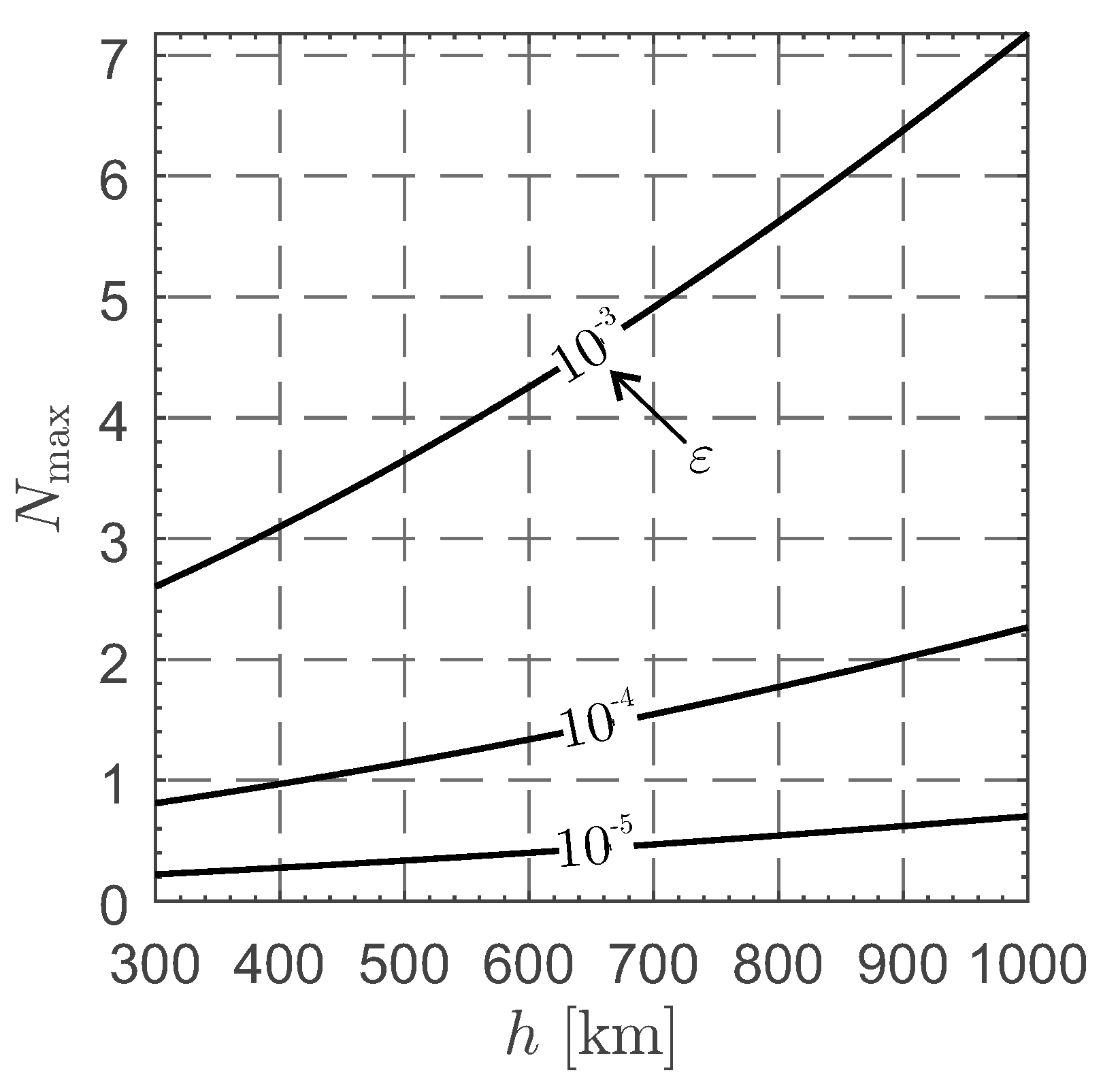
3. Numerical Simulations and Mission Application
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| transverse plasma brake-induced acceleration [] | |
| d | relative distance [m] |
| standard gravity [] | |
| h | altitude [m] |
| i | iteration index |
| Boltzmann constant [] | |
| tether length [m] | |
| m | spacecraft mass [kg] |
| mean molecular mass of the incoming flow [kg] | |
| N | number of revolutions of the virtual point |
| O | origin of |
| r | orbital radius [km] |
| mean Earth’s radius [km] | |
| t | time [s] |
| T | ionosphere temperature [K] |
| tether voltage [V] | |
| x | transverse relative coordinate [km] |
| y | radial relative coordinate [km] |
| dimensionless parameter () | |
| Earth’s gravitational parameter [] | |
| rotating reference system | |
| orbital angular velocity [] | |
| Subscripts | |
| c | numerical |
| d | decay |
| max | maximum |
| min | minimum |
| N | relative to N revolutions of the virtual point |
| 0 | initial conditions |
References
- Li, Q.B.; Wu, K.J.; Niu, C.Y. Determination of the Number of Space Debris’ Materials Based on Spectral Information. Guang Pu Xue Yu Guang Pu Fen Xi/Spectrosc. Spectr. Anal. 2017, 37, 2679–2684. [Google Scholar] [CrossRef]
- Zhang, H.; Li, Z.; Wang, W.; Zhang, Y.; Wang, H. Geostationary Orbital Debris Collision Hazard after a Collision. Aerospace 2022, 9, 258. [Google Scholar] [CrossRef]
- Rossi, A.; Colombo, C.; Tsiganis, K.; Beck, J.; Rodriguez, J.B.; Walker, S.; Letterio, F.; Dalla Vedova, F.; Schaus, V.; Popova, R.; et al. ReDSHIFT: A Global Approach to Space Debris Mitigation. Aerospace 2018, 5, 64. [Google Scholar] [CrossRef]
- Hu, M.; Ruan, Y.; Zhou, H.; Xu, J.; Xue, W. Long-Term Orbit Prediction and Deorbit Disposal Investigation of MEO Navigation Satellites. Aerospace 2022, 9, 266. [Google Scholar] [CrossRef]
- Zhang, Y.; An, F.; Liao, S.; Wu, C.; Liu, J.; Li, Y. Study on Numerical Simulation Methods for Hypervelocity Impact on Large-Scale Complex Spacecraft Structures. Aerospace 2022, 9, 12. [Google Scholar] [CrossRef]
- Kvell, U.; Di Cara, D.; Janhunen, P.; Noorma, M.; Gonzalez del Amo, J.A. Deorbiting strategies: Comparison between electrostatic plasma brake and conventional propulsion. In Proceedings of the 47th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, San Diego, CA, USA, 31 July–3 August 2011. Paper AIAA 2011-5920. [Google Scholar] [CrossRef]
- Ham, C.; Ngo, D. Deorbiting of LEO small satellites without a conventional propulsion system. In Proceedings of the IEEE SOUTHEASTCON, Charlotte, NC, USA, 30 March–2 April 2017. [Google Scholar] [CrossRef]
- Lv, S.; Zhang, H.; Zhang, Y.; Ning, B.; Qi, R. Design of an Integrated Platform for Active Debris Removal. Aerospace 2022, 9, 339. [Google Scholar] [CrossRef]
- Yakovlev, M. The IADC space debris mitigation guidelines and support document. In Proceedings of the Fourth European Conference on Space Debris, European Space Agency (ESA), Darmstadt, Germany, 18–20 April 2005. [Google Scholar]
- Lambert, F.; Christiansen, E. The Inter-Agency Space Debris Coordination Committee (IADC) protection manual. In Proceedings of the Fourth European Conference on Space Debris, European Space Agency (ESA), Darmstadt, Germany, 18–20 April 2005. [Google Scholar]
- Popova, R.; Schaus, V. The Legal Framework for Space Debris Remediation as a Tool for Sustainability in Outer Space. Aerospace 2018, 5, 55. [Google Scholar] [CrossRef]
- Janhunen, P. Electrostatic plasma brake for deorbiting a satellite. J. Propuls. Power 2010, 26, 370–372. [Google Scholar] [CrossRef]
- Zhong, R.; Zhu, Z.H. Dynamics of nanosatellite deorbit by bare electrodynamic tether in low earth orbit. J. Spacecr. Rocket. 2013, 50, 691–700. [Google Scholar] [CrossRef]
- Janhunen, P. Simulation study of the plasma-brake effect. Ann. Geophys. 2014, 32, 1207–1216. [Google Scholar] [CrossRef]
- Janhunen, P.; Toivanen, P.K.; Envall, J.; Slavinskis, A. Using charged tether Coulomb drag: E-sail and plasma brake. In Proceedings of the 5th International Conference on Tethers in Space, Ann Arbor, Michigan, 24–26 May 2016. [Google Scholar]
- Janhunen, P. On the feasibility of a negative polarity electric sail. Ann. Geophys. 2009, 27, 1439–1447. [Google Scholar] [CrossRef]
- Janhunen, P. Electric sail for spacecraft propulsion. J. Propuls. Power 2004, 20, 763–764. [Google Scholar] [CrossRef]
- Mengali, G.; Quarta, A.A.; Janhunen, P. Electric sail performance analysis. J. Spacecr. Rocket. 2008, 45, 122–129. [Google Scholar] [CrossRef]
- Huo, M.; Mengali, G.; Quarta, A.A. Optimal planetary rendezvous with an electric sail. Aircr. Eng. Aerosp. Technol. 2016, 88, 515–522. [Google Scholar] [CrossRef]
- Bassetto, M.; Quarta, A.A.; Mengali, G. Locally-optimal electric sail transfer. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 233, 166–179. [Google Scholar] [CrossRef]
- Mengali, G.; Quarta, A.A.; Janhunen, P. Considerations of electric sailcraft trajectory design. JBIS-J. Br. Interplanet. Soc. 2008, 61, 326–329. [Google Scholar]
- Quarta, A.A.; Mengali, G. Electric sail mission analysis for outer solar system exploration. J. Guid. Control. Dyn. 2010, 33, 740–755. [Google Scholar] [CrossRef]
- Janhunen, P.; Lebreton, J.P.; Merikallio, S.; Paton, M.; Mengali, G.; Quarta, A.A. Fast E-sail Uranus Entry Probe Mission. Planet. Space Sci. 2014, 104, 141–146. [Google Scholar] [CrossRef]
- Huo, M.; Mengali, G.; Quarta, A.A. Mission Design for an Interstellar Probe with E-Sail Propulsion System. JBIS-J. Br. Interplanet. Soc. 2015, 68, 128–134. [Google Scholar]
- Matloff, G.L. The Solar-Electric Sail: Application to Interstellar Migration and Consequences for SETI. Universe 2022, 8, 252. [Google Scholar] [CrossRef]
- Bonnal, C.; Ruault, J.M.; Desjean, M.C. Active debris removal: Recent progress and current trends. Acta Astronaut. 2015, 85, 51–60. [Google Scholar] [CrossRef]
- Mark, C.P.; Kamath, S. Review of active space debris removal methods. Space Policy 2019, 47, 194–206. [Google Scholar] [CrossRef]
- Roberts, P.C.E.; Harkness, P.G. Drag sail for end-of-life disposal from low Earth orbit. J. Spacecr. Rocket. 2007, 44, 1196–1203. [Google Scholar] [CrossRef]
- Underwood, C.; Viquerat, A.; Schenk, M.; Taylor, B.; Massimiani, C.; Duke, R.; Stewart, B.; Fellowes, S.; Bridges, C.; Aglietti, G.; et al. InflateSail de-orbit flight demonstration results and follow-on drag-sail applications. Acta Astronaut. 2019, 162, 344–358. [Google Scholar] [CrossRef]
- Hoyt, R.P.; Barnes, I.M.; Voronka, N.R.; Slostad, J.T. The Terminator Tape™: A cost-effective de-orbit module for end-of-life disposal of LEO satellites. In Proceedings of the AIAA Space 2009 Conference and Exposition, Pasadena, CA, USA, 14–17 September 2009. [Google Scholar]
- Valmorbida, A.V.; Olivieri, L.; Brunello, A.; Sarego, G.; Sánchez-Arriaga, G.; Lorenzini, E.C. Validation of enabling technologies for deorbiting devices based on electrodynamic tethers. Acta Astronaut. 2022, 198, 707–719. [Google Scholar] [CrossRef]
- Iakubivskyi, I.; Janhunen, P.; Praks, J.; Allik, V.; Bussov, K.; Clayhills, B.; Dalbins, J.; Eenmäe, T.; Ehrpais, H.; Envall, J.; et al. Coulomb drag propulsion experiments of ESTCube-2 and FORESAIL-1. Acta Astronaut. 2020, 177, 771–783. [Google Scholar] [CrossRef]
- Janhunen, P.; Toivanen, P.K.; Polkko, J.; Merikallio, S.; Salminen, P.; Haeggström, E.; Seppänen, H.; Kurppa, R.; Ukkonen, J.; Kiprich, S.; et al. Electric solar wind sail: Toward test missions. Rev. Sci. Instrum. 2010, 81, 111301. [Google Scholar] [CrossRef]
- Bassetto, M.; Niccolai, L.; Quarta, A.A.; Mengali, G. A comprehensive review of Electric Solar Wind Sail concept and its applications. Prog. Aerosp. Sci. 2022, 128, 1–27. [Google Scholar] [CrossRef]
- Seppänen, H.; Rauhala, T.; Kiprich, S.; Ukkonen, J.; Simonsson, M.; Kurppa, R.; Janhunen, P.; Hæggström, E. One kilometer (1 km) electric solar wind sail tether produced automatically. Rev. Sci. Instrum. 2013, 84, 095102. [Google Scholar] [CrossRef]
- Seppänen, H.; Kiprich, S.; Kurppa, R.; Janhunen, P.; Hæggström, E. Wire-to-wire bonding of μm-diameter aluminum wires for the electric solar wind sail. Microelectron. Eng. 2011, 88, 3267–3269. [Google Scholar] [CrossRef]
- Rauhala, T.; Seppänen, H.; Ukkonen, J.; Kiprich, S.; Maconi, G.; Janhunen, P.; Hæggström, E. Automatic 4-wire Heytether production for the electric solar wind sail. In Proceedings of the International Microelectronics Assembly and Packing Society Topical Workshop and Tabletop Exhibition on Wire Bonding, San Jose, CA, USA; 2013. [Google Scholar]
- Lätt, S.; Slavinskis, A.; Ilbis, E.; Kvell, U.; Voormansik, K.; Kulu, E.; Pajusalu, M.; Kuuste, H.; Sünter, I.; Eenmäe, T.; et al. ESTCube-1 nanosatellite for electric solar wind sail in-orbit technology demonstration. Proc. Est. Acad. Sci. 2014, 63, 200–209. [Google Scholar] [CrossRef]
- Slavinskis, A.; Pajusalu, M.; Kuuste, H.; Ilbis, E.; Eenmäe, T.; Sünter, I.; Laizans, K.; Ehrpais, H.; Liias, P.; Kulu, E.; et al. ESTCube-1 in-orbit experience and lessons learned. IEEE Aerosp. Electron. Syst. Mag. 2015, 30, 12–22. [Google Scholar] [CrossRef]
- Olesk, A. Mediatization of a Research Group: The Estonian Student Satellite ESTCube-1. Sci. Commun. 2019, 41, 196–221. [Google Scholar] [CrossRef]
- Olesk, A.; Noorma, M. Chapter 9 - The Engagement Activities of ESTCube-1: How Estonia Built and Fell in Love With a Tiny Satellite. In Space Science and Public Engagement; Kaminski, A.P., Ed.; Elsevier: Amsterdam, The Netherlands, 2021; pp. 169–183. [Google Scholar] [CrossRef]
- Envall, J.; Janhunen, P.; Toivanen, P.K.; Pajusalu, M.; Ilbis, E.; Kalde, J.; Averin, M.; Kuuste, H.; Laizans, K.; Allik, V.; et al. E-sail test payload of the ESTCube-1 nanosatellite. Proc. Est. Acad. Sci. 2014, 63, 210–221. [Google Scholar] [CrossRef]
- Iakubivskyi, I.; Ehrpais, H.; Dalbins, J.; Oro, E.; Kulu, E.; Kütt, J.; Janhunen, P.; Slavinskis, A.; Ilbis, E.; Ploom, I.; et al. ESTCube-2 mission analysis: Plasma brake experiment for deorbiting. In Proceedings of the 67th International Astronautical Congress (IAC), Guadalajara, Mexico, 26–30 September 2016. Paper IAC-16,E2,4,4,x33190. [Google Scholar]
- Dalbins, J.; Allaje, K.; Iakubivskyi, I.; Kivastik, J.; Komarovskis, R.O.; Plans, M.; Sünter, I.; Teras, H.; Ehrpais, H.; Ilbis, E.; et al. ESTCube-2: The Experience of Developing a Highly Integrated CubeSat Platform. In Proceedings of the 2022 IEEE Aerospace Conference (AERO), Big Sky, MT, USA, 5–12 March 2022; pp. 1–16. [Google Scholar] [CrossRef]
- Kestilä, A.; Tikka, T.; Peitso, P.; Rantanen, J.; Näsilä, A.; Nordling, K.; Saari, H.; Vainio, R.; Janhunen, P.; Praks, J.; et al. Aalto-1 nanosatellite—Technical description and mission objectives. Geosci. Instrum. Methods Data Syst. 2013, 2, 121–130. [Google Scholar] [CrossRef]
- Khurshid, O.; Selkäinaho, J.; Soken, H.E.; Kallio, E.; Visala, A. Small satellite attitude determination during plasma brake deorbiting experiment. Acta Astronaut. 2016, 129, 52–58. [Google Scholar] [CrossRef]
- Khurshid, O.; Tikka, T.; Praks, J.; Hallikainen, M. Accomodating the plasma brake experiment on-board the Aalto-1 satellite. Proc. Est. Acad. Sci. 2014, 63, 258–266. [Google Scholar] [CrossRef]
- Mughal, M.R.; Praks, J.; Vainio, R.; Janhunen, P.; Envall, J.; Näsilä, A.; Oleynik, P.; Niemelä, P.; Nyman, S.; Slavinskis, A.; et al. Aalto-1, multi-payload CubeSat: In-orbit results and lessons learned. Acta Astronaut. 2021, 187, 557–568. [Google Scholar] [CrossRef]
- O’Reilly, D.; Herdrich, G.; Kavanagh, D.F. Electric Propulsion Methods for Small Satellites: A Review. Aerospace 2021, 8, 22. [Google Scholar] [CrossRef]
- Palmroth, M.; Praks, J.; Vainio, R.; Janhunen, P.; Kilpua, E.K.J.; Afanasiev, A.; Ala-Lahti, M.; Alho, A.; Asikainen, T.; Asvestari, E.; et al. FORESAIL-1 CubeSat mission to measure radiation belt losses and demonstrate deorbiting. J. Geophys. Res. Space Phys. 2019, 124, 5783–5799. [Google Scholar] [CrossRef]
- Niccolai, L.; Bassetto, M.; Quarta, A.A.; Mengali, G. Trajectory approximation of a Coulomb drag-based deorbiting. Aerospace, 2022; Submitted. [Google Scholar]
- Clohessy, W.H.; Wiltshire, R.S. Terminal Guidance System for Satellite Rendezvous. J. Aerosp. Sci. 1960, 27, 653–658. [Google Scholar] [CrossRef]
- Orsini, L.; Niccolai, L.; Mengali, G.; Quarta, A.A. Plasma Brake Model for Preliminary Mission Analysis. Acta Astronaut. 2018, 144, 297–304. [Google Scholar] [CrossRef]
- Chobotov, V.A. (Ed.) Orbital Mechanics; AIAA Education Series; American Institute of Aeronautics and Astronautics, Inc.: Reston, Virginia, 2002; Chapter 7; pp. 155–159. [Google Scholar] [CrossRef]
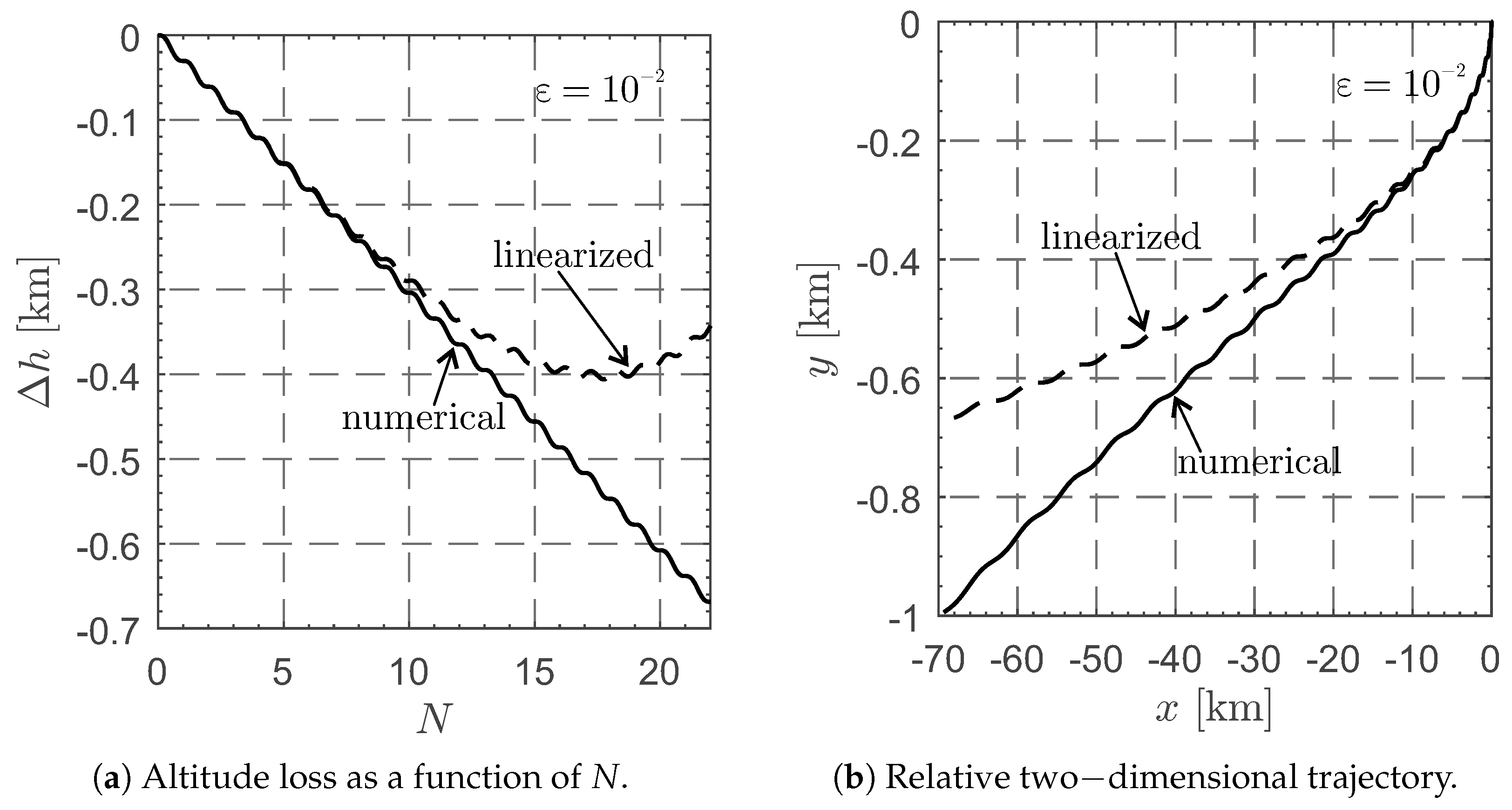
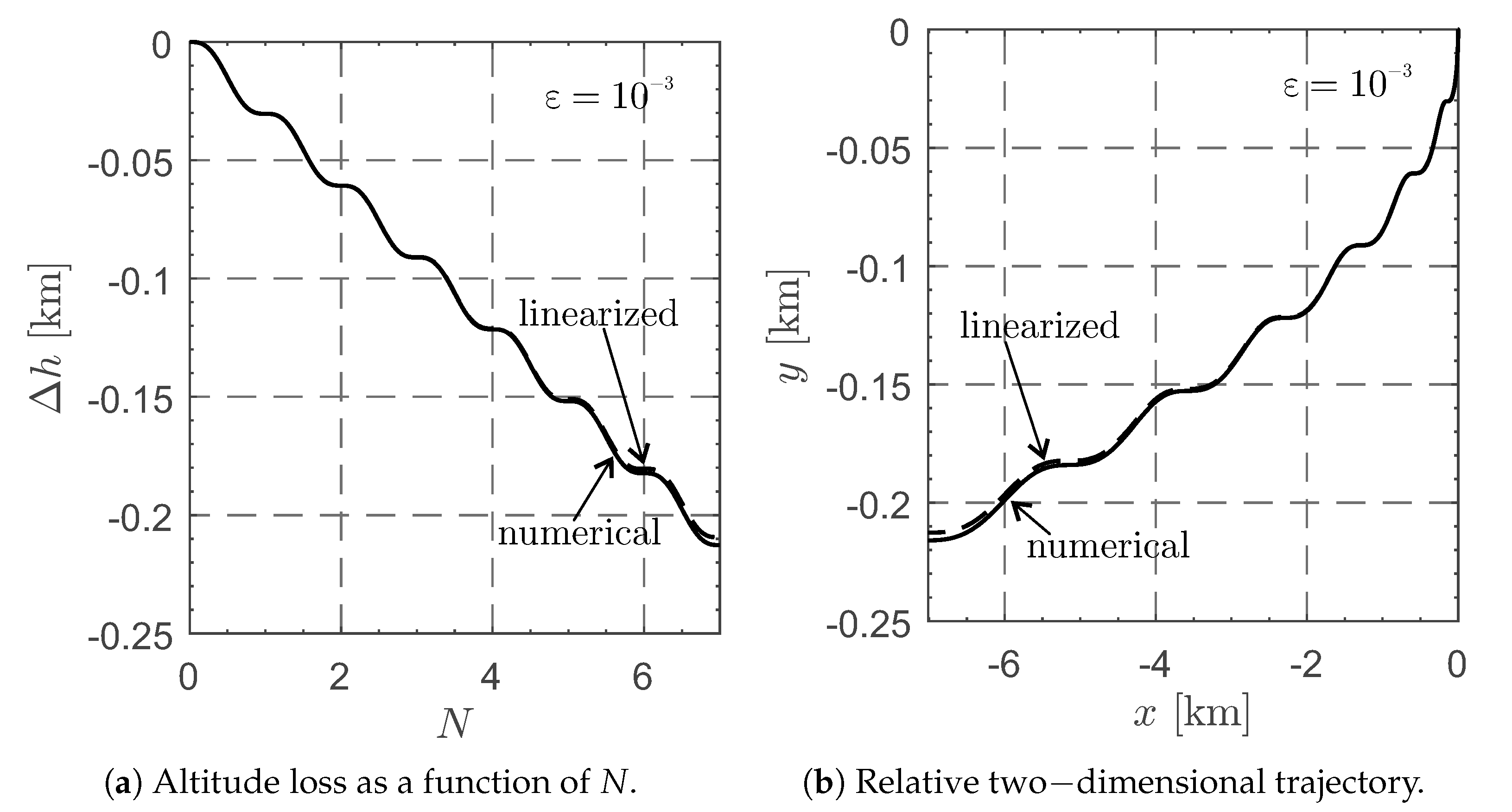
| m (kg) | (m) | (V) | (mm/s) | |
|---|---|---|---|---|
| spacecraft ① | 1 | 25 | −500 | 0.0014 |
| spacecraft ② | 4 | 100 | −1000 | 0.0020 |
| spacecraft ③ | 10 | 300 | −1000 | 0.0024 |
| Spacecraft ① | Spacecraft ② | Spacecraft ③ | |
|---|---|---|---|
| N | 3 | 2 | 2 |
| Numerical Integration | Algorithm | ||||
|---|---|---|---|---|---|
| (Years) | (s) | (Years) | (s) | Error(%) | |
| spacecraft ① | 3.5632 | 2061 | 3.5697 | 0.1 | 0.1835 |
| spacecraft ② | 2.5006 | 1356 | 2.5026 | 0.1 | 0.0794 |
| spacecraft ③ | 2.0838 | 1162 | 2.0859 | 0.1 | 0.0969 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bassetto, M.; Niccolai, L.; Quarta, A.A.; Mengali, G. Rapid Evaluation of the Decay Time of a Plasma Brake-Based CubeSat. Aerospace 2022, 9, 636. https://doi.org/10.3390/aerospace9110636
Bassetto M, Niccolai L, Quarta AA, Mengali G. Rapid Evaluation of the Decay Time of a Plasma Brake-Based CubeSat. Aerospace. 2022; 9(11):636. https://doi.org/10.3390/aerospace9110636
Chicago/Turabian StyleBassetto, Marco, Lorenzo Niccolai, Alessandro A. Quarta, and Giovanni Mengali. 2022. "Rapid Evaluation of the Decay Time of a Plasma Brake-Based CubeSat" Aerospace 9, no. 11: 636. https://doi.org/10.3390/aerospace9110636
APA StyleBassetto, M., Niccolai, L., Quarta, A. A., & Mengali, G. (2022). Rapid Evaluation of the Decay Time of a Plasma Brake-Based CubeSat. Aerospace, 9(11), 636. https://doi.org/10.3390/aerospace9110636






