Innovative Scaled Test Platform e-Genius-Mod—Scaling Methods and Systems Design
Abstract
:1. Introduction
1.1. Future Challenges
- Reduce CO2 and NOx emissions
- Reduce noise emission
- Taxi emission free
1.2. Accelerate Technology Readiness
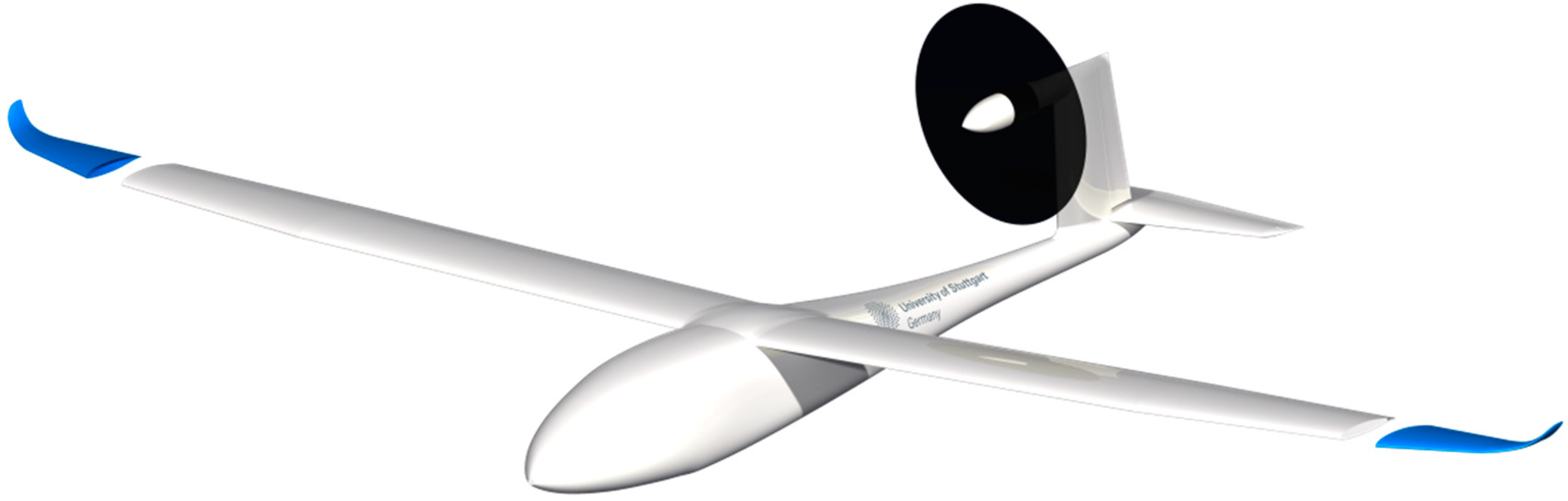
2. Technology Test Bed e-Genius-Mod
2.1. Scaling
- Dimensions
- Aerodynamics
- Inertia
2.1.1. Scaling Factor
- Flight conditions and test area
- Handling and transportability
- Environmental influences
- Payload test equipment
- Scalability of new technologies which shall be investigated
2.1.2. Airfoil Scaling
- Thickness / chord ratio
- Airfoil camber
- Location of maximum thickness
- Location of maximum chamber
- Leading edge radius
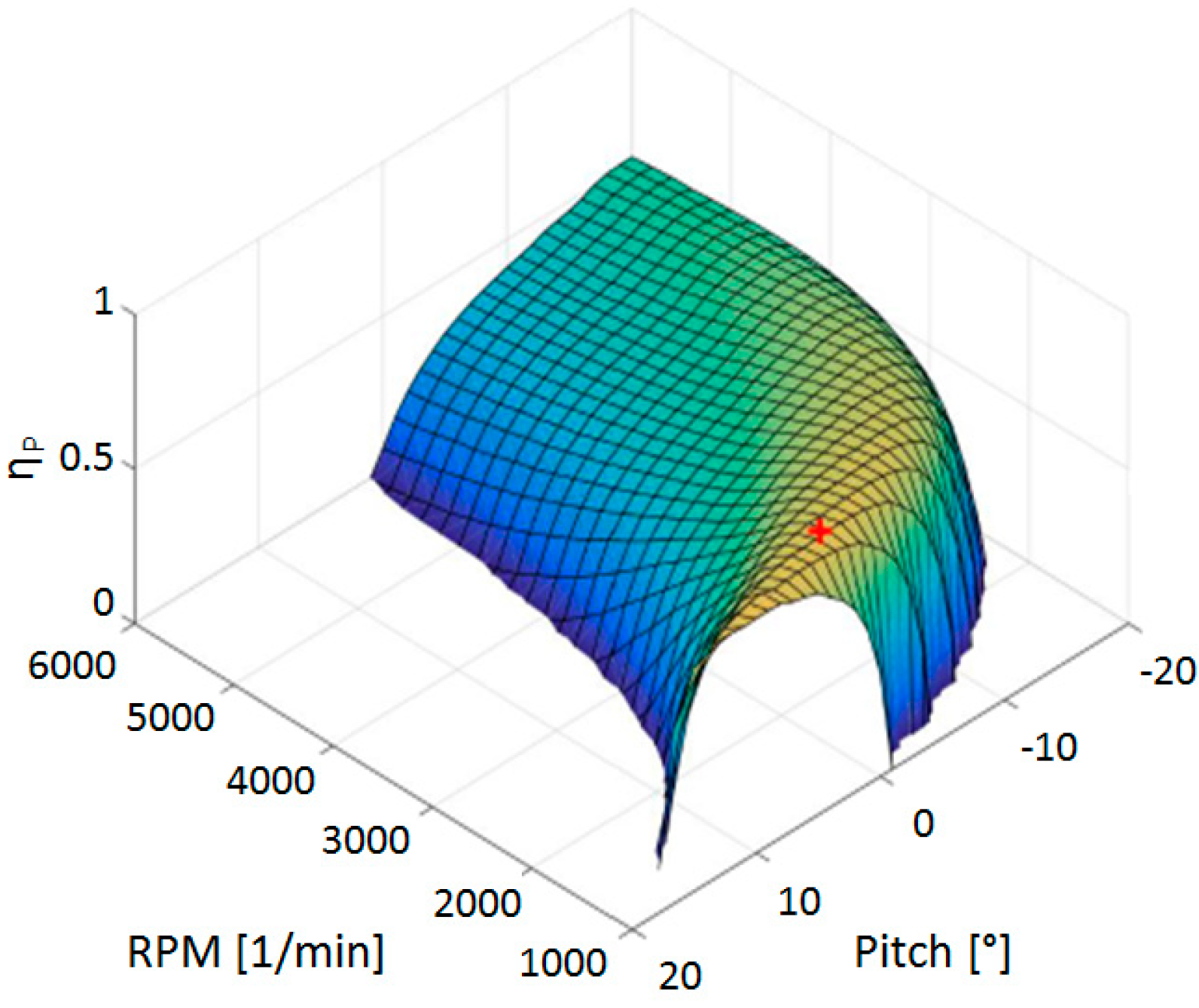
2.1.3. Variable Pitch Propeller
- The fixed propeller can efficiently cover a large portion of the flight envelope. In cruise flight (best range or best endurance), the fixed propeller works very close to its optimum, and the VPP barely gives any performance improvement (less than 2%). This is due to the e-Genius being a 33.3% scaled model, and having a smaller flight envelope than that of a full-scale aircraft.
- During climbing flight, the VPP improves performance by approximately 25%. However, the e-Genius-Mod, being a UAS, will mostly fly at low altitude (<500m). Consequently, climbing will be very short when compared to the cruise flight, thus limiting the performance improvement.
- The electric engine has a very good efficiency over a wide range of RPM, therefore the advantage of running the engine near its point of best efficiency (the way it would be done on a piston-engine aircraft) does not translate to the e-Genius model.
- The pitch variation system itself weighs 400 g, and due to its position at the aft of the fuselage this weight needs to be counterbalanced in the nose to keep a neutral center of gravity. Consequently, the overall weight penalty ranges from 400 to 1200 g (depending if the payload itself can be used as ballast to counterbalance this without adding overall weight, but this may not always be possible). Therefore, in most flight situations the increased weight of the variable-pitch propeller might negate the possible performance improvements.
- If the runway is particularly short, take-off performance can be noticeably improved.
- If the flight tasks require flying at either very low or very high speeds, outside the range where the fixed propeller performs well.
3. Results
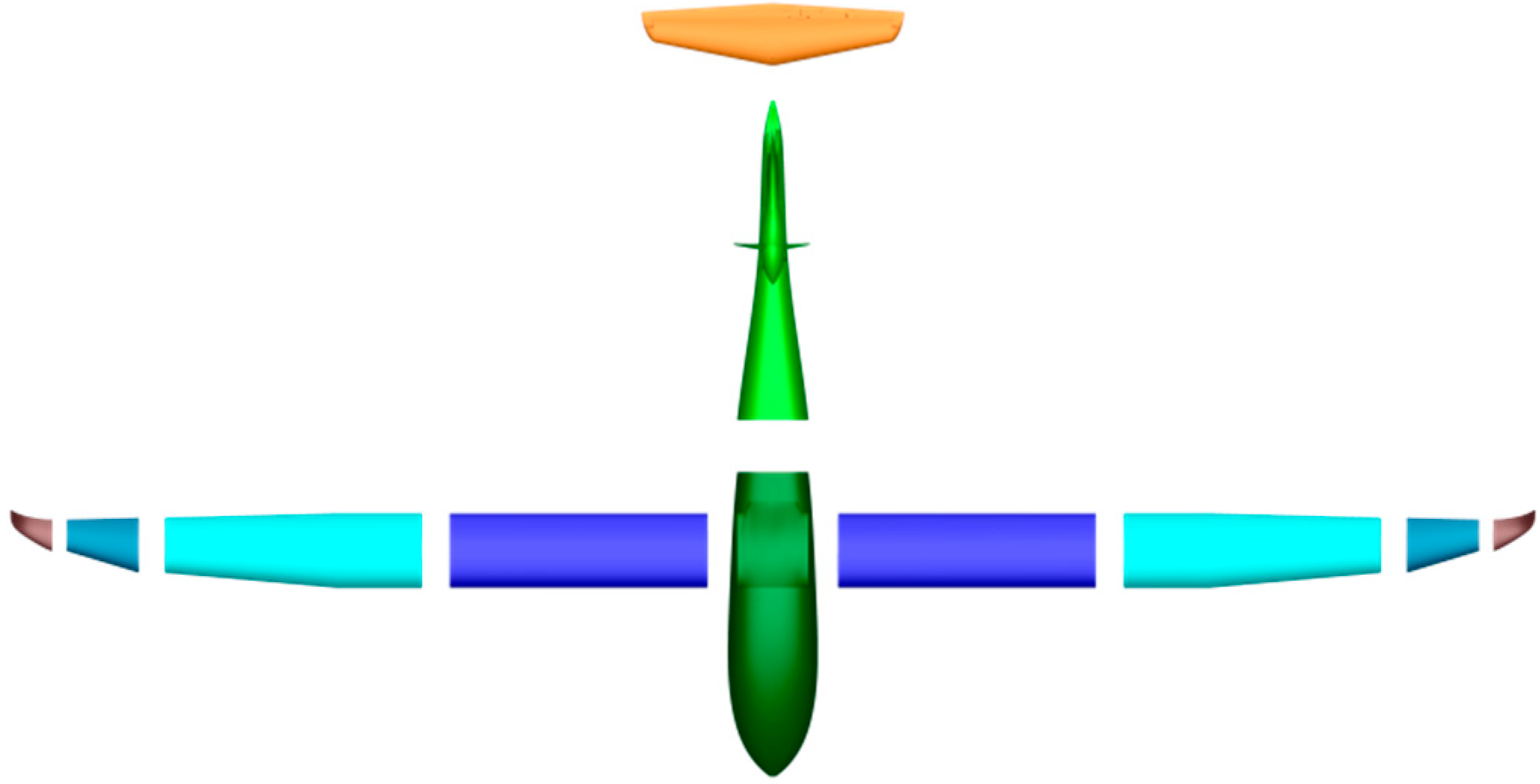
3.1. Flexible Airframe Configuration
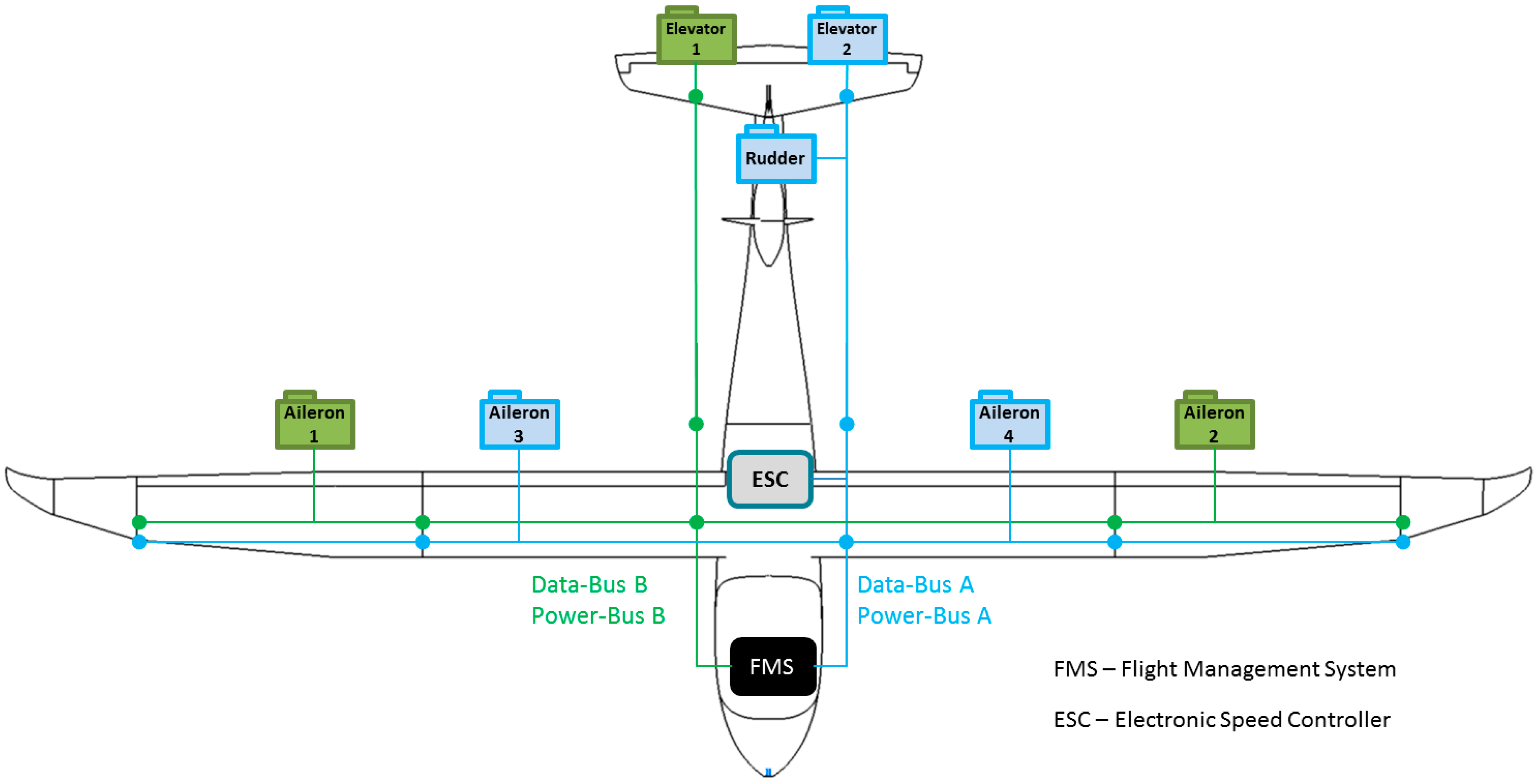
3.2. Systems Design
3.3. Free–Flight Model e-Genius Mod
- Inertial measurement unit—data of flight attitude (angle of orientation, acceleration, rotation rate)
- Air data boom—angle of attack, side slip, static and dynamic pressure
- GPS—position of the aircraft
- Actuator feedback—control surface angle
- Monitoring sensors—battery current/voltage/temperature, engine RPM/temperature
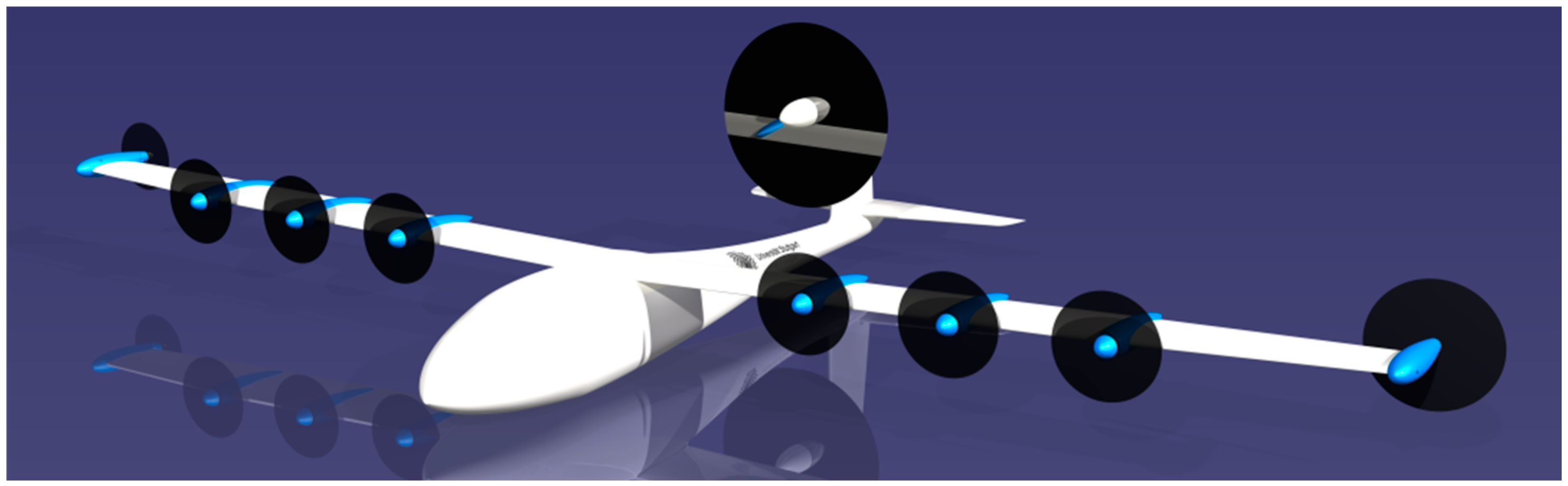
3.4. Application Example—Investigation of a Wing Tip Propulsion System
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bergmann, D.; Denzel, J.; Strohmayer, A. UAS as flexible and innovative test platform for aircraft configuration and systems testing. In Proceedings of the 8th EASN-CEAS International Workshop on Manufacturing for Growth & Innovation, Glasgow, UK, 4–7 September 2018. [Google Scholar]
- Kittmann, K. Entwicklung einer modularen Messplattform zur Analyse des Potenzials von Freiflugmessungen. Ph.D. Thesis, University of Stuttgart, Institute of Aircraft Design, Stuttgart, Germany, 2013. [Google Scholar] [CrossRef]
- Wolowicz, C.H.; Bowman, J.S., Jr.; Gilbert, W.P. Similitude Requirements and Scaling Relationships as Applied to Model Testing; NASA Technical Paper 1435; National Aeronautics and Space Administration, Scientific and Technical Information Branch: Washington, DC, USA, 1979. [Google Scholar]
- European Commission; Directorate-General for Research and Innovation; Directorate General for Mobility and Transport. Flightpath 2050 Europe’s Vision for Aviation, Report of the High Level Group on Aviation Research; Publications Office of the European Union, European Union: Luxembourg, 2011; ISBN 978-92-79-19724-6. [Google Scholar] [CrossRef]
- IATA Technology Roadmap 2013, 4th Edition. 2013. Available online: https://www.iata.org/whatwedo/environment/Documents/technology-roadmap-2013.pdf (accessed on 30 November 2018).
- Schmollgruber, P.; Gobert, J.-L.; Gall, P.-E.; Goraj, Z.; Jentink, H.W.; Näs, A.; Voit-Nitschmann, R. An innovative evaluation platform for new aircraft concepts. Aeronautical J. 2010, 114, 451–456. [Google Scholar]
- Jordan, T.; Langford, W.; Hill, J. Airborne Subscale Transport Aircraft Research Testbed—Aircraft Model Development. In Proceedings of the AIAA Guidance, Navigation, and Control Conference and Exhibit, San Francisco, CA, USA, 15–18 August 2005. [Google Scholar] [CrossRef]
- Jouannet, C.; Lundström, D.; Amadori, K.; Berry, P. Design of a Very Light Jet and a Dynamically Scaled Demonstrator. In Proceedings of the 46th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 7–8 January 2008. [Google Scholar] [CrossRef]
- Chambers, J.R. Modeling Flight, The Role of Scaled Free-Flight Models in Support of NASA’s Aerospace Programs; NASA Technical Report, National Aeronautics and Space Administration; NASA Headquarters: Washington, DC, UAS, 2010; ISBN 978-0-16-084633-5. [Google Scholar]
- Nguewo, D. Erstellung und Optimierung der Skalierungsgesetze zur Abschätzung der Aerodynamik und der Eigendynamik eines Flugzeugs auf der Basis von frei fliegenden Modellen. Ph.D. Thesis, University of Stuttgart, Institute of Aircraft Design, Stuttgart, Germany, 2007. [Google Scholar] [CrossRef]
- Baden, A. Entwurf eines Freiflugmodells des E-Motorseglers e-Genius unter Berücksichtigung der aerodynamischen Vergleichbarkeit. Bachelor’s Thesis, University of Stuttgart, Institute of Aircraft Design, Stuttgart, Germany, 2016. [Google Scholar]
- van Gorcum, J.J. Ready for Take-off: Scaled Flight Testing—An investigation into influence of scaling on the aerodynamic properties. Master’s Thesis, Delft University of Technology, Delft, The Netherlands, 2017. [Google Scholar]
- Lutz, T. Profilentwurf, Skript zur Vorlesung und Seminar; Lecture Notes; University of Stuttgart, Institute of Aerodynamics and Gas Dynamics: Stuttgart, Germany, 2007. [Google Scholar]
- Kugler, L. Analysis and characterization of the variable pitch propeller for the Scaled-down e-Genius. Bachelor’s Thesis, University of Stuttgart, Institute of Aircraft Design, Stuttgart, Germany, 2018. [Google Scholar]
- McCrink, M.H.; Gregory, J.W. Blade Element Momentum Modeling of Low-Re Small UAS Electric Propulsion Systems. In Proceedings of the 33rd AIAA Applied Aerodynamics Conference, Dallas, TX, USA, 22–26 June 2015. [Google Scholar] [CrossRef]



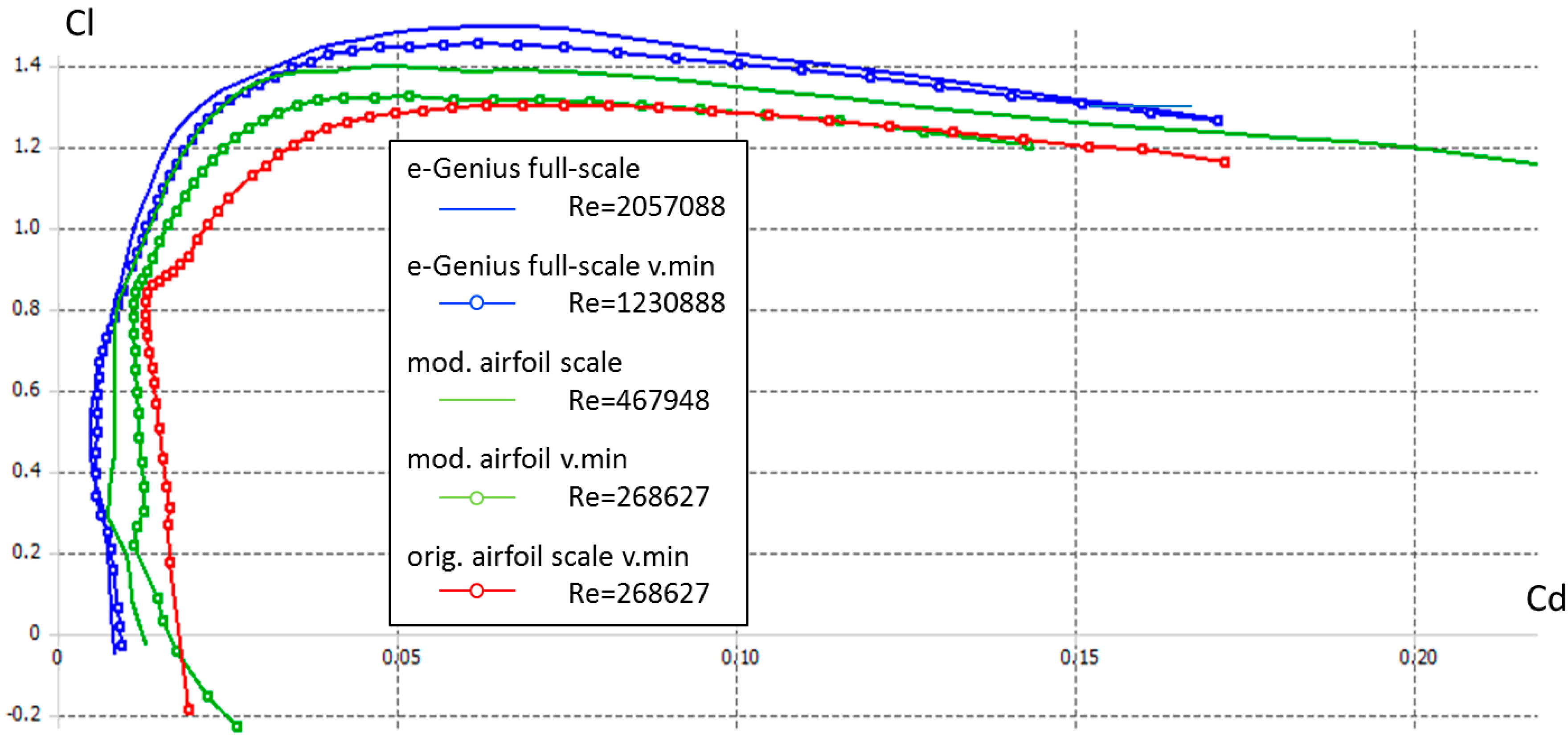
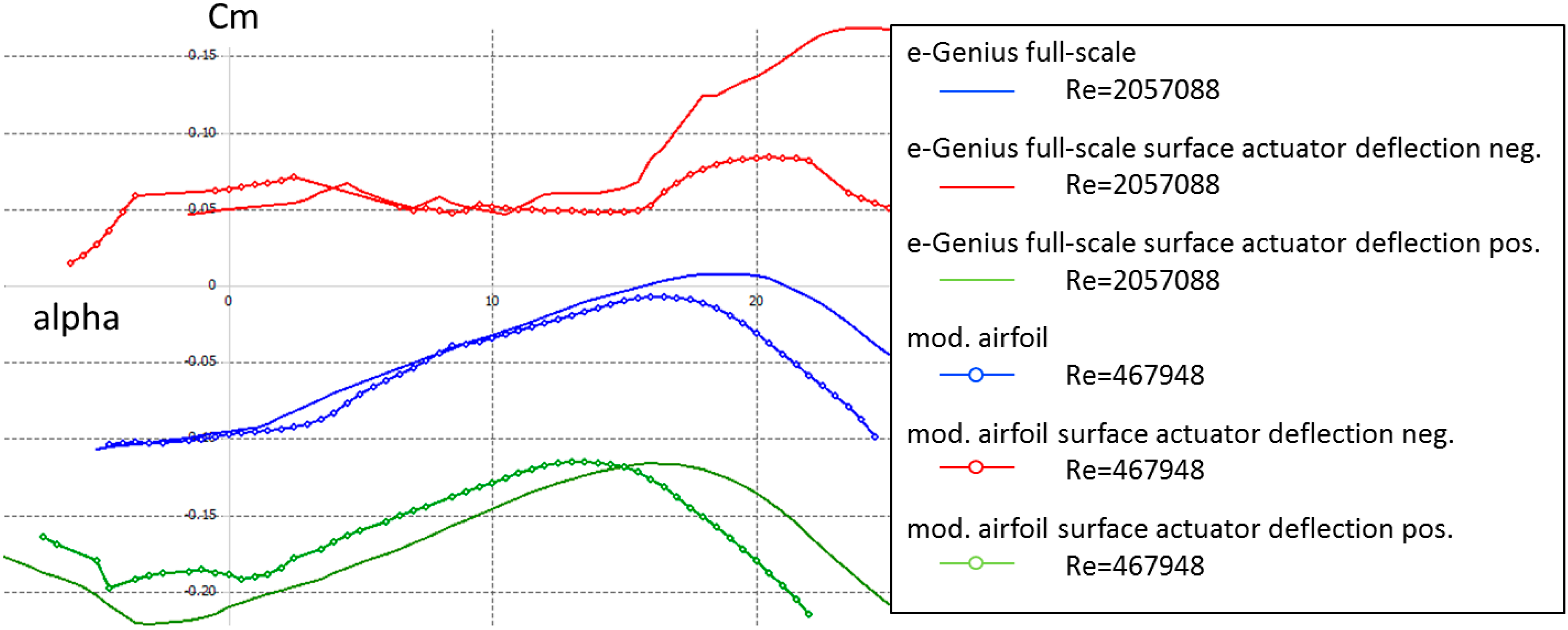
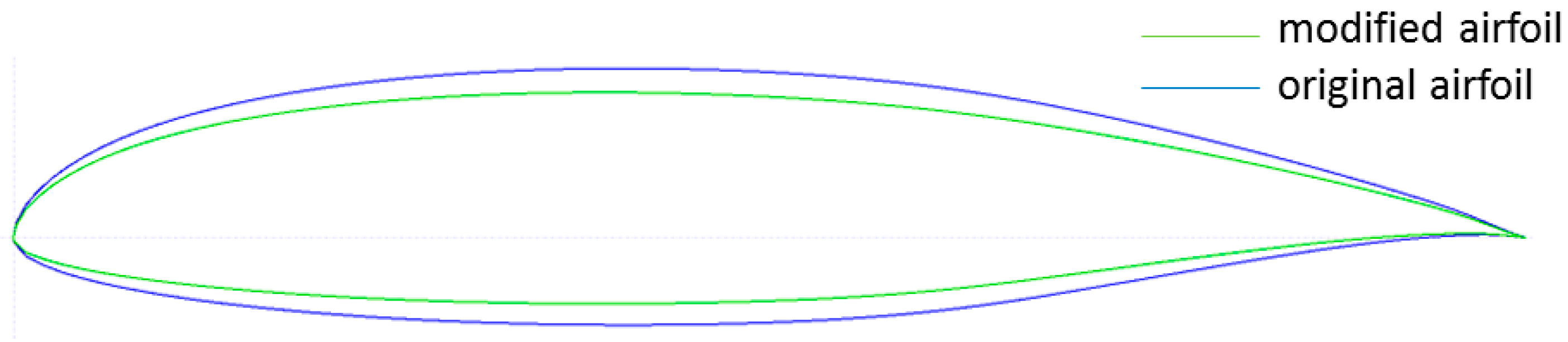


| Profil Parameter | Original Full-Scale Airfoil | Modified Scale Airfoil |
|---|---|---|
| Thickness/chord ratio (%) | 17.01 | 14.00 |
| Airfoil camber (%) | 2.71 | 2.62 |
| Location of maximum thickness (%) | 40.40 | 39.39 |
| Location of maximum camber (%) | 39.39 | 38.38 |
| Leading-edge radius (m) | 0.02026 | 0.01383 |
| Wingspan | 5.62 m |
| Aspect ratio | 20.16 |
| Length | 2.95 m |
| MTOM | 41 kg |
| Electric drive power | 5 kw |
| Payload | up to 10 kg |
| Design speed | 24.8 m/s |
| Max. speed | 35.6 m/s |
| Airframe | 2 Segments |
| Wing | 8 Segments |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bergmann, D.P.; Denzel, J.; Baden, A.; Kugler, L.; Strohmayer, A. Innovative Scaled Test Platform e-Genius-Mod—Scaling Methods and Systems Design. Aerospace 2019, 6, 20. https://doi.org/10.3390/aerospace6020020
Bergmann DP, Denzel J, Baden A, Kugler L, Strohmayer A. Innovative Scaled Test Platform e-Genius-Mod—Scaling Methods and Systems Design. Aerospace. 2019; 6(2):20. https://doi.org/10.3390/aerospace6020020
Chicago/Turabian StyleBergmann, Dominique Paul, Jan Denzel, Asmus Baden, Lucas Kugler, and Andreas Strohmayer. 2019. "Innovative Scaled Test Platform e-Genius-Mod—Scaling Methods and Systems Design" Aerospace 6, no. 2: 20. https://doi.org/10.3390/aerospace6020020
APA StyleBergmann, D. P., Denzel, J., Baden, A., Kugler, L., & Strohmayer, A. (2019). Innovative Scaled Test Platform e-Genius-Mod—Scaling Methods and Systems Design. Aerospace, 6(2), 20. https://doi.org/10.3390/aerospace6020020






