Experimental and Numerical Study on the Plasma-Laser-Induced Ignition of Strut Stabilizer at Different Locations
Abstract
1. Introduction
2. Materials and Methods
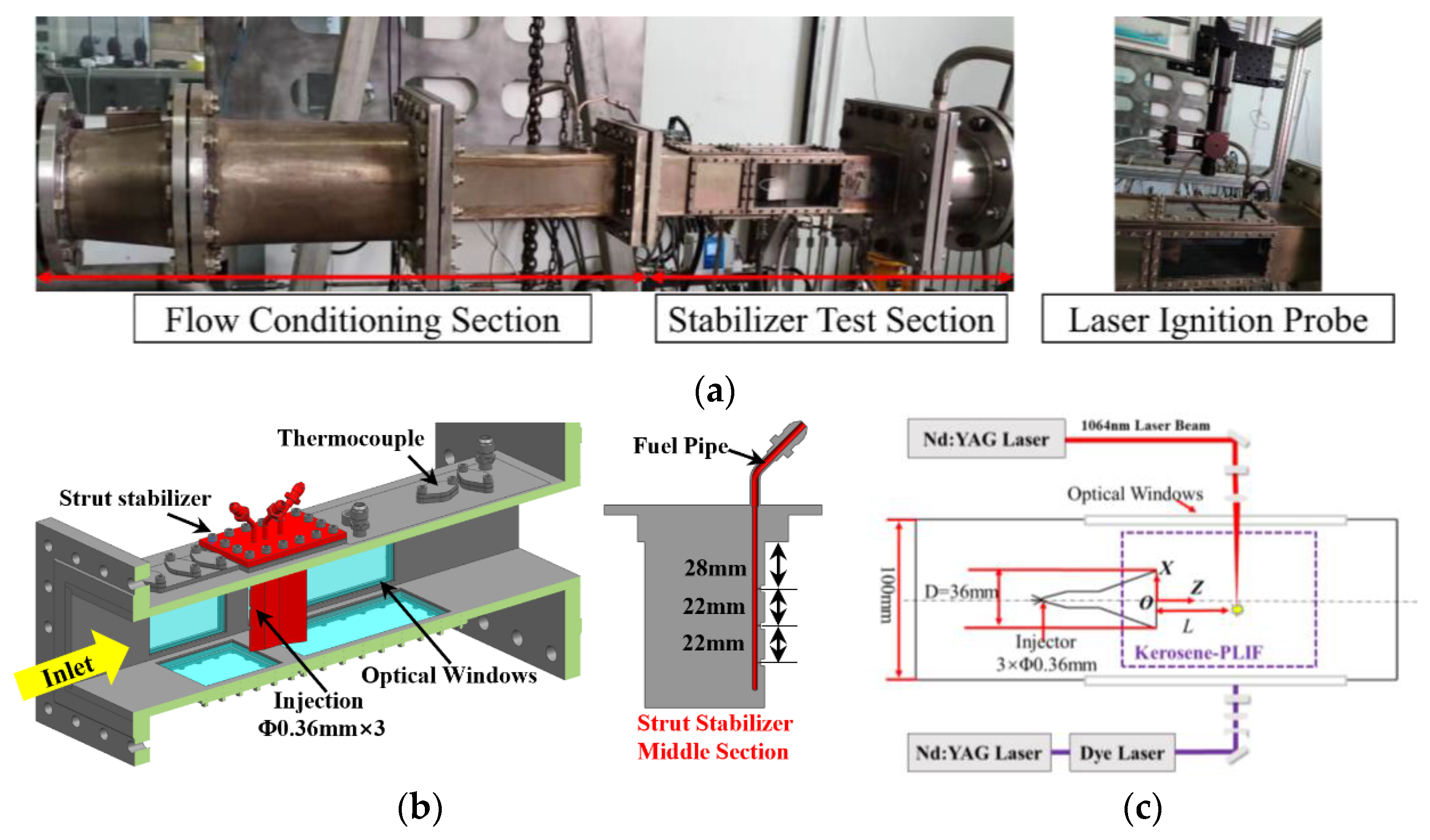
2.1. Experimental Methods
2.2. Numerical Simulation Methods
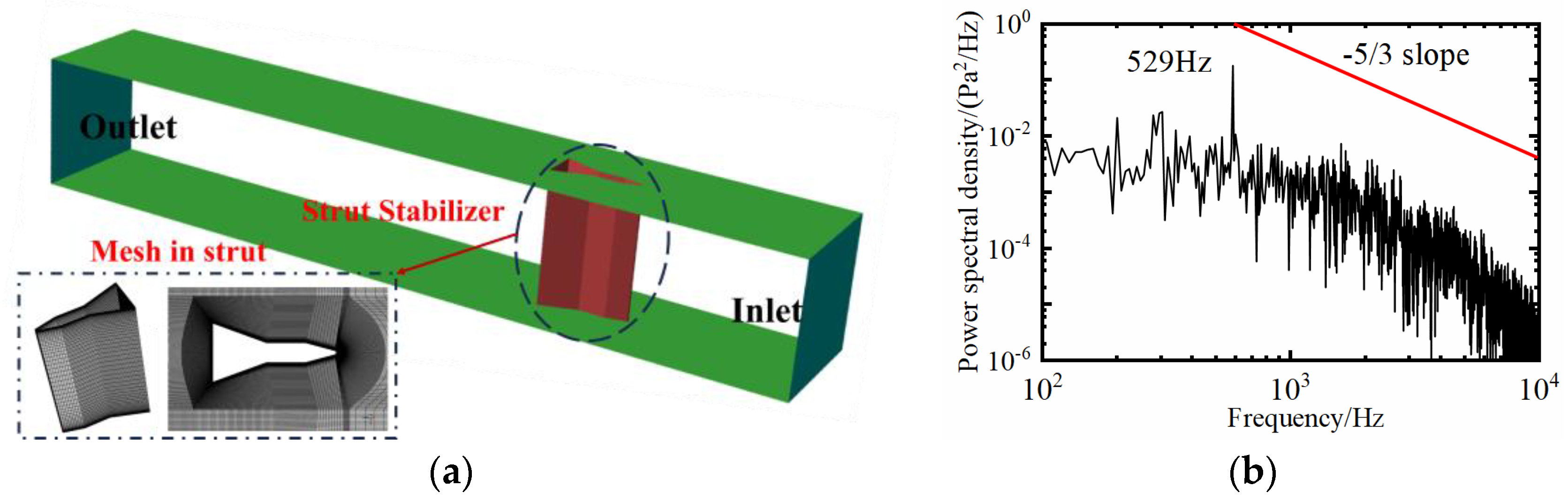
2.2.1. Computational Setup and Flow Conditions
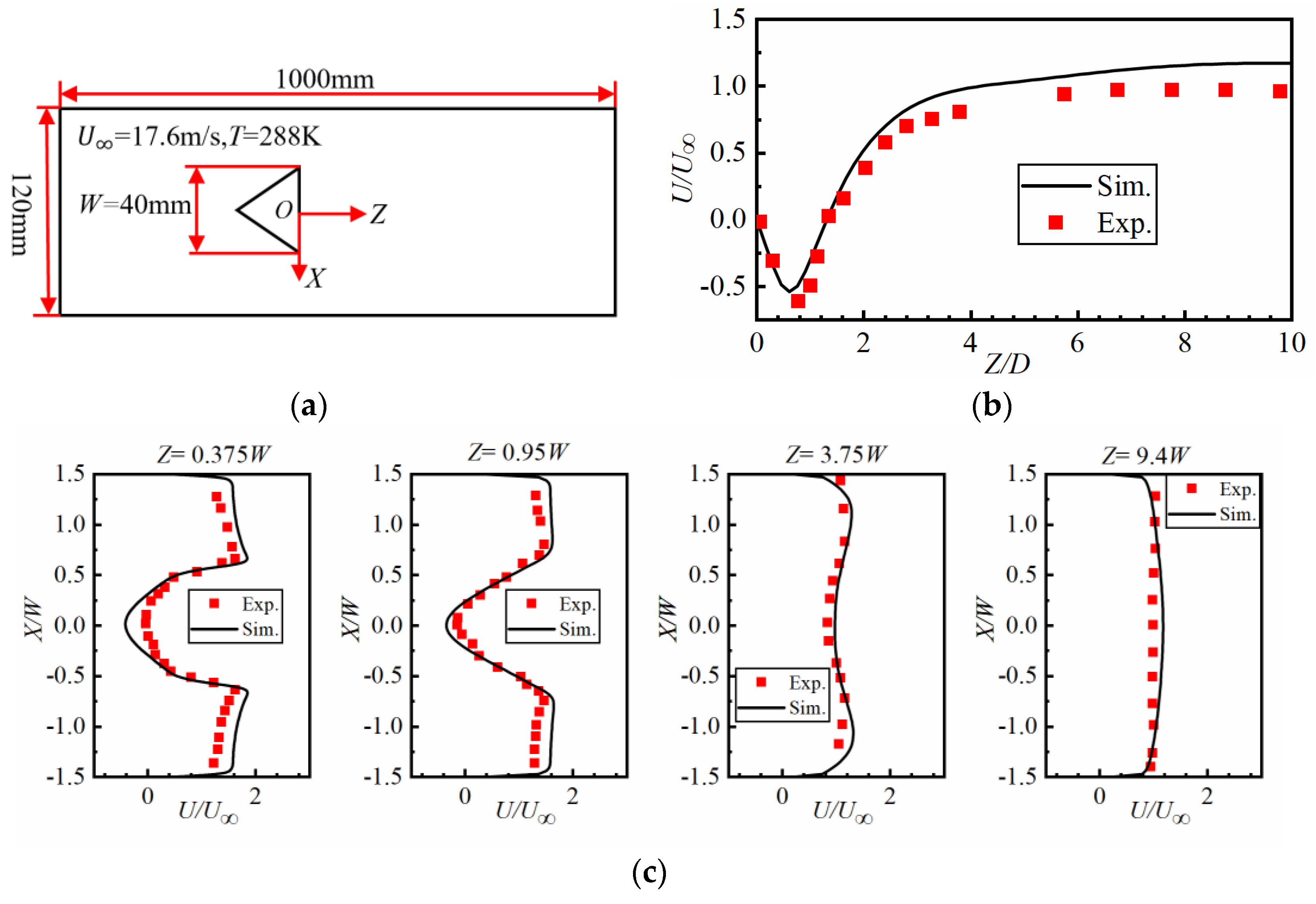
2.2.2. Validation of the Numerical Simulation Methods
3. Results and Discussion
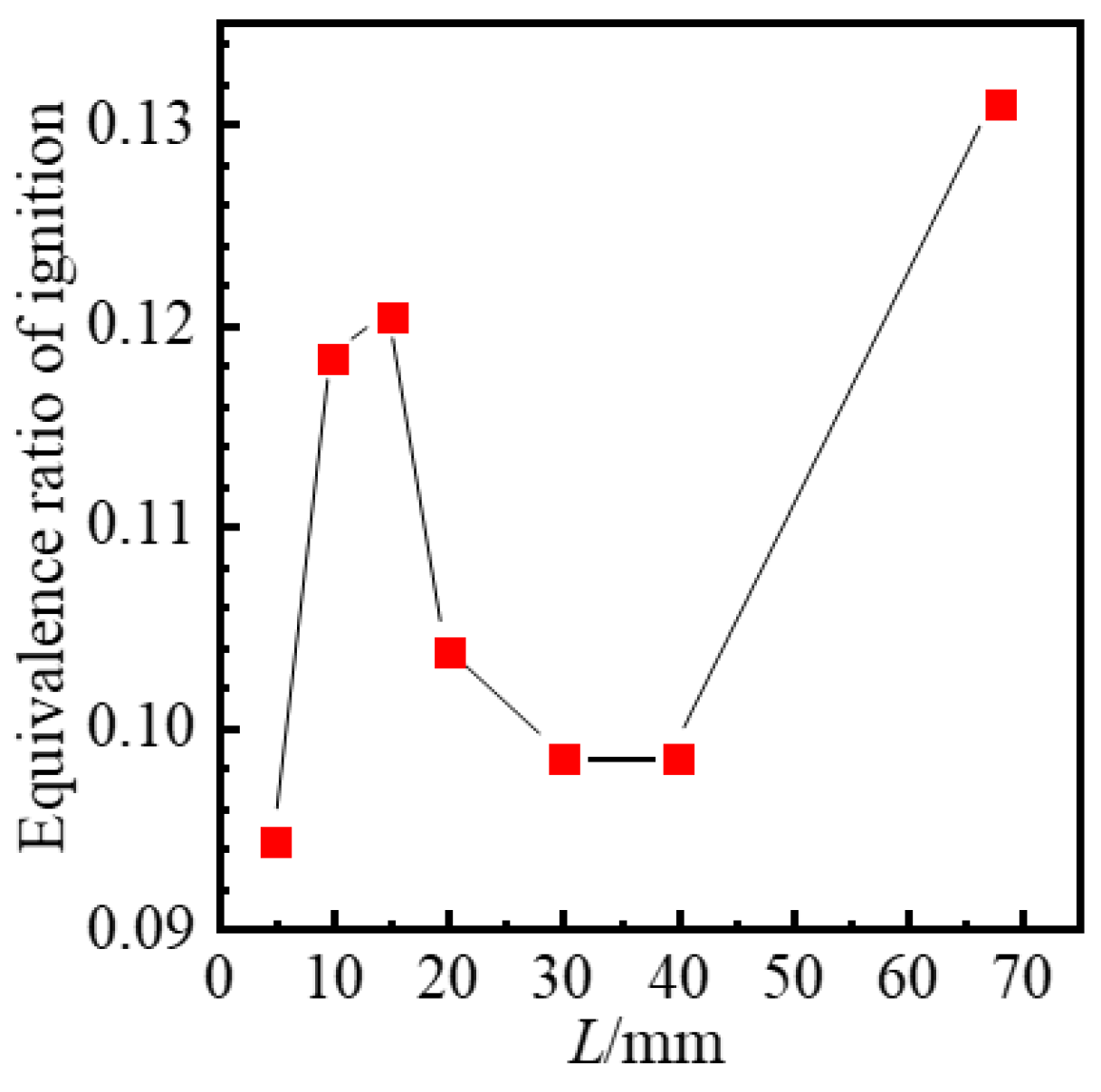
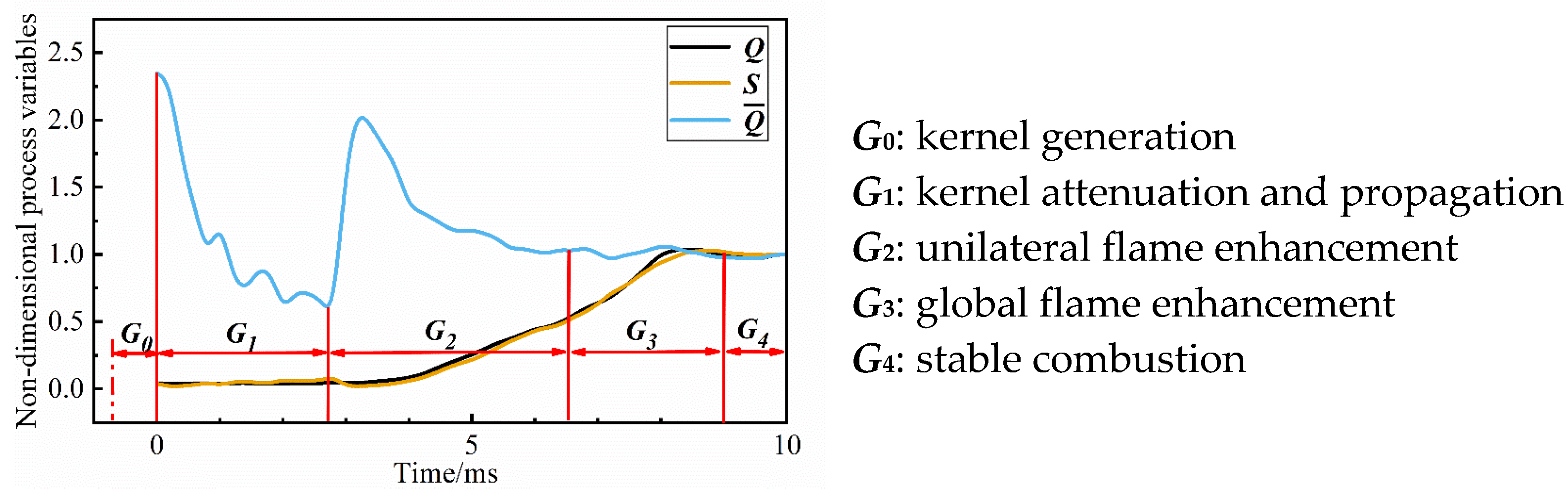
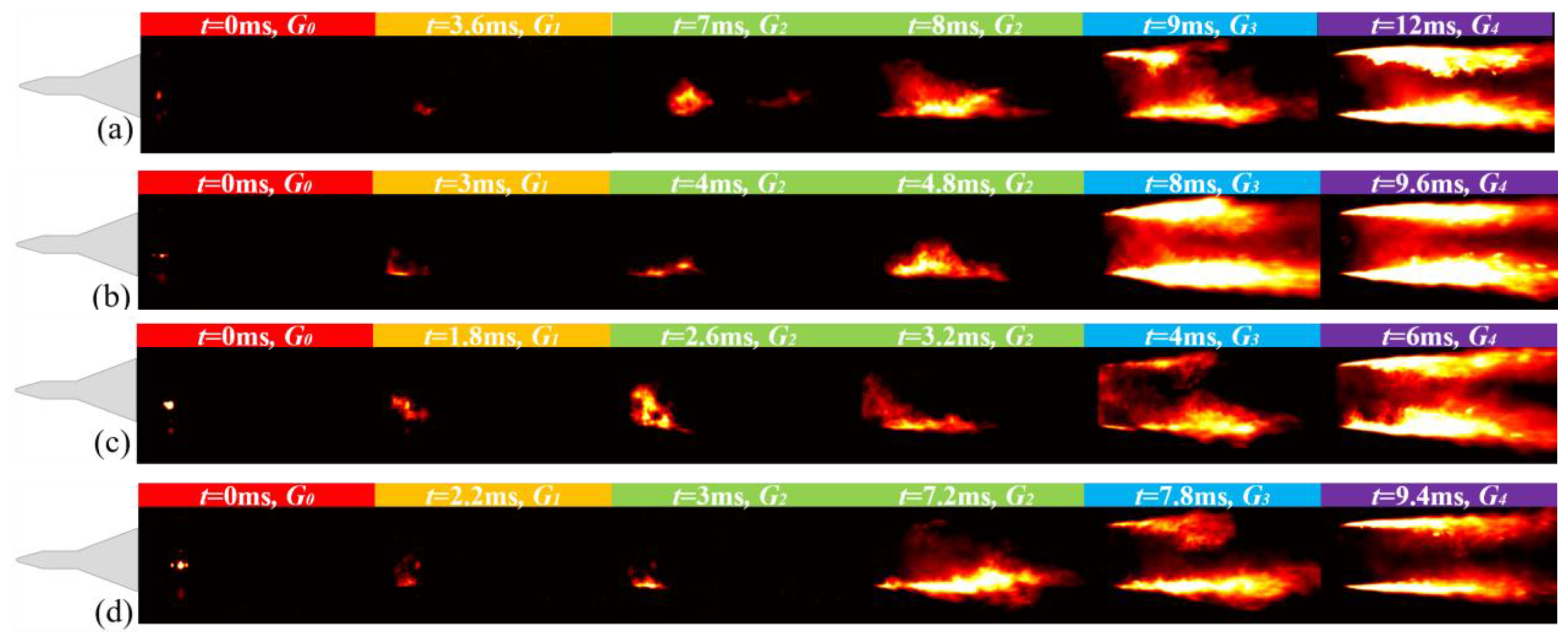
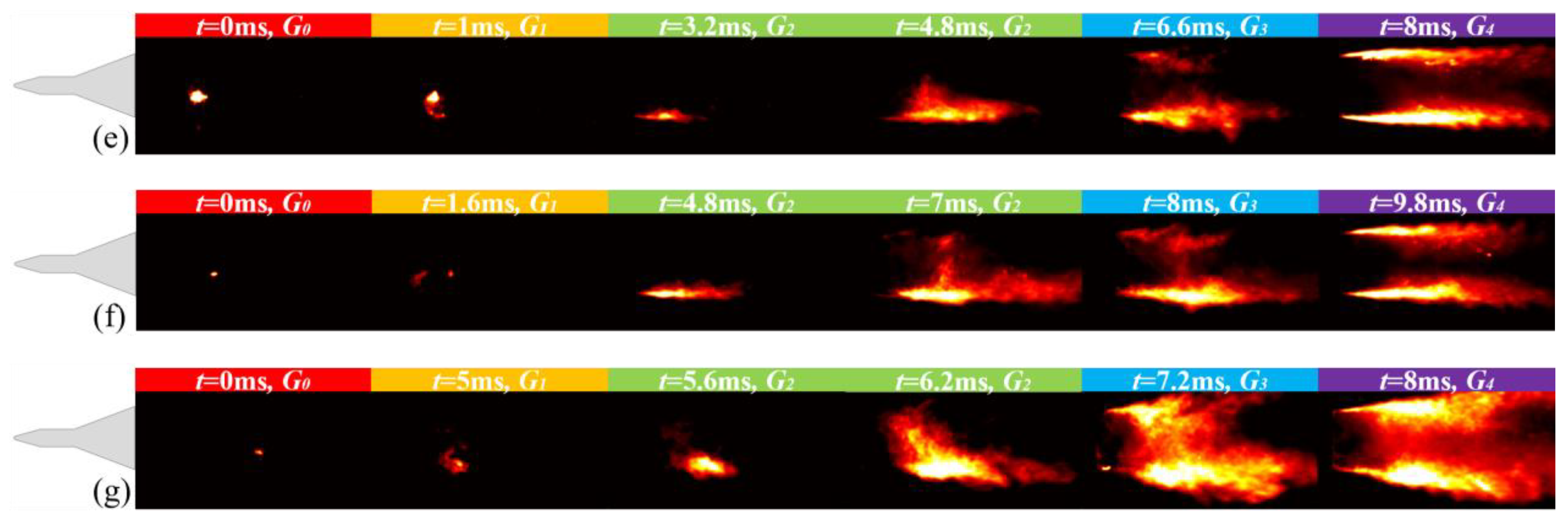
3.1. Ignition Performance and Processes at Different Locations
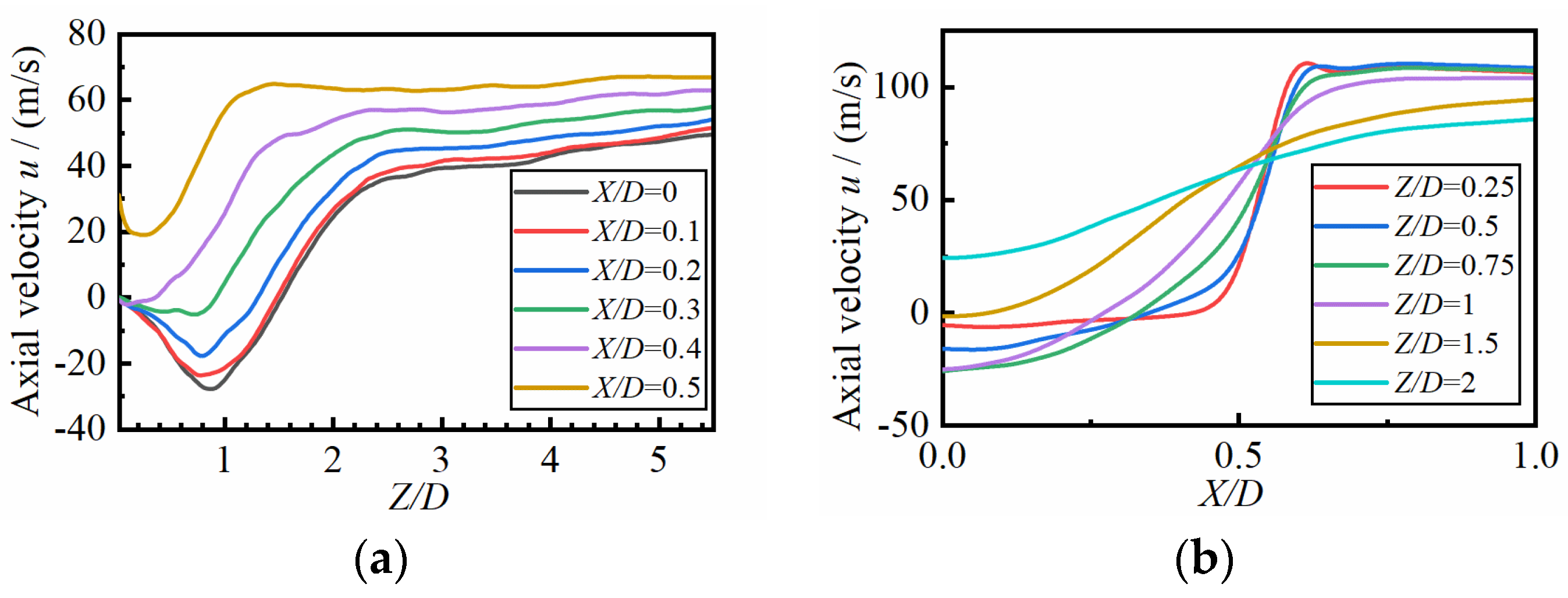
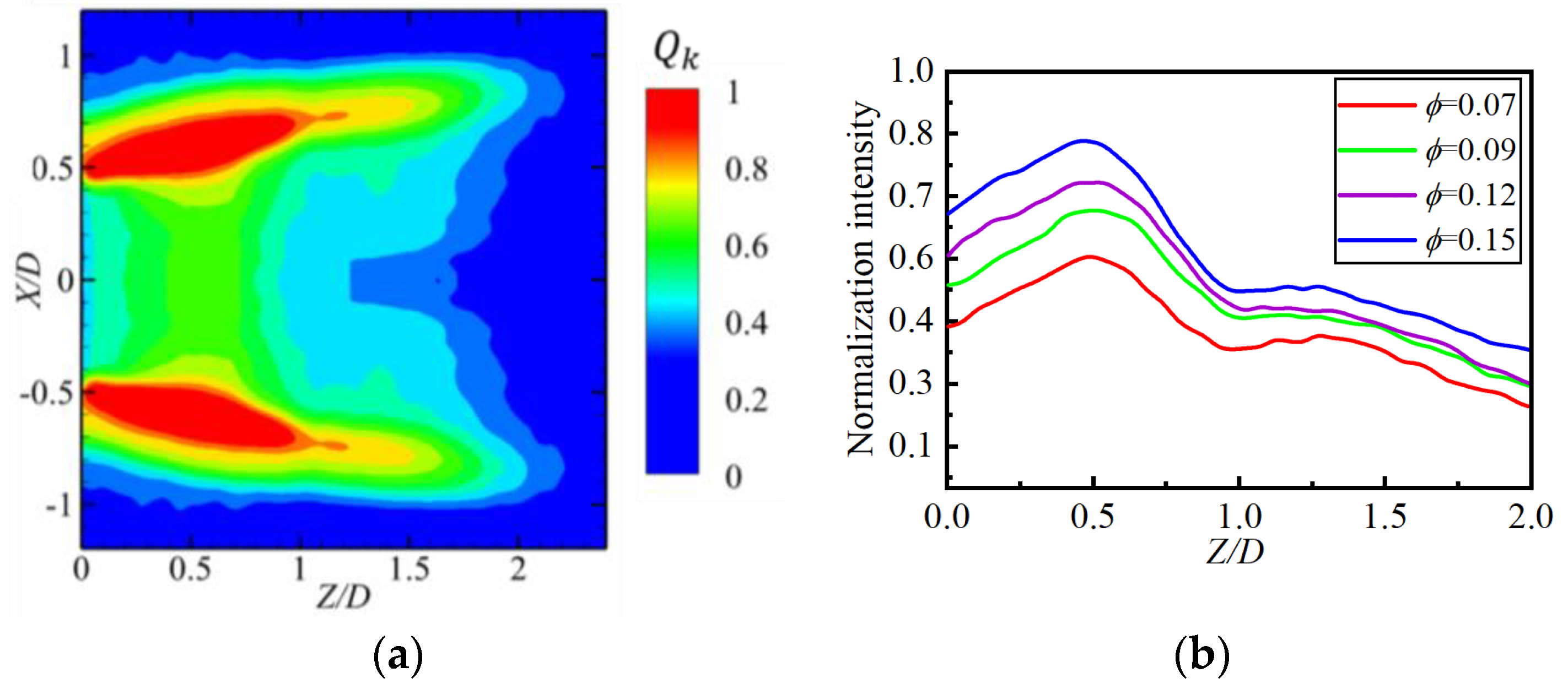
3.2. Non-Reacting Flow Field
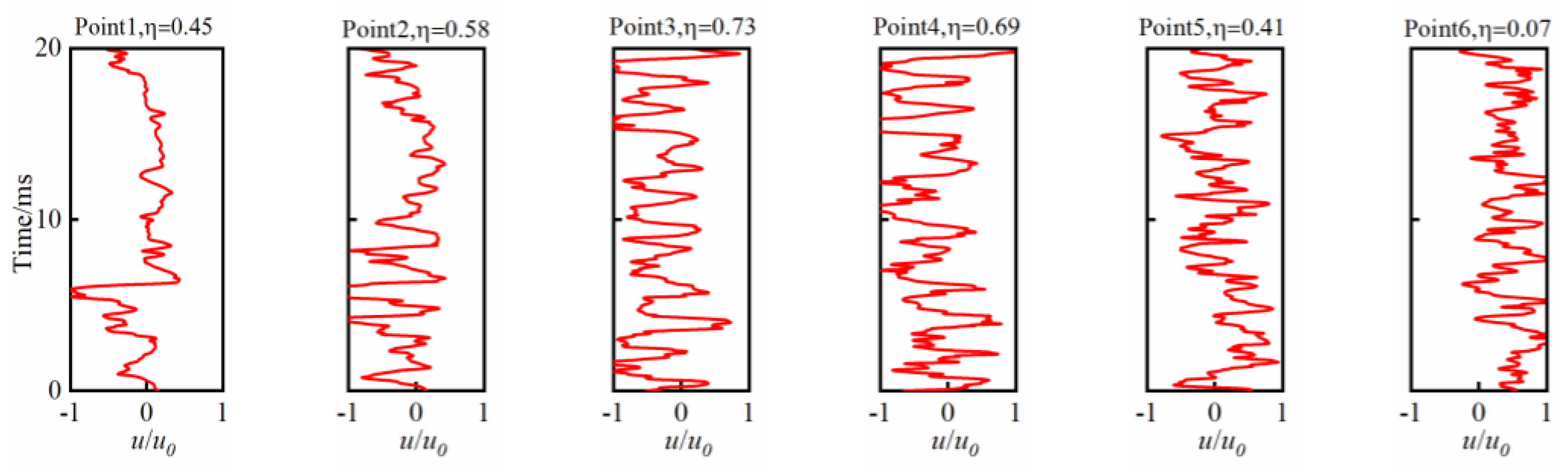
3.3. Kerosene Distribution Characteristics
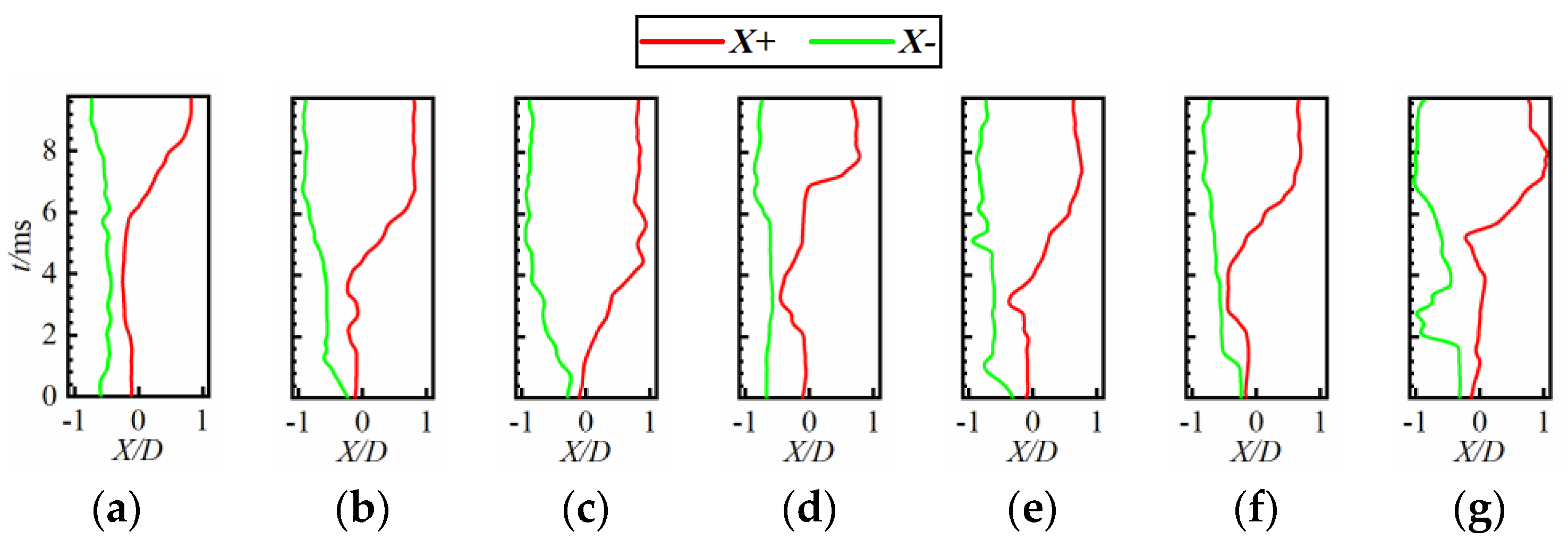
3.4. Flame Propagation Trajectory
4. Conclusions
- (1)
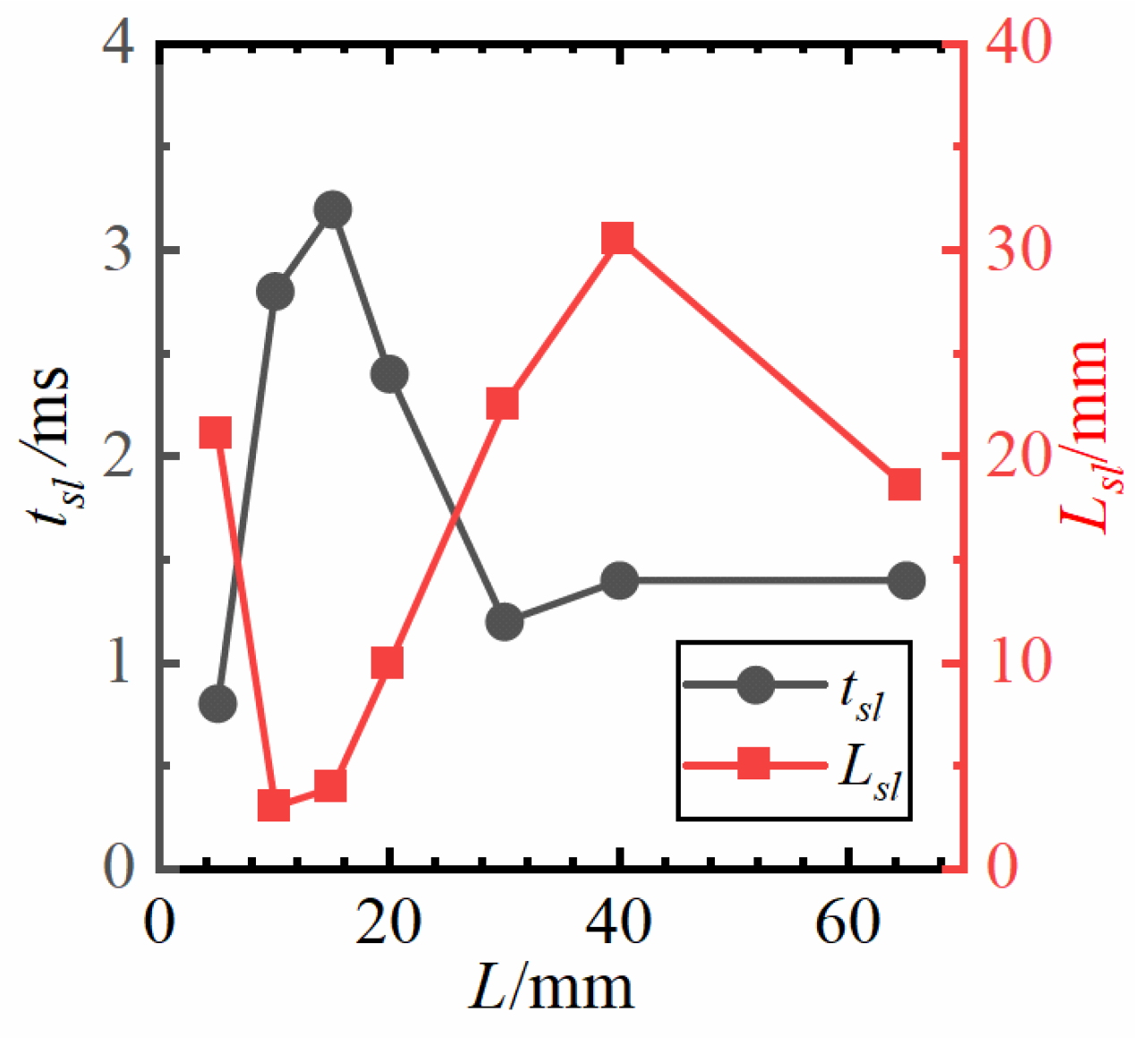
- The ignition test results show that when the ignition distance increases, the equivalence ratio of ignition first decreases (L = 5–15 mm) and then increases (L = 20–40 mm). When the ignition location is outside the recirculation zone (L = 65 mm), the ignition equivalence ratio reaches its highest value.
- (2)
- For different ignition locations, after the formation of the flame kernel, it propagates to the shear layer, the flame then rapidly expands, and the entire combustion chamber steadily burns. The flame propagation trajectory is related to the ignition location. When the ignition position is close to the tail edge of the stabilizer, the flame propagates directly downstream to the shear layer. When the ignition position is far from the stabilizer but still within the recirculation zone, the flame propagates upstream into the shear layer in a U-shaped pattern. When the ignition location is outside the recirculation zone, the flame also propagates in a U-shaped pattern, first into the recirculation zone and then into the shear layer.
- (3)
- When the ignition position is within the recirculation zone, the time for the flame to propagate to the shear layer is directly related to the ignition performance. If the flame stays in the shear layer for a longer time, there will be more energy loss during the flame propagation process, and the ignition performance deteriorates. Due to the different propagation modes at different ignition positions, it is difficult to determine the ignition performance based on the length of the flame propagation path. When the ignition position is outside the recirculation zone, the flame propagation distance upstream is shorter. Although the ignition equivalence ratio is relatively high, the time for the flame to propagate to the shear layer is shorter.
- (4)
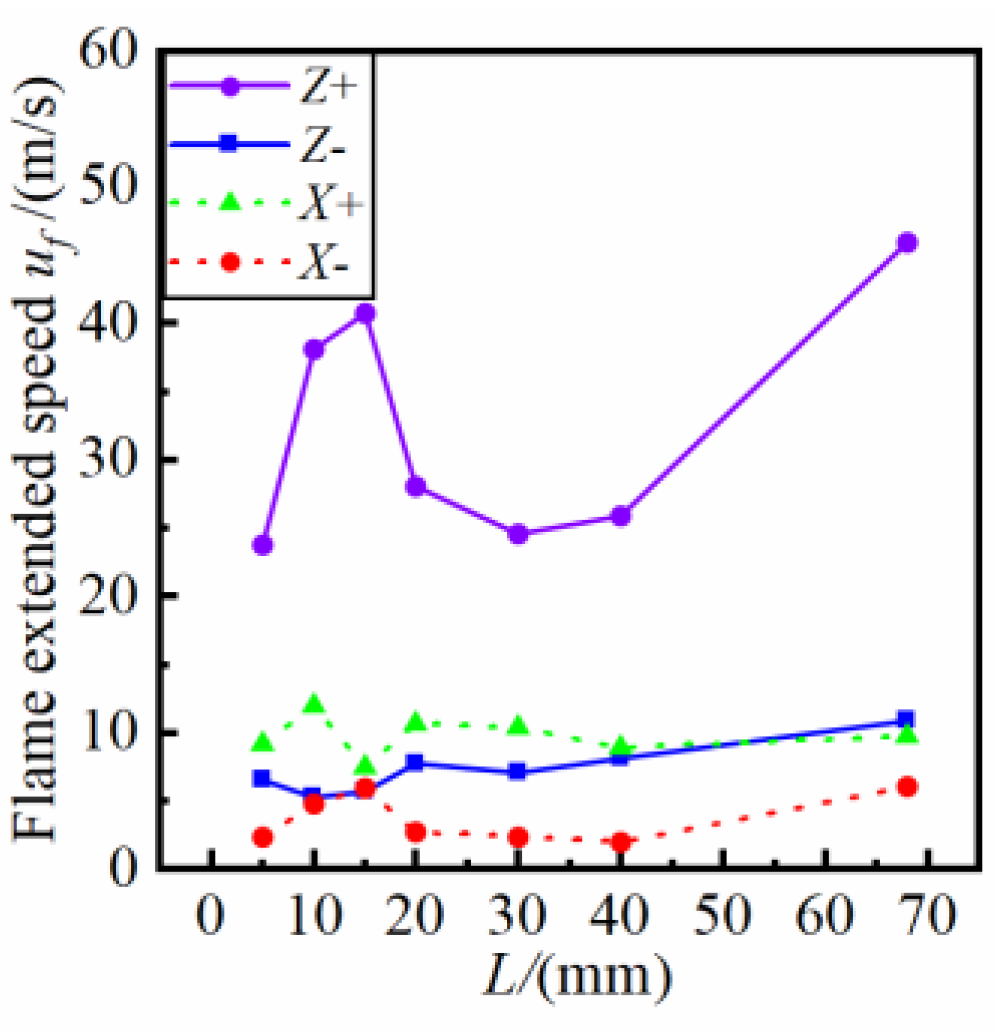
- The downstream extension speed of the flame after propagating to the shear layer is directly related to the ignition fuel-air ratio. When the latter is higher, the expansion angle of the flame is greater. However, for the shear layer, a position farther away from the centerline leads to a greater axial velocity. The downstream extension speed of the flame is consistent with the axial velocity of the shear layer. The expansion of flames downstream is mainly affected by the turbulent velocity within the shear layer. The speed of flame propagation upstream is in the range of 5–11 m/s, independent of the equivalence ratio and ignition oil gas ratio, and mainly driven by the turbulent flame speed and flame dilatation.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Inamura, T.; Takahashi, M.; Kumakawa, A. Combustion characteristics of a liquid-fueled ramjet combustor. J. Propuls. Power 2001, 17, 860–868. [Google Scholar] [CrossRef]
- Wadia, A.R.; James, F.D. F110-GE-132: Enhanced Power Through Low-Risk Derivative Technology. J. Turbomach. 2001, 123, 544–551. [Google Scholar] [CrossRef]
- Li, W.; Tan, X.; Huang, X.; Wei, Y.; Deng, Y. Application of double-wall cooling structure in the integrated strut flame stabilizer. Therm. Sci. Eng. Prog. 2022, 36, 101526. [Google Scholar] [CrossRef]
- Ebrahimi, H. Overview of gas turbine augmentor design, operation, and combustion oscillation. In Proceedings of the 42nd AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Sacramento, CA, USA, 9–12 July 2006. [Google Scholar]
- Dagaut, P.; Cathonnet, M. The ignition, oxidation, and combustion of kerosene: A review of experimental and kinetic modeling. Prog. Energy Combust. Sci. 2006, 32, 48–92. [Google Scholar] [CrossRef]
- Clenci, A.; Berquez, J.; Stoica, R.; Niculescu, R.; Cioc, B.; Zaharia, C.; Iorga-Simăn, V. Experimental investigation of the effect of an afterburner on the light-off performance of an exhaust after-treatment system. Energy Rep. 2022, 8, 406–418. [Google Scholar] [CrossRef]
- Huang, Y.; He, X.; Jin, Y.; Zhu, H.; Zhu, Z. Effect of non-uniform inlet profile on the combustion performance of an afterburner with bluff body. Energy 2021, 216, 119142. [Google Scholar] [CrossRef]
- Tonarely, M.E.; Fortin, M.K.; Genova, T.; Morales, A.; Stiehl, B.; Ahmed, K.A. C2*/CH* Intensity Ratios of Bluff Body Stabilized Flames Approaching Lean Blowout at Elevated Pressures. In Proceedings of the AIAA SCITECH 2022 Forum, San Diego, CA, USA, 3–7 January 2022. [Google Scholar]
- Ivanov, V.S.; Frolov, S.M.; Zangiev, A.E.; Zvegintsev, V.I.; Shamshin, I.O. Hydrogen fueled detonation ramjet: Conceptual design and test fires at Mach 1.5 and 2.0. Aerosp. Sci. Technol. 2021, 109, 106459. [Google Scholar] [CrossRef]
- Tsuboi, S.; Miyokawa, S.; Matsuda, M.; Yokomori, T.; Iida, N. Influence of spark discharge characteristics on ignition and combustion process and the lean operation limit in a spark ignition engine. Appl. Energy 2019, 250, 617–632. [Google Scholar] [CrossRef]
- Morales, A.J.; Lasky, I.M.; Geikie, M.K.; Engelmann, C.A.; Ahmed, K.A. Mechanisms of flame extinction and lean blowout of bluff body stabilized flames. Combust. Flame 2019, 203, 31–45. [Google Scholar] [CrossRef]
- Badawy, T.; Bao, X.C.; Xu, H. Impact of spark plug gap on flame kernel propagation and engine performance. Appl. Energy 2017, 191, 311–327. [Google Scholar] [CrossRef]
- Tilz, A.; Kiesling, C.; Meyer, G.; Nickl, A.; Pirker, G.; Wimmer, A. Experimental investigation of the influence of ignition system parameters on combustion behavior in large lean burn spark ignited gas engines. Exp. Therm. Fluid Sci. 2020, 119, 110176. [Google Scholar] [CrossRef]
- Zhu, S.; Akehurst, S.; Lewis, A.; Yuan, H. A review of the pre-chamber ignition system applied on future low-carbon spark ignition engines. Renew. Sustain. Energy Rev. 2022, 154, 111872. [Google Scholar] [CrossRef]
- Lovett, J.; Brogan, T.; Philippona, D.; Kiel, B.; Thompson, T. Development needs for advanced afterburner designs. In Proceedings of the 40th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Fort Lauderdale, FL, USA, 11–14 July 2004; p. 4192. [Google Scholar]
- Morsy, M.H. Review and recent developments of laser ignition for internal combustion engines applications. Renew. Sustain. Energy Rev. 2012, 16, 4849–4875. [Google Scholar] [CrossRef]
- Patane, P.; Nandgaonkar, M. Multipoint laser ignition system and its applications to IC engines. Opt. Laser Technol. 2020, 130, 106305. [Google Scholar] [CrossRef]
- O’Briant, S.A.; Gupta, S.B.; Vasu, S.S. Laser ignition for aerospace propulsion. Propuls. Power Res. 2016, 5, 1–21. [Google Scholar] [CrossRef]
- Pavel, N.; Bärwinkel, M.; Heinz, P.; Brüggemann, D.; Dearden, G.; Croitoru, G.; Grigore, O.V. Laser ignition-Spark plug development and application in reciprocating engines. Prog. Quantum Electron. 2018, 58, 1–32. [Google Scholar] [CrossRef]
- Ju, Y.; Sun, W. Plasma assisted combustion: Dynamics and chemistry. Prog. Energy Combust. Sci. 2015, 48, 21–83. [Google Scholar] [CrossRef]
- Andrey, S.; Nickolay, A. Plasma-assisted ignition and combustion. Prog. Energy Combust. Sci. 2013, 39, 61–110. [Google Scholar]
- Gerhard, K.; Georg, F.; Ernst, W. Novel miniaturized high-energy Nd-YAG laser for spark ignition in internal combustion engines. Optical. Eng. 2009, 48, 014202. [Google Scholar]
- Gupta, S.B. Fouling Mitigation for Laser Igniters in Natural Gas Engines. ASME J. Eng. Gas Turbines Power 2022, 144, 041020. [Google Scholar] [CrossRef]
- Tsunekane, M.; Inohara, T.; Ando, A.; Kido, N.; Kanehara, K.; Taira, T. High peak power, passively q-switched microlaser for ignition of engines. IEEE J. Quantum Electron. 2010, 46, 277–284. [Google Scholar] [CrossRef]
- Moesl, K.G.; Vollmer, K.G.; Sattelmayer, T.; Eckstein, J.; Kopecek, H. Experimental study on laser-induced ignition of swirl-stabilized kerosene flames. J. Eng. Foe Gas Turbines Power 2009, 131, 021501. [Google Scholar] [CrossRef]
- You, B.; Liu, X.; Kong, L.; Yang, J.; Chen, M.; Zheng, H.; Li, S. Experimental study on direct ignition of blast furnace gas by plasma igniter in a gas turbine combustor. Part C J. Mech. Eng. Sci. 2022, 236, 9306–9315. [Google Scholar] [CrossRef]
- Kopecek, H.; Maier, H.; Reider, G.; Winter, F.; Wintner, E. Laser ignition of methane–air mixtures at high pressures. Exp. Therm. Fluid Sci. 2003, 27, 499–503. [Google Scholar] [CrossRef]
- Seunghyun, J.; Jay, P. Laser ignition energy for turbulent premixed hydrogen air jets. Combust. Flame 2022, 236, 111767. [Google Scholar]
- Weinrotter, M.; Kopecek, H.; Wintner, E.; Lackner, M.; Winter, F. Application of laser ignition to hydrogen–air mixtures at high pressures. Int. J. Hydrog. Energy 2005, 30, 319–326. [Google Scholar] [CrossRef]
- Weinrotter, M.; Kopecek, H.; Tesch, M.; Wintner, E.; Lackner, M.; Winter, F. Laser ignition of ultra-lean methane/hydrogen/air mixtures at high temperature and pressure. Exp. Therm. Fluid Sci. 2005, 29, 569–577. [Google Scholar] [CrossRef]
- An, B.; Wang, Z.; Yang, L.; Li, X.; Liu, C. The ignition characteristics of the close dual-point laser ignition in a cavity based scramjet combustor. Exp. Therm. Fluid Sci. 2019, 101, 136–140. [Google Scholar] [CrossRef]
- Lyon, E.; Kuang, Z.; Cheng, H.; Page, V.; Shenton, T.; Dearden, G. Multi-point laser spark generation for internal combustion engines using a spatial light modulator. J. Phys. D Appl. Phys. 2014, 47, 475501. [Google Scholar] [CrossRef]
- Mastorakos, E. Ignition of turbulent non-premixed flames. Prog. Energy Combust. Sci. 2009, 35, 57–97. [Google Scholar] [CrossRef]
- Sitte, M.P.; Bach, E.; Kariuki, J.; Bauer, H.J.; Mastorakos, E. Simulations and experiments on the ignition probability in turbulent premixed bluff-body flames. Combust. Theory Model. 2016, 20, 548–565. [Google Scholar] [CrossRef]
- Bourgouin, J.F.; Durox, D.; Schuller, T.; Beaunier, J.; Candel, S. Ignition dynamics of an annular combustor equipped with multiple swirling injectors. Combust. Flame 2013, 160, 1398–1413. [Google Scholar] [CrossRef]
- Marchione, T.; Ahmed, S.F.; Mastorakos, E. Ignition of turbulent swirling n-heptane spray flames using single and multiple sparks. Combust. Flame 2009, 156, 166–180. [Google Scholar] [CrossRef]
- Wang, X.; Huang, Y.; Liu, Y.; Sun, L. Effect of the ignition location on lean light-off limits for a gas turbine combustor. Combust. Flame 2022, 245, 112295. [Google Scholar] [CrossRef]
- Ciardiello, R.; de Oliveira, P.M.; Skiba, A.W.; Mastorakos, E.; Allison, P.M. Effect of spark location and laminar flame speed on the ignition transient of a premixed annular combustor. Combust. Flame 2020, 221, 296–310. [Google Scholar] [CrossRef]
- Ciardiello, R.; Pathania, R.S.; Allison, P.M.; de Oliveira, P.M.; Mastorakos, E. Ignition probability and lean ignition behavior of a swirled premixed bluff body stabilized annular combustor. J. Eng. Gas Turbines Power 2021, 143, 041024. [Google Scholar] [CrossRef]
- Ciardiello, R. Transient phenomena in annular combustors. Doctoral Dissertation, University of Cambridge, Cambridge, UK, 2021. [Google Scholar]
- Lin, B.X.; Wu, Y.; Xu, M.X.; Chen, Z.G. Experimental investigation on spark ignition and flame propagation of swirling kerosene spray flames. Fuel 2021, 303, 121254. [Google Scholar] [CrossRef]
- Mao, Y.; Yu, L.; Wu, Z.; Tao, W.; Wang, S.; Ruan, C.; Zhu, L.; Lu, X. Experimental and kinetic modeling study of ignition characteristics of RP-3 kerosene over low-to-high temperature ranges in a heated rapid compression machine and a heated shock tube. Combust. Flame 2019, 203, 157–169. [Google Scholar] [CrossRef]
- Hinze, J.O. Experimental investigation on secondary currents in the turbulent flow through a straight conduit. Appl. Sci. Res. 1973, 28, 453–465. [Google Scholar] [CrossRef]
- Ducros, F.; Nicoud, F.; Poinsot, T. Wall-adapting local eddy-viscosity models for simulations in complex geometries. Numer. Methods Fluid Dyn. VI 1998, 6, 293–299. [Google Scholar]
- Sjunnesson, A.; Henriksson, R.; Löfström, C. CARS measurements and visualization of reacting flows in bluffbody stabilized flame. In Proceedings of the 28th Joint Propulsion Conference and Exhibit, Nashville, TN, USA, 6–8 July 1992. [Google Scholar]
- Otsu, N. A threshold selection method from gray-level histograms. IEEE Trans. Syst. Man Cybern. 1979, 9, 62–66. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jia, X.; Hu, B.; Zhao, W.; Zeng, W.; Peng, J.; Zhao, Q. Experimental and Numerical Study on the Plasma-Laser-Induced Ignition of Strut Stabilizer at Different Locations. Aerospace 2024, 11, 652. https://doi.org/10.3390/aerospace11080652
Jia X, Hu B, Zhao W, Zeng W, Peng J, Zhao Q. Experimental and Numerical Study on the Plasma-Laser-Induced Ignition of Strut Stabilizer at Different Locations. Aerospace. 2024; 11(8):652. https://doi.org/10.3390/aerospace11080652
Chicago/Turabian StyleJia, Xin, Bin Hu, Wei Zhao, Wen Zeng, Jiangbo Peng, and Qingjun Zhao. 2024. "Experimental and Numerical Study on the Plasma-Laser-Induced Ignition of Strut Stabilizer at Different Locations" Aerospace 11, no. 8: 652. https://doi.org/10.3390/aerospace11080652
APA StyleJia, X., Hu, B., Zhao, W., Zeng, W., Peng, J., & Zhao, Q. (2024). Experimental and Numerical Study on the Plasma-Laser-Induced Ignition of Strut Stabilizer at Different Locations. Aerospace, 11(8), 652. https://doi.org/10.3390/aerospace11080652





