Investigations on Trimming Strategy and Unsteady Aerodynamic Characteristics of Tiltrotor in Conversion Procedure
Abstract
1. Introduction
2. Aerodynamic Trimming Method of Tiltrotor in Conversion Procedure
2.1. Numerical Methods for Aerodynamic Forces Simulation of Tiltrotor
2.2. Flight Dynamic and Balance Equations of Tiltrotor
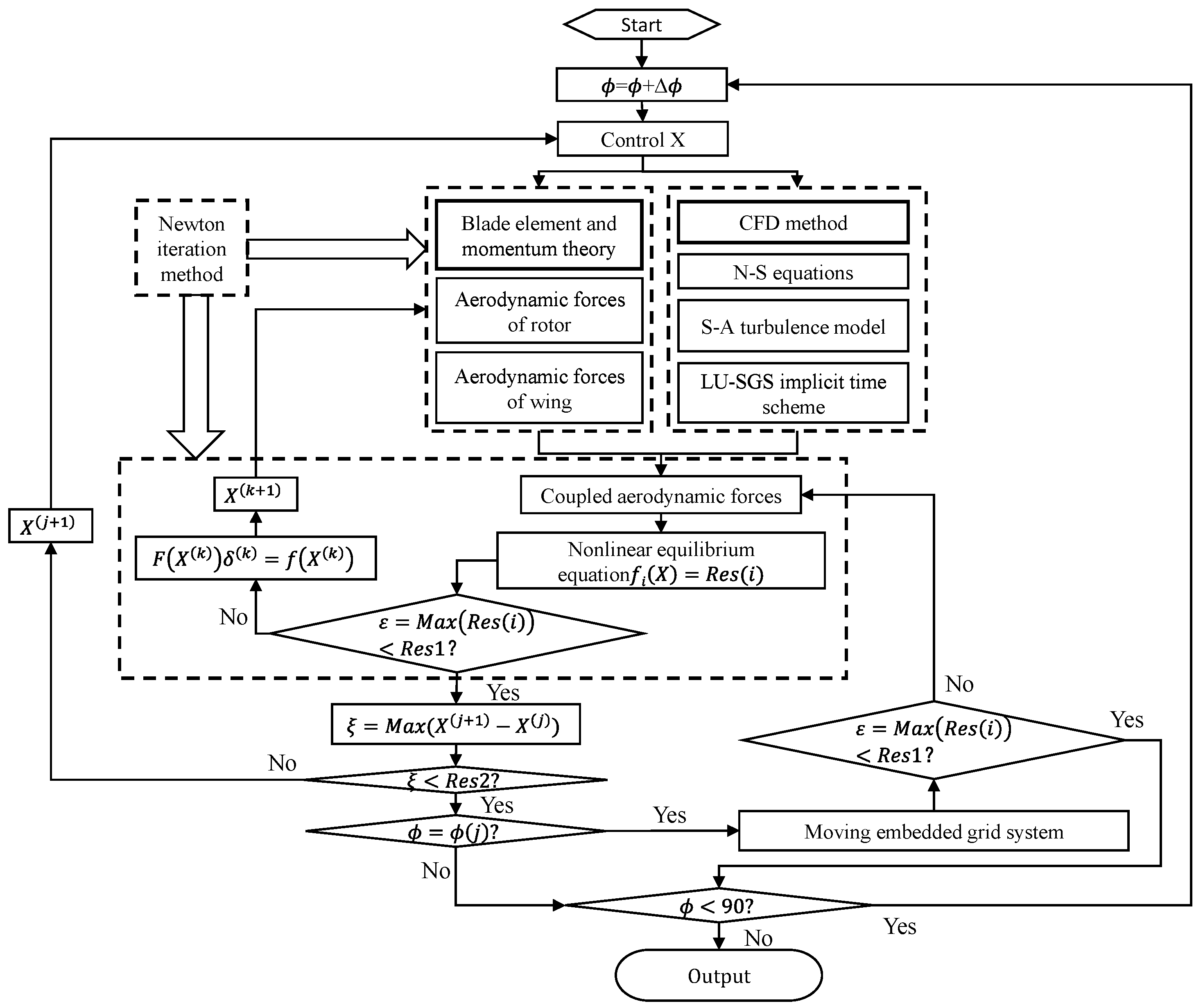
2.3. Coupling Trimming Method of Tiltrotor and Wing in Conversion Procedure
- Definition of the initial value ,
- Calculation of ,
- If is satisfied, the solution of the equations is , and the solution is completed; otherwise, the next step is entered,
- Computation of the Jacobian matrix ,
- Equation AZ = Res is solved, where , and is calculated,
- Calculation of ,
- The iteration step is updated by , and step 2 is returned until convergence.
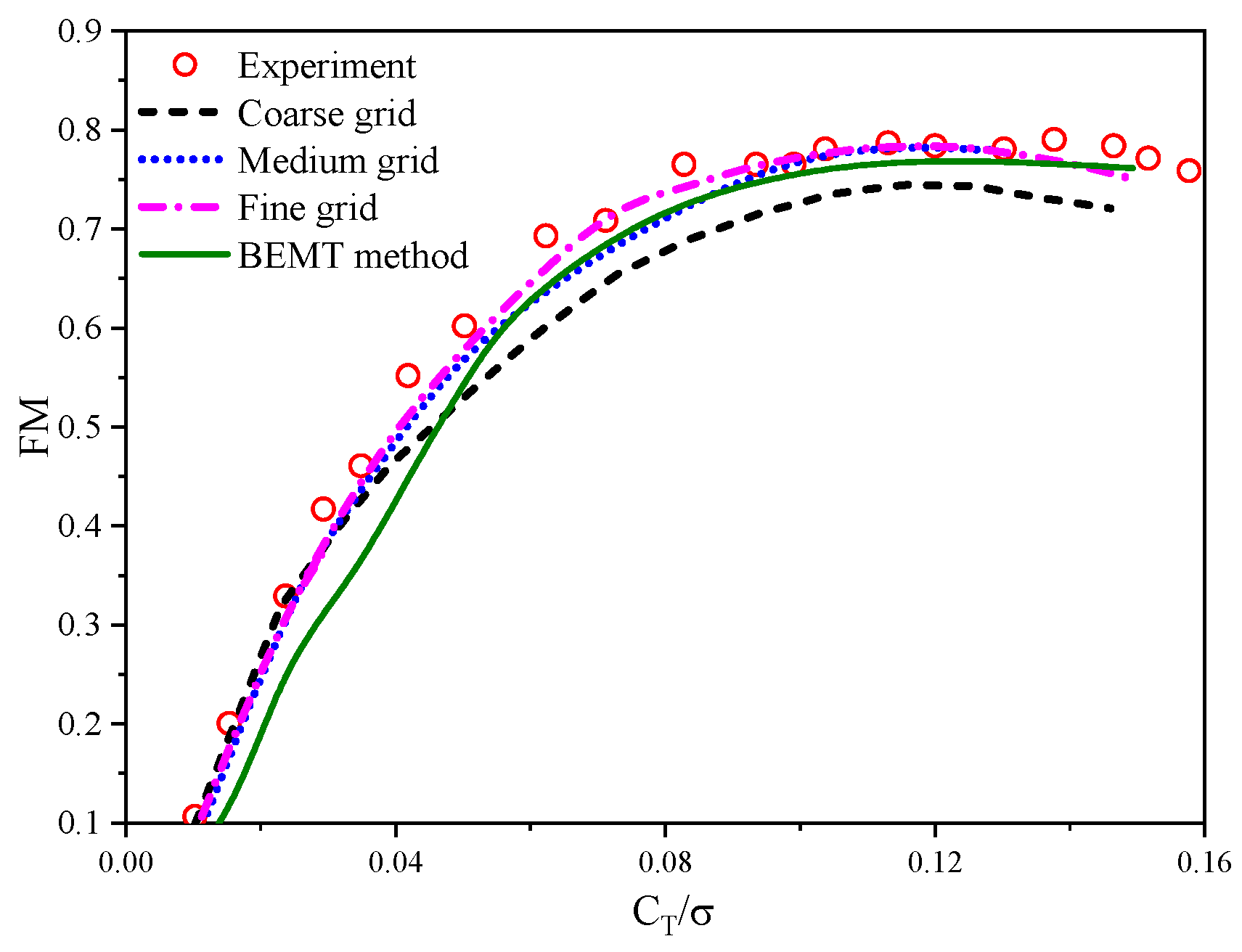
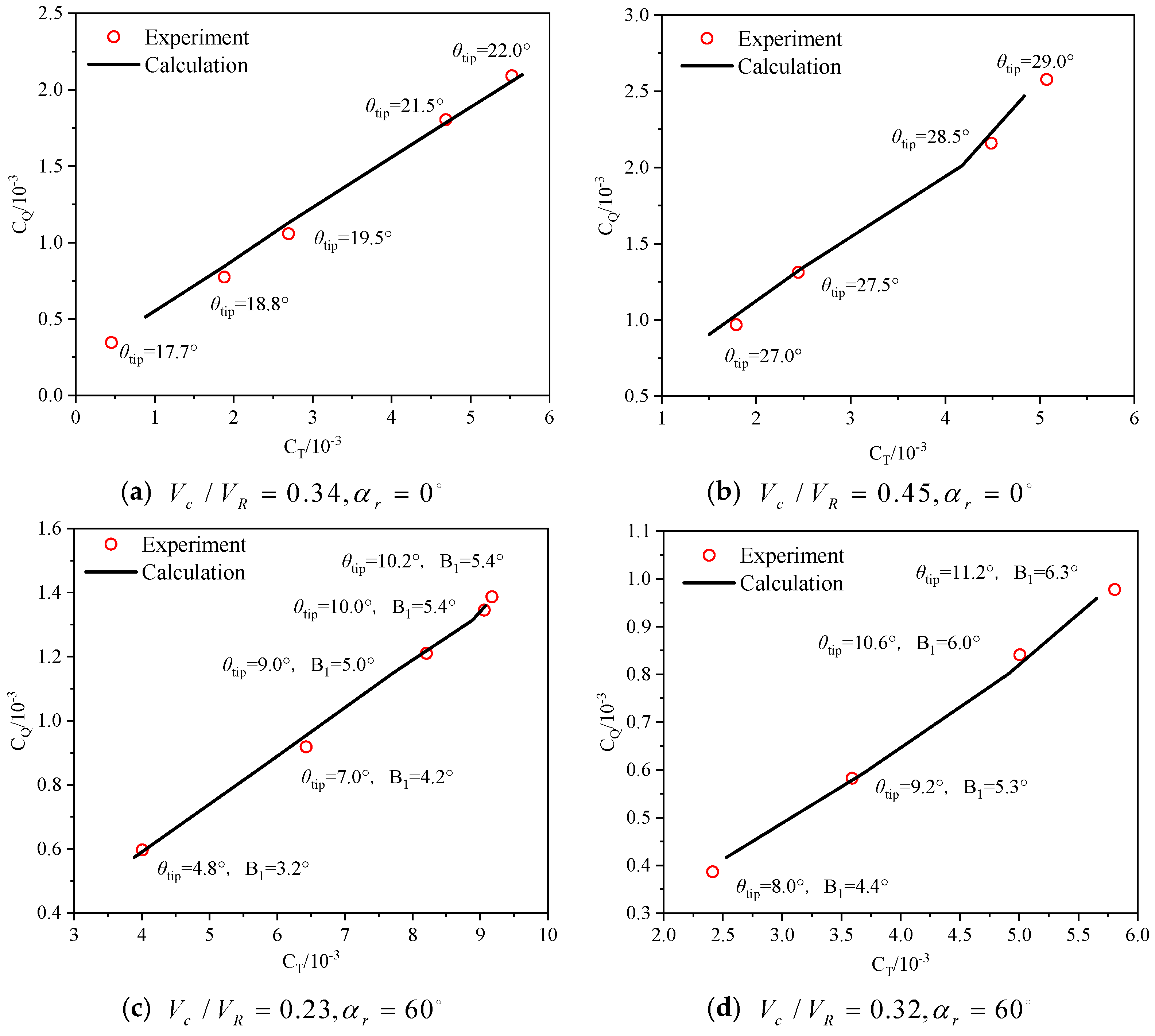
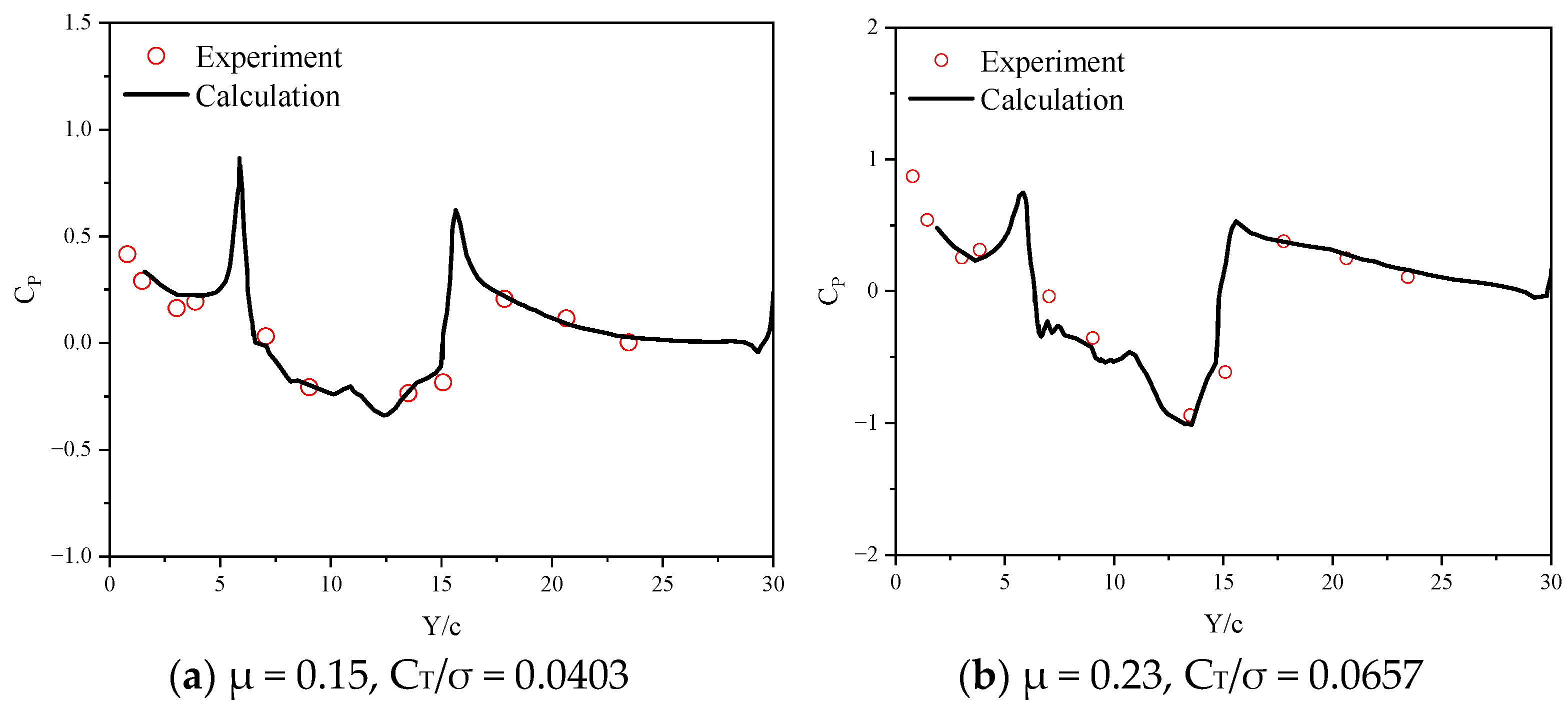
2.4. Verification of the Trimming Method
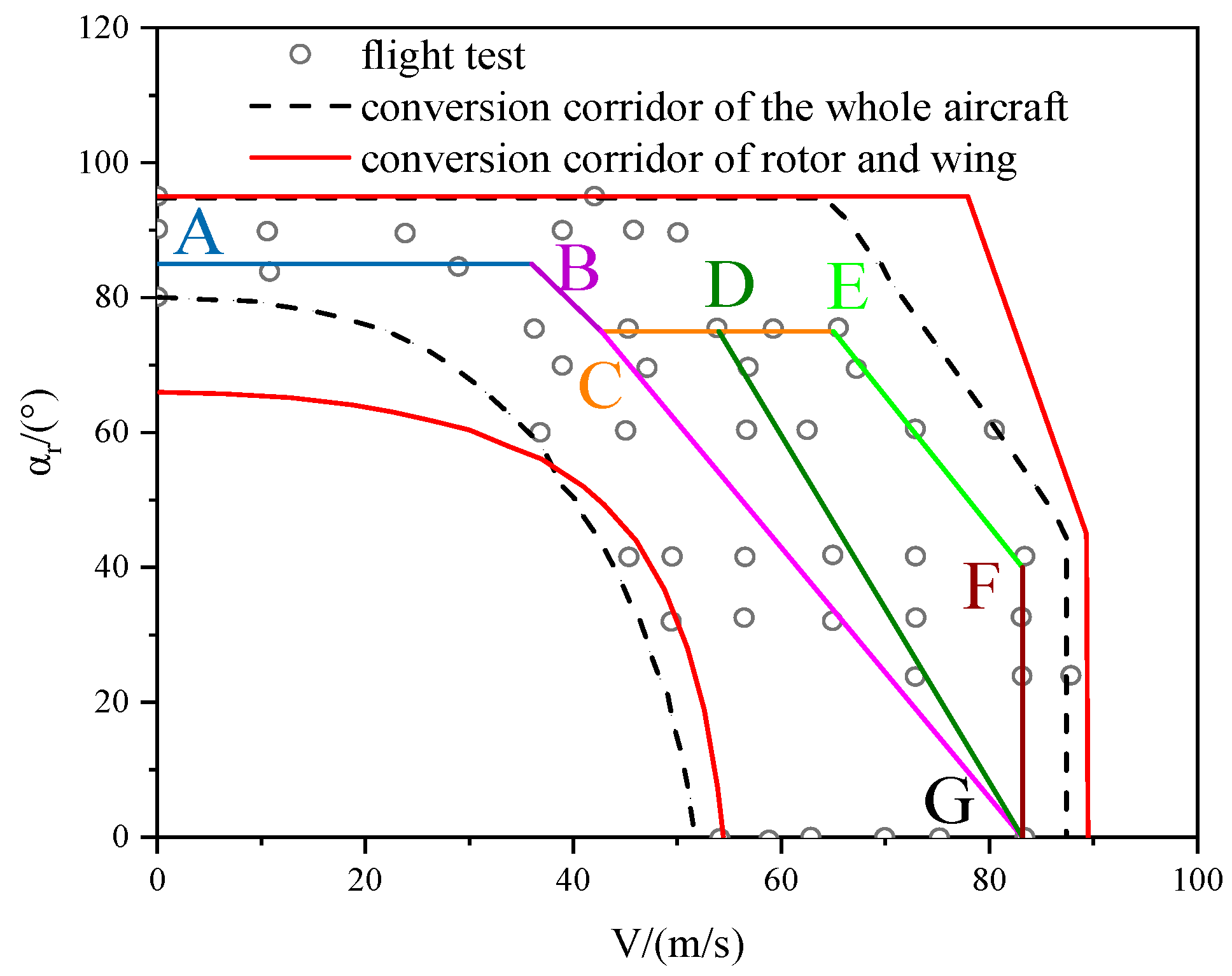
3. Analyses of Conversion Corridor
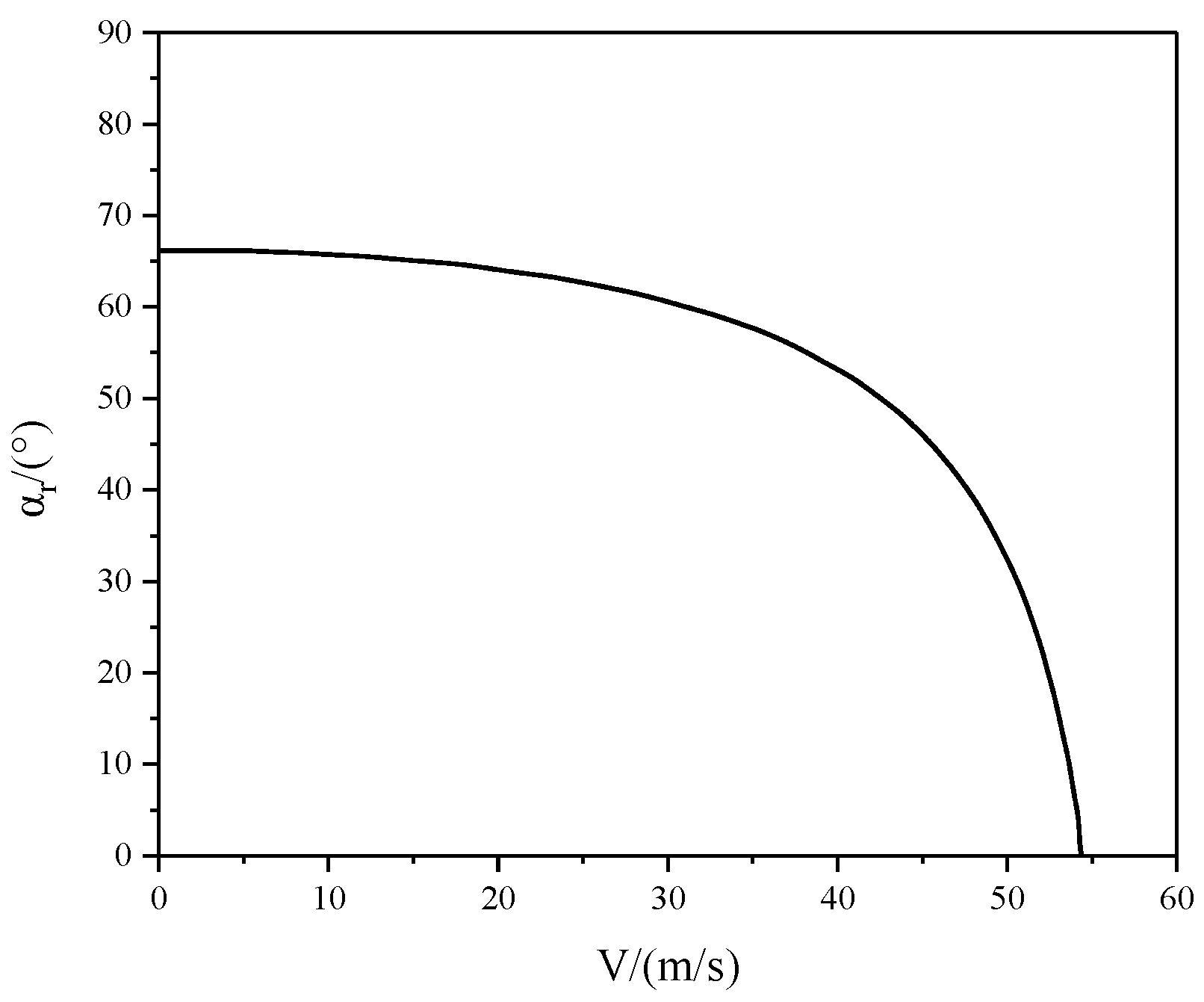
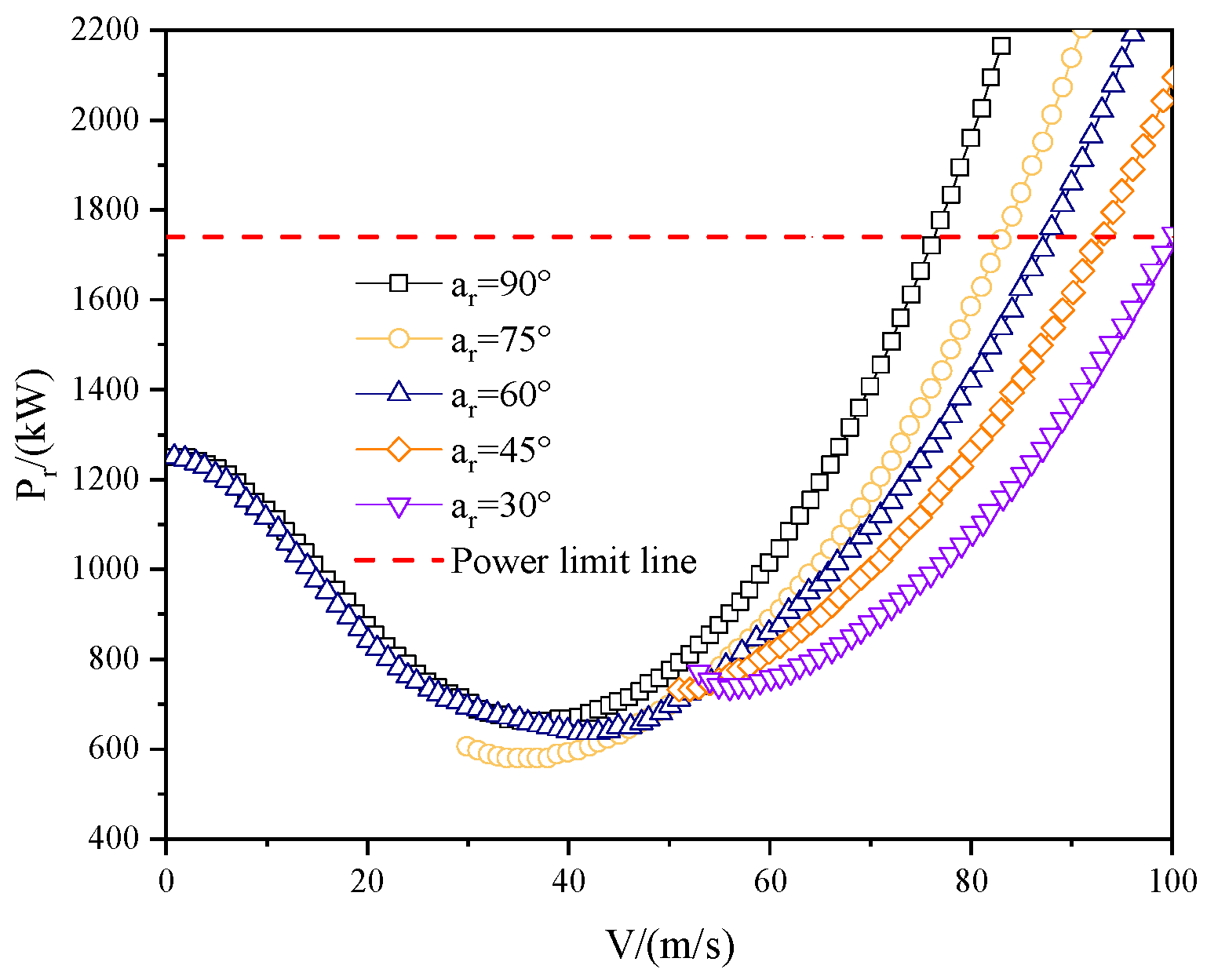
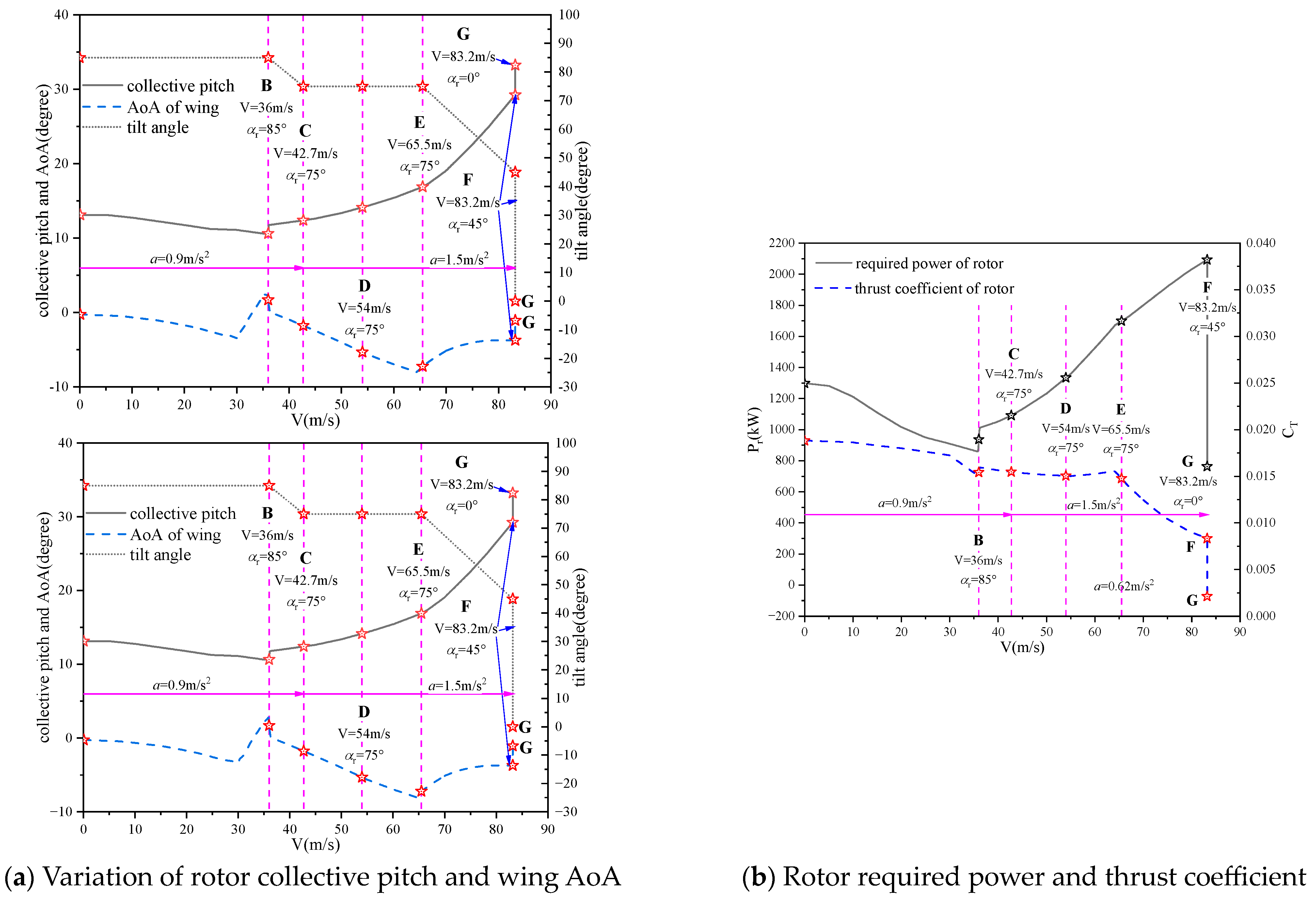
3.1. Tilt-Angle–Speed Envelope on Low-Speed Boundary
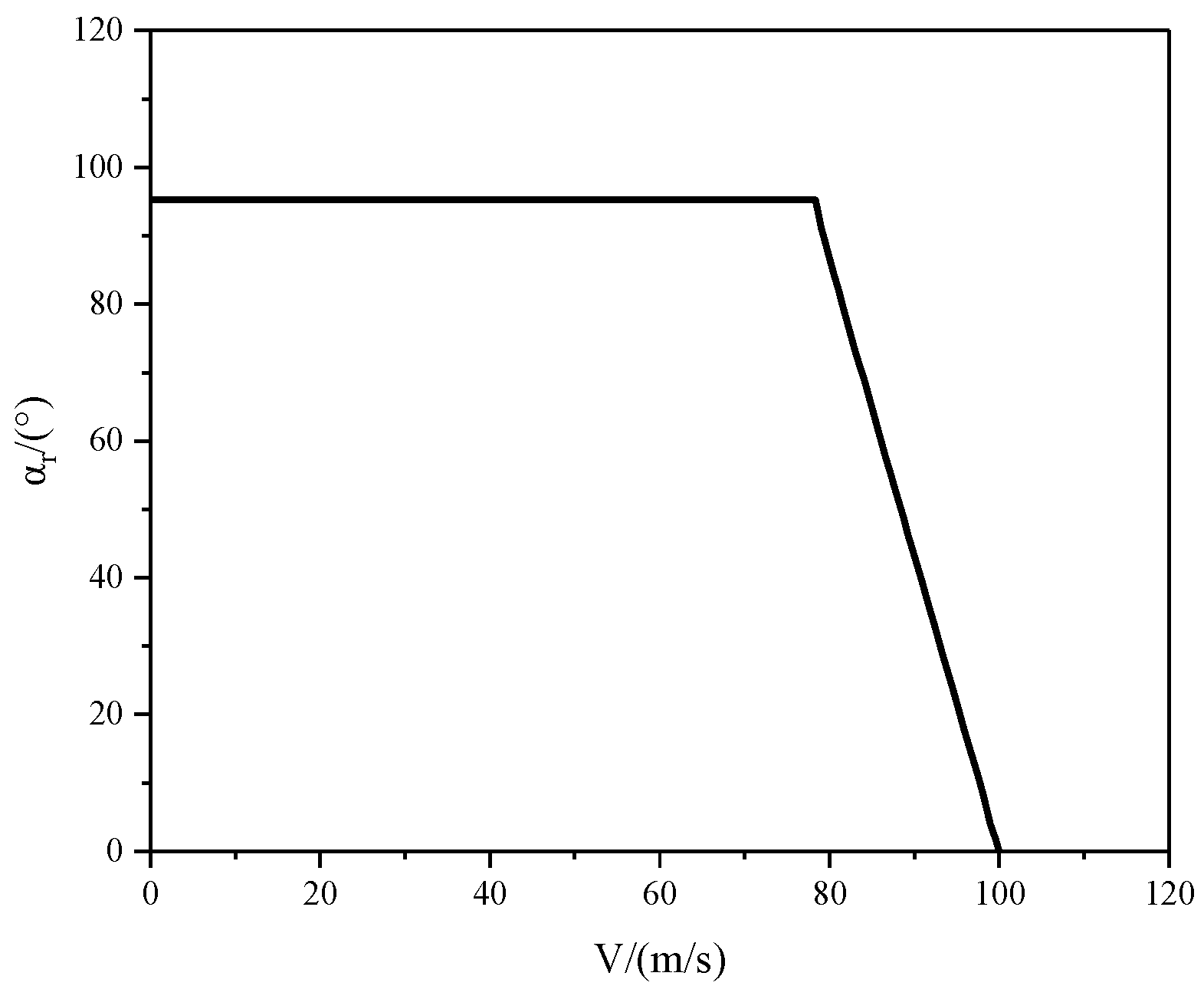
3.2. Tilt-Angle–Speed Envelope on High-Speed Boundary
4. Aerodynamic Characteristics of Tiltrotor in Conversion Procedure
4.1. Aerodynamic Characteristics of Rotor and Wing at Fixed Tilt Angle
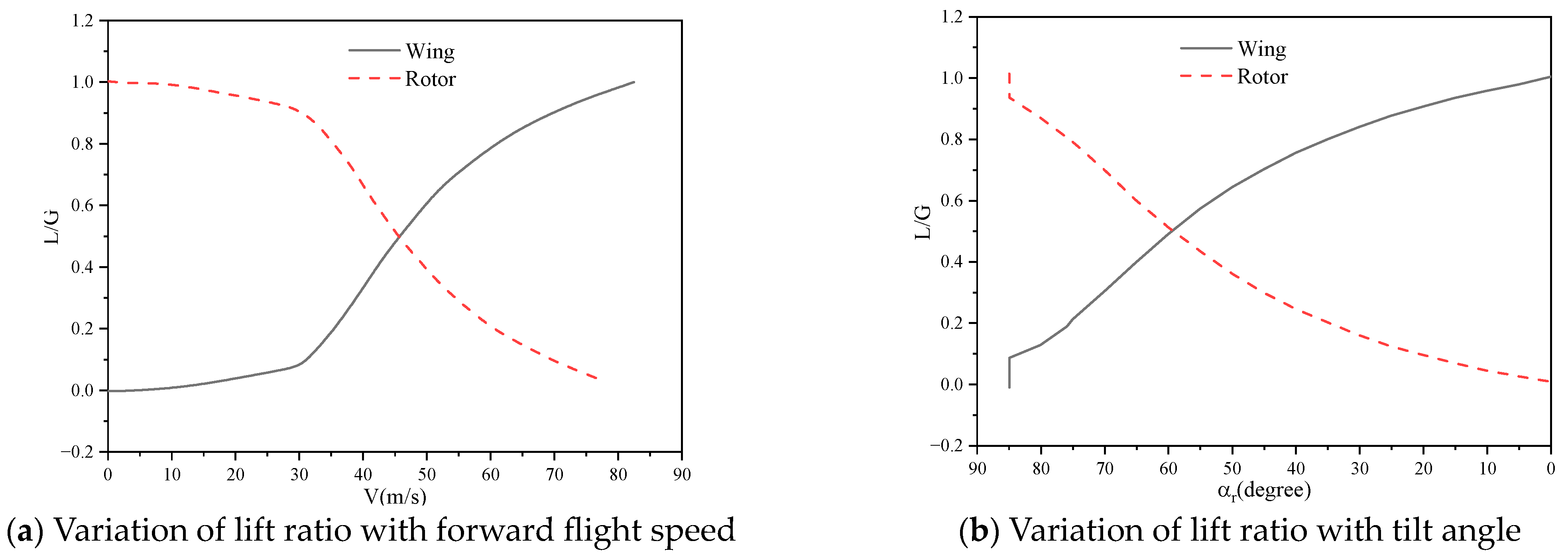
4.1.1. Variation of Aerodynamic Forces of Rotor and Wing
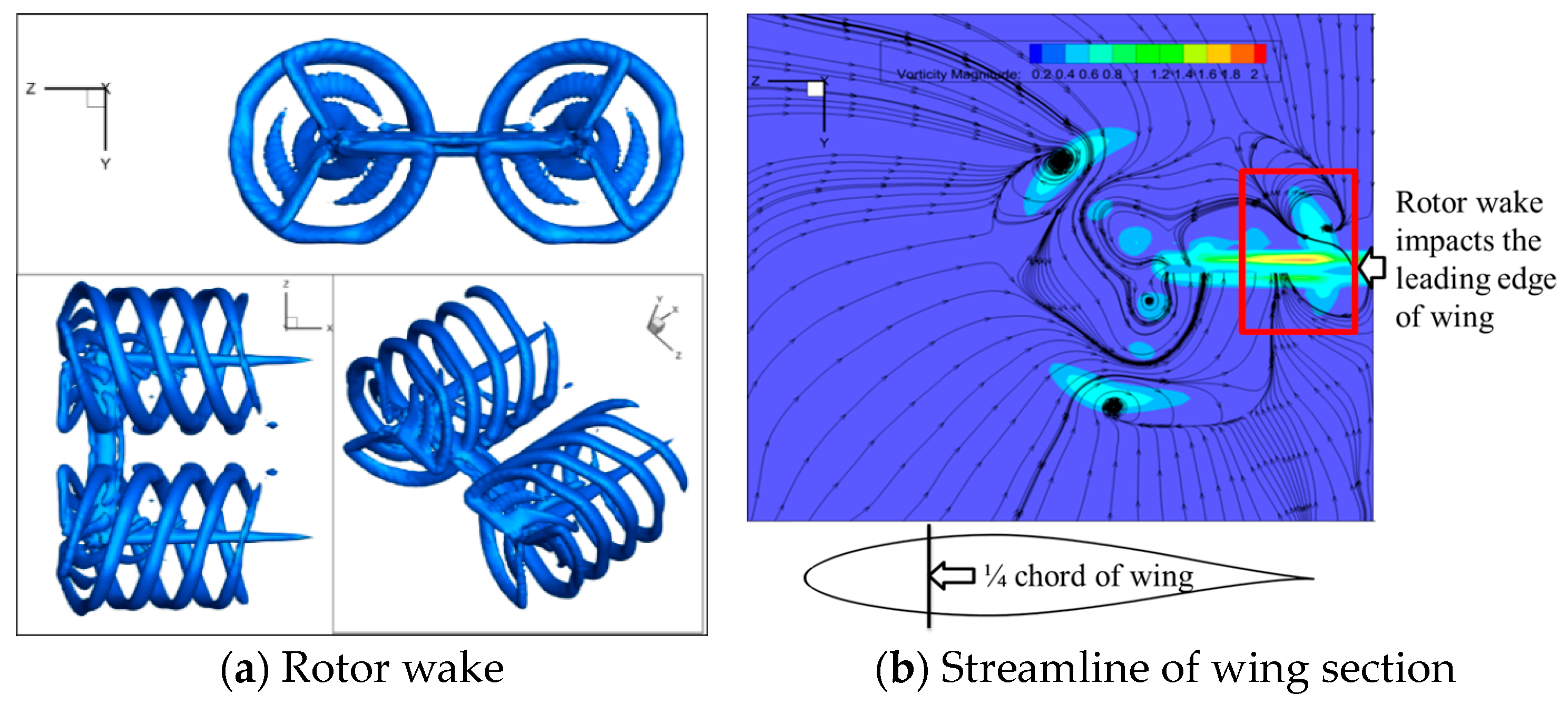
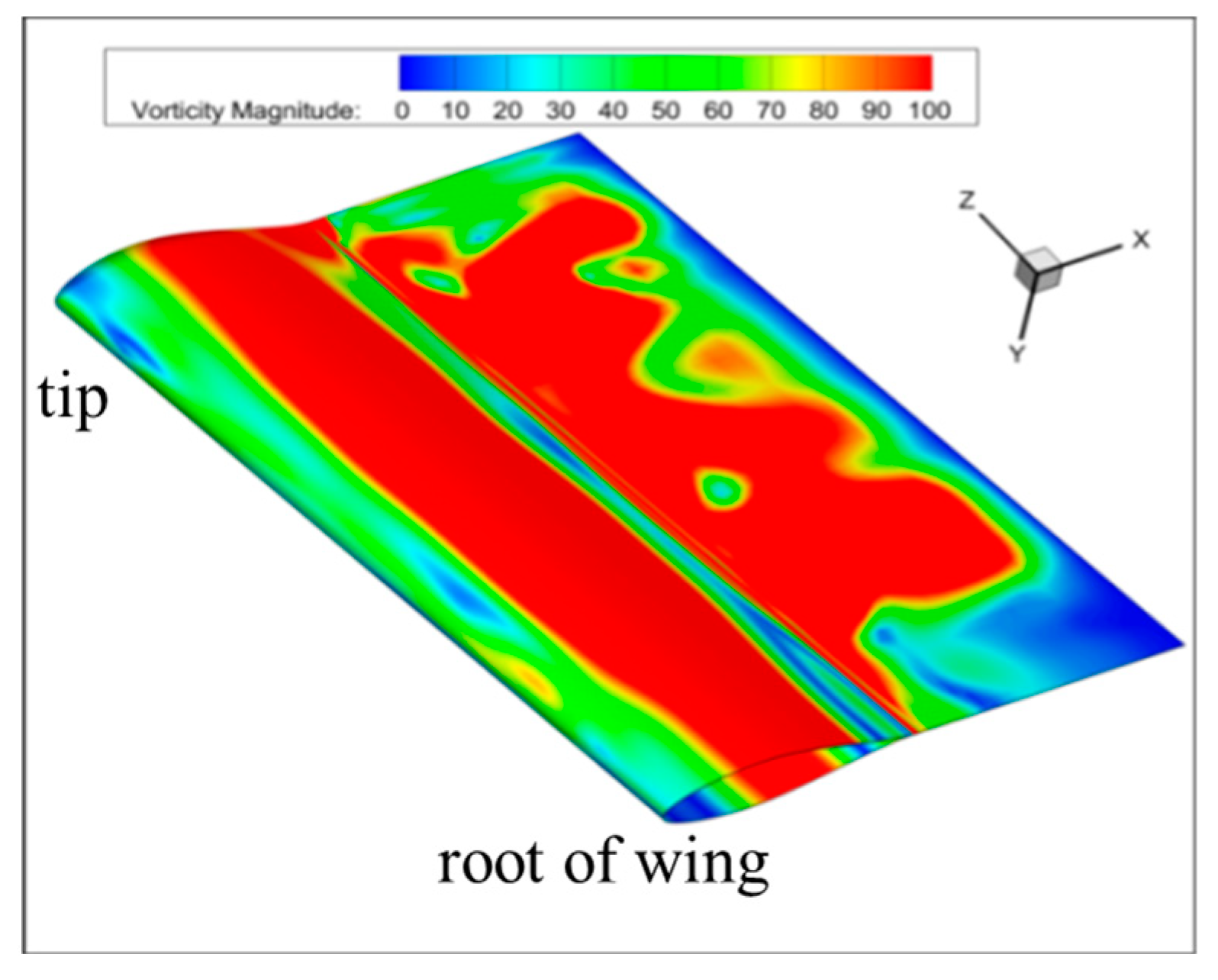
4.1.2. Aerodynamic Interaction of Rotor and Wing
- (1)
- Case 1: αr = 0° (fixed-wing mode)
- (2)
- Case 2: αr = 30° (conversion mode)
4.2. Aerodynamic Characteristics of Rotor/Wing in Continuous Conversion Procedure
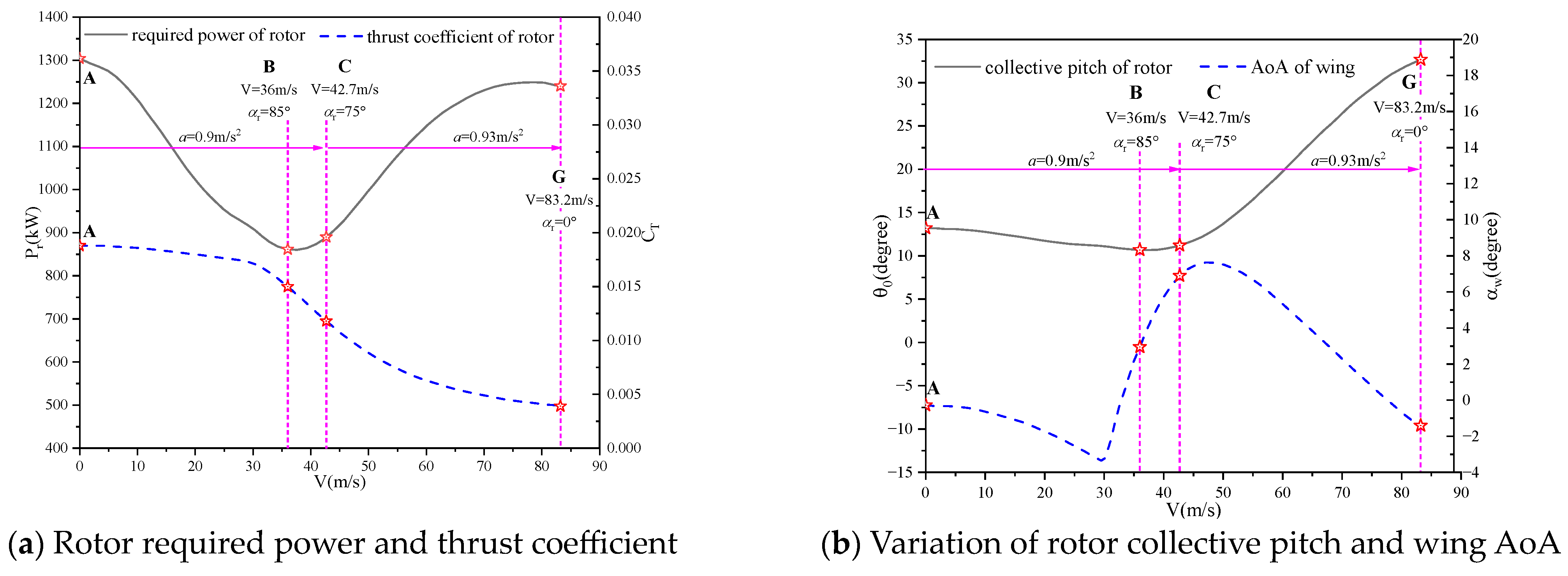
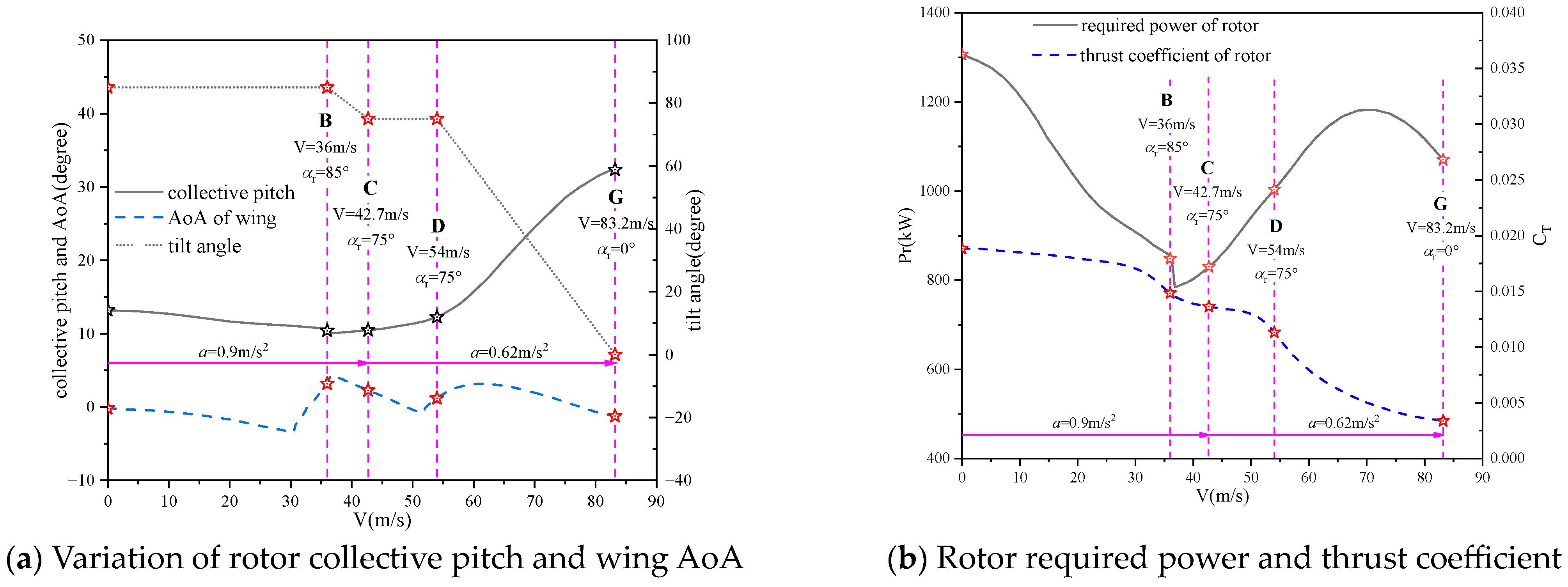
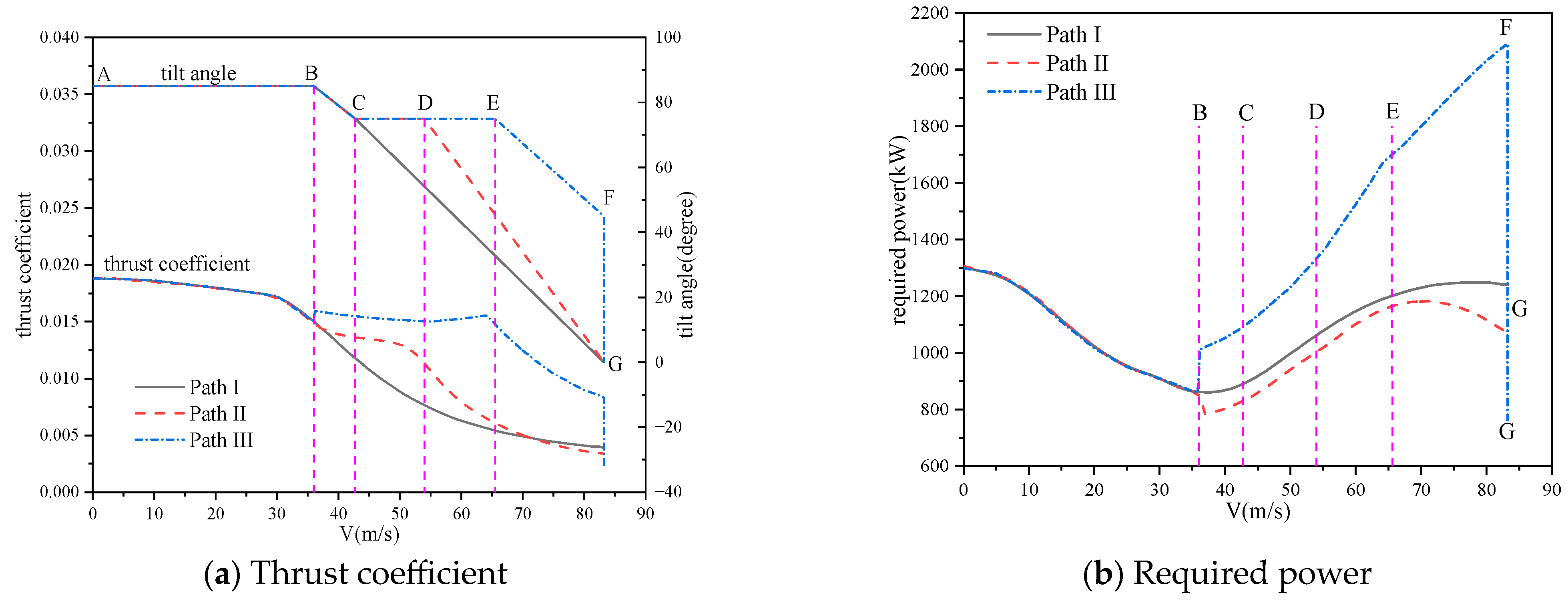
4.2.1. Influences of Paths on Aerodynamic Characteristics of Tiltrotor
4.2.2. Influences of Tilting Angular Velocity
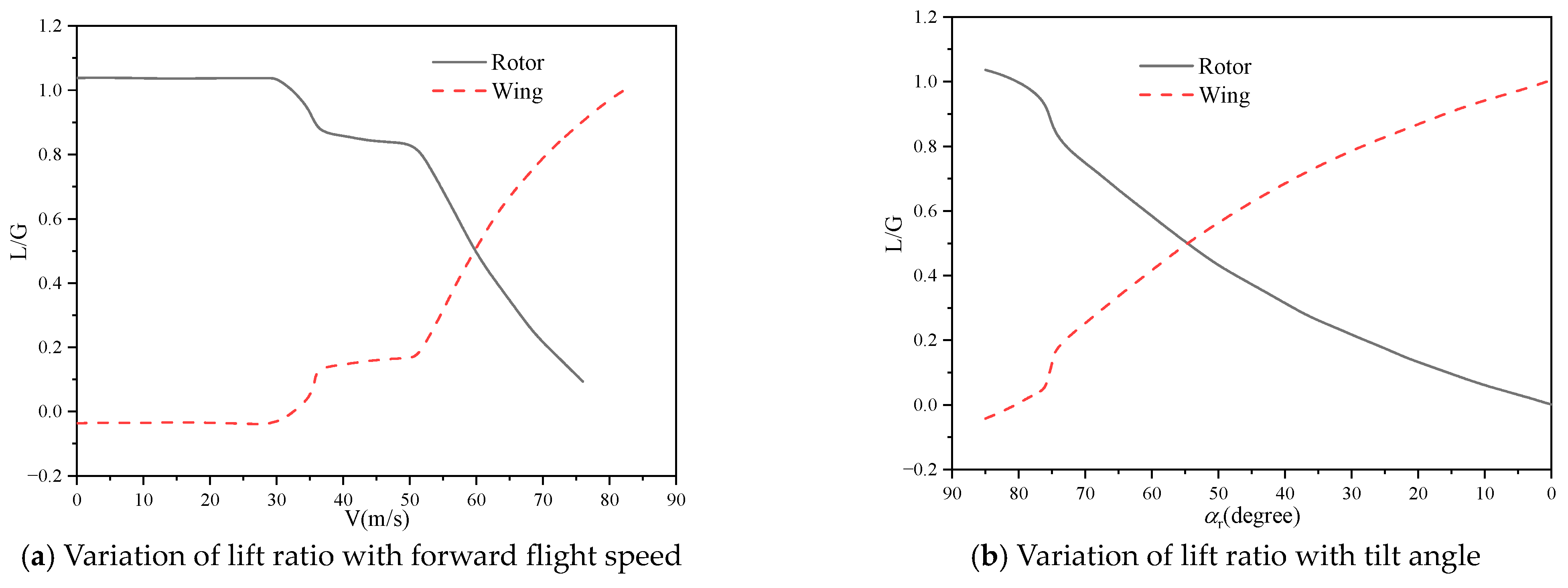
4.2.3. Evolutions of Aerodynamic Forces for Tiltrotor and Wing
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Risen, T. Future vertical lift demonstrators. Aerosp. Am. 2018, 56, 23–25. [Google Scholar]
- Belardo, A.M.; Beretta, B.J.; Marano, A.D. Design strategy of the wing of the Next Generation Civil TiltRotor Technology Demonstrator. In Proceedings of the 3rd Euro-Mediterranean Conference on Structural Dynamics and Vibroacoustics, Napoli, Italy, 17–19 February 2020. [Google Scholar]
- Marano, A.D.; Diodati, G.; Paletta, N. Structural scalability preliminary studies for the next generation civil tiltrotor composite wing. Aerospace 2023, 10, 478. [Google Scholar] [CrossRef]
- Garcia, A.J.; Barakos, G.N. Numerical simulations on the ERICA tiltrotor. Aerosp. Sci. Technol. 2017, 64, 171–191. [Google Scholar] [CrossRef]
- Ma, J.; Lu, Y.; Xu, X.; Yue, H. Research on near field aeroacoustics suppression of tilt-rotor aircraft based on rotor phase control. Appl. Acoust. 2022, 186, 108451. [Google Scholar] [CrossRef]
- Xu, P.; Yang, Y.; Zhou, J.; Zhou, G. Aerodynamic characteristic analysis of V-22 tilt-rotor aircraft in hover. J. Phys. Conf. Ser. 2022, 2280, 012020. [Google Scholar] [CrossRef]
- Chen, H. Numerical calculations on the unsteady aerodynamic force of the tilt-rotor aircraft in conversion mode. Int. J. Aerosp. Eng. 2019, 2019, 2147068. [Google Scholar] [CrossRef]
- Sheng, C.; Narramore, J.C. Computational simulation and analysis of bell boeing quad tiltrotor aero interaction. J. Am. Helicopter Soc. 2009, 54, 42002. [Google Scholar] [CrossRef]
- Jung, Y.S.; You, J.Y.; Kwon, O.J. Numerical investigation of prop-rotor and tail-wing aerodynamic interference for a tilt-rotor UAV configuration. J. Mech. Sci. Technol. 2014, 28, 2609–2617. [Google Scholar] [CrossRef]
- Truong, H.; Marouf, A.; Hoarau, Y. Aerodynamic drag reduction of a tilt rotor aircraft using zero-net-mass-flux devices. arXiv 2023, arXiv:2310.20023v1. [Google Scholar]
- Muscarello, V.; Colombo, F.; Quaranta, G.; Masarati, P. Aeroelastic rotorcraft–pilot couplings in tiltrotor aircraft. J. Guid. Control. Dyn. 2019, 42, 524–537. [Google Scholar] [CrossRef]
- Ferguson, S.W. A Mathematical Model for Real Time Flight Simulation of a Generic Tilt Rotor Aircraft; NASA CR-166536; NASA: Washington, DC, USA, 1988. [Google Scholar]
- Appleton, W.; Filippone, A.; Bojdo, N. Interaction effects on the conversion corridor of tiltrotor aircraft. Aeronaut. J. 2021, 125, 2065–2086. [Google Scholar] [CrossRef]
- Sheng, H.; Zhang, C.; Xiang, Y. Mathematical modeling and stability analysis of tiltrotor aircraft. Drones 2022, 6, 92. [Google Scholar] [CrossRef]
- Wu, D.; Li, H.; Li, S. Flight dynamics study of a small tilt rotor UAV with tail propeller. In Proceedings of the 16th AIAA Aviation Technology, Integration, and Operations Conference, Washington, DC, USA, 13–17 June 2016; p. 3450. [Google Scholar]
- Govdeli, Y.; Muzaffar, S.M.; Raj, R.; Elhadidi, B.; Kayacan, E. Learning control of tandem-wing tilt-rotor UAV with unsteady aerodynamic model. In Proceedings of the 2019 IEEE International Conference on Fuzzy Systems (FUZZ-IEEE), New Orleans, LA, USA, 23–26 June 2019; pp. 1–6. [Google Scholar]
- Govdeli, Y.; Muzaffar, S.M.; Raj, R.; Elhadidi, B.; Kayacan, E. Unsteady aerodynamic modeling and control of pusher and tilt-rotor quadplane configurations. Aerosp. Sci. Technol. 2019, 94, 105421. [Google Scholar] [CrossRef]
- Lyu, Z.; Wang, Z.; Duan, D.; Lin, L.; Li, J.; Yang, Y.; Chen, Y.; Li, Y. Tilting path optimization of tilt quad rotor in conversion process based on ant colony optimization algorithm. IEEE Access 2020, 8, 140777–140791. [Google Scholar] [CrossRef]
- Zhang, H.; Zhao, Q.; Zhao, G.; Wang, B. Preliminary aerodynamic design strategy for prop-rotors based on multifidelity methods. J. Aerosp. Eng. 2023, 36, 04023067. [Google Scholar] [CrossRef]
- Li, P.; Zhao, Q.; Zhu, Q. CFD calculations on the unsteady aerodynamic characteristics of a tilt-rotor in a conversion mode. Chin. J. Aeronaut. 2015, 28, 1593–1605. [Google Scholar] [CrossRef]
- Zhao, Q.; Zhao, G.; Wang, B.; Wang, Q.; Shi, Y.; Xu, G. Robust Navier-Stokes method for predicting unsteady flowfield and aerodynamic characteristics of helicopter rotor. Chin. J. Aeronaut. 2018, 31, 214–224. [Google Scholar] [CrossRef]
- Zhao, G.; Zhao, Q.; Li, P.; Wang, B. Numerical Investigations for Passive and Active Flow Control on Tiltrotor/Wing Aerodynamic Interactions. J. Aerosp. Eng. 2021, 34, 04021094. [Google Scholar] [CrossRef]
- Felker, F.F.; Betzina, M.D.; Signor, D.B. Performance and Loads Data from a Hover Test of a Full-Scale XV-15 Rotor; NASA-TM-86833; NASA: Washington, DC, USA, 1986. [Google Scholar]
- He, J. Variational iteration method-some recent results and new interpretations. J. Comput. Appl. Math. 2007, 207, 3–17. [Google Scholar] [CrossRef]
- Yang, Z.; Sankar, L.N.; Smith, M.J.; Bauchau, O. Recent improvements to a hybrid method for rotors in forward flight. J. Aircr. 2002, 39, 804–812. [Google Scholar] [CrossRef]
- Company, B.H. Advancement of Proprotor Technology: Task 2 Wind Tunnel Test Results; NASA CR-114363; NASA: Washington, DC, USA, 1971. [Google Scholar]
- Mineck, R.E.; Gorton, S.A. Steady and Periodic Pressure Measurements on a Generic Helicopter Fuselage Model in the Presence of a Rotor; TM-2000-210286; NASA: Washington, DC, USA, 2000. [Google Scholar]
- Harendra, P.B.; Joglekar, M.J.; Gaffey, T.M.; Marr, R.L. A mathematical model for real time flight simulation of the Bell model 301 tilt rotor research aircraft. In V/STOL Tilt Rotor Study; NASA CR-114614; NASA: Washington, DC, USA, 1973; Volume 5. [Google Scholar]









| Type and State | θ0 | A1 | B1 | CT/σ |
|---|---|---|---|---|
| Experiment (μ = 0.15) | 10.3 | −2.7 | 2.4 | 0.0403 |
| Calculation (μ = 0.15) | 8.0 | −1.6 | 2.4 | |
| Experiment (μ = 0.23) | 10.4 | −0.4 | 3.8 | 0.0657 |
| Calculation (μ = 0.23) | 9.6 | −0.11 | 3.5 |
| Main Parameters of XV-15 | Value | Main Parameters of XV-15 | Value |
|---|---|---|---|
| Total mass M/(kg) | 5897 | Area of wing Sw/(m2) | 16.82 |
| Engine nacelle angle αr/() | 0–95 | Installation angle of wing iw(°) | 0 |
| Rotor radius R/(m) | 3.81 | Rated power of engine Pn/(kw) | 1737.5 |
| Rotor revolution Ω/(r/min) | 589 | Transmission loss coefficient ηp | 0.95 |
| Average chord length of wing c/(m) | 1.6 |
| Tile Angle of Rotor | Proportion of Rotor Lift | Proportion of Wing Lift |
|---|---|---|
| (Hover) | 100.0% | 0.0% |
| 41.2% | 58.8% | |
| 10.5% | 89.5% | |
| (Cruise) | 0.0% | 100% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, G.; Cui, Z.; Zhao, Q.; Chen, X.; Li, P. Investigations on Trimming Strategy and Unsteady Aerodynamic Characteristics of Tiltrotor in Conversion Procedure. Aerospace 2024, 11, 632. https://doi.org/10.3390/aerospace11080632
Zhao G, Cui Z, Zhao Q, Chen X, Li P. Investigations on Trimming Strategy and Unsteady Aerodynamic Characteristics of Tiltrotor in Conversion Procedure. Aerospace. 2024; 11(8):632. https://doi.org/10.3390/aerospace11080632
Chicago/Turabian StyleZhao, Guoqing, Zhuangzhuang Cui, Qijun Zhao, Xi Chen, and Peng Li. 2024. "Investigations on Trimming Strategy and Unsteady Aerodynamic Characteristics of Tiltrotor in Conversion Procedure" Aerospace 11, no. 8: 632. https://doi.org/10.3390/aerospace11080632
APA StyleZhao, G., Cui, Z., Zhao, Q., Chen, X., & Li, P. (2024). Investigations on Trimming Strategy and Unsteady Aerodynamic Characteristics of Tiltrotor in Conversion Procedure. Aerospace, 11(8), 632. https://doi.org/10.3390/aerospace11080632






