A Frequency Domain PID Control Strategy for an In-House Friction and Wear Test Rig
Abstract
1. Introduction
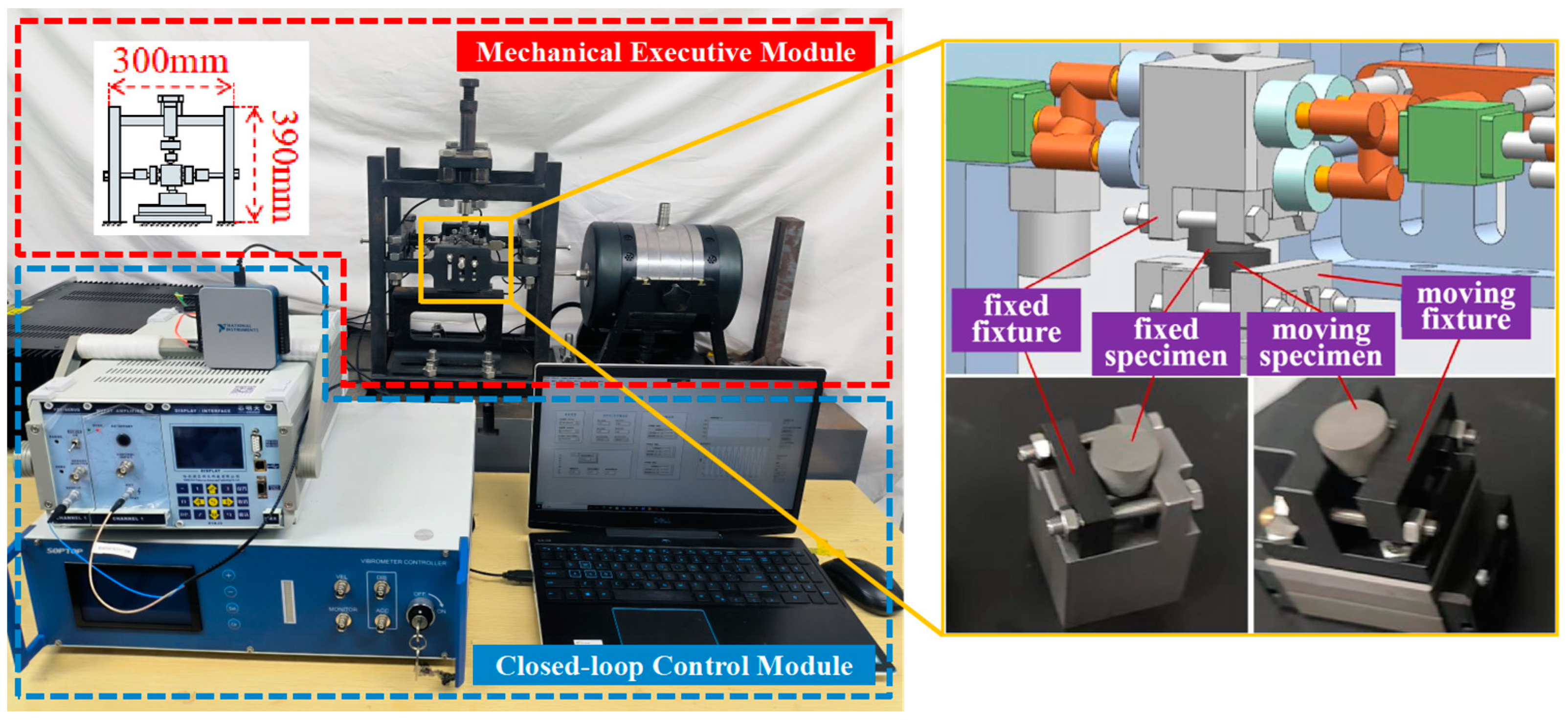
2. Improvement of the In-House Friction and Wear Test Rig
2.1. Mechanical Operations Module
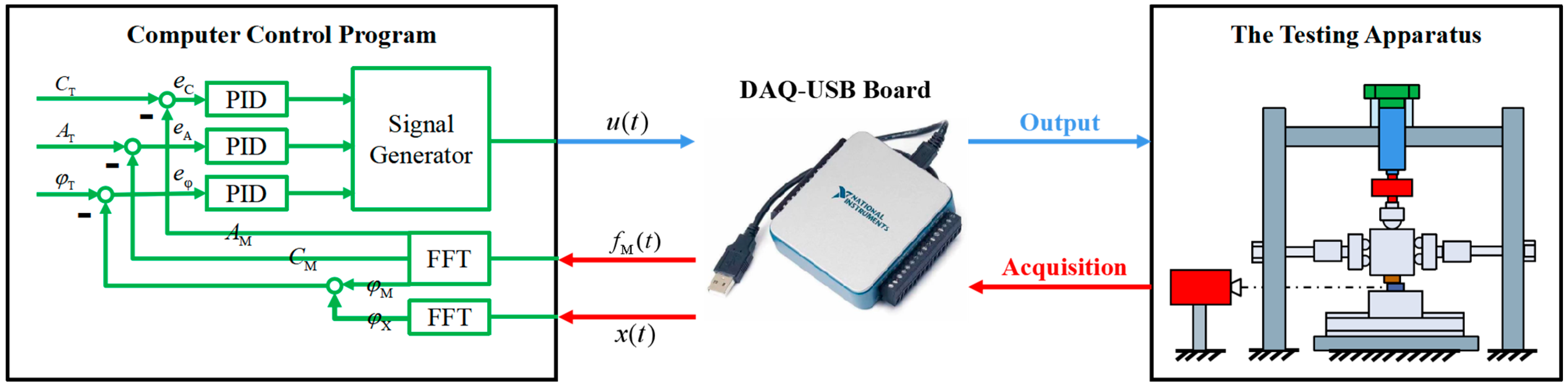
2.2. Closed-Loop Control Module
3. Control Performance of the Test Rig
3.1. Parameter Configuration of the PID Controller
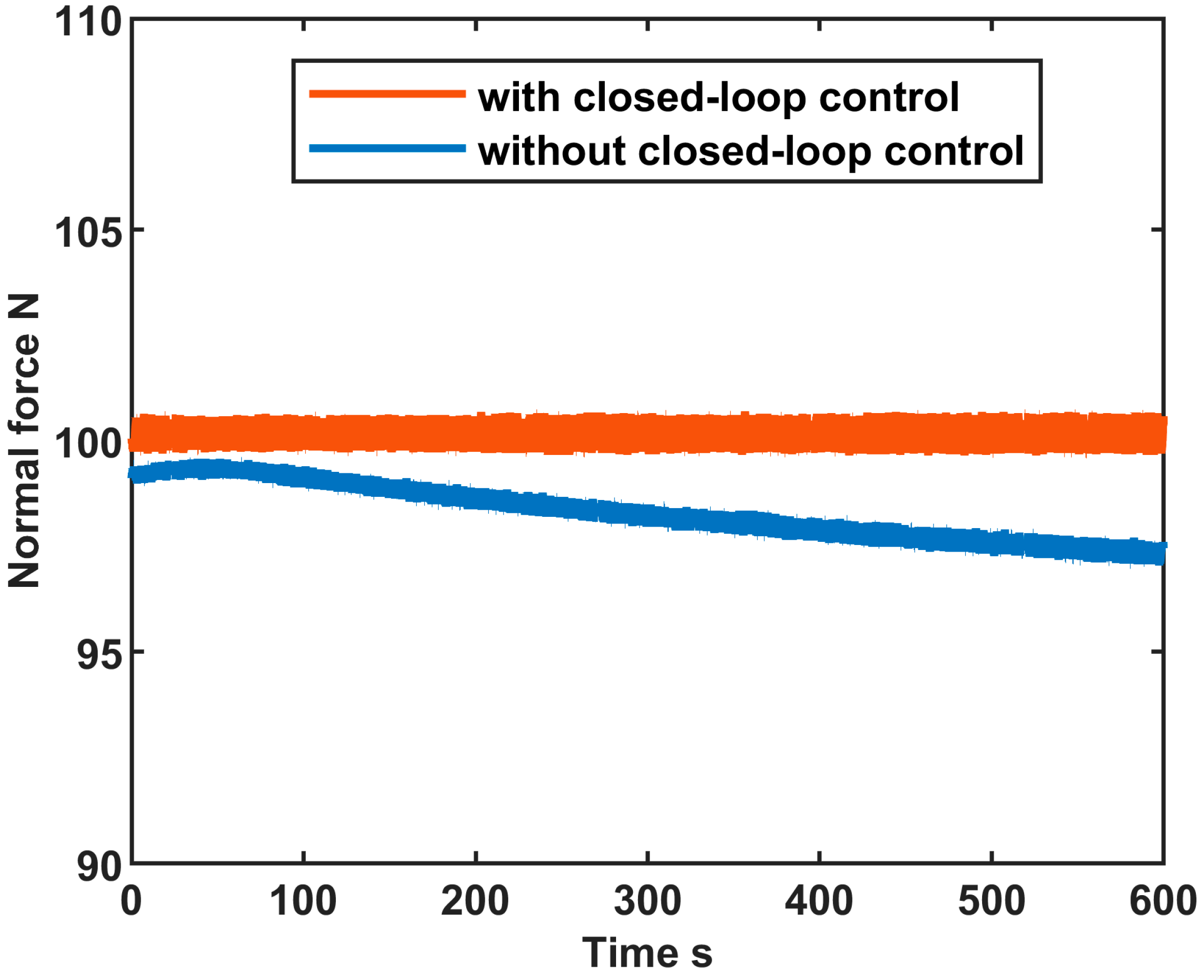
3.2. Performance of the Closed-Loop Control Module
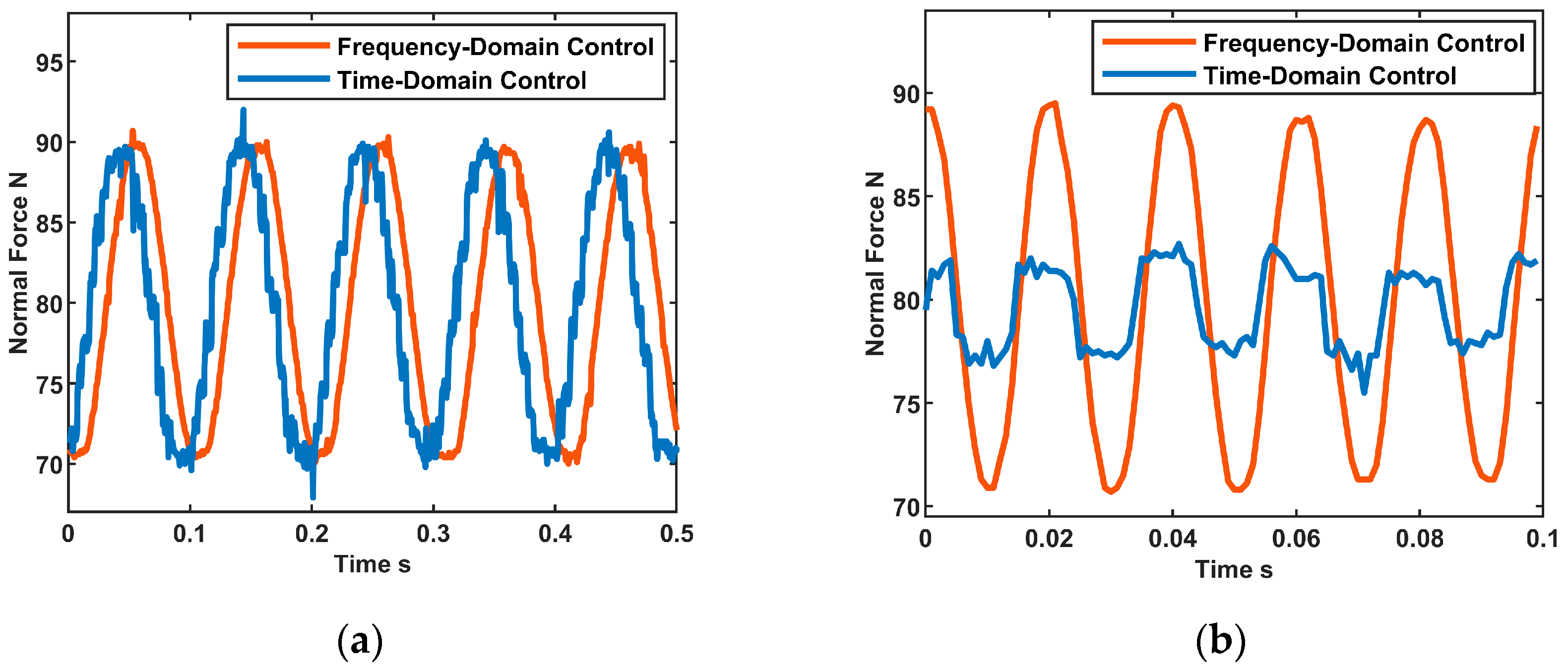
3.3. Performance Comparison of Frequency-Domain and Time-Domain PID Control Strategies
4. Friction Tests Results
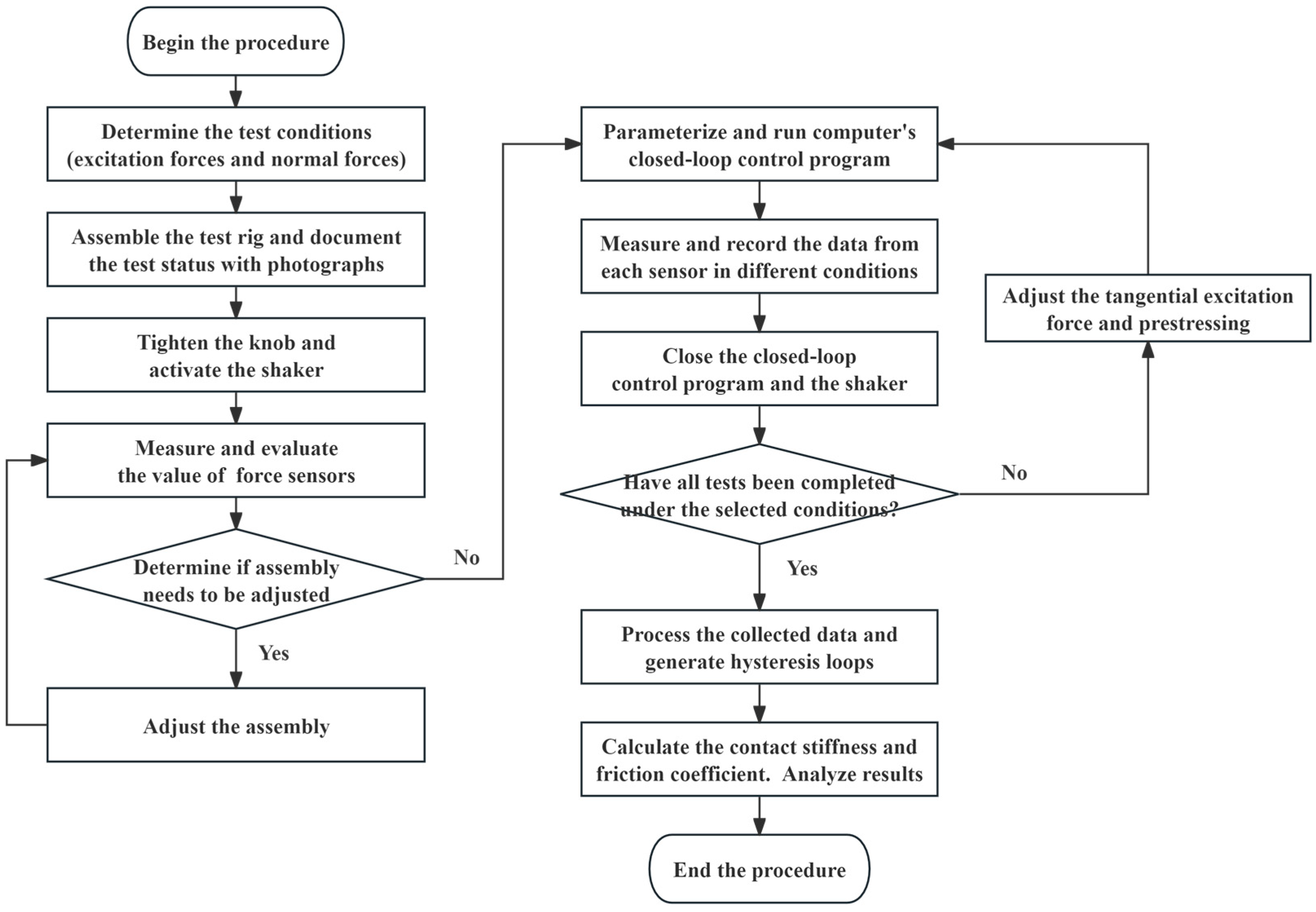
4.1. Experimental Preparation
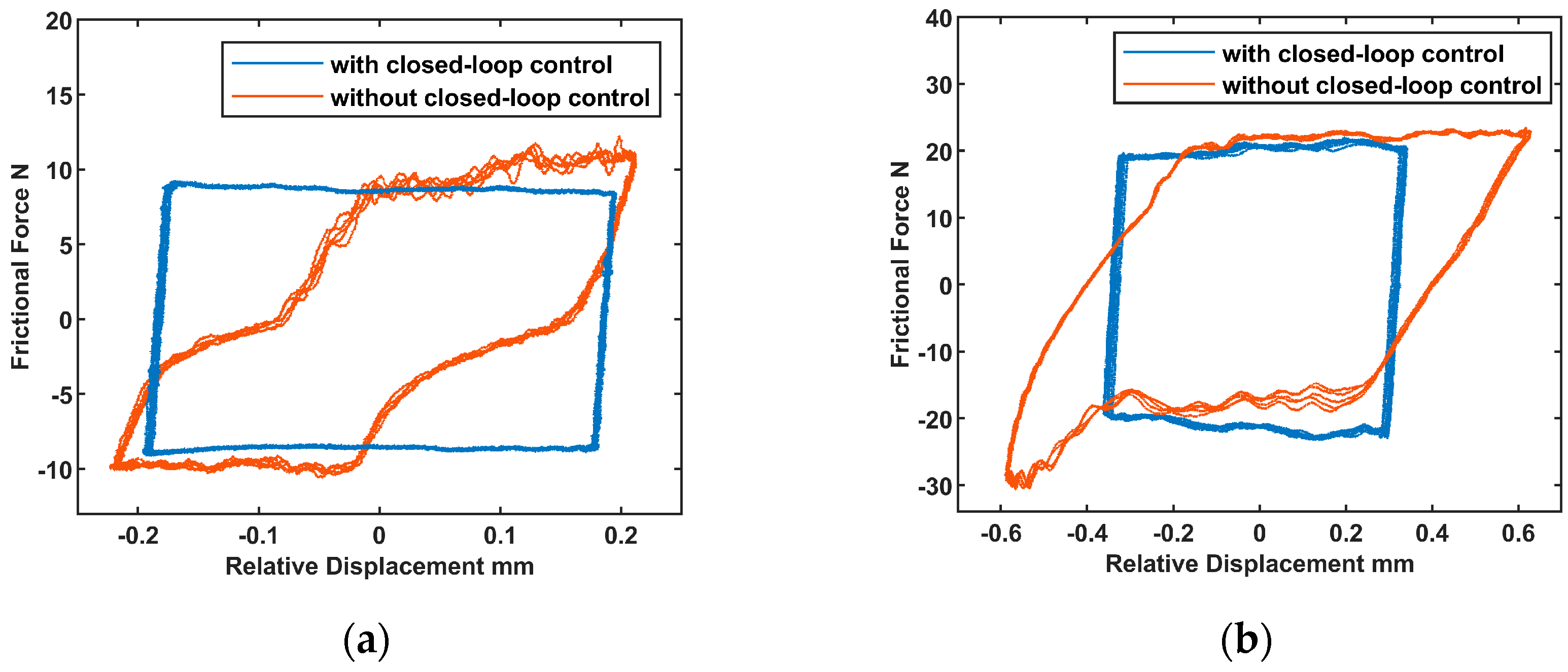
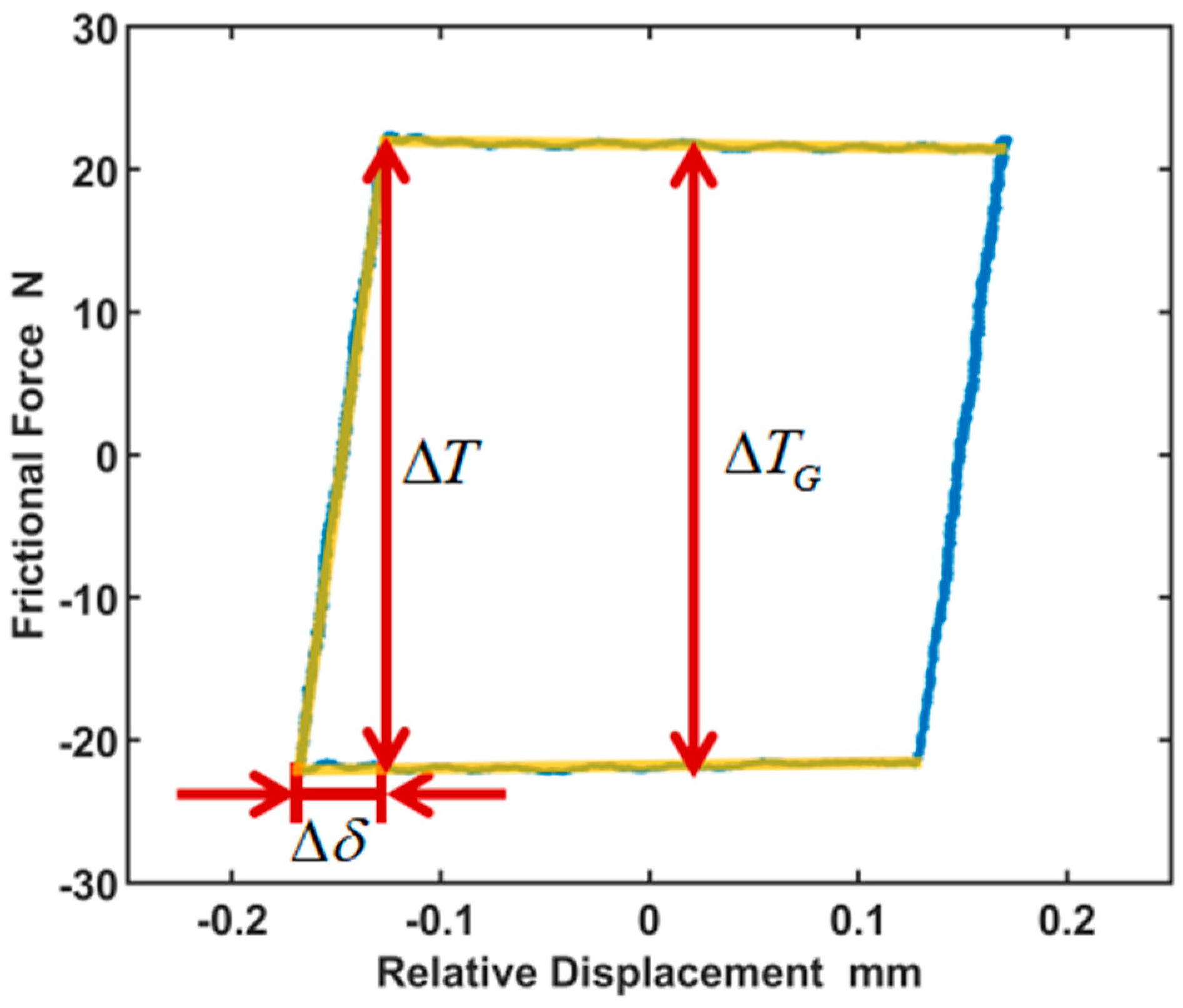
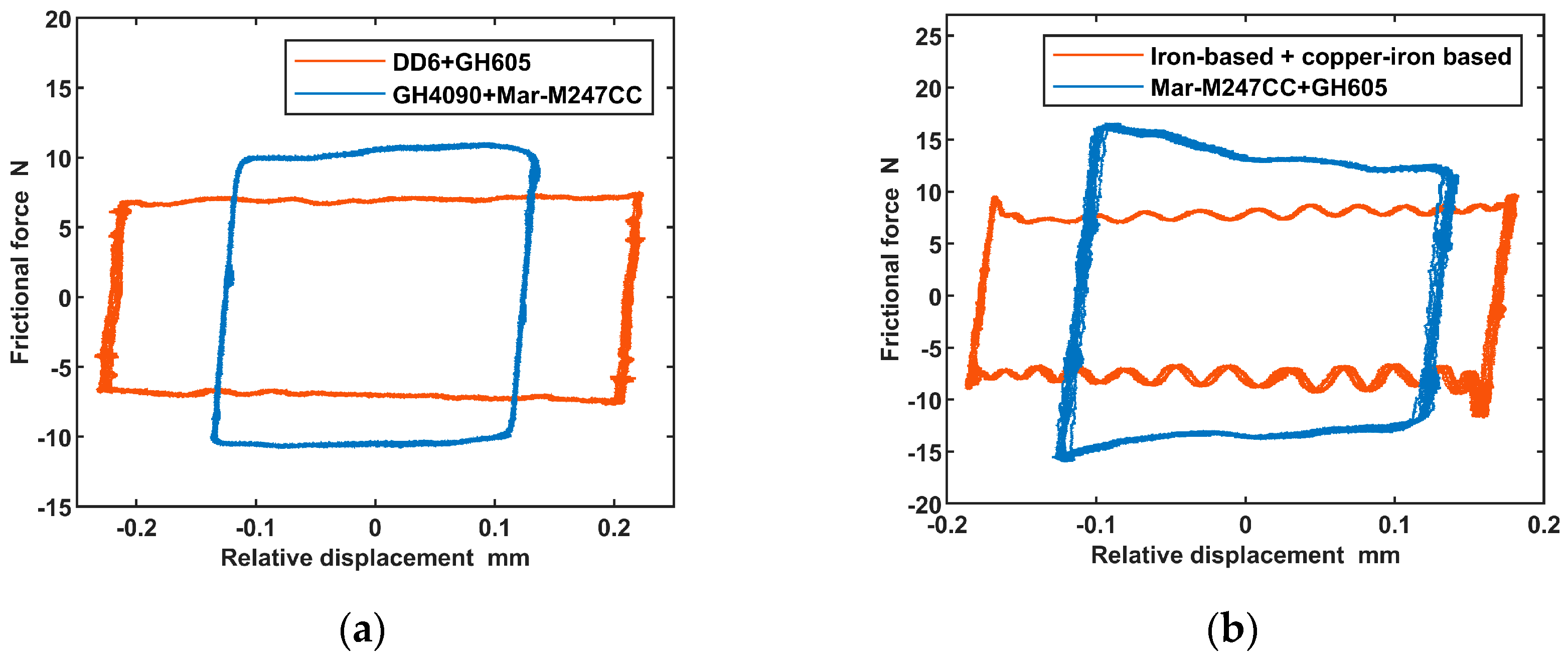
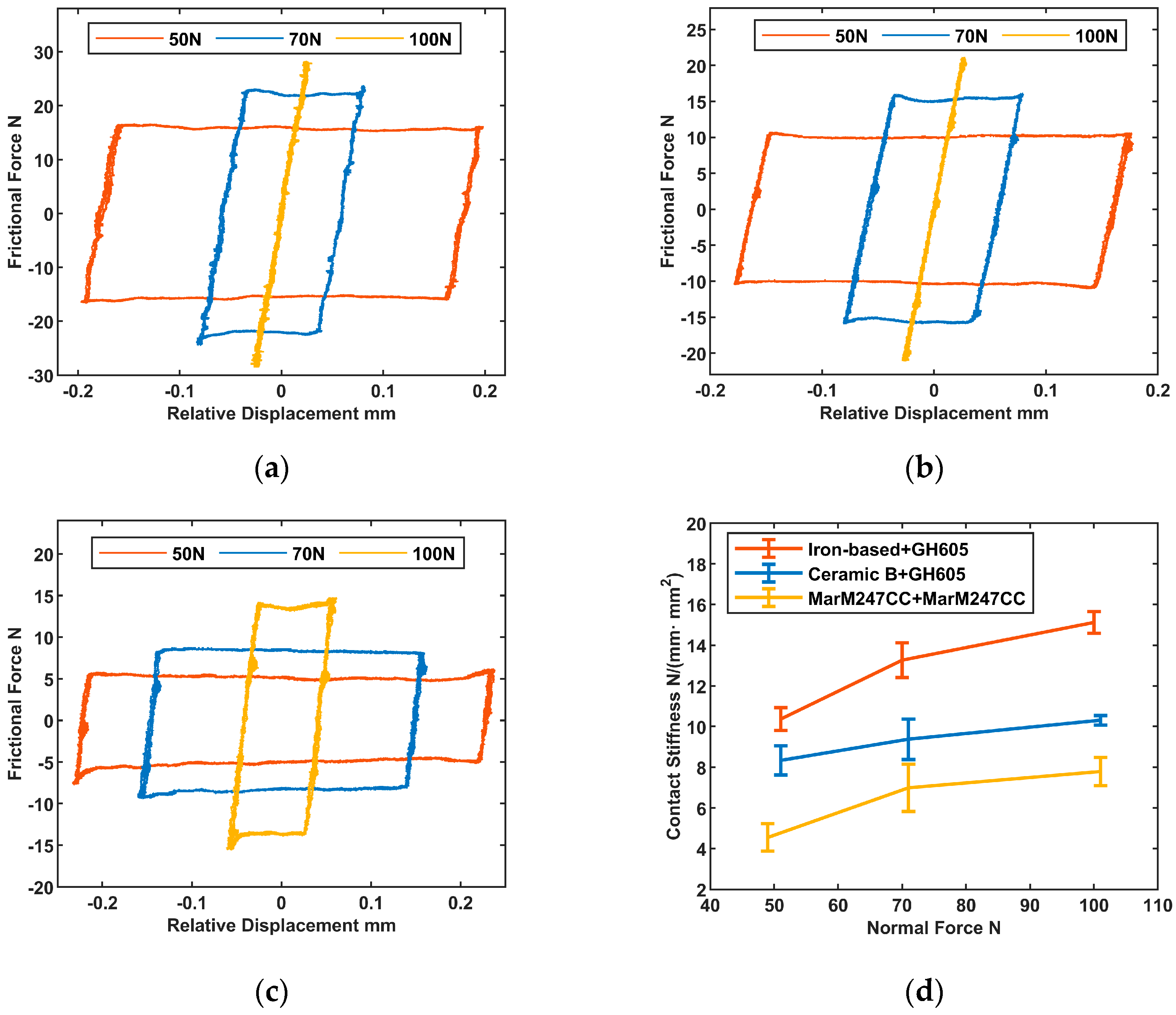
4.2. Hysteresis Loops of Different Friction Materials
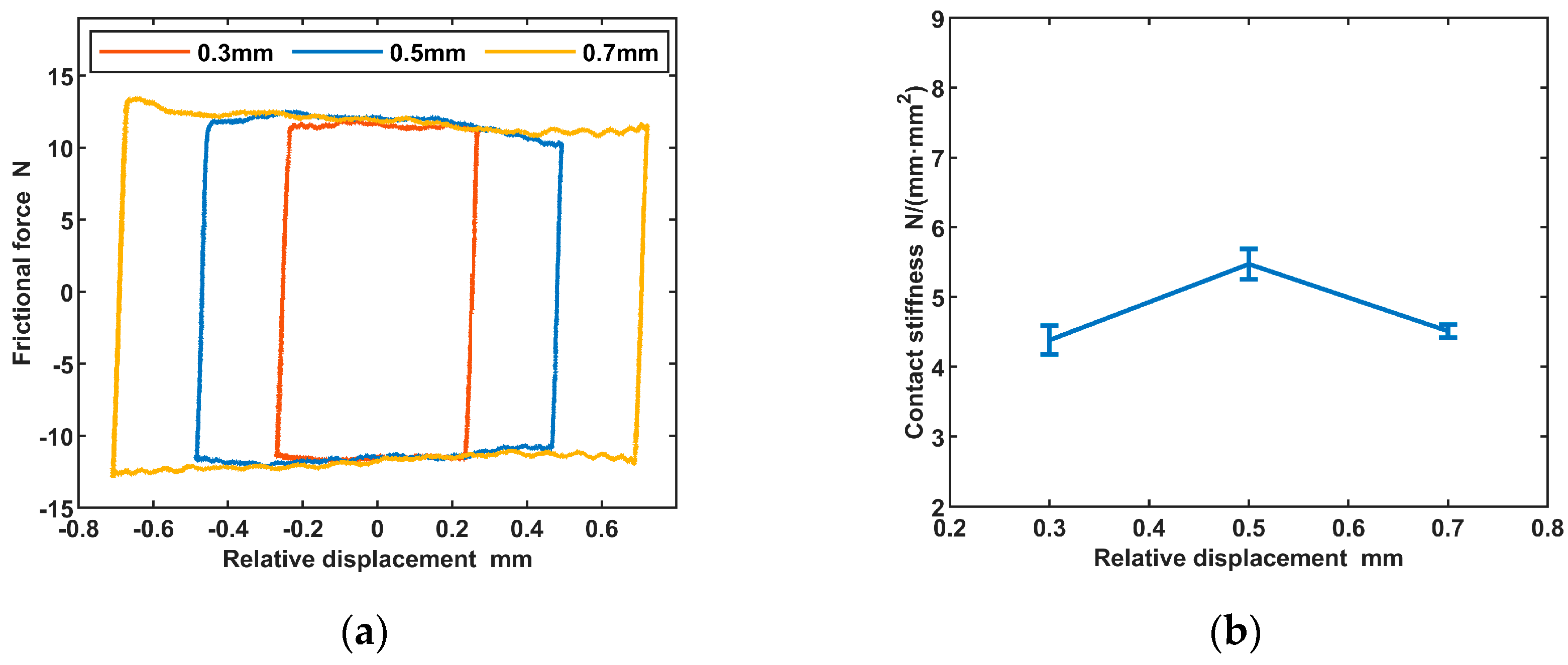
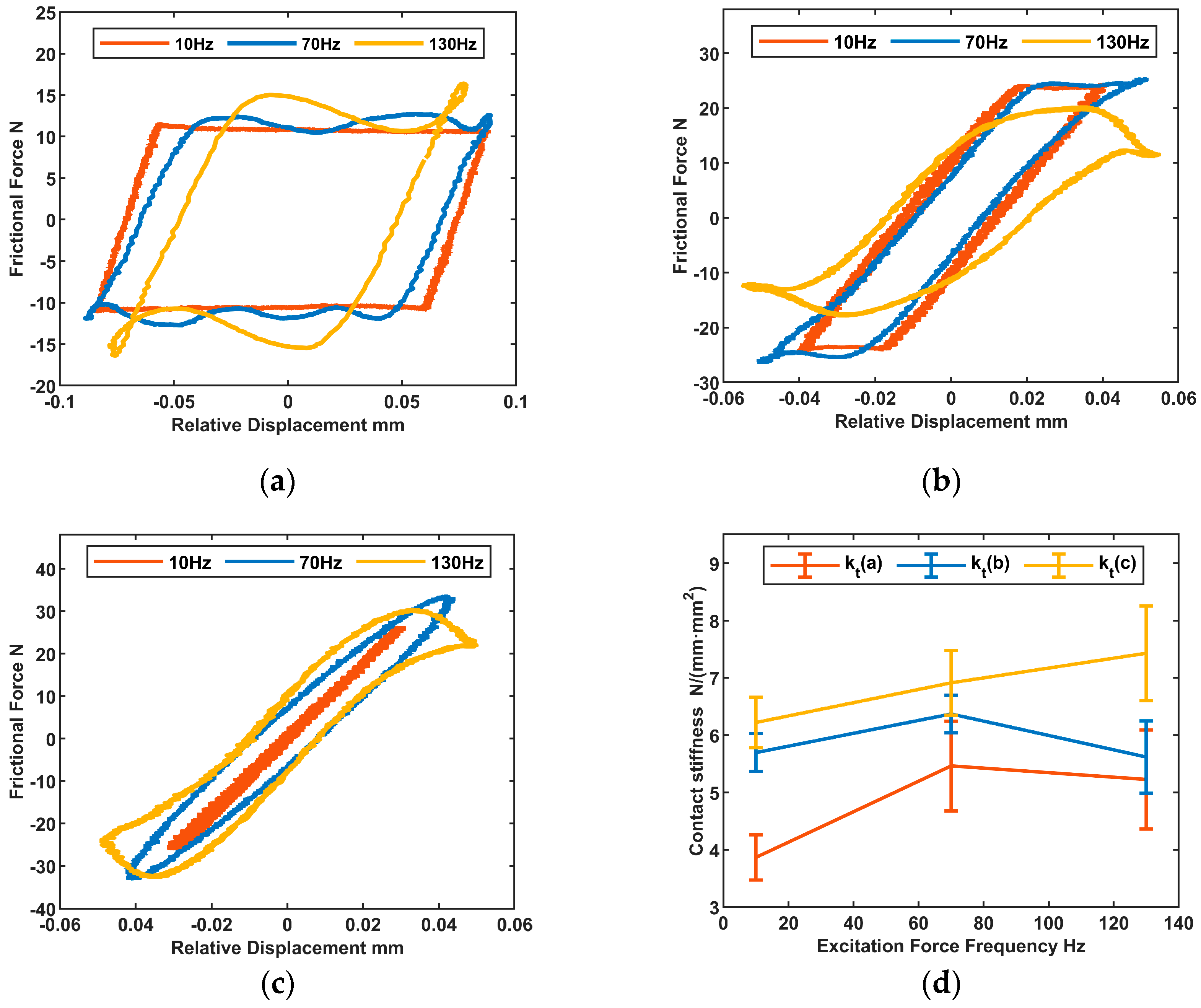
4.3. Effect of Tangential Excitation Level on Contact Parameters
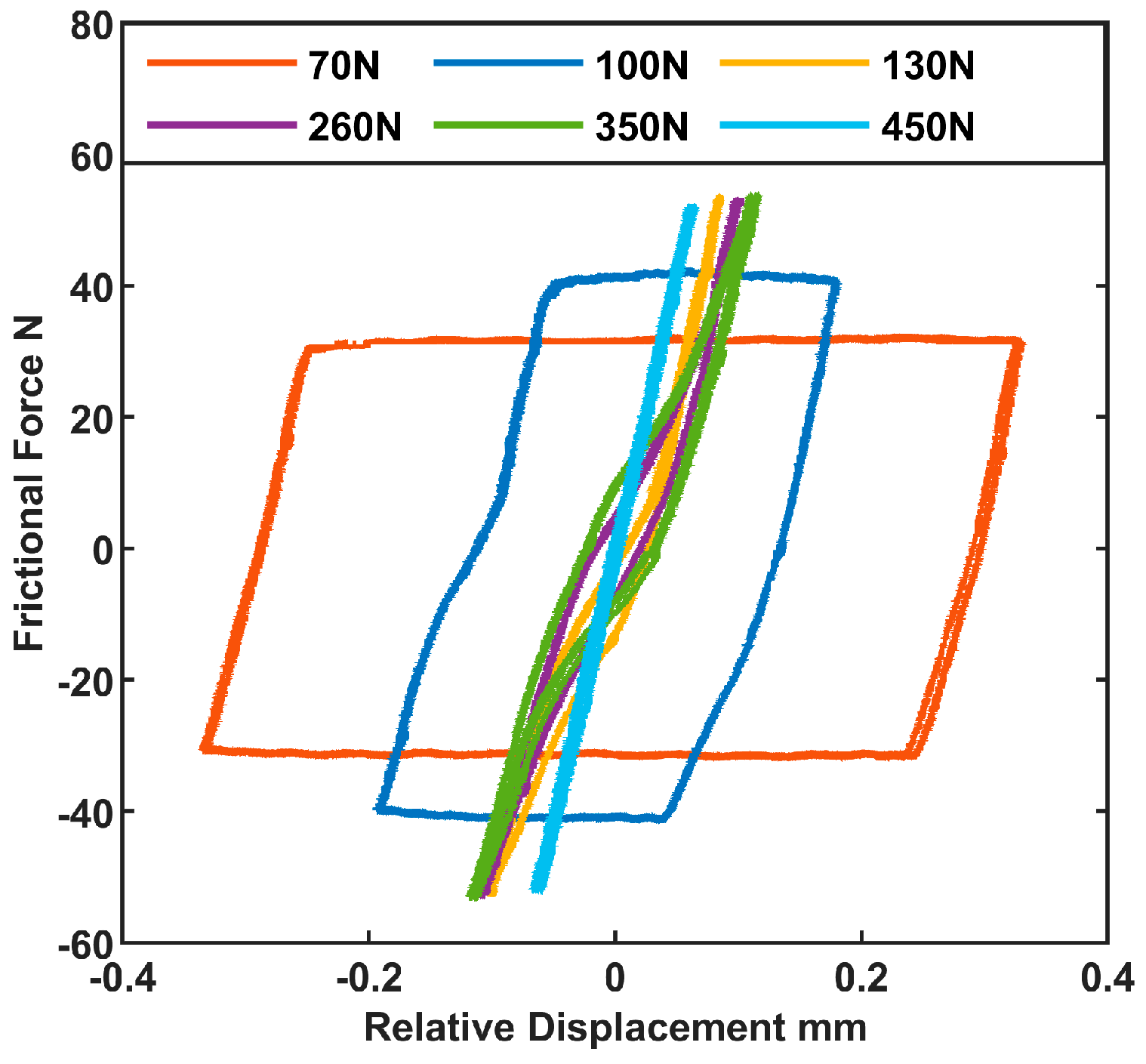
4.4. Effect of Normal Force on Contact Parameters
5. Conclusions
- (1)
- The test rig can output normal force with stable constant (0–300 N) and harmonic (0–50 N) components with given amplitude and phase lag in the friction tests. The frequency-domain PID controller avoids the frequent control of the traditional PID controller in the time-domain, which tracks the reference signal step-by-step. The control error is significantly reduced (from 0.97% to 0.44% at 10 Hz and from 6.30% to 0.54% at 50 Hz). With a standard error threshold of 3%, the controller’s operating frequency is increased from 20 Hz to 300 Hz.
- (2)
- Through conducting friction tests on typical materials, it was found that, in general, contact stiffness tends to rise with an increase in normal force. However, the relationship between the friction coefficient and the normal force does not demonstrate a clear pattern.
- (3)
- Variations in tangential excitation amplitude and frequency significantly impact the shape of the hysteresis loop while having minimal influence on the contact parameters. Consequently, contact parameters obtained under low-frequency, low-amplitude tangential excitation can be extrapolated to high-frequency, high-amplitude conditions. The friction and wear test rig demonstrates high reliability under low tangential excitation, which holds considerable implications for engineering applications.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gao, Q.; Fan, Y.; Wu, Y.; Li, L. A harmonic balance-based method to predict nonlinear forced response and temperature rise of dry friction systems including frictional heat transfer. Nonlinear Dyn. 2023, 111, 14263–14291. [Google Scholar] [CrossRef]
- Gola, M.M. A general geometrical theory of turbine blade underplatform asymmetric dampers. Mech. Syst. Signal Process. 2023, 191, 1–23. [Google Scholar]
- Fantetti, A.; Setchfield, R.; Schwingshackl, C. Nonlinear dynamics of turbine bladed disk with friction dampers: Experiment and simulation. Int. J. Mech. Sci. 2023, 257, 1–20. [Google Scholar] [CrossRef]
- Li, D.W.; Botto, D.; Xu, C.; Gola, M. Fretting wear of bolted joint interfaces. Wear 2020, 458–459, 1–11. [Google Scholar] [CrossRef]
- Fantetti, A.; Tamatam, L.R.; Volvert, M.; Lawal, I.; Liu, L.; Salles, L.; Brake, M.R.W.; Schwingshackl, C.W.; Nowell, D. The impact of fretting wear on structural dynamics: Experiment and Simulation. Tribol. Int. 2019, 138, 111–124. [Google Scholar] [CrossRef]
- Sun, J.Y.; Yang, H.Y.; Li, D.W.; Xu, C. Experimental investigation on acoustic emission in fretting friction and wear of bolted joints. J. Sound Vib. 2023, 558, 1–18. [Google Scholar] [CrossRef]
- Ranjan, P.; Pandey, A.K. Experimental characterization and parameter identification of bolted joints under vibratory loading. Tribol. Int. 2023, 186, 1–11. [Google Scholar]
- Vingsbo, O.; Söderberg, S. On fretting maps. Wear 1988, 126, 131–147. [Google Scholar] [CrossRef]
- Schwingshackl, C.W.; Petrov, E.P.; Ewins, D.J. Measured and estimated friction interface parameters in a nonlinear dynamic analysis. Mech. Syst. Signal Process. 2012, 28, 574–584. [Google Scholar] [CrossRef]
- Wang, K.; Wu, J.W.; Zhao, G.Y.; Li, Z.K. Study on Friction Contact Characteristics of Turbine Shroud Damper. Aero-engine 2018, 44, 70–74. [Google Scholar]
- Chen, L.L.; Ma, Y.H.; Li, D.; Hong, J. Measurement of Contact Stiffness during Microslio. J. Aerosp. Power 2010, 25, 936–942. [Google Scholar]
- Yu, S.J.; Zhu, E.; Yao, X.F. Experiment Study on Friction Characteristics of Spherical Plain Bearings for Large Civil Aircraft Lifiting Devices. Civ. Aircr. Des. Res. 2023, 1, 38–43. [Google Scholar]
- Zhang, D.W.; Yang, Y.C.; Lv, S.C.; Tian, C.; Li, Z.J. Fretting behavior of static metal seal and test rig for fretting friction with low/high temperature. Tribol. Int. 2023, 187, 1–13. [Google Scholar] [CrossRef]
- Wu, Y.; Li, L.; Fan, Y.; Ma, H.Y.; Wang, W.J.; Christen, J.L.; Ichchou, M. Design of semi-active dry friction dampers for steady-state vibration: Sensitivity analysis and experimental studies. J. Sound Vib. 2019, 459, 114850. [Google Scholar] [CrossRef]
- Cui, X.; Gao, Y.; Muggleton, J.; Liu, Y. Superimposed imaging of acoustic wave reflections for the detection of underground nonmetallic pipelines. Mech. Syst. Signal Process. 2024, 209, 111127. [Google Scholar] [CrossRef]
- Wu, Y.; Chen, J.; Fan, Y.; Li, L.; Jiang, Z. An MFC-based friction damper with adjustable normal force: Conception, modelling, and experiment. Mech. Syst. Signal Process. 2024, 215, 111450. [Google Scholar] [CrossRef]
- Gao, Q.; Fan, Y.; Wu, Y.G.; Li, L.; Zhang, D.Y. Insight into the influence of frictional heat on the modal characteristics and interface temperature of frictionally damped turbine blades. J. Sound Vib. 2024, 581, 118410. [Google Scholar] [CrossRef]
- Ma, H.; Li, L.; Wu, Y.G.; Fan, Y.; Gao, Q. Design of dry friction dampers for thin-walled structures by an accelerated dynamic Lagrange method. J. Sound Vib. 2020, 489, 115550. [Google Scholar] [CrossRef]
- Sun, H.; Zhang, D.; Wu, Y.; Shen, Q.; Hu, D. A semi-analytical multi-harmonic balance method on full-3D contact model for dynamic analysis of dry friction systems. Chin. J. Aeronaut. 2024, 37, 309–329. [Google Scholar] [CrossRef]
- Firrone, C.M.; Zucca, S.; Gola, M.M. The effect of underplatform dampers on the forced response of bladed disks by a coupled static/dynamic harmonic balance method. Int. J. Non-Linear Mech. 2011, 46, 363–375. [Google Scholar] [CrossRef]
- Zucca, S.; Firrone, C.M. Nonlinear dynamics of mechanical systems with friction contacts: Coupled static and dynamic Multi-Harmonic Balance Method and multiple solutions. J. Sound Vib. 2014, 333, 916–926. [Google Scholar] [CrossRef]
- Lakshminarayana, R.T.; Botto, D.; Zucca, Z. A novel test rig to study the effect of fretting wear on the forced response dynamics with a friction contact. Nonlinear Dyn. 2021, 105, 1405–1426. [Google Scholar]
- Tamatam, L.R.; Botto, D.; Zucca, Z. A coupled approach to model wear effect on shrouded bladed disk dynamics. Int. J. Mech. Sci. 2023, 237, 1–17. [Google Scholar] [CrossRef]
- Gao, Q.; Fan, Y.; Wu, Y.G.; Liu, J.L.; Wang, J.; Li, L. A novel test apparatus to study the mechanism of harmonic normal force on fretting wear. Tribol. Int. 2024, 191, 109091. [Google Scholar] [CrossRef]
- Moura, J.P.; Neto, J.V.D.F.; Rêgo, P.H.M. A neuro-fuzzy model for online optimal tuning of PID controllers in industrial system applications to the mining sector. IEEE Trans. Fuzzy Syst. 2020, 288, 1864–1877. [Google Scholar] [CrossRef]
- Liu, J.; Li, T.; Zhang, Z.; Chen, J. NARX prediction-based parameters online tuning method of intelligent PID system. IEEE Access 2020, 8, 130922–130936. [Google Scholar] [CrossRef]
- Lavella, M.; Botto, D.; Gola, M.M. Design of a high-precision, flat-on-flat fretting test apparatus with high temperature capability. Wear 2013, 302, 1073–1081. [Google Scholar] [CrossRef]


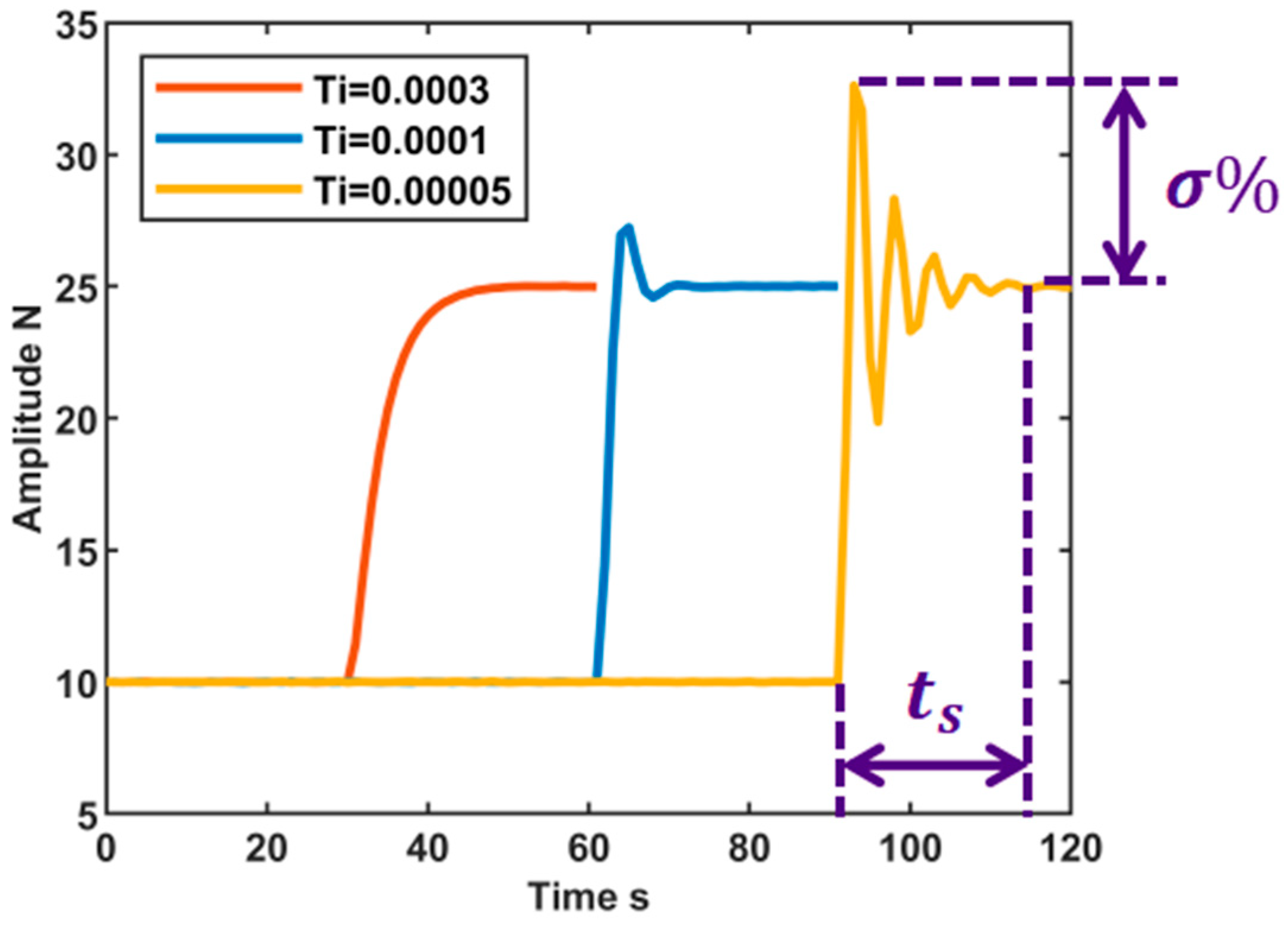



| 0.0003 | 19 s | 0 |
| 0.0001 | 11 s | 8.9% |
| 0.00005 | 25 s | 30.5% |
| A | C | |||
|---|---|---|---|---|
| parameter | 0.0005 | 0.0005 | 0.003 | |
| 0.0001 | 0.0001 | 0.0001 | ||
| 0.0001 | 0.0001 | 0.0001 | ||
| 10 s | 9 s | 7 s | ||
| 7.4% | 6.0% | 3.5% | ||
| Technical Parameter | Specification | |
|---|---|---|
| Range of Applied Normal Force | Constant (C) | 15 N ≤ C ≤ 300 N |
| Time-varying Amplitude (A) | ≤50 N | |
| ) | ≤300 Hz | |
| Range of Relative Displacement | Amplitude | ≤2 mm |
| Frequency | ≤150 Hz | |
| Measurement Accuracy | Force | 0.01 N |
| Displacement | 0.1 μm | |
| Control Accuracy | Force Amplitude | ≤3% |
| Force Phase | ≤5° | |
| RD/mm | 0.3 | 0.5 | 0.7 |
|---|---|---|---|
| μ | 0.385 | 0.381 | 0.394 |
| 10 | 70 | 130 | |
|---|---|---|---|
| μ(a) | 0.272 | 0.297 | 0.219 |
| μ(b) | 0.340 | 0.352 | 0.226 |
| μ(c) | - | 0.331 | 0.272 |
| 50 N | 70 N | 100 N | ||
|---|---|---|---|---|
| μ | Iron-based + GH605 | 0.3095 | 0.3151 | 0.2566 |
| Ceramic B + GH605 | 0.1905 | 0.2104 | 0.1891 | |
| Mar-M247C + Mar-M247CC | 0.1226 | 0.1088 | 0.1307 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, D.; Wang, J.; Li, H.; Meng, G.; Li, A. A Frequency Domain PID Control Strategy for an In-House Friction and Wear Test Rig. Aerospace 2024, 11, 623. https://doi.org/10.3390/aerospace11080623
Li D, Wang J, Li H, Meng G, Li A. A Frequency Domain PID Control Strategy for an In-House Friction and Wear Test Rig. Aerospace. 2024; 11(8):623. https://doi.org/10.3390/aerospace11080623
Chicago/Turabian StyleLi, Di, Jing Wang, Hongguang Li, Guang Meng, and Anlue Li. 2024. "A Frequency Domain PID Control Strategy for an In-House Friction and Wear Test Rig" Aerospace 11, no. 8: 623. https://doi.org/10.3390/aerospace11080623
APA StyleLi, D., Wang, J., Li, H., Meng, G., & Li, A. (2024). A Frequency Domain PID Control Strategy for an In-House Friction and Wear Test Rig. Aerospace, 11(8), 623. https://doi.org/10.3390/aerospace11080623





