Wind Tunnel Experiment and Numerical Simulation of Secondary Flow Systems on a Supersonic Wing
Abstract
1. Introduction
2. Geometrical Models and Performance Indicator Definitions
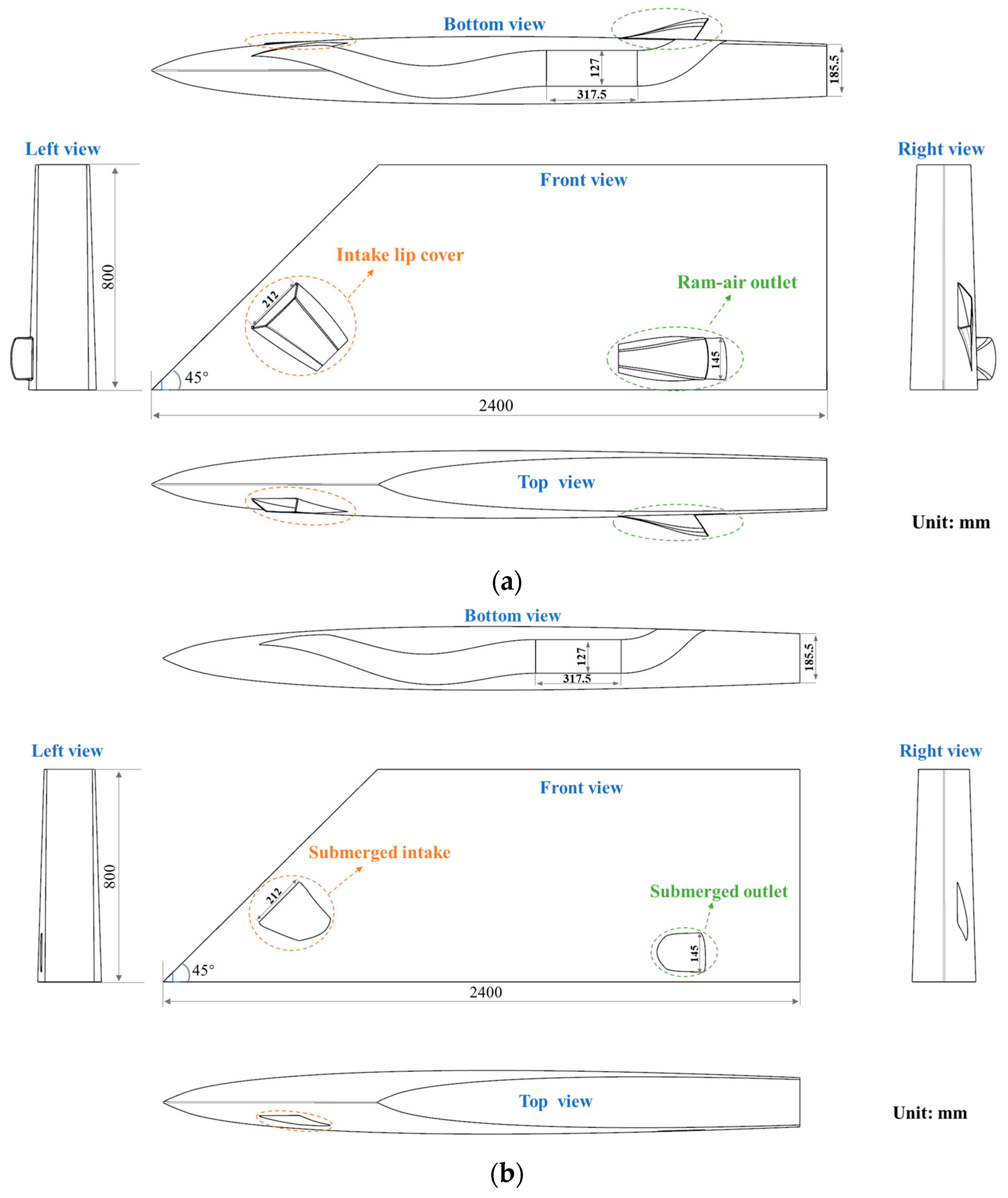
2.1. Geometrical Models
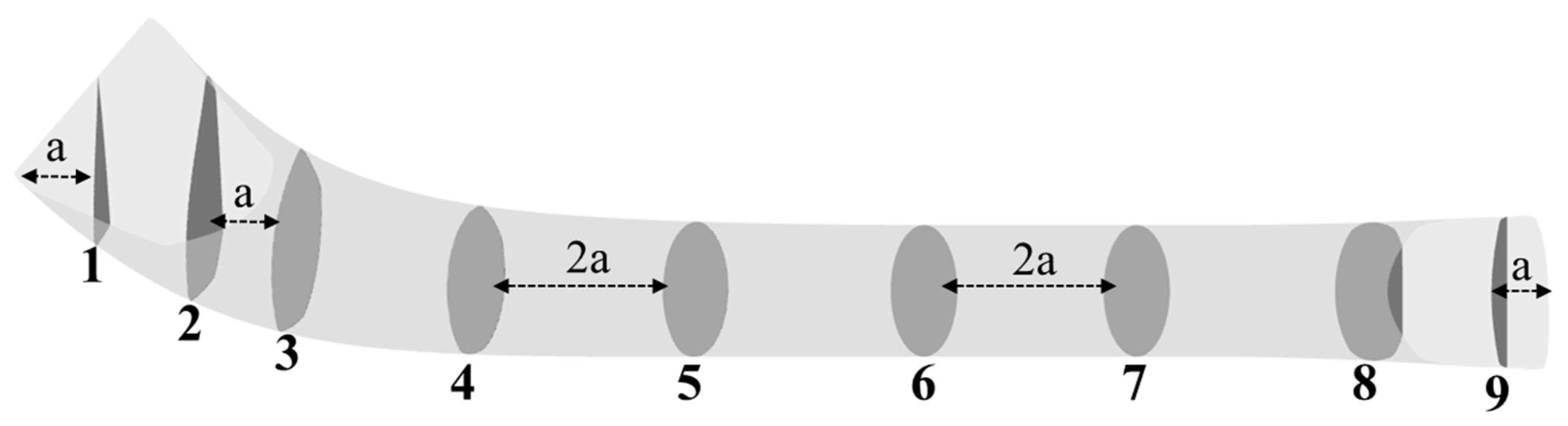
2.2. Parameterization
3. Methods
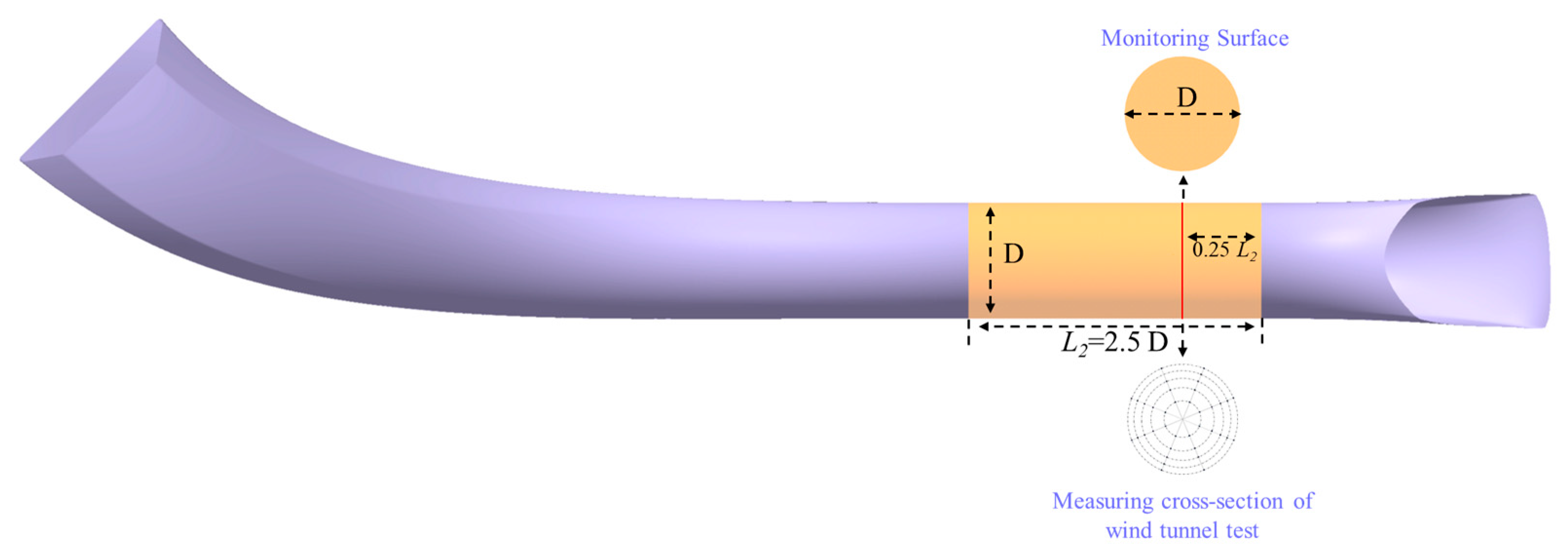
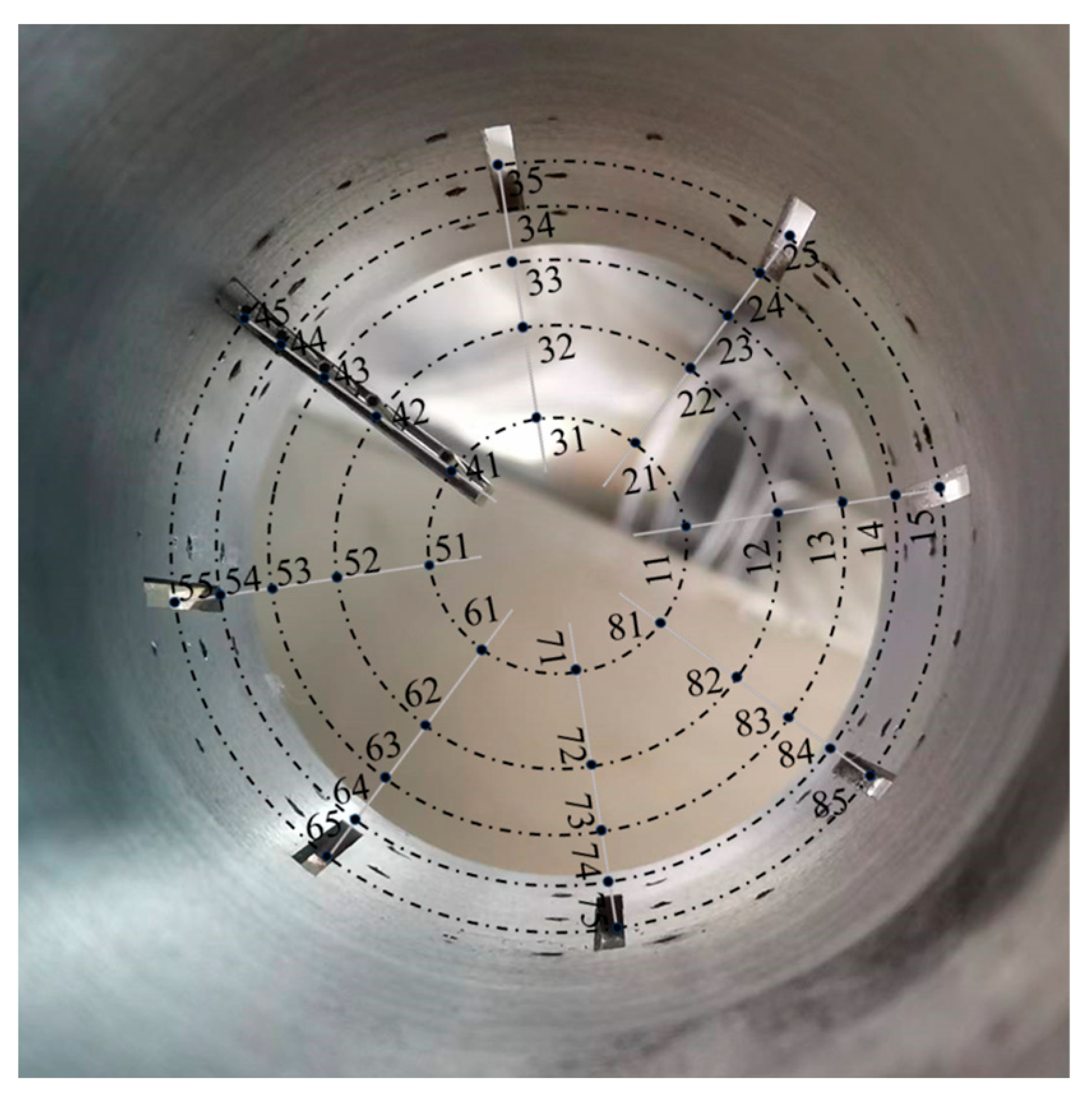
3.1. Experiment
3.2. Numerical Simulation
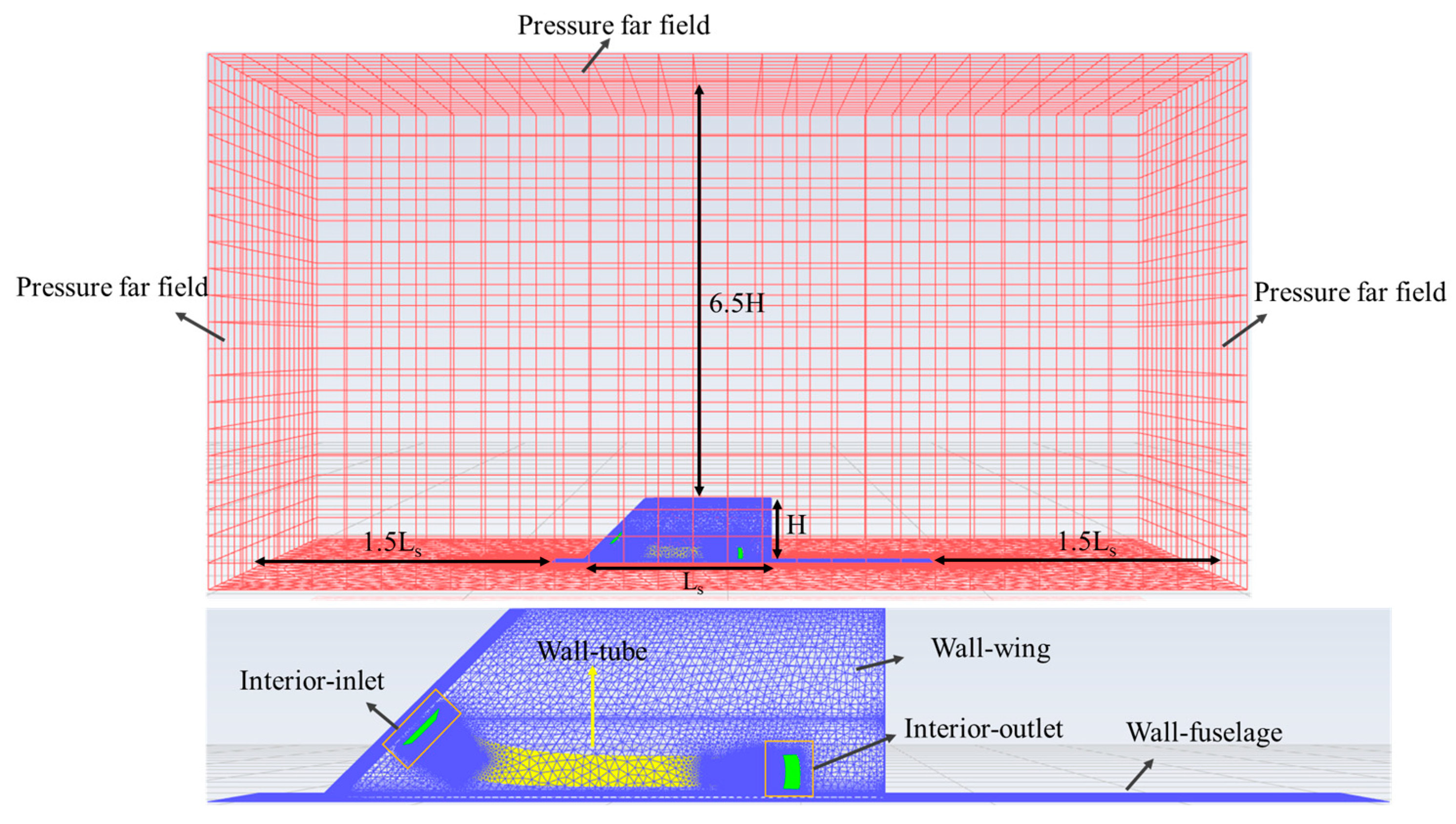
3.2.1. Numerical Methods
3.2.2. Grid Independence Verification
4. Result and Discussion
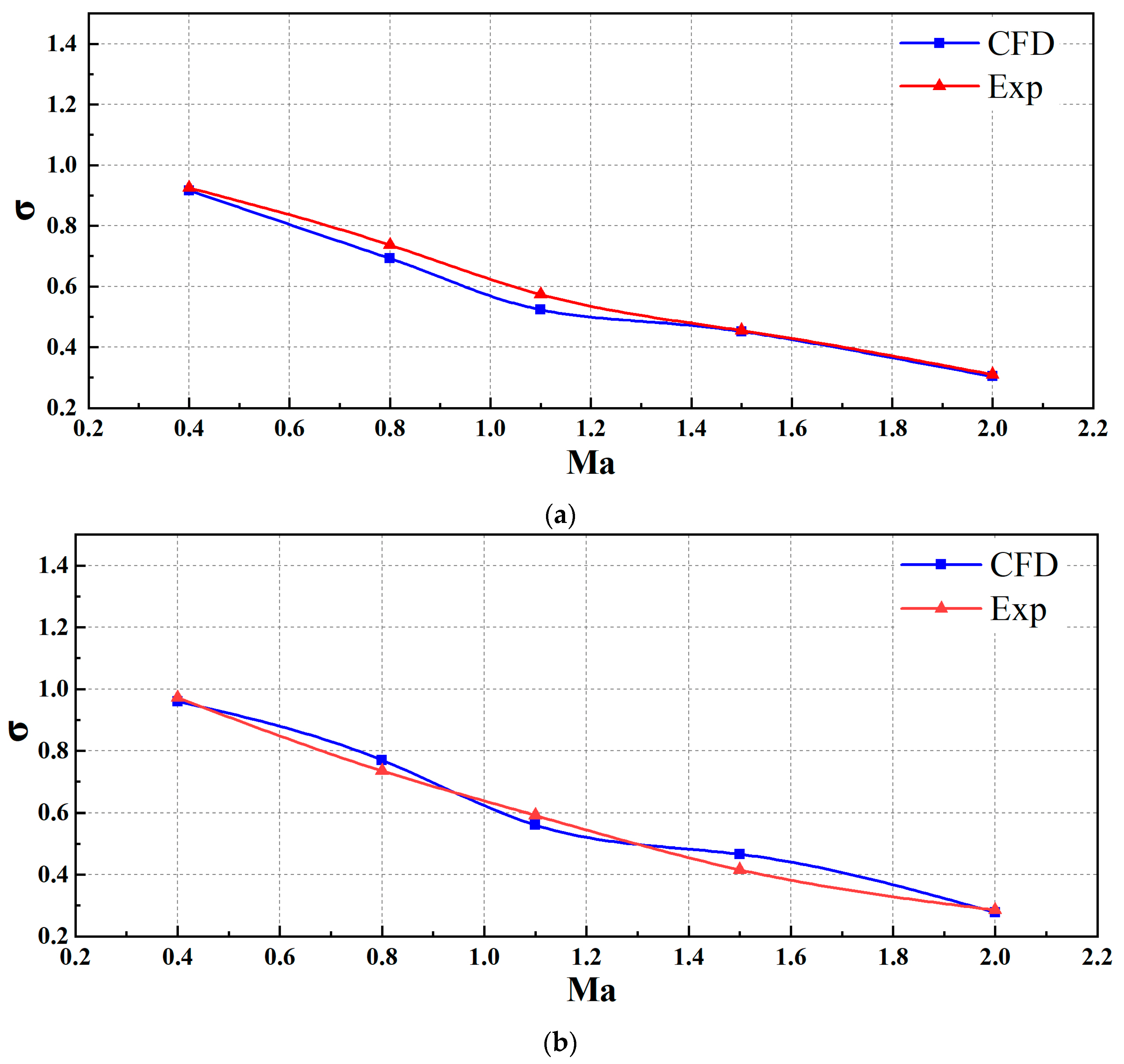
4.1. Analysis of Experimental Results
4.2. Analysis of Simulation Results
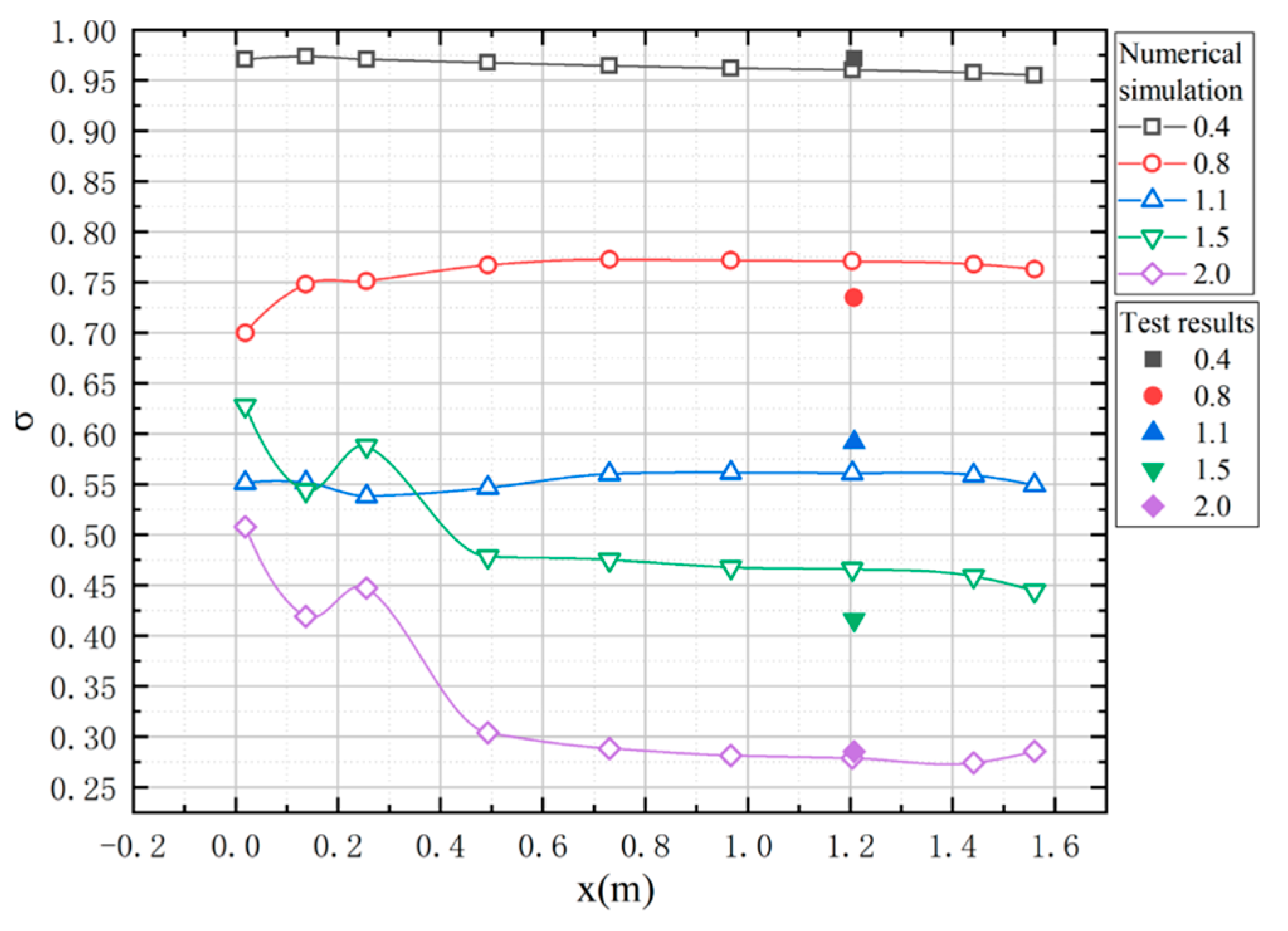
4.2.1. Analysis of Total Pressure Recovery ()
4.2.2. Analysis of Total Pressure Distortion ()
4.3. Flow Characteristics Analysis in the Ram-Air Secondary Flow System
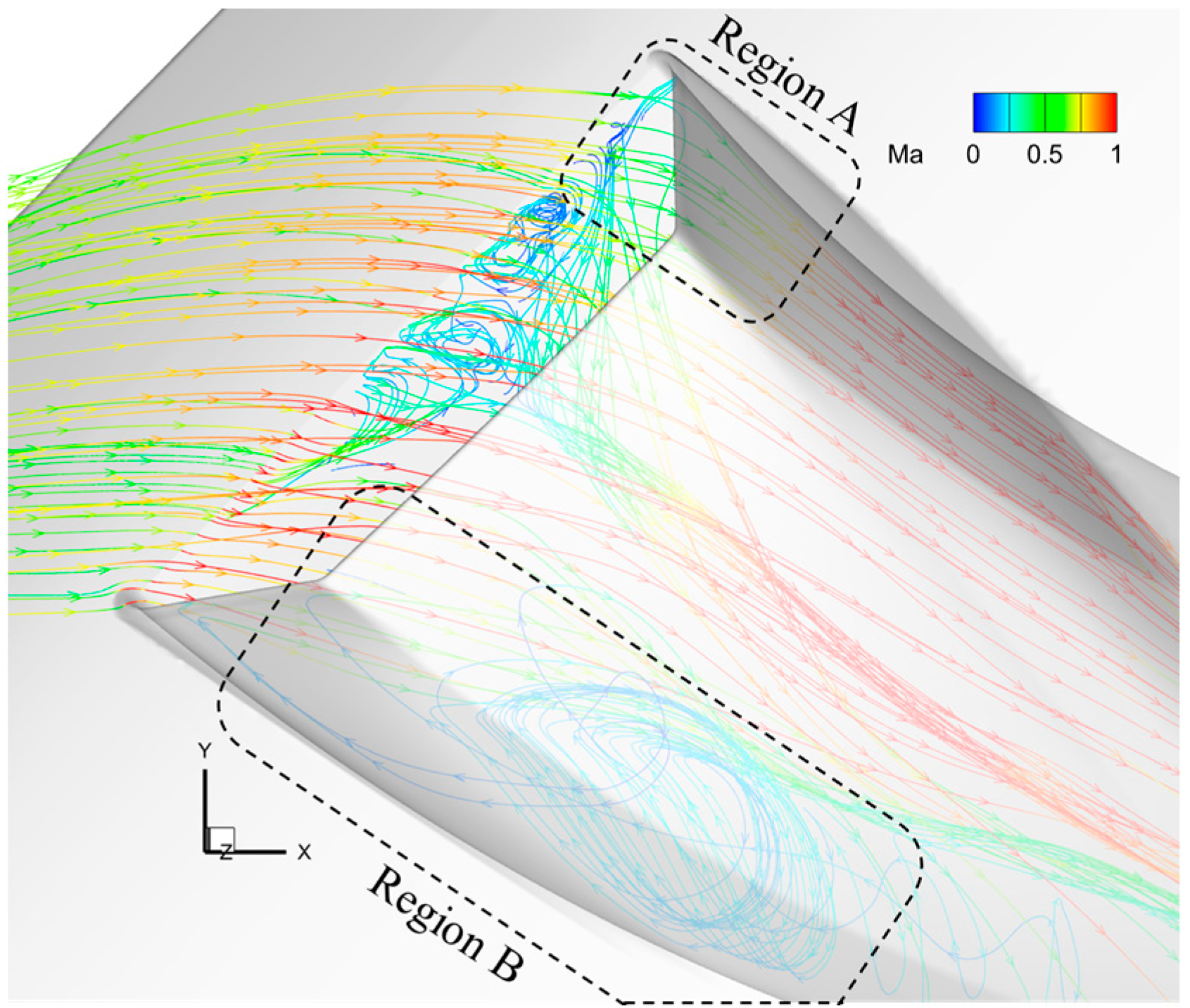
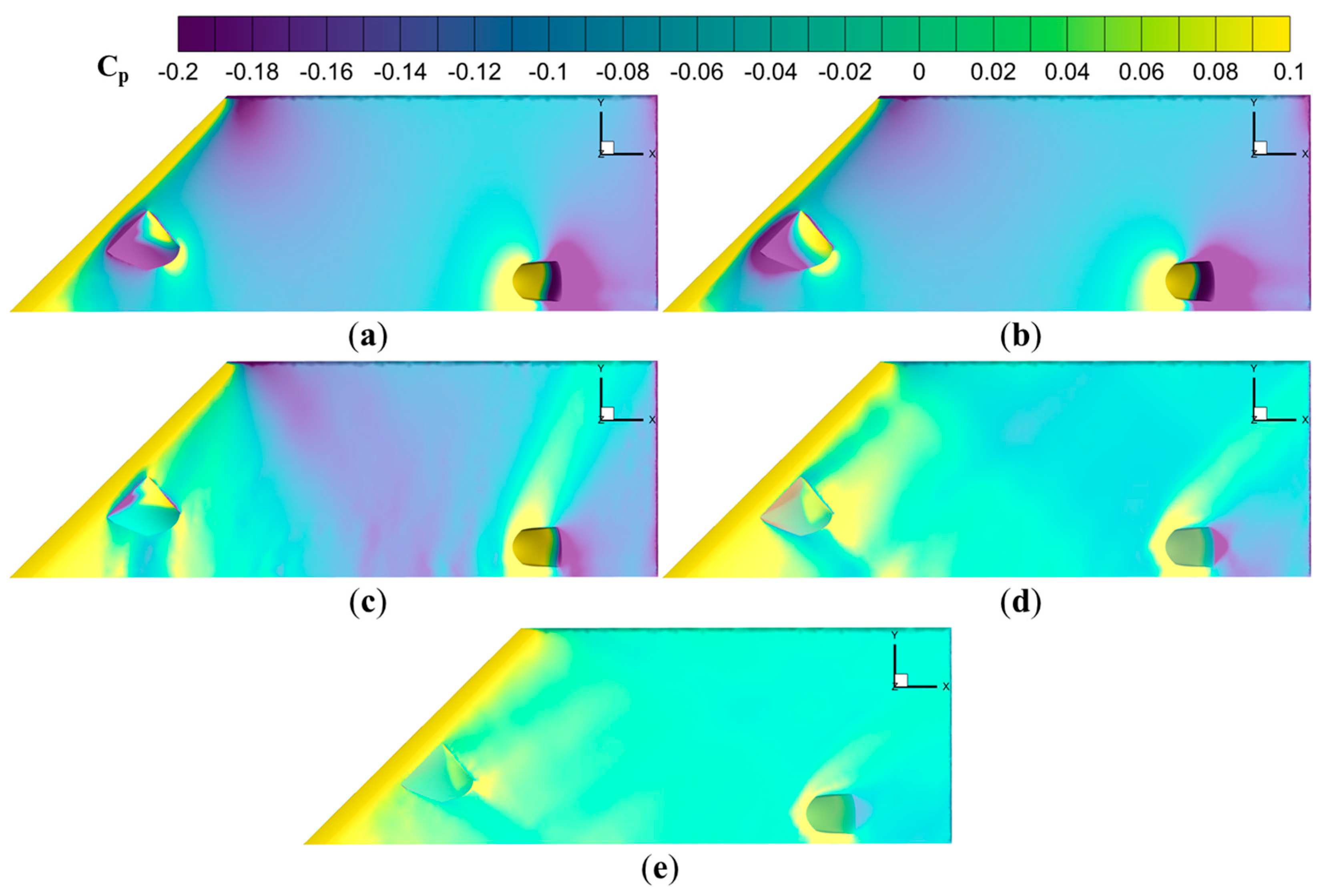
4.3.1. Flow Characteristics on the Wing
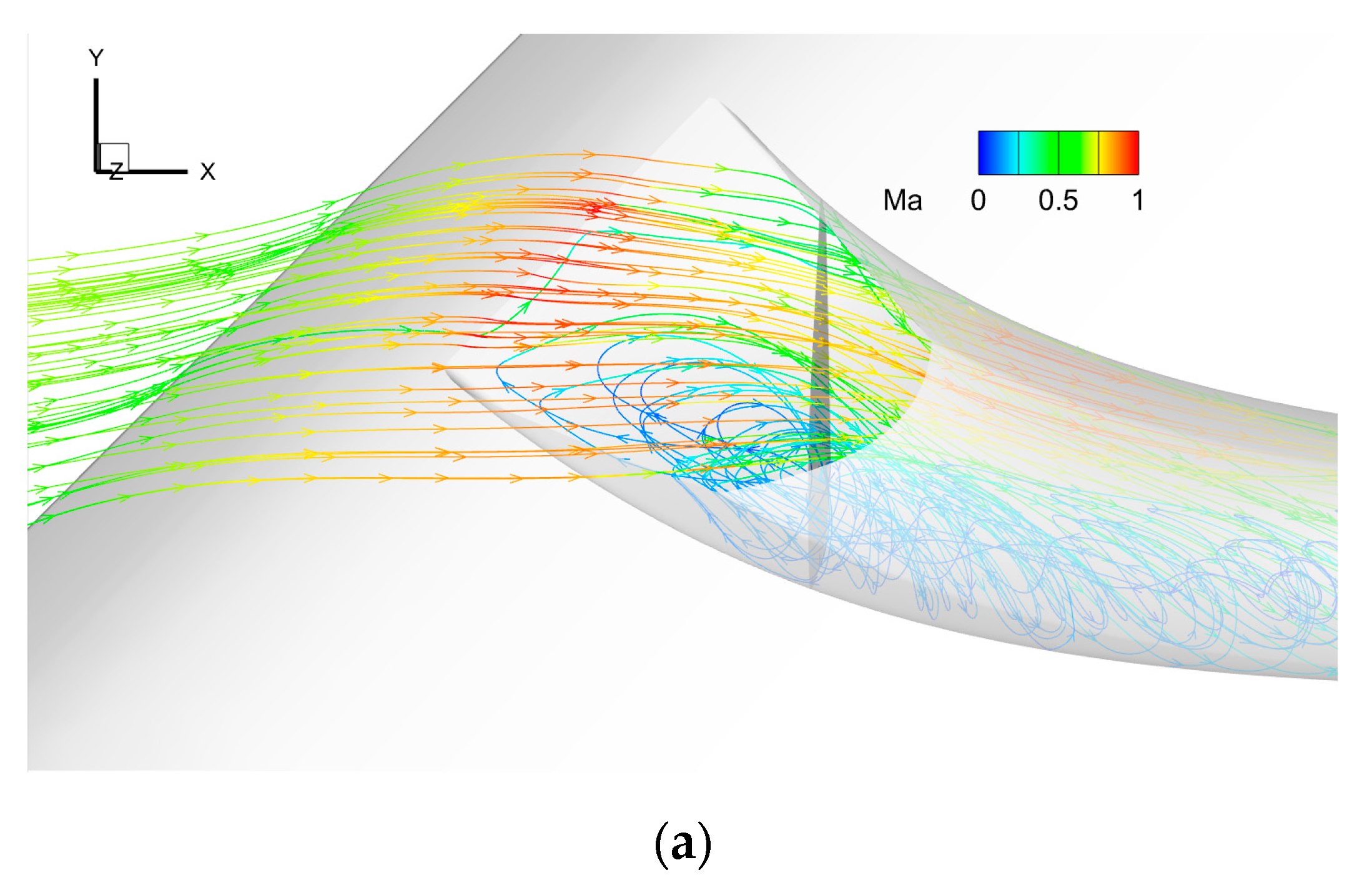
4.3.2. Flow Characteristics at the Intake
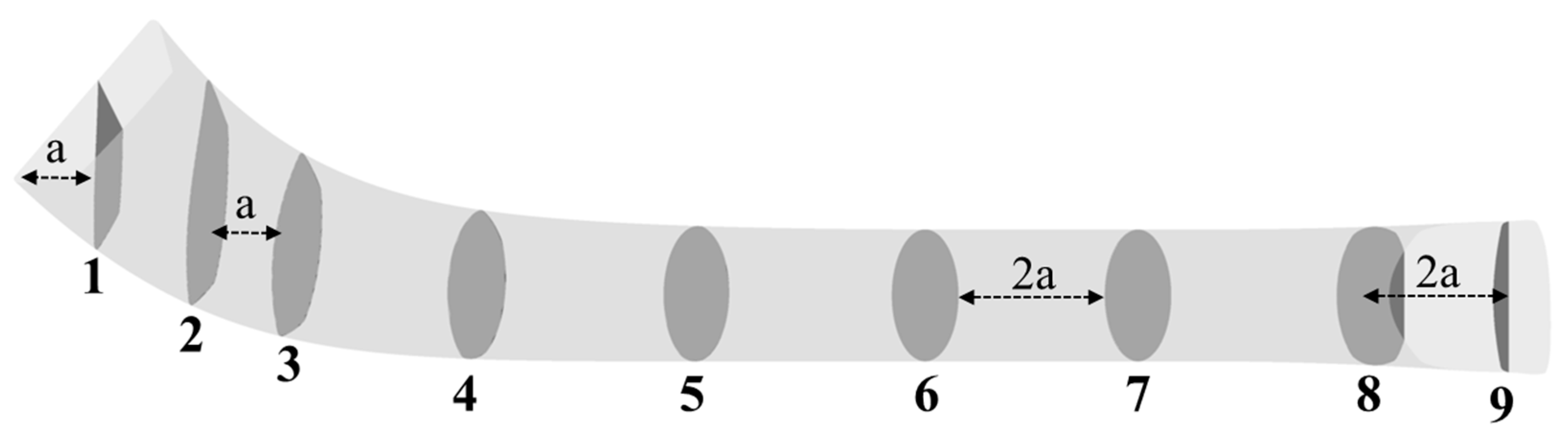
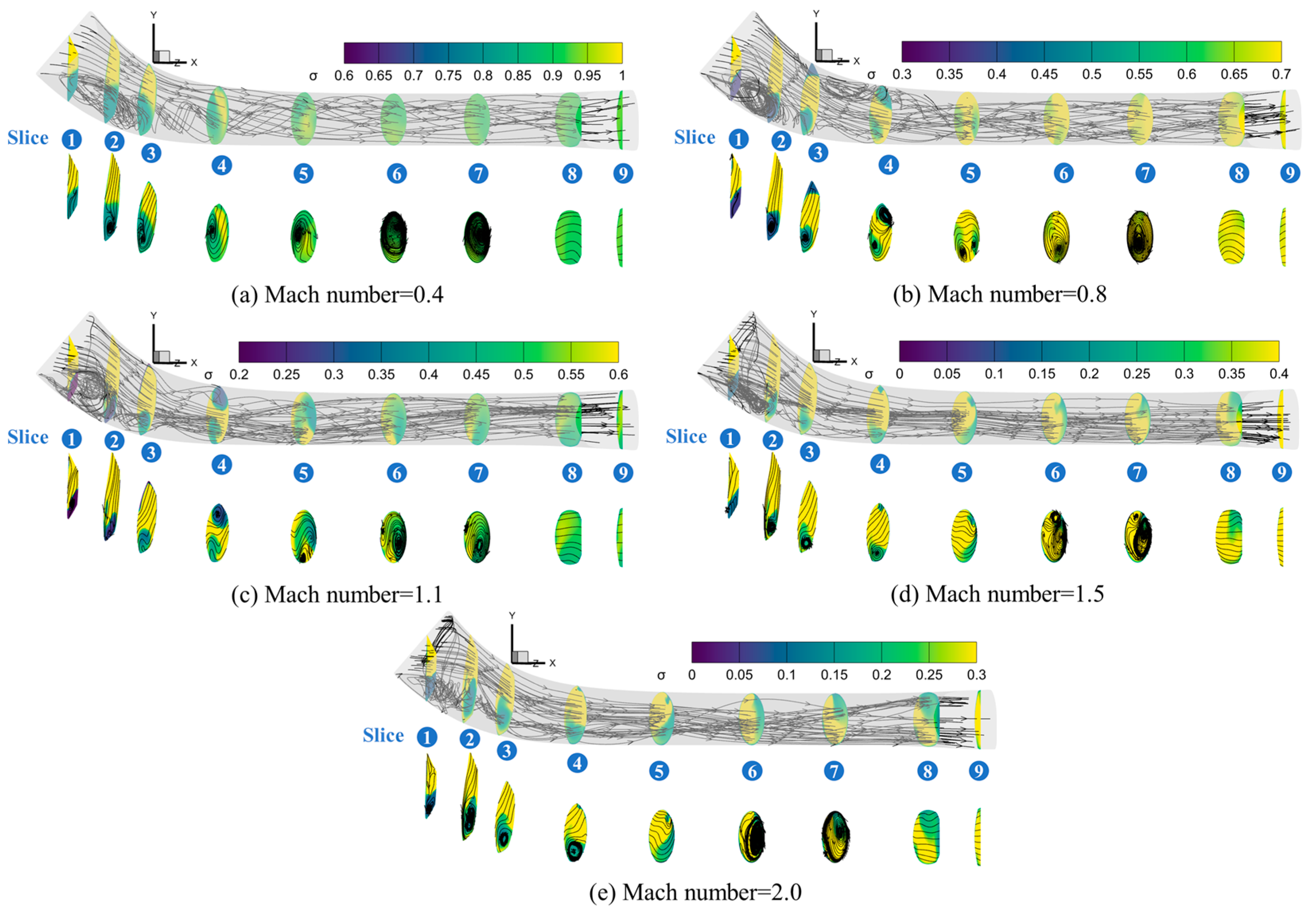
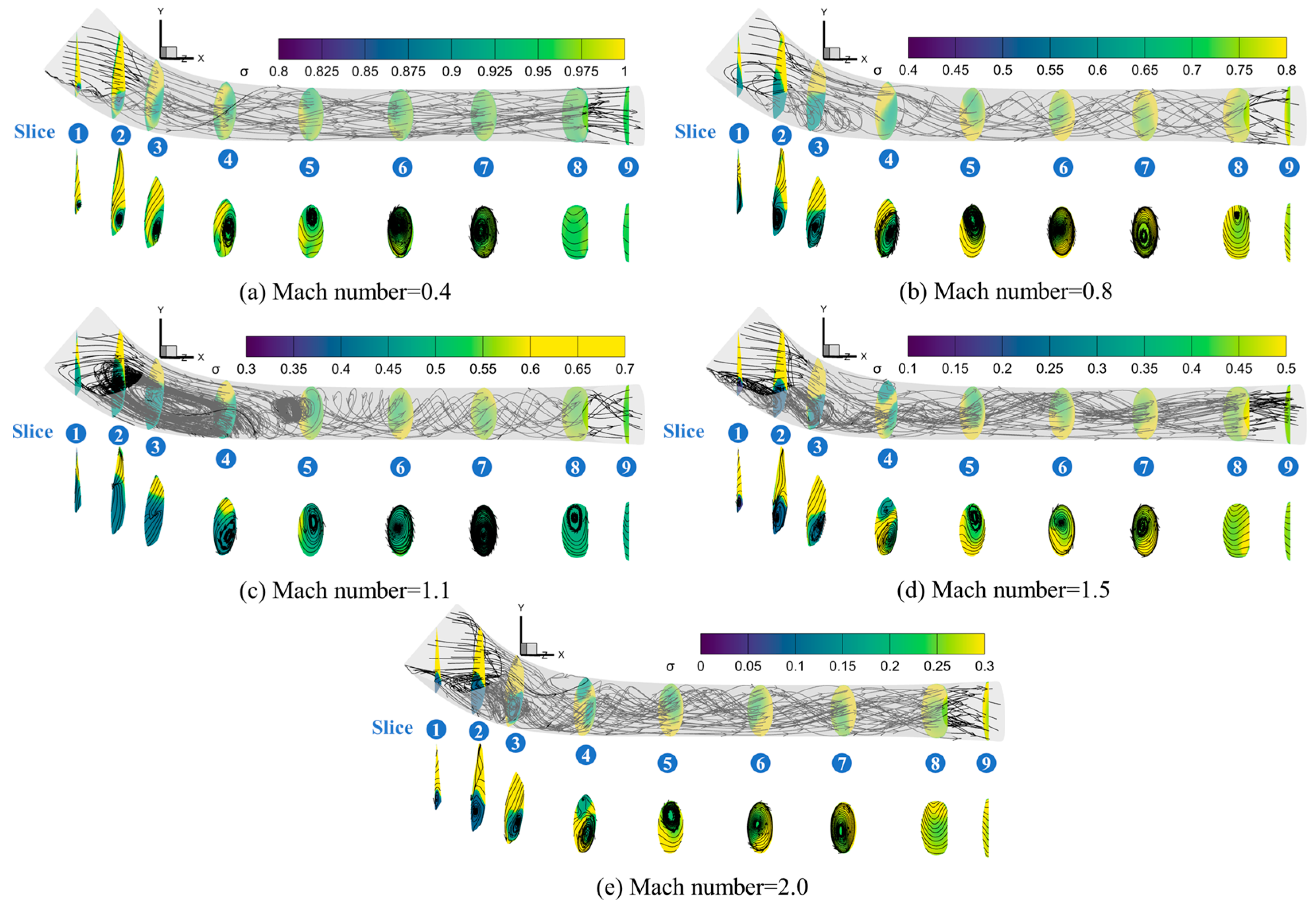
4.3.3. Flow Characteristics in the Tube
4.4. Flow Characteristics Analysis in the Submerged Secondary Flow System
4.4.1. Flow Characteristics on the Wing
4.4.2. Flow Characteristics at the Intake
4.4.3. Flow Characteristics in the Tube
5. Conclusions
- (1)
- It demonstrates that the simulation method can accurately predict the total pressure recoveries, contrasting with experimental results. The relative errors are 8% for the ram-air system and 10% for the submerged system between calculation and experimental results, respectively.
- (2)
- The experiment and simulation results indicate that the total pressure recovery decreases and the total pressure distortion increases with the increase in Mach numbers. As the Mach number increases from 0.4 to 2, the total pressure recovery of the ram-air secondary flow system decreases by 68% and 71% for the submerged system, respectively. Moreover, the total pressure distortion of the ram-air and submerged secondary flow systems is increased by 19.7 times and 8.3 times, respectively.
- (3)
- There are two primary flow characteristics in the secondary flow system. Firstly, the separations in the tube are primarily impacted by adverse pressure gradients. The flow separation at the intake mainly affects the total pressure recovery. Secondly, the three-dimensional vortices in the center of the tube are caused by the transition of the cross-section shape. The strength of three-dimensional vortices modifies the total pressure distribution over the cross-section and influences the total pressure distortion at the outlet.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| the maximum centerline offset of the y–x plane | |
| the maximum centerline offset of the z–x plane | |
| the length of the cylinder tube | |
| the monitor number | |
| the average total pressure measured by the monitor | |
| the total pressure of the inflow | |
| total pressure distortion coefficient | |
| total pressure recovery |
References
- Sziroczak, D.; Smith, H. A review of design issues specific to hypersonic flight vehicles. Prog. Aerosp. Sci. 2016, 84, 1–28. [Google Scholar] [CrossRef]
- Ferri, A. Review of scramjet propulsion technology. J. Aircraft 1968, 5, 3–10. [Google Scholar] [CrossRef]
- Anderson, B. Factors which influence the analysis and design of ejector nozzles. In Proceedings of the 10th Aerospace Sciences Meeting, San Diego, CA, USA, 17–19 January 1972; p. 46. [Google Scholar] [CrossRef][Green Version]
- Collie, W.; Burgun, R.; Heinzen, S.; Hall, C., Jr.; Chokani, N. Advanced propulsion system design and integration for a turbojet powered unmanned aerial vehicle. In Proceedings of the 41st Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 2003; p. 415. [Google Scholar] [CrossRef]
- Heiser, W.H. Ejector thrust augmentation. J. Propuls. Power 2010, 26, 1325–1330. [Google Scholar] [CrossRef]
- Li, N.; Chang, J.; Jiang, C.; Yu, D.; Bao, W.; Song, Y.; Jiao, X. Unstart/restart hysteresis characteristics analysis of an over–under TBCC inlet caused by backpressure and splitter. Aerosp. Sci. Technol. 2018, 72, 418–425. [Google Scholar] [CrossRef]
- Seddon, J.; Goldsmith, E.L. Intake Aerodynamics; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1985. [Google Scholar]
- Frank, J.L.; Taylor, R.A. Comparison of Drag, Pressure Recovery, and Surface Pressure of a Scoop-Type Inlet and an NACA Submerged Inlet at Transonic Speeds; National Advisory Committee for Aeronautics: Washington, DC, USA, 1951. Available online: https://ntrs.nasa.gov/citations/19930086824 (accessed on 23 July 2023).
- Mossman, E.A.; Randall, L.M. An Experimental Investigation of the Design Variables for NACA Submerged Duct Entrances; National Advisory Committee for Aeronautics: Washington, DC, USA, 1948. Available online: https://ntrs.nasa.gov/citations/19930093809 (accessed on 23 July 2023).
- Martin, N.J.; Holzhauser, C.A. An Experimental Investigation at Large Scale of Several Configurations of an NACA Submerged Air Intake; National Advisory Committee for Aeronautics: Washington, DC, USA, 1948. Available online: https://ntrs.nasa.gov/citations/19930085452 (accessed on 23 July 2023).
- Taylor, R.A. Some Effects of Side-Wall Modifications on the Drag and Pressure Recovery of an NACA Submerged Inlet at Transonic Speeds; National Advisory Committee for Aeronautics: Washington, DC, USA, 1952. Available online: https://ntrs.nasa.gov/citations/19930087954 (accessed on 10 September 2023).
- Tan, H.J.; Guo, R.W. Design and wind tunnel study of a top-mounted diverter less inlet. Chin. J. Aeronaut. 2004, 17, 72–78. [Google Scholar] [CrossRef]
- Sun, S.; Guo, R.; Wu, Y. Characterization and Performance Enhancement of Submerged Inlet with Flush-Mounted Planar Side Entrance. J. Propuls. Power 2012, 23, 987–995. [Google Scholar] [CrossRef]
- Xie, W.; Yang, S.; Zeng, C.; Liao, K.; Ding, R.; Zhang, L.; Guo, S. Effects of forebody boundary layer on the performance of a submerged inlet. Aeronaut. J. 2021, 125, 1260–1281. [Google Scholar] [CrossRef]
- Lee, C.; Boedicker, C. Subsonic diffuser design and performance for advanced fighter aircraft. In Proceedings of the Aircraft Design Systems and Operations Meeting, Colorado Springs, CO, USA, 14–16 October 1985. [Google Scholar] [CrossRef]
- Yu, A.Y. A Study of the Design Method and an Investigation of the Aerodynamic Performance for a Submerged Inlet under a Stealthy Shaped Fuselage; Nanjing University of Aeronautics and Astronautics: Nanjing, China, 2003. [Google Scholar]
- Lee, B.J.; Kim, C. Automated design methodology of turbulent internal flow using discrete adjoint formulation. Aerosp. Sci. Technol. 2007, 11, 63–173. [Google Scholar] [CrossRef]
- Mayer, D.; Anderson, B.; Johnson, T. 3D subsonic diffuser design and analysis. In Proceedings of the 34th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Cleveland, OH, USA, 13–15 July 1998; p. 3418. [Google Scholar] [CrossRef]
- Anabtawi, A.; Blackwelder, R.; Lissaman, P.; Liebeck, R. An experimental study of vortex generators in boundary layer ingesting diffusers with a centerline offset. In Proceedings of the 35th Joint Propulsion Conference and Exhibit, Los Angeles, CA, USA, 20–24 June 1999; p. 2110. [Google Scholar] [CrossRef]
- Brear, M.J.; Warfield, Z.; Mangus, J.F.; Braddom, S.; Paduano, J.D.; Philhower, J.S. Flow separation within the engine inlet of an uninhabited combat air vehicle (UCAV). J. Fluids Eng. 2004, 126, 266–272. [Google Scholar] [CrossRef]
- Ming, L.; Wu, Y.; Yang, Z.; Zhu, Z.; Ouyang, H.; Li, L. Large eddy simulation investigation of S-shaped intake distortion and swirl characteristics. Aerosp. Sci. Technol. 2023, 141, 108578. [Google Scholar] [CrossRef]
- Abdolahipour, S.; Mani, M.; Taleghani, A.S. Parametric study of a frequency-modulated pulse jet by measurements of flow characteristics. Phys. Scr. 2021, 96, 125012. [Google Scholar] [CrossRef]
- Abdolahipour, S.; Mani, M.; Shams Taleghani, A. Experimental investigation of flow control on a high-lift wing using modulated pulse jet vortex generator. J. Aerosp. Eng. 2022, 35, 05022001. [Google Scholar] [CrossRef]
- Abdolahipour, S.; Mani, M.; Shams Taleghani, A. Pressure improvement on a supercritical high-lift wing using simple and modulated pulse jet vortex generator. Flow Turbul. Combust. 2022, 109, 65–100. [Google Scholar] [CrossRef]
- Mohammadi, M.; Taleghani, A.S. Active flow control by dielectric barrier discharge to increase stall angle of a NACA0012 airfoil. Arab. J. Sci. Eng. 2014, 39, 2363–2370. [Google Scholar] [CrossRef]
- Taleghani, A.S.; Shadaram, A.; Mirzaei, M. Effects of duty cycles of the plasma actuators on improvement of pressure distribution above a NLF0414 airfoil. IEEE Trans. Plasma Sci. 2012, 40, 1434–1440. [Google Scholar] [CrossRef]
- Taleghani, A.S.; Shadaram, A.; Mirzaei, M.; Abdolahipour, S. Parametric study of a plasma actuator at unsteady actuation by measurements of the induced flow velocity for flow control. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 173. [Google Scholar] [CrossRef]
- Noori, M.S.; Taleghani, A.S.; Rahni, M.T. Surface acoustic waves as control actuator for drop removal from solid surface. Fluid Dyn. Res. 2021, 53, 045503. [Google Scholar] [CrossRef]
- Rahni, M.T.; Taleghani, A.S.; Sheikholeslam, M.; Ahmadi, G. Computational simulation of water removal from a flat plate, using surface acoustic waves. Wave Motion 2022, 111, 102867. [Google Scholar] [CrossRef]
- Abdolahipour, S. Effects of low and high frequency actuation on aerodynamic performance of a supercritical airfoil. Front. Mech. Eng. 2023, 9, 1290074. [Google Scholar] [CrossRef]
- Su, W.Y.; Zhang, Y.; Jin, Z.G. Adaptive passive control on hypersonic inlet boundary layer separation. J. Propuls. Technol. 2011, 32, 455–460. [Google Scholar] [CrossRef]
- Harouni, A.G. Flow control of a boundary layer ingesting serpentine diffuser via blowing and suction. Aerosp. Sci. Technol. 2014, 39, 472–480. [Google Scholar] [CrossRef]
- Kumar, R.A.; Rajesh, G. Physics of vacuum generation in zero-secondary flow ejectors. Phys. Fluid 2018, 30, 066102. [Google Scholar] [CrossRef]
- Zhang, K.Y.; Zhang, R.X.; Xu, H. Investigation of single expansion ram-airp nozzle. J. Propuls. Technol. 2001, 22, 380–382. [Google Scholar]
- Abdollahzadeh, M.; Rodrigues, F.; Pascoa, J.; Oliveira, P. Numerical design and analysis of a multi-DBD actuator configuration for the experimental testing of ACHEON nozzle model. Aerosp. Sci. Technol. 2015, 41, 259–273. [Google Scholar] [CrossRef]
- Ma, S.; Tan, J.; Zhang, Z.; Zhu, W.; Jiang, H. Influence of secondary flow ejection on the performance of conformal asymmetric nozzle. J. Natl. Univ. Def. Technol. 2018, 40, 44–51. [Google Scholar] [CrossRef]
- Cheng, D.; Zhang, Y.; Gao, W.; Xue, Y. Investigation of a Variable Axisymmetric Inlet with Local Secondary Flow Recirculation. J. Propuls. Technol. 2019, 40, 2003–2011. [Google Scholar]
- NASA/TP-2005-213766; Berrier, B.L.; Carter, M.B.; Allan, B.G. High Reynolds Number Investigation of a Flush-Mounted, S-Duct Inlet with Large Amounts of Boundary Layer Ingestion. National Advisory Committee for Aeronautics: Washington, DC, USA, 2005. Available online: https://ntrs.nasa.gov/api/citations/20050229940/downloads/20050229940.pdf (accessed on 18 November 2023).
- Shams Taleghani, A.; Ghajar, A. Aerodynamic characteristics of a delta wing aircraft underground effect. Front. Mech. Eng. 2024, 10, 1355711. [Google Scholar] [CrossRef]
- Shams Taleghani, A.; Ghajar, A.; Masdari, M. Experimental study of ground effect on horizontal tail effectiveness of a conceptual advanced jet trainer. J. Aerosp. Eng. 2020, 33, 05020001. [Google Scholar] [CrossRef]
- Spalart, P.; Allmaras, S.A. one-equation turbulence model for aerodynamic flows. In Proceedings of the 30th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1992; p. 439. [Google Scholar] [CrossRef]
- Li, W.; Liu, Y. Numerical investigation of corner separation flow using Spalart-Allmaras model with various modifications. Aerosp. Sci. Technol. 2022, 127, 107682. [Google Scholar] [CrossRef]
- Babcock, D.A.; Tobaldini Neto, L.; Davis, Z.S. Summary of the 4th Propulsion Aerodynamics Workshop: S-duct Results. In Proceedings of the AIAA Propulsion and Energy 2019 Forum, Indianapolis, IN, USA, 19–22 August 2019; p. 3845. [Google Scholar] [CrossRef]
- Kennedy, S.; Robinson, T.; Spence, S.; Richardson, J. Computational Investigation of Inlet Distortion at High Angles of Attack. J. Aircr. 2014, 51, 361–376. [Google Scholar] [CrossRef]
- Berens, T.M.; Delot, A.-L.; Chevalier, M.; van Muijden, J. Numerical simulations for high offset intake diffuser flows. In Proceedings of the 52nd Aerospace Sciences Meeting, National Harbor, MD, USA, 13–17 January 2014; p. 0371. [Google Scholar] [CrossRef][Green Version]
- Moura Lima, L.S.; Batista de Jesus, A.; Huebner, R. Analysis of Geometric Features and Mesh Parameters on the 3rd PAW S-duct Case. In Proceedings of the 53rd AIAA/SAE/ASEE Joint Propulsion Conference, Atlanta, GA, USA, 10–12 July 2017; p. 4910. Available online: https://arc.aiaa.org/doi/pdf/10.2514/6.2017-4910 (accessed on 9 March 2024).








| Experimental Conditions | Mach Number | Total Temperature/K | Total Pressure/Pa |
|---|---|---|---|
| 1 | 0.4 | 297.37 | 104,517 |
| 2 | 0.8 | 325.03 | 114,824 |
| 3 | 1.1 | 357.89 | 129,843 |
| 4 | 1.5 | 417.82 | 177,629 |
| 5 | 2.0 | 518.67 | 209,392 |
| Measurement Points Circle Serial Number | Distance from Center of Circle ri (mm) | Relative Distance from the Center of Circle ri/R |
|---|---|---|
| 1 | 10.033 | 0.316 |
| 2 | 17.399 | 0.548 |
| 3 | 22.447 | 0.707 |
| 4 | 26.575 | 0.837 |
| 5 | 30.131 | 0.949 |
| Mesh Cells (Million) | |||
|---|---|---|---|
| Grid 1 | 0.9 | 0.7043 | 0.054 |
| Grid 2 | 1.8 | 0.6935 | 0.032 |
| Grid 3 | 3.6 | 0.6924 | 0.033 |
| Grid 4 | 7.2 | 0.6925 | 0.033 |
| Mach Number | ||
|---|---|---|
| 0.4 | 0.9254 | 0.0144 |
| 0.8 | 0.7368 | 0.0505 |
| 1.1 | 0.5733 | 0.0615 |
| 1.5 | 0.4553 | 0.1049 |
| 2.0 | 0.3104 | 0.1104 |
| Mach Number | ||
|---|---|---|
| 0.4 | 0.9716 | 0.02653 |
| 0.8 | 0.7351 | 0.01861 |
| 1.1 | 0.5918 | 0.04352 |
| 1.5 | 0.416 | 0.02509 |
| 2.0 | 0.2853 | 0.11008 |
| Mach Number | Start Position | End Position |
|---|---|---|
| 0.4 | Slices 1 | Slices 3 |
| 0.8 | Slices 1 | Slices 2 |
| 1.1 | Slices 1 | Slices 2 |
| 1.5 | Slices 1 | Slices 2 |
| 2.0 | Slices 1 | Slices 2 |
| Mach Number | Start Position | End Position |
|---|---|---|
| 0.4 | Slices 1 | Slices 1 |
| 0.8 | Slices 1 | Slices 3 |
| 1.1 | Slices 1 | Slices 4 |
| 1.5 | Slices 1 | Slices 3 |
| 2.0 | Slices 1 | Slices 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, S.; Lin, Z.; Gao, Z.; Miao, S.; Li, J.; Zeng, L.; Pan, D. Wind Tunnel Experiment and Numerical Simulation of Secondary Flow Systems on a Supersonic Wing. Aerospace 2024, 11, 618. https://doi.org/10.3390/aerospace11080618
Zhang S, Lin Z, Gao Z, Miao S, Li J, Zeng L, Pan D. Wind Tunnel Experiment and Numerical Simulation of Secondary Flow Systems on a Supersonic Wing. Aerospace. 2024; 11(8):618. https://doi.org/10.3390/aerospace11080618
Chicago/Turabian StyleZhang, Sheng, Zheng Lin, Zeming Gao, Shuai Miao, Jun Li, Lifang Zeng, and Dingyi Pan. 2024. "Wind Tunnel Experiment and Numerical Simulation of Secondary Flow Systems on a Supersonic Wing" Aerospace 11, no. 8: 618. https://doi.org/10.3390/aerospace11080618
APA StyleZhang, S., Lin, Z., Gao, Z., Miao, S., Li, J., Zeng, L., & Pan, D. (2024). Wind Tunnel Experiment and Numerical Simulation of Secondary Flow Systems on a Supersonic Wing. Aerospace, 11(8), 618. https://doi.org/10.3390/aerospace11080618






