Ground-Based Characterisation of a Compact Instrument for Gamma-ray Burst Detection on a CubeSat Platform
Abstract
1. Introduction
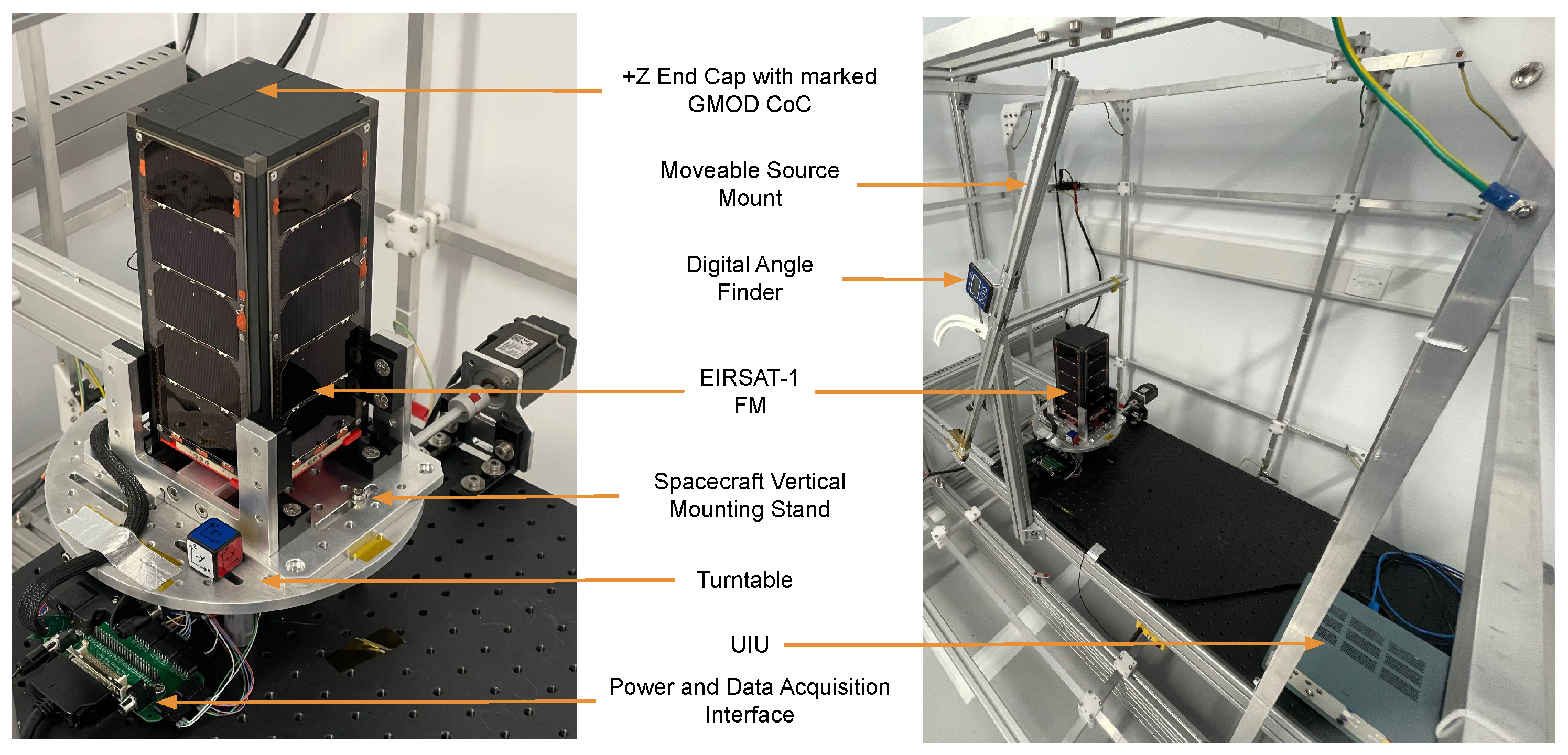
2. Characterisation Test Set-Up
2.1. Energy Calibration Hardware Set-Up

2.2. Angular Measurements Hardware Set-Up
2.3. Data Acquisition and Scripting
3. Energy Calibration
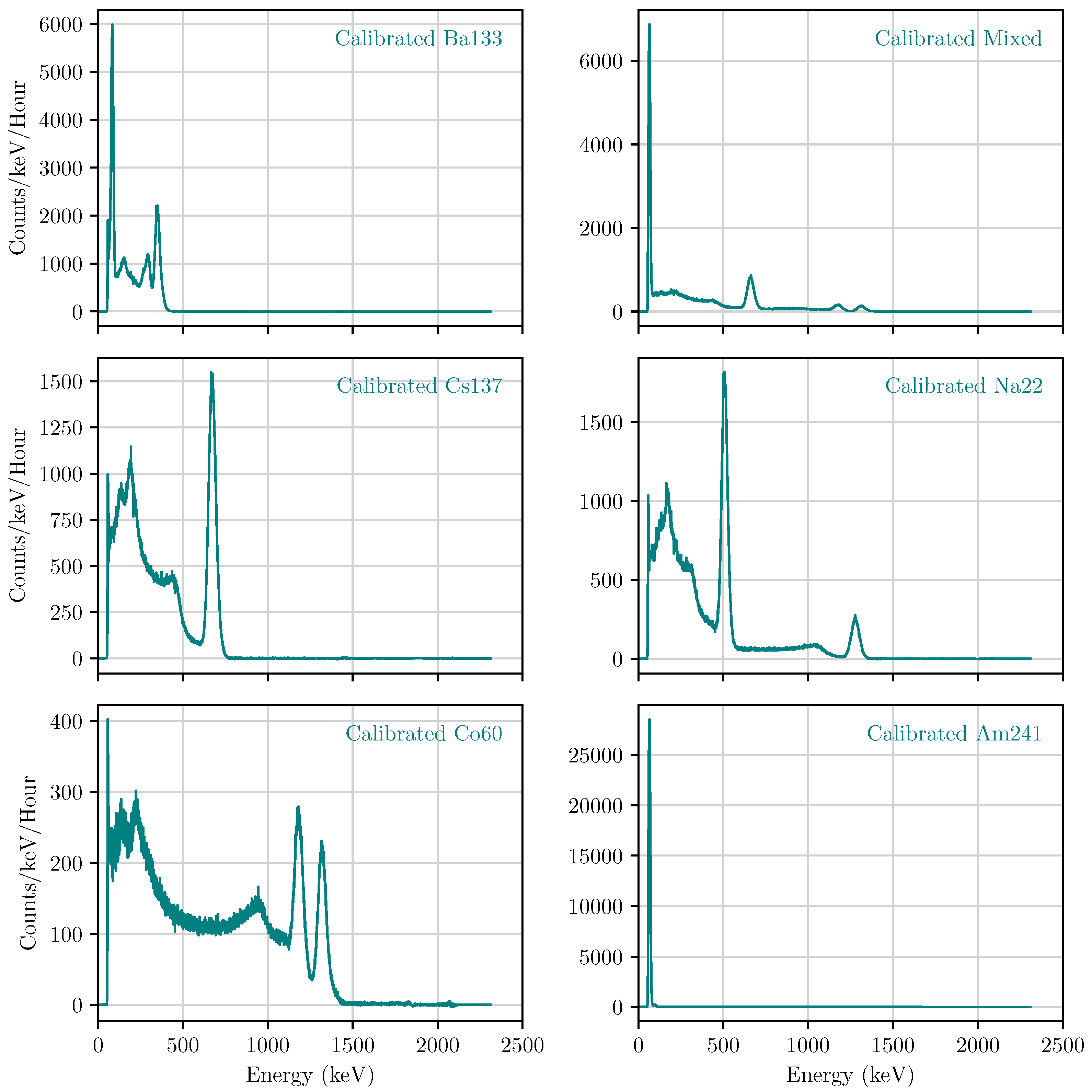
3.1. Single-Source Spectra
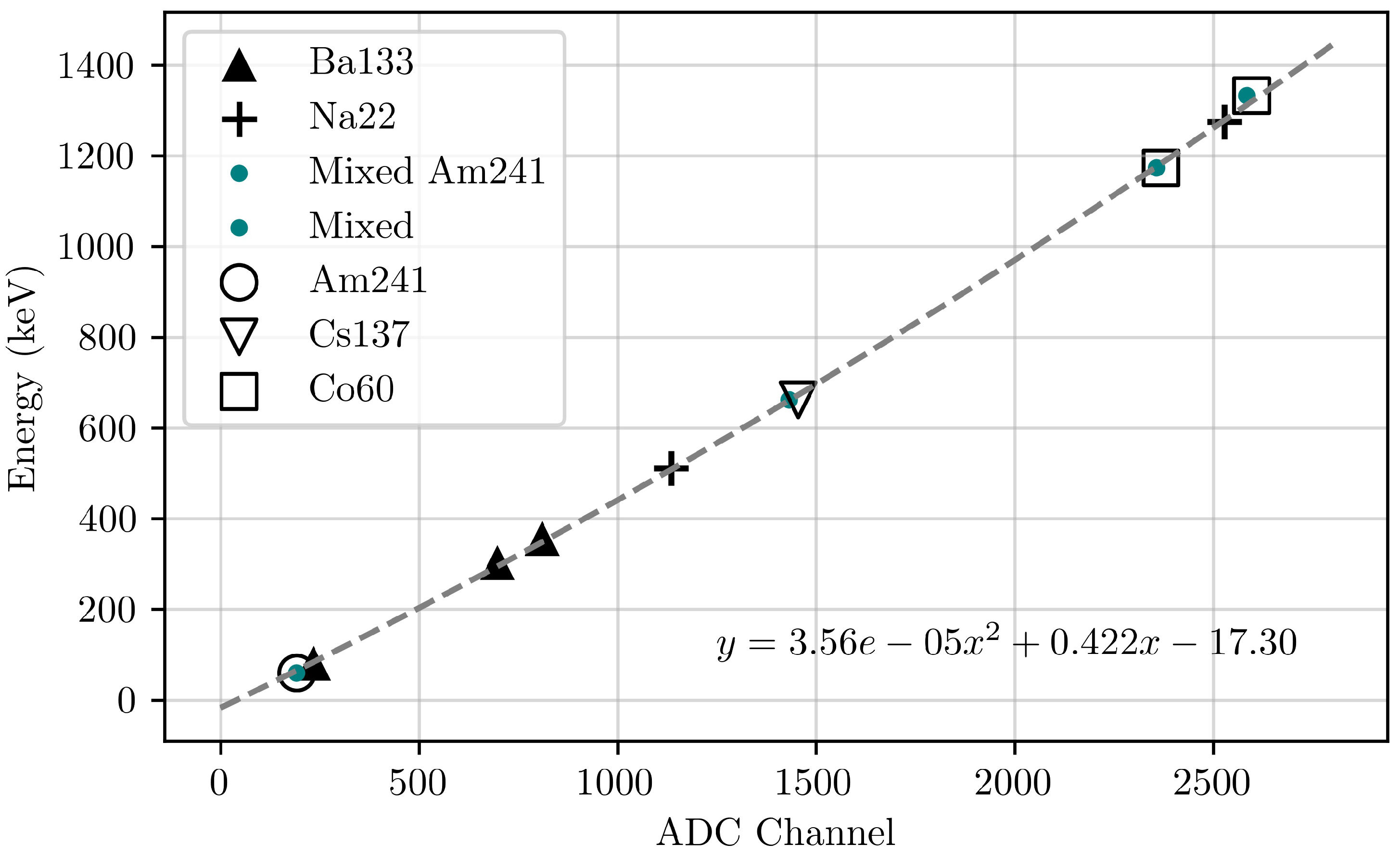
3.2. Channel–Energy Relation
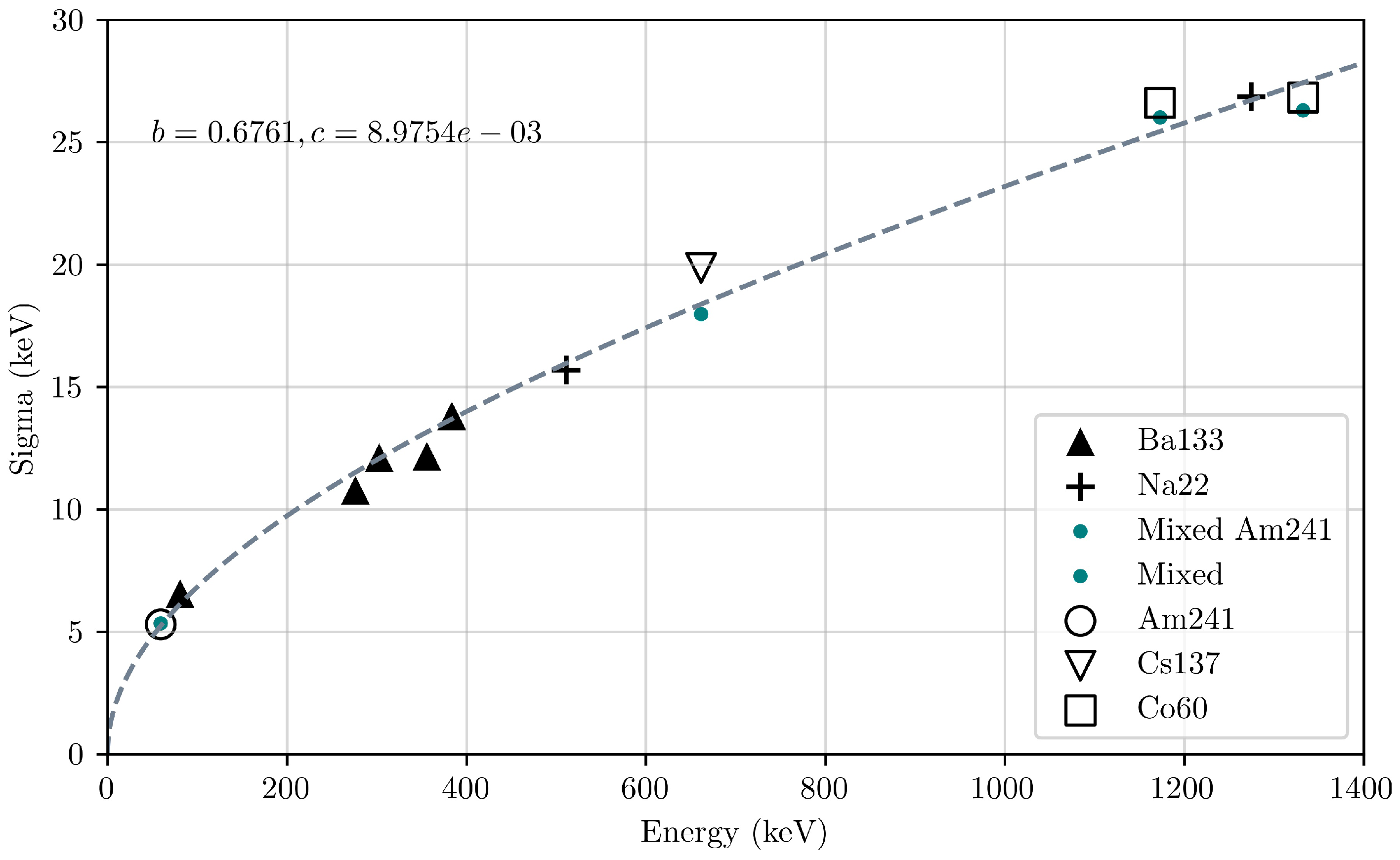
4. Energy Resolution
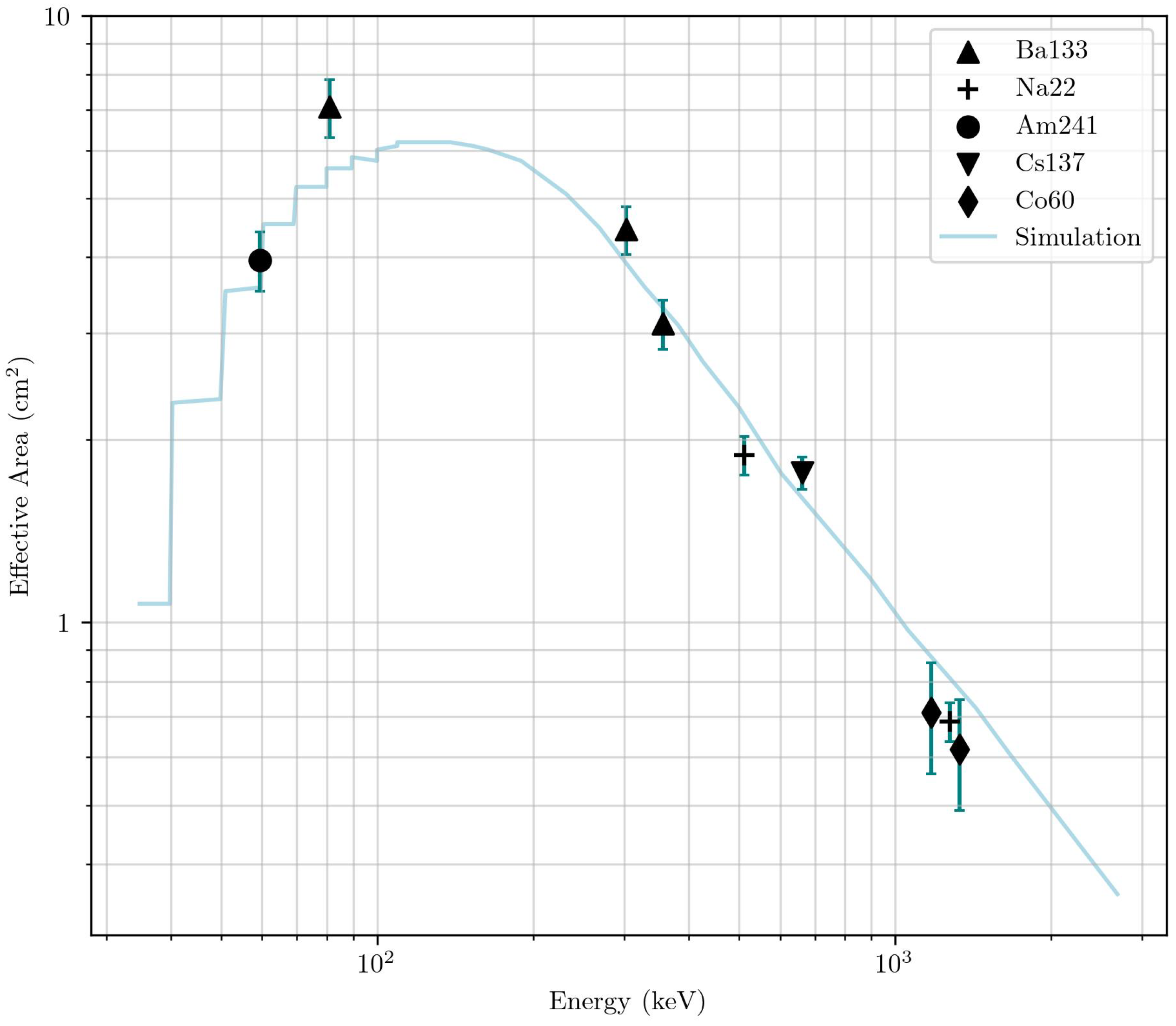
5. Effective Area
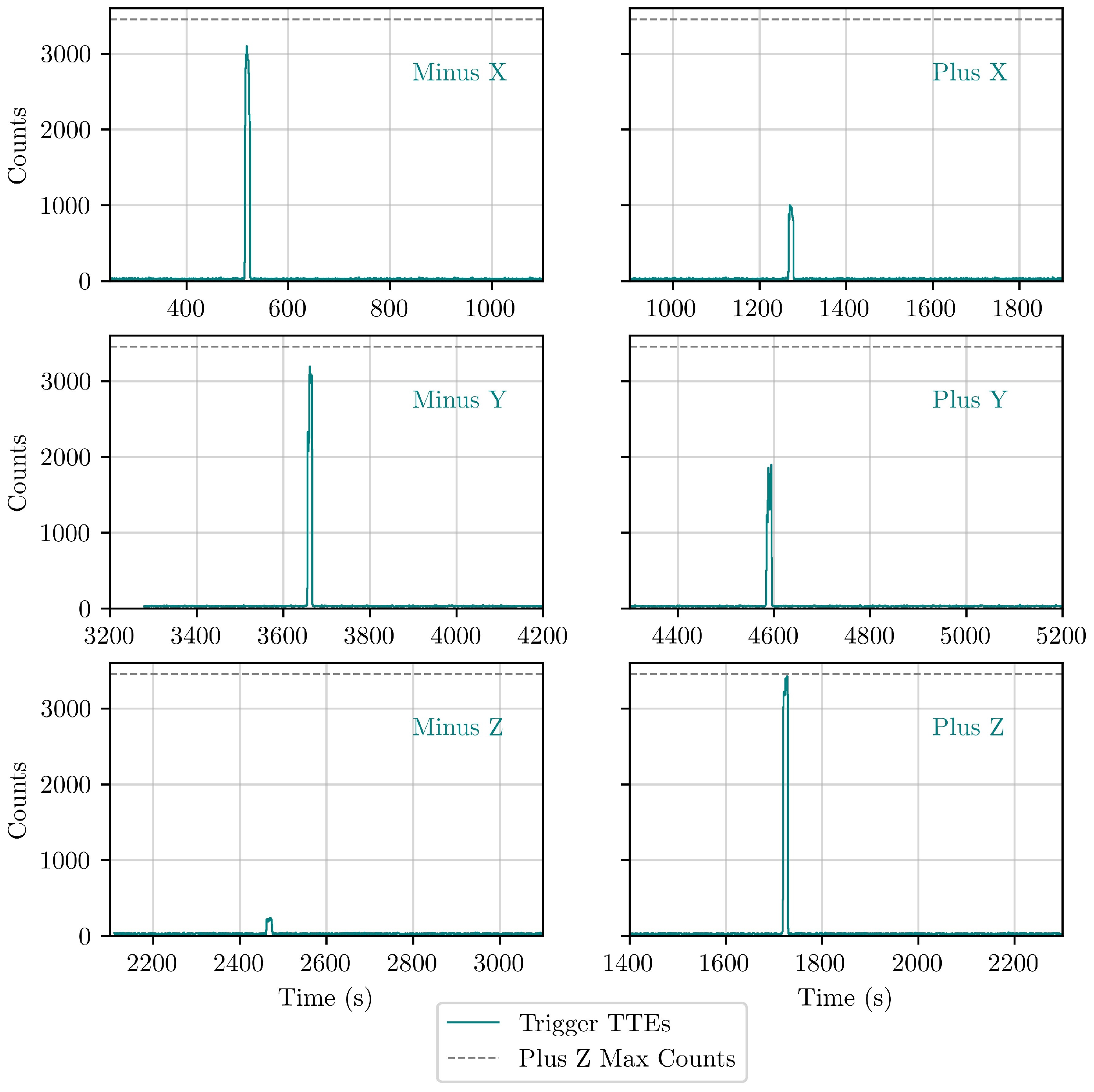
6. Trigger Algorithm Testing
7. Characterisation Simulations
7.1. MEGALib
- Geometry for MEGAlib (Geomega);
- A Cosmic Simulator for MEGAlib (Cosima);
- Real Event Analyser (Revan).
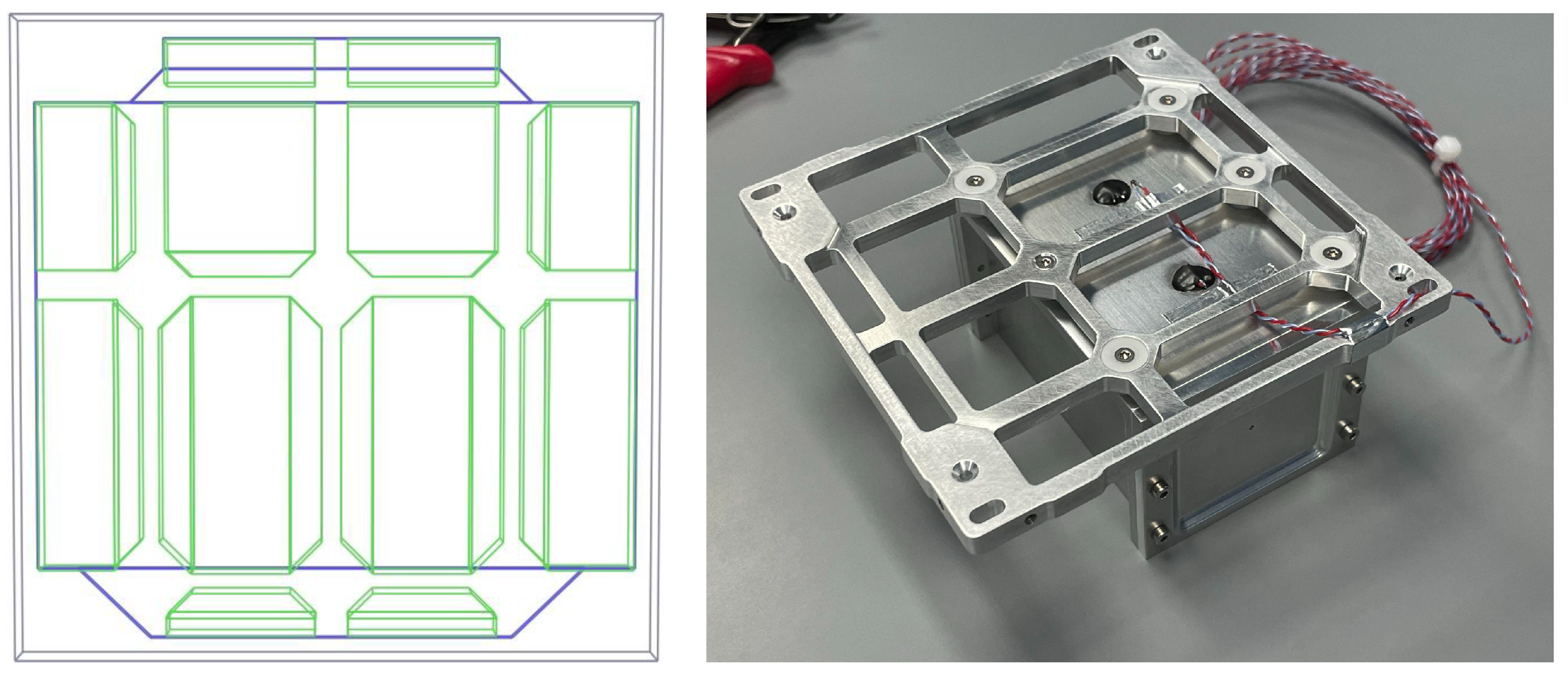
7.2. EIRSAT-1 MEGAlib Model
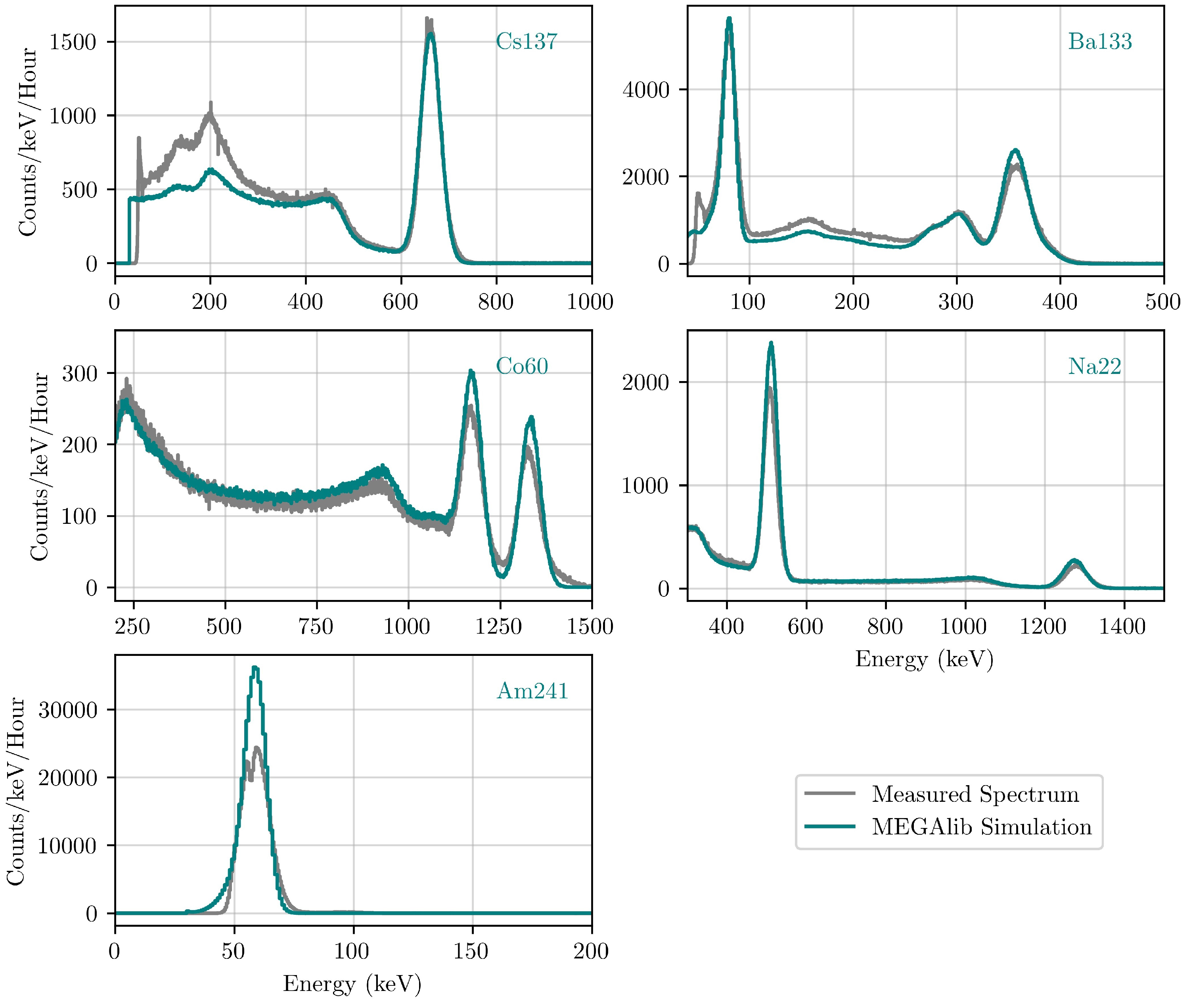
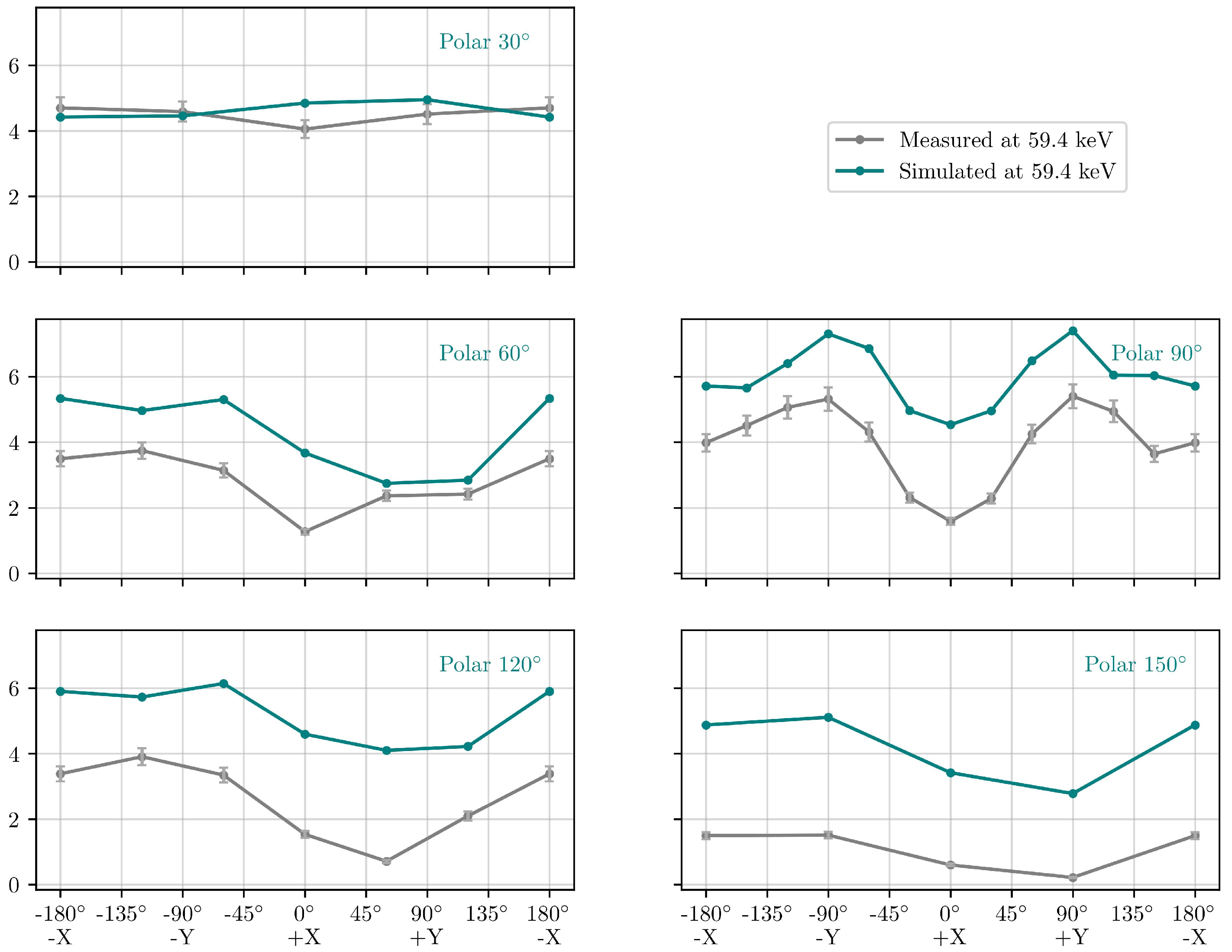
7.3. Simulations and Comparison with Physical Measurements
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Vedrenne, G.; Atteia, J.L. Gamma-ray Bursts: The Brightest Explosions in the Universe. Phys. Today 2010, 63, 56–57. [Google Scholar] [CrossRef]
- Luongo, O.; Muccino, M. A Roadmap to Gamma-ray Bursts: New Developments and Applications to Cosmology. Galaxies 2021, 9, 77. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Cahillane, C.; et al. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef] [PubMed]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. Gravitational Waves and Gamma-rays from a Binary Neutron Star Merger: GW170817 and GRB 170817A. Astrophys. J. 2017, 848, L13. [Google Scholar] [CrossRef]
- Meegan, C.; Lichti, G.; Bhat, P.N.; Bissaldi, E.; Briggs, M.S.; Connaughton, V.; Diehl, R.; Fishman, G.; Greiner, J.; Hoover, A.S.; et al. The Fermi Gamma-ray Burst Monitor. Astrophys. J. 2009, 702, 791–804. [Google Scholar] [CrossRef]
- Atwood, W.B.; Abdo, A.A.; Ackermann, M.; Althouse, W.; Anderson, B.; Axelsson, M.; Baldini, L.; Ballet, J.; Band, D.L.; Barbiellini, G.; et al. The Large Area Telescope on the Fermi Gamma-ray Space Telescope Mission. Astrophys. J. 2009, 697, 1071–1102. [Google Scholar] [CrossRef]
- Gehrels, N.; Chincarini, G.; Giommi, P.; Mason, K.O.; Nousek, J.A.; Wells, A.A.; White, N.E.; Barthelmy, S.D.; Burrows, D.N.; Cominsky, L.R.; et al. The Swift Gamma-ray Burst Mission. Astrophys. J. 2004, 611, 1005–1020. [Google Scholar] [CrossRef]
- Winkler, C.; Courvoisier, T.J.-L.; Di Cocco, G.; Gehrels, N.; Giménez, A.; Grebenev, S.; Hermsen, W.; Mas-Hesse, J.M.; Lebrun, F.; Lund, N.; et al. The INTEGRAL mission. Astron. Astrophys. 2003, 411, L1–L6. [Google Scholar] [CrossRef]
- von Kienlin, A.; Meegan, C.A.; Paciesas, W.S.; Bhat, P.N.; Bissaldi, E.; Briggs, M.S.; Burns, E.; Cleveland, W.H.; Gibby, M.H.; Giles, M.M.; et al. The Fourth Fermi-GBM Gamma-ray Burst Catalog: A Decade of Data. Astrophys. J. 2020, 893, 46. [Google Scholar] [CrossRef]
- Bailes, M.; Berger, B.K.; Brady, P.R.; Branchesi, M.; Danzmann, K.; Evans, M.; Holley-Bockelmann, K.; Iyer, B.R.; Kajita, T.; Katsa-nevas, S.; et al. Gravitational-wave physics and astronomy in the 2020s and 2030s. Nat. Rev. Phys. 3 2021, 3, 344–366. [Google Scholar] [CrossRef]
- The CubeSat Program. CubeSat Design Specification Rev. 14. California Polytechnic State University. In Technical Report CP-CDS-R14; California Polytechnic State University (Cal Poly): San Luis Obispo, CA, USA, 2020. [Google Scholar]
- Bloser, P.; Murphy, D.; Fiore, F.; Perkins, J. CubeSats for Gamma-ray Astronomy. In Handbook of X-ray and Gamma-ray Astrophysics; Springer Nature: Singapore, 2022; pp. 1–33. [Google Scholar] [CrossRef]
- Serjeant, S.; Elvis, M.; Tinetti, G. The future of astronomy with small satellites. Nat. Astron. 2020, 4, 1031–1038. [Google Scholar] [CrossRef]
- Mero, B.; Quillien, K.; McRobb, M.; Chesi, S.; Marshall, R.; Gow, A.; Clark, C.; Anciaux, M.; Cardoen, P.; Keyser, J.D.; et al. PICASSO: A State of the Art CubeSat. In Proceedings of the 29th Annual AIAA/USU Small Satellite Conference, Logan, UT, USA, 8–13 August 2015. Technical Session III: Next on the Pad: SSC15-III-2. [Google Scholar]
- Liddle, J.D.; Holt, A.P.; Jason, S.J.; O’Donnell, K.A.; Stevens, E.J. Space science with CubeSats and nanosatellites. Nat. Astron. 2020, 4, 1026–1030. [Google Scholar] [CrossRef]
- Nieto-Peroy, C.; Emami, M.R. CubeSat Mission: From Design to Operation. Appl. Sci. 2019, 9, 3110. [Google Scholar] [CrossRef]
- Twiggs, R. Origin of CubeSat. In Small Satellite: Past, Present and Future; Helvajian, H., Janson, S.W., Eds.; The Aerospace Press: El Segundo, CA, USA, 2008; Chapter 5; pp. 151–173. [Google Scholar]
- Straub, J.; Villela, T.; Costa, C.A.; Brandão, A.M.; Bueno, F.T.; Leonardi, R. Towards the Thousandth CubeSat: A Statistical Overview. Int. J. Aerosp. Eng. 2019, 2019, 5063145. [Google Scholar] [CrossRef]
- Shkolnik, E.L. On the Verge of an Astronomy CubeSat Revolution. Nat. Astron. 2018, 2, 374–378. [Google Scholar] [CrossRef]
- Engel, K.; Lewis, T.; Stein, M.; Venters, T.; Ahlers, M.; Albert, A.; Allen, A.; Solares, H.; Anandagoda, S.; Andersen, T.; et al. Advancing the Landscape of Multimessenger Science in the Next Decade. arXiv 2022, arXiv:2203.10074. [Google Scholar] [CrossRef]
- Racusin, J.; Perkins, J.S.; Briggs, M.S.; de Nolfo, G.; Krizmanic, J.; Caputo, R.; McEnery, J.E.; Shawhan, P.; Morris, D.; Connaughton, V.; et al. BurstCube: A CubeSat for Gravitational Wave Counterparts. arXiv 2017, arXiv:1708.09292. [Google Scholar] [CrossRef]
- Joens, A.; Brewer, I.; Briggs, M.; Bruno, A.; Burns, E.; Caputo, R.; Cenko, B.; de Nolfo, G.; Goldstein, A.; Griffin, S.; et al. BurstCube: A CubeSat for gravitational wave counterparts. In Proceedings of the Space Telescopes and Instrumentation 2022: Ultraviolet to Gamma Ray, Montréal, QC, Canada, 17–22 July 2022; Volume 12181, p. 121811N. [Google Scholar]
- Hui, C.M.; Briggs, M.S.; Goldstein, A.; Jenke, P.; Kocevski, D.; Wilson-Hodge, C.A. MoonBEAM: A Beyond-LEO Gamma-ray Burst Detector for Gravitational-Wave Astronomy. Deep Space Gatew. Concept Sci. Workshop 2018, 2063, 3060. [Google Scholar]
- Fuschino, F.; Campana, R.; Labanti, C.; Evangelista, Y.; Feroci, M.; Burderi, L.; Fiore, F.; Ambrosino, F.; Baldazzi, G.; Bellutti, P.; et al. HERMES: An ultra-wide band X and gamma-ray transient monitor on board a nano-satellite constellation. Nucl. Instruments Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2019, 936, 199–203. [Google Scholar] [CrossRef]
- Pál, A.; Ohno, M.; Mészáros, L.; Werner, N.; Ripa, J.; Frajt, M.; Hirade, N.; Hudec, J. GRBAlpha: A 1U CubeSat mission for validating timing-based gamma-ray burst localization. In Proceedings of the Space Telescopes and Instrumentation 2020: Ultraviolet to Gamma Ray, Online, 14–18 December 2020; Volume 11444, pp. 825–833. [Google Scholar] [CrossRef]
- Werner, N.; Řípa, J.; Pál, A.; Ohno, M.; Tarcai, N.; Torigoe, K.; Tanaka, K.; Uchida, N.; Mészáros, L.; Galgóczi, G.; et al. CAMELOT: Cubesats Applied for MEasuring and LOcalising Transients mission overview. In Proceedings of the Space Telescopes and Instrumentation 2018: Ultraviolet to Gamma Ray, Austin, TX, USA, 10–15 June 2018; Volume 10699, pp. 672–686. [Google Scholar] [CrossRef]
- David, M.; Flanagan, J.; Joseph, W.T.; Maeve, D.; Jessica, E.; Andrew, G.; Conor, O.; Lana, S.; Daire, S.; Sarah, W.; et al. EIRSAT-1—The Educational Irish Research Satellite. In Proceedings of the 2nd Symposium on Space Educational Activities, Barcelona, Spain, 27–28 April 2018; pp. 201–205. [Google Scholar]
- Maeve, D.; David, M.; Jack, R.; Joseph, T.; Sarah, W.; Reddy, A.S.K.; Rachel, D.; Jessica, E.; Gabriel, F.; Joseph, M.; et al. Update on the status of the Educational Irish Research Satellite (EIRSAT-1). In Proceedings of the 4th Symposium on Space Educational Activities, Barcelona, Spain, 27–29 April 2022; pp. 294–299. [Google Scholar] [CrossRef]
- Murphy, D.; Ulyanov, A.; McBreen, S.; Doyle, M.; Dunwoody, R.; Mangan, J.; Thompson, J.; Shortt, B.; Martin-Carrillo, A.; Hanlon, L. A compact instrument for gamma-ray burst detection on a Cubesat platform I: Design drivers and expected performance. Exp. Astron. 2021, 52, 59–84. [Google Scholar] [CrossRef]
- Murphy, D.; Ulyanov, A.; McBreen, S.; Mangan, J.; Dunwoody, R.; Doyle, M.; O’Toole, C.; Thompson, J.; Reilly, J.; Walsh, S.; et al. A compact instrument for gamma-ray burst detection on a CubeSat platform II. Exp. Astron. 2022, 53, 961–990. [Google Scholar] [CrossRef] [PubMed]
- Dunwoody, R.; Doyle, M.; Murphy, D.; Finneran, G.; O’Callaghan, D.; Reilly, J.; Thompson, J.; Akarapu, S.K.R.; de Barra, C.; Cotter, L.; et al. Development, description, and validation of the operations manual for EIRSAT-1, a 2U CubeSat with a gamma-ray burst detector. J. Astron. Telesc. Instruments Syst. 2023, 9, 037001. [Google Scholar] [CrossRef]
- Dirk, M.; Jörg, A.; Alf, O.; Hans, K.; Hans, B.; Amir, H.; Akif, A.; Suleyman, A.; Bahram, N.; Jahanzad, T.; et al. SIPHRA 16-Channel Silicon Photomultiplier Readout ASIC. In Proceedings of the 6th International Workshop on Analogue and Mixed-Signal Integrated Circuits for Space Applications, Gothenburg, Sweden, 12–16 June 2016. [Google Scholar] [CrossRef]
- Mangan, J.; Murphy, D.; Dunwoody, R.; Doyle, M.; Ulyanov, A.; Hanlon, L.; Shortt, B.; McBreen, S. Embedded Firmware Development for a Novel CubeSat Gamma-ray Detector. In Proceedings of the 2021 IEEE 8th International Conference on Space Mission Challenges for Information Technology (SMC-IT), Pasadena, CA, USA, 26–30 July 2021; pp. 14–22. [Google Scholar] [CrossRef]
- Joseph, M.; David, M.; Rachel, D.; Maeve, D.; Alexey, U.; Mike, H.; Reddy, A.S.K.; Jessica, E.; Gabriel, F.; Fergal, M.; et al. Experiences in firmware development for a CubeSat instrument payload. In Proceedings of the 4th Symposium on Space Educational Activities 2022, Barcelona, Spain, 27–29 April 2022. [Google Scholar] [CrossRef]
- Ulyanov, A.; Morris, O.; Hanlon, L.; McBreen, S.; Foley, S.; Roberts, O.J.; Tobin, I.; Murphy, D.; Wade, C.; Nelms, N.; et al. Performance of a monolithic LaBr3:Ce crystal coupled to an array of silicon photomultipliers. Nucl. Instruments Methods Phys. Res. A 2016, 810, 107–119. [Google Scholar] [CrossRef]
- Ulyanov, A.; Morris, O.; Roberts, O.J.; Tobin, I.; Hanlon, L.; McBreen, S.; Murphy, D.; Nelms, N.; Shortt, B. Localisation of gamma-ray interaction points in thick monolithic CeBr3 and LaBr3:Ce scintillators. Nucl. Instruments Methods Phys. Res. A 2017, 844, 81–89. [Google Scholar] [CrossRef]
- Murphy, D.; Mangan, J.; Ulyanov, A.; Walsh, S.; Dunwoody, R.; Hanlon, L.; Shortt, B.; McBreen, S. Balloon flight test of a CeBr3 detector with silicon photomultiplier readout. Exp. Astron. 2021, 52, 1–34. [Google Scholar] [CrossRef] [PubMed]
- Bissaldi, E.; von Kienlin, A.; Lichti, G.; Steinle, H.; Bhat, P.N.; Briggs, M.S.; Fishman, G.J.; Hoover, A.S.; Kippen, R.M.; Krumrey, M.; et al. Ground-based calibration and characterization of the Fermi gamma-ray burst monitor detectors. Exp. Astron. 2009, 24, 47–88. [Google Scholar] [CrossRef]
- Bandyopadhyay, R.M.; Silk, J.; Taylor, J.E.; Maccarone, T.J. On the origin of the 511-keV emission in the Galactic Centre. Mon. Not. R. Astron. Soc. 2009, 392, 1115–1123. [Google Scholar] [CrossRef]
- Ramaty, R.; Lingenfelter, R.E. Gamma-ray Lines from Solar Flares. In Solar Gamma-, X-, and EUV Radiation; Kane, S.R., Ed.; Springer: Amsterdam, The Netherlands, 1975; Volume 68, p. 363. [Google Scholar]
- Tierney, D.; McBreen, S.; Hanlon, L.; Foley, S.; Martin-Carrillo, A.; Topinka, M.; Meehan, S. Spectral Cross-Calibration of Fermi-GBM and INTEGRAL-ISGRI using Gamma-ray Bursts. In Proceedings of the Gamma Ray Bursts 2010, Annapolis, MD, USA, 1–4 November 2010; Volume 1358, pp. 397–400. [Google Scholar] [CrossRef]
- Doyle, M.; Gloster, A.; Griffin, M.; Hibbett, M.; Kyle, J.; O’Toole, C.; Mangan, J.; Murphy, D.; Wong, N.L.; Akarapu, S.K.R.; et al. Design, development, and testing of flight software for EIRSAT-1: A university-class CubeSat enabling astronomical research. In Proceedings of the Software and Cyberinfrastructure for Astronomy VII, Montreal, QC, Canada, 17–23 July 2022; Volume 12189, p. 1218915. [Google Scholar] [CrossRef]
- Zoglauer, A.; Andritschke, R.; Schopper, F. MEGAlib – The Medium Energy Gamma-ray Astronomy Library. New Astron. Rev. 2006, 50, 629–632. [Google Scholar] [CrossRef]
- Be, M.-M.; Chiste, V.; Dulieu, C.; Kellett, M.A.; Mougeot, X.; Arinc, A.; Chechev, V.P.; Kuzmenko, N.K.; Kibedi, T.; Luca, A.; et al. LNE-LNHB/CEA Table de Radionucleides. 2016. Bureau International des Poids et Mesures, Pavillon de Breteuil, F-92310 Sevres, France. Available online: http://www.bipm.org/utils/common/pdf/monographieRI/Monographie_BIPM-5_Tables_Vol8.pdf (accessed on 9 July 2024).
- Bright Ascension Ltd. User Manual—COAST MCS, BAL-COAST-MCS-UM-21.2. 2021. Available online: https://brightascension.com/portal/knowledge-base/mcs-user-manual/ (accessed on 23 June 2023).
- Knoll, G. Radiation Detection and Measurement; Wiley: Hoboken, NJ, USA, 2010. [Google Scholar]
- Andritschke, R.; Zoglauer, A.; Kanbach, G.; Bloser, P.F.; Schopper, F. The Compton and pair creation telescope MEGA. Exp. Astron. 2005, 20, 395–403. [Google Scholar] [CrossRef]
- Zoglauer, A.; Weidenspointner, G.; Galloway, M.; Boggs, S.E.; Wunderer Cosima, C.B. The cosmic simulator of MEGAlib. In Proceedings of the 2009 IEEE Nuclear Science Symposium Conference Record (NSS/MIC), Orlando, FL, USA, 24 October–1 November 2009; pp. 2053–2059. [Google Scholar] [CrossRef]
- Agostinelli, S.; Allison, J.; Amako, K.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.; Axen, D.; Banerjee, S.; Barrand, G.; et al. GEANT4—A simulation toolkit. Nucl. Instrum. Meth. A 2003, 506, 250–303. [Google Scholar] [CrossRef]
- Livermore Low-Energy Package (2023). Available online: https://twiki.cern.ch/twiki/bin/view/Geant4/LoweMigratedLivermore (accessed on 20 June 2023).










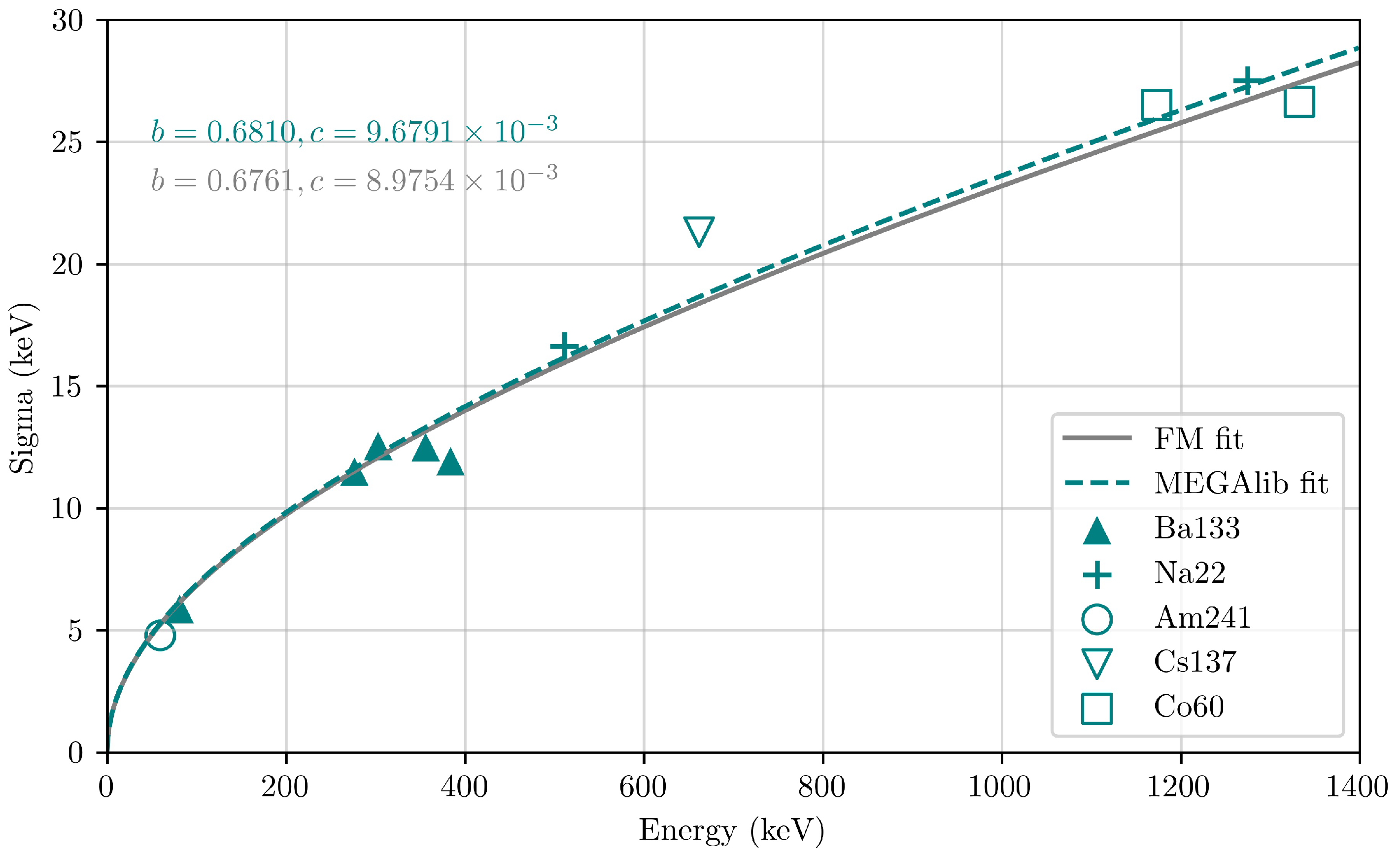
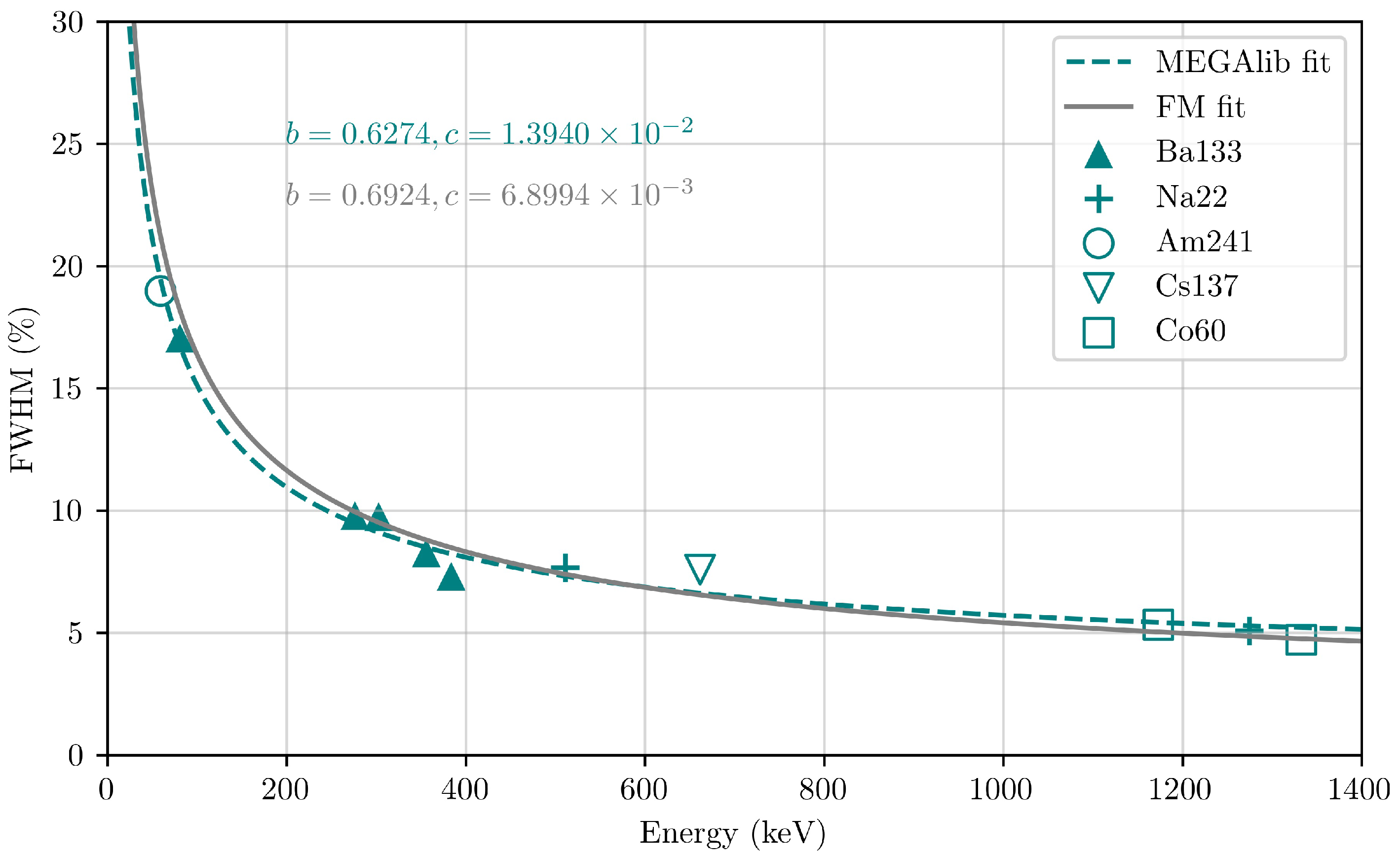
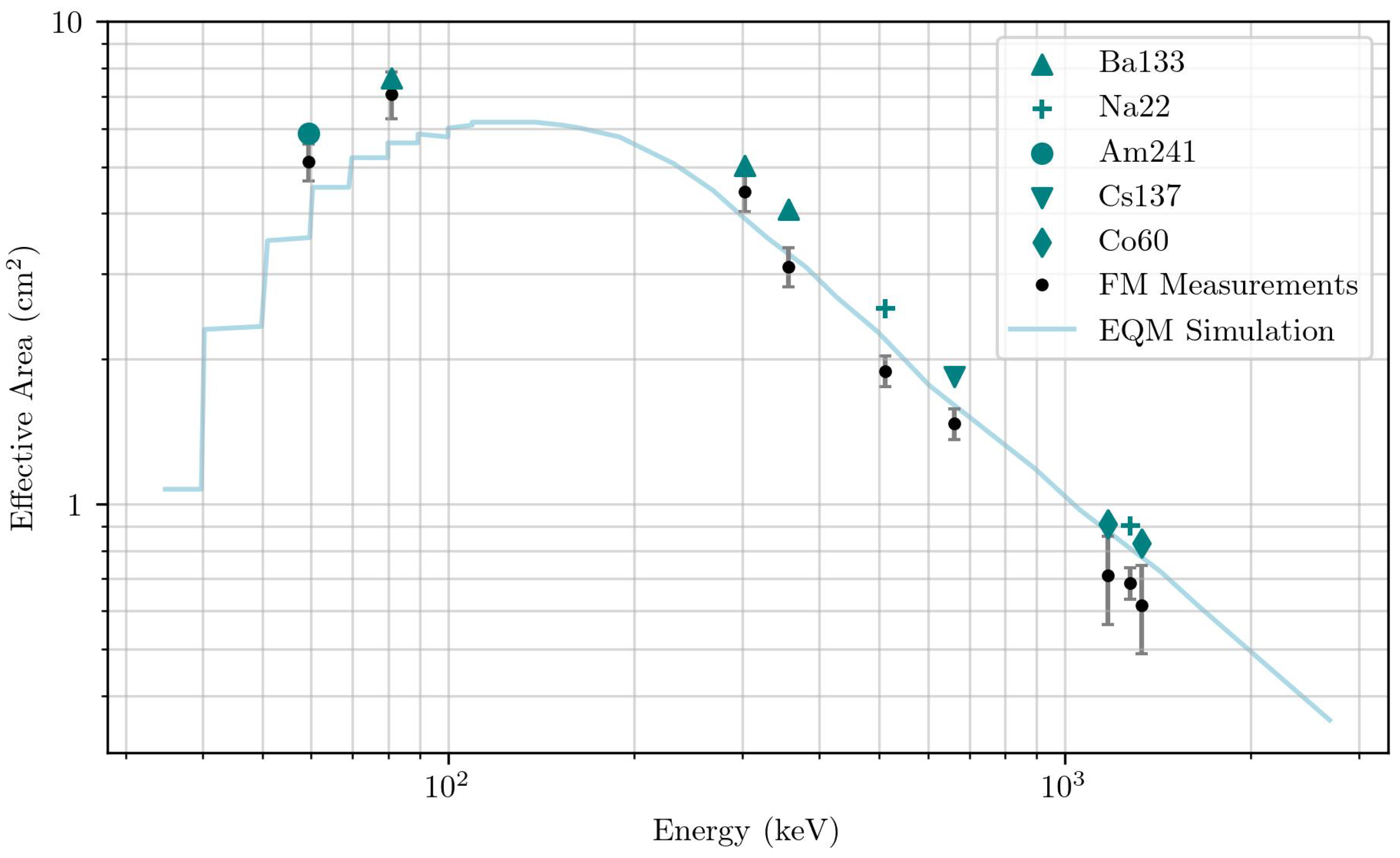
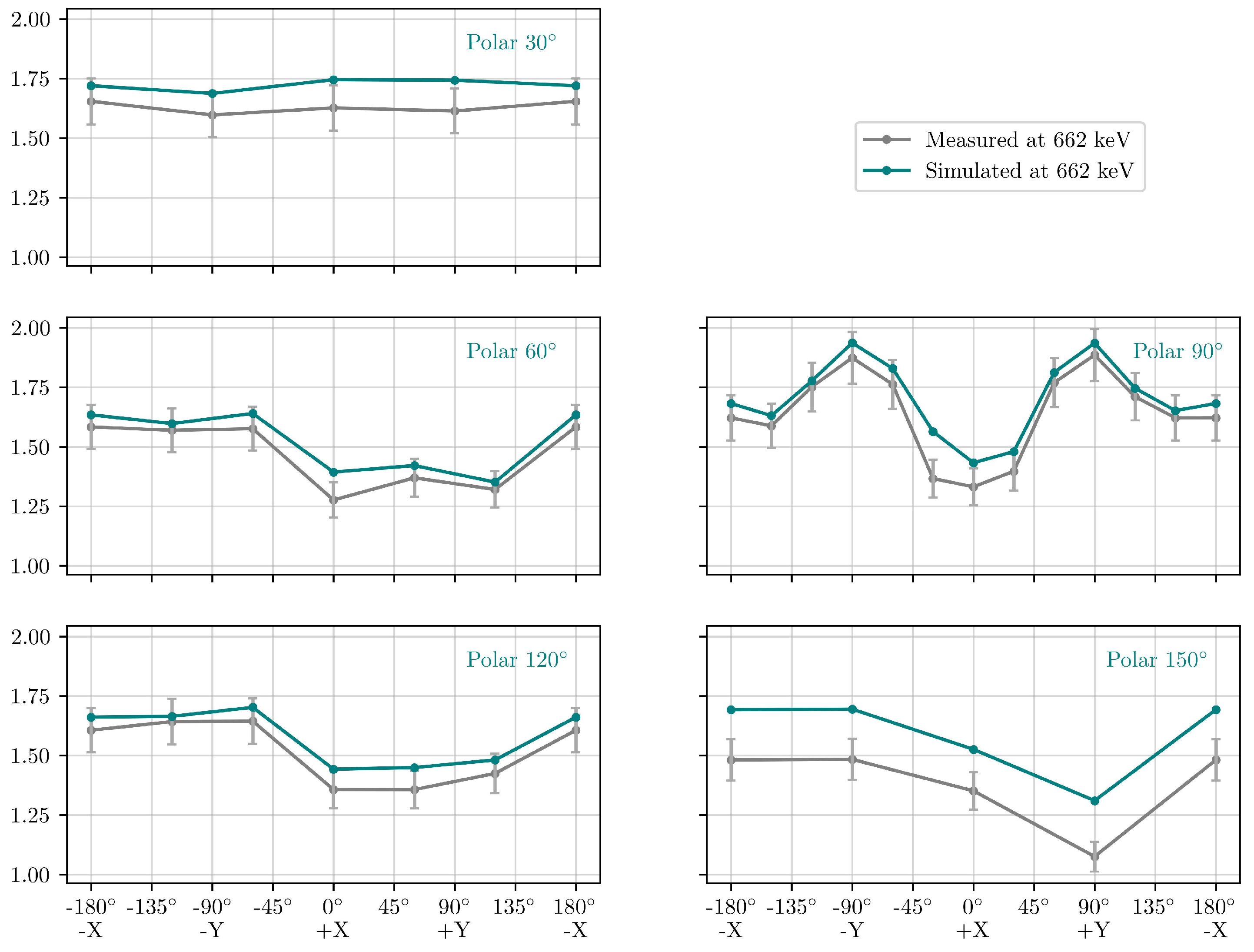
| Source No. | Source Name | Nuclide | Line Origin | Line Energy (keV) | Transition Probability | Half-Life (Years) | Activity (µCi) |
|---|---|---|---|---|---|---|---|
| 1 | Americium | 241Am | 59.40 * | 0.359 * | 432.2 | 11.16 | |
| 2 | Cobalt | 60Co | 1173.23 * | 0.998 * | 5.26 | 12.28 | |
| 60Co | 1332.49 * | 0.999 * | 5.26 | 12.28 | |||
| 3 | Barium | 133Ba | 80.99 † | 0.901 † | 10.5 | 11.42 | |
| 133Ba | 276.39 † | 0.075 † | 10.5 | 11.42 | |||
| 133Ba | 302.85 † | 0.191 † | 10.5 | 11.42 | |||
| 133Ba | 356.01 † | 0.636 † | 10.5 | 11.42 | |||
| 133Ba | 383.85 † | 0.091 † | 10.5 | 11.42 | |||
| 4 | Mixed Source | 241Am | 59.40 * | 0.359 * | 432.2 | - | |
| 137Cs | Ba-137m | 661.66 * | 0.850 * | 30.17 | - | ||
| 60Co | 1173.23 * | 0.998 * | 5.26 | - | |||
| 60Co | 1332.49 * | 0.999 * | 5.26 | - | |||
| 5 | Sodium | 22Na | annihilation | 511 * | 1.798 * | 2.6 | 10 |
| 22Na | 1274.54 * | 0.999 * | 2.6 | 10 | |||
| 6 | Caesium | 137Cs | Ba-137m | 661.66 * | 0.850 * | 30.17 | 11.16 |
| Source No. | Source Name | Location with Respect to Centre of EIRSAT-1 (mm) | Location Relative to CoC (mm) | Int. (Hours) | ||||
|---|---|---|---|---|---|---|---|---|
| X | Y | Z | X | Y | Z | |||
| 1 | Americium | −10.57 | −14.50 | 298.5 | 0.00 | 0.00 | 234.6 | 12 |
| 2 | Cobalt | −10.57 | −14.50 | 113.5 | 0.00 | 0.00 | 49.6 | 12 |
| 3 | Barium | −10.57 | −14.50 | 216.5 | 0.00 | 0.00 | 152.6 | 12 |
| 4 | Mixed Source | −10.57 | −14.50 | 113.5 | 0.00 | 0.00 | 49.6 | 12 |
| 5 | Sodium | −10.57 | −14.50 | 252.5 | 0.00 | 0.00 | 188.6 | 12 |
| 6 | Cesium | −10.57 | 272.0 | 5.50 | 0.00 | −286.5 | −58.4 | 12 |
| Polar Angle (°) | Azimuthal Angle (°) | Distance to Centre of Spacecraft (mm) | Integration (s) | ||
|---|---|---|---|---|---|
| X | Y | Z | |||
| 0 | 0 | −1.057 | −1.450 | 28.890 | 3112.56 |
| 30 | 0 | 10.193 | −1.450 | 25.876 | 8815.00 |
| 90 | −1.057 | 9.800 | 25.876 | 6226.38 | |
| 180 | −12.307 | −1.450 | 25.876 | 6137.81 | |
| 270 | −1.057 | −12.700 | 25.876 | 6201.48 | |
| 60 | 0 | 18.429 | −1.450 | 17.640 | 9337.74 |
| 60 | 8.686 | 15.425 | 17.640 | 7057.31 | |
| 120 | −10.799 | 15.425 | 17.640 | 6720.92 | |
| 180 | −20.543 | −1.450 | 17.640 | 6895.48 | |
| 240 | −10.799 | −18.325 | 17.640 | 8116.63 | |
| 300 | 8.686 | −18.325 | 17.640 | 8021.03 | |
| 90 | 0 | 21.443 | −1.450 | 6.390 | 12,597.20 |
| 30 | 18.429 | 9.800 | 6.390 | 8079.04 | |
| 60 | 10.193 | 18.036 | 6.390 | 6063.91 | |
| 90 | −1.057 | 21.050 | 6.390 | 5528.77 | |
| 120 | −12.307 | 18.036 | 6.390 | 5759.64 | |
| 150 | −20.543 | 9.800 | 6.390 | 6256.82 | |
| 180 | −23.557 | −1.450 | 6.390 | 6549.98 | |
| 210 | −20.543 | −12.700 | 6.390 | 6635.26 | |
| 240 | −12.307 | −20.936 | 6.390 | 5773.07 | |
| 270 | −1.057 | −23.950 | 6.390 | 5364.51 | |
| 300 | 10.193 | −20.936 | 6.390 | 5981.63 | |
| 330 | 18.429 | −12.700 | 6.390 | 7814.70 | |
| 120 | 0 | 18.429 | −1.450 | −4.860 | 8490.85 |
| 60 | 8.686 | 15.4250 | −4.860 | 6611.60 | |
| 120 | −10.799 | 15.425 | −4.860 | 8862.90 | |
| 180 | −20.543 | −1.450 | −4.860 | 6699.69 | |
| 240 | −10.799 | −18.325 | −4.860 | 7760.87 | |
| 300 | 8.686 | −18.325 | −4.860 | 9409.55 | |
| 150 | 0 | 10.193 | −1.450 | −13.096 | 13,941.38 |
| 90 | −1.057 | 9.800 | −13.096 | 15,991.81 | |
| 180 | −12.307 | −1.450 | −13.096 | 3324.10 | |
| 270 | −1.057 | −12.700 | −13.096 | 9837.67 | |
| 180 | 0 | −1.0570 | −1.450 | −16.110 | 50,397.94 |
| Polar Angle | Peak Energy (keV) | Measured (cm2) | Simulated (cm2) |
|---|---|---|---|
| 0 | 662 | 1.72 | 1.86 |
| 59.4 | 5.23 | 5.33 | |
| 180 | 662 | 0.73 | 1.15 |
| 59.4 | 0.05 | 0.74 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dunwoody, R.; Murphy, D.; Uliyanov, A.; Mangan, J.; Doyle, M.; Thompson, J.; de Barra, C.; Hanlon, L.; McKeown, D.; Shortt, B.; et al. Ground-Based Characterisation of a Compact Instrument for Gamma-ray Burst Detection on a CubeSat Platform. Aerospace 2024, 11, 578. https://doi.org/10.3390/aerospace11070578
Dunwoody R, Murphy D, Uliyanov A, Mangan J, Doyle M, Thompson J, de Barra C, Hanlon L, McKeown D, Shortt B, et al. Ground-Based Characterisation of a Compact Instrument for Gamma-ray Burst Detection on a CubeSat Platform. Aerospace. 2024; 11(7):578. https://doi.org/10.3390/aerospace11070578
Chicago/Turabian StyleDunwoody, Rachel, David Murphy, Alexey Uliyanov, Joseph Mangan, Maeve Doyle, Joseph Thompson, Cuan de Barra, Lorraine Hanlon, David McKeown, Brian Shortt, and et al. 2024. "Ground-Based Characterisation of a Compact Instrument for Gamma-ray Burst Detection on a CubeSat Platform" Aerospace 11, no. 7: 578. https://doi.org/10.3390/aerospace11070578
APA StyleDunwoody, R., Murphy, D., Uliyanov, A., Mangan, J., Doyle, M., Thompson, J., de Barra, C., Hanlon, L., McKeown, D., Shortt, B., & McBreen, S. (2024). Ground-Based Characterisation of a Compact Instrument for Gamma-ray Burst Detection on a CubeSat Platform. Aerospace, 11(7), 578. https://doi.org/10.3390/aerospace11070578






