Seasonal Variations in Lunar-Assisted GEO Transfer Capability for Southward Launch
Abstract
1. Introduction
2. Problem Description
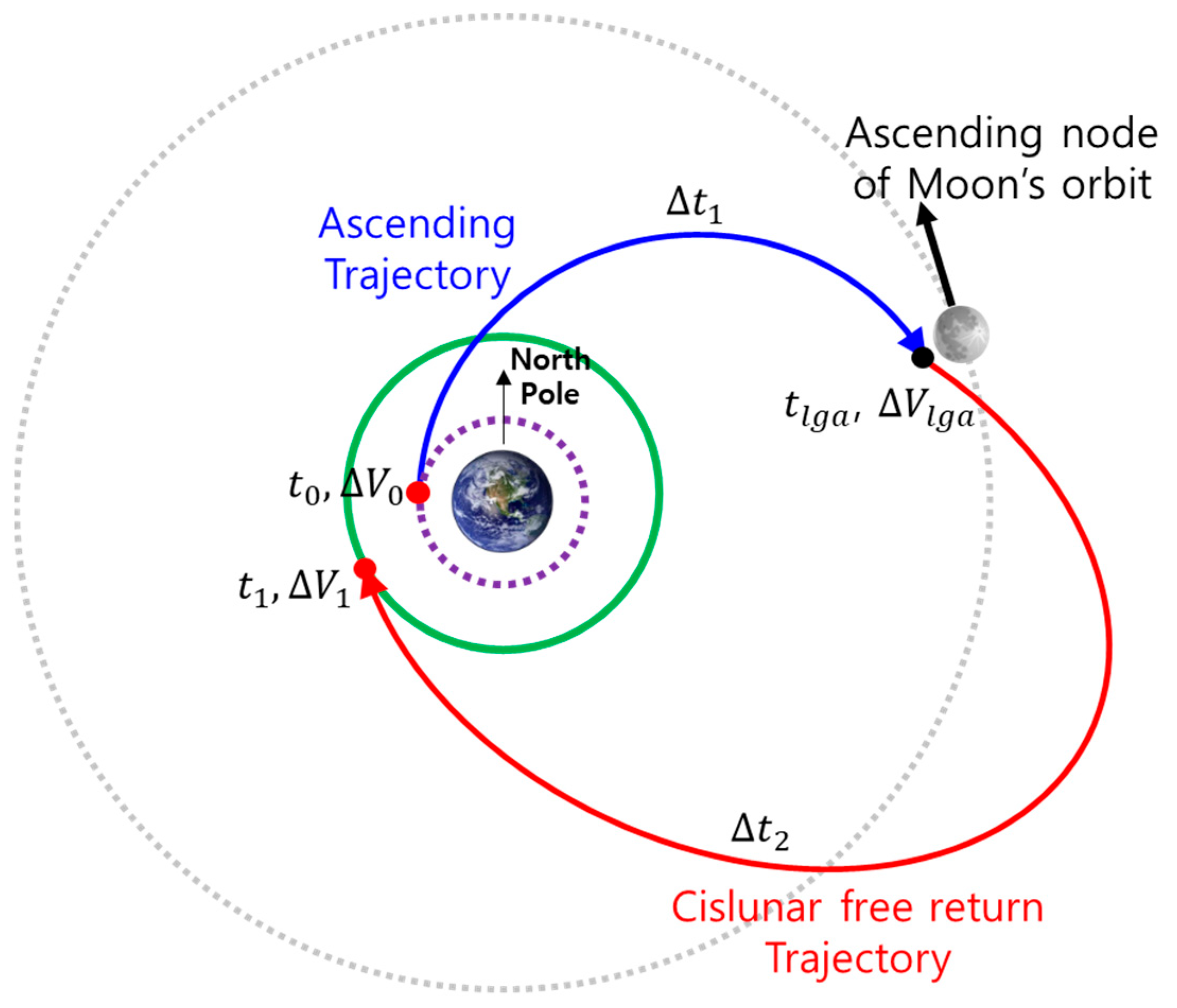
2.1. Possible Paths
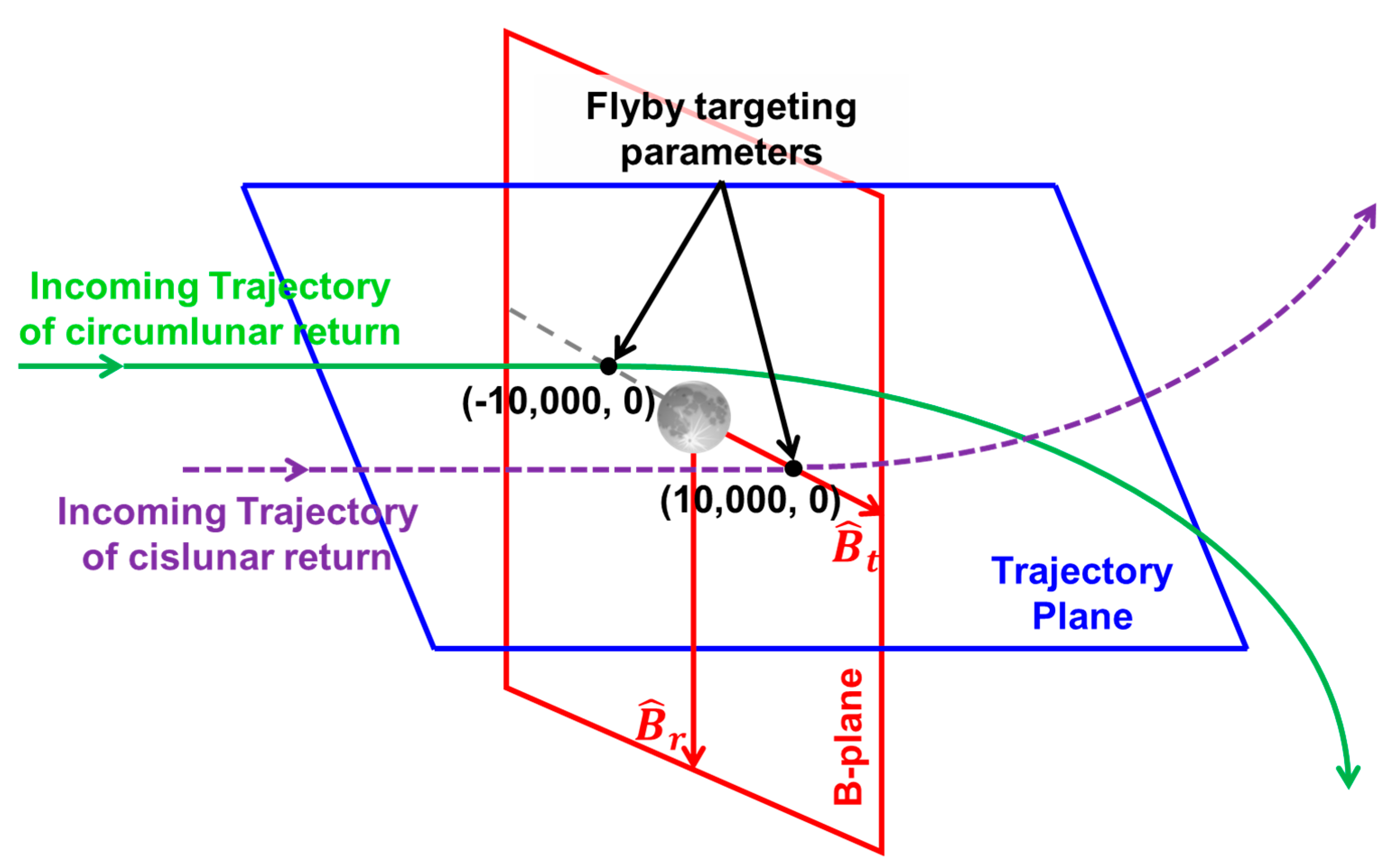
2.2. B-Plane
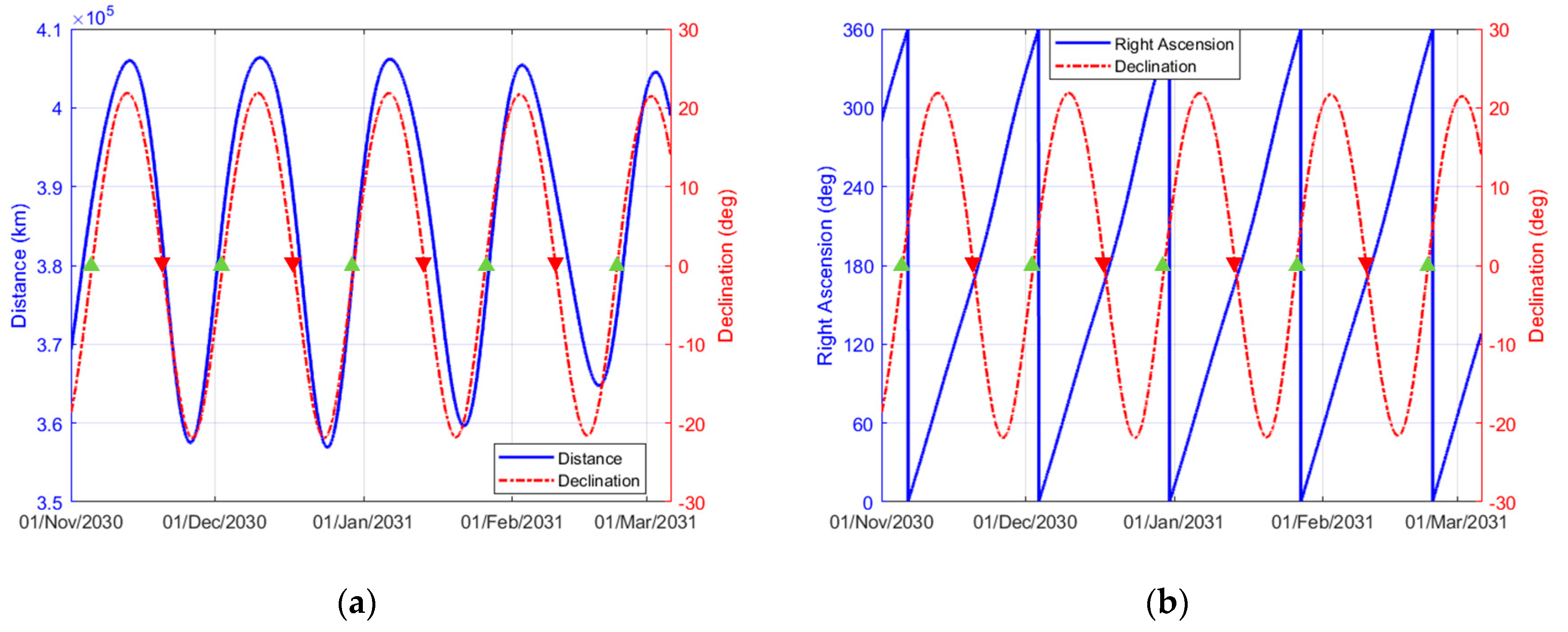
2.3. Moon’s Position and Necessary Condition for LGA
3. Dynamic Model and Numerical Search Method
3.1. Dynamic and Propagation Model
3.2. Mission Scenario and Initial Conditions (I.Cs)
3.3. Numerical Search Model
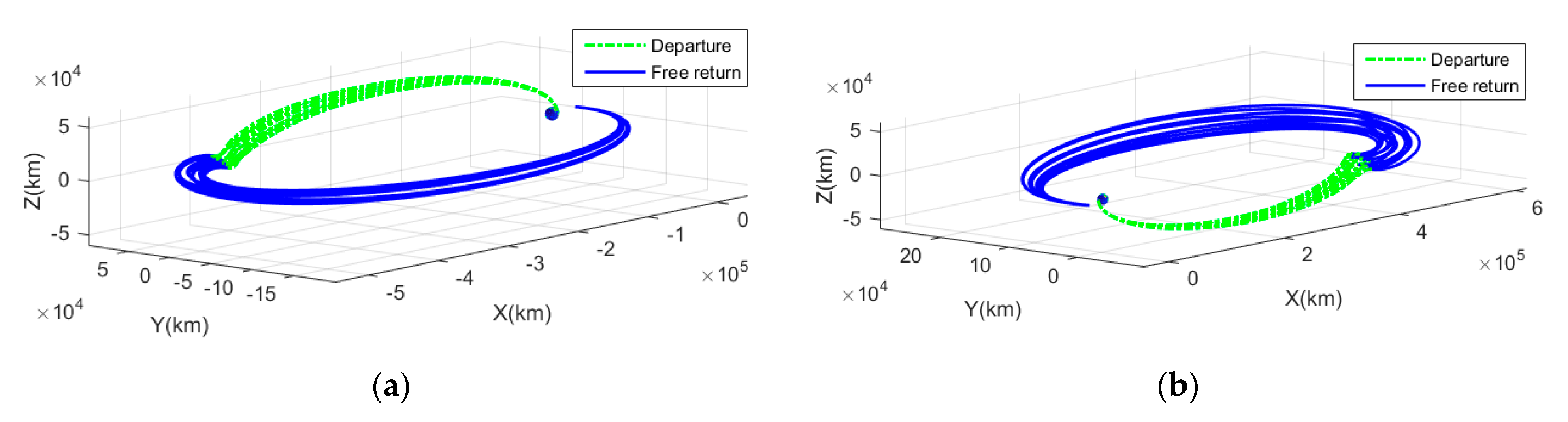
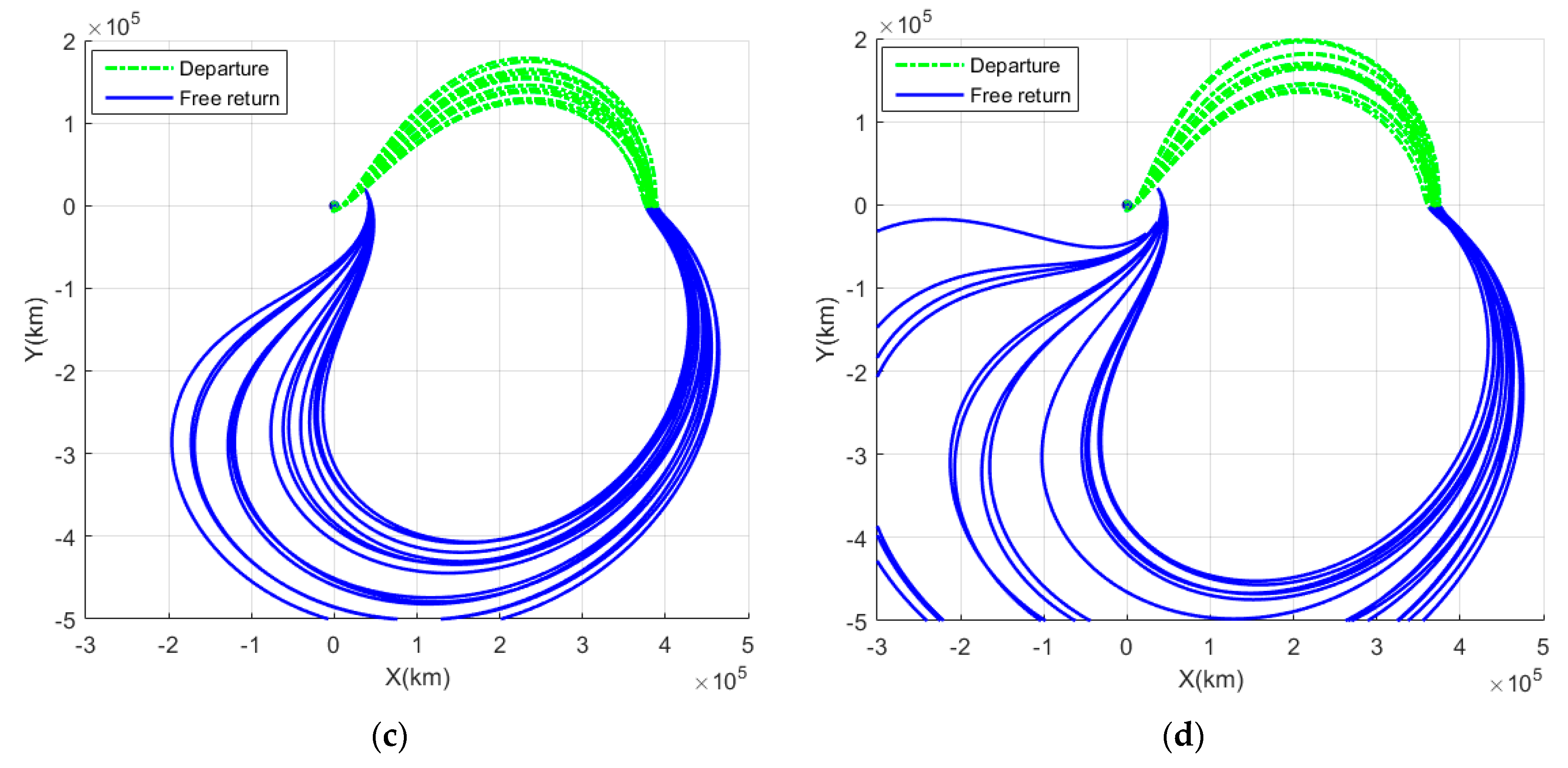
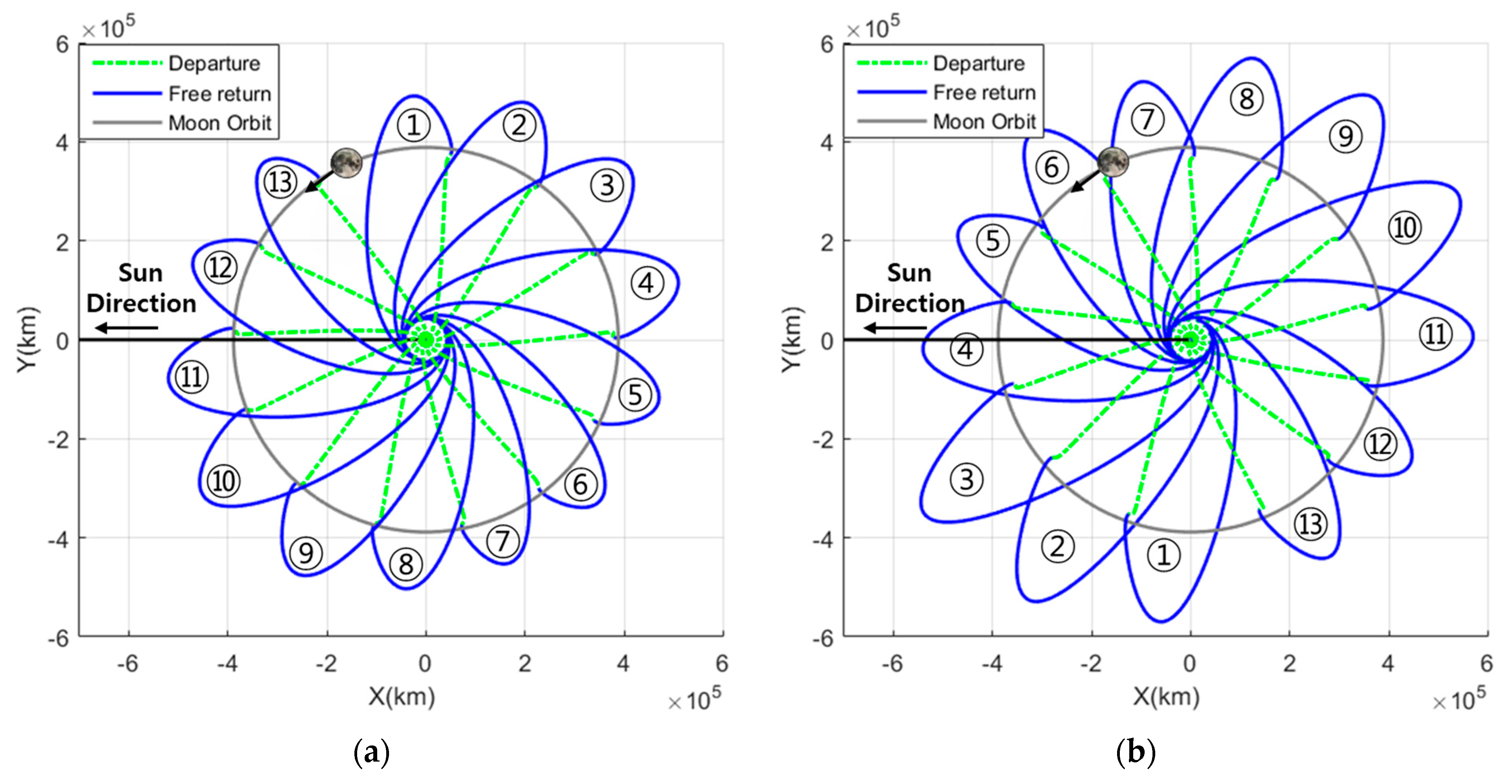
4. Simulation Results
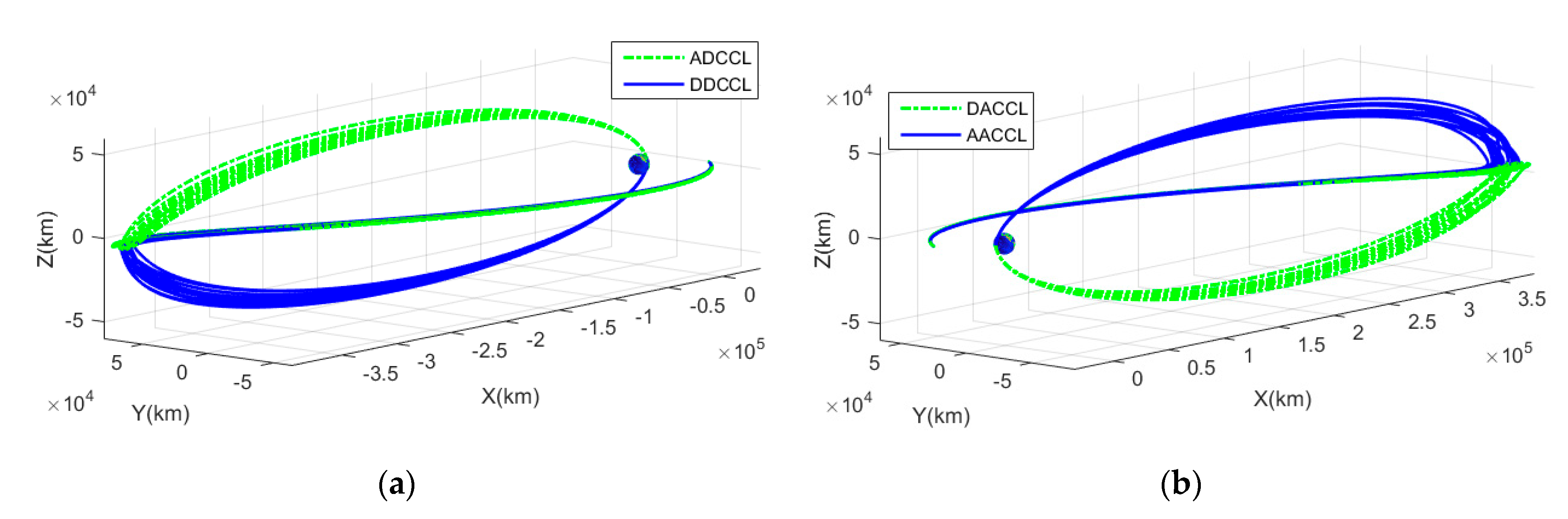
4.1. Trajectory Overview
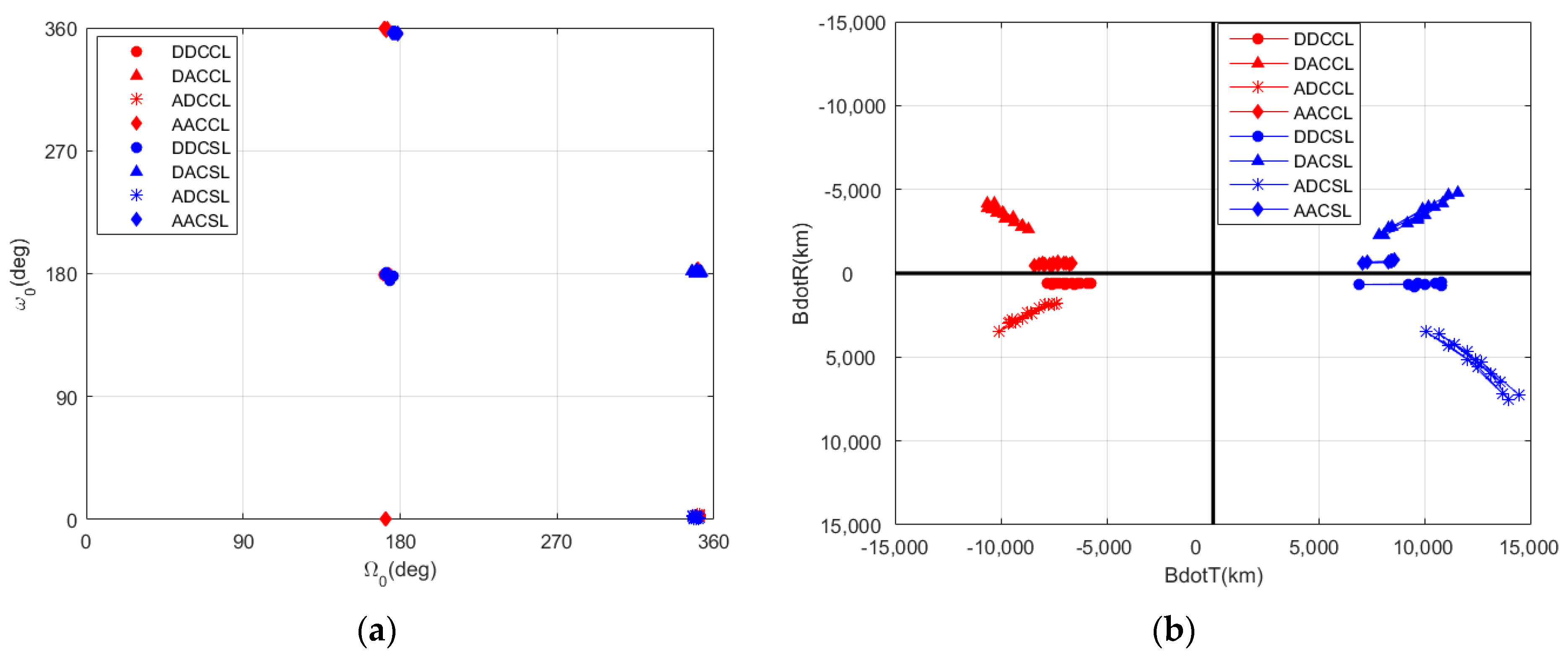
4.2. Converged Geometry
- for AACCL, [170.5°, 172.5°], [357.9°, 360.4°]
- for AACSL, [175.2°, 179.0°], [355.6°, 356.6°]
- for DDCCL, [170.5°, 172.5°], [178.3°, 180.9°]
- for DDCSL, [171.5°, 175.5°], [174.6°, 180.5°]
- for ADCCL, [350.0°, 352.0°], [1.0°, 3.6°]
- for ADCSL, [347.5°, 351.5°], [0.0°, 3.1°]
- for DACCL, [349.5°, 351.2°], [180.6°, 183.4°]
- for DACSL, [347.9°, 353.0°], [180.0°, 182.7°]
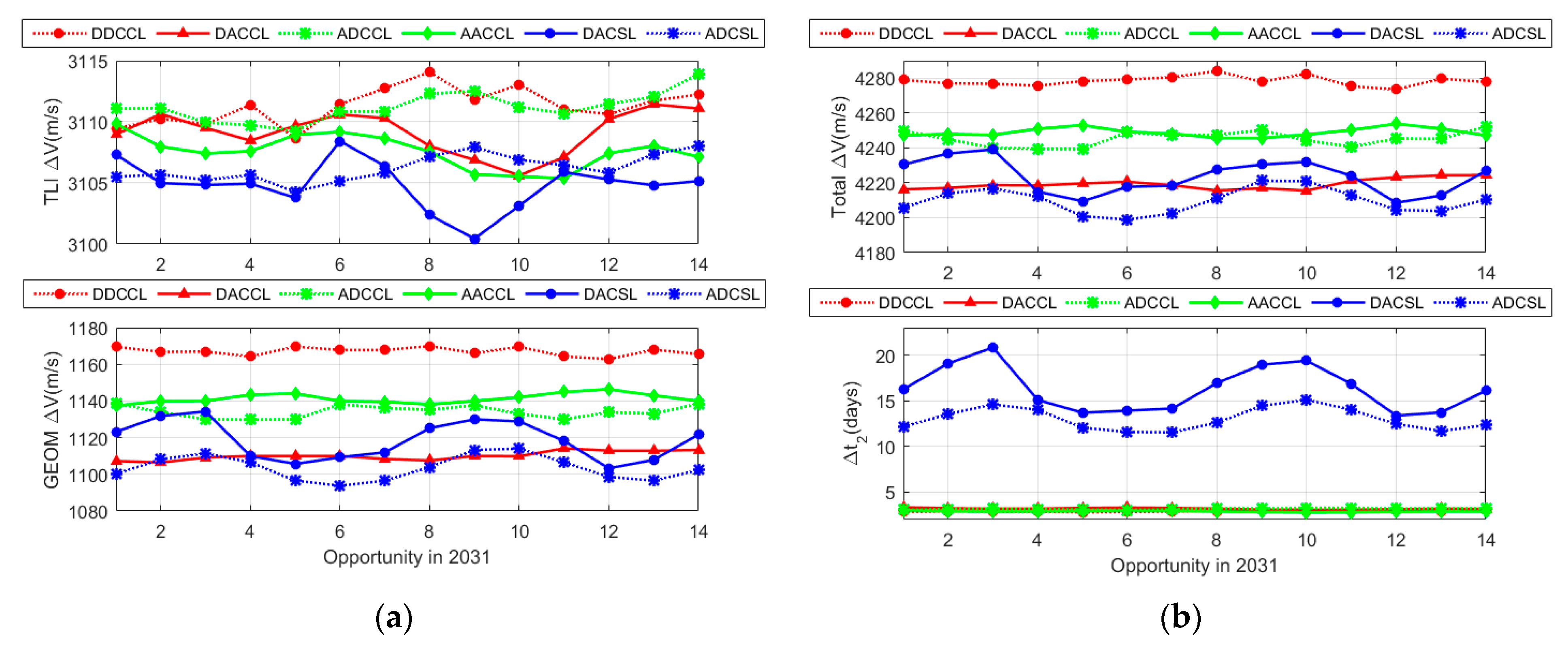
4.3. ΔV and
- for DDCCL, [4.31, 5.24], [2.80, 2.98]
- for DACCL, [4.31, 5.20], [3.08, 3.34]
- for ADCCL, [4.28, 5.35], [3.05, 3.25]
- for AACCL, [4.26, 5.20], [2.79, 3.01]
- for ADCSL, [4.22, 5.31], [11.55, 15.12]
- for DACSL, [3.97, 5.28], [13.38, 20.83]
4.4. Analysis of Seasonal Factors
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| No. | (UTC) | (m/s) | (UTC) | (m/s) | (UTC) | (m/s) |
|---|---|---|---|---|---|---|
| 1 | 2031-04-01 10:56:33 | 3104.73 | 2031-04-06 00:48:27 | 1128.24 | 2031-04-25 14:18:52 | 4232.97 |
| 2 | 2031-04-28 09:04:54 | 3105.50 | 2031-05-03 09:33:04 | 1119.24 | 2031-05-20 00:04:52 | 4224.74 |
| 3 | 2031-05-24 07:19:55 | 3111.06 | 2031-05-30 17:36:16 | 1127.16 | 2031-06-17 12:01:10 | 4238.22 |
| 4 | 2031-06-22 05:10:11 | 3107.85 | 2031-06-26 21:23:02 | 1133.16 | 2031-07-15 18:14:41 | 4241.01 |
| 5 | 2031-10-09 22:28:58 | 3106.66 | 2031-10-14 08:23:44 | 1128.13 | 2031-11-02 23:33:25 | 4234.79 |
| 6 | 2031-11-05 20:34:32 | 3106.75 | 2031-11-10 15:59:50 | 1119.80 | 2031-11-27 06:39:31 | 4226.55 |
| 7 | 2031-12-02 18:38:18 | 3108.42 | 2031-12-07 22:37:28 | 1124.91 | 2031-12-24 22:02:37 | 4233.33 |
| 8 | 2031-12-30 16:35:13 | 3110.13 | 2032-01-04 02:05:38 | 1142.91 | 2032-01-25 10:59:48 | 4253.04 |
| No. | (UTC) | (m/s) | (UTC) | (m/s) | (UTC) | |
|---|---|---|---|---|---|---|
| 1 | 2031-04-14 09:34:40 | 3105.04 | 2031-04-19 04:37:12 | 1137.45 | 2031-05-11 08:33:31 | 4242.50 |
| 2 | 2031-05-11 07:52:28 | 3105.51 | 2031-05-16 07:32:05 | 1134.80 | 2031-06-05 14:15:37 | 4240.31 |
| 3 | 2031-06-07 05:43:38 | 3106.07 | 2031-06-12 09:43:42 | 1147.32 | 2031-07-05 05:14:03 | 4253.38 |
| 4 | 2031-10-22 21:02:37 | 3103.24 | 2031-10-27 12:35:10 | 1133.56 | 2031-11-17 12:47:03 | 4236.80 |
| 5 | 2031-11-18 19:05:01 | 3104.38 | 2031-11-23 15:42:32 | 1133.56 | 2031-12-13 05:57:33 | 4237.94 |
| 6 | 2031-12-15 17:09:26 | 3104.71 | 2031-12-20 18:01:28 | 1149.38 | 2032-01-13 00:00:40 | 4254.08 |
References
- Choi, S.J.; Kang, H.; Lee, K.; Swon, S. A Pattern Search Method to Optimize Mars Exploration Trajectories. Aerospace 2023, 10, 827. [Google Scholar] [CrossRef]
- Lovell, J.B. The Effect of Space Launch Vehicle Trajectory Parameters on Payload Capability and Their Relation to Interplanetary Mission Design. Master’s Thesis, Virginia Polytechnic Institute, Blacksburg, VA, USA, 1969. [Google Scholar]
- Ivashkin, V.V.; Tupitsyn, N.N. Use of the Moon’s Gravitational Field to Inject a Space Vehicle into a Stationary Earth-Satellite Orbit. Cosm. Res. 1971, 9, 151–159. [Google Scholar]
- Graziani, F.; Castronuovo, M.M.; Teorilatto, P. Geostationary Orbits from Mid-Latitude Launch Sites via Lunar Gravity Assist. In Proceedings of the AAS/AIAA Astrodynamics Specialist Conference, Victoria, BC, Canada, 16–19 August 1993; Volume 84, pp. 561–571. [Google Scholar]
- Circi, C.; Graziani, F.; Teofillatto, P. Moon assisted out of plane maneuvers of earth spacecraft. J. Astronaut. Sci. 2001, 49, 363–383. [Google Scholar] [CrossRef]
- Ocampo, C. Transfer to earth centered orbits via lunar gravity assist. Acta Astronaut. 2003, 52, 173–179. [Google Scholar] [CrossRef]
- Ocampo, C. Trajectory analysis for the lunar flyby rescue of AsiaSat-3/HGS-1. Ann. N. Y. Acad. Sci. 2005, 1065, 232–253. [Google Scholar] [CrossRef] [PubMed]
- Ramanan, R.V.; Adimurthy, V. Precise lunar gravity assist transfers to geostationary orbits. J. Guid. Control Dyn. 2006, 29, 500–502. [Google Scholar] [CrossRef]
- Choi, S.J.; Carrico, J.; Loucks, M.; Lee, H.; Kwon, S. Geostationary Orbit Transfer with Lunar Gravity Assist from Non-equatorial Launch Site. J. Astronaut. Sci. 2021, 68, 1014–1033. [Google Scholar] [CrossRef]
- Policastri, L.; Intelisano, M.; Loucks, M.; West, S. Mission Design for the Sherpa GEO Pathfinder. In Proceedings of the AAS/AIAA Astrodynamics Specialist Conference, Charlotte, NC, USA, 7–11 August 2022. [Google Scholar]
- Short, C.R.; Howell, K.C. Lagrangian coherent structures in various map representations for application to multi-body gravitational regimes. Acta Astronaut. 2014, 94, 592–607. [Google Scholar] [CrossRef]
- Bakhtiari, M.; Abbasali, E.; Sabzy, S.; Kosari, A. Natural coupled orbit—Attitude periodic motions in the perturbed-CRTBP including radiated primary and oblate secondary. Astrodynamics 2022, 7, 229–249. [Google Scholar] [CrossRef]
- Schwaniger, A.J. Trajectories in the Earth-Moon Space with Symmetrical Free Return Properties; NASA TN D-1833; National Aeronautics and Space Administration: Washington, DC, USA, 1963.
- Choi, S.J.; Lee, D.; Kim, I.K.; Kwon, J.W.; Koo, C.H.; Moon, S.; Kim, C.; Min, S.Y.; Rew, D.Y. Trajectory Optimization for a lunar orbiter using a pattern search method. Proc. Inst. Mech. Eng. G J. Aerosp. Eng. 2017, 231, 1325–1337. [Google Scholar] [CrossRef]
- Folta, D.; Demcak, S.; Young, B.; Berry, K. Transfer trajectory design for the Mars atmosphere and volatile evolution (MAVEN) mission. In Proceedings of the AAS/AIAA Space Flight Mechanics Meeting, Kauai, HI, USA, 10–14 February 2013. [Google Scholar]
- Ansys Systems Tool Kit/Astrogator, Software Package, Ver. 12.1.0. Available online: https://help.agi.com/stk/#gator/eq-bplane.htm (accessed on 7 April 2024).
- Berry, M.M.; Coppola, V.T. Integration of orbit trajectories in the presence of multiple full gravitational fields. In Proceedings of the AAS/AIAA Space Flight Mechanics Meeting, Galveston, TX, USA, 27–31 January 2008. [Google Scholar]
- Miele, A.; Mancuso, S. Optimal Trajectories for Earth-Moon-Earth Flight. Acta Astronaut. 2001, 49, 59–71. [Google Scholar] [CrossRef]
- Vallado, D.A. Fundamentals of Astrodynamics and Applications, 3rd ed.; Springer: New York, NY, USA, 2007; pp. 322–339. [Google Scholar]
- Berry, M.M. Comparisons between Newton-Raphson and Broyden’s methods for trajectory design problems. In Proceedings of the AAS/AIAA Astrodynamics Specialist Conference, Girdwood, AK, USA, 31 July–4 August 2011. [Google Scholar]
- Kluever, C.A. Optimal Geostationary Orbit Transfer using Onboard Chemical-Electric Propulsion. J. Spacecr. Rocket. 2012, 49, 1174–1182. [Google Scholar] [CrossRef]


| Model | Earth Propagator | Moon Propagator |
|---|---|---|
| Gravitational field () | WGS84–EGM96 21 × 21 | LP150Q 48 × 48 |
| Atmospheric drag | Jacchia–Roberts | – |
| Solar radiation pressure | Dual cone | Dual cone |
| Third bodies () | Sun, Moon | Earth, Sun |
| SOI distance | 925,000 km | 66,185 km |
| Type (Departure/Node) | (km) | (°) | (°) | (°) | (°) | |
|---|---|---|---|---|---|---|
| Ascending/Ascending | 6678 | 0.0 | 80.0 | 167 | 0.0 | 0.0 |
| Ascending/Descending | 6678 | 0.0 | 80.0 | 347 | 0.0 | 0.0 |
| Descending/Ascending | 6678 | 0.0 | 80.0 | 347 | 180.0 | 0.0 |
| Descending/Descending | 6678 | 0.0 | 80.0 | 167 | 180.0 | 0.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Choi, S.-J.; Lee, H. Seasonal Variations in Lunar-Assisted GEO Transfer Capability for Southward Launch. Aerospace 2024, 11, 321. https://doi.org/10.3390/aerospace11040321
Choi S-J, Lee H. Seasonal Variations in Lunar-Assisted GEO Transfer Capability for Southward Launch. Aerospace. 2024; 11(4):321. https://doi.org/10.3390/aerospace11040321
Chicago/Turabian StyleChoi, Su-Jin, and Hoonhee Lee. 2024. "Seasonal Variations in Lunar-Assisted GEO Transfer Capability for Southward Launch" Aerospace 11, no. 4: 321. https://doi.org/10.3390/aerospace11040321
APA StyleChoi, S.-J., & Lee, H. (2024). Seasonal Variations in Lunar-Assisted GEO Transfer Capability for Southward Launch. Aerospace, 11(4), 321. https://doi.org/10.3390/aerospace11040321