Study on RP-3 Aviation Fuel Vapor Concentration
Abstract
:1. Introduction
2. Fuel Vapor Concentration Study in the Equilibrium State
2.1. Fuel Composition and Calculation Model
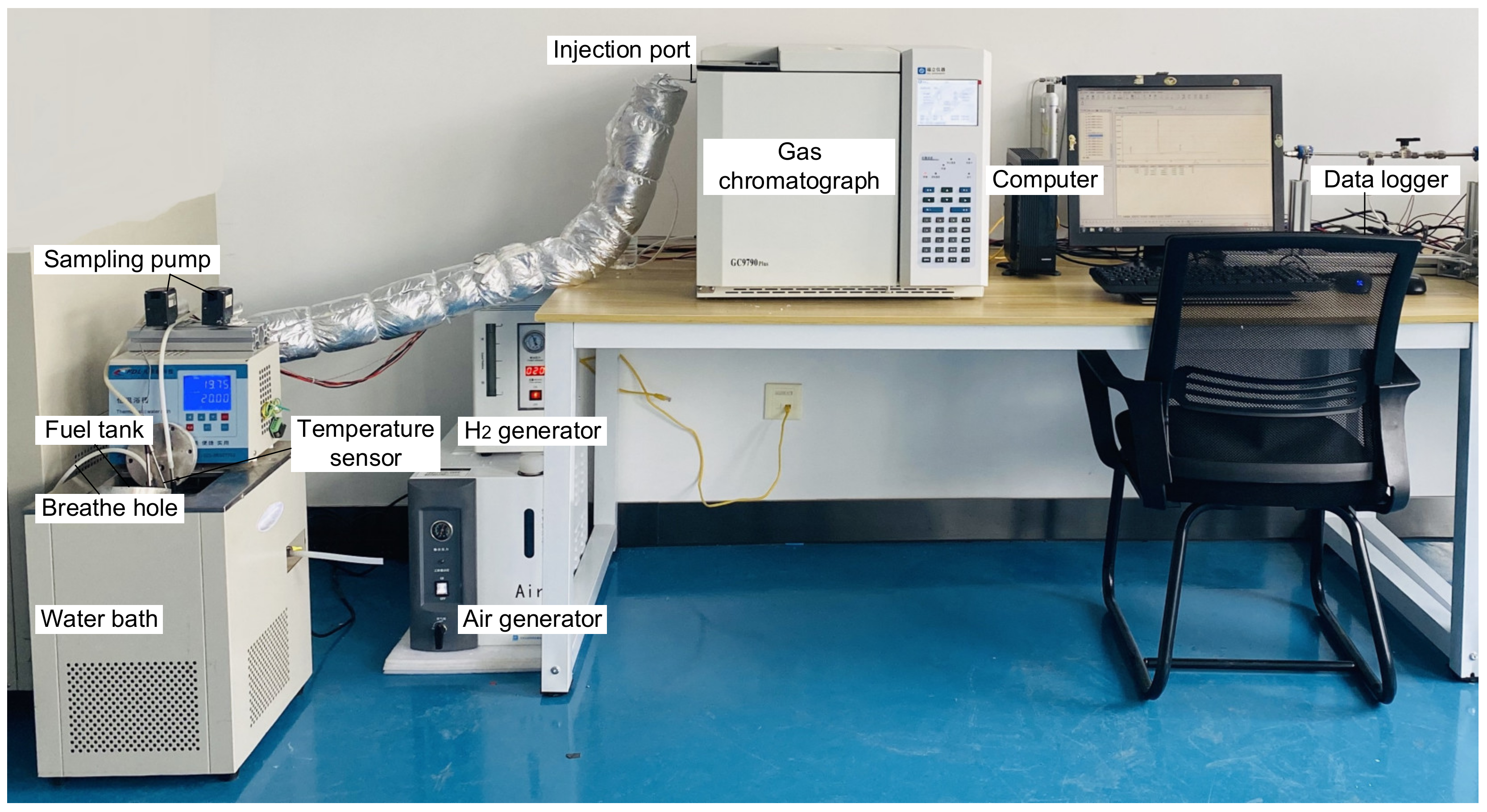
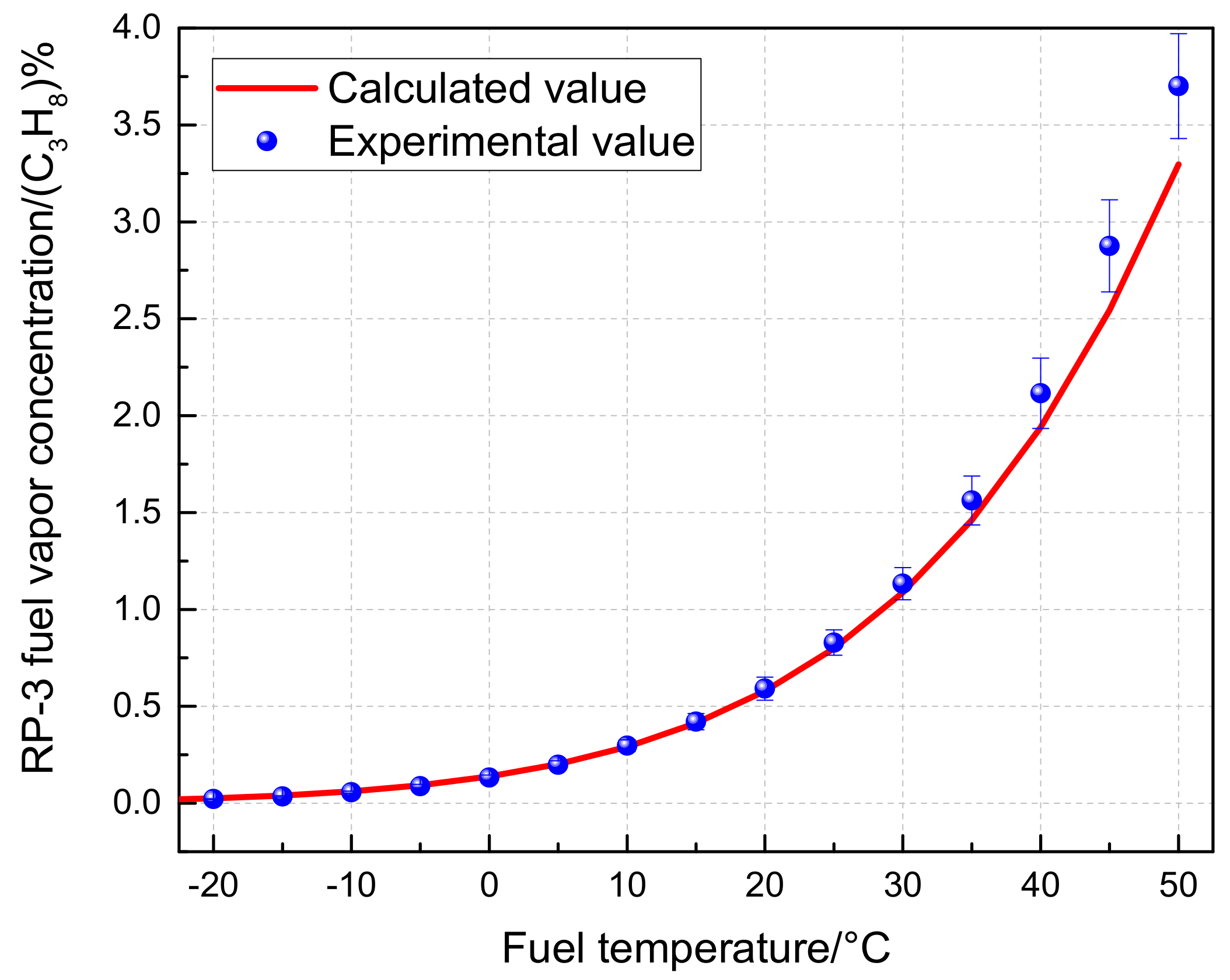
2.2. Experimental Verification
- Connect the experimental system and fill the fuel tank with RP-3 fuel to a fuel loading ratio of 50%, ensuring that the sampling rod port is positioned 1 cm away from the fuel surface.
- Set the fuel temperature by adjusting the water bath temperature and heating the fuel tank until it reaches the predetermined temperature. Maintain the temperature for 2 h to ensure thermal stability.
- Measure the fuel vapor concentration by taking three readings from each of the two sampling ports under the same fuel temperature. An interval of 1 h is provided between each measurement to ensure the fuel tank reaches a stable mass transfer state.
- Modify the working conditions by resetting the water bath temperature and repeat step (3).
- Upon completion of all experiments, close the water bath, and allow the fuel to cool before returning it to the fuel storage tank.
2.3. Results and Analysis
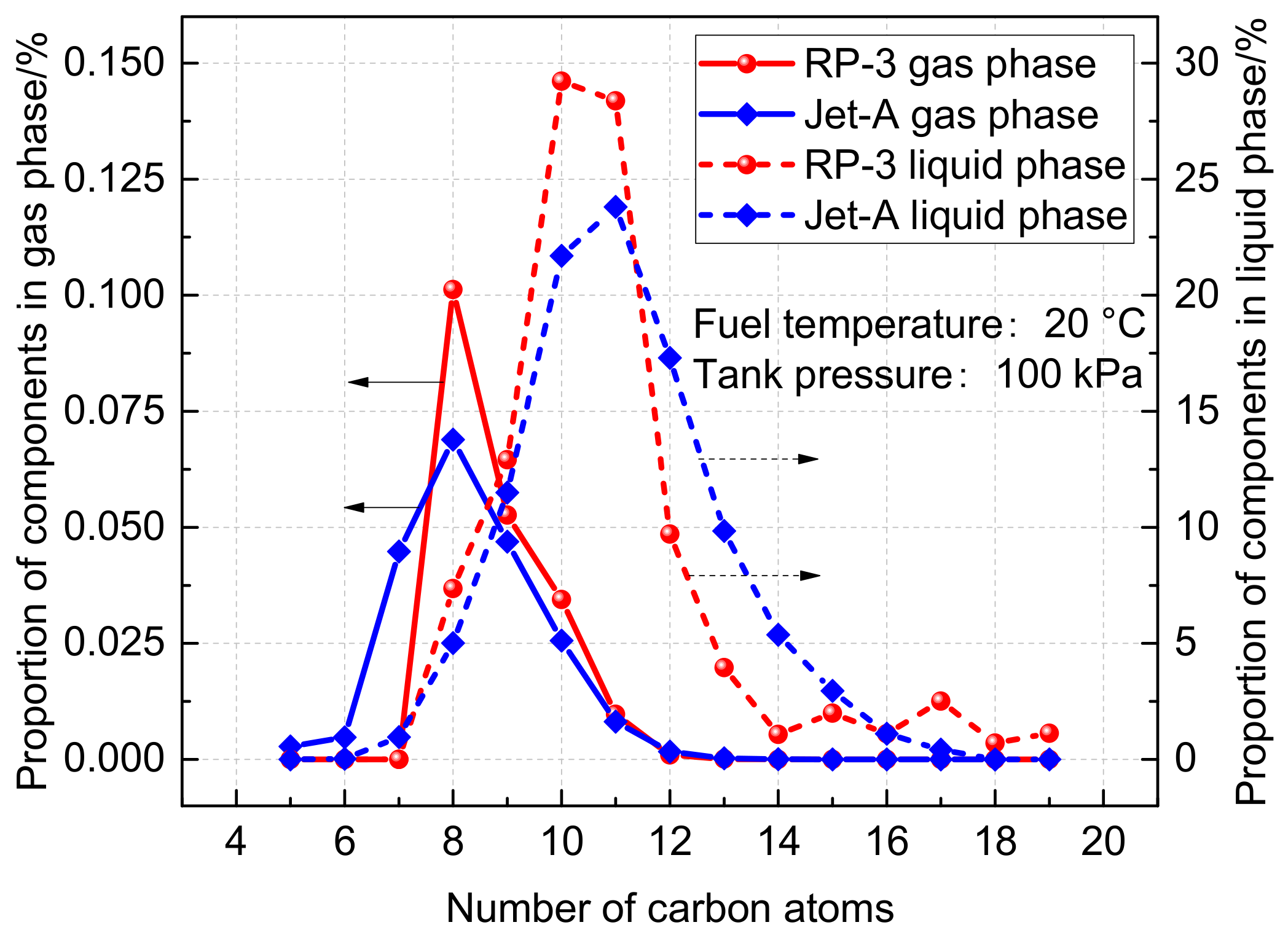
2.3.1. Composition Difference between RP-3 and Jet-A Fuels
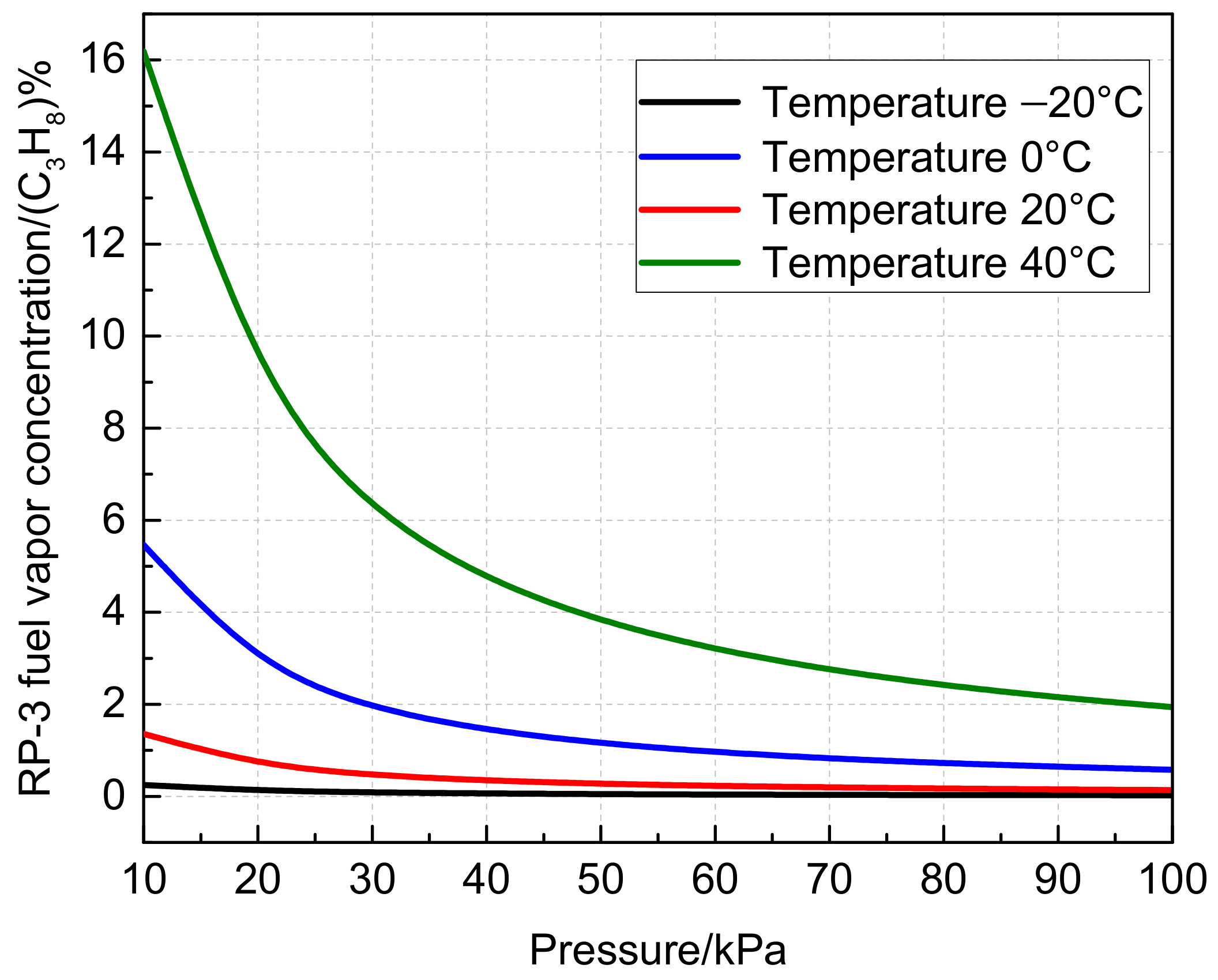
2.3.2. Influence of Temperature and Pressure
3. Fuel Vapor Concentration Study in the Transient State
3.1. Transient Mathematical Model
3.1.1. Model Assumptions
- (1)
- The gas in the fuel tank ullage is assumed to be uniformly mixed and treated as an ideal gas, while aviation fuel is treated as an incompressible fluid.
- (2)
- All radiation effects, including solar radiation, ground radiation, and radiation from the fuel tank wall, are neglected.
- (3)
- Trace gases, such as carbon dioxide and water vapor, are disregarded in both the air and fuel.
- (4)
- The fuel tank size is assumed to be 1 m × 1 m × 1 m.
- (5)
- The fuel tank is considered a lumped heat capacity system, meaning that the internal thermal resistance of the object can be ignored compared to the thermal resistance of convection heat transfer.
- (6)
- The relationship between temperature and pressure with flight altitude is based on reference [24]. Moreover, the total temperature of the external environment after aerodynamic heating is determined by the following equation:
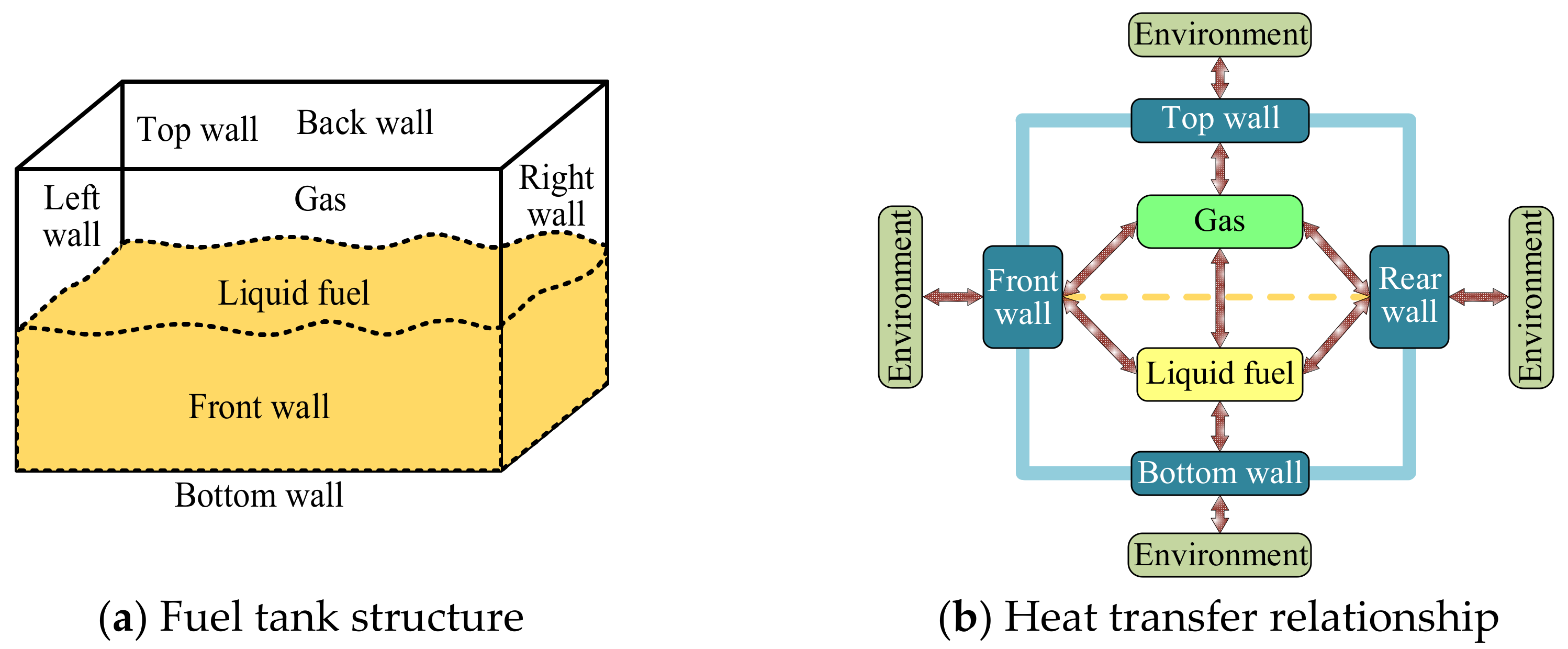
3.1.2. Heat Transfer Model
3.1.3. Mass Transfer Model
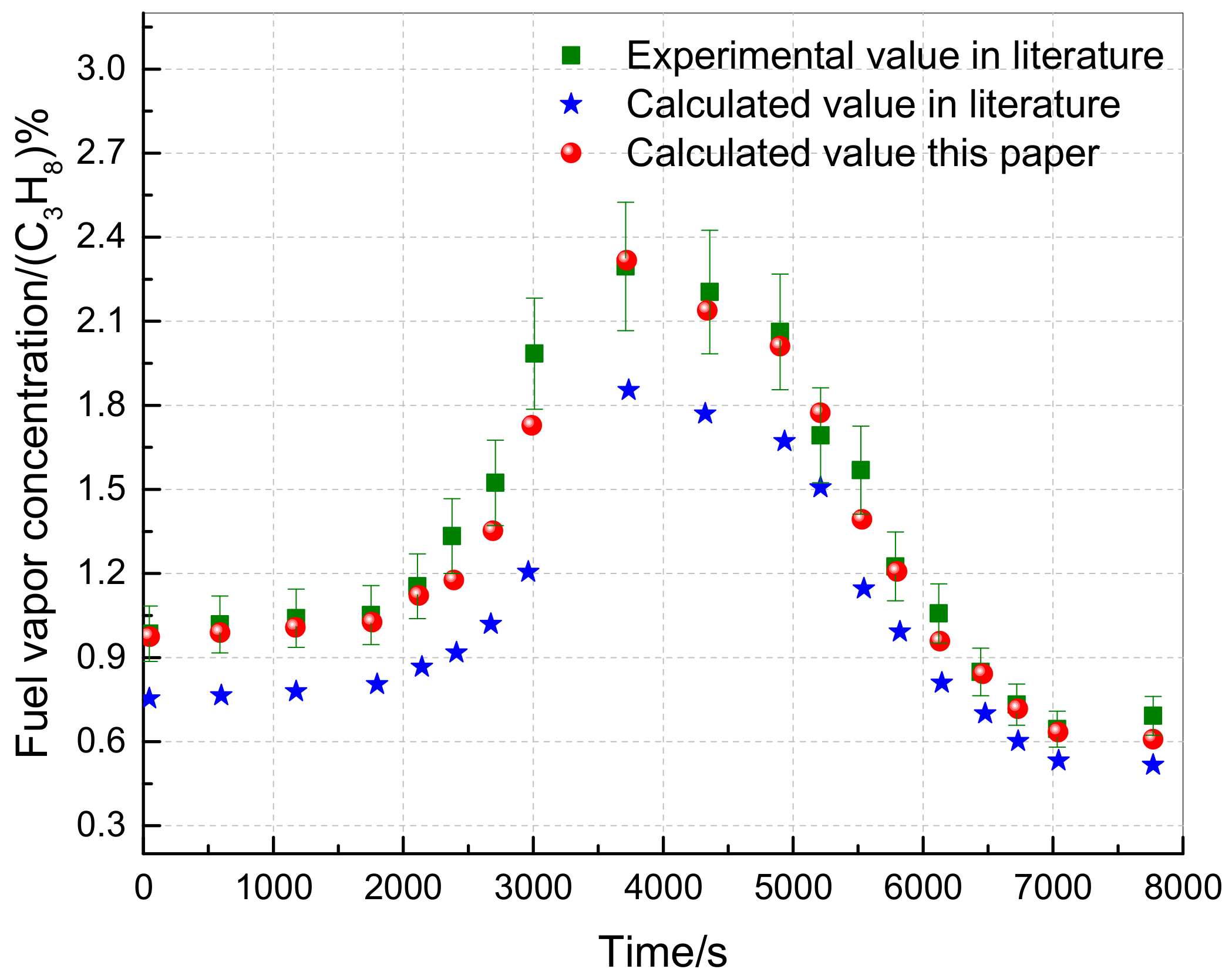
3.2. Model Verification
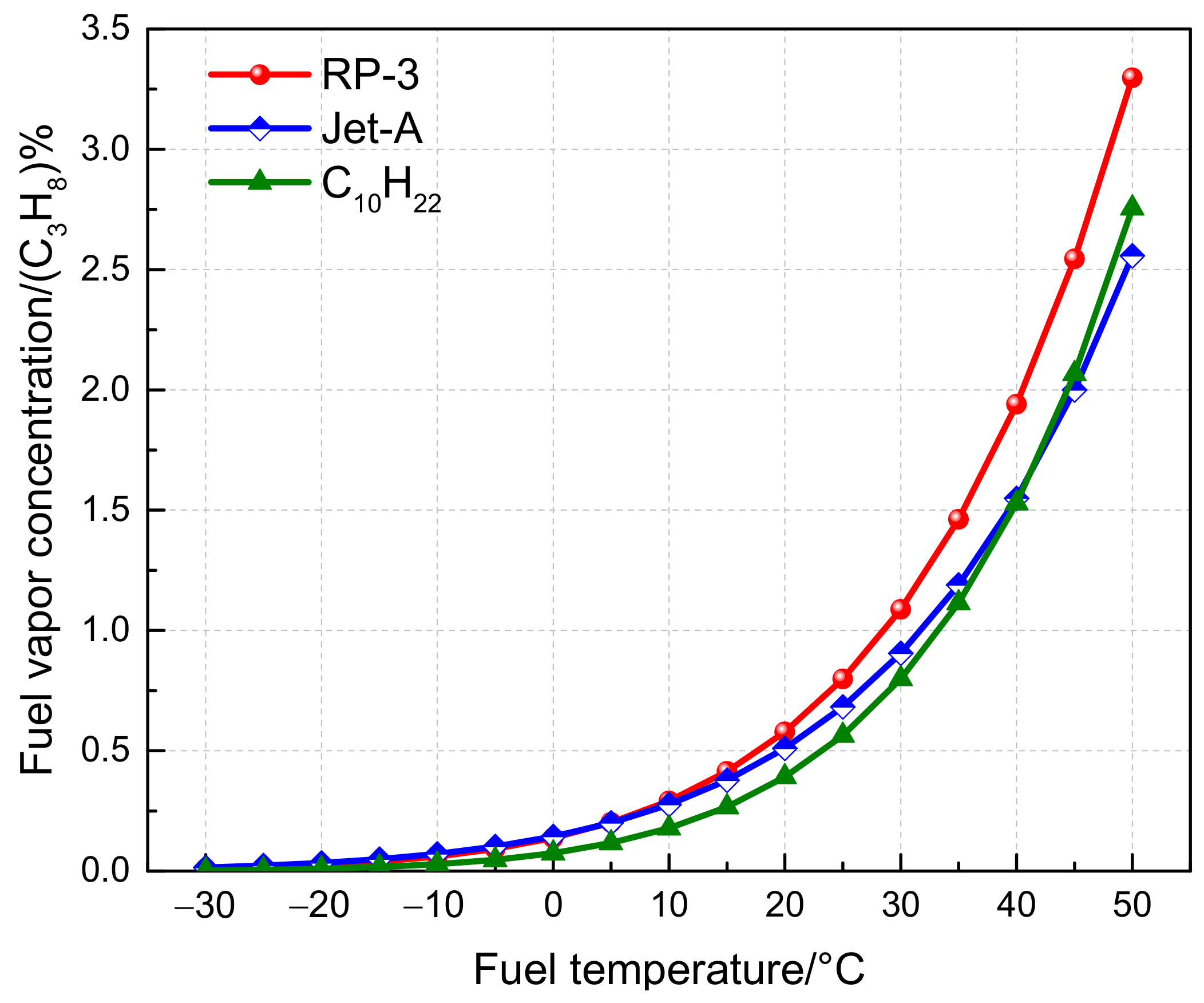
3.3. Results and Analysis
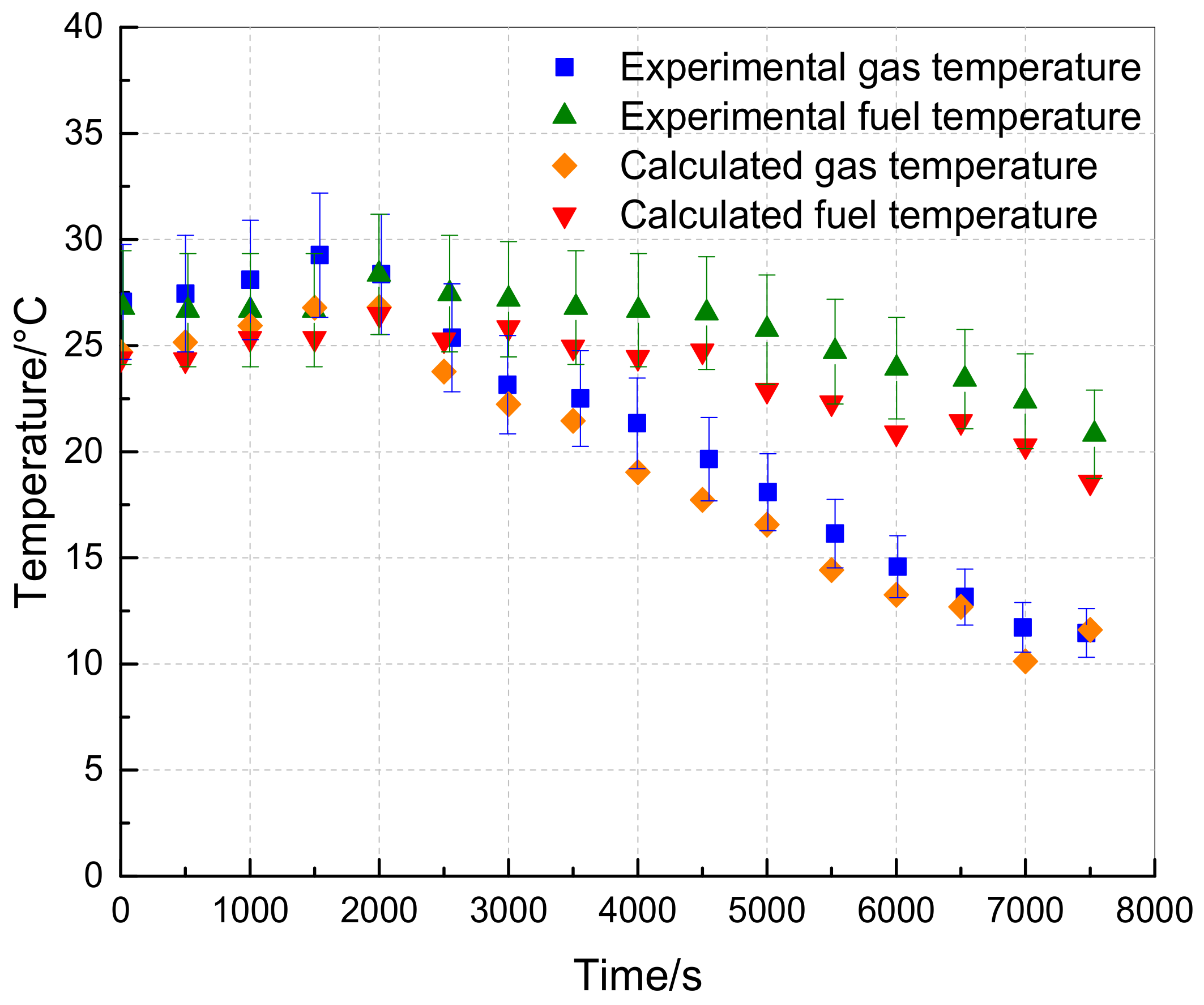
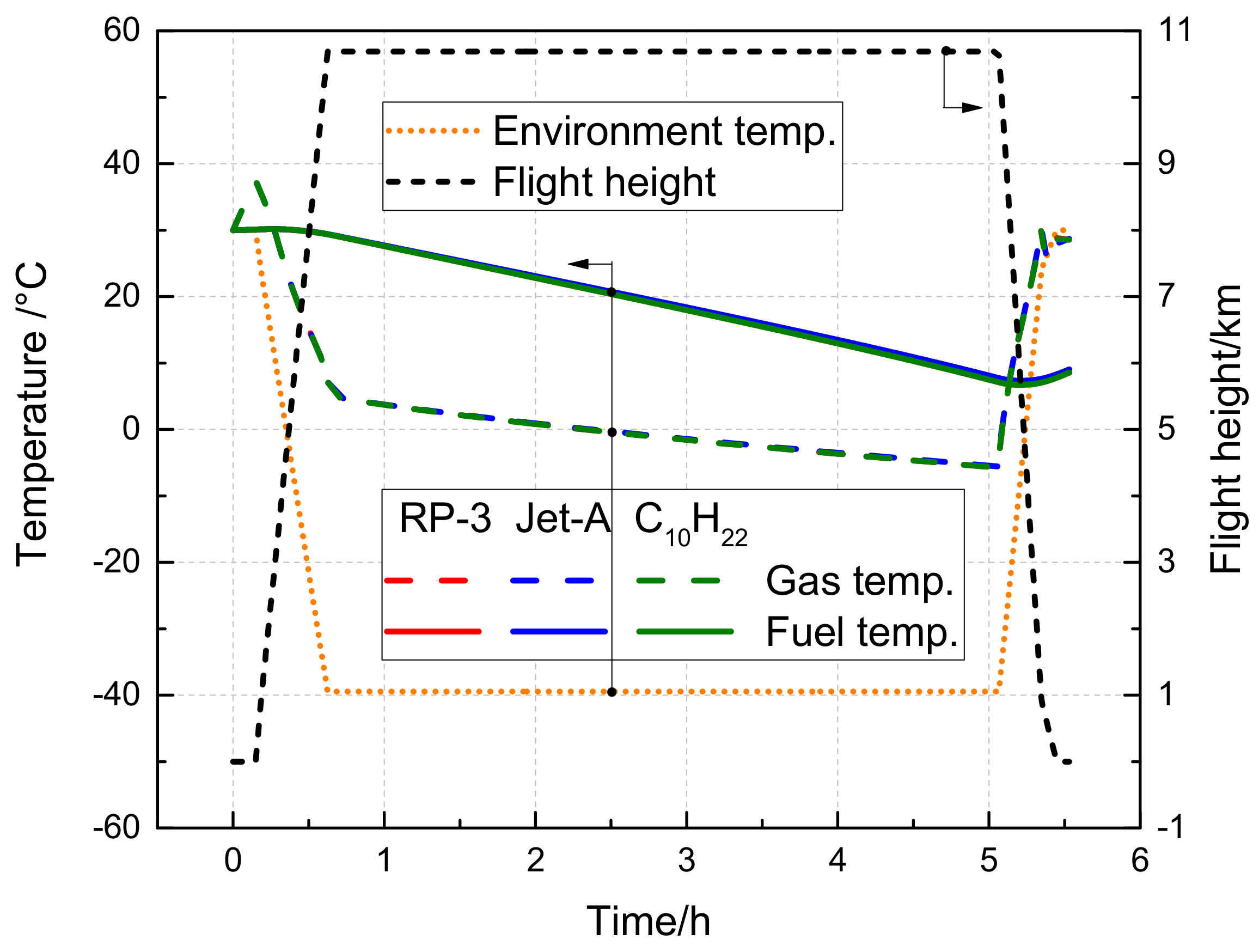
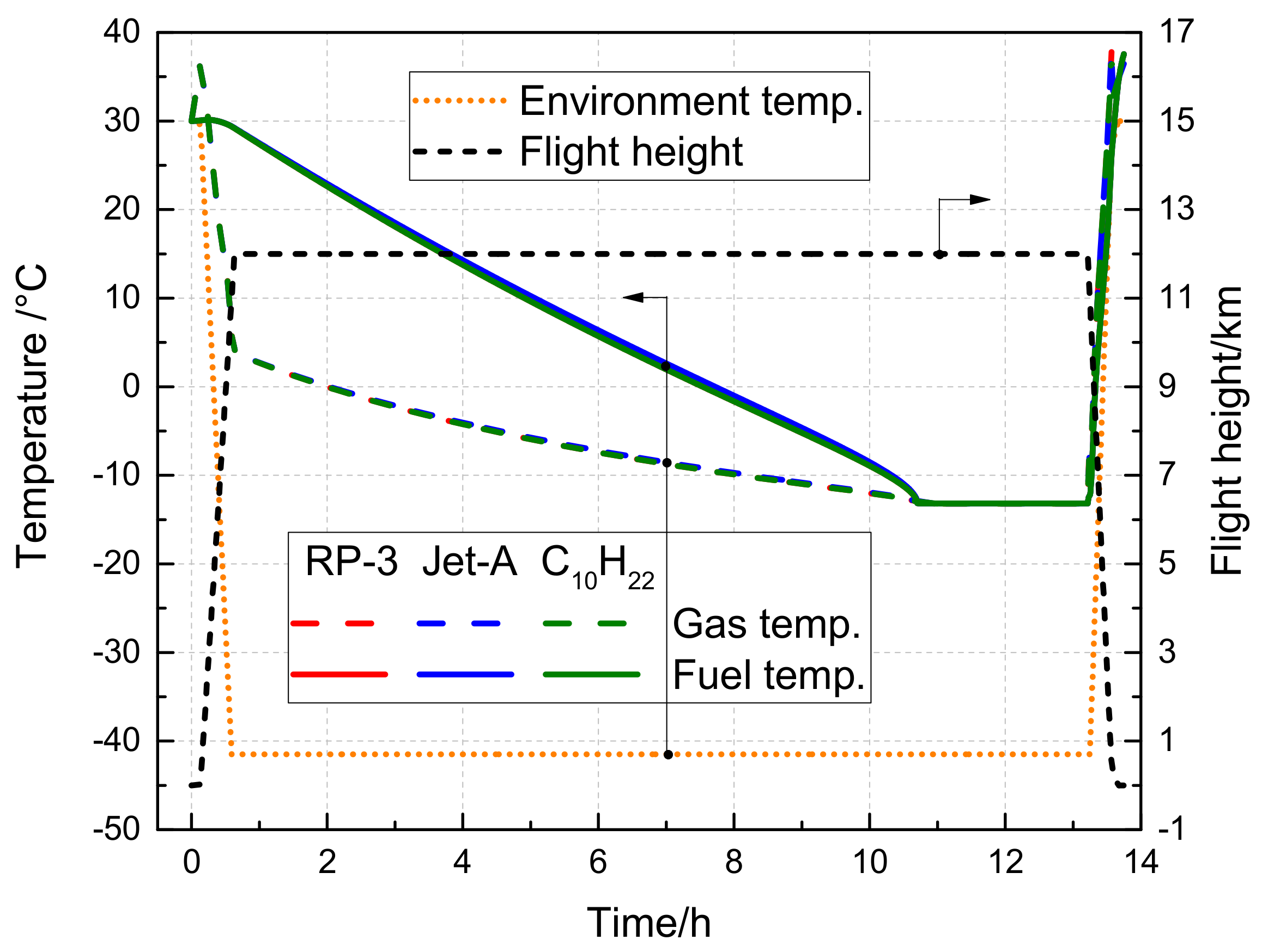
3.3.1. Effect of the Flight State on Fuel Tank Temperature
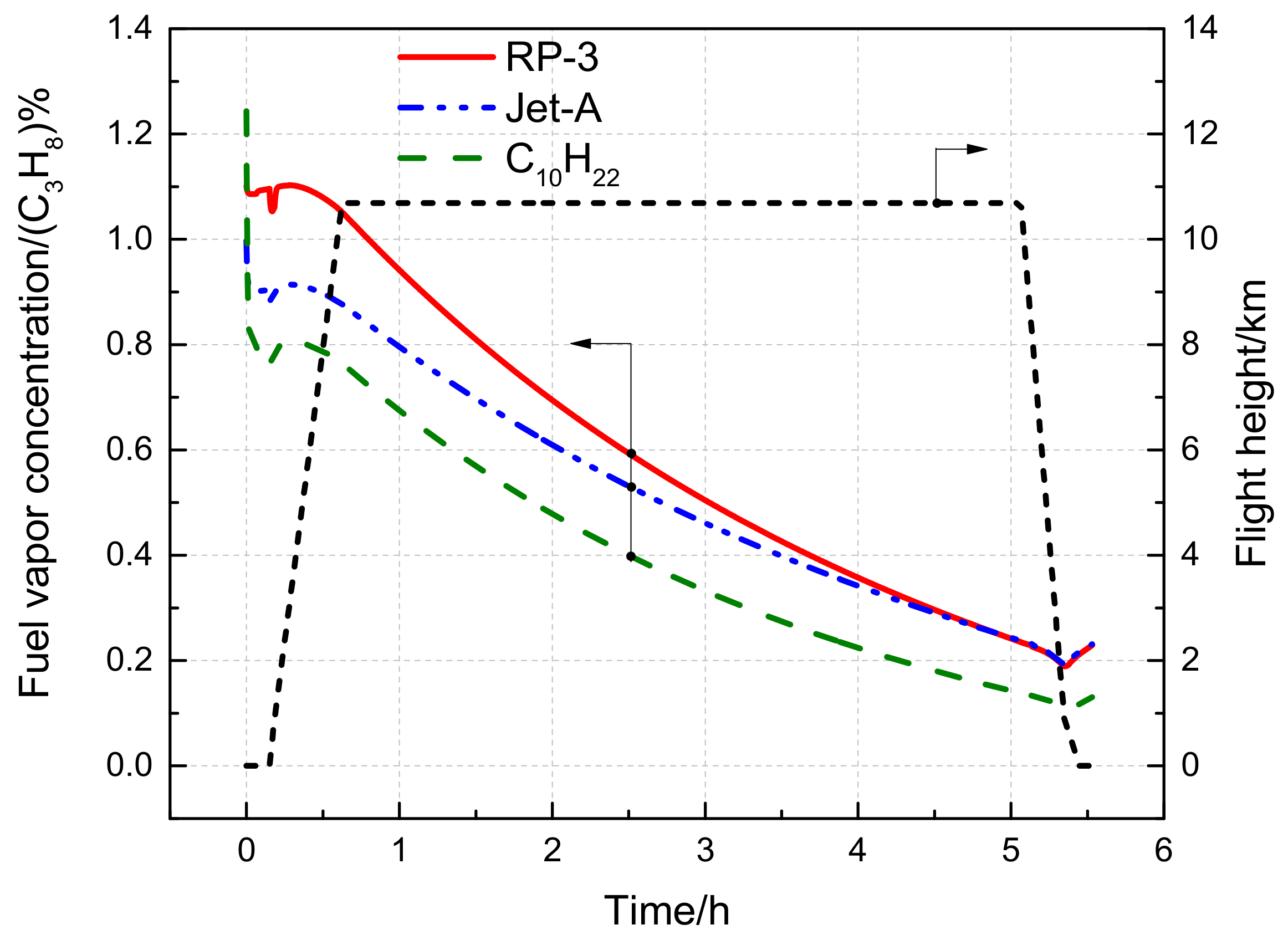
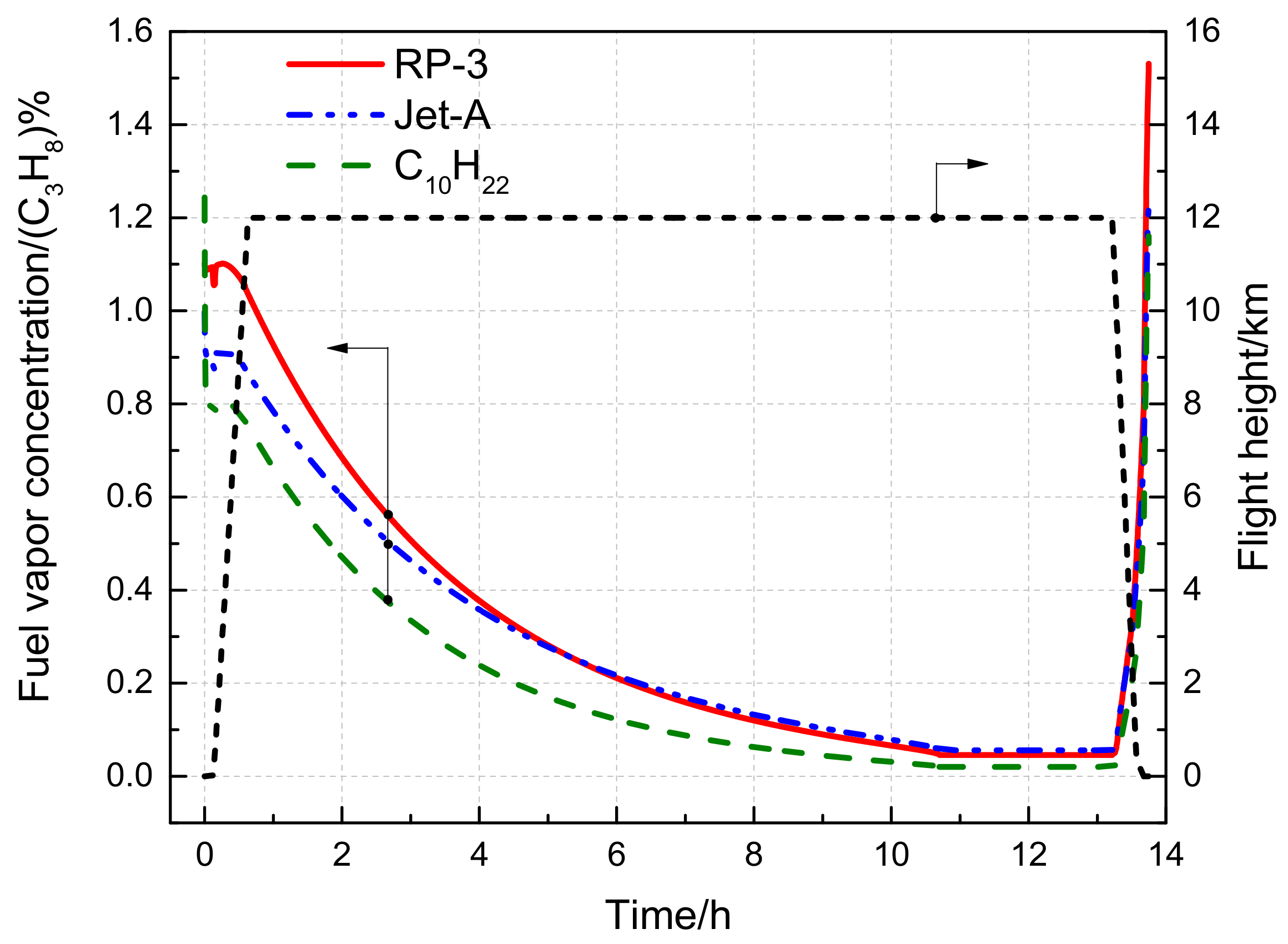
3.3.2. Effect of the Flight State on the Fuel Vapor Concentration
4. Discussion
- The fuel vapor concentrations in the fuel tank ullage exhibit significant differences between RP-3 and Jet-A aviation fuels. During short voyages, the fuel vapor concentration of RP-3 is consistently higher than that of Jet-A. Conversely, during long voyages, the fuel vapor concentration of Jet-A is marginally higher than that of RP-3 towards the end of the cruise phase.
- RP-3 cannot be simplified as C10H22 when calculating fuel vapor concentration. The fuel vapor concentration calculated based on RP-3 as a single-component fuel is lower than the value calculated using a multicomponent model. For instance, at 40 °C and atmospheric pressure, the difference between the two approaches is 21.19%, and similar results were obtained under flight conditions.
- Assessing fuel tank flammability based on fuel vapor concentration is more reasonable than using fuel temperature as a criterion. In the flight state, the temperature change curves of the gas and fuel for RP-3, Jet-A, and C10H22 show little difference. If the current airworthiness certification’s method of assessing fuel tank flammability based on temperature is adopted, the impact on RP-3 and Jet-A will not be significant. However, the significant differences in fuel vapor concentrations between RP-3 and Jet-A determine the flammability of the fuel tank.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Smith, D.E. Fuel Tank Inerting Systems for Civil Aircraft. Ph.D. Thesis, College of Engineering, Colorado State University, Fort Collins, FL, USA, 2014. [Google Scholar]
- Peng, X.T.; Wang, H.M.; Huang, L.; Liu, G.T.; Wang, C.C.; Feng, S.Y. Performance of an oxygen-consuming inerting system for an aircraft fuel tank with RP-3 aviation fuel in flight. Aerosp. Sci. Technol. 2022, 123, 107446. [Google Scholar] [CrossRef]
- Marciello, V.; Di Stasio, M.; Ruocco, M.; Trifari, V.; Nicolosi, F.; Meindl, M.; Lemoine, B.; Caliandro, P. Design exploration for sustainable regional Hybrid-Electric aircraft: A study based on technology forecasts. Aerospace 2023, 10, 165. [Google Scholar] [CrossRef]
- Fuel Flammability Task Group. A Review of the Flammability Hazard of Jet a Fuel Vapor in Civil Transport Aircraft Fuel Tanks; 20591 Report No. DOT/FAA/AR-98/26; U.S. Department of Transportation Federal Aviation Administration, Office of Aviation Research: Washington, DC, USA, 1998.
- Feng, S.Y.; Peng, X.T.; Chen, C.; Zhang, R.H.; Liu, W.H. Effect of air supplementation on the performance of an onboard catalytic inerting system. Aerosp. Sci. Technol. 2019, 97, 105605. [Google Scholar] [CrossRef]
- Li, J.; Yang, W.; Pei, Y. Vulnerability assessment for fire and explosion suppression measures of aircraft fuel system. Adv. Mater. Res. 2012, 510, 64–69. [Google Scholar] [CrossRef]
- Cican, G.; Frigioescu, T.F.; Crunteanu, D.E.; Cristea, L. Micro turbojet engine nozzle ejector impact on the acoustic emission, thrust force and fuel consumption analysis. Aerospace 2023, 10, 162. [Google Scholar] [CrossRef]
- China Civil Aviation Regulations, Part 25, Airworthiness Standards for Transport Aircraft [CCAR-25-R4], Civil Aviation Administration Order No. 209, 2011.12.7. Available online: http://www.caac.gov.cn/XXGK/XXGK/MHGZ/201606/P020160622405532063536.pdf (accessed on 3 April 2023).
- Zimmerman, S.P.; Keneshea, T.J. Handbook of Aviation Fuel Properties; Coordinating Research Council: Alpharetta, GA, USA, 1983; CRC Rep. No. 635. [Google Scholar]
- Fu, W.; Li, M.; Tao, Z. World Aviation Fuel Specifications and Progress, 1st ed.; China Petrochemical Press: Beijing, China, 2011; pp. 19–54. [Google Scholar]
- Dubovkin, H. Jet Fuel Performance Manual, 1st ed.; Aviation Industry Press: Beijing, China, 1990; pp. 98–125. [Google Scholar]
- Wang, S.Y. Research on Fuel Tank Flammability Assessment Technology Based on Domestic Fuel Physical-Chemical Characteristics. Ph.D. Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2012. [Google Scholar]
- Shepherd, J.E.; Nuyt, C.D.; Lee, J.J. Flash Point and Chemical Composition of Aviation Kerosene (Jet-A); Rep. No. NTSB12-98-CB-0415; California Institute of Technology: Pasadena, CA, USA, 2000. [Google Scholar]
- Woodrow, J.E.; Seiber, J.N. The Laboratory Characterization of Jet Fuel Vapor under Simulated Flight Conditions; 89557-0187 Report No. NTSB12-97-SP-0255; Center for Environmental Sciences and Engineering, University of Nevada: Reno, NV, USA, 1997. [Google Scholar]
- Ochs, R.I. Vaporization of JP-8 Jet Fuel in a Simulated Aircraft Fuel Tank Under Varying Ambient Conditions; 20591; FAA Report, Report No. DOT/FAA/AR-TT09/42; Air Traffic Organization NextGen & Operations Planning Office of Research and Technology Development: Washington, DC, USA, 2009.
- Summer, S.M. Mass Loading Effects on Fuel Vapor Concentrations in an Aircraft Fuel Tank Ullage; FAA Report, Report No. DOT/FAA/AR-TN99/65; U.S. Department of Transportation, Federal Aviation Administration: Washington, DC, USA, 1999.
- Polymeropoulos, C.E.; Ochs, R. Jet-A vaporization in a simulated aircraft fuel tank (Including Sub-Atmospheric Pressures and Low Temperatures). In Proceedings of the Fourth International Aircraft Fire and Cabin Research Conference, Lisbon, Portugal, 15–18 November 2004. [Google Scholar]
- Shao, L. Theoretical and Experimental Study of Oxygen Consumed Inerting Technology for Aircraft Fuel Tank. Ph.D. Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2018. [Google Scholar]
- Mze-Ahmed, A.; Hadj-Ali, K.; Devar, P.; Dagaut, P. Kinetics of oxidation of a reformulated jet fuel (1-hexanol/jet a-1) in a jet-stirred reactor: Experimental and modeling study. Combust. Sci. Technol. 2012, 79, 1039–1050. [Google Scholar] [CrossRef]
- Zheng, D.; Yu, W.M.; Zhong, B.J. RP-3 aviation kerosene surrogate fuel and the chemical reaction kinetic model. Acta. Phys. Chim. Sin. 2015, 31, 636–642. [Google Scholar]
- Xu, J.Q.; Guo, J.J.; Liu, A.K. Construction of autoignition mechanisms for the combustion of RP-3 surrogate fuel and kinetics simulation. Acta. Phys. Chim. Sin. 2015, 4, 643–652. [Google Scholar]
- Dadia, D. Modeling Wing Tank Flammability. Ph.D. Thesis, The State University of New Jersey, New Brunswick, NJ, USA, 2009. [Google Scholar]
- Bruce, E.P.; John, M.P.; John, P.O. The Properties of Gases and Liquids, 5th ed.; McGraw-Hill Companies Press: New York, NY, USA, 2001; pp. 32–57. [Google Scholar]
- Shou, R.; He, H. Aircraft Environmental Control, 1st ed.; Beijing University of Aeronautics and Astronautics Press: Beijing, China, 2004; pp. 110–146. [Google Scholar]
- Sanchez, F.; Liscouët-Hanke, S.; Bhise, T. Influence of ventilation flow rate and gap distance on the radiative heat transfer in aircraft avionics bays. Aerospace 2022, 9, 806. [Google Scholar] [CrossRef]
- Zhao, X.; Su, L.; Jiang, J.; Deng, W.; Zhao, D. A review of working fluids and flow state effects on thermal performance of micro-channel oscillating heat pipe for aerospace heat dissipation. Aerospace 2023, 10, 179. [Google Scholar] [CrossRef]
- Shi, Y.; Ding, S.; Liu, P.; Qiu, T.; Liu, C.; Qiu, C.; Ye, D. Swirl flow and heat transfer in a Rotor-Stator cavity with consideration of the inlet seal thermal deformation effect. Aerospace 2023, 10, 134. [Google Scholar] [CrossRef]
- Incropera, F.; DeWitt, D. Fundamentals of Heat and Mass Transfer, 4th ed.; Wiley Press: New York, NY, USA, 2011; pp. 132–151. [Google Scholar]

| Fuel Type | Alkanes/% | Cycloalkanes/% | Aromatic Hydrocarbons/% | Olefins/% | Others/% |
|---|---|---|---|---|---|
| RP-3 | 53.0 | 37.7 | 4.6 | 2.0 | 2.7 |
| Jet-A | 39.1 | 23.2 | 37.4 | 0 | 0.3 |
| Carbon Atom Numbers Ci | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| RP-3 mole fraction/% | 0 | 0 | 0 | 7.36 | 12.91 | 29.22 | 28.37 | 9.71 | 3.96 | 1.08 | 1.99 | 1. 06 | 2.51 | 0.7 | 1.13 |
| Jet-A mole fraction/% | 0.032 | 0.22 | 1.08 | 2.85 | 7.77 | 15.6 | 20 | 18.1 | 15.2 | 10.5 | 5.49 | 2.1 | 0.82 | 0.13 | 0.108 |
| Molar mass Mi/×103 kg·mol−1 | 72 | 86 | 100 | 114 | 128 | 142 | 156 | 170 | 184 | 198 | 212 | 226 | 240 | 254 | 268 |
| A | 3.98 | 4.00 | 4.02 | 4.05 | 4.07 | 4.07 | 4.10 | 4.12 | 4.13 | 4.14 | 4.15 | 4.15 | 4.14 | 4.13 | 4.14 |
| B | 1064.84 | 1170.88 | 1263.91 | 1356.36 | 1438.03 | 1495.17 | 1569.57 | 1639.27 | 1690.67 | 1740.88 | 1789.95 | 1830.51 | 1865.10 | 1894.30 | 1932.80 |
| D | 232.01 | 224.32 | 216.43 | 209.64 | 202.69 | 193.86 | 187.70 | 181.84 | 174.22 | 167.72 | 161.38 | 154.45 | 149.20 | 143.30 | 137.60 |
| Equipment | Manufacturer | Model | Range | Precision |
|---|---|---|---|---|
| Fuel tank | — | DN10 cm × Length 10 cm | — | — |
| Water bath | FDL | DC-303 | 243.15~373.15 K | ±0.1 K |
| Temperature sensor | Shenpeng | T-type thermocouple | 273.15~373.15 K | ±0.1 K |
| Sampling pump | JH | JH24-80 | 0–12 L/min | — |
| Gas chromatograph | Fuli | GC9790plus | — | ±1.0% |
| H2 generator | Hongyi | HYH-300B | — | — |
| Air generator | Hongyi | HY-3A 3L | — | — |
| Data logger | HIOKI | LR8432 | — | — |
| State | Flight Information (Short Voyage) | Flight Information (Long Voyage) | ||||
|---|---|---|---|---|---|---|
| Block Time/min | Height/m | Fuel Consumption/kg | Block Time/min | Height/m | Fuel Consumption/kg | |
| Slide out | 9 | 0 | 90 | 7 | 0 | 189 |
| Take off | 2 | 0→900 | 210 | 2 | 0→457 | 630 |
| Climb out | 26.5 | 900→10,688 | 1604 | 29 | 457→12,000 | 4992 |
| Cruise | 266.4 | 10,688 | 9429 | 755 | 12,000 | 67,987 |
| Decline | 17 | 10,688→900 | 146 | 21 | 12,000→457 | 373 |
| Approach | 6 | 900→0 | 120 | 6 | 457→0 | 240 |
| Slide in | 5 | 0 | 503. | 5 | 0 | 135 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, X.; Fan, D.; Qiu, D.; Feng, S.; Peng, H.; Bai, W. Study on RP-3 Aviation Fuel Vapor Concentration. Aerospace 2023, 10, 497. https://doi.org/10.3390/aerospace10060497
Peng X, Fan D, Qiu D, Feng S, Peng H, Bai W. Study on RP-3 Aviation Fuel Vapor Concentration. Aerospace. 2023; 10(6):497. https://doi.org/10.3390/aerospace10060497
Chicago/Turabian StylePeng, Xiaotian, Donghao Fan, Doudou Qiu, Shiyu Feng, Hao Peng, and Wentao Bai. 2023. "Study on RP-3 Aviation Fuel Vapor Concentration" Aerospace 10, no. 6: 497. https://doi.org/10.3390/aerospace10060497
APA StylePeng, X., Fan, D., Qiu, D., Feng, S., Peng, H., & Bai, W. (2023). Study on RP-3 Aviation Fuel Vapor Concentration. Aerospace, 10(6), 497. https://doi.org/10.3390/aerospace10060497





