Aerodynamic Optimization Design of Supersonic Wing Based on Discrete Adjoint
Abstract
1. Introduction
2. Numerical Simulation Method and Discrete Adjoint Equation
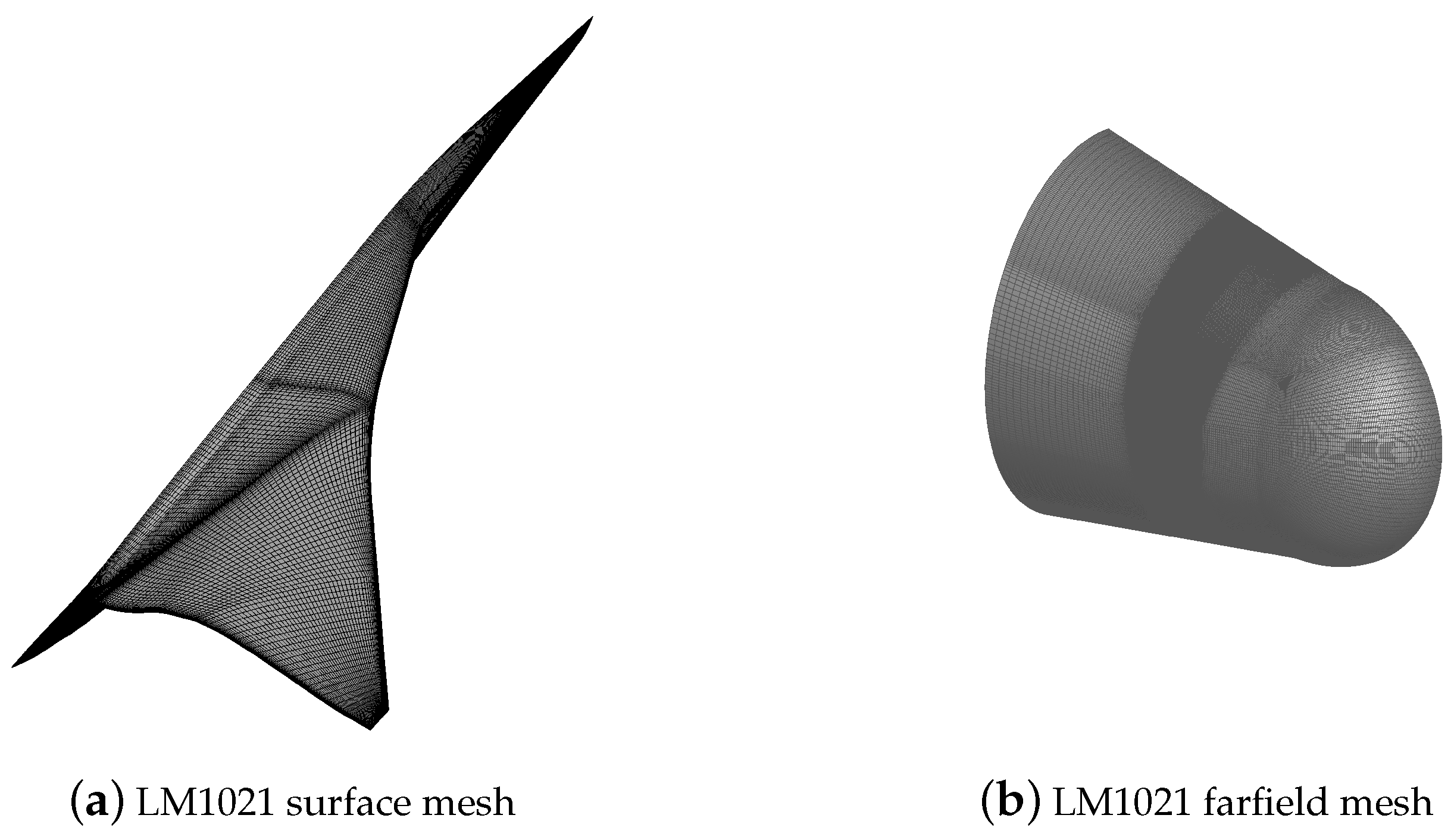
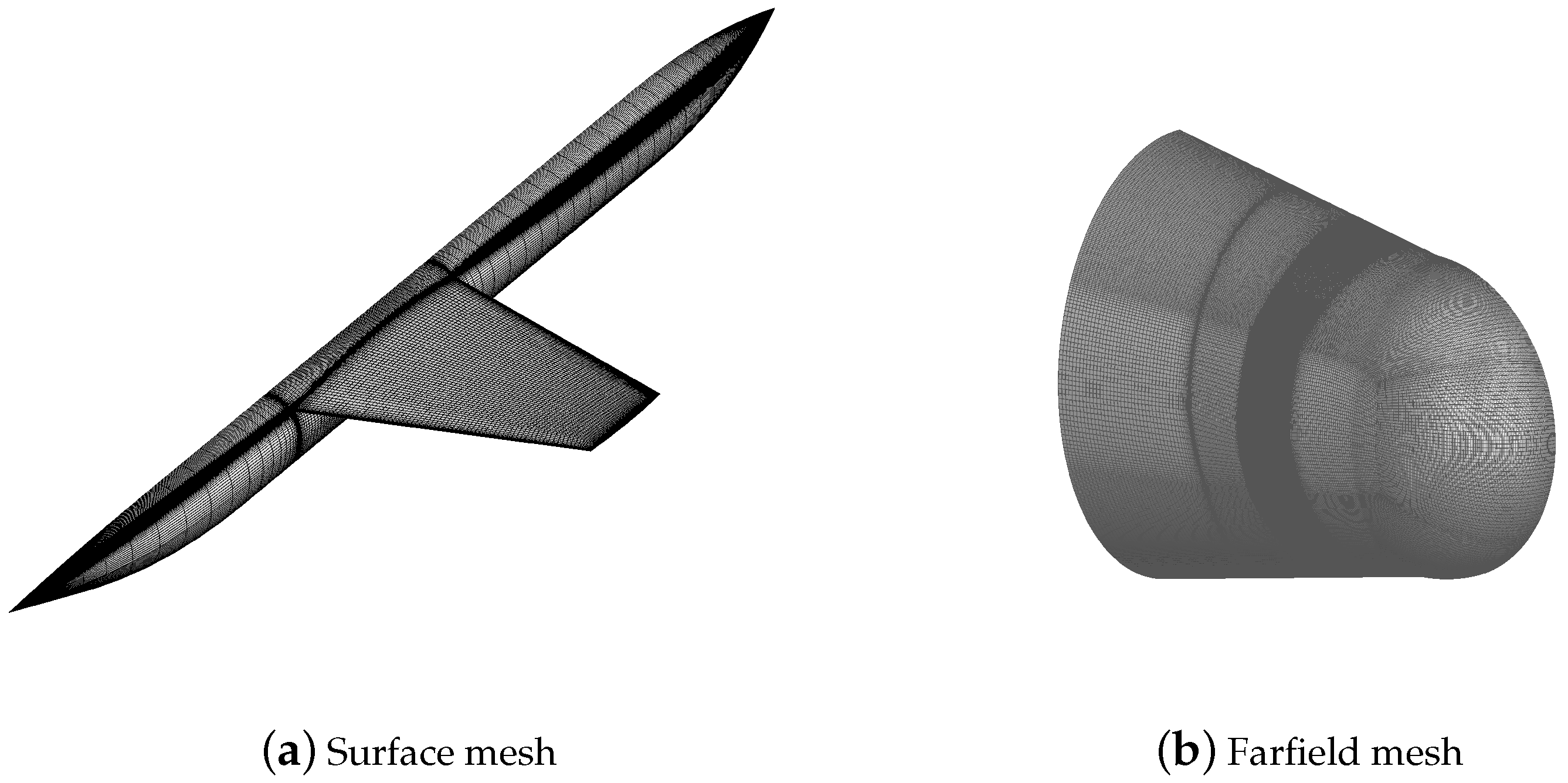
2.1. Numerical Simulation Method
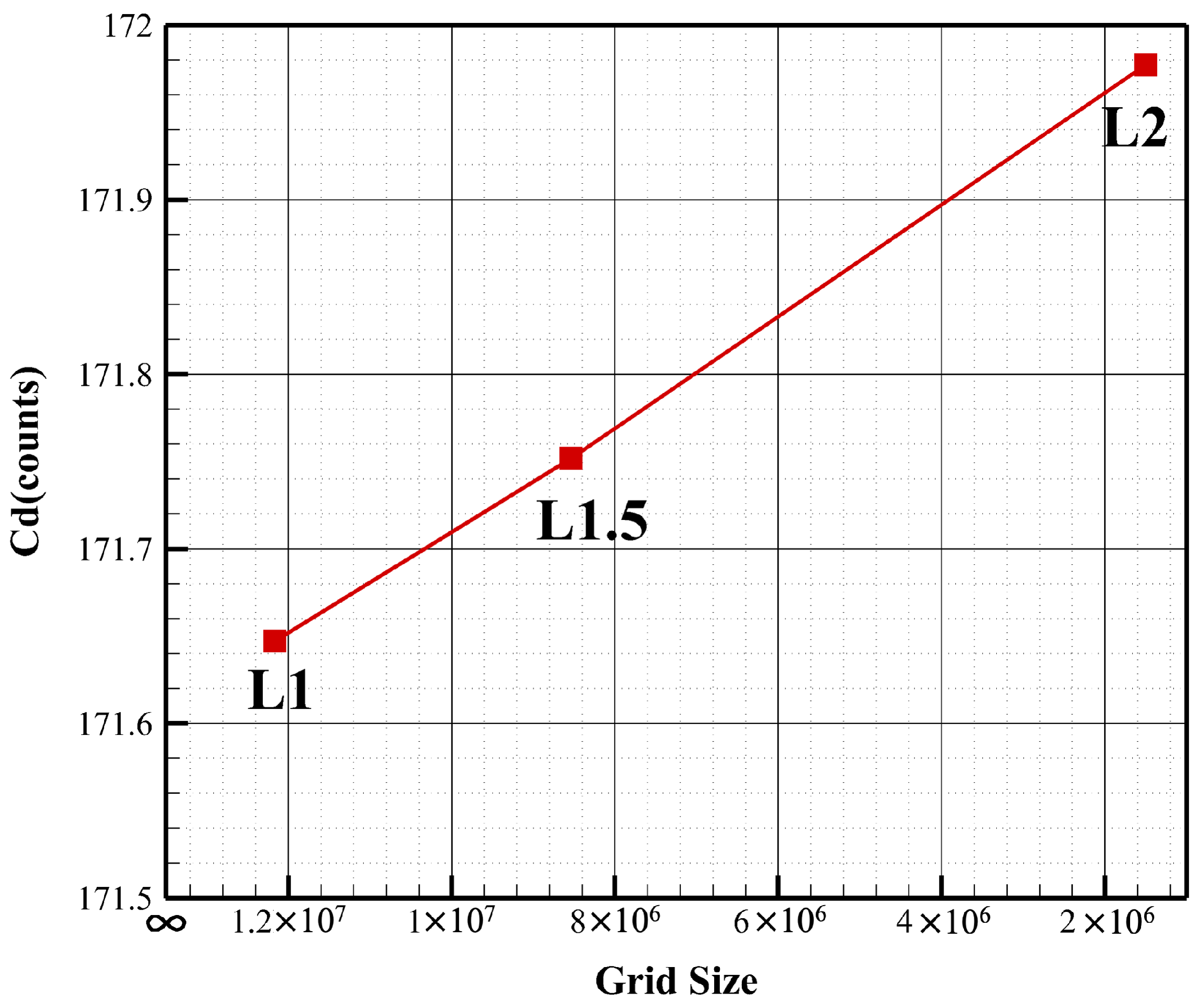
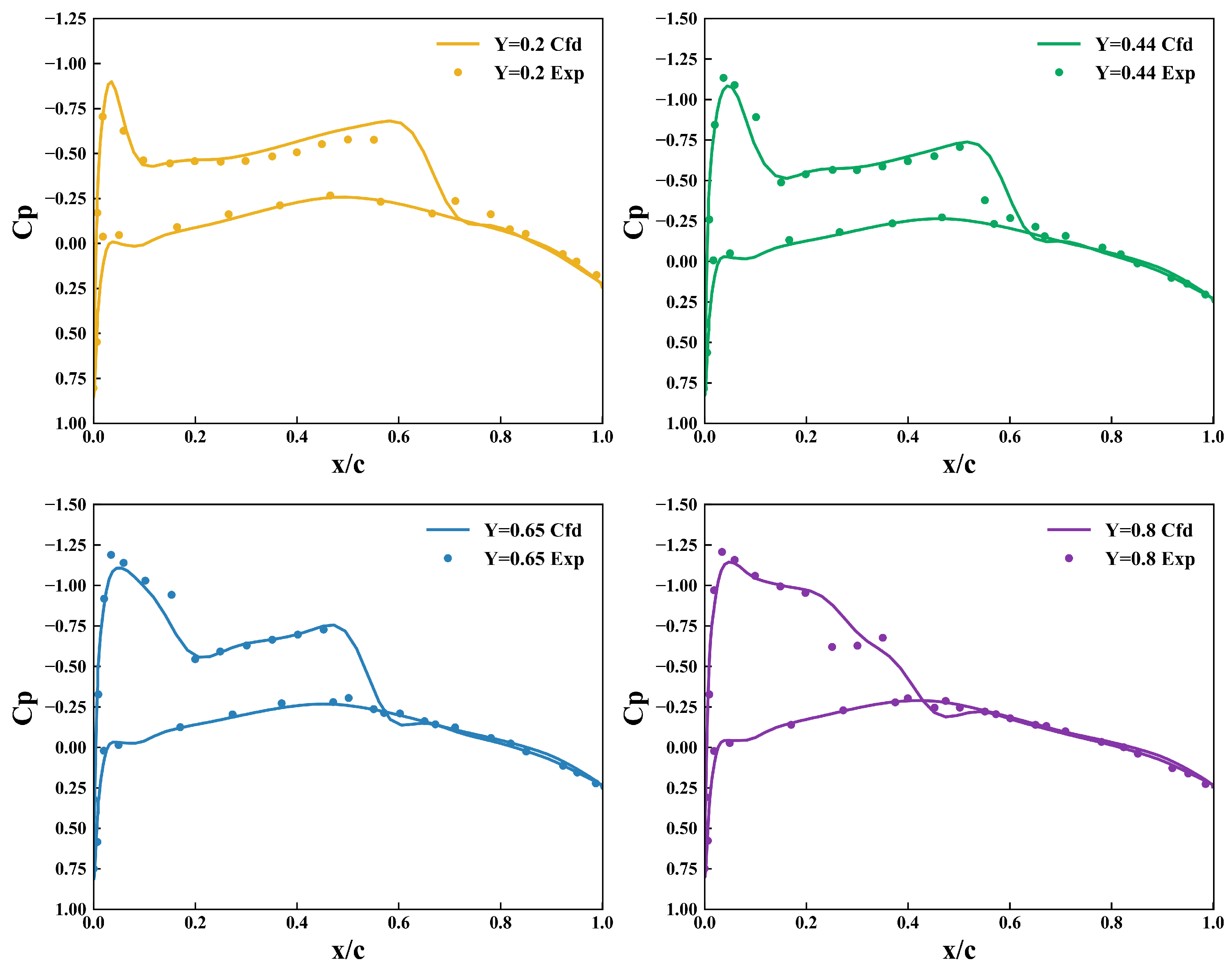
2.2. Validation of Simulation Method
2.3. Discrete Adjoint Equation
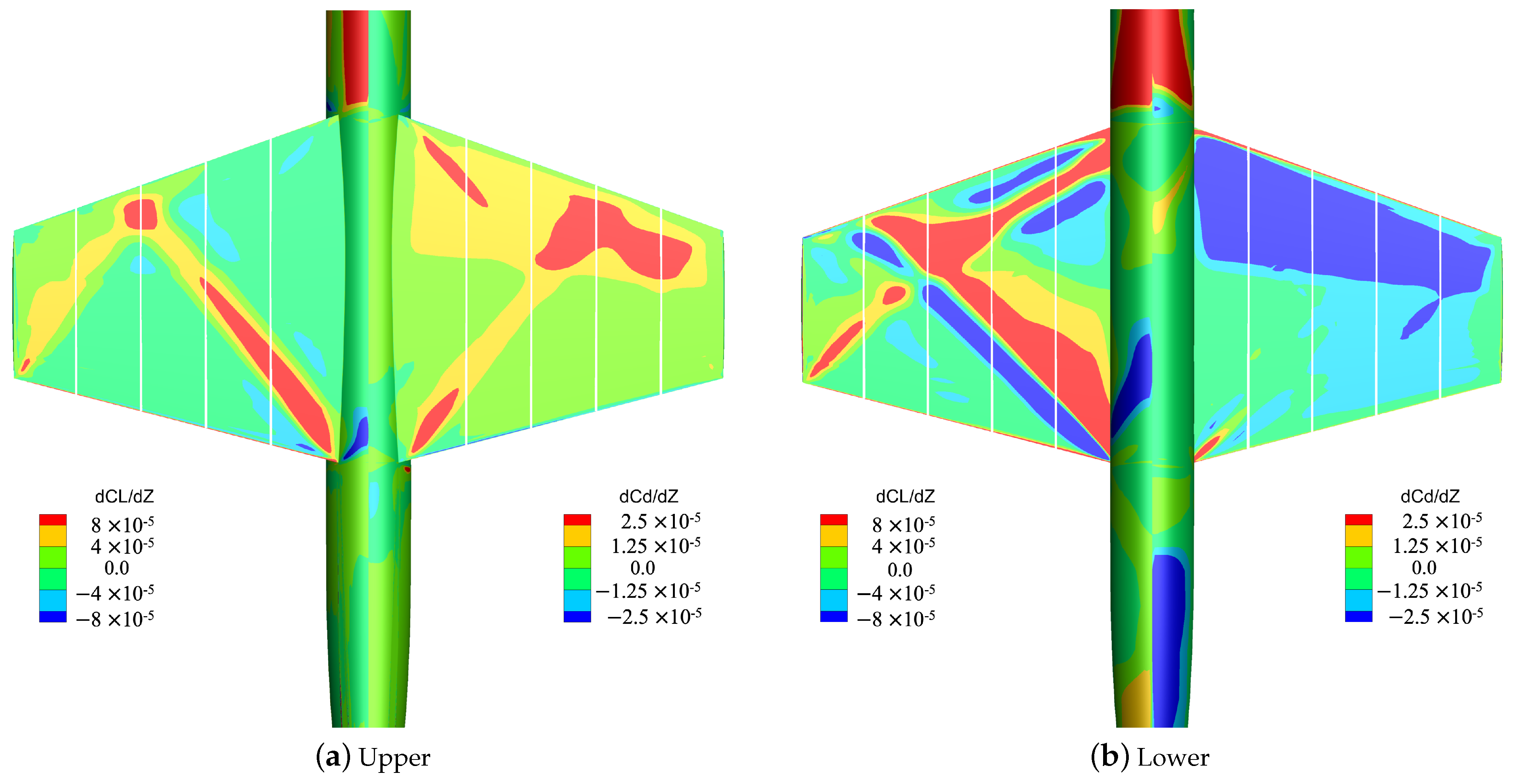
2.4. Gradient Verification
3. Optimization Design Framework for Supersonic Wing
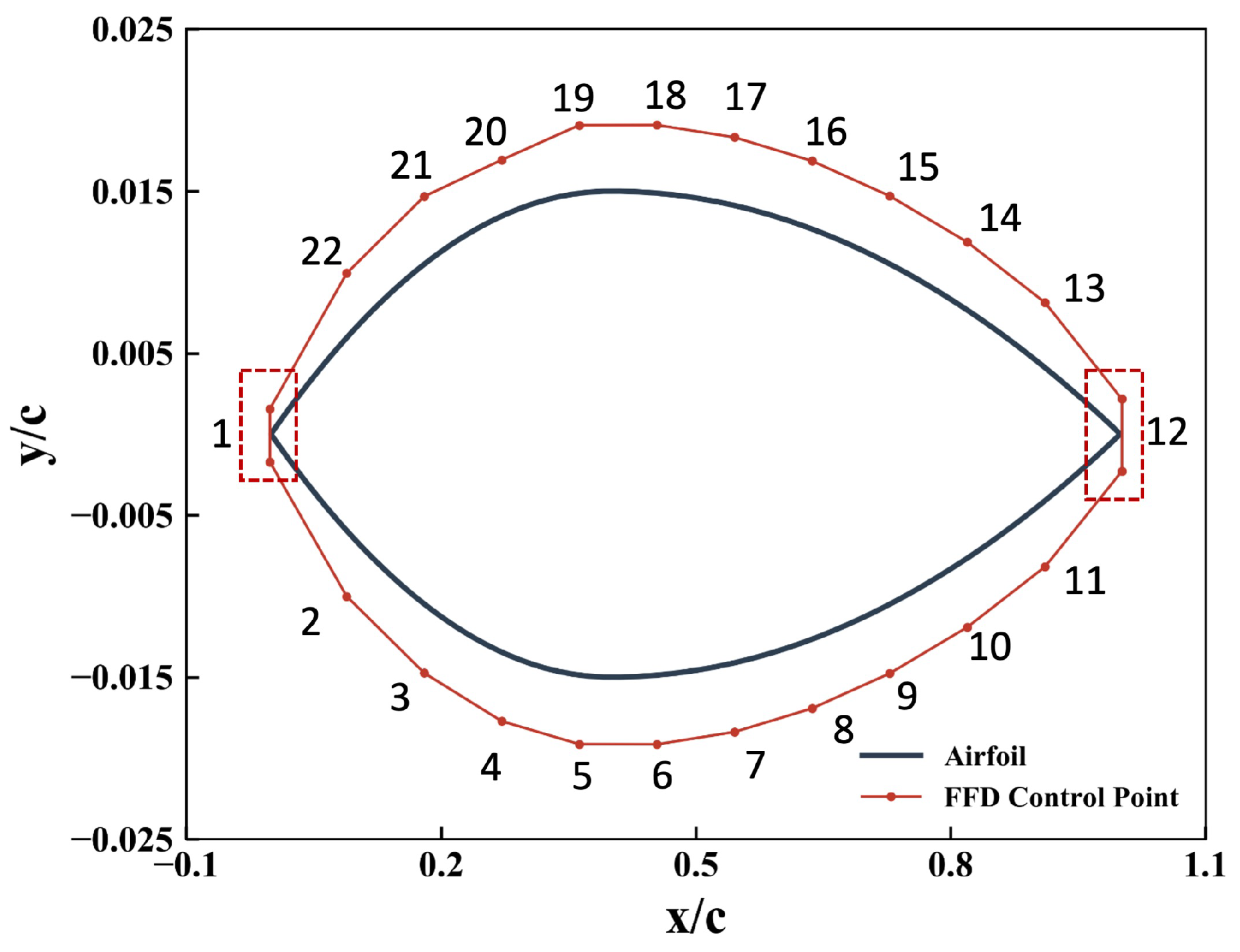
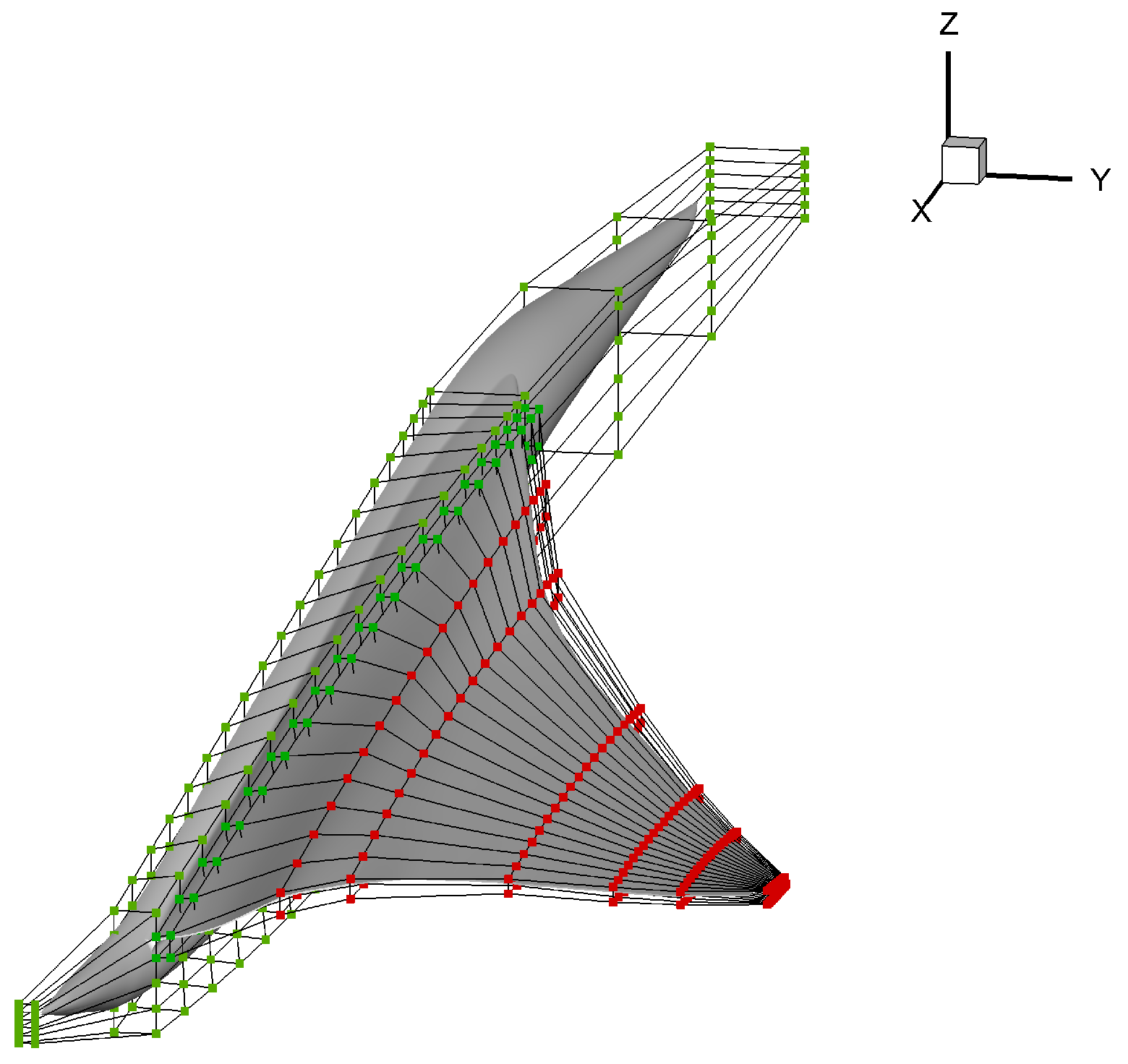
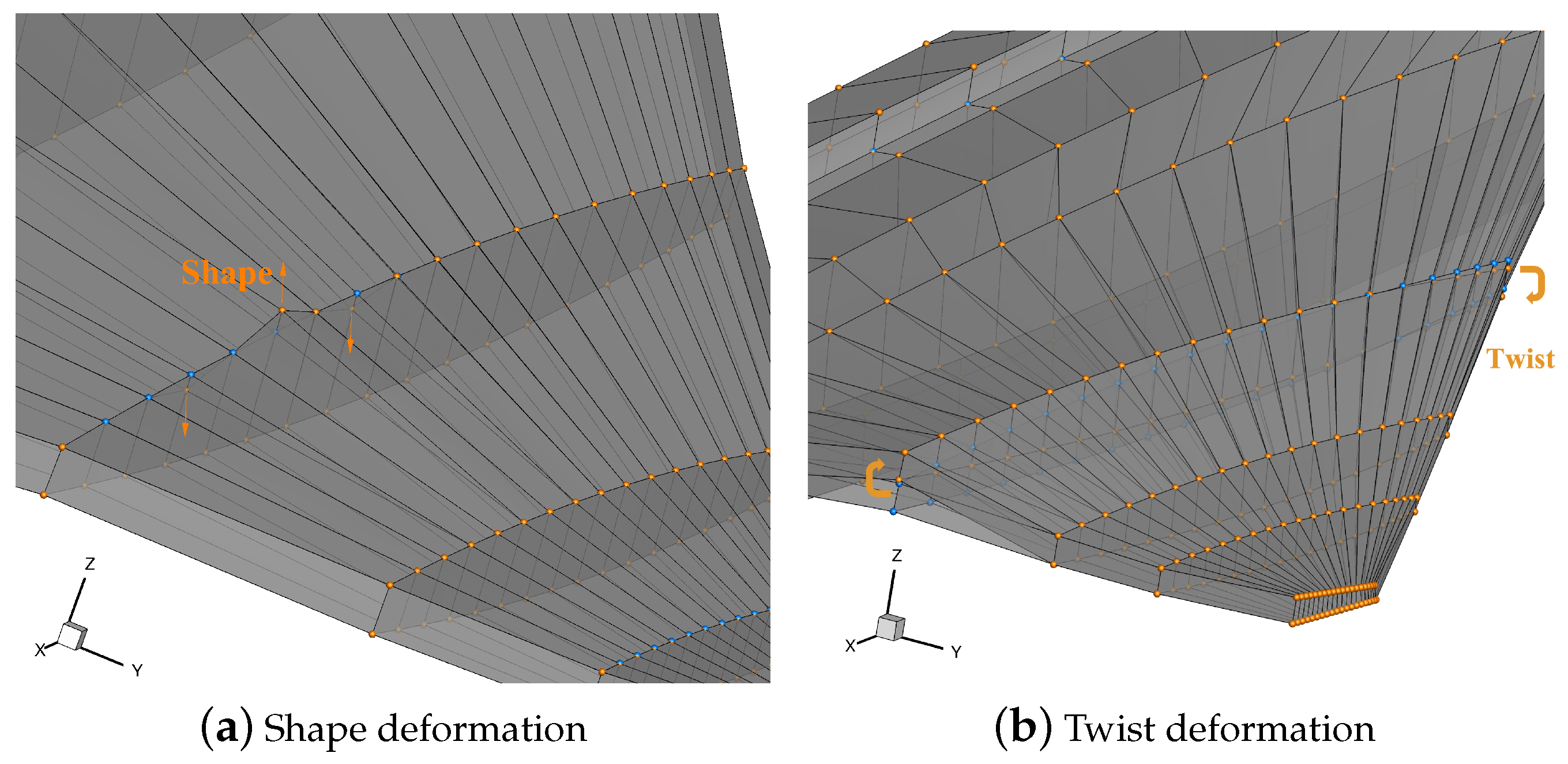
3.1. FFD Geometry Parameterization
3.2. IDW Mesh Warping
3.3. SNOPT Optimization Algorithm
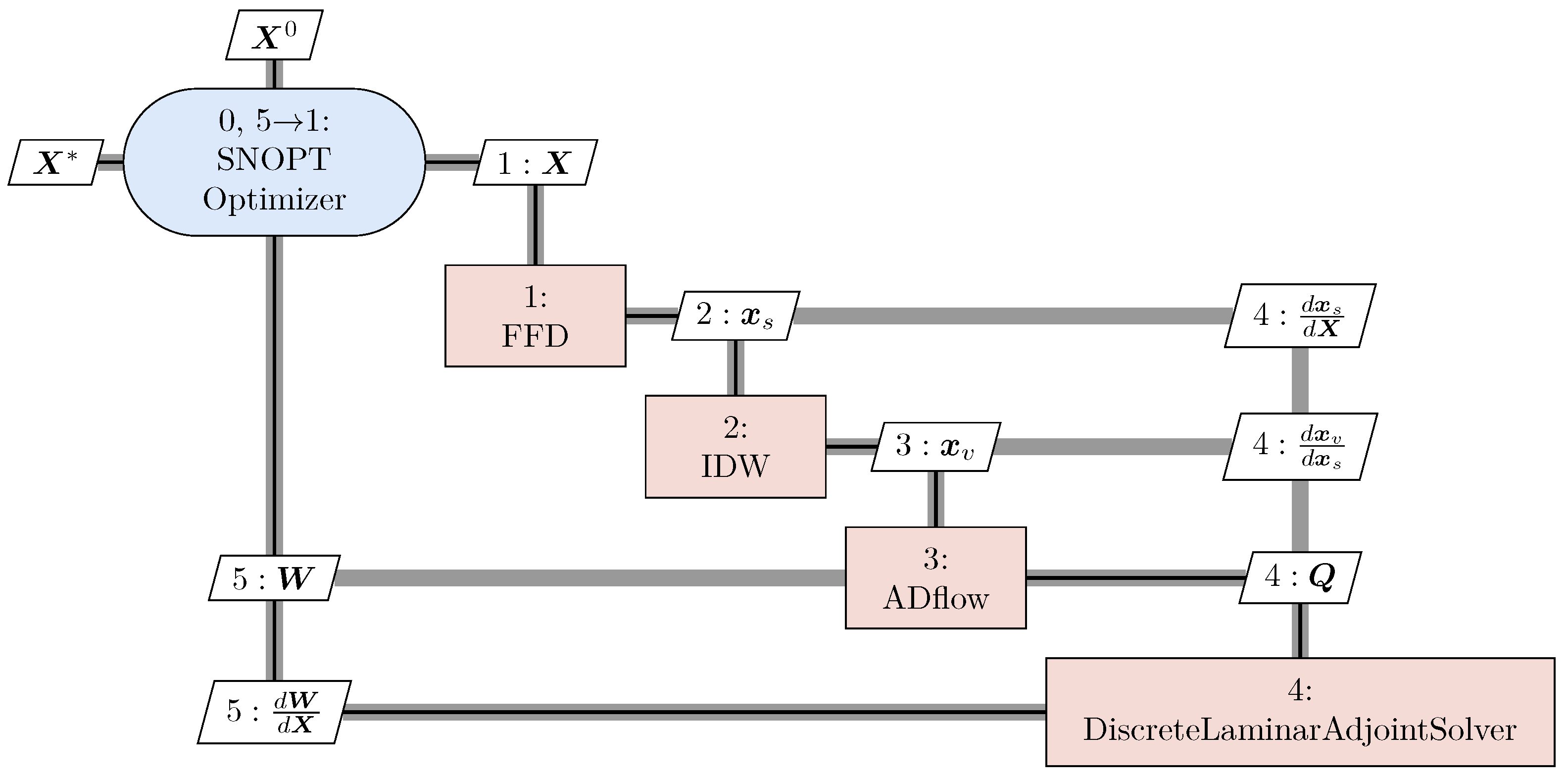
3.4. Optimization Framework
- 1
- Constructing the initial model and the corresponding mesh, and giving the initial design variable ;
- 2
- The surface mesh is parameterized using the FFD method;
- 3
- Based on the deformed surface mesh, the deformed volume mesh is obtained through IDW mesh warping technology;
- 4
- Using ADflow solver to solve the RANS equation to obtain the convergent flow solution vector and the aerodynamic objective function value ;
- 5
- Based on the flow field results, ADflow constructs the adjoint equation and solves it through the GMRES algorithm. Finally, the gradient of the aerodynamic objective function with respect to the design variables is obtained;
- 6
- Combining the calculation results of the flow field in step 4 and the gradient information in step 5, the SNOPT optimization algorithm is used to update the design variables;
- 7
- Repeating steps 2 to 6 until the optimization design converges.
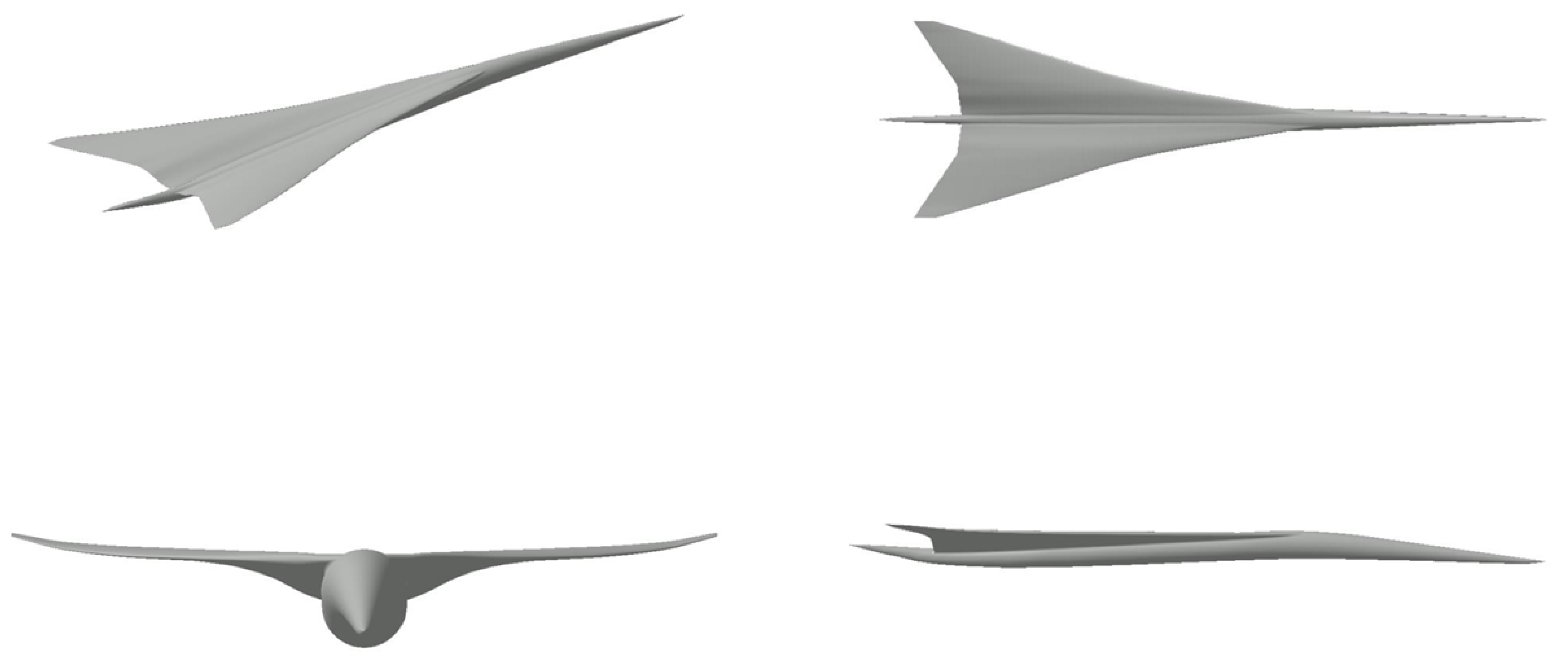
4. Aerodynamic Optimization Design of Subsonic Leading Edge Configuration
4.1. Optimization Problem
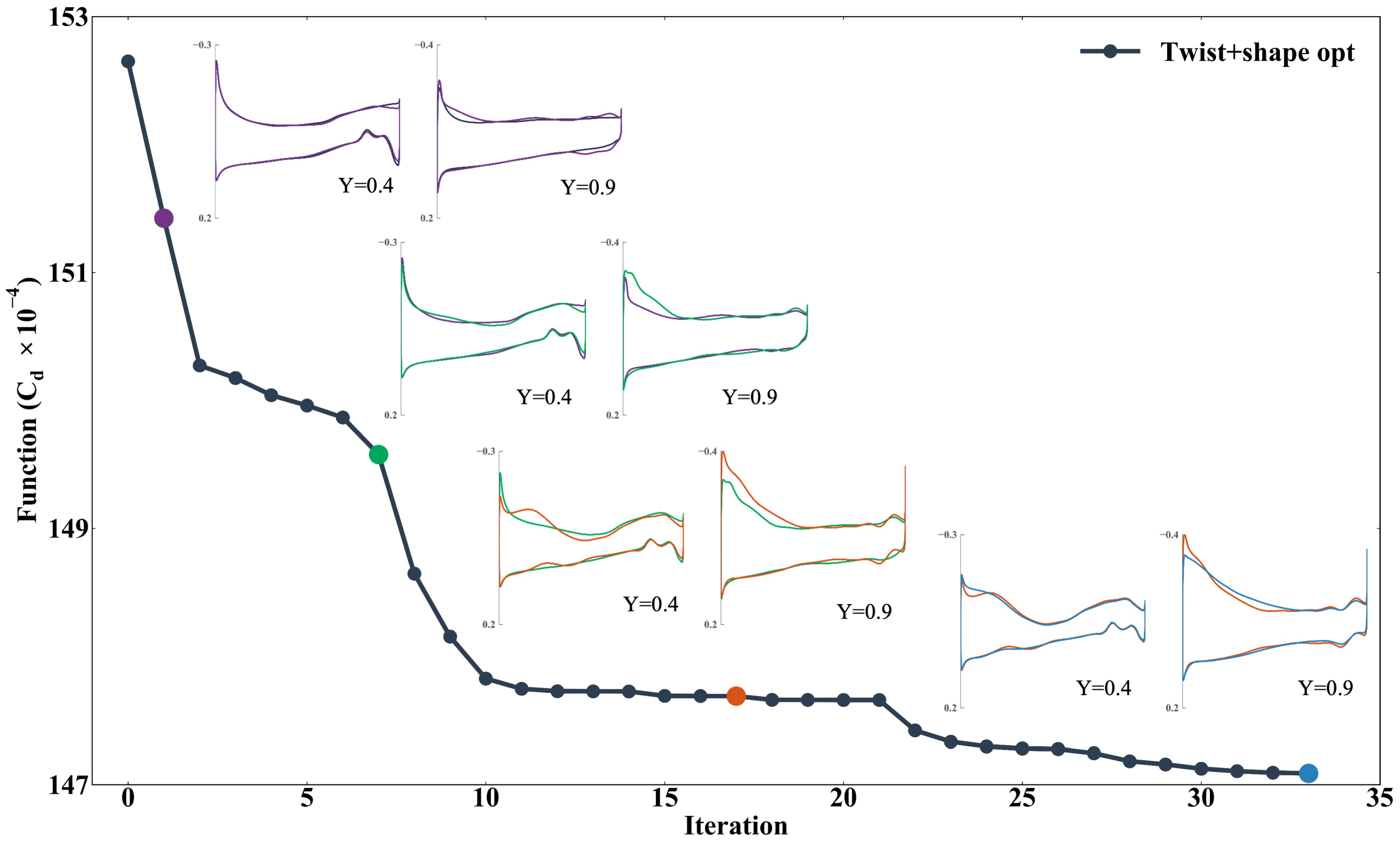
4.2. Optimization Results
5. Aerodynamic Optimization Design of Supersonic Leading Edge Configuration
5.1. Optimization Problem
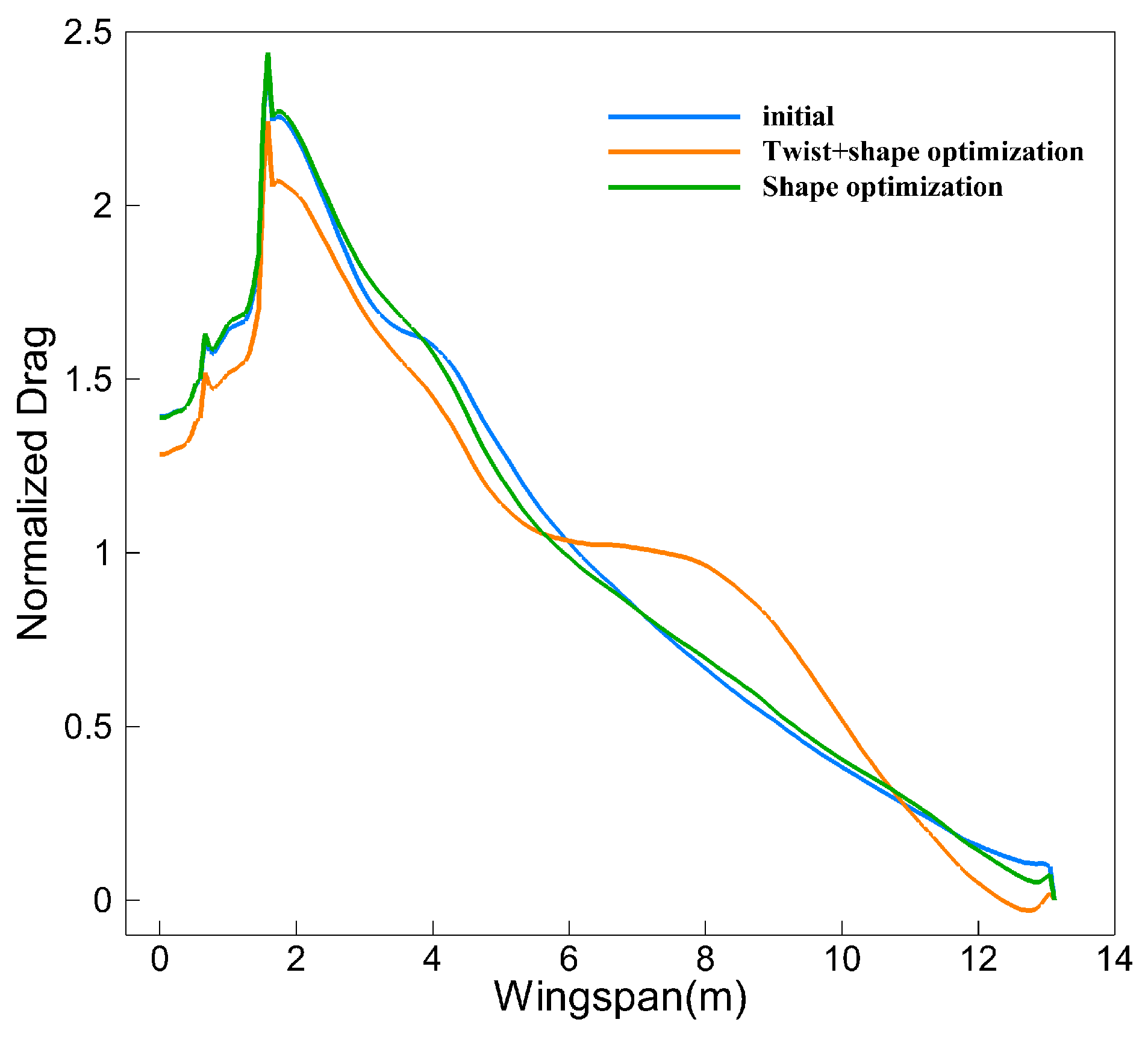
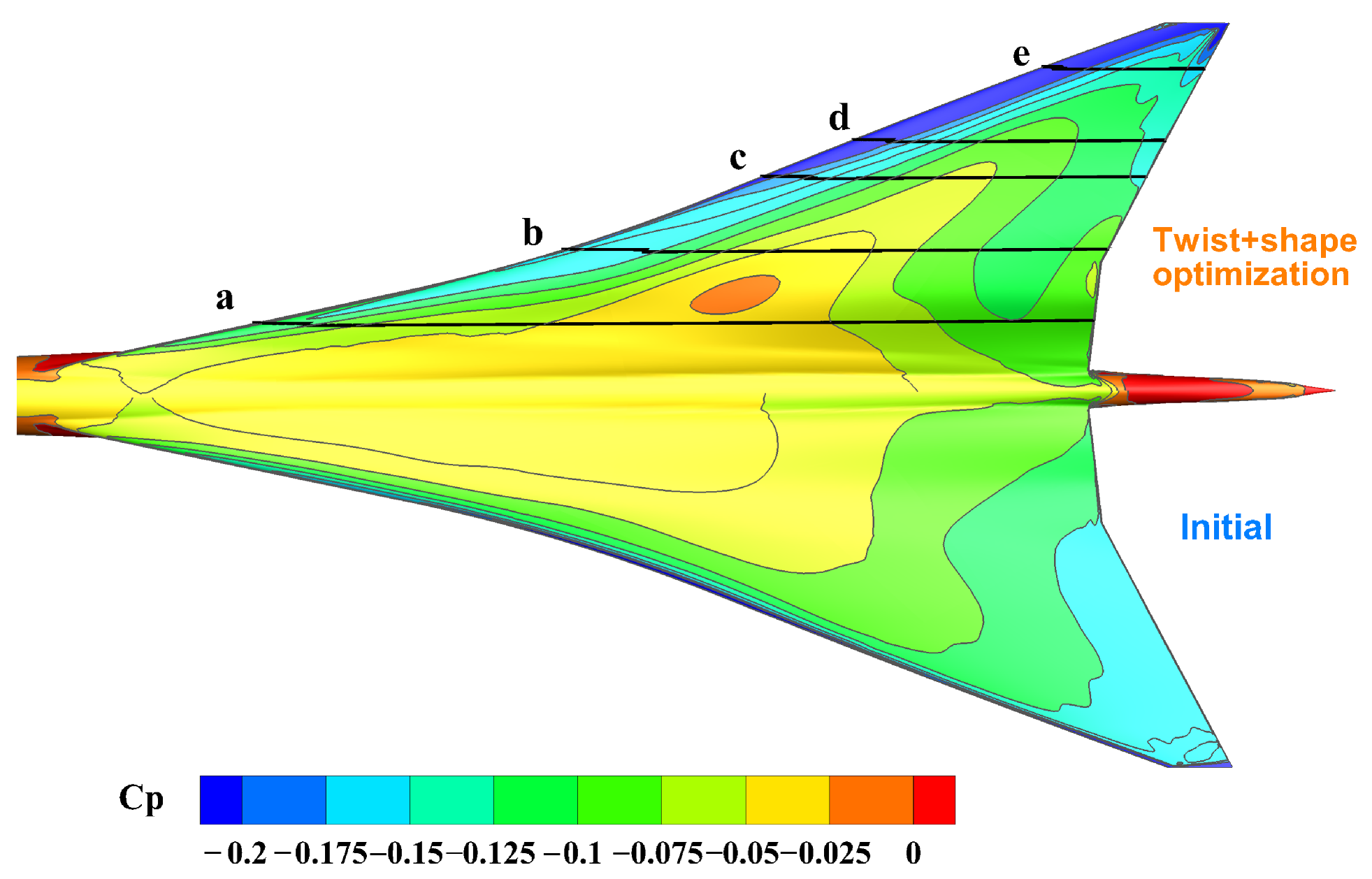
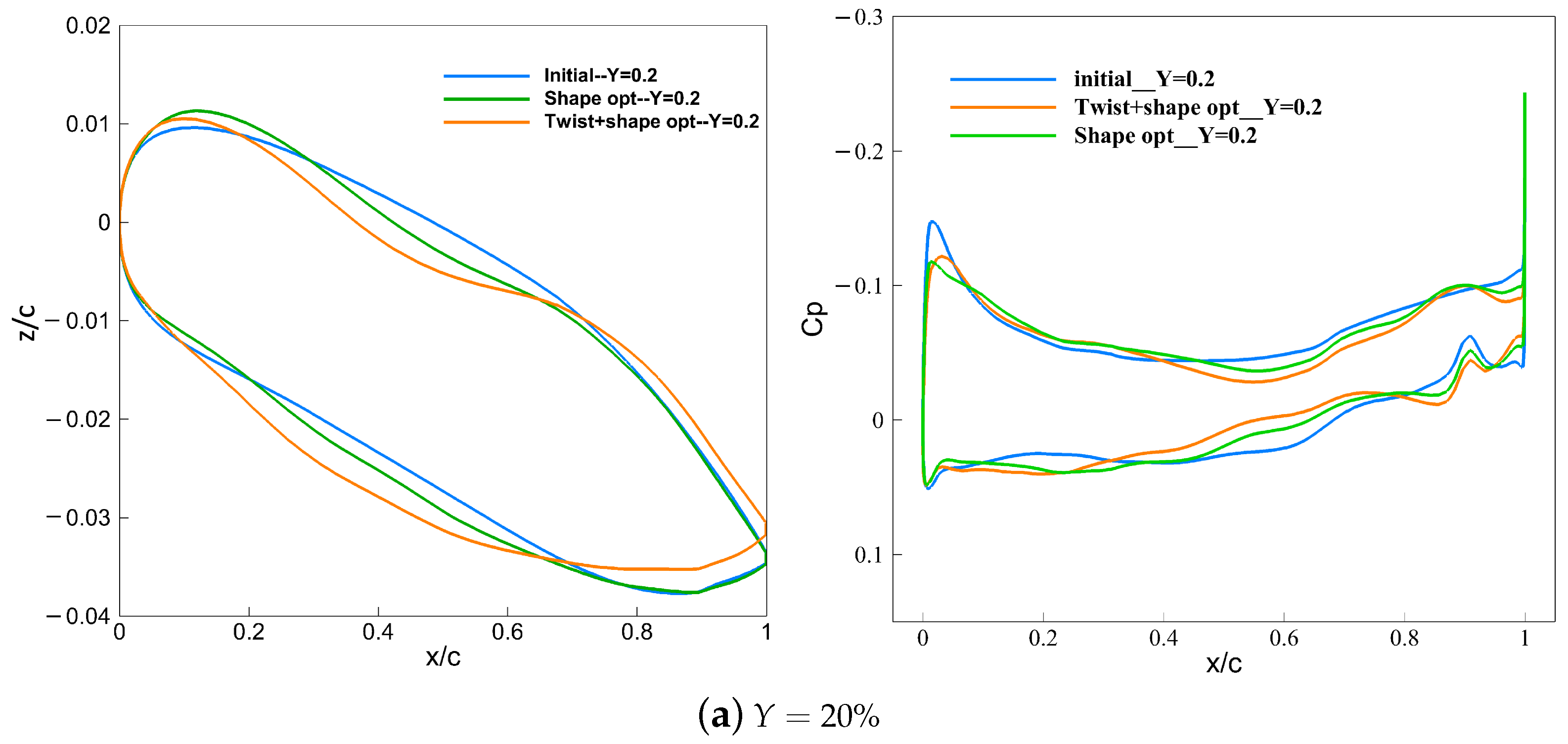
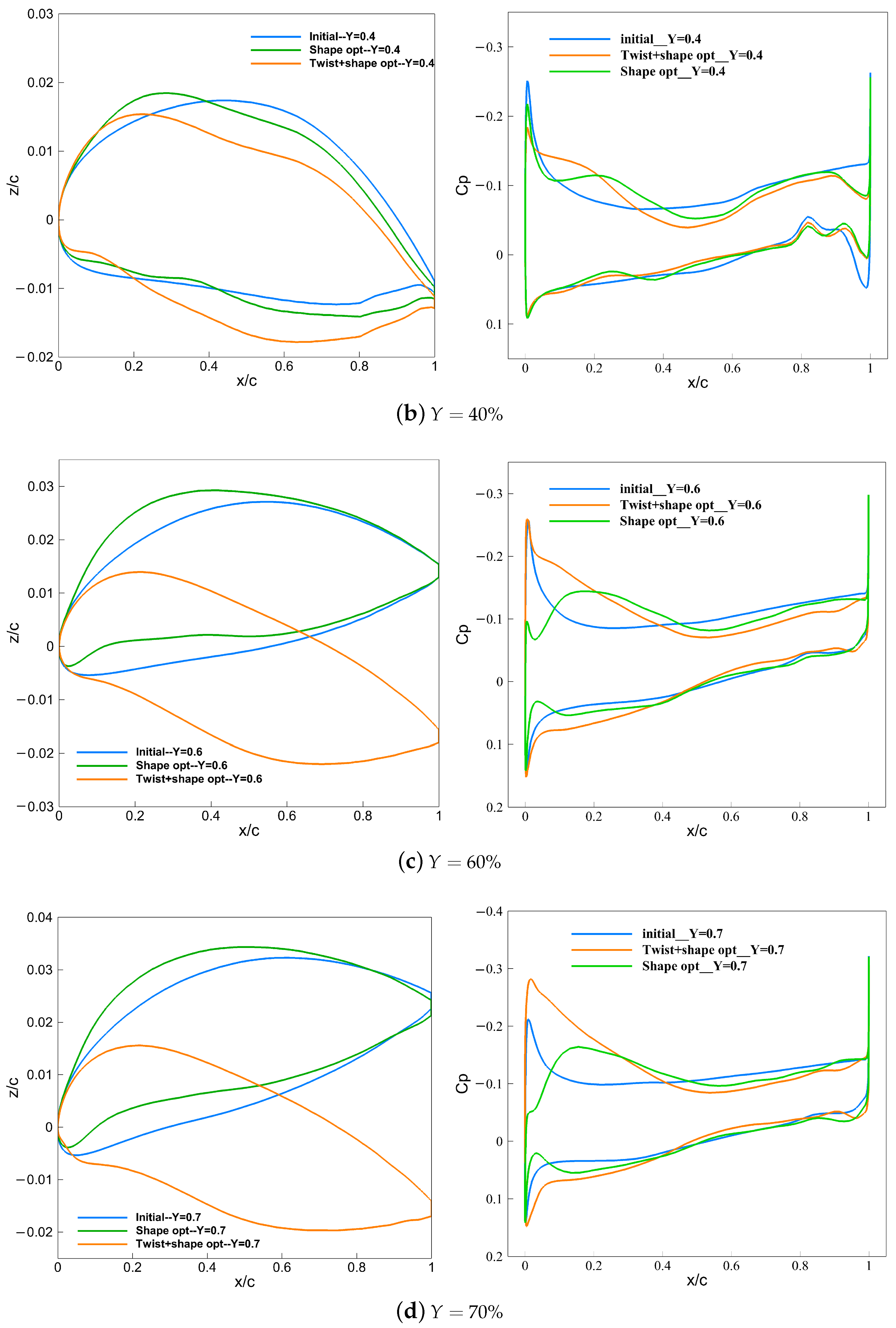
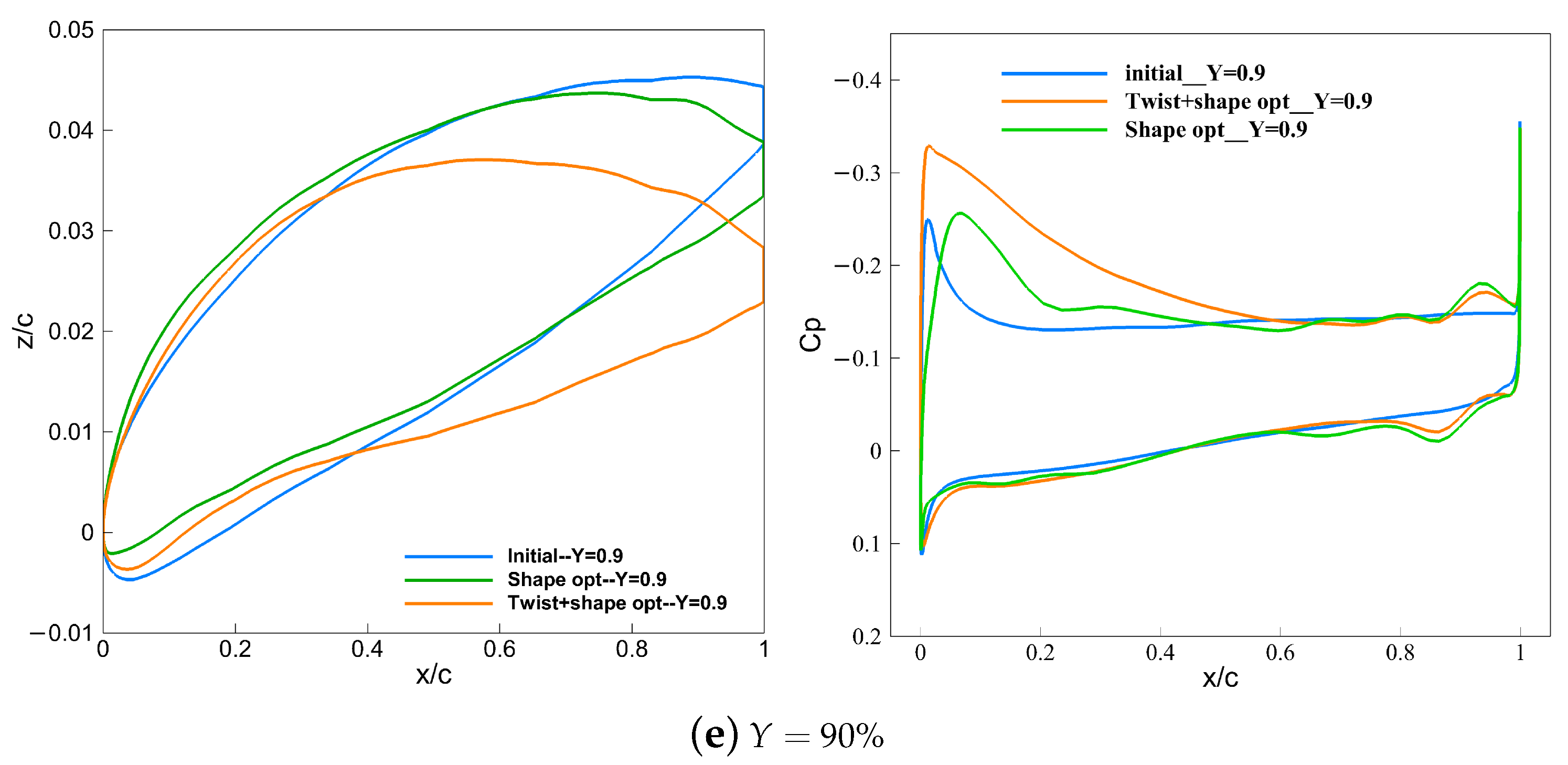
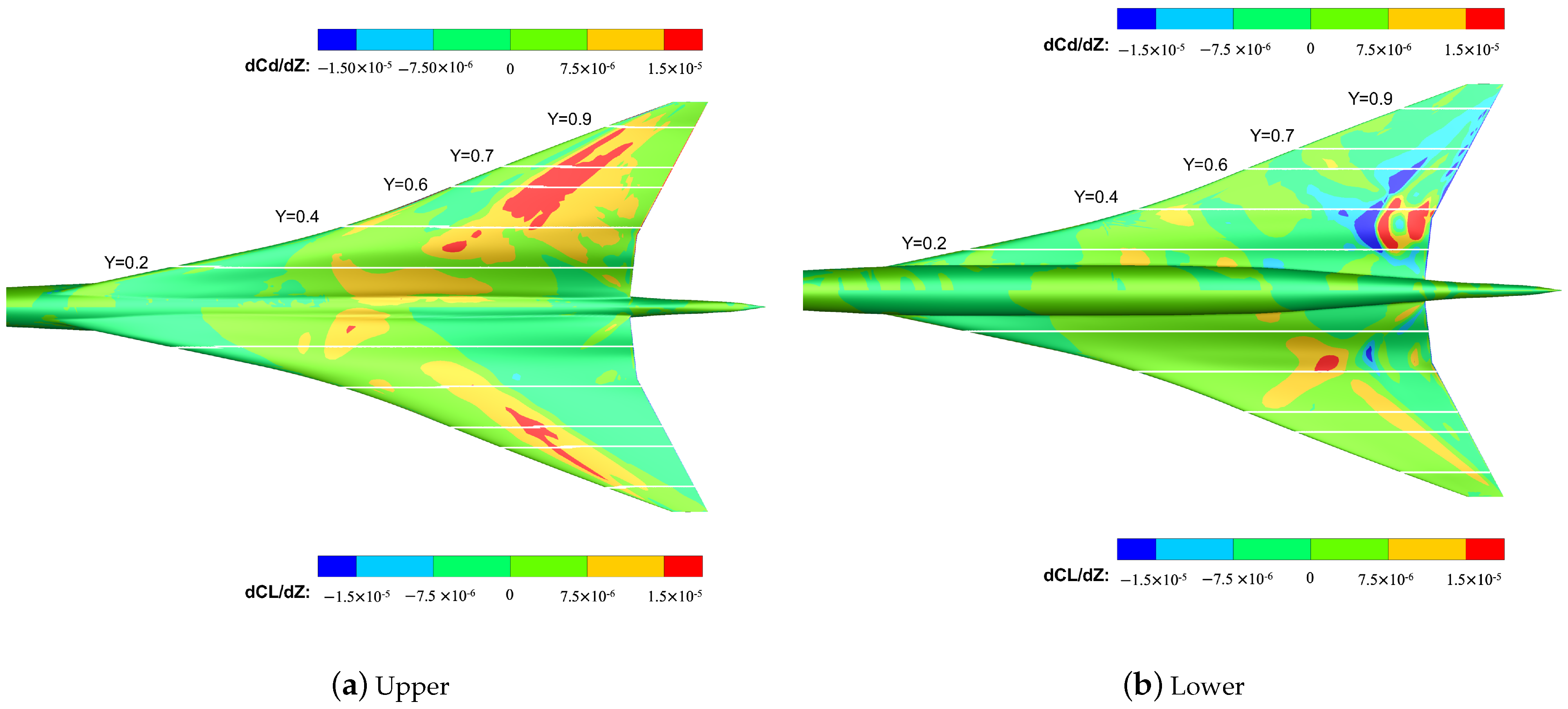
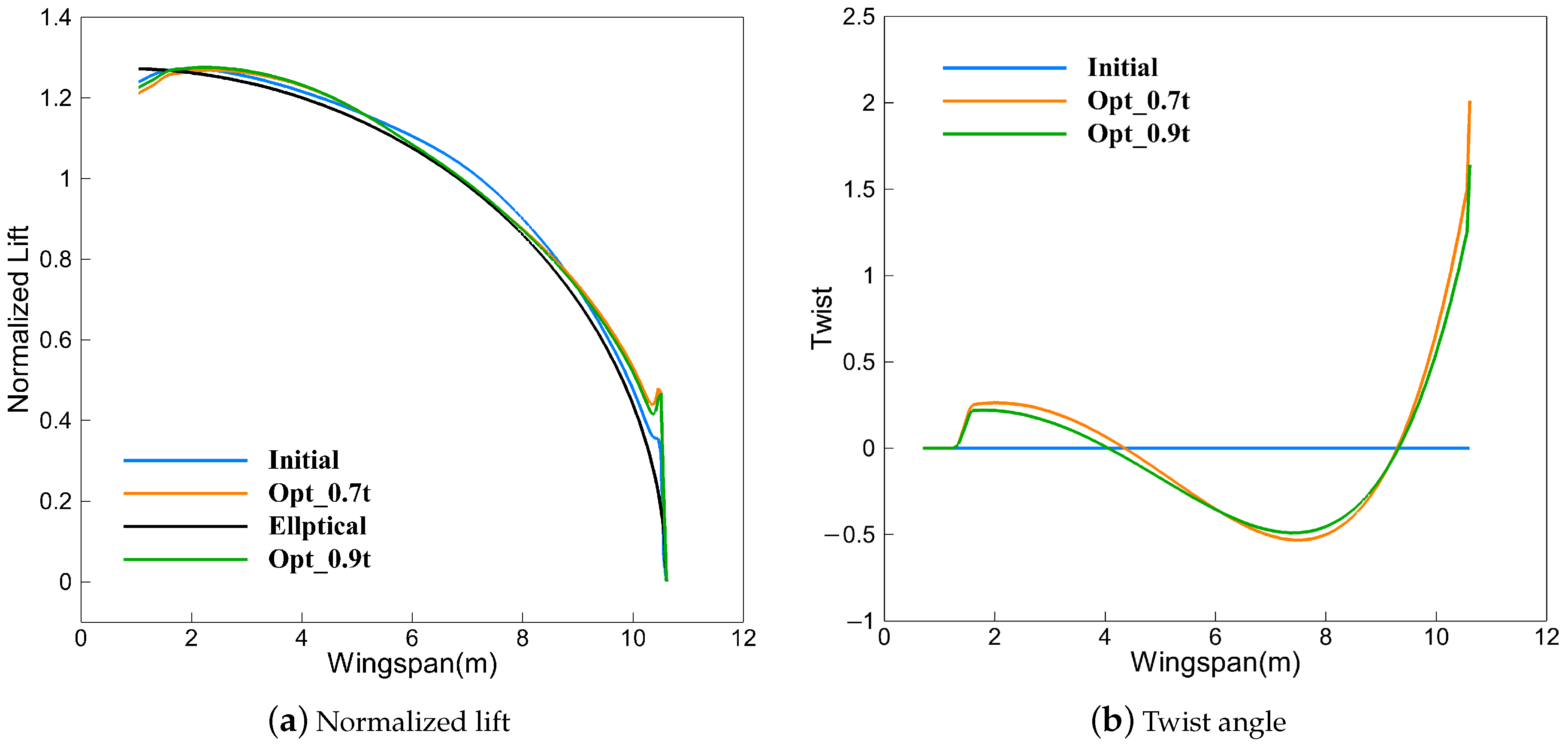
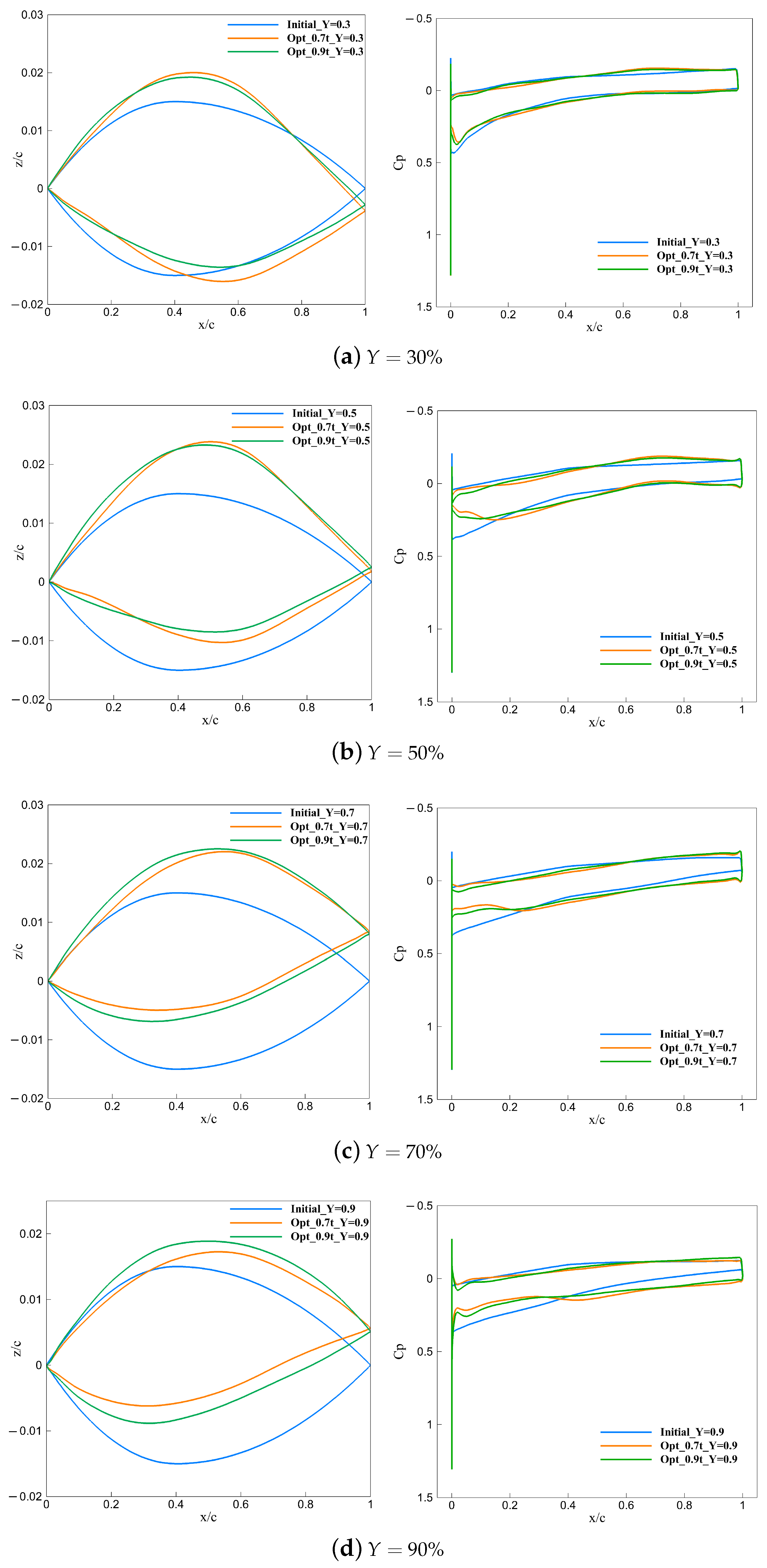
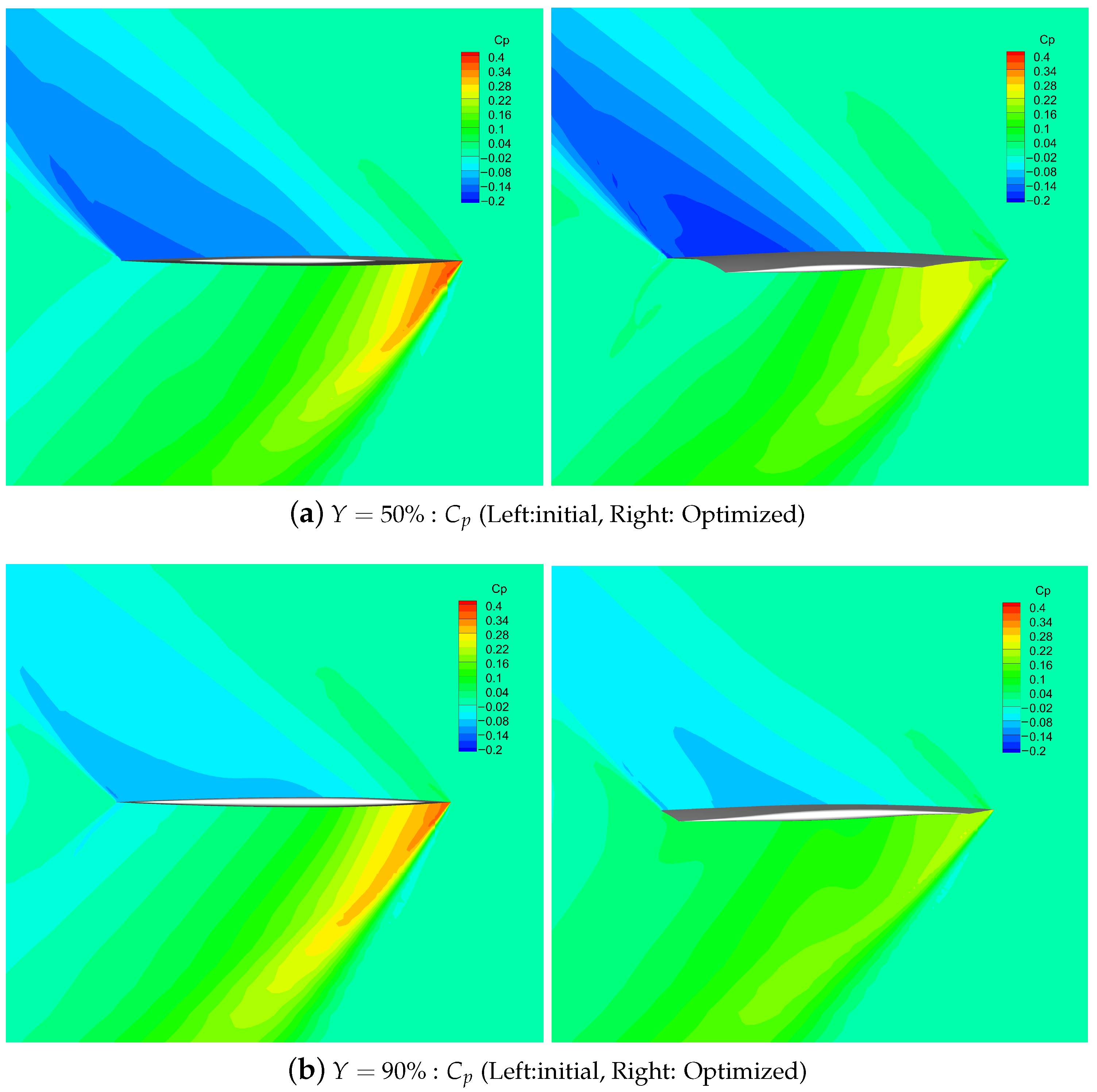
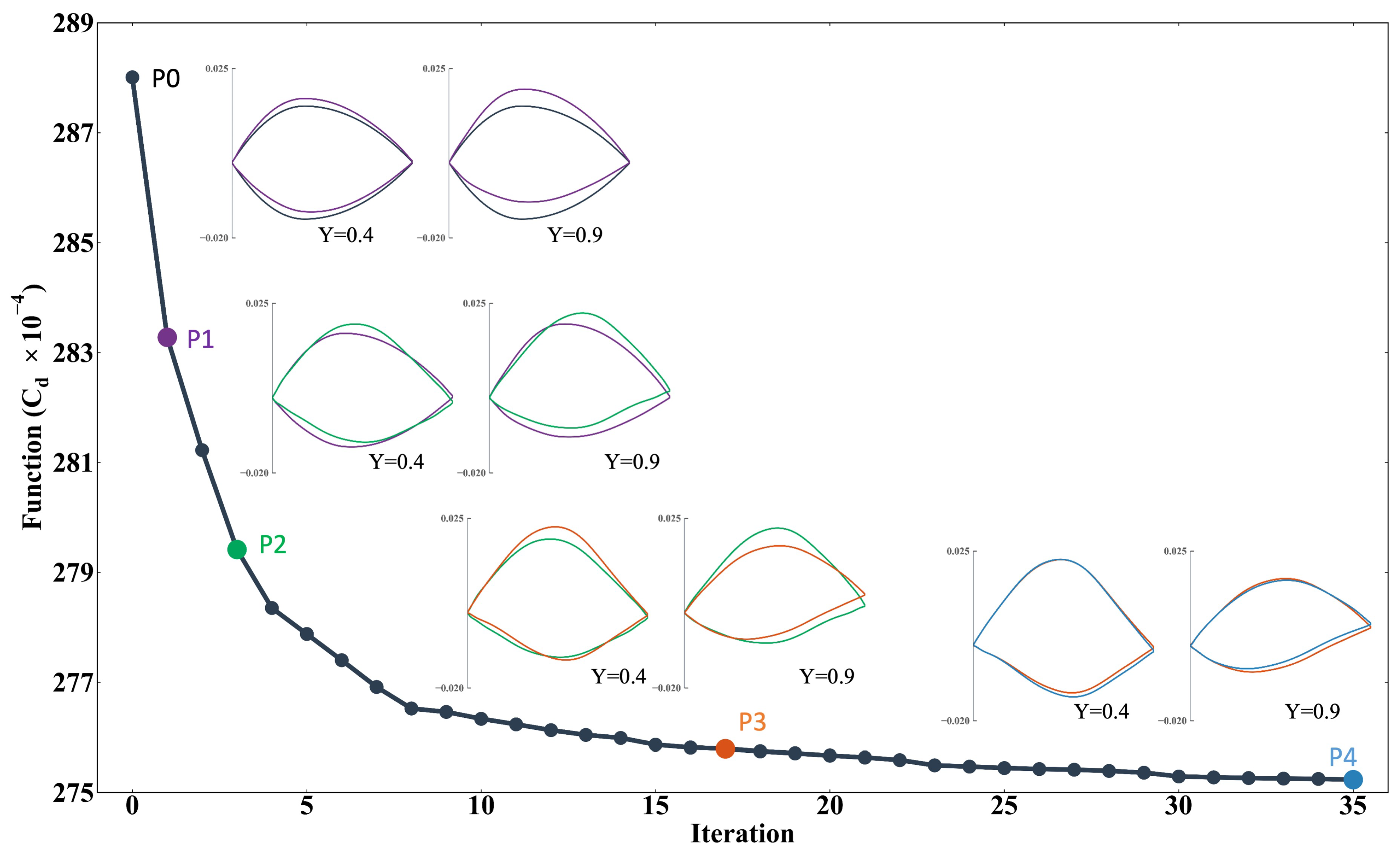
5.2. Optimization Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Han, Z.; Qiao, J.; Ding, Y.; Wang, G.; Song, B.; Song, W. Key technologies for next-generation environmentally-friendly supersonic transport aircraft: A review of recent progress. Acta Aerodyn. 2019, 37, 620–635. (In Chinese) [Google Scholar]
- Ang, H. General layout design analysis of large aircraft. Aviat. Manuf. Technol. 2009, 52, 40–43. (In Chinese) [Google Scholar]
- Gao, P. Civil aircraft design reference model Tu-144 supersonic transport aircraft. Civ. Aircr. Des. Res. 2015, 4. (In Chinese) [Google Scholar] [CrossRef]
- Sun, Y.; Smith, H. Review and prospect of supersonic business jet design. Prog. Aerosp. Sci. 2017, 90, 12–38. [Google Scholar] [CrossRef]
- Pietremont, N.; Deremaux, Y. Executive Public Summary of the Three Preliminary Aircraft Configuration Families; HISAC Publishable Activity Report; HISAC: Ormond Beach, FL, USA, 2005. [Google Scholar]
- Hanai, T.; Yoshida, K.; Usuki, K.; Tamaki, T. Research trend in supersonic transport. J. Jpn. Soc. Aeronaut. Space Sci. 1989, 37, 1–13. [Google Scholar]
- Chen, L.; Yang, X. Research progress and development trend of American supersonic business jet. Aeronaut. Sci. Technol. 2014, 11–15. (In Chinese) [Google Scholar] [CrossRef]
- Jameson, A. Advances in aerodynamic shape optimization. In Computational Fluid Dynamics 2004; Springer: Berlin/Heidelberg, Germany, 2006; pp. 687–698. [Google Scholar]
- Wu, H.; Da, X.; Wang, D.; Huang, X. Multi-Row Turbomachinery Aerodynamic Design Optimization by an Efficient and Accurate Discrete Adjoint Solver. Aerospace 2023, 10, 106. [Google Scholar] [CrossRef]
- Semlitsch, B.; Huscava, A. Shape Optimisation of Turbomachinery Components. In Proceedings of the 8th European Congress on Computational Methods in Applied Sciences and Engineering-ECCOMAS Congress 2022, Oslo, Norway, 5–9 June 2022. [Google Scholar]
- Rao, H.; Chen, Y.; Shi, Y.; Yang, T.; Liu, H. Adjoint-Based Aerodynamic Design Optimization and Drag Reduction Analysis of a Military Transport Aircraft Afterbody. Aerospace 2023, 10, 331. [Google Scholar] [CrossRef]
- Lyu, Z.; Xu, Z.; Martins, J. Benchmarking optimization algorithms for wing aerodynamic design optimization. In Proceedings of the Proceedings of the 8th International Conference on Computational Fluid Dynamics, Chengdu, China, 14–18 July 2014; Volume 11, p. 585. [Google Scholar]
- Mader, C.A.; Martins, J.R.; Alonso, J.J.; Van Der Weide, E. ADjoint: An approach for the rapid development of discrete adjoint solvers. AIAA J. 2008, 46, 863–873. [Google Scholar] [CrossRef]
- Marta, A.; Mader, C.; Martins, J.; Van der Weide, E.; Alonso, J. A methodology for the development of discrete adjoint solvers using automatic differentiation tools. Int. J. Comput. Fluid Dyn. 2007, 21, 307–327. [Google Scholar] [CrossRef]
- Chan, M.K.Y. Supersonic Aircraft Optimization for Minimizing Drag and Sonic Boom; Stanford University: Stanford, CA, USA, 2003. [Google Scholar]
- Choi, S.; Alonso, J.J.; Kroo, I.M.; Wintzer, M. Multifidelity design optimization of low-boom supersonic jets. J. Aircr. 2008, 45, 106–118. [Google Scholar] [CrossRef]
- Kirz, J. Surrogate-Based Low-Boom Low-Drag Nose Design for the JAXA S4 Supersonic Airliner. In Proceedings of the AIAA SCITECH 2022 Forum, San Diego, CA, USA, 3–7 January 2022; p. 0706. [Google Scholar]
- Kiyici, F.; Aradag, S. Design and optimization of a supersonic business jet. In Proceedings of the 22nd AIAA Computational Fluid Dynamics Conference, Dallas, TX, USA, 22–26 June 2015; p. 3064. [Google Scholar]
- Li, L.; Bai, J.; Guo, T.; Fu, Z.; Chen, S. Aerodynamic optimization design of supersonic airliner wing based on adjoint method. J. Northwestern Polytech. Univ. 2017, 35, 843–849. (In Chinese) [Google Scholar]
- Liu, S.; Bai, J.; Yu, P.; Chen, B.; Zhou, B. Aerodynamic optimization design of supersonic airliner considering sonic boom characteristics. J. Northwestern Polytech. Univ. 2020, 38, 271. (In Chinese) [Google Scholar] [CrossRef]
- Liu, B.; Hao, H.; Li, D.; Liang, Y. Adjoint optimization considering both aerodynamic and near-field sonic boom characteristics. Acta Aerodyn. Sin. 2022, 40, 1–11. (In Chinese) [Google Scholar]
- Seraj, S.; Martins, J.R. Aerodynamic Shape Optimization of a Supersonic Transport Considering Low-Speed Stability. In Proceedings of the AIAA Scitech 2022 Forum, San Diego, CA, USA, 3–7 January 2022; p. 2177. [Google Scholar]
- Bons, N.; Martins, J.R.; Mader, C.A.; McMullen, M.S.; Suen, M. High-fidelity aerostructural optimization studies of the Aerion AS2 supersonic business jet. In Proceedings of the AIAA Aviation 2020 Forum, Online, 15–19 June 2020; p. 3182. [Google Scholar]
- Morgenstern, J.; Norstrud, N.; Sokhey, J.; Martens, S.; Alonso, J.J. Advanced Concept Studies for Supersonic Commercial Transports Entering Service in the 2018 to 2020 Period; Technical Report; Lockheed Martin Corporation: Palmdale, CA, USA, 2013. [Google Scholar]
- Mangano, M.; Martins, J.R. Multipoint aerodynamic shape optimization for subsonic and supersonic regimes. J. Aircr. 2021, 58, 650–662. [Google Scholar] [CrossRef]
- Kenway, G.K.; Mader, C.A.; He, P.; Martins, J.R. Effective adjoint approaches for computational fluid dynamics. Prog. Aerosp. Sci. 2019, 110, 100542. [Google Scholar] [CrossRef]
- Spalart, P.; Allmaras, S. A one-equation turbulence model for aerodynamic flows. In Proceedings of the 30th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1992; p. 439. [Google Scholar] [CrossRef]
- Jameson, A.; Schmidt, W.; Turkel, E. Numerical solution of the Euler equations by finite volume methods using Runge Kutta time stepping schemes. In Proceedings of the 14th Fluid and Plasma Dynamics Conference, Palo Alto, CA, USA, 23–25 June 1981; p. 1259. [Google Scholar]
- Klopfer, G.; Hung, C.; Van der Wijngaart, R.; Onufer, J. A diagonalized diagonal dominant alternating direction implicit (D3ADI) scheme and subiteration correction. In Proceedings of the 29th AIAA, Fluid Dynamics Conference, Albuquerque, NM, USA, 15–18 June 1998; p. 2824. [Google Scholar]
- Biros, G.; Ghattas, O. Parallel Lagrange–Newton–Krylov–Schur methods for PDE-constrained optimization. Part I: The Krylov–Schur solver. SIAM J. Sci. Comput. 2005, 27, 687–713. [Google Scholar] [CrossRef]
- Knoll, D.A.; Keyes, D.E. Jacobian-free Newton–Krylov methods: A survey of approaches and applications. J. Comput. Phys. 2004, 193, 357–397. [Google Scholar] [CrossRef]
- Yildirim, A.; Kenway, G.K.; Mader, C.A.; Martins, J.R. A Jacobian-free approximate Newton–Krylov startup strategy for RANS simulations. J. Comput. Phys. 2019, 397, 108741. [Google Scholar] [CrossRef]
- Yoon, S.; Jameson, A. Lower-upper symmetric-Gauss-Seidel method for the Euler and Navier-Stokes equations. AIAA J. 1988, 26, 1025–1026. [Google Scholar] [CrossRef]
- Mayeur, J.; Dumont, A.; Destarac, D.; Gleize, V. Reynolds-averaged Navier–Stokes simulations on NACA0012 and ONERA-M6 wing with the ONERA elsA solver. AIAA J. 2016, 54, 2671–2687. [Google Scholar] [CrossRef]
- Jameson, A. Aerodynamic Shape Optimization Using the Adjoint Method; Von Karman Institute: Brussels, Belgium, 2003. [Google Scholar]
- Mavriplis, D.J. Discrete adjoint-based approach for optimization problems on three-dimensional unstructured meshes. AIAA J. 2007, 45, 741–750. [Google Scholar] [CrossRef]
- He, P.; Mader, C.A.; Martins, J.R.; Maki, K.J. An aerodynamic design optimization framework using a discrete adjoint approach with OpenFOAM. Comput. Fluids 2018, 168, 285–303. [Google Scholar] [CrossRef]
- Rashad, R.; Zingg, D.W. Aerodynamic shape optimization for natural laminar flow using a discrete-adjoint approach. AIAA J. 2016, 54, 3321–3337. [Google Scholar] [CrossRef]
- Shi, Y.; Mader, C.A.; He, S.; Halila, G.L.; Martins, J.R. Natural laminar-flow airfoil optimization design using a discrete adjoint approach. AIAA J. 2020, 58, 4702–4722. [Google Scholar] [CrossRef]
- Kenway, G.K.; Martins, J.R. Multipoint high-fidelity aerostructural optimization of a transport aircraft configuration. J. Aircr. 2014, 51, 144–160. [Google Scholar] [CrossRef]
- Albring, T.; Sagebaum, M.; Gauger, N.R. New Results in Numerical and Experimental Fluid Mechanics X: Contributions to the 19th STAB/DGLR Symposium Munich, Germany, 2014; Chapter A Consistent and Robust Discrete Adjoint Solver for the SU2 Framework—Validation and Application; Springer International Publishing: Cham, Switzerland, 2016; pp. 77–86. [Google Scholar] [CrossRef]
- Othmer, C. A continuous adjoint formulation for the computation of topological and surface sensitivities of ducted flows. Int. J. Numer. Methods Fluids 2008, 58, 861–877. [Google Scholar] [CrossRef]
- Andreoli, M.; Ales, J.; Désidéri, J.A. Free-Form-Deformation Parameterization for Multilevel 3D Shape Optimization in Aerodynamics. Ph.D. Thesis, Institute National de Recherche en Informatique et en Automatique, INRIA, Sophia Antipolis, France, 2003. [Google Scholar]
- Luke, E.; Collins, E.; Blades, E. A fast mesh deformation method using explicit interpolation. J. Comput. Phys. 2012, 231, 586–601. [Google Scholar] [CrossRef]
- Roache, P.J.; Ghia, K.N.; White, F.M. Editorial policy statement on the control of numerical accuracy. J. Fluids Eng. 1986, 108, 2. [Google Scholar] [CrossRef]
- Roache, P.J. A method for uniform reporting of grid refinement studies. ASME-Publ.-Fed 1993, 158, 109. [Google Scholar] [CrossRef]

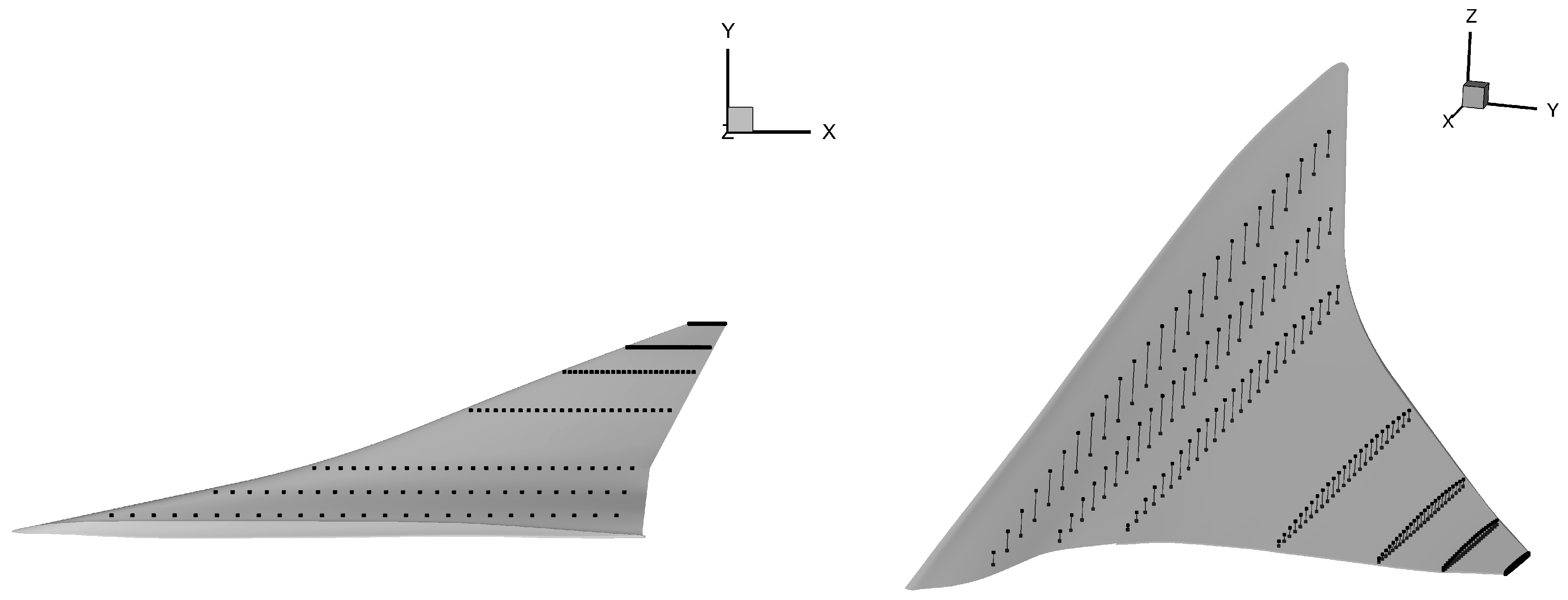









| Var | Finite Difference | Adjoint Equation | Rel. Error | |
|---|---|---|---|---|
| 4 | 0.0024191310 | 0.0024183648 | ||
| 6 | 0.0018757146 | 0.0018757950 | ||
| 9 | 0.0008184027 | 0.0008184097 | ||
| 16 | −0.0007589824 | −0.0007589494 | ||
| 20 | −0.0018793665 | −0.0018790061 |
| Var | Finite Difference | Adjoint Equation | Rel. Error | |
|---|---|---|---|---|
| 4 | −0.0466292505 | −0.0466005468 | ||
| 6 | −0.0361944061 | −0.0361960611 | ||
| 9 | −0.0157922613 | −0.0157923472 | ||
| 16 | 0.0146452139 | 0.0146445737 | ||
| 20 | 0.0362649685 | 0.0362579965 |
| Parameters | Value |
|---|---|
| Length of fuselage (m) | 70.1 |
| Semi-span (m) | 12.8 |
| Reference semi-area () | 153.2 |
| Average aerodynamic chord length (m) | 21.0 |
| Sweep angle of inner wing section () | 78 |
| Sweep angle of outer wing section () | 69 |
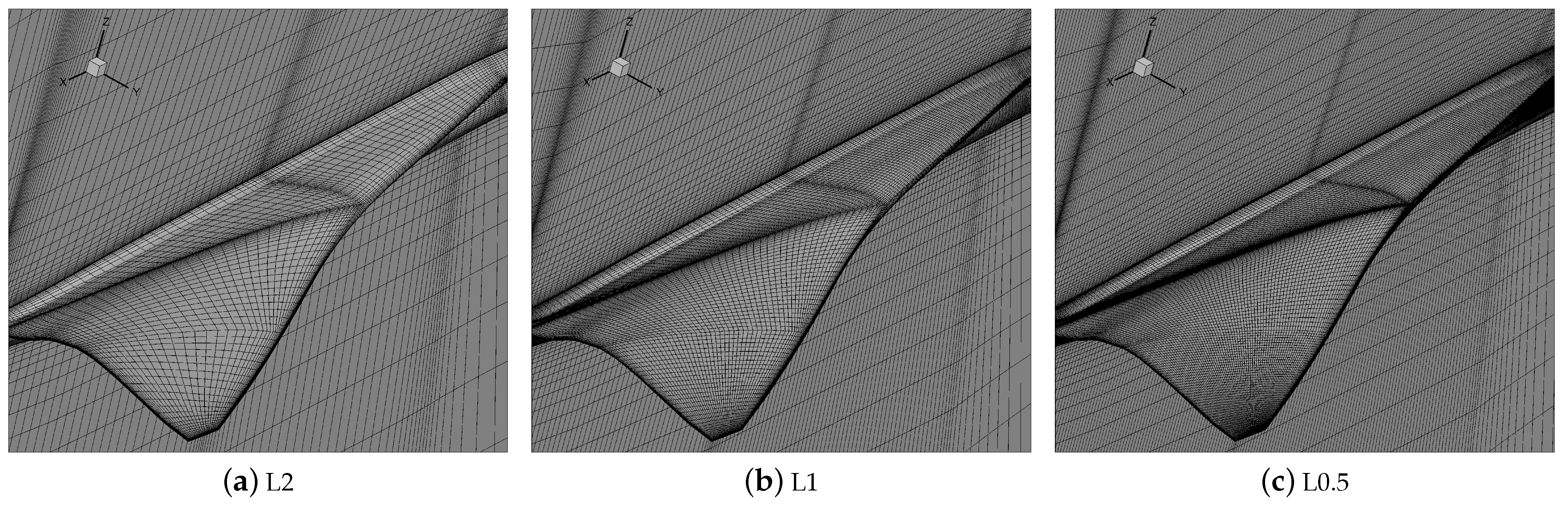
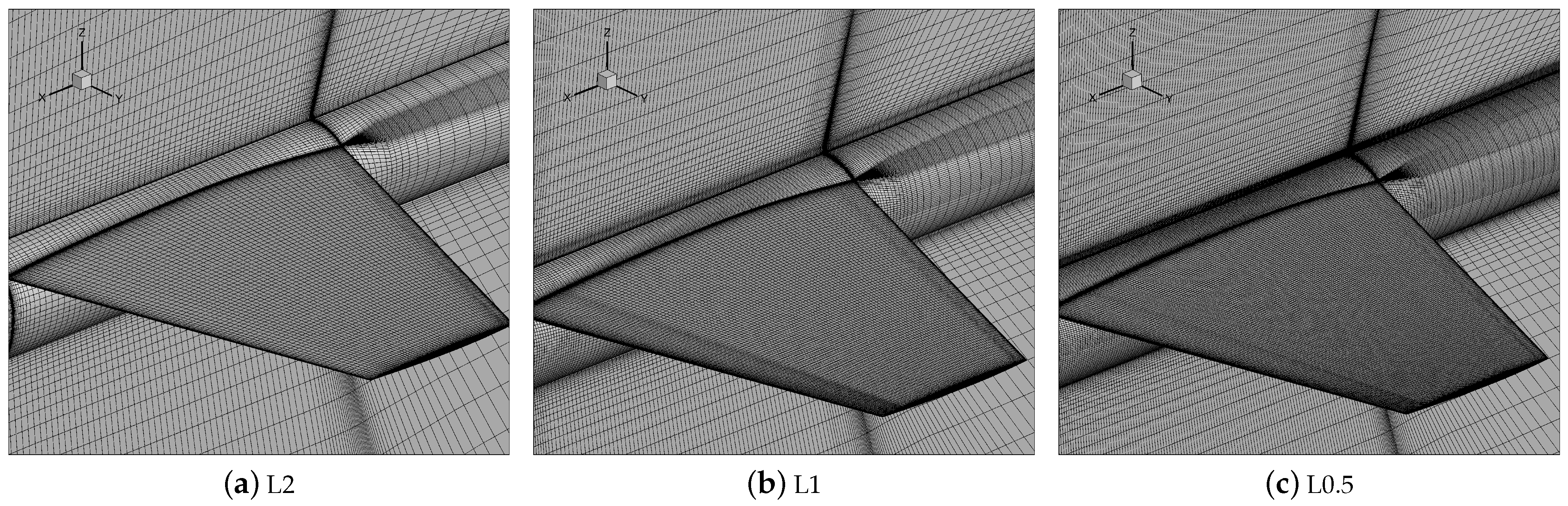
| Grid Level | Grid Size (Million) | (Counts) | GCI/% | Order p |
|---|---|---|---|---|
| L2 | 1.3 | 160.88 | / | |
| L1 | 5.3 | 152.87 | 1.488 | |
| L0.5 | 22.6 | 151.39 | 0.266 | 3.57 |
| Category | Name | Quantity |
|---|---|---|
| Objective | min | 1 |
| Design Variables | Shape | 240 |
| Twist | 5 | |
| AoA | 1 | |
| Constraints | = 0.142 | 1 |
| 1 | ||
| 1 | ||
| 1 |
| Configuration | (Counts) | (Counts) | (Counts) | AoA (Degree) | |||
|---|---|---|---|---|---|---|---|
| Initial | 0.142 | 152.87 | / | 86.88 | 66.00 | 2.15 | 0.0039 |
| Twist + Shape | 0.142 | 147.09 | −5.78 | 81.32 | 65.77 | 1.85 | 0.0040 |
| Shape | 0.142 | 149.00 | −3.87 | 83.06 | 65.94 | 2.04 | 0.0040 |
| Parameters | Value |
|---|---|
| Length of fuselage (m) | 48.7 |
| Semi-span(m) | 10.5 |
| Reference semi-area () | 71.4 |
| Aspect ratio | 2.6 |
| Average aerodynamic chord length (m) | 7.8 |
| Chord length of wing root | 10.4 |
| Chord length of wing tip | 4.4 |
| Sweep angle of leading edge () | 19.7 |
| Grid Level | Grid Size (Million) | (Counts) | GCI/% | Order p |
|---|---|---|---|---|
| L2 | 3.0 | 289.56 | / | |
| L1 | 6.5 | 288.01 | 0.870 | |
| L0.5 | 14.0 | 287.13 | 0.487 | 2.27 |
| Category | Name | Quantity |
|---|---|---|
| Objective | min | 1 |
| Design Variables | Shape | 120 |
| Twist | 3 | |
| AoA | 1 | |
| Constraints | = 0.195 | 1 |
| 1 | ||
| 1 | ||
| 1 |
| Configuration | (Counts) | (Counts) | (Counts) | AoA (Degree) | |||
|---|---|---|---|---|---|---|---|
| Initial | 0.195 | 288.01 | / | 211.46 | 76.55 | 2.97 | 0.03 |
| Opt_0.7t | 0.195 | 274.97 | −13.04 | 198.30 | 76.67 | 3.00 | 0.02 |
| Opt_0.9t | 0.195 | 277.91 | −10.10 | 201.26 | 76.65 | 2.98 | 0.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rao, H.; Shi, Y.; Bai, J.; Chen, Y.; Yang, T.; Li, J. Aerodynamic Optimization Design of Supersonic Wing Based on Discrete Adjoint. Aerospace 2023, 10, 420. https://doi.org/10.3390/aerospace10050420
Rao H, Shi Y, Bai J, Chen Y, Yang T, Li J. Aerodynamic Optimization Design of Supersonic Wing Based on Discrete Adjoint. Aerospace. 2023; 10(5):420. https://doi.org/10.3390/aerospace10050420
Chicago/Turabian StyleRao, Hanyue, Yayun Shi, Junqiang Bai, Yifu Chen, Tihao Yang, and Junfu Li. 2023. "Aerodynamic Optimization Design of Supersonic Wing Based on Discrete Adjoint" Aerospace 10, no. 5: 420. https://doi.org/10.3390/aerospace10050420
APA StyleRao, H., Shi, Y., Bai, J., Chen, Y., Yang, T., & Li, J. (2023). Aerodynamic Optimization Design of Supersonic Wing Based on Discrete Adjoint. Aerospace, 10(5), 420. https://doi.org/10.3390/aerospace10050420





