Numerical Investigation of Asymmetric Mach 2.5 Turbulent Shock Wave Boundary Layer Interaction
Abstract
1. Introduction
2. Experiment
3. Computational Methods
3.1. Overview of Simulations
3.2. Governing Equations and Discretization
3.3. Non-Dimensionalization
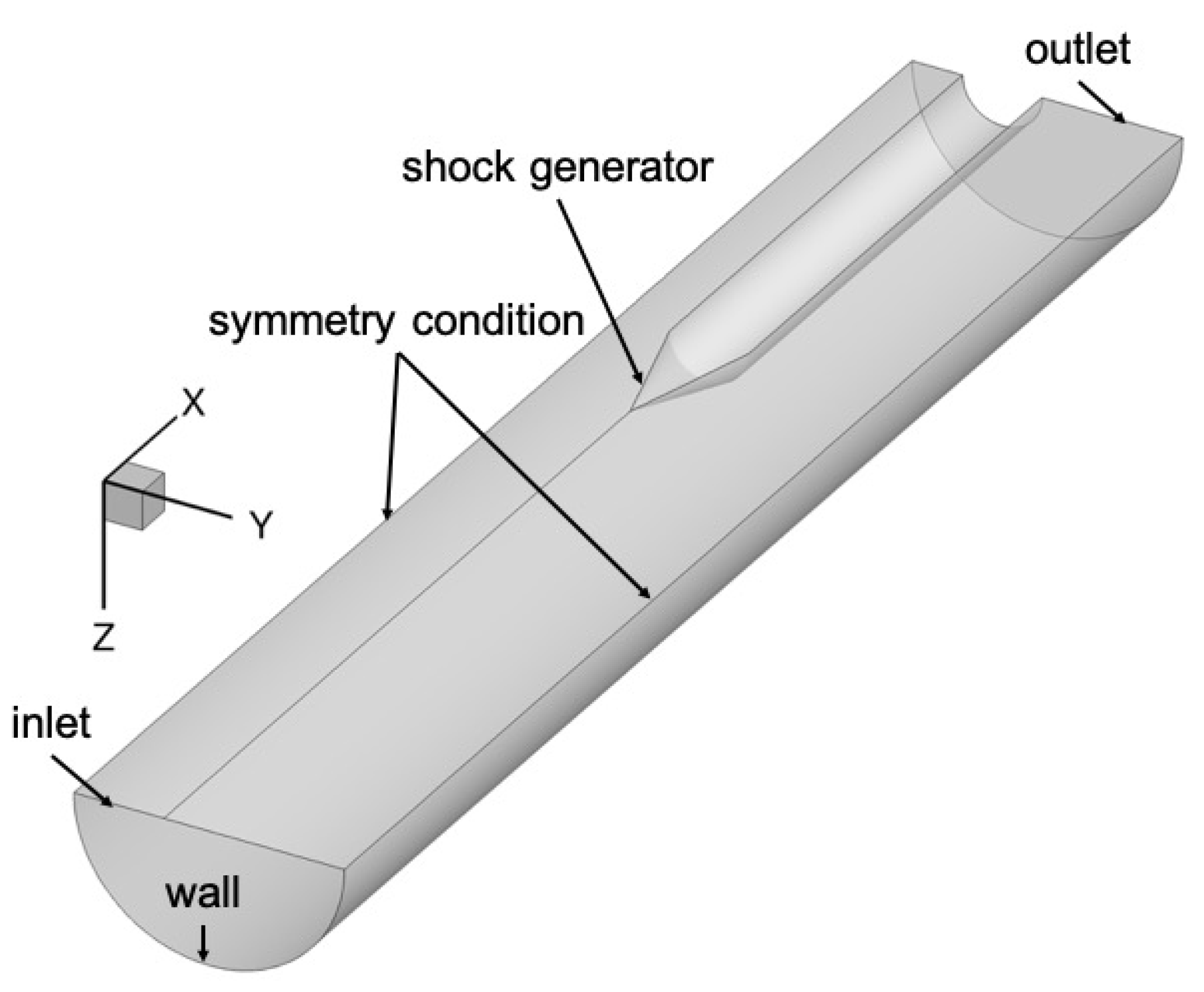
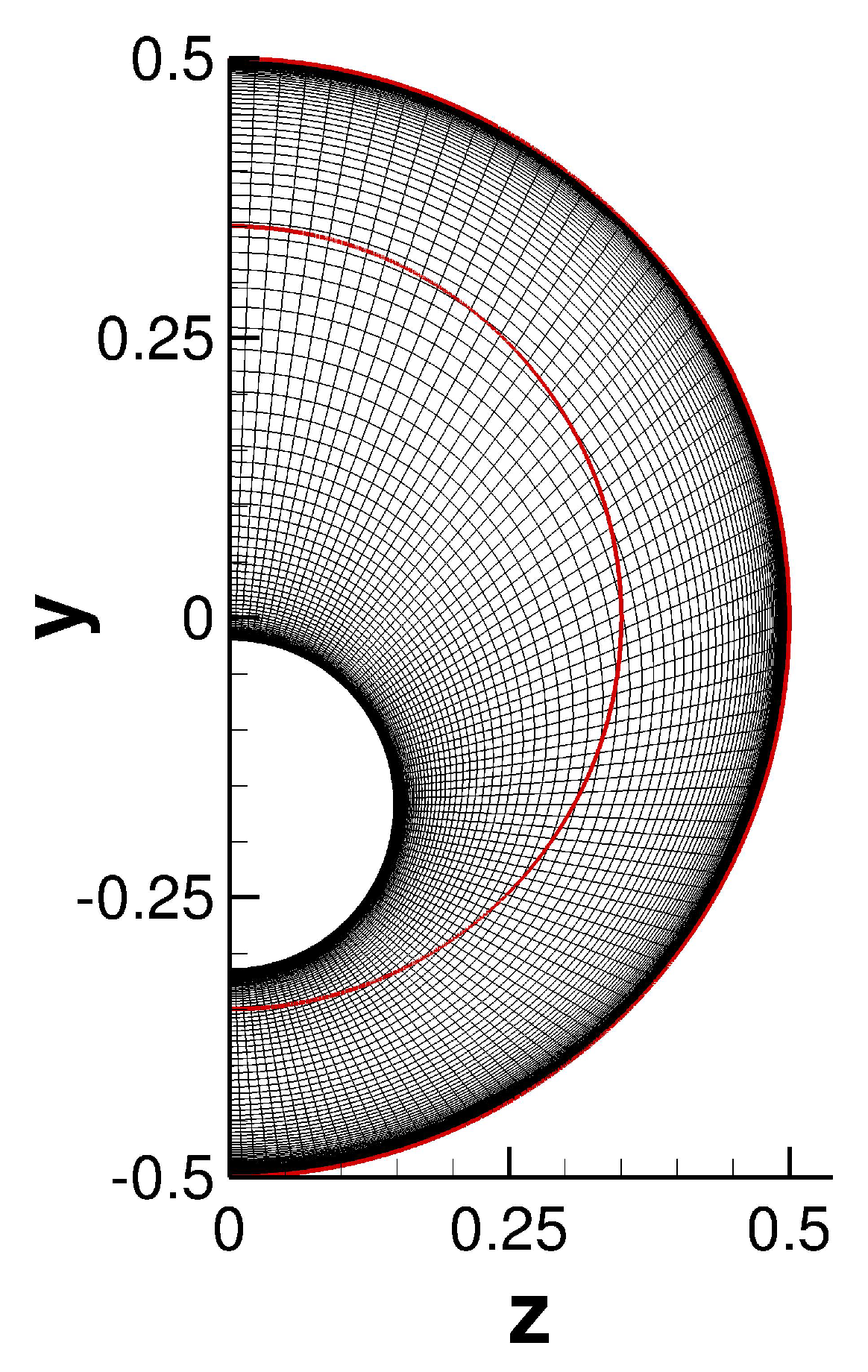
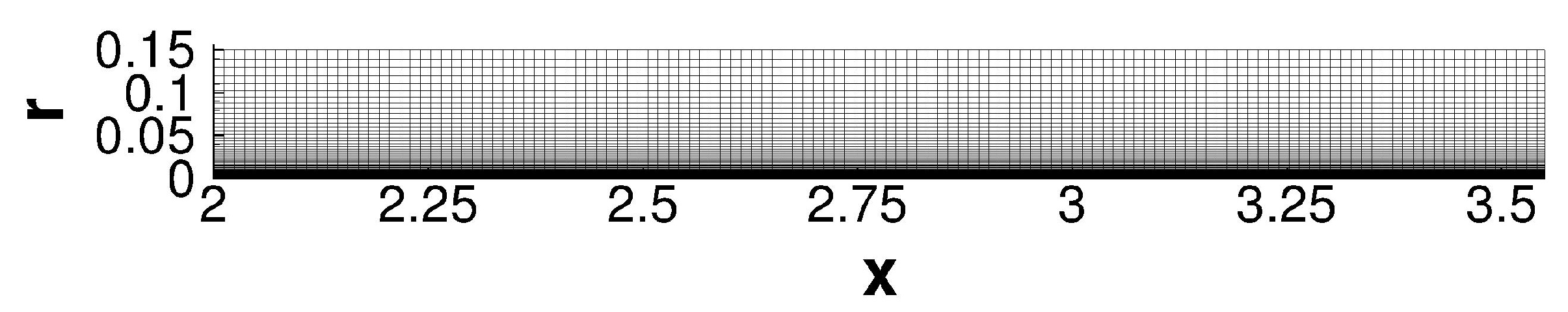
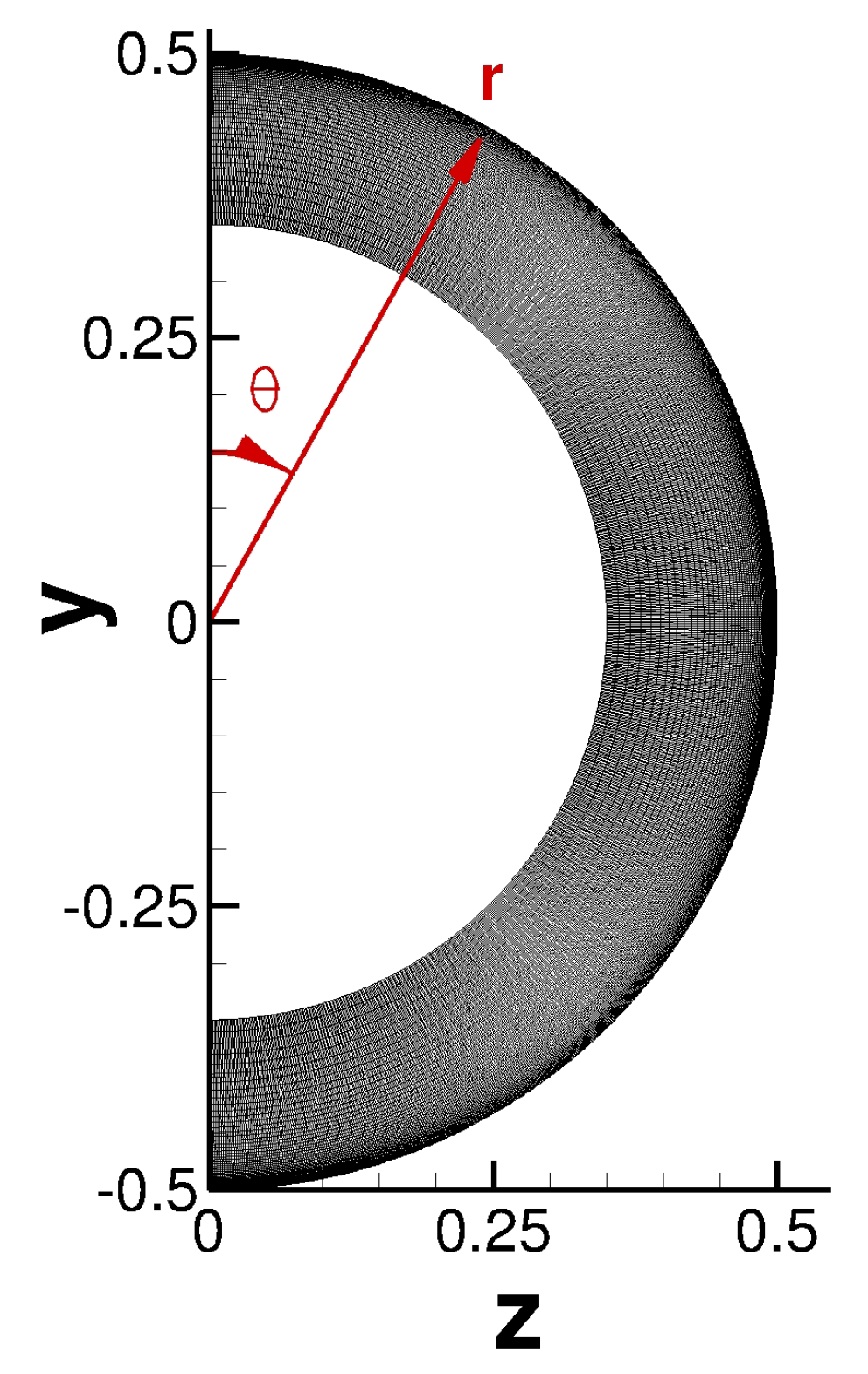
3.4. Computational Grids
3.5. Boundary Conditions
3.6. Proper Orthogonal Decomposition
4. Results
4.1. Turbulent Approach Boundary Layer
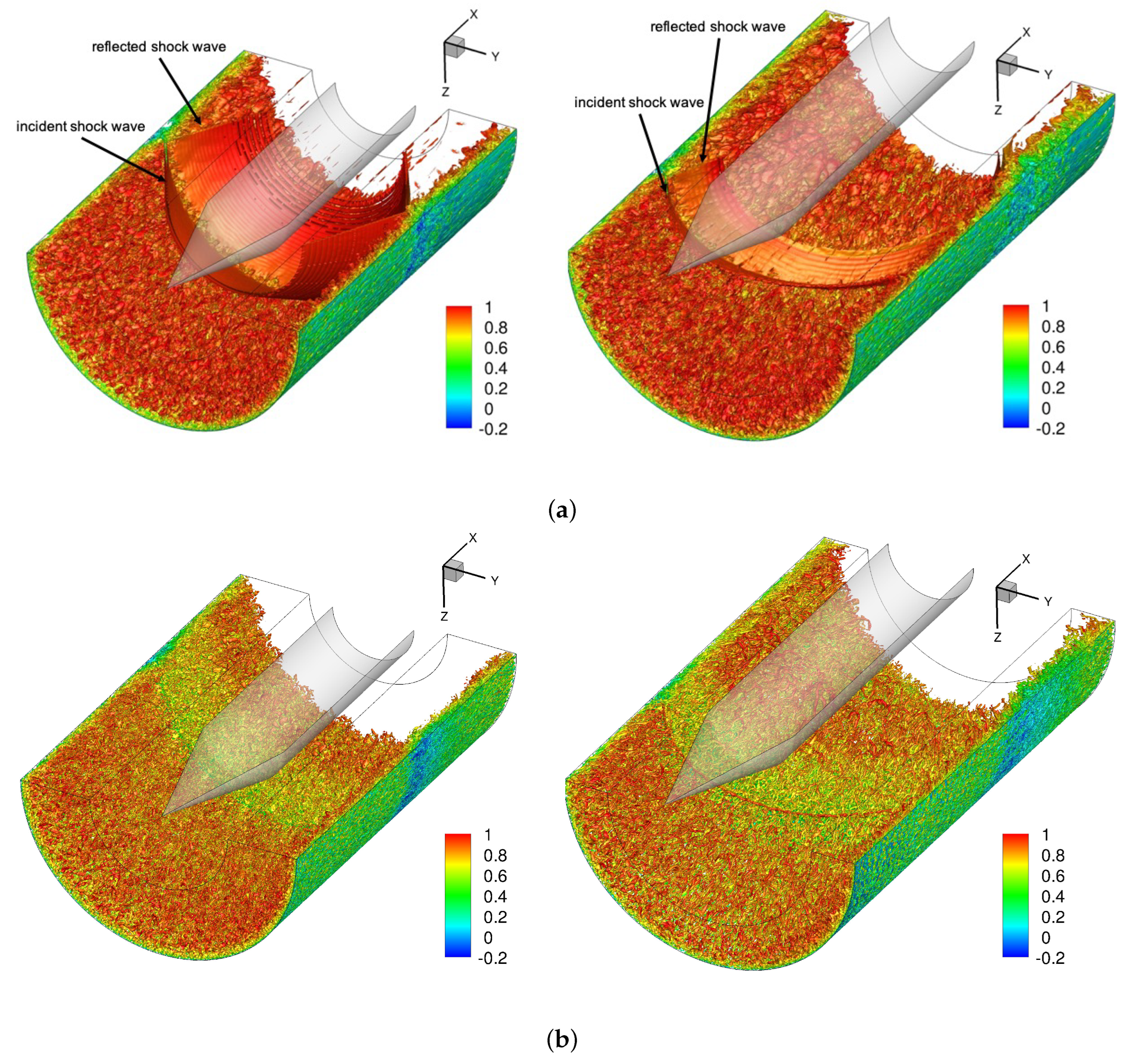
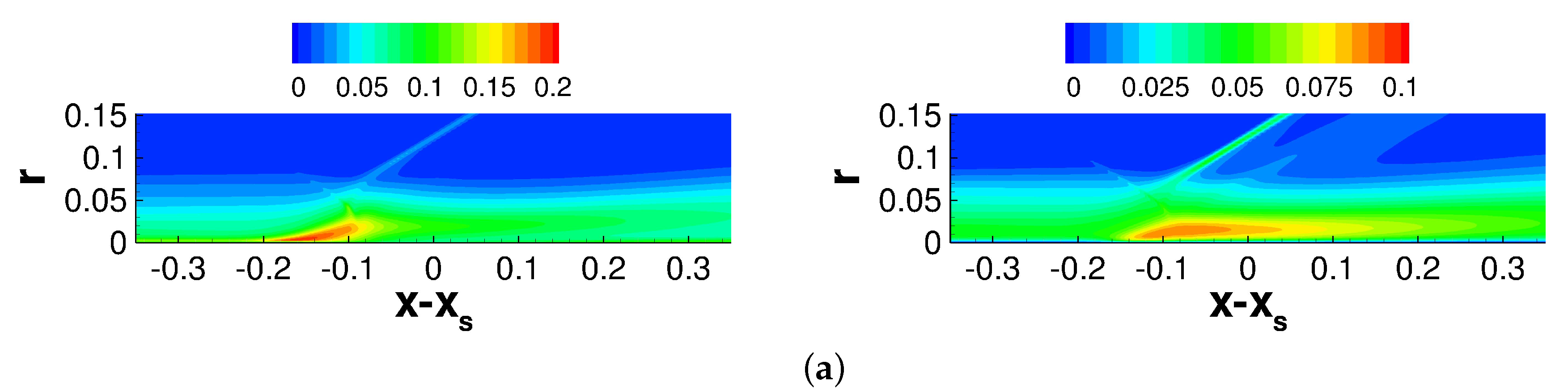
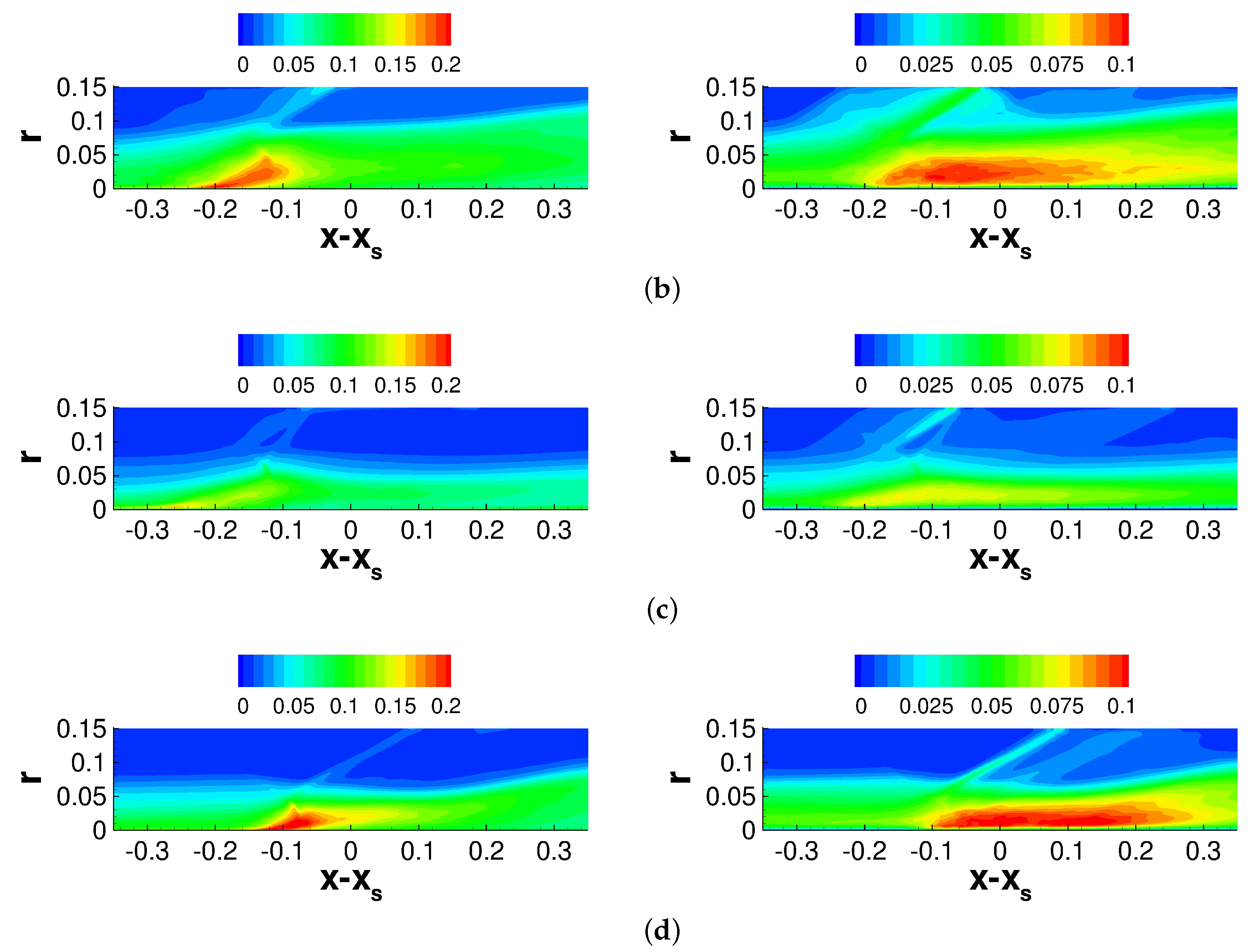
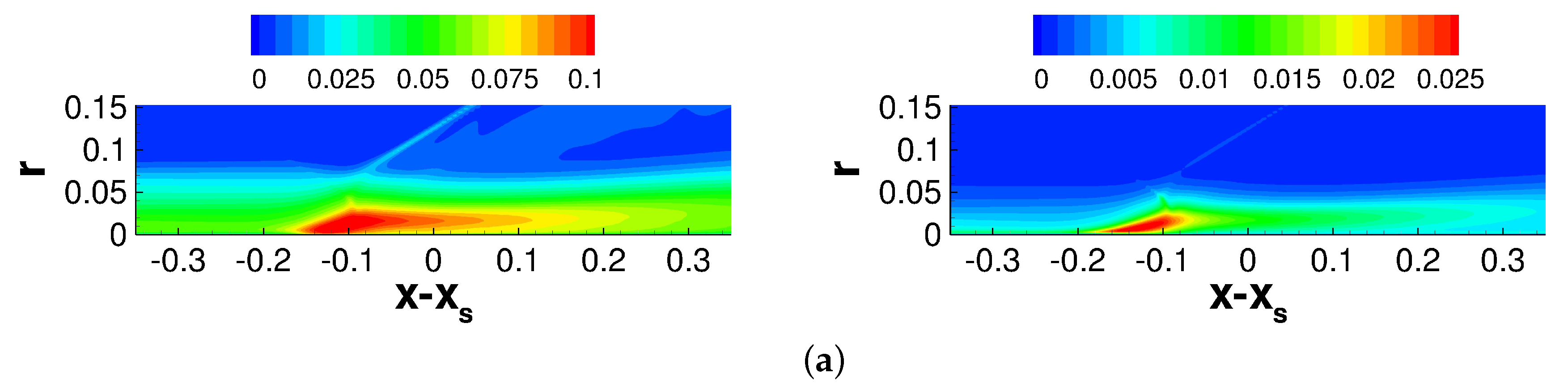
4.2. Instantaneous Flow Fields
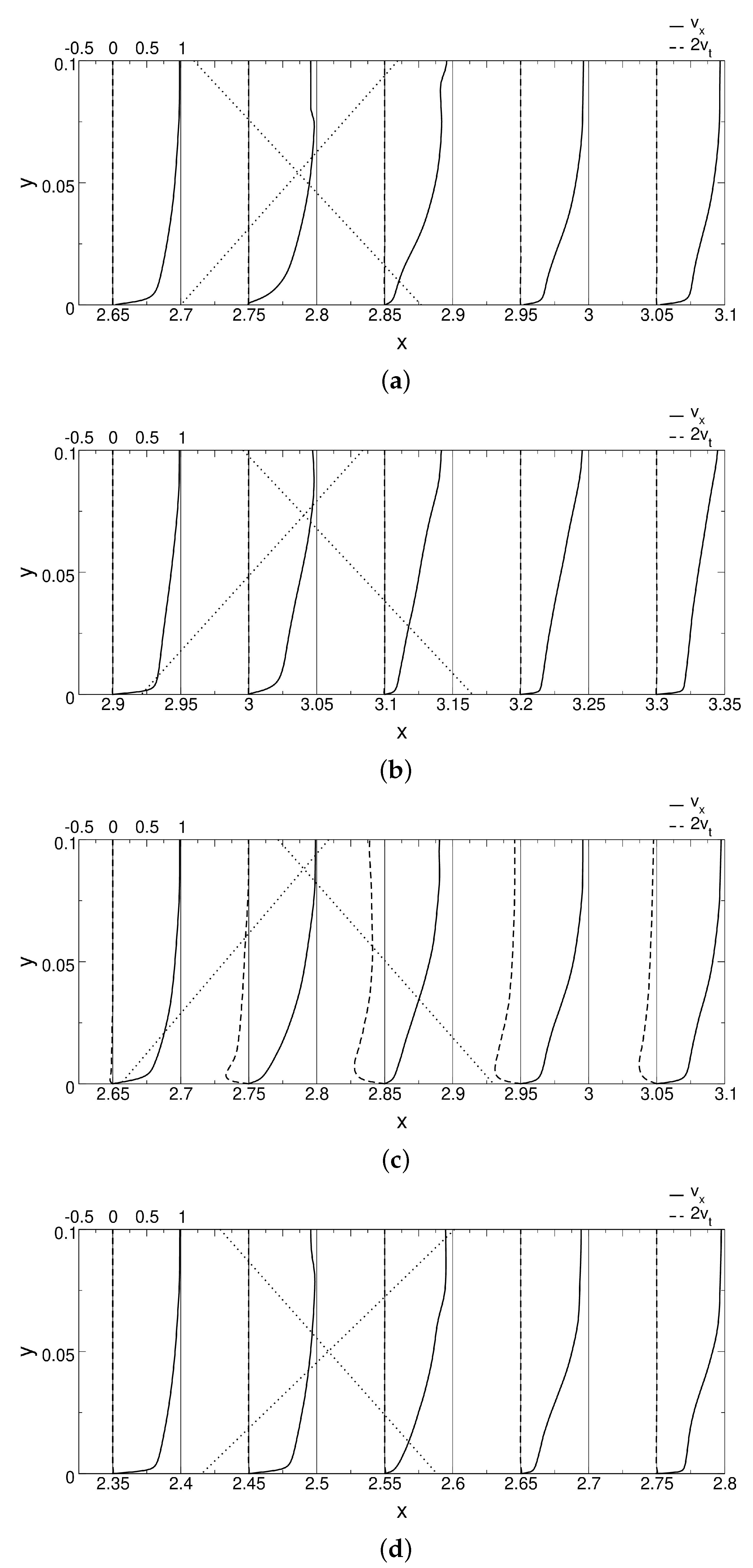
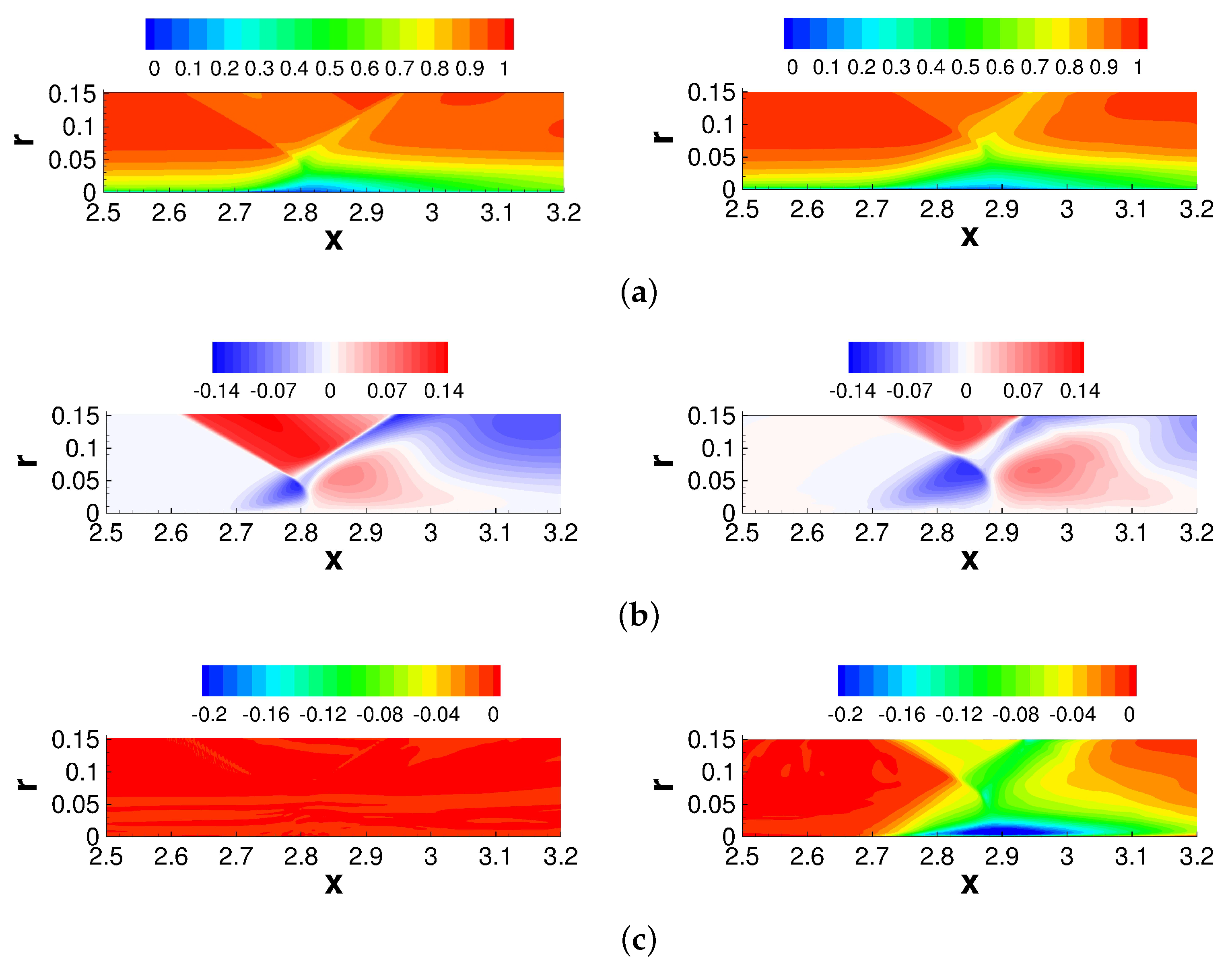
4.3. Mean Flow Analysis
4.4. Turbulent Statistics
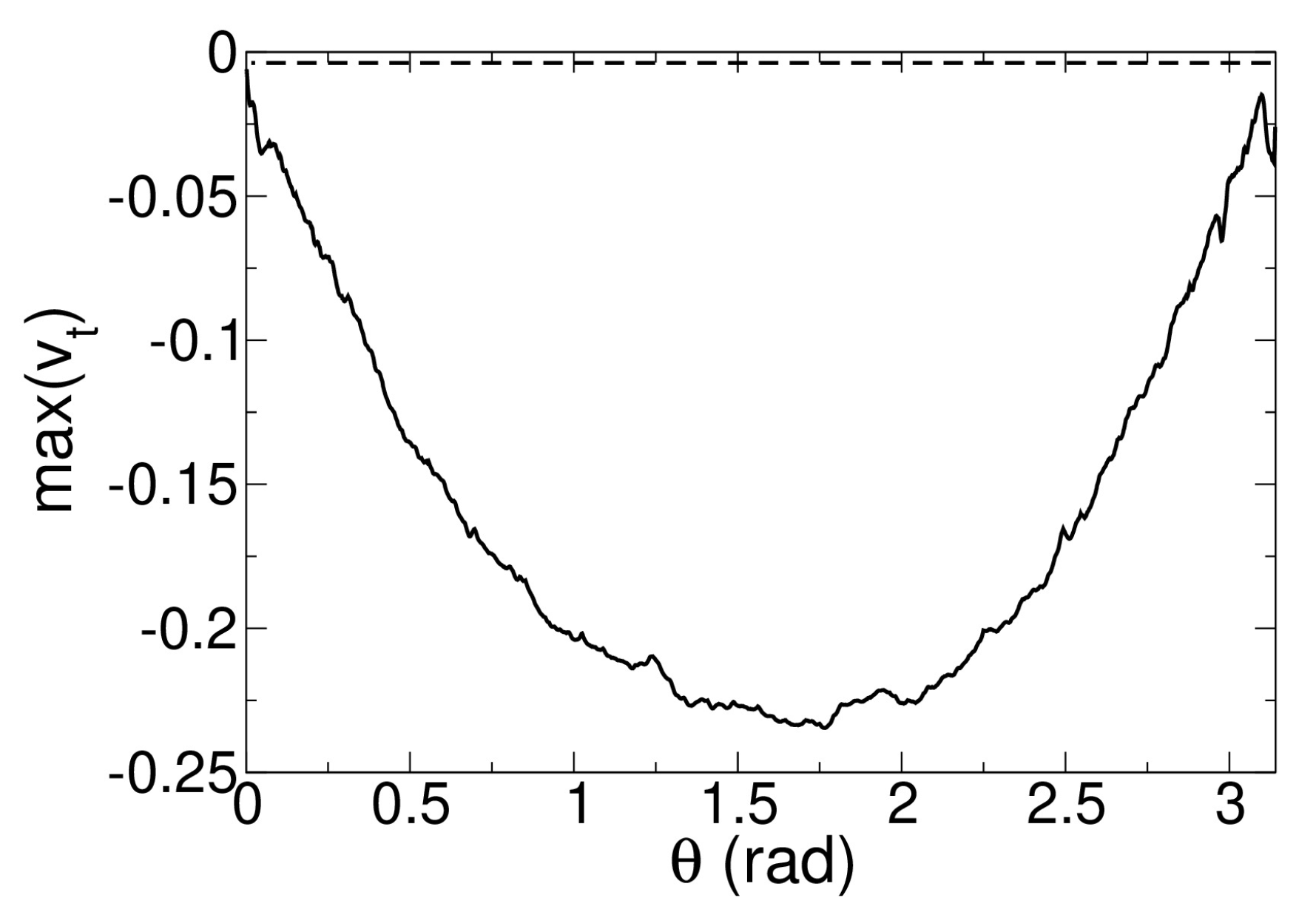
4.5. Unsteady Flow Analysis
4.5.1. Fourier Transforms of Wall-Pressure Coefficient
4.5.2. Probability Density Function of Axial Skin-Friction Coefficient
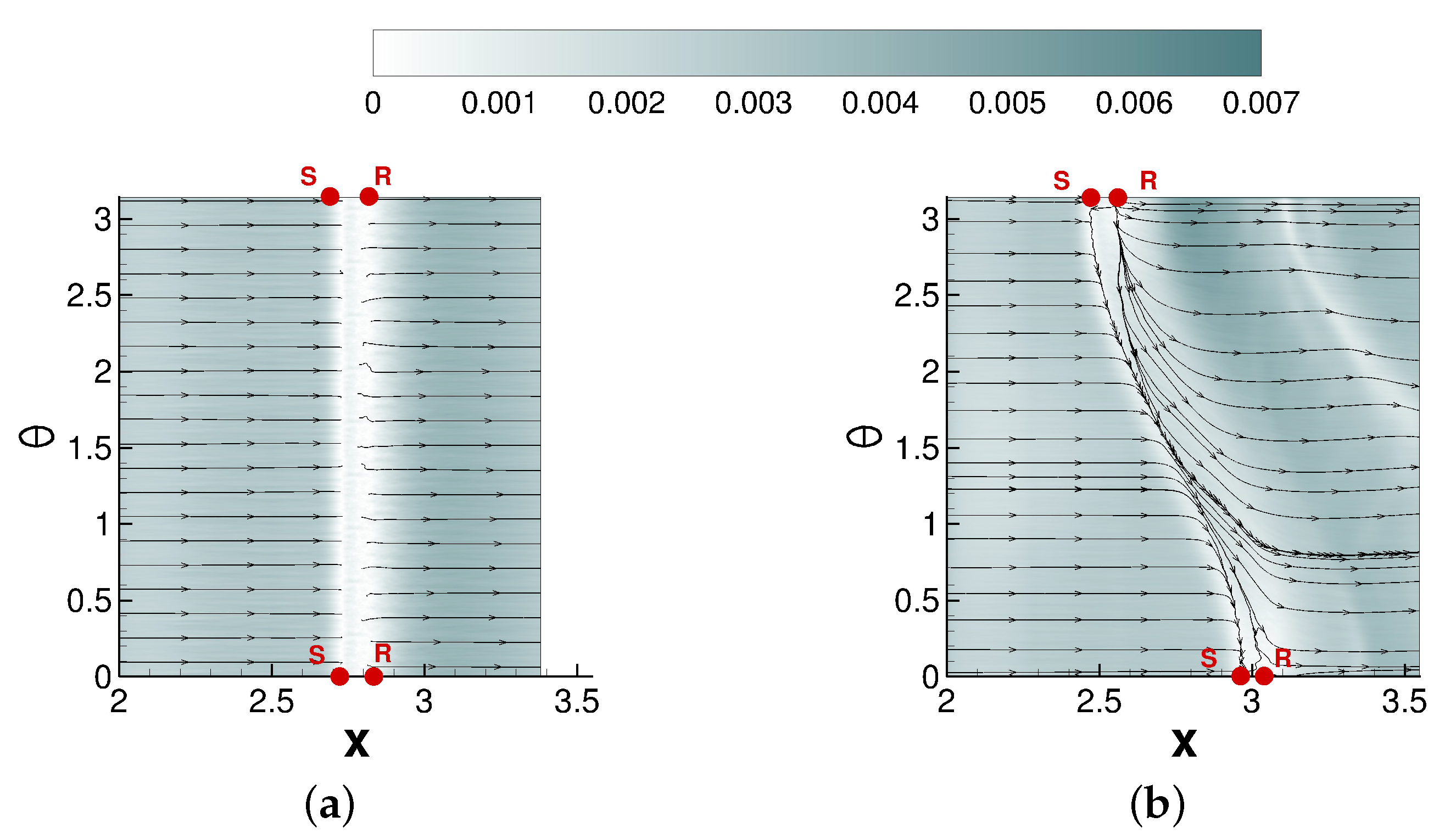
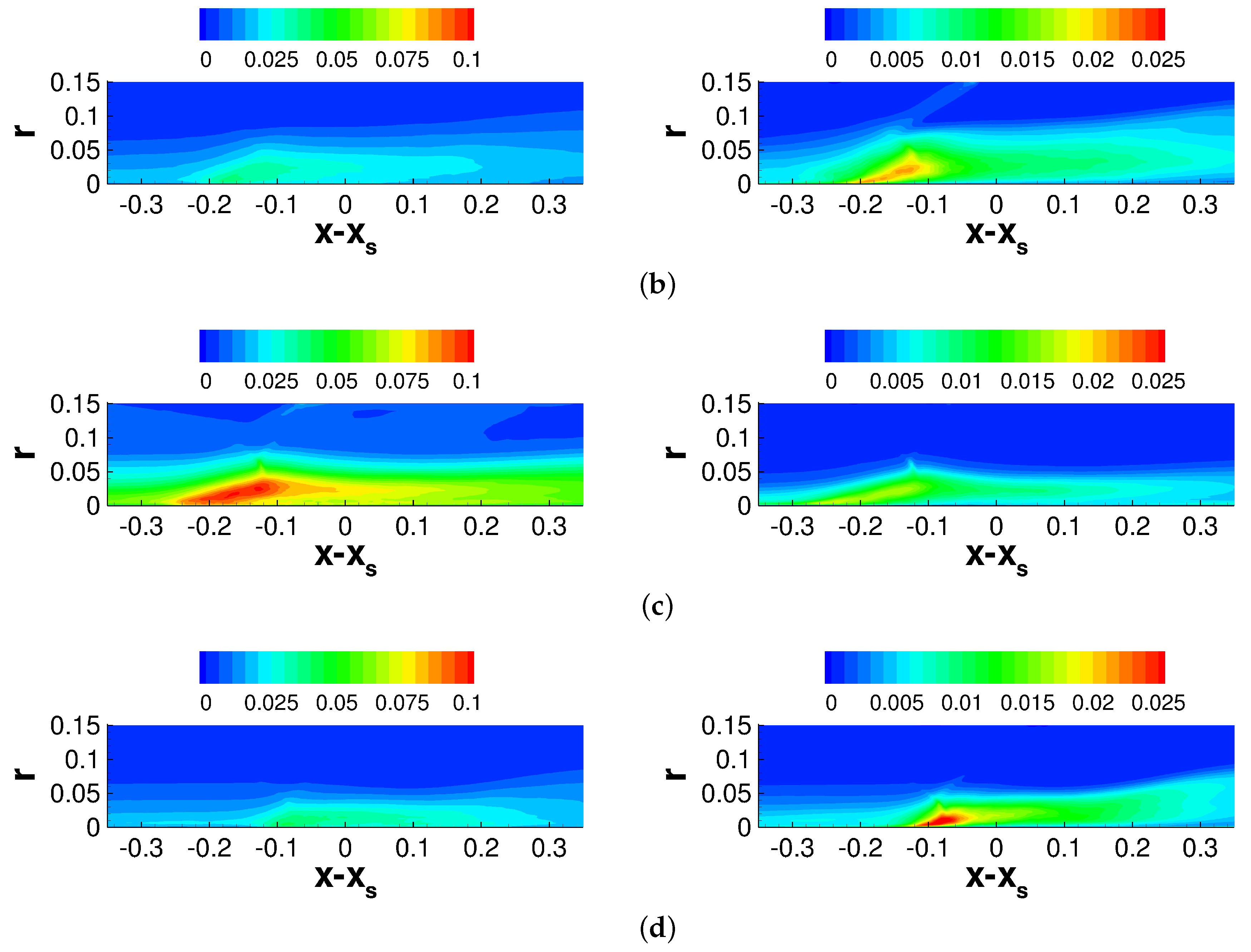
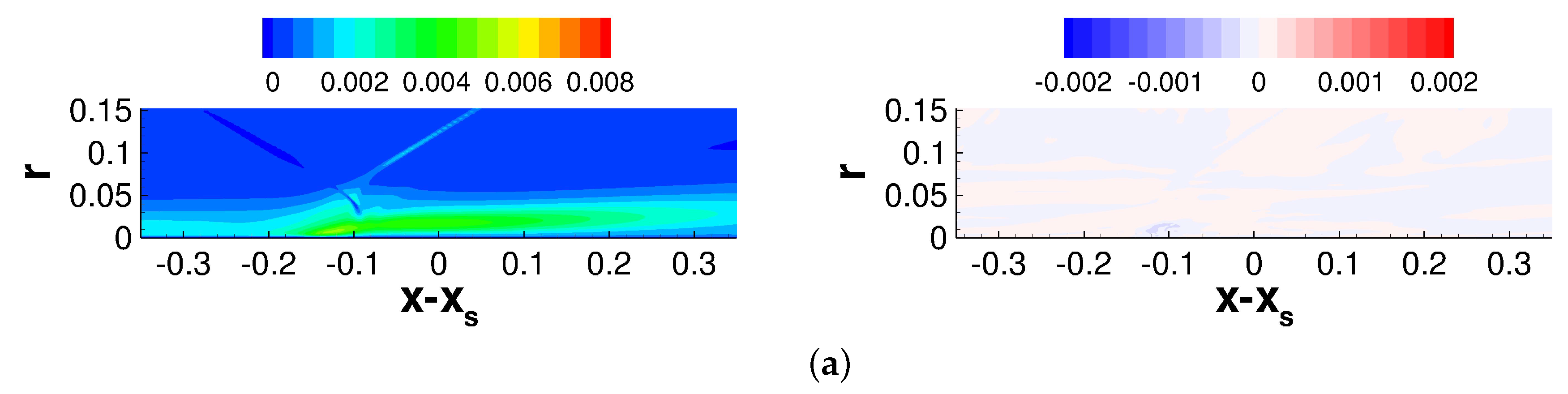
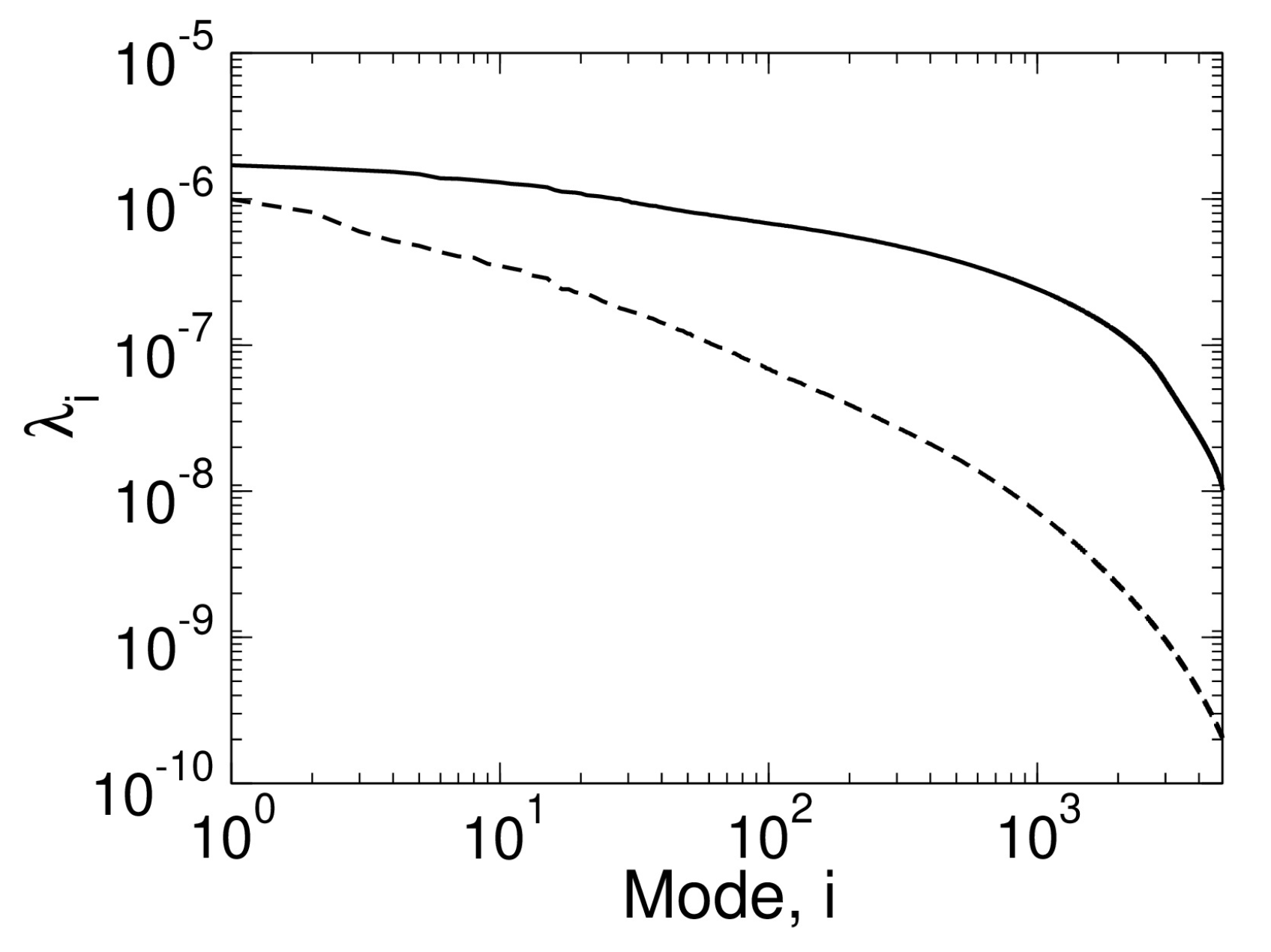
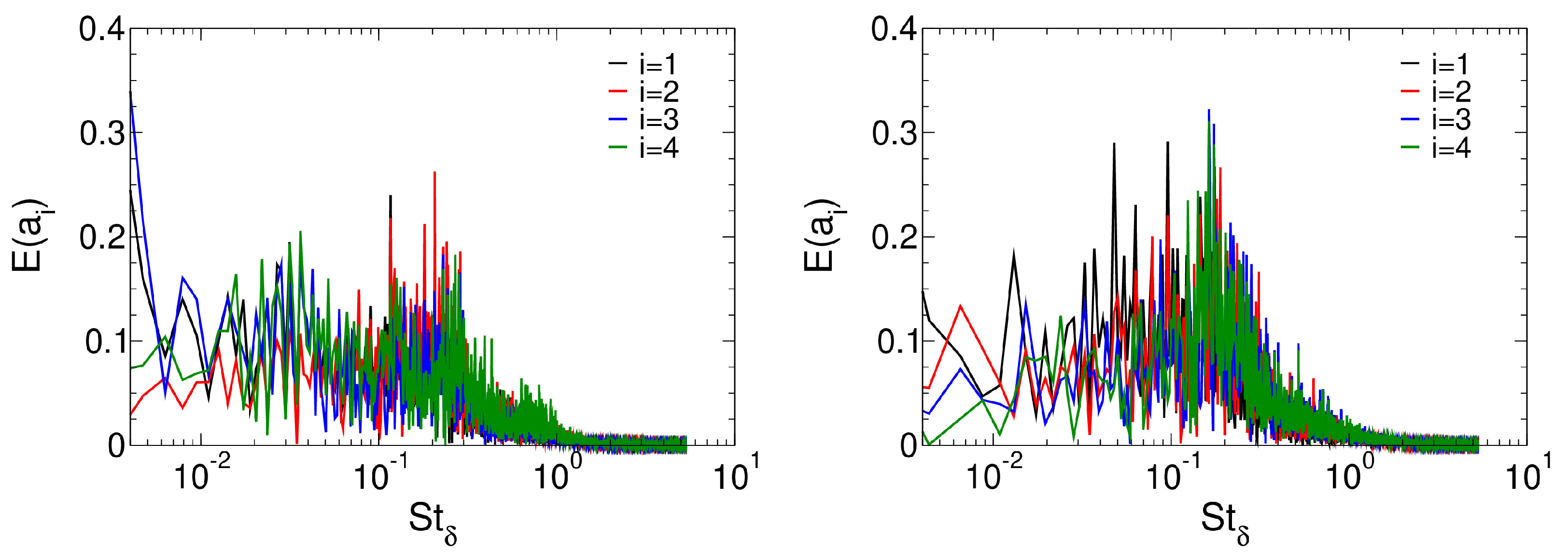
4.5.3. Proper Orthogonal Decomposition of Pressure Coefficient
5. Discussion
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| ai | Time coefficient |
| Cf | Skin-friction coefficient |
| Cp | Pressure coefficient |
| D | Test section diameter |
| E | Amplitude |
| f | Frequency |
| H | Shape factor |
| k | Turbulent kinetic energy |
| M | Mach number |
| n | Fourier mode number |
| p | Pressure |
| Pr | Prandtl number |
| Re | Reynolds number |
| rSG | Shock generator centerbody radius |
| St | Strouhal number |
| t | Time |
| T | Temperature |
| vx,vr,vt | Velocities |
| r,θ,x | Cylindrical coordinates |
| Greek Symbols | |
| α | Shock generator cone half-angle |
| γ | Ratio of specific heats |
| δ | Boundary layer thickness |
| δ∗ | Displacement thickness |
| ε | turbulent dissipation rate |
| ϑ | momentum thickness |
| λ | Eigenvalue |
| μ | Dynamic viscosity |
| v | Kinematic viscosity |
| ρ | Density |
| Subscripts | |
| i | POD mode number |
| incomp | Incompressible |
| rms | Root-mean-square |
| ∞ | Approach flow freestream conditions |
| Superscripts | |
| + | In wall units |
| * | Dimensional quantity |
| ’ | Fluctuation |
References
- Clemens, N.T.; Narayanaswamy, V. Low-Frequency Unsteadiness of Shock Wave/Turbulent Boundary Layer Interactions. Annu. Rev. Fluid Mech. 2014, 46, 469–492. [Google Scholar] [CrossRef]
- Piponniau, S.; Dussauge, J.P.; Debieve, J.F.; Dupont, P. A simple model for low-frequency unsteadiness in shock-induced separation. J. Fluid Mech. 2009, 629, 87–108. [Google Scholar] [CrossRef]
- Gaitonde, D.V. Progress in shock wave/boundary layer interactions. Prog. Aerosp. Sci. 2015, 72, 80–99. [Google Scholar] [CrossRef]
- Agostini, L.; Larchevêque, L.; Dupont, P. Mechanism of shock unsteadiness in separated shock/boundary-layer interactions. Phys. Fluids 2015, 27, 126103. [Google Scholar] [CrossRef]
- Touber, E.; Sandham, N.D. Large-eddy simulation of low-frequency unsteadiness in a turbulent shock-induced separation bubble. Theor. Comput. Fluid Dyn. 2009, 23, 79–107. [Google Scholar] [CrossRef]
- Threadgill, J.A.; Little, J.C. An inviscid analysis of swept oblique shock reflections. J. Fluid Mech. 2020, 890, A22. [Google Scholar] [CrossRef]
- Dussauge, J.P.; Dupont, P.; Debiève, J.F. Unsteadiness in shock wave boundary layer interactions with separation. Aerosp. Sci. Technol. 2006, 10, 85–91. [Google Scholar] [CrossRef]
- Ganapathisubramani, B.; Clemens, N.; Dolling, D. Effects of upstream boundary layer on the unsteadiness of shock-induced separation. J. Fluid Mech. 2007, 585, 369–394. [Google Scholar] [CrossRef]
- Priebe, S.; Tu, J.H.; Rowley, C.W.; Martín, M.P. Low-frequency dynamics in a shock-induced separated flow. J. Fluid Mech. 2016, 807, 441–477. [Google Scholar] [CrossRef]
- Gaitonde, D.V.; Adler, M.C. Dynamics of Three-Dimensional Shock-Wave/Boundary-Layer Interactions. Annu. Rev. Fluid Mech. 2023, 55, 291–321. [Google Scholar] [CrossRef]
- Panaras, A.G. Review of the physics of swept-shock/boundary layer interactions. Prog. Aerosp. Sci. 1996, 32, 173–244. [Google Scholar] [CrossRef]
- Erengil, M.E.; Dolling, D.S. Effects of Sweepback on Unsteady Separation in Mach 5 Compression Ramp Interactions. AIAA J. 1993, 31, 302–311. [Google Scholar] [CrossRef]
- Settles, G.S.; Teng, H.Y. Cylindrical and Conical Flow Regimes of Three-Dimensional Shock/Boundary-Layer Interactions. AIAA J. 1984, 22, 194–200. [Google Scholar] [CrossRef]
- Vanstone, L.; Musta, M.N.; Seckin, S.; Clemens, N. Experimental study of the mean structure and quasi-conical scaling of a swept-compression-ramp interaction at Mach 2. J. Fluid Mech. 2018, 841, 1–27. [Google Scholar] [CrossRef]
- Huang, X.; Estruch-Samper, D. Low-frequency unsteadiness of swept shock-wave/turbulent-boundary-layer interaction. J. Fluid Mech. 2018, 856, 797–821. [Google Scholar] [CrossRef]
- Arora, N.; Mears, L.; Alvi, F.S. Unsteady Characteristics of a Swept-Shock/Boundary-Layer Interaction at Mach 2. AIAA J. 2019, 57, 4548–4559. [Google Scholar] [CrossRef]
- Adler, M.C.; Gaitonde, D.V. Dynamics of strong swept-shock/turbulent-boundary-layer interactions. J. Fluid Mech. 2020, 896, A29. [Google Scholar] [CrossRef]
- Lee, S.; Gross, A. Numerical Investigation of Sweep Effect on Turbulent Shock-Wave Boundary-Layer Interaction. AIAA J. 2022, 60, 712–730. [Google Scholar] [CrossRef]
- Fang, J.; Yao, Y.; Zheltovodov, A.A.; Lu, L. Investigation of Three-Dimensional Shock Wave/Turbulent-Boundary-Layer Interaction Initiated by a Single Fin. AIAA J. 2017, 55, 509–523. [Google Scholar] [CrossRef]
- Mears, L.J.; Baldwin, A.; Ali, M.Y.; Kumar, R.; Alvi, F.S. Spatially resolved mean and unsteady surface pressure in swept SBLI using PSP. Exp. Fluids 2020, 61, 92. [Google Scholar] [CrossRef]
- Baldwin, A.; Mears, L.J.; Kumar, R.; Alvi, F.S. Effects of Reynolds Number on Swept Shock-Wave/Boundary-Layer Interactions. AIAA J. 2021, 59, 3883–3899. [Google Scholar] [CrossRef]
- Vanstone, L.; Clemens, N.T. Proper Orthogonal Decomposition Analysis of Swept-Ramp Shock-Wave/Boundary-Layer Unsteadiness at Mach 2. AIAA J. 2019, 57, 3395–3409. [Google Scholar] [CrossRef]
- Adler, M.C.; Gaitonde, D.V. Flow Similarity in Strong Swept-Shock/Turbulent-Boundary-Layer Interactions. AIAA J. 2019, 57, 1579–1593. [Google Scholar] [CrossRef]
- Padmanabhan, S.; Maldonado, J.C.; Threadgill, J.A.; Little, J.C. Experimental Study of Swept Impinging Oblique Shock/Boundary-Layer Interactions. AIAA J. 2021, 59, 140–149. [Google Scholar] [CrossRef]
- Mears, L.J.; Sellappan, P.; Alvi, F.S. Three-Dimensional Flowfield in a Fin-Generated Shock Wave/Boundary-Layer Interaction Using Tomographic PIV. AIAA J. 2021, 59, 4869–4880. [Google Scholar] [CrossRef]
- Zuo, F.Y.; Memmolo, A.; Huang, G.p.; Pirozzoli, S. Direct numerical simulation of conical shock wave–turbulent boundary layer interaction. J. Fluid Mech. 2019, 877, 167–195. [Google Scholar] [CrossRef]
- Kussoy, M.; Viegas, J.; Horstman, C. Investigation of a Three-Dimensional Shock Wave Separated Turbulent Boundary Layer. AIAA J. 1980, 18, 1477–1484. [Google Scholar] [CrossRef]
- Davis, D.O. CFD Validation Experiment of a Mach 2.5 Axisymmetric Shock-Wave/Boundary-Layer Interaction. In Proceedings of the Fluids Engineering Division Summer Meeting. American Society of Mechanical Engineers, Seoul, Republic of Korea, 26–31 July 2015; Volume 57212, p. V001T06A001. [Google Scholar]
- Bruce, P.; Burton, D.; Titchener, N.; Babinsky, H. Corner effect and separation in transonic channel flows. J. Fluid Mech. 2011, 679, 247–262. [Google Scholar] [CrossRef]
- Burton, D.; Babinsky, H. Corner separation effects for normal shock wave/turbulent boundary layer interactions in rectangular channels. J. Fluid Mech. 2012, 707, 287–306. [Google Scholar] [CrossRef]
- Chou, J.H.; Childs, M.E.; Wong, R.S. An Experimental Study of Three-Dimensional Shock Wave/Turbulent Boundary Layer Interactions in a Supersonic Flow. In Proceedings of the 18th Fluid Dynamics and Plasmadynamics and Lasers Conference, Cincinnati, OH, USA, 16–18 July 1985; AIAA Paper 1985-1556. AIAA: Reston, VA, USA, 1985. [Google Scholar]
- Sasson, J.; Reising, H.H.; Davis, D.O.; Barnhart, P.J. Summary of Shock Wave Turbulent Boundary Layer Interaction Experiments In a Circular Test Section; AIAA Paper 2023-0442; AIAA: Reston, VA, USA, 2023. [Google Scholar]
- Settles, G.S.; Dodson, L.J. Supersonic and Hypersonic Shock/Boundary-Layer Interaction Database. AIAA J. 1994, 32, 1377–1383. [Google Scholar] [CrossRef]
- Sasson, J. Conical Shock Wave Turbulent Boundary Layer Interactions in a Circular Test Section at Mach 2.5. Ph.D. Thesis, Case Western Reserve University, Cleveland, OH, USA, 2022. [Google Scholar]
- Sasson, J.; Barnhart, P. Spectral Analysis of a Shock Wave Turbulent Boundary Layer Interaction In a Circular Test Section. In Proceedings of the AIAA SCITECH 2023 Forum, National Harbor, MD, USA, 23–27 January 2023; AIAA Paper 2023-2255. AIAA: Reston, VA, USA, 2023. [Google Scholar]
- Reising, H.H.; Davis, D.O. Development and Assessment of a New Particle Image Velocimetry System in the NASA GRC 225 cm2 Wind Tunnel. In Proceedings of the AIAA SCITECH 2023 Forum, National Harbor, MD, USA, 23–27 January 2023; AIAA Paper 2023-0631. AIAA: Reston, VA, USA, 2023. [Google Scholar]
- Mosele, J.P.; Gross, A.; Slater, J. Numerical Investigation of Mach 2.5 Axisymmetric Turbulent Shock Wave Boundary Layer Interactions. Aerospace 2023, 10, 159. [Google Scholar] [CrossRef]
- Souverein, L.; Bakker, P.; Dupont, P. A scaling analysis for turbulent shock-wave/boundary-layer interactions. J. Fluid Mech. 2013, 714, 505–535. [Google Scholar] [CrossRef]
- Gross, A.; Fasel, H.F. High-Order Accurate Numerical Method for Complex Flows. AIAA J. 2008, 46, 204–214. [Google Scholar] [CrossRef]
- Gross, A.; Fasel, H.F. Numerical investigation of supersonic flow for axisymmetric cones. Math. Comput. Simul. 2010, 81, 133–142. [Google Scholar] [CrossRef]
- Wilcox, D.C. Turbulence Modeling for CFD, 3rd ed.; DCW Industries: New York, NY, USA, 2006. [Google Scholar]
- Wilcox, D.C. Formulation of the k-w Turbulence Model Revisited. AIAA J. 2008, 46, 2823–2838. [Google Scholar] [CrossRef]
- Carr, J.C.; Beatson, R.K.; Cherrie, J.B.; Mitchell, T.J.; Fright, W.R.; McCallum, B.C.; Evans, T.R. Reconstruction and Representation of 3D Objects with Radial Basis Functions. In Proceedings of the 28th Annual Conference on Computer Graphics and Interactive Techniques, Los Angeles, CA, USA, 12–17 August 2001; pp. 67–76. [Google Scholar]
- Poletto, R.; Craft, T.; Revell, A. A New Divergence Free Synthetic Eddy Method for the Reproduction of Inlet Flow Conditions for LES. Flow Turbul. Combust. 2013, 91, 519–539. [Google Scholar] [CrossRef]
- Lumley, J.L. The structure of inhomogeneous turbulent flows. In Atmospheric Turbulence and Radio Wave Propagation; National Institute of Informatics: Tokyo, Japan, 1967. [Google Scholar]
- Gross, A.; Lee, S. Numerical analysis of laminar and turbulent shock-wave boundary layer interactions. In Proceedings of the 2018 Fluid Dynamics Conference, Atlanta, GA, USA, 25–29 June 2018; AIAA Paper 2018-4033. AIAA: Reston, VA, USA, 2018. [Google Scholar]
- Sirovich, L. Turbulence and the Dynamics of Coherent Structures. Quaterly Appl. Math. 1987, 45, 561–590. [Google Scholar] [CrossRef]
- Hunt, J.; Wray, A.; Moin, P. Eddies, stream, and convergence zones in turbulent flows. In Studying Turbulence Using Numerical Simulation Databases, 2. Proceedings of the 1988 Summer Program; Center for Turbulence Research: Stanford, CA, USA, 1988; Volume 193. [Google Scholar]
- Anderson, J.D. Modern Compressible Flow: With Historical Perspective; McGraw-Hill: New York, NY, USA, 1990; Volume 12. [Google Scholar]
- Tobak, M.; Peake, D.J. Topology of three-dimensional separated flows. Annu. Rev. Fluid Mech. 1982, 14, 61–85. [Google Scholar] [CrossRef]
- Adler, M.C.; Gaitonde, D.V. Unsteadiness in swept-compression-ramp shock/turbulent-boundary-layer interactions. In Proceedings of the 55th AIAA Aerospace Sciences Meeting, Grapevine, TX, USA, 9–13 January 2017; AIAA Paper 2017-0987. AIAA: Reston, VA, USA, 2017. [Google Scholar]
- Arora, N.; Ali, M.Y.; Zhang, Y.; Alvi, F.S. Flowfield Measurements in a Mach 2 Fin-Generated Shock/Boundary-Layer Interaction. AIAA J. 2018, 56, 3963–3974. [Google Scholar] [CrossRef]
- Berkooz, G.; Holmes, P.; Lumley, J.L. The proper orthogonal decomposition in the analysis of turbulent flows. Annu. Rev. Fluid Mech. 1993, 25, 539–575. [Google Scholar] [CrossRef]
- Zuo, F.Y.; Yu, M.; Pirozzoli, S. Modal Analysis of Separation Bubble Unsteadiness in Conical Shock Wave/Turbulent Boundary Layer Interaction. AIAA J. 2022, 60, 5123–5135. [Google Scholar] [CrossRef]
- Gross, A.; Little, J.; Fasel, H.F. Numerical investigation of unswept and swept turbulent shock-wave boundary layer interactions. Aerosp. Sci. Technol. 2022, 123, 107455. [Google Scholar] [CrossRef]
- Mosele, J.P.G.; Gross, A.; Slater, J.W. Numerical Investigation of Flow Over Periodic Hill. In Proceedings of the AIAA AVIATION 2022 Forum, Gravesend, UK, 27 June–20 July 2022; AIAA Paper 2022-3228. AIAA: Reston, VA, USA, 2022. [Google Scholar]



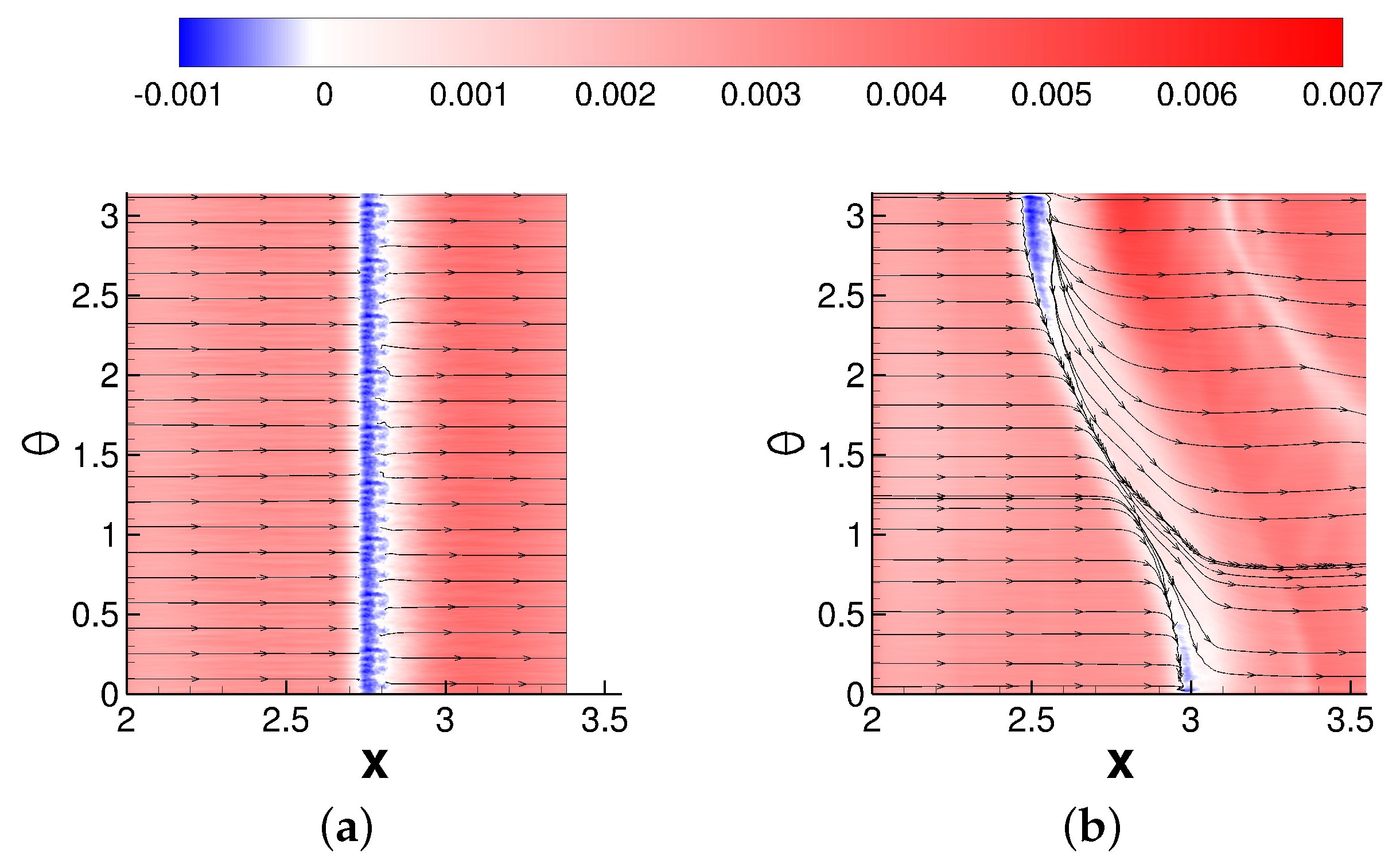
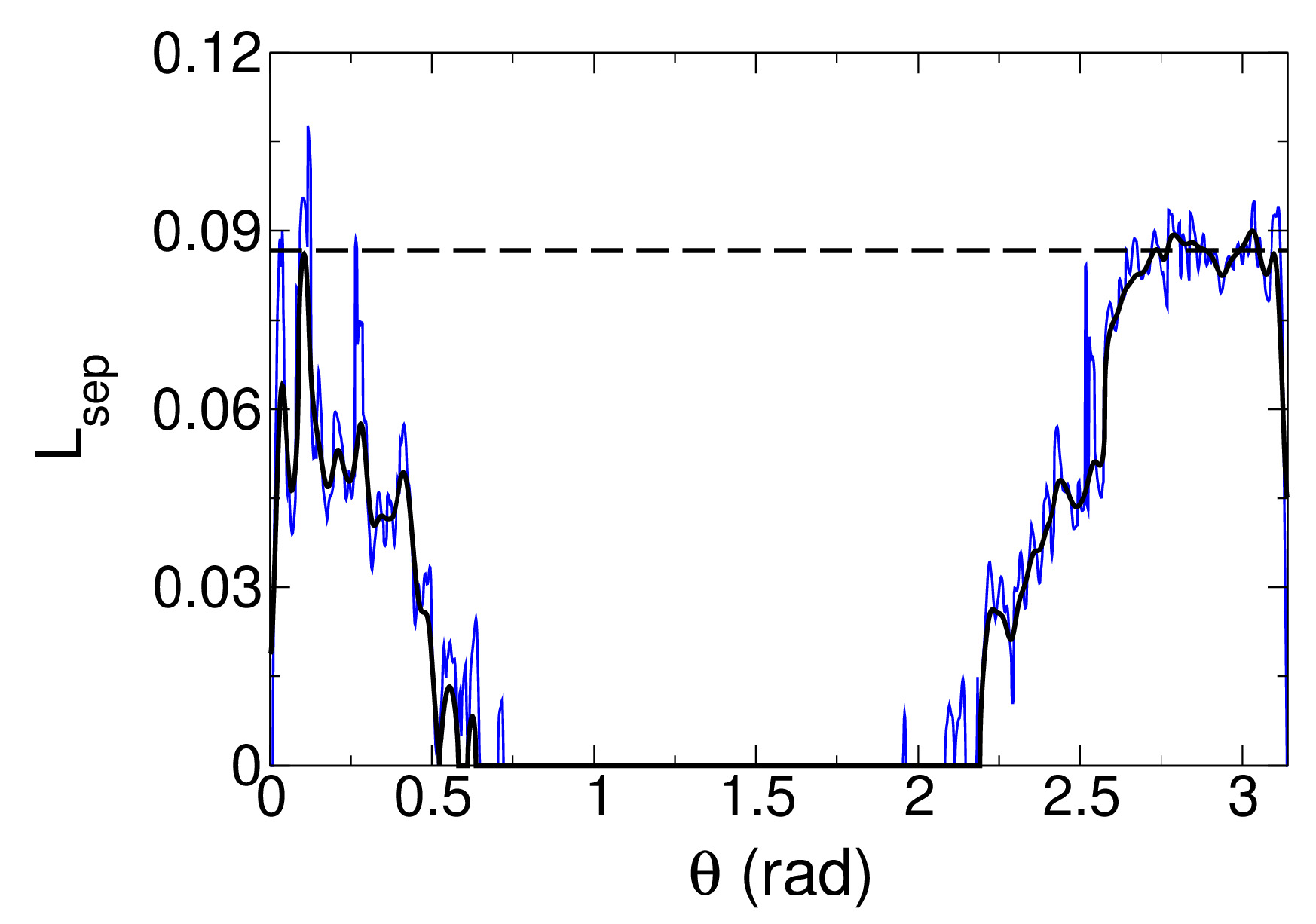
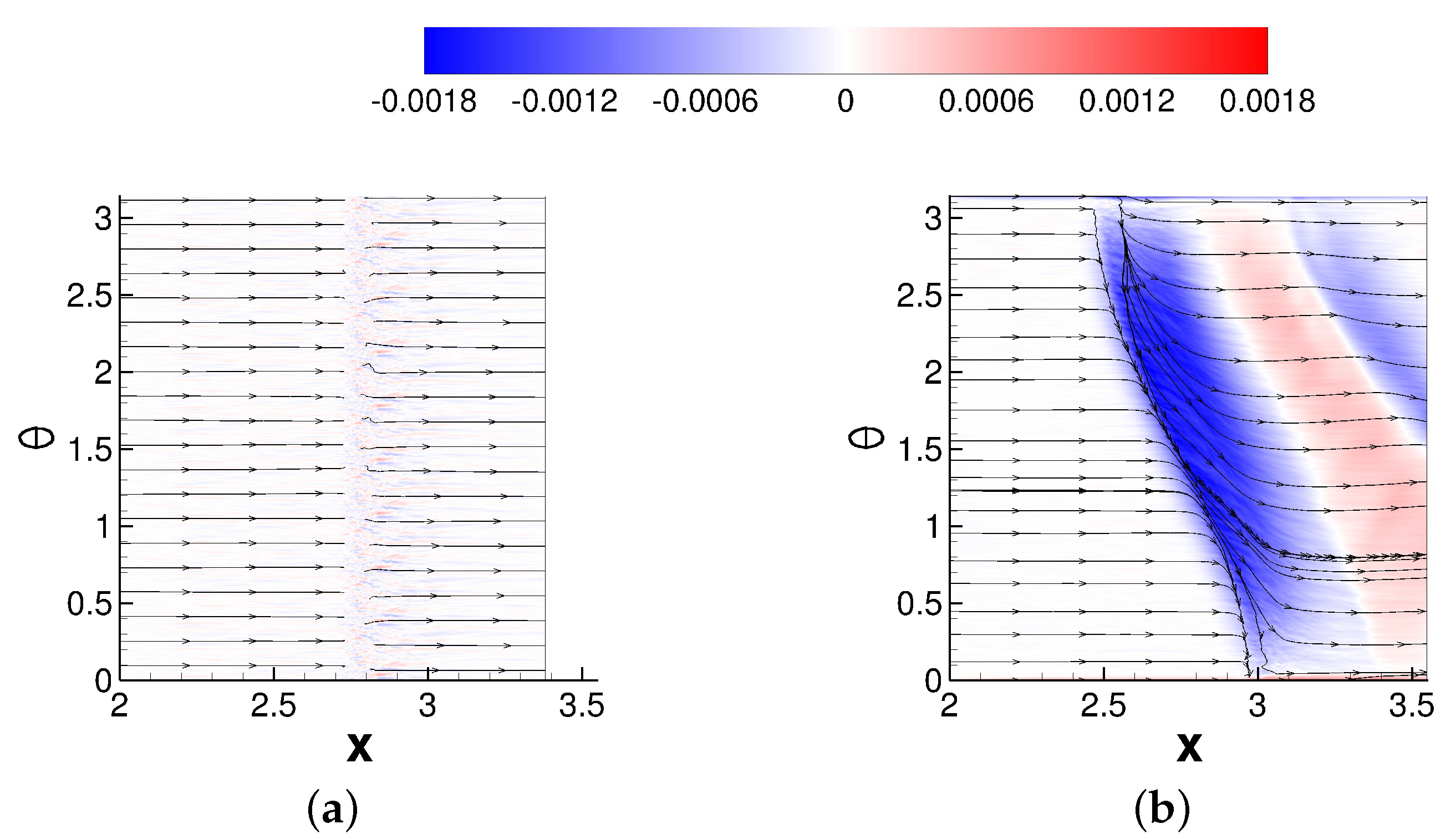
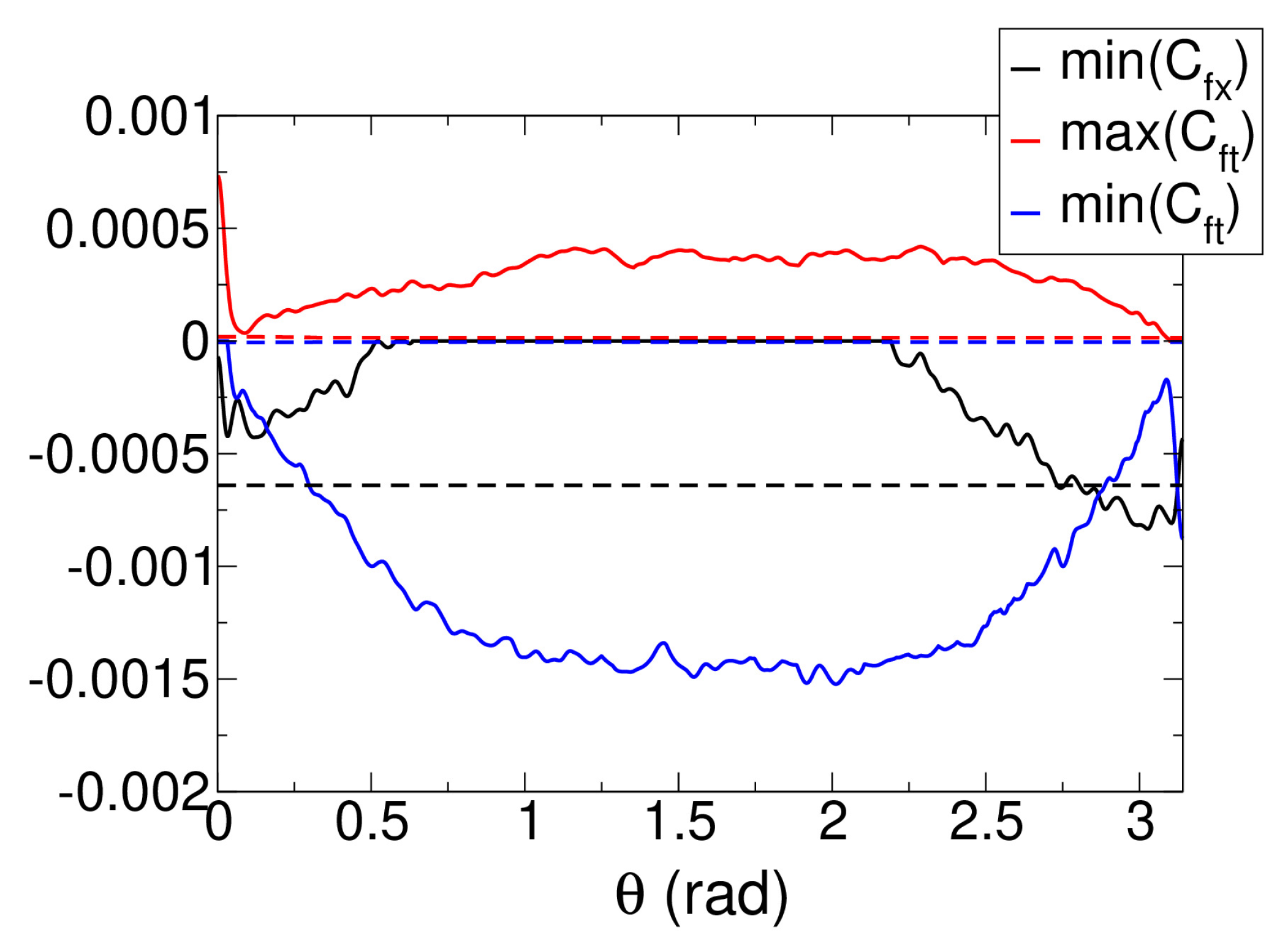







| Property | Axisymmetric [37] | Asymmetric |
|---|---|---|
| Case | ||
|---|---|---|
| Baseline | ||
| x-refine | ||
| r-refine | ||
| -refine |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mosele, J.-P.; Gross, A.; Slater, J. Numerical Investigation of Asymmetric Mach 2.5 Turbulent Shock Wave Boundary Layer Interaction. Aerospace 2023, 10, 417. https://doi.org/10.3390/aerospace10050417
Mosele J-P, Gross A, Slater J. Numerical Investigation of Asymmetric Mach 2.5 Turbulent Shock Wave Boundary Layer Interaction. Aerospace. 2023; 10(5):417. https://doi.org/10.3390/aerospace10050417
Chicago/Turabian StyleMosele, John-Paul, Andreas Gross, and John Slater. 2023. "Numerical Investigation of Asymmetric Mach 2.5 Turbulent Shock Wave Boundary Layer Interaction" Aerospace 10, no. 5: 417. https://doi.org/10.3390/aerospace10050417
APA StyleMosele, J.-P., Gross, A., & Slater, J. (2023). Numerical Investigation of Asymmetric Mach 2.5 Turbulent Shock Wave Boundary Layer Interaction. Aerospace, 10(5), 417. https://doi.org/10.3390/aerospace10050417






