Aerodynamics and Power Balance of a Distributed Aft-Fuselage Boundary Layer Ingesting Aircraft †
Abstract
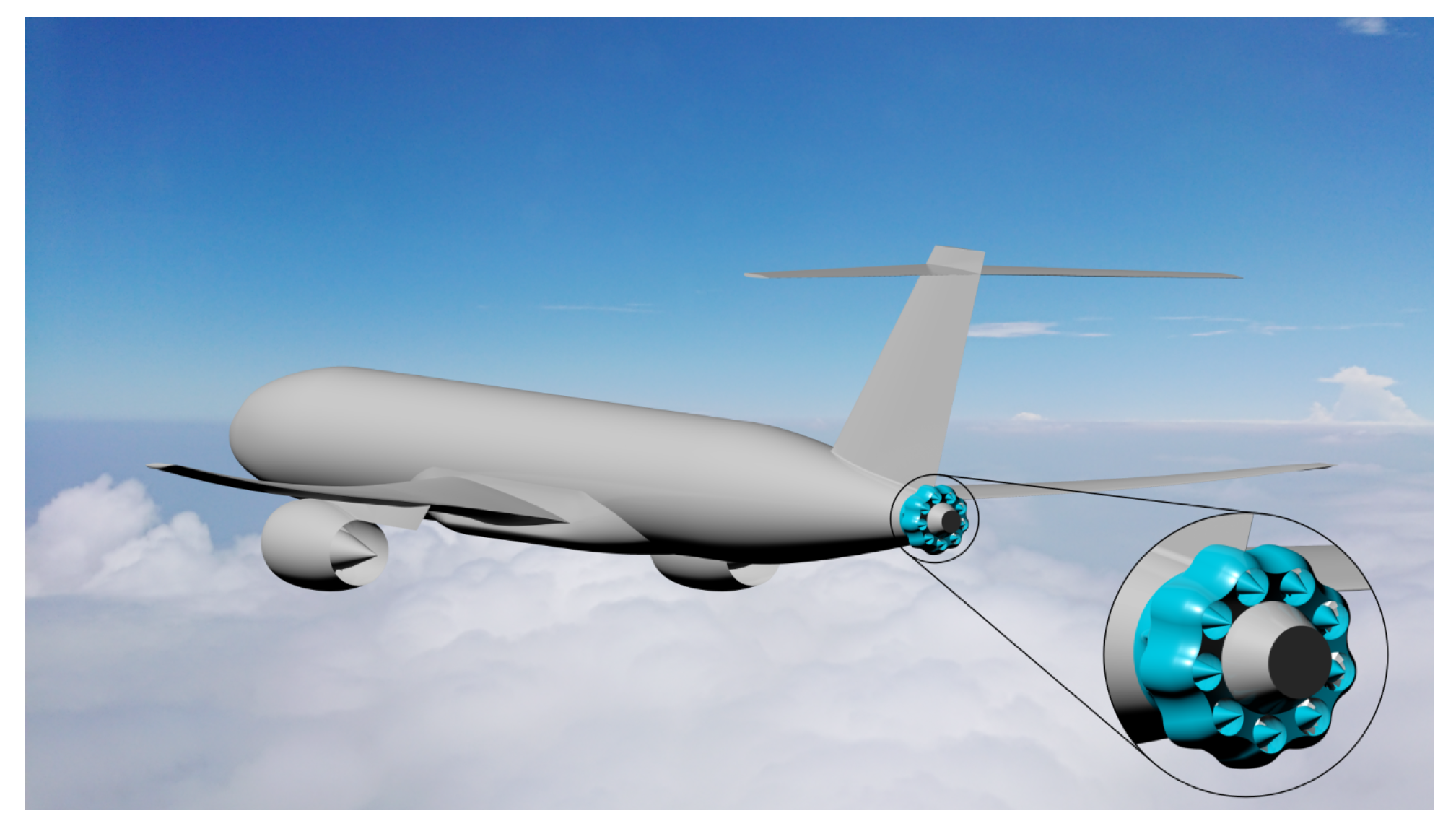
1. Introduction
2. Computational Method
2.1. Computational Simulations
2.2. Application of Power Balance Method
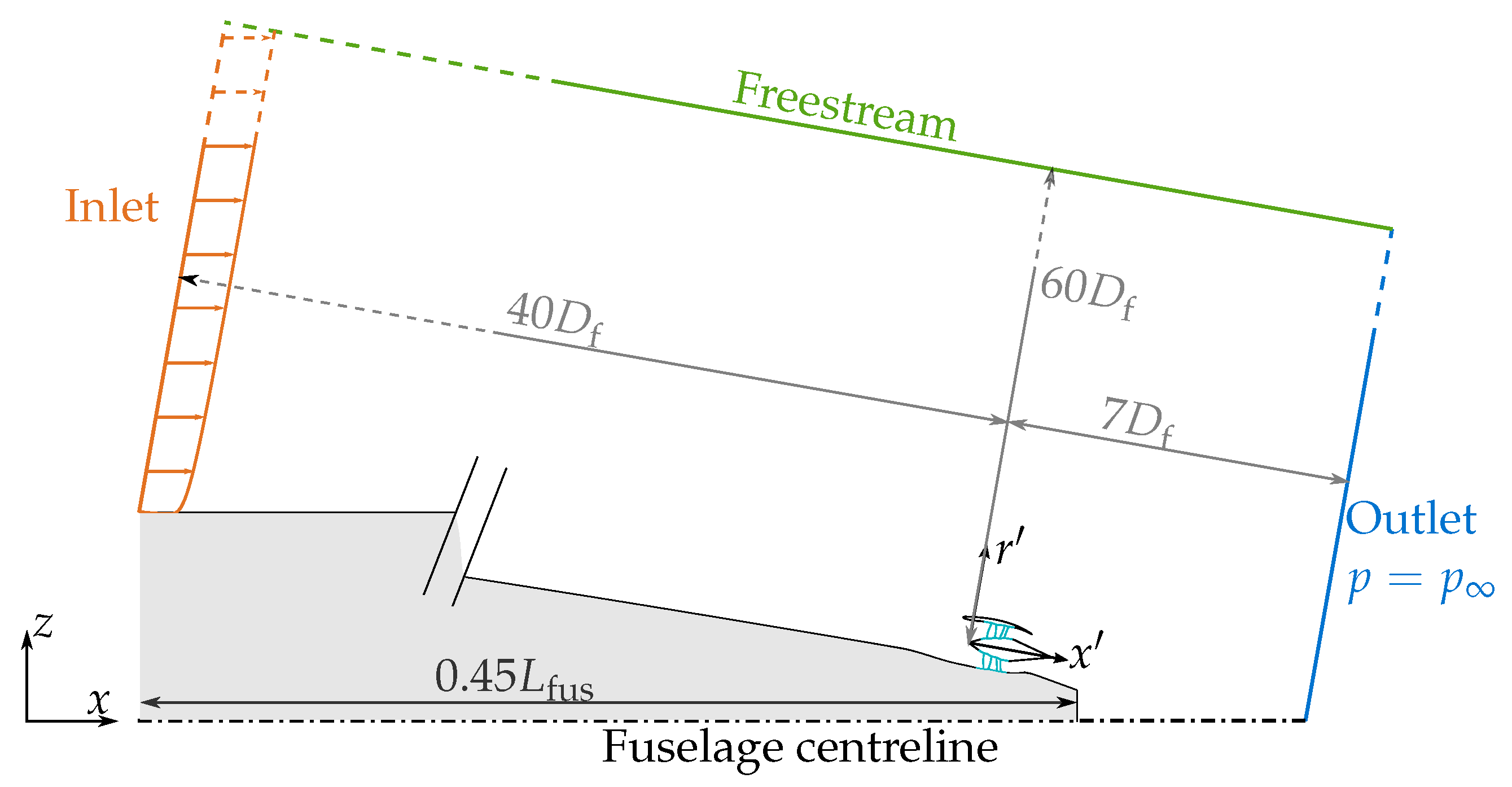
2.2.1. Control Volume
2.2.2. Formulation
3. Test Cases
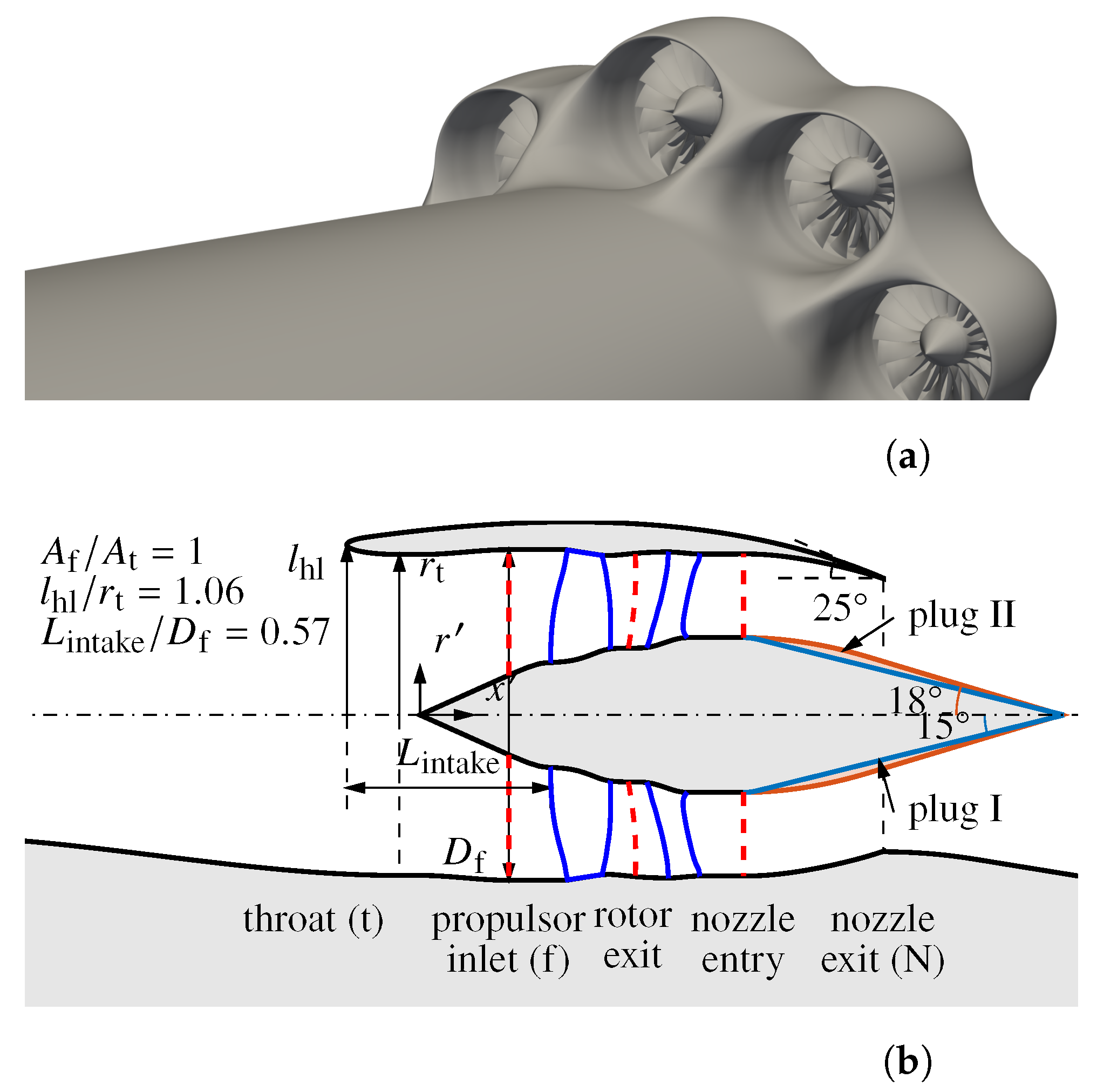
3.1. Installation and Fan Geometries
3.2. Operating Conditions
4. Flow Field
4.1. Fuselage Contraction
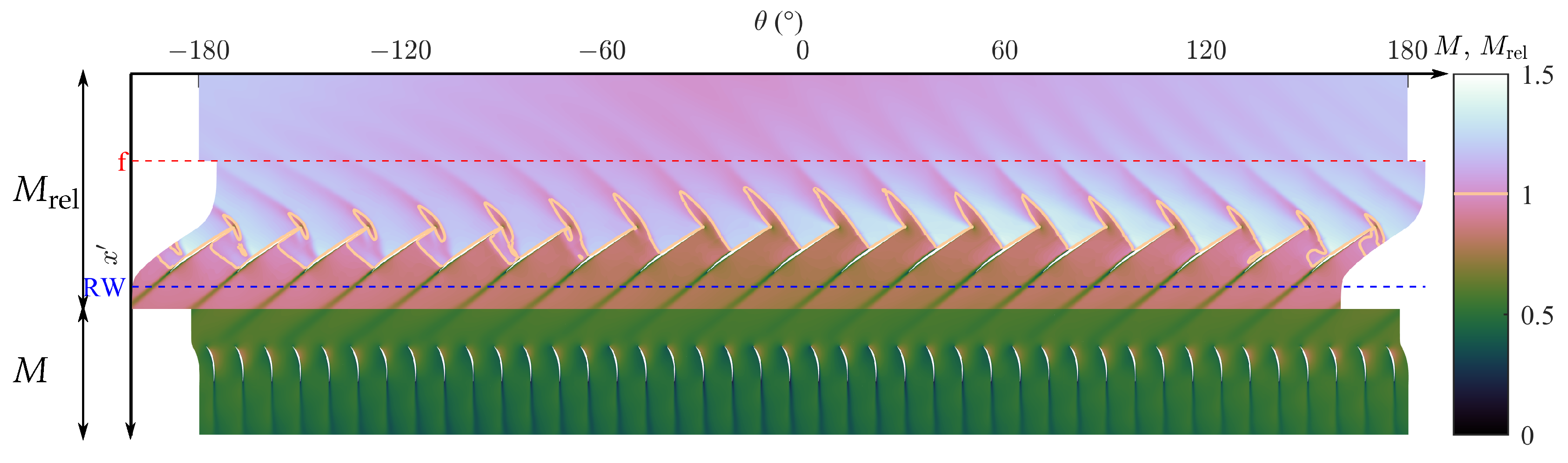
4.2. Cowl
4.3. Exhaust Jet and After-Body
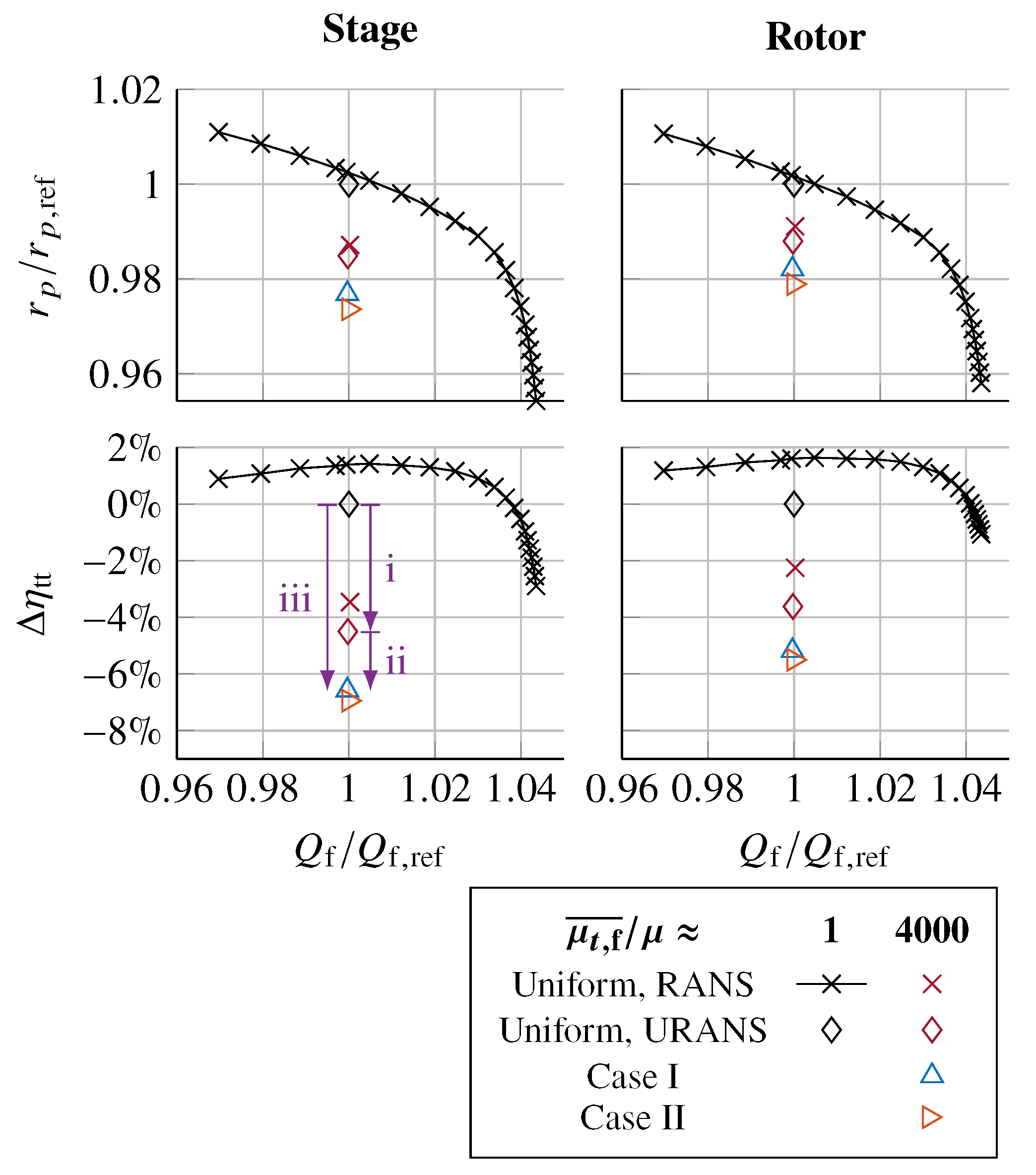
4.4. Fan Aerodynamics
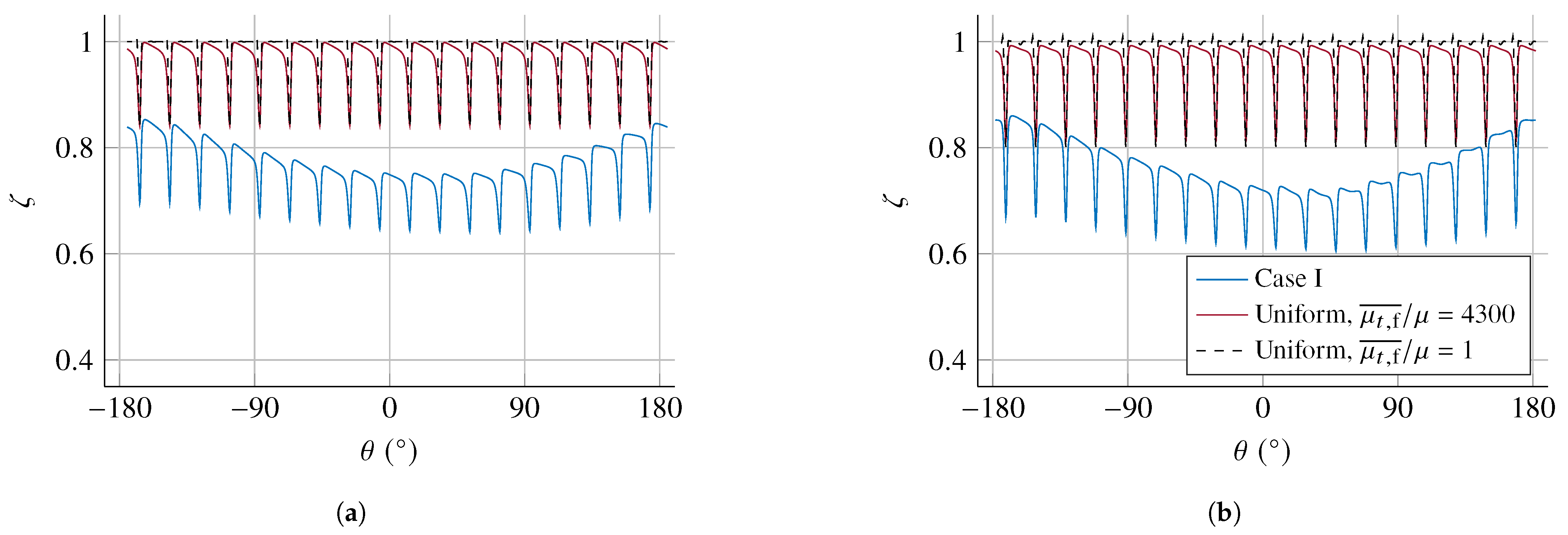
4.4.1. Effect of Fuselage BL Turbulence
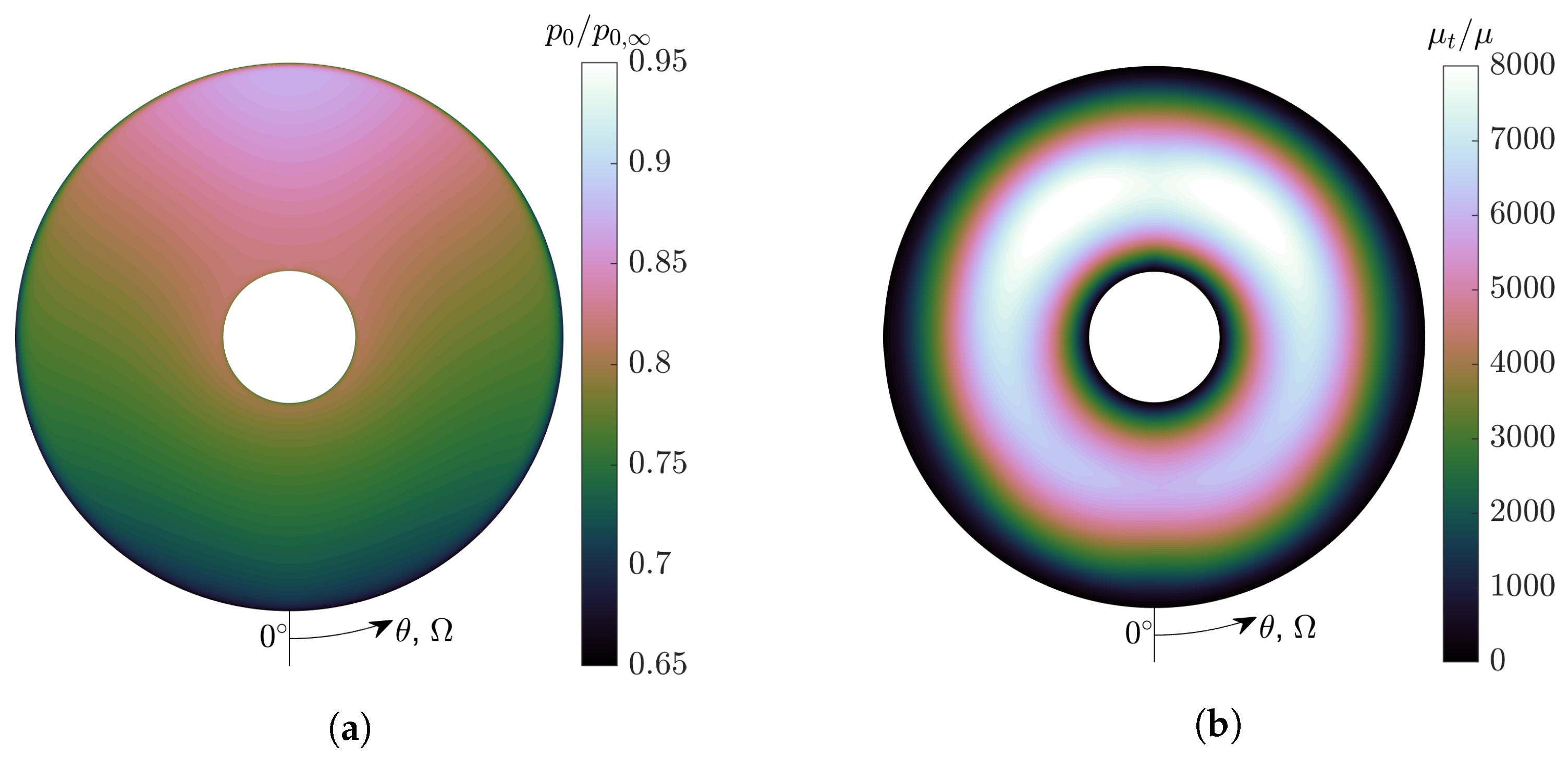
4.4.2. Inlet Distortion
4.4.3. Internal Flow Field
5. Mechanical Power Balance
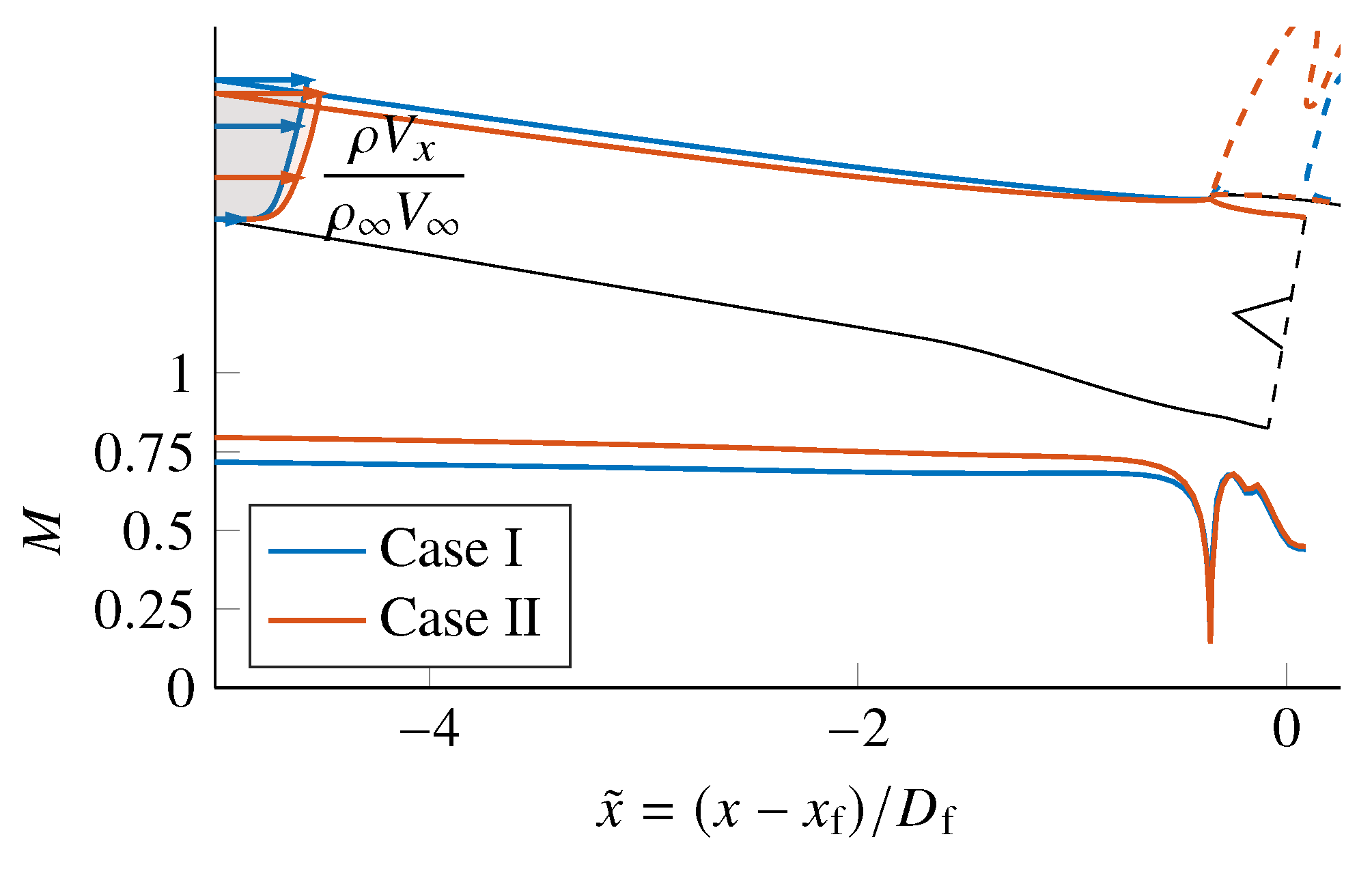
 ). This implies that the reversed flow takes part in the mixing with the jet, which has a higher velocity than case I. The higher velocity gradient increases the shear stress and, therefore, dissipation. The stronger shock and flow separation over the cowl has approximately tripled the dissipation in region B, but the contribution to the total dissipation remains small. Down the fuselage ramp, the dissipation also increases because the velocity gradient is higher in the thinner boundary layer.
). This implies that the reversed flow takes part in the mixing with the jet, which has a higher velocity than case I. The higher velocity gradient increases the shear stress and, therefore, dissipation. The stronger shock and flow separation over the cowl has approximately tripled the dissipation in region B, but the contribution to the total dissipation remains small. Down the fuselage ramp, the dissipation also increases because the velocity gradient is higher in the thinner boundary layer.6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Area |
| A∞ | Area of the captured streamtube at upstream freestream condition, i.e. |
| At | Area at the intake throat |
| Power coefficient, | |
| Df | Propulsor diameter at propulsor inlet |
| Mechanical energy deposition rate | |
| Viscous stress work deposition rate | |
| Pressure work deposition rate | |
| Kinetic energy deposition rate | |
| F | Net force acting on the aircraft portion surrounded by the control volume |
| H | Boundary layer shape factor |
| h | Height from surface |
| h0 | Specific total enthalpy |
| i | Incidence angle, positive if stagnation point is on blade pressure surface |
| Lfus | Fuselage length |
| lhl | Distance between intake highlight and propulsor rotational axis |
| Lintake | Intake length |
| Mass flow rate | |
| M; Mrel | Mach number; Mach number in the relative frame of reference |
| Outward surface normal unit vector | |
| N | Number of BLI propulsors in the aircraft concept |
| NΩ | Normalised fan rotational speed, NΩ = ΩDf/ |
| p | Pressure |
| PS | Propulsor shaft power |
| PV | Net pressure-volume power |
| q∞ | Freestream dynamic head, |
| Qf | Non-dimensional mass flow rate at propulsor inlet, Qf = |
| rp | Total-total pressure ratio, rp = |
| rT | Total-total temperature ratio, rT = |
| Surface | |
| Stationary viscous walls | |
| Moving surfaces | |
| s | Specific entropy |
| VMW | Wall velocity vector |
| V; V | Velocity vector; velocity magnitude |
| Control volume | |
| Non-dimensional distance from propulsor inlet, | |
| x’, r’, θ | Propulsor-centric cylindrical coordinate system, centred at fan spinner tip |
| x, y, z | Fuselage-centric Cartesian coordinate system |
| δ | Boundary layer thickness |
| δ* | Boundary layer displacement thickness, |
| Entropy function relative to freestream, | |
| ηtt | Total-total isentropic efficiency, ηtt = |
| μ; μt | Dynamic viscosity; turbulent/eddy viscosity |
| Spalart-Allmaras (S-A) turbulence model working variable | |
| ρ | Density |
| ; τ | Viscous stress tensor; surface viscous stress vector τ = |
| Φ | Viscous dissipation rate |
| ϕ | Rate of dissipation per unit volume, ϕ = |
| Ω | Fan rotational speed |
| Averaged quantity | |
| Stagnation quantity | |
| Freestream quantity | |
| Quantity at exit. For rotor and stage quantites, they are evaluated at rotor exit and nozzle entry planes respectively | |
| Quantity relating to the external boundaries | |
| Quantity at the boundary layer edge | |
| Quantity at propulsor inlet | |
| Quantity relating to in/out flow boundaries | |
| Isentropic quantity | |
| Quantity at nozzle exit | |
| Quantity relating to the propulsor | |
| BL | Boundary layer |
| BLI | Boundary layer ingestion |
| CAD | Computer-aided design |
| CFD | Computational fluid dynamics |
| CRM | Common Research Model |
| CV | Control volume |
| LES | Large eddy simulation |
| RANS | Reynolds-averaged Navier-Stokes |
| TKE | Turbulent kinetic energy |
| URANS | Unsteady Reynolds-averaged Navier-Stokes |
Appendix A. Grid Dependency Study

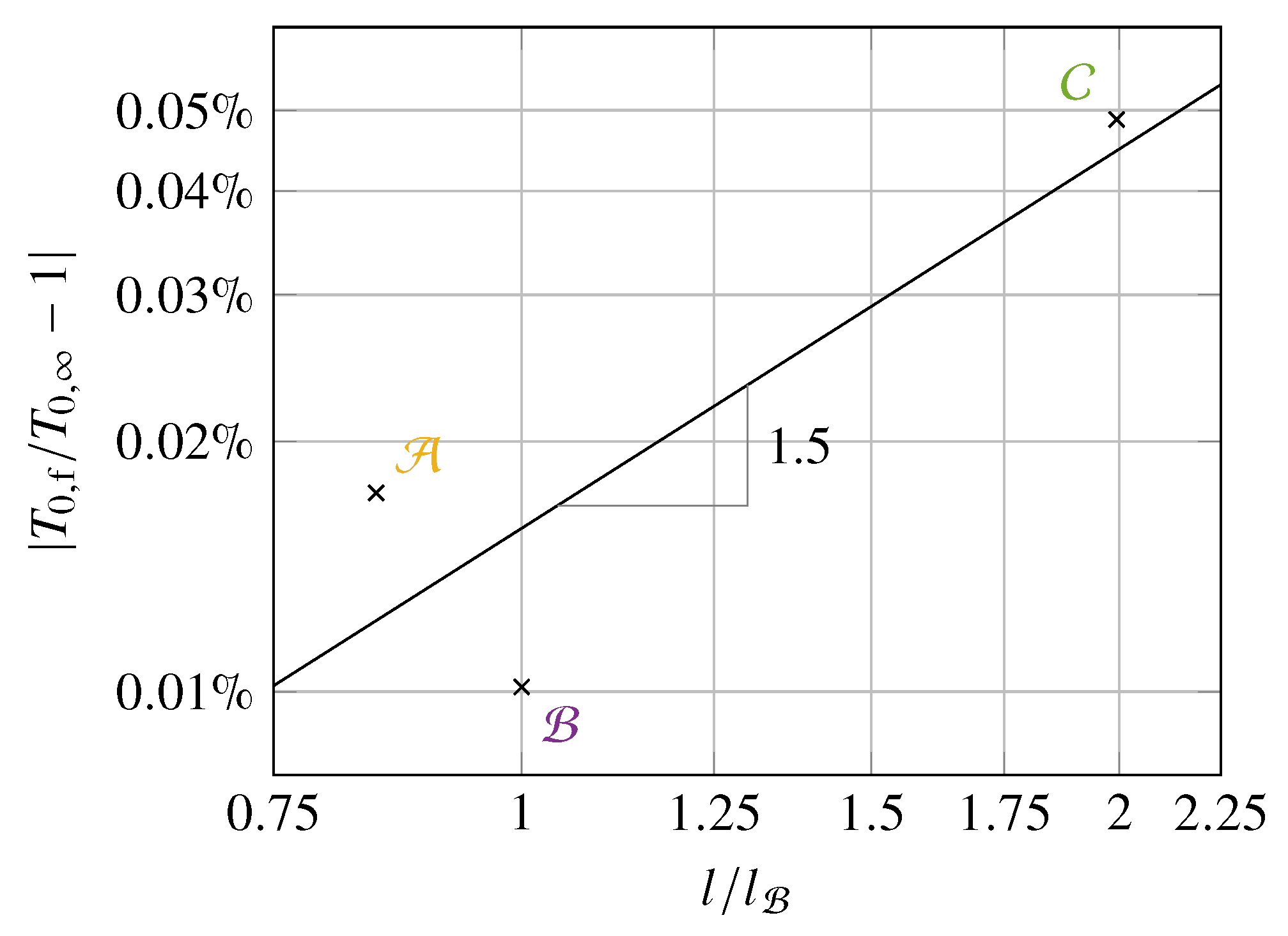
Appendix B. Power Balance Method
References
- Tse, T.S.; Hall, C.A. Flow Field and Power Balance of a Distributed Aft-fuselage Boundary Layer Ingesting Aircraft. In Proceedings of the AIAA Propulsion and Energy 2020 Forum, Virtual Event, 24–28 August 2020. [Google Scholar] [CrossRef]
- Hileman, J.I.; Spakovszky, Z.S.; Drela, M.; Sargeant, M.A.; Jones, A. Airframe Design for Silent Fuel-Efficient Aircraft. J. Aircr. 2010, 47, 956–969. [Google Scholar] [CrossRef]
- Drela, M. Development of the D8 Transport Configuration. In Proceedings of the 29th AIAA Applied Aerodynamics Conference, Honolulu, HI, USA, 27–30 June 2011. [Google Scholar] [CrossRef]
- Welstead, J.; Felder, J.L. Conceptual Design of a Single-Aisle Turboelectric Commercial Transport with Fuselage Boundary Layer Ingestion. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016. [Google Scholar] [CrossRef]
- Seitz, A.; Habermann, A.L.; Peter, F.; Troeltsch, F.; Castillo Pardo, A.; Della Corte, B.; van Sluis, M.; Goraj, Z.; Kowalski, M.; Zhao, X.; et al. Proof of Concept Study for Fuselage Boundary Layer Ingesting Propulsion. Aerospace 2021, 8, 16. [Google Scholar] [CrossRef]
- Seitz, A.; Gologan, C. Parametric design studies for propulsive fuselage aircraft concepts. CEAS Aeronaut. J. 2015, 6, 69–82. [Google Scholar] [CrossRef]
- Steiner, H.J.; Seitz, A.; Wieczorek, K.; Plötner, K.; Isikveren, A.T.; Hornung, M. Multi-disciplinary design and feasibility study of distributed propulsion systems. In Proceedings of the 28th International Congress of the Aeronautical Sciences (ICAS), Brisbane, Australia, 23–28 September 2012. [Google Scholar]
- National Academies of Sciences, Engineering, and Medicine. Commercial Aircraft Propulsion and Energy Systems Research; The National Academies Press: Washington, DC, USA, 2016. [Google Scholar] [CrossRef]
- Cumpsty, N.; Heyes, A. Jet Propulsion, 3rd ed.; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar] [CrossRef]
- Epstein, A.H.; O’Flarity, S.M. Considerations for Reducing Aviation’s CO2 with Aircraft Electric Propulsion. J. Propuls. Power 2019, 35, 572–582. [Google Scholar] [CrossRef]
- Jansen, R.H.; Celestina, M.L.; Kim, H.D. Electrical Propulsive Fuselage Concept for Transonic Transport Aircraft. In Proceedings of the 24th ISABE Conference, Canberra, Australia, 22–27 September 2019. [Google Scholar]
- Plas, A.P.; Sargeant, M.A.; Madani, V.; Crichton, D.; Greitzer, E.M.; Hynes, T.P.; Hall, C.A. Performance of a Boundary Layer Ingesting (BLI) propulsion system. In Proceedings of the 45th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2007; Volume 8, pp. 5368–5388. [Google Scholar] [CrossRef]
- Gunn, E.J.; Hall, C.A. Aerodynamics of Boundary Layer Ingesting Fans. In Proceedings of the ASME Turbo Expo 2014: Turbine Technical Conference and Exposition, Düsseldorf, Germany, 16–20 June 2014;A; Volume 1A, p. V01AT01A024. [Google Scholar] [CrossRef]
- Gunn, E. Aerodynamics of Boundary Layer Ingesting Fans. Ph.D. Thesis, University of Cambridge, Cambridge, UK, 2015. [Google Scholar]
- Giesecke, D.; Friedrichs, J. Aerodynamic Comparison Between Circumferential and Wing-Embedded Inlet Distortion for an Ultra-High Bypass Ratio Fan Stage. In Proceedings of the ASME Turbo Expo 2019: Turbomachinery Technical Conference and Exposition, Phoenix, AZ, USA, 17–21 July 2019. [Google Scholar] [CrossRef]
- Drela, M. Power Balance in Aerodynamic Flows. AIAA J. 2009, 47, 1761–1771. [Google Scholar] [CrossRef]
- Brandvik, T.; Pullan, G. An Accelerated 3D Navier–Stokes Solver for Flows in Turbomachines. J. Turbomach. 2011, 133, 021025. [Google Scholar] [CrossRef]
- Spalart, P.R.; Allmaras, S.R. A One-Equation Turbulence Model for Aerodynamic Flows. La Recherche Aérospatiale 1994, 5–21. [Google Scholar]
- Liu, Y.; Lu, L.; Fang, L.; Gao, F. Modification of Spalart–Allmaras Model with Consideration of Turbulence Energy Backscatter Using Velocity Helicity. Phys. Lett. A 2011, 375, 2377–2381. [Google Scholar] [CrossRef]
- Vassberg, J.C.; Tinoco, E.N.; Mani, M.; Rider, B.; Zickuhr, T.; Levy, D.W.; Brodersen, O.P.; Eisfeld, B.; Crippa, S.; Wahls, R.A.; et al. Summary of the Fourth AIAA Computational Fluid Dynamics Drag Prediction Workshop. J. Aircr. 2014, 51, 1070–1089. [Google Scholar] [CrossRef]
- Tinoco, E.N.; Brodersen, O.P.; Keye, S.; Laflin, K.R.; Feltrop, E.; Vassberg, J.C.; Mani, M.; Rider, B.; Wahls, R.A.; Morrison, J.H.; et al. Summary Data from the Sixth AIAA CFD Drag Prediction Workshop: CRM Cases. J. Aircr. 2018, 55, 1352–1379. [Google Scholar] [CrossRef]
- Masaki, A.; Ogushi, S.; Tsuruta, R.; Nishiwaki, D.; Sato, T.; Okai, K.; Kazawa, J.; Masaki, D.; Harada, M. Assessment of the Influence of Boundary Layer Ingestion (BLI) on the Axial Fan. J. Phys. Conf. Ser. 2021, 1909, 012081. [Google Scholar] [CrossRef]
- Jerez Fidalgo, V. Fan-Distortion Interaction in Novel Aircraft Installations. Ph.D. Thesis, University of Cambridge, Cambridge, UK, 2012. [Google Scholar]
- Jameson, A. Time Dependent Calculations Using Multigrid, with Applications to Unsteady Flows Past Airfoils and Wings. In Proceedings of the 10th Computational Fluid Dynamics Conference, Honolulu, HI, USA, 24–26 June 1991; p. 1596. [Google Scholar] [CrossRef]
- Ayachit, U. The ParaView Guide; Kitware Inc.: Clifton Park, NY, USA, 2019; Updated for ParaView version 5.6. [Google Scholar]
- Pandya, S.A.; Uranga, A.; Espitia, A.; Huang, A. Computational Assessment of the Boundary Layer Ingesting Nacelle Design of the D8 Aircraft. In Proceedings of the 52nd Aerospace Sciences Meeting, National Harbor, MD, USA, 13–17 January 2014. [Google Scholar] [CrossRef]
- Maldonado, Y.B.; Giannakakis, P.; Rodriguez, B.; Tantot, N. Book-keeping investigations for BLI aircraft. In Proceedings of the AIAA Propulsion and Energy 2020 Forum, Virtual Event, 24–28 August 2020. [Google Scholar] [CrossRef]
- Vassberg, J.C.; DeHaan, M.A.; Rivers, M.S.; Wahls, R.A. Retrospective on the Common Research Model for Computational Fluid Dynamics Validation Studies. J. Aircr. 2018, 55, 1325–1337. [Google Scholar] [CrossRef]
- Korsia, J.J.; De Spiegeleer, G. VITAL, An European R&D Program for Greener Aero-engines. In Proceedings of the 25th International Congress of the Aeronautical Sciences, Hamburg, Germany, 3–8 September 2006. [Google Scholar]
- Spalart, P.R.; Rumsey, C.L. Effective Inflow Conditions for Turbulence Models in Aerodynamic Calculations. AIAA J. 2007, 45, 2544–2553. [Google Scholar] [CrossRef]
- Jerez Fidalgo, V.; Hall, C.A.; Colin, Y. A Study of Fan-Distortion Interaction Within the NASA Rotor 67 Transonic Stage. J. Turbomach. 2012, 134, 051011. [Google Scholar] [CrossRef]
- Heinlein, G.; Chen, J.; Bakhle, M. Aerodynamic Behavior of a Coupled Boundary Layer Ingesting Inlet – Distortion Tolerant Fan. In Proceedings of the AIAA Propulsion and Energy 2020 Forum, Virtual Event, 24–28 August 2020. [Google Scholar] [CrossRef]
- Castillo Pardo, A.; Mehdi, A.; Pachidis, V.; MacManus, D.G. Numerical Study of the Effect of Multiple Tightly-Wound Vortices on a Transonic Fan Stage Performance. In Proceedings of the ASME Turbo Expo 2014, Düsseldorf, Germany, 16–20 June 2014; Volume 1A, p. V01AT01A033. [Google Scholar] [CrossRef]
- Tucker, P.G. Trends in turbomachinery turbulence treatments. Prog. Aerosp. Sci. 2013, 63, 1–32. [Google Scholar] [CrossRef]
- Hue, D.; Chanzy, Q.; Landier, S. DPW-6: Drag Analyses and Increments Using Different Geometries of the Common Research Model Airliner. J. Aircr. 2018, 55, 1509–1521. [Google Scholar] [CrossRef]
- Sanders, D.S.; Laskaridis, P. Full-Aircraft Energy-Based Force Decomposition Applied to Boundary-Layer Ingestion. AIAA J. 2020, 58, 4357–4373. [Google Scholar] [CrossRef]

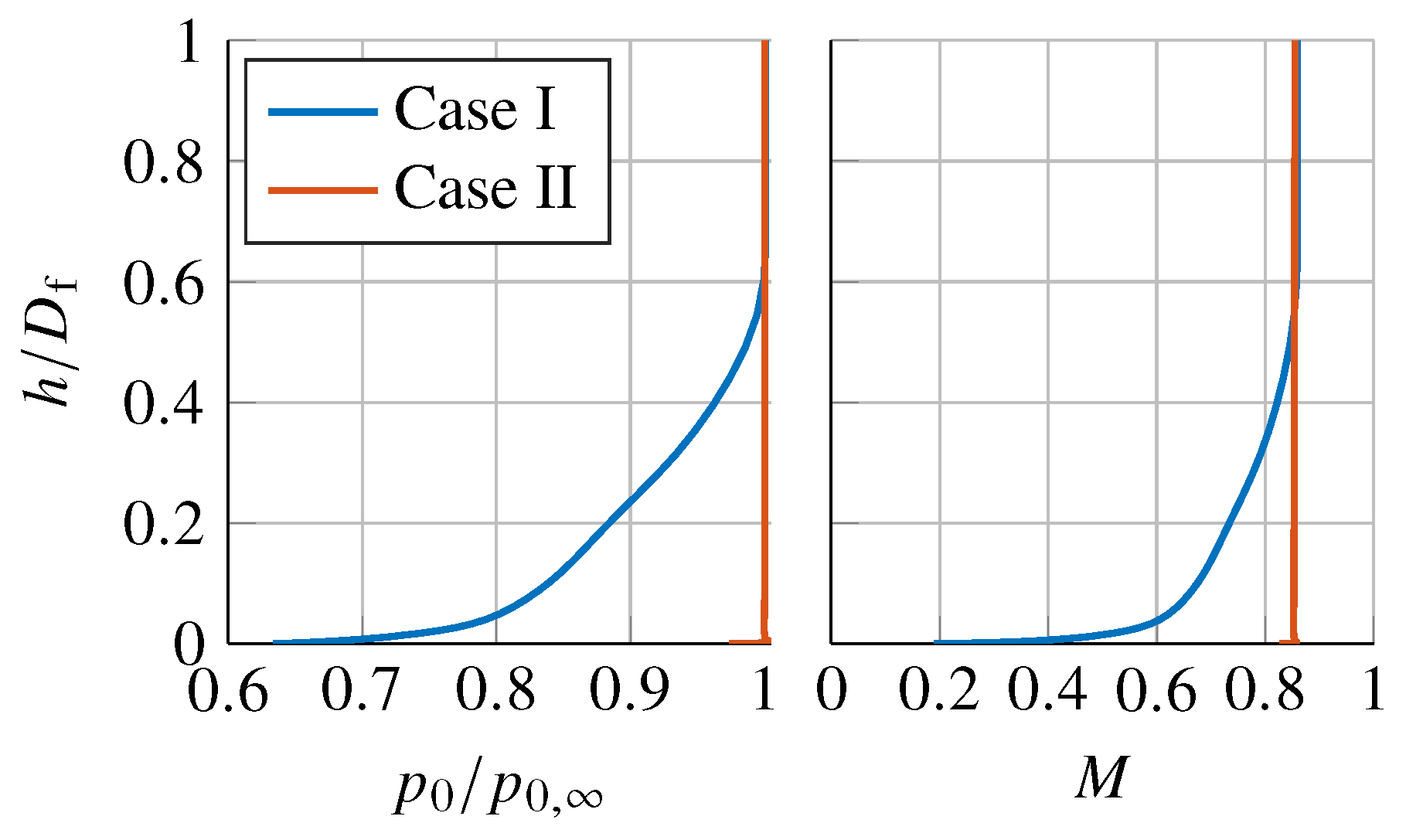
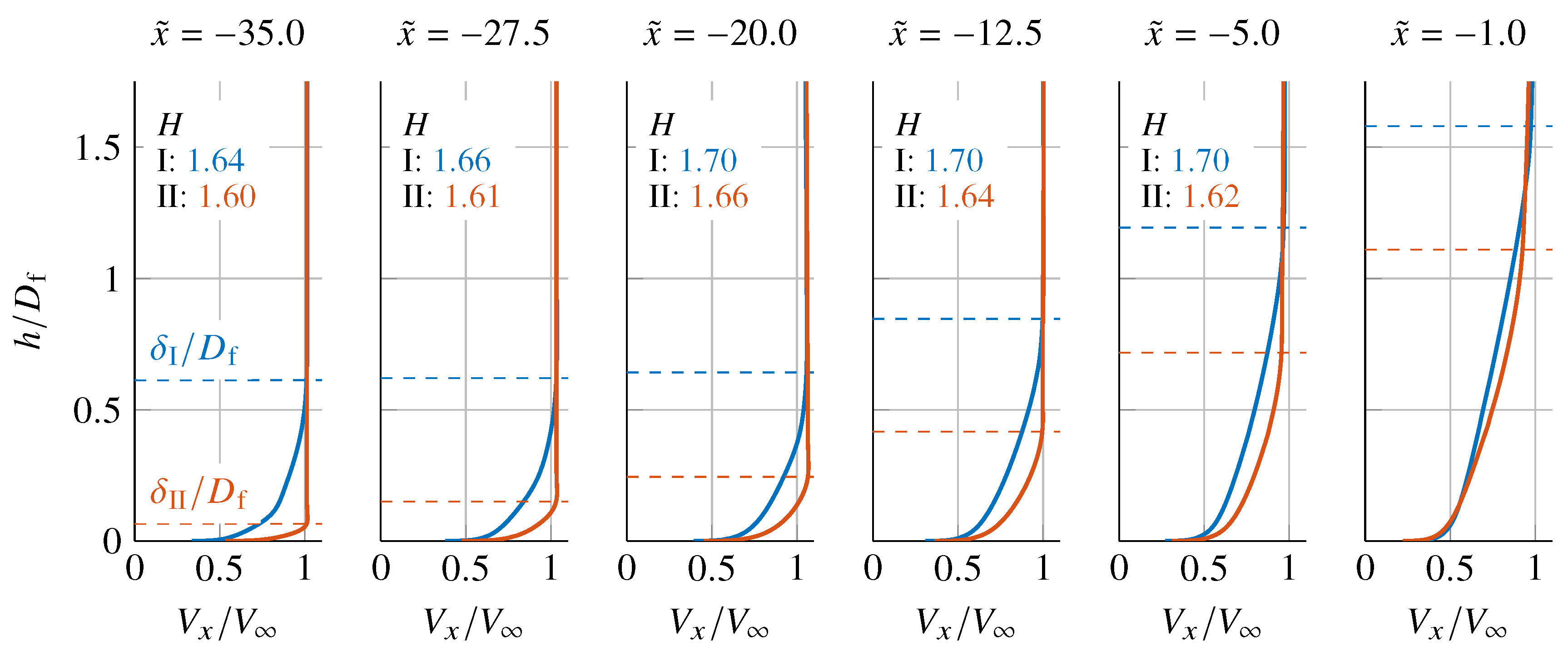


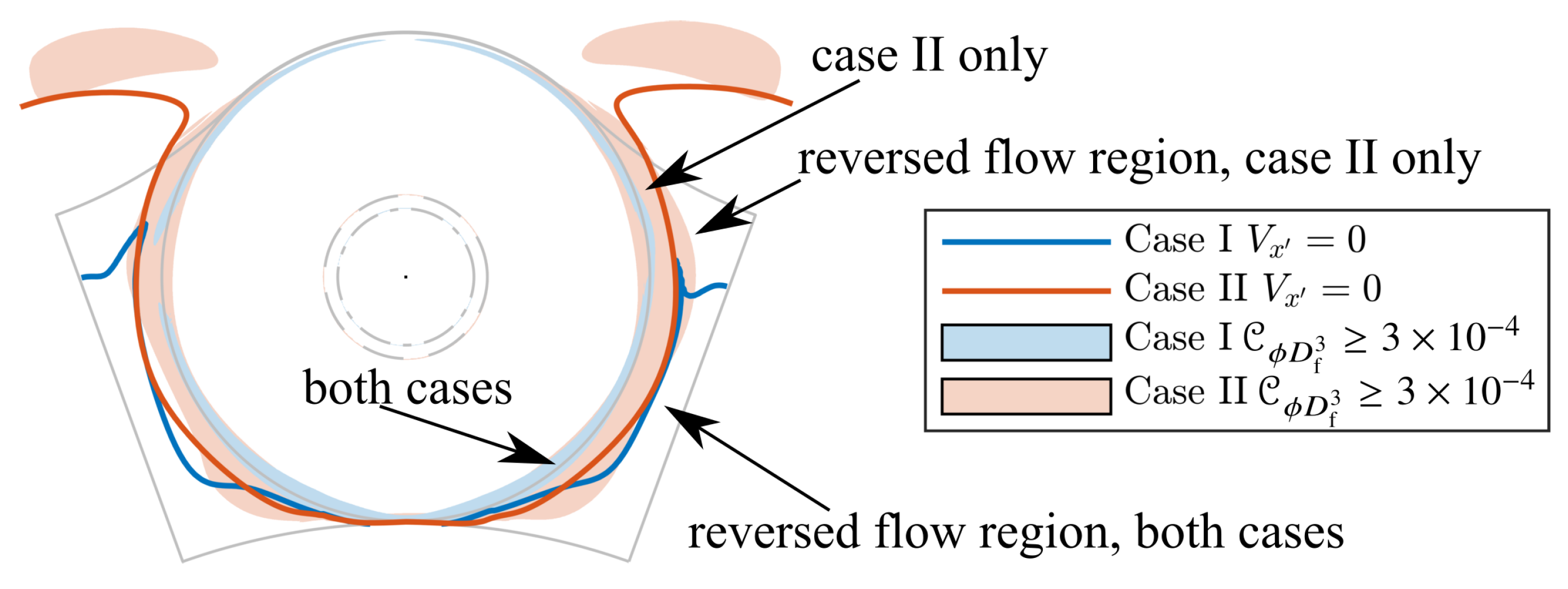
 , and case II
, and case II  .
.
 , and case II
, and case II  .
.
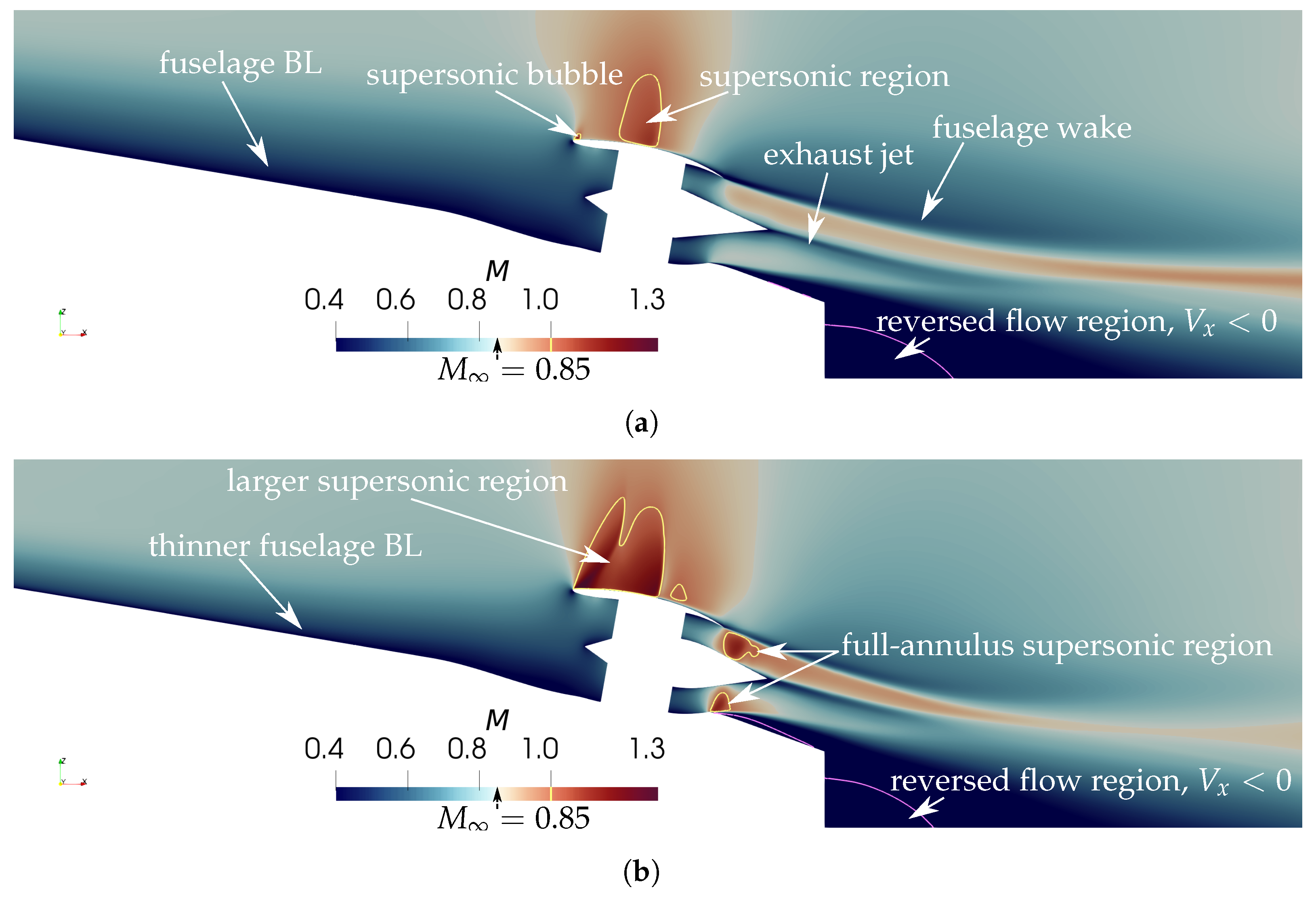
 ) denotes the cut plane for Figure 11.
) denotes the cut plane for Figure 11.
 ) denotes the cut plane for Figure 11.
) denotes the cut plane for Figure 11.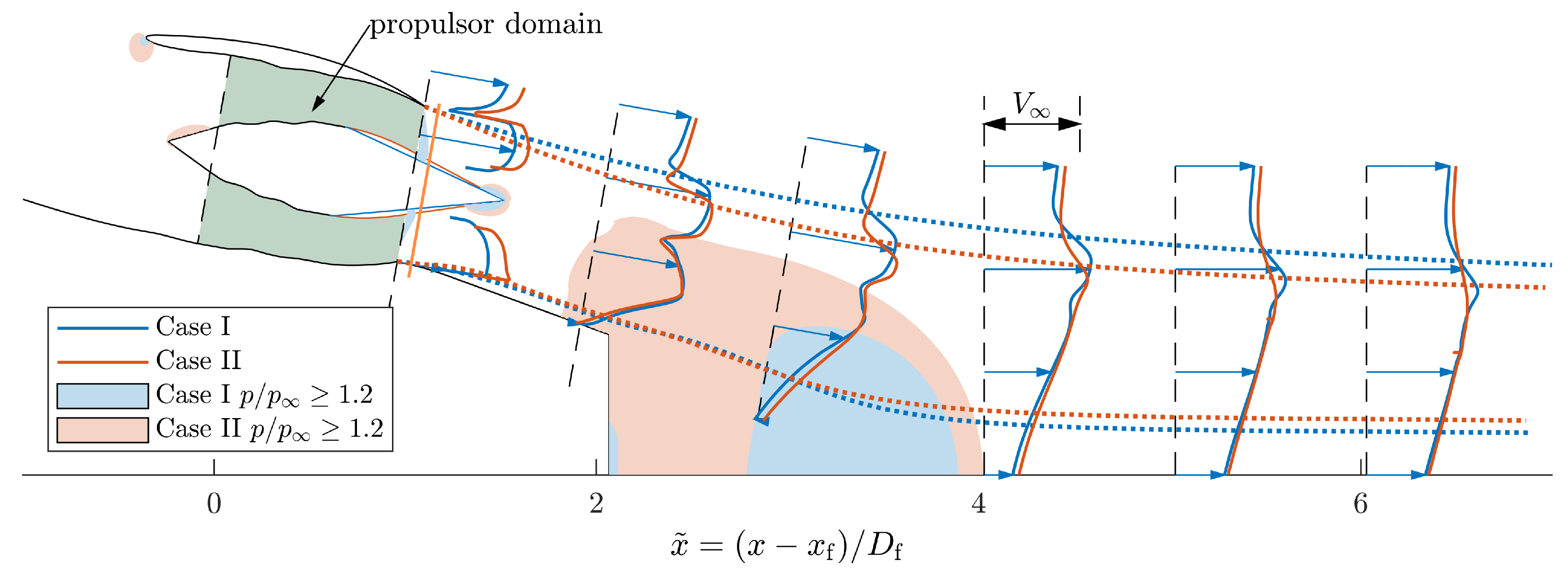


| Parameter | Value |
|---|---|
| Cruise altitude | 12 km (FL394) |
| 0.85 | |
| Pa | |
| K | |
| N s m−2 | |
| 1005 J kg−1 K−1 s | |
| 1.4 | |
| 1 | |
| ≈ [30] |
| Test Case | Inlet Profile | Intake Flow Ratio | ||
|---|---|---|---|---|
| Case I (BLI) | Realistic BL profile | 1.00 | 0.224 | 0.637 |
| Case II (Thin BL) | Uniform | 0.95 | 0.117 | 0.687 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tse, T.S.; Hall, C.A. Aerodynamics and Power Balance of a Distributed Aft-Fuselage Boundary Layer Ingesting Aircraft. Aerospace 2023, 10, 122. https://doi.org/10.3390/aerospace10020122
Tse TS, Hall CA. Aerodynamics and Power Balance of a Distributed Aft-Fuselage Boundary Layer Ingesting Aircraft. Aerospace. 2023; 10(2):122. https://doi.org/10.3390/aerospace10020122
Chicago/Turabian StyleTse, Tze Sing, and Cesare A. Hall. 2023. "Aerodynamics and Power Balance of a Distributed Aft-Fuselage Boundary Layer Ingesting Aircraft" Aerospace 10, no. 2: 122. https://doi.org/10.3390/aerospace10020122
APA StyleTse, T. S., & Hall, C. A. (2023). Aerodynamics and Power Balance of a Distributed Aft-Fuselage Boundary Layer Ingesting Aircraft. Aerospace, 10(2), 122. https://doi.org/10.3390/aerospace10020122







