Fatigue Analysis of Flexible Joint Elastomers Combining Ogden Second-Order Constitutive Model with Cracking Energy Density
Abstract
1. Introduction
2. The Calculated Expression of the CED for Silicone Rubber Elastomers
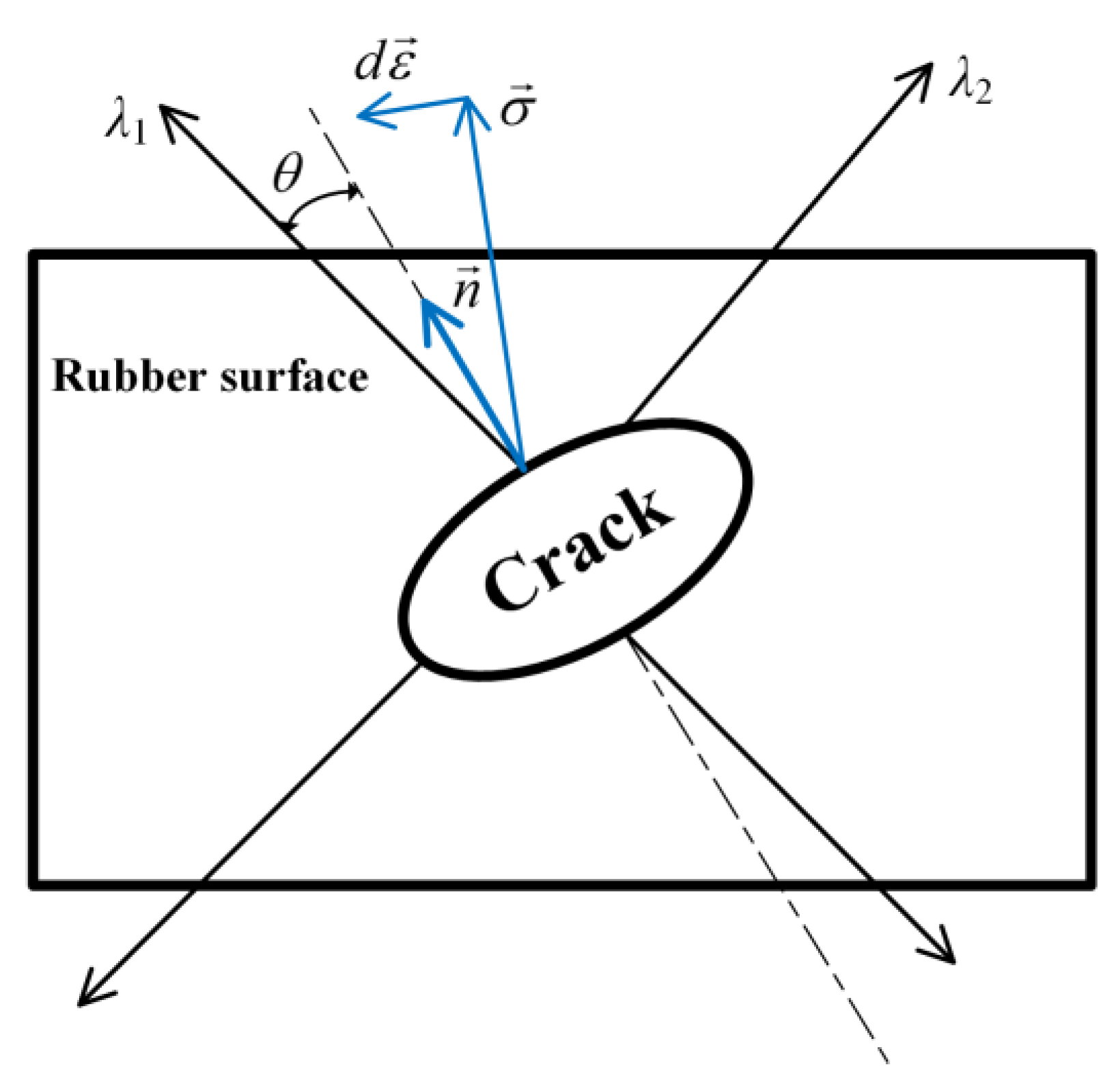
2.1. General Expression of CED Increment under Finite Strain and Non-Linear Elastic Conditions
2.2. The CED Expression of Elastomers Using Ogden Second-Order Constitutive Model
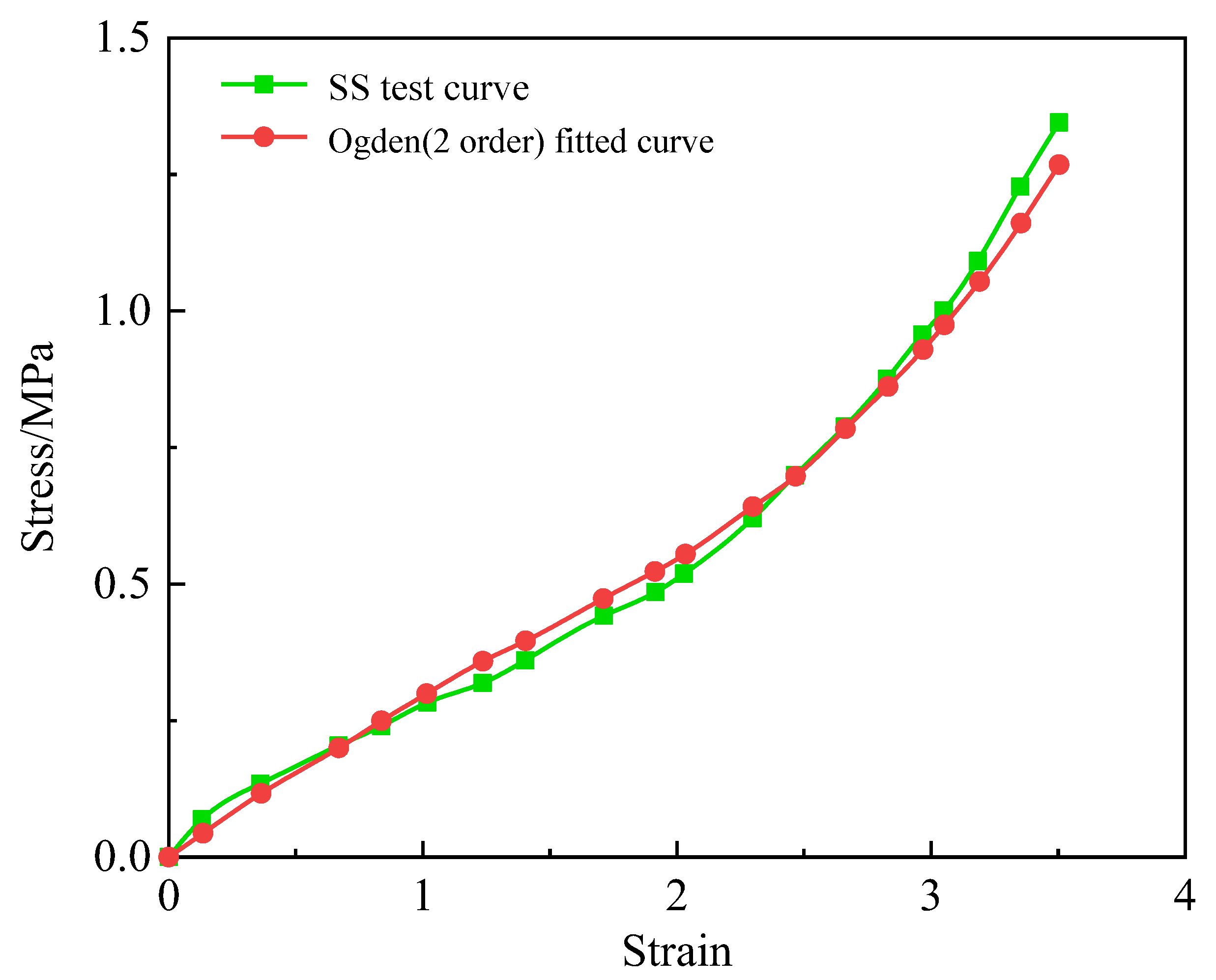
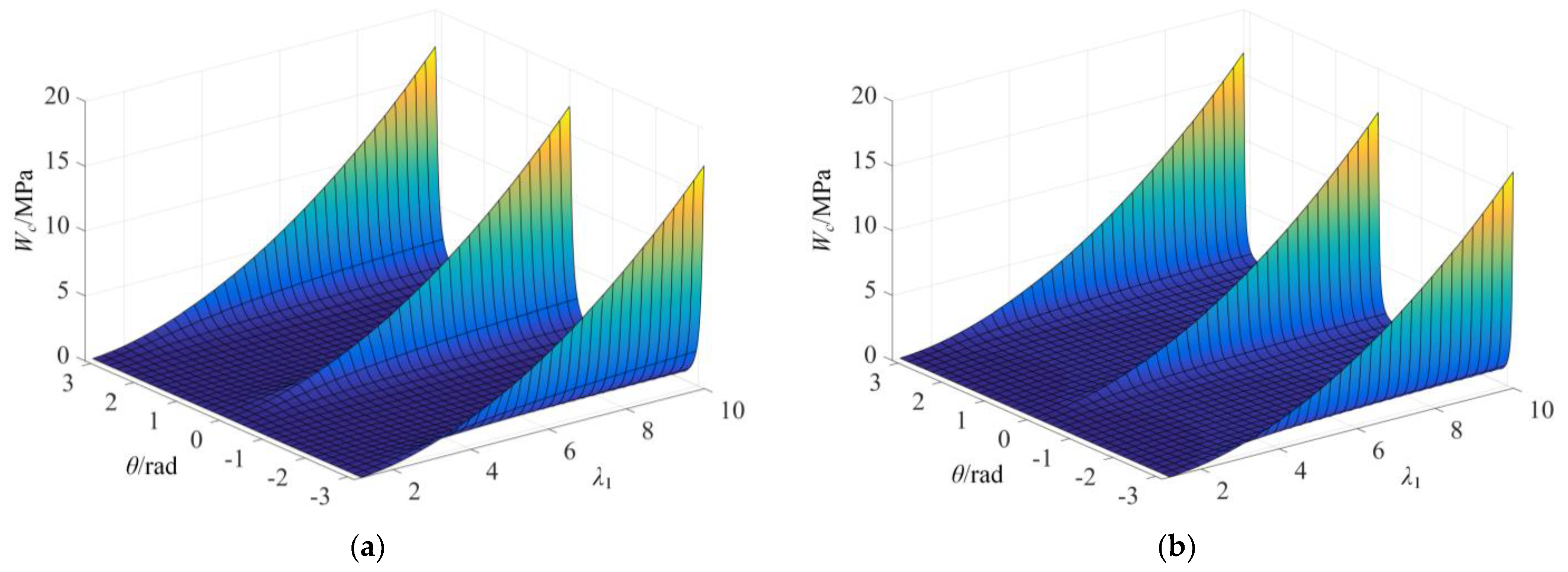
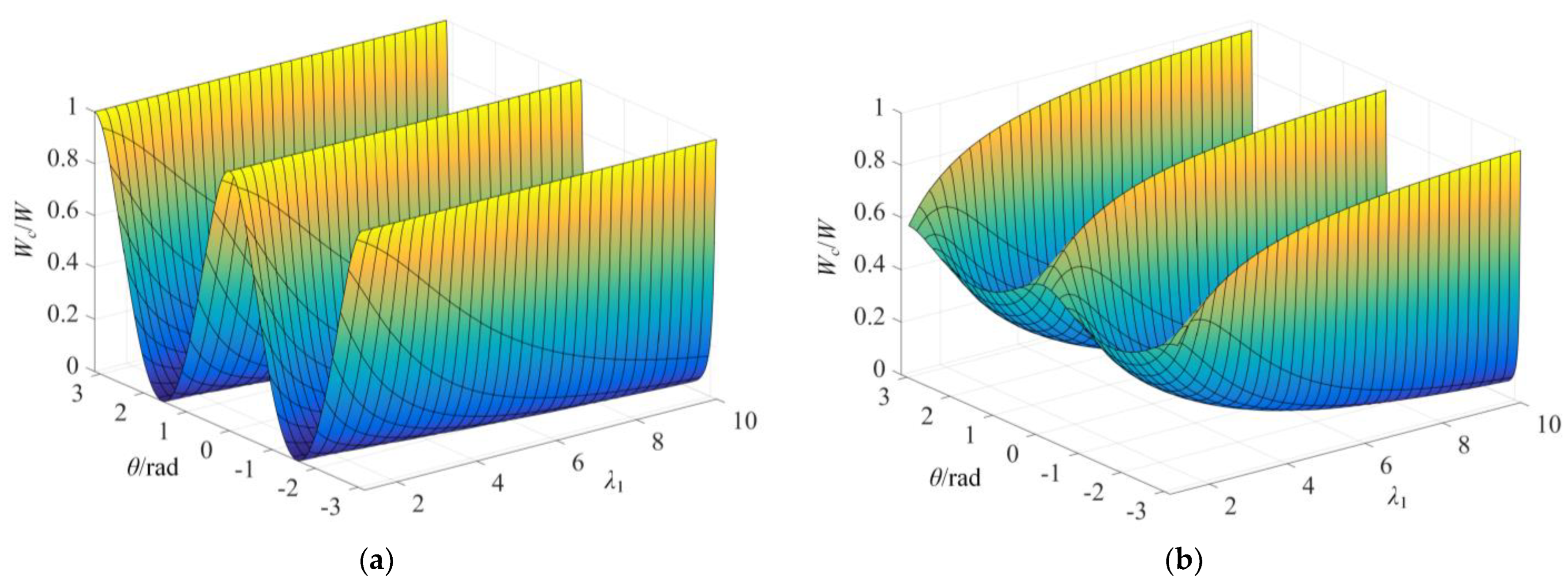
2.3. Verification of CED Distribution for Elastomer Material under Typical Strain State
3. Fatigue Life Prediction of the Flexible Joint Elastomers
3.1. Fatigue Life Calculation of Silicone Rubber with CED
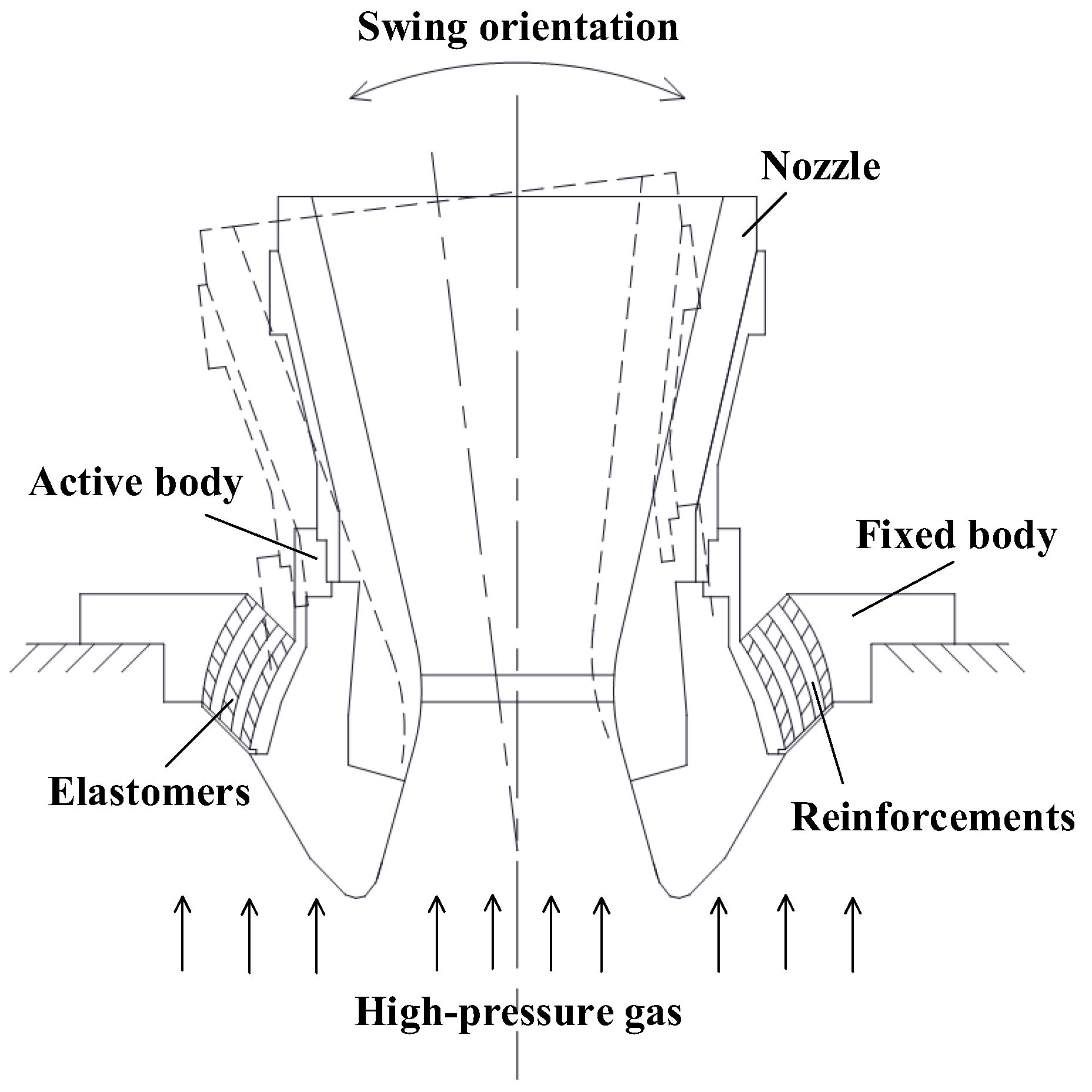
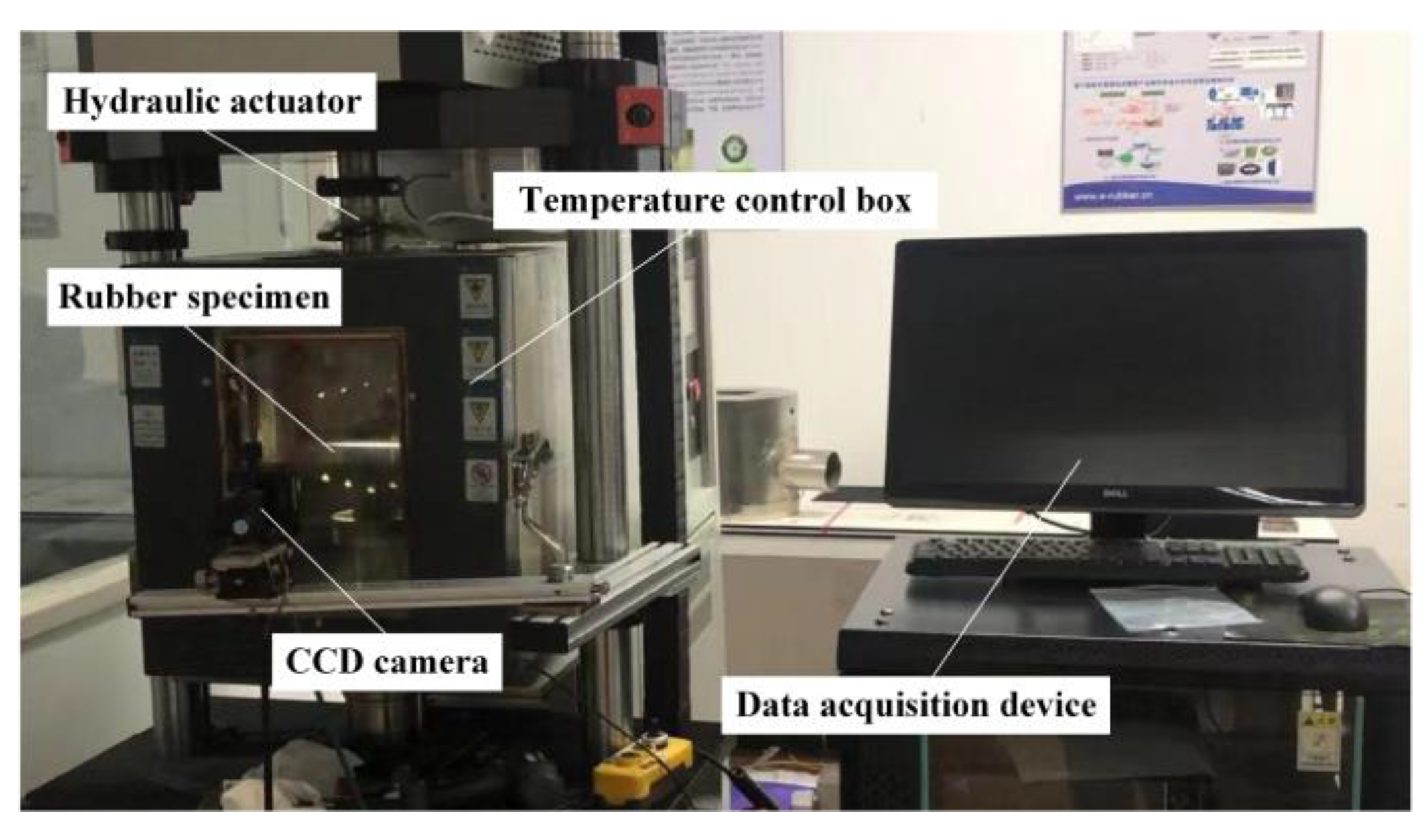
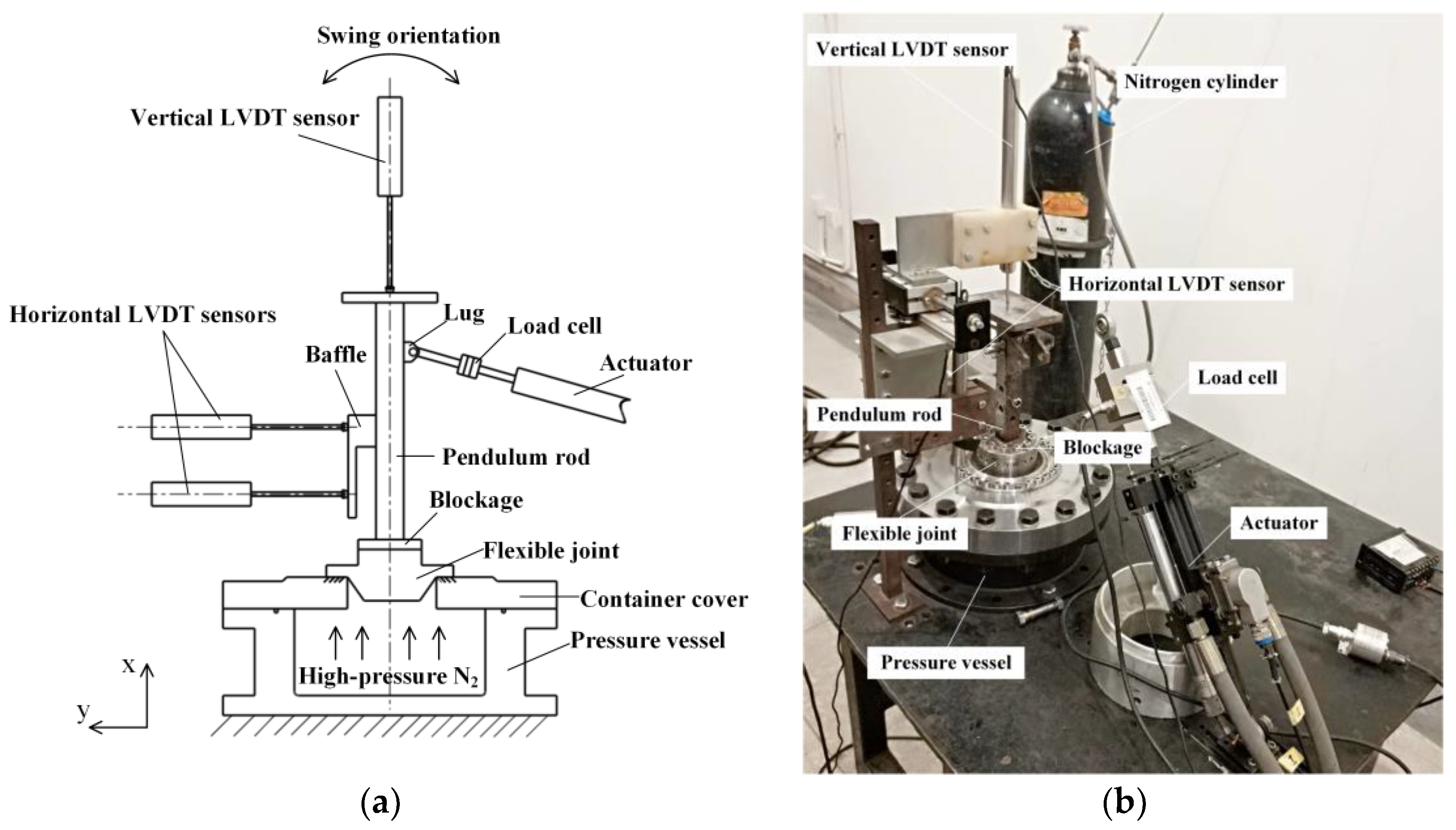
3.2. Swing Fatigue Life Test of the Flexible Joint
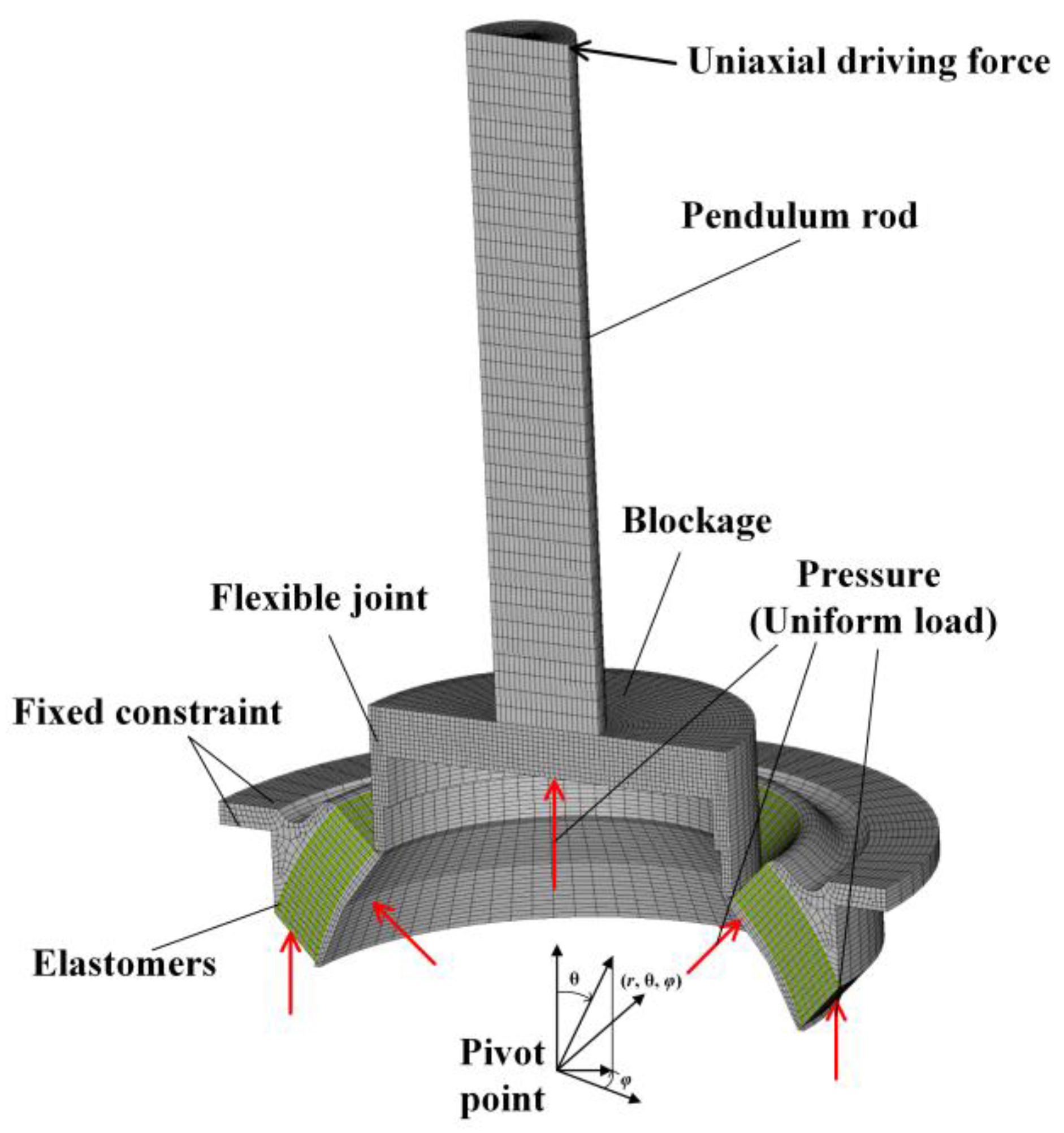
3.3. Finite Element Model of the Flexible Joint
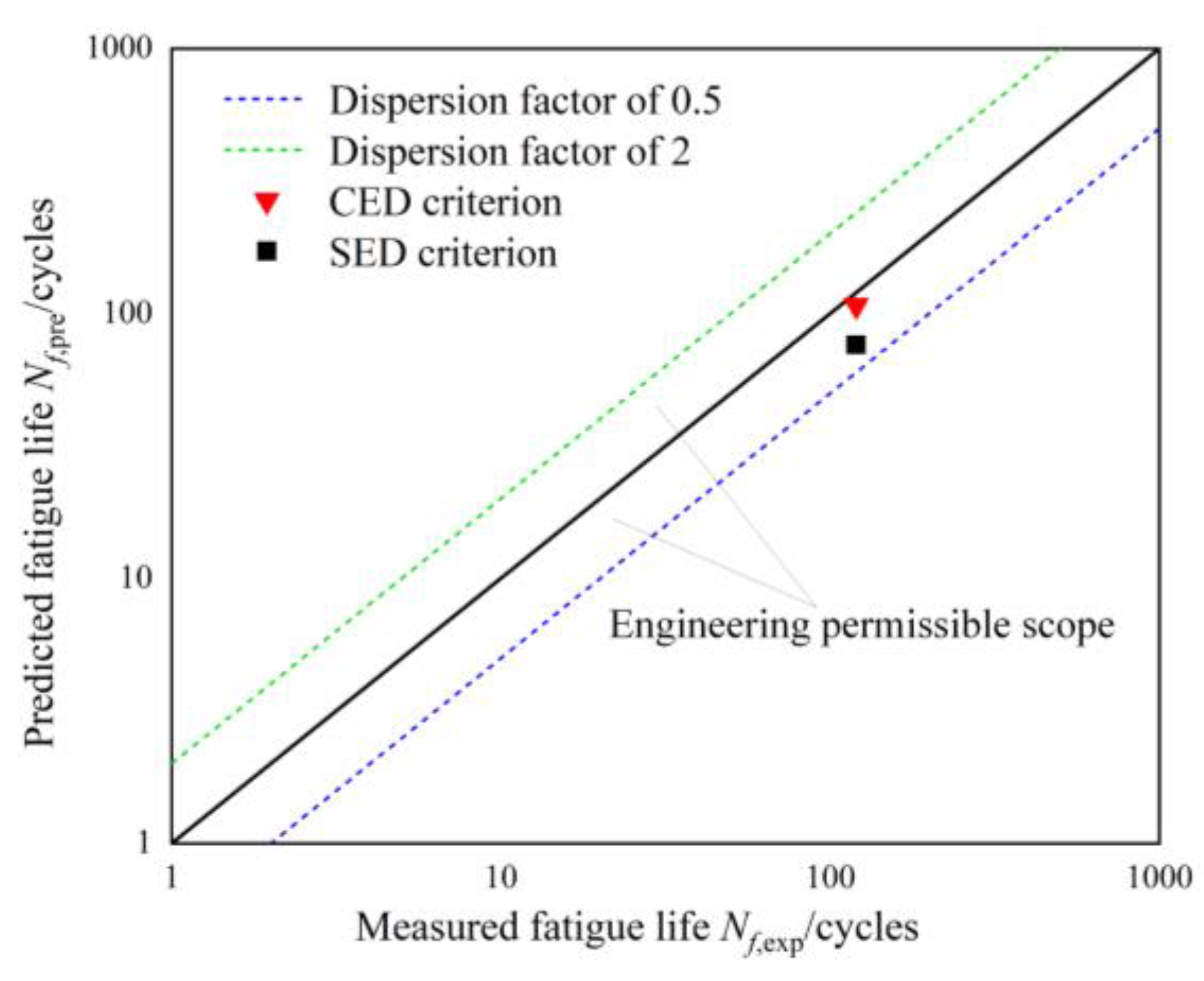
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| b | biaxial parameter |
| CED, Wc | cracking energy density |
| right Cauchy–Green strain tensor | |
| CCD | charge coupled device |
| c | crack length |
| c0 | inherent crack length |
| cf | permissible crack length |
| dc/dN | crack growth rate |
| dWc | increment of Wc |
| increment of the composite strain | |
| F0 | material constant determined using the least squares method |
| FEA/FE | finite element analysis/finite element |
| f [T(c,t)] | crack growth model of rubber material |
| J | elastic volume ratio before and after deformation |
| LVDT | linear variable differential transformer |
| Nf,pre | predicted life of the flexible joint in the finite element analysis |
| Nf,exp | measured life of the flexible joint in the swing fatigue test |
| unit normal vector of a potential cracking plane under the undeformed configuration in the Lagrange coordinate system | |
| unit normal vector of the cracking plane at a certain point in the rubber | |
| p | hydrostatic pressure in the plane stress state |
| R | ratio of minimum to maximum tearing energy |
| rc | critical crack growth rate |
| r, θ and φ | radial direction, angular direction and circumferential direction in finite element model of the flexible joint, respectively |
| SED, W | strain energy density |
| second Piola–Kirchhoff stress tensor | |
| Tc | critical tearing energy |
| Tmax | maximum tearing energy |
| t | loading time |
| θ | crack angle |
| λ1, λ2 and λ3 | first, second and third principal elongation, respectively |
| μ1, α1, μ2 and α2 | material constants independent of deformation, which are obtained by fitting the material test data |
| composite stress vector of rubber fatigue crack |
References
- Caveny, L.H.; Geisler, R.L.; Ellis, R.A.; Moore, T.L. Solid Rocket Enabling Technologies and Milestones in the United States. J. Propuls. Power 2003, 19, 1038–1066. [Google Scholar] [CrossRef]
- Ren, J.; Zhang, X.; Yang, J.; Wang, C.; Liu, Y.; Yang, W. Structural Analysis and Testing of a Miniature Flexible Joint under Pressure and Vector Loading. J. Mech. Sci. Technol. 2014, 28, 3637–3643. [Google Scholar] [CrossRef]
- Su, H.; Ren, J.; Xue, M.; Tong, Y.; Zheng, Q.; Yang, J. Influence of Pressure and Deflection Loads on the Critical Behavior of Flexible Joint. Compos. Struct. 2017, 180, 772–781. [Google Scholar] [CrossRef]
- Hu, J.; Ren, J.; Zhang, Z.; Xue, M.; Tong, Y.; Zou, J.; Zheng, Q.; Tang, H. A Pressure, Amplitude and Frequency Dependent Hybrid Damping Mechanical Model of Flexible Joint. J. Sound Vib. 2020, 471, 115173. [Google Scholar] [CrossRef]
- Wan, N.; Shi, H.; Qu, Z.; Liu, K. Analysis of Fatigue Properties and Vectoring Life of the Flexible Joint. J. Propuls. Technol. 2022, 43, 200932. (In Chinese) [Google Scholar] [CrossRef]
- Liu, W.; Yang, X.; Wang, Z.; Jia, M.; Zhao, Y. Analysis of Load-Bearing Safety and Reliability of Solid Rocket Motor Flexible Joint Structure. Eng. Fail. Anal. 2022, 144, 106952. [Google Scholar] [CrossRef]
- Griffith, A.A. The Phenomena of Rupture and Flow in Solids. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 1920, 221, 163–198. [Google Scholar] [CrossRef]
- Paris, P.C.; Gomez, M.P.; Anderson, W.P. A Rational Analytic Theory of Fatigue. Trends Eng. 1961, 13, 9–14. [Google Scholar]
- Paris, P.C.; Erdogan, F. A Critical Analysis of Crack Propagation Laws. J. Basic Eng. 1963, 85, 528–533. [Google Scholar] [CrossRef]
- Mirzaei, M.; Provan, J.W. A New Method for the Analysis and Assessment of Fatigue Crack Closure. I: Modeling. Theor. Appl. Fract. Mec. 1992, 18, 47–58. [Google Scholar] [CrossRef]
- Shariati, M.; Mirzaei, M.; Nejad, R.M. An Applied Method for Fatigue Life Assessment of Engineering Components Using Rigid-Insert Crack Closure Model. Eng. Fract. Mech. 2018, 204, 421–433. [Google Scholar] [CrossRef]
- Nejad, R.M.; Liu, Z. Analysis of Fatigue Crack Growth under Mixed Mode Loading Conditions for a Pearlitic Grade 900A Steel Used in Railway Applications. Eng. Fract. Mech. 2021, 247, 107672. [Google Scholar] [CrossRef]
- Nejad, R.M.; Berto, F. Fatigue Fracture and Fatigue Life Assessment of Railway Wheel Using Nonlinear Model for Fatigue Crack Growth. Int. J. Fatigue 2021, 153, 106516. [Google Scholar] [CrossRef]
- Ma, T.; Zhou, C.; Chang, L.; He, X. Multiaxial Low Cycle Fatigue Behavior and Life Prediction of CP-Ti under Non-Proportional Stress-Controlled Mode. Fatigue Fract. Eng. Mater. Struct. 2022, 46, 341–356. [Google Scholar] [CrossRef]
- Nejad, R.M.; Aliakbari, K.; Abbasnia, S.K.; Langari, J. Failure Analysis of Overdrive Gear of Passenger Car Gearbox Fabricated from Powder Metallurgy. Eng. Fail. Anal. 2022, 141, 106683. [Google Scholar] [CrossRef]
- Rivlin, R.S.; Thomas, A.G. Rupture of Rubber. Ⅰ. Characteristic Energy for Tearing. J. Polym. Sci. 1953, 10, 291–318. [Google Scholar] [CrossRef]
- Thomas, A.G. Rupture of Rubber. V. Cut Growth in Natural Rubber Vulcanizates. Rubber Chem. Technol. 1959, 32, 477–489. [Google Scholar] [CrossRef]
- Saintier, N.; Cailletaud, G.; Piques, R. Crack Initiation and Propagation under Multiaxial Fatigue in a Natural Rubber. Int. J. Fatigue 2006, 28, 61–72. [Google Scholar] [CrossRef]
- Mars, W.V.; Fatemi, A. Multiaxial Fatigue of Rubber: Part I: Equivalence Criteria and Theoretical Aspects. Fatigue Fract. Eng. Mater. Struct. 2005, 28, 515–522. [Google Scholar] [CrossRef]
- Mars, W.V.; Fatemi, A. Multiaxial Fatigue of Rubber: Part II: Experimental Observations and Life Predictions. Fatigue Fract. Eng. Mater. Struct. 2005, 28, 523–538. [Google Scholar] [CrossRef]
- Boulenouar, A.; Benseddiq, N.; Merzoug, M.; Benamara, N.; Mazari, M. A Strain Energy Density Theory for Mixed Mode Crack Propagation in Rubber-like Materials. J. Theor. App. Mech. 2016, 54, 1417–1431. [Google Scholar] [CrossRef]
- El Yaagoubi, M.; Meier, J. Crack Growth Angle Prediction of an Internal Crack under Mixed Mode Load for Unfilled Elastomer Using the Strain Energy Density Factor. Polym. Eng. Sci. 2021, 61, 2183–2192. [Google Scholar] [CrossRef]
- Belkhiria, S.; Hamdi, A.; Fathallah, R. Cracking Energy Density for Rubber Materials: Computation and Implementation in Multiaxial Fatigue Design. Polym. Eng. Sci. 2020, 60, 2190–2203. [Google Scholar] [CrossRef]
- Mars, W.V. Cracking Energy Density as a Predictor of Fatigue Life under Multiaxial Conditions. Rubber Chem. Technol. 2002, 75, 1–17. [Google Scholar] [CrossRef]
- Tee, Y.L.; Loo, M.S.; Andri, A. Recent Advances on Fatigue of Rubber after the Literature Survey by Mars and Fatemi in 2002 and 2004. Int. J. Fatigue 2018, 110, 115–129. [Google Scholar] [CrossRef]
- Pan, Z.; Lai, Y.; Wang, Y.; Duan, W.; Qiao, Y.; Liu, Y.; Liu, Y.; Song, C. Fatigue Life Prediction and Effects of Cerium Oxide-Filled Vulcanized Natural Rubber on Fatigue Life under Multiaxial Loading. Fatigue Fract. Eng. Mater. Struct. 2021, 44, 3349–3362. [Google Scholar] [CrossRef]
- Wang, X.; Shangguan, W.; Li, M.; Duan, X.; Yan, J. A Method for Calculating Cracking Energy Density of Rubber Components under Different Hyperelastic Models and Multiaxial Stress States. Eng. Mech. 2015, 32, 197–205. (In Chinese) [Google Scholar] [CrossRef]
- Wang, X.; Shangguan, W.; Zeng, X.; Duan, X.; Yan, J. A Method to Predict Fatigue Performances of Rubber Isolator Based on the Cracking Energy Density and the Fatigue Crack Growth Characteristic of Rubber Material. J. Vib. Shock 2016, 35, 70–74. (In Chinese) [Google Scholar] [CrossRef]
- Peng, Y.; Liu, G.; Quan, Y.; Zeng, Q. Cracking Energy Density Calculation of Hyperelastic Constitutive Model and Its Application in Rubber Fatigue Life Estimations. J. Appl. Polym. Sci. 2016, 133, 44195. [Google Scholar] [CrossRef]
- Luo, R.; Wu, W. Fatigue Failure Analysis of Anti-Vibration Rubber Spring. Eng. Fail. Anal. 2006, 13, 110–116. [Google Scholar] [CrossRef]
- Duan, X.; Shangguan, W.; Li, M.; Rakheja, S. Measurement and Modelling of the Fatigue Life of Rubber Mounts for an Automotive Powertrain at High Temperatures. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2016, 230, 942–954. [Google Scholar] [CrossRef]
- Peng, X.; Li, L. State of the Art of Constitutive Relations of Hyperelastic Materials. Chin. J. Theor. Appl. Mech. 2020, 52, 1221–1232. (In Chinese) [Google Scholar] [CrossRef]
- Mars, W.V. Multiaxial Fatigue of Rubber. Ph.D. Thesis, The University of Toledo, Toledo, OH, USA, 2001. [Google Scholar]
- Zine, A.; Benseddiq, N.; Abdelaziz, M.N. Rubber Fatigue Life under Multiaxial Loading: Numerical and Experimental Investigations. Int. J. Fatigue 2011, 33, 1360–1368. [Google Scholar] [CrossRef]
- Hao, M.; Huang, Y.; Su, Z.; Wang, J. Dynamic Mechanical Properties of Phenyl Silicone Rubber. J. Mater. Eng. 2012, 10, 35–38. (In Chinese) [Google Scholar] [CrossRef]
- Puglisi, G.; Saccomandi, G. Multi-Scale Modelling of Rubber-Like Materials and Soft Tissues: An Appraisal. Proc. R. Soc. A Math. Phys. Eng. Sci. 2016, 472, 20160060. [Google Scholar] [CrossRef]
- Aït-Bachir, M.; Mars, W.V.; Verron, E. Energy Release Rate of Small Cracks in Hyperelastic Materials. Int. J. Non Linear Mech. 2012, 47, 22–29. [Google Scholar] [CrossRef]
- Marco, Y.; Huneau, B.; Masquelier, I.; Le Saux, V.; Charrier, P. Prediction of Fatigue Properties of Natural Rubber Based on the Descriptions of the Cracks Population and of the Dissipated Energy. Polym. Test. 2017, 59, 67–74. [Google Scholar] [CrossRef]
- Lake, G.J.; Lindley, P.B. Cut Growth and Fatigue of Rubbers. II. Experiments on a Noncrystallizing Rubber. J. Appl. Polym. Sci. 1965, 38, 301–313. [Google Scholar] [CrossRef]
- Lindley, P.B. Non-Relaxing Crack Growth and Fatigue in a Non-Crystallizing Rubber. Rubber Chem. Technol. 1974, 47, 1253–1264. [Google Scholar] [CrossRef]
- Mars, W.V.; Fatemi, A. Fatigue Crack Nucleation and Growth in Filled Natural Rubber. Fatigue Fract. Eng. Mater. Struct. 2003, 26, 779–789. [Google Scholar] [CrossRef]
- Tao, G.; Xia, Z. A Non-Contact Real-Time Strain Measurement and Control System for Multiaxial Cyclic/Fatigue Tests of Polymer Materials by Digital Image Correlation Method. Polym. Test. 2005, 24, 844–855. [Google Scholar] [CrossRef]





| Hyperelastic Parameters | μ1 | α1 | μ2 | α2 |
|---|---|---|---|---|
| Fitting results | 1.83663 × 10−5 | 10.303 | 21.539 | 0.02659 |
| Characteristic Parameters | rc (m/one cycle) | Tc (kJ/m2) | F0 | c0 (m) |
|---|---|---|---|---|
| Test results | 1.8068 × 10−6 | 3.1136 | 1.24116 | 5.2334 × 10−5 |
| Dangerous Element | Wc,max/MPa | θ/rad | Nf,pre/cycles | Nf,exp/cycles | Nf,pre/Nf,exp |
|---|---|---|---|---|---|
| No.7505 | 5.585 | θ = 0, ±π | 107 | 120 | 1/1.12 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Ren, J.; Xue, M.; Tong, Y.; Zheng, Q.; Tang, H. Fatigue Analysis of Flexible Joint Elastomers Combining Ogden Second-Order Constitutive Model with Cracking Energy Density. Aerospace 2023, 10, 123. https://doi.org/10.3390/aerospace10020123
Zhang J, Ren J, Xue M, Tong Y, Zheng Q, Tang H. Fatigue Analysis of Flexible Joint Elastomers Combining Ogden Second-Order Constitutive Model with Cracking Energy Density. Aerospace. 2023; 10(2):123. https://doi.org/10.3390/aerospace10020123
Chicago/Turabian StyleZhang, Jinyao, Junxue Ren, Muyao Xue, Yue Tong, Qing Zheng, and Haibin Tang. 2023. "Fatigue Analysis of Flexible Joint Elastomers Combining Ogden Second-Order Constitutive Model with Cracking Energy Density" Aerospace 10, no. 2: 123. https://doi.org/10.3390/aerospace10020123
APA StyleZhang, J., Ren, J., Xue, M., Tong, Y., Zheng, Q., & Tang, H. (2023). Fatigue Analysis of Flexible Joint Elastomers Combining Ogden Second-Order Constitutive Model with Cracking Energy Density. Aerospace, 10(2), 123. https://doi.org/10.3390/aerospace10020123






