Numerical Analysis of Unsteady Characteristics of the Second Throat of a Transonic Wind Tunnel
Abstract
:1. Introduction
2. Numerical Methods
2.1. Pulsating Pressure and Shock Position
2.2. Governing Equation
2.3. Boundary Conditions
2.4. Algorithm Verification
3. Results and Discussion
3.1. The Pressure Disturbance Frequency Is 5 Hz
3.2. Pressure Disturbance Frequency Is 10 Hz~2500 Hz
4. Conclusions
- (1)
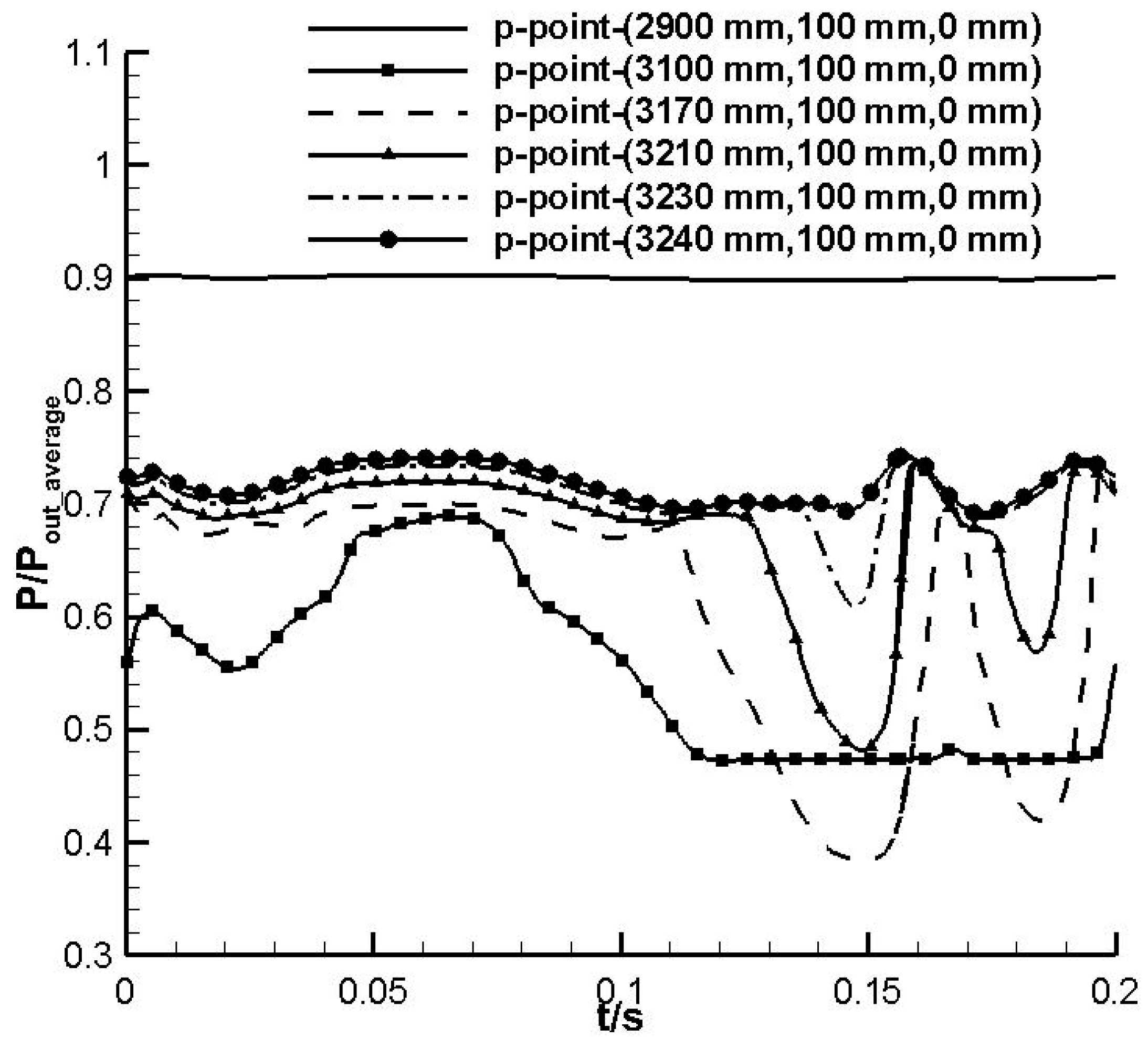
- Forced shock oscillations are caused by the propagation of a wavefront originating from downstream pressure perturbations that periodically alter the shock position and the flow field structure downstream of the shock, including boundary layer thickness, the size of the separation zone, and the size of the wake mixing zone. The shock boundary layer interference is very sensitive to the shock wave position. Due to the large shock wave displacement, a relatively long straight section needs to be provided in the second throat.
- (2)
- Compared with the outlet back pressure, the pressure change near the shock wave has a phase delay. The pressure disturbance shows a significant amplification effect; in particular, the closer the shock wave is, the more obvious it is. This leads to overshoot when the shock moves upstream or downstream. Under the same back pressure, the shock wave position in the unsteady state is different from the position in the steady state.
- (3)
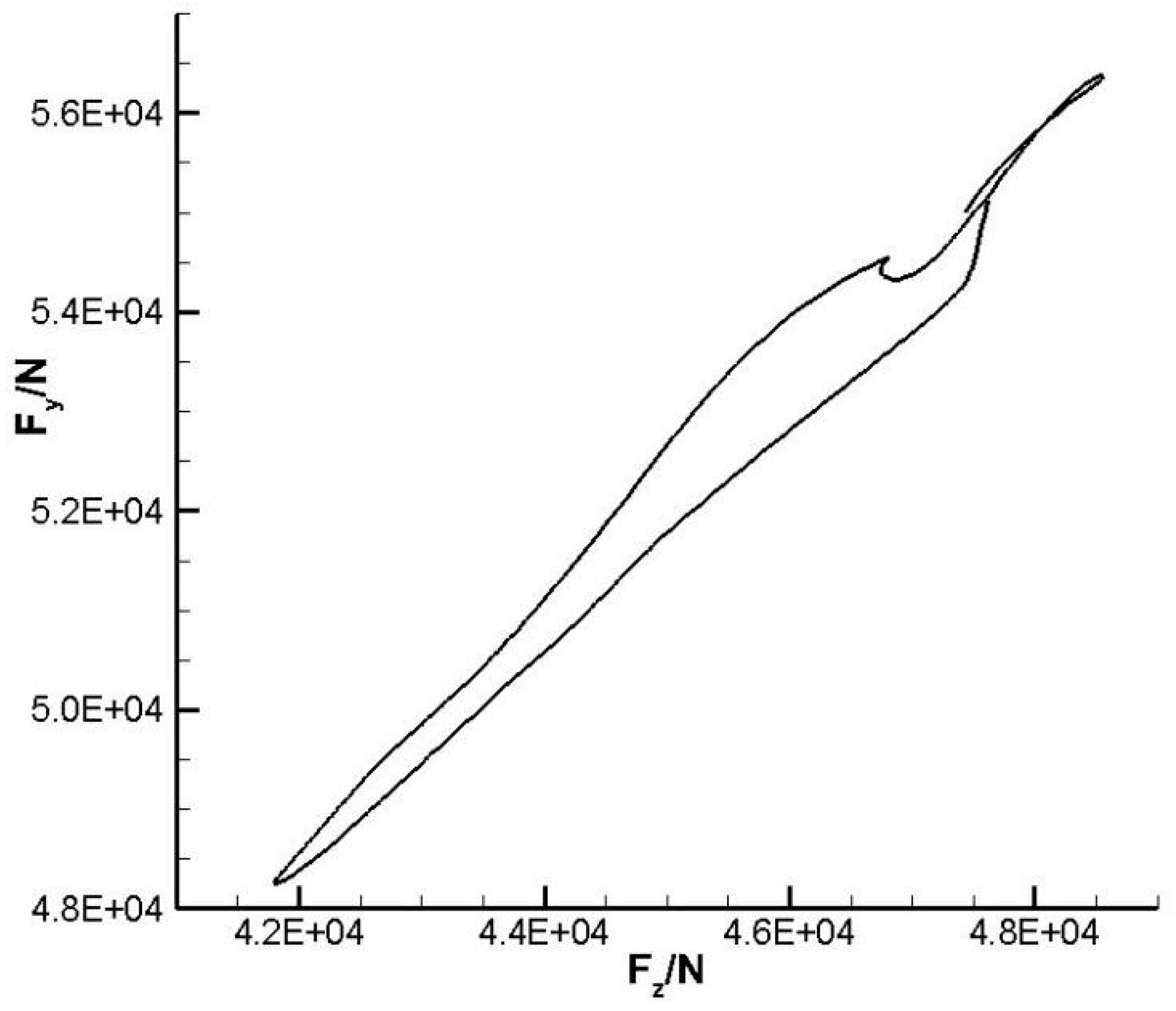
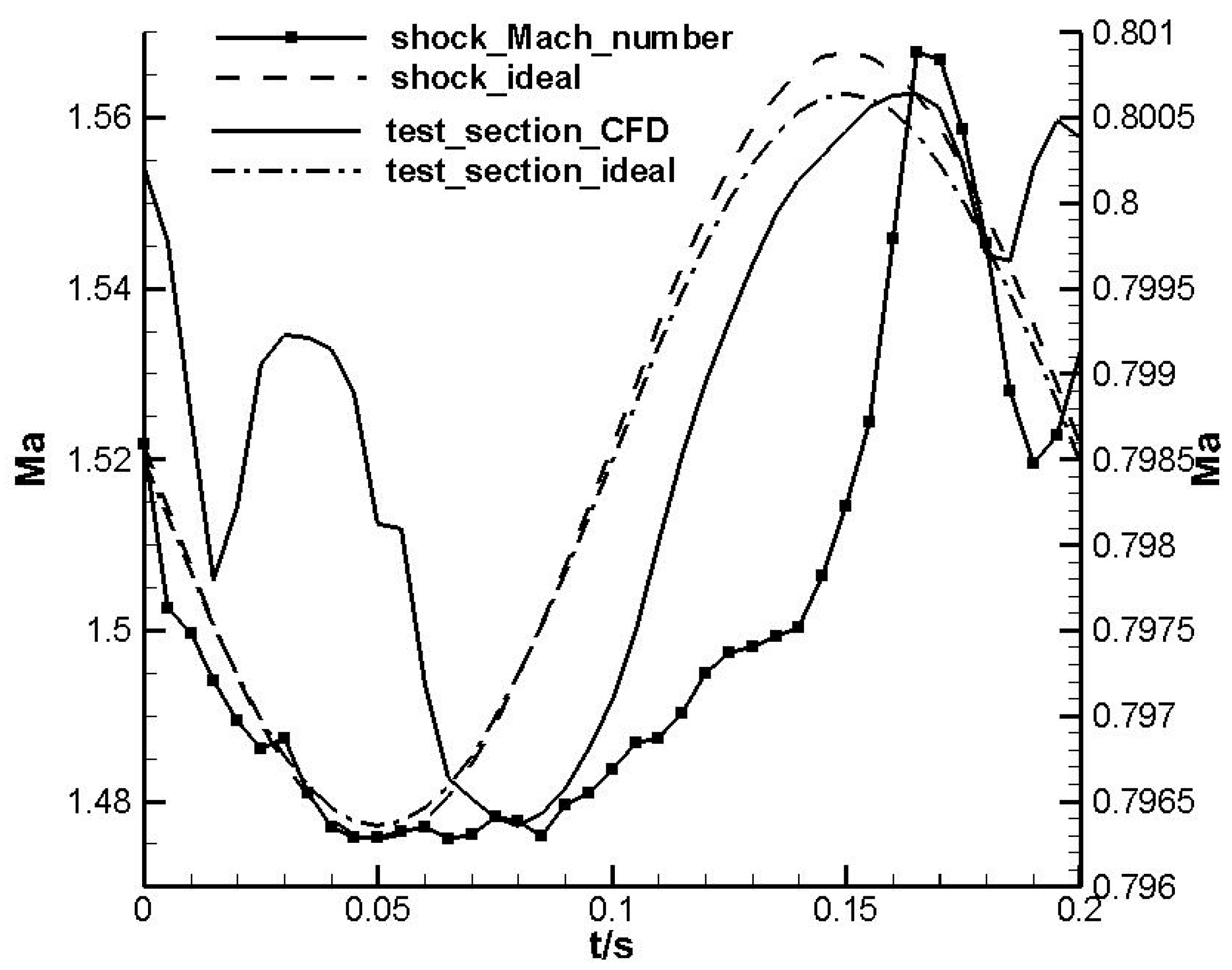
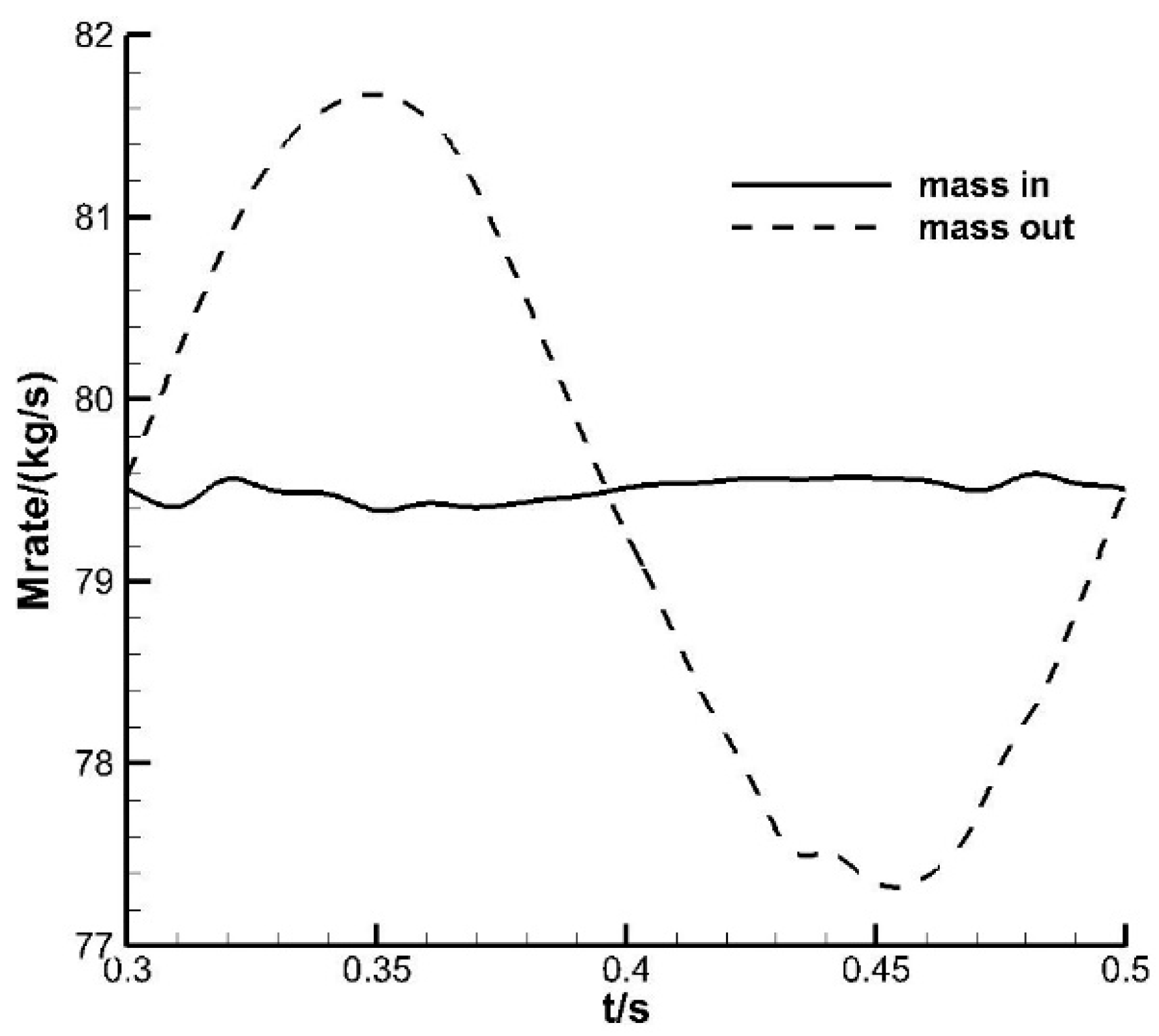
- The variation trend in the second throat wall force, wavefront Mach number, and Mach number in the test section with time is consistent with the downstream disturbance, but does not have a complete follow-up effect. It shows that the pressure disturbance will propagate into the test section through the boundary layer or shock gap, but it is still recommended to use the second throat choking to control the Mach number stability of the test section.
- (4)
- The dynamic characteristics of shock oscillation are related to the amplitude and frequency of the applied pressure disturbance. The shock displacement decreases with the increase in the excitation frequency. When the frequency is higher than 125 Hz, the flow field is no longer sensitive to the forced excitation.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Robert, L.C. The New French High Speed Wind Runnel. National Advisory Committee for Aeronautics, Washington, NACA TM-205. 1923. Available online: https://digital.library.unt.edu/ark:/67531/metadc277372/ (accessed on 10 August 2023).
- Knight, W. ltalian and French Experiments on Wind Tunnels. NACA TN-17; 1920. Available online: https://ntrs.nasa.gov/api/citations/19930080818/downloads/19930080818.pdf (accessed on 10 August 2023).
- Kilgore, R.A. Evolution and Development of Cryogenic Wind Tunnels. AIAA paper 2005-457. In Proceedings of the 43rd AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 10–13 January 2005. [Google Scholar]
- Jones, G.; Balakrishna, S.; DeMoss, J.; Goodliff, S.; Bailey, M. Influences of models on the unsteady pressure characteristics of the NASA national transonic facility. AIAA paper 2015-1557. In Proceedings of the 53rd AIAA Aerospace Sciences Meeting, Kissmmee, FL, USA, 5–9 January 2015. [Google Scholar]
- Mabey, D.G. Some Remarks on the Design of Transonic Tunnels with Low Levels of Flow Unsteadiness; NASA-CR-2722; Royal Aircraft Establishment: Bedford, UK, 1976. [Google Scholar]
- Long, D.F. Acoustic testing in transonic wind tunnels. AIAA paper 2006–0921. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 2006. [Google Scholar]
- Guo, D.M.; Luo, J.B.; Fang, D.N. Key scientific issues on the design and construction of large wind tunnels. Bull. Natl. Nat. Sci. Found. China 2017, 5, 420–427. (In Chinese) [Google Scholar]
- Chen, J.M.; Wu, S.H.; Chen, Z.H.; Lyu, J.L.; Pei, H.T. Experimental research on noise reduction for continuous transonic wind tunnel loop. J. Northwest. Polytech. Univ. 2020, 38, 855–861. (In Chinese) [Google Scholar] [CrossRef]
- Brooks, J.D.; Stainback, P.C.; Brooks, C.W., Jr. Additional flow quality measurements in the Langley Research Center 8-Foot Transonic Pressure Tunnel. AIAA paper 80-0434. In Proceedings of the 11th Aerodynamic Testing Conference, Colorado Springs, CO, USA, 18–20 March 1980. [Google Scholar]
- Barnwell, R.W.; Edwards, C.L.W.; Kilgore, R.A.; Dresstt, D.A. Optimum transonic wind tunnel. AIAA paper 1986-0755. In Proceedings of the 14th Aerodynamic Testing Conference, West Palm Beach, FL, USA, 5–7 March 1986. [Google Scholar]
- Mabey, D.G. Further Noise Measurements in a Slotted Cryogenic Wind Tunnel; RAE-TM-AERO-2201; Royal Aircraft Establishment: Bedford, UK, 1990. [Google Scholar]
- Sawyer, R.S.; Krynytzky, A.J. Overview of the NWTC studies and experiments. AIAA paper 97-0092. In Proceedings of the 35th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1997. [Google Scholar]
- Mckinney, M.O.; Scheiman, J. Evaluation of Turbulence Reduction Devices for the Langley 8-Foot Transonic Pressure Tunnel; NASA TM-81792; NASA Langley Research Center: Hampton, VA, USA, 1981.
- Stainback, P.C.; Harvey, W.D.; Owen, F.K. Evaluation of flow quality in two NASA transonic wind tunnels. NASA-TP-1737. In Proceedings of the AIAA 12th Fluid and Plasma Dynamics Conference, Williamsburg, VA, USA, 23–25 July 1981. [Google Scholar]
- Owen, F.K.; Stainback, P.C.; Harvey, W.D. Evaluation of flow quality in two NASA transonic wind tunnels. J. Aircr. 1981, 18, 451–457. [Google Scholar] [CrossRef]
- Bo, X.; Enmin, Z.; Song, C.; Wen, Z.; Kai, L.; Xinfu, L. Research on key technologies of debugging and operating in 0.6 m × 0.6 m continuous transonic wind tunnel. J. Exp. Fluid Mech. 2016, 30, 81–86. (In Chinese) [Google Scholar]
- Yoshinaga, T.; Fujii, K.; Nagai, S.; Yamaguchi, T. Suppression of infrasonic noise emanating from the NAL 1 m × 1 m supersonic wind tunnel. AIAA paper 97-0664. In Proceedings of the 35th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1997. [Google Scholar]
- Liao, D.X.; Chen, W.H.; Peng, L. Research on the intellectualization of large-scale wind tunnel equipment. Control. Inf. Technol. 2019, 1, 18–22. (In Chinese) [Google Scholar]
- Sajben, M.; Kroutil, J.C. Effects of initial boundary-layer thickness on transonic diffuser flows. AIAA J. 1981, 19, 1386–1393. [Google Scholar] [CrossRef]
- Grzona, A.; Olivier, H. Shock train generated turbulence inside a nozzle with a small opening angle. Exp. Fluids 2011, 51, 621–639. [Google Scholar] [CrossRef]
- Bogar, T.J. Structure of self-excited oscillations in transonic diffuser flows. AIAA J. 1986, 24, 54–61. [Google Scholar] [CrossRef]
- Bogar, T.J.; Sajben, M. Response of transonic diffuser flows to abrupt increases of back pressure wall pressure measurements. AIAA paper 87-1356. In Proceedings of the 19th AIAA, Fluid Dynamics, Plasma Dynamics, and Lasers Conference, Honolulu, HI, USA, 8–10 June 1987. [Google Scholar]
- Robinet, J.C.; Casalis, G. Shock oscillations in diffuser modeled by a selective noise amplification. AIAA J. 1999, 37, 453–459. [Google Scholar] [CrossRef]
- Matsuo, K.; Miyazato, Y.; Kim, H.D. Shock train and pseudo-shock phenomena in internal gas flows. Prog. Aerosp. Sci. 1999, 35, 33–100. [Google Scholar] [CrossRef]
- Bogar, T.J.; Sajben, M.; Kroutil, J.C. Characteristic frequencies of transonic diffuser flow oscillations. AIAA J. 1983, 21, 1232–1240. [Google Scholar] [CrossRef]
- Handa, T.; Masuda, M.; Matsuo, K. Mechanism of shock wave oscillation in transonic diffusers. AIAA J. 2003, 41, 64–70. [Google Scholar] [CrossRef]
- Bur, R.; Benay, R.; Galli, A.; Berthouze, P. Experimental and numerical study of forced shock-wave oscillations in a transonic channel. Aerosp. Sci. Technol. 2006, 10, 265–278. [Google Scholar] [CrossRef]
- Hsieh, T.; Wardlaw, A.B., Jr.; Collins, P. Numerical investigation of unsteady inlet flowfields. AIAA J. 1987, 25, 75–81. [Google Scholar] [CrossRef]
- Rodi, P.E.; Emami, S. Unsteady pressure behavior in a ramjet scramjet inlet. J. Propuls. Power 1996, 12, 486–493. [Google Scholar] [CrossRef]
- Olson, B.J.; Lele, S.K. Low-frequency unsteadiness in nozzle flow separation. AIAA paper 2012-2974. In Proceedings of the 42nd AIAA Fluid Dynamics Conference and Exhibit, New Orleans, LA, USA, 25–28 June 2012. [Google Scholar]
- Bruce, P.J.K.; Babinsky, H. An experimental study of transonic shock-boundary layer interactions subject to downstream pressure perturbations. Aerosp. Sci. Technol. 2010, 14, 134–142. [Google Scholar] [CrossRef]
- Biswas, D. Unsteady 3D Navier-Stokes simulations on characteristic frequency and length scales in transonic diffuser. AIAA paper 2004-2715. In Proceedings of the 34th AIAA Fluid Dynamics Conference and Exhibit, Portland, OR, USA, 28 June–1 July 2004. [Google Scholar]
- Bruce, P.J.K.; Babinsky, H.; Tartinville, B.; Hirsch, C. Experimental and numerical study of oscillating transonic shock waves in ducts. AIAA J. 2011, 49, 1710–1720. [Google Scholar] [CrossRef]
- Cong, C.H.; Liao, D.X.; Chen, J.M. Numerical investigation on performance of second throat in transonic wind tunnel. J. Aerosp. Power 2010, 25, 2050–2056. (In Chinese) [Google Scholar]
- Georgiadis, N.J.; Vyas, M.A.; Yoder, D.A. Wind-US Code Contributions to the First AIAA Shock Boundary Layer Interaction Prediction Workshop; NASA-TM-217837; NASA Glenn Research Center: Cleveland, OH, USA, 2013.
- Verma, S.B.; Manisankar, C. Origin of flow asymmetry in planar nozzles with separation. Shock Waves 2014, 24, 191–209. [Google Scholar] [CrossRef]
- Bourgoing, A.; Reijasse, P. Experimental analysis of unsteady separated flows in a supersonic planar nozzle. Shock Waves 2005, 14, 251–258. [Google Scholar] [CrossRef]
- Doerffer, P.; Szulc, O.; Magagnato, F. Shock wave-boundary layer interaction in forced shock oscillations. J. Therm. Sci. 2003, 12, 10–15. [Google Scholar] [CrossRef]
- Chen, W.H.; Wang, Y.X.; Wang, C.Q.; Wu, J.Q. Fault diagnosis of the wind tunnel structure based on finite element method. J. Exp. Fluid Mech. 2011, 25, 63–67. (In Chinese) [Google Scholar]
- Cong, C.H.; Qin, H.G.; Ren, Z.B.; Chen, J.M. Numerical simulation of flow field characterisctics of impacting T-junction duct in wind tunnel. J. Aerosp. Power 2020, 35, 235–243. (In Chinese) [Google Scholar]
- Rose, J.B.R.; Jinu, G.R.; Brindha, C.J. A numerical optimization of high altitude testing facility for wind tunnel experiments. Chin. J. Aeronaut. 2015, 28, 636–648. [Google Scholar] [CrossRef]
- Long, D.F. An experimental evaluation of test section noise in transonic wind tunnels. AIAA paper 90-1419. In Proceedings of the 16th Aerodynamic Ground Testing Conference, Seattle, WA, USA, 18–20 June 1990. [Google Scholar]
- Hashimoto, A.; Kohzai, M.; Aoyama, T.; Murayama, M. Wall interference analysis of transonic wind tunnel with porous wall model. AIAA paper 2011-3017. In Proceedings of the 29th AIAA Applied Aerodynamics Conference, Honolulu, HI, USA, 27–30 June 2011. [Google Scholar]


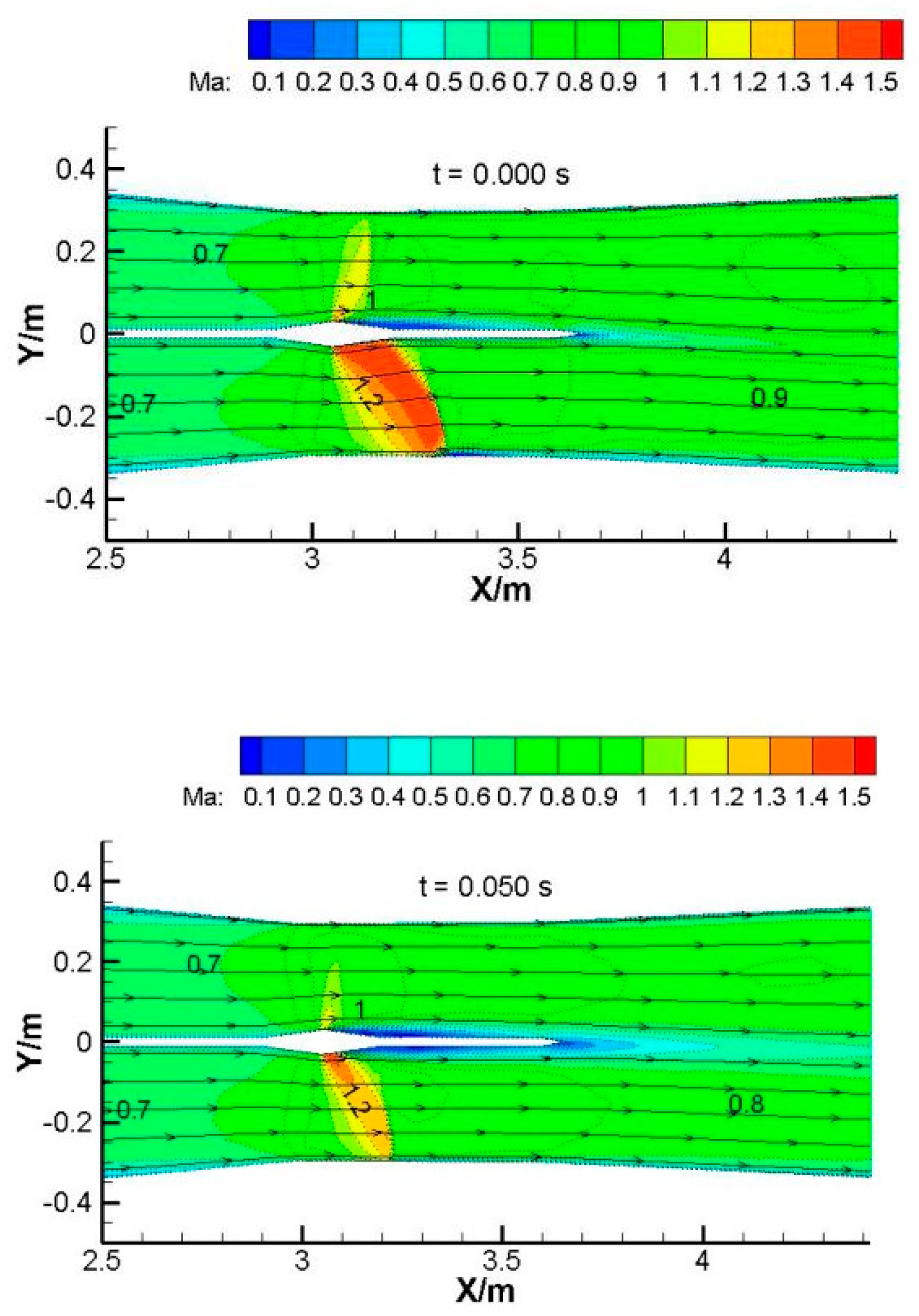
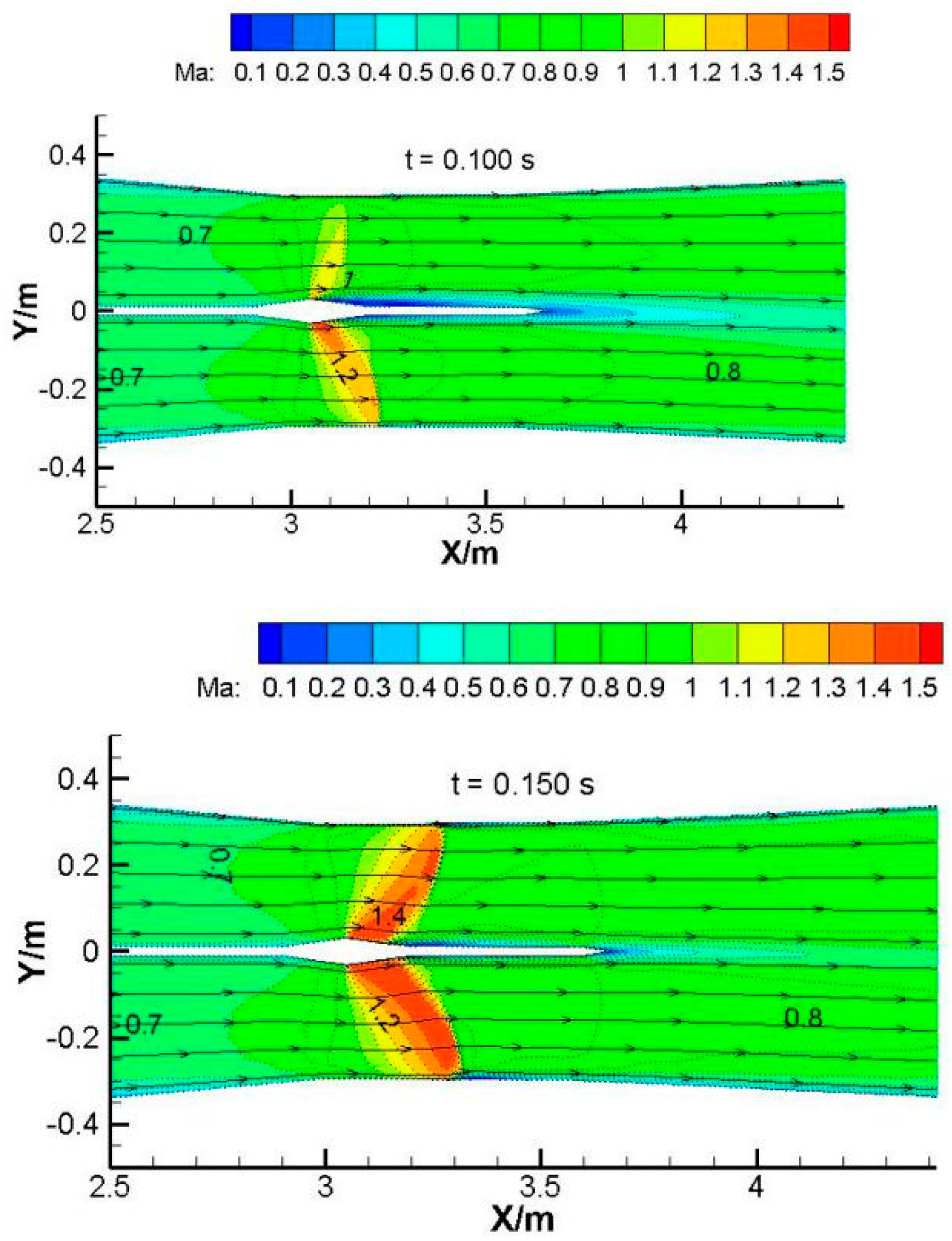
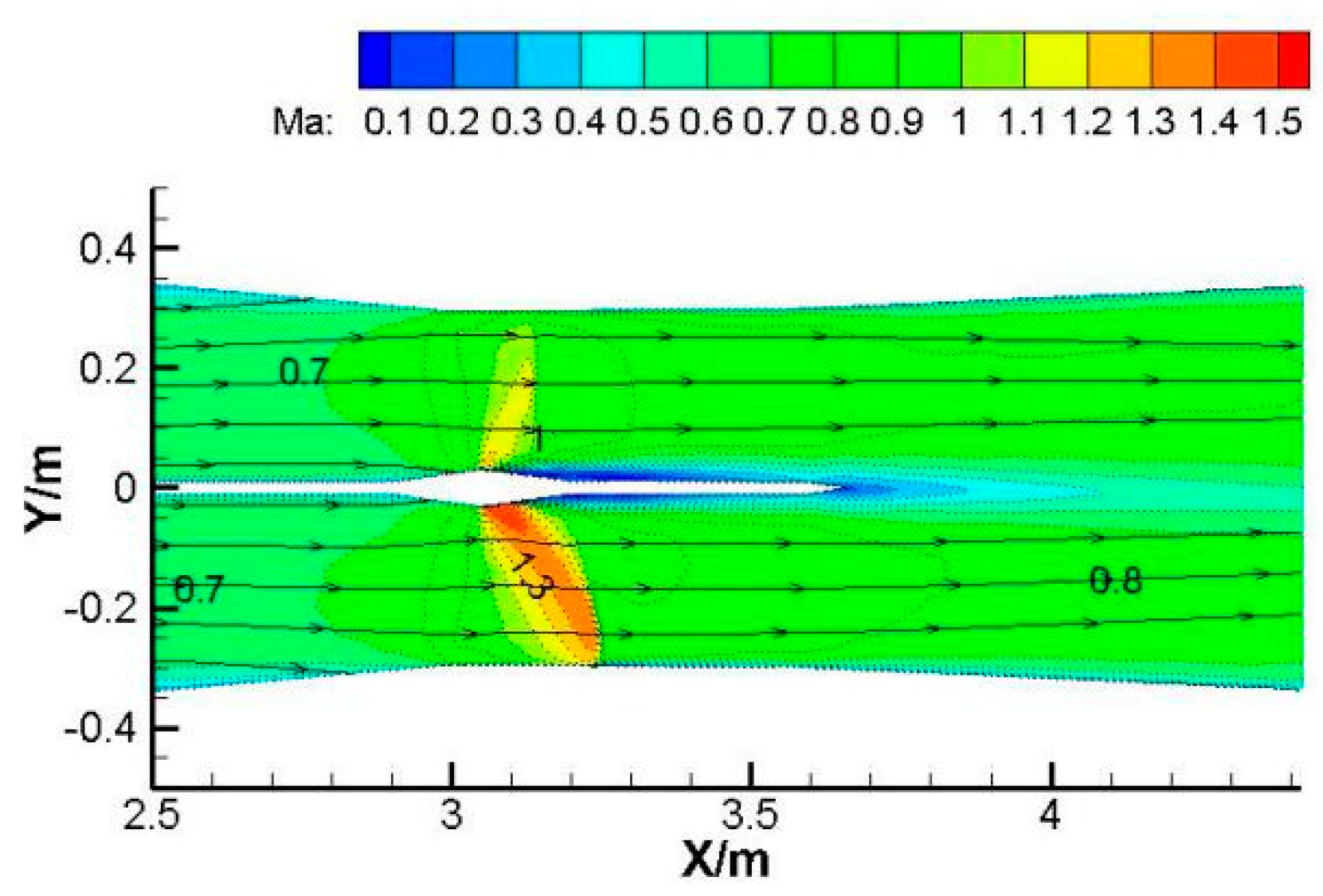
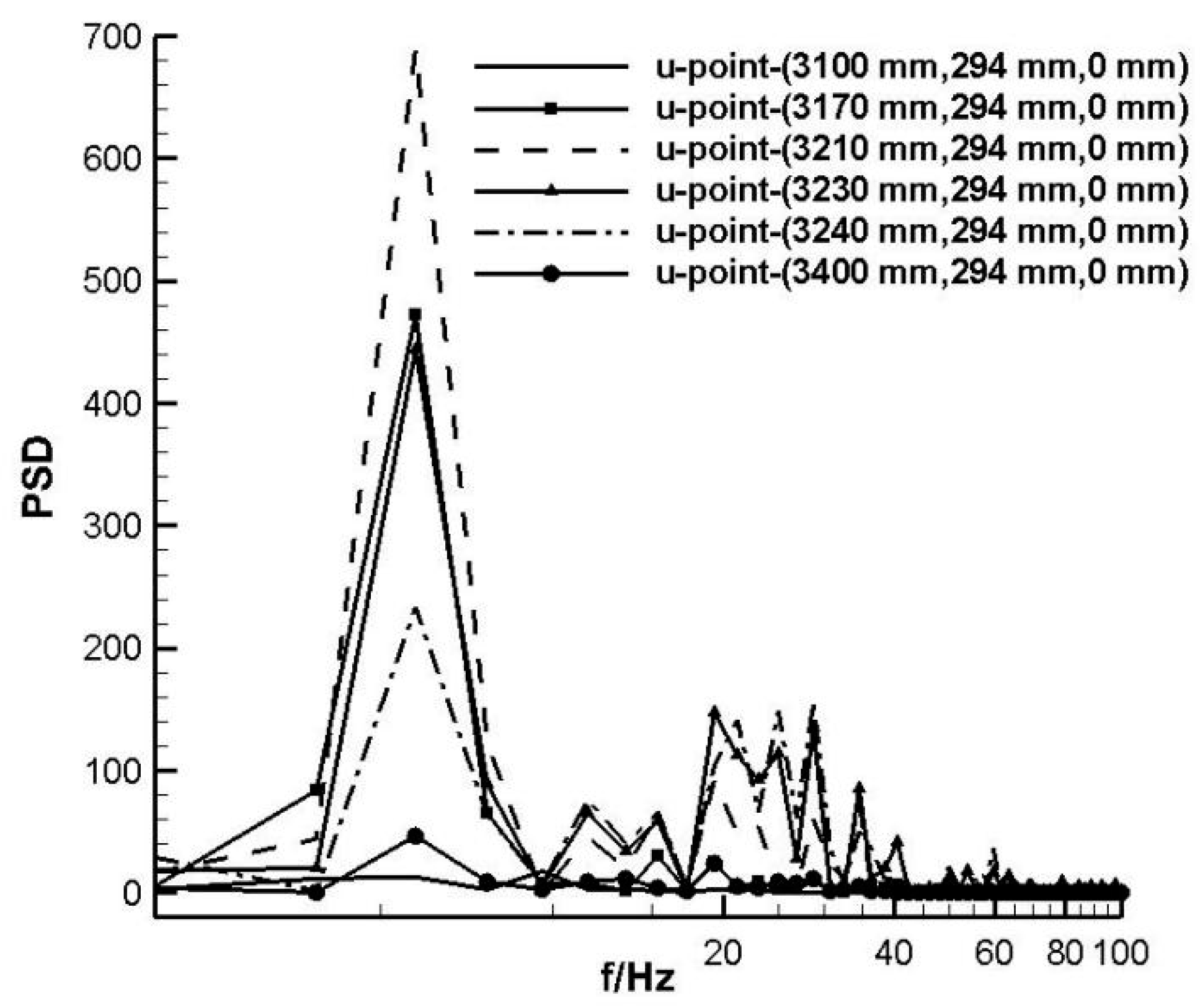


| Locations | y × z Cross-Sectional Size/mm | x-Coordinates/mm |
|---|---|---|
| test section entrance | 600 × 600 | (0, 0, 0) |
| model area center of the test section | 600 × 600 | (1300, 0, 0) |
| test section exit | 600 × 600 | (2350, 0, 0) |
| support section exit | 680 × 680 | (2500, 0, 0) |
| contraction section exit | Variable size × 680 | (3000, 0, 0) |
| straight section exit | Variable size × 680 | (3600, 0, 0) |
| diffuser section exit | 680 × 680 | (4500, 0, 0) |
| computational domain exit | 860 × 860 | (18,000, 0, 0) |
| Pressure Perturbation Amplitude | Frequency/Hz | Physical Time Step/s |
|---|---|---|
| 1% | 5 | 5 × 10−4 |
| 1% | 10 | 2.5 × 10−4 |
| 1% | 25 | 1 × 10−4 |
| 1% | 50 | 5 × 10−5 |
| 1% | 125 | 2 × 10−4 |
| 1%, 2%, 5%, 10% | 500 | 5 × 10−6 |
| 1% | 2500 | 1 × 10−6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cong, C.; Qin, H.; Yi, X. Numerical Analysis of Unsteady Characteristics of the Second Throat of a Transonic Wind Tunnel. Aerospace 2023, 10, 956. https://doi.org/10.3390/aerospace10110956
Cong C, Qin H, Yi X. Numerical Analysis of Unsteady Characteristics of the Second Throat of a Transonic Wind Tunnel. Aerospace. 2023; 10(11):956. https://doi.org/10.3390/aerospace10110956
Chicago/Turabian StyleCong, Chenghua, Honggang Qin, and Xingyou Yi. 2023. "Numerical Analysis of Unsteady Characteristics of the Second Throat of a Transonic Wind Tunnel" Aerospace 10, no. 11: 956. https://doi.org/10.3390/aerospace10110956
APA StyleCong, C., Qin, H., & Yi, X. (2023). Numerical Analysis of Unsteady Characteristics of the Second Throat of a Transonic Wind Tunnel. Aerospace, 10(11), 956. https://doi.org/10.3390/aerospace10110956






