A New Range Equation for Hybrid Aircraft Design
Abstract
:1. Introduction
- The power split is a third variable unlaced from the fuel and the battery mass, so that it is possible to choose a power management strategy and have a multiple-segments cruise, each with its power split;
- The equation can use weight fractions, which do not depend on the power split. Thus, the fuel and battery mass are defined a priori and do not depend on the power split, so even the aircraft’s total mass is constant;
- The equation uses the state of charge and the fuel mass instead of the energy level;
- The power split must be defined at the mechanical power level rather than at the energy source level. The reason is that the first value can be easily measured and used in the control system logic.
2. Analytical Model
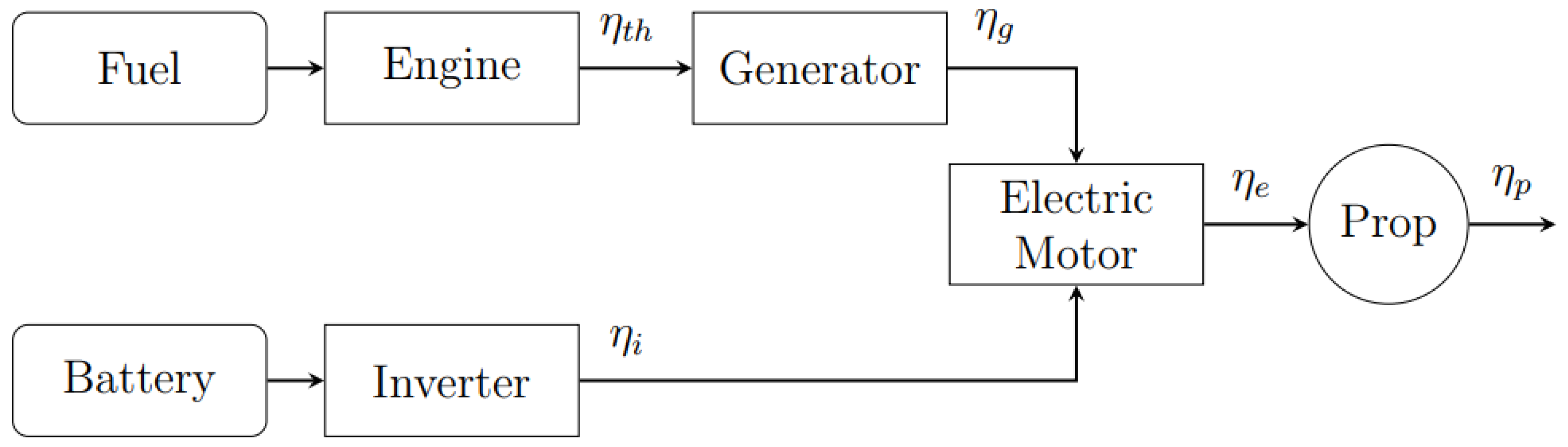
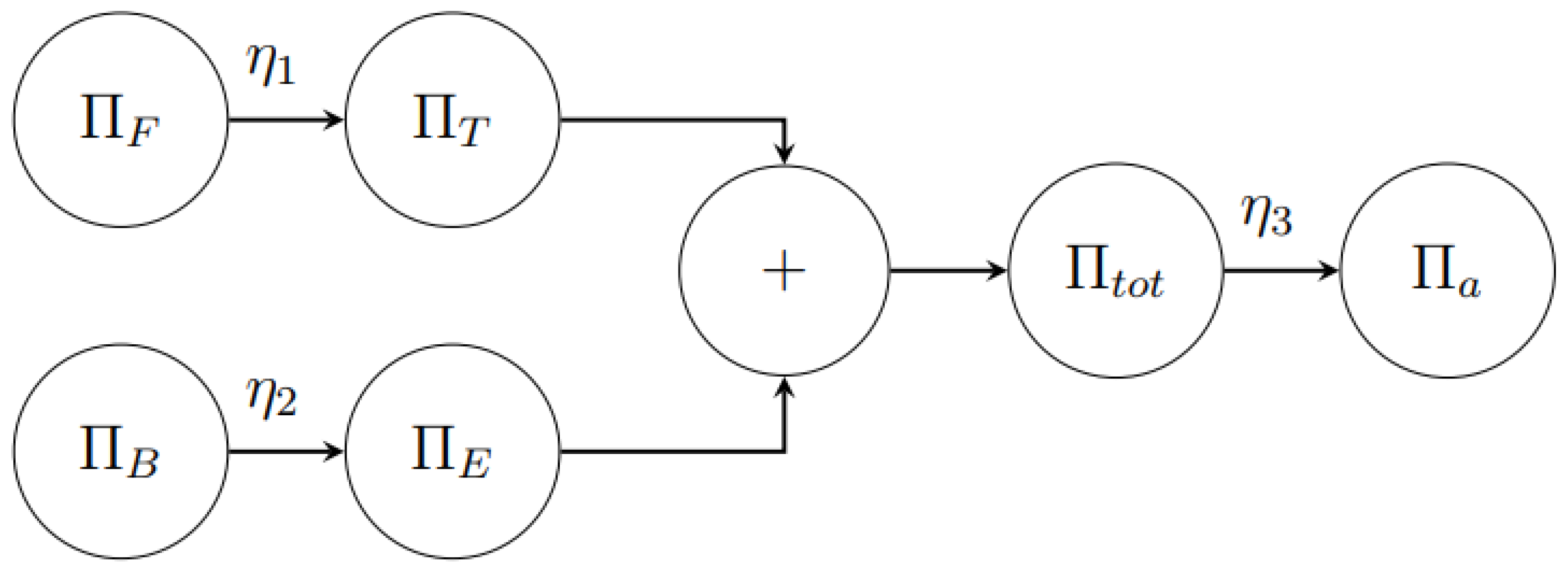
2.1. The “Virtual Aircraft” Method
2.1.1. Virtual Thermal Aircraft (VTA)
2.1.2. Virtual Electrical Aircraft (VEA)
2.1.3. Overall Range
3. Reference Aircraft
4. Results
4.1. Range Equation from the Literature [15,17]
- The power split is constant, and the cruise ends when one of the energy sources does;
- The aircraft consumes all the energy sources, and, if one runs out before the other, the power split cannot be constant for all the cruise. (i.e., if the batteries are below then the must go to 0);
- The energy sources run out exactly at the same time.
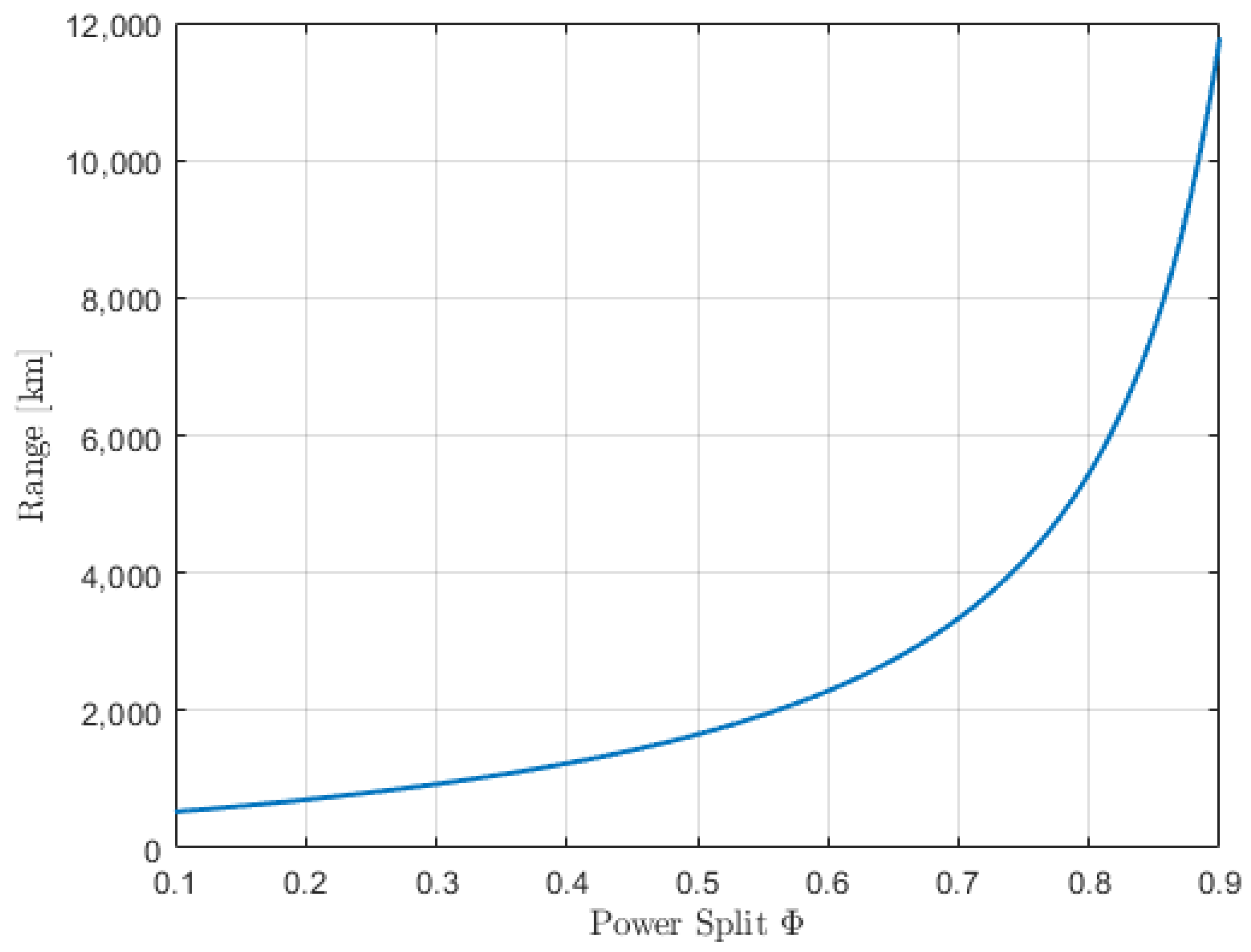
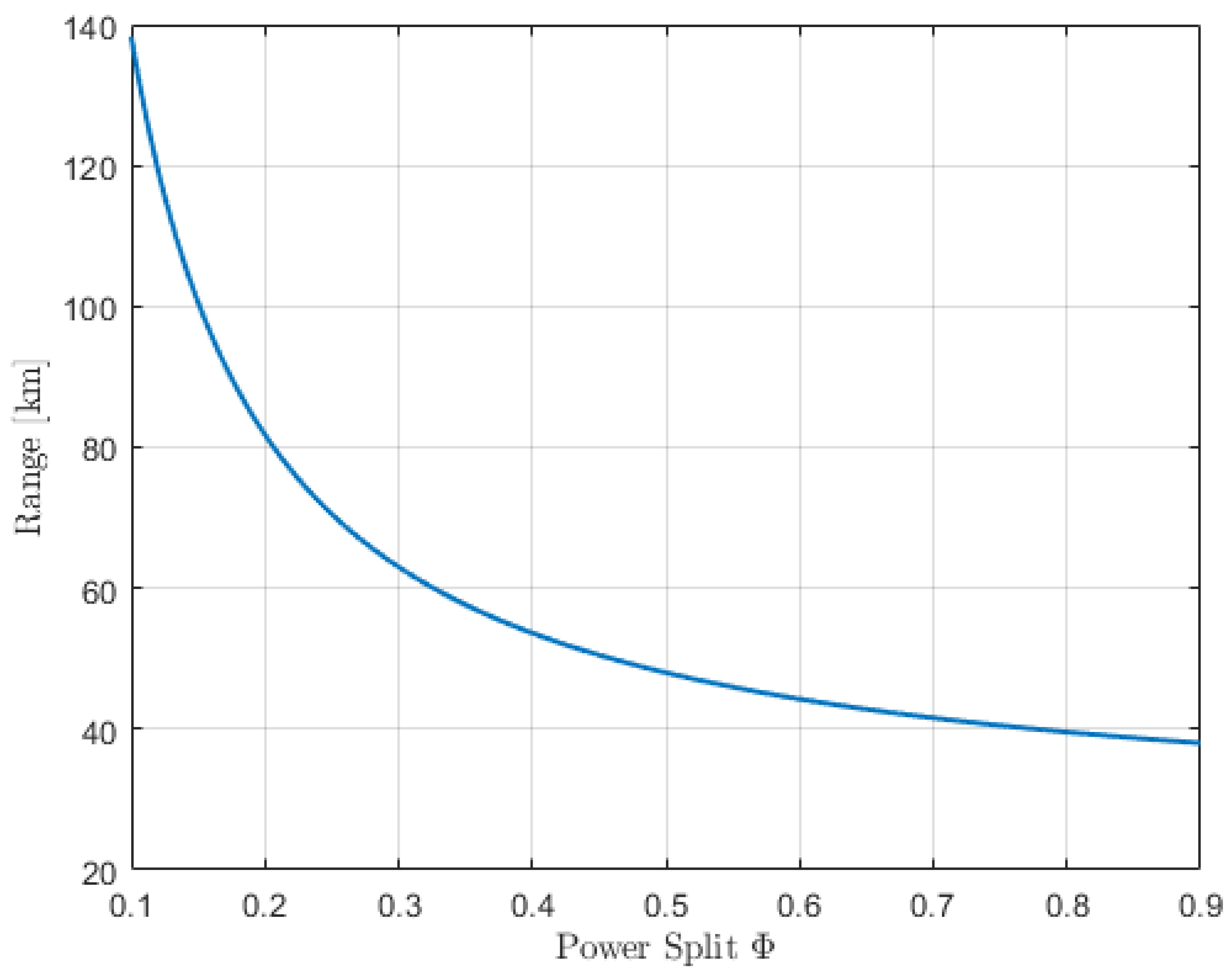
4.2. Present New Range Equation
5. Validation with a Numerical Approach
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Proton Exchange Membrane Fuel Cell | |
| maximum take off mass | |
| empty operative mass | |
| battery pack mass | |
| payload mass | |
| propeller efficiency | |
| electric motor efficiency | |
| inverter efficiency | |
| thermal motor efficiency | |
| generator efficiency | |
| total power fraction | |
| power split | |
| E | aerodynamic efficiency |
| Virtual Electric Aircraft Range | |
| Virtual Thermal Aircraft Range | |
| Hybrid Aircraft Range | |
| Fuel energy density | |
| Battery energy density | |
| Battery initial cruise state of charge | |
| Battery end of cruise state of charge | |
| mass fractions |
References
- Bacchini, A.; Cestino, E. Key aspects of electric vertical take-off and landing conceptual design. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2019, 234, 774–787. [Google Scholar] [CrossRef]
- Brelje, B.J.; Martins, J.R. Electric, hybrid, and turboelectric fixed-wing aircraft: A review of concepts, models, and design approaches. Prog. Aerosp. Sci. 2019, 104, 1–19. [Google Scholar] [CrossRef]
- Hepperle, M. Electric Flight—Potential and Limitations. In Proceedings of the NATO MPAVT-209-09, Energy Efficient Technologies and Concepts of Operation, Lisbon, Portugal, 22–24 October 2012. [Google Scholar]
- Romeo, G.; Cestino, E.; Correa, G.; Borello, F. A Fuel Cell Based Propulsion System for General Aviation Aircraft: The ENFICA-FC Experience. SAE Int. J. Aerosp. 2011, 4, 724–737. [Google Scholar] [CrossRef]
- Correa, G.; Santarelli, M.; Borello, F.; Cestino, E.; Romeo, G. Flight test validation of the dynamic model of a fuel cell system for ultra-light aircraft. Proc. Inst. of Mech. Eng. Part G J. Aerosp. Eng. 2015, 229, 917–932. [Google Scholar] [CrossRef]
- Romeo, G.; Cestino, E.; Pacino, M.; Borello, F.; Correa, G. Design and testing of a propeller for a two-seater aircraft powered by fuel cells. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2012, 226, 804–816. [Google Scholar] [CrossRef]
- Schmollgruber, P.; Döll, C.; Hermetz, J.; Liaboeuf, R.; Ridel, M.; Cafarelli, I.; Atinault, O.; François, C.; Paluch, B. Multidisciplinary Exploration of DRAGON: An ONERA Hybrid Electric Distributed Propulsion Concept. In Proceedings of the 2019 AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 7–11 January 2019. [Google Scholar] [CrossRef]
- C.F.M.Air. Blu Spark Hybrid Technology. Available online: https://cfm-air.net/projects/blu-spark-hybrid-technology/ (accessed on 8 October 2023).
- Diamond Aircraft. Diamond Aircraft Presents the World’s First Serial Hybrid Electric Aircraft “DA36 E-Star”. Available online: https://www.diamondaircraft.com/ (accessed on 8 October 2023).
- Raymer, D.P. Aircraft Design: A Conceptual Approach, 6th ed.; AIAA: Reston, VA, USA, 2018. [Google Scholar]
- Adu-Gyamfi, B.A.; Good, C. Pre-design strategies and sizing techniques for dual-energy aircraft. Aircr. Eng. Aerosp. Technol. 2014, 86, 525–542. [Google Scholar] [CrossRef]
- Finger, F.; Braun, C.; Bil, C. Initial Sizing Methodology for Hybrid-Electric General Aviation Aircraft. J. Aircr. 2019, 57, 245–255. [Google Scholar] [CrossRef]
- Wroblewski, G.E.; Ansell, P.J. A Bréguet Range Equation for Hybrid-Electric Jet Aircraft Sizing and Analysis. In Proceedings of the 2020 AIAA/IEEE Electric Aircraft Technologies Symposium (EATS), New Orleans, LA, USA, 26–28 August 2020; pp. 1–24. [Google Scholar]
- Kiesewetter, L.; Shakib, K.; Singh, P.; Rahman, M.; Khandelwal, B.; Kumar, S.; Shah, K. A holistic review of the current state of research on aircraft design concepts and consideration for advanced air mobility applications. Prog. Aerosp. Sci. 2023, 142, 100949. [Google Scholar] [CrossRef]
- de Vries, R.; Hoogreef, M.F.M.; Vos, R. Range Equation for Hybrid-Electric Aircraft with Constant Power Split. J. Aircr. 2020, 57, 552–557. [Google Scholar] [CrossRef]
- Rohacs, J.; Rohacs, D. Energy coefficients for comparison of aircraft supported by different propulsion systems. Energy 2020, 191, 116391. [Google Scholar] [CrossRef]
- Batra, A.; Raute, R.; Camilleri, R. On the Range Equation for Hybrid-Electric Aircraft. Aerospace 2023, 10, 687. [Google Scholar] [CrossRef]
- Finger, F.; de Vries, R.; Vos, R.; Braun, C.; Bil, C. A Comparison of Hybrid-Electric Aircraft Sizing Methods. In Proceedings of the AIAA SciTech 2020 Forum, Orlando, FL, USA, 6–10 January 2020; pp. 1–31. [Google Scholar]
- Adu-Gyamfi, B.A.; Good, C. Electric aviation: A review of concepts and enabling technologies. Transp. Eng. 2022, 9, 100134. [Google Scholar] [CrossRef]

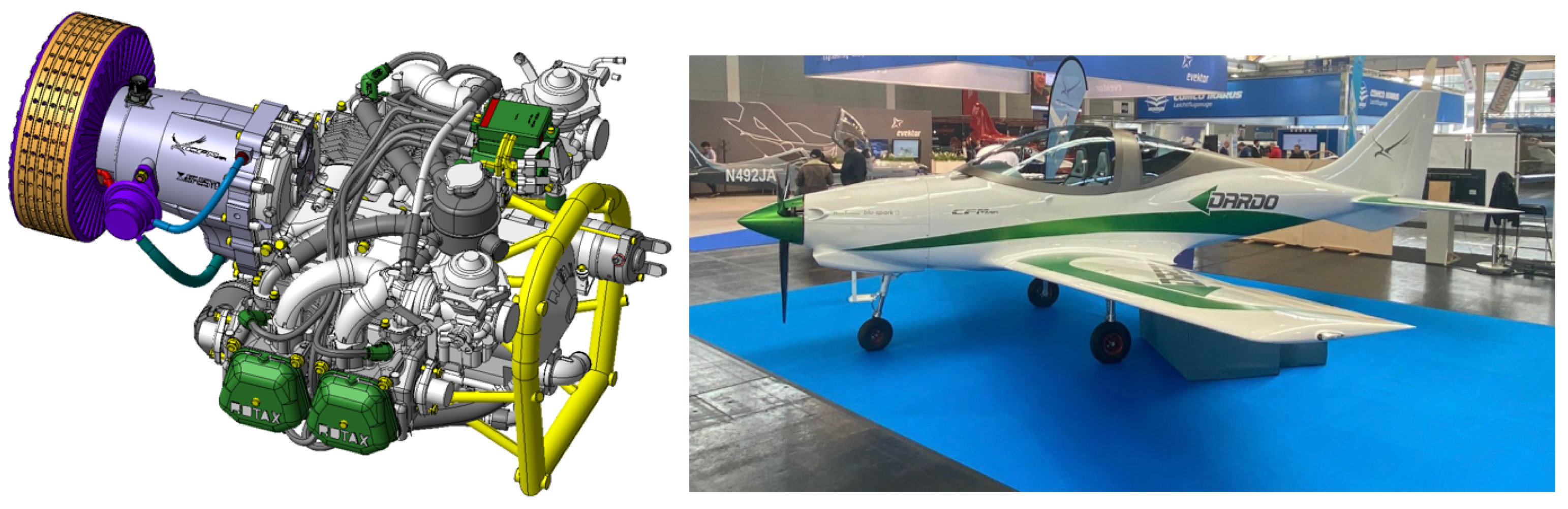

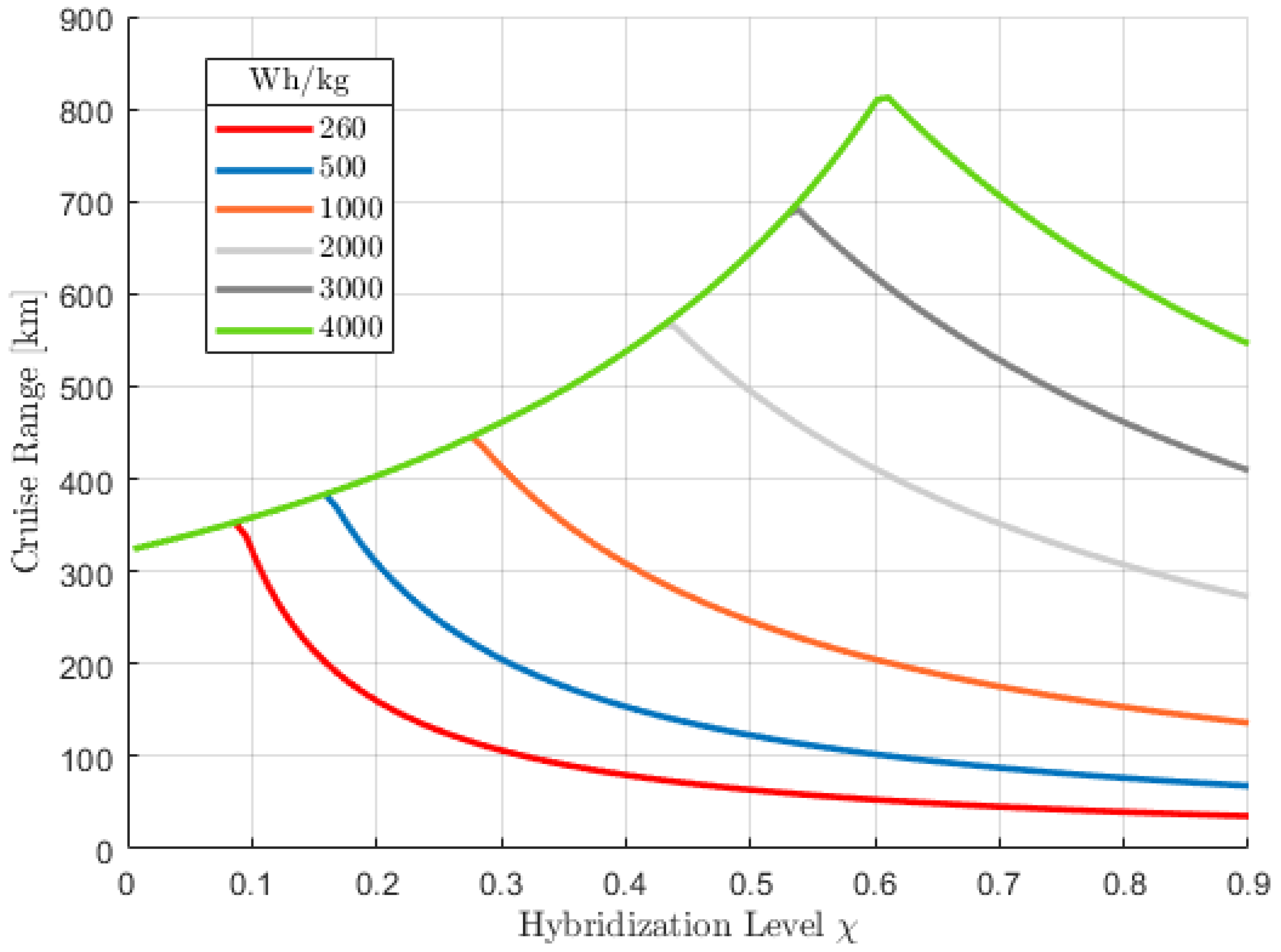
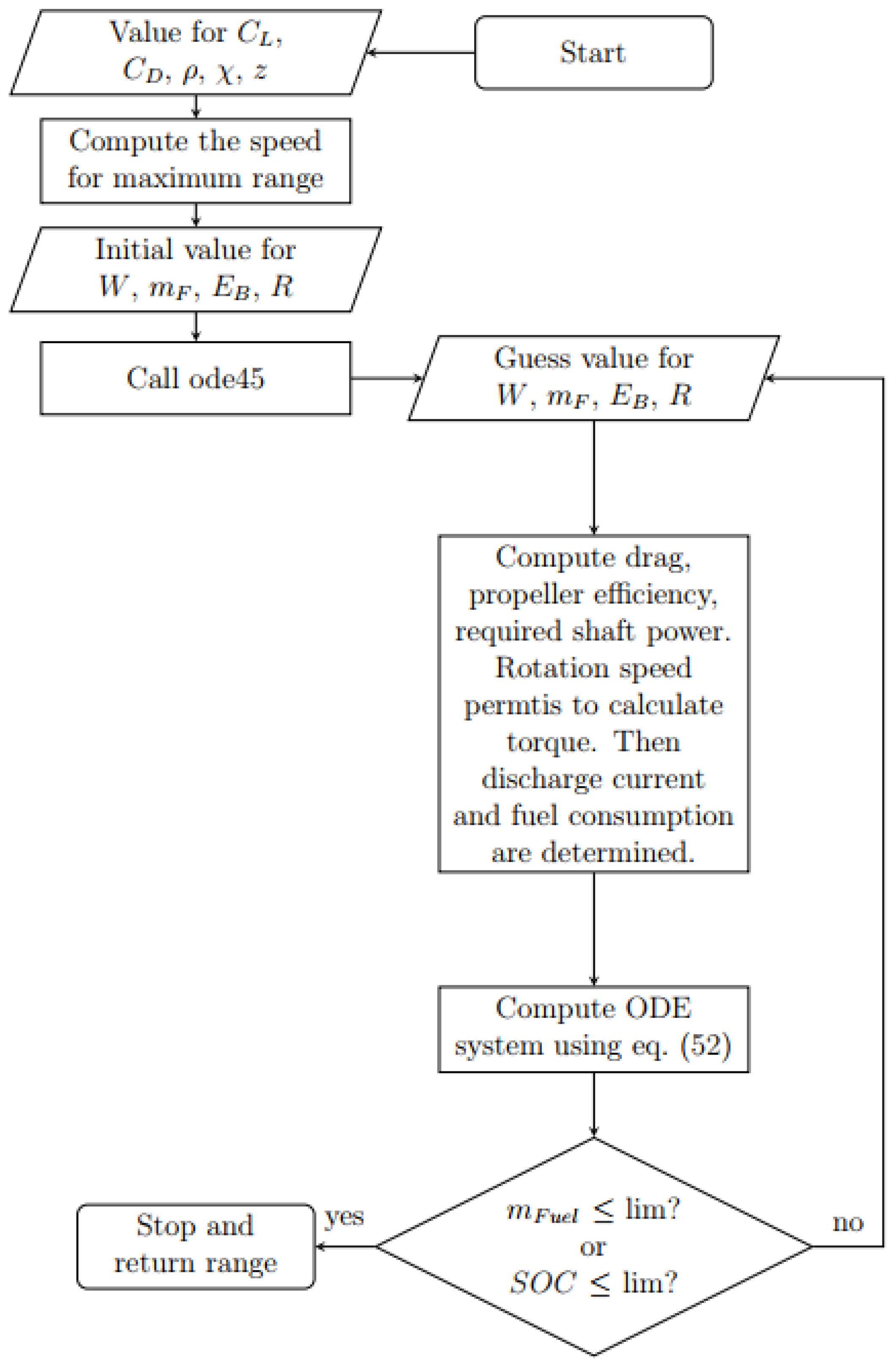
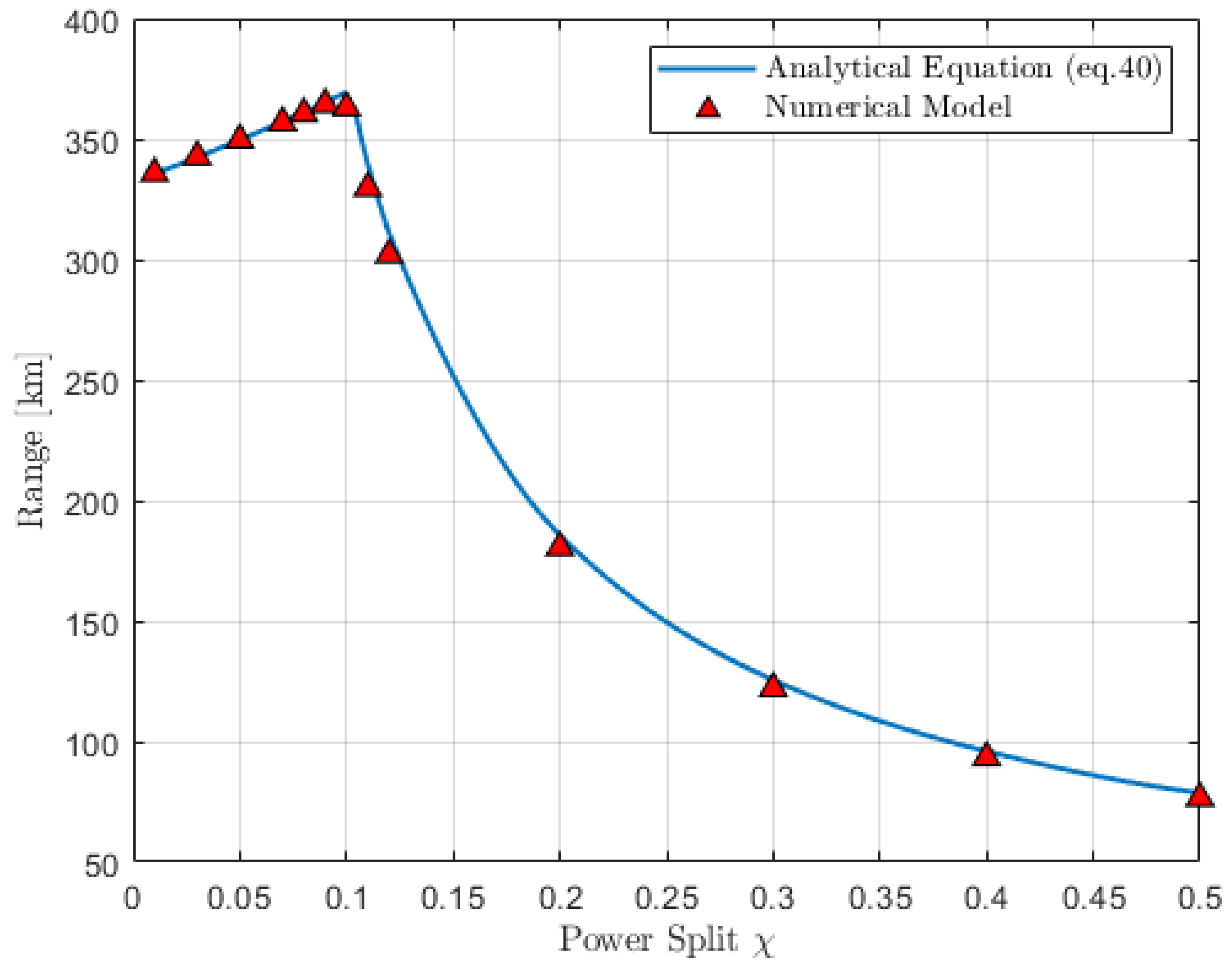
| Parallel | Series | |
|---|---|---|
| Fraction | Value | Ref. Par. | Value |
|---|---|---|---|
| 750 kg | |||
| 43 MJ/kg | |||
| 0.936 MJ/kg (260 Wh/kg) | |||
| E | 13 | ||
| 0.29 | |||
| 1 | 0.95 | ||
| 0.8 |
| Analytical Model Input | Numerical Model Input | ||
|---|---|---|---|
| 747 kg | |||
| 497 kg | |||
| 48 kg | |||
| 170 kg | |||
| 32 kg | |||
| 15 kg | |||
| 1 | 1 | ||
| Aircraft polar curve | |||
| ICE map | |||
| EM and inverter map | |||
| Propeller map | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cestino, E.; Pisu, D.; Sapienza, V.; Chesta, L.; Martilla, V. A New Range Equation for Hybrid Aircraft Design. Aerospace 2023, 10, 955. https://doi.org/10.3390/aerospace10110955
Cestino E, Pisu D, Sapienza V, Chesta L, Martilla V. A New Range Equation for Hybrid Aircraft Design. Aerospace. 2023; 10(11):955. https://doi.org/10.3390/aerospace10110955
Chicago/Turabian StyleCestino, Enrico, Davide Pisu, Vito Sapienza, Lorenzo Chesta, and Valentina Martilla. 2023. "A New Range Equation for Hybrid Aircraft Design" Aerospace 10, no. 11: 955. https://doi.org/10.3390/aerospace10110955
APA StyleCestino, E., Pisu, D., Sapienza, V., Chesta, L., & Martilla, V. (2023). A New Range Equation for Hybrid Aircraft Design. Aerospace, 10(11), 955. https://doi.org/10.3390/aerospace10110955