Estimating the Future Function of the Nipsa Reservoir due to Climate Change and Debris Sediment Factors
Abstract
1. Introduction
2. Materials and Methods
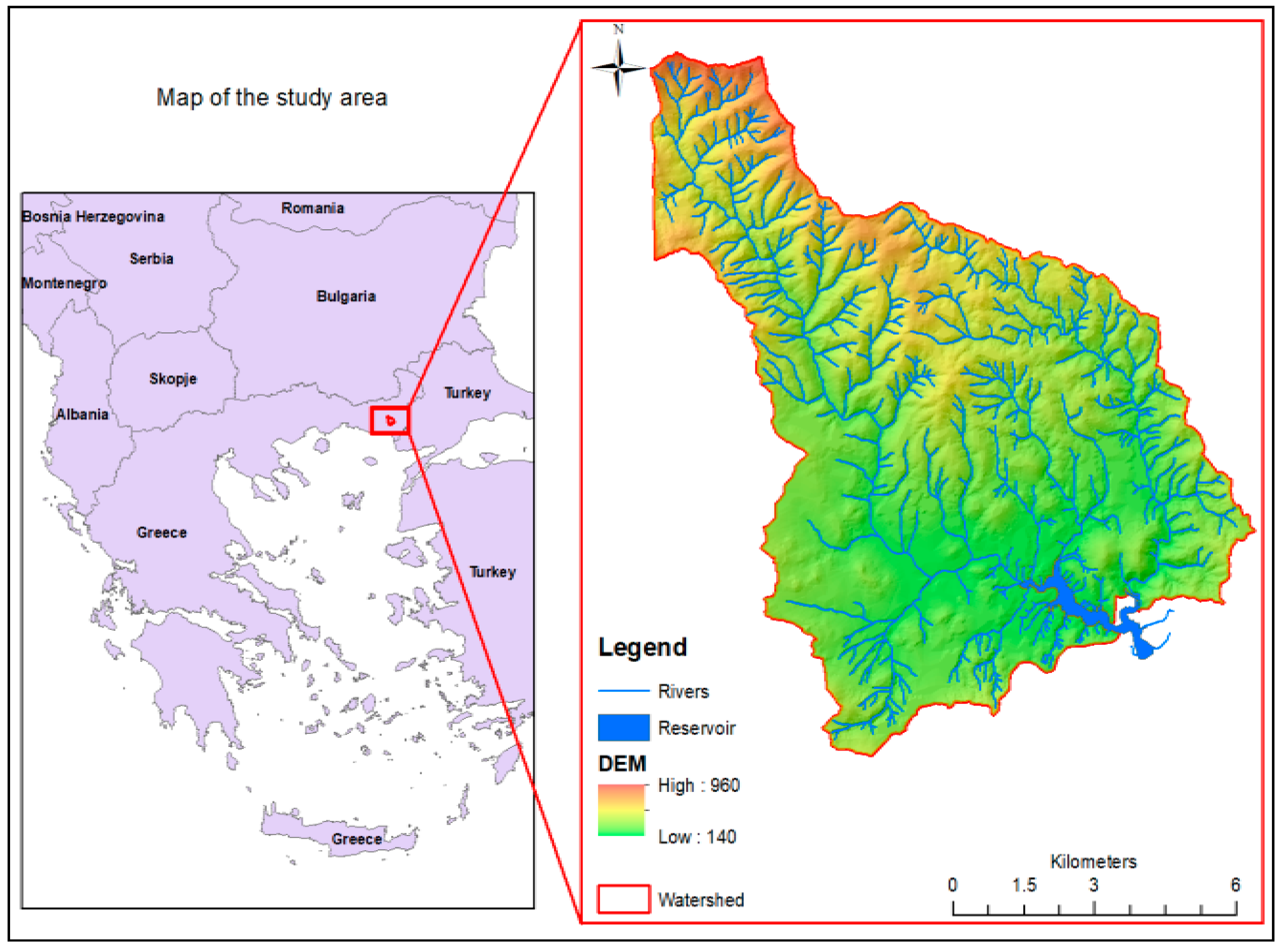
2.1. Study Area
Methodology
2.2. Sediment Yield Estimation
2.2.1. Universal Soil Loss Equation (USLE)
2.2.2. The Gavrilovic Method
2.3. Debris-Flow Spread and Deposition Simulation
2.3.1. TopRunDF Model
2.3.2. Mobility Coefficient
2.4. Water Balance Modelling
2.5. Climate Change Scenarios
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Sanmartin, J.F.; García, L.A.; Torres, A.M.; Boueno, I.E. Review article: Climate change impacts on dam safety. Nat. Hazards Earth Syst. Sci. 2018, 18, 2471–2488. [Google Scholar] [CrossRef]
- Skoulikaris, C.; Ganoulis, J. Multipurpose hydropower projects economic assessment under climate change conditions. Fresenious Environ. Bull. 2017, 26, 5599–5607. [Google Scholar]
- Kaffas, Κ.; Hrissanthou, V. Computation of hourly sediment discharges and annual sediment yields by means of two soil erosion models in a mountainous basin. Int. J. Riv. Basin Manag. 2017, 17, 63–77. [Google Scholar] [CrossRef]
- DeNoyelles, F.; Kastens, J.H. Reservoir sedimentation challenges Kansas. Trans. Kans. Acad. Sci. 2016, 119, 69–81. [Google Scholar] [CrossRef]
- Field, C.B.; Barros, V.R.; Dokken, D.J.; Mach, K.J.; Mastrandrea, M.D.; Bilir, T.E.; Chatterjee, M.; Ebi, K.L.; Estrada, Y.O.; Genova, R.C. Intergovernmental Panel on Climate Change (IPCC). Climate Change: Impacts, Adaptation, and Vulnerability, Part A: Global and Sectoral Aspects, Contribution of Working Group II to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Kundzewicz, Z.W.; Kanae, S.; Seneviratne, S.I.; Handmer, J.; Nicholls, N.; Peduzzi, P.; Mechler, R.; Bouwer, L.M.; Arnell, N.; Mach, K. Flood risk and climate change: Global and regional perspectives. Hydrol. Sci. J. 2014, 59, 1–28. [Google Scholar] [CrossRef]
- Biao, E.I. Assessing the impacts of climate change on river discharge dynamics in Oueme river basin (Benin, West Africa). Hydrology 2017, 4, 47. [Google Scholar] [CrossRef]
- Spartalis, S.; Iliadis, L.; Maris, F. An innovative risk evaluation system estimating its own fuzzy entropy. Math. Comput. Model. 2007, 46, 260–267. [Google Scholar] [CrossRef]
- Karamage, F.; Zhang, C.; Kayiranga, A.; Shao, H.; Fang, X.; Ndayisaba, F.; Nahayo, L.; Mupenzi, C.; Tian, G. USLE-Based assessment of soil erosion by water in the Nyabarongo River catchment, Rwanda. Int. J. Environ. Res. Public Health 2016, 13, 835. [Google Scholar] [CrossRef] [PubMed]
- Kostadinov, S.; Braunović, S.; Dragićević, S.; Zlatić, M.; Dragović, N.; Rakonjac, N. Effects of erosion control works: Case study—Grdelica Gorge, the South Morava River (Serbia). Water 2018, 10, 1094. [Google Scholar] [CrossRef]
- Bagarello, V.; Ferro, V.; Giordano, G.; Mannocchi, F.; Todisco, F.; Vergni, L. Statistical check of USLE-M and USLE-MM to predict bare plot soil loss in two Italian environments. Land Degrad. Dev. 2018, 29, 2614–2628. [Google Scholar] [CrossRef]
- Van der Knijff, J.M.; Jones, R.J.A.; Montanarella, L. Soil Erosion Risk Assessment in Europe; EUR 19044 EN; Office for Official Publications of the European Communities: Luxembourg, 2000; p. 3. [Google Scholar]
- Papaioannou, G.; Maris, F.; Loukas, A. Estimation of the erosion of the mountainous watershed of River Kosynthos (in Greek). In Proceedings of the Common Conference of EYE-EEDYP with Title Integrated Water Resources Management under Climatic Changes, Volos, Greece, 27–30 May 2009; pp. 453–460. [Google Scholar]
- Ali, S.A.; Hagos, H. Estimation of soil erosion using USLE and GIS in Awassa Catchment, Rift valley, Central Ethiopia. Geoderma Reg. 2016, 7, 159–166. [Google Scholar] [CrossRef]
- Kronvang, B.; Andersen, H.E.; Larsen, S.E.; Audet, J. Importance of bank erosion for sediment input, storage and export at the catchment scale. J. Soil. Sedim. 2013, 13, 230. [Google Scholar] [CrossRef]
- Alexandridis, T.K.; Monachou, S.; Skoulikaris, C.; Kalopesa, E.; Zalidis, G.C. Investigation of the temporal relation of remotely sensed coastal water quality with GIS modeled upstream soil erosion. Hydrol. Process. 2015, 29, 2373–2384. [Google Scholar] [CrossRef]
- Auddino, M.; Dominici, R.; Viscomi, A. Evaluation of yield sediment in the Sfalassà Fiumara (southwestern, Calabria) by using Gavrilović method in GIS environment. Rend. Online Soc. Geol. Ital. 2015, 33, 3–7. [Google Scholar]
- Scheidl, C.; Rickenmann, D. Empirical prediction of debris-flow mobility and deposition on fans. Earth Surf. Process. Landf. 2010, 35, 157–173. [Google Scholar] [CrossRef]
- Kappes, M.S.; Malet, J.P.; Remaître, A.; Horton, P.; Jaboyedoff, M.; Bell, R. Assessment of debris-flow susceptibility at medium-scale in the Barcelonnette Basin, France. Nat. Hazards Earth Syst. Sci. 2011, 11, 627–641. [Google Scholar] [CrossRef]
- Scheidl, C.; Chiari, M.; Rickenmann, D. The use of airborne LiDAR data for the analysis of debris flow events in Switzerland. Nat. Hazards Earth Syst. Sci. 2008, 8, 1113–1127. [Google Scholar] [CrossRef]
- Vasileiou, A.; Maris, F.; Varsami, G. Estimation of sedimentation to the torrential sedimentation fan of the Dadia stream with the use of the TopRunDF and the GIS models. Adv. Res. Aquat. Environ. Environ. Earth Sci. 2013, 3, 207–214. [Google Scholar] [CrossRef]
- TechnoLogismiki Works 2013. Water Budget v9.0—Computational Issue. Available online: http://www.technologismiki.com (accessed on 26 September 2013).
- Arsenault, R.; Poulin, A.; Côté, P.; Brissette, F. Comparison of stochastic optimization algorithms in hydrological model calibration. J. Hydrol. Eng. 2014, 19, 1374–1384. [Google Scholar] [CrossRef]
- Farmer, W.H.; Vogel, R.M. Research Article: On the deterministic and stochastic use of hydrologic models. Water Resour. Res. 2016, 52, 5619–5633. [Google Scholar] [CrossRef]
- Thuiller, W.; Lavergne, S.; Roquet, C.; Boulangeat, I.; Lafourcade, B.; Araujo, M.B. Consequences of climate change on the tree of life in Europe. Nat. Int. J. Sci. 2011, 470, 531–534. [Google Scholar] [CrossRef] [PubMed]
- Phillips, S.J.; Dudík, M.; Schapire, R.E. Maxent software for modeling species niches and distributions (Version 3.4.1). Available online: http://biodiversityinformatics.amnh.org/open_source/maxent/ (accessed on 28 February 2019).
- Panagos, P.; Meusburger, K.; Ballabio, C.; Borrelli, P.; Alewell, C. Soil erodibility in Europe: A high-resolution dataset based on LUCAS. Sci. Total Environ. 2014, 479–480, 189–200. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Thompson, D.K.; Marshall, G.A.; Tymstra, C.; Carr, R.; Flannigan, M.K. Increasing frequency of extreme fire weather in Canada with climate change. Clim. Ch. 2015, 130, 573–586. [Google Scholar] [CrossRef]
- Belanovic, S.S.; Perović, V.; Vidojević, D.; Kostadinov, S.; Knezevic, M.; Kadović, R.; Košanin, O. Assessment of soil erosion intensity in Kolubara District, Serbia. Fresenius Environ. Bull. 2013, 22, 1556–1563. [Google Scholar]
- Zivotic, L.; Perović, V.; Jaramaz, D.; Djordevic, A.; Petrović, R.; Todorovic, M. Application of USLE, GIS, and remote sensing in the assessment of soil erosion rates in Southeastern Serbia. Pol. J. Environ. Stud. 2012, 21, 1929–1935. [Google Scholar]
- Dragičević, N.; Karleuša, B.; Ožanić, N. A review of the Gavrilovic method (erosion potential method) application. Gradjevinar 2016, 68, 715–725. [Google Scholar] [CrossRef]



| ID | GAVRILOVIC | USLE | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| x | y | φ | J | T | h | R | LS | K | C | |
| 1 | 0,1111 | 0,62 | 0,25 | 13,19 | 1,505 | 481,2 | 381,696 | 1,334 | 0,553 | 0,048 |
| 2 | 0,1516 | 0,83 | 0,45 | 16,39 | 1,505 | 481,2 | 381,696 | 2,753 | 0,806 | 0,026 |
| 3 | 0,2366 | 0,65 | 0,35 | 12,62 | 1,505 | 481,2 | 381,696 | 1,159 | 0,596 | 0,097 |
| 4 | 0,2287 | 0,71 | 0,40 | 14,61 | 1,505 | 481,2 | 381,696 | 2,172 | 0,663 | 0,021 |
| 5 | 0,2913 | 0,54 | 0,15 | 10,48 | 1,505 | 481,2 | 381,696 | 0,804 | 0,454 | 0,130 |
| 6 | 0,2677 | 0,74 | 0,20 | 8,33 | 1,505 | 481,2 | 381,696 | 0,688 | 0,695 | 0,049 |
| 7 | 0,1416 | 0,67 | 0,15 | 11,20 | 1,505 | 481,2 | 381,696 | 0,887 | 0,649 | 0,022 |
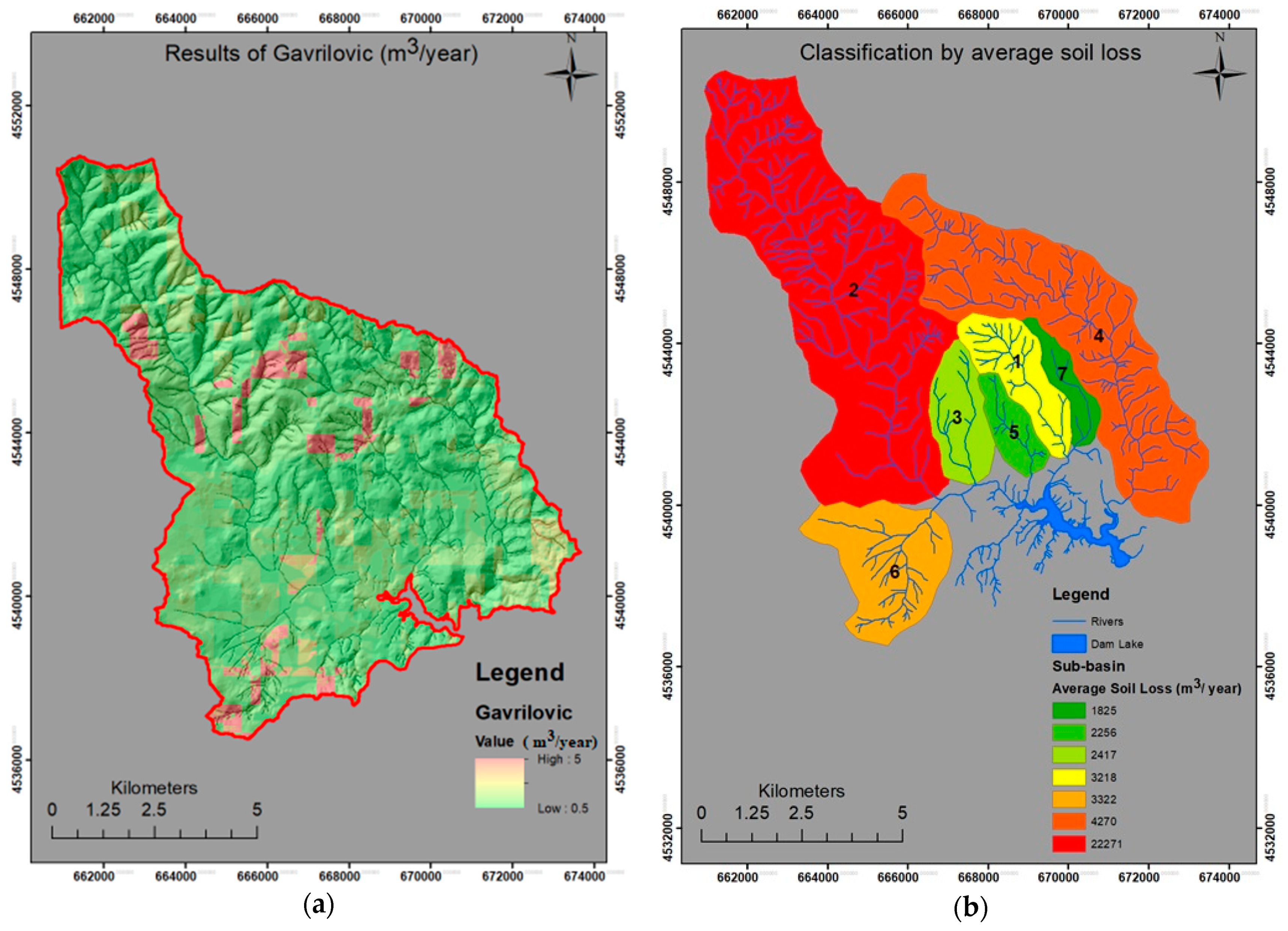
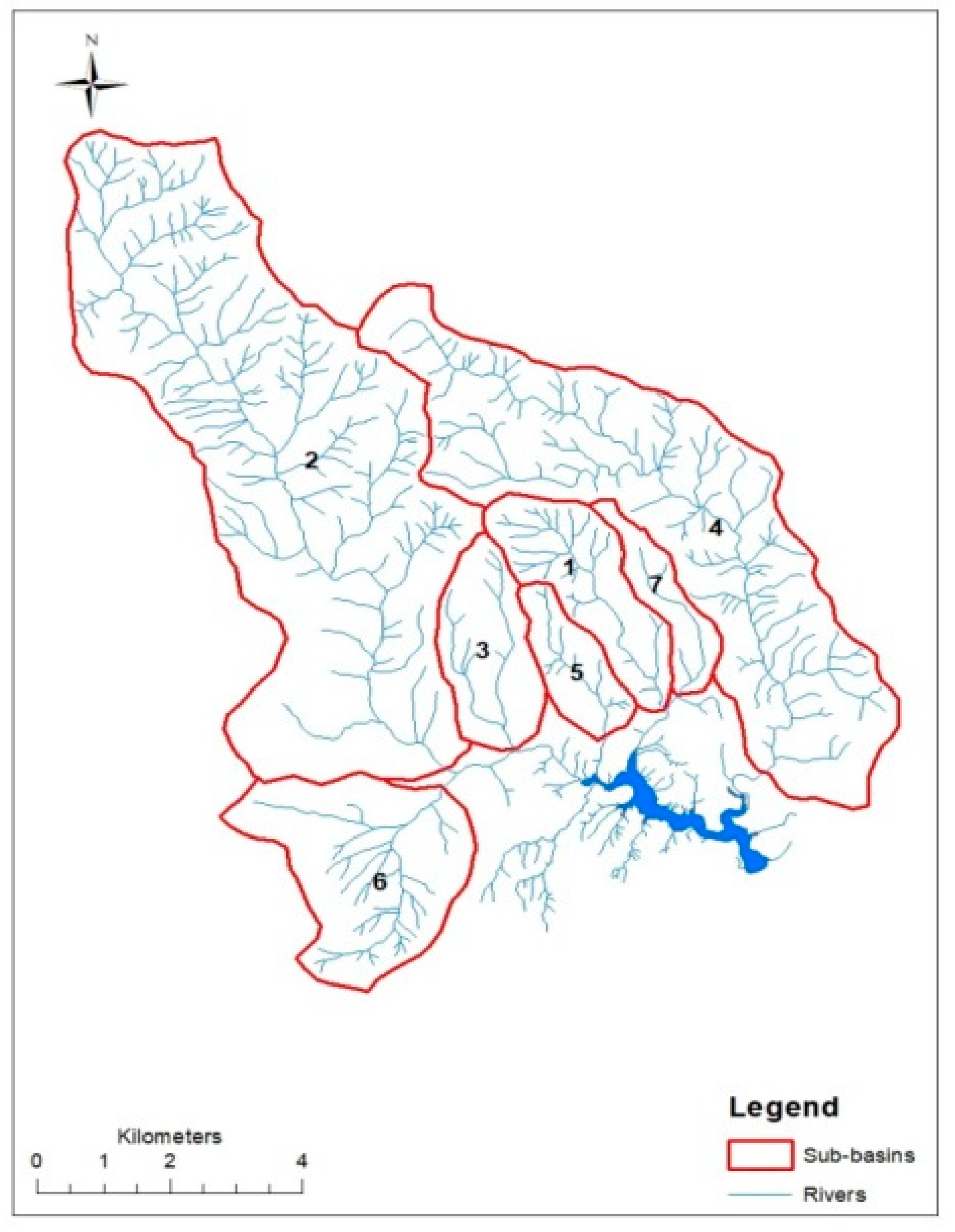
| ID | USLE (m3/Year) | Gavrilovic (m3/Year) | Average (m3/Year) | Maximum Discharge (m3/sec) |
|---|---|---|---|---|
| 1 | 3150 | 3286 | 3218 | 57.35 |
| 2 | 22959 | 21583 | 22271 | 87.02 |
| 3 | 2129 | 2705 | 2417 | 67.35 |
| 4 | 4150 | 4390 | 4270 | 79.38 |
| 5 | 2350 | 2162 | 2256 | 62.79 |
| 6 | 3522 | 3122 | 3322 | 59.82 |
| 7 | 1928 | 1722 | 1825 | 45.78 |
| Oct | Nov | Dec | Jan | Feb | Mar | Apr | Μay | Jun | Jul | Aug | Sep | Average | Months/Scenariοs |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 49.0 | 34.4 | 55.1 | 8.7 | 10.1 | 62.9 | 64.1 | 64.1 | 63.7 | 55.4 | 36.3 | 26.6 | 42.75 | HadCM2_GGd |
| 28.0 | 14.2 | 19.7 | 2.1 | 44.9 | 33.4 | 26.3 | 18.4 | 23.4 | 29.1 | 39.9 | 28.1 | 25.63 | ECHAM 4 |
| 26.0 | 9.7 | 33.5 | 1.2 | 31.4 | 19.0 | 18.8 | 12.3 | 18.7 | 26.3 | 35.3 | 26.7 | 21.58 | CSIRO-MK2 |
| 28.2 | 12.6 | 9.0 | 0.7 | 29.9 | 15.1 | 24.9 | 2.4 | 21.1 | 37.6 | 61.8 | 34.2 | 15.94 | CGCM1 |
| 18.8 | 3.7 | 8.8 | 3.1 | 4.7 | 12.6 | 15.1 | 16.4 | 17.1 | 14.8 | 16.0 | 14.3 | 12.11 | CCSR-98 |
| Oct | Nov | Dec | Jan | Feb | Mar | Apr | Μay | Jun | Jul | Aug | Sep | Sum | Months/Scenariοs |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1.40 | 1.79 | 2.99 | 2.55 | 0.32 | 1.01 | 1.22 | 1.39 | 0.88 | 0.68 | 0.27 | 0.67 | 15.17 | Base Scenarios |
| 0.71 | 1.18 | 1.34 | 2.55 | 0.29 | 0.12 | 0.12 | 0.50 | 0.32 | 0.32 | 0.30 | 0.17 | 7.92 | HadCM2_GGd |
| 1.01 | 1.54 | 2.40 | 2.77 | 0.18 | 0.22 | 0.24 | 0.26 | 0.67 | 0.67 | 0.48 | 0.16 | 10.60 | ECHAM 4 |
| 1.03 | 1.62 | 1.99 | 0.32 | 0.22 | 0.26 | 0.26 | 0.28 | 0.71 | 0.71 | 0.50 | 0.17 | 8.09 | CSIRO-MK2 |
| 1.00 | 1.57 | 2.72 | 0.32 | 0.23 | 1.16 | 1.52 | 1.43 | 0.69 | 0.69 | 0.42 | 0.10 | 11.86 | CGCM1 |
| 1.14 | 1.73 | 2.73 | 2.56 | 0.31 | 0.28 | 0.28 | 0.27 | 0.73 | 0.73 | 0.58 | 0.23 | 11.55 | CCSR-98 |
| 2000–2020 | 2021–2040 | 2041–2060 | 2061–2080 | 2081–2100 | Average | Years/Scenarios |
|---|---|---|---|---|---|---|
| 39.22 | 40.89 | 42.45 | 44.16 | 47.03 | 42.75 | HadCM2_GGd |
| 22.79 | 24.12 | 25.22 | 26.77 | 29.25 | 25.63 | ECHAM 4 |
| 20.19 | 20.57 | 21.16 | 22.09 | 23.89 | 21.58 | CSIRO-MK2 |
| 15.37 | 15.71 | 15.91 | 16.08 | 16.63 | 15.94 | CGCM1 |
| 11.5 | 11.94 | 12.05 | 12.2 | 12.86 | 12.11 | CCSR-98 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maris, F.; Vasileiou, A.; Tsiamantas, P.; Angelidis, P. Estimating the Future Function of the Nipsa Reservoir due to Climate Change and Debris Sediment Factors. Climate 2019, 7, 76. https://doi.org/10.3390/cli7060076
Maris F, Vasileiou A, Tsiamantas P, Angelidis P. Estimating the Future Function of the Nipsa Reservoir due to Climate Change and Debris Sediment Factors. Climate. 2019; 7(6):76. https://doi.org/10.3390/cli7060076
Chicago/Turabian StyleMaris, Fotios, Apostolos Vasileiou, Panagiotis Tsiamantas, and Panagiotis Angelidis. 2019. "Estimating the Future Function of the Nipsa Reservoir due to Climate Change and Debris Sediment Factors" Climate 7, no. 6: 76. https://doi.org/10.3390/cli7060076
APA StyleMaris, F., Vasileiou, A., Tsiamantas, P., & Angelidis, P. (2019). Estimating the Future Function of the Nipsa Reservoir due to Climate Change and Debris Sediment Factors. Climate, 7(6), 76. https://doi.org/10.3390/cli7060076







