The Relationship between Madden–Julian Oscillation Moist Convective Circulations and Tropical Cyclone Genesis
Abstract
1. Introduction
1.1. Madden Julian Oscillation (MJO) Overview
1.2. MJO Impacts on Tropical Cyclones (TCs)
1.3. Motivation
2. Materials and Methods
2.1. Data
2.1.1. GPCP Rainfall Estimates
2.1.2. IGRA Sounding Data
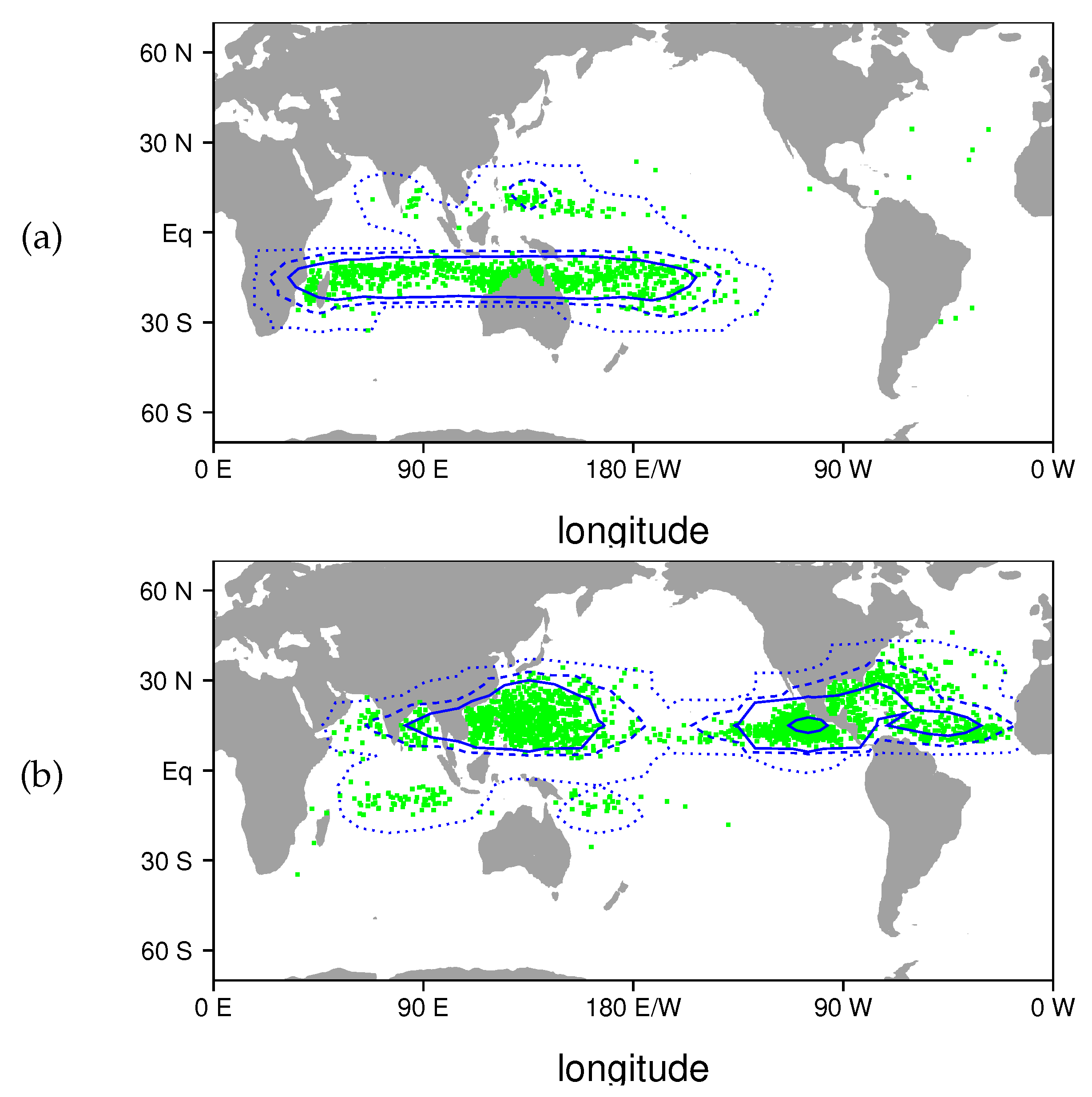
2.1.3. IBTRACS Tropical Cyclone Data
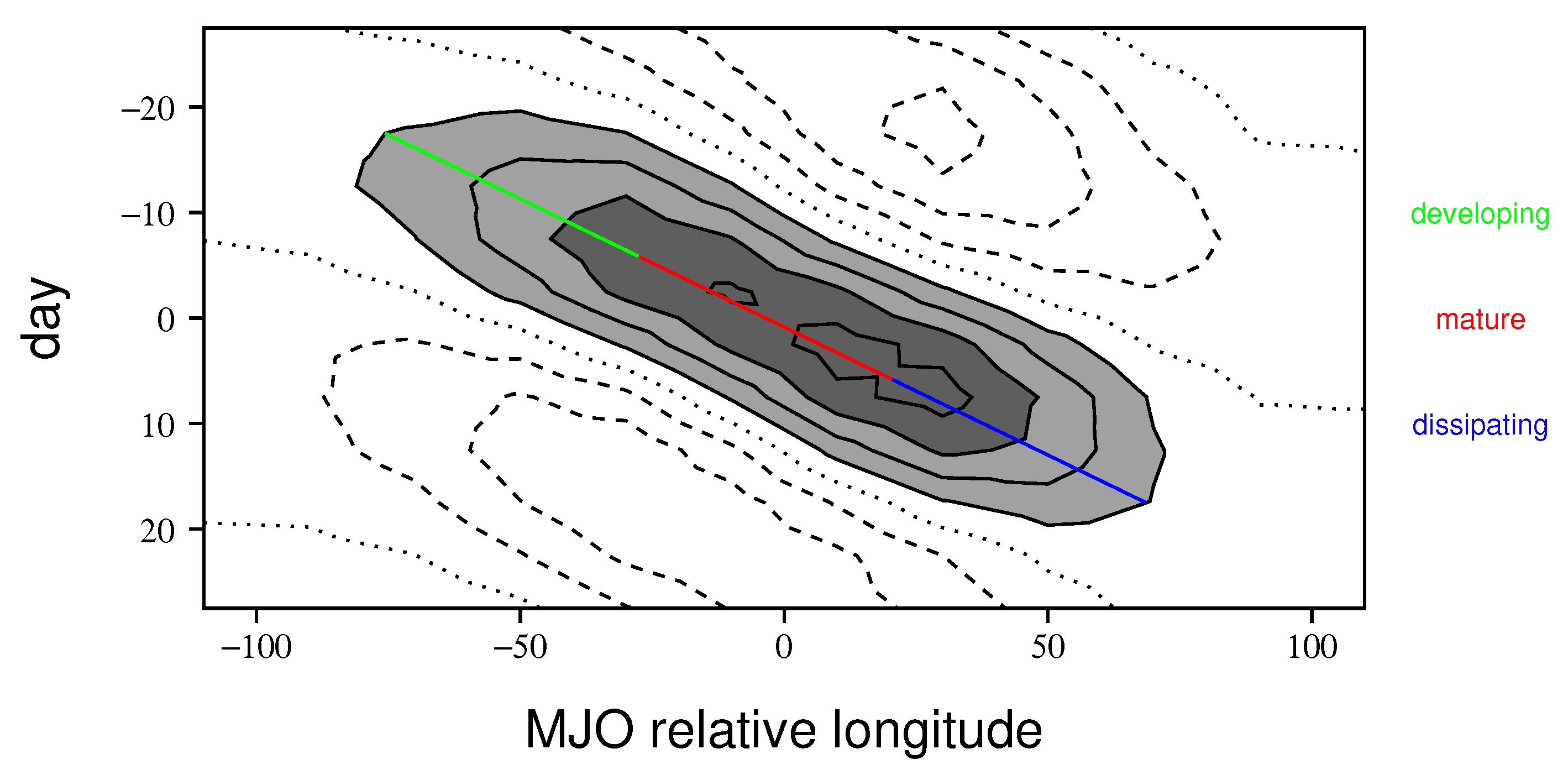
2.2. Identifying and Compositing MJOs
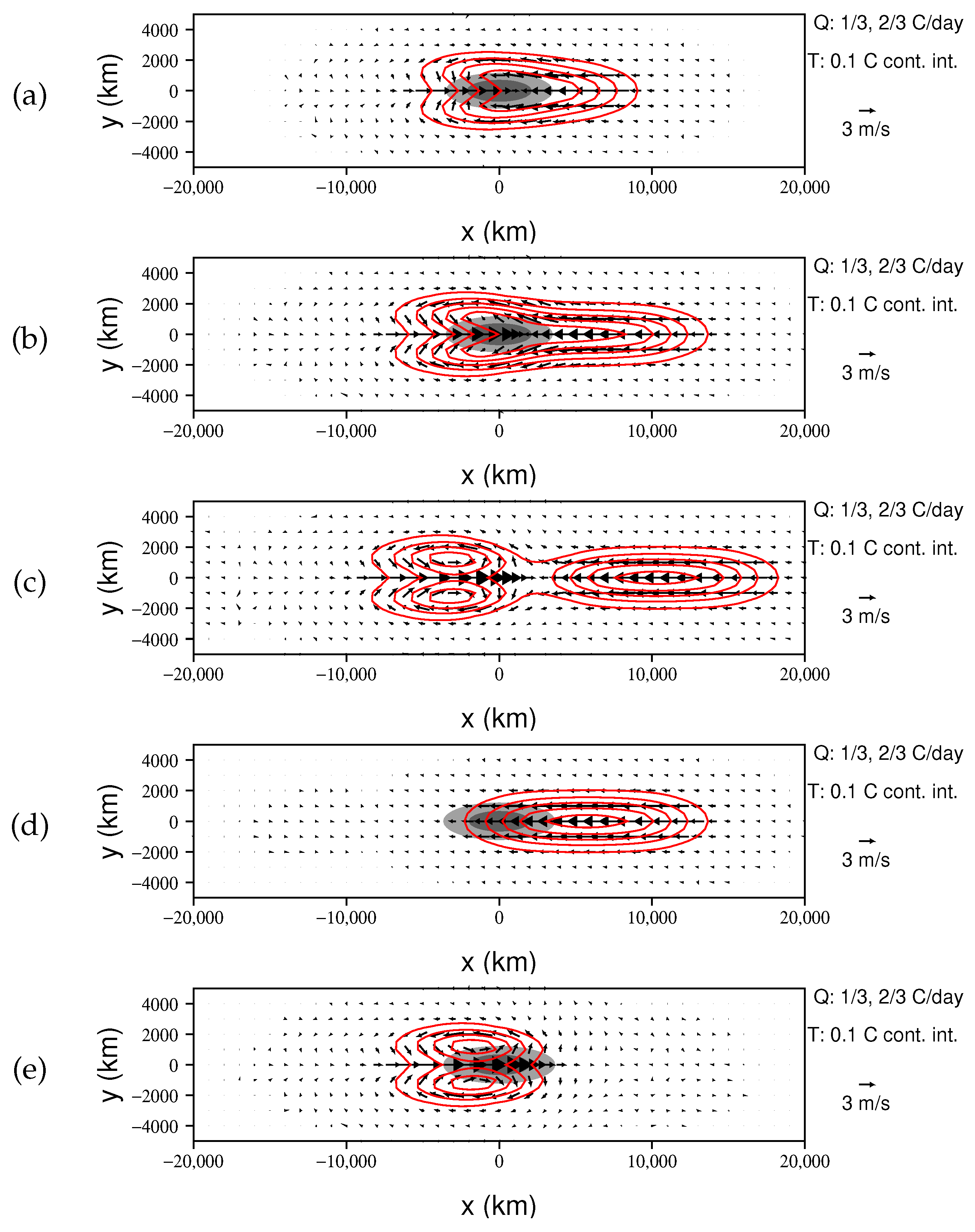
2.3. Kelvin/Rossby Wave Decomposition
3. Results
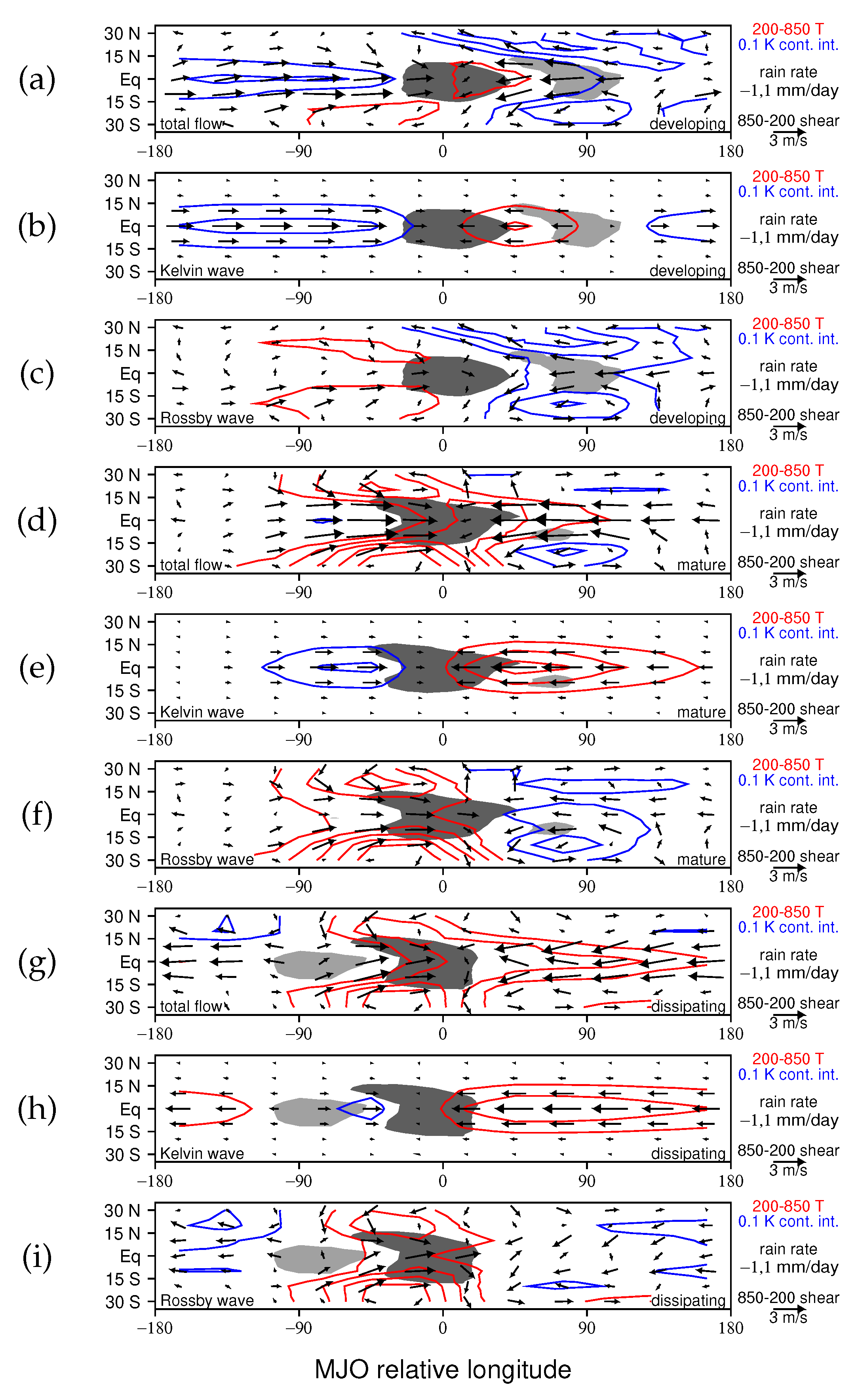
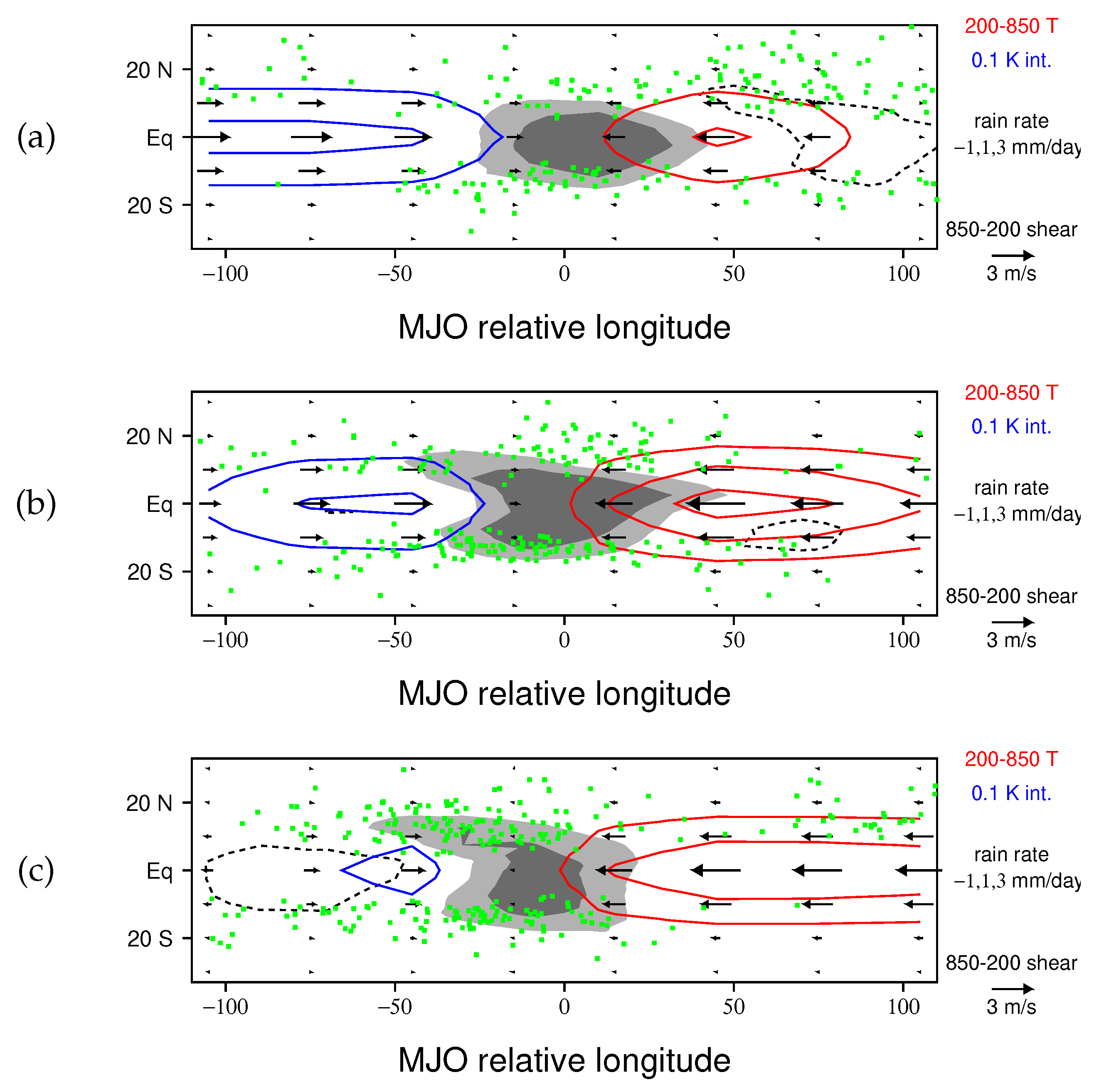
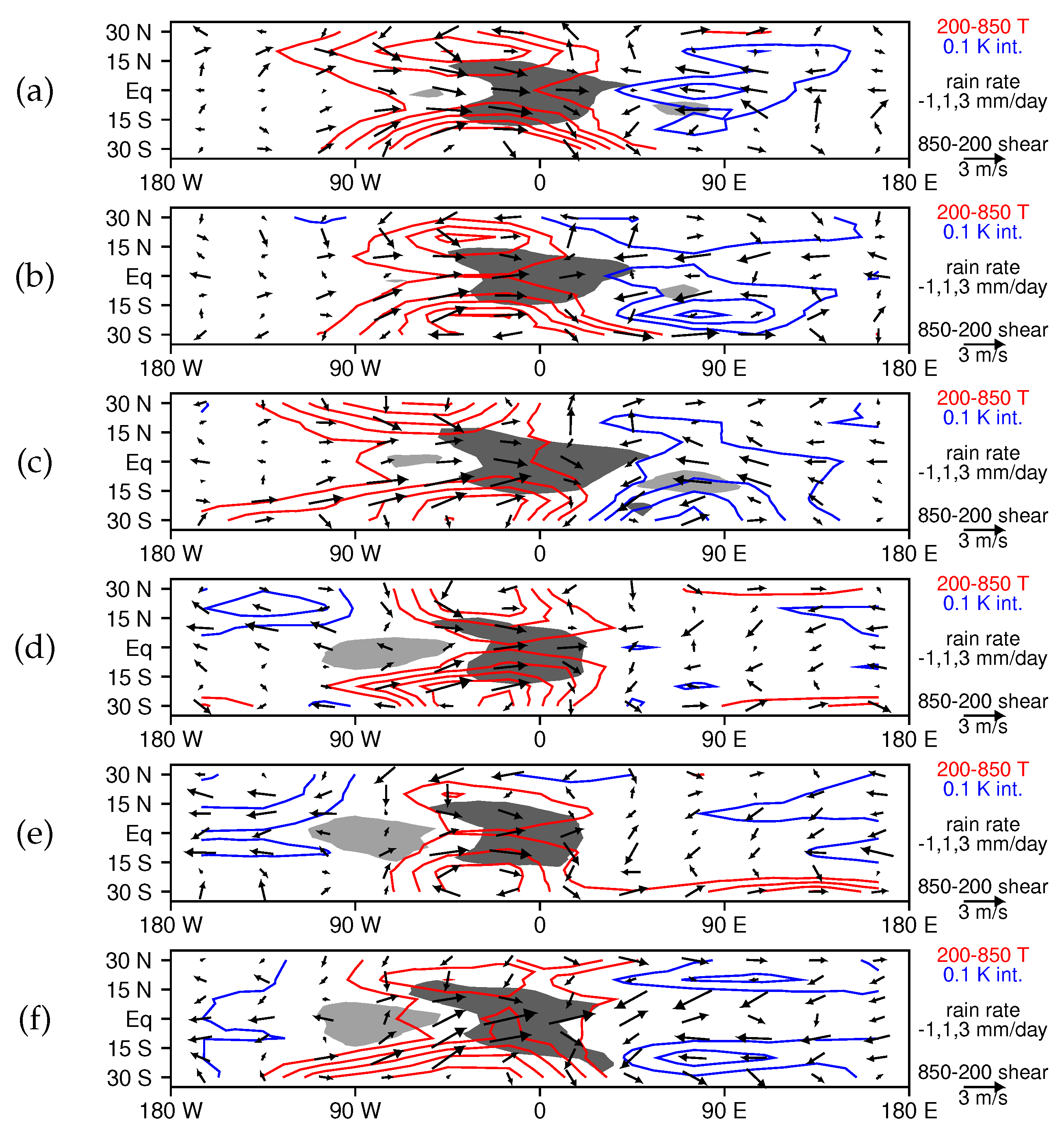
3.1. Composite MJO Circulations
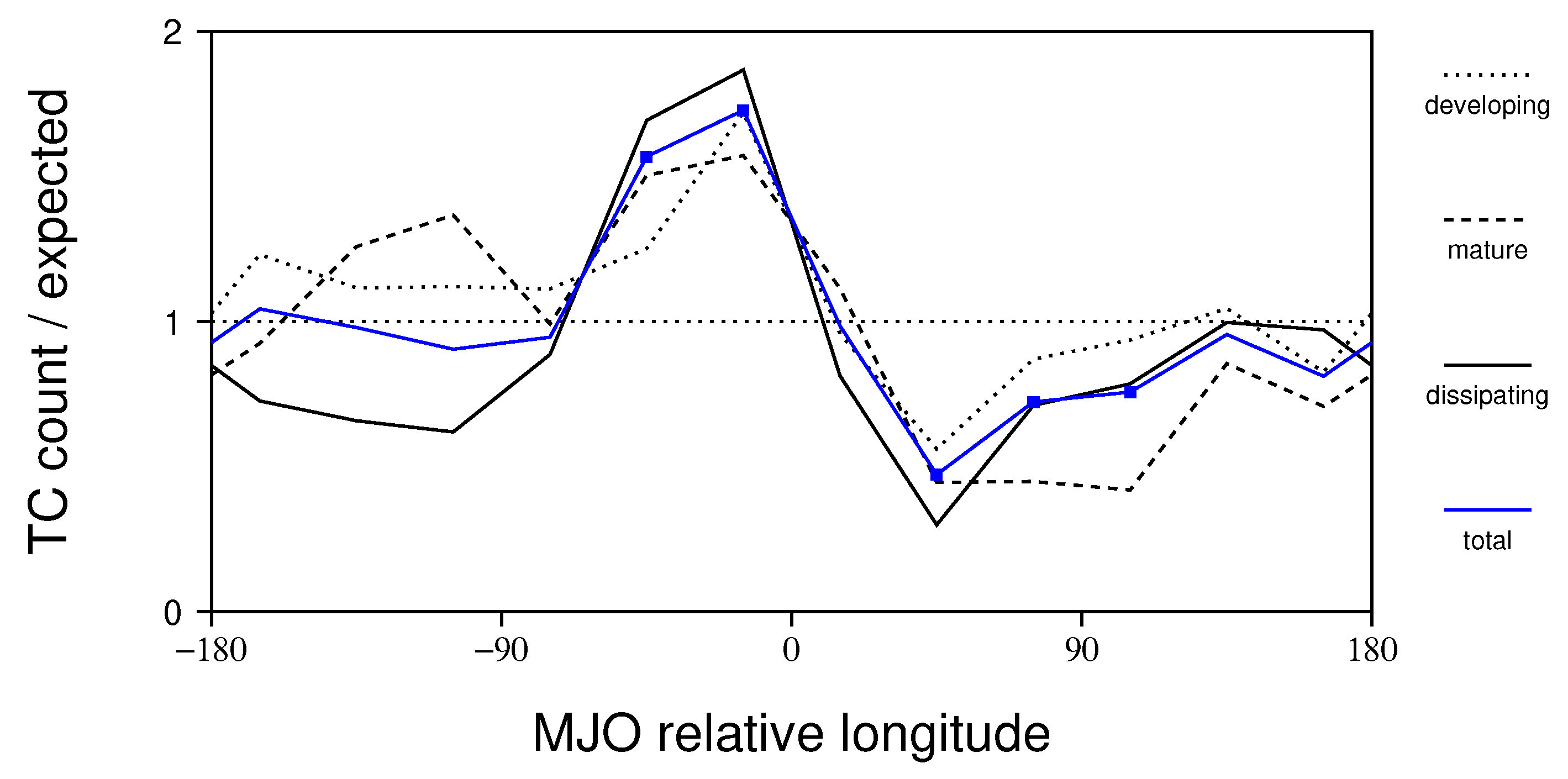
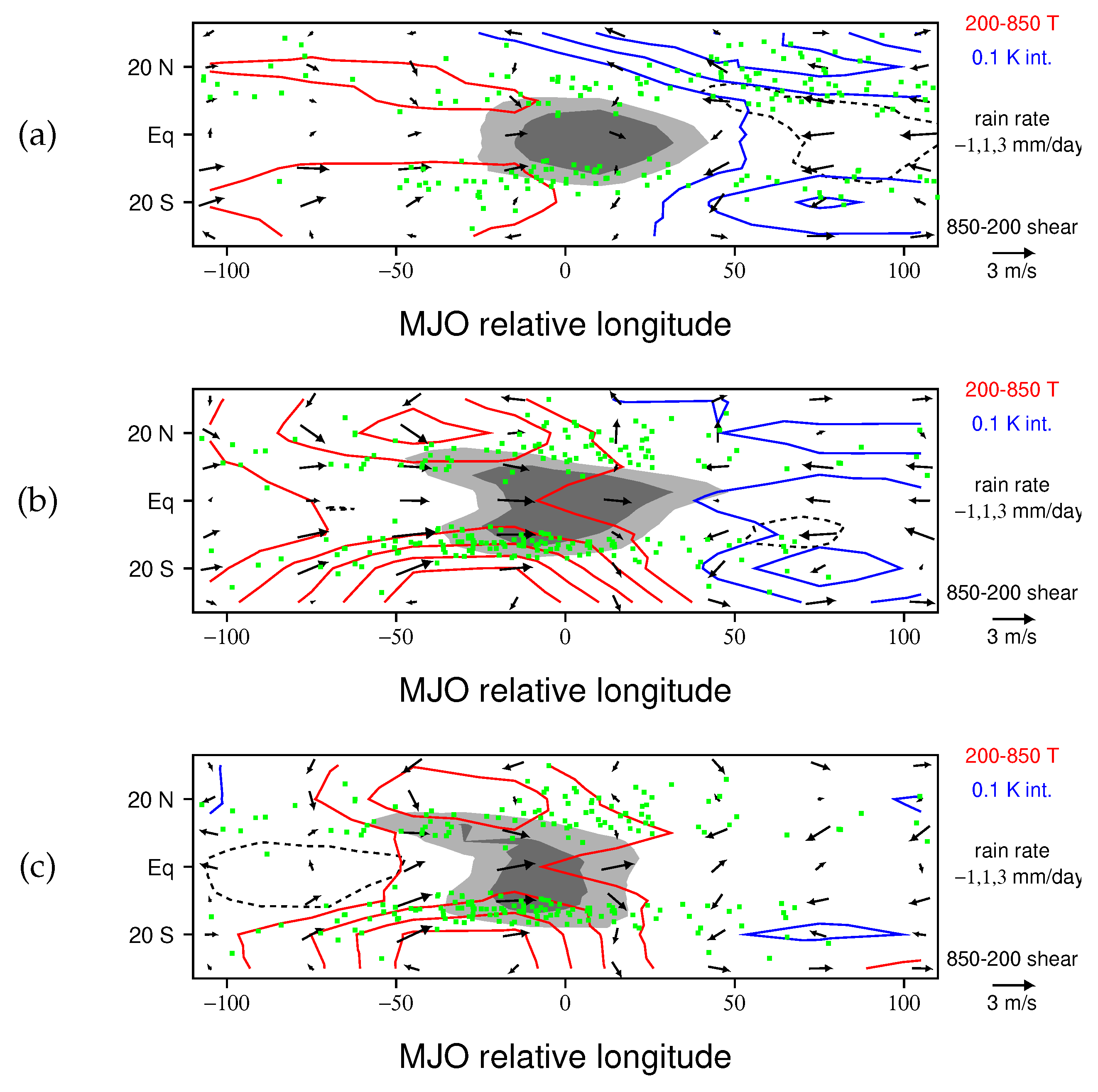
3.2. Kelvin and Rossby Wave Impacts on TC Genesis
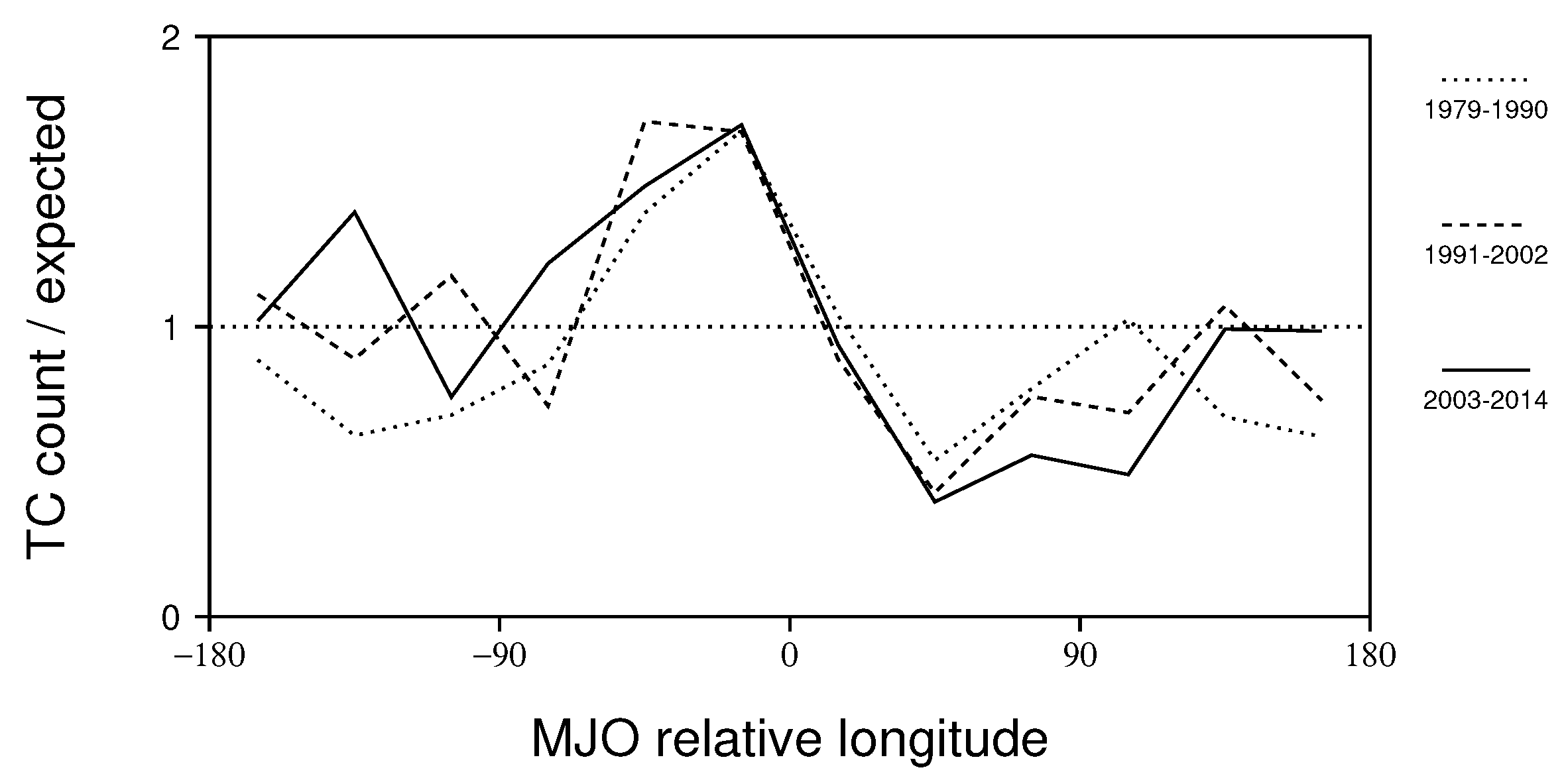
3.3. Changes in MJO Circulations and TC Impacts over Time
4. Discussion
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Madden, R.A.; Julian, P.R. Detection of a 40–50 day oscillation in the zonal wind in the tropical Pacific. J. Atmos. Sci. 1971, 28, 702–708. [Google Scholar] [CrossRef]
- Madden, R.A.; Julian, P.R. Description of global-scale circulation cells in the tropics with a 40–50 day period. J. Atmos. Sci. 1972, 29, 1109–1123. [Google Scholar] [CrossRef]
- Madden, R.A.; Julian, P.R. Observations of the 40–50-day tropical oscillation—A review. Mon. Weather Rev. 1994, 122, 814–837. [Google Scholar] [CrossRef]
- Zhang, C. Madden-julian oscillation. Rev. Geophys. 2005, 43, RG2003. [Google Scholar] [CrossRef]
- Wheeler, M.; Kiladis, G.N.; Webster, P.J. Large-scale dynamical fields associated with convectively coupled equatorial waves. J. Atmos. Sci. 2000, 57, 613–640. [Google Scholar] [CrossRef]
- Sobel, A.; Kim, D. The MJO-Kelvin wave transition. Geophys. Res. Lett. 2012, 39, L20808. [Google Scholar] [CrossRef]
- Haertel, P.; Straub, K.; Budsock, A. Transforming circumnavigating Kelvin waves that initiate and dissipate the Madden–Julian Oscillation. Q. J. R. Meteorol. Soc. 2015, 141, 1586–1602. [Google Scholar] [CrossRef]
- Wu, M.L.C.; Schubert, S.; Huang, N.E. The development of the South Asian summer monsoon and the intraseasonal oscillation. J. Clim. 1999, 12, 2054–2075. [Google Scholar] [CrossRef]
- Lorenz, D.J.; Hartmann, D.L. The effect of the MJO on the North American monsoon. J. Clim. 2006, 19, 333–343. [Google Scholar] [CrossRef]
- Haertel, P.; Boos, W.R. Global association of the Madden-Julian Oscillation with monsoon lows and depressions. Geophys. Res. Lett. 2017, 44, 8065–8074. [Google Scholar] [CrossRef]
- Mundhenk, B.D.; Barnes, E.A.; Maloney, E.D.; Baggett, C.F. Skillful empirical subseasonal prediction of landfalling atmospheric river activity using the Madden–Julian oscillation and quasi-biennial oscillation. NPJ Clim. Atmos. Sci. 2018, 1, 1–7. [Google Scholar] [CrossRef]
- Liang, Y.; Fedorov, A.V. Linking the Madden–Julian Oscillation, tropical cyclones and westerly wind bursts as part of El Niño development. Clim. Dyn. 2021, 57, 1039–1060. [Google Scholar] [CrossRef]
- Liang, Y.; Fedorov, A.V.; Haertel, P. Intensification of Westerly Wind Bursts Caused by the Coupling of the Madden-Julian Oscillation to SST During El Niño Onset and Development. Geophys. Res. Lett. 2021, 48, e2020GL089395. [Google Scholar] [CrossRef]
- Hu, S.; Fedorov, A.V. The extreme El Niño of 2015–2016: The role of westerly and easterly wind bursts, and preconditioning by the failed 2014 event. Clim. Dyn. 2019, 52, 7339–7357. [Google Scholar] [CrossRef]
- Waliser, D.; Lau, K.; Stern, W.; Jones, C. Potential predictability of the Madden–Julian oscillation. Bull. Am. Meteorol. Soc. 2003, 84, 33–50. [Google Scholar] [CrossRef]
- Neena, J.; Lee, J.Y.; Waliser, D.; Wang, B.; Jiang, X. Predictability of the Madden–Julian oscillation in the intraseasonal variability hindcast experiment (ISVHE). J. Clim. 2014, 27, 4531–4543. [Google Scholar] [CrossRef]
- Liebmann, B.; Hendon, H.H.; Glick, J.D. The relationship between tropical cyclones of the Western Pacific and Indian Oceans and the Madden-Julian oscillation. J. Meteorol. Soc. Jpn. Ser. II 1994, 72, 401–412. [Google Scholar] [CrossRef]
- Kim, J.H.; Ho, C.H.; Kim, H.S.; Sui, C.H.; Park, S.K. Systematic variation of summertime tropical cyclone activity in the western North Pacific in relation to the Madden–Julian oscillation. J. Clim. 2008, 21, 1171–1191. [Google Scholar] [CrossRef]
- Chen, J.M.; Wu, C.H.; Chung, P.H.; Sui, C.H. Influence of intraseasonal–interannual oscillations on tropical cyclone genesis in the western North Pacific. J. Clim. 2018, 31, 4949–4961. [Google Scholar] [CrossRef]
- Hall, J.D.; Matthews, A.J.; Karoly, D.J. The modulation of tropical cyclone activity in the Australian region by the Madden–Julian oscillation. Mon. Weather Rev. 2001, 129, 2970–2982. [Google Scholar] [CrossRef]
- Bessafi, M.; Wheeler, M.C. Modulation of south Indian Ocean tropical cyclones by the Madden–Julian oscillation and convectively coupled equatorial waves. Mon. Weather Rev. 2006, 134, 638–656. [Google Scholar] [CrossRef]
- Krishnamohan, K.; Mohanakumar, K.; Joseph, P. The influence of Madden–Julian oscillation in the genesis of north Indian Ocean tropical cyclones. Theor. Appl. Climatol. 2012, 109, 271–282. [Google Scholar] [CrossRef]
- Wheeler, M.C.; Hendon, H.H. An all-season real-time multivariate MJO index: Development of an index for monitoring and prediction. Mon. Weather Rev. 2004, 132, 1917–1932. [Google Scholar] [CrossRef]
- Girishkumar, M.; Suprit, K.; Vishnu, S.; Prakash, V.T.; Ravichandran, M. The role of ENSO and MJO on rapid intensification of tropical cyclones in the Bay of Bengal during October–December. Theor. Appl. Climatol. 2015, 120, 797–810. [Google Scholar] [CrossRef]
- Bhardwaj, P.; Singh, O.; Pattanaik, D.; Klotzbach, P.J. Modulation of Bay of Bengal tropical cyclone activity by the Madden-Julian oscillation. Atmos. Res. 2019, 229, 23–38. [Google Scholar] [CrossRef]
- Maloney, E.D.; Hartmann, D.L. Modulation of eastern North Pacific hurricanes by the Madden–Julian oscillation. J. Clim. 2000, 13, 1451–1460. [Google Scholar] [CrossRef]
- Barrett, B.S.; Leslie, L.M. Links between tropical cyclone activity and Madden–Julian oscillation phase in the North Atlantic and northeast Pacific basins. Mon. Weather Rev. 2009, 137, 727–744. [Google Scholar] [CrossRef]
- Klotzbach, P.J.; Oliver, E.C. Modulation of Atlantic basin tropical cyclone activity by the Madden–Julian oscillation (MJO) from 1905 to 2011. J. Clim. 2015, 28, 204–217. [Google Scholar] [CrossRef]
- Camargo, S.J.; Wheeler, M.C.; Sobel, A.H. Diagnosis of the MJO modulation of tropical cyclogenesis using an empirical index. J. Atmos. Sci. 2009, 66, 3061–3074. [Google Scholar] [CrossRef]
- Wang, B.; Moon, J.Y. An anomalous genesis potential index for MJO modulation of tropical cyclones. J. Clim. 2017, 30, 4021–4035. [Google Scholar] [CrossRef]
- Haertel, P. Kelvin/Rossby wave partition of Madden-Julian oscillation circulations. Climate 2020, 9, 2. [Google Scholar] [CrossRef]
- Nakazawa, T. Tropical super clusters within intraseasonal variations over the Western Pacific. J. Meteorol. Soc. Jpn. Ser. II 1988, 66, 823–839. [Google Scholar] [CrossRef]
- Haertel, P.T.; Kiladis, G.N. Dynamics of 2-day equatorial waves. J. Atmos. Sci. 2004, 61, 2707–2721. [Google Scholar] [CrossRef]
- Kiladis, G.N.; Wheeler, M.C.; Haertel, P.T.; Straub, K.H.; Roundy, P.E. Convectively coupled equatorial waves. Rev. Geophys. 2009, 47, RG2003. [Google Scholar] [CrossRef]
- Xie, P.; Janowiak, J.E.; Arkin, P.A.; Adler, R.; Gruber, A.; Ferraro, R.; Huffman, G.J.; Curtis, S. GPCP pentad precipitation analyses: An experimental dataset based on gauge observations and satellite estimates. J. Clim. 2003, 16, 2197–2214. [Google Scholar] [CrossRef]
- Adler, R.F.; Sapiano, M.R.; Huffman, G.J.; Wang, J.J.; Gu, G.; Bolvin, D.; Chiu, L.; Schneider, U.; Becker, A.; Nelkin, E.; et al. The Global Precipitation Climatology Project (GPCP) monthly analysis (new version 2.3) and a review of 2017 global precipitation. Atmosphere 2018, 9, 138. [Google Scholar] [CrossRef] [PubMed]
- Durre, I.; Vose, R.S.; Wuertz, D.B. Overview of the integrated global radiosonde archive. J. Clim. 2006, 19, 53–68. [Google Scholar] [CrossRef]
- Durre, I.; Yin, X.; Vose, R.S.; Applequist, S.; Arnfield, J. Enhancing the data coverage in the integrated global radiosonde archive. J. Atmos. Ocean. Technol. 2018, 35, 1753–1770. [Google Scholar] [CrossRef]
- Hung, M.P.; Lin, J.L.; Wang, W.; Kim, D.; Shinoda, T.; Weaver, S.J. MJO and convectively coupled equatorial waves simulated by CMIP5 climate models. J. Clim. 2013, 26, 6185–6214. [Google Scholar] [CrossRef]
- Jiang, X.; Waliser, D.E.; Xavier, P.K.; Petch, J.; Klingaman, N.P.; Woolnough, S.J.; Guan, B.; Bellon, G.; Crueger, T.; DeMott, C.; et al. Vertical structure and physical processes of the Madden-Julian oscillation: Exploring key model physics in climate simulations. J. Geophys. Res. Atmos. 2015, 120, 4718–4748. [Google Scholar] [CrossRef]
- Knapp, K.R.; Diamond, H.J.; Kossin, J.P.; Kruk, M.C.; Schreck, C.J. NCDC International Best Track Archive for Climate Stewardship (IBTrACS) Project, Version 4; NOAA National Centers for Environmental Information: Hancock County, MI, USA, 2019. [Google Scholar] [CrossRef]
- Knapp, K.R.; Kruk, M.C.; Levinson, D.H.; Diamond, H.J.; Neumann, C.J. The international best track archive for climate stewardship (IBTrACS) unifying tropical cyclone data. Bull. Am. Meteorol. Soc. 2010, 91, 363–376. [Google Scholar] [CrossRef]
- Haertel, P. Prospects for Erratic and Intensifying Madden-Julian Oscillations. Climate 2020, 8, 24. [Google Scholar] [CrossRef]
- Emanuel, K.A.; David Neelin, J.; Bretherton, C.S. On large-scale circulations in convecting atmospheres. Q. J. R. Meteorol. Soc. 1994, 120, 1111–1143. [Google Scholar] [CrossRef]
- Wheeler, M.; Kiladis, G.N. Convectively coupled equatorial waves: Analysis of clouds and temperature in the wavenumber–frequency domain. J. Atmos. Sci. 1999, 56, 374–399. [Google Scholar] [CrossRef]
- Haertel, P.T.; Kiladis, G.N.; Denno, A.; Rickenbach, T.M. Vertical-mode decompositions of 2-day waves and the Madden–Julian oscillation. J. Atmos. Sci. 2008, 72, 813–833. [Google Scholar] [CrossRef]
- Fulton, S.R.; Schubert, W.H. Vertical normal mode transforms: Theory and application. Mon. Weather Rev. 1985, 113, 647–658. [Google Scholar] [CrossRef]
- Matsuno, T. Quasi-geostrophic motions in the equatorial area. J. Meteorol. Soc. Jpn. Ser. II 1966, 44, 25–43. [Google Scholar] [CrossRef]
- Gill, A. Some simple solutions for heat-induced tropical circulation. Q. J. R. Meteorol. Soc. 1980, 106, 447–462. [Google Scholar] [CrossRef]
- Haertel, P. Kelvin and Rossby Wave Contributions to the Mechanisms of the Madden–Julian Oscillation. Geosciences 2022, 12, 314. [Google Scholar] [CrossRef]
- Lin, X.; Johnson, R.H. Heating, moistening, and rainfall over the Western Pacific warm pool during TOGA COARE. J. Atmos. Sci. 1996, 53, 3367–3383. [Google Scholar] [CrossRef]
- Sobel, A.; Maloney, E. Moisture modes and the eastward propagation of the MJO. J. Atmos. Sci. 2013, 70, 187–192. [Google Scholar] [CrossRef]
- Adames, Á.F.; Wallace, J.M. Three-dimensional structure and evolution of the moisture field in the MJO. J. Atmos. Sci. 2015, 72, 3733–3754. [Google Scholar] [CrossRef]
- Haertel, P. Sensitivity of the Madden Julian Oscillation to Ocean Warming in a Lagrangian Atmospheric Model. Climate 2018, 6, 45. [Google Scholar] [CrossRef]
- Maloney, E.D.; Adames, Á.F.; Bui, H.X. Madden–Julian oscillation changes under anthropogenic warming. Nat. Clim. Chang. 2019, 9, 26–33. [Google Scholar] [CrossRef]
- Fuchs, Ž.; Raymond, D.J. A simple model of intraseasonal oscillations. J. Adv. Model. Earth Syst. 2017, 9, 1195–1211. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haertel, P. The Relationship between Madden–Julian Oscillation Moist Convective Circulations and Tropical Cyclone Genesis. Climate 2023, 11, 134. https://doi.org/10.3390/cli11070134
Haertel P. The Relationship between Madden–Julian Oscillation Moist Convective Circulations and Tropical Cyclone Genesis. Climate. 2023; 11(7):134. https://doi.org/10.3390/cli11070134
Chicago/Turabian StyleHaertel, Patrick. 2023. "The Relationship between Madden–Julian Oscillation Moist Convective Circulations and Tropical Cyclone Genesis" Climate 11, no. 7: 134. https://doi.org/10.3390/cli11070134
APA StyleHaertel, P. (2023). The Relationship between Madden–Julian Oscillation Moist Convective Circulations and Tropical Cyclone Genesis. Climate, 11(7), 134. https://doi.org/10.3390/cli11070134





