Conceptual Neighborhood Graphs of Topological Relations in
Abstract
1. Introduction
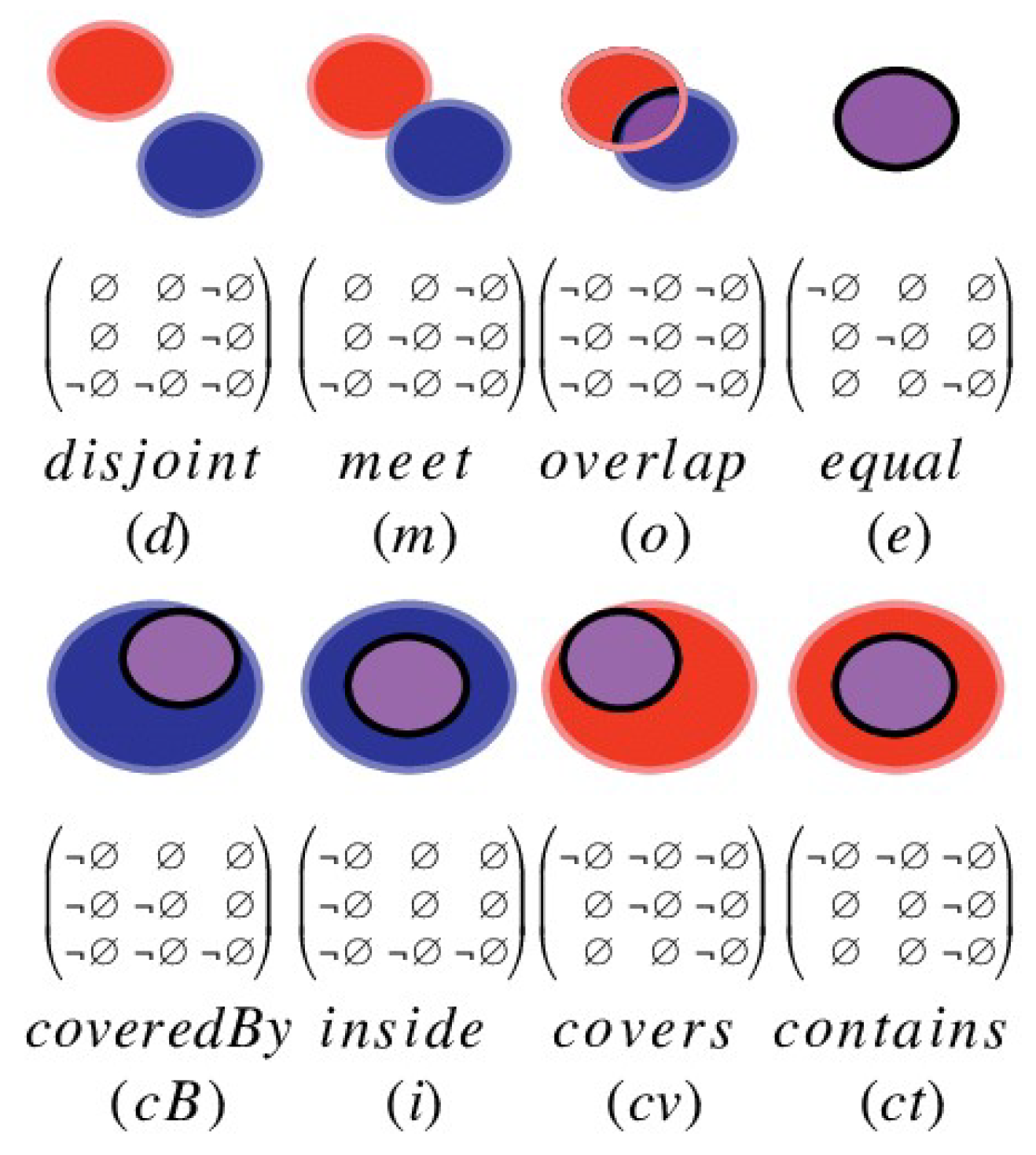
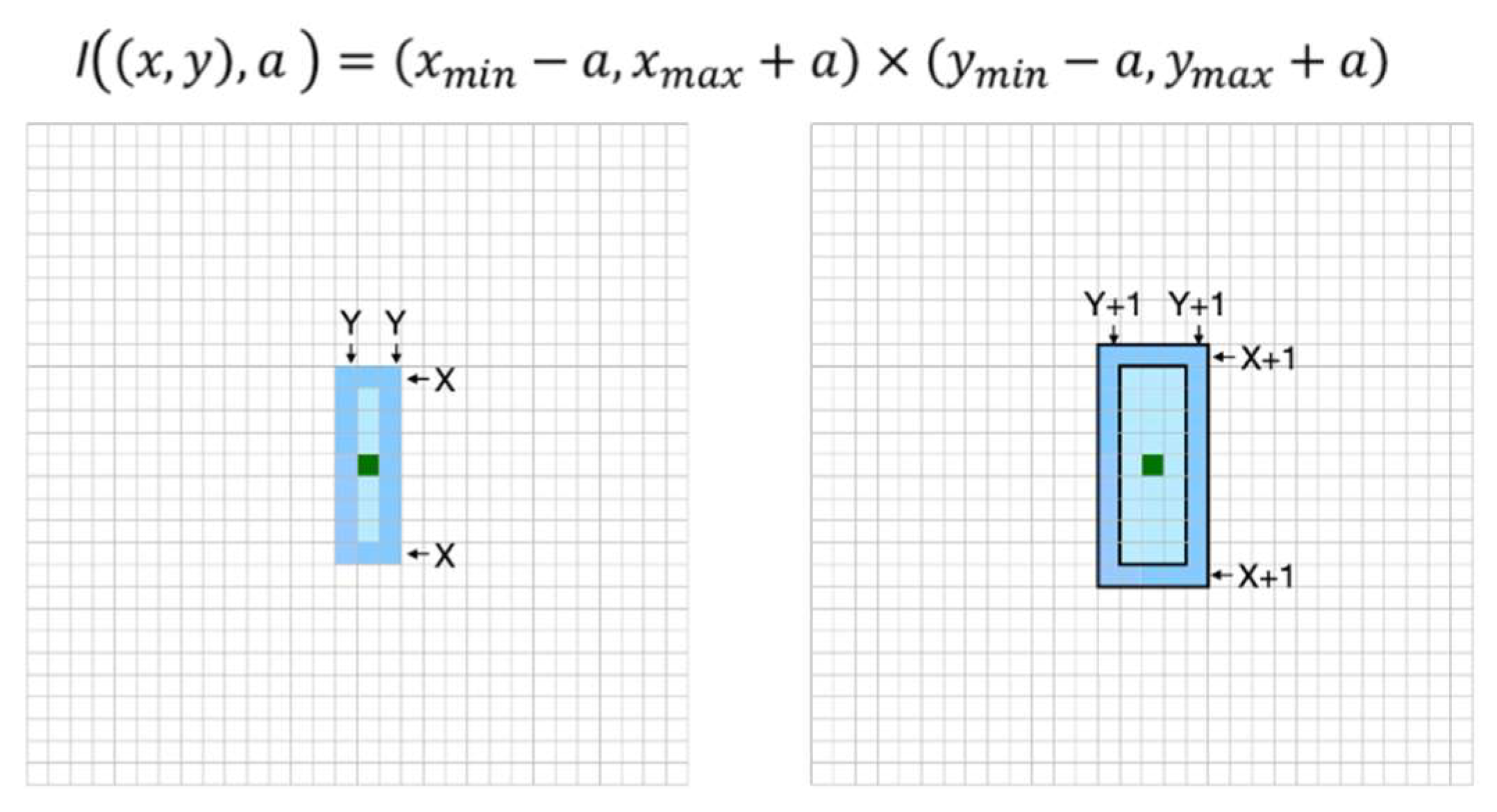
2. Region–Region Relations in Continuous and Discretized Spaces
- Vectorized and localized:
- Vectorized and globalized:
- Rasterized and localized:
- Rasterized and globalized:
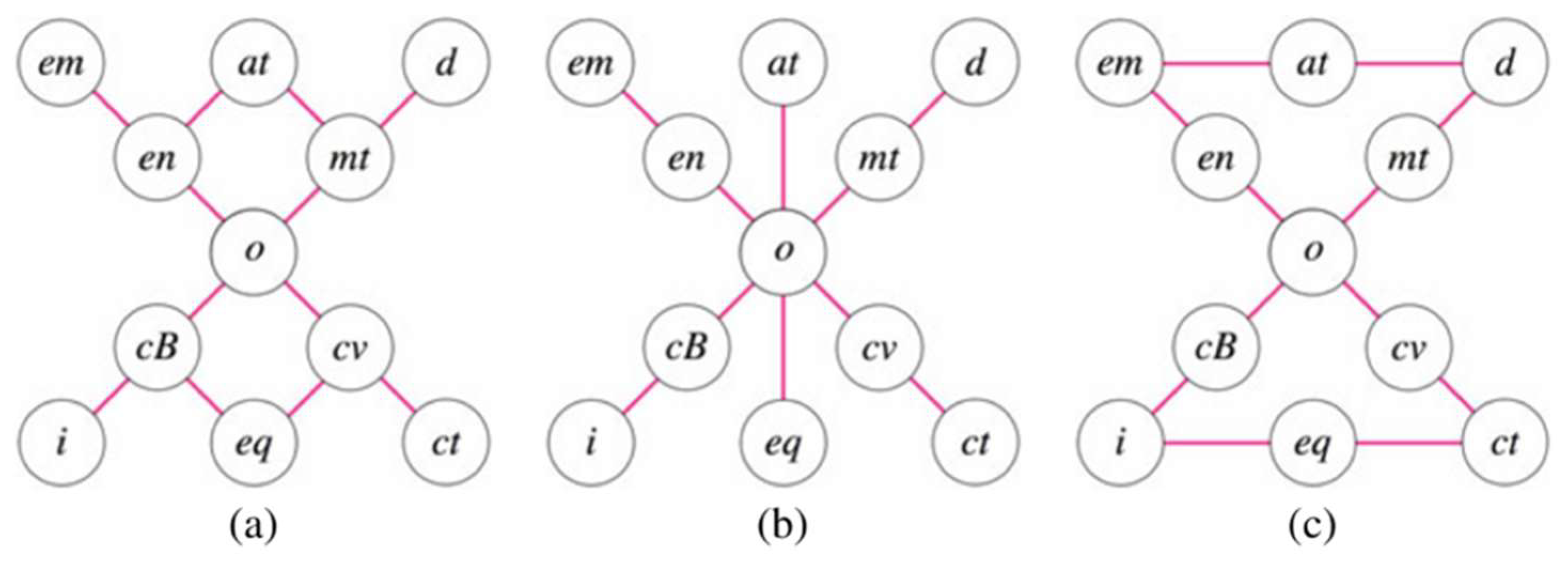
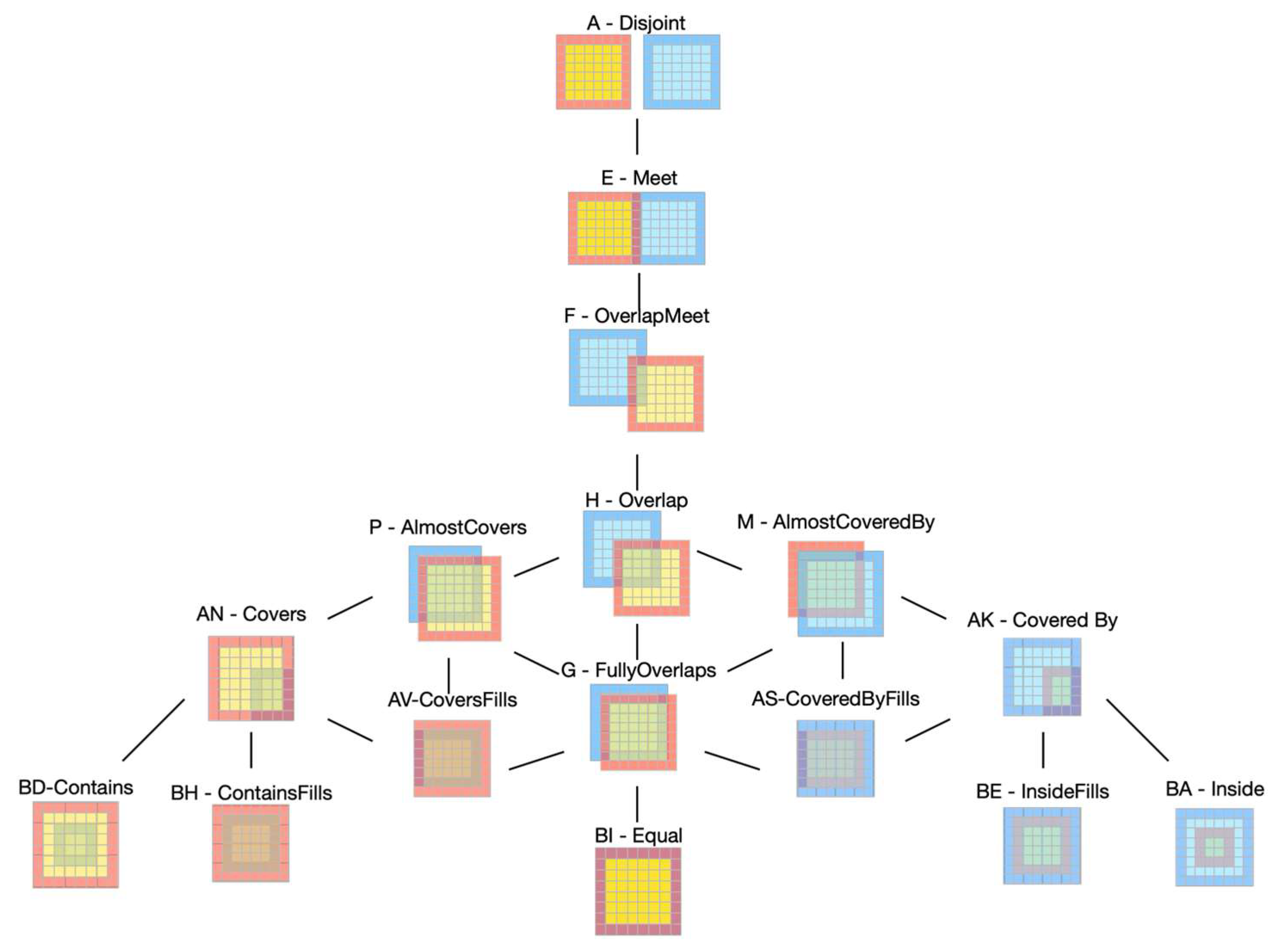
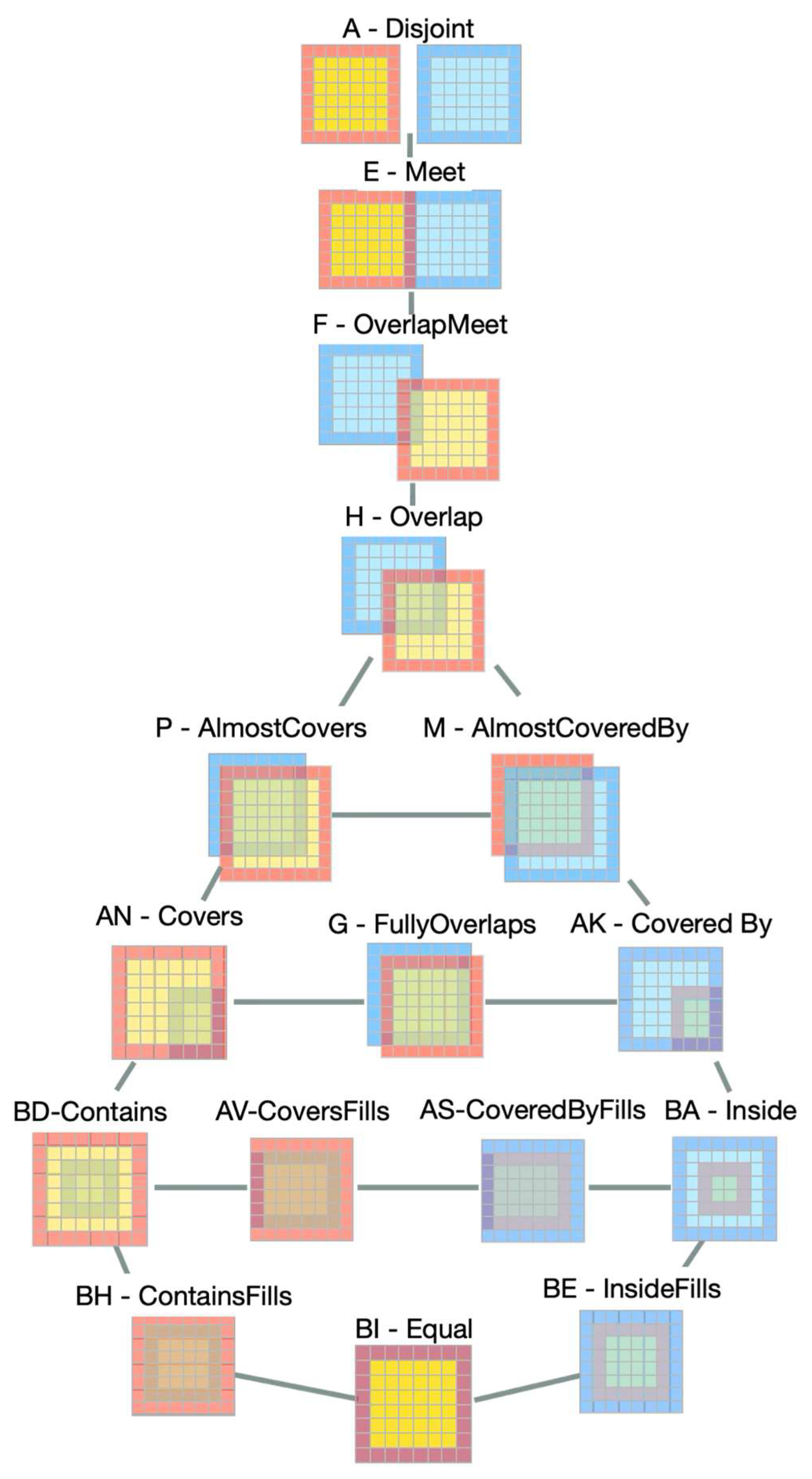
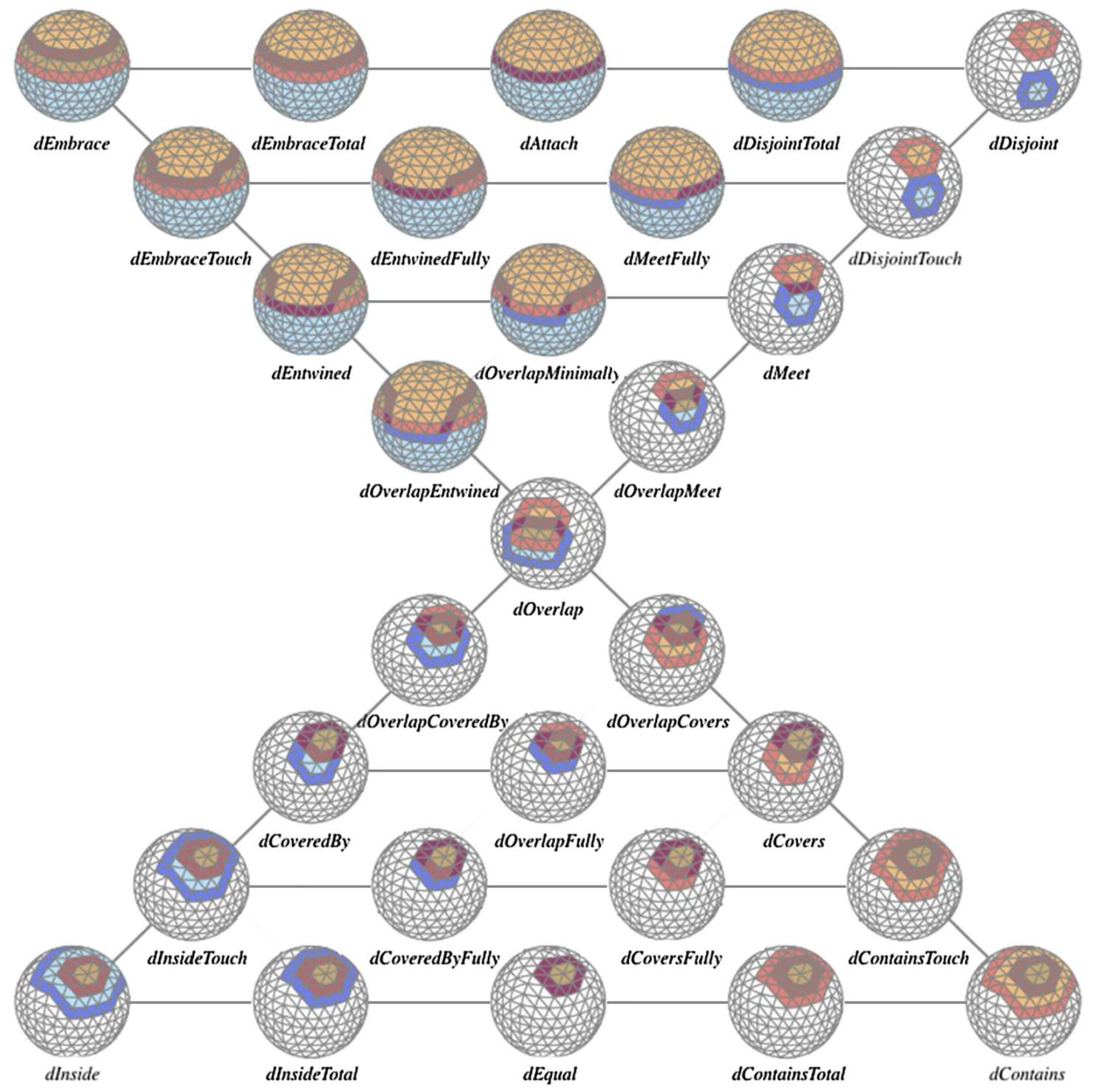
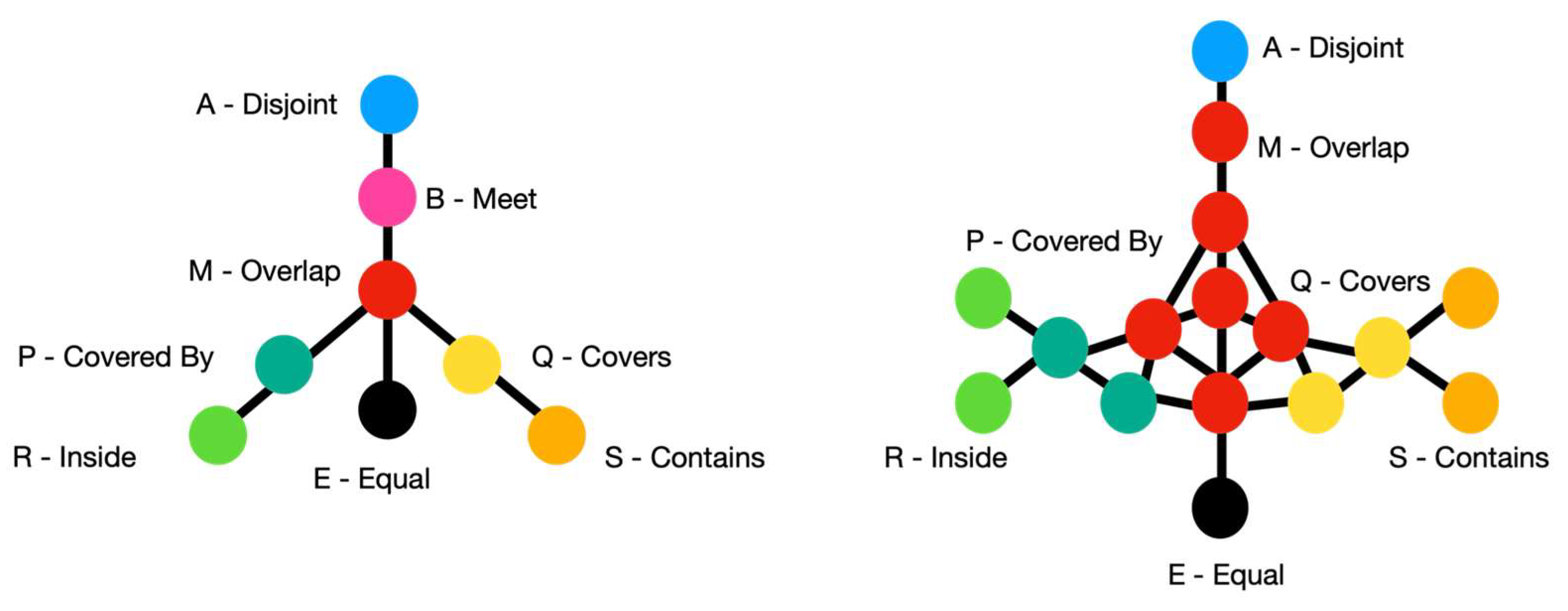
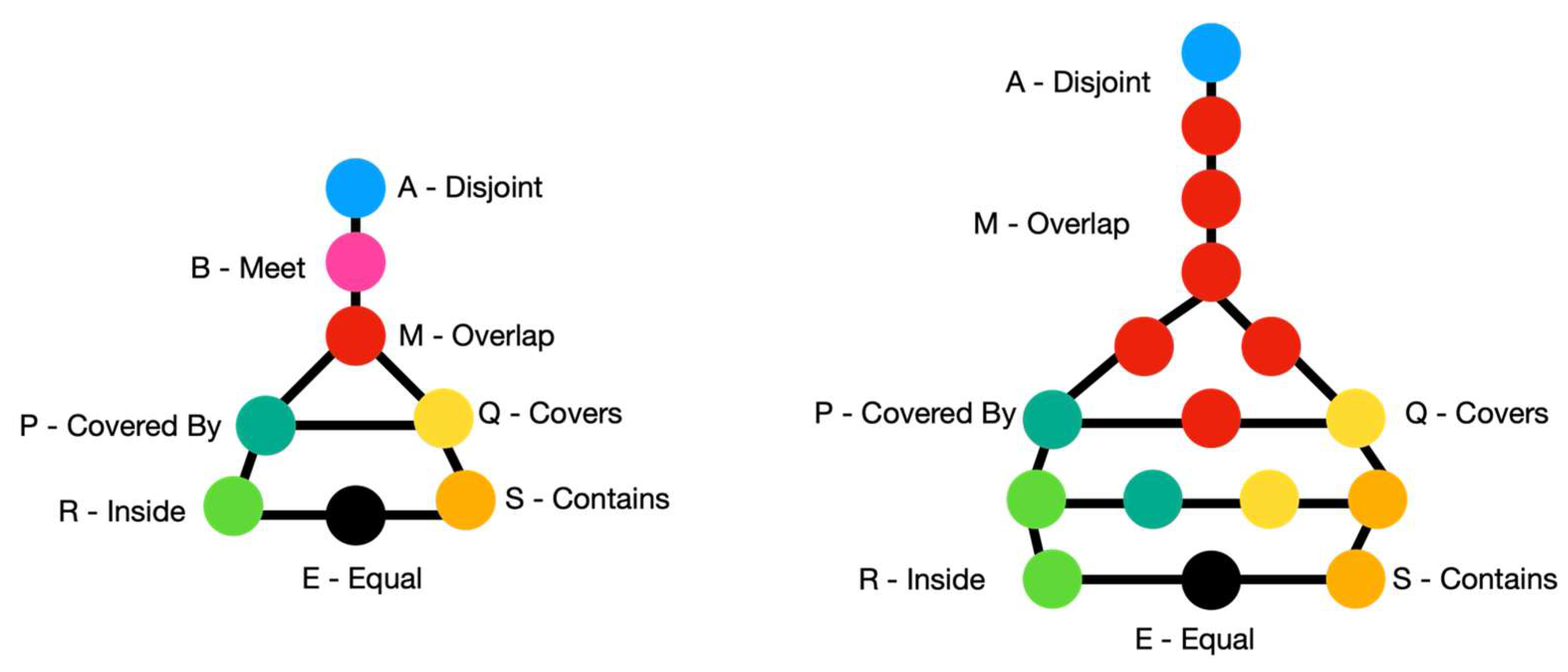
3. Conceptual Neighborhood Graphs
- Freksa [9] approached the problem through considering the ordering of the boundary points of the semi-intervals within a space based on the corresponding mathematical changes exhibited by the objects.
- Egenhofer and Al-Taha [13] approached the problem through studying deformational paths based on the various strategies, starting at all possible configurations and creating a union of the various deformation paths.
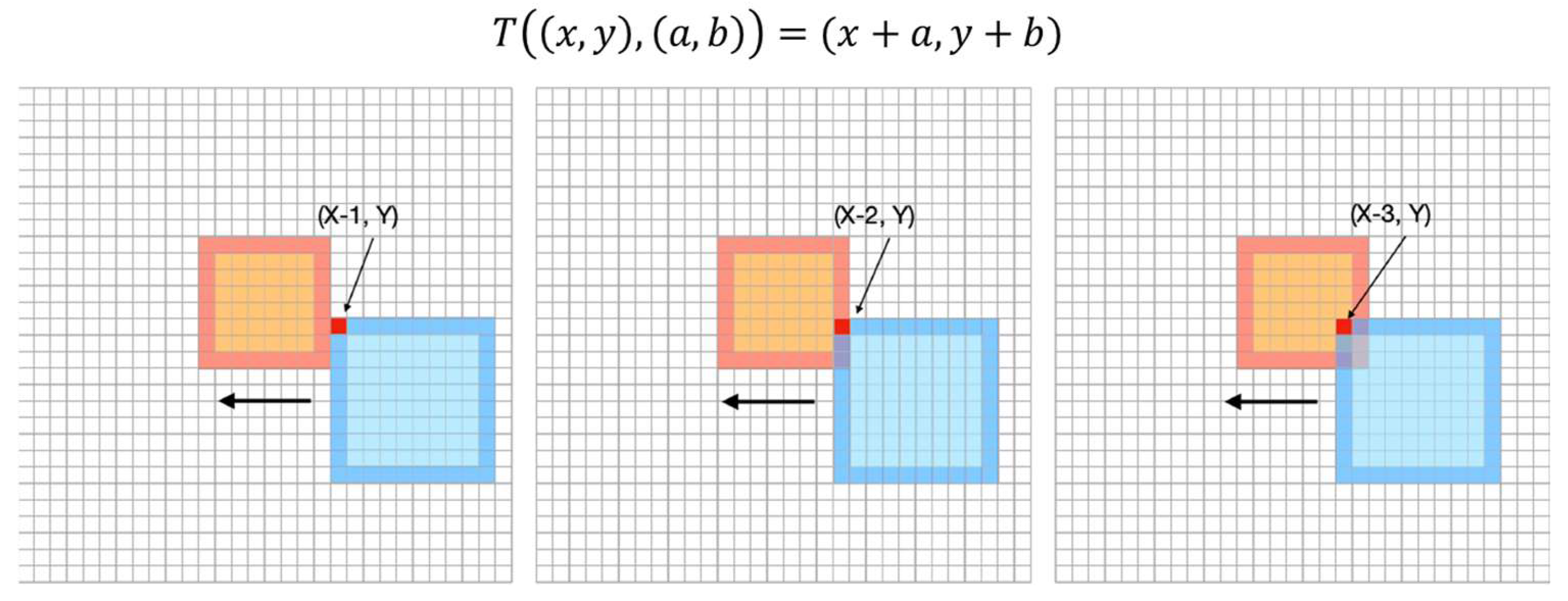
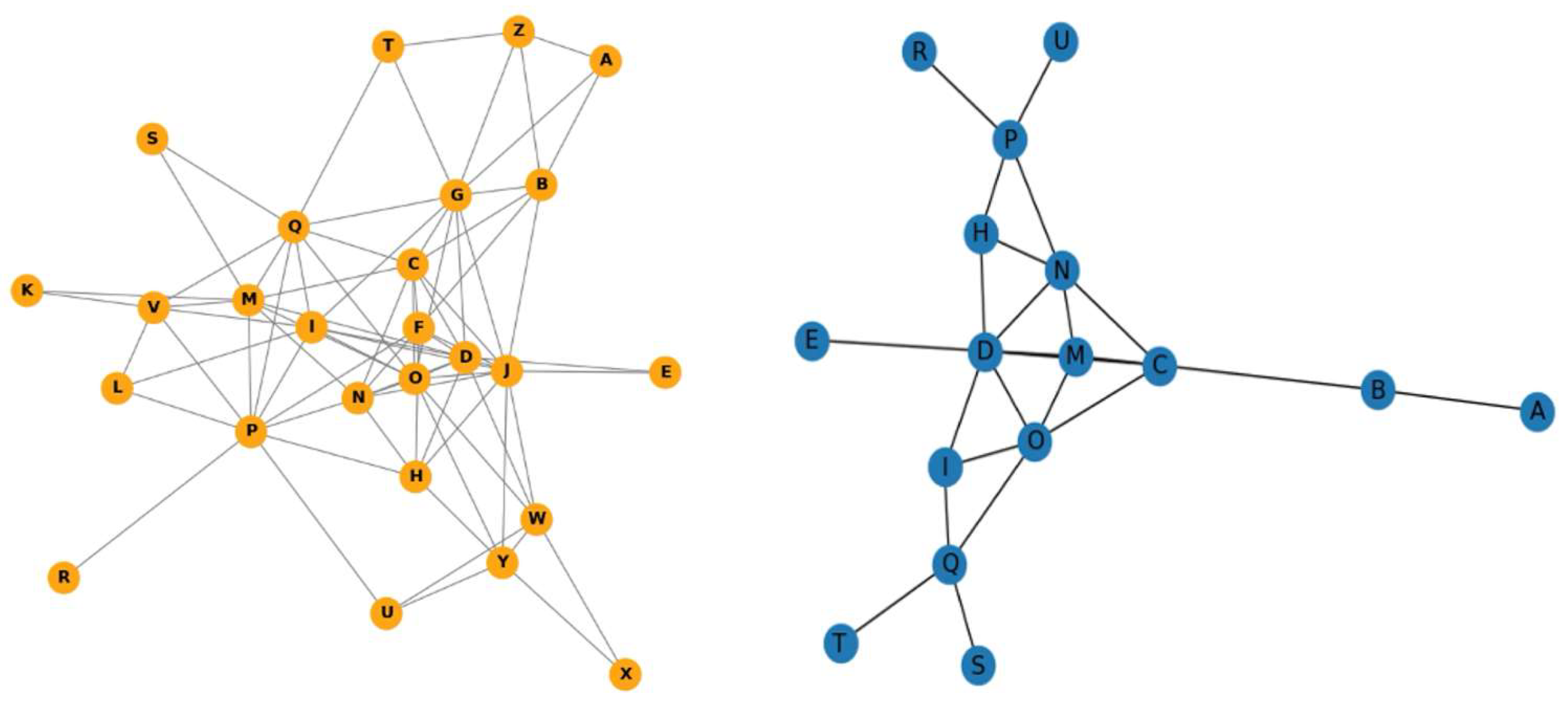
4. Simulation Methodology for Establishing Conceptual Neighborhood Graphs
5. Processing the Simulations into Conceptual Neighborhood Graphs
5.1. Translation Conceptual Neighborhood Graph
5.2. Isotropic Scaling Conceptual Neighborhood Graph
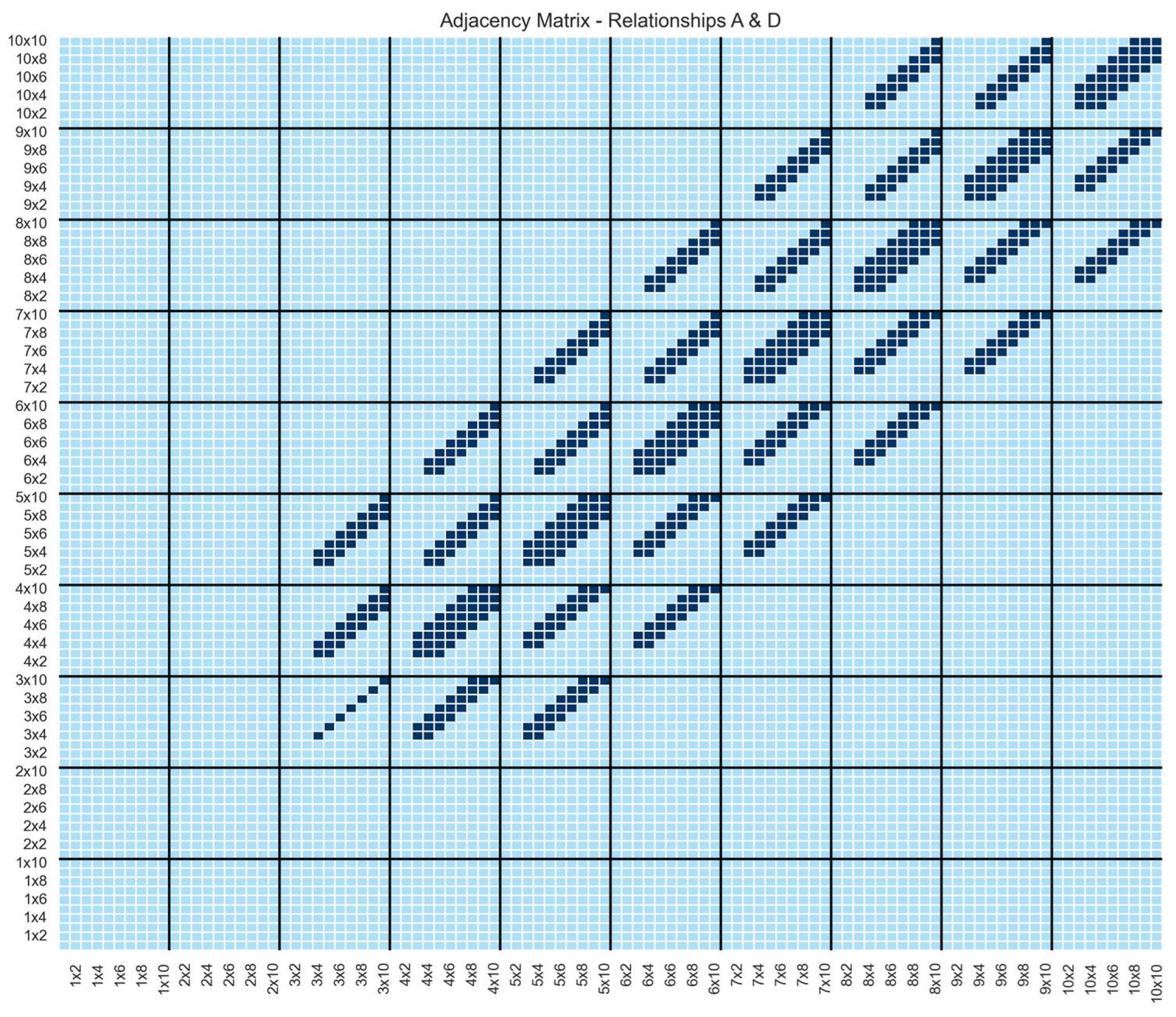
6. Litmus Test for Conceptual Neighborhoods from Simulation: The Hyperraster
7. Utility of the Outcome: Processing Pixelated Maps in Various Circumstances
8. Conclusions and Future Work
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Egenhofer, M.; Mark, D. Naïve geography. In Proceedings of the International Conference on Spatial Information Theory, Semmering, Austria, 21–23 September 1995; Frank, A., Kuhn, W., Eds.; Springer: Berlin, Germany, 1995; pp. 1–15. [Google Scholar]
- Landau, B.; Jackendoff, R. Whence and whither in spatial language and spatial cognition? Behav. Brain Sci. 1993, 16, 255–265. [Google Scholar] [CrossRef]
- Dube, M.; Egenhofer, M. Surrounds in partitions. In Proceedings of the 22nd ACM SIGSPATIAL International Conference on Advances in Geographic Information Systems, Dallas, TX, USA, 4–7 November 2014; Huang, Y., Schneider, M., Gertz, M., Krumm, J., Sankaranarayanan, J., Eds.; Association for Computing Machinery: Washington, DC, USA, 2014; pp. 233–242. [Google Scholar]
- Worboys, M.; Duckham, M. Qualitative-geometric surrounds relations between disjoint regions. Int. J. Geogr. Inf. Sci. 2021, 35, 1032–1063. [Google Scholar] [CrossRef]
- Tyler, A.; Evans, V. Applying cognitive linguistics to pedagogical grammar: The case of over. Cogn. Linguist. Second. Lang. Acquis. Foreign Lang. Teach. 2004, 1, 257–280. [Google Scholar]
- Aajami, R. A cognitive linguistic study of the English preposition ‘in’. J. Coll. Educ. Women 2019, 30, 37–49. [Google Scholar] [CrossRef]
- Aajami, R. A cognitive linguistic study of the English prepositions ‘above’, ‘on’, and ‘over’. J. Lang. Linguist. Stud. 2022, 18, 1. [Google Scholar]
- Yue, F.; Cao, S.; Huang, J. The semantic analysis of the preposition through from the perspective of cognitive linguistics. Theory Pract. Lang. Stud. 2022, 12, 400–406. [Google Scholar] [CrossRef]
- Freksa, C. Temporal reasoning based on semi-intervals. Artif. Intell. 1992, 54, 199–227. [Google Scholar] [CrossRef]
- Dube, M.; Egenhofer, M. An ordering of convex topological relations. In Proceedings of the Geographic Information Science: 7th International Conference, Columbus, OH, USA, 18–21 September 2012; Xiao, N., Kwan, M., Goodchild, M., Shekhar, S., Eds.; Springer: Berlin, Germany, 2012; pp. 72–86. [Google Scholar]
- Clementini, E.; Sharma, J.; Egenhofer, M. Modelling topological spatial relations: Strategies for query processing. Comput. Graph. 1994, 18, 815–822. [Google Scholar] [CrossRef]
- Egenhofer, M. SpatialSQL: A query and presentation language. IEEE Trans. Knowl. Data Eng. 1994, 6, 86–95. [Google Scholar] [CrossRef]
- Egenhofer, M.; Al-Taha, K. Reasoning about gradual changes of topological relationships. In Theories and Methods of Spatio-Temporal Reasoning in Geographic Space: International Conference GIS—From Space to Territory; Frank, A., Campari, I., Formentini, U., Eds.; Springer: Berlin, Germany, 1992; pp. 196–219. [Google Scholar]
- Cohn, A.; Bennett, B.; Gooday, J.; Gotts, N. Representing and reasoning with qualitative spatial relations about regions. In Spatial and Temporal Reasoning; Stock, O., Ed.; Springer: Berlin, Germany, 1997; pp. 97–134. [Google Scholar]
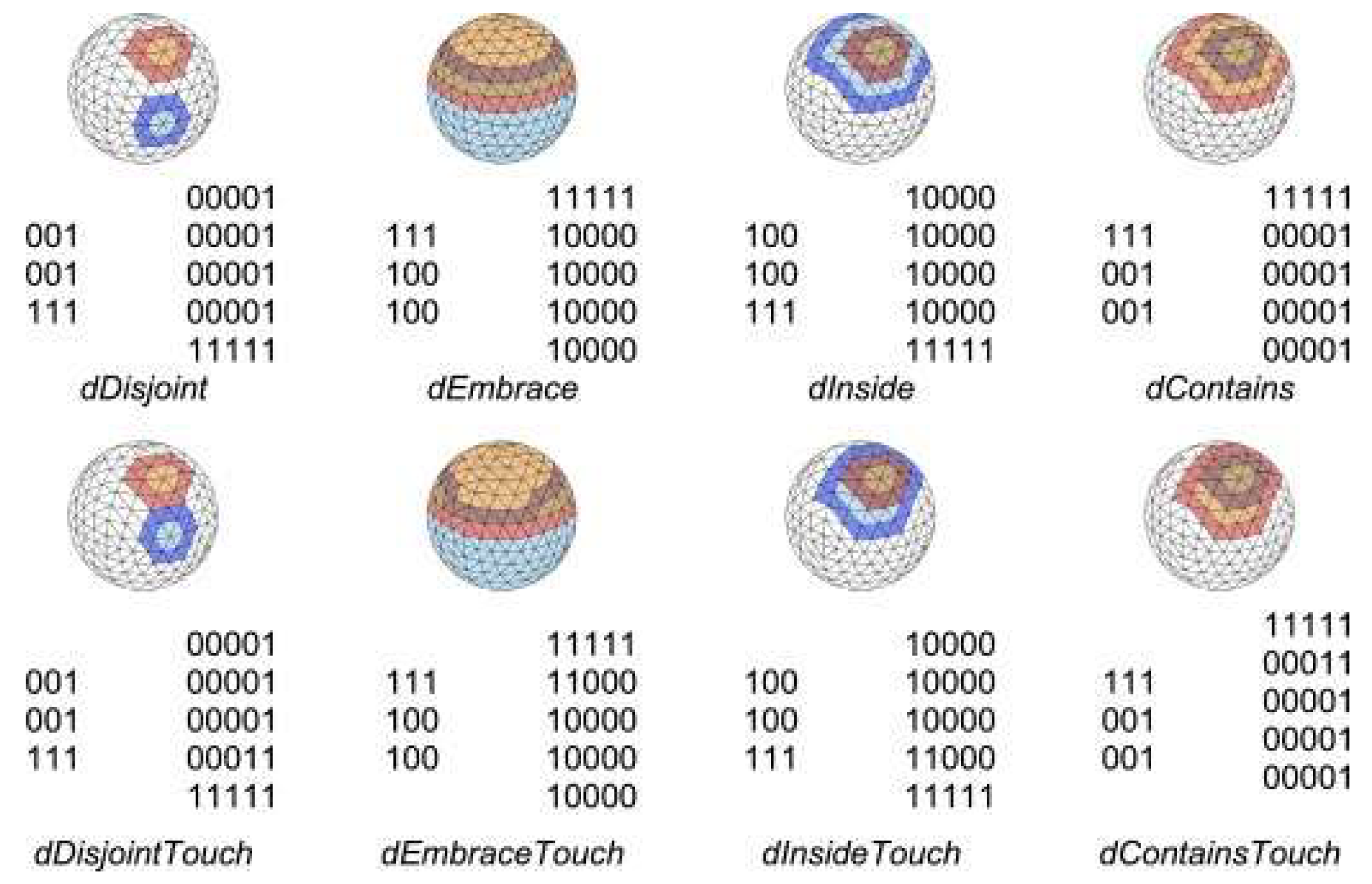
- Egenhofer, M. Spherical topological relations. J. Data Semant. III 2005, 1, 25–49. [Google Scholar]
- Egenhofer, M. The family of conceptual neighborhood graphs for region-region relations. In Proceedings of the Geographic Information Science: 6th International Conference, Zurich, Switzerland, 14–17 September 2010; Fabrikant, S., Reichenbacher, T., van Krevald, M., Schlieder, C., Eds.; Springer: Berling, Germany, 2010; pp. 42–55. [Google Scholar]
- Dube, M. Beyond homeomorphic deformations: Neighborhoods of topological changes. In Advancing Geographic Information Science: The Past and Next Twenty Years; Onsrud, H., Kuhn, W., Eds.; GSDI Press: Needham, MA, USA, 2016; pp. 137–151. [Google Scholar]
- Hall, B. Identification of Conceptual Neighborhoods and Topological Relations in . Master’s Thesis, University of Maine, Orono, ME, USA, 2024. [Google Scholar]
- Freundschuh, S.; Sharma, M. Spatial image schemata, locative terms, and geographic spaces in children’s narrative: Fostering spatial skills in children. Cartographica 1995, 32, 38–49. [Google Scholar] [CrossRef]
- Regier, T.; Xu, Y. The Sapir-Whorf hypothesis and inference under uncertainty. Wiley Interdiscip. Rev. Cogn. Sci. 2017, 8, 1440. [Google Scholar] [CrossRef] [PubMed]
- Xie, Y.; Wang, Z.; Mai, G.; Li, Y.; Jia, X.; Gao, S.; Wang, S. Geo-foundation models: Reality, gaps, and opportunities. In Proceedings of the 31st ACM International Conference on Advances in Geographic Information Systems, Hamburg, Germany, 13–16 November 2023; Damiani, M., Renz, M., Eldawy, A., Kroger, P., Nascimento, M., Eds.; ACM Press: Washington, DC, USA, 2023; pp. 1–4. [Google Scholar]
- Egenhofer, M.; Sharma, J. Topological relations between regions in and . In International Symposium on Spatial Databases; Abel, D., Ooi, B., Eds.; Springer: Berlin, Germany, 1993; pp. 316–336. [Google Scholar]
- Dube, M. An embedding Graph for 9-Intersection Topological Spatial Relations. Master’s Thesis, University of Maine, Orono, ME, USA, 2009. [Google Scholar]
- Dube, M.; Egenhofer, M. Binary relations on the digital sphere. Int. J. Approx. Reason. 2020, 116, 62–84. [Google Scholar]
- Dunstch, I. A tutorial on relation algebras and their application in spatial reasoning. In Proceedings of the International Conference on Spatial Information Theory, Stade, Germany, 25 August 1999. [Google Scholar]
- Winter, S. Topological relations between discrete regions. In International Symposium on Spatial Databases; Egenhofer, M., Herring, J., Eds.; Springer: Berlin, Germany, 1995; pp. 310–327. [Google Scholar]
- Kuipers, B. Modelling spatial knowledge. Cogn. Sci. 1978, 2, 129–153. [Google Scholar]
- Egenhofer, M.; Herring, J. Categorizing binary topological relations between regions, lines, and points in geographic databases. The 1990, 9, 76. [Google Scholar]
- Egenhofer, M.; Franzosa, R. Point-set topological spatial relations. Int. J. Geogr. Inf. Syst. 1991, 5, 161–174. [Google Scholar] [CrossRef]
- Kurata, Y. The 9+-intersection: A universal framework for modelling topological relations. In International Conference on Geographic Information Science; Cova, T., Miller, H., Beard, K., Frank, A., Goodchild, M., Eds.; Springer: Berlin, Germany, 2008; pp. 181–198. [Google Scholar]
- Clarke, B. A calculus of individuals based on connection. Notre Dame J. Form. Log. 1981, 22, 204–218. [Google Scholar]
- Randell, D.; Cui, Z.; Cohn, A. A spatial logic based on regions and connection. In Principles of Knowledge Representation and Reasoning; Nebel, B., Rich, C., Swartout, W., Eds.; Morgan Kaufmann Publishers: San Francisco, CA, USA, 1992; pp. 165–176. [Google Scholar]
- Sindoni, G.; Stell, J. The logic of discrete qualitative relations. In International Conference on Spatial Information Theory; Clementini, E., Donnelly, M., Yuan, M., Kray, C., Fogliaroni, P., Ballatore, A., Eds.; Schloss Dagstuhl-Leibniz-Zentrum fur Informatik: Waderm, Germany, 2017; pp. 1:1–1:15. [Google Scholar]
- Shariff, A.; Egenhofer, M.; Mark, D. Natural-language spatial relations between linear and areal objects: The topology and metric of English-language terms. Int. J. Geogr. Inf. Sci. 1998, 12, 215–245. [Google Scholar]
- Egenhofer, M.; Dube, M. Topological relations from metric refinements. In Proceedings of the 17th ACM SIGSPATIAL International Conference on Advances in Geographic Information Systems, Seattle, WA, USA, 4–6 November 2009; Wolfson, O., Agrawal, D., Liu, C., Eds.; ACM Press: Washington, DC, USA, 2009; pp. 158–167. [Google Scholar]
- Dube, M.; Barrett, J.; Egenhofer, M. From metric to topology: Determining relations in discrete space. In International Conference on Spatial Information Theory; Fabrikant, S., Raubal, M., Bertolotto, M., Davies, C., Freundschuh, S., Bell, S., Eds.; Springer: Berlin, Germany, 2015; pp. 151–171. [Google Scholar]
- Klippel, A.; Li, R.; Yang, J.; Hardisty, F.; Xu, S. The Egenhofer-Cohn hypothesis or topological relativity? In Cognitive and Linguistic Aspects of Geographic Space: New Perspectives on Geographic Information Research; Raubal, M., Mark, D., Frank, A., Eds.; Springer: Berlin, Germany, 2013; pp. 195–214. [Google Scholar]
- Egenhofer, M.; Mark, D. Modelling conceptual neighborhoods of topological line-region relations. Int. J. Geogr. Inf. Syst. 1995, 9, 555–565. [Google Scholar]
- Allen, J. Maintaining knowledge about temporal intervals. Commun. ACM 1983, 26, 832–843. [Google Scholar]
- Jiang, J.; Worboys, M.; Nittel, S. Qualitative change detection using sensor networks based on connectivity information. GeoInformatica 2011, 15, 305–328. [Google Scholar] [CrossRef]
- Agrawal, R.; Imielinski, T.; Swami, A. Mining association rules between sets of items in large databases. In Proceedings of the 1993 ACM SIGMOD International Conference on the Management of Data, Washington, DC, USA, 26–28 May 1993; Buneman, P., Jajodia, S., Eds.; ACM Press: Washington, DC, USA, 1993; pp. 207–216. [Google Scholar]
- Baumann, P.; Misev, D.; Merticariu, V.; Huu, B. Datacubes: Towards space/time analysis-ready data. In Service-Oriented Mapping: Changing Paradigm in Map Production and Geoinformation Management; Doellner, J., Jobst, M., Schmitz, P., Eds.; Springer: Berlin, Germany, 2018; pp. 269–299. [Google Scholar]
- Ferreira, K.; de Oliveira, A.; Monteiro, A.; de Almeida, D. Temporal GIS and Spatiotemporal Data Sources. Braz. J. Cartogr. 2016, 68, 1191–1202. [Google Scholar] [CrossRef]
- Dube, M.; Egenhofer, M.; Barrett, J.; Simpson, N. Beyond the digital Jordan curve: Unconstrained simple pixel-based raster relations. J. Comput. Lang. 2019, 54, 100906. [Google Scholar] [CrossRef]
- Vince, A.; Little, C. Discrete Jordan curve theorems. J. Comb. Theory Ser. B 1989, 47, 251–261. [Google Scholar] [CrossRef]








Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Published by MDPI on behalf of the International Society for Photogrammetry and Remote Sensing. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hall, B.P.; Dube, M.P.
Conceptual Neighborhood Graphs of Topological Relations in
Hall BP, Dube MP.
Conceptual Neighborhood Graphs of Topological Relations in
Hall, Brendan Patrick, and Matthew Paul Dube.
2025. "Conceptual Neighborhood Graphs of Topological Relations in
Hall, B. P., & Dube, M. P.
(2025). Conceptual Neighborhood Graphs of Topological Relations in