Geometric Phase Effects in Ultracold Chemical Reactions
Abstract
1. Introduction
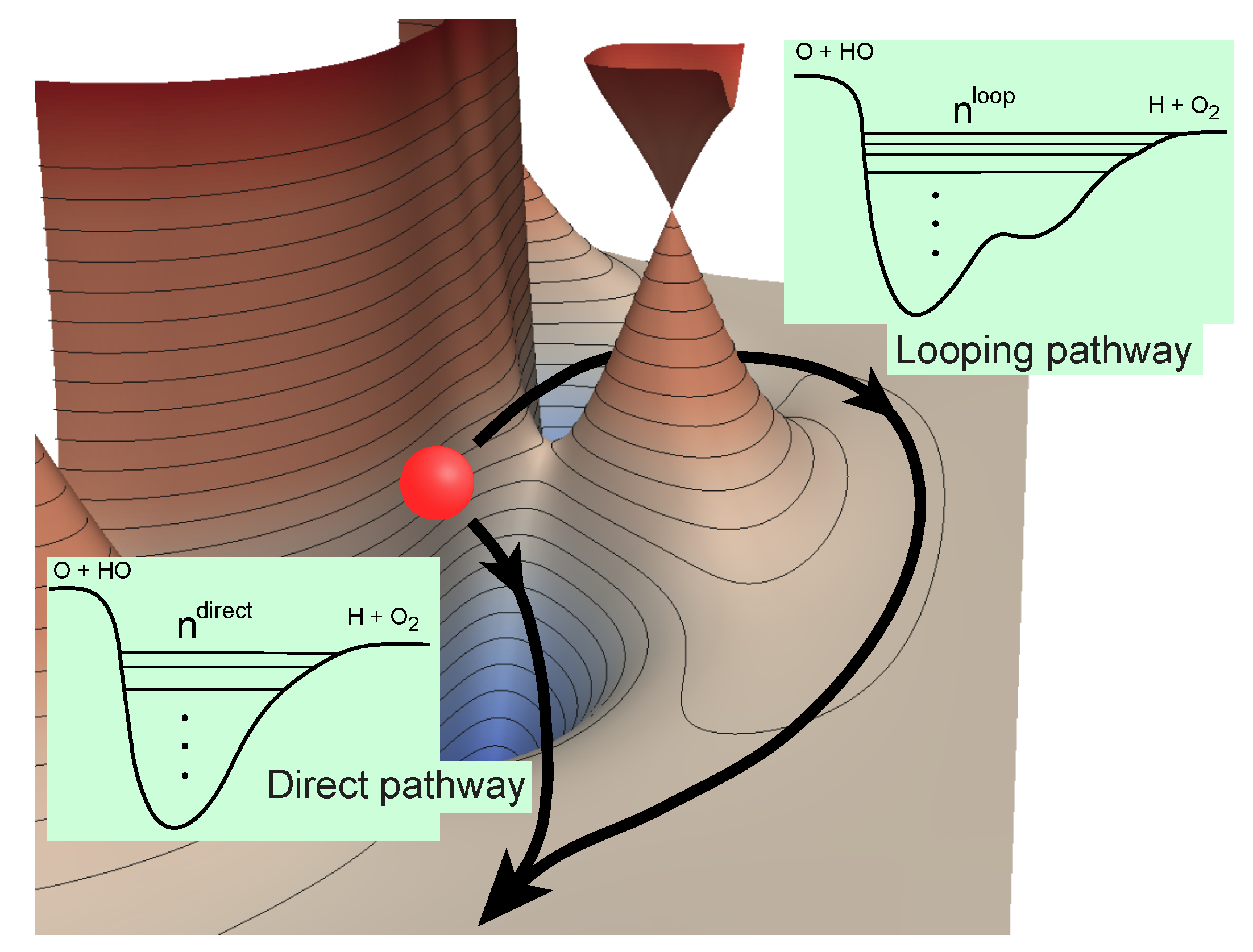
2. Mechanism of GP Effect in Ultracold Chemical Reactions
3. Quantum Scattering Method
4. Results
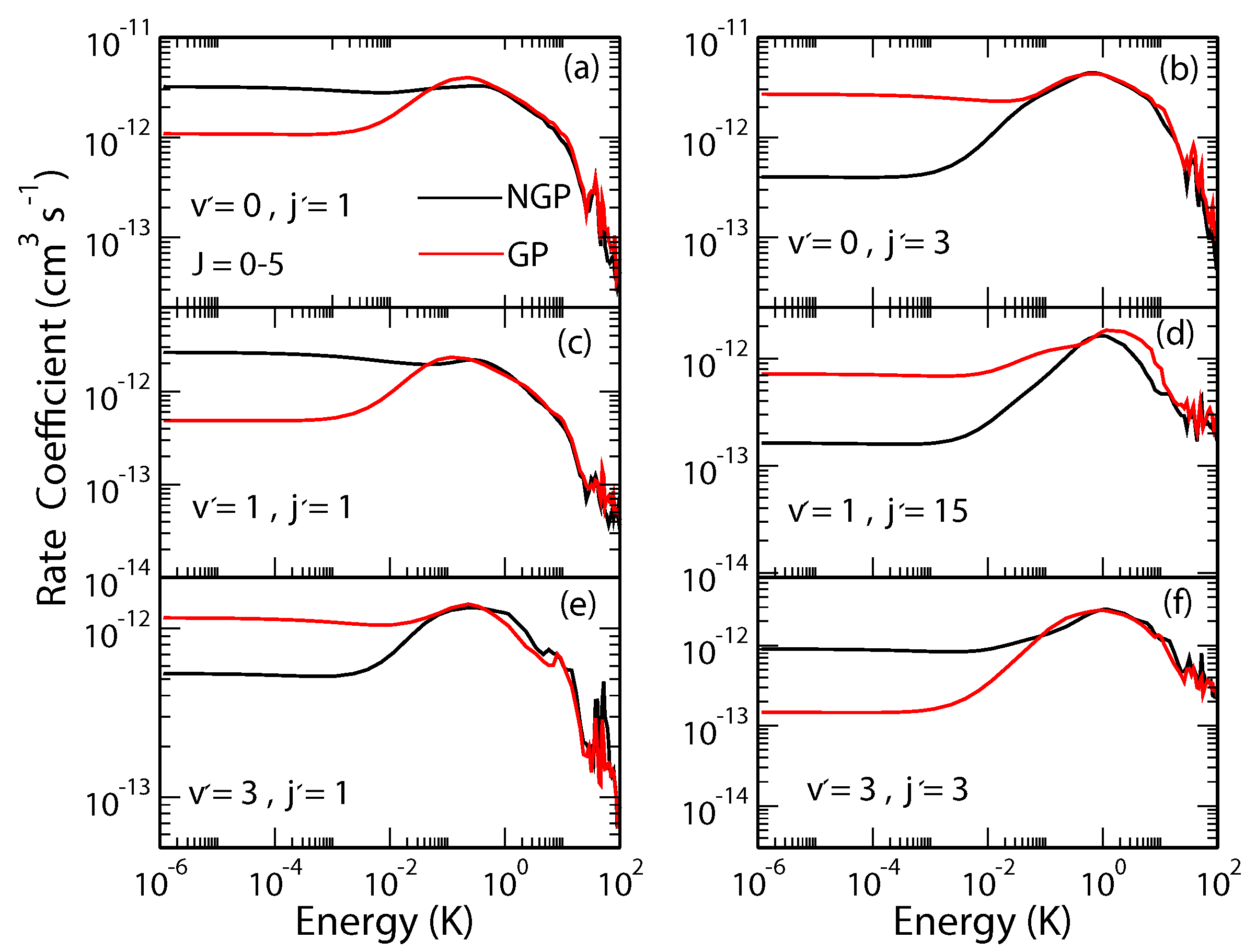
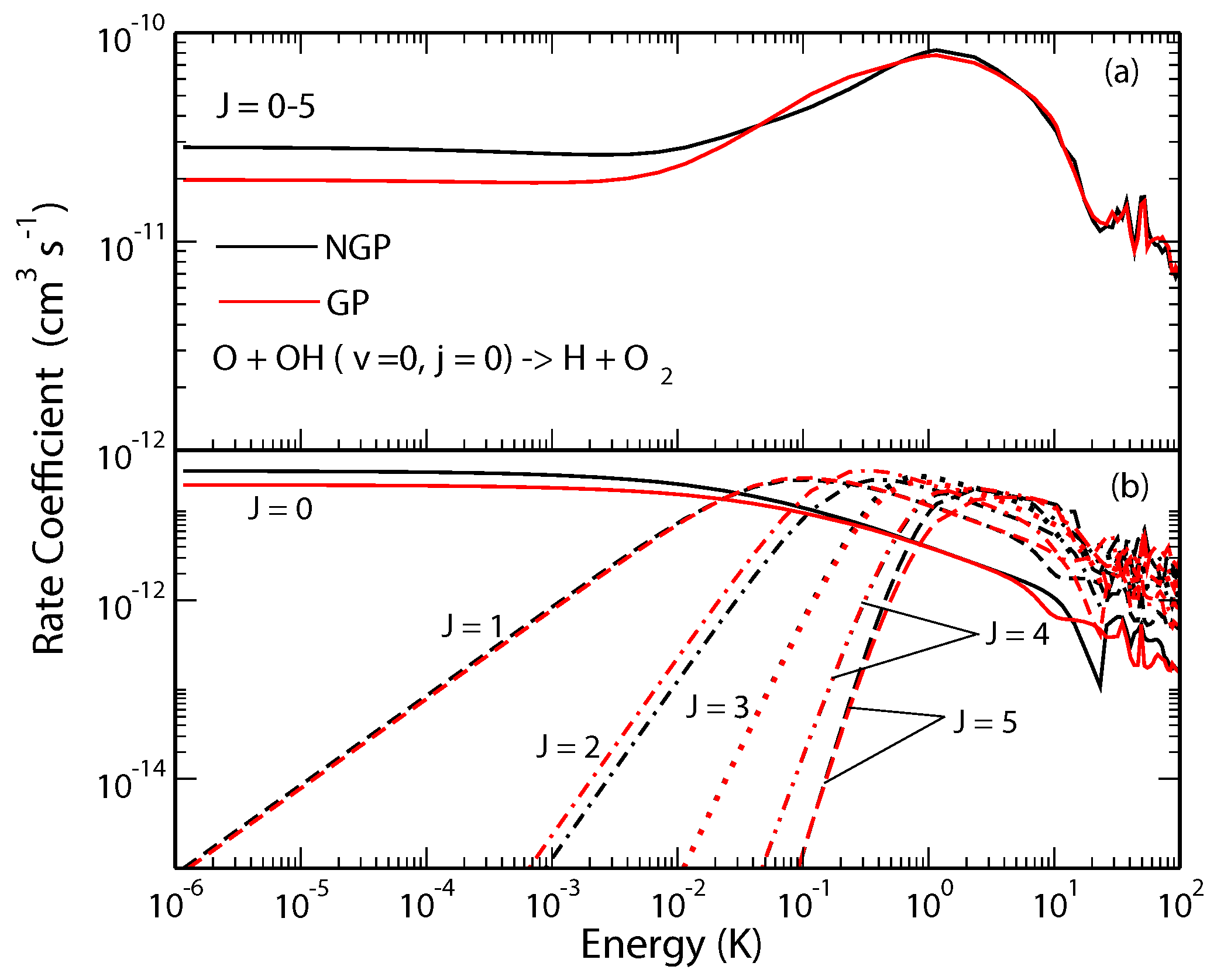
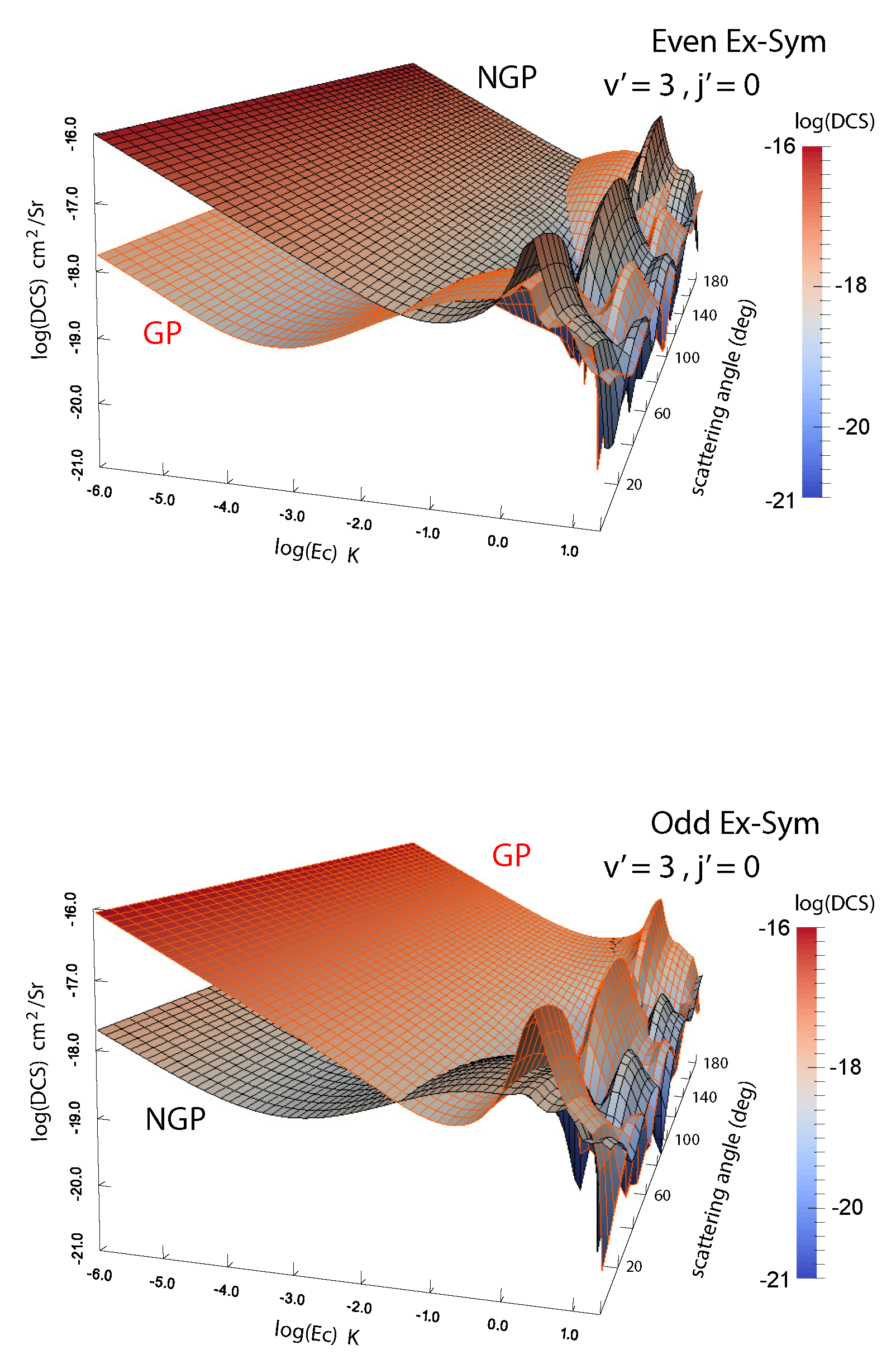
4.1. O + OH→H + O Reaction
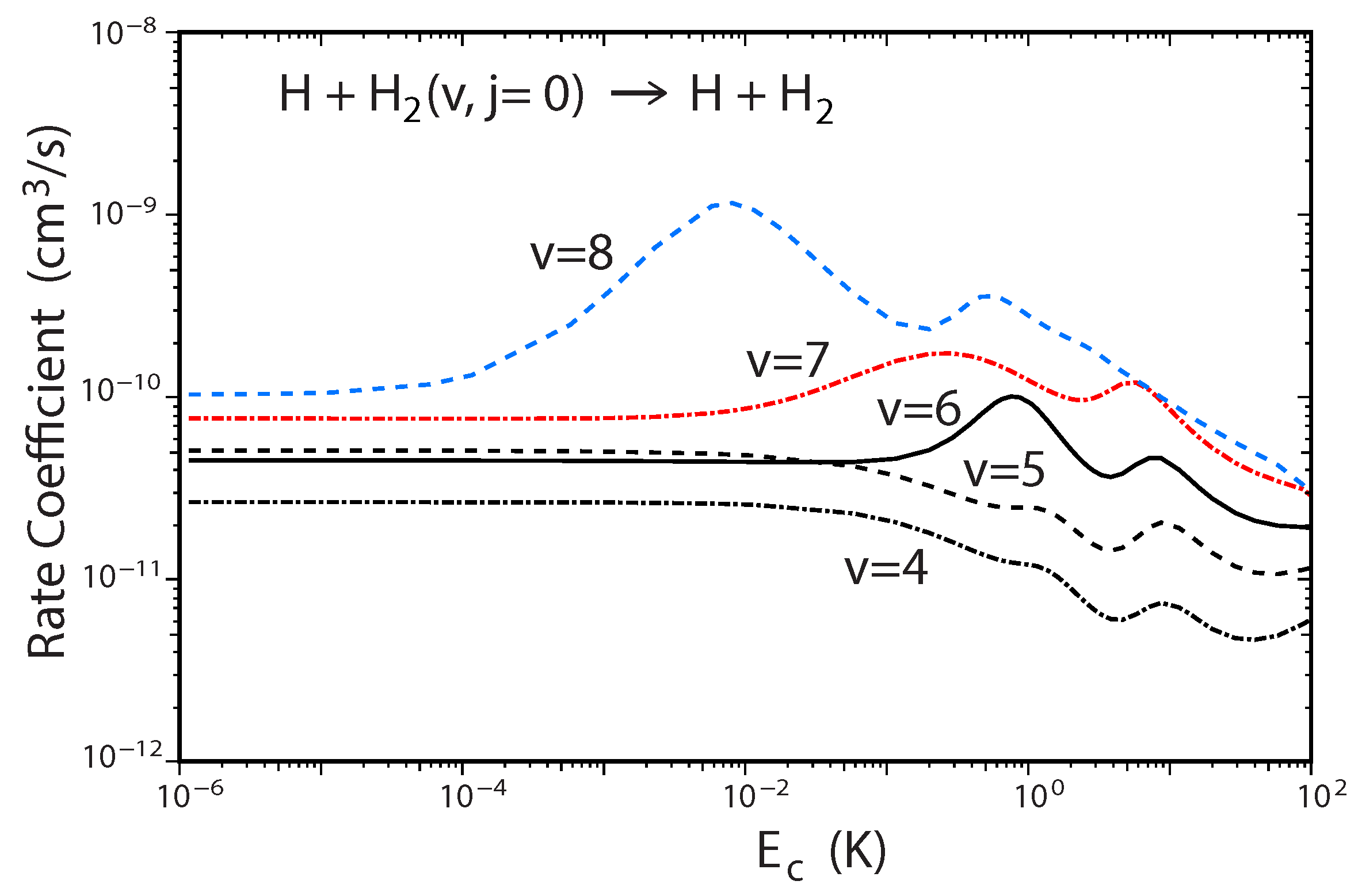
4.2. Hydrogen Exchange Reaction
5. Non-Adiabatic Effects
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Longuet-Higgins, H.C.; Öpik, U.; Pryce, M.H.L.; Sack, R.A. Studies of the Jahn-Teller effect. II. The dynamical problem. Proc. R. Soc. Lond. Ser. A 1958, 244, 1–16. [Google Scholar]
- Herzberg, G.; Longuet-Higgins, H.C. Intersection of potential energy surfaces in polyatomic molecules. Discuss. Faraday Soc. 1963, 35, 77–82. [Google Scholar] [CrossRef]
- Kendrick, B.K. The effects of pseudomagnetic fields in molecular spectra and scattering. Int. J. Quantum Chem. 1997, 64, 581–597. [Google Scholar] [CrossRef]
- Kendrick, B.K. Geometric phase effects in the vibrational spectrum of Na3. Phys. Rev. Lett. 1997, 79, 2431–2434. [Google Scholar] [CrossRef]
- Kendrick, B.K.; Pack, R.T. Geometric phase effects in the resonance spectrum, state-to-state transition probabilities and bound states of HO2. J. Chem. Phys. 1997, 106, 3519–3539. [Google Scholar] [CrossRef]
- Babikov, D.; Kendrick, B.K.; Zhang, P.; Morokuma, K. Cyclic-N3. II. Significant geometric phase effects in the vibrational spectra. J. Chem. Phys. 2005, 122, 044315. [Google Scholar] [CrossRef] [PubMed]
- Babikov, D.; Kendrick, B.K. The infrared spectrum of cyclic-N3: Theoretical prediction. J. Chem. Phys. 2010, 133, 174310. [Google Scholar] [CrossRef]
- Mead, C.A.; Truhlar, D.G. On the determination of Born-Oppenheimer nuclear motion wave functions including complications due to conical intersections and identical nuclei. J. Chem. Phys. 1979, 70, 2284–2296, 1983, 78, 6344E. [Google Scholar] [CrossRef]
- Mead, C.A. The molecular Aharonov-Bohm effect in bound states. Chem. Phys. 1980, 49, 23–32. [Google Scholar] [CrossRef]
- Mead, C.A. Superposition of reactive and nonreactive scattering amplitudes in the presence of a conical intersection. J. Chem. Phys. 1980, 72, 3839–3840. [Google Scholar] [CrossRef]
- Mead, C.A. The geometric phase in molecular systems. Rev. Mod. Phys. 1992, 64, 51–85. [Google Scholar] [CrossRef]
- Berry, M.V. Quantal phase factors accompanying adiabatic changes. Proc. R. Soc. Lond. Ser. A 1984, 392, 45–57. [Google Scholar] [CrossRef]
- Bohm, A. Quantum Mechanics: Foundations and Applications, 3rd ed.; Springer: New York, NY, USA, 2001; Chapters XII and XIII. [Google Scholar]
- Kendrick, B.K. Geometric phase effects in chemical reaction dynamics and molecular spectra. J. Phys. Chem. A 2003, 107, 6739–6756. [Google Scholar] [CrossRef]
- Kuppermann, A.; Wu, Y.S.M. The geometric phase effect shows up in chemical reactions. Chem. Phys. Lett. 1993, 205, 577–586, 1993, 213, 636E. [Google Scholar] [CrossRef]
- Wu, Y.S.M.; Kuppermann, A. The importance of the geometric phase effect for the H + D2→ HD + D reaction. Chem. Phys. Lett. 1995, 235, 105–110. [Google Scholar] [CrossRef]
- Kuppermann, A.; Wu, Y.S.M. The quantitative prediction and lifetime of a pronounced reactive scattering resonance. Chem. Phys. Lett. 1995, 241, 229–240. [Google Scholar] [CrossRef]
- Kendrick, B.K. Quantum reactive scattering calculations for the H + D2→HD + D reaction. J. Chem. Phys. 2001, 114, 8796–8819. [Google Scholar] [CrossRef]
- Kendrick, B.; Pack, R.T. Geometric phase effects in H + O2 scattering. I. Surface function solutions in the presence of a conical intersection. J. Chem. Phys. 1996, 104, 7475–7501. [Google Scholar] [CrossRef]
- Kendrick, B.; Pack, R.T. Geometric phase effects in H + O2 scattering. II. Recombination resonances and state-to-state transition probabilities at thermal energies. J. Chem. Phys. 1996, 104, 7502–7514. [Google Scholar] [CrossRef]
- Kendrick, B.K. Geometric phase effects in the H + D2→HD + D reaction. J. Chem. Phys. 2000, 112, 5679–5704, 2001, 114, 4335E. [Google Scholar] [CrossRef]
- Kendrick, B.K. Quantum reactive scattering calculations for the D + H2→HD + H reaction. J. Chem. Phys. 2003, 118, 10502–10522. [Google Scholar] [CrossRef]
- Juanes-Marcos, J.C.; Althorpe, S.C. Geometric phase effects in the H + H2 reaction: Quantum wave packet calculations of integral and differential cross sections. J. Chem. Phys. 2005, 122, 204324. [Google Scholar] [CrossRef] [PubMed]
- Juanes-Marcos, J.C.; Althorpe, S.C.; Wrede, E. Theoretical study of geometric phase effects in the hydrogen-exchange reaction. Science 2005, 309, 1227–1230. [Google Scholar] [CrossRef] [PubMed]
- Althorpe, S.C. General explanations of geometric phase effects in reactive systems: Unwinding the nuclear wave function using simple topology. J. Chem. Phys. 2006, 124, 084105. [Google Scholar] [CrossRef] [PubMed]
- Althorpe, S.C.; Stecher, T.; Bouakline, F. Effect of the geometric phase on nuclear dynamics at a conical intersection: Extension of a recent topological approach from one to two coupled surfaces. J. Chem. Phys. 2008, 129, 214117. [Google Scholar] [CrossRef] [PubMed]
- Von Busch, H.; Eckel, V.; Dev, H.; Kasahara, S.; Wang, J.; Demtröder, W.; Sebald, P.; Meyer, W. Unambiguous Proof for Berry’s Phase in the Sodium Trimer: Analysis of the Transition A2E′′←X2E′. Phys. Rev. Lett. 1998, 81, 4584–4587. [Google Scholar] [CrossRef]
- Keil, M.; Krämer, H.-G.; Kudell, A.; Baig, M.A.; Zhu, J.; Demtröder, W.; Meyer, W. Rovibrational structures of the pseudorotating lithium trimer 21Li3: Rotationally resolved spectroscopy and ab initio calculations of the A2E′′←X2E′ system. J. Chem. Phys. 2000, 113, 7414–7431. [Google Scholar] [CrossRef]
- Rohlfing, E.A.; Valentini, J.J. Laser-excited fluorescence spectroscopy of the jet-cooled copper trimer. Chem. Phys. Lett. 1986, 126, 113–118. [Google Scholar] [CrossRef]
- Kliner, D.A.V.; Adleman, D.E.; Zare, R.N. Comparison of experimental and theoretical integral cross sections for D + H2(v = 1,j = 1)→ HD(v′ = 1,j′) + H. J. Chem. Phys. 1991, 95, 1648–1662. [Google Scholar] [CrossRef]
- Adleman, D.E.; Shafer, N.E.; Kliner, D.A.V.; Zare, R.N. Measurement of relative state-to-state rate constants for the reaction D + H2(v,j)→ HD(v′,j′) + H. J. Chem. Phys. 1992, 97, 7323–7341. [Google Scholar] [CrossRef]
- Kitsopoulos, T.N.; Buntine, M.A.; Baldwind, D.P.; Zare, R.N.; Chandler, D.W. Reaction product imaging: The H + D2 reaction. Science 1993, 260, 1605–1610. [Google Scholar] [CrossRef] [PubMed]
- Jankunas, J.; Sneha, M.; Zare, R.N.; Bouakline, F.; Althorpe, S.C. Hunt for geometric phase effects in H + HD→ H + HD(v′,j′). J. Chem. Phys. 2013, 139, 144316. [Google Scholar] [CrossRef] [PubMed]
- Jankunas, J.; Sneha, M.; Zare, R.N.; Bouakline, F.; Althorpe, S.C.; Herráez-Aguilar, D.; Aoiz, F.J. Is the simplest chemical reaction really so simple? Proc. Natl. Acad. Sci. USA 2014, 111, 15–20. [Google Scholar] [CrossRef] [PubMed]
- Sneha, M.; Gao, H.; Zare, R.N.; Jambrina, P.J.; Menéndez, M.; Aoiz, F.J. Multiple scattering mechanisms causing interference effects in the differential cross sections of H + D2→ HD(v′ = 4,j′) + D at 3.26 eV collision energy. J. Chem. Phys. 2016, 145, 024308. [Google Scholar] [CrossRef] [PubMed]
- Yuan, D.; Guan, Y.; Chen, W.; Zhao, H.; Yu, S.; Luo, C.; Tan, Y.; Xie, T.; Wang, X.; Sun, Z.; et al. Observation of the geometric phase effect in the H + HD→ H2 + D reaction. Science 2018, 362, 1289–1293. [Google Scholar] [CrossRef]
- Izmaylov, A.F.; Li, J.; Joubert-Doriol, L. Diabatic definition of geometric phase effects. J. Chem. Theory Comput. 2016, 12, 5278–5283. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Joubert-Doriol, L.; Izmaylov, A.F. Geometric phase effects in excited state dynamics through a conical intersection in large molecules: N-dimensional linear vibronic coupling model study. J. Chem. Phys. 2017, 147, 064106. [Google Scholar] [CrossRef]
- Ryabinkin, I.G.; Joubert-Doriol, L.; Izmaylov, A.F. Geometric phase effects in nonadiabatic dynamics near conical intersections. Acc. Chem. Res. 2017, 50, 1785–1793. [Google Scholar] [CrossRef]
- Xie, C.; Kendrick, B.K.; Yarkony, D.R.; Guo, H. Constructive and destructive interference in nonadiabatic tunneling via conical intersections. J. Chem. Theory Comput. 2017, 13, 1902–1910. [Google Scholar] [CrossRef]
- Xie, C.; Malbon, C.L.; Yarkony, D.R.; Guo, H. Dynamic mapping of conical intersection seams: A general method for incorporating the geometric phase in adiabatic dynamics in polyatomic systems. J. Chem. Phys. 2017, 147, 044109. [Google Scholar] [CrossRef]
- Levinson, N. On the uniqueness of the potential in a Schrödinger equation for a given asymptotic phase. Kgl. Danske Videnskab Selskab. Mat. Fys. Medd. 1949, 25, 9. [Google Scholar]
- Kendrick, B.K.; Hazra, J.; Balakrishnan, N. The geometric phase controls ultracold chemistry. Nat. Commun. 2015, 6, 7918. [Google Scholar] [CrossRef] [PubMed]
- Hazra, J.; Kendrick, B.K.; Balakrishnan, N. Importance of geometric phase effects in ultracold chemistry. J. Phys. Chem. A 2015, 119, 12291–12303. [Google Scholar] [CrossRef] [PubMed]
- Kendrick, B.K.; Hazra, J.; Balakrishnan, N. The geometric phase appears in the ultracold hydrogen exchange reaction. Phys. Rev. Lett. 2015, 115, 153201. [Google Scholar] [CrossRef] [PubMed]
- Kendrick, B.K.; Hazra, J.; Balakrishnan, N. Geometric phase effects in the ultracold D + HD↔D + HD, D + HD↔H + D2 reactions. New J. Phys. 2016, 18, 12320. [Google Scholar]
- Kendrick, B.K.; Hazra, J.; Balakrishnan, N. Geometric phase effects in ultracold H + H2 reaction. J. Chem. Phys. 2016, 145, 164303. [Google Scholar] [CrossRef] [PubMed]
- Hazra, J.; Kendrick, B.K.; Balakrishnan, N. Geometric phase effects in ultracold hydrogen exchange reaction. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 194004. [Google Scholar] [CrossRef]
- Croft, J.F.E.; Hazra, J.; Balakrishnan, N.; Kendrick, B.K. Symmetry and the geometric phase in ultracold hydrogen-exchange reactions. J. Chem. Phys. 2017, 147, 074302. [Google Scholar] [CrossRef]
- Pack, R.T.; Parker, G.A. Quantum reactive scattering in three dimensions using hyperspherical (APH) coordinates. Theory. J. Chem. Phys. 1987, 87, 3888–3921. [Google Scholar] [CrossRef]
- Kendrick, B.K.; Pack, R.T.; Walker, R.B.; Hayes, E.F. Hyperspherical surface functions for nonzero total angular momentum. I. Eckart singularities. J. Chem. Phys. 1999, 110, 6673–6693. [Google Scholar] [CrossRef]
- Balakrishnan, N.; Kharchenko, V.; Forrey, R.C.; Dalgarno, A. Complex scattering lengths in multi-channel atom-molecule collisions. Chem. Phys. Lett. 1997, 280, 5–9. [Google Scholar] [CrossRef]
- Kendrick, B.K. Non-adiabatic quantum reactive scattering in hyperspherical coordinates. J. Chem. Phys. 2018, 148, 044116. [Google Scholar] [CrossRef] [PubMed]
- Kendrick, B.K. Non-adiabatic quantum reactive scattering calculations for the ultracold hydrogen exchange reaction: H + H2(v = 4 − 8, j = 0) → H + H2(v′, j′). Chem. Phys. 2018, 515, 387–399. [Google Scholar] [CrossRef]
- Londoño, B.E.; Mahecha, J.E.; Luc-Koenig, E.; Crubellier, A. Shape resonances in ground-state diatomic molecules: General trends and the example of RbCs. Phys. Rev. A 2010, 81, 012510. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kendrick, B.K.; Balakrishnan, N. Geometric Phase Effects in Ultracold Chemical Reactions. Atoms 2019, 7, 65. https://doi.org/10.3390/atoms7030065
Kendrick BK, Balakrishnan N. Geometric Phase Effects in Ultracold Chemical Reactions. Atoms. 2019; 7(3):65. https://doi.org/10.3390/atoms7030065
Chicago/Turabian StyleKendrick, Brian K., and N. Balakrishnan. 2019. "Geometric Phase Effects in Ultracold Chemical Reactions" Atoms 7, no. 3: 65. https://doi.org/10.3390/atoms7030065
APA StyleKendrick, B. K., & Balakrishnan, N. (2019). Geometric Phase Effects in Ultracold Chemical Reactions. Atoms, 7(3), 65. https://doi.org/10.3390/atoms7030065




