Quadrupole Effects in the Photoionisation of Sodium 3s in the Vicinity of the Dipole Cooper Minimum
Abstract
1. Introduction
2. Theory
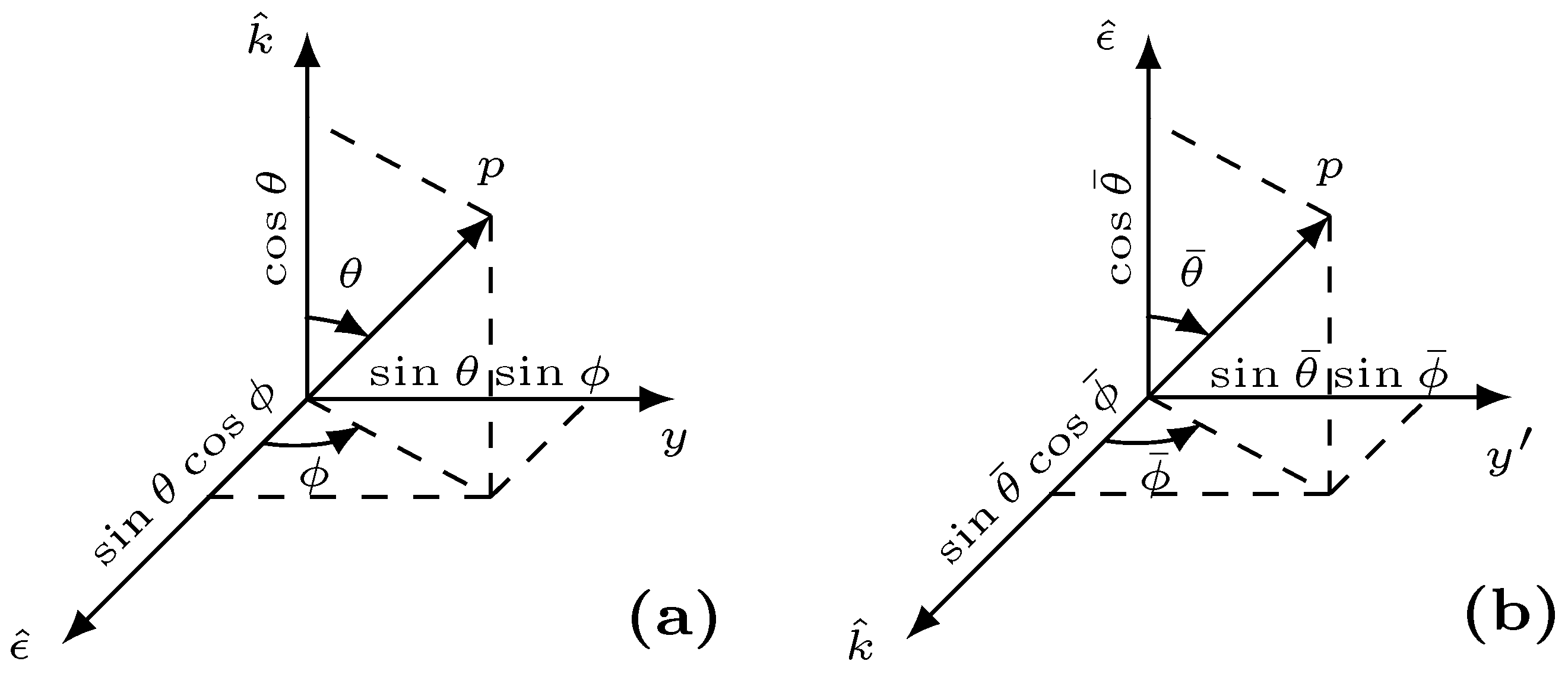
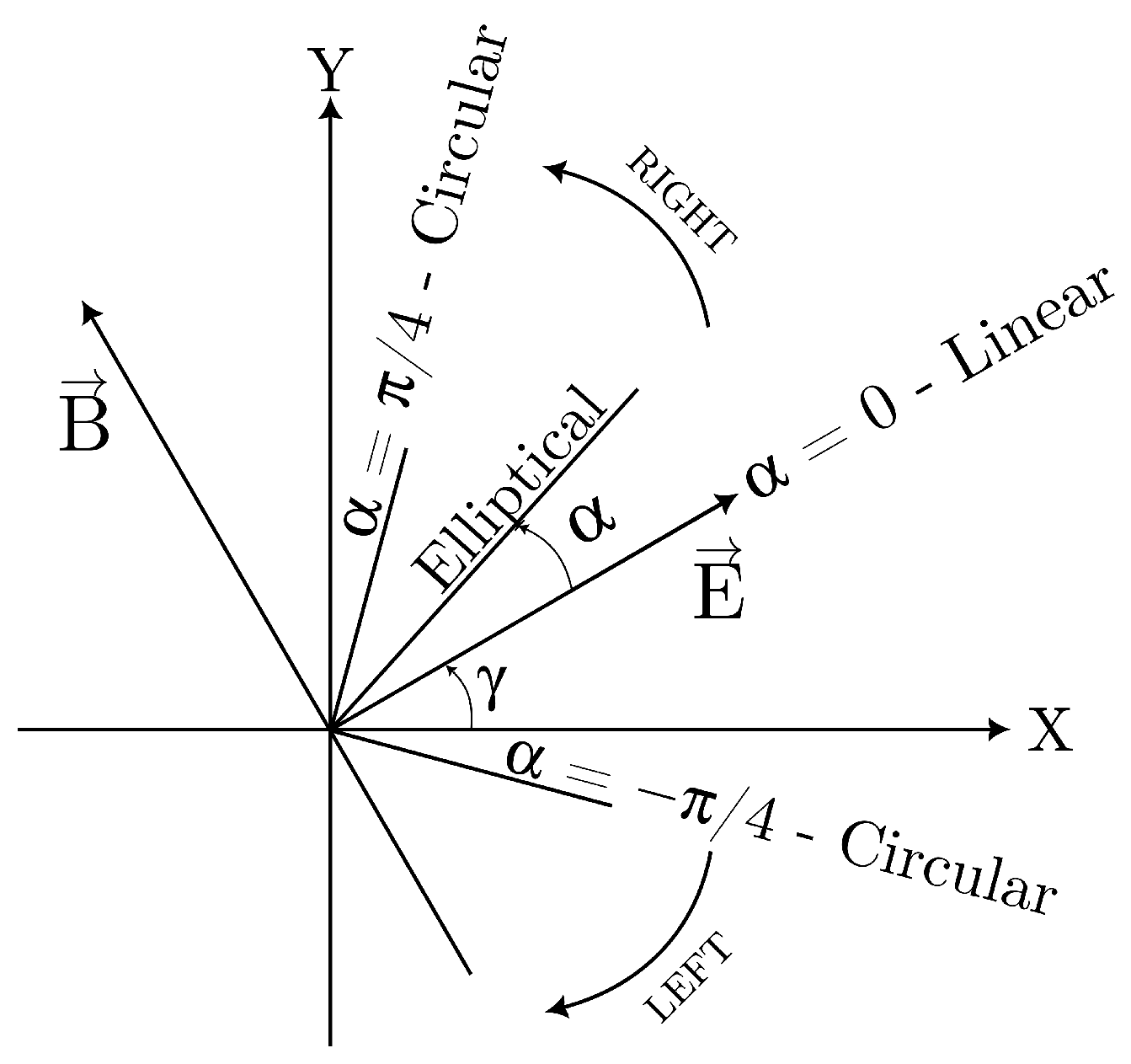
2.1. Photoionisation Dynamics Based on Helicity Formalism
2.2. Dipole Approximation
2.3. Beyond Dipole Approximation
3. Results and Discussion
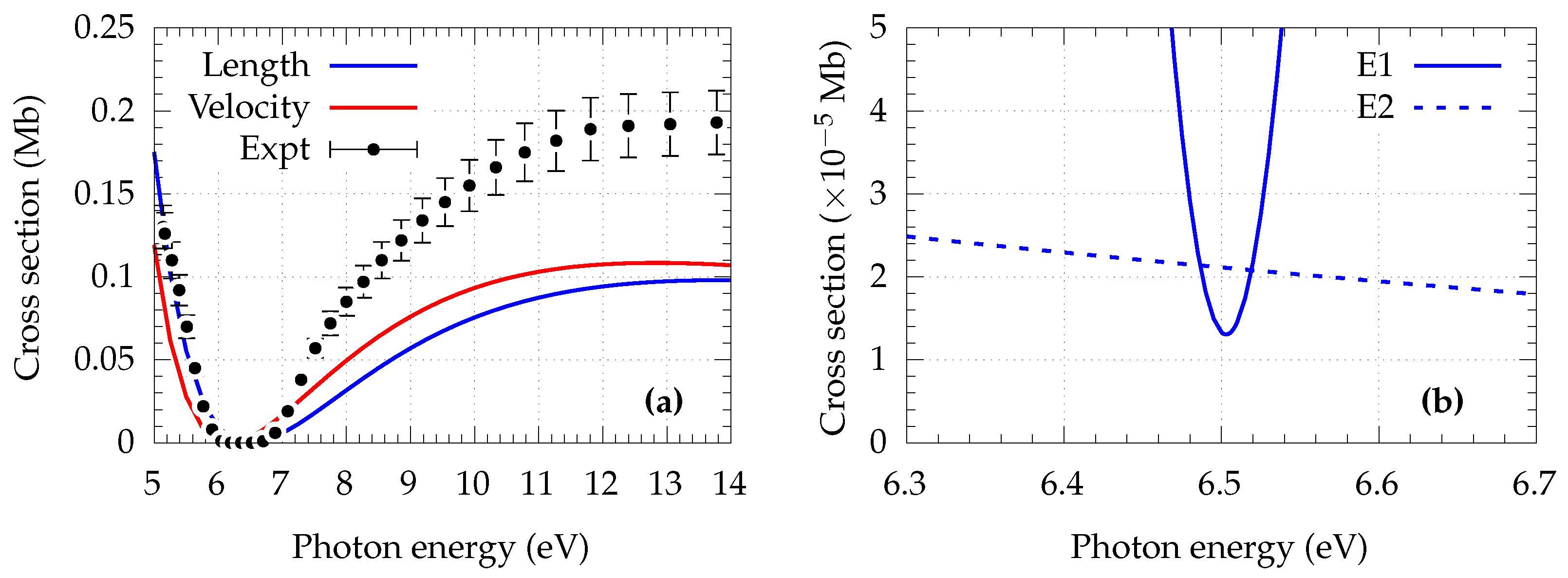
3.1. Cross-Section
3.2. Dipole Parameter,
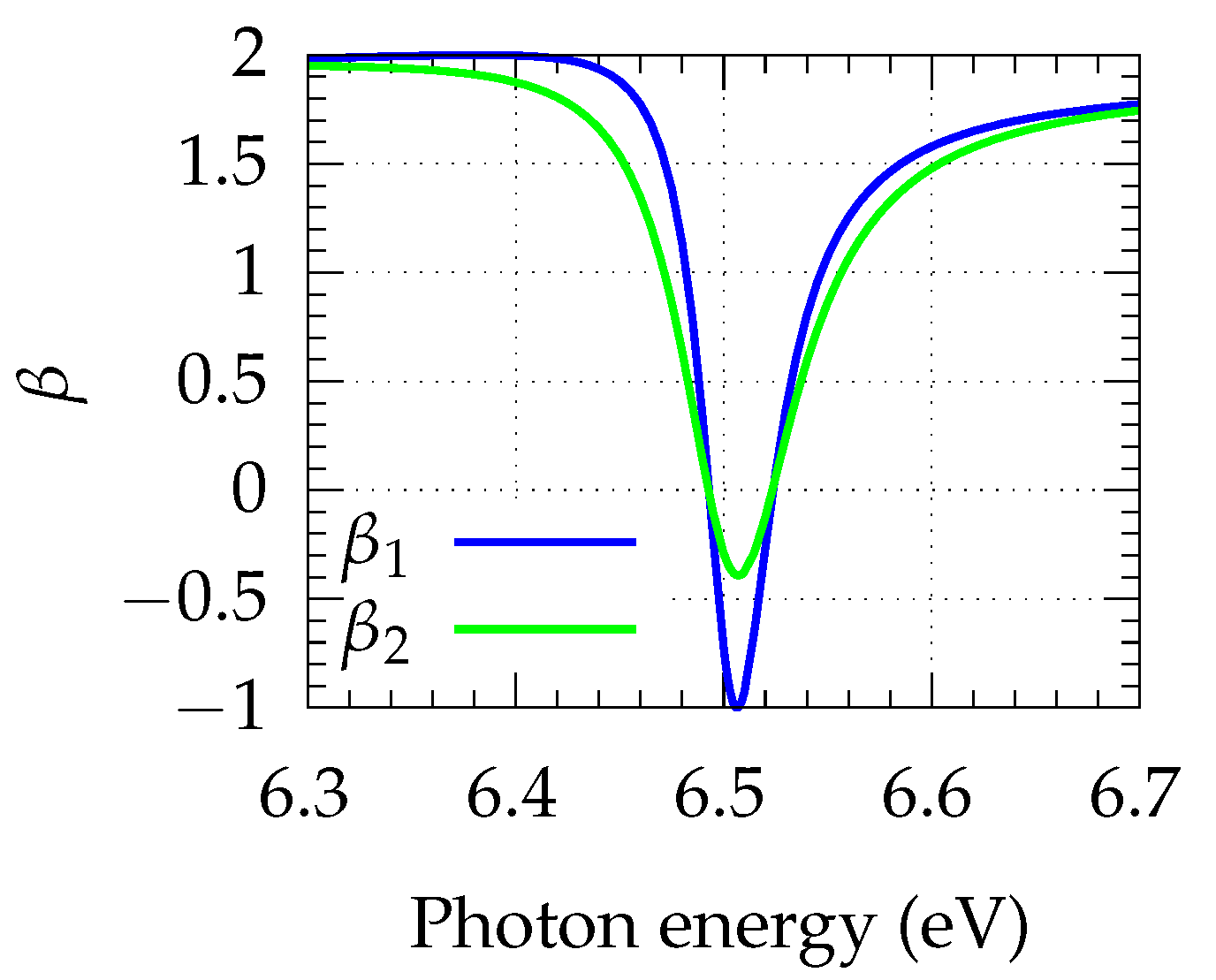
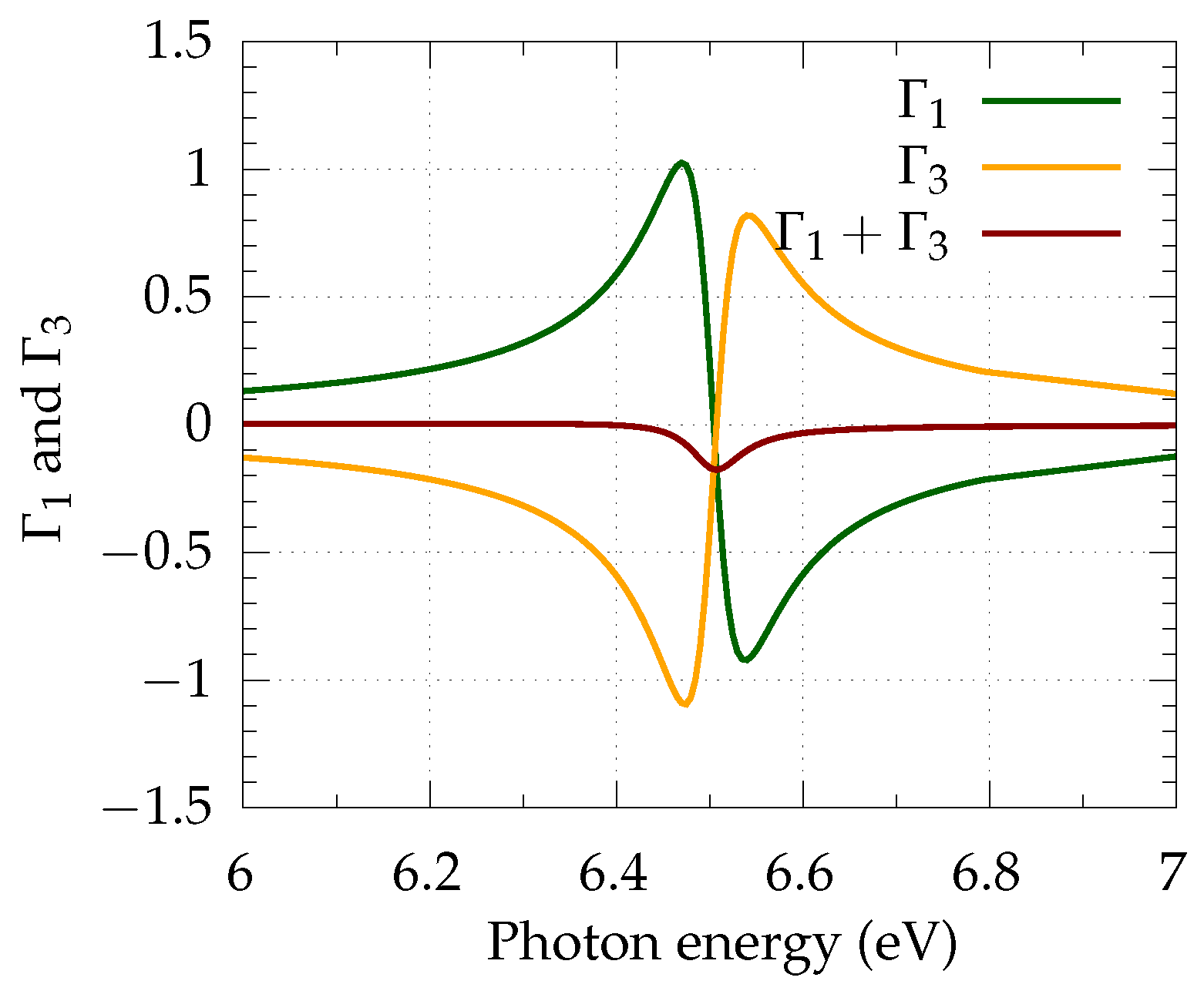
3.3. Quadrupole Parameters and
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hemmers, O.; Guillemin, R.; Lindle, D.W. Nondipole effects in soft X-ray photoemission. Radiat. Phys. Chem. 2004, 70, 123–147. [Google Scholar] [CrossRef]
- Guillemin, R.; Hemmers, O.; Lindle, D.W.; Manson, S.T. Experimental investigation of nondipole effects in photoemission at the advanced light source. Radiat. Phys. Chem. 2006, 75, 2258–2274. [Google Scholar] [CrossRef]
- Hemmers, O.; Guillemin, R.; Rolles, D.; Wolska, A.; Lindle, D.W.; Kanter, E.P.; Krässig, B.; Southworth, S.H.; Wehlitz, R.; Zimmermann, B.; et al. Low-energy nondipole effects in molecular nitrogen valence-shell photoionization. Phys. Rev. Lett. 2006, 97, 103006. [Google Scholar] [CrossRef] [PubMed]
- Cherepkov, N.A.; Semenov, S.K. Non-dipole effects in spin polarization of photoelectrons from Xe 4p and 5p shells. J. Phys. B At. Mol. Opt. Phys. 2001, 34, L211. [Google Scholar] [CrossRef]
- Khalil, T.; Schmidtke, B.; Drescher, M.; Müller, N.; Heinzmann, U. Experimental verification of quadrupole-dipole interference in spin-resolved photoionization. Phys. Rev. Lett. 2002, 89, 053001. [Google Scholar] [CrossRef]
- Jensen, S.V.B.; Madsen, L.B. Propagation time and nondipole contributions to intraband high-order harmonic generation. Phys. Rev. A 2022, 105, L021101. [Google Scholar] [CrossRef]
- Tyndall, N.B.; Ramsbottom, C.A.; Ballance, C.P.; Hibbert, A. Photoionization of Co+ and electron-impact excitation of Co2+ using the Dirac R-matrix method. Mon. Not. R. Astron. Soc. 2016, 462, 3350–3360. [Google Scholar] [CrossRef][Green Version]
- Emma, P.; Akre, R.; Arthur, J.; Bionta, R.; Bostedt, C.; Bozek, J.; Brachmann, A.; Bucksbaum, P.; Coffee, R.; Decker, F.J.; et al. First lasing and operation of an ångstrom-wavelength free-electron laser. Nat. Photonics 2010, 4, 641–647. [Google Scholar] [CrossRef]
- McNeil, B.W.; Thompson, N.R. X-ray free-electron lasers. Nat. Photonics 2010, 4, 814–821. [Google Scholar] [CrossRef]
- Ishikawa, T.; Aoyagi, H.; Asaka, T.; Asano, Y.; Azumi, N.; Bizen, T.; Ego, H.; Fukami, K.; Fukui, T.; Furukawa, Y.; et al. A compact X-ray free-electron laser emitting in the sub-ångström region. Nat. Photonics 2012, 6, 540–544. [Google Scholar] [CrossRef]
- Lindle, D.W.; Hemmers, O. Breakdown of the dipole approximation in soft-X-ray photoemission. J. Electron Spectrosc. Relat. Phenom. 1999, 100, 297–311. [Google Scholar] [CrossRef]
- Bechler, A.; Pratt, R. Higher retardation and multipole corrections to the dipole angular distribution of 1s photoelectrons at low energies. Phys. Rev. A 1989, 39, 1774. [Google Scholar] [CrossRef]
- Bechler, A.; Pratt, R. Higher multipole and retardation corrections to the dipole angular distributions of L-shell photoelectrons ejected by polarized photons. Phys. Rev. A 1990, 42, 6400. [Google Scholar] [CrossRef] [PubMed]
- Cooper, J.W. Multipole corrections to the angular distribution of photoelectrons at low energies. Phys. Rev. A 1990, 42, 6942. [Google Scholar] [CrossRef] [PubMed]
- Cooper, J.W. Erratum: Multipole corrections to the angular distribution of photoelectrons at low energies [Phys. Rev. A 42, 6942 (1990)]. Phys. Rev. A 1992, 45, 3362. [Google Scholar] [CrossRef] [PubMed]
- Cooper, J. Photoelectron-angular-distribution parameters for rare-gas subshells. Phys. Rev. A 1993, 47, 1841. [Google Scholar] [CrossRef]
- Krässig, B.; Jung, M.; Gemmell, D.; Kanter, E.; LeBrun, T.; Southworth, S.; Young, L. Nondipolar asymmetries of photoelectron angular distributions. Phys. Rev. Lett. 1995, 75, 4736. [Google Scholar] [CrossRef]
- Hemmers, O.; Fisher, G.; Glans, P.; Hansen, D.; Wang, H.; Whitfield, S.; Wehlitz, R.; Levin, J.; Sellin, I.; Perera, R.C.; et al. Beyond the dipole approximation: Angular-distribution effects in valence photoemission. J. Phys. B At. Mol. Opt. Phys. 1997, 30, L727. [Google Scholar] [CrossRef]
- Dolmatov, V.K.; Manson, S.T. Enhanced nondipole effects in low energy photoionization. Phys. Rev. Lett. 1999, 83, 939. [Google Scholar] [CrossRef]
- Amusia, M.Y.; Baltenkov, A.; Felfli, Z.; Msezane, A. Large nondipole correlation effects near atomic photoionization thresholds. Phys. Rev. A 1999, 59, R2544. [Google Scholar] [CrossRef]
- Derevianko, A.; Hemmers, O.; Oblad, S.; Glans, P.; Wang, H.; Whitfield, S.B.; Wehlitz, R.; Sellin, I.A.; Johnson, W.; Lindle, D.W. Electric-octupole and pure-electric-quadrupole effects in soft-X-ray photoemission. Phys. Rev. Lett. 2000, 84, 2116. [Google Scholar] [CrossRef] [PubMed]
- Amusia, M.Y.; Baltenkov, A.; Chernysheva, L.; Felfli, Z.; Msezane, A. Nondipole parameters in angular distributions of electrons in photoionization of noble-gas atoms. Phys. Rev. A 2001, 63, 052506. [Google Scholar] [CrossRef]
- Johnson, W.R.; Cheng, K. Strong nondipole effects in low-energy photoionization of the 5 s and 5 p subshells of xenon. Phys. Rev. A 2001, 63, 022504. [Google Scholar] [CrossRef]
- Cherepkov, N.A.; Semenov, S.K. On quadrupole resonances in atomic photoionization. J. Phys. B At. Mol. Opt. Phys. 2001, 34, L495. [Google Scholar] [CrossRef]
- Hemmers, O.; Guillemin, R.; Kanter, E.; Krässig, B.; Lindle, D.W.; Southworth, S.; Wehlitz, R.; Baker, J.; Hudson, A.; Lotrakul, M.; et al. Dramatic Nondipole Effects in Low-Energy Photoionization: Experimental and Theoretical Study of Xe 5 s. Phys. Rev. Lett. 2003, 91, 053002. [Google Scholar] [CrossRef]
- Trzhaskovskaya, M.; Nikulin, V.; Nefedov, V.; Yarzhemsky, V. Non-dipole second order parameters of the photoelectron angular distribution for elements Z = 1–100 in the photoelectron energy range 1–10 keV. At. Data Nucl. Data Tables 2006, 92, 245–304. [Google Scholar] [CrossRef]
- Trzhaskovskaya, M.; Nefedov, V.; Yarzhemsky, V. Photoelectron angular distribution parameters for elements Z = 1 to Z = 54 in the photoelectron energy range 100–5000 eV. At. Data Nucl. Data Tables 2001, 77, 97–159. [Google Scholar] [CrossRef]
- Leuchs, G.; Smith, S.; Dixit, S.; Lambropoulos, P. Observation of interference between quadrupole and dipole transitions in low-energy (2-eV) photoionization from a sodium Rydberg state. Phys. Rev. Lett. 1986, 56, 708. [Google Scholar] [CrossRef]
- Martin, N.; Thompson, D.; Bauman, R.; Caldwell, C.; Krause, M.; Frigo, S.; Wilson, M. Electric-dipole–quadrupole interference of overlapping autoionizing levels in photoelectron energy spectra. Phys. Rev. Lett. 1998, 81, 1199. [Google Scholar] [CrossRef]
- Grum-Grzhimailo, A. Non-dipole effects in magnetic dichroism in atomic photoionization. J. Phys. B At. Mol. Opt. Phys. 2001, 34, L359. [Google Scholar] [CrossRef]
- Krässig, B.; Kanter, E.; Southworth, S.; Guillemin, R.; Hemmers, O.; Lindle, D.W.; Wehlitz, R.; Martin, N. Photoexcitation of a dipole-forbidden resonance in helium. Phys. Rev. Lett. 2002, 88, 203002. [Google Scholar] [CrossRef] [PubMed]
- Kanter, E.; Krässig, B.; Southworth, S.; Guillemin, R.; Hemmers, O.; Lindle, D.W.; Wehlitz, R.; Amusia, M.Y.; Chernysheva, L.; Martin, N. E 1-E 2 interference in the vuv photoionization of He. Phys. Rev. A 2003, 68, 012714. [Google Scholar] [CrossRef]
- Lépine, F.; Zamith, S.; de Snaijer, A.; Bordas, C.; Vrakking, M. Observation of large quadrupolar effects in a slow photoelectron imaging experiment. Phys. Rev. Lett. 2004, 93, 233003. [Google Scholar] [CrossRef] [PubMed]
- Dolmatov, V.; Bailey, D.; Manson, S. Gigantic enhancement of atomic nondipole effects: The 3 s→ 3 d resonance in Ca. Phys. Rev. A 2005, 72, 022718. [Google Scholar] [CrossRef]
- Deshmukh, P.; Banerjee, T.; Varma, H.R.; Hemmers, O.; Guillemin, R.; Rolles, D.; Wolska, A.; Yu, S.; Lindle, D.W.; Johnson, W.; et al. Theoretical and experimental demonstrations of the existence of quadrupole Cooper minima. J. Phys. B At. Mol. Opt. Phys. 2008, 41, 021002. [Google Scholar] [CrossRef][Green Version]
- Argenti, L.; Moccia, R. Nondipole effects in helium photoionization. J. Phys. B At. Mol. Opt. Phys. 2010, 43, 235006. [Google Scholar] [CrossRef]
- Pradhan, G.; Jose, J.; Deshmukh, P.; LaJohn, L.; Pratt, R.; Manson, S. Cooper minima: A window on nondipole photoionization at low energy. J. Phys. B At. Mol. Opt. Phys. 2011, 44, 201001. [Google Scholar] [CrossRef]
- Gryzlova, E.; Grum-Grzhimailo, A.; Strakhova, S.; Meyer, M. Non-dipole effects in the angular distribution of photoelectrons in sequential two-photon double ionization: Argon and neon. J. Phys. B At. Mol. Opt. Phys. 2013, 46, 164014. [Google Scholar] [CrossRef]
- Gryzlova, E.; Grum-Grzhimailo, A.; Kuzmina, E.; Strakhova, S. Sequential two-photon double ionization of noble gases by circularly polarized XUV radiation. J. Phys. B At. Mol. Opt. Phys. 2014, 47, 195601. [Google Scholar] [CrossRef]
- Ilchen, M.; Hartmann, G.; Gryzlova, E.; Achner, A.; Allaria, E.; Beckmann, A.; Braune, M.; Buck, J.; Callegari, C.; Coffee, R.; et al. Symmetry breakdown of electron emission in extreme ultraviolet photoionization of argon. Nat. Commun. 2018, 9, 4659. [Google Scholar] [CrossRef]
- Huang, K.N. Theory of angular distribution and spin polarization of photoelectrons. Phys. Rev. A 1980, 22, 223. [Google Scholar] [CrossRef]
- Huang, K.N. Addendum to “Theory of angular distribution and spin polarization of photoelectrons”. Phys. Rev. A 1982, 26, 3676. [Google Scholar] [CrossRef]
- Derevianko, A.; Johnson, W.; Cheng, K. Non-dipole effects in photoelectron angular distributions for rare gas atoms. At. Data Nucl. Data Tables 1999, 73, 153–211. [Google Scholar] [CrossRef][Green Version]
- Dyall, K.; Grant, I.; Johnson, C.; Parpia, F.; Plummer, E. GRASP: A general-purpose relativistic atomic structure program. Comput. Phys. Commun. 1989, 55, 425–456. [Google Scholar] [CrossRef]
- Parpia, F.A.; Fischer, C.F.; Grant, I.P. GRASP92: A package for large-scale relativistic atomic structure calculations. Comput. Phys. Commun. 1996, 94, 249–271. [Google Scholar] [CrossRef]
- Jönsson, P.; Gaigalas, G.; Bieroń, J.; Fischer, C.F.; Grant, I. New version: Grasp2K relativistic atomic structure package. Comput. Phys. Commun. 2013, 184, 2197–2203. [Google Scholar] [CrossRef]
- Fritzsche, S. The Ratip program for relativistic calculations of atomic transition, ionization and recombination properties. Comput. Phys. Commun. 2012, 183, 1525–1559. [Google Scholar] [CrossRef]
- Hütten, K.; Mittermair, M.; Stock, S.O.; Beerwerth, R.; Shirvanyan, V.; Riemensberger, J.; Duensing, A.; Heider, R.; Wagner, M.S.; Guggenmos, A.; et al. Ultrafast quantum control of ionization dynamics in krypton. Nat. Commun. 2018, 9, 719. [Google Scholar] [CrossRef] [PubMed]
- Perry-Sassmannshausen, A.; Buhr, T.; Borovik, A., Jr.; Martins, M.; Reinwardt, S.; Ricz, S.; Stock, S.; Trinter, F.; Müller, A.; Fritzsche, S.; et al. Multiple photodetachment of carbon anions via single and double core-hole creation. Phys. Rev. Lett. 2020, 124, 083203. [Google Scholar] [CrossRef]
- Schippers, S.; Beerwerth, R.; Bari, S.; Buhr, T.; Holste, K.; Kilcoyne, A.D.; Perry-Sassmannshausen, A.; Phaneuf, R.A.; Reinwardt, S.; Savin, D.W.; et al. Near L-edge single and multiple photoionization of doubly charged iron ions. Astrophys. J. 2021, 908, 52. [Google Scholar] [CrossRef]
- Hosea, N.M.; Jose, J.; Varma, H.R. Near-threshold Cooper minimum in the photoionisation of the 2p subshell of sodium atom and its impact on the angular distribution parameter. J. Phys. B At. Mol. Opt. Phys. 2022, 55, 135001. [Google Scholar] [CrossRef]
- Lee, C. Spin polarization and angular distribution of photoelectrons in the Jacob-Wick helicity formalism. Application to autoionization resonances. Phys. Rev. A 1974, 10, 1598. [Google Scholar] [CrossRef]
- Landau, L.; Lifshitz, E. A Shorter Course of Theoretical Physics. Vol. 2: Quantum Mechanics; Pergamon: Oxford, UK, 1974. [Google Scholar]
- Seaton, M.J. A comparison of theory and experiment for photo-ionization cross-sections II. Sodium and the alkali metals. Proc. R. Soc. Lond. Ser. A. Math. Phys. Sci. 1951, 208, 418–430. [Google Scholar]
- Hudson, R.D.; Carter, V.L. Atomic absorption cross sections of lithium and sodium between 600 and 1000 Å. J. Opt. Soc. Am. 1967, 57, 651–654. [Google Scholar] [CrossRef]
- Manson, S.T.; Starace, A.F. Photoelectron angular distributions: Energy dependence for s subshells. Rev. Mod. Phys. 1982, 54, 389. [Google Scholar] [CrossRef]
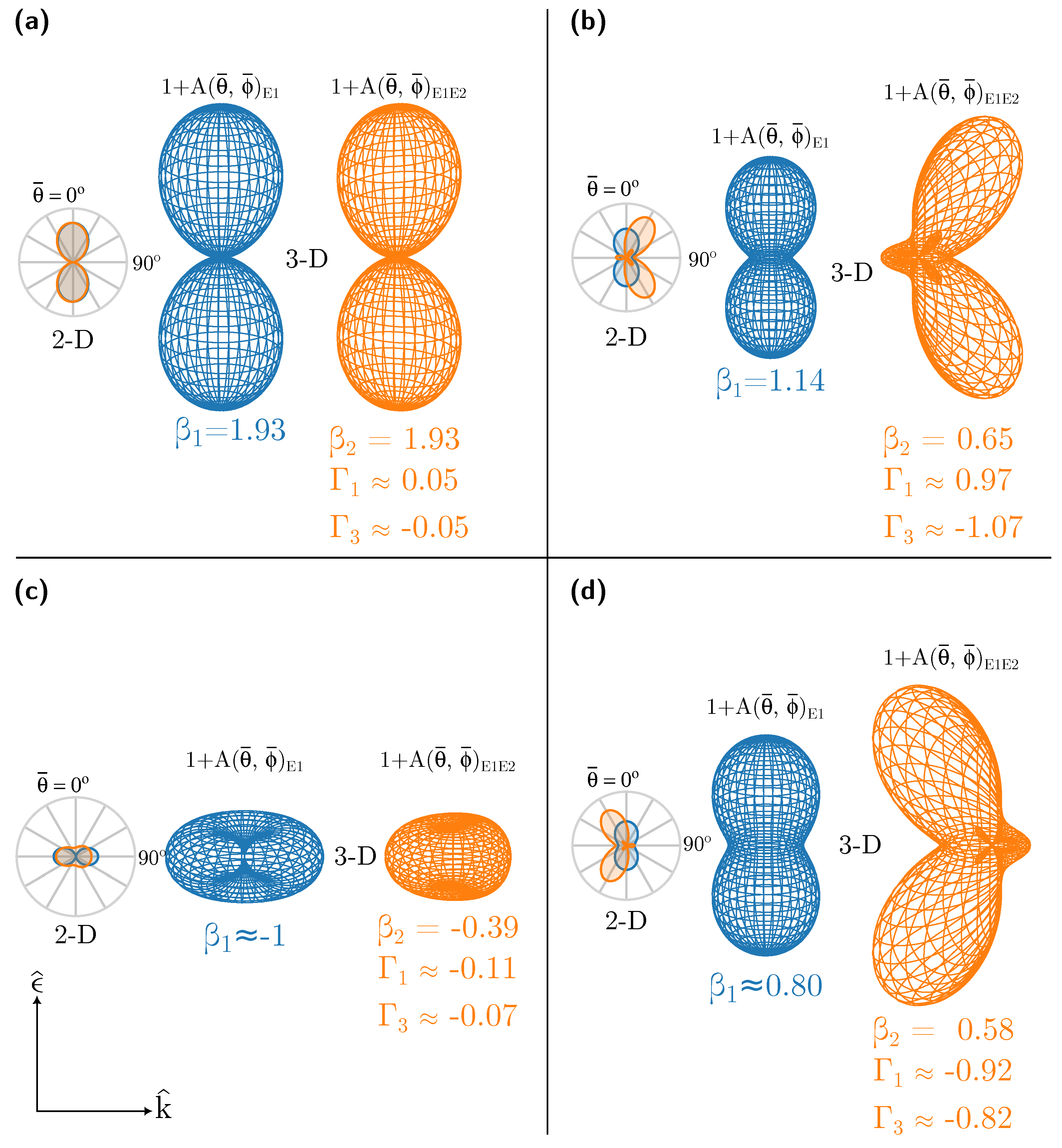
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hosea, N.M.; Jose, J.; Varma, H.R.; Deshmukh, P.C.; Manson, S.T. Quadrupole Effects in the Photoionisation of Sodium 3s in the Vicinity of the Dipole Cooper Minimum. Atoms 2023, 11, 125. https://doi.org/10.3390/atoms11100125
Hosea NM, Jose J, Varma HR, Deshmukh PC, Manson ST. Quadrupole Effects in the Photoionisation of Sodium 3s in the Vicinity of the Dipole Cooper Minimum. Atoms. 2023; 11(10):125. https://doi.org/10.3390/atoms11100125
Chicago/Turabian StyleHosea, Nishita M., Jobin Jose, Hari R. Varma, Pranawa C. Deshmukh, and Steven T. Manson. 2023. "Quadrupole Effects in the Photoionisation of Sodium 3s in the Vicinity of the Dipole Cooper Minimum" Atoms 11, no. 10: 125. https://doi.org/10.3390/atoms11100125
APA StyleHosea, N. M., Jose, J., Varma, H. R., Deshmukh, P. C., & Manson, S. T. (2023). Quadrupole Effects in the Photoionisation of Sodium 3s in the Vicinity of the Dipole Cooper Minimum. Atoms, 11(10), 125. https://doi.org/10.3390/atoms11100125






