Modeling and Testing Screening Mechanisms in the Laboratory and in Space
Abstract
:| Contents | ||
| 1. | Introduction ..................................................................................................................................................... | 2 |
| 2. | Summary of Theories...................................................................................................................................... | 3 |
| 2.1. Thin-Shell Scenarios............................................................................................................................. | 3 | |
| 2.2. Galileons................................................................................................................................................ | 5 | |
| 3. | Numerical Methods and Technical Considerations................................................................................... | 7 |
| 3.1. Relaxation Method................................................................................................................................ | 7 | |
| 3.2. Finite Element Codes............................................................................................................................ | 10 | |
| 3.3. Forces on Extended Objects................................................................................................................. | 13 | |
| 4. | Laboratory Tests............................................................................................................................................... | 14 |
| 4.1. Direct Force Measurements................................................................................................................. | 15 | |
| 4.2. Indirect Measurements......................................................................................................................... | 17 | |
| 5. | Astrophysical Scales........................................................................................................................................ | 17 |
| 5.1. Stars........................................................................................................................................................ | 17 | |
| 5.1.1. Hydrostatic Equilibrium—Stellar Structure and Evolution.............................................. | 17 | |
| 5.1.2. Out of Equilibrium—Stellar Oscillations............................................................................ | 19 | |
| 5.2. Screening Maps..................................................................................................................................... | 20 | |
| 5.3. Galaxy Morphology............................................................................................................................. | 21 | |
| 5.3.1. Thin-Shell Screened Theories............................................................................................... | 21 | |
| 5.3.2. Vainshtein Screened Theories............................................................................................... | 22 | |
| 5.4. Halo Properties..................................................................................................................................... | 22 | |
| 5.5. Splashback............................................................................................................................................. | 23 | |
| 6. | Conclusions...................................................................................................................................................... | 25 |
| References................................................................................................................................................................... | 26 | |
1. Introduction
2. Summary of Theories
2.1. Thin-Shell Scenarios
- 1.
- , with const and n (chameleons),
- 2.
- , with const and M (symmetrons).
2.2. Galileons
3. Numerical Methods and Technical Considerations
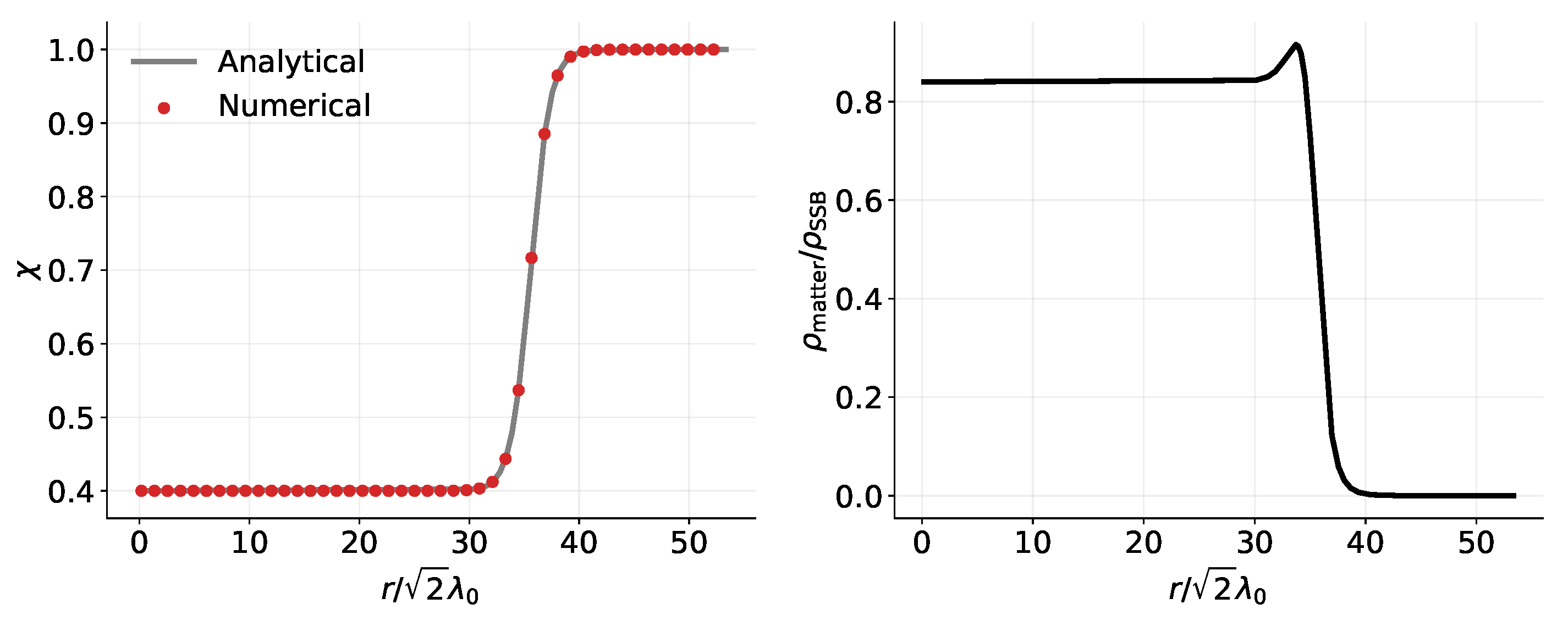
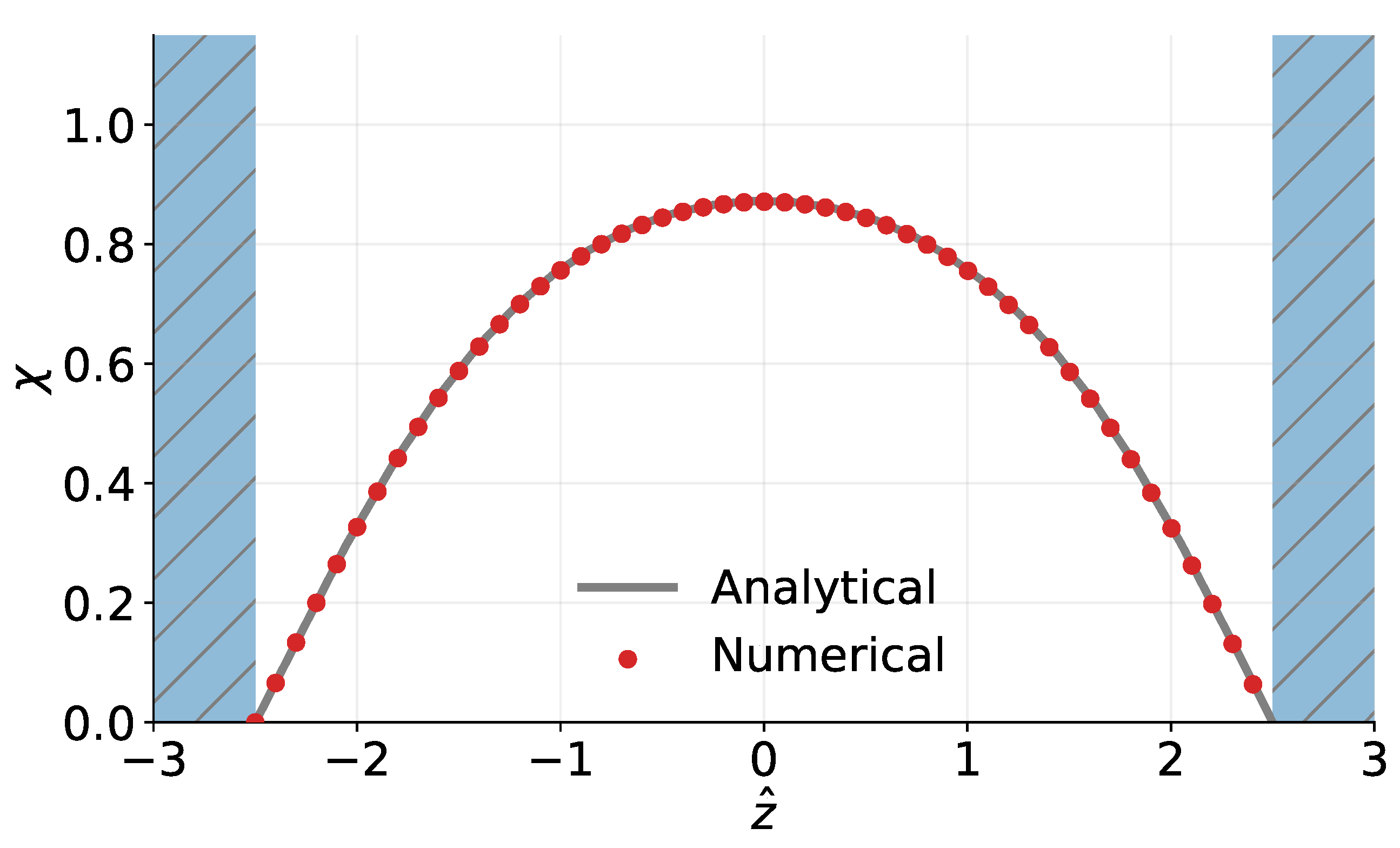
3.1. Relaxation Method
3.2. Finite Element Codes
3.3. Forces on Extended Objects
4. Laboratory Tests
4.1. Direct Force Measurements
4.2. Indirect Measurements
5. Astrophysical Scales
5.1. Stars
5.1.1. Hydrostatic Equilibrium—Stellar Structure and Evolution
5.1.2. Out of Equilibrium—Stellar Oscillations
5.2. Screening Maps
5.3. Galaxy Morphology
5.3.1. Thin-Shell Screened Theories
5.3.2. Vainshtein Screened Theories
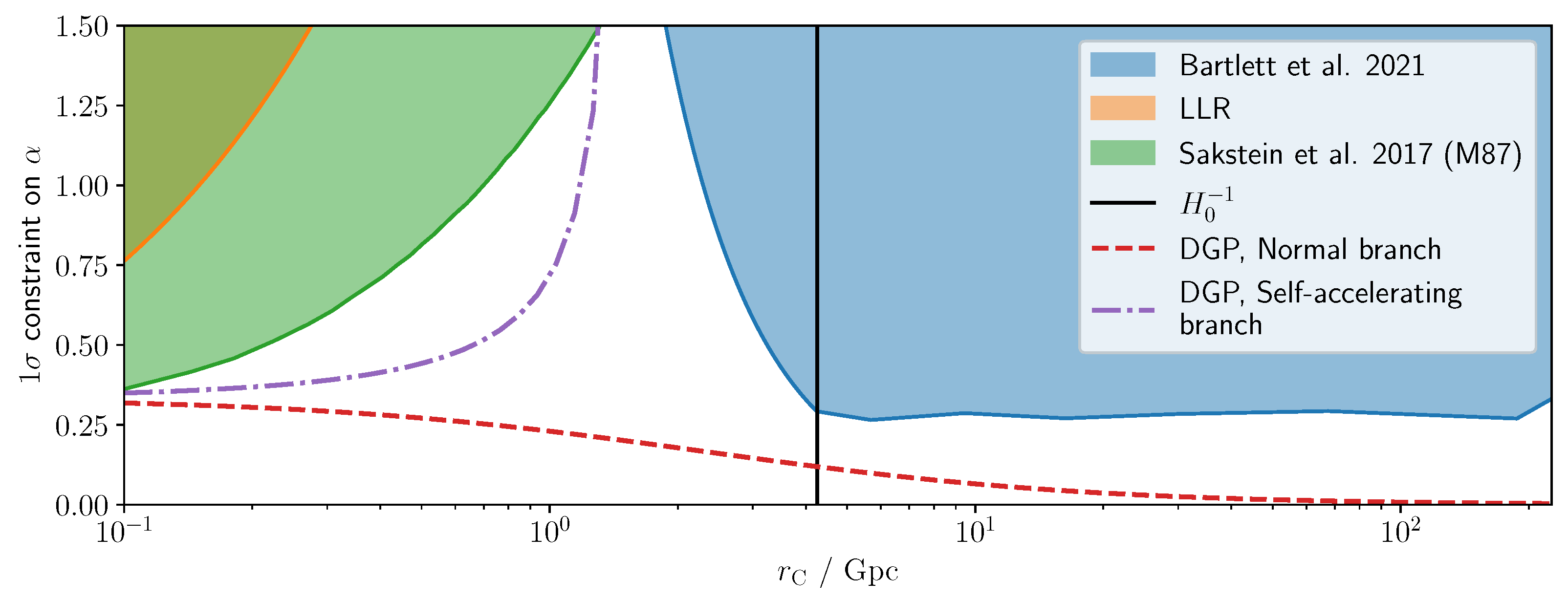
5.4. Halo Properties
5.5. Splashback
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Perlmutter, S. Measurements of Ω and Λ from 42 high redshift supernovae. Astrophys. J. 1999, 517, 565–586. [Google Scholar] [CrossRef]
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Gillil, R.L.; Hogan, C.J.; Jha, S.; Kirshner, R.P.; et al. Observational evidence from supernovae for an accelerating universe and a cosmological constant. Astron. J. 1998, 116, 1009–1038. [Google Scholar] [CrossRef] [Green Version]
- Copeland, E.J.; Sami, M.; Tsujikawa, S. Dynamics of dark energy. Int. J. Mod. Phys. D 2006, 15, 1753–1936. [Google Scholar] [CrossRef] [Green Version]
- Silvestri, A.; Trodden, M. Approaches to Understanding Cosmic Acceleration. Rept. Prog. Phys. 2009, 72, 096901. [Google Scholar] [CrossRef] [Green Version]
- Clifton, T.; Ferreira, P.G.; Padilla, A.; Skordis, C. Modified Gravity and Cosmology. Phys. Rept. 2012, 513, 1–189. [Google Scholar] [CrossRef] [Green Version]
- Joyce, A.; Jain, B.; Khoury, J.; Trodden, M. Beyond the Cosmological Standard Model. Phys. Rept. 2015, 568, 1–98. [Google Scholar] [CrossRef] [Green Version]
- Koyama, K. Cosmological Tests of Modified Gravity. Rept. Prog. Phys. 2016, 79, 046902. [Google Scholar] [CrossRef] [Green Version]
- Ade, P.A.; Aghanim, N.; Arnaud, M.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Bay, A.J.; Barreiro, R.B.; Bartolo, N.; Battaner, E.; et al. Planck 2015 results. XIV. Dark energy and modified gravity. Astron. Astrophys. 2016, 594, A14. [Google Scholar] [CrossRef] [Green Version]
- Pogosian, L.; Silvestri, A. What can cosmology tell us about gravity? Constraining Horndeski gravity with Σ and μ. Phys. Rev. 2016, D94, 104014. [Google Scholar] [CrossRef] [Green Version]
- Will, C.M. Theory and Experiment in Gravitational Physics; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Khoury, J.; Weltman, A. Chameleon cosmology. Phys. Rev. D 2004, 69, 044026. [Google Scholar] [CrossRef]
- Khoury, J.; Weltman, A. Chameleon fields: Awaiting surprises for tests of gravity in space. Phys. Rev. Lett. 2004, 93, 171104. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Brax, P.; van de Bruck, C.; Davis, A.C.; Khoury, J.; Weltman, A. Detecting dark energy in orbit: The cosmological chameleon. Phys. Rev. D 2004, 70, 123518. [Google Scholar] [CrossRef] [Green Version]
- Hinterbichler, K.; Khoury, J. Symmetron Fields: Screening Long-Range Forces Through Local Symmetry Restoration. Phys. Rev. Lett. 2010, 104, 231301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hinterbichler, K.; Khoury, J.; Levy, A.; Matas, A. Symmetron Cosmology. Phys. Rev. D 2011, 84, 103521. [Google Scholar] [CrossRef] [Green Version]
- Vainshtein, A.I. To the problem of nonvanishing gravitation mass. Phys. Lett. B 1972, 39, 393–394. [Google Scholar] [CrossRef]
- Nicolis, A.; Rattazzi, R.; Trincherini, E. Galileon as a local modification of gravity. Phys. Rev. D 2009, 79, 064036. [Google Scholar] [CrossRef] [Green Version]
- Babichev, E.; Deffayet, C. An introduction to the Vainshtein mechanism. Class. Quant. Grav. 2013, 30, 184001. [Google Scholar] [CrossRef]
- Burrage, C.; Sakstein, J. A Compendium of Chameleon Constraints. J. Cosmol. Astropart. Phys. 2016, 11, 045. [Google Scholar] [CrossRef] [Green Version]
- Burrage, C.; Sakstein, J. Tests of Chameleon Gravity. Living Rev. Relativ. 2018, 21, 1. [Google Scholar] [CrossRef] [Green Version]
- Sakstein, J. Astrophysical tests of screened modified gravity. Int. J. Mod. Phys. D 2018, 27, 1848008. [Google Scholar] [CrossRef] [Green Version]
- Baker, T.; Barreira, A.; Desmond, H.; Ferreira, P.; Jain, B.; Koyama, K.; Li, B.; Lombriser, L.; Nicola, A.; Sakstein, J.; et al. Novel Probes Project: Tests of gravity on astrophysical scales. Rev. Mod. Phys. 2021, 93, 015003. [Google Scholar] [CrossRef]
- Brax, P.; Casas, S.; Desmond, H.; Elder, B. Testing Screened Modified Gravity. Universe 2021, 8, 11. [Google Scholar] [CrossRef]
- Elder, B.; Vardanyan, V.; Akrami, Y.; Brax, P.; Davis, A.C.; Decca, R.S. Classical symmetron force in Casimir experiments. Phys. Rev. D 2020, 101, 064065. [Google Scholar] [CrossRef] [Green Version]
- Babichev, E.; Deffayet, C.; Ziour, R. k-MOUFLAGE Gravity. Int. J. Mod. Phys. D 2009, 18, 2147–2154. [Google Scholar] [CrossRef] [Green Version]
- Dvali, G.; Gabadadze, G.; Porrati, M. 4D gravity on a brane in 5D Minkowski space. Phys. Lett. B 2000, 485, 208–214. [Google Scholar] [CrossRef] [Green Version]
- Horndeski, G.W. Second-Order Scalar-Tensor Field Equations in a Four-Dimensional Space. Int. J. Theor. Phys. 1974, 10, 363–384. [Google Scholar] [CrossRef]
- Babichev, E.; Esposito-Farèse, G. Time-dependent spherically symmetric covariant Galileons. Phys. Rev. D 2013, 87, 044032. [Google Scholar] [CrossRef] [Green Version]
- de Felice, A.; Tsujikawa, S. Cosmology of a Covariant Galileon Field. Phys. Rev. Lett. 2010, 105, 111301. [Google Scholar] [CrossRef] [Green Version]
- Renk, J.; Zumalacárregui, M.; Montanari, F.; Barreira, A. Galileon gravity in light of ISW, CMB, BAO and H0 data. J. Cosmol. Astropart. Phys. 2017, 2017, 020. [Google Scholar] [CrossRef] [Green Version]
- LIGO Scientific Collaboration; Virgo Collaboration. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef] [Green Version]
- Ezquiaga, J.M.; Zumalacárregui, M. Dark Energy After GW170817: Dead Ends and the Road Ahead. Phys. Rev. Lett. 2017, 119, 251304. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Barreira, A.; Li, B.; Hellwing, W.A.; Baugh, C.M.; Pascoli, S. Nonlinear structure formation in the cubic Galileon gravity model. J. Cosmol. Astropart. Phys. 2013, 2013, 027. [Google Scholar] [CrossRef] [Green Version]
- Hui, L.; Nicolis, A.; Stubbs, C.W. Equivalence principle implications of modified gravity models. Phys. Rev. D 2009, 80, 104002. [Google Scholar] [CrossRef] [Green Version]
- Hui, L.; Nicolis, A. Proposal for an Observational Test of the Vainshtein Mechanism. Phys. Rev. Lett. 2012, 109, 051304. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hui, L.; Nicolis, A. No-Hair Theorem for the Galileon. Phys. Rev. Lett. 2013, 110, 241104. [Google Scholar] [CrossRef] [Green Version]
- Schmidt, F.; Hu, W.; Lima, M. Spherical collapse and the halo model in braneworld gravity. Phys. Rev. D 2010, 81, 063005. [Google Scholar] [CrossRef] [Green Version]
- Sakstein, J.; Jain, B.; Heyl, J.S.; Hui, L. Tests of Gravity Theories Using Supermassive Black Holes. Astrophys. J. Lett. 2017, 844, L14. [Google Scholar] [CrossRef] [Green Version]
- Falck, B.; Koyama, K.; Zhao, G.B.; Li, B. The Vainshtein Mechanism in the Cosmic Web. J. Cosmol. Astropart. Phys. 2014, 07, 058. [Google Scholar] [CrossRef] [Green Version]
- Cardoso, A.; Koyama, K.; Seahra, S.S.; Silva, F.P. Cosmological perturbations in the DGP braneworld: Numeric solution. Phys. Rev. D 2008, 77, 083512. [Google Scholar] [CrossRef] [Green Version]
- Schmidt, F. Self-consistent cosmological simulations of DGP braneworld gravity. Phys. Rev. D 2009, 80, 043001. [Google Scholar] [CrossRef] [Green Version]
- Khoury, J.; Wyman, M. N-body simulations of DGP and degravitation theories. Phys. Rev. D 2009, 80, 064023. [Google Scholar] [CrossRef] [Green Version]
- Chan, K.C.; Scoccimarro, R. Large-scale structure in brane-induced gravity. II. Numerical simulations. Phys. Rev. D 2009, 80, 104005. [Google Scholar] [CrossRef] [Green Version]
- Davis, A.C.; Li, B.; Mota, D.F.; Winther, H.A. Structure Formation in the Symmetron Model. Astrophys. J. 2012, 748, 61. [Google Scholar] [CrossRef] [Green Version]
- Clampitt, J.; Jain, B.; Khoury, J. Halo Scale Predictions of Symmetron Modified Gravity. J. Cosmol. Astropart. Phys. 2012, 1201, 030. [Google Scholar] [CrossRef] [Green Version]
- Brax, P.; Davis, A.C.; Li, B.; Winther, H.A.; Zhao, G.B. Systematic Simulations of Modified Gravity: Symmetron and Dilaton Models. J. Cosmol. Astropart. Phys. 2012, 1210, 002. [Google Scholar] [CrossRef] [Green Version]
- Llinares, C.; Mota, D.F. Cosmological simulations of screened modified gravity out of the static approximation: Effects on matter distribution. Phys. Rev. 2014, D89, 084023. [Google Scholar] [CrossRef] [Green Version]
- Noller, J.; von Braun-Bates, F.; Ferreira, P.G. Relativistic scalar fields and the quasistatic approximation in theories of modified gravity. Phys. Rev. 2014, D89, 023521. [Google Scholar] [CrossRef] [Green Version]
- Vardanyan, V. Aspects of Cosmic Acceleration. Ph.D. Thesis, Leiden University, Leiden, The Netherlands, 2019. [Google Scholar]
- Contigiani, O.; Vardanyan, V.; Silvestri, A. Splashback radius in symmetron gravity. Phys. Rev. D 2019, 99, 064030. [Google Scholar] [CrossRef] [Green Version]
- Upadhye, A. Symmetron dark energy in laboratory experiments. Phys. Rev. Lett. 2013, 110, 031301. [Google Scholar] [CrossRef] [Green Version]
- Li, B.; Zhao, G.B.; Teyssier, R.; Koyama, K. ECOSMOG: An Efficient COde for Simulating MOdified Gravity. J. Cosmol. Astropart. Phys. 2012, 2012, 051. [Google Scholar] [CrossRef]
- Llinares, C.; Mota, D.F.; Winther, H.A. ISIS: A new N-body cosmological code with scalar fields based on RAMSES. Code presentation and application to the shapes of clusters. Astron. Astrophys. 2014, 562, A78. [Google Scholar] [CrossRef] [Green Version]
- Puchwein, E.; Baldi, M.; Springel, V. Modified Gravity-GADGET: A new code for cosmological hydrodynamical simulations of modified gravity models. Mon. Not. R. Astron. Soc. 2013, 436, 348. [Google Scholar] [CrossRef] [Green Version]
- Winther, H.A.; Schmidt, F.; Barreira, A.; Arnold, C.; Bose, S.; Llinares, C.; Baldi, M.; Falck, B.; Hellwing, W.A.; Koyama, K.; et al. Modified Gravity N-body Code Comparison Project. Mon. Not. R. Astron. Soc. 2015, 454, 4208–4234. [Google Scholar] [CrossRef] [Green Version]
- Briddon, C.; Burrage, C.; Moss, A.; Tamosiunas, A. SELCIE: A tool for investigating the chameleon field of arbitrary sources. J. Cosmol. Astropart. Phys. 2021, 2021, 043. [Google Scholar] [CrossRef]
- Alnaes, M.S.; Blechta, J.; Hake, J.; Johansson, A.; Kehlet, B.; Logg, A.; Richardson, C.; Ring, J.; Rognes, M.E.; Wells, G.N. The FEniCS Project Version 1.5. Arch. Numer. Softw. 2015, 3, 9–23. [Google Scholar] [CrossRef]
- Logg, A.; Mardal, K.A.; Wells, G. Automated Solution of Differential Equations by the Finite Element Method; Springer: Berlin, Germany, 2012. [Google Scholar] [CrossRef]
- Cimrman, R.; Lukeš, V.; Rohan, E. Multiscale finite element calculations in Python using SfePy. arXiv 2018, arXiv:1810.00674. [Google Scholar] [CrossRef] [Green Version]
- Braden, J.; Burrage, C.; Elder, B.; Saadeh, D. φenics: Vainshtein screening with the finite element method. J. Cosmol. Astropart. Phys. 2021, 2021, 010. [Google Scholar] [CrossRef]
- Tamosiunas, A.; Briddon, C.; Burrage, C.; Cutforth, A.; Moss, A.; Vincent, T. Chameleon screening in cosmic voids. J. Cosmol. Astropart. Phys. 2022, 2022, 056. [Google Scholar] [CrossRef]
- Tamosiunas, A.; Briddon, C.; Burrage, C.; Cui, W.; Moss, A. Chameleon screening depends on the shape and structure of NFW halos. J. Cosmol. Astropart. Phys. 2022, 2022, 047. [Google Scholar] [CrossRef]
- Lévy, H.; Bergé, J.; Uzan, J.P. Solving nonlinear Klein-Gordon equations on unbounded domains via the finite element method. Phys. Rev. D 2022, 106, 124021. [Google Scholar] [CrossRef]
- Grosch, C.E.; Orszag, S.A. Numerical Solution of Problems in Unbounded Regions: Coordinate Transforms. J. Comput. Phys. 1977, 25, 273–295. [Google Scholar] [CrossRef]
- Burrage, C.; Copeland, E.J.; Moss, A.; Stevenson, J.A. The shape dependence of chameleon screening. J. Cosmol. Astropart. Phys. 2018, 2018, 056. [Google Scholar] [CrossRef] [Green Version]
- Burrage, C.; Copeland, E.J.; Hinds, E.A. Probing Dark Energy with Atom Interferometry. J. Cosmol. Astropart. Phys. 2015, 2015, 042. [Google Scholar] [CrossRef]
- Burrage, C.; Copeland, E.J. Using Atom Interferometry to Detect Dark Energy. Contemp. Phys. 2016, 57, 164–176. [Google Scholar] [CrossRef] [Green Version]
- Hamilton, P.; Jaffe, M.; Haslinger, P.; Simmons, Q.; Müller, H.; Khoury, J. Atom-interferometry constraints on dark energy. Science 2015, 349, 849–851. [Google Scholar] [CrossRef] [Green Version]
- Burrage, C.; Kuribayashi-Coleman, A.; Stevenson, J.; Thrussell, B. Constraining symmetron fields with atom interferometry. J. Cosmol. Astropart. Phys. 2016, 1612, 041. [Google Scholar] [CrossRef] [Green Version]
- Jaffe, M.; Haslinger, P.; Xu, V.; Hamilton, P.; Upadhye, A.; Elder, B.; Khoury, J.; Müller, H. Testing sub-gravitational forces on atoms from a miniature, in-vacuum source mass. Nat. Phys. 2017, 13, 938. [Google Scholar] [CrossRef] [Green Version]
- Sabulsky, D.O.; Dutta, I.; Hinds, E.A.; Elder, B.; Burrage, C.; Copeland, E.J. Experiment to detect dark energy forces using atom interferometry. Phys. Rev. Lett. 2019, 123, 061102. [Google Scholar] [CrossRef] [Green Version]
- Brax, P.; Burrage, C. Atomic Precision Tests and Light Scalar Couplings. Phys. Rev. D 2011, 83, 035020. [Google Scholar] [CrossRef] [Green Version]
- Brax, P.; Davis, A.C.; Elder, B. Screened scalar fields in hydrogen and muonium. Phys. Rev. D 2023, 107, 044008. [Google Scholar] [CrossRef]
- Cronenberg, G.; Brax, P.; Filter, H.; Geltenbort, P.; Jenke, T.; Pignol, G.; Pitschmann, M.; Thalhammer, M.; Abele, H. Acoustic Rabi oscillations between gravitational quantum states and impact on symmetron dark energy. Nat. Phys. 2018, 14, 1022–1026. [Google Scholar] [CrossRef] [Green Version]
- Decca, R.S.; Lopez, D.; Chan, H.B.; Fischbach, E.; Krause, D.E.; Jamell, C.R. Constraining new forces in the Casimir regime using the isoelectronic technique. Phys. Rev. Lett. 2005, 94, 240401. [Google Scholar] [CrossRef] [Green Version]
- Decca, R.S.; Lopez, D.; Fischbach, E.; Krause, D.E. Measurement of the Casimir Force between Dissimilar Metals. Phys. Rev. Lett. 2003, 91, 050402. [Google Scholar] [CrossRef] [Green Version]
- Almasi, A.; Brax, P.; Iannuzzi, D.; Sedmik, R.I.P. Force sensor for chameleon and Casimir force experiments with parallel-plate configuration. Phys. Rev. 2015, D91, 102002. [Google Scholar] [CrossRef] [Green Version]
- Sedmik, R.; Brax, P. Status Report and first Light from Cannex: Casimir Force Measurements between flat parallel Plates. In Journal of Physics: Conference Series, Proceedings of the 10th International Conference on Precision Physics of Simple Atomic Systems (PSAS 2018), Vienna, Austria, 14–18 May 2018; IOP Publishing Ltd.: Bristol, UK, 2018; Volume 1138, p. 012014. [Google Scholar] [CrossRef]
- Brax, P.; van de Bruck, C.; Davis, A.C.; Shaw, D.J.; Iannuzzi, D. Tuning the Mass of Chameleon Fields in Casimir Force Experiments. Phys. Rev. Lett. 2010, 104, 241101. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Adelberger, E.G. Sub-millimeter tests of the gravitational inverse square law. In Proceedings of the 2nd Meeting on CPT and Lorentz Symmetry, Bloomington, IN, USA, 15–18 August 2002; pp. 9–15. [Google Scholar] [CrossRef] [Green Version]
- Brax, P.; Davis, A.C.; Elder, B. Casimir Tests of Scalar-Tensor Theories. Phys. Rev. D 2022, 107, 084025. [Google Scholar] [CrossRef]
- Sparnaay, M.J. Measurements of attractive forces between flat plates. Physica 1958, 24, 751–764. [Google Scholar] [CrossRef]
- van Blokland, P.H.G.M.; Overbeek, J.T.G. van der Waals forces between objects covered with a chromium layer. J. Chem. Soc. Faraday Trans. 1 1978, 74, 2637–2651. [Google Scholar] [CrossRef]
- Lamoreaux, S.K. Demonstration of the Casimir force in the 0.6 to 6 micrometers range. Phys. Rev. Lett. 1997, 78, 5–8, Erratum in Phys. Rev. Lett. 1998, 81, 5475. [Google Scholar] [CrossRef] [Green Version]
- Decca, R.S.; Lopez, D.; Fischbach, E.; Klimchitskaya, G.L.; Krause, D.E.; Mostepanenko, V.M. Precise comparison of theory and new experiment for the Casimir force leads to stronger constraints on thermal quantum effects and long-range interactions. Annals Phys. 2005, 318, 37–80. [Google Scholar] [CrossRef] [Green Version]
- Mohideen, U.; Roy, A. Precision measurement of the Casimir force from 0.1 to 0.9 micrometers. Phys. Rev. Lett. 1998, 81, 4549–4552. [Google Scholar] [CrossRef] [Green Version]
- Harris, B.W.; Chen, F.; Mohideen, U. Precision measurement of the Casimir force using gold surfaces. Phys. Rev. A 2000, 62, 052109. [Google Scholar] [CrossRef] [Green Version]
- Chen, Y.J.; Tham, W.K.; Krause, D.E.; Lopez, D.; Fischbach, E.; Decca, R.S. Stronger Limits on Hypothetical Yukawa Interactions in the 30-8000 nm Range. Phys. Rev. Lett. 2016, 116, 221102. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bressi, G.; Carugno, G.; Onofrio, R.; Ruoso, G. Measurement of the Casimir force between parallel metallic surfaces. Phys. Rev. Lett. 2002, 88, 041804. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zou, J.; Marcet, Z.; Rodriguez, A.W.; Reid, M.T.H.; McCauley, A.P.; Kravchenko, I.I.; Lu, T.; Bao, Y.; Johnson, S.G.; Chan, H.B. Casimir forces on a silicon micromechanical chip. Nat. Commun. 2013, 4, 1845. [Google Scholar] [CrossRef] [Green Version]
- Neto, P.A.M.; Lambrecht, A.; Reynaud, S. Roughness correction to the Casimir force: Beyond the Proximity Force Approximation. EPL (Europhys. Lett.) 2005, 69, 924–930. [Google Scholar] [CrossRef] [Green Version]
- Rodrigues, R.B.; Neto, P.A.M.; Lambrecht, A.; Reynaud, S. Lateral Casimir Force beyond the Proximity-Force Approximation. Phys. Rev. Lett. 2006, 96, 100402. [Google Scholar] [CrossRef]
- Lambrecht, A.; Reynaud, S. Casimir force between metallic mirrors. Eur. Phys. J. D 2000, 8, 309–318. [Google Scholar] [CrossRef] [Green Version]
- Reynaud, S.; Lambrecht, A. 407Casimir forces and vacuum energy. In Quantum Optics and Nanophotonics; Oxford University Press: Oxford, UK, 2017. [Google Scholar] [CrossRef]
- Dalvit, D.A.R.; Onofrio, R. On the use of the proximity force approximation for deriving limits to short-range gravitational-like interactions from sphere-plane Casimir force experiments. Phys. Rev. D 2009, 80, 064025. [Google Scholar] [CrossRef] [Green Version]
- Krause, D.E.; Decca, R.S.; Lopez, D.; Fischbach, E. Experimental Investigation of the Casimir Force beyond the Proximity-Force Approximation. Phys. Rev. Lett. 2007, 98, 050403. [Google Scholar] [CrossRef]
- Brax, P.; van de Bruck, C.; Davis, A.C.; Mota, D.F.; Shaw, D.J. Detecting chameleons through Casimir force measurements. Phys. Rev. 2007, D76, 124034. [Google Scholar] [CrossRef] [Green Version]
- Brax, P.; Davis, A.C. Casimir, Gravitational and Neutron Tests of Dark Energy. Phys. Rev. D 2015, 91, 063503. [Google Scholar] [CrossRef] [Green Version]
- Upadhye, A.; Gubser, S.S.; Khoury, J. Unveiling chameleons in tests of gravitational inverse-square law. Phys. Rev. D 2006, 74, 104024. [Google Scholar] [CrossRef] [Green Version]
- Upadhye, A. Dark energy fifth forces in torsion pendulum experiments. Phys. Rev. D 2012, 86, 102003. [Google Scholar] [CrossRef] [Green Version]
- Elder, B.; Khoury, J.; Haslinger, P.; Jaffe, M.; Müller, H.; Hamilton, P. Chameleon Dark Energy and Atom Interferometry. Phys. Rev. D 2016, 94, 044051. [Google Scholar] [CrossRef] [Green Version]
- Koyama, K.; Sakstein, J. Astrophysical probes of the Vainshtein mechanism: Stars and galaxies. Phys. Rev. D 2015, 91, 124066. [Google Scholar] [CrossRef] [Green Version]
- Saito, R.; Yamauchi, D.; Mizuno, S.; Gleyzes, J.; Langlois, D. Modified gravity inside astrophysical bodies. J. Cosmol. Astropart. Phys. 2015, 2015, 008. [Google Scholar] [CrossRef] [Green Version]
- Gleyzes, J.; Langlois, D.; Piazza, F.; Vernizzi, F. Exploring gravitational theories beyond Horndeski. J. Cosmol. Astropart. Phys. 2015, 2015, 018. [Google Scholar] [CrossRef] [Green Version]
- Sakstein, J. Hydrogen Burning in Low Mass Stars Constrains Scalar-Tensor Theories of Gravity. Phys. Rev. Lett. 2015, 115, 201101. [Google Scholar] [CrossRef] [Green Version]
- Sakstein, J. Testing gravity using dwarf stars. Phys. Rev. D 2015, 92, 124045. [Google Scholar] [CrossRef] [Green Version]
- Ségransan, D.; Delfosse, X.; Forveille, T.; Beuzit, J.L.; Udry, S.; Perrier, C.; Mayor, M. Accurate masses of very low mass stars. III. 16 new or improved masses. Astron. Astrophys. 2000, 364, 665–673. [Google Scholar]
- Paxton, B.; Bildsten, L.; Dotter, A.; Herwig, F.; Lesaffre, P.; Timmes, F. Modules for Experiments in Stellar Astrophysics (MESA). Astrophys. J. Suppl. Ser. 2011, 192, 3. [Google Scholar] [CrossRef]
- Paxton, B.; Cantiello, M.; Arras, P.; Bildsten, L.; Brown, E.F.; Dotter, A.; Mankovich, C.; Montgomery, M.H.; Stello, D.; Timmes, F.X.; et al. Modules for Experiments in Stellar Astrophysics (MESA): Planets, Oscillations, Rotation, and Massive Stars. Astrophys. J. Suppl. Ser. 2013, 208, 4. [Google Scholar] [CrossRef] [Green Version]
- Paxton, B.; Marchant, P.; Schwab, J.; Bauer, E.B.; Bildsten, L.; Cantiello, M.; Dessart, L.; Farmer, R.; Hu, H.; Langer, N.; et al. Modules for Experiments in Stellar Astrophysics (MESA): Binaries, Pulsations, and Explosions. Astrophys. J. Suppl. Ser. 2015, 220, 15. [Google Scholar] [CrossRef]
- Paxton, B.; Schwab, J.; Bauer, E.B.; Bildsten, L.; Blinnikov, S.; Duffell, P.; Farmer, R.; Goldberg, J.A.; Marchant, P.; Sorokina, E.; et al. Modules for Experiments in Stellar Astrophysics (MESA): Convective Boundaries, Element Diffusion, and Massive Star Explosions. Astrophys. J. Suppl. Ser. 2018, 234, 34. [Google Scholar] [CrossRef]
- Paxton, B.; Smolec, R.; Schwab, J.; Gautschy, A.; Bildsten, L.; Cantiello, M.; Dotter, A.; Farmer, R.; Goldberg, J.A.; Jermyn, A.S.; et al. Modules for Experiments in Stellar Astrophysics (MESA): Pulsating Variable Stars, Rotation, Convective Boundaries, and Energy Conservation. Astrophys. J. Suppl. Ser. 2019, 243, 10. [Google Scholar] [CrossRef]
- Jermyn, A.S.; Bauer, E.B.; Schwab, J.; Farmer, R.; Ball, W.H.; Bellinger, E.P.; Dotter, A.; Joyce, M.; Marchant, P.; Mombarg, J.S.G.; et al. Modules for Experiments in Stellar Astrophysics (MESA): Time-Dependent Convection, Energy Conservation, Automatic Differentiation, and Infrastructure. arXiv 2022, arXiv:2208.03651. [Google Scholar] [CrossRef]
- Chang, P.; Hui, L. Stellar Structure and Tests of Modified Gravity. Astrophys. J. 2011, 732, 25. [Google Scholar] [CrossRef] [Green Version]
- Saltas, I.D.; Lopes, I. Obtaining Precision Constraints on Modified Gravity with Helioseismology. Phys. Rev. Lett. 2019, 123, 091103. [Google Scholar] [CrossRef] [Green Version]
- Saltas, I.D.; Christensen-Dalsgaard, J. Searching for dark energy with the Sun. Astron. Astrophys. 2022, 667, A115. [Google Scholar] [CrossRef]
- Jain, B.; Vikram, V.; Sakstein, J. Astrophysical Tests of Modified Gravity: Constraints from Distance Indicators in the Nearby Universe. Astrophys. J. 2013, 779, 39. [Google Scholar] [CrossRef] [Green Version]
- Sakstein, J. Stellar oscillations in modified gravity. Phys. Rev. D 2013, 88, 124013. [Google Scholar] [CrossRef] [Green Version]
- Desmond, H.; Sakstein, J.; Jain, B. Five percent measurement of the gravitational constant in the Large Magellanic Cloud. Phys. Rev. D 2021, 103, 024028. [Google Scholar] [CrossRef]
- Desmond, H.; Jain, B.; Sakstein, J. Local resolution of the Hubble tension: The impact of screened fifth forces on the cosmic distance ladder. Phys. Rev. D 2019, 100, 043537. [Google Scholar] [CrossRef] [Green Version]
- Desmond, H.; Sakstein, J. Screened fifth forces lower the TRGB-calibrated Hubble constant too. Phys. Rev. D 2020, 102, 023007. [Google Scholar] [CrossRef]
- Sakstein, J.; Desmond, H.; Jain, B. Screened fifth forces mediated by dark matter-baryon interactions: Theory and astrophysical probes. Phys. Rev. D 2019, 100, 104035. [Google Scholar] [CrossRef] [Green Version]
- Högås, M.; Mörtsell, E. Impact of symmetron screening on the Hubble tension: New constraints using cosmic distance ladder data. Phys. Rev. D 2023, 108, 024007. [Google Scholar] [CrossRef]
- Sakstein, J.; Kenna-Allison, M.; Koyama, K. Stellar pulsations in beyond Horndeski gravity theories. J. Cosmol. Astropart. Phys. 2017, 2017, 007. [Google Scholar] [CrossRef] [Green Version]
- Zhao, G.B.; Li, B.; Koyama, K. Testing Gravity Using the Environmental Dependence of Dark Matter Halos. Phys. Rev. Lett. 2011, 107, 071303. [Google Scholar] [CrossRef] [Green Version]
- Schmidt, F. Dynamical masses in modified gravity. Phys. Rev. D 2010, 81, 103002. [Google Scholar] [CrossRef] [Green Version]
- Cabré, A.; Vikram, V.; Zhao, G.B.; Jain, B.; Koyama, K. Astrophysical tests of gravity: A screening map of the nearby universe. J. Cosmol. Astropart. Phys. 2012, 2012, 034. [Google Scholar] [CrossRef] [Green Version]
- Yang, X.; Mo, H.J.; van den Bosch, F.C.; Pasquali, A.; Li, C.; Barden, M. Galaxy Groups in the SDSS DR4. I. The Catalog and Basic Properties. Astrophys. J. 2007, 671, 153–170. [Google Scholar] [CrossRef] [Green Version]
- Abell, G.O.; Corwin, H.G., Jr.; Olowin, R.P. A Catalog of Rich Clusters of Galaxies. Astrophys. J. Suppl. Ser. 1989, 70, 1. [Google Scholar] [CrossRef] [Green Version]
- Ebeling, H.; Voges, W.; Bohringer, H.; Edge, A.C.; Huchra, J.P.; Briel, U.G. Properties of the X-ray-brightest Abell-type clusters of galaxies (XBACs) from ROSAT All-Sky Survey data—I. The sample. Mon. Not. R. Astron. Soc. 1996, 281, 799–829. [Google Scholar] [CrossRef] [Green Version]
- Karachentsev, I.D.; Karachentseva, V.E.; Huchtmeier, W.K.; Makarov, D.I. A Catalog of Neighboring Galaxies. Astron. J. 2004, 127, 2031–2068. [Google Scholar] [CrossRef]
- Lavaux, G.; Hudson, M.J. The 2M++ galaxy redshift catalogue. Mon. Not. R. Astron. Soc. 2011, 416, 2840–2856. [Google Scholar] [CrossRef]
- Zhao, G.B.; Li, B.; Koyama, K. N-body simulations for f(R) gravity using a self-adaptive particle-mesh code. Phys. Rev. D 2011, 83, 044007. [Google Scholar] [CrossRef] [Green Version]
- Desmond, H.; Ferreira, P.G.; Lavaux, G.; Jasche, J. Reconstructing the gravitational field of the local Universe. Mon. Not. R. Astron. Soc. 2018, 474, 3152–3161. [Google Scholar] [CrossRef] [Green Version]
- Behroozi, P.S.; Wechsler, R.H.; Wu, H.Y. The ROCKSTAR Phase-space Temporal Halo Finder and the Velocity Offsets of Cluster Cores. Astrophys. J. 2013, 762, 109. [Google Scholar] [CrossRef] [Green Version]
- Skillman, S.W.; Warren, M.S.; Turk, M.J.; Wechsler, R.H.; Holz, D.E.; Sutter, P.M. Dark Sky Simulations: Early Data Release. arXiv 2014, arXiv:1407.2600. [Google Scholar]
- Lehmann, B.V.; Mao, Y.Y.; Becker, M.R.; Skillman, S.W.; Wechsler, R.H. The Concentration Dependence of the Galaxy-Halo Connection: Modeling Assembly Bias with Abundance Matching. Astrophys. J. 2017, 834, 37. [Google Scholar] [CrossRef]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. A Universal Density Profile from Hierarchical Clustering. Astrophys. J. 1997, 490, 493–508. [Google Scholar] [CrossRef]
- Cole, S.; Lacey, C. The structure of dark matter haloes in hierarchical clustering models. Mon. Not. R. Astron. Soc. 1996, 281, 716. [Google Scholar] [CrossRef] [Green Version]
- Jasche, J.; Wandelt, B.D. Bayesian inference from photometric redshift surveys. Mon. Not. R. Astron. Soc. 2012, 425, 1042–1056. [Google Scholar] [CrossRef] [Green Version]
- Jasche, J.; Wandelt, B.D. Bayesian physical reconstruction of initial conditions from large-scale structure surveys. Mon. Not. R. Astron. Soc. 2013, 432, 894–913. [Google Scholar] [CrossRef]
- Jasche, J.; Kitaura, F.S.; Wandelt, B.D.; Enßlin, T.A. Bayesian power-spectrum inference for large-scale structure data. Mon. Not. R. Astron. Soc. 2010, 406, 60–85. [Google Scholar] [CrossRef] [Green Version]
- Jasche, J.; Leclercq, F.; Wandelt, B.D. Past and present cosmic structure in the SDSS DR7 main sample. J. Cosmol. Astropart. Phys. 2015, 2015, 036. [Google Scholar] [CrossRef] [Green Version]
- Lavaux, G.; Jasche, J. Unmasking the masked Universe: The 2M++ catalogue through Bayesian eyes. Mon. Not. R. Astron. Soc. 2016, 455, 3169–3179. [Google Scholar] [CrossRef] [Green Version]
- Shao, S.; Li, B.; Cautun, M.; Wang, H.; Wang, J. Screening maps of the local Universe I - Methodology. Mon. Not. R. Astron. Soc. 2019, 489, 4912–4925. [Google Scholar] [CrossRef]
- Li, B.; Zhao, G.B.; Koyama, K. Exploring Vainshtein mechanism on adaptively refined meshes. J. Cosmol. Astropart. Phys. 2013, 2013, 023. [Google Scholar] [CrossRef] [Green Version]
- Li, B.; Barreira, A.; Baugh, C.M.; Hellwing, W.A.; Koyama, K.; Pascoli, S.; Zhao, G.B. Simulating the quartic Galileon gravity model on adaptively refined meshes. J. Cosmol. Astropart. Phys. 2013, 2013, 012. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Mo, H.J.; Yang, X.; Jing, Y.P.; Lin, W.P. ELUCID—Exploring the Local Universe with the Reconstructed Initial Density Field. I. Hamiltonian Markov Chain Monte Carlo Method with Particle Mesh Dynamics. Astrophys. J. 2014, 794, 94. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Mo, H.J.; Yang, X.; Zhang, Y.; Shi, J.; Jing, Y.P.; Liu, C.; Li, S.; Kang, X.; Gao, Y. ELUCID—Exploring the Local Universe with ReConstructed Initial Density Field III: Constrained Simulation in the SDSS Volume. Astrophys. J. 2016, 831, 164. [Google Scholar] [CrossRef] [Green Version]
- Naidoo, K.; Hellwing, W.; Bilicki, M.; Libeskind, N.; Pfeifer, S.; Hoffman, Y. Constrained simulations of the local Universe with Modified Gravity. arXiv 2022, arXiv:2209.14386. [Google Scholar] [CrossRef]
- Kourkchi, E.; Tully, R.B.; Eftekharzadeh, S.; Llop, J.; Courtois, H.M.; Guinet, D.; Dupuy, A.; Neill, J.D.; Seibert, M.; Andrews, M.; et al. Cosmicflows-4: The Catalog of ∼10,000 Tully-Fisher Distances. Astrophys. J. 2020, 902, 145. [Google Scholar] [CrossRef]
- Doumler, T.; Gottlöber, S.; Hoffman, Y.; Courtois, H. Reconstructing cosmological initial conditions from galaxy peculiar velocities—III. Constrained simulations. Mon. Not. R. Astron. Soc. 2013, 430, 912–923. [Google Scholar] [CrossRef] [Green Version]
- Zaroubi, S.; Hoffman, Y.; Fisher, K.B.; Lahav, O. Wiener Reconstruction of the Large-Scale Structure. Astrophys. J. 1995, 449, 446. [Google Scholar] [CrossRef]
- Doumler, T.; Hoffman, Y.; Courtois, H.; Gottlöber, S. Reconstructing cosmological initial conditions from galaxy peculiar velocities—I. Reverse Zeldovich Approximation. Mon. Not. R. Astron. Soc. 2013, 430, 888–901. [Google Scholar] [CrossRef] [Green Version]
- Winther, H.A.; Koyama, K.; Manera, M.; Wright, B.S.; Zhao, G.B. COLA with scale-dependent growth: Applications to screened modified gravity models. J. Cosmol. Astropart. Phys. 2017, 2017, 006. [Google Scholar] [CrossRef] [Green Version]
- Tassev, S.; Zaldarriaga, M.; Eisenstein, D.J. Solving large scale structure in ten easy steps with COLA. J. Cosmol. Astropart. Phys. 2013, 2013, 036. [Google Scholar] [CrossRef] [Green Version]
- Fasiello, M.; Vlah, Z. Screening in perturbative approaches to LSS. Phys. Lett. B 2017, 773, 236–241. [Google Scholar] [CrossRef]
- Jain, B.; VanderPlas, J. Tests of modified gravity with dwarf galaxies. J. Cosmol. Astropart. Phys. 2011, 2011, 032. [Google Scholar] [CrossRef] [Green Version]
- Vikram, V.; Cabré, A.; Jain, B.; VanderPlas, J.T. Astrophysical tests of modified gravity: The morphology and kinematics of dwarf galaxies. J. Cosmol. Astropart. Phys. 2013, 2013, 020. [Google Scholar] [CrossRef] [Green Version]
- Giovanelli, R.; Haynes, M.P.; Kent, B.R.; Perillat, P.; Saintonge, A.; Brosch, N.; Catinella, B.; Hoffman, G.L.; Stierwalt, S.; Spekkens, K.; et al. The Arecibo Legacy Fast ALFA Survey. 1. Science goals, survey design and strategy. Astron. J. 2005, 130, 2598–2612. [Google Scholar] [CrossRef]
- Abazajian, K.N.; Adelman-McCarthy, J.K.; Agüeros, M.A.; Allam, S.S.; Prieto, C.A.; An, D.; Anderson, K.S.; Anderson, S.F.; Annis, J.; Bahcall, N.A.; et al. The Seventh Data Release of the Sloan Digital Sky Survey. Astrophys. J. Suppl. 2009, 182, 543–558. [Google Scholar] [CrossRef]
- Desmond, H.; Ferreira, P.G.; Lavaux, G.; Jasche, J. Fifth force constraints from the separation of galaxy mass components. Phys. Rev. D 2018, 98, 064015. [Google Scholar] [CrossRef] [Green Version]
- Desmond, H.; Ferreira, P.G.; Lavaux, G.; Jasche, J. Fifth force constraints from galaxy warps. Phys. Rev. D 2018, 98, 083010. [Google Scholar] [CrossRef] [Green Version]
- Desmond, H.; Ferreira, P.G.; Lavaux, G.; Jasche, J. The Fifth Force in the Local Cosmic Web. Mon. Not. R. Astron. Soc. 2019, 483, L64–L68. [Google Scholar] [CrossRef]
- Desmond, H.; Ferreira, P.G. Galaxy morphology rules out astrophysically relevant Hu-Sawicki f (R ) gravity. Phys. Rev. D 2020, 102, 104060. [Google Scholar] [CrossRef]
- Bartlett, D.J.; Desmond, H.; Ferreira, P.G. Calibrating galaxy formation effects in galactic tests of fundamental physics. Phys. Rev. D 2021, 103, 123502. [Google Scholar] [CrossRef]
- Asvathaman, A.; Heyl, J.S.; Hui, L. Eötvös experiments with supermassive black holes. Mon. Not. R. Astron. Soc. 2017, 465, 3261–3266. [Google Scholar] [CrossRef] [Green Version]
- Bartlett, D.J.; Desmond, H.; Ferreira, P.G. Constraints on Galileons from the positions of supermassive black holes. Phys. Rev. D 2021, 103, 023523. [Google Scholar] [CrossRef]
- Bartlett, D.J.; Desmond, H.; Devriendt, J.; Ferreira, P.G.; Slyz, A. Spatially offset black holes in the Horizon-AGN simulation and comparison to observations. Mon. Not. R. Astron. Soc. 2021, 500, 4639–4657. [Google Scholar] [CrossRef]
- Khoury, J. Les Houches Lectures on Physics Beyond the Standard Model of Cosmology. arXiv 2013, arXiv:1312.2006. [Google Scholar]
- Murphy, T.W., Jr.; Adelberger, E.G.; Battat, J.B.R.; Hoyle, C.D.; Johnson, N.H.; McMillan, R.J.; Stubbs, C.W.; Swanson, H.E. APOLLO: Millimeter lunar laser ranging. Class. Quantum Gravity 2012, 29, 184005. [Google Scholar] [CrossRef]
- Schmidt, F.; Lima, M.V.; Oyaizu, H.; Hu, W. Non-linear Evolution of f(R) Cosmologies III: Halo Statistics. Phys. Rev. D 2009, 79, 083518. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Hu, W. Chameleon Halo Modeling in f(R) Gravity. Phys. Rev. D 2011, 84, 084033. [Google Scholar] [CrossRef] [Green Version]
- Pourhasan, R.; Afshordi, N.; Mann, R.B.; Davis, A.C. Chameleon Gravity, Electrostatics, and Kinematics in the Outer Galaxy. J. Cosmol. Astropart. Phys. 2011, 12, 005. [Google Scholar] [CrossRef] [Green Version]
- Lombriser, L.; Koyama, K.; Zhao, G.B.; Li, B. Chameleon f(R) gravity in the virialized cluster. Phys. Rev. D 2012, 85, 124054. [Google Scholar] [CrossRef] [Green Version]
- Lombriser, L.; Li, B.; Koyama, K.; Zhao, G.B. Modeling halo mass functions in chameleon f(R) gravity. Phys. Rev. D 2013, 87, 123511. [Google Scholar] [CrossRef] [Green Version]
- Lombriser, L.; Koyama, K.; Li, B. Halo modelling in chameleon theories. J. Cosmol. Astropart. Phys. 2014, 03, 021. [Google Scholar] [CrossRef] [Green Version]
- Shi, D.; Li, B.; Han, J.; Gao, L.; Hellwing, W.A. Exploring the liminality: Properties of haloes and subhaloes in borderline f(R) gravity. Mon. Not. R. Astron. Soc. 2015, 452, 3179–3191. [Google Scholar] [CrossRef] [Green Version]
- Mitchell, M.A.; Arnold, C.; He, J.H.; Li, B. A general framework to test gravity using galaxy clusters II: A universal model for the halo concentration in f(R) gravity. Mon. Not. R. Astron. Soc. 2019, 487, 1410–1425. [Google Scholar] [CrossRef]
- Barreira, A.; Li, B.; Hellwing, W.A.; Lombriser, L.; Baugh, C.M.; Pascoli, S. Halo model and halo properties in Galileon gravity cosmologies. J. Cosmol. Astropart. Phys. 2014, 2014, 029. [Google Scholar] [CrossRef] [Green Version]
- Mitchell, M.A.; Hernández-Aguayo, C.; Arnold, C.; Li, B. A general framework to test gravity using galaxy clusters IV: Cluster and halo properties in DGP gravity. Mon. Not. R. Astron. Soc. 2021, 508, 4140–4156. [Google Scholar] [CrossRef]
- Pizzuti, L.; Saltas, I.D.; Umetsu, K.; Sartoris, B. Probing vainsthein-screening gravity with galaxy clusters using internal kinematics and strong and weak lensing. Mon. Not. Roy. Astron. Soc. 2022, 512, 4280–4290. [Google Scholar] [CrossRef]
- Falck, B.; Koyama, K.; Zhao, G.B. Cosmic Web and Environmental Dependence of Screening: Vainshtein vs. Chameleon. J. Cosmol. Astropart. Phys. 2015, 2015, 049. [Google Scholar] [CrossRef] [Green Version]
- Diemer, B.; Kravtsov, A.V. Dependence of the outer density profiles of halos on their mass accretion rate. Astrophys. J. 2014, 789, 1. [Google Scholar] [CrossRef]
- More, S.; Miyatake, H.; Takada, M.; Diemer, B.; Kravtsov, A.V.; Dalal, N.K.; More, A.; Murata, R.; Melbaum, R.; Rozo, E.; et al. Detection of the Splashback Radius and Halo Assembly bias of Massive Galaxy Clusters. Astrophys. J. 2016, 825, 39. [Google Scholar] [CrossRef] [Green Version]
- Baxter, E.; Chang, C.; Jain, B.; Adhikari, S.; Dalal, N.; Kravtsov, A.; More, S.; Rozo, E.; Rykoff, E.; Sheth, R.K. The Halo Boundary of Galaxy Clusters in the SDSS. Astrophys. J. 2017, 841, 18. [Google Scholar] [CrossRef] [Green Version]
- Chang, C.; Baxter, E.; Jain, B.; Sánchez, C.; Adhikari, S.; Varga, T.N.; Fang, Y.; Rozo, E.; Rykoff, E.S.; Kravtsov, A.; et al. The Splashback Feature around DES Galaxy Clusters: Galaxy Density and Weak Lensing Profiles. Astrophys. J. 2018, 864, 83. [Google Scholar] [CrossRef] [Green Version]
- Shin, T.H.; Adhikari, S.; Baxter, E.J.; Chang, C.; Jain, B.; Battaglia, N.; Bleem, L.; Bocquet, S.; DeRose, J.; Gruen, D.; et al. Measurement of the Splashback Feature around SZ-selected Galaxy Clusters with DES, SPT and ACT. Mon. Not. R. Astron. Soc. 2018, 487, 2900–2918. [Google Scholar] [CrossRef] [Green Version]
- Umetsu, K.; Diemer, B. Lensing Constraints on the Mass Profile Shape and the Splashback Radius of Galaxy Clusters. Astrophys. J. 2017, 836, 231. [Google Scholar] [CrossRef]
- Contigiani, O.; Hoekstra, H.; Bahé, Y.M. Weak lensing constraints on splashback around massive clusters. Mon. Not. R. Astron. Soc. 2018, 485, 408–415. [Google Scholar] [CrossRef]
- Adhikari, S.; Sakstein, J.; Jain, B.; Dalal, N.; Li, B. Splashback in galaxy clusters as a probe of cosmic expansion and gravity. J. Cosmol. Astropart. Phys. 2018, 11, 033. [Google Scholar] [CrossRef] [Green Version]
- Fillmore, J.A.; Goldreich, P. Self-similar gravitational collapse in an expanding universe. Astrophys. J. 1984, 281, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Bertschinger, E. Self-similar secondary infall and accretion in an Einstein-de Sitter universe. Astrophys. J. Suppl. Ser. 1985, 58, 39–65. [Google Scholar] [CrossRef]
- Lithwick, Y.; Dalal, N. Self-Similar Solutions of Triaxial Dark Matter Halos. Astrophys. J. 2011, 734, 100. [Google Scholar] [CrossRef]
- Shi, X. The outer profile of dark matter haloes: An analytical approach. Mon. Not. R. Astron. Soc. 2016, 459, 3711–3720. [Google Scholar] [CrossRef] [Green Version]
- Amendola, L.; Appleby, S.; Avgoustidis, A.; Bacon, D.; Baker, T.; Baldi, M.; Bartolo, N.; Blanchard, A.; Bonvin, C.; Borgani, S.; et al. Cosmology and fundamental physics with the Euclid satellite. Living Rev. Relativ. 2018, 21, 2. [Google Scholar] [CrossRef] [Green Version]
- Gubitosi, G.; Piazza, F.; Vernizzi, F. The Effective Field Theory of Dark Energy. J. Cosmol. Astropart. Phys. 2013, 2013, 032. [Google Scholar] [CrossRef] [Green Version]
- Gleyzes, J.; Langlois, D.; Piazza, F.; Vernizzi, F. Essential Building Blocks of Dark Energy. J. Cosmol. Astropart. Phys. 2013, 2013, 025. [Google Scholar] [CrossRef] [Green Version]
- Creminelli, P.; Gleyzes, J.; Hui, L.; Simonović, M.; Vernizzi, F. Single-Field Consistency Relations of Large Scale Structure. Part III: Test of the Equivalence Principle. J. Cosmol. Astropart. Phys. 2014, 2014, 009. [Google Scholar] [CrossRef] [Green Version]
- Bellini, E.; Sawicki, I. Maximal freedom at minimum cost: Linear large-scale structure in general modifications of gravity. J. Cosmol. Astropart. Phys. 2014, 2014, 050. [Google Scholar] [CrossRef]
- Hu, B.; Raveri, M.; Frusciante, N.; Silvestri, A. Effective Field Theory of Cosmic Acceleration: An implementation in CAMB. Phys. Rev. D 2014, 89, 103530. [Google Scholar] [CrossRef] [Green Version]
- Raveri, M.; Hu, B.; Frusciante, N.; Silvestri, A. Effective Field Theory of Cosmic Acceleration: Constraining dark energy with CMB data. Phys. Rev. D 2014, 90, 043513. [Google Scholar] [CrossRef] [Green Version]
- Zumalacárregui, M.; Bellini, E.; Sawicki, I.; Lesgourgues, J.; Ferreira, P.G. hi_class: Horndeski in the Cosmic Linear Anisotropy Solving System. J. Cosmol. Astropart. Phys. 2017, 2017, 019. [Google Scholar] [CrossRef] [Green Version]
- Bellini, E.; Sawicki, I.; Zumalacárregui, M. hi_class: Background Evolution, Initial Conditions and Approximation Schemes. J. Cosmol. Astropart. Phys. 2020, 2020, 008. [Google Scholar] [CrossRef] [Green Version]
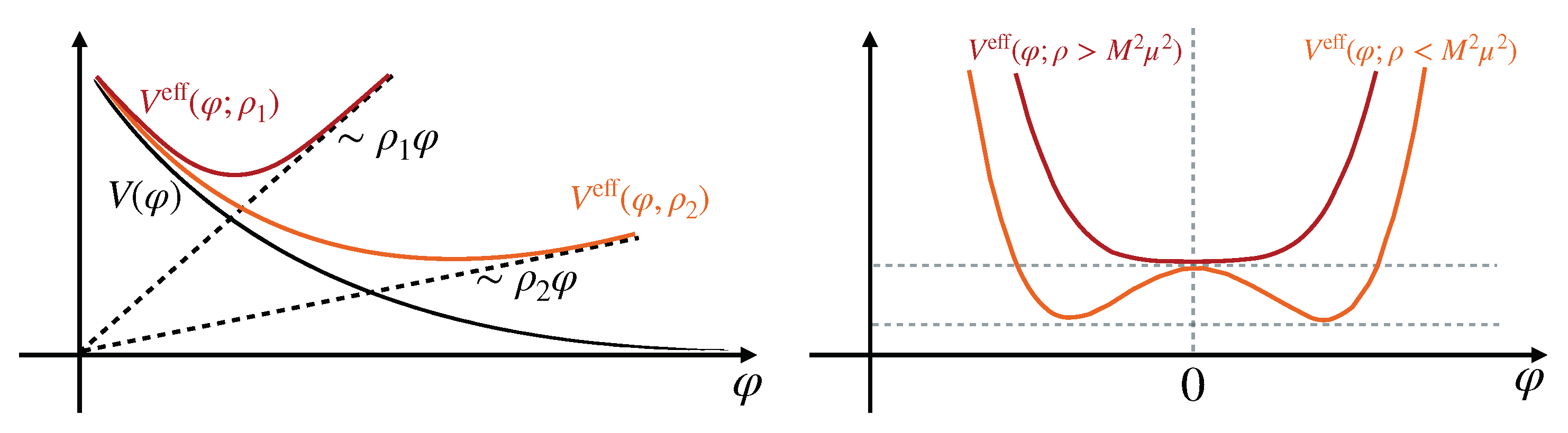

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vardanyan, V.; Bartlett, D.J. Modeling and Testing Screening Mechanisms in the Laboratory and in Space. Universe 2023, 9, 340. https://doi.org/10.3390/universe9070340
Vardanyan V, Bartlett DJ. Modeling and Testing Screening Mechanisms in the Laboratory and in Space. Universe. 2023; 9(7):340. https://doi.org/10.3390/universe9070340
Chicago/Turabian StyleVardanyan, Valeri, and Deaglan J. Bartlett. 2023. "Modeling and Testing Screening Mechanisms in the Laboratory and in Space" Universe 9, no. 7: 340. https://doi.org/10.3390/universe9070340
APA StyleVardanyan, V., & Bartlett, D. J. (2023). Modeling and Testing Screening Mechanisms in the Laboratory and in Space. Universe, 9(7), 340. https://doi.org/10.3390/universe9070340





