Recent Achievements of the ERNA Collaboration
Abstract
1. Introduction
2. Materials and Methods
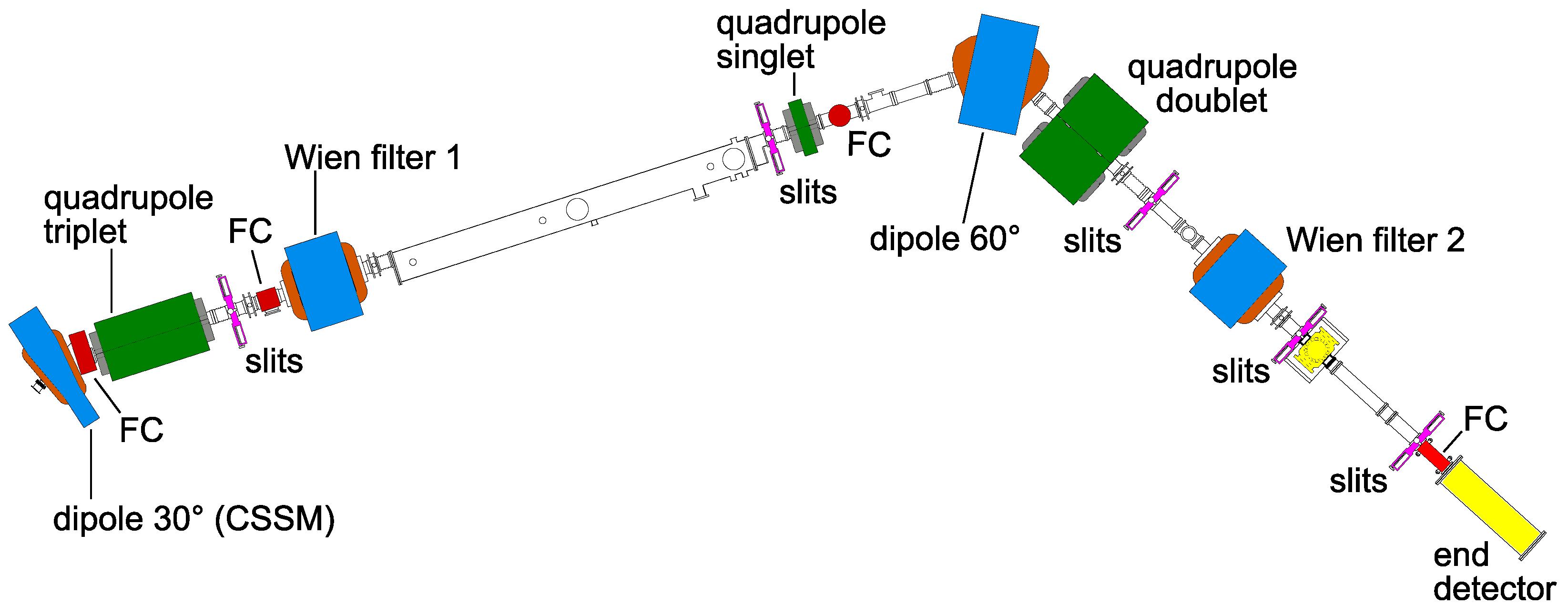
2.1. Recoil Mass Separator ERNA
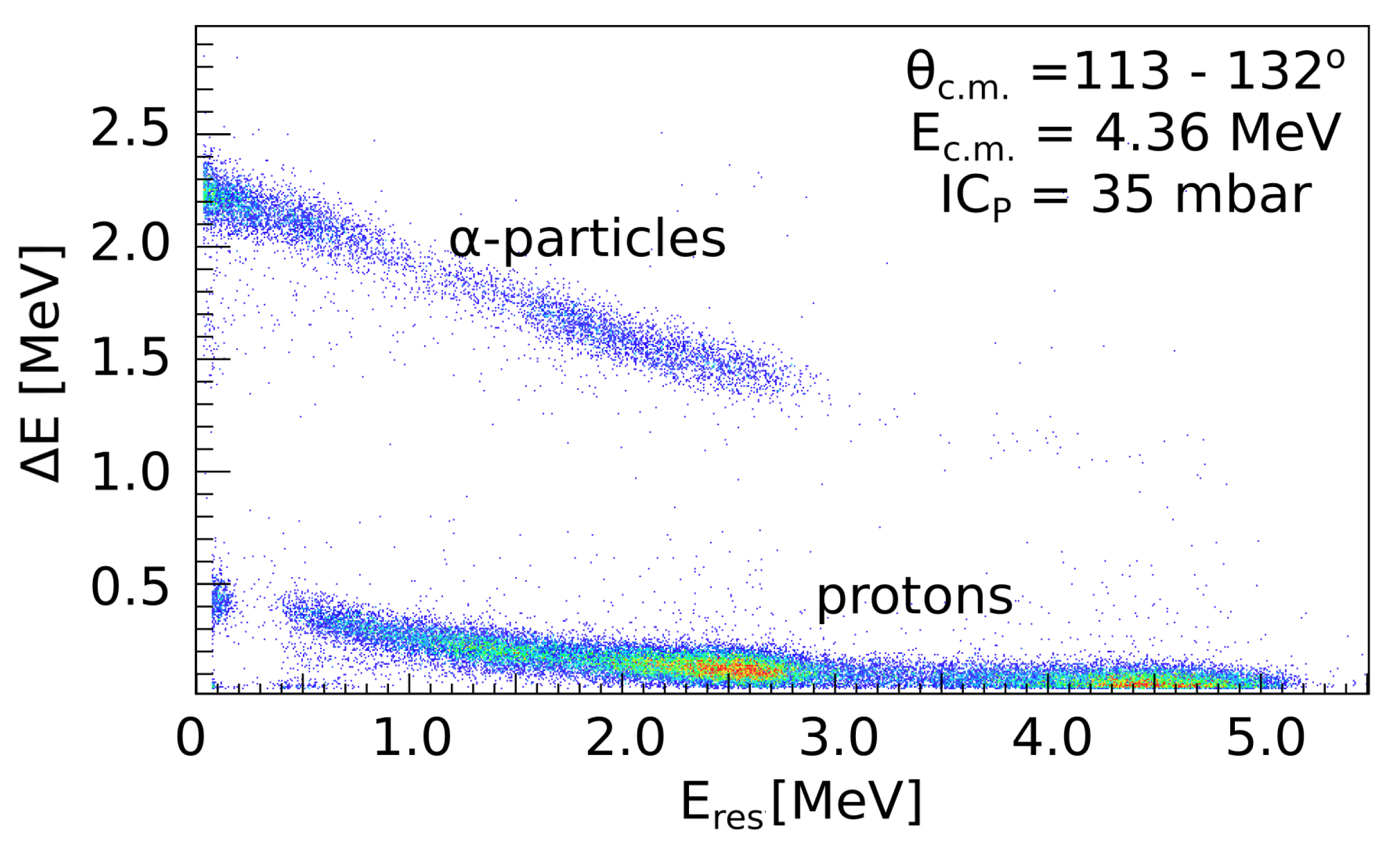
2.2. Charged Particle Spectroscopy
3. Results
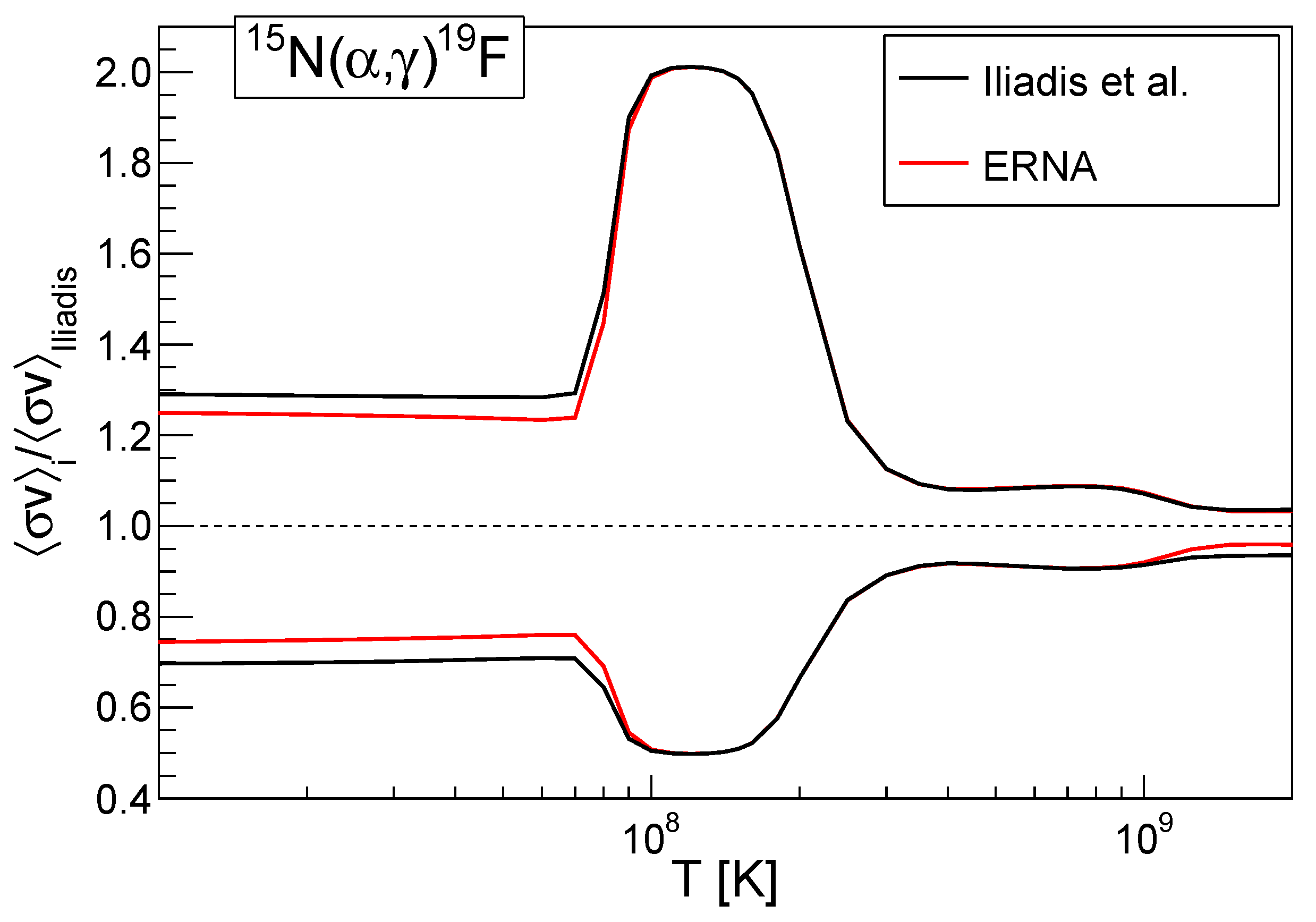
3.1. Measurement of the Reaction Cross Section
3.2. Measurement of the C+C Fusion Processes Cross Section
4. Discussion
5. Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Rolfs, C.E.; Rodney, W.S. Cauldrons in the Cosmos: Nuclear Astrophysics; The University of Chicago Press: Chicago, IL, USA, 1988. [Google Scholar]
- Rolfs, C.; Barnes, C. Radiative Capture Reactions in Nuclear Astrophysics. Annu. Rev. Nucl. Part. Sci. 1990, 40, 45–78. [Google Scholar] [CrossRef]
- Brune, C.R.; Davids, B. Radiative Capture Reactions in Astrophysics. Annu. Rev. Nucl. Part. Sci. 2015, 65, 87–112. [Google Scholar] [CrossRef]
- Di Leva, A.; De Cesare, M.; Schürmann, D.; De Cesare, N.; D’Onofrio, A.; Gialanella, L.; Kunz, R.; Imbriani, G.; Ordine, A.; Roca, V.; et al. Recoil separator ERNA: Measurement of 3He(α,γ)7Be. Nucl. Instr. Meth. A 2008, 595, 381–390. [Google Scholar] [CrossRef]
- Di Leva, A.; Imbriani, G.; Buompane, R.; Gialanella, L.; Best, A.; Cristallo, S.; De Cesare, M.; D’Onofrio, A.; Duarte, J.G.; Gasques, L.R.; et al. Measurement of 1323 and 1487 keV resonances in 15N(α,γ)19F with the recoil separator ERNA. Phys. Rev. C 2017, 95, 045803. [Google Scholar] [CrossRef]
- Zickefoose, J.; Leva, A.D.; Strieder, F.; Gialanella, L.; Imbriani, G.; Cesare, N.D.; Rolfs, C.; Schweitzer, J.; Spillane, T.; Straniero, O.; et al. Measurement of the 12C(12C,p)23Na cross section near the Gamow energy. Phys. Rev. C 2018, 97, 065806. [Google Scholar] [CrossRef]
- Terrasi, F.; Marzaioli, F.; Buompane, R.; Passariello, I.; Porzio, G.; Capano, M.; Helama, S.; Oinonen, M.; Nöjd, P.; Uusitalo, J.; et al. Can the 14C Production in 1055 CE be Affected by SN1054? Radiocarbon 2020, 62, 1403–1418. [Google Scholar] [CrossRef]
- Brandi, F.; Labate, L.; Rapagnani, D.; Buompane, R.; Leva, A.d.; Gialanella, L.; Gizzi, L.A. Optical and spectroscopic study of a supersonic flowing helium plasma: Energy transport in the afterglow. Sci. Rep. 2020, 10, 5087. [Google Scholar] [CrossRef]
- Buompane, R.; Di Leva, A.; Gialanella, L.; D’Onofrio, A.; De Cesare, M.; Duarte, J.G.; Fülöp, Z.; Gasques, L.R.; Gyürky, G.; Morales-Gallegos, L.; et al. Determination of the 7Be(p,γ)8B cross section at astrophysical energies using a radioactive 7Be ion beam. Phys. Lett. B 2021, 824, 136819. [Google Scholar] [CrossRef]
- Santonastaso, C.; Buompane, R.; Di Leva, A.; Morales-Gallegos, L.; Itaco, N.; Landi, G.; Neitzert, H.; Rapagnani, D.; Gialanella, L. Change in the 7Be half-life in different environments. Nuovo Cimento 2021, 100, 44. [Google Scholar]
- Ruiz, C.; Greife, U.; Hager, U. Recoil separators for radiative capture using radioactive ion beams. Eur. Phys. J. A 2014, 50, 99. [Google Scholar] [CrossRef]
- Schürmann, D.; Strieder, F.; Di Leva, A.; Gialanella, L.; De Cesare, N.; D’Onofrio, A.; Imbriani, G.; Klug, J.; Lubritto, C.; Ordine, A.; et al. Recoil separator ERNA: Charge state distribution, target density, beam heating, and longitudinal acceptance. Nucl. Instr. Meth. A 2004, 531, 428–434. [Google Scholar] [CrossRef]
- Gialanella, L.; Strieder, F.; Brand, K.; Campajola, L.; D’Onofrio, A.; Greife, U.; Huttel, E.; Petrazzuolo, F.; Roca, V.; Rolfs, C.; et al. A recoil separator for the measurement of radiative capture reactions. Nucl. Instr. Meth. A 1996, 376, 174–184. [Google Scholar] [CrossRef]
- Rogalla, D.; Theis, S.; Campajola, L.; D’Onofrio, A.; Gialanella, L.; Greife, U.; Imbriani, G.; Ordine, A.; Roca, V.; Rolfs, C.; et al. Recoil separator ERNA: Ion beam purification. Nucl. Instr. Meth. A 1999, 437, 266–273. [Google Scholar] [CrossRef]
- Gialanella, L.; Strieder, F.; Campajola, L.; D’Onofrio, A.; Greife, U.; Gyurky, G.; Imbriani, G.; Oliviero, G.; Ordine, A.; Roca, V.; et al. Absolute cross section of p(7Be,γ)8B using a novel approach. Eur. Phys. J. A 2000, 7, 303–305. [Google Scholar] [CrossRef]
- Rogalla, D.; Schürmann, D.; Strieder, F.; Aliotta, M.; DeCesare, N.; DiLeva, A.; Lubritto, C.; D’Onofrio, A.; Gialanella, L.; Imbriani, G.; et al. Recoil separator ERNA: Acceptances in angle and energy. Nucl. Instr. Meth. A 2003, 513, 573–578. [Google Scholar] [CrossRef]
- Gialanella, L.; Schürmann, D.; Strieder, F.; Di Leva, A.; De Cesare, N.; D’Onofrio, A.; Imbriani, G.; Klug, J.; Lubritto, C.; Ordine, A.; et al. Recoil separator ERNA: Gas target and beam suppression. Nucl. Instr. Meth. A 2004, 522, 432–438. [Google Scholar] [CrossRef]
- deBoer, R.J.; Görres, J.; Wiescher, M.; Azuma, R.E.; Best, A.; Brune, C.R.; Fields, C.E.; Jones, S.; Pignatari, M.; Sayre, D.; et al. The 12C(α,γ)16O reaction and its implications for stellar helium burning. Rev. Mod. Phys. 2017, 89, 035007. [Google Scholar] [CrossRef]
- Smith, M.S.; Blackmon, J.C.; Koehler, P.E.; Lewis, T.A.; McConnell, J.W.; Milner, W.T.; Pierce, D.E.; Shapira, D.; Bardayan, D.W.; Chen, A.A.; et al. Commissioning of the Daresbury recoil seperator for nuclear astrophysics measurements at the Holifield radioactive ion beam facility. In Stellar Evolution, Stellar Explosions and Galactic Chemical Evolution, Proceedings of the 2nd Oak Ridge Symposium on Atomic and Nuclear Astrophysics, Oak Ridge, Tennessee, 2–6 December 1997; Mezzacappa, A., Ed.; IOP Publishing Ltd.: Bristol, UK, 1998; p. 511. ISBN 9780750305556. [Google Scholar]
- Hutcheon, D.A.; Bishop, S.; Buchmann, L.; Chatterjee, M.L.; Chen, A.A.; D’Auria, J.M.; Engel, S.; Gigliotti, D.; Greife, U.; Hunter, D.; et al. The DRAGON facility for nuclear astrophysics at TRIUMF-ISAC: Design, construction and operation. Nucl. Instr. Meth. A 2003, 498, 190–210. [Google Scholar] [CrossRef]
- Couder, M.; Angulo, C.; Galster, W.; Graulich, J.S.; Leleux, P.; Lipnik, P.; Tabacaru, G.; Vanderbist, F. Performance of the ARES recoil separator for (p,γ) reaction measurements. Nucl. Instr. Meth. A 2003, 506, 26–34. [Google Scholar] [CrossRef]
- Ikeda, N.; Sagara, K.; Tsuruta, K.; Oba, H.; Ohta, T.; Noguchi, Y.; Ichikawa, K.; Miwa, Y.; Morinobu, S. Facilities for direct measurement of 12C(α,γ)16O reaction cross section at KUTL. Nucl. Phys. A 2003, 718, 558. [Google Scholar] [CrossRef]
- Meisel, Z.; Moran, M.; Gilardy, G.; Schmitt, J.; Seymour, C.; Couder, M. Energy acceptance of the St. George recoil separator. Nucl. Instr. Meth. A 2017, 850, 48–53. [Google Scholar] [CrossRef]
- Miskovich, S.A. Commissioning of the Separator for Capture Reactions in Astrophysics. Ph.D. Thesis, Michigan State University, East Lansing, MI, USA, 2021. [Google Scholar]
- Terrasi, F.; Rogalla, D.; De Cesare, N.; D’Onofrio, A.; Lubritto, C.; Marzaioli, F.; Passariello, I.; Rubino, M.; Sabbarese, C.; Casa, G.; et al. A new AMS facility in Caserta/Italy. Nucl. Instr. Meth. B 2007, 259, 14–17. [Google Scholar] [CrossRef]
- Gialanella, L.; Greife, U.; De Cesare, N.; D’Onofrio, A.; Romano, M.; Campajola, L.; Formicola, A.; Fulop, Z.; Gyurky, G.; Imbriani, G.; et al. Off-line production of a 7Be radioactive ion beam. Nucl. Instr. Meth. B 2002, 197, 150–154. [Google Scholar] [CrossRef]
- Limata, B.N.; Gialanella, L.; Leva, A.D.; Cesare, N.D.; D’Onofrio, A.; Gyurky, G.; Rolfs, C.; Romano, M.; Rogalla, D.; Rossi, C.; et al. 7Be radioactive beam production at CIRCE and its utilization in basic and applied physics. Nucl. Instr. Meth. B 2008, 266, 2117–2121. [Google Scholar] [CrossRef]
- Schürmann, D.; Di Leva, A.; Gialanella, L.; De Cesare, M.; De Cesare, N.; Imbriani, G.; D’Onofrio, A.; Romano, M.; Romoli, M.; Terrasi, F. A windowless hydrogen gas target for the measurement of 7Be(p, γ)8B with the recoil separator ERNA. Eur. Phys. J. A 2013, 49, 80. [Google Scholar] [CrossRef]
- Rapagnani, D.; Buompane, R.; Di Leva, A.; Gialanella, L.; Busso, M.; De Cesare, M.; De Stefano, G.; Duarte, J.; Gasques, L.; Morales-Gallegos, L.; et al. A supersonic jet target for the cross section measurement of the 12C(α,γ)16O reaction with the recoil mass separator ERNA. Nucl. Instr. Meth. B 2017, 407, 217–221. [Google Scholar] [CrossRef]
- Romoli, M.; Morales-Gallegos, L.; Aliotta, M.; Bruno, C.G.; Buompane, R.; D’Onofrio, A.; Davinson, T.; De Cesare, M.; Di Leva, A.; Di Meo, P.; et al. Development of a two-stage detection array for low-energy light charged particles in nuclear astrophysics applications. Eur. Phys. J. A 2018, 54, 142. [Google Scholar] [CrossRef]
- Morales-Gallegos, L.; Aliotta, M.; Bruno, C.; Buompane, R.; Davinson, T.; De Cesare, M.; Di Leva, A.; D’Onofrio, A.; Duarte, J.; Gasques, L.; et al. Reduction of deuterium content in carbon targets for 12C+12C reaction studies of astrophysical interest. Eur. Phys. J. A 2018, 54. [Google Scholar] [CrossRef]
- Brune, C.R.; Sayre, D.B. Energy deconvolution of cross-section measurements with an application to the 12C(α,γ)16O reaction. Nucl. Instr. Meth. A 2013, 698, 49–59. [Google Scholar] [CrossRef]
- Iliadis, C. Nuclear Physics of Stars; WILEY-VCH Verlag GmbH & Co. KGaA, Weinheim: Berlin, Germany, 2007. [Google Scholar]
- Schürmann, D.; Di Leva, A.; Gialanella, L.; Rogalla, D.; Strieder, F.; De Cesare, N.; D’Onofrio, A.; Imbriani, G.; Kunz, R.; Lubritto, C.; et al. First direct measurement of the total cross-section of 12C(α,γ)16O. Eur. Phys. J. A 2005, 26, 301–305. [Google Scholar] [CrossRef]
- Matei, C.; Buchmann, L.; Hannes, W.R.; Hutcheon, D.A.; Ruiz, C.; Brune, C.R.; Caggiano, J.; Chen, A.A.; D’Auria, J.; Laird, A.; et al. Measurement of the Cascade Transition via the First Excited State of 16O in the 12C(α,γ)16O Reaction, and Its S Factor in Stellar Helium Burning. Phis. Rev. Lett. 2006, 97, 242503. [Google Scholar] [CrossRef] [PubMed]
- Schürmann, D.; Di Leva, A.; Gialanella, L.; Kunz, R.; Strieder, F.; De Cesare, N.; De Cesare, M.; D’Onofrio, A.; Fortak, K.; Imbriani, G.; et al. Study of the 6.05 MeV cascade transition in 12C(α,γ)16O. Phys. Lett. B 2011, 703, 557–561. [Google Scholar] [CrossRef]
- Buompane, R.; De Cesare, N.; Di Leva, A.; D’Onofrio, A.; Gialanella, L.; Romano, M.; De Cesare, M.; Duarte, J.G.; Fülöp, Z.; Morales-Gallegos, L.; et al. Test measurement of 7Be(p,γ)8B with the recoil mass separator ERNA. Eur. Phys. J. A 2018, 54, 92. [Google Scholar] [CrossRef]
- Cristallo, S.; Di Leva, A.; Imbriani, G.; Piersanti, L.; Abia, C.; Gialanella, L.; Straniero, O. Effects of nuclear cross sections on 19F nucleosynthesis at low metallicities. Astron. Astroph. 2014, 570, A46. [Google Scholar] [CrossRef][Green Version]
- Forestini, M.; Goriely, S.; Jorissen, A.; Arnould, M. Fluorine production in thermal pulses on the asymptotic giant branch. Astron. Astrophys. 1992, 261, 157. [Google Scholar]
- Abia, C.; Recio-Blanco, A.; de Laverny, P.; Cristallo, S.; Domínguez, I.; Straniero, O. Fluorine in Asymptotic Giant Branch Carbon Stars Revisited. Astrophys. J. 2009, 694, 971–977. [Google Scholar] [CrossRef]
- Woosley. Supernova neutrinos, neutral currents and the origin of fluorine. Nature 1988, 334, 45–47. [Google Scholar] [CrossRef]
- Meynet, G.; Arnould, M. Synthesis of 19F in Wolf-Rayet stars. Astron. Astrophys. 2000, 335, 176–180. [Google Scholar]
- Straniero, O.; Gallino, R.; Busso, M.; Chiefei, A.; Raiteri, C.M.; Limongi, M.; Salaris, M. Radiative 13C Burning in Asymptotic Giant Branch Stars and s-Processing. Astrophys. J. Lett. 1995, 440, L85. [Google Scholar] [CrossRef]
- Gallino, R.; Arlandini, C.; Busso, M.; Lugaro, M.; Travaglio, C.; Straniero, O.; Chieffi, A.; Limongi, M. Evolution and Nucleosynthesis in Low-Mass Asymptotic Giant Branch Stars. II. Neutron Capture and the S-Process. Astrophys. J. 1998, 497, 388–403. [Google Scholar] [CrossRef]
- Wilmes, S.; Wilmes, V.; Staudt, G.; Mohr, P.; Hammer, J.W. The 15N(α,γ)19F reaction and nucleosynthesis of 19F. Phys. Rev. C 2002, 66, 065802. [Google Scholar] [CrossRef]
- Rogers, D.W.O.; Beukens, R.P.; Diamond, W.T. Resonances in the 15N(α,γ)19F Reaction Between Eα=1.68 and 2.72MeV. Can. J. Phys. 1972, 50, 2428–2443. [Google Scholar] [CrossRef]
- Price, P.C. The Radiative Capture of Alpha Particles in 15N. Proc. Phys. Society. Sect. A 1957, 70, 661–667. [Google Scholar] [CrossRef]
- de Oliveira, F.; Coc, A.; Aguer, P.; Angulo, C.; Bogaert, G.; Kiener, J.; Lefebvre, A.; Tatischeff, V.; Thibaud, J.P.; Fortier, S.; et al. Determination of α-widths in 19F relevant to fluorine nucleosynthesis. Nucl. Phys. A 1996, 597, 231–252. [Google Scholar] [CrossRef]
- Di Leva, A.; Pezzella, A.; De Cesare, N.; D’Onofrio, A.; Gialanella, L.; Romano, M.; Romoli, M.; Schürmann, D.; Terrasi, F.; Imbriani, G. 14,15N beam from cyanide compounds. Nucl. Instr. Meth. A 2012, 689, 98–101. [Google Scholar] [CrossRef]
- Straniero, O.; Piersanti, L.; Cristallo, S. Do we really know Mup (i.e., the transition mass between Type Ia and core-collapse supernova progenitors)? J. Phys. Conf. Ser. (NPA6) 2016, 665, 012008. [Google Scholar] [CrossRef]
- Tang, X.; Bucher, B.; Fang, X.; Notani, M.; Tan, W.P.; Li, Y.; Mooney, P.; Esbensen, H.; Jiang, C.L.; Rehm, K.E.; et al. How does the carbon fusion reaction happen in stars? Nucl. Phys. At. Energy 2013, 14, 224–232. [Google Scholar] [CrossRef]
- Strieder, F.; Rolfs, C. Reaction data for light element nucleosynthesis. Prog. Part. Nucl. Phys. 2007, 59, 562–578. [Google Scholar] [CrossRef]
- Barrón-Palos, L.; Aguilera, E.F.; Aspiazu, J.; Huerta, A.; Martínez-Quiroz, E.; Monroy, R.; Moreno, E.; Murillo, G.; Ortíz, M.E.; Policroniades, R.; et al. Absolute cross sections measurement for the 12C+12C system at astrophysically relevant energies. Nucl. Phys. A 2006, 779, 318–332. [Google Scholar] [CrossRef]
- Angulo, C. Experimental Tools for Nuclear Astrophysics. Lect. Notes Phys. 2009, 764, 253–282. [Google Scholar]
- Jiang, C.L.; Back, B.B.; Esbensen, H.; Janssens, R.F.; Rehm, K.E.; Charity, R.J. Origin and Consequences of 12C+12C Fusion Resonances at Deep Sub-barrier Energies. Phys. Rev. Lett. 2013, 110, 072701. [Google Scholar] [CrossRef] [PubMed]
- Patterson, J.R.; Winkler, H.; Zaidins, C.S. Experimental investigation of the stellar nuclear reaction 12C+12C at low energies. Astrophys. J. 1969, 157, 367–373. [Google Scholar] [CrossRef]
- Mazarakis, M.G.; Stephens, W.E. Experimental Measurements of the 12C+12C Nuclear Reactions at Low Energies. Phys. Rev. C 1973, 7. [Google Scholar] [CrossRef]
- Becker, H.W.; Kettner, K.U.; Rolfs, C.; Trautvetter, H.P. The 12C+12C Reaction at Subcoulomb Energies (II). Z. Phys. A 1981, 303, 305–312. [Google Scholar] [CrossRef]
- High, M.D.; Cûjec, B. The 12C+12C sub-coulomb fusion cross section. Nucl. Phys. A 1977, 282, 181–188. [Google Scholar] [CrossRef]
- Kettner, K.U.; Lorenz-Wirzba, H.; Rolfs, C. The 12C+12C reaction at subcoulomb energies (I). Z. Phys. A 1980, 298, 65–75. [Google Scholar] [CrossRef]
- Rosales, P.; Aguilera, E.F.; Martinez-Quiroz, E.; Murillo, G.; Policroniades, R.; Varela, A.; Moreno, E.; Fernández, M.; Berdejo, H.; Aspiazu1, J.; et al. Sub-Coulomb fusion excitation function for 12C+12C. Rev. Mex. De Fis. S4 2003, 49, 88–91. [Google Scholar]
- Aguilera, E.F.; Rosales, P.; Martinez-Quiroz, E.; Murillo, G.; Fernández, M.; Berdejo, H.; Lizcano, D.; Gómez-Camacho, A.; Policroniades, R.; Varela, A.; et al. New γ-ray measurements for 12C+12C sub-Coulomb fusion: Toward data unification. Phys. Rev. C 2006, 73, 064601. [Google Scholar] [CrossRef]
- Spillane, T.; Raiola, F.; Rolfs, C.; Schürmann, D.; Strieder, F.; Zeng, S.; Becker, H.W.; Bordeanu, C.; Gialanella, L.; Romano, M.; et al. 12C+12C Fusion Reactions near the Gamow Energy. Phys. Rev. Lett. 2007, 98, 122501. [Google Scholar] [CrossRef]
- Jiang, C.L.; Santiago-Gonzalez, D.; Almaraz-Calderon, S.; Rehm, K.E.; Back, B.B.; Auranen, K.; Avila, M.L.; Ayangeakaa, A.D.; Bottoni, S.; Carpenter, M.P.; et al. Reaction rate for carbon burning in massive stars. Phys. Rev. C 2018, 97, 012801. [Google Scholar] [CrossRef]
- Fruet, G.; Courtin, S.; Heine, M.; Jenkins, D.G.; Adsley, P.; Brown, A.; Canavan, R.; Catford, W.N.; Charon, E.; Curien, D.; et al. Advances in the Direct Study of Carbon Burning in Massive Stars. Phys. Rev. Lett. 2020, 124, 192701. [Google Scholar] [CrossRef] [PubMed]
- Tan, W.P.; Boeltzig, A.; Dulal, C.; deBoer, R.J.; Frentz, B.; Henderson, S.; Howard, K.B.; Kelmar, R.; Kolata, J.J.; Long, J.; et al. New Measurement of 12C+12C Fusion Reaction at Astrophysical Energies. Phys. Rev. Lett. 2020, 124, 192702. [Google Scholar] [CrossRef]
- Tumino, A.; Spitaleri, C.; Cognata, M.L.; Cherubini, S.; Guardo, G.L.; Gulino, M.; Hayakawa, S.; Indelicato, I.; Lamia, L.; Petrascu, H.; et al. An increase in the 12C+12C fusion rate from resonances at astrophysical energies. Nature 2018, 557, 687–690. [Google Scholar] [CrossRef] [PubMed]
- Mukhamedzhanov, A.M.; Pang, D.Y. Astrophysical factors of 12C+12C fusion from Trojan horse method. Phys. Rev. C 2019, 99, 064618. [Google Scholar] [CrossRef]
- Tumino, A.; Spitaleri, C.; Cognata, M.L.; Cherubini, S.; Guardo, G.L.; Gulino, M.; Hayakawa, S.; Indelicato, I.; Lamia, L.; Petrascu, H.; et al. Reply to the Comments on the 12C+12C fusion S*-factor. arXiv 2018, arXiv:1807.06148. [Google Scholar]
- Longland, R.; Iliadis, C.; Champagne, A.E.; Newton, J.R.; Ugalde, C.; Coc, A.; Fitzgerald, R. Charged-particle thermonuclear reaction rates: I. Monte Carlo method and statistical distributions. Nucl. Phys. A 2010, 841, 1–30. [Google Scholar] [CrossRef]
- Iliadis, C.; Longland, R.; Champagne, A.E.; Coc, A.; Fitzgerald, R. Charged-particle thermonuclear reaction rates: II. Tables and graphs of reaction rates and probability density functions. Nucl. Phys. A 2010, 841, 31–250. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Buompane, R.; Di Leva, A.; Gialanella, L.; Imbriani, G.; Morales-Gallegos, L.; Romoli, M. Recent Achievements of the ERNA Collaboration. Universe 2022, 8, 135. https://doi.org/10.3390/universe8020135
Buompane R, Di Leva A, Gialanella L, Imbriani G, Morales-Gallegos L, Romoli M. Recent Achievements of the ERNA Collaboration. Universe. 2022; 8(2):135. https://doi.org/10.3390/universe8020135
Chicago/Turabian StyleBuompane, Raffaele, Antonino Di Leva, Lucio Gialanella, Gianluca Imbriani, Lizeth Morales-Gallegos, and Mauro Romoli. 2022. "Recent Achievements of the ERNA Collaboration" Universe 8, no. 2: 135. https://doi.org/10.3390/universe8020135
APA StyleBuompane, R., Di Leva, A., Gialanella, L., Imbriani, G., Morales-Gallegos, L., & Romoli, M. (2022). Recent Achievements of the ERNA Collaboration. Universe, 8(2), 135. https://doi.org/10.3390/universe8020135





