Searches for Violation of CPT Symmetry and Lorentz Invariance with Astrophysical Neutrinos
Abstract
1. Introduction
2. Lorentz Invariance and CPT Symmetry Violations
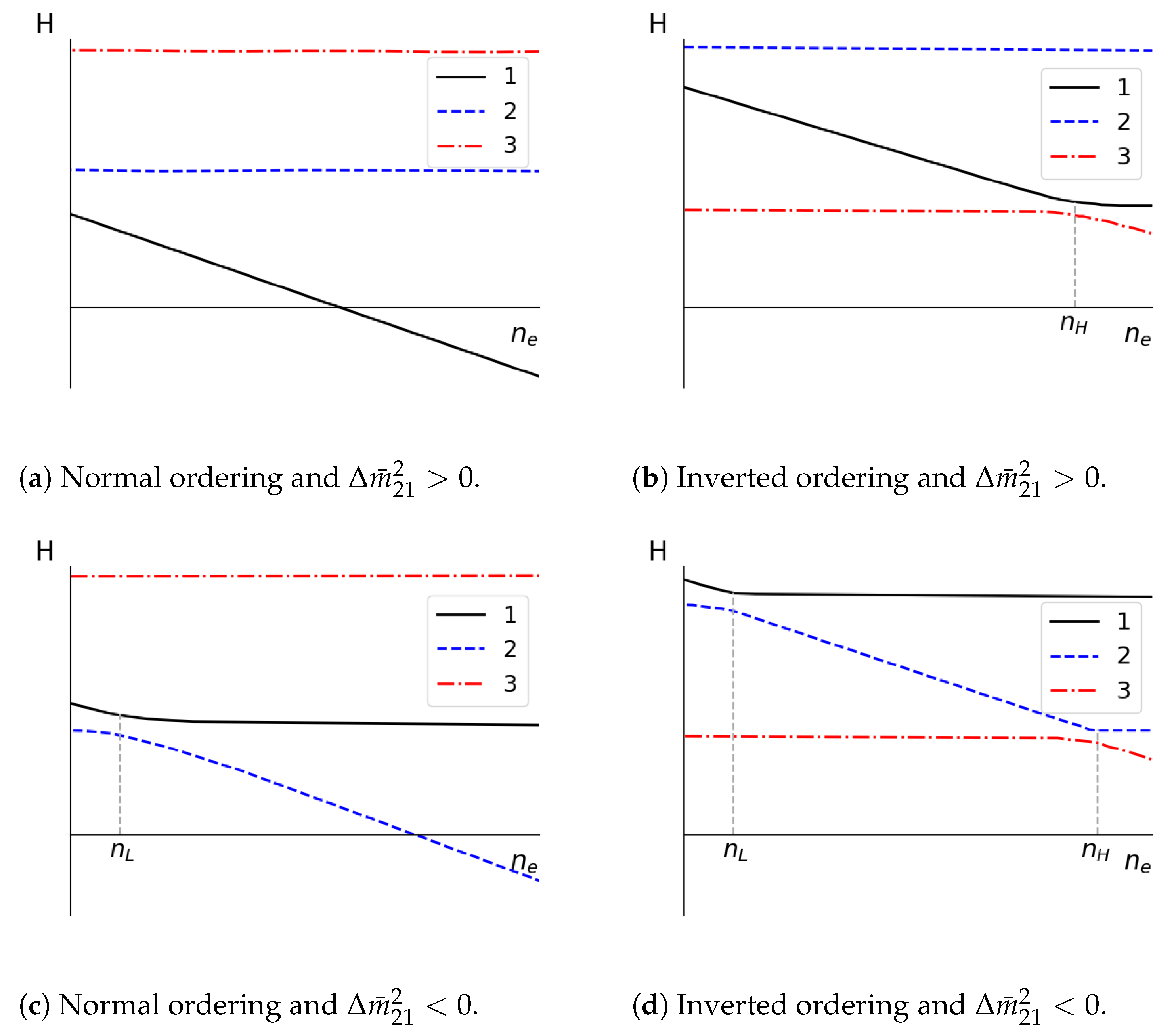
3. Limits from Supernova Events
3.1. CPT Violation Measurements from Supernovae
3.2. LIV Measurements from Supernovae
4. High Energy Astrophysical Environments
4.1. LIV Limit Measurements
4.2. CPT Limit Measurements
5. Outlook and Future Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| SM | The Standard Model of Elementary Particles |
| SME | Standard Model Extension |
| LIV | Lorentz Invariance Violation |
| PMNS | Pontecorvo–Maki–Nakagawa–Sakata |
| SN | Supernova |
| GRB | Gamma-Ray Burst |
References
- Rosner, J.L. Resource letter: The Standard model and beyond. Am. J. Phys. 2003, 71, 302. [Google Scholar] [CrossRef]
- Luders, G. Proof of the TCP theorem. Ann. Phys. 1957, 2, 1. [Google Scholar] [CrossRef]
- Srednicki, M. Quantum Field Theory; Cambridge University Press: New York, NY, USA, 2007; pp. 543–561. [Google Scholar]
- Greenberg, O.W. CPT violation implies violation of Lorentz invariance. Phys. Rev. Lett. 2002, 89, 231602. [Google Scholar] [CrossRef] [PubMed]
- Chaichian, M.; Dolgov, A.D.; Novikov, V.A.; Tureanu, A. CPT Violation Does Not Lead to Violation of Lorentz Invariance and Vice Versa. Phys. Lett. B 2011, 699, 177. [Google Scholar] [CrossRef]
- Tasson, J.D. Gravity Effects on Antimatter in the Standard-Model Extension. Int. J. Mod. Phys. Conf. Ser. 2014, 30, 1460273. [Google Scholar] [CrossRef]
- Zyla, P.A.; Barnett, R.M.; Beringer, J.; Dahl, O.; Dwyer, D.A.; Groom, D.E.; Lin, C.-J.; Lugovsky, K.S.; Pianori, E.; Robinson, D.J.; et al. Review of Particle Physics. Prog. Theor. Exp. Phys. 2020, 2020, 083C01. [Google Scholar] [CrossRef]
- Kostelecky, A.; Mewes, M. Neutrinos with Lorentz-violating operators of arbitrary dimension. Phys. Rev. D 2012, 85, 096005. [Google Scholar] [CrossRef]
- Colladay, D.; Kostelecky, V.A. Lorentz violating extension of the standard model. Phys. Rev. D 1998, 58, 116002. [Google Scholar] [CrossRef]
- Davis, R., Jr.; Harmer, D.S.; Hoffman, K.C. Search for neutrinos from the sun. Phys. Rev. Lett. 1968, 20, 1205. [Google Scholar] [CrossRef]
- Bionta, R.M.; Blewitt, G.; Bratton, C.B.; Casper, D.; Ciocio, A.; Claus, R.; Cortez, B.; Crouch, M.; Dye, S.T.; Errede, S.; et al. Observation of a Neutrino Burst in Coincidence with Supernova SN 1987a in the Large Magellanic Cloud. Phys. Rev. Lett. 1987, 58, 1494. [Google Scholar] [CrossRef]
- Alekseev, E.N.; Alekseeva, L.N.; Volchenko, V.I.; Krivosheina, I.V. Detection of the Neutrino Signal From SN1987A in the LMC Using the Inr Baksan Underground Scintillation Telescope. Phys. Lett. B 1988, 205, 209. [Google Scholar] [CrossRef]
- Aglietta, M.; Badino, G.; Bologna, G.; Castagnoli, C.; Castellina, A.; Fulgione, W.; Galeotti, P.; Saavedra, O.; Trinchero, G.; Vernetto, S.; et al. On the event observed in the Mont Blanc Underground Neutrino observatory during the occurrence of Supernova 1987a. Europhys. Lett. 1987, 3, 1315. [Google Scholar] [CrossRef]
- Hirata, K. et al. [Kamiokande-II Collaboration] Observation of a Neutrino Burst from the Supernova SN 1987a. Phys. Rev. Lett. 1987, 58, 1490. [Google Scholar] [CrossRef]
- Aartsen, M.G. et al. [IceCube Collaboration] First observation of PeV-energy neutrinos with IceCube. Phys. Rev. Lett. 2013, 111, 021103. [Google Scholar] [CrossRef] [PubMed]
- Aartsen, M.G. et al. [IceCube Collaboration] Evidence for High-Energy Extraterrestrial Neutrinos at the IceCube Detector. Science 2013, 342, 1242856. [Google Scholar] [CrossRef]
- Aartsen, M.G. et al. [IceCube Collaboration] Observation of High-Energy Astrophysical Neutrinos in Three Years of IceCube Data. Phys. Rev. Lett. 2014, 113, 101101. [Google Scholar] [CrossRef]
- Greus, F.S.; Losa, A.S. Multimessenger Astronomy with Neutrinos. Universe 2021, 7, 397. [Google Scholar] [CrossRef]
- Antonelli, V.; Miramonti, L.; Torri, M.D.C. Phenomenological Effects of CPT and Lorentz Invariance Violation in Particle and Astroparticle Physics. Symmetry 2020, 12, 1821. [Google Scholar] [CrossRef]
- Diaz, J.S. Testing Lorentz and CPT invariance with neutrinos. Symmetry 2016, 8, 105. [Google Scholar] [CrossRef]
- Greaves, H.; Thomas, T. On the CPT theorem. Stud. Hist. Phil. Sci. B 2014, 45, 46. [Google Scholar] [CrossRef][Green Version]
- Murayama, H.; Yanagida, T. LSND, SN1987A, and CPT violation. Phys. Lett. B 2001, 520, 263. [Google Scholar] [CrossRef]
- Barenboim, G.; Borissov, L.; Lykken, J.D.; Smirnov, A.Y. Neutrinos as the Messengers of CPT Violation. J. High Energy Phys. 2002, 10, 1. [Google Scholar] [CrossRef]
- Gonzalez-Garcia, M.C.; Maltoni, M.; Schwetz, T. Status of the CPT violating interpretations of the LSND signal. Phys. Rev. D 2003, 68, 053007. [Google Scholar] [CrossRef]
- De Gouvêa, A.; Kelly, K.J. Neutrino vs. Antineutrino Oscillation Parameters at DUNE and Hyper-Kamiokande. Phys. Rev. D 2017, 96, 095018. [Google Scholar] [CrossRef]
- De Gouvea, A.; Pena-Garay, C. Probing new physics by comparing solar and KamLAND data. Phys. Rev. D 2005, 71, 093002. [Google Scholar] [CrossRef]
- Giunti, C.; Laveder, M. Hint of CPT Violation in Short-Baseline Electron Neutrino Disappearance. Phys. Rev. D 2010, 82, 113009. [Google Scholar] [CrossRef]
- Barenboim, G.; Beacom, J.F.; Borissov, L.; Kayser, B. CPT Violation and the Nature of Neutrinos. Phys. Lett. B 2002, 537, 227. [Google Scholar] [CrossRef]
- Moura, C.A.; on behalf of the DUNE Collaboration. Physics Beyond the Standard Model with DUNE: Prospects for Exploring Lorentz and CPT Violation. In Proceedings of the Eighth Meeting on CPT and Lorentz Symmetry, Bloomington, IN, USA, 12–16 May 2019; Lehnert, R., Ed.; World Scientific Publishing Co. Pte. Ltd.: Singapore, 2020; pp. 150–153. [CrossRef]
- Barenboim, G.; Ternes, C.A.; Tórtola, M. “Neutrinos, DUNE and the world best bound on CPT invariance. Phys. Lett. B 2018, 780, 631. [Google Scholar] [CrossRef]
- Carrasco, J.C.; Díaz, F.N.; Gago, A.M. Probing CPT breaking induced by quantum decoherence at DUNE. Phys. Rev. D 2019, 99, 075022. [Google Scholar] [CrossRef]
- Majhi, R.; Singha, D.K.; Deepthi, K.N.; Mohanta, R. Constraining CPT violation with Hyper-Kamiokande and ESSnuSB. Phys. Rev. D 2021, 104, 055002. [Google Scholar] [CrossRef]
- Gando, A. et al. [KamLAND Collaboration] Reactor On-Off Antineutrino Measurement with KamLAND. Phys. Rev. D 2013, 88, 033001. [Google Scholar] [CrossRef]
- Adey, D. et al. [Daya Bay Collaboration] Measurement of the Electron Antineutrino Oscillation with 1958 Days of Operation at Daya Bay. Phys. Rev. Lett. 2018, 121, 241805. [Google Scholar] [CrossRef] [PubMed]
- Choi, J.H. et al. [RENO Collaboration] Observation of Energy and Baseline Dependent Reactor Antineutrino Disappearance in the RENO Experiment. Phys. Rev. Lett. 2016, 116, 211801. [Google Scholar] [CrossRef] [PubMed]
- Abe, Y. et al. [Double Chooz Collaboration] Improved measurements of the neutrino mixing angle θ13 with the Double Chooz detector. J. High Energy Phys. 2014, 10, 086, Erratum in J. High Energy Phys. 2015, 2, 74. [Google Scholar] [CrossRef]
- Abe, K. et al. [Super-Kamiokande Collaboration] Search for Differences in Oscillation Parameters for Atmospheric Neutrinos and Antineutrinos at Super-Kamiokande. Phys. Rev. Lett. 2011, 107, 241801. [Google Scholar] [CrossRef]
- Adamson, P. et al. [MINOS Collaboration] Measurement of Neutrino and Antineutrino Oscillations Using Beam and Atmospheric Data in MINOS. Phys. Rev. Lett. 2013, 110, 251801. [Google Scholar] [CrossRef] [PubMed]
- Abe, K. et al. [T2K Collaboration] T2K measurements of muon neutrino and antineutrino disappearance using 3.13 × 1021 protons on target. Phys. Rev. D 2021, 103, L011101. [Google Scholar] [CrossRef]
- Hampel, W. et al. [GALLEX Collaboration] Final results of the Cr-51 neutrino source experiments in GALLEX. Phys. Lett. B 1998, 420, 114. [Google Scholar] [CrossRef]
- Altmann, M. et al. [GNO Collaboration] Complete results for five years of GNO solar neutrino observations. Phys. Lett. B 2005, 616, 174. [Google Scholar] [CrossRef]
- Abdurashitov, J.N. et al. [SAGE Collaboration] Measurement of the solar neutrino capture rate with gallium metal. III: Results for the 2002–2007 data-taking period. Phys. Rev. C 2009, 80, 015807. [Google Scholar] [CrossRef]
- Aharmim, B. et al. [SNO Collaboration] Combined Analysis of all Three Phases of Solar Neutrino Data from the Sudbury Neutrino Observatory. Phys. Rev. C 2013, 88, 025501. [Google Scholar] [CrossRef]
- Bellini, G. et al. [Borexino Collaboration] Final results of Borexino Phase-I on low energy solar neutrino spectroscopy. Phys. Rev. D 2014, 89, 112007. [Google Scholar] [CrossRef]
- Abe, K. et al. [Super-Kamiokande Collaboration] Solar Neutrino Measurements in Super-Kamiokande-IV. Phys. Rev. D 2016, 94, 052010. [Google Scholar] [CrossRef]
- Adamson, P. et al. [MINOS Collaboration] Combined analysis of νμ disappearance and νμ→νe appearance in MINOS using accelerator and atmospheric neutrinos. Phys. Rev. Lett. 2014, 112, 191801. [Google Scholar] [CrossRef] [PubMed]
- Adamson, P. et al. [NOvA Collaboration] Constraints on Oscillation Parameters from νe Appearance and νμ Disappearance in NOvA. Phys. Rev. Lett. 2017, 118, 231801. [Google Scholar] [CrossRef]
- Esteban, I.; Gonzalez-Garcia, M.C.; Maltoni, M.; Schwetz, T.; Zhou, A. The fate of hints: Updated global analysis of three-flavor neutrino oscillations. J. High Energy Phys. 2020, 9, 178. [Google Scholar] [CrossRef]
- De Salas, P.F.; Forero, D.V.; Gariazzo, S.; Martínez-Miravé, P.; Mena, O.; Ternes, C.A.; Tórtola, M.; Valle, J.W.F. 2020 global reassessment of the neutrino oscillation picture. J. High Energy Phys. 2021, 2, 71. [Google Scholar] [CrossRef]
- Abe, K. et al. [T2K Collaboration] Improved constraints on neutrino mixing from the T2K experiment with 3.13 × 1021 protons on target. Phys. Rev. D 2021, 103, 112008. [Google Scholar] [CrossRef]
- Guo, W.L.; Xing, Z.Z.; Zhou, S. Neutrino Masses, Lepton Flavor Mixing and Leptogenesis in the Minimal Seesaw Model. Int. J. Mod. Phys. E 2007, 16, 1. [Google Scholar] [CrossRef]
- Roberts, Á. Astrophysical Neutrinos in Testing Lorentz Symmetry. Galaxies 2021, 9, 47. [Google Scholar] [CrossRef]
- Diaz, J.S.; Kostelecky, V.A.; Mewes, M. Perturbative Lorentz and CPT violation for neutrino and antineutrino oscillations. Phys. Rev. D 2009, 80, 076007. [Google Scholar] [CrossRef]
- Mattingly, D. Modern tests of Lorentz invariance. Living Rev. Rel. 2005, 8, 5. [Google Scholar] [CrossRef]
- Liberati, S. Tests of Lorentz invariance: A 2013 update. Class. Quant. Grav. 2013, 30, 133001. [Google Scholar] [CrossRef]
- Kostelecky, V.A.; Mewes, M. Electrodynamics with Lorentz-violating operators of arbitrary dimension. Phys. Rev. D 2009, 80, 015020. [Google Scholar] [CrossRef]
- Kostelecký, A.; Mewes, A. Fermions with Lorentz-violating operators of arbitrary dimension. Phys. Rev. D 2013, 88, 096006. [Google Scholar] [CrossRef]
- Coleman, S.R.; Glashow, S.L. High-energy tests of Lorentz invariance. Phys. Rev. D 1999, 59, 116008. [Google Scholar] [CrossRef]
- Amelino-Camelia, G. Doubly special relativity. Nature 2002, 418, 34. [Google Scholar] [CrossRef]
- Amelino-Camelia, G. Doubly special relativity: First results and key open problems. Int. J. Mod. Phys. D 2002, 11, 1643. [Google Scholar] [CrossRef]
- Torri, M.D.C.; Antonelli, V.; Miramonti, L. Homogeneously Modified Special relativity (HMSR): A new possible way to introduce an isotropic Lorentz invariance violation in particle standard model. Eur. Phys. J. C 2019, 79, 808. [Google Scholar] [CrossRef]
- Addazi, A.; Alvarez-Muniz, J.; Batista, R.A.; Amelino-Camelia, G.; Antonelli, V.; Arzano, M.; Asorey, M.; Atteia, J.L.; Bahamonde, S.; Bajardi, F.; et al. Quantum gravity phenomenology at the dawn of the multi-messenger era—A review. arXiv 2021, arXiv:2111.05659. [Google Scholar]
- Mirizzi, A.; Tamborra, I.; Janka, H.T.; Saviano, N.; Scholberg, K.; Bollig, R.; Hudepohl, L.; Chakraborty, S. Supernova Neutrinos: Production, Oscillations and Detection. Riv. Nuovo Cim. 2016, 39, 1. [Google Scholar] [CrossRef]
- Duan, H.; Fuller, G.M.; Qian, Y.Z. Collective Neutrino Oscillations. Ann. Rev. Nucl. Part. Sci. 2010, 60, 569. [Google Scholar] [CrossRef]
- Vissani, F. Comparative analysis of SN1987A antineutrino fluence. J. Phys. G 2015, 42, 013001. [Google Scholar] [CrossRef]
- Jaffe, A.H.; Turner, M.S. Gamma-rays and the decay of neutrinos from SN1987A. Phys. Rev. D 1997, 55, 7951. [Google Scholar] [CrossRef]
- Schmid, H.; Raffelt, G.; Leike, A. The Bremsstrahlung process tau-neutrino —> electron-neutrino e+ e− gamma. Phys. Rev. D 1998, 58, 113004. [Google Scholar] [CrossRef]
- Raffelt, G.G. What Have We Learned From SN 1987A? Mod. Phys. Lett. A 1990, 5, 2581. [Google Scholar] [CrossRef]
- Abi, B. et al. [DUNE Collaboration] Deep Underground Neutrino Experiment (DUNE), Far Detector Technical Design Report, Volume II: DUNE Physics. arXiv 2020, arXiv:2002.03005. [Google Scholar]
- Abe, K.; Abe, T.; Aihara, H.; Fukuda, Y.; Hayato, Y.; Huang, K.; Ichikawa, A.K.; Ikeda, M.; Inoue, K.; Ishino, H.; et al. Letter of Intent: The Hyper-Kamiokande Experiment—Detector Design and Physics Potential. arXiv 2011, arXiv:1109.3262. [Google Scholar]
- Minakata, H.; Uchinami, S. Testing CPT symmetry with supernova neutrinos. Phys. Rev. D 2005, 72, 105007. [Google Scholar] [CrossRef]
- Dighe, A.S.; Smirnov, A.Y. Identifying the neutrino mass spectrum from the neutrino burst from a supernova. Phys. Rev. D 2000, 62, 033007. [Google Scholar] [CrossRef]
- Amelino-Camelia, G.; Ellis, J.R.; Mavromatos, N.E.; Nanopoulos, D.V.; Sarkar, S. Tests of quantum gravity from observations of gamma-ray bursts. Nature 1998, 393, 763. [Google Scholar] [CrossRef]
- Garay, L.J. Thermal properties of space-time foam. Phys. Rev. D 1998, 58, 124015. [Google Scholar] [CrossRef]
- Stodolsky, L. The Speed of Light and the Speed of Neutrinos. Phys. Lett. B 1998, 201, 353. [Google Scholar] [CrossRef]
- Chakraborty, S.; Mirizzi, A.; Sigl, G. Testing Lorentz invariance with neutrino bursts from supernova neutronization. Phys. Rev. D 2013, 87, 017302. [Google Scholar] [CrossRef]
- Ellis, J.R.; Harries, N.; Meregaglia, A.; Rubbia, A.; Sakharov, A. Probes of Lorentz Violation in Neutrino Propagation. Phys. Rev. D 2008, 78, 033013. [Google Scholar] [CrossRef]
- Ellis, J.; Janka, H.T.; Mavromatos, N.E.; Sakharov, A.S.; Sarkisyan, E.K.G. Probing Lorentz Violation in Neutrino Propagation from a Core-Collapse Supernova. Phys. Rev. D 2012, 85, 045032. [Google Scholar] [CrossRef]
- Lund, T.; Wongwathanarat, A.; Janka, H.T.; Muller, E.; Raffelt, G. Fast time variations of supernova neutrino signals from 3-dimensional models. Phys. Rev. D 2012, 86, 105031. [Google Scholar] [CrossRef]
- Kostelecky, V.A.; Russell, N. Data Tables for Lorentz and CPT Violation. arXiv 2008, arXiv:0801.0287. [Google Scholar]
- Diaz, J.S.; Kostelecky, A.; Mewes, M. Testing Relativity with High-Energy Astrophysical Neutrinos. Phys. Rev. D 2014, 89, 043005. [Google Scholar] [CrossRef]
- Waxman, E.; Bahcall, J.N. High-energy neutrinos from cosmological gamma-ray burst fireballs. Phys. Rev. Lett. 1997, 78, 2292. [Google Scholar] [CrossRef]
- Vietri, M. On the energy of neutrinos from gamma-ray bursts. Astrophys. J. 1998, 507, 40. [Google Scholar] [CrossRef]
- Jacob, U.; Piran, T. Neutrinos from gamma-ray bursts as a tool to explore quantum-gravity-induced Lorentz violation. Nat. Phys. 2007, 3, 87. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Liu, R.Y.; Wang, X.Y. Testing the equivalence principle and Lorentz invariance with PeV neutrinos from blazar flares. Phys. Rev. Lett. 2016, 116, 151101. [Google Scholar] [CrossRef]
- Maccione, L.; Liberati, S.; Mattingly, D.M. Violations of Lorentz invariance in the neutrino sector after OPERA. J. Cosmol. Astropart. Phys. 2013, 3, 39. [Google Scholar] [CrossRef]
- Jentschura, U.D.; Nándori, I.; Ehrlich, R. Calculation of the decay rate of tachyonic neutrinos against charged-lepton-pair and neutrino-pair Cerenkov radiation. J. Phys. G 2017, 44, 105201. [Google Scholar] [CrossRef]
- Somogyi, G.; Nándori, I.; Jentschura, U.D. Neutrino Splitting for Lorentz-Violating Neutrinos: Detailed Analysis. Phys. Rev. D 2019, 100, 035036. [Google Scholar] [CrossRef]
- Cohen, A.G.; Glashow, S.L. Pair Creation Constrains Superluminal Neutrino Propagation. Phys. Rev. Lett. 2011, 107, 181803. [Google Scholar] [CrossRef]
- Stecker, F.W. Limiting superluminal electron and neutrino velocities using the 2010 Crab Nebula flare and the IceCube PeV neutrino events. Astropart. Phys. 2014, 56, 16. [Google Scholar] [CrossRef]
- Wang, K.; Xi, S.Q.; Shao, L.; Liu, R.Y.; Li, Z.; Zhang, Z.K. Limiting Superluminal Neutrino Velocity and Lorentz Invariance Violation by Neutrino Emission from the Blazar TXS 0506+056. Phys. Rev. D 2020, 102, 063027. [Google Scholar] [CrossRef]
- Stecker, F.W.; Scully, S.T.; Liberati, S.; Mattingly, D. Searching for Traces of Planck-Scale Physics with High Energy Neutrinos. Phys. Rev. D 2015, 91, 045009. [Google Scholar] [CrossRef]
- Borriello, E.; Chakraborty, S.; Mirizzi, A.; Serpico, P.D. Stringent constraint on neutrino Lorentz-invariance violation from the two IceCube PeV neutrinos. Phys. Rev. D 2013, 87, 116009. [Google Scholar] [CrossRef]
- Aartsen, M.G. et al. [IceCube Collaboration, Fermi-LAT, MAGIC, AGILE, ASAS-SN, HAWC, H.E.S.S., INTEGRAL, Kanata, Kiso, Kapteyn, Liverpool Telescope, Subaru, Swift NuSTAR, VERITAS and VLA/17B-403] Multimessenger observations of a flaring blazar coincident with high-energy neutrino IceCube-170922A. Science 2018, 361, 1378. [Google Scholar] [CrossRef]
- Paiano, S.; Falomo, R.; Treves, A.; Scarpa, R. The redshift of the BL Lac object TXS 0506+056. Astrophys. J. Lett. 2018, 854, L32. [Google Scholar] [CrossRef]
- Laha, R. Constraints on neutrino speed, weak equivalence principle violation, Lorentz invariance violation, and dual lensing from the first high-energy astrophysical neutrino source TXS 0506+056. Phys. Rev. D 2019, 100, 103002. [Google Scholar] [CrossRef]
- Ellis, J.; Mavromatos, N.E.; Sakharov, A.S.; Sarkisyan-Grinbaum, E.K. Limits on Neutrino Lorentz Violation from Multimessenger Observations of TXS 0506+056. Phys. Lett. B 2019, 789, 352. [Google Scholar] [CrossRef]
- Wei, J.J.; Zhang, B.B.; Shao, L.; Gao, H.; Li, Y.; Yin, Q.Q.; Wu, X.F.; Wang, X.Y.; Zhang, B.; Dai, Z.G. Multimessenger tests of Einstein’s weak equivalence principle and Lorentz invariance with a high-energy neutrino from a flaring blazar. J. High Energy Astrophys. 2019, 22, 1. [Google Scholar] [CrossRef]
- Stecker, F.W.; Scully, S.T. Propagation of Superluminal PeV IceCube Neutrinos: A High Energy Spectral Cutoff or New Constraints on Lorentz Invariance Violation. Phys. Rev. D 2014, 90, 043012. [Google Scholar] [CrossRef]
- Stecker, F.W. Tests of Lorentz Invariance Using High Energy Astrophysics Observations. In CPT and Lorentz Symmetry, Proceedings of the Sixth Meeting, Bloomington, IN, USA, 17–21 June 2013; Kostelecky, V.A., Ed.; World Scientific Publishing Co. Pte. Ltd.: Singapore, 2014; pp. 73–76. [Google Scholar] [CrossRef]
- Hooper, D.; Morgan, D.; Winstanley, E. Lorentz and CPT invariance violation in high-energy neutrinos. Phys. Rev. D 2005, 72, 065009. [Google Scholar] [CrossRef]
- Buoninfante, L.; Capolupo, A.; Giampaolo, S.M.; Lambiase, G. Revealing neutrino nature and CPT violation with decoherence effects. Eur. Phys. J. C 2020, 80, 1009. [Google Scholar] [CrossRef]
- Adrian-Martinez, S. et al. [KM3Net Collaboration] Letter of intent for KM3NeT 2.0. J. Phys. G 2016, 43, 084001. [Google Scholar] [CrossRef]
- Aartsen, M.G. et al. [IceCube-Gen2 Collaboration] IceCube-Gen2: The window to the extreme Universe. J. Phys. G 2021, 48, 060501. [Google Scholar] [CrossRef]
- Anchordoqui, L.A.; Goldberg, H.; Gonzalez-Garcia, M.C.; Halzen, F.; Hooper, D.; Sarkar, S.; Weiler, T.J. Probing Planck scale physics with IceCube. Phys. Rev. D 2005, 72, 065019. [Google Scholar] [CrossRef]
- Mehta, P.; Winter, W. Interplay of energy dependent astrophysical neutrino flavor ratios and new physics effects. J. Cosmol. Astropart. Phys. 2011, 3, 41. [Google Scholar] [CrossRef]
- Klapdor-Kleingrothaus, H.V.; Pas, H.; Sarkar, U. Effects of quantum space-time foam in the neutrino sector. Eur. Phys. J. A 2000, 8, 577. [Google Scholar] [CrossRef]
- Abi, B. et al. [DUNE Collaboration] Supernova neutrino burst detection with the Deep Underground Neutrino Experiment. Eur. Phys. J. C 2021, 81, 423. [Google Scholar] [CrossRef]
| Neutrino | Anti-Neutrino | |
|---|---|---|
| (eV) | [48] | [33] |
| (eV) | [39] | [39] |
| [48] | [33] | |
| [50] | [34] | |
| [39] | [39] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moura, C.A.; Rossi-Torres, F. Searches for Violation of CPT Symmetry and Lorentz Invariance with Astrophysical Neutrinos. Universe 2022, 8, 42. https://doi.org/10.3390/universe8010042
Moura CA, Rossi-Torres F. Searches for Violation of CPT Symmetry and Lorentz Invariance with Astrophysical Neutrinos. Universe. 2022; 8(1):42. https://doi.org/10.3390/universe8010042
Chicago/Turabian StyleMoura, Celio A., and Fernando Rossi-Torres. 2022. "Searches for Violation of CPT Symmetry and Lorentz Invariance with Astrophysical Neutrinos" Universe 8, no. 1: 42. https://doi.org/10.3390/universe8010042
APA StyleMoura, C. A., & Rossi-Torres, F. (2022). Searches for Violation of CPT Symmetry and Lorentz Invariance with Astrophysical Neutrinos. Universe, 8(1), 42. https://doi.org/10.3390/universe8010042






