Dynamics and Merger Rate of Primordial Black Holes in a Cluster
Abstract
1. Introduction
2. The Fokker–Planck Equation Approach
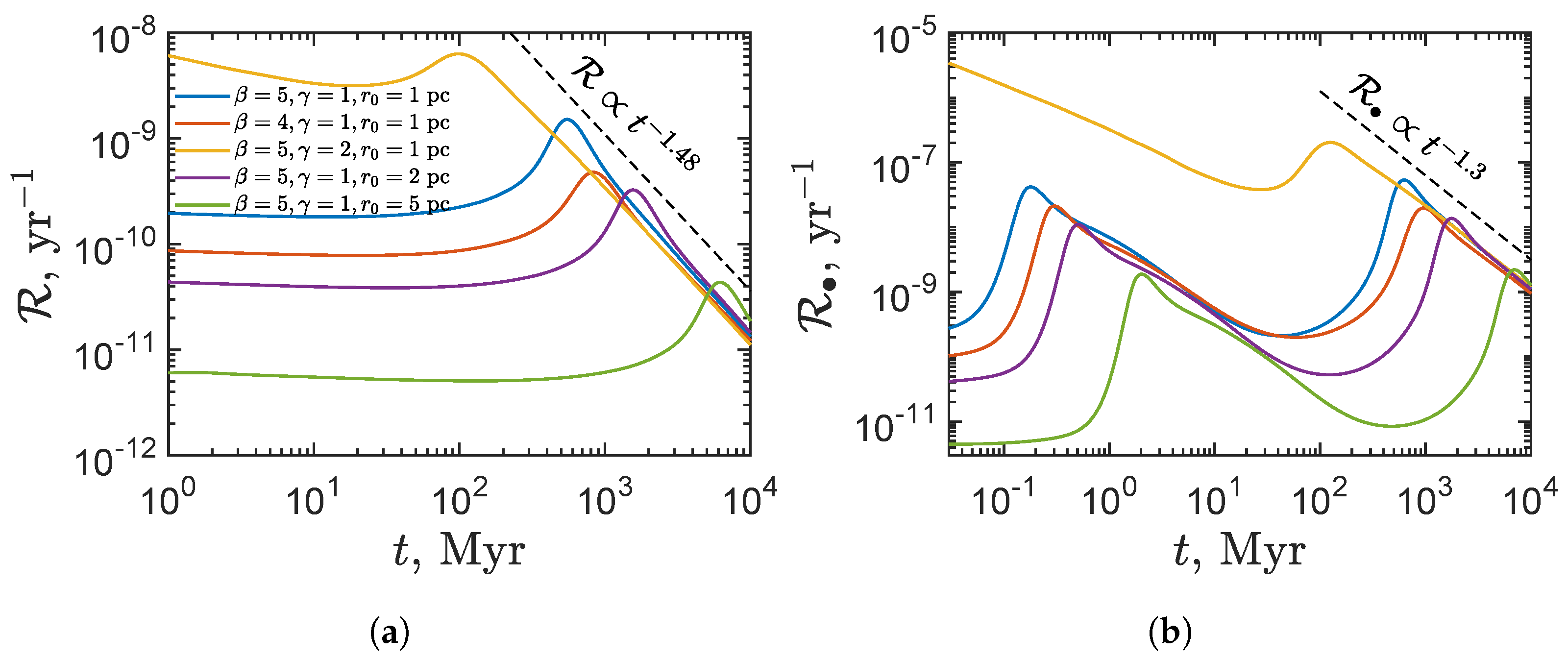
3. The Merging of Primordial Black Holes
4. Evolution of PBHs Clusters
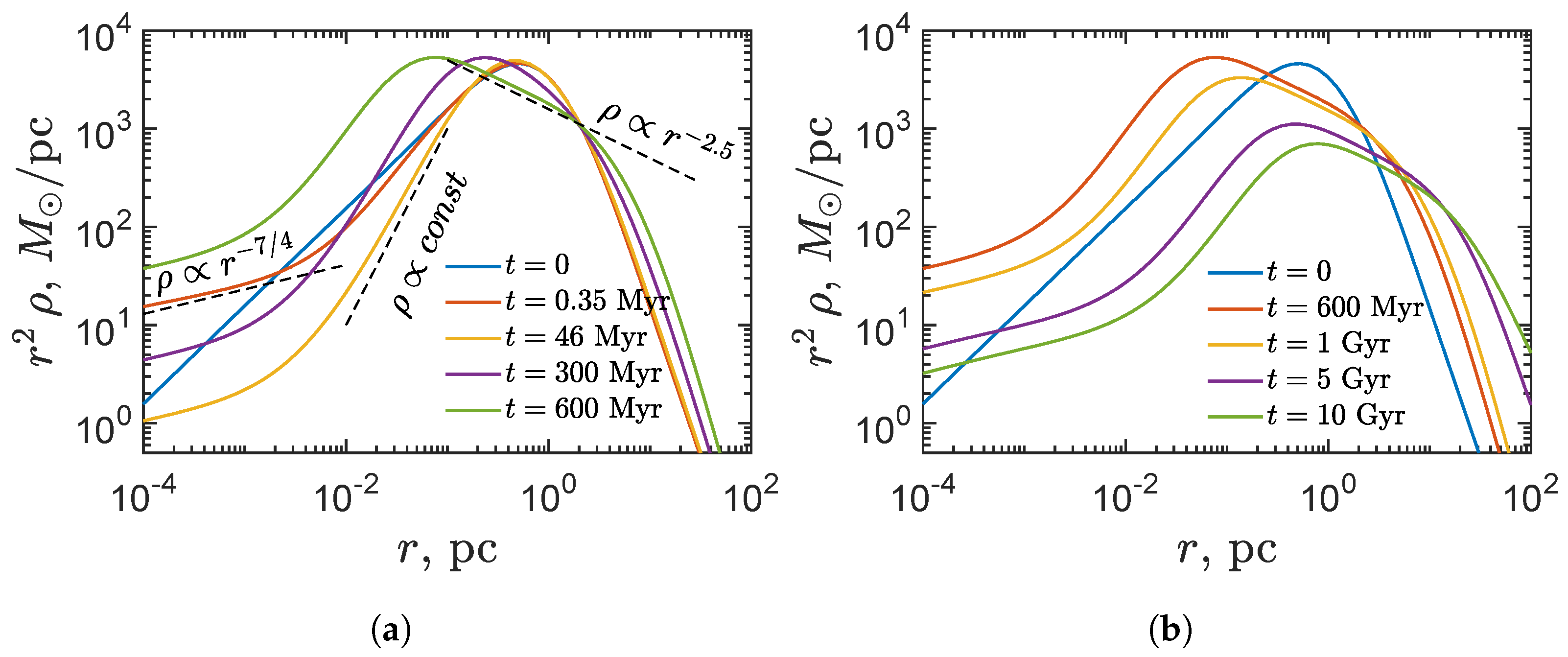
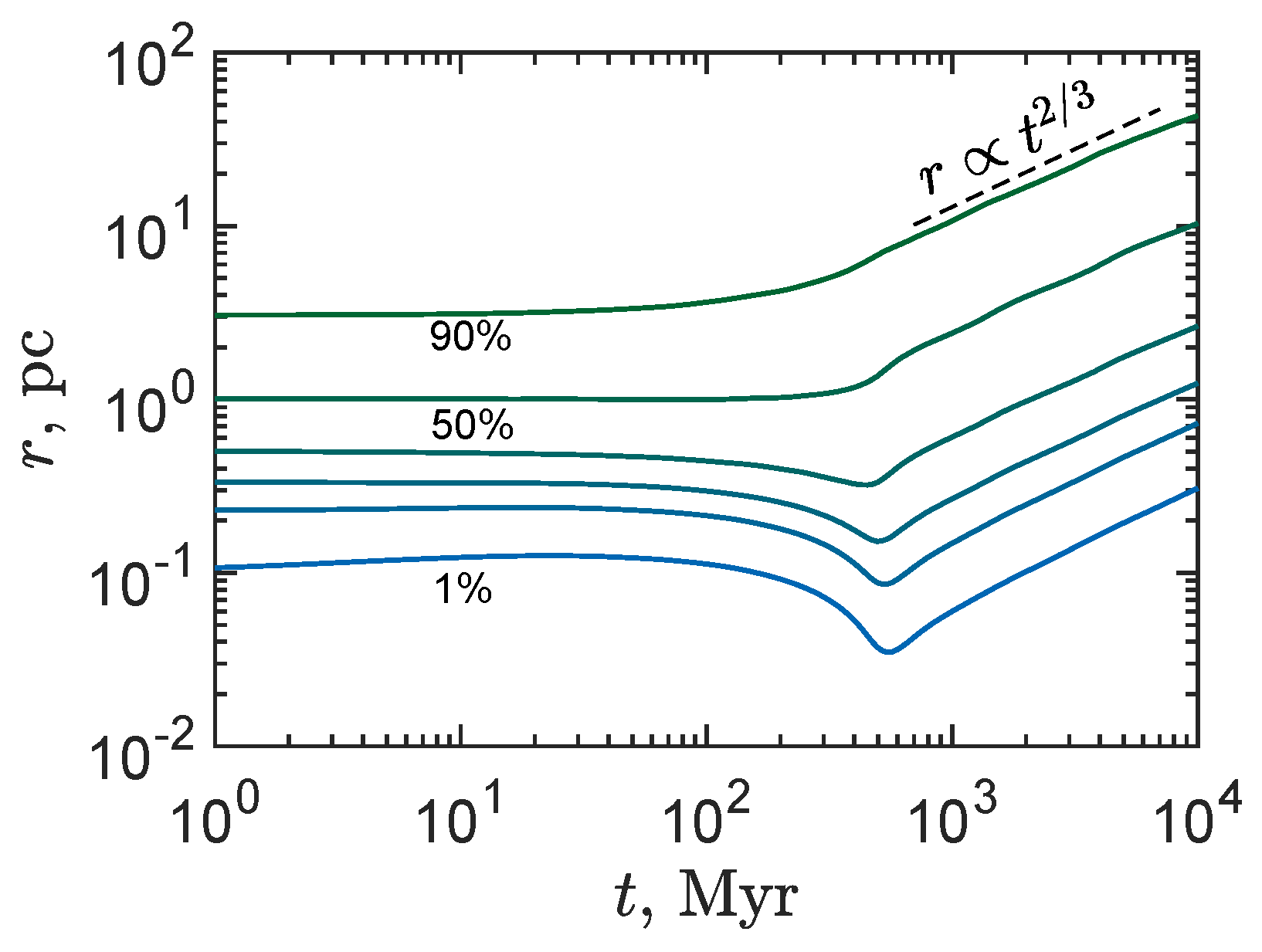
4.1. PBHs Clusters with Monochromatic Mass Spectra
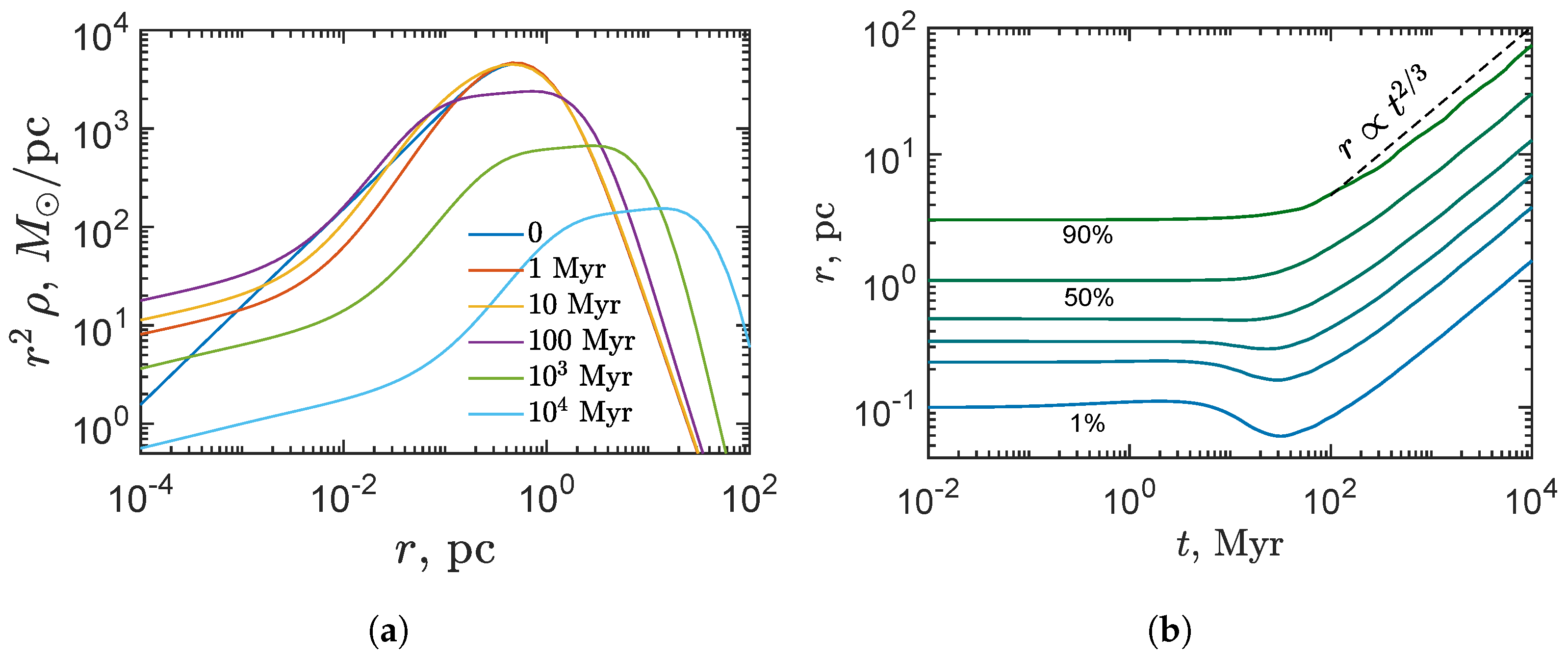
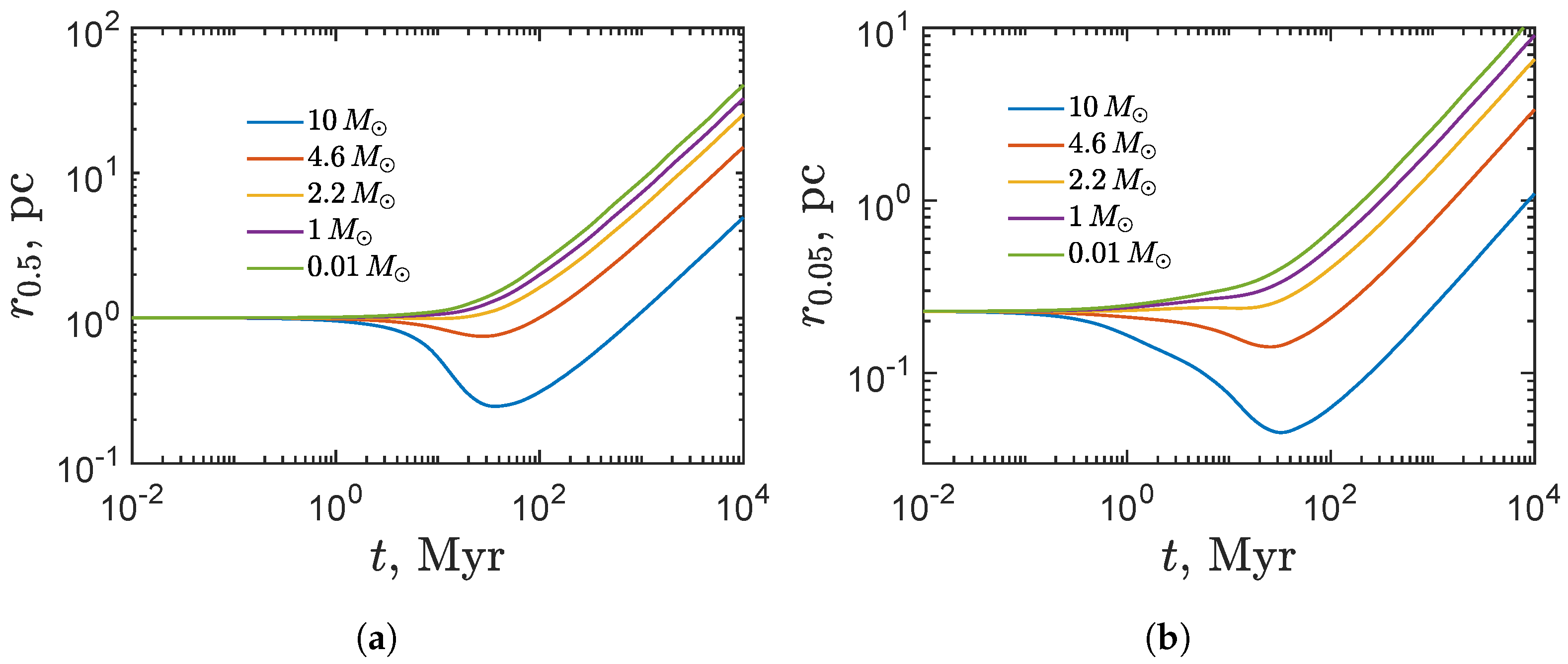
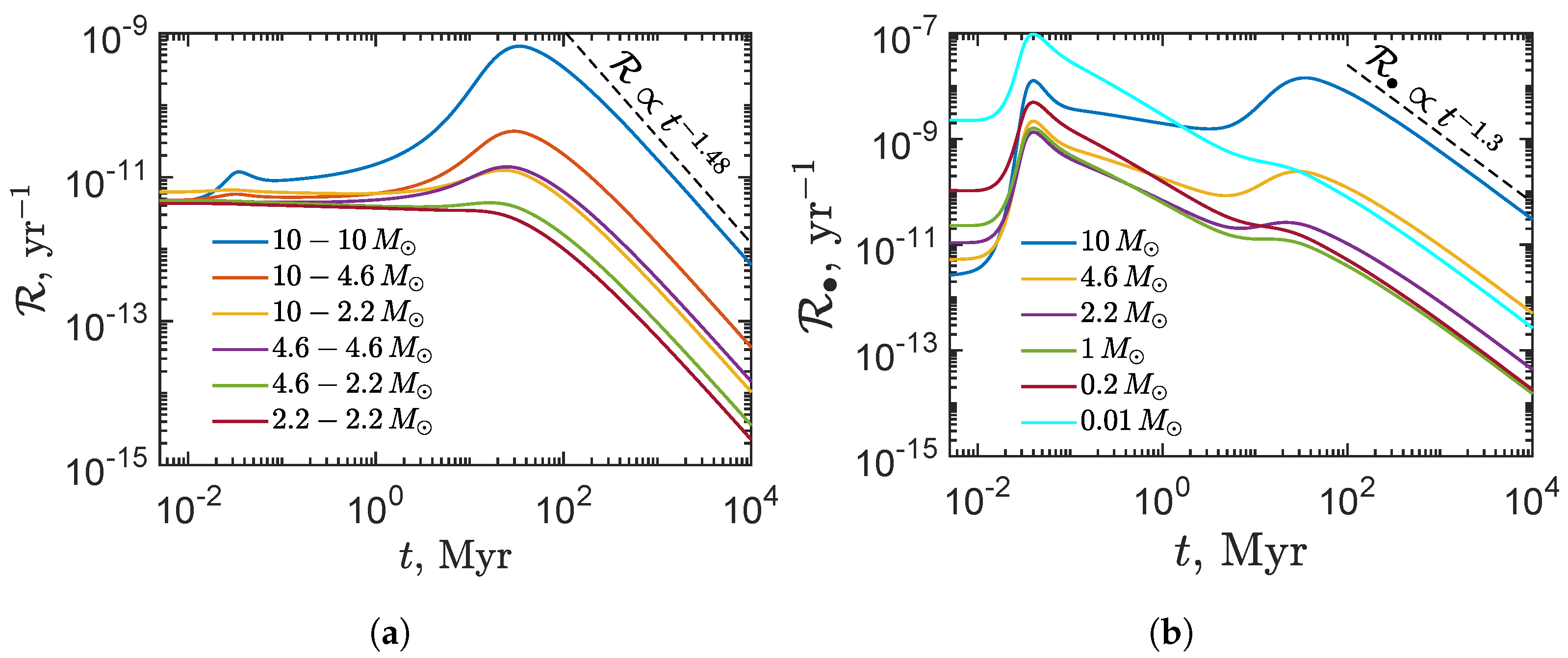
4.2. PBHs Clusters with Wide Mass Spectra
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zel’dovich, Y.B.; Novikov, I.D. The Hypothesis of Cores Retarded during Expansion and the Hot Cosmological Model. Sov. Astron. 1967, 10, 602. [Google Scholar]
- Hawking, S. Gravitationally collapsed objects of very low mass. Mon. Not. R. Astron. Soc. 1971, 152, 75. [Google Scholar] [CrossRef]
- Carr, B.J.; Hawking, S.W. Black holes in the early Universe. Mon. Not. R. Astron. Soc. 1974, 168, 399–416. [Google Scholar] [CrossRef]
- Dolgov, A.; Silk, J. Baryon isocurvature fluctuations at small scales and baryonic dark matter. Phys. Rev. D 1993, 47, 4244–4255. [Google Scholar] [CrossRef]
- Garcia-Bellido, J.; Linde, A.D.; Wands, D. Density perturbations and black hole formation in hybrid inflation. Phys. Rev. D 1996, 54, 6040–6058. [Google Scholar] [CrossRef] [PubMed]
- Jedamzik, K.; Niemeyer, J.C. Primordial black hole formation during first-order phase transitions. Phys. Rev. D 1999, 59, 124014. [Google Scholar] [CrossRef]
- Khlopov, M.Y. Primordial black holes. Res. Astron. Astrophys. 2010, 10, 495–528. [Google Scholar] [CrossRef]
- Kawasaki, M.; Kitajima, N.; Yanagida, T.T. Primordial black hole formation from an axionlike curvaton model. Phys. Rev. D 2013, 87, 063519. [Google Scholar] [CrossRef]
- Garcia-Bellido, J.; Ruiz Morales, E. Primordial black holes from single field models of inflation. Phys. Dark Univ. 2017, 18, 47–54. [Google Scholar] [CrossRef]
- Cotner, E.; Kusenko, A. Primordial Black Holes from Supersymmetry in the Early Universe. Phys. Rev. Lett. 2017, 119, 031103. [Google Scholar] [CrossRef]
- Belotsky, K.M.; Dokuchaev, V.I.; Eroshenko, Y.N.; Esipova, E.A.; Khlopov, M.Y.; Khromykh, L.A.; Kirillov, A.A.; Nikulin, V.V.; Rubin, S.G.; Svadkovsky, I.V. Clusters of Primordial Black Holes. Eur. Phys. J. C 2019, 79, 246. [Google Scholar] [CrossRef]
- Cotner, E.; Kusenko, A.; Sasaki, M.; Takhistov, V. Analytic description of primordial black hole formation from scalar field fragmentation. J. Cosmol. Astropart. Phys. 2019, 2019, 077. [Google Scholar] [CrossRef]
- Kusenko, A.; Sasaki, M.; Sugiyama, S.; Takada, M.; Takhistov, V.; Vitagliano, E. Exploring Primordial Black Holes from the Multiverse with Optical Telescopes. Phys. Rev. Lett. 2020, 125, 181304. [Google Scholar] [CrossRef] [PubMed]
- Carr, B.; Kuhnel, F. Primordial Black Holes as Dark Matter: Recent Developments. Ann. Rev. Nucl. Part. Sci. 2020, 70, 355–394. [Google Scholar] [CrossRef]
- Green, A.M.; Kavanagh, B.J. Primordial black holes as a dark matter candidate. J. Phys. Nucl. Phys. 2021, 48, 043001. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Bird, S.; Cholis, I.; Muñoz, J.B.; Ali-Haïmoud, Y.; Kamionkowski, M.; Kovetz, E.D.; Raccanelli, A.; Riess, A.G. Did LIGO Detect Dark Matter? Phys. Rev. Lett. 2016, 116, 201301. [Google Scholar] [CrossRef]
- Blinnikov, S.; Dolgov, A.; Porayko, N.K.; Postnov, K. Solving puzzles of GW150914 by primordial black holes. J. Cosmol. Astropart. Phys. 2016, 2016, 036. [Google Scholar] [CrossRef]
- Clesse, S.; García-Bellido, J. The clustering of massive Primordial Black Holes as Dark Matter: Measuring their mass distribution with advanced LIGO. Phys. Dark Univ. 2017, 15, 142–147. [Google Scholar] [CrossRef]
- Raidal, M.; Vaskonen, V.; Veermäe, H. Gravitational waves from primordial black hole mergers. J. Cosmol. Astropart. Phys. 2017, 2017, 037. [Google Scholar] [CrossRef]
- Clesse, S.; García-Bellido, J. Seven hints for primordial black hole dark matter. Phys. Dark Univ. 2018, 22, 137–146. [Google Scholar] [CrossRef]
- Raidal, M.; Spethmann, C.; Vaskonen, V.; Veermäe, H. Formation and evolution of primordial black hole binaries in the early universe. J. Cosmol. Astropart. Phys. 2019, 2019, 018. [Google Scholar] [CrossRef]
- Ali-Haïmoud, Y.; Kovetz, E.D.; Kamionkowski, M. Merger rate of primordial black-hole binaries. Phys. Rev. D 2017, 96, 123523. [Google Scholar] [CrossRef]
- Kimura, R.; Suyama, T.; Yamaguchi, M.; Zhang, Y.L. Reconstruction of primordial power spectrum of curvature perturbation from the merger rate of primordial black hole binaries. J. Cosmol. Astropart. Phys. 2021, 2021, 031. [Google Scholar] [CrossRef]
- Sasaki, M.; Takhistov, V.; Vardanyan, V.; Zhang, Y.l. Establishing the Non-Primordial Origin of Black Hole-Neutron Star Mergers. arXiv 2021, arXiv:2110.09509. [Google Scholar]
- Dolgov, A.D.; Kuranov, A.G.; Mitichkin, N.A.; Porey, S.; Postnov, K.A.; Sazhina, O.S.; Simkin, I.V. On mass distribution of coalescing black holes. J. Cosmol. Astropart. Phys. 2020, 2020, 017. [Google Scholar] [CrossRef]
- Inman, D.; Ali-Haïmoud, Y. Early structure formation in primordial black hole cosmologies. Phys. Rev. D 2019, 100, 083528. [Google Scholar] [CrossRef]
- Kashlinsky, A. LIGO gravitational wave detection, primordial black holes and the near-IR cosmic infrared background anisotropies. Astrophys. J. Lett. 2016, 823, L25. [Google Scholar] [CrossRef]
- Bañados, E.; Venemans, B.P.; Mazzucchelli, C.; Farina, E.P.; Walter, F.; Wang, F.; Decarli, R.; Stern, D.; Fan, X.; Davies, F.B.; et al. An 800-million-solar-mass black hole in a significantly neutral Universe at a redshift of 7.5. Nature 2018, 553, 473–476. [Google Scholar] [CrossRef]
- Matsuoka, Y.; Onoue, M.; Kashikawa, N.; Strauss, M.A.; Iwasawa, K.; Lee, C.H.; Imanishi, M.; Nagao, T.; Akiyama, M.; Asami, N.; et al. Discovery of the First Low-luminosity Quasar at z > 7. Astrophys. J. Lett. 2019, 872, L2. [Google Scholar] [CrossRef]
- Yang, J.; Wang, F.; Fan, X.; Hennawi, J.F.; Davies, F.B.; Yue, M.; Banados, E.; Wu, X.B.; Venemans, B.; Barth, A.J.; et al. Pōniuā’ena: A Luminous z = 7.5 Quasar Hosting a 1.5 Billion Solar Mass Black Hole. Astrophys. J. Lett. 2020, 897, L14. [Google Scholar] [CrossRef]
- Kohri, K.; Nakama, T.; Suyama, T. Testing scenarios of primordial black holes being the seeds of supermassive black holes by ultracompact minihalos and CMB μ distortions. Phys. Rev. D 2014, 90, 083514. [Google Scholar] [CrossRef]
- Valiante, R.; Agarwal, B.; Habouzit, M.; Pezzulli, E. On the Formation of the First Quasars. Publ. Astron. Soc. Aust. 2017, 34, e031. [Google Scholar] [CrossRef]
- Inayoshi, K.; Visbal, E.; Haiman, Z. The Assembly of the First Massive Black Holes. Ann. Rev. Astron. Astrophys. 2020, 58, 27–97. [Google Scholar] [CrossRef]
- Eroshenko, Y. Mergers of primordial black holes in extreme clusters and the H0 tension. Phys. Dark Univ. 2021, 32, 100833. [Google Scholar] [CrossRef]
- Rubin, S.G.; Khlopov, M.Y.; Sakharov, A.S. Primordial black holes from nonequilibrium second order phase transition. Grav. Cosmol. 2000, 6, 51–58. [Google Scholar]
- Rubin, S.G.; Sakharov, A.S.; Khlopov, M.Y. The Formation of Primary Galactic Nuclei during Phase Transitions in the Early Universe. J. Exp. Theor. Phys. 2001, 92, 921–929. [Google Scholar] [CrossRef]
- Khlopov, M.Y.; Rubin, S.G.; Sakharov, A.S. Primordial structure of massive black hole clusters. Astropart. Phys. 2005, 23, 265–277. [Google Scholar] [CrossRef]
- Ding, Q.; Nakama, T.; Silk, J.; Wang, Y. Detectability of gravitational waves from the coalescence of massive primordial black holes with initial clustering. Phys. Rev. D 2019, 100, 103003. [Google Scholar] [CrossRef]
- Matsubara, T.; Terada, T.; Kohri, K.; Yokoyama, S. Clustering of primordial black holes formed in a matter-dominated epoch. Phys. Rev. D 2019, 100, 123544. [Google Scholar] [CrossRef]
- Young, S.; Byrnes, C.T. Initial clustering and the primordial black hole merger rate. J. Cosmol. Astropart. Phys. 2020, 2020, 004. [Google Scholar] [CrossRef]
- Kawasaki, M.; Murai, K.; Nakatsuka, H. Strong clustering of primordial black holes from Affleck-Dine mechanism. J. Cosmol. Astropart. Phys. 2021, 2021, 025. [Google Scholar] [CrossRef]
- Afshordi, N.; McDonald, P.; Spergel, D.N. Primordial Black Holes as Dark Matter: The Power Spectrum and Evaporation of Early Structures. Astrophys. J. Lett. 2003, 594, L71–L74. [Google Scholar] [CrossRef]
- Jedamzik, K. Primordial black hole dark matter and the LIGO/Virgo observations. J. Cosmol. Astropart. Phys. 2020, 2020, 022. [Google Scholar] [CrossRef]
- De Luca, V.; Desjacques, V.; Franciolini, G.; Riotto, A. The clustering evolution of primordial black holes. J. Cosmol. Astropart. Phys. 2020, 2020, 028. [Google Scholar] [CrossRef]
- Dokuchaev, V.I.; Eroshenko, Y.N. A Stochastic Model for Correlations between Central Black Hole Masses and Galactic Bulge Velocity Dispersions. Astron. Lett. 2001, 27, 759–764. [Google Scholar] [CrossRef]
- Trashorras, M.; García-Bellido, J.; Nesseris, S. The Clustering Dynamics of Primordial Black Boles in N-Body Simulations. Universe 2021, 7, 18. [Google Scholar] [CrossRef]
- Korol, V.; Mandel, I.; Miller, M.C.; Church, R.P.; Davies, M.B. Merger rates in primordial black hole clusters without initial binaries. Mon. Not. R. Astron. Soc. 2020, 496, 994–1000. [Google Scholar] [CrossRef]
- Toshchenko, K.A.; Belotsky, K.M. Studying method of microlensing effect estimation for a cluster of primordial black holes. J. Phys. Conf. Ser. 2019, 1390, 012087. [Google Scholar] [CrossRef]
- Cohn, H. Numerical integration of the Fokker-Planck equation and the evolution of star clusters. Astrophys. J. 1979, 234, 1036–1053. [Google Scholar] [CrossRef]
- Cohn, H. Late core collapse in star clusters and the gravothermal instability. Astrophys. J. 1980, 242, 765–771. [Google Scholar] [CrossRef]
- Quinlan, G.D.; Shapiro, S.L. Dynamical Evolution of Dense Clusters of Compact Stars. Astrophys. J. 1989, 343, 725. [Google Scholar] [CrossRef]
- Chernoff, D.F.; Weinberg, M.D. Evolution of Globular Clusters in the Galaxy. Astrophys. J. 1990, 351, 121. [Google Scholar] [CrossRef]
- Murphy, B.W.; Cohn, H.N.; Durisen, R.H. Dynamical and Luminosity Evolution of Active Galactic Nuclei: Models with a Mass Spectrum. Astrophys. J. 1991, 370, 60. [Google Scholar] [CrossRef]
- Vasiliev, E. A New Fokker-Planck Approach for the Relaxation-driven Evolution of Galactic Nuclei. Astrophys. J. 2017, 848, 10. [Google Scholar] [CrossRef]
- Merritt, D. Evolution of Nuclear Star Clusters. Astrophys. J. 2009, 694, 959–970. [Google Scholar] [CrossRef][Green Version]
- Merritt, D. Gravitational Encounters and the Evolution of Galactic Nuclei. I. Method. Astrophys. J. 2015, 804, 52. [Google Scholar] [CrossRef]
- Lynden-Bell, D.; Wood, R. The gravo-thermal catastrophe in isothermal spheres and the onset of red-giant structure for stellar systems. Mon. Not. R. Astron. Soc. 1968, 138, 495. [Google Scholar] [CrossRef]
- Spitzer, L. Dynamical Evolution of Globular Clusters; Princeton University Press: Princeton, NJ, USA, 1987. [Google Scholar]
- Heggie, D.C. Binary evolution in stellar dynamics. Mon. Not. R. Astron. Soc. 1975, 173, 729–787. [Google Scholar] [CrossRef]
- Hut, P.; McMillan, S.; Goodman, J.; Mateo, M.; Phinney, E.S.; Pryor, C.; Richer, H.B.; Verbunt, F.; Weinberg, M. Binaries in Globular Clusters. Publ. Astron. Soc. Pac. 1992, 104, 981. [Google Scholar] [CrossRef]
- Breen, P.G.; Heggie, D.C. Gravothermal oscillations in multicomponent models of star clusters. Mon. Not. R. Astron. Soc. 2012, 425, 2493–2500. [Google Scholar] [CrossRef][Green Version]
- Goodman, J.; Hut, P. Primordial binaries and globular cluster evolution. Nature 1989, 339, 40–42. [Google Scholar] [CrossRef]
- Heggie, D.C.; Trenti, M.; Hut, P. Star clusters with primordial binaries-I. Dynamical evolution of isolated models. Mon. Not. R. Astron. Soc. 2006, 368, 677–689. [Google Scholar] [CrossRef][Green Version]
- Shapiro, S.L. Star clusters, self-interacting dark matter halos, and black hole cusps: The fluid conduction model and its extension to general relativity. Phys. Rev. D 2018, 98, 023021. [Google Scholar] [CrossRef] [PubMed]
- Mouri, H.; Taniguchi, Y. Runaway Merging of Black Holes: Analytical Constraint on the Timescale. Astrophys. J. Lett. 2002, 566, L17–L20. [Google Scholar] [CrossRef]
- García-Bellido, J.; Jaraba, S.; Kuroyanagi, S. The stochastic gravitational wave background from close hyperbolic encounters of primordial black holes in dense clusters. arXiv 2021, arXiv:2109.11376. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. The Classical Theory of Fields, 4th Revised English ed.; Morton, H., Translator; Pergamon Press: Oxford, UK, 1975. [Google Scholar]
- Stasenko, V.D.; Kirillov, A.A. The Merger Rate of Black Holes in a Primordial Black Hole Cluster. Physics 2021, 3, 372–378. [Google Scholar] [CrossRef]
- Merritt, D. Dynamics and Evolution of Galactic Nuclei; Princeton University Press: Princeton, NJ, USA, 2013. [Google Scholar]
- Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, A.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. Population Properties of Compact Objects from the Second LIGO-Virgo Gravitational-Wave Transient Catalog. Astrophys. J. Lett. 2021, 913, L7. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stasenko, V.D.; Kirillov, A.A.; Belotsky, K.M. Dynamics and Merger Rate of Primordial Black Holes in a Cluster. Universe 2022, 8, 41. https://doi.org/10.3390/universe8010041
Stasenko VD, Kirillov AA, Belotsky KM. Dynamics and Merger Rate of Primordial Black Holes in a Cluster. Universe. 2022; 8(1):41. https://doi.org/10.3390/universe8010041
Chicago/Turabian StyleStasenko, Viktor D., Alexander A. Kirillov, and Konstantin M. Belotsky. 2022. "Dynamics and Merger Rate of Primordial Black Holes in a Cluster" Universe 8, no. 1: 41. https://doi.org/10.3390/universe8010041
APA StyleStasenko, V. D., Kirillov, A. A., & Belotsky, K. M. (2022). Dynamics and Merger Rate of Primordial Black Holes in a Cluster. Universe, 8(1), 41. https://doi.org/10.3390/universe8010041






