Quark Clusters, QCD Vacuum and the Cosmological 7Li, Dark Matter and Dark Energy Problems
Abstract
1. Introduction
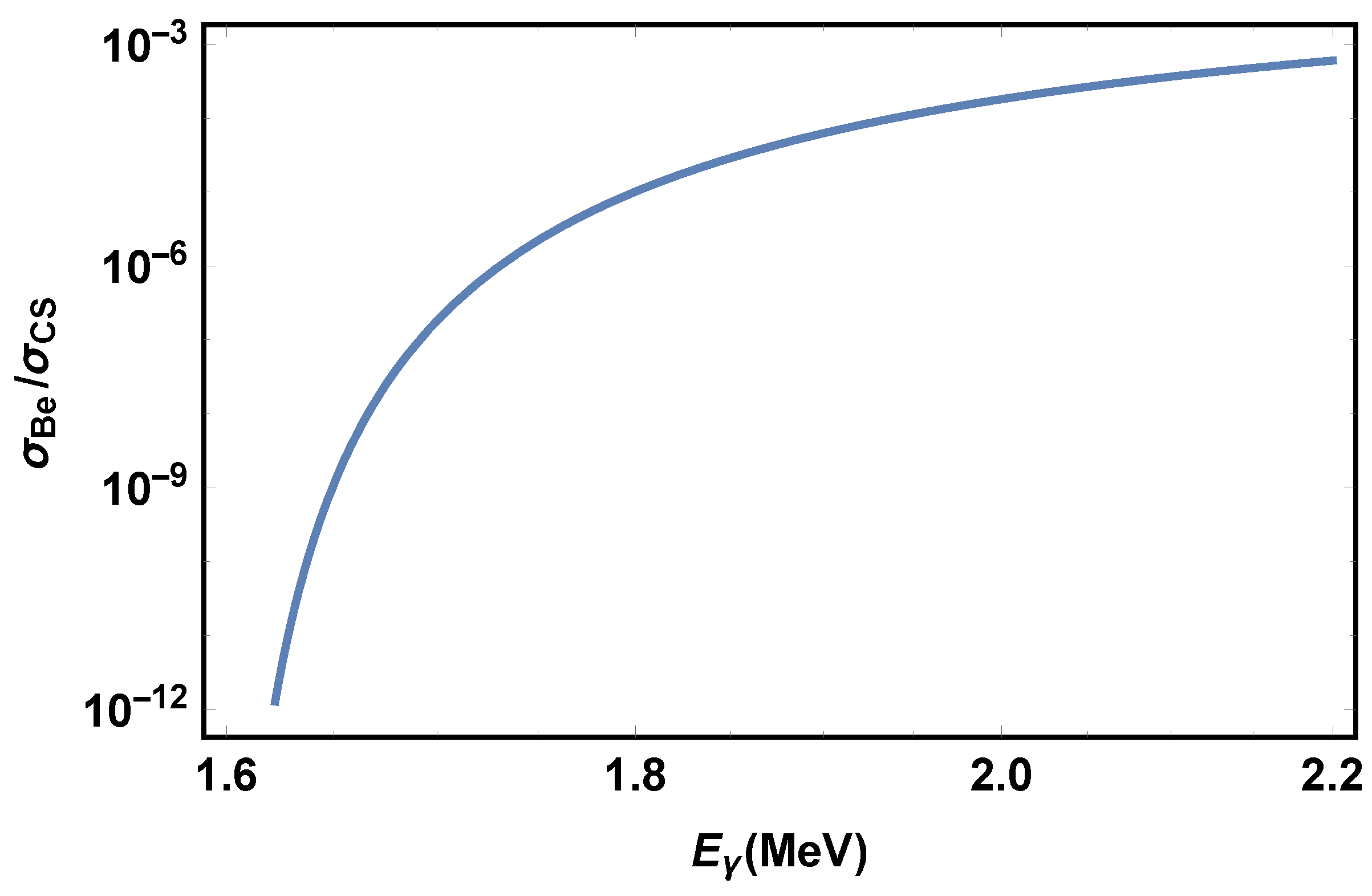
2. Post-BBN 7Be Destruction
2.1. The Mono-Chromatic ∼2 MeV Line
2.2. The SQC Size and Baryon Number
3. SQCs and Dark Energy (DE)
4. SQCs as Cold Dark Matter (CDM)
4.1. The Pre-LGB Era
4.2. The Post-LGB Era
5. Discussion
- 1.
- The evolution of the SQC and of the DM densities: We first recall, from Equation (9), that the density inside an SQC evolves from to after the LGB phase. Leakage further decreases the SQC density to an asymptotic value of at (which we associate with ). The minimum possible value of an SQC would be given by the bare quarks masses, or % of their original mass. However, we find and to be on the order of tens of percent each (see Section 3), and an SQC would lose a fraction of its mass to DE over the age of the universe. Meaning that the DM content in today’s universe is a fraction of that in the pre-BBN era according to our model.Averaging the total SQC mass over the Hubble volume gives us the DM density in our model. The ratio of the total amount of DM to that of the baryonic matter evolves from a maximum value of before LGB decay to after the LGB decay to photons just before leakage starts. At full leakage, which we associate with , the ratio isAssuming as measured today and extrapolating back to the LGB era, it means that the amount of DM content is larger than what we used in Section 2, where we set (i.e., when taking ).
- 2.
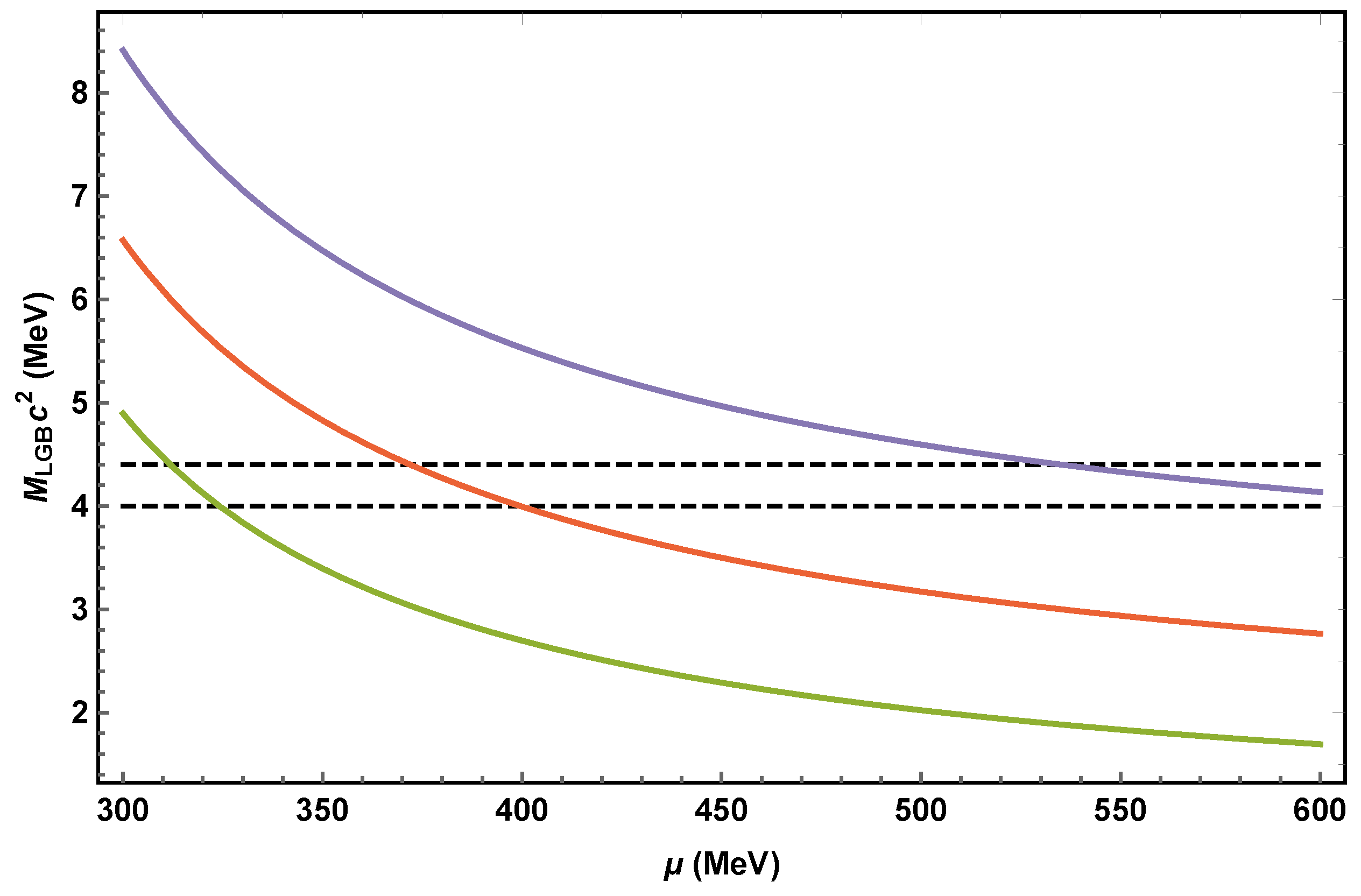
- The 7Li problem revisited: The solution to the cosmological 7Li problem presented in Section 2 did not take into account the further loss of gluonic energy due to in-SQC vacuum leakage following LGB decay as discussed above. From Equation (14), and when plugged into Equation (4), we obtainEquation (5) becomesThat is, a smaller percentage of the gluonic energy of the SQCs converted to LGBs could resolve the 7Li problem. For , we recover the values we arrived at in Section 2, while for , we obtain , which is less than the maximum expected from the 2SC-phase.The ratio between the DE density and the DM energy density at is (from Equation (7)),To have as measured in today’s universe requires , in which case Equation (14) gives and . On the other hand, the resolution of the Hubble tension suggests that a favored parameter combination lies around , as illustrated in Figure 4. This discrepancy could potentially be mitigated by relaxing some of the approximations made in our cosmological model or by adopting a more realistic approach to the tunneling process, moving beyond the simple assumption of exponential decay.
- 3.
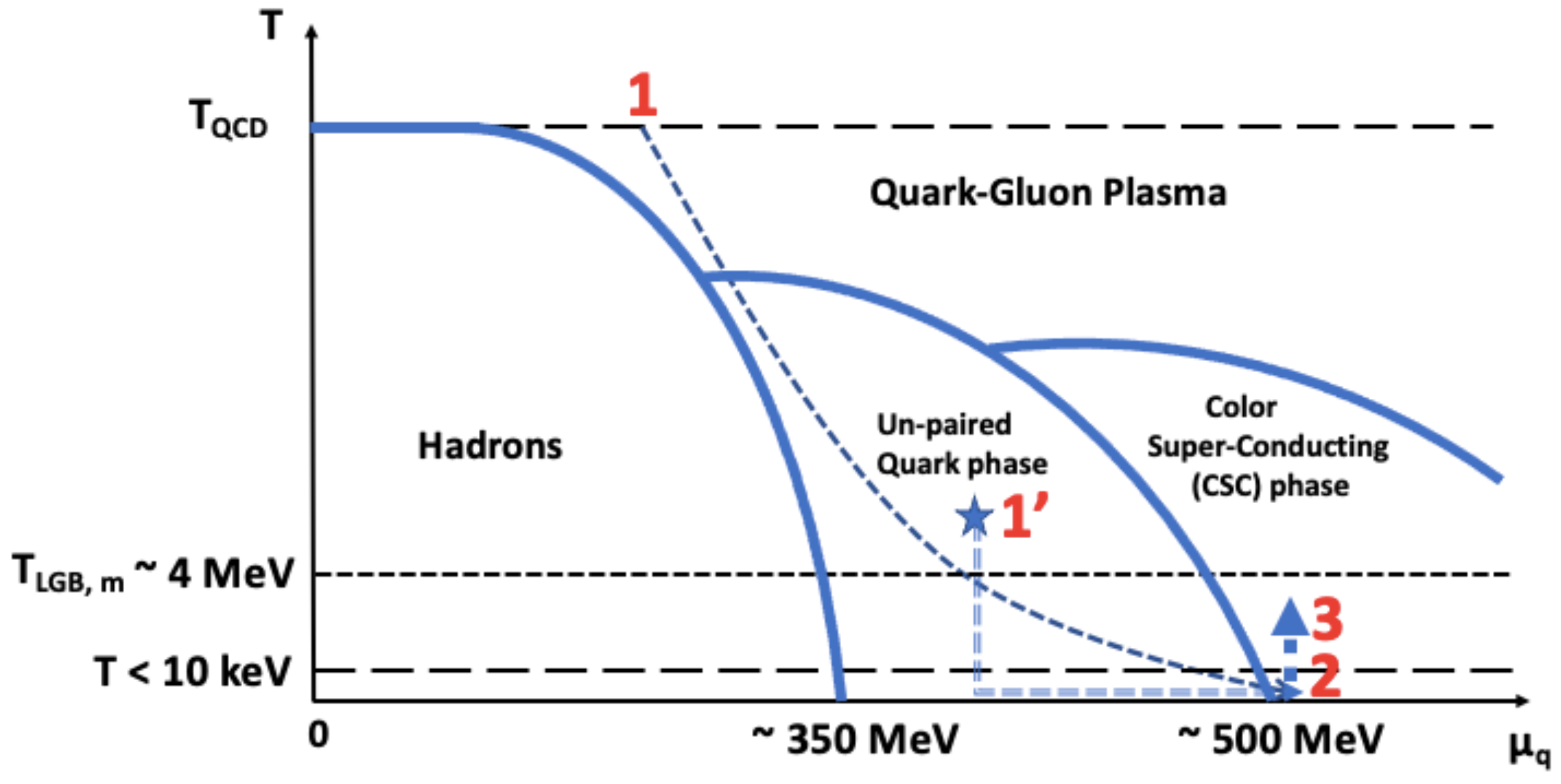
- The CSC phase and neutron stars: Figure 2 shows a suggested pathway, starting at point “”, an NS core could take to enter the CSC phase since conversion following SQC capture is suppressed (see Section 4). An NS born with (or which acquiring through evolution) a core in the unpaired phase could transition to the CSC phase by a sequence of cooling (to keV temperature via the URCA process, e.g., [36]) and compression (to MeV via mass accretion). Take an NS with a core making up a fraction of the total mass. The energy released from the conversion of gluonic condensation (e.g., LGBs) to photons is . Comparing this to the NS binding energy , we conclude that NSs with compactness parametermay be completely obliterated in the process; the NS mass and radius are in units of solar mass, , and cm, respectively. NSs with higher compactness parameters would lose mass, leaving behind a pure CSC core. In this latter case, the conversion to a CSC star puts a constraint on due to the black hole limit 2 with as the radius of the CSC star. With , this gives which is consistent with the MeV (i.e., a 2SC-like phase) adopted in our model. Thus, if some NSs follow a path as suggested in Figure 2, the resulting photon fireball may have interesting implications for explosive astrophysics.
- 4.
- SQC–hadron decoupling: Following the leakage and loss of more gluonic energy, it is not unreasonable to assume that the quarks within the SQC become undressed and should in principle decouple (or at least experience some level of decoupling) from the strong interaction. They would still rely on gluons to remain bound while exhibiting minimal interaction with hadrons. We speculate that DM and hadrons may represent separate phases of quark matter within the framework of QCD, characterized by distinct vacuum properties. If our model is correct, it allows a unique connection between cosmology and the properties of the QCD vacuum in the CSC quark phase. An estimate of the parameter from cosmological observations may be an indication of the contribution of the QCD condensates in CSC to the SQC mass, which may have implications, albeit indirect, to the mass of hadrons. The exact details of the SQC–hadron decoupling remain to be worked out.
- 5.
- SQCs and LGBs in today’s detectors: SQCs (the DM in our model; see Section 4) would interact only gravitationally and would thus evade detection in current DM experiments. Instead, we propose that our model can be tested by experiments that can access the 2SC phase at temperatures below the LGB melting temperature; i.e., at MeV (see Appendix C). The Compressed Baryonic Matter experiment (CBM) at FAIR explores the QCD phase diagram in the region of high baryon densities (representative of neutron star densities) and moderate temperatures using high-energy nucleus–nucleus collisions (e.g., [37,38]). We note that the 2SC phase carries MeV LGBs (i.e., can solve the cosmological 7Li problem) at a baryonic density as low as a few times that of nuclear matter (see Figure 3) and that LGBs form on strong interaction timescales and decay to MeV photons on timescales of ∼ s. Thus, experiments such as the CBM at FAIR could potentially be used to detect the photons from the LGB decay.The width of the line can be found in Equation (A8) in Appendix C, and for typical values, it is expected to be <0.1 MeV. If this width were to be measured, it would provide a direct estimation of the 2SC dielectric constant, which could then be used to infer the ratio and consequently determine (as per Equation (A8)). However, it remains unclear whether and how the CBM experiment could explore the temperature regime relevant to our study. More significantly, the 2 MeV line may be overshadowed by the anticipated high low-energy electromagnetic background in high-energy ion–ion collisions. Even if the background were to diminish as the QGP transitions into the 2SC phase and subsequently cools down to the LGB phase ( MeV), detecting the 2 MeV line would likely prove challenging. Calculating the strength of the LGB 2 MeV line is beyond the scope of this paper.
- 6.
- The stability of the CSC phase: Our findings seem to hint at the standard neutral 2SC phase (adopted in our theoretical framework) as the unspecified CSC phase. However, the 2SC phase may be unstable at small superconducting gap () values due to the mismatch in the up and down quarks chemical potential [39]. It suggests that either the 2SC phase is stable in the regime of chemical potential () and values we used or that another stable 2SC-like phase exists in nature and remains to be identified. A 2SCus phase, which has u-s pairs instead of u-d pairs, is also a candidate. This phase, however, faces a similar challenge in that the u and s Fermi momenta are split apart by the strange-quark mass (combined with electrical neutrality; e.g., [40]). The color-flavor-locked phase may be one candidate if the strange-quark effective mass is small or alternatively a crystalline quark phase if strange-quark mass is heavier (e.g., [41]). However, these phases do not possess properties that allow for the conversion of gluonic energy to photons. Despite its limitations, the u-d 2SC phase remains one of the candidate phases for dense quark matter (adopted in our model), pending the determination of its exact phase structure.Another possibility is collective excitations (i.e., phonons) of SQCs, which would decay directly to photons via phonon-to-photon conversion channels. Mono-chromatic photons of energy MeV would result if the SQC radius were fm. Physically, the decaying particle is not an LGB but is instead a long-wavelength mode that is resonant with the quark cluster containing it. In this scenario, higher resonant modes should also be excited, and these could affect the very sensitive D abundance, which is not desired. Superconducting strings [42] are an interesting avenue to explore in this context. If such domain walls could form during the cosmic QCD phase, one could imagine a scenario where they would evolve into 2SC-dominated strings in the post-BBN era. It remains to be shown that LGBs as described in our model could form in this case.
- 7.
- Matter–antimatter annihilation and SQC size: We hypothesize that each quark cluster is born with an anti-matter deficit of , meaning that there is one extra baryon per quark–antiquark pair; is the baryon-to-photon ratio. After annihilation (on timescales of s with mbarn), a cluster has only baryons left in it; this assumes that annihilation does not destroy the cluster, and instead it reduces it into a pure baryon cluster of radius where “f” stands for final. Here, fm is the typical size of an SQC set by the photon mean-free-path in the 2SC phase. In other words, we claim that the maximum size of the “shrapnel” of the annihilated much bigger parent cluster is on the order of (see Section 2.2). In this case, a cluster’s birth radius can be obtained from , which gives fm. Some constraints and implications to consider in the future include the following. (i) Annihilation should also yield pions. These would decay on weak-interaction timescales, and if their mean-free-path turns out to be much smaller than that of photons, they may affect the ∼ fm SQCs. (ii) While cluster formation (when the universe has aged such that its temperature is in the tens of MeV) is followed rapidly by annihilation, we must avoid the re-creation of matter–antimatter pairs, i.e., ensure that pair-creation timescales exceed the Hubble expansion timescale. (iii) In the framework we outline here, SQCs would require a formation mechanism that is different from that of the much larger cosmic strange-quark nuggets [14] (which require a first-order QCD phase transition) and Axion quark nuggets [15] (which require their co-existence with Axions). Additionally, the mechanism by which these nuggets can convert their stored gluonic energy into MeV photons is a serious limitation.
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Pedagogical Framework
Appendix A.1. A Quantum Chromodynamics (QCD) Phase Diagram
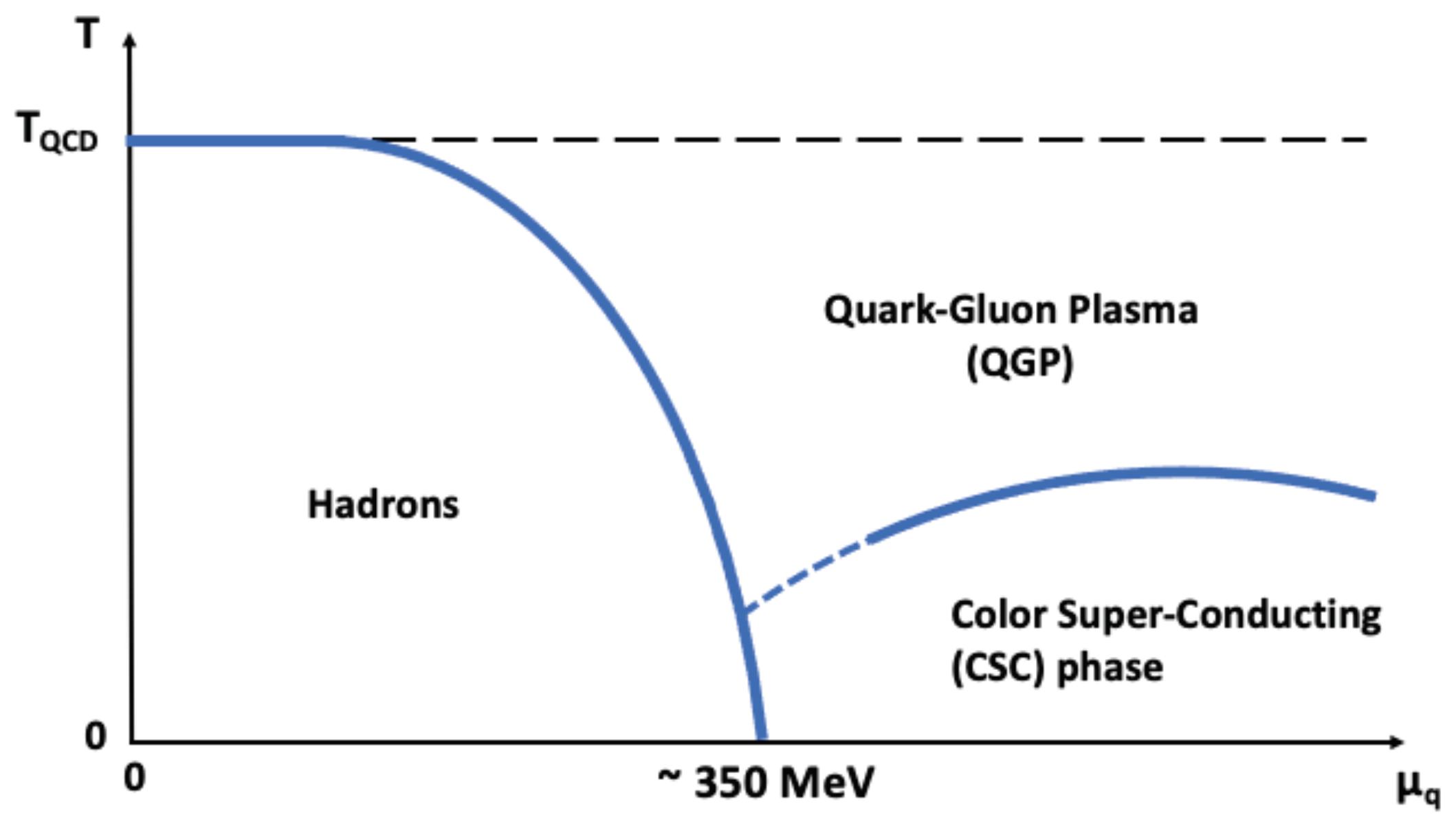
Appendix A.2. Our Model in a Nutshell
Appendix B. Post-BBN 7Be Destruction
Appendix C. Light Glueballs (LGBs) in the 2SC Phase
Appendix C.1. The Confined SUc(2) Phase and Its Vacuum
Appendix D. Our Cosmology
References
- Hoyle, F.; Tayler, R.J. The Mystery of the Cosmic Helium Abundance. Nature 1964, 203, 1108. [Google Scholar] [CrossRef]
- Peebles, J.P.E. Primordial Helium Abundance and the Primordial Fireball. II. Astrophys. J. 1966, 146, 542. [Google Scholar] [CrossRef]
- Wagoner, R.V.; Fowler, W.A.; Hoyle, F. On the Synthesis of Elements at Very High Temperatures. Astrophys. J. 1967, 148, 3. [Google Scholar] [CrossRef]
- Tytler, D.; O’Meara, J.M.; Suzuki, N.; Lubin, D. Review of Big Bang Nucleosynthesis and Primordial Abundances. Phys. Scripta 2000, T85, 12. [Google Scholar] [CrossRef]
- Lang, K.R. Astrophysical Formulae, Volume II: Space, Time, Matter and Cosmology; Springer: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Khatri, R.; Sunyaev, R.A. Time of primordial 7Be conversion into 7Li, energy release and doublet of narrow cosmological neutrino lines. Astron. Lett. 2011, 37, 367. [Google Scholar] [CrossRef]
- Spite, F.; Spite, M. Abundance of lithium in un-evolved stars and old disk stars: Interpretation and consequences. Astron. Astrophys. 1982, 115, 357. [Google Scholar]
- Fields, B.D. The Primordial Lithium Problem. Annu. Rev. Nucl. Part. Sci. 2011, 61, 47. [Google Scholar] [CrossRef]
- Berezinsky, V.S.; Bulanov, S.V.; Dogiel, V.A.; Ginzburg, V.L.; Ptuskin, V.S. Astrophysics of Cosmic Rays; North-Holland: Amsterdam, The Netherlands, 1990; 534p. [Google Scholar]
- Kawasaki, M.; Moroi, T. Electromagnetic Cascade in the Early Universe and Its Application to the Big Bang Nucleosynthesis. Astrophys. J. 1995, 452, 506. [Google Scholar] [CrossRef][Green Version]
- Poulin, V.; Serpico, P.D. Loophole to the universal photon spectrum in electromagnetic cascades: Application to the “cosmological lithium problem”. Phys. Rev. Lett. 2015, 114, 091101. [Google Scholar] [CrossRef]
- Protheroe, R.J.; Stanev, T.; Berezinsky, V.S. Electromagnetic cascades and cascade nucleosynthesis in the early Universe. Phys. Rev. D 1995, 51, 4134. [Google Scholar] [CrossRef][Green Version]
- Kawasaki, M.; Kohri, K.; Moroi, T.; Murai, K.; Murayama, H. Big Bang nucleosynthesis with sub-GeV massive decaying particles. J. Cosmol. Astropart. Phys. 2020, 2020, 048. [Google Scholar] [CrossRef]
- Witten, E. Cosmic separation of phases. Phys. Rev. D 1984, 30, 272. [Google Scholar] [CrossRef]
- Zhitnitsky, A.R. ‘Nonbaryonic’ dark matter as baryonic colour superconductor. J. Cosmol. Astropart. Phys. 2003, 2003, 010. [Google Scholar] [CrossRef]
- Ishida, H.; Kusakabe, M.; Okada, H. Effects of long-lived 10 MeV-scale sterile neutrinos on primordial elemental abundances and the effective neutrino number. Phys. Rev. D 2014, 90, 083519. [Google Scholar] [CrossRef]
- Rüster, S.B.; Shovkovy, I.A.; Rischke, D.H. Phase diagram of dense neutral three-flavor quark matter. Nucl. Phys. A 2004, 743, 127. [Google Scholar] [CrossRef]
- Alford, M.G.; Schmitt, A.; Rajagopal, K.; Schäfer, T. Color superconductivity in dense quark matter. Rev. Mod. Phys. 2008, 80, 1455. [Google Scholar] [CrossRef]
- Baym, G.; Hatsuda, T.; Kojo, T.; Powell, P.D.; Song, Y.; Takatsuka, T. From hadrons to quarks in neutron stars: A review. Rep. Prog. Phys. 2018, 81, 056902. [Google Scholar] [CrossRef]
- Brodsky, S.J.; Roberts, C.D.; Shrock, R.; Tandy, P.C. Confinement contains condensates. Phys. Rev. C 2012, 85, 065202. [Google Scholar] [CrossRef]
- Coleman, S.R. Fate of the false vacuum: Semiclassical theory. Phys. Rev. D 1977, 15, 2929, Erratum in Phys. Rev. D 1977, 16, 1248. [Google Scholar] [CrossRef]
- Cohen-Tannoudji, C.; Diu, B.; Laloe, F. Quantum Mechanics; Hermann and John Wiley & Sons: Paris, France, 2006. [Google Scholar]
- Kamionkowski, M.; Riess, A.G. The Hubble Tension and Early Dark Energy. Ann. Rev. Nucl. Part. Sci. 2023, 73, 153–180. [Google Scholar] [CrossRef]
- Schöneberg, N.; Abellán, G.F.; Sánchez, A.P.; Witte, S.J.; Poulin, V.; Lesgourgues, J. The H0 Olympics: A fair ranking of proposed models. Phys. Rep. 2022, 984, 1–55. [Google Scholar] [CrossRef]
- Garrett, K.; Gintaras, D. Dark Matter: A Primer. Adv. Astron. 2011, 2011, 968283. [Google Scholar] [CrossRef]
- Schumann, M. Dark Matter 2014. Eur. Phys. J. Web Conf. 2015, 96, 01027. [Google Scholar] [CrossRef]
- Bertone, G.; Hooper, D. History of dark matter. Rev. Mod. Phys. 2018, 90, 045002. [Google Scholar] [CrossRef]
- Kisslinger, L.S.; Das, D. A brief review of dark matter. Int. J. Mod. Phys. A 2019, 34, 1930013. [Google Scholar] [CrossRef]
- Oks, E. Brief Review of Recent Advances in Understanding Dark Matter and Dark Energy. New Astron. Rev. 2021, 93, 101632. [Google Scholar] [CrossRef]
- Arbey, A.; Mahmoudi, F. Dark matter and the early Universe: A review. Prog. Part. Nucl. Phys. 2021, 119, 103865. [Google Scholar] [CrossRef]
- Matsumoto, R. et al. [Super-Kamiokande Collaboration] Search for proton decay via p→μ+K0 in 0.37 megaton-years exposure of Super-Kamiokande. arXiv 2002, arXiv:2208.13188. [Google Scholar]
- Sofue, Y. Rotation Curve of the Milky Way and the Dark Matter Density. Galaxies 2020, 8, 37. [Google Scholar] [CrossRef]
- Kanno, S.; Soda, J. Exact Coleman-De Luccia instantons. Int. J. Mod. Phys. 2012, 21, 1250040. [Google Scholar] [CrossRef]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. The Structure of Cold Dark Matter Halos. Astrophys. J. 1996, 462, 563. [Google Scholar] [CrossRef]
- Abel, T.; Bryan, G.L.; Norman, M.L. The Formation of the First Star in the Universe. Science 2002, 295, 93. [Google Scholar] [CrossRef]
- Paczyński, B. Carbon Ignition in Degenerate Stellar Cores. Astrophys. J. 1972, 11, 53. [Google Scholar]
- Ablyazimov, T.; Abuhoza, A.; Adak, R.P.; Adamczyk, M.; Agarwal, K.; Aggarwal, M.M.; Ahammed, Z.; Ahmad, F.; Ahmad, N.; Ahmad, S.; et al. Challenges in QCD matter physics—The scientific programme of the Compressed Baryonic Matter experiment at FAIR. Eur. Phys. J. 2017, 53, 60. [Google Scholar] [CrossRef]
- Senger, P. Status of the Compressed Baryonic Matter experiment at FAIR. Int. J. Mod. Phys. E 2020, 29, 2030001. [Google Scholar] [CrossRef]
- Huang, M.; Shovkovy, I.A. Chromomagnetic instability in dense quark matter. Phys. Rev. D 2004, 70, 051501. [Google Scholar] [CrossRef][Green Version]
- Alford, M.; Kouvaris, C.; Rajagopal, K. Evaluating the gapless color-flavor locked phase. Phys. Rev. D 2005, 71, 054009. [Google Scholar] [CrossRef]
- Cao, G.; He, L.; Zhuang, P. Solid-state calculation of crystalline color superconductivity. Phys. Rev. 2015, 91, 114021. [Google Scholar] [CrossRef]
- Haber, A.; Schmitt, A. New color-magnetic defects in dense quark matter. J. Phys. Nucl. Phys. 2018, 45, 065001. [Google Scholar] [CrossRef]
- Guenther, J.N. Overview of the QCD phase diagram—Recent progress from the lattice. Eur. Phys. J. A 2021, 57, 136. [Google Scholar] [CrossRef] [PubMed]
- Alford, M. Color superconducting quark matter. Annu. Rev. Nucl. Part. Sci. 2001, 51, 131. [Google Scholar] [CrossRef]
- Svensson, R.; Zdziarski, A.A. Photon-Photon Scattering of Gamma Rays at Cosmological Distances. Astrophys. J. 1990, 349, 415. [Google Scholar] [CrossRef]
- Ouyed, R.; Sannino, F. The Glueball sector of two-flavor Color Superconductivity. Phys. Lett. B 2001, 511, 66. [Google Scholar] [CrossRef][Green Version]
- Sannino, F.; Marchal, N.; Schäfer, W. Partial Deconfinement in Color Superconductivity. Phys. Rev. D 2002, 66, 016007. [Google Scholar] [CrossRef][Green Version]
- Rischke, D.H.; Son, D.T.; Stephanov, M.A. Asymptotic Deconfinement in High-Density QCD. Phys. Rev. Lett. 2001, 87, 062001. [Google Scholar] [CrossRef]
- Alford, M.; GRajagopal, K.; Reddy, S.; FWilczek, F. Minimal color-flavor-locked–nuclear interface. Phys. Rev. D 2001, 64, 074017. [Google Scholar] [CrossRef]
- Shovkovy, I.A. Lectures delivered at the IARD 2004 conference, Saas Fee, Switzerland, June 12–19, and at the Helmholtz International Summer School and Workshop on Hot points in Astrophysics and Cosmology, JINR, Dubna, Russia, Aug. 2–13. arXiv 2004, arXiv:nucl-th/0410091. [Google Scholar]
- Satz, H. Phase transitions in QCD. Nucl. Phys. A 2001, 681, 3. [Google Scholar] [CrossRef][Green Version]
- Addazi, A.; Marcianò, A.; Pasechnik, R.; Prokhorov, G. Mirror symmetry of quantum Yang-Mills vacua and cosmological implications. Eur. Phys. J. 2019, 79, 251. [Google Scholar] [CrossRef]
- Aghanim, N. et al. [Planck Collaboration] Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2018, 641, A6. [Google Scholar]
- Ryden, B. Introduction to Cosmology, 2nd ed.; Cambridge University Press: Cambridge, UK, 2016. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ouyed, R.; Leahy, D.; Koning, N.; Jaikumar, P. Quark Clusters, QCD Vacuum and the Cosmological 7Li, Dark Matter and Dark Energy Problems. Universe 2024, 10, 115. https://doi.org/10.3390/universe10030115
Ouyed R, Leahy D, Koning N, Jaikumar P. Quark Clusters, QCD Vacuum and the Cosmological 7Li, Dark Matter and Dark Energy Problems. Universe. 2024; 10(3):115. https://doi.org/10.3390/universe10030115
Chicago/Turabian StyleOuyed, Rachid, Denis Leahy, Nico Koning, and Prashanth Jaikumar. 2024. "Quark Clusters, QCD Vacuum and the Cosmological 7Li, Dark Matter and Dark Energy Problems" Universe 10, no. 3: 115. https://doi.org/10.3390/universe10030115
APA StyleOuyed, R., Leahy, D., Koning, N., & Jaikumar, P. (2024). Quark Clusters, QCD Vacuum and the Cosmological 7Li, Dark Matter and Dark Energy Problems. Universe, 10(3), 115. https://doi.org/10.3390/universe10030115






