Accurate Closed-Form Solution for Moving Underwater Vehicle Localization Using Two-Way Travel Time
Abstract
:1. Introduction
- a new time delay measurement model that accounts for the AUV motion during the TWTT is proposed in the paper. it is unbiased and accurate for the moving AUV localization.
- the CRLBs are derived using the proposed model for the two cases in range-only AUV localization. it is proved that the AUV localization accuracy can be improved by measuring the AUV depth.
- a set of closed-form solutions are constructed using time delay measurement for the two cases in range-only AUV localization. The proposed solutions are computationally efficient and can achieve the CRLB analytically. Simulations validate the performance of the proposed solutions.
2. Models for AUV Localization
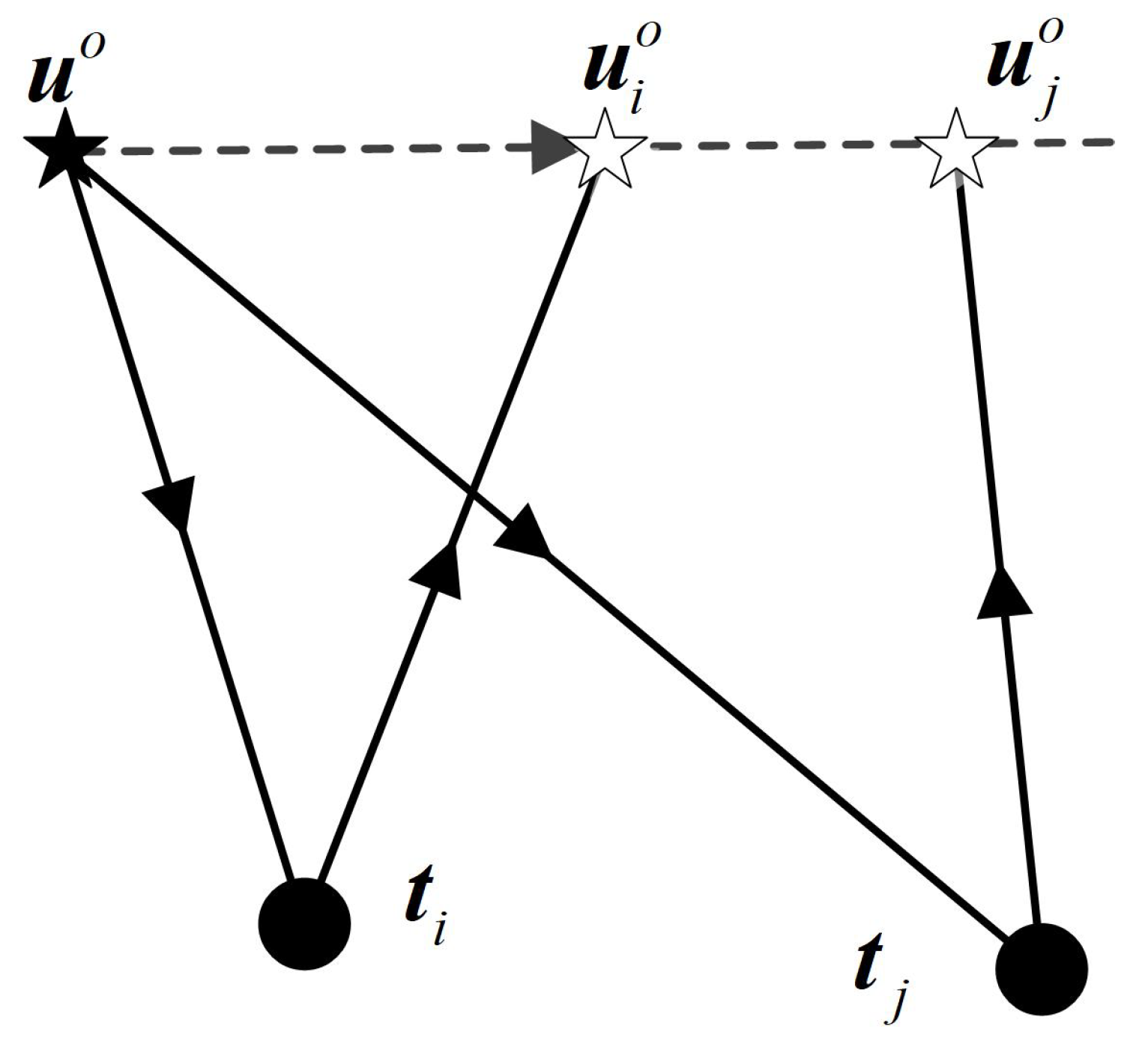
2.1. Moving Model
2.2. AUV Motion Effect and Static Model
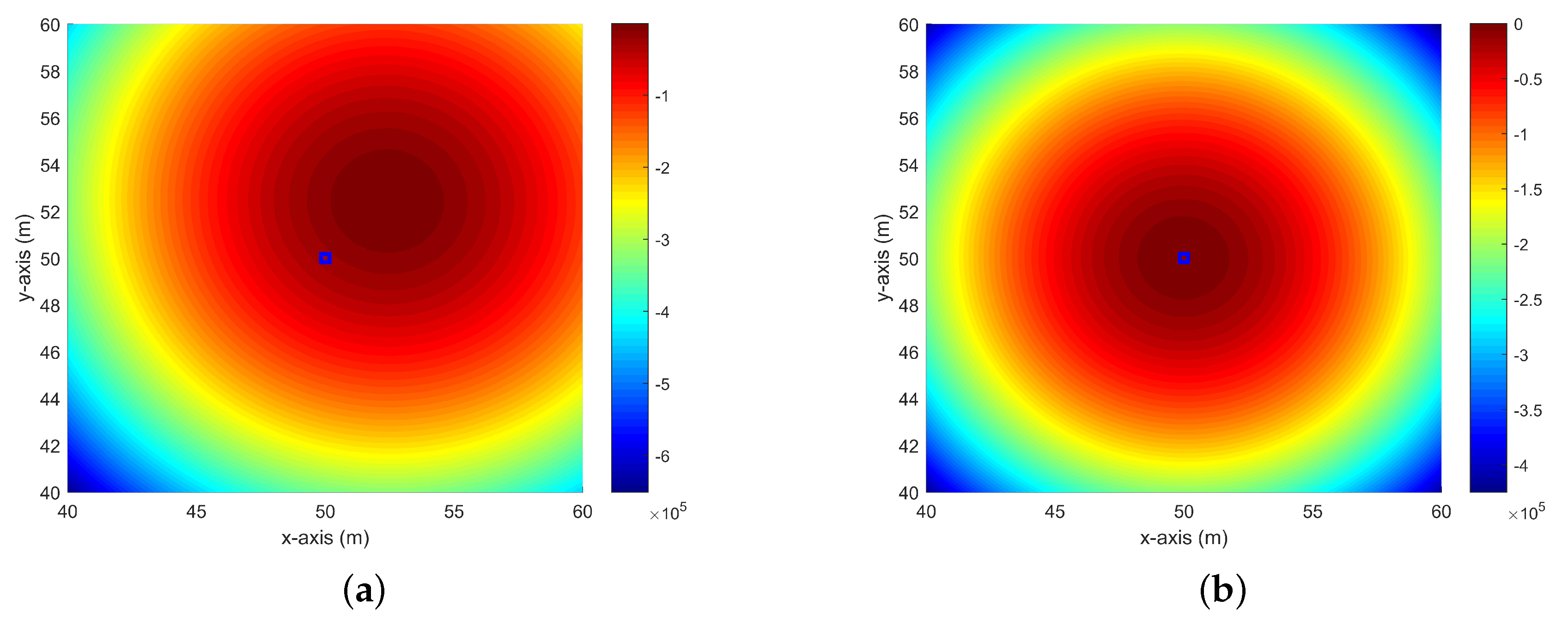
3. CRLB
3.1. AUV Localization with Unknown Depth
3.2. AUV Localization with Known Depth
3.3. Comparisons of the CRLBs of Proposed Cases
4. Closed-Form Solution
4.1. AUV Localization with Unknown Depth
4.1.1. First Stage
4.1.2. Second Stage
4.1.3. Third Stage
4.2. AUV Localization with Known Depth
4.2.1. First Stage
4.2.2. Second Stage
4.2.3. Third Stage
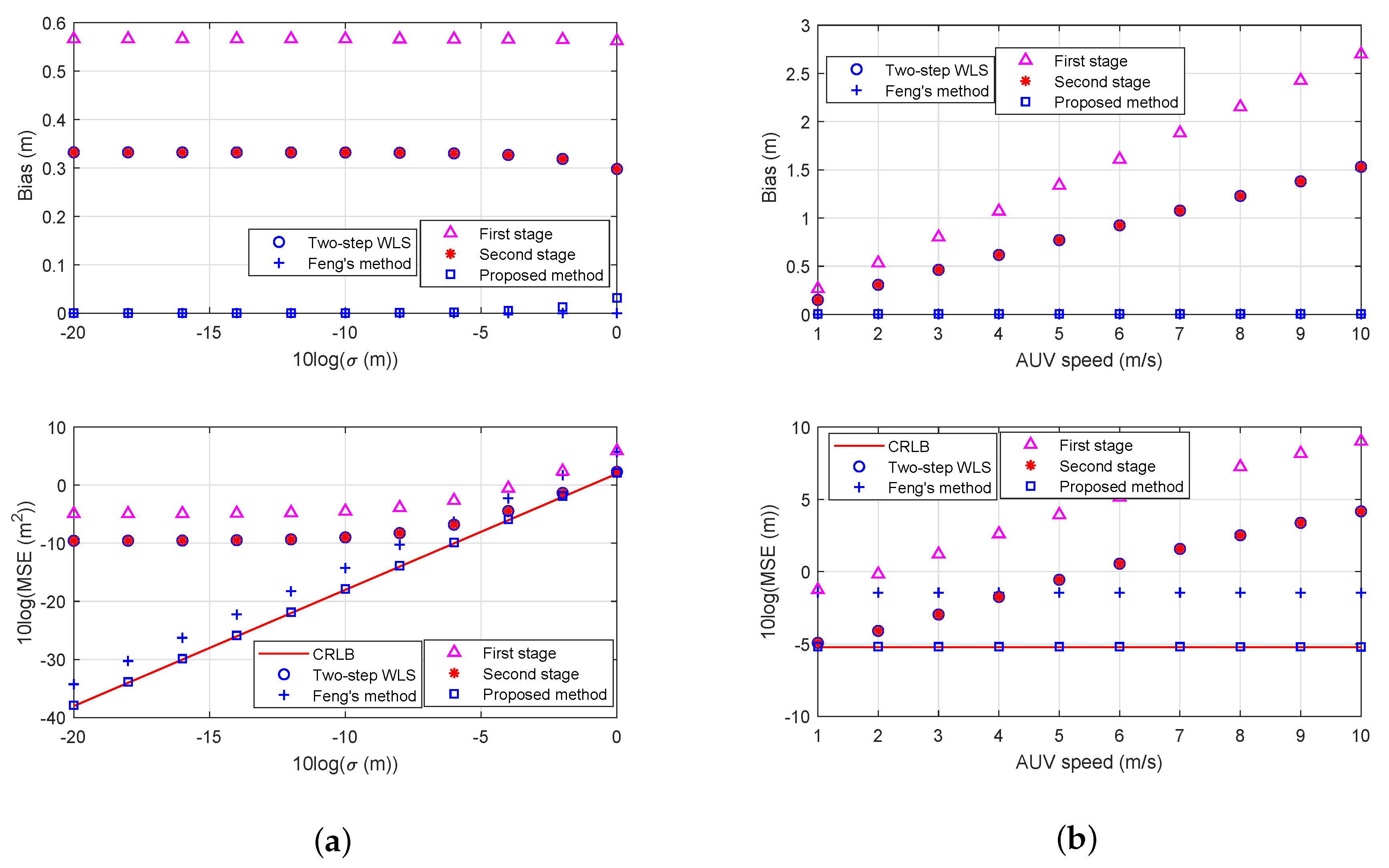
4.3. Performance Analysis
5. Simulation
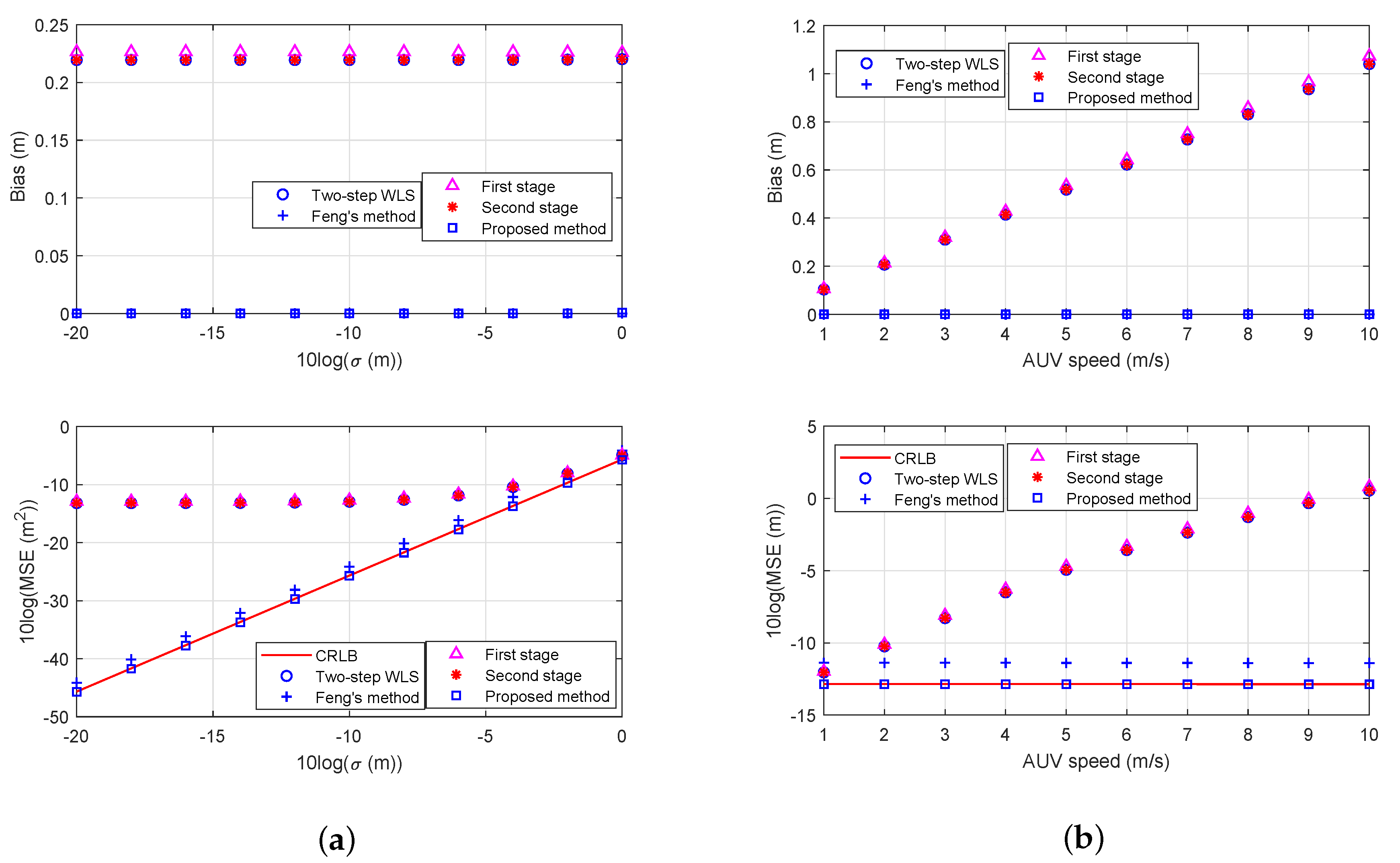
5.1. AUV Localization with Unknown Depth
5.2. AUV Localization with Known Depth
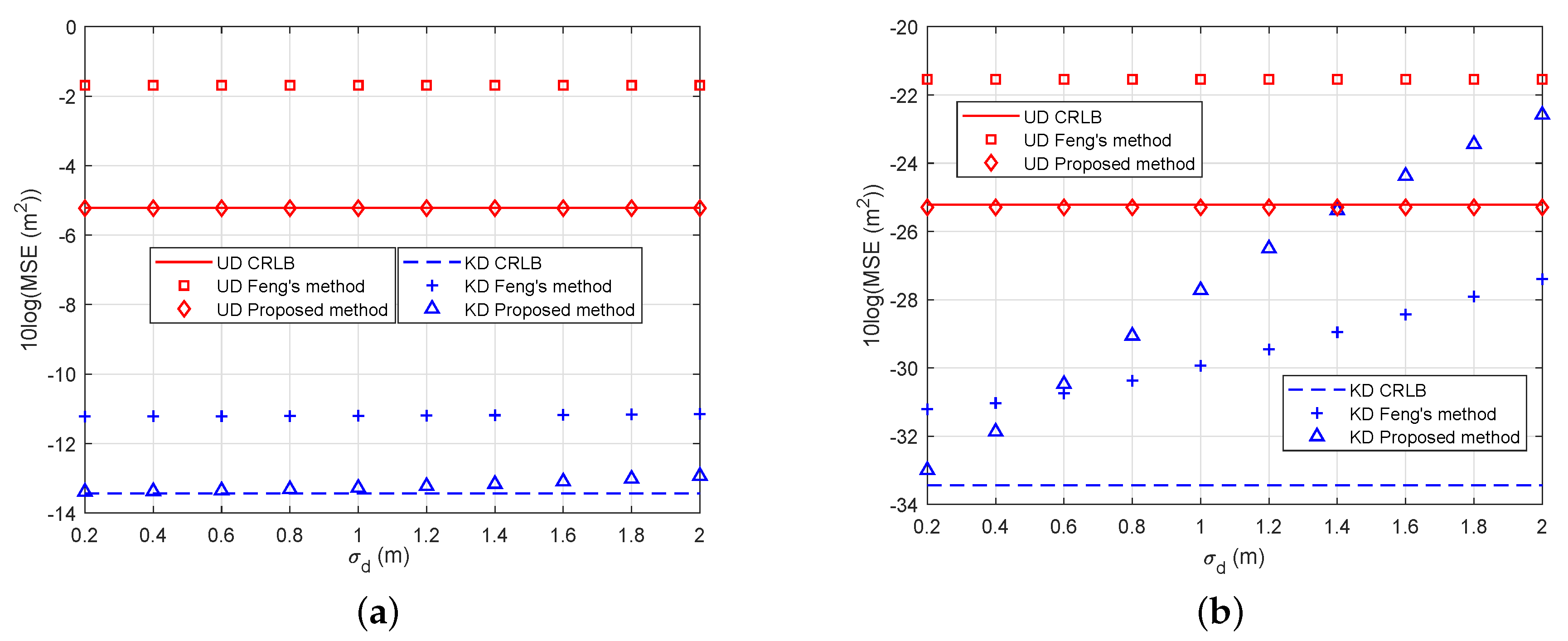
5.3. AUV Localization Performance When Error Occurring in Depth
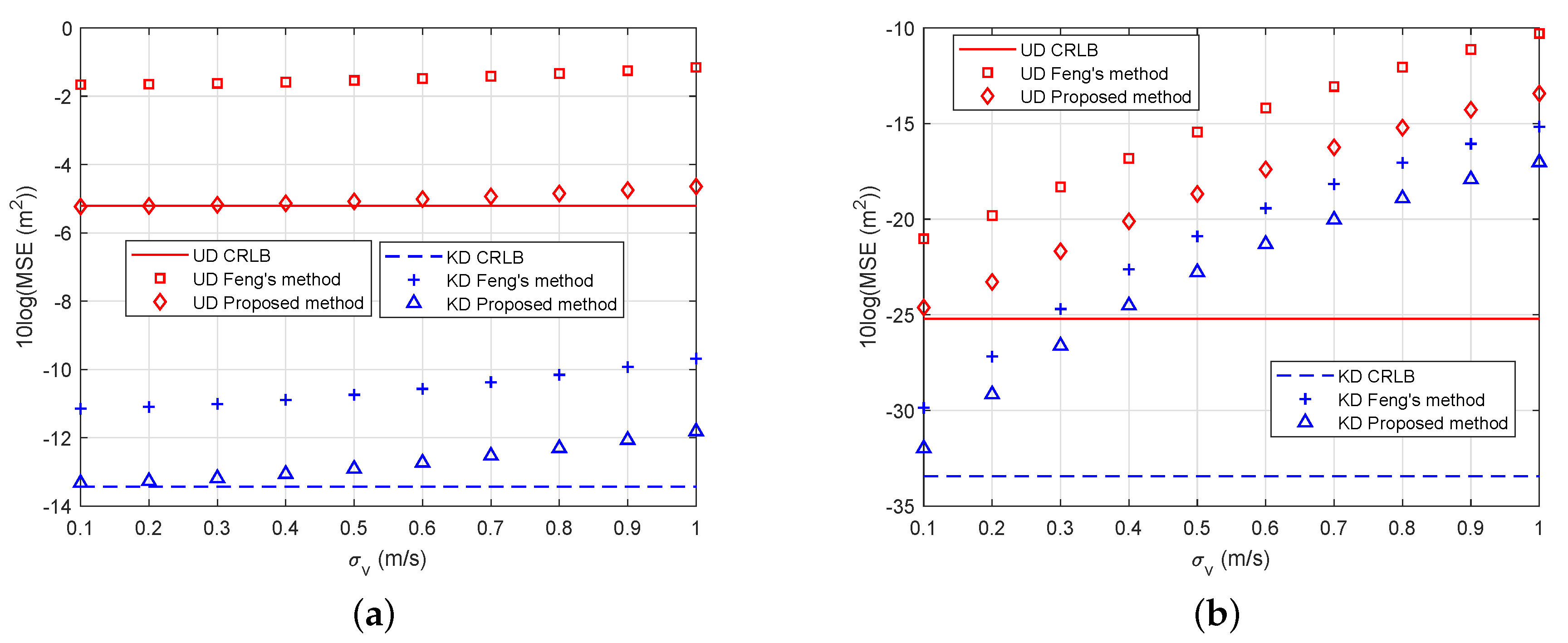
5.4. AUV Localization Performance When Error Occurring in Velocity
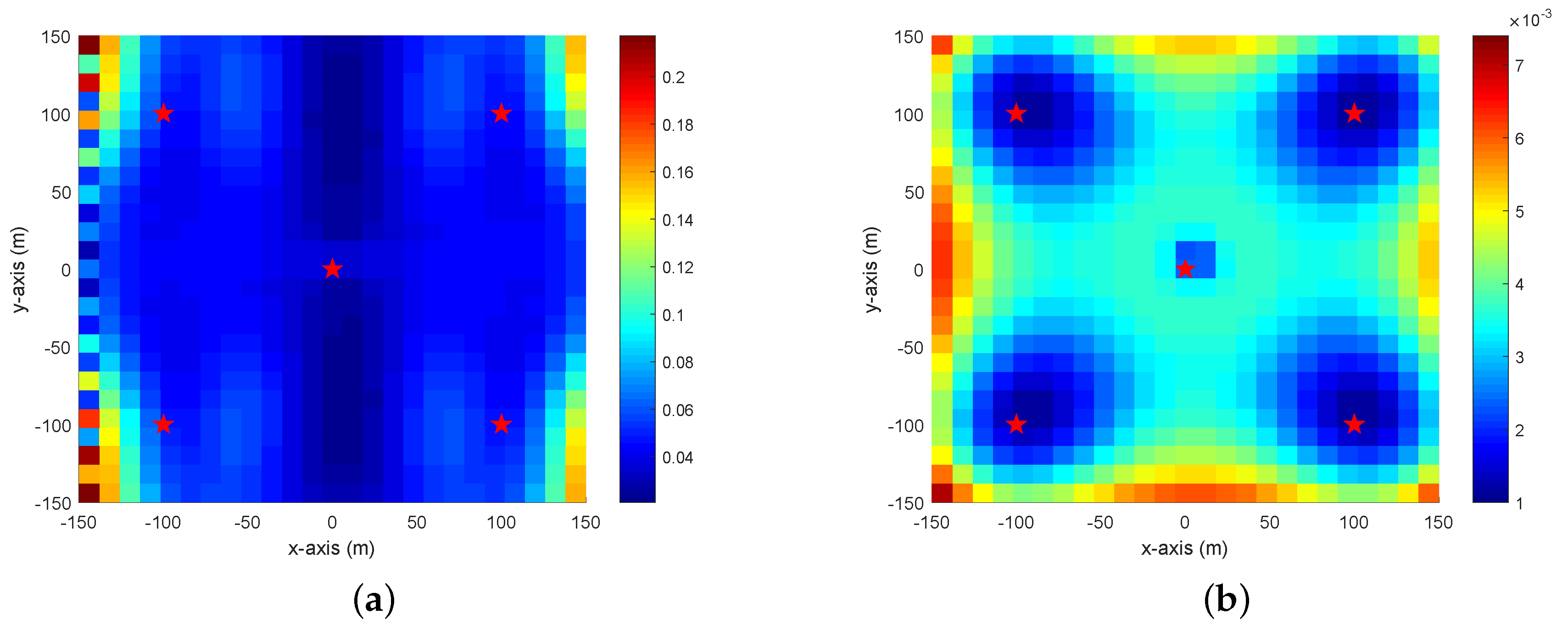
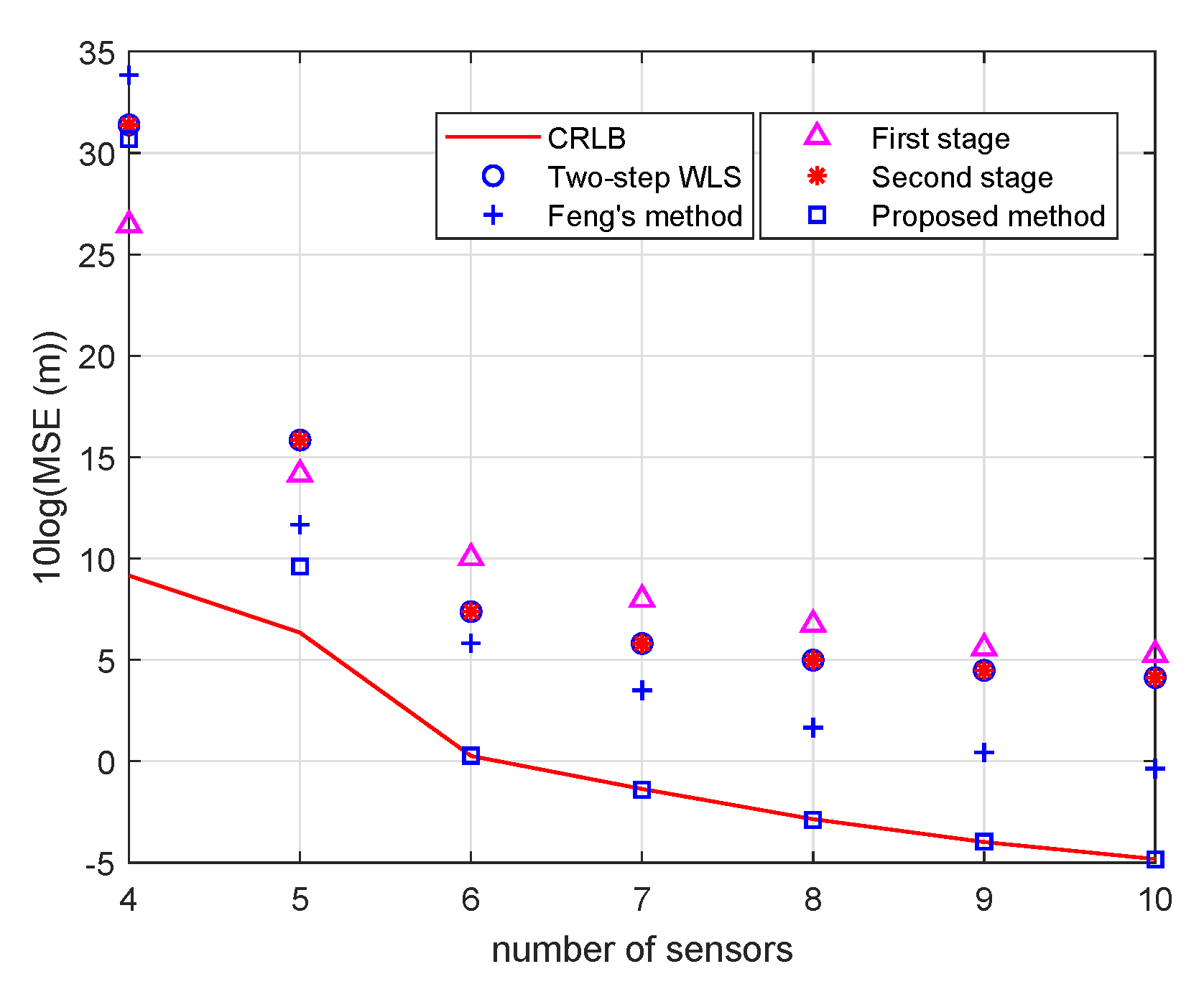
5.5. AUV Localization Performance with Randomly Generated Scenarios
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| TWTT | Two-Way Travel Time |
| OWTT | One-Way Travel Time |
| AUV | Autonomous Underwater Vehicle |
| LBL | Long Baseline |
| CRLB | Cramér–Rao Lower Bounds |
| MSE | Mean Square Error |
Appendix A
References
- Chen, L.; Wang, S.; McDonald-Maier, K.; Hu, H. Towards autonomous localization and mapping of AUVs: A survey. Int. J. Intell. Unmanned Syst. 2013, 1, 97–120. [Google Scholar] [CrossRef] [Green Version]
- Fang, J.; He, Z. Robust modified newton algorithms using tikhonov regularization for TDOA source localization. Circuits Syst. Signal Process. 2019, 38, 5342–5359. [Google Scholar] [CrossRef]
- Chen, X.; Wang, D.; Liu, R.; Yin, J.; Wu, Y. Structural total least squares algorithm for locating multiple disjoint sources based on AOA/TOA/FOA in the presence of system error. Front. Inf. Technol. Electron. Eng. 2018, 19, 917–936. [Google Scholar] [CrossRef]
- Yan, Y.; Shen, X.; Hua, F.; Zhong, X. On the Semidefinite Programming Algorithm for Energy-Based Acoustic Source Localization in Sensor Networks. IEEE Sens. J. 2018, 18, 8835–8846. [Google Scholar] [CrossRef]
- Paull, L.; Saeedi, S.; Seto, M.; Li, H. AUV navigation and localization: A review. IEEE J. Ocean. Eng. 2014, 39, 131–149. [Google Scholar] [CrossRef]
- Jia, T.; Wang, H.; Shen, X.; Liu, X.; Jing, H. Bearing-only multiple sources localization and the spatial spectrum. In Proceedings of the IEEE Oceans Conference, Aberdeen, UK, 19–22 June 2017; pp. 1–5. [Google Scholar]
- Gao, J.; Shen, X.; Mei, H.; Jia, T.; Wang, L.; Wang, H. A range-angle based self-localization scheme for MUANS. In Proceedings of the IEEE Oceans Conference, Aberdeen, UK, 19–22 June 2017; pp. 1–4. [Google Scholar]
- Gao, J.; Shen, X.; Mei, H.; Zhang, Z. Dynamic Reference Selection-Based Self-Localization Algorithm for Drifted Underwater Acoustic Networks. Sensors 2019, 19, 3920. [Google Scholar] [CrossRef] [Green Version]
- Zhong, X.; Premkumar, A.; Wang, H. Multiple wideband acoustic source tracking in 3-D space using a distributed acoustic vector sensor array. IEEE Sens. J. 2014, 14, 2502–2513. [Google Scholar] [CrossRef]
- Renner, C. Packet-based ranging with a low-power, low-cost acoustic modem for micro AUVs. In Proceedings of the International ITG Conference on Systems, Communications, and Coding, Hamburg, Germany, 6–9 February 2017; pp. 1–6. [Google Scholar]
- Sabet, M.T.; Sarhadi, P.; Zarini, M. Extended and Unscented Kalman filters for parameter estimation of an autonomous underwater vehicle. Ocean Eng. 2014, 91, 329–339. [Google Scholar] [CrossRef]
- Donovan, G.T. Position error correction for an autonomous underwater vehicle inertial navigation system (INS) using a particle filter. IEEE J. Ocean. Eng. 2012, 37, 431–445. [Google Scholar] [CrossRef]
- Yan, W.; Fang, X.; Li, J. Formation optimization for AUV localization with range-dependent measurements noise. IEEE Commun. Lett. 2014, 18, 1579–1582. [Google Scholar] [CrossRef]
- Jia, T.; Shen, X.; Wang, H. Multistatic Sonar Localization With a Transmitter. IEEE Access 2019, 7, 111192–111203. [Google Scholar] [CrossRef]
- Newman, P.; Leonard, J. Pure range-only sub-sea SLAM. In Proceedings of the IEEE International Conference on Robotics and Automation, Taipei, Taiwan, 14–19 September 2003; Volume 2, pp. 1921–1926. [Google Scholar]
- Olson, E.; Leonard, J.J.; Teller, S. Robust range-only beacon localization. IEEE J. Ocean. Eng. 2006, 31, 949–958. [Google Scholar] [CrossRef] [Green Version]
- Kussat, N.H.; Chadwell, C.D.; Zimmerman, R. Absolute positioning of an autonomous underwater vehicle using GPS and acoustic measurements. IEEE J. Ocean. Eng. 2005, 30, 153–164. [Google Scholar] [CrossRef]
- Webster, S.E.; Eustice, R.M.; Singh, H.; Whitcomb, L.L. Advances in single-beacon one-way-travel-time acoustic navigation for underwater vehicles. Int. J. Robot. Res. 2012, 31, 935–950. [Google Scholar] [CrossRef]
- Webster, S.E.; Walls, J.M.; Whitcomb, L.L.; Eustice, R.M. Decentralized extended information filter for single-beacon cooperative acoustic navigation: Theory and experiments. IEEE Trans. Robot. 2013, 29, 957–974. [Google Scholar] [CrossRef]
- Zhang, J.; Han, Y.; Zheng, C.; Sun, D. Underwater target localization using long baseline positioning system. Appl. Acoust. 2016, 111, 129–134. [Google Scholar] [CrossRef]
- Li, Z.; Dosso, S.E.; Sun, D. Motion-compensated acoustic localization for underwater vehicles. IEEE J. Ocean. Eng. 2016, 41, 840–851. [Google Scholar] [CrossRef]
- Jia, T.; Ho, K.C.; Wang, H.; Shen, X. Effect of Sensor Motion on Time Delay and Doppler Shift Localization: Analysis and Solution. IEEE Trans. Signal Process. 2019, 67, 5881–5895. [Google Scholar] [CrossRef]
- Kay, S.M. Fundamentals of Statistical Signal Processing: Estimation Theory; PTR Prentice Hall: Upper Saddle River, NJ, USA, 2010. [Google Scholar]
- Chan, Y.T.; Hang, H.Y.C.; Ching, P.C. Exact and approximate maximum likelihood localization algorithms. IEEE Trans. Veh. Technol. 2006, 55, 10–16. [Google Scholar] [CrossRef]
- Feng, J.; Ding, S.; Hui, J. A solution of locating a moving object using long-base line acoustic system. Acta Acust. 1996, 21, 832–837. [Google Scholar]
- Chan, Y.T.; Ho, K.C. A simple and efficient estimator for hyperbolic location. IEEE Trans. Signal Process. 1994, 42, 1905–1915. [Google Scholar] [CrossRef] [Green Version]
- Yang, L.; Yang, L.; Ho, K.C. Moving target localization in multistatic sonar by differential delays and Doppler shifts. IEEE Signal Process. Lett. 2016, 23, 1160–1164. [Google Scholar] [CrossRef]
- Beck, A.; Stoica, P.; Li, J. Exact and approximate solutions of source localization problems. IEEE Trans. Signal Process. 2008, 56, 1770–1778. [Google Scholar] [CrossRef]
- Ho, K.C.; Lu, X.; Kovavisaruch, L. Source localization using TDOA and FDOA measurements in the presence of receiver location errors: Analysis and solution. IEEE Trans. Signal Process. 2007, 55, 684–696. [Google Scholar] [CrossRef]
- Simakov, S. Localization in airborne multistatic sonars. IEEE J. Ocean. Eng. 2008, 33, 278–288. [Google Scholar] [CrossRef]
- Diamant, R.; Lampe, L. Underwater localization with time synchronization and propagation speed uncertainties. IEEE Trans. Mob. Comput. 2013, 12, 1257–1269. [Google Scholar] [CrossRef]
- Rui, L.; Ho, K.C. Efficient closed-form estimators for multistatic sonar localization. IEEE Trans. Aerosp. Electron. Syst. 2015, 51, 600–614. [Google Scholar] [CrossRef]
- Li, J.; Gao, H.; Zhang, S.; Chang, S.; Chen, J.; Liu, Z. Self-localization of autonomous underwater vehicles with accurate sound travel time solution. Comput. Electr. Eng. 2016, 50, 26–38. [Google Scholar] [CrossRef]
- Carter, G. Time delay estimation for passive sonar signal processing. IEEE Trans. Acoust. Speech Signal Process. 1981, 29, 463–470. [Google Scholar] [CrossRef] [Green Version]
- Gholami, M.R.; Gezici, S.; Strom, E.G. Improved position estimation using hybrid TW-TOA and TDOA in cooperative networks. IEEE Trans. Signal Process. 2012, 60, 3770–3785. [Google Scholar] [CrossRef]
- Gao, S.; Zhang, S.; Wang, G.; Li, Y. Robust second-order cone relaxation for TW-TOA-based localization with clock imperfection. IEEE Signal Process. Lett. 2016, 23, 1047–1051. [Google Scholar] [CrossRef]
- Sahinoglu, Z. Improving range accuracy of IEEE 802.15. 4a radios in the presence of clock frequency offsets. IEEE Commun. Lett. 2011, 15, 244–246. [Google Scholar] [CrossRef]
- Jia, T.; Wang, H.; Shen, X.; Jiang, Z.; He, K. Target localization based on structured total least squares with hybrid TDOA-AOA measurements. Signal Process. 2018, 143, 211–221. [Google Scholar] [CrossRef]
- Wei, H.; Wan, Q.; Chen, Z.; Ye, S. A novel weighted multidimensional scaling analysis for time-of-arrival-based mobile location. IEEE Trans. Signal Process. 2008, 56, 3018–3022. [Google Scholar] [CrossRef]
- Foy, W.H. Position-location solutions by Taylor-series estimation. IEEE Trans. Aerosp. Electron. Syst. 1976, 12, 187–194. [Google Scholar] [CrossRef]




| No. i | m | m | m |
|---|---|---|---|
| 1 | 100 | 100 | 30 |
| 2 | 100 | −100 | 30 |
| 3 | −100 | −100 | 30 |
| 4 | −100 | 100 | 30 |
| 5 | 0 | 0 | 0 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jia, T.; Wang, H.; Shen, X.; Yan, Y. Accurate Closed-Form Solution for Moving Underwater Vehicle Localization Using Two-Way Travel Time. Electronics 2020, 9, 565. https://doi.org/10.3390/electronics9040565
Jia T, Wang H, Shen X, Yan Y. Accurate Closed-Form Solution for Moving Underwater Vehicle Localization Using Two-Way Travel Time. Electronics. 2020; 9(4):565. https://doi.org/10.3390/electronics9040565
Chicago/Turabian StyleJia, Tianyi, Haiyan Wang, Xiaohong Shen, and Yongsheng Yan. 2020. "Accurate Closed-Form Solution for Moving Underwater Vehicle Localization Using Two-Way Travel Time" Electronics 9, no. 4: 565. https://doi.org/10.3390/electronics9040565
APA StyleJia, T., Wang, H., Shen, X., & Yan, Y. (2020). Accurate Closed-Form Solution for Moving Underwater Vehicle Localization Using Two-Way Travel Time. Electronics, 9(4), 565. https://doi.org/10.3390/electronics9040565





