A Mathematical Method of Current-Carrying Capacity for Shore Power Cables in Port Microgrids
Abstract
1. Introduction
2. Materials and Methods
2.1. Shore Power Cable Model
2.1.1. Simulation Model
2.1.2. Physical Model
2.2. Mathematical Algorithm for Ampacity Calculation of Shore Power Cable Using Heat Balance Equation
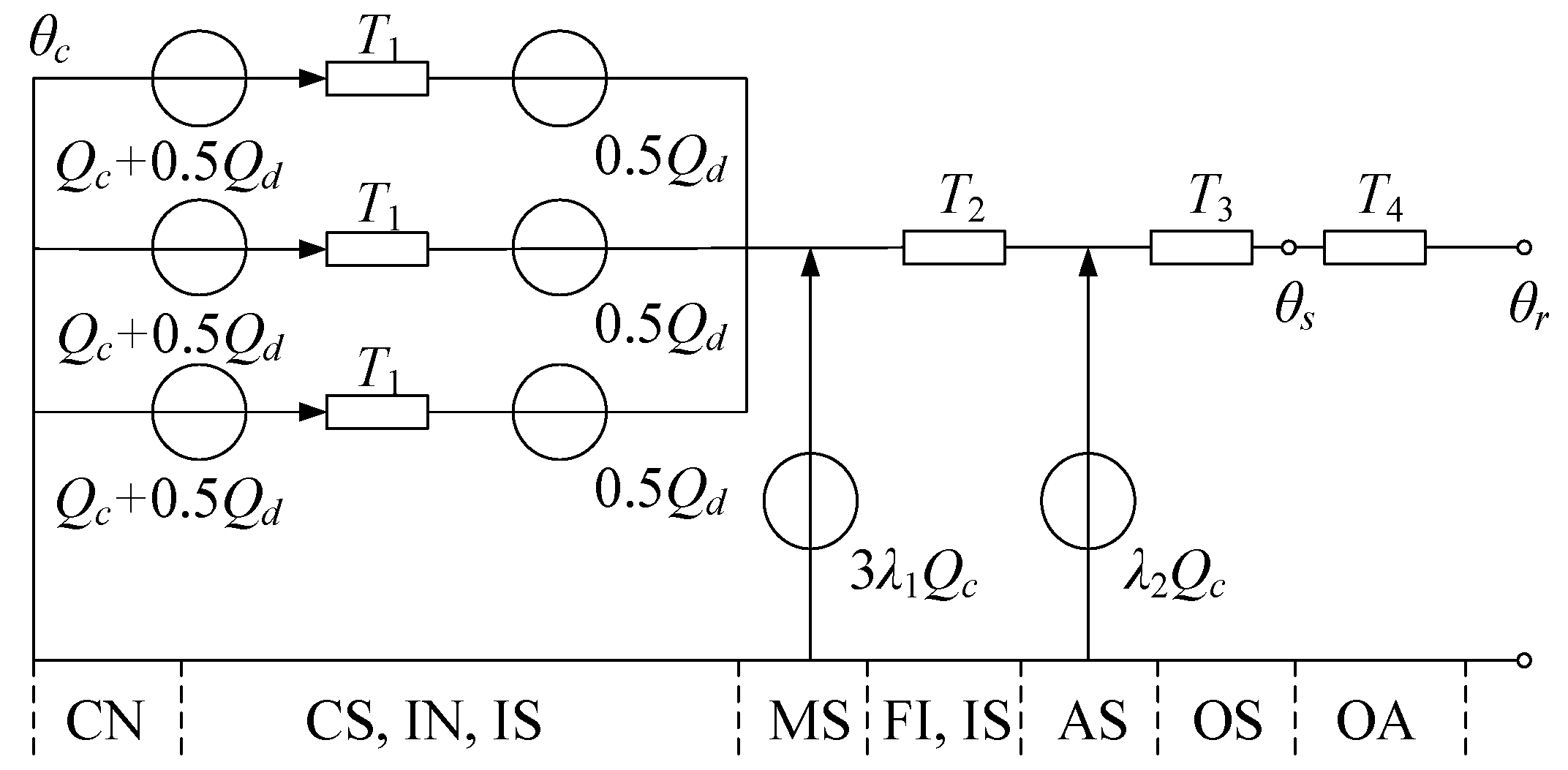
2.2.1. Thermal Circuit Model of Shore Power Cables
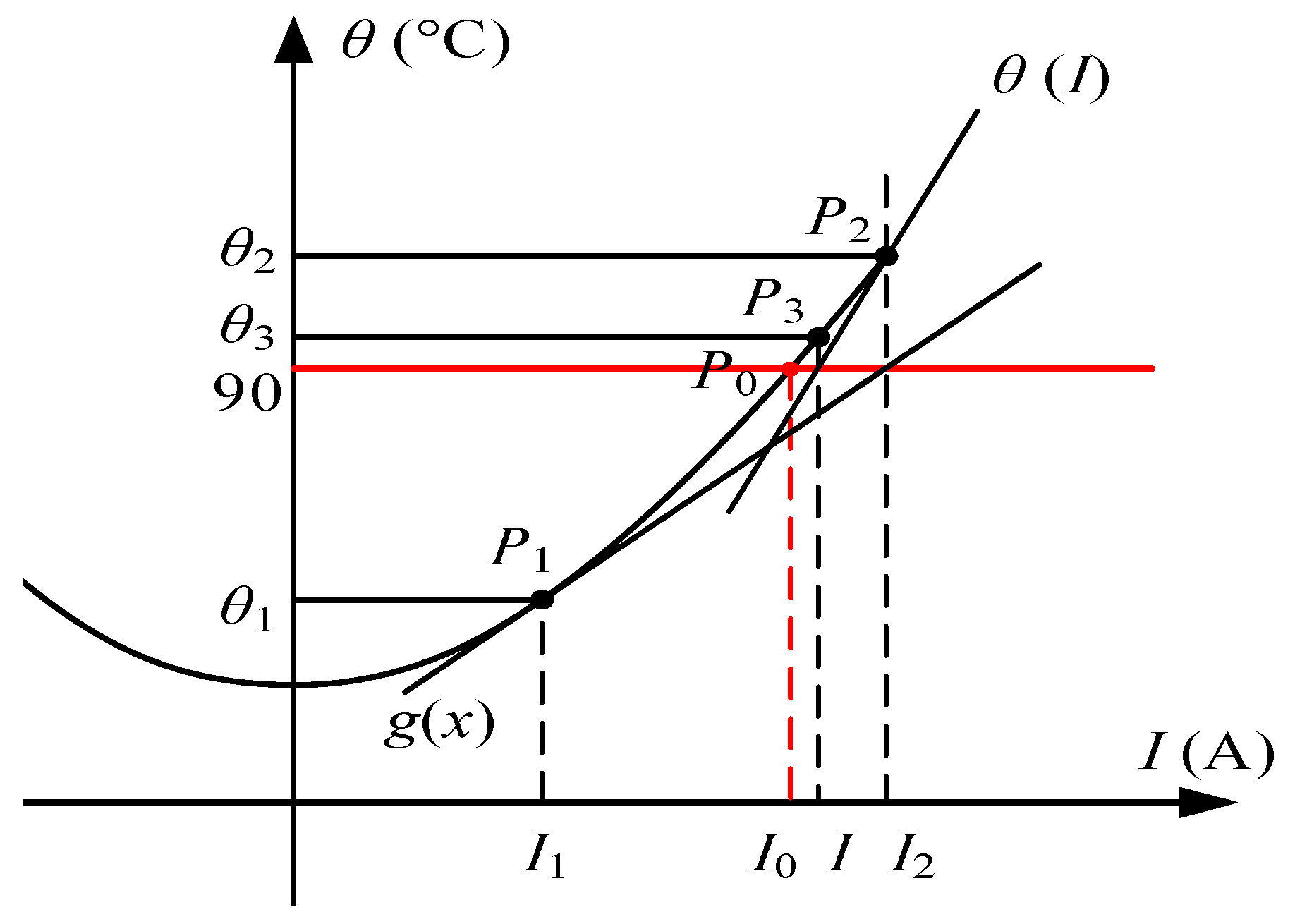
2.2.2. Newton–Raphson Method of Current-Carrying Capacity
2.2.3. Heat Balance Equation (HBE) and Solution for Shore Power Cables
2.3. Methodology of Mathematical Model for Current-Carrying Capacity
3. Results
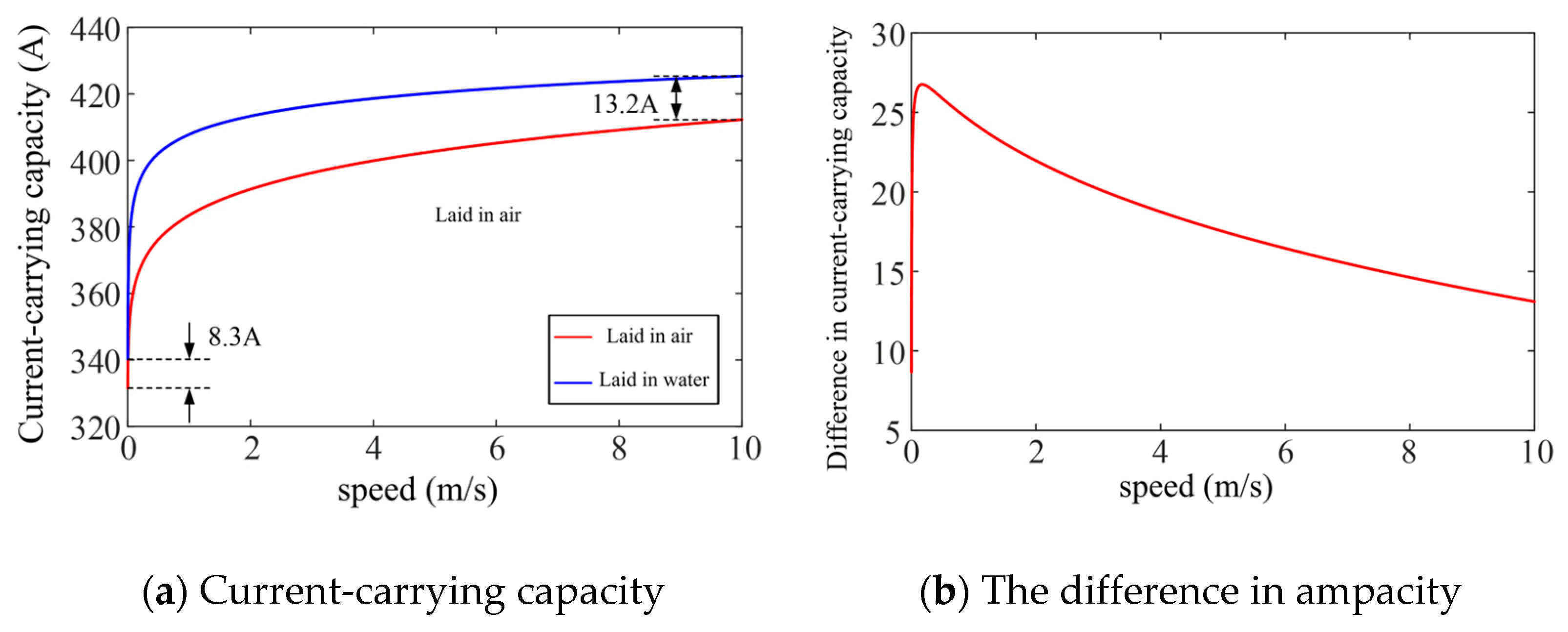
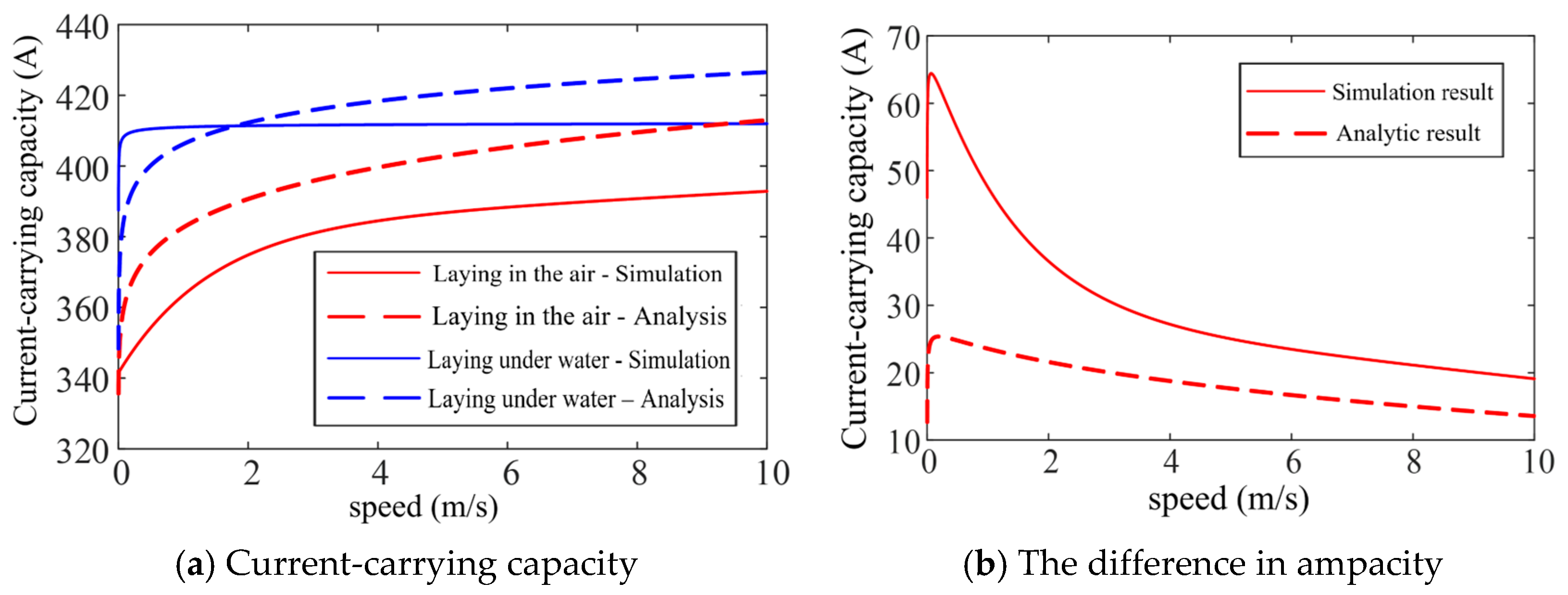
3.1. Influence of Wind and Water Speed
3.2. Influence of Ambient Temperature
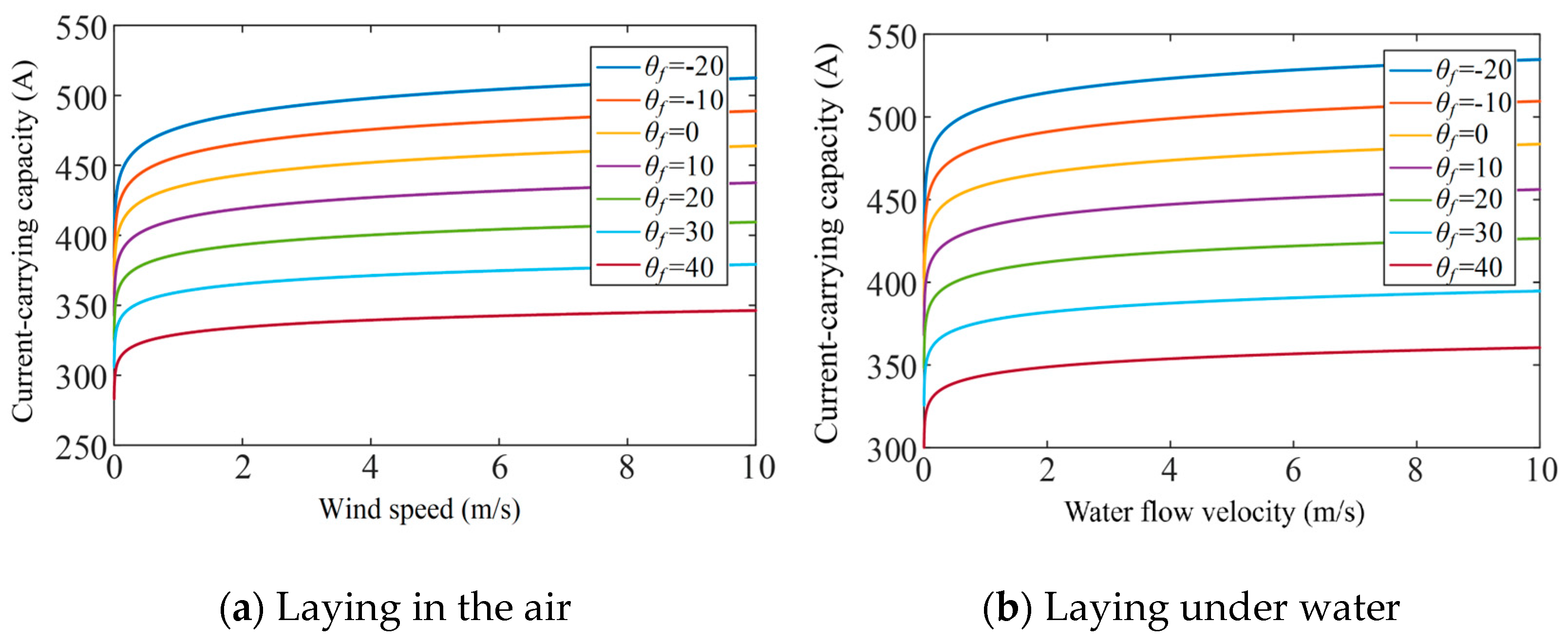
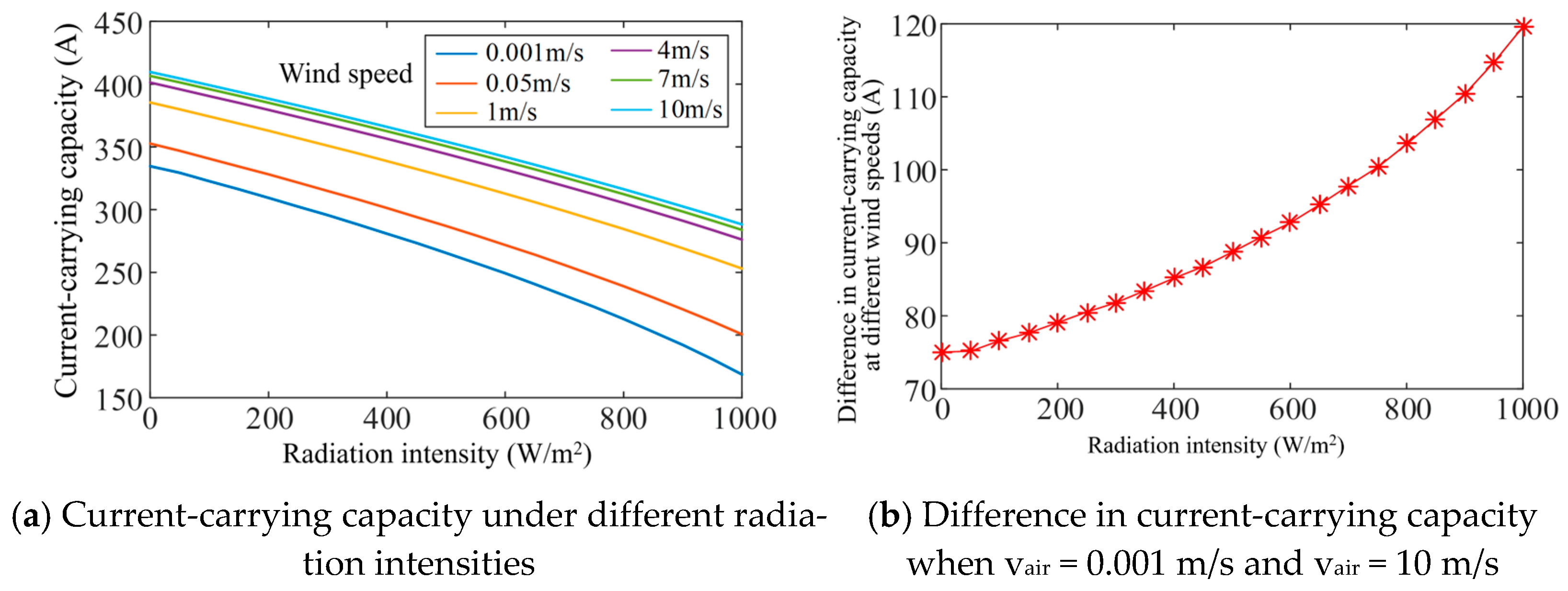
3.3. Effect of Solar Radiations
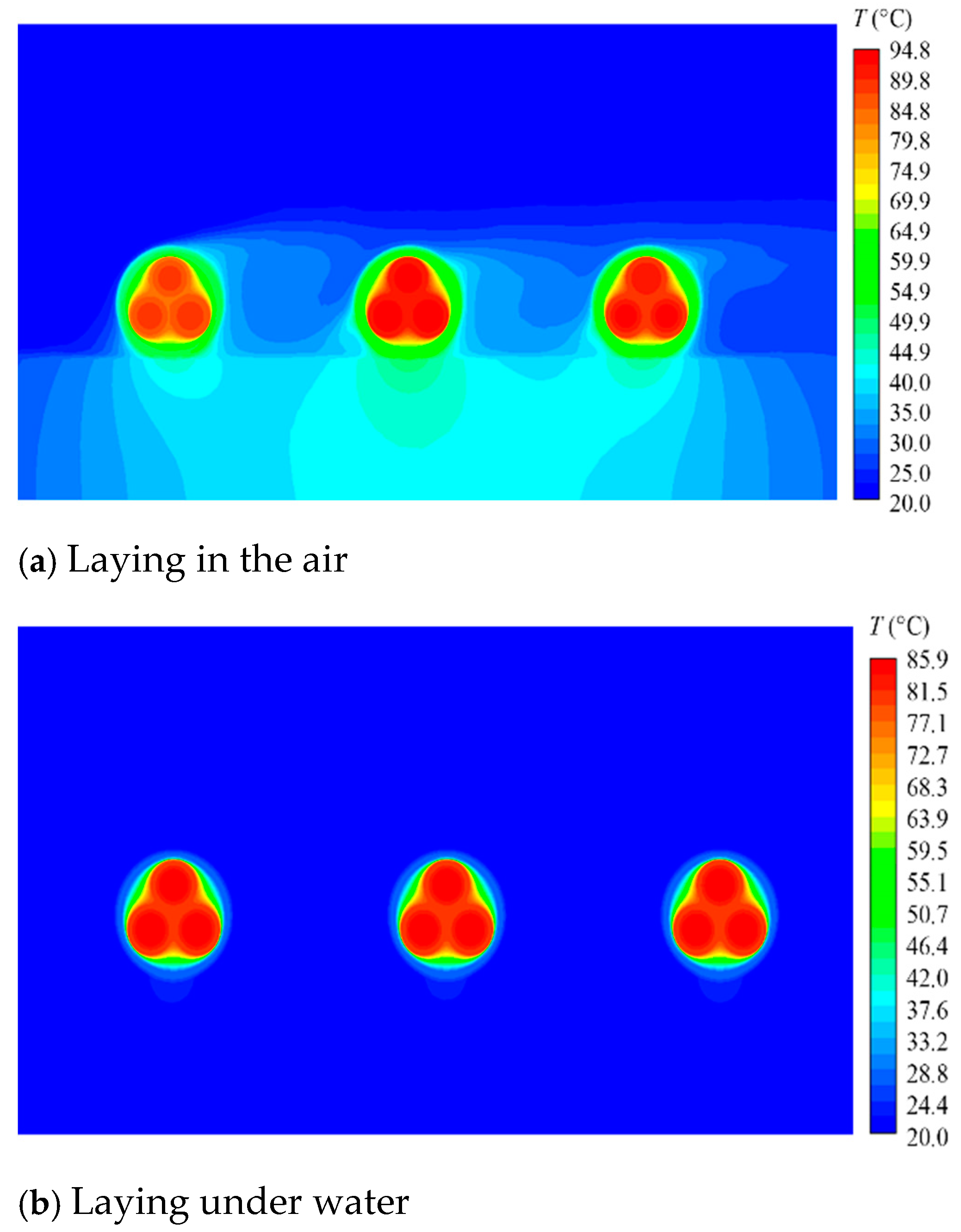
3.3.1. Laying in the Air
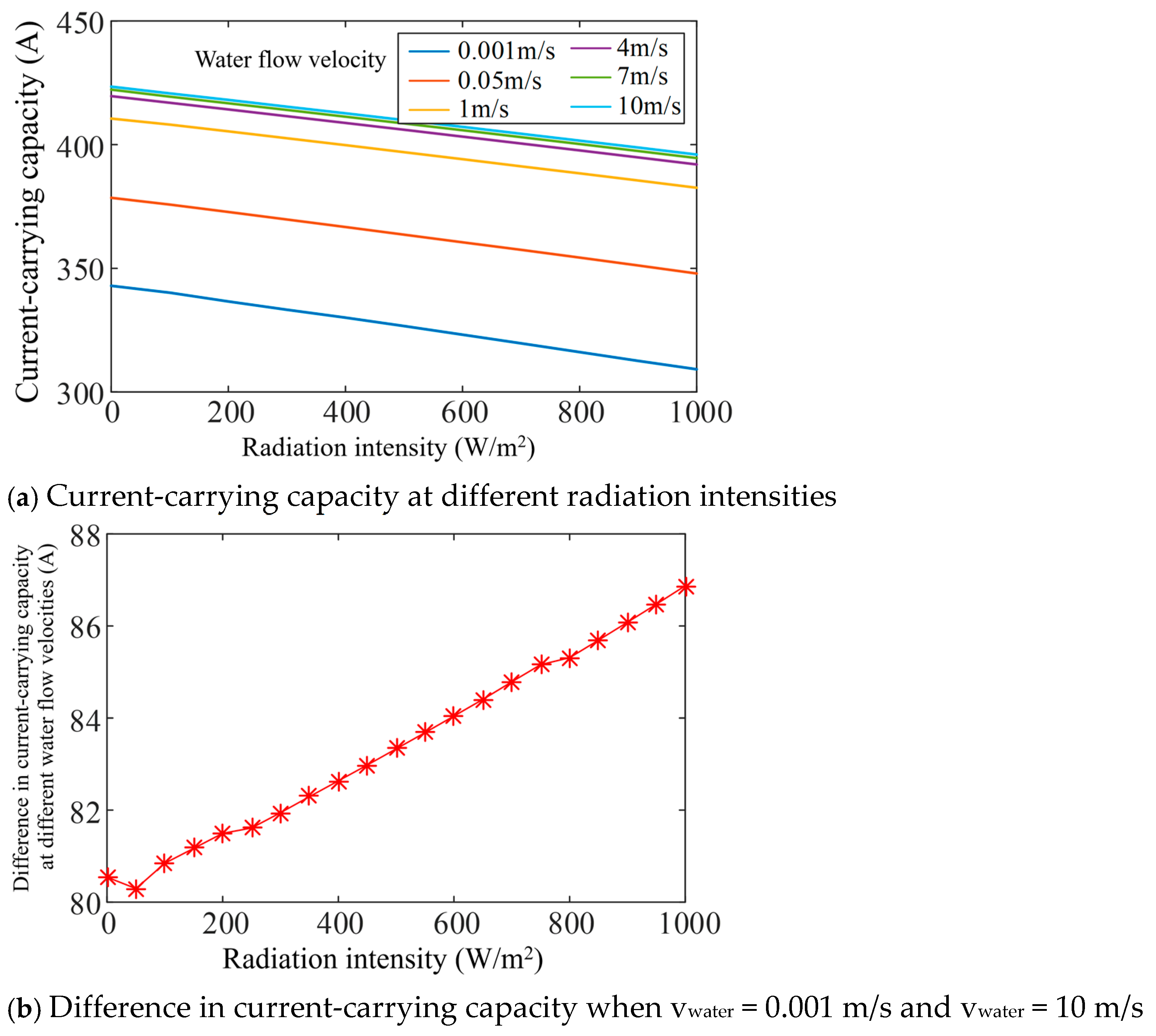
3.3.2. Laying Under Water
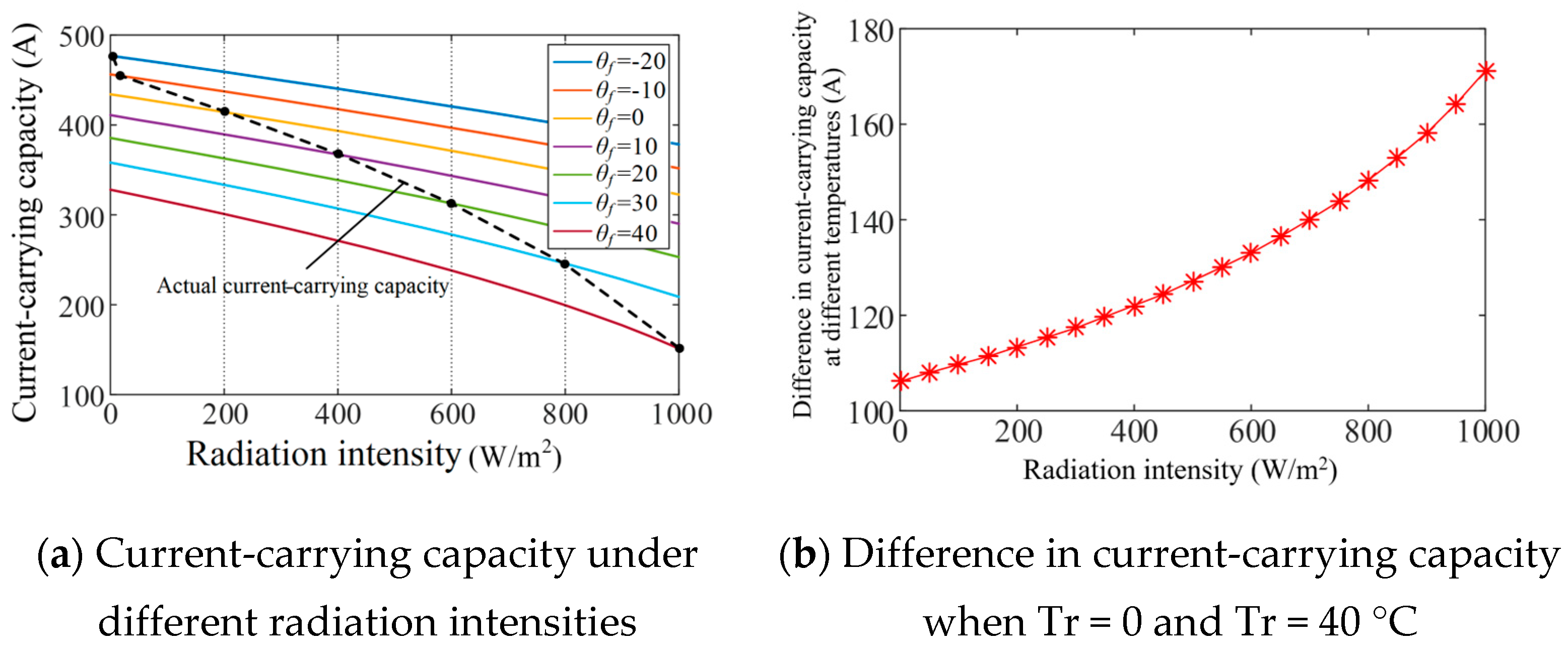
3.4. Influence of Exterior Thermal Resistance T4
3.4.1. Calculation of Exterior Thermal Resistance T4 When Laying in the Air
3.4.2. Calculation of Exterior Thermal Resistance T4 When Laying Under Water
4. Conclusions
5. Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- IEC 60287-1-1:2023; Electric Cables–Calculation of the Current Rating–Part 1-1: Current Rating Equations (100% Load Factor) and Calculation of Losses–General. International Electrotechnical Commission: Geneva, Switzerland, 2023.
- Zeng, T.; Yang, G.; Yuan, M.; Chen, Y.; Fang, F.; Xu, X.; Lv, S.; Liu, G. Theoretical Modeling and Experimental Verification of AC Resistance in Large Cross-Section XLPE Single-Core Cables. In Proceedings of the 2024 The 9th International Conference on Power and Renewable Energy (ICPRE), Guangzhou, China, 20–23 September 2024; IEEE: New York, NY, USA, 2024. [Google Scholar]
- Liu, Z.; Ouyang, B.; Liu, S.; Chu, F.; Li, T. Discussion on the Improvement of Current Carrying Capacity of AC 500 kV Submarine Cable. IOP Conf. Ser. Mater. Sci. Eng. 2019, 563, 052106. [Google Scholar] [CrossRef]
- Zhang, L.; He, Y.; Liu, Y.; Yang, F.; He, T.; Liu, L.; Wang, S.; Liu, H. Temperature analysis based on multi-coupling field and ampacity optimization calculation of shore power cable considering tide effect. IEEE Access 2020, 8, 119785–119794. [Google Scholar] [CrossRef]
- Chen, Y.; Wang, S.; Hao, Y.; Yao, K.; Li, H.; Jia, F.; Yue, D.; Shi, Q.; Cheng, Y.; Huang, X. Temperature Monitoring for 500 kV Oil-Filled Submarine Cable Based on BOTDA Distributed Optical Fiber Sensing Technology: Method and Application. IEEE Trans. Instrum. Meas. 2022, 71, 3504510. [Google Scholar] [CrossRef]
- Yang, L.; Hu, Z.; Hao, Y.; Qiu, W.; Li, L. Internal temperature measurement and conductor temperature calculation of XLPE power cable based on optical fiber at different radial positions. Eng. Fail. Anal. 2021, 125, 105407. [Google Scholar] [CrossRef]
- Cherukupalli, S.; Anders, G.J. Distributed Fiber Optic Sensing and Dynamic Rating of Power Cables; John Wiley & Sons: Hoboken, NJ, USA, 2019. [Google Scholar]
- Zhang, Y.; Cen, Z.; Zhang, P.; Li, Q.; Hao, Y. Simulation Analysis of Burial Depth State of Optical Fiber Composite Submarine Cable Based on Temperature Field Variation. In Proceedings of the 2023 IEEE 4th International Conference on Electrical Materials and Power Equipment (ICEMPE), Shanghai, China, 7–10 May 2023; IEEE: New York, NY, USA, 2023; pp. 1–4. [Google Scholar]
- Xu, Z.; Hu, Z.; Zhao, L.; Zhang, Y.; Yang, Z.; Hu, S.; Li, Y. Application of temperature field modeling in monitoring of optic-electric composite submarine cable with insulation degradation. Measurement 2019, 133, 479–494. [Google Scholar] [CrossRef]
- Chen, X.; Yu, J.; Yu, L.; Zhou, H. Numerical analysis of thermo-electric field for AC XLPE cable in DC operation based on conduction current measurement. IEEE Access 2018, 7, 8226–8234. [Google Scholar] [CrossRef]
- Henke, A.; Frei, S. Fast analytical approaches for the transient axial temperature distribution in single wire cables. IEEE Trans. Ind. Electron. 2021, 69, 4158–4166. [Google Scholar] [CrossRef]
- Xiong, L.; Chen, Y.; Jiao, Y.; Wang, J.; Hu, X. Study on the effect of cable group laying mode on temperature field distribution and cable ampacity. Energies 2019, 12, 3397. [Google Scholar] [CrossRef]
- Hadi, M.K. Utilization of the finite element method for the calculation and examination of underground power cable ampacity. Int. J. Appl. Power Eng. 2019, 8, 257–264. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, X.; Zhang, H.; Liu, J.; Zhang, C.; Jiao, J. Analysis on the temperature field and the ampacity of XLPE submarine HV cable based on electro-thermal-flow multiphysics coupling simulation. Polymers 2020, 12, 952. [Google Scholar] [CrossRef] [PubMed]
- Elsaid, A.M.; Zahran, M.S.; Abdel Moneim, S.A.; Lasheen, A.; Mohamed, I.G. A recent review on ventilation and cooling of underground high-voltage cable tunnels. J. Therm. Anal. Calorim. 2024, 149, 8927–8978. [Google Scholar] [CrossRef]
- Che, C.; Yan, B.; Fu, C.; Li, G.; Qin, C.; Liu, L. Improvement of cable current carrying capacity using COMSOL software. Energy Rep. 2022, 8, 931–942. [Google Scholar] [CrossRef]
- Wang, W.; Bai, X.; Si, W.; Zhao, Y.; Yao, W.; Liu, G.; Liu, H.; Yan, L.; Yang, D.; Meng, X.; et al. Review of Calculation Methods of Power Cables Temperature based on Thermal Circuit Model. In Proceedings of the 2024 IEEE 7th Advanced Information Technology, Electronic and Automation Control Conference (IAEAC), Chongqing, China, 15–17 March 2024; IEEE: New York, NY, USA, 2024; Volume 7, pp. 1711–1714. [Google Scholar]
- Ma, A.; Zheng, M.; Zhang, S.; Qin, T. Thermal circuit model of DC submarine cable considering the influence of seawater property variations. Electr. Power Syst. Res. 2024, 230, 110268. [Google Scholar] [CrossRef]
- Wang, X.W.; Zhao, J.P.; Zhang, Q.G.; Lv, B.; Chen, L.C.; Zhang, Y.; Yang, J.H. Real-time calculation of transient ampacity of trench laying cables based on the thermal circuit model and the temperature measurement. In Proceedings of the 2019 IEEE 8th International Conference on Advanced Power System Automation and Protection (APAP), Xi’an, China, 21–24 October 2019; IEEE: New York, NY, USA, 2019. [Google Scholar]
- Wang, P.Y.; Ma, H.; Liu, G.; Han, Z.Z.; Guo, D.M.; Xu, T.; Kang, L.Y. Dynamic thermal analysis of high-voltage power cable insulation for cable dynamic thermal rating. IEEE Access 2019, 7, 56095–56106. [Google Scholar] [CrossRef]
- Tang, J.; Fang, X.; Luo, X.; Qin, T.; Yang, T.; Ma, C.; Zhang, Y. Research on the Method of Improving the Current-carrying Capacity of Power Cable. J. Phys. Conf. Ser. 2022, 2247, 012042. [Google Scholar] [CrossRef]
- Sun, D.; Dong, Q. Cable Steady-State Ampacity Correction Method based on Multi Physical Field Coupling Algorithm. Electroteh. Electron. Autom. 2022, 70, 19–27. [Google Scholar]
- Zhang, H.; Ding, R.; Lin, P.; Zeng, Y.; Lyu, D.; Liu, G. Application of Thermal Resistance Dynamic Characteristics on High-Voltage Cable Ampacity Based on Field Circuit Coupling Method. In Proceedings of the 2022 12th International Conference on Power and Energy Systems (ICPES), Guangzhou, China, 23–25 December 2022; IEEE: New York, NY, USA, 2022; pp. 116–122. [Google Scholar]
- Ge, J.; Wang, M.; Yang, Y.; Jing, Q.; Zhang, L.; He, J.; Zhou, Y.; Chang, Z.; Deng, J. Multi-Physics Simulation Study on Current Carrying Characteristics of High Voltage AC Submarine Cable Under Multiple Working Conditions. In Proceedings of the 2024 IEEE PES 16th Asia-Pacific Power and Energy Engineering Conference (APPEEC), Nanjing, China, 25–27 October 2024; pp. 1–5. [Google Scholar]
- You, F.; Yusoh, M.A.T.M. A novel model to identify the performance of power cable on the offshore network by considering dynamic environmental impact. J. Phys. Conf. Ser. 2022, 2260, 012046. [Google Scholar] [CrossRef]
- Tang, C.; Zhong, L.; Zeng, T.; Jiang, B.; Liu, D.; Cheng, R.; Yuan, C. Simulation and Analysis of Submarine Cable Based on Electro-Thermal-Flow Multi-physics Coupling. In Proceedings of the 2023 IEEE 4th International Conference on Electrical Materials and Power Equipment (ICEMPE), Shanghai, China, 7–10 May 2023; IEEE: New York, NY, USA, 2023; pp. 1–4. [Google Scholar]
- You, F.; Yusoh, M.A.T.M.; Ali, N.H.B.N.; Yang, H. Analysis on Influencing Factors of Temperature Distribution of Shore Power Cable. IEEE Access 2024, 12, 137988–137999. [Google Scholar] [CrossRef]
- Bergman, T.L. Fundamentals of Heat and Mass Transfer; John Wiley & Sons: Hoboken, NJ, USA, 2020. [Google Scholar]
- Myklemyr, A. Analysis of Natural Convection and High Voltage AC Cable Rating in Naturally Ventilated Tunnels. Master’s Thesis, Norwegian University of Life Sciences, Ås, Norway, 2023. [Google Scholar]
- Dehbani, M.; Rahimi, M.; Rahimi, Z. A review on convective heat transfer enhancement using ultrasound. Appl. Therm. Eng. 2022, 208, 118273. [Google Scholar] [CrossRef]






| Parameter | Relative Dielectric Constant | Conductivity (S/m) | Specific Heat Capacity J/(kg·K) | Heat Conductivity W/(m·K) | Yang’s Modulus (GPa) | Poisson’s Ratio |
|---|---|---|---|---|---|---|
| Conductor | Tend to ∞ | 58 × 106 | 385 | 400 | 120 | 0.34 |
| Insulation layer | 3 | Tends to 0 | 2300 | 0.2875 | 1.1 | 0.42 |
| Conductor shied | 100 | 6 | 1005 | 0.2875 | 1.7 | 0.41 |
| Filler | 1.6 | Tends to 0 | 1883 | 0.0169 | 1.1 | 0.44 |
| Inner sheath | 3.6 | Tends to 0 | 2100 | 0.2 | 0.64 | 0.41 |
| Aluminum sheath | Tends to ∞ | 38 × 106 | 871 | 273 | 70 | 0.33 |
| Outer sheath | 3.5 | Tends to 0 | 2070 | 0.2 | 0.2 | 0.42 |
| Radiation Intensity H (W/m2) | Wind Speed vair (m/s) | Current-Carrying Capacity Difference (A) | |
|---|---|---|---|
| 0.001 m/s | 10 m/s | ||
| 0 | 334.6 | 409.7 | 75.1 |
| 200 | 309.4 | 388.5 | 79.1 |
| 400 | 280.8 | 366.0 | 85.2 |
| 600 | 249.2 | 342.0 | 92.8 |
| 800 | 212.7 | 316.3 | 103.6 |
| 1000 | 168.6 | 288.2 | 119.6 |
| Radiation Intensity H (W/m2) | Ambient Temperature Tr ( °C) | Current-Carrying Capacity Difference (A) | |
|---|---|---|---|
| 0 °C | 40 °C | ||
| 0 | 434.2 | 328.0 | 106.2 |
| 200 | 414.3 | 301.0 | 113.3 |
| 400 | 393.4 | 271.3 | 122.1 |
| 600 | 371.3 | 238.2 | 133.1 |
| 800 | 347.9 | 199.6 | 148.3 |
| 1000 | 322.6 | 151.5 | 171.1 |
| Radiation Intensity H (W/m2) | Water Flow Velocity vwater (m/s) | Current-Carrying Capacity Difference (A) | |
|---|---|---|---|
| 0.001 m/s | 10 m/s | ||
| 0 | 342.9 | 423.5 | 80.6 |
| 200 | 336.6 | 418.1 | 81.5 |
| 400 | 330.1 | 412.7 | 82.6 |
| 600 | 323.2 | 407.2 | 84 |
| 800 | 316.2 | 401.6 | 85.4 |
| 1000 | 309.2 | 396.0 | 86.8 |
| Radiation Intensity H (W/m2) | Ambient Temperature Tr (°C) | Current-Carrying Capacity Difference (A) | |
|---|---|---|---|
| 0 °C | 40 °C | ||
| 0 | 465.0 | 348.0 | 117.0 |
| 200 | 460.2 | 341.3 | 118.9 |
| 400 | 455.3 | 334.7 | 120.6 |
| 600 | 450.4 | 327.9 | 122.5 |
| 800 | 445.4 | 321.0 | 124.4 |
| 1000 | 440.3 | 313.9 | 126.4 |
| Ambient Temperature Tr (°C) | Wind Speed Vair (m/s) | |||||
|---|---|---|---|---|---|---|
| 0.001 | 0.05 | 1 | 4 | 7 | 10 | |
| −20 | 0.813 | 0.580 | 0.268 | 0.158 | 0.125 | 0.107 |
| −10 | 0.761 | 0.552 | 0.261 | 0.155 | 0.123 | 0.106 |
| 0 | 0.712 | 0.524 | 0.254 | 0.153 | 0.122 | 0.105 |
| 10 | 0.666 | 0.497 | 0.247 | 0.150 | 0.120 | 0.103 |
| 20 | 0.623 | 0.471 | 0.240 | 0.147 | 0.118 | 0.102 |
| 30 | 0.582 | 0.446 | 0.233 | 0.144 | 0.116 | 0.101 |
| 40 | 0.544 | 0.423 | 0.225 | 0.142 | 0.114 | 0.099 |
| Ambient Temperature Tr (°C) | Water Flow Velocity Vwater (m/s) | |||||
|---|---|---|---|---|---|---|
| 0.001 | 0.05 | 1 | 4 | 7 | 10 | |
| −20 | 0.702 | 0.321 | 0.101 | 0.054 | 0.041 | 0.035 |
| −10 | 0.662 | 0.312 | 0.099 | 0.053 | 0.041 | 0.035 |
| 0 | 0.623 | 0.302 | 0.098 | 0.053 | 0.041 | 0.035 |
| 10 | 0.587 | 0.292 | 0.097 | 0.053 | 0.041 | 0.035 |
| 20 | 0.552 | 0.283 | 0.096 | 0.052 | 0.041 | 0.035 |
| 30 | 0.519 | 0.273 | 0.095 | 0.052 | 0.040 | 0.034 |
| 40 | 0.488 | 0.263 | 0.094 | 0.052 | 0.040 | 0.034 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
You, F.; Yusoh, M.A.T.M.; Nik Ali, N.H.; Yang, H. A Mathematical Method of Current-Carrying Capacity for Shore Power Cables in Port Microgrids. Electronics 2025, 14, 1749. https://doi.org/10.3390/electronics14091749
You F, Yusoh MATM, Nik Ali NH, Yang H. A Mathematical Method of Current-Carrying Capacity for Shore Power Cables in Port Microgrids. Electronics. 2025; 14(9):1749. https://doi.org/10.3390/electronics14091749
Chicago/Turabian StyleYou, Fei, Mohd Abdul Talib Mat Yusoh, Nik Hakimi Nik Ali, and Hao Yang. 2025. "A Mathematical Method of Current-Carrying Capacity for Shore Power Cables in Port Microgrids" Electronics 14, no. 9: 1749. https://doi.org/10.3390/electronics14091749
APA StyleYou, F., Yusoh, M. A. T. M., Nik Ali, N. H., & Yang, H. (2025). A Mathematical Method of Current-Carrying Capacity for Shore Power Cables in Port Microgrids. Electronics, 14(9), 1749. https://doi.org/10.3390/electronics14091749







