Compact Modeling and Exploration of the Light Metal Insertion Effect for a Voltage-Controlled Spin–Orbit Torque Magnetic Tunnel Junction
Abstract
1. Introduction
2. Compact Model for the VCSOT-MTJ Device
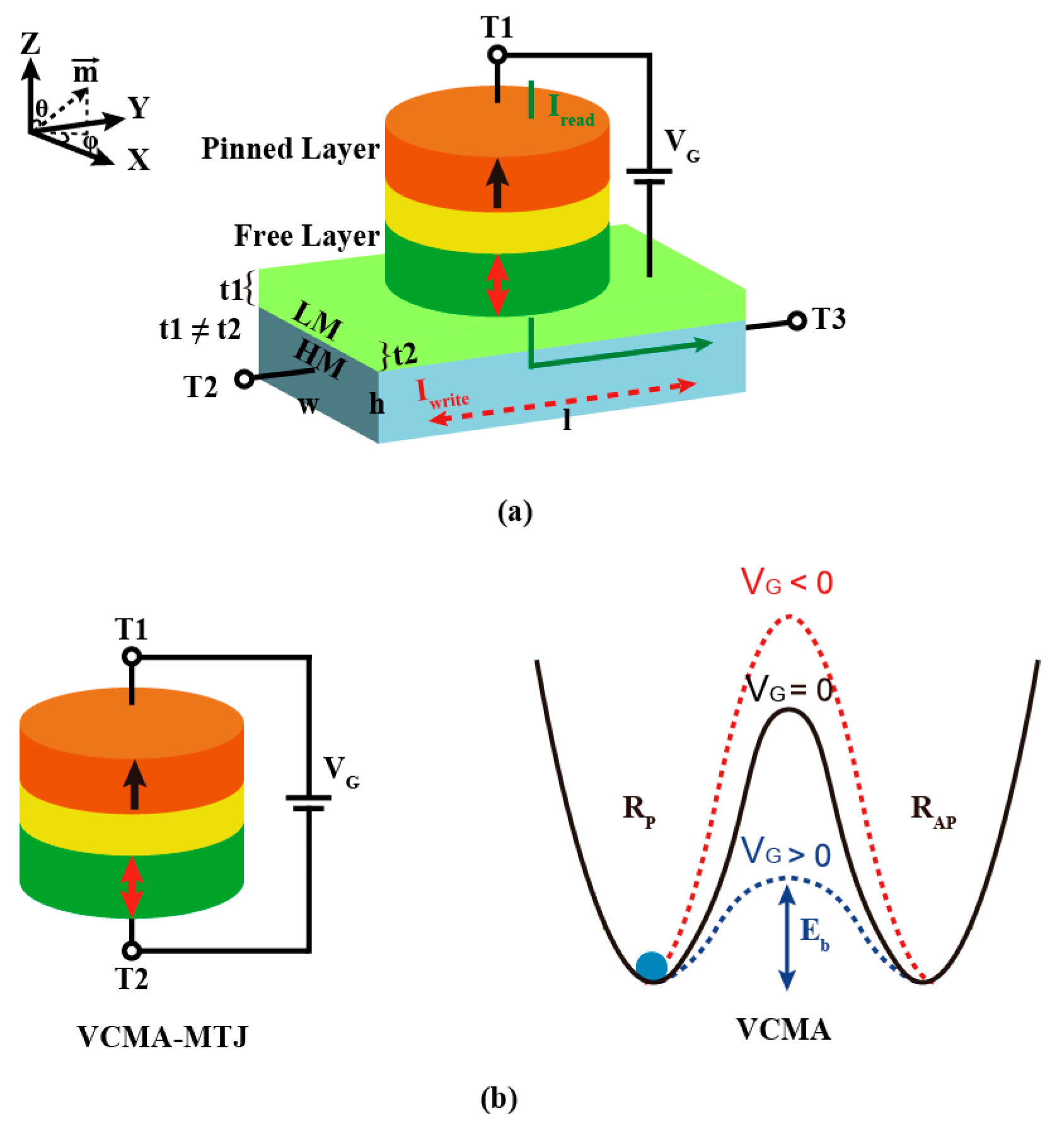
2.1. Structure of the VCSOT-MTJ
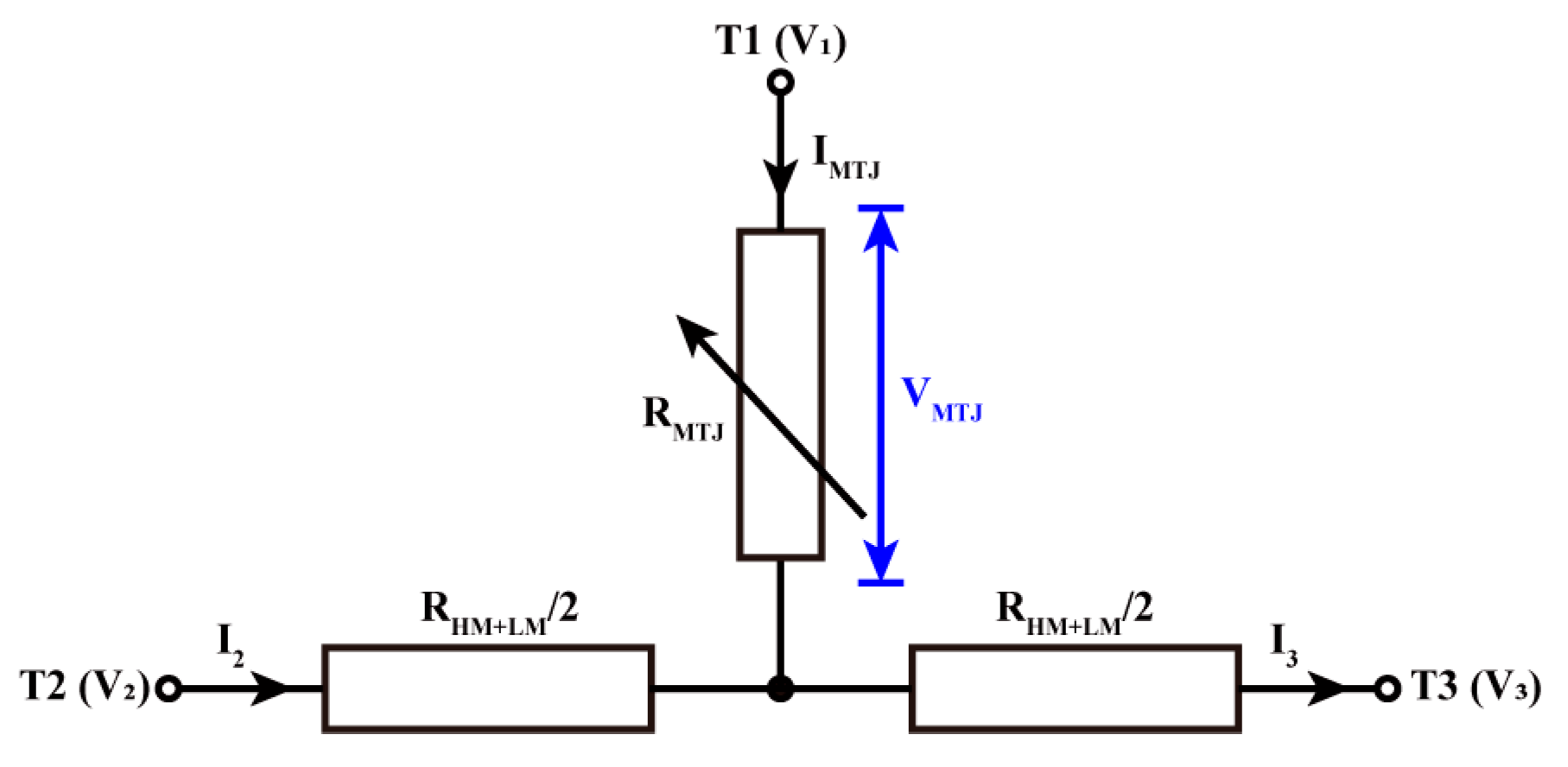
2.2. Electrical Model of the VCSOT-MTJ
2.3. Model of Tunneling Magnetoresistance in VCSOT-MTJ
2.4. Magnetization Dynamics Model of the VCSOT-MTJ
2.5. Effective Magnetic Field
3. Electrical Compact Model and Simulations
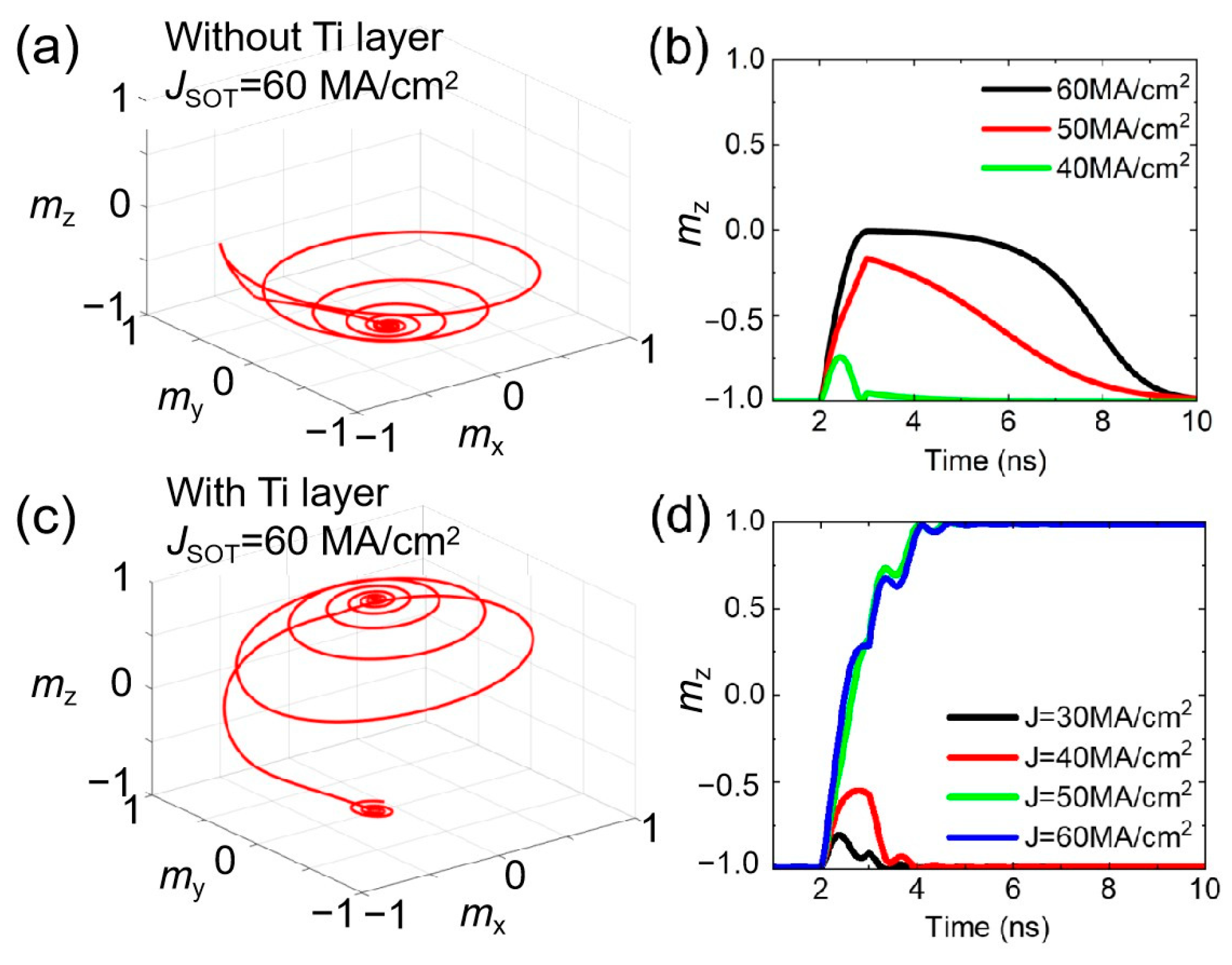
3.1. Field-Free SOT Switching Without VCMA Effect
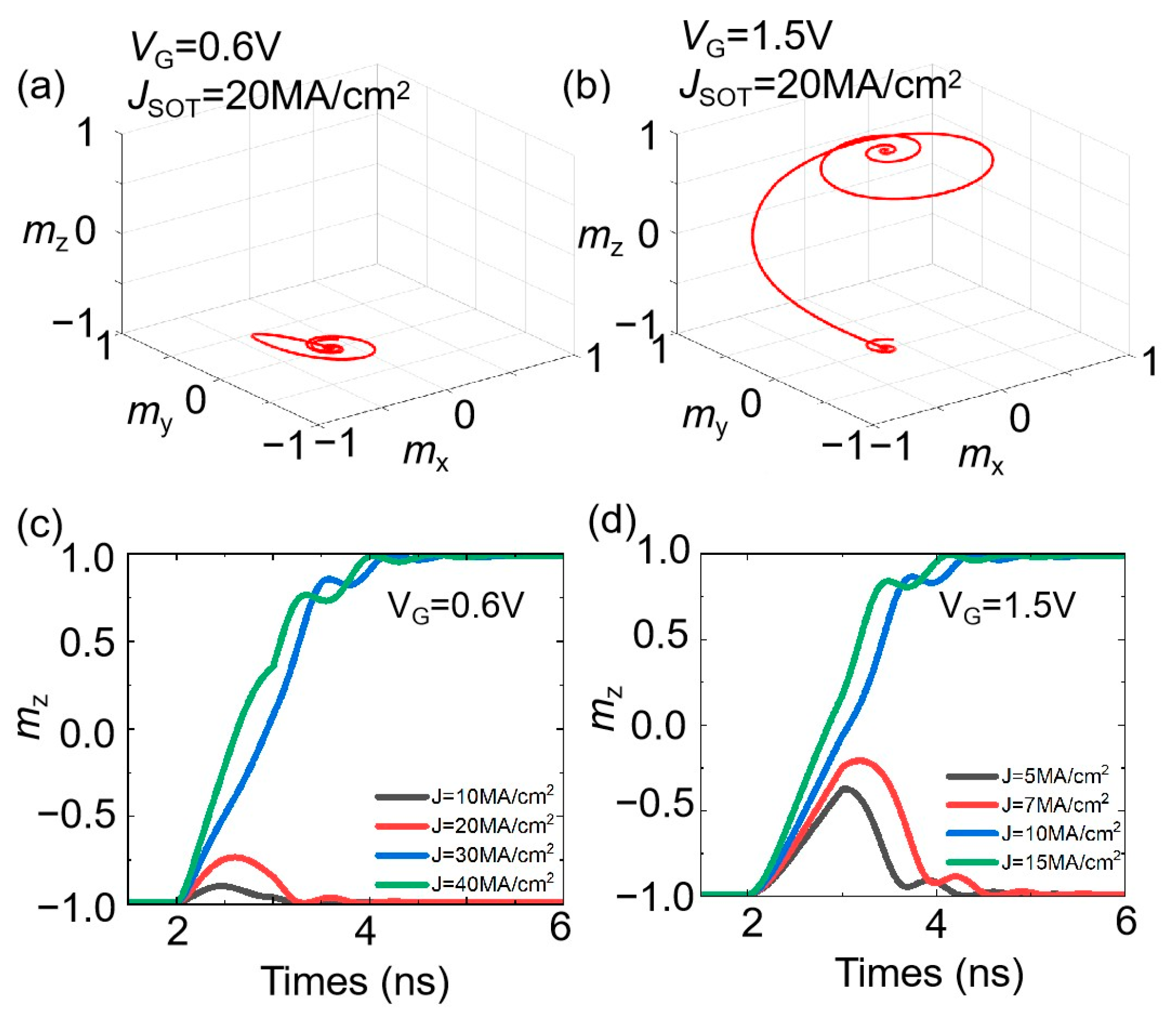
3.2. DC Simulation of the VCSOT-MTJ
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Swami, S.; Mohanram, K. Reliable nonvolatile memories: Techniques and measures. IEEE Des. Test 2017, 34, 31–41. [Google Scholar] [CrossRef]
- Xia, Y.; Zhang, K.; Zeng, L.; Zhang, Y.; Zhao, W.; Zhang, D. A Novel Non-Voltatile Magnetic Majority Gate with Voltage-Gated Spin-Orbit Torque Magnetic Tunnel Junction Cascaded in Series for Low Power and High Reliability. IEEE-NANO 2023, 2023, 661–665. [Google Scholar] [CrossRef]
- Kang, W.; Ran, Y.; Zhang, Y.; Lv, W.; Zhao, W. Modeling and exploration of the voltage-controlled magnetic anisotropy effect for the next-generation low-power and high-speed MRAM applications. IEEE Trans. Nanotechnol. 2017, 16, 387–395. [Google Scholar] [CrossRef]
- Halawani, Y. Impact of Emerging Memory Technologies on the Energy Efficiency of Wireless Sensor Nodes. Ph.D. Thesis, Khalifa University, Abu Dhabi, United Arab Emirates, 2014. [Google Scholar]
- Apalkov, D.; Khvalkovskiy, A.; Watts, S.; Nikitin, V.; Tang, X.; Lottis, D.; Moon, K.; Luo, X.; Chen, E.; Ong, A.; et al. Spin-transfer torque magnetic random access memory (STT-MRAM). JETC 2013, 9, 1–35. [Google Scholar] [CrossRef]
- Halawani, Y.; Mohammad, B.; Al-Qutayri, M.; Saleh, H. Modeling of STT-MTJ for low power embedded memory applications: A comparative review. In Proceedings of the 2013 IEEE 20th International Conference on Electronics, Circuits, and Systems (ICECS), Abu Dhabi, United Arab Emirates, 8–11 December 2013; pp. 719–722. [Google Scholar] [CrossRef]
- Kang, W.; Lv, W.; Zhang, Y.; Zhao, W. Low store power high-speed high-density nonvolatile SRAM design with spin Hall effect-driven magnetic tunnel junctions. IEEE Trans. Nanotechnol. 2016, 16, 148–154. [Google Scholar] [CrossRef]
- Wang, Z.; Zhao, W.; Deng, E.; Klein, J.O.; Chappert, C. Perpendicular-anisotropy magnetic tunnel junction switched by spin-Hall-assisted spin-transfer torque. J. Phys. D Appl. Phys. 2015, 48, 065001. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, D.; Zhang, K.; Zeng, L.; Wang, Y.; Hou, Z.; Zhang, Y.; Zhao, W. Magnetic nonvolatile SRAM based on voltage-gated spin-orbit-torque magnetic tunnel junctions. IEEE Trans. Electron. Devices 2020, 67, 1965–1971. [Google Scholar] [CrossRef]
- Salehi, S.; Mashhadi, M.B.; Zaeemzadeh, A.; Rahnavard, N.; DeMara, R.F. Energy-aware adaptive rate and resolution sampling of spectrally sparse signals leveraging VCMA-MTJ devices. IEEE J. Emerg. Sel. Top. Circuits Syst. 2018, 8, 679–692. [Google Scholar] [CrossRef]
- Zhang, K.; Zhang, D.; Wang, C.; Zeng, L.; Wang, Y.; Zhao, W. Compact modeling and analysis of voltage-gated spin-orbit torque magnetic tunnel junction. IEEE Access 2020, 8, 50792–50800. [Google Scholar] [CrossRef]
- Li, Y.; Edmonds, K.W.; Liu, X.; Zheng, H.; Wang, K. Manipulation of magnetization by spin–orbit torque. Adv. Quantum Technol. 2019, 2, 1800052. [Google Scholar] [CrossRef]
- Razavi, A.; Wu, H.; Shao, Q.; Fang, C.; Dai, B.; Wong, K.; Hang, X.; Yu, G.; Wang, K.L. Deterministic spin–orbit torque switching by a light-metal insertion. Nano Lett. 2020, 20, 3703–3709. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Liu, Z.; Peng, S.; Lu, J.; Liu, J.; Li, X.; Lu, S.; Otani, Y.; Zhao, W. Selective Data Writing in IrMn-Based Perpendicular Magnetic Tunnel Junction Array Through Voltage-Gated Spin-Orbit Torque. IEEE Electron Device Lett. 2024, 45, 921–924. [Google Scholar] [CrossRef]
- Wan, Q.; Shi, Y. Neuromorphic Devices for Brain-inspired Computing: Artificial Intelligence, Perception, and Robotics; John Wiley & Sons: Hoboken, NJ, USA, 2022. [Google Scholar]
- Brinkman, W.F.; Dynes, R.C.; Rowell, J.M. Tunneling conductance of asymmetrical barriers. J. Appl. Phys. 1970, 41, 1915–1921. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhao, W.; Lakys, Y.; Klein, J.O.; Kim, J.V.; Ravalosona, D.; Chappert, C. Compact modeling of perpendicular-anisotropy CoFeB/MgO magnetic tunnel junctions. IEEE Trans. Nanotechnol. 2012, 59, 819–826. [Google Scholar] [CrossRef]
- Pathak, S.; Youm, C.; Hong, J. Impact of spin-orbit torque on spin-transfer torque switching in magnetic tunnel junctions. Sci. Rep. 2020, 10, 2799. [Google Scholar] [CrossRef] [PubMed]
- Li, S.; Jiang, Y. Field-free switching model of spin–orbit torque (SOT)-MTJ device with thermal effect based on voltage-controlled magnetic anisotropy (VCMA). AIP Adv. 2023, 13, 025030. [Google Scholar] [CrossRef]
- Oh, Y.W.; Chris Baek, S.; Kim, Y.M.; Lee, K.D.; Yang, G.G.; Park, E.S.; Lee, K.S.; Kim, K.W.; Go, G. Field-Free Switching of perpendicular magnetization through spin-orbit torque in antiferromagnet/ferromagnet/oxide structures. Nat. Nanotechnol. 2016, 11, 878–884. [Google Scholar] [CrossRef] [PubMed]
- Fina, I.; Quintana, A.; Marti, X.; Sanchez, F.; Foerster, M.; Aballe, L.; Sort, J.; Fontcuberta, J. Reversible and magnetically unassisted voltage-driven switching of magnetization in FeRh/PMN-PT. Appl. Phys. Lett. 2018, 113, 152901. [Google Scholar] [CrossRef]
- Zhang, C.; Takeuchi, Y.; Fukami, S.; Ohno, H. Field-free and sub-ns magnetization switching of magnetic tunnel junctions by combining spin-transfer torque and spin orbit torque. Appl. Phys. Lett. 2021, 118, 092406. [Google Scholar] [CrossRef]
| Parameter | Description | Default Value |
|---|---|---|
| D | Diameter of MTJ | 50 nm |
| AMTJ | Surface area of MTJ | π × D2/4 |
| tf | Free layer height | 1.1 nm |
| tox | Oxide layer thickness | 1.4 nm |
| Ms | Saturation magnetization | 6.25 × 105 A/m |
| Ki(0) | Interfacial PMA at 0 V | 3.2 × 10−4 J/m2 |
| α | Gilbert damping factor | 0.05 |
| P | Spin polarization | 0.58 |
| R·A | Resistance–area product | 650 Ω·µm2 |
| TMR(0) | TMR at 0 V | 100% |
| β | VCMA coefficient | 110 fJ/V·m |
| LIrMn | IrMn length | 60 nm |
| TIrMn | IrMn thickness | 3 nm |
| Resistivity | 5.48 × 10−8 Ω·m | |
| Resistivity | 42 × 10−8 Ω·m | |
| Spin Hall angle | 0.25 | |
| Ratio between and | 0.8 | |
| γ | Gyromagnetic ratio | 2.2127 × 105 m/(A·s) |
| Vacuum permeability | 1.2566 × 10−6 H/m |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, W.; Lu, J.; Wang, C.; Wang, D. Compact Modeling and Exploration of the Light Metal Insertion Effect for a Voltage-Controlled Spin–Orbit Torque Magnetic Tunnel Junction. Electronics 2025, 14, 1272. https://doi.org/10.3390/electronics14071272
Li W, Lu J, Wang C, Wang D. Compact Modeling and Exploration of the Light Metal Insertion Effect for a Voltage-Controlled Spin–Orbit Torque Magnetic Tunnel Junction. Electronics. 2025; 14(7):1272. https://doi.org/10.3390/electronics14071272
Chicago/Turabian StyleLi, Weixiang, Jiaqi Lu, Chengzhi Wang, and Dongsheng Wang. 2025. "Compact Modeling and Exploration of the Light Metal Insertion Effect for a Voltage-Controlled Spin–Orbit Torque Magnetic Tunnel Junction" Electronics 14, no. 7: 1272. https://doi.org/10.3390/electronics14071272
APA StyleLi, W., Lu, J., Wang, C., & Wang, D. (2025). Compact Modeling and Exploration of the Light Metal Insertion Effect for a Voltage-Controlled Spin–Orbit Torque Magnetic Tunnel Junction. Electronics, 14(7), 1272. https://doi.org/10.3390/electronics14071272






