Research on Predictive Control Strategy for Phase Shift Full Bridge Transform Based on Improved Nonlinear Disturbance Observer
Abstract
1. Introduction
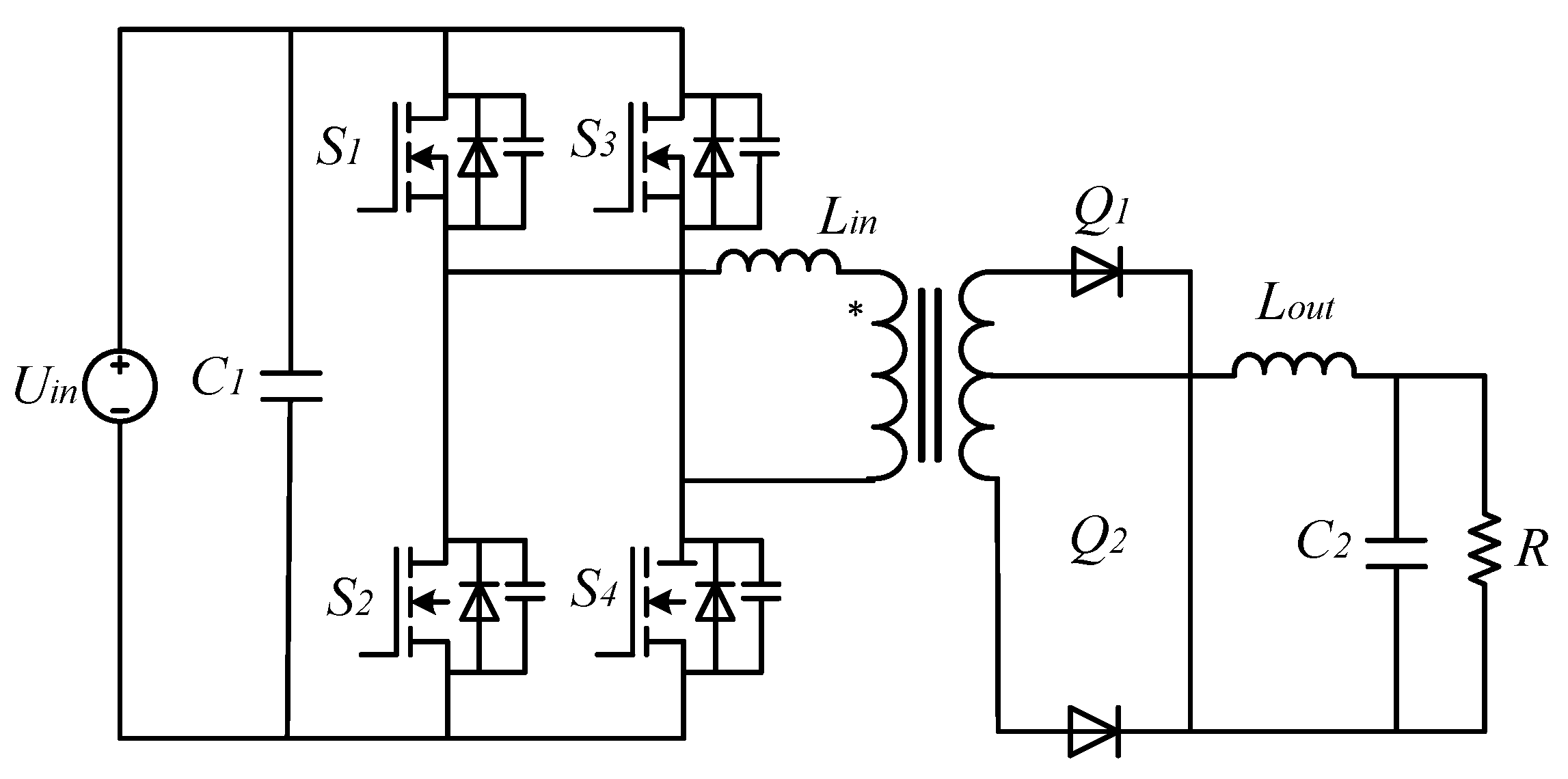
2. PSFB Converter Modeling
2.1. PSFB Working Principle
2.2. Equivalent Mathematical Model
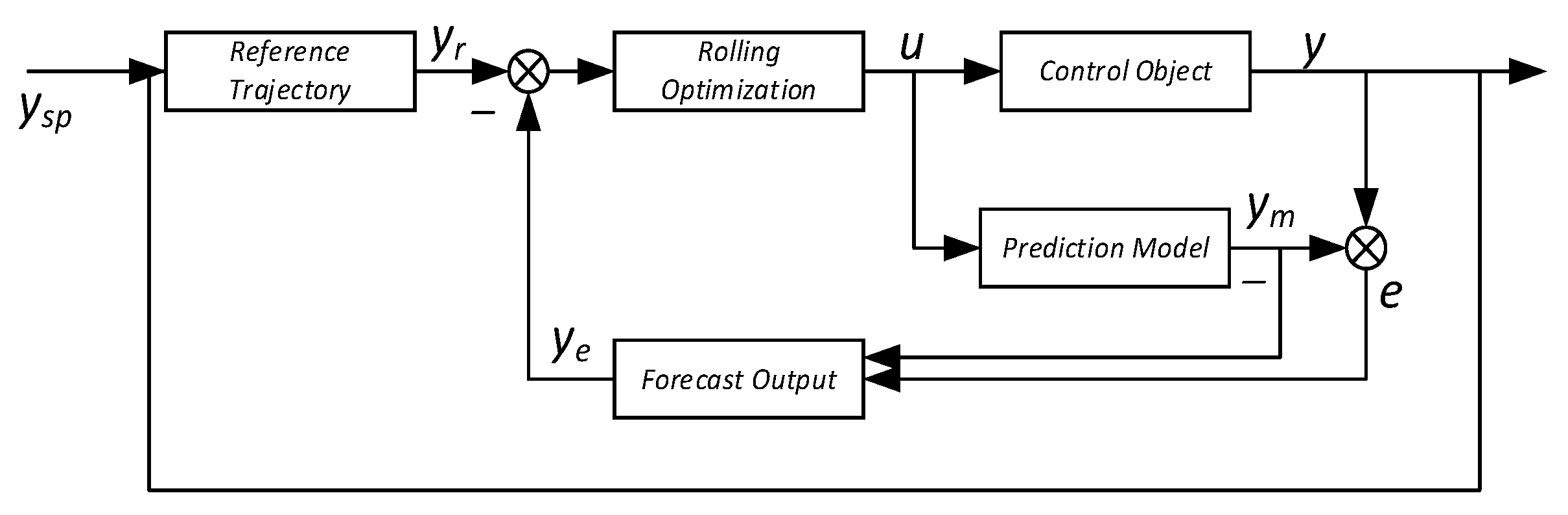
2.3. Control Framework Design
3. PSFB Controller Design
3.1. Model Predictive Control Strategy
3.2. Disturbance Observer Design
3.3. Duty Cycle Compensation and Phase Shift Control
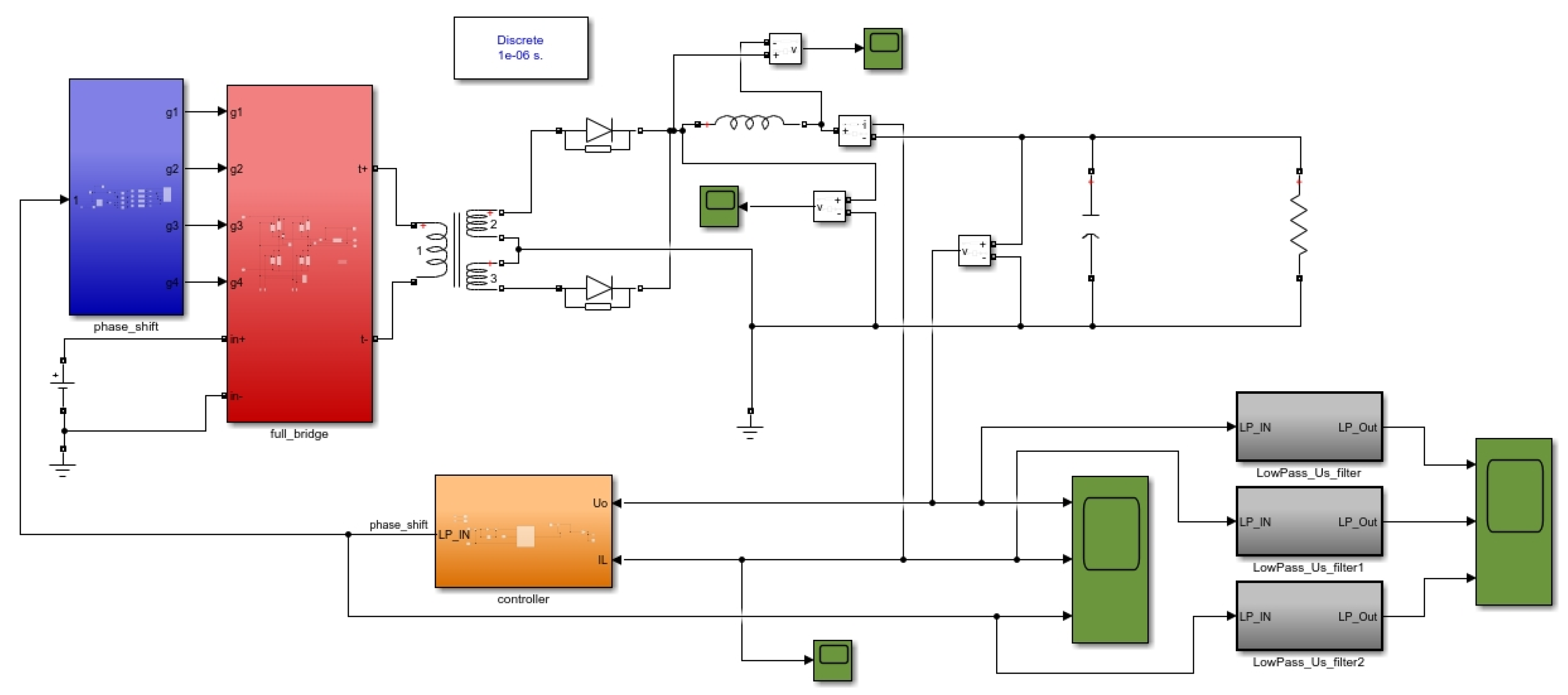
4. Simulation Verification
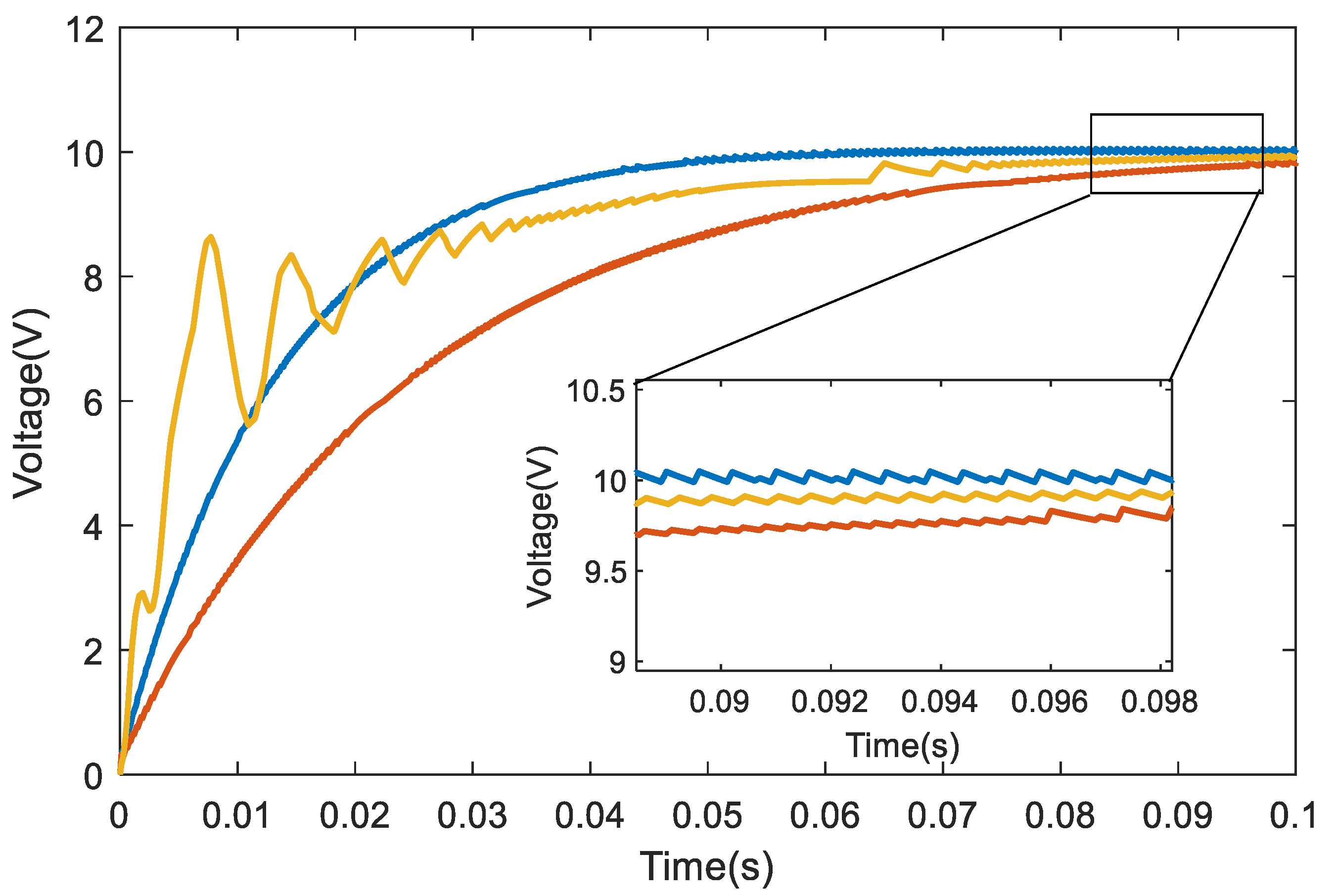
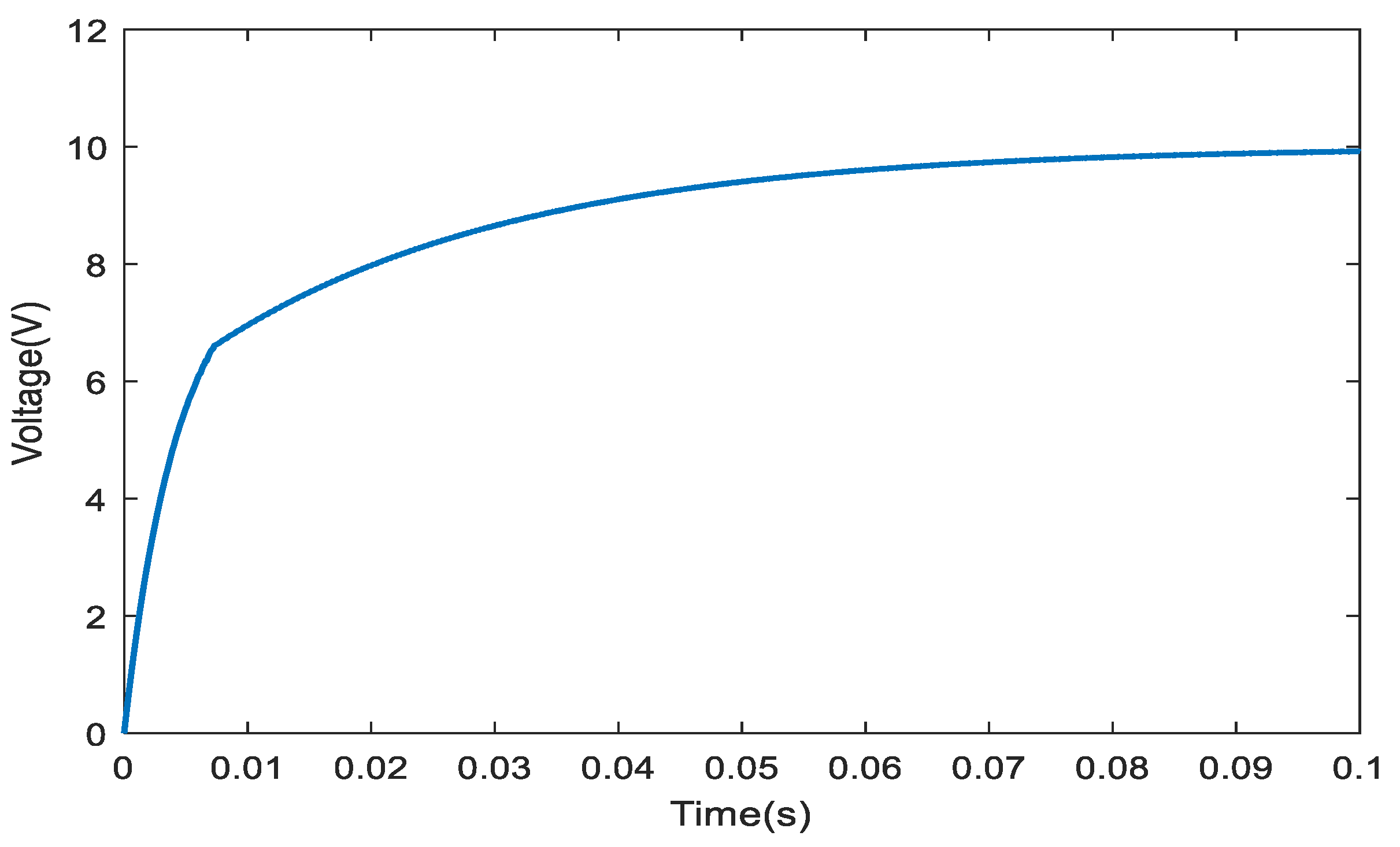
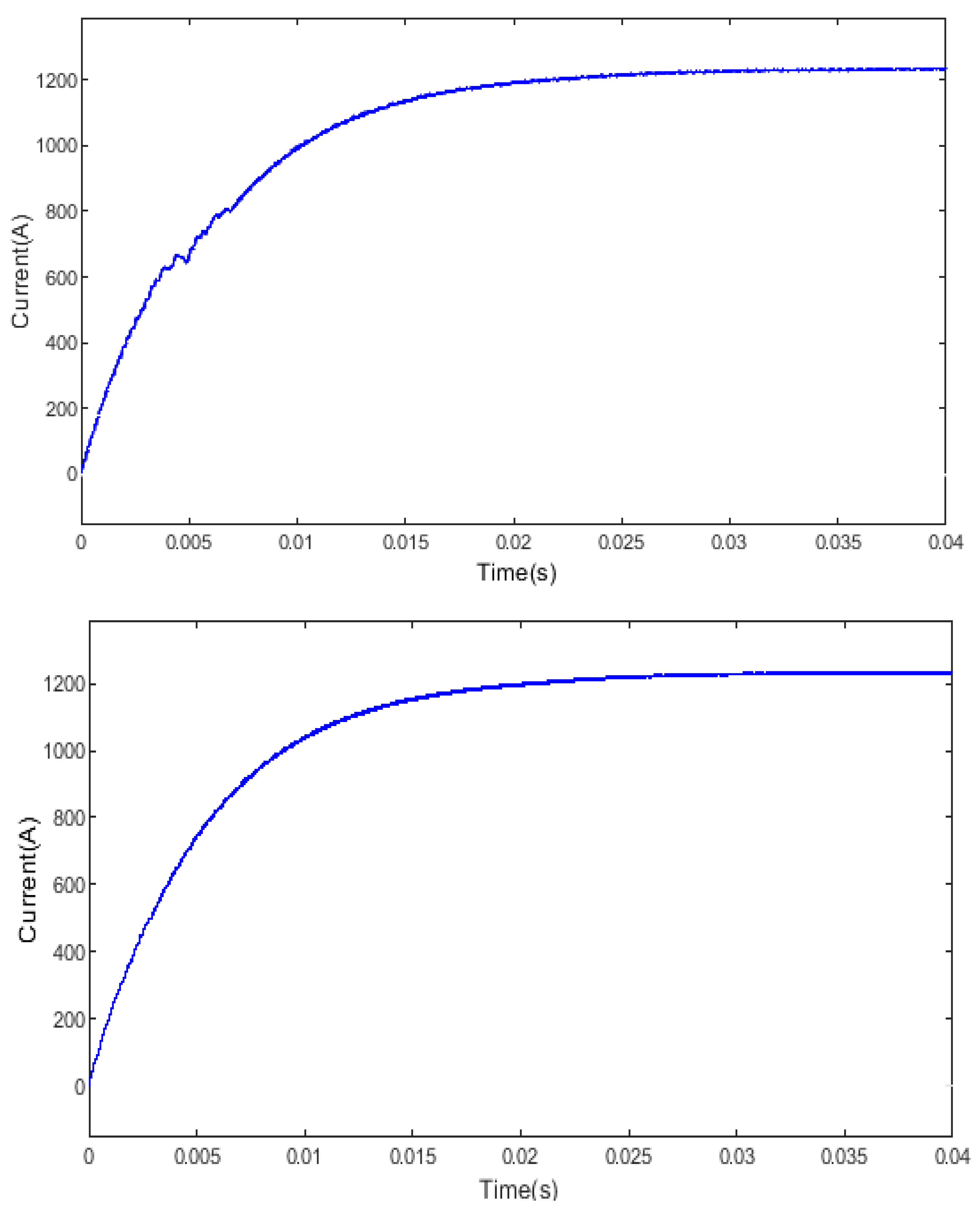
4.1. Comparison Experiment of Traditional PI Control
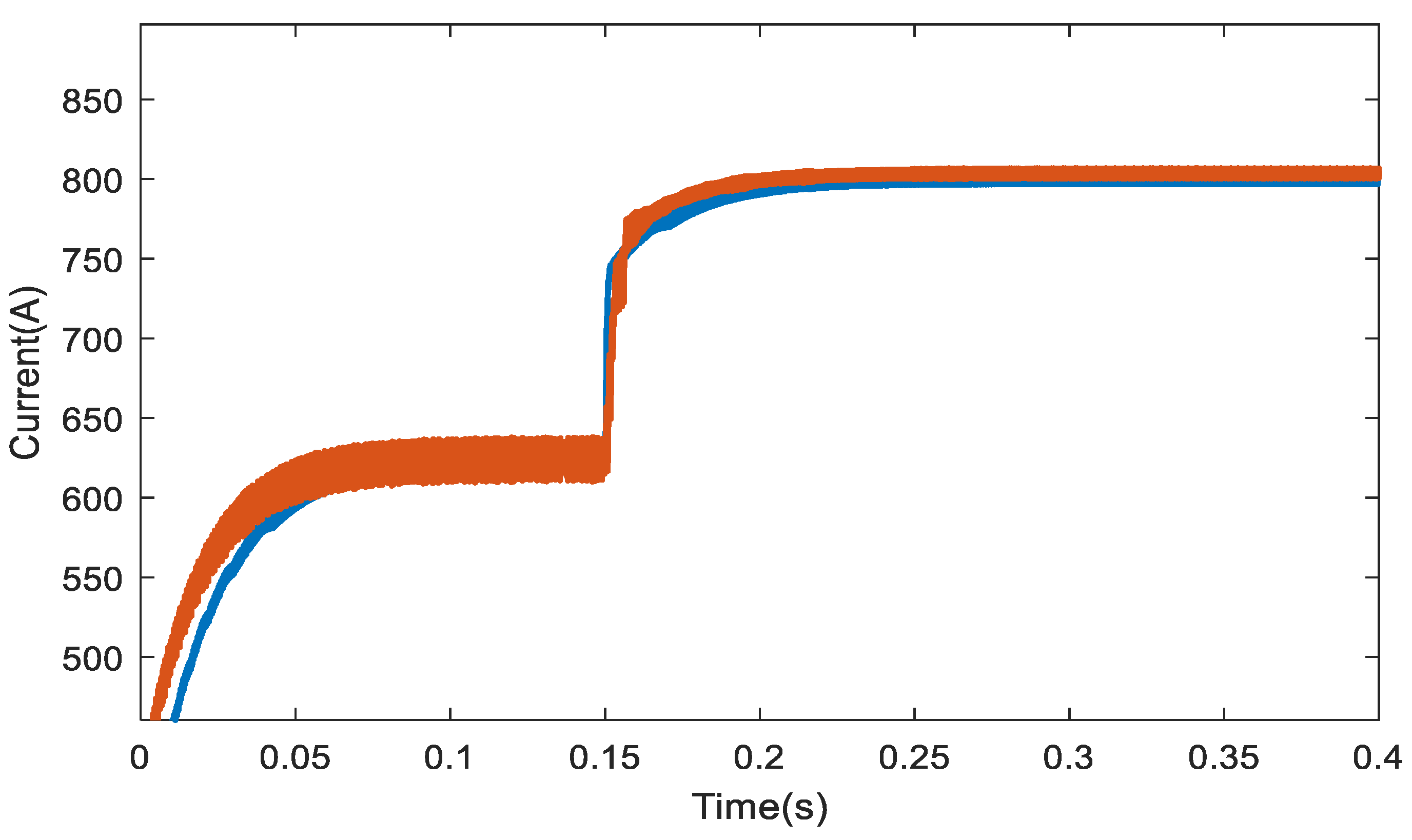
4.2. Load Mutation Experiment
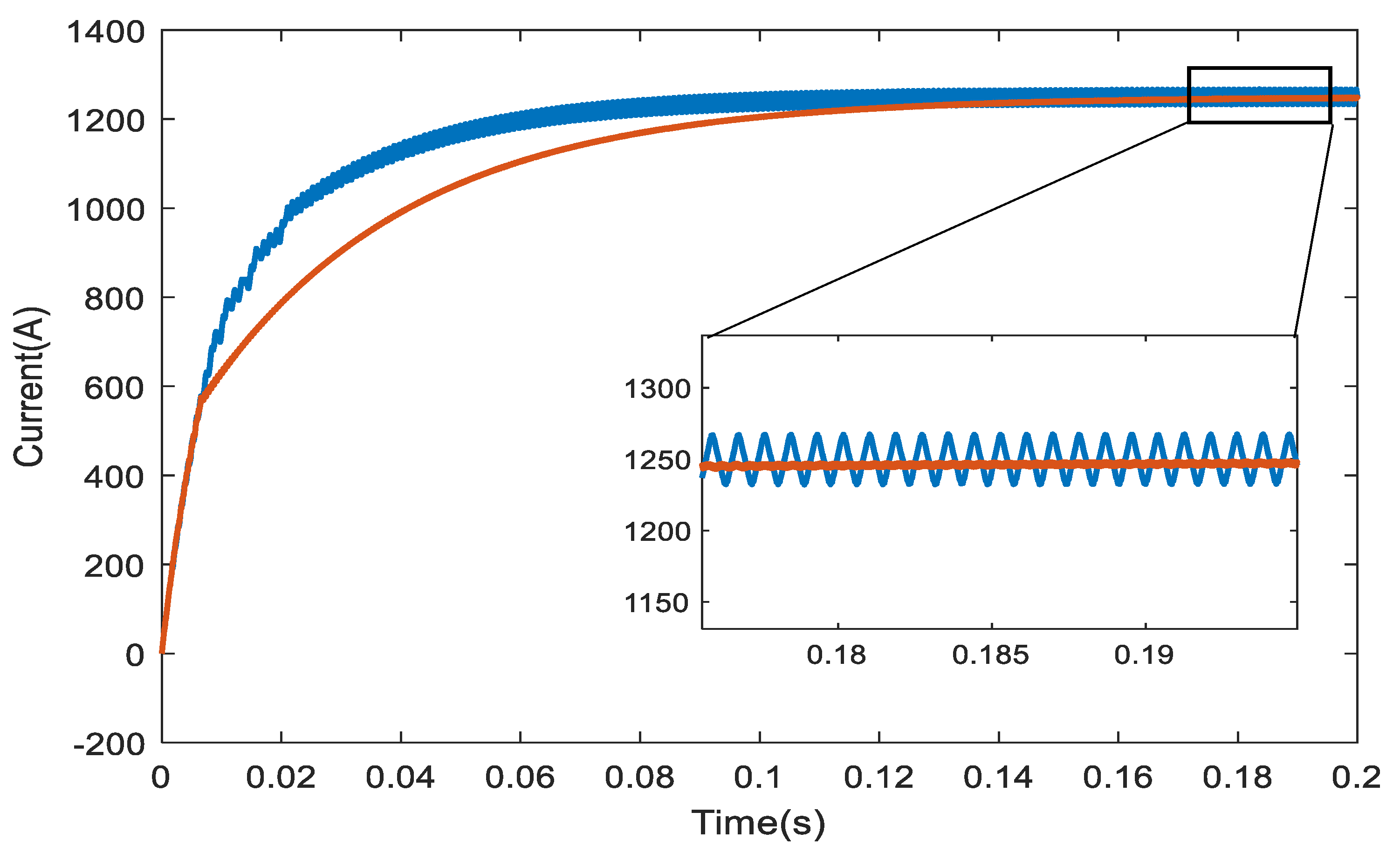
4.3. Inductance Parameter Mismatch Experiment
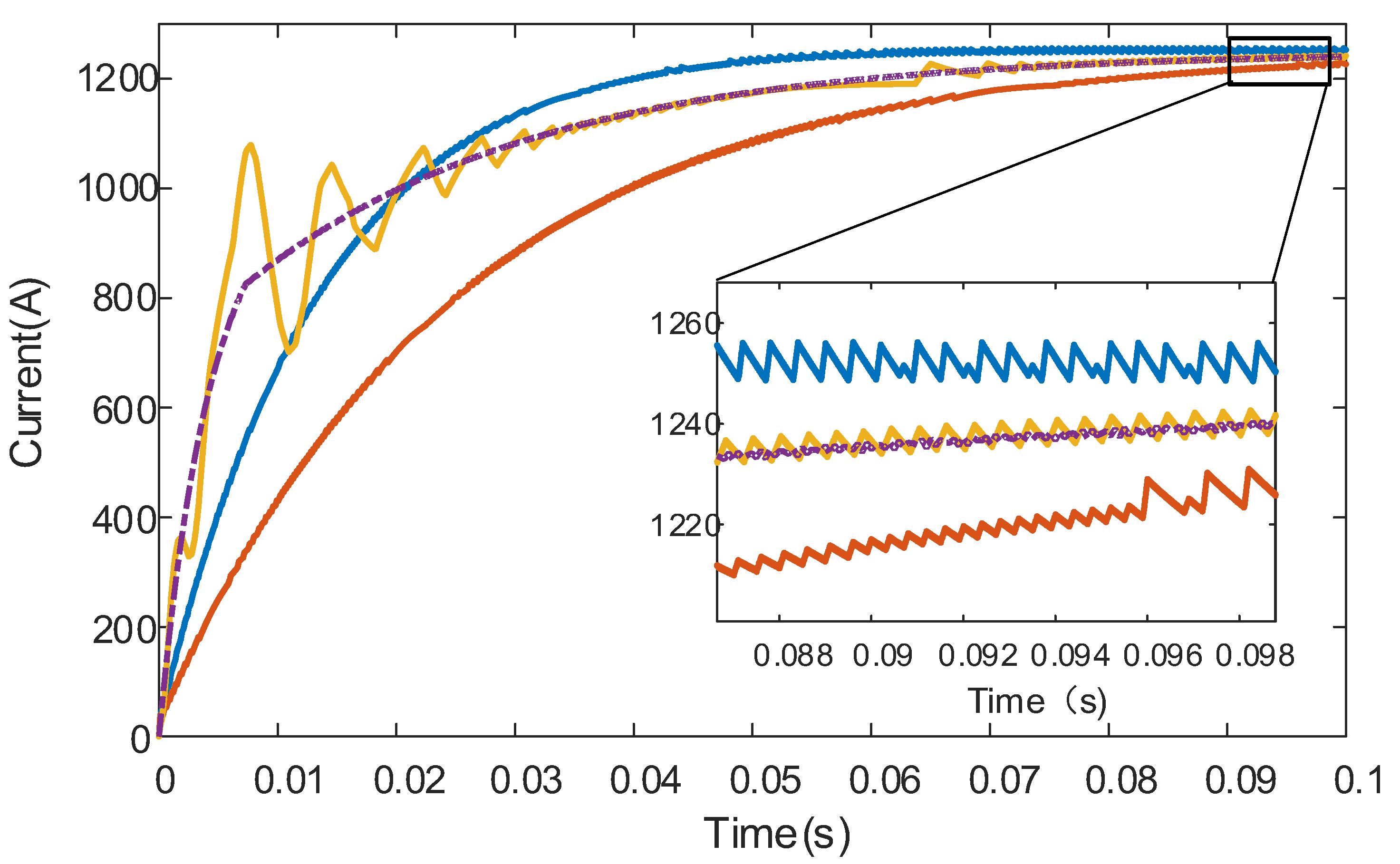
4.4. Current Random Disturbance Experiment
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gugua, E.C.; Ujah, C.O.; Asadu, C.O.; Von Kallon, D.V.; Ekwueme, B.N. Electroplating in the modern era, improvements and challenges: A review. Hybrid Adv. 2024, 7, 100286. [Google Scholar] [CrossRef]
- Nayanasiri, D.; Li, Y. Step-Down DC–DC Converters: An Overview and Outlook. Electronics 2022, 11, 1693. [Google Scholar] [CrossRef]
- Gao, Y.; Tang, Y.; Yao, F.; Ge, L.; Guo, Y.; Sun, H. Paralleled Variable Inductor Phase-Shifted Full-Bridge Converter with Full-Load Range ZVS and Low Duty Cycle Loss. IEEE Trans. Ind. Electron. 2024, 71, 4673–4684. [Google Scholar] [CrossRef]
- Yen, W.-W.; Chao, P.C.-P. A ZVS Phase-Shift Full-Bridge Converter With Input Current Steering to Reduce EMI Noise. IEEE Trans. Power Electron. 2022, 37, 11937–11950. [Google Scholar] [CrossRef]
- Xu, Z.; Pan, J. Research review of phase-shifted full-bridge ZVS DC converters. J. Power Sources 2022, 20, 11–27. [Google Scholar]
- Wang, P.; Xu, Z.; Wang, L.; Gao, L.; Wang, X. Hybrid Optimal Control Strategy for Efficiency and Dynamic Performance of dual active bridge DC-DC Converter based on triple Phase shift. Trans. China Electrotech. Soc. 2019, 37, 4720–4731. [Google Scholar]
- Wang, H.; Liu, Z.; Lv, J. Research on Model Predictive Control of Interleaved Parallel bidirectional DC-DC Converter. Guangxi Electr. Power 2022, 45, 49–54. [Google Scholar]
- Meng, L.; Hu, X. Dynamic compensation control of Buck bidirectional DC-DC Converter based on Model Predictive control. J. Power Sources 2024, 22, 90–97. [Google Scholar]
- Ullah, B.; Ullah, H.; Khalid, S. Direct Model Predictive Control of Noninverting Buck-boost DC-DC Converter. CES Trans. Electr. Mach. Syst. 2022, 6, 332–339. [Google Scholar] [CrossRef]
- Sadiq, M.; Aragon, C.A.; Terriche, Y.; Ali, S.W.; Su, C.-L.; Buzna, Ľ.; Elsisi, M.; Lee, C.-H. Continuous-Control-Set Model Predictive Control for Three-Level DC–DC Converter with Unbalanced Loads in Bipolar Electric Vehicle Charging Stations. Mathematics 2022, 10, 3444. [Google Scholar] [CrossRef]
- Razani, R.; Mohamed, Y.A.-R.I. Model Predictive Control of Non-Isolated DC/DC Modular Multilevel Converter Improving the Dynamic Response. IEEE Open J. Power Electron. 2022, 3, 303–316. [Google Scholar] [CrossRef]
- Dang, C.; Wang, F.; Mu, X.; Liu, D.; Tong, X.; Song, W.; Huang, J. Double vector predictive constant frequency control of Vienna Rectifier with inductance parameter identification. Proc. CSEE 2022, 42 (Suppl. S1), 246–255. [Google Scholar]
- Feng, X.; Cui, X.; Shao, K. A linear active disturbance rejection control strategy for Vienna Rectifier. Power Electron. Technol. 2020, 54, 103–105. [Google Scholar]
- Fu, X.; Wang, L.; Wang, X. Nonlinear sliding mode control strategy for grid-connected photovoltaic inverters. J. Shenyang Univ. Technol. 2022, 44, 694–699. [Google Scholar]
- Wang, R.; Li, J.; Sun, Q.; Zhang, H.; Wei, Z.; Wang, P. Energy Transfer Converter Between Electric Vehicles: DC–DC Converter Based on Virtual Power Model Predictive Control. IEEE Trans. Consum. Electron. 2023, 69, 556–567. [Google Scholar] [CrossRef]
- Tan, B.; Li, H.; Zhao, D.; Liang, Z.; Ma, R.; Huangfu, Y. Finite-control-set model predictive control of interleaved DC-DC boost converter Based on Kalman the observer. eTransportation 2022, 11, 100158. [Google Scholar] [CrossRef]
- Zhang, W.; Chen, L. Model Predictive Control Strategy for Three-phase Inverter Based on Disturbance Suppression. Electr. Drive 2019, 51, 43–50. [Google Scholar]
- Babayomi, O.O.; Zhang, Z.; Li, Z.; Park, K.-B. Bidirectional DC-DC converters for distributed energy resources: Robust predictive control with structurally-adaptive extended state observers. Int. J. Electr. Power Energy Syst. 2024, 158, 109913. [Google Scholar] [CrossRef]
- Chen, W.; Zeng, S.; Zhang, G.; Shi, T.; Xia, C. A modified double vectors model predictive torque control of permanent magnet synchronous motor. IEEE Trans. Power Electron. 2019, 34, 11419–11428. [Google Scholar] [CrossRef]
- Kang, S.; Soh, J.; Kim, R. Symmetrical three-vector-based model predictive control with deadbeat solution for IPMSM in rotating reference frame. IEEE Trans. Ind. Electron. 2020, 67, 159–168. [Google Scholar] [CrossRef]
- Li, X.; Peng, T.; Dan, H.; Zhang, G.; Tang, W.; Wheeler, P. A modulated model predictive control scheme for the brushless doubly fed induction machine. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 6, 1681–1691. [Google Scholar] [CrossRef]
- Guo, L.; Jin, Y.; Cao, L.; Dai, L.; Luo, K. Hybrid single and double-voltage vector-based model predictive common-mode voltage reduction method for 2-level voltage source inverters. IET Power Electron. 2019, 12, 2086–2094. [Google Scholar] [CrossRef]
- Xu, H. Research on High-Reliability and High-Power Electroplating Power Supply. Master’s Thesis, Zhengzhou University, Zhengzhou, China, 2015. [Google Scholar]
- Capua, G.D.; Shirsavar, S.A.; Hallworth, M.A.; Femia, N. An enhanced model for small-signal analysis of the phase-shifted full-bridge converter. IEEE Trans. Power Electron. 2015, 30, 1567–1576. [Google Scholar] [CrossRef]
- Sun, T. Model Prediction of Direct Velocity Weak Field Control Strategy for Permanent Magnet Synchronous Motor Parameter Identification. Master’s Thesis, Xi’an University of Technology, Xi’an, China, 2023. [Google Scholar]
- Sun, X.; Zhou, Y.; Chen, G.; Ren, B. Model predictive control of a phase-shifted full-bridge dc-dc converter. In Proceedings of the 2020 IEEE 9th International Power Electronics and Motion Control Conference (IPEMC2020-ECCE Asia), Virtual, 29 November–2 December 2020; IEEE: New York, NY, USA; pp. 2710–2714. [Google Scholar]
- Liu, X.; Li, K.; Zhang, Q.; Zhang, C. Single-loop predictive control of PMSM based on nonlinear disturbance observers. Proc. CSEE 2018, 38, 2153–2162. [Google Scholar]
- Liu, Z.; Yuan, S.; Zheng, L.; Ma, Y.; Sun, Y. An improved NMPC-NDOB scheme for trajectory tracking of unmanned surface vessel. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2022, 236, 1012–1024. [Google Scholar] [CrossRef]
- Cao, L.; Li, Y.; Li, X.; Guo, L.; Jin, N.; Cao, H. A Dual-Vector Modulated Model Predictive Control Method for Voltage Source Inverters with a New Duty Cycle Calculation Method. Energies 2020, 13, 4200. [Google Scholar] [CrossRef]


| Argument | Numerical Value | Unit |
|---|---|---|
| Input voltage | 800 | Uin/V |
| Input filter capacitor | 250 | C/uF |
| Output filtering inductance | 1 | L/mH |
| Output filter capacitor | 600 | C/uF |
| Rated output voltage | 10 | Uout/V |
| Output current | 1250 | Iout/A |
| Switching frequency | 10 | f/KHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Y.; Liu, C.; Zhao, Q.; Liu, L. Research on Predictive Control Strategy for Phase Shift Full Bridge Transform Based on Improved Nonlinear Disturbance Observer. Electronics 2025, 14, 1002. https://doi.org/10.3390/electronics14051002
Wu Y, Liu C, Zhao Q, Liu L. Research on Predictive Control Strategy for Phase Shift Full Bridge Transform Based on Improved Nonlinear Disturbance Observer. Electronics. 2025; 14(5):1002. https://doi.org/10.3390/electronics14051002
Chicago/Turabian StyleWu, Yunbo, Cheng Liu, Qing Zhao, and Lixin Liu. 2025. "Research on Predictive Control Strategy for Phase Shift Full Bridge Transform Based on Improved Nonlinear Disturbance Observer" Electronics 14, no. 5: 1002. https://doi.org/10.3390/electronics14051002
APA StyleWu, Y., Liu, C., Zhao, Q., & Liu, L. (2025). Research on Predictive Control Strategy for Phase Shift Full Bridge Transform Based on Improved Nonlinear Disturbance Observer. Electronics, 14(5), 1002. https://doi.org/10.3390/electronics14051002






