Design of 2.5 Bit Programmable Metasurface Unit Cell for Electromagnetic Manipulation
Abstract
1. Introduction
2. High-Quantitative-Accuracy Metasurface Unit Cell Modeling
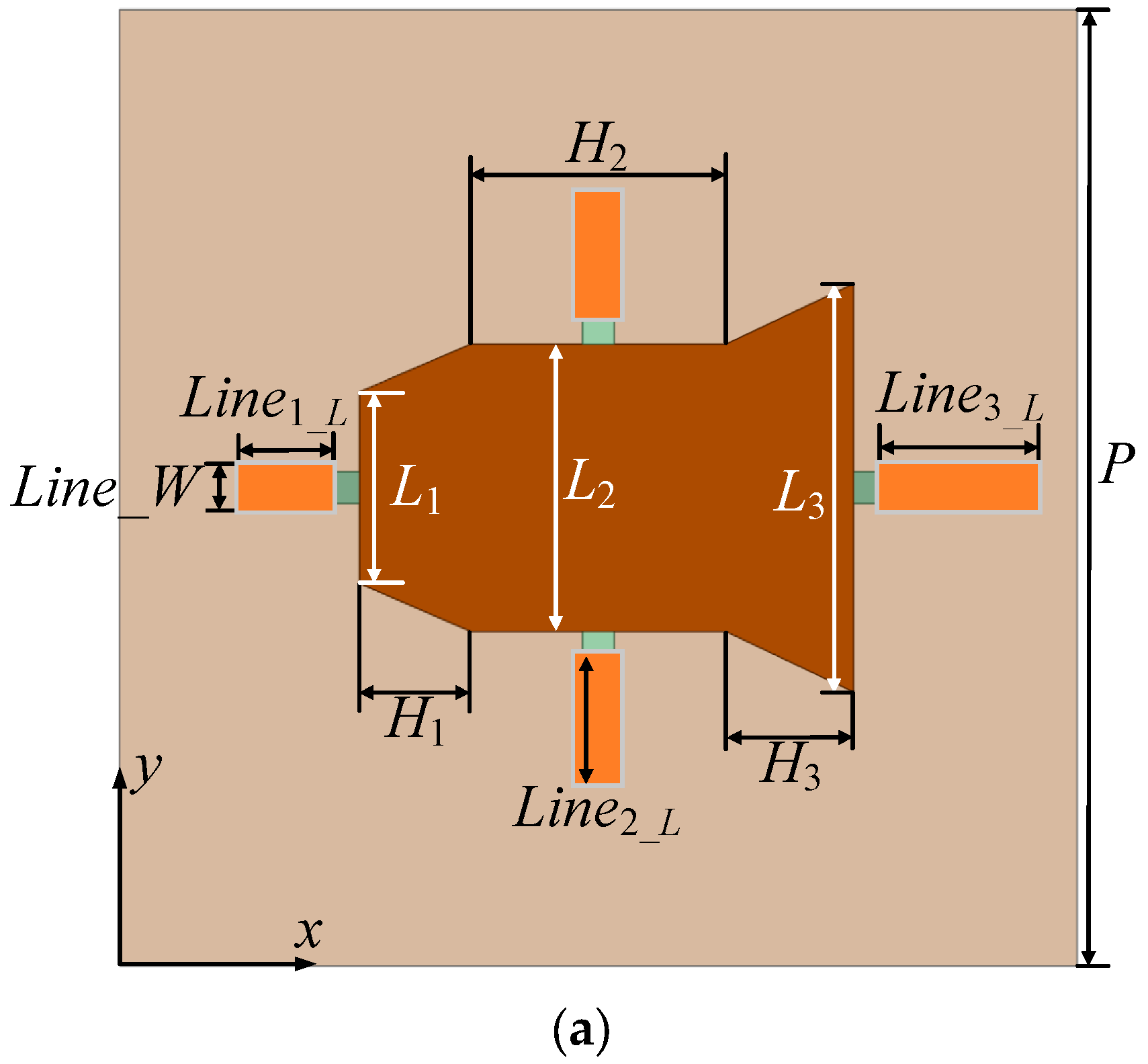
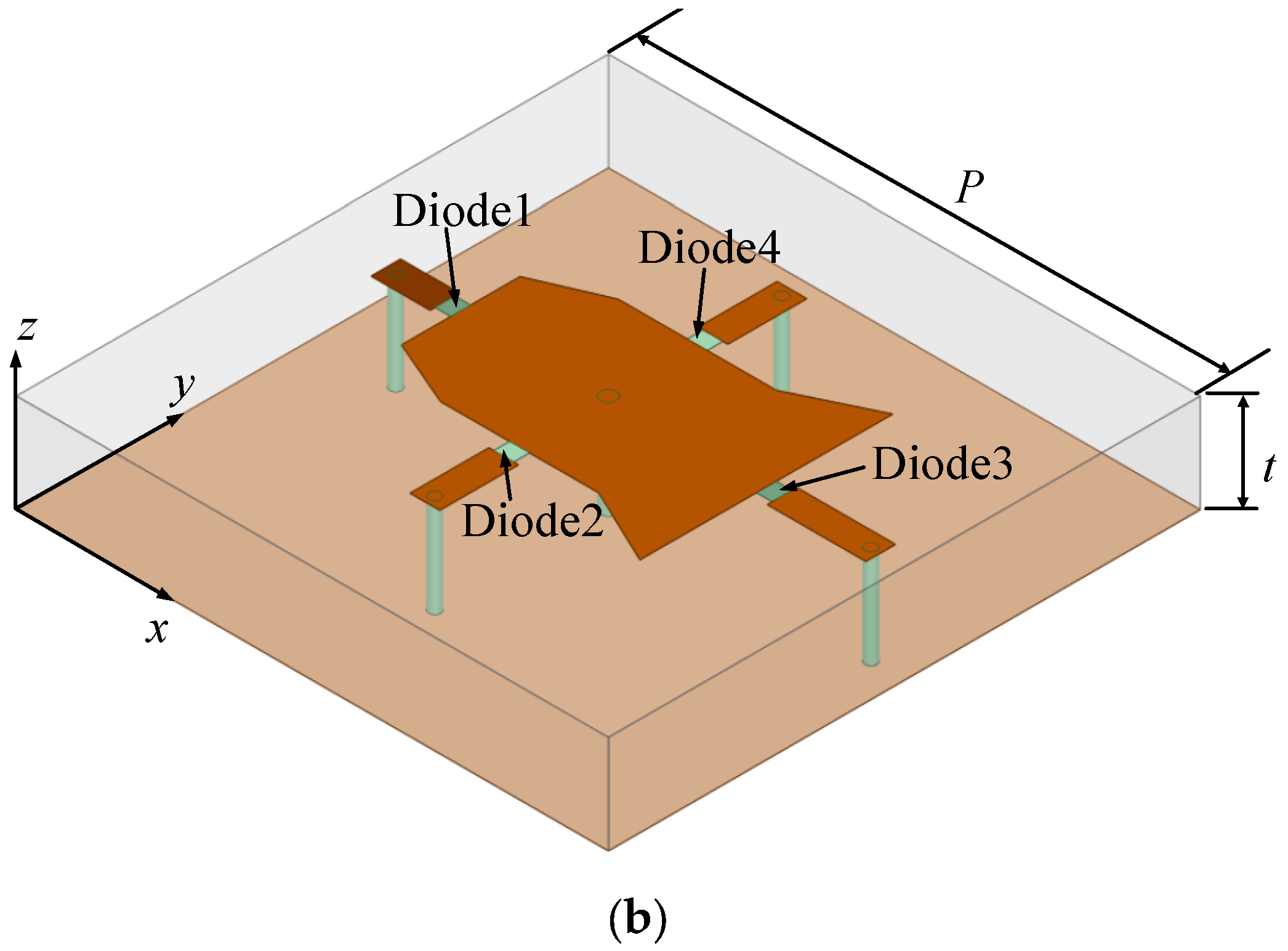
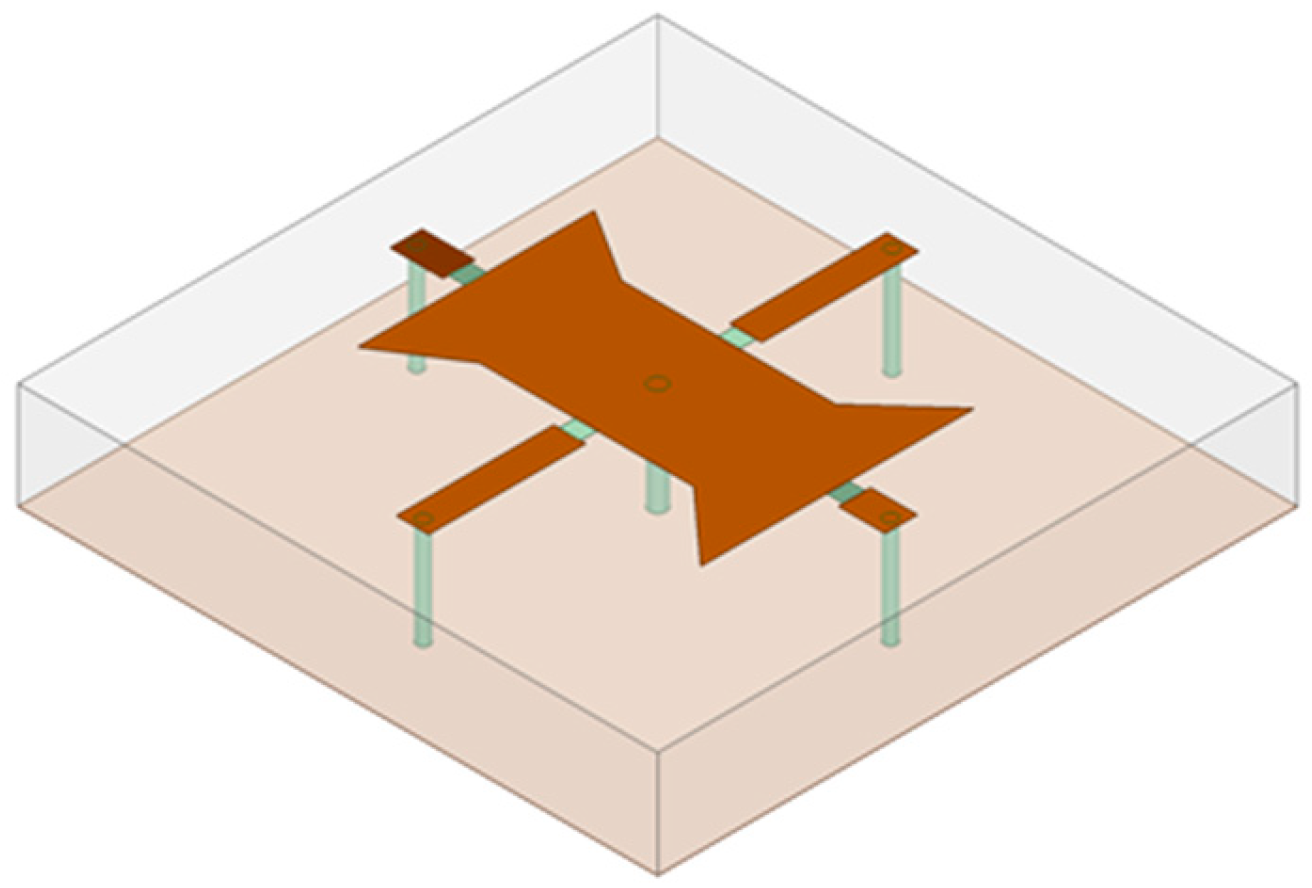
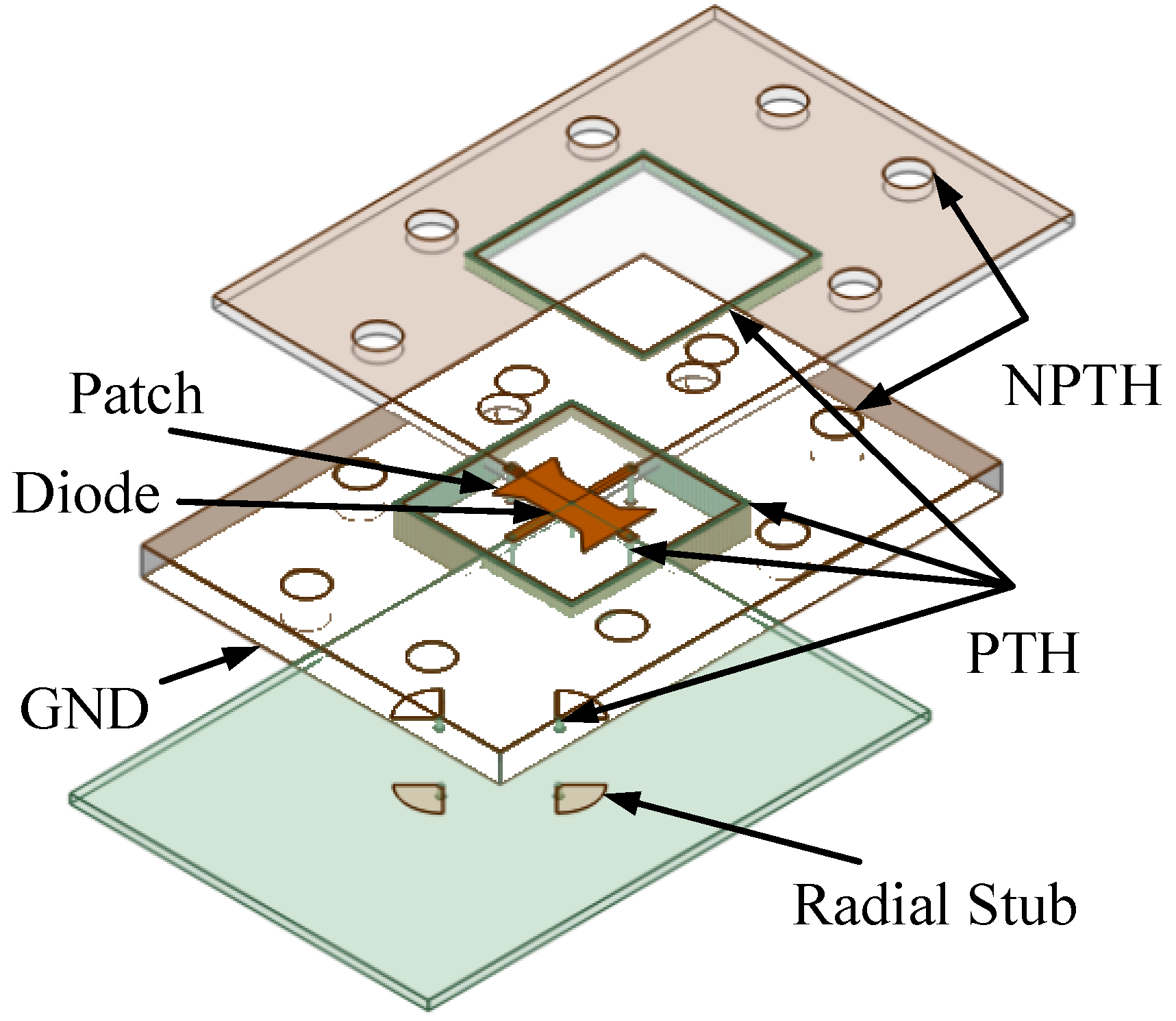
Unit Design
3. Optimization Algorithm
3.1. Genetic Optimization Algorithm
3.2. Algorithmic Framework
3.3. Design of the Fitness Function
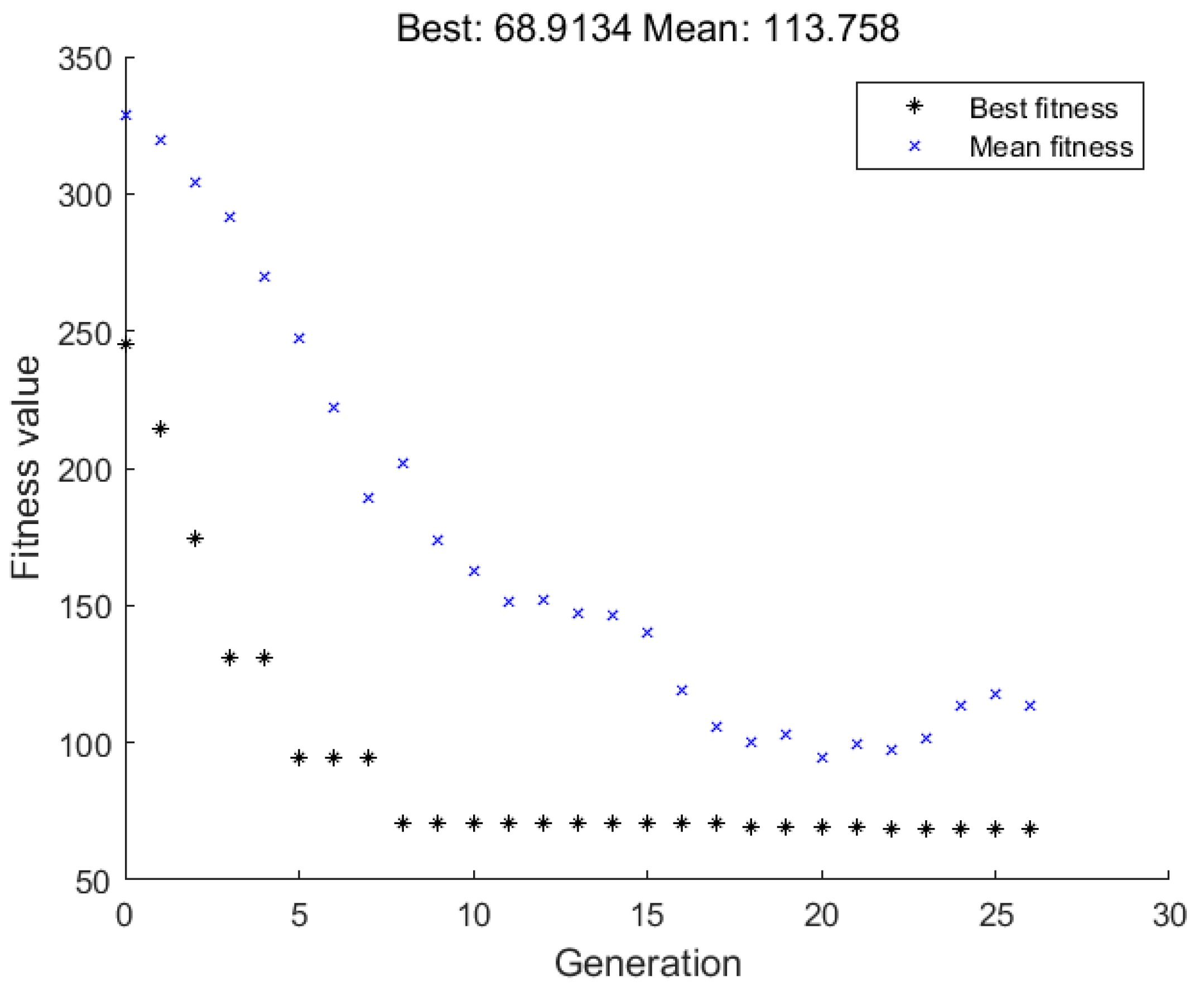
3.4. Algorithmic Implementation
4. Optimization and Measurement Results
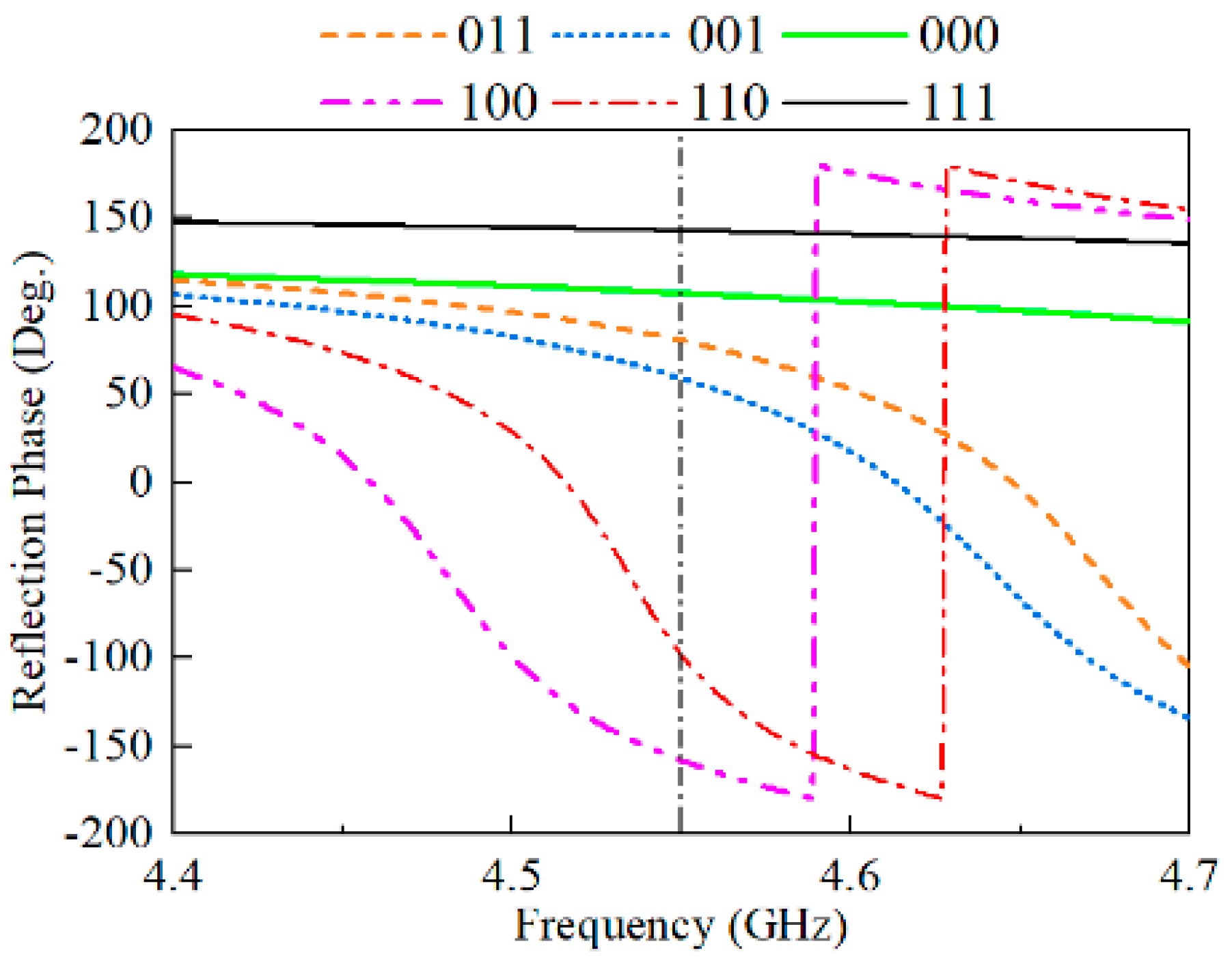
4.1. Metasurface Unit Optimization Results
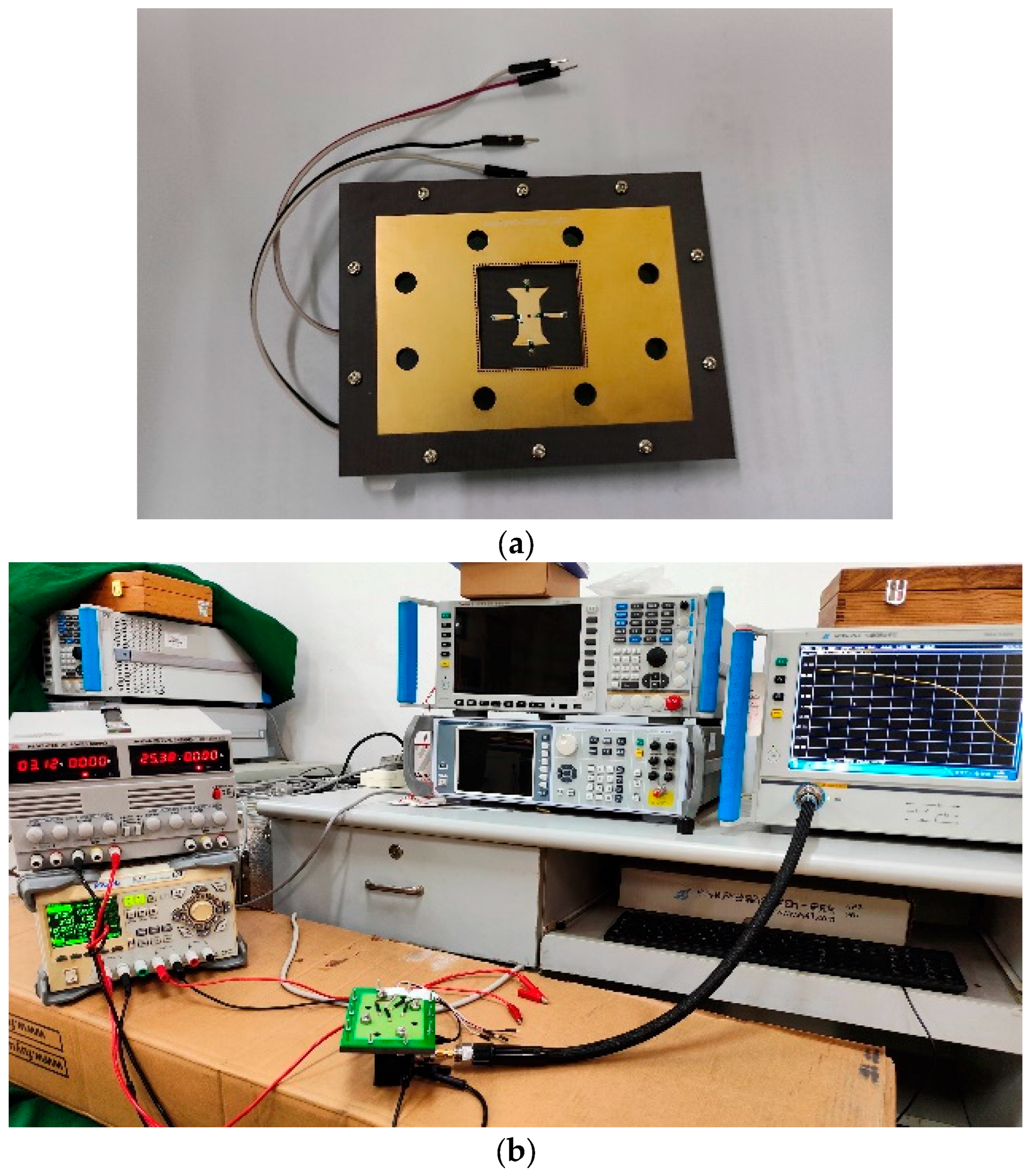
4.2. Unit Measurement
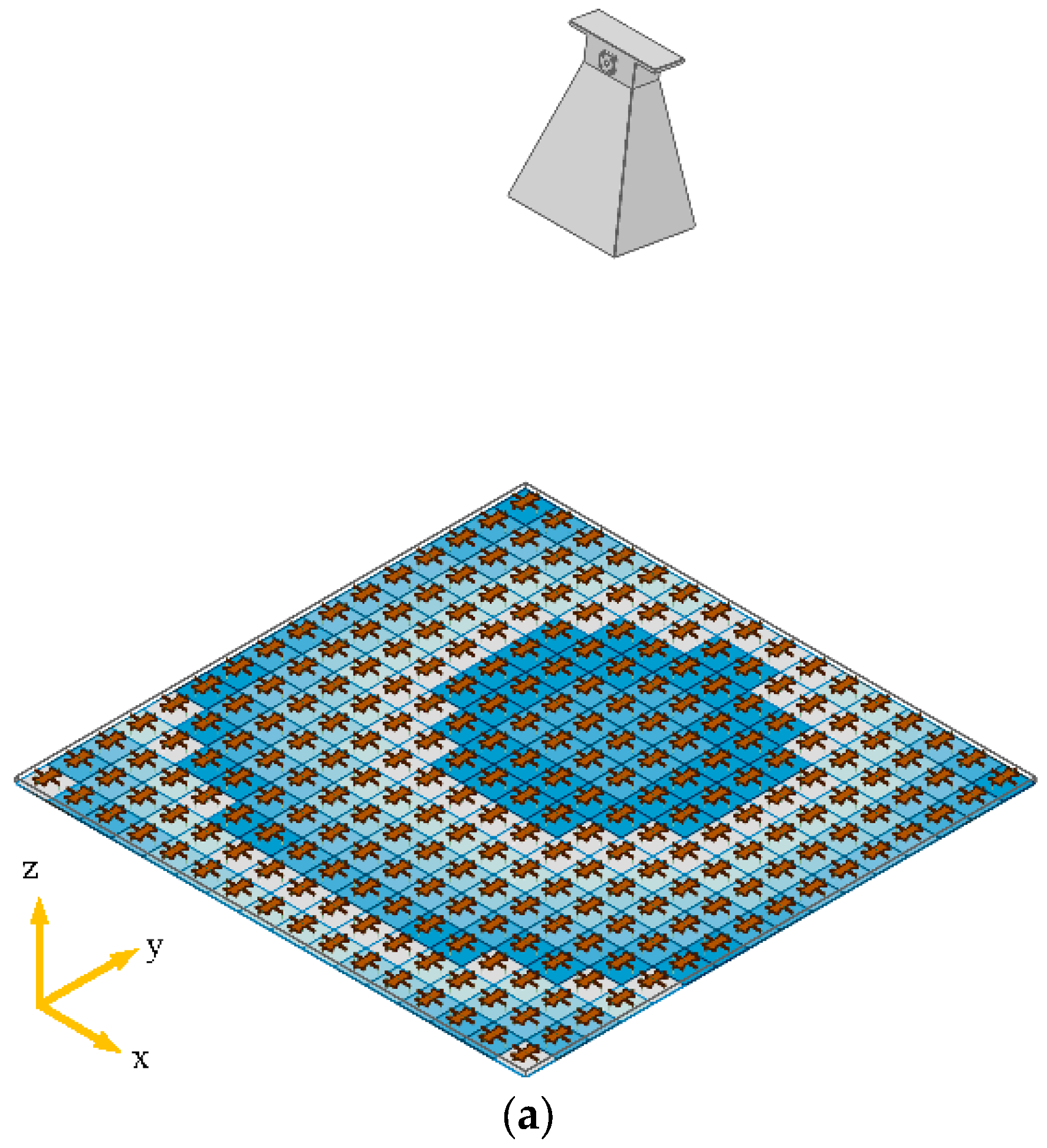
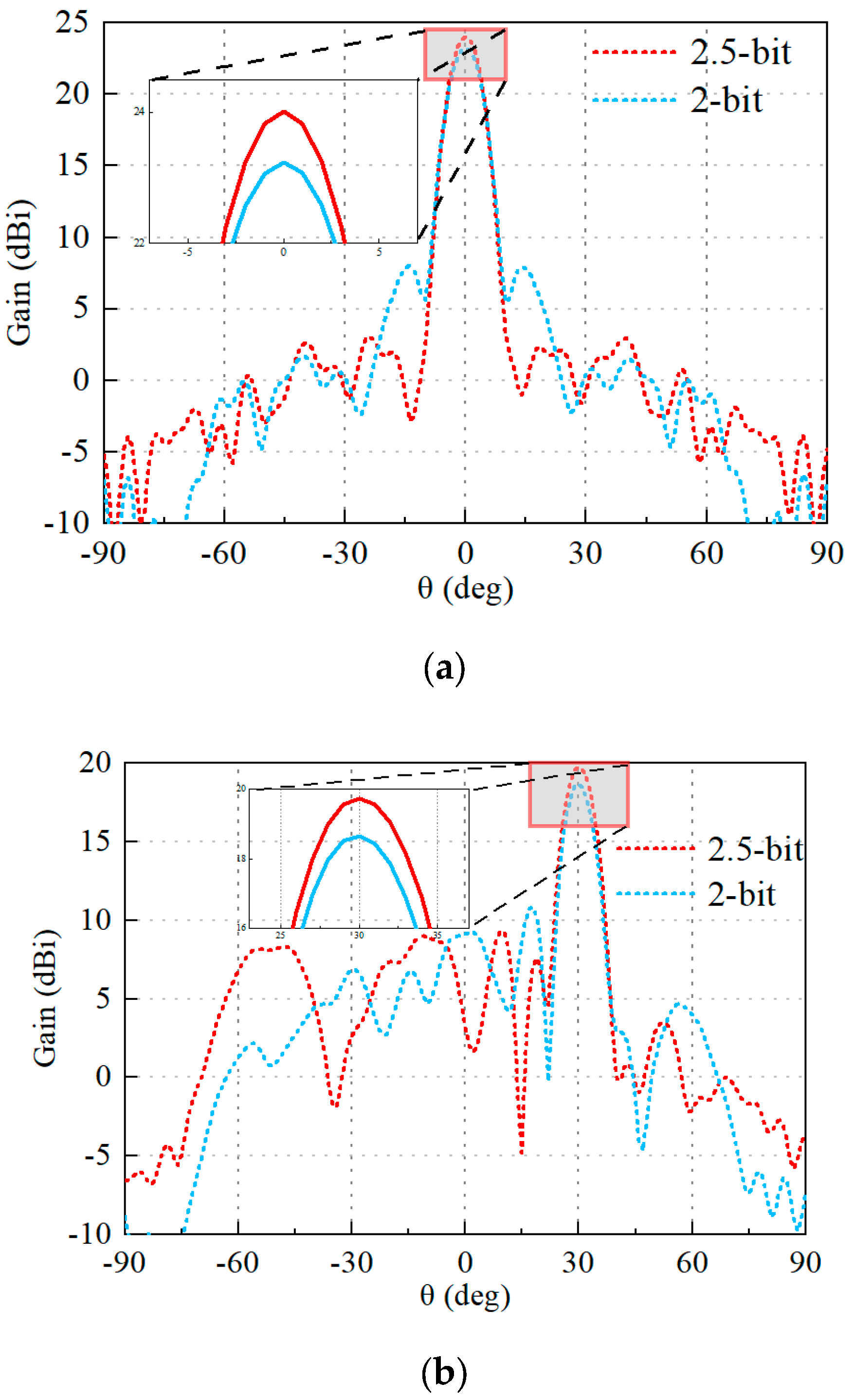
4.3. The Metasurface Antenna Design and Simulation Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Naqvi, A.H.; Lim, S. Review of recent phased arrays for millimeter-wave wireless communication. Sensors 2018, 18, 3194. [Google Scholar] [CrossRef] [PubMed]
- Nayeri, P.; Yang, F.; Elsherbeni, A.Z. Beam-scanning reflectarray antennas: A technical overview and state of the art. IEEE Antennas Propag. Mag. 2015, 57, 32–47. [Google Scholar] [CrossRef]
- Cui, T.J.; Qi, M.Q.; Wan, X.; Zhao, J.; Cheng, Q. Coding metamaterials, digital metamaterials and programmable metamaterials. Light. Sci. Appl. 2014, 3, e218. [Google Scholar] [CrossRef]
- Singh, K.; Saikia, M.; Thiyagarajan, K.; Thalakotuna, D.; Esselle, K.; Kodagoda, S. Multi-functional reconfigurable intelligent surfaces for enhanced sensing and communication. Sensors 2023, 23, 8561. [Google Scholar] [CrossRef] [PubMed]
- Gao, L.; Zhou, Y.; Zhu, H.; Zheng, P.; Liu, J.; He, Z.; Xu, Z.; Cui, Y. Reconfigurable amplitude-phase-coding metasurface with flexible beamforming capability. Electronics 2023, 12, 4565. [Google Scholar] [CrossRef]
- Selvaraj, M.; Vijay, R.; Anbazhagan, R.; Rengarajan, A. Reconfigurable metasurface: Enabling tunable reflection in 6g wireless communications. Sensors 2023, 23, 9166. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Wang, W.; Moitra, P.; Kravchenko, I.I.; Briggs, D.P.; Valentine, J. Dielectric meta-reflectarray for broadband linear polarization conversion and optical vortex generation. Nano Lett. 2014, 14, 1394–1399. [Google Scholar] [CrossRef] [PubMed]
- Zhang, T.Y.; Sun, S.; Gou, Y.; Wang, H.L.; Ma, H.F.; Cui, T.J. Frequency-multiplexed holographic-reflective coding metasurface for independent controls of surface wave and spatially propagating wave. Adv. Opt. Mater. 2023, 11, 202202832. [Google Scholar] [CrossRef]
- Lin, R.J.; Su, V.-C.; Wang, S.; Chen, M.K.; Chung, T.L.; Chen, Y.H.; Kuo, H.Y.; Chen, J.-W.; Chen, J.; Huang, Y.-T.; et al. Achromatic metalens array for full-colour light-field imaging. Nat. Nanotechnol. 2019, 14, 227–231. [Google Scholar] [CrossRef]
- Han, J.; Li, L.; Ma, X.; Gao, X.; Mu, Y.; Liao, G.; Luo, Z.J.; Cui, T.J. Adaptively smart wireless power transfer using 2-bit programmable metasurface. IEEE Trans. Ind. Electron. 2022, 69, 8524–8534. [Google Scholar] [CrossRef]
- Zheng, G.; Mühlenbernd, H.; Kenney, M.; Li, G.; Zentgraf, T.; Zhang, S. Metasurface holograms reaching 80% efficiency. Nat. Nanotechnol. 2015, 10, 308–312. [Google Scholar] [CrossRef]
- Wang, Y.; Guan, C.; Ding, X.; Zhang, K.; Wang, J.; Burokur, S.N.; Wu, Q. Huygens’ metasurface with stable transmission response under wide range of incidence angle. IEEE Antennas Wirel. Propag. Lett. 2022, 21, 630–634. [Google Scholar] [CrossRef]
- Yang, H.; Yang, F.; Xu, S.; Li, M.; Cao, X.; Gao, J.; Zheng, Y. A study of phase quantization effects for reconfigurable reflectarray antennas. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 302–305. [Google Scholar] [CrossRef]
- Yang, H.; Yang, F.; Xu, S.; Mao, Y.; Li, M.; Cao, X.; Gao, J. A 1-bit 10 × 10 reconfigurable reflectarray antenna: Design, optimization, and experiment. IEEE Trans. Antennas Propag. 2016, 64, 2246–2254. [Google Scholar] [CrossRef]
- Liang, J.C.; Cheng, Q.; Gao, Y.; Xiao, C.; Gao, S.; Zhang, L.; Jin, S.; Cui, T.J. An angle-insensitive 3-bit reconfigurable intelligent surface. iEEE Trans. Antennas Propag. 2022, 70, 8798–8808. [Google Scholar] [CrossRef]
- Trampler, M.E.; Lovato, R.E.; Gong, X. Dual-resonance continuously beam-scanning x-band reflectarray antenna. IEEE Trans. Antennas Propag. 2020, 68, 6080–6087. [Google Scholar] [CrossRef]
- Tang, W.; Dai, J.Y.; Chen, M.; Li, X.; Cheng, Q.; Jin, S.; Wong, K.K.; Cui, T.J. Programmable metasurface-based rf chain-free 8psk wireless transmitter. Electron. Lett. 2019, 55, 417–420. [Google Scholar] [CrossRef]
- Li, P.; Ren, J.; Chen, Y.; Ren, X.; Xu, K.-D.; Yin, Y.-Z.; Shen, M. Design of low-cost single-layer 2-bit reflective programmable metasurface based on folded ground. IEEE Trans. Microw. Theory Tech. 2023, 71, 3455–3465. [Google Scholar] [CrossRef]
- Xiang, B.J.; Dai, X.; Luk, K.-M. A Wideband low-cost reconfigurable reflectarray antenna with 1-bit resolution. IEEE Trans. Antennas Propag. 2022, 70, 7439–7447. [Google Scholar] [CrossRef]
- Kim, J.; Kim, J.; Oh, J.H.; Wi, S.H.; Oh, J. Rotated feed-combined reconfigurable transmit ris with disparate deployment of 1-bit hybrid units for b5g/6g. IEEE Trans. Antennas Propag. 2023, 71, 5457–5462. [Google Scholar] [CrossRef]
- Fazal, D.; Hong, I.-P. A new unit-cell design for a 2-bit reflective metasurface for ris applications. Electronics 2023, 12, 4220. [Google Scholar] [CrossRef]
- Rains, J.; Kazim, J.U.R.; Zhang, L.; Abbasi, Q.H.; Imran, M.; Tukmanov, A. 2.75-bit reflecting unit cell design for reconfigurable intelligent surfaces. In Proceedings of the 2021 IEEE International Symposium on Antennas and Propagation and USNC-URSI Radio Science Meeting (APS/URSI), Singapore, 4–10 December 2021; pp. 335–336. [Google Scholar]
- Saifullah, Y.; Zhang, F.; Yang, G.-M.; Xu, F. 3-bit programmable reflective metasurface. In Proceedings of the 2018 12th International Symposium on Antennas, Propagation and EM Theory (ISAPE), Hangzhou, China, 3–6 December 2018; pp. 1–2. [Google Scholar]
- Wang, X.; Xia, D.; Li, G.; Ma, X.; Xu, P.; Han, J.; Liu, H.; Li, L. Design and waveguide measurement of 2-bit reconfigurable amplification metasurface cell. IEEE Antennas Wirel. Propag. Lett. 2023, 22, 2090–2094. [Google Scholar] [CrossRef]
- Holland, J.H. Genetic algorithms and the optimal allocation of trials. SIAM J. Comput. 1973, 2, 88–105. [Google Scholar] [CrossRef]
- Deb, K.; Pratap, A.; Agarwal, S.; Meyarivan, T. A fast and elitist multiobjective genetic algorithm: NSGA-II. IEEE Trans. Evol. Comput. 2002, 6, 182–197. [Google Scholar] [CrossRef]
- Zitzler, E.; Laumanns, M.; Thiele, L. SPEA2: Improving the Strength Pareto Evolutionary Algorithm; TIK-Report; ETH Zurich: Zurich, Switzerland, 2001; Volume 103. [Google Scholar] [CrossRef]
- WoodyBuendia. Hfss-Matlab-Api. Available online: https://github.com/WoodyBuendia/HFSS-MATLAB-API (accessed on 10 March 2024).
- KORVIN011. Ads-Matlab-Interface. Available online: https://github.com/korvin011/ADS-Matlab-Interface (accessed on 10 March 2024).
- Wu, L.-X.; Hu, Q.; Luo, X.-Y.; Zhao, J.; Jiang, T.; Chen, K.; Feng, Y. Wideband Dual-Feed Dual-Polarized Reflectarray Antenna Using Anisotropic Metasurface. IEEE Antennas Wirel. Propag. Lett. 2022, 21, 129–133. [Google Scholar] [CrossRef]
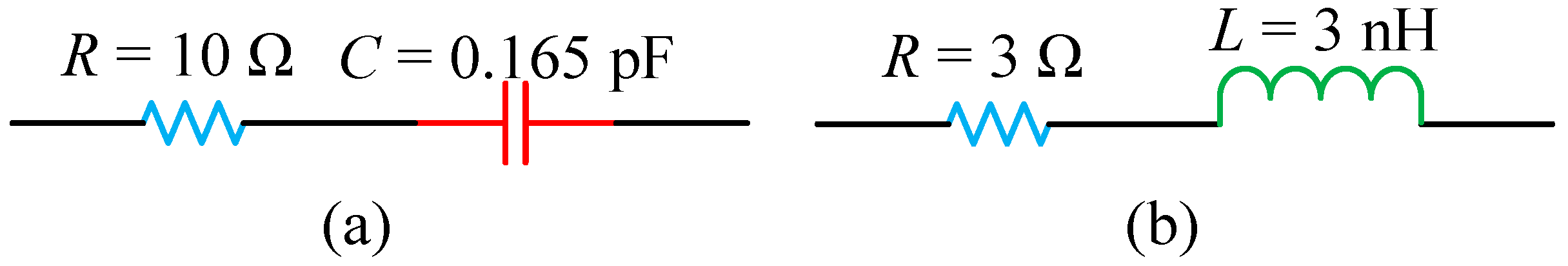




| Diode 1 | ON | OFF | |||
|---|---|---|---|---|---|
| Diode 3 | ON | OFF | ON | OFF | |
| Diodes 2 and 4 | |||||
| ON | 000 | 001 | 100 | 101 | |
| OFF | 010 | 011 | 110 | 111 | |
| Operating state | 100 | 110 | 001 | 011 | 010/000 | 101/111 |
| Reflection phase | −155° | −97° | −29° | 37° | 95° | 136° |
| Parameters | H1 | H2 | H3 | L1 | L2 |
| Length/mm | 3.48 | 10.07 | 3.40 | 11.08 | 6.66 |
| Parameters | L3 | Line1_L | Line2_L | Line3_L | |
| Length/mm | 12.80 | 2.48 | 7.37 | 2.18 |
| Reference | [30] | [19] | [18] | [22] | [23] | This Work |
|---|---|---|---|---|---|---|
| Quantization Accuracy | - | 1-bit | 2-bit | 2.75-bit | 3-bit | 2.5-bit |
| Quantization Error | - | - | - | ≈56.3% | ≈88.9% | 13.1% |
| Number of Diodes | - | 1 | 2 | 3 | 4 | 4 |
| Aperture Size | 7.75λ × 7.75λ | 6λ × 6λ | 8λ × 8λ | - | - | 8λ × 8λ |
| SLLs (dB) | ≈−20 | −13.8 | −14 | - | - | −20 |
| Reconfigurable | No | Yes | Yes | Yes | Yes | Yes |
| Aperture Efficiency | 37.4% | 24.0% | 28.2% | - | - | 31.9% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luan, Y.; Lu, Y.; Ren, J.; Sun, F. Design of 2.5 Bit Programmable Metasurface Unit Cell for Electromagnetic Manipulation. Electronics 2024, 13, 1648. https://doi.org/10.3390/electronics13091648
Luan Y, Lu Y, Ren J, Sun F. Design of 2.5 Bit Programmable Metasurface Unit Cell for Electromagnetic Manipulation. Electronics. 2024; 13(9):1648. https://doi.org/10.3390/electronics13091648
Chicago/Turabian StyleLuan, Yuchen, Yuyang Lu, Jian Ren, and Fukun Sun. 2024. "Design of 2.5 Bit Programmable Metasurface Unit Cell for Electromagnetic Manipulation" Electronics 13, no. 9: 1648. https://doi.org/10.3390/electronics13091648
APA StyleLuan, Y., Lu, Y., Ren, J., & Sun, F. (2024). Design of 2.5 Bit Programmable Metasurface Unit Cell for Electromagnetic Manipulation. Electronics, 13(9), 1648. https://doi.org/10.3390/electronics13091648






