Recent Status and Prospects of Low-Temperature Drift Resistors
Abstract
1. Introduction
2. Theory and Hypothesis of TCR
2.1. Electron Mean Free Path
2.2. Balance between Two Temperature Coefficients
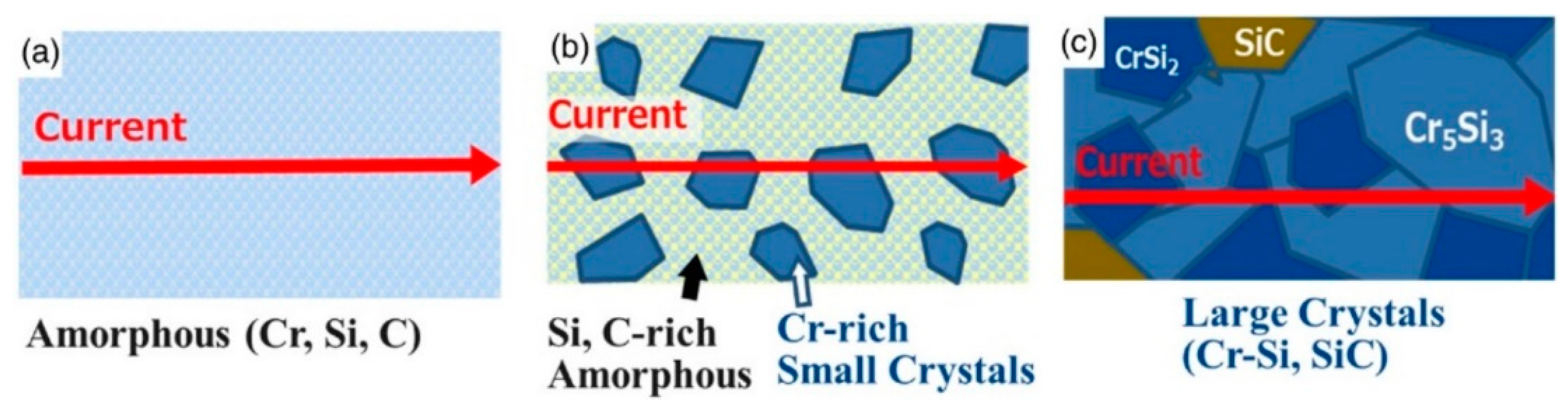
3. Current Advances in LTDRs
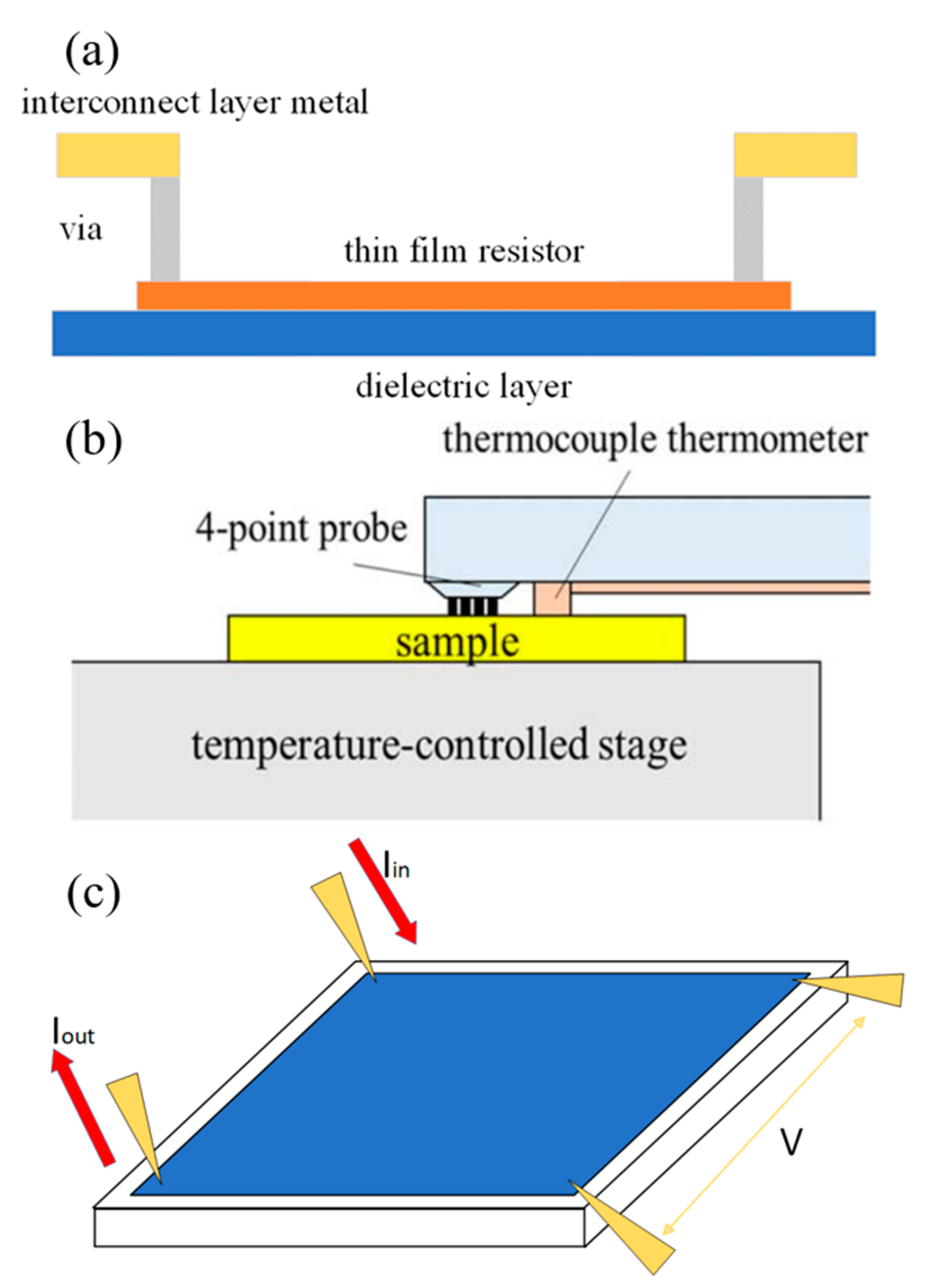
3.1. Methods for Measurement and Characterization
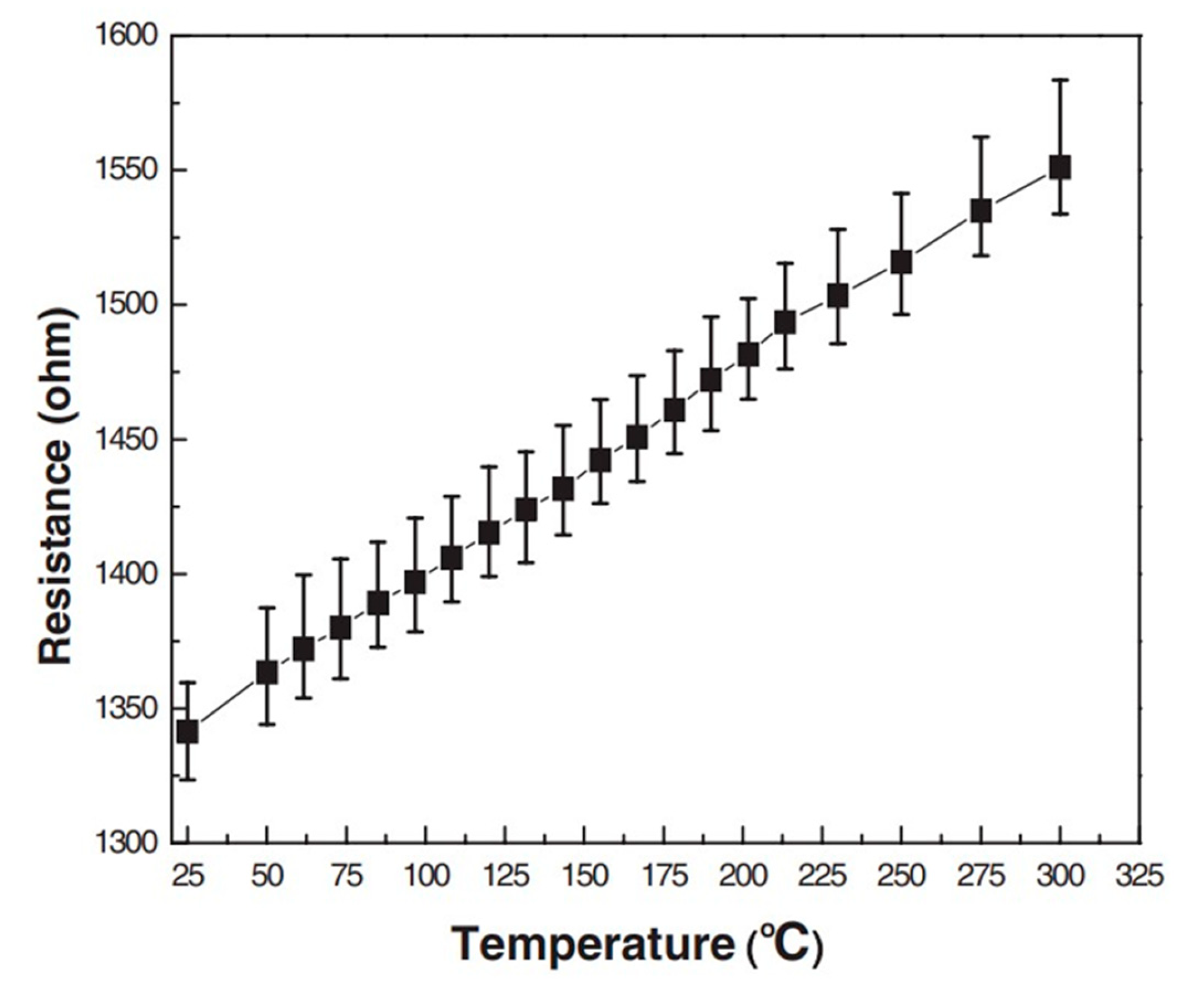
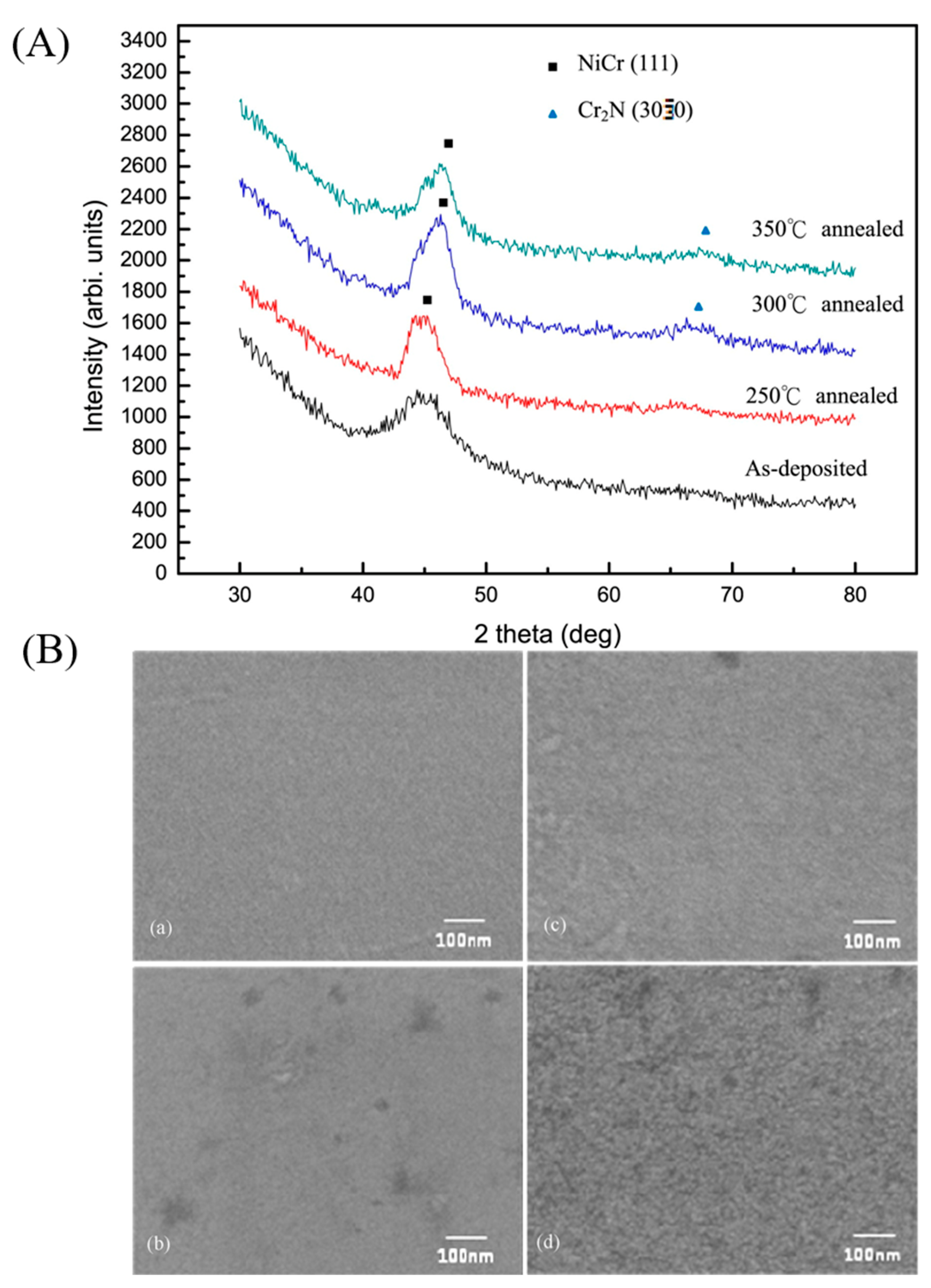
3.2. Types and Composition of Materials in LTDRs
| Material | Resistivity (μΩ·cm) | TCR (ppm/°C) |
|---|---|---|
| Cu | 0.000172 | >3900 |
| Ag | 0.000158 | 3800 |
| Ni | 0.00068 | 6900 |
| Cr | 0.00129 | 3000 |
| CuNi | 110 | <100 |
| AlV | 200 | <100 |
| CrNi | 110 | <100 |
| CuGe | 100 | <100 |
| CrSi | 2600 | 200 |
| MoSi | 1300 | 125 |
| Square Resistor Resistance | Film Material |
|---|---|
| 200 Ω/sq | MoSi2/NiCr |
| 2 kΩ/sq | SiCr/Cr-SiO |
| 20 kΩ/sq | SiCr |
| at. % Cr | ρ (Ω·cm) | TCR (ppm/℃) |
|---|---|---|
| 30 | 0.0058 | +100 |
| 27 | 0.0074 | ~0 |
| 25 | 0.0095 | −100 |
| 19 | 0.026 | −500 |
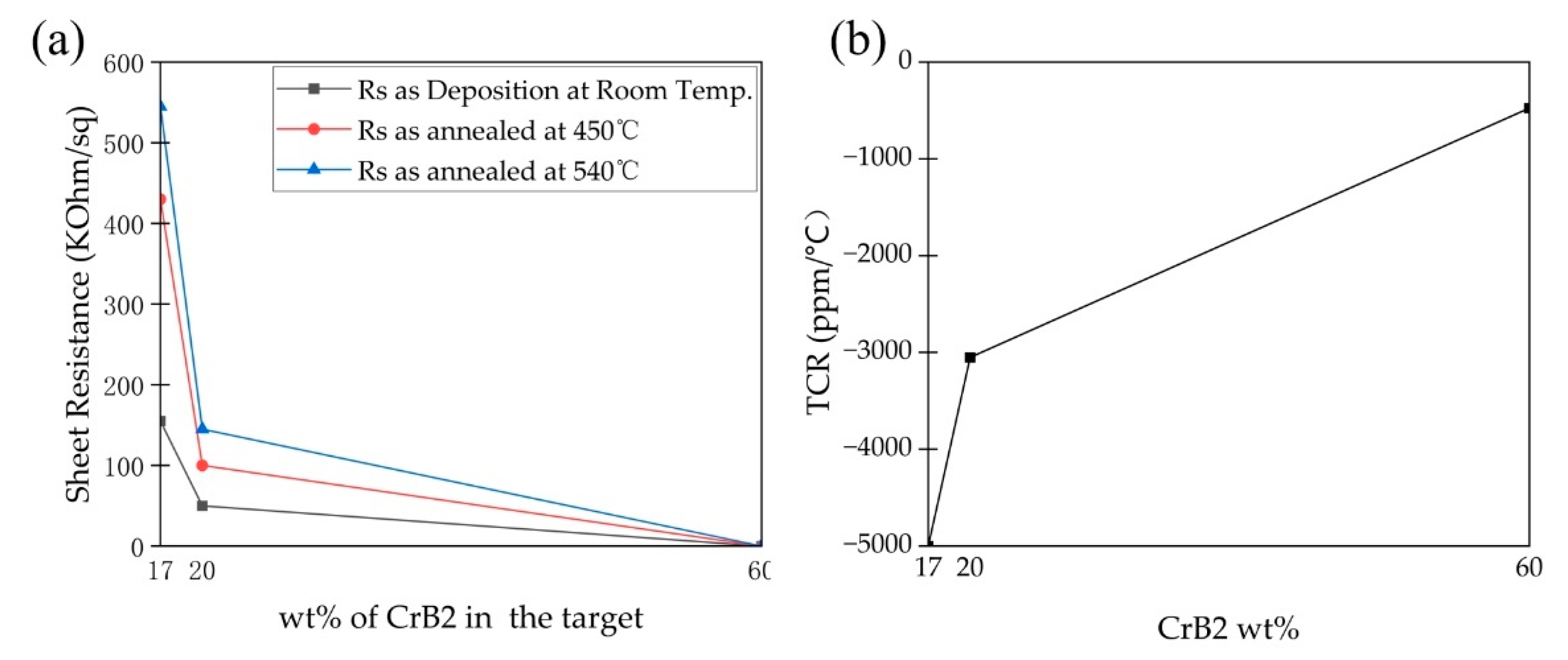
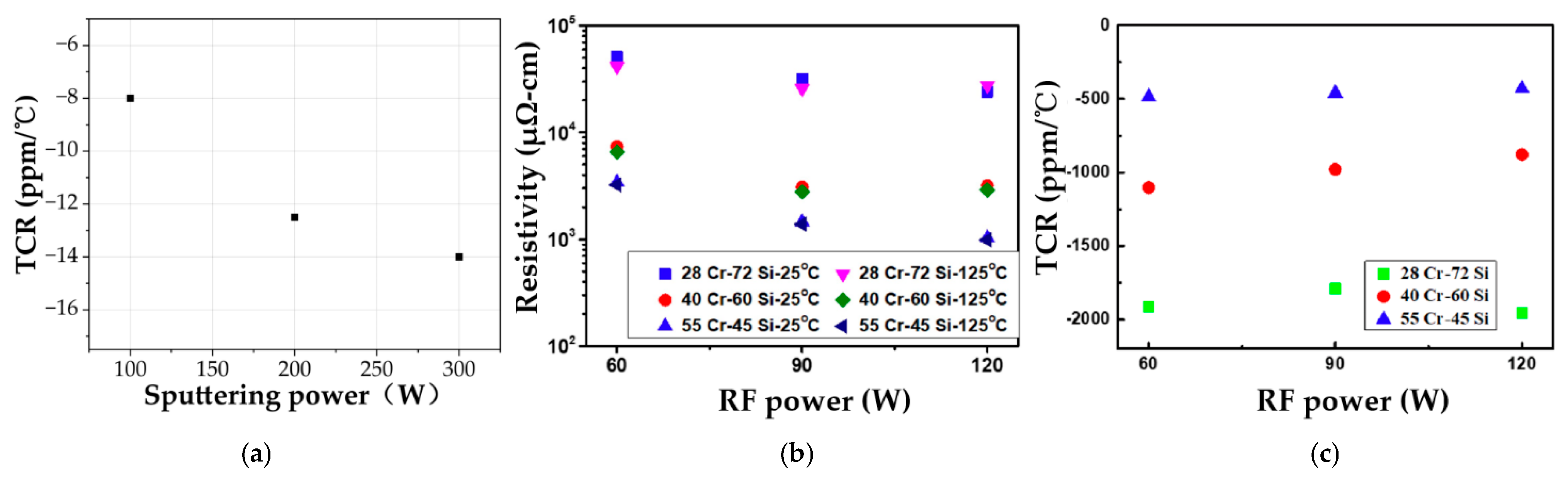
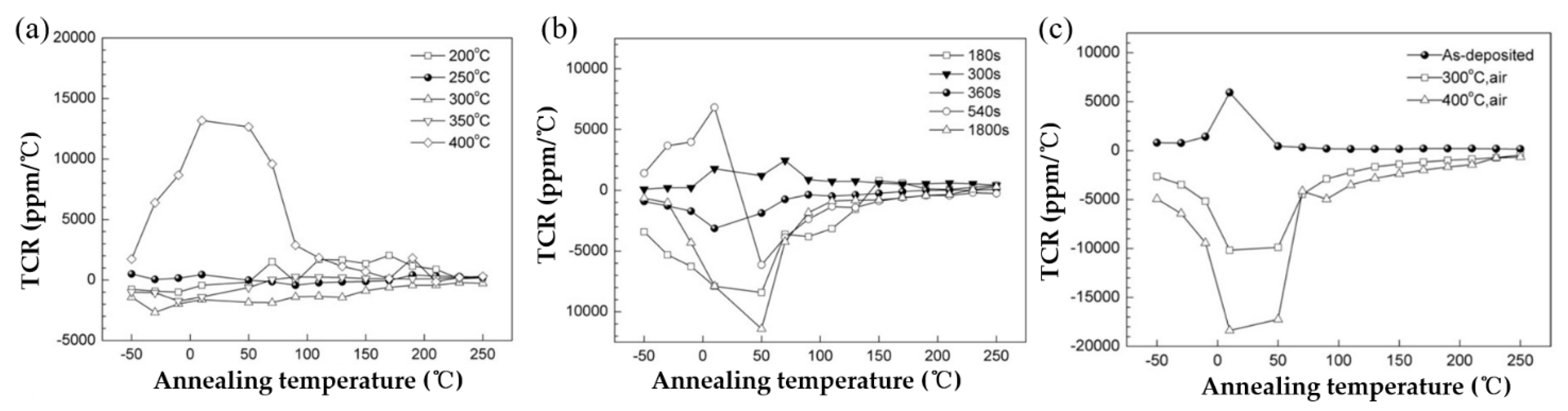
3.3. Fabrication of LTDRs




| Resistor Type | Thickness (nm) | Resistivity (Ohm·cm) | Current (A/cm2) | Tambient (°C) | Tresistor (°C) | EA (eV) |
|---|---|---|---|---|---|---|
| Cr(70%)Si(30%) | 5 | 5.5 × 10−3 | 1.0 × 107 | 25 | \ | \ |
| CrSi | 10 | 2.0 × 10−3 | 3.2 × 106 | 150–225 | 380–480 | 3.0 |
| CrSi | 10 | 2.7× 10−4 | 1.0 × 107 | \ | 386 | 1.46 |
| CrSi | <10 | <1.5 × 10−3 | 1.0 × 107 | 190–230 | n/a | 0.71–0.84 |
4. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Correction Statement
References
- Cheng, H.Y.; Chen, Y.C.; Li, C.L.; Li, P.J.; Houng, M.P.; Yang, C.F. Developments of the Physical and Electrical Properties of NiCr and NiCrSi Single-Layer and Bi-Layer Nano-Scale Thin-Film Resistors. Nanomaterials 2016, 6, 39. [Google Scholar] [CrossRef] [PubMed]
- Stewart, K.A.; Kimura, K.; Ring, M.; Noldus, K.; Hulse, P.; Jerome, R.C.; Hasegawa, A.; Gambino, J.P.; Price, D.T. Assessing SiCr resistor drift for automotive analog ICs. In Proceedings of the 2021 IEEE International Reliability Physics Symposium (IRPS), Monterey, CA, USA, 21–25 March 2021. [Google Scholar] [CrossRef]
- Tseng, H.W.; Feng, D.J.Y.; Li, C.L.; Yang, C.F. Effects of deposition parameters on properties of high resistance CrSi-based thin-film resistors. Int. J. Mod. Phys. B 2021, 35, 2150040. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, Z.; He, N.; Zhang, S.; Gu, L.; Zhang, L. Electrical Properties of CrSi Thin Film Resistors for High Precision Analog Circuits. In Proceedings of the 2022 10th International Symposium on Next-Generation Electronics (ISNE), Wuxi, China, 12–14 May 2023; pp. 1–3. [Google Scholar]
- Kwon, Y.C.; Seol, H.C.; Hong, S.K.; Kwon, O.K. Process Optimization of Integrated SiCr Thin-Film Resistor for High-Performance Analog Circuits. IEEE T Electron. Dev. 2014, 61, 8–14. [Google Scholar] [CrossRef]
- Gladun, C.; Heinrich, A.; Schumann, J.; Pitschke, W.; Vinzelberg, H. Transport-Properties of Nanodisperse Crxsi1-X Thin-Films. Int. J. Electron. 1994, 77, 301–308. [Google Scholar] [CrossRef]
- Burkov, A.T.; Vinzelberg, H.; Schumann, J.; Nakama, T.; Yagasaki, K. Strongly nonlinear electronic transport in Cr-Si composite films. J. Appl. Phys. 2004, 95, 7903–7907. [Google Scholar] [CrossRef]
- Cuong, N.D.; Kim, D.J.; Kang, B.D.; Yoon, S.G. Structural and electrical properties of TiNxOy thin-film resistors for 30 dB applications of π-type attenuator. J. Electrochem. Soc. 2006, 153, G856–G859. [Google Scholar] [CrossRef]
- Belser, R.B.; Hicklin, W.H. Temperature Coefficients of Resistance of Metallic Films in the Temperature Range 25° to 600°C. J. Appl. Phys. 1959, 30, 313–322. [Google Scholar] [CrossRef]
- Lacy, F. Evaluating the Resistivity-Temperature Relationship for RTDs and Other Conductors. IEEE Sens. J. 2011, 11, 1208–1213. [Google Scholar] [CrossRef]
- Lacy, F. Developing a theoretical relationship between electrical resistivity, temperature, and film thickness for conductors. Nanoscale Res. Lett. 2011, 6, 636. [Google Scholar] [CrossRef]
- Mooij, J.H. Electrical-Conduction in Concentrated Disordered Transition-Metal Alloys. Phys. Status Solidi A 1973, 17, 521–530. [Google Scholar] [CrossRef]
- Nocerino, G.; Singer, K.E. The electrical and compositional structure of thin Ni-Cr films. Thin Solid. Films 1979, 57, 343–348. [Google Scholar] [CrossRef]
- Ito, N.; Maekawa, K.; Kunimune, Y.; Hasegawa, E.; Abe, K.; Shiraishi, N.; Takahashi, Y.; Tonegawa, T.; Tsuchiya, Y.; Inoue, M. The effect of microstructures on the electrical properties of Cr-Si-C thin film resistors. Jpn. J. Appl. Phys. 2022, 61, SJ1004. [Google Scholar] [CrossRef]
- Glang, R.; Holmwood, R.A.; Herd, S.R. Resistivity and Structure of Cr-Sio Cermet Films. J. Vac. Sci. Technol. 1967, 4, 163–170. [Google Scholar] [CrossRef]
- Devries, J.W.C. Resistivity of Thin Au Films as a Function of Grain Diameter and Temperature. J. Phys. F Met. Phys. 1987, 17, 1945–1952. [Google Scholar] [CrossRef]
- Fuchs, K. The conductivity of thin metallic films according to the electron theory of metals. P. Camb. Philos. Soc. 1938, 34, 100–108. [Google Scholar] [CrossRef]
- Ma, H.; Ma, Y.; Han, X.; Chen, J.; Yang, Z.; Ta, S.W.; Zhang, Y.; Zhang, Z.Y.; Cao, Z. Influence of Al doping and annealing on the microstructures and electrical properties of CrSi films prepared by magnetron co-sputtering. Vacuum 2023, 210, 111904. [Google Scholar] [CrossRef]
- Chung, K.C.; Lee, W.H. Effect of pretreatment on Al2O3 substrate by depositing Al2O3 film on the properties of Ni-Cr-Si based thin film resistor. Mater. Chem. Phys. 2019, 234, 311–317. [Google Scholar] [CrossRef]
- Gao, C.W.; Zhang, D.C. Establishment and verification of resistance temperature coefficient model of P-type non-uniformly doped resistance. J. Micromech. Microeng. 2022, 32, 105006. [Google Scholar] [CrossRef]
- Cheng, Y.L.; Wei, B.J.; Shih, F.H.; Wang, Y.L. Stability and Reliability of Ti/TiN as a Thin Film Resistor. Ecs J. Solid. State Sc. 2013, 2, Q12–Q15. [Google Scholar] [CrossRef]
- Sumida, T. Recent Advances in Thick-Film Resistors and Target Applications. IEEE Power Electron. 2022, 9, 70–72. [Google Scholar] [CrossRef]
- Lu, Y.; Li, Y.; Peng, R.; Shi, L.; Li, F.; Chen, D.; Wen, Q.; Yang, C.; Yang, Q. Low temperature coefficient of resistance and high conductivity: Enhanced Cu–substituted LaCo0.4Ni0.6O3 ceramics for functional material. Ceram. Int. 2022, 48, 27899–27904. [Google Scholar] [CrossRef]
- Uang, C.-W.; Chuang, H.-M.; Shen-Fu, T.; Thei, K.-B.; Lai, P.-H.; Fu, S.-I.; Tsai, Y.-Y.; Liu, W.-C. Temperature-dependent characteristics of diffused and polysilicon resistors for ULSI applications. In Proceedings of the Fourth International Workshop on Junction Technology, 2004. IWJT’04, Shanghai, China, 16 March 2004; pp. 293–296. [Google Scholar]
- Hur, S.G.; Kim, D.J.; Kang, Y.D.; Yoon, S.G. Effect of the deposition temperature on temperature coefficient of resistance in CuNi thin film resistors. J. Vac. Sci. Technol. B 2004, 22, 2698–2701. [Google Scholar] [CrossRef]
- Ramadan, A.A.; Gould, R.D.; Ashour, A. On the Van der Pauw method of resistivity measurements. Thin Solid. Films 1994, 239, 272–275. [Google Scholar] [CrossRef]
- Sun, Y.-C.; Zhang, L.-Z. Measurement of sheet resistance for microa- reas by using a modified van der pauw’s method. Acta Phys. Sin. 1994, 43, 530–539. [Google Scholar] [CrossRef]
- Nava, F.; Tien, T.; Tu, K.N. Temperature-Dependence of Semiconducting and Structural-Properties of Cr-Si Thin-Films. J. Appl. Phys. 1985, 57, 2018–2025. [Google Scholar] [CrossRef]
- Jankowski, A.F.; Hayes, J.P. Ti-Cr-Al-O thin film resistors. Thin Solid. Films 2002, 420, 487–491. [Google Scholar] [CrossRef]
- Perillo, P.M.; Rodriguez, D.F. Influence of low temperature annealing time on CdS thin films. Physica B 2024, 680, 415828. [Google Scholar] [CrossRef]
- Chuang, N.C.; Lin, J.T.; Chen, H.R. TCR control of Ni-Cr resistive film deposited by DC magnetron sputtering. Vacuum 2015, 119, 200–203. [Google Scholar] [CrossRef]
- Waits, R.K. Silicide Resistors for Integrated Circuits. Pract. Inst. Electr. Elect. 1971, 59, 1425–1429. [Google Scholar] [CrossRef]
- Chang, L.C.; Sung, M.C.; Chen, Y.I. Effects of bias voltage and substrate temperature on the mechanical properties and oxidation behavior of CrSiN films. Vacuum 2021, 194, 110580. [Google Scholar] [CrossRef]
- Choi, J.Y.; Choi, C.H.; Cho, K.H.; Seong, T.G.; Nahm, S.; Kang, C.Y.; Yoon, S.J.; Kim, J.H. Effect of oxygen vacancy and Mn-doping on electrical properties of Bi4Ti3O12 thin film grown by pulsed laser deposition. Acta Mater. 2009, 57, 2454–2460. [Google Scholar] [CrossRef]
- Tian, G.K.; Shi, T.T.; Li, X.Y.; Lu, X.B.; Wang, Y.Y.; Liu, C. Low resistivity and near-zero temperature drift ZrB2-Ag composite films prepared by DC magnetron co-sputtering. Mater. Lett. 2022, 307, 130992. [Google Scholar] [CrossRef]
- Liang, Z.W.; Yang, S.A.; Wang, H.S.; Li, Y.L.; Li, J.F.; Hou, B.K.; Li, J.H.; Wang, J.; Wu, L.; Zhang, H.; et al. Temperature coefficient of resistance improvement in La0.67Ca0.33MnO3 polycrystalline ceramics with vanadium addition. Ceram. Int. 2023, 49, 13578–13585. [Google Scholar] [CrossRef]
- Waits, R.K. Sputtered Silicon-Chromium Resistive Films. J. Vac. Sci. Technol. 1969, 6, 308–315. [Google Scholar] [CrossRef]
- Wu, F.; McLaurin, A.W.; Henson, K.E.; Managhan, D.G.; Thomasson, S.L. The effects of the process parameters on the electrical and microstructure characteristics of the CrSi thin resistor films: Part I. Thin Solid. Films 1998, 332, 418–422. [Google Scholar] [CrossRef]
- Mott, N. Mechanisms for the Oxidation of Silicon and the Formation of Charged Defects. Proc. R. Soc. Lon. Ser.-A 1981, 376, 207–215. [Google Scholar] [CrossRef]
- Fang, Y.K.; Hsu, S.L. Observations on the Phase-Transformation and Its Effect on the Resistivity of Wsi2 Films Prepared by Low-Pressure Chemical Vapor-Deposition. J. Appl. Phys. 1985, 57, 2980–2982. [Google Scholar] [CrossRef]
- Ghandhi, S.K.; Field, R.J.; Shealy, J.R. Highly Oriented Zinc-Oxide Films Grown by the Oxidation of Diethylzinc. Appl. Phys. Lett. 1980, 37, 449–451. [Google Scholar] [CrossRef]
- Mol, H.A.; Sarro, P.M.; Schellevis, H.; Hou, Y. Process for low temperature deposition of Strain Gauge materials based on Chromium Nitride Thin Films. In Proceedings of the ENSORS, 2011 IEEE, Limerick, Ireland, 28–31 October 2011; pp. 226–229. [Google Scholar]
- Anttila, A.; Koskinen, J.; Lappalainen, R.; Hirvonen, J.P.; Stone, D.; Paszkiet, C. Comparison of diamond-like coatings deposited with c+ and various hydrocarbon ion-beams. Appl. Phys. Lett. 1987, 50, 132–134. [Google Scholar] [CrossRef][Green Version]
- Fitzgerald, E.A.; Xie, Y.H.; Green, M.L.; Brasen, D.; Kortan, A.R.; Michel, J.; Mii, Y.J.; Weir, B.E. Totally Relaxed Gexsi1-X Layers with Low Threading Dislocation Densities Grown on Si Substrates. Appl. Phys. Lett. 1991, 59, 811–813. [Google Scholar] [CrossRef]
- Wang, F.; Chen, J.; Wang, X.; Chang, X.; Ran, M.; Yang, Y.; Yang, W. Preparation technology of CrSi thin film resistor with low temperature coefficient. Micronanoelectron. Technol. 2017, 54, 355–359. [Google Scholar] [CrossRef]
- Cuong, N.D.; Kim, D.J.; Kang, B.D.; Yoon, S.G. Effects of nitrogen concentration on structural and electrical properties of titanium nitride for thin-film resistor applications. Electrochem. Solid. St. 2006, 9, G279–G281. [Google Scholar] [CrossRef]
- Stöber, L.; Konrath, J.P.; Krivec, S.; Patocka, F.; Schwarz, S.; Bittner, A.; Schneider, M.; Schmid, U. Impact of sputter deposition parameters on molybdenum nitride thin film properties. J. Micromech. Microeng. 2015, 25, 074001. [Google Scholar] [CrossRef]
- Vanden, N.; Heinrich, A.; Klostermann, K.; Sobe, G. Ir Absorption in Crsio Thin-Films. Phys. Status Solidi A 1986, 93, 163–170. [Google Scholar] [CrossRef]
- Sonoda, K.; Shiraishi, N.; Maekawa, K.; Ito, N.; Hasegawa, E.; Ogata, T. Modeling electrical resistivity of CrSi thin films. Solid. State Electron. 2022, 198, 108471. [Google Scholar] [CrossRef]
- van den Broek, J.J.; Donkers, J.J.T.M.; van der Rijt, R.A.F.; Janssen, J.T.M. Metal film precision resistors: Resistive metal films and a new resistor concept. Philips J. Res. 1998, 51, 429–447. [Google Scholar] [CrossRef]
- Lai, L.F.; Zeng, W.J.; Fu, X.Z.; Sun, R.; Du, R.X. Annealing effect on the electrical properties and microstructure of embedded Ni-Cr thin film resistor. J. Alloy Compd. 2012, 538, 125–130. [Google Scholar] [CrossRef]
- Brynsvold, R.R.; Manning, K. Constant-current stressing of SiCr-based thin-film resistors: Initial “Wearout” investigation. IEEE Trans. Device Mater. Reliab. 2007, 7, 259–269. [Google Scholar] [CrossRef]
- Li, Y.; Huiskamp, P. A novel degradation mechanism in SiCr-O based thin film resistors under temperature and current stress. In Proceedings of the 2012 IEEE International Reliability Physics Symposium (IRPS), Anaheim, CA, USA, 15–19 April 2012. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, F.; Zhang, L.; Wu, B.; Deng, Y.; Xu, K. Recent Status and Prospects of Low-Temperature Drift Resistors. Electronics 2024, 13, 4197. https://doi.org/10.3390/electronics13214197
Liu F, Zhang L, Wu B, Deng Y, Xu K. Recent Status and Prospects of Low-Temperature Drift Resistors. Electronics. 2024; 13(21):4197. https://doi.org/10.3390/electronics13214197
Chicago/Turabian StyleLiu, Fang, Lei Zhang, Bo Wu, Yongfeng Deng, and Kai Xu. 2024. "Recent Status and Prospects of Low-Temperature Drift Resistors" Electronics 13, no. 21: 4197. https://doi.org/10.3390/electronics13214197
APA StyleLiu, F., Zhang, L., Wu, B., Deng, Y., & Xu, K. (2024). Recent Status and Prospects of Low-Temperature Drift Resistors. Electronics, 13(21), 4197. https://doi.org/10.3390/electronics13214197





