Safety Assessment for Full Flight between Beidou Radio Determination Satellite Service Airborne Equipment and 5G System
Abstract
1. Introduction
2. System Models
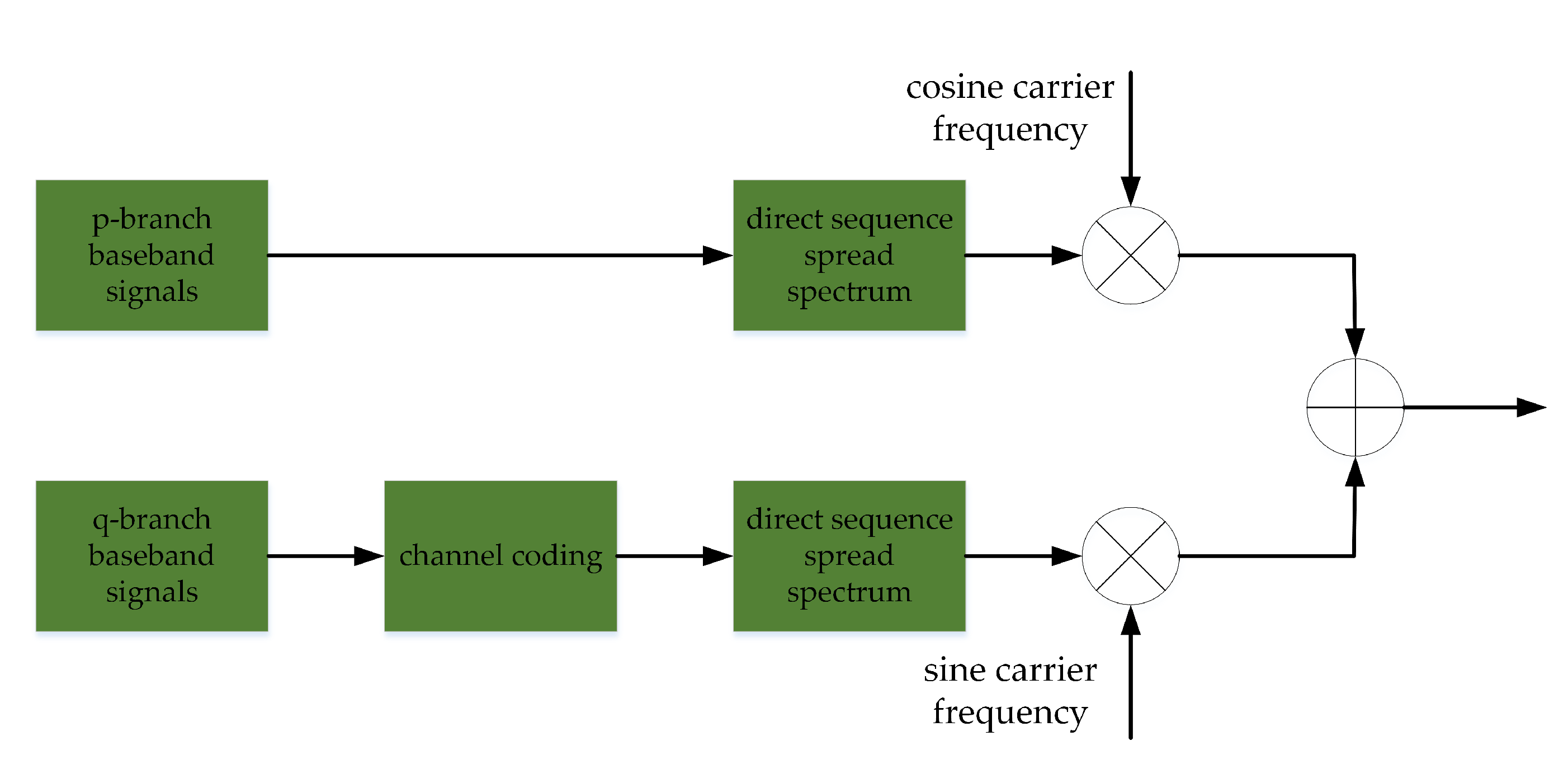
2.1. RDSS Signal Model
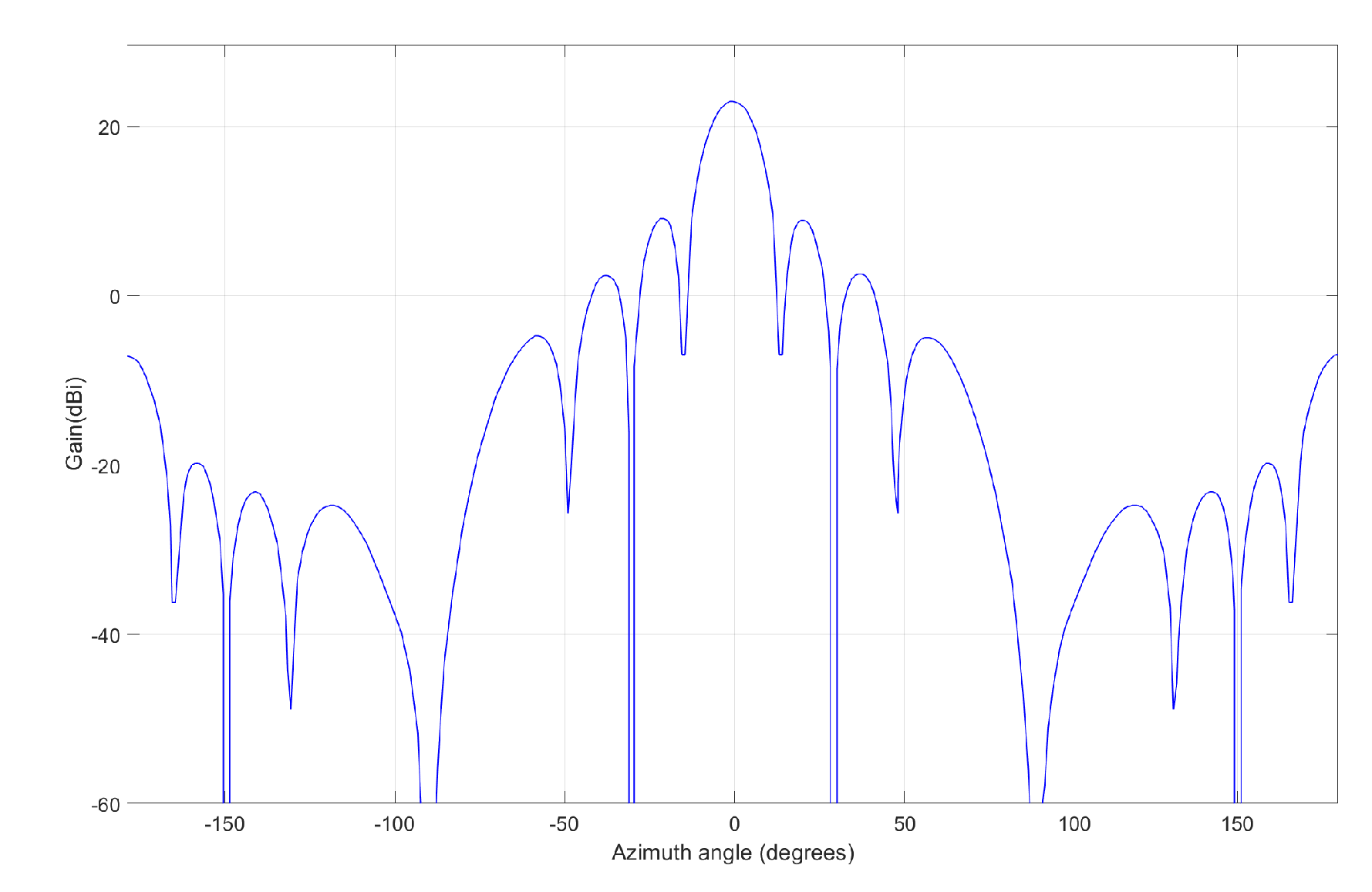
2.2. 5G Antenna Model
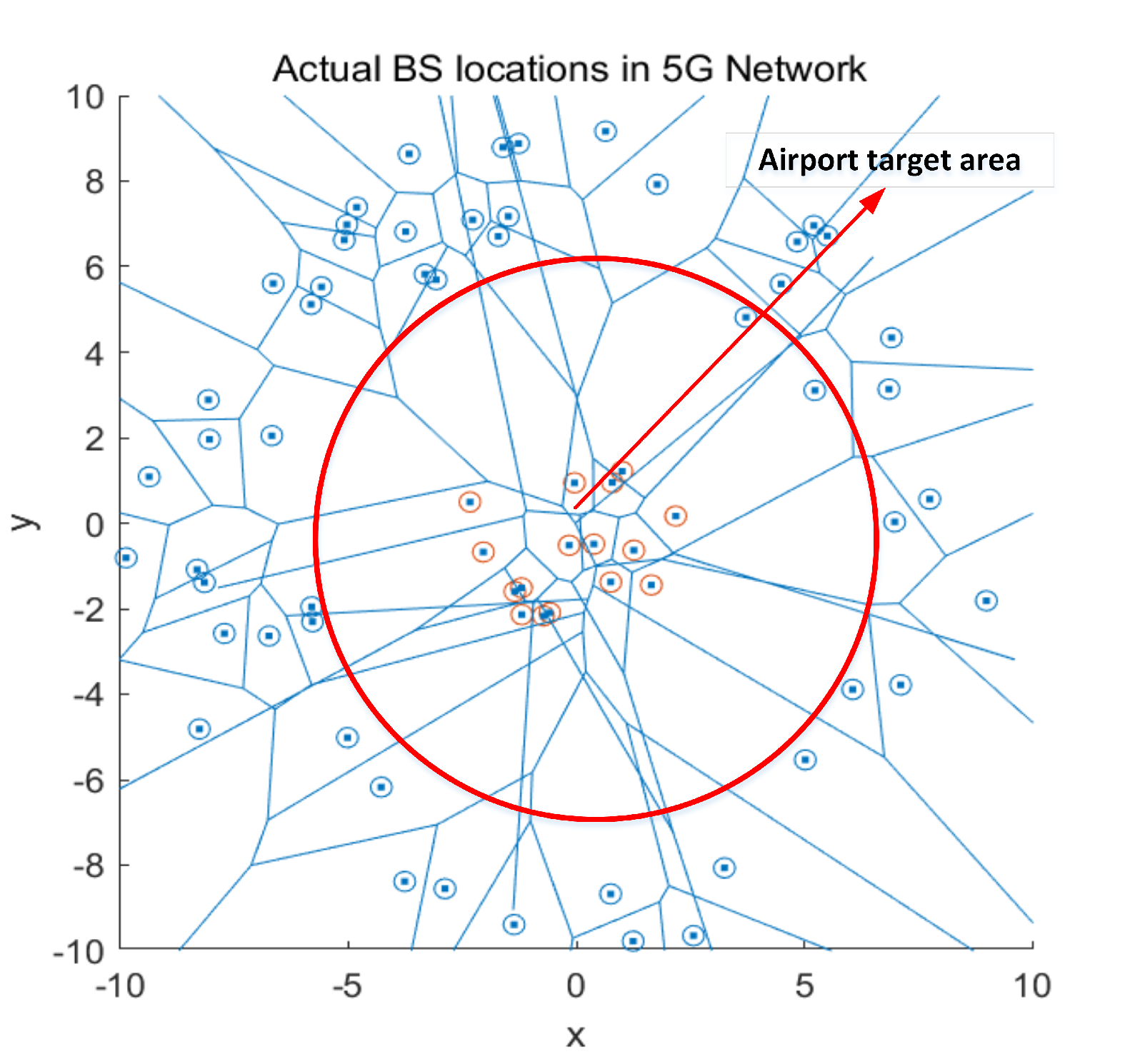
2.3. Base Station Distribution Modelling
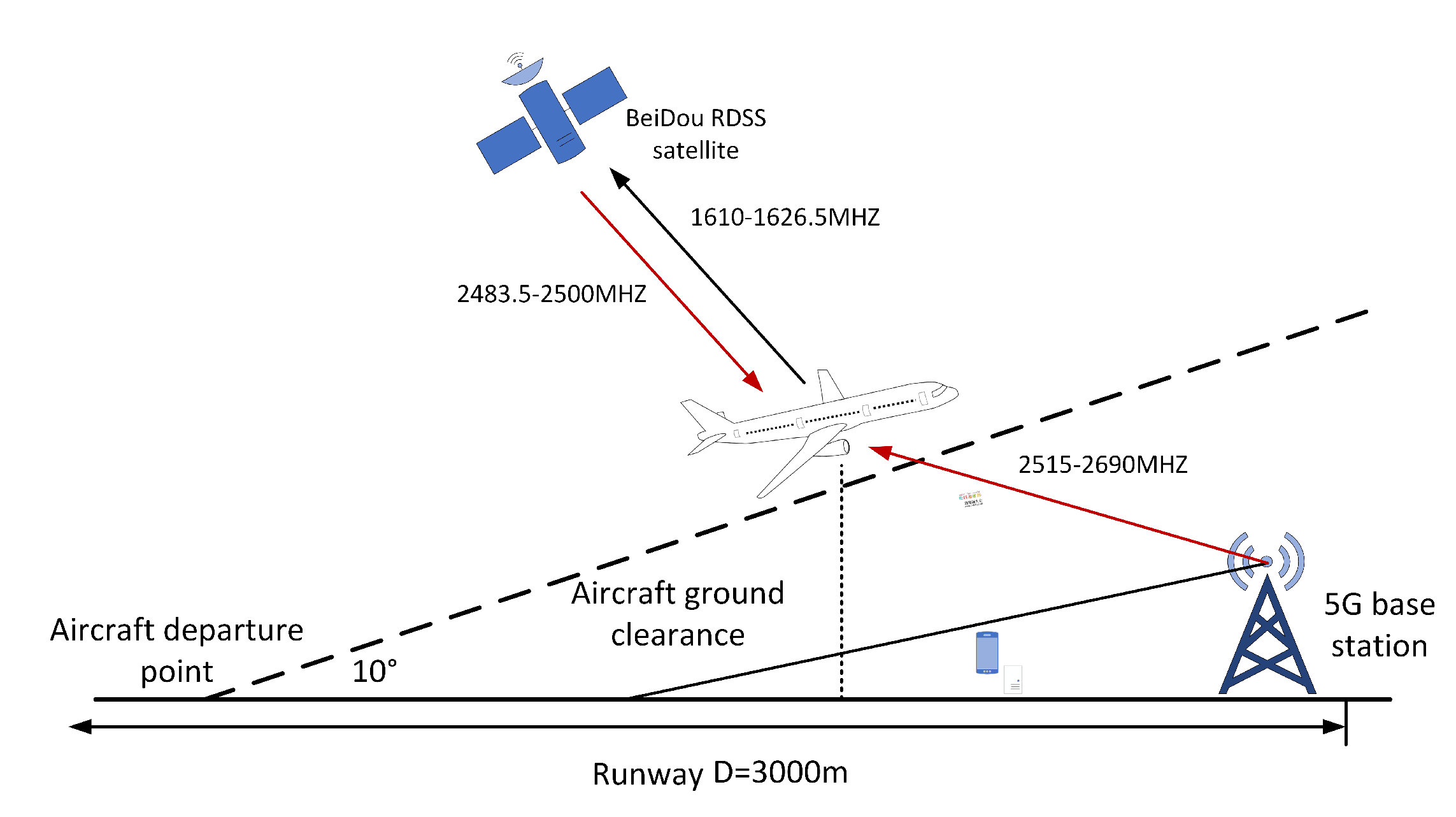
3. Takeoff and Landing Phase Assessment
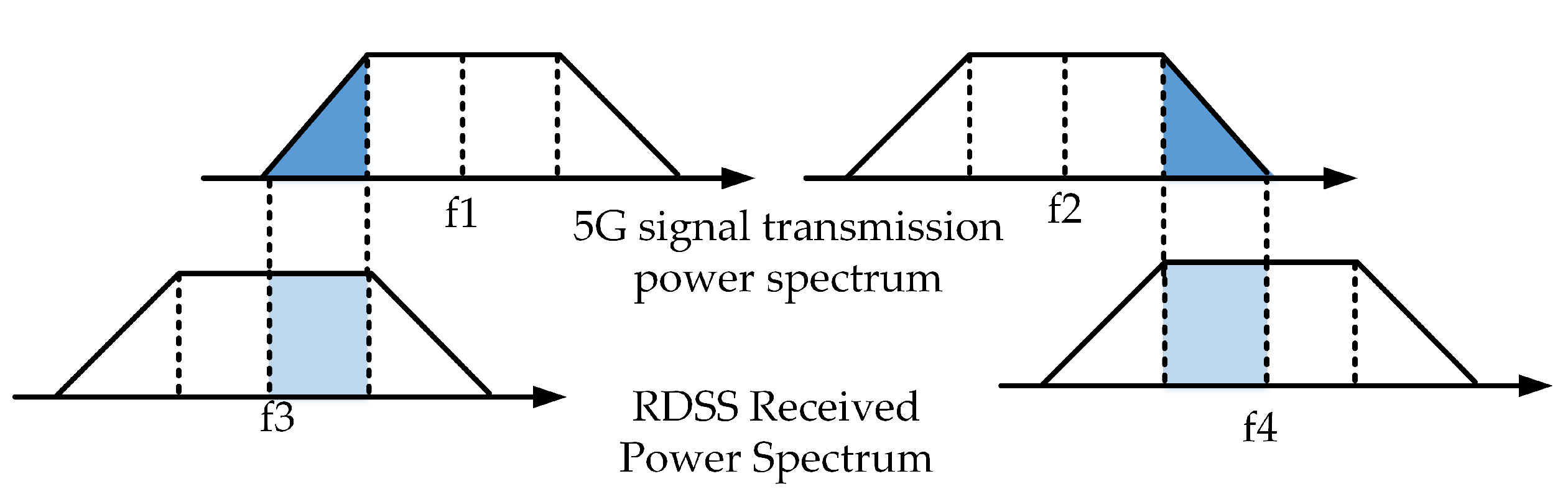
3.1. Interference Scene Building
3.2. Interference Assessment Methods
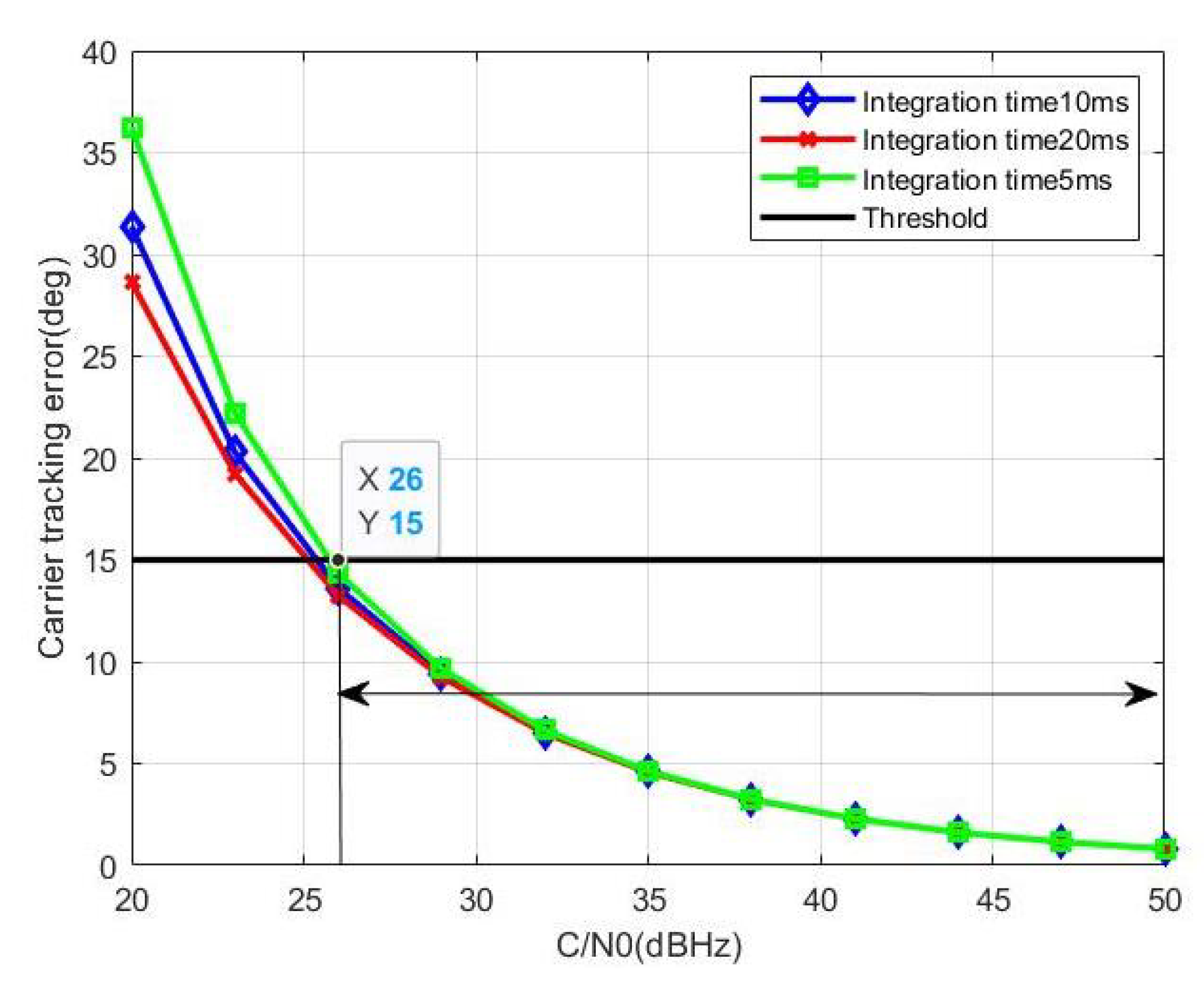
3.3. Simulation Results and Analysis
4. Cruise Phase Assessment
4.1. Interference Scene Building
4.2. Interference Assessment Methods
4.2.1. Fuselage Modelling
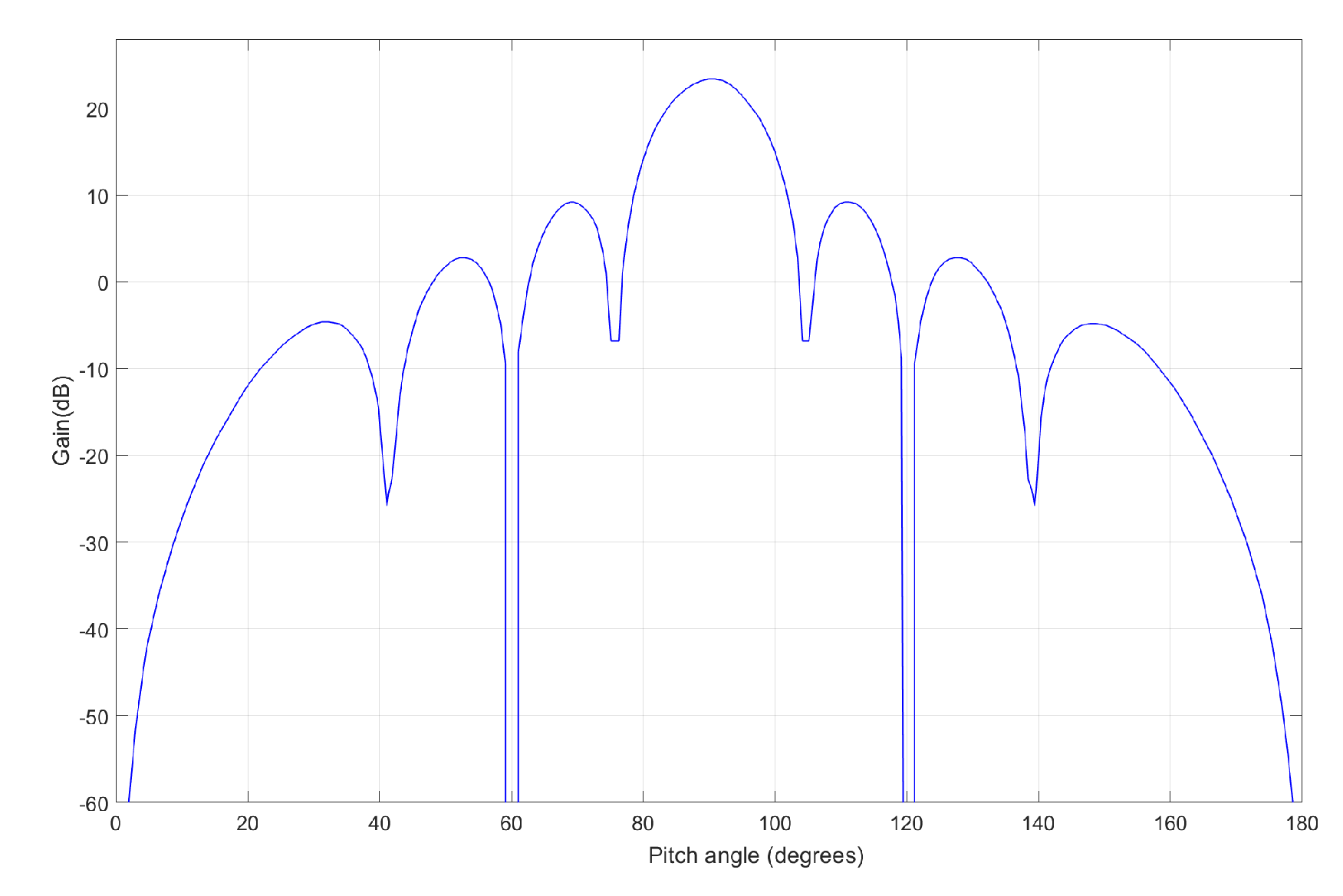
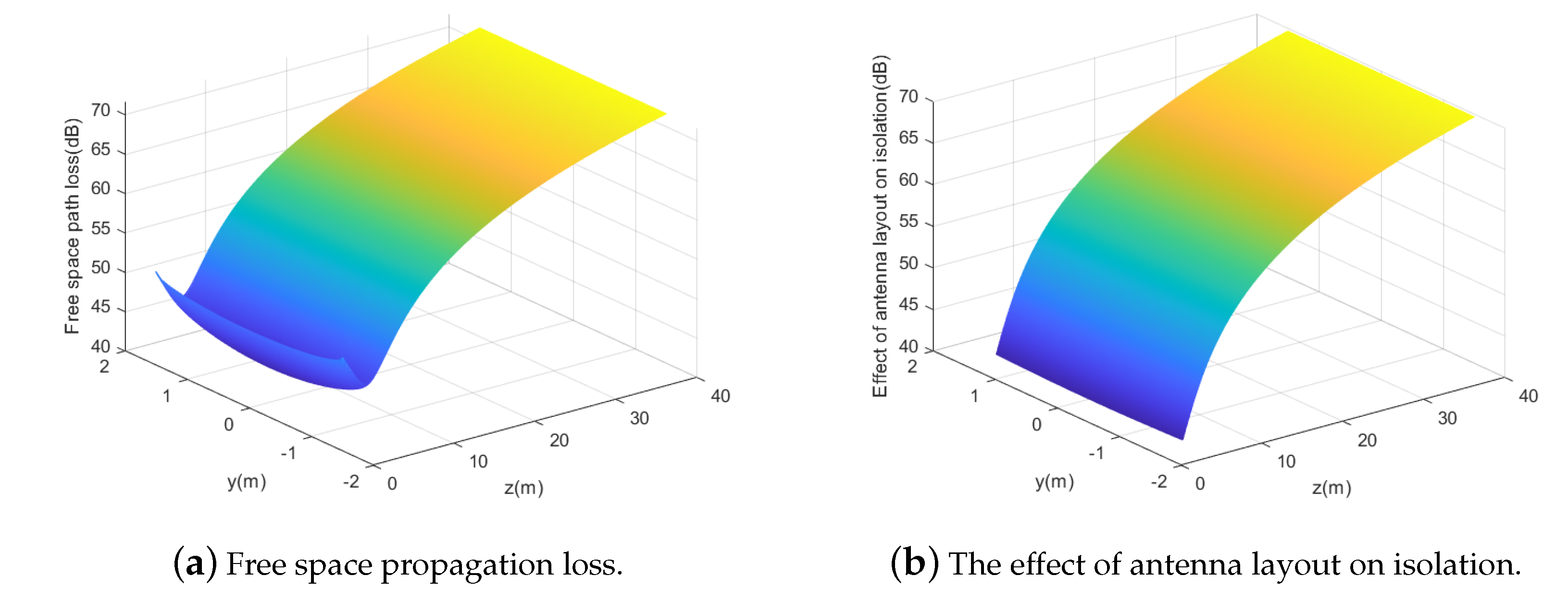
4.2.2. Airborne Antenna Isolation Calculation
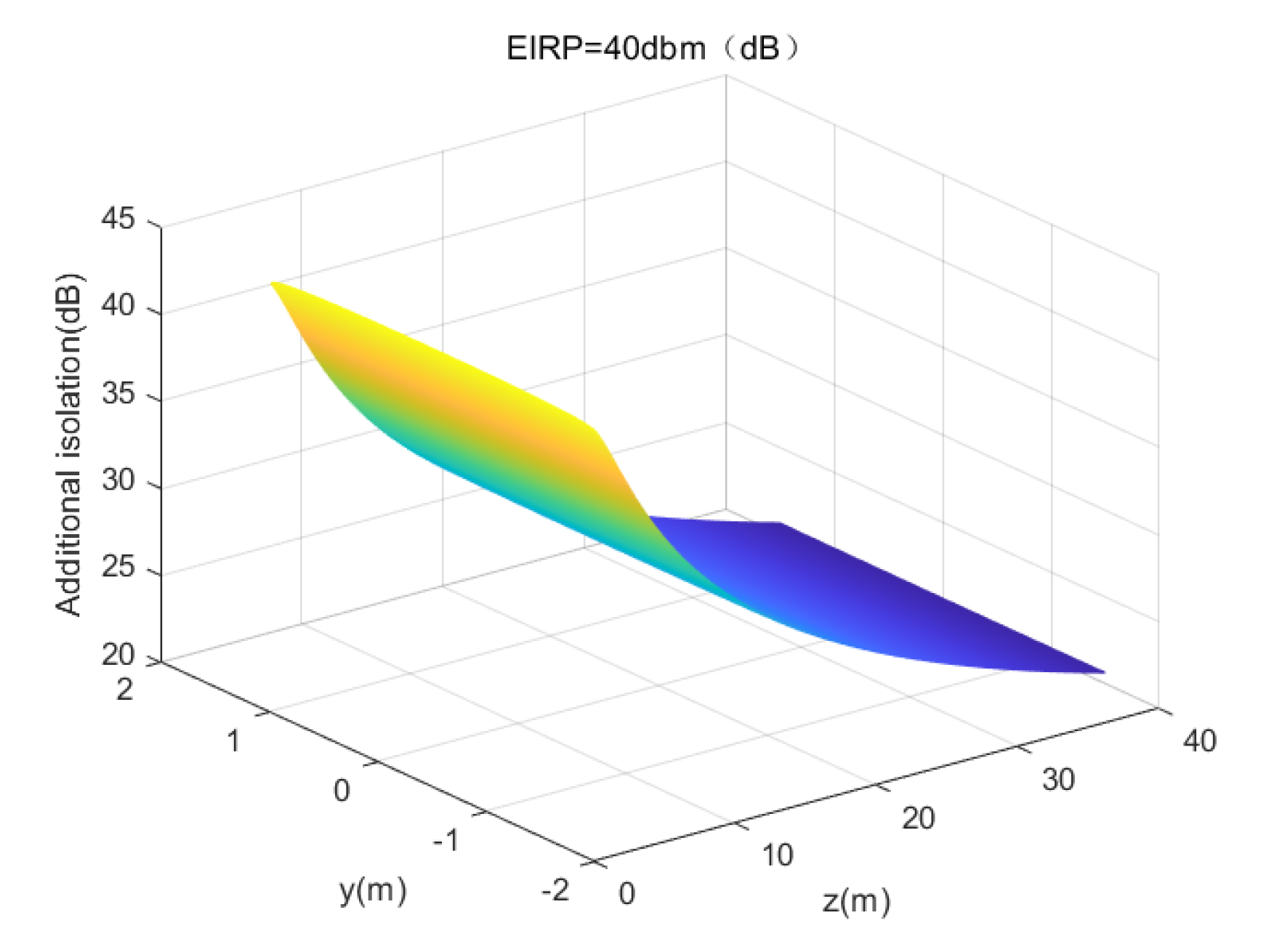
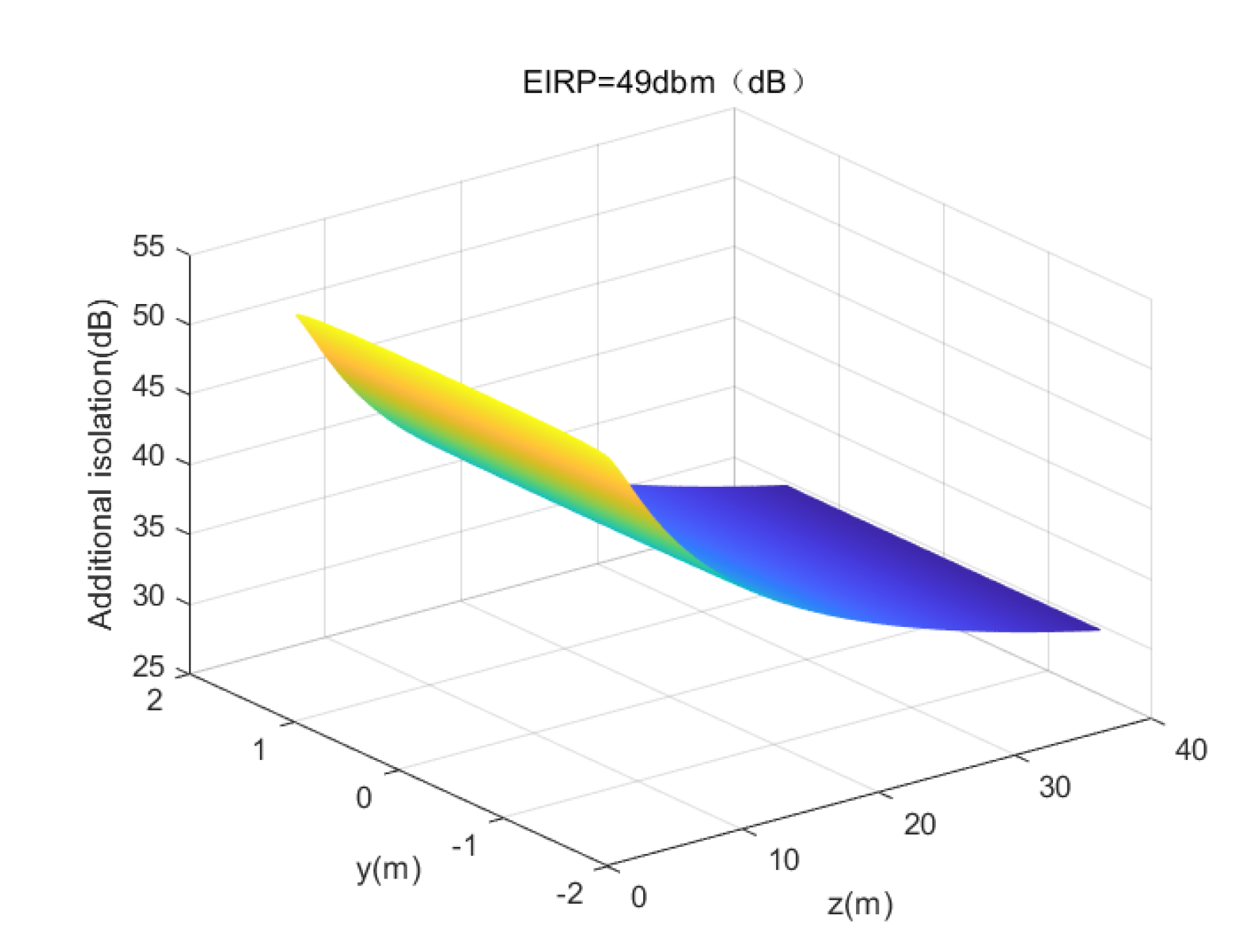
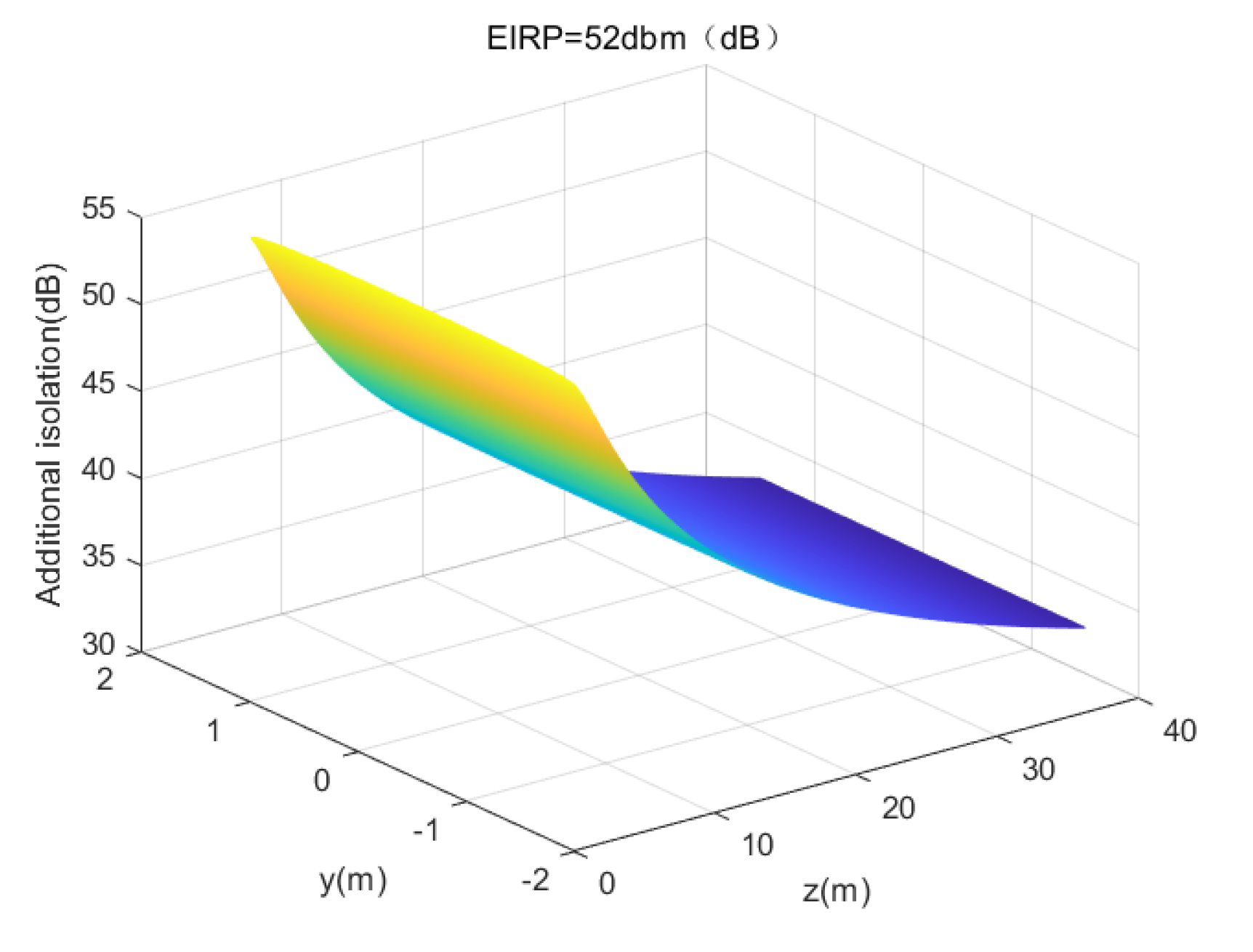
4.3. Simulation Results and Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sun, F.; Liu, S.; Zhu, X.; Men, B. Research and progress of Beidou satellite navigation system. Sci. China Inf. Sci. 2012, 55, 2899–2907. [Google Scholar] [CrossRef]
- Sun, Y.; Xue, R.; Zhao, D.; Wang, D. Radio frequency compatibility evaluation of S band navigation signals for future BeiDou. Sensors 2017, 17, 1039. [Google Scholar] [CrossRef] [PubMed]
- Ren, H.; Tang, C.; Guo, R.; Zhang, T.; Xing, N.; Hu, X.; Li, X. Precision analysis of BDS-3 GEO satellite orbit determination using RDSS. Sci. Sin. Phys. Mech. Astron. 2021, 51, 019510. [Google Scholar]
- 3GPP TS 38.104; 3rd Generation Partnership Project; Technical Specification Group Radio Access Network NR Base Station (BS) radio transmission and reception (Release 16). 3GPP: Sophia Antipolis, France, 2020.
- Jessner, A. Industrial interference and radio astronomy. Adv. Radio Sci. 2013, 11, 251–258. [Google Scholar] [CrossRef]
- Tong, W.; Zou, D.; Han, T.; Zhang, X.; Shen, P.; Lu, X.; Wang, P.; Yin, T. A New Type of 5G-Oriented Integrated BDS/SON High-Precision Positioning. Remote Sens. 2021, 13, 4261. [Google Scholar] [CrossRef]
- RTCA. Assessment of the Lightsquared Ancillary Terrestrial Component Radio Frequency Interference Impact on GNSS L1 Band Airborne Receiver Operations; RTCA: Washington, DC, USA, 2011. [Google Scholar]
- O’Driscoll, C.; Rao, M.; Borio, D.; Cano, E.; Fortuny, J.; Bastide, F.; Hayes, D. Compatibility analysis between LightSquared signals and L1/E1 GNSS reception. In Proceedings of the 2012 IEEE/ION Position, Location and Navigation Symposium, Myrtle Beach, NC, USA, 23–26 April 2012; pp. 447–454. [Google Scholar]
- Godet, J. GPS/GALILEO radio frequency compatibility analysis. In Proceedings of the 13th International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GPS 2000), Salt Lake City, UT, USA, 22–25 September 2000; pp. 1782–1790. [Google Scholar]
- Rao, M.; O’Driscoll, C.; Borio, D. Light Squared effects on estimated C/N0 pseudoranges and positions. GPS Solut. 2014, 18, 1–13. [Google Scholar]
- Zhang, X. Research on the performance testing of Beidou RDSS navigational surveillance service. J. Civ. Aviat. Univ. China 2017, 35, 1–5. [Google Scholar]
- Wang, F. Analysis of 4G interference to BeiDou RDSS system and countermeasure strategies. Glob. Position. Syst. 2019, 44, 76–81. [Google Scholar] [CrossRef]
- Han, X. A tractable approach to coexistence analysis between COMPASS and TD-LTE system. In Proceedings of the 2014 21st International Conference on Telecommunications (ICT), Lisbon, Portugal, 4–7 May 2014; pp. 77–81. [Google Scholar] [CrossRef]
- An, N.; Wang, C.; Wang, W. Interference Coexistence of 5G NR and LTE System Based on 2.1GHz. In Proceedings of the 2020 12th International Conference on Communication Software and Networks (ICCSN), Chongqing, China, 12–15 June 2020; pp. 90–94. [Google Scholar] [CrossRef]
- Zhang, T.; Ge, X.; Liu, Z.; Zhang, W. Analysis and countermeasures of Beidou S signal affected by ground 5G interference. Radio Eng. 2021, 51, 1037–1041. [Google Scholar]
- Wang, Q.; Cheng, F.; Zhang, T. 5G Impacts analysis on the Beidou RDSS system in 2.5 GHz band. GPS Solut. 2023, 27, 24. [Google Scholar] [CrossRef]
- Wang, D.; Guo, R.; Xing, N. Performance analysis of two RDSS positioning modes of BeiDou-3 system. Astrodynamics 2022, 6, 317–327. [Google Scholar] [CrossRef]
- Jing, P.; Zhang, Y.; Zhan, J.; Ou, G. Research on key techniques of the Beidou RDSS receiver test system. J. Astronaut. Metrol. Meas. 2016, 36, 95. [Google Scholar]
- Won, J.H.; Eissfeller, B.; Schmitz-Peiffer, A.; Floch, J.J.; Zanier, F.; Colzi, E. Trade-off between data rate and signal power split in GNSS signal design. IEEE Trans. Aerosp. Electron. Syst. 2012, 48, 2260–2281. [Google Scholar] [CrossRef]
- Kaplan, E.D.; Hegarty, C. Understanding GPS: Principles and Applications, 2nd ed.; Artech House: Norwood, MA, USA, 2006. [Google Scholar]
- 3GPP TR 37.842; Radio Frequency (RF) Requirement Back-Ground for Active Antenna System (AAS) Base Station (BS).V13.2.0. 3GPP: Sophia Antipolis, France, 2017.
- Gkonis, P.K.; Trakadas, P.T.; Kaklamani, D.I. A comprehensive study on simulation techniques for 5G networks: State of the art results, analysis, and future challenges. Electronics 2020, 9, 468. [Google Scholar]
- Dangi, R.; Lalwani, P.; Choudhary, G.; You, I.; Pau, G. Study and investigation on 5G technology: A systematic review. Sensors 2021, 22, 26. [Google Scholar] [PubMed]
- ITU-R M.2101; Modelling and Simulation of IMT Networks for Use in SHARING and compatibility Studies. ITU-R: Geneva, Switzerland, 2017.
- Andrews, J.G.; Baccelli, F.; Ganti, R.K. A tractable approach to coverage and rate in cellular networks. IEEE Trans. Commun. 2011, 59, 3122–3134. [Google Scholar]
- ITU-R. Report M.2412; Guidelines for Evaluation of Radio Interface Technologies for IMT-2020. ITU-R: Geneva, Switzerland, 2017.
- 3GPP TR 36.942; Radio Frequency (RF) System Scenarios. V15.0.0. 3GPP: Sophia Antipolis, France, 2018.
- BD420007; Performance Requirements and Test Methods for RDSS Units of Beidou User Terminals. NSTDA: Beijing, China, 2015.
- Lei, L.; Hou, J. Analysis of interference of 5G system to ATG system in 3.5 GHz. J. Radio Wave Sci. 2021, 36, 477–482. [Google Scholar]
- Liu, L.; Wang, B.; Kadoch, M. Analysis of Interference from ATG Uplink to Terrestrial 5G Base Stations in 2.1 GHz Band. In Proceedings of the 2022 International Conference on Information Processing and Network Provisioning (ICIPNP), Beijing, China, 20–21 October 2022; pp. 43–46. [Google Scholar] [CrossRef]
- Zhang, S.W.; Hou, J.J.; Wang, J. Research on the form and capacity of 5G-based ATG networking. Mob. Communition 2020, 44, 1–6. [Google Scholar]
- Li, H.; Bolsover, M.; Ye, J.; Yan, L. The Role of Electromagnetic Compatibility Qualification Considerations in Airborne System Integration Programs. In Proceedings of the 2014 Asia-Pacific International Symposium on Aerospace Technology, APISAT2014, Shanghai, China, 24–26 September 2014; Volume 99, pp. 208–213. [Google Scholar] [CrossRef]
- Liu, R.H.; Wang, X. Analysis of compatibility between Beidou radio determination satellite service airborne equipment and iridium airborne earth station. Sci. Technol. Eng. 2021, 21, 4103–4109. [Google Scholar]








| Dissemination Environment | Flight Altitude (m) | Horizontal Distance (m) | Aggregate Interference (dBm) |
|---|---|---|---|
| EDDB−KIAD Free Space | 75.31 | 50.8 | −121.85 |
| 89.62 | 158 | −127.87 | |
| 103.93 | 251 | −131.39 | |
| KJFK−KLAX Free Space | 27.5 | 84.6 | −119.67 |
| 47 | 171.6 | −125.69 | |
| 66.50 | 258.5 | −129.21 | |
| EDDB−KIAD RMA | 75.31 | 50.8 | −126.60 |
| 89.62 | 158 | −141.03 | |
| 103.93 | 251 | −149.47 | |
| KJFK−KLAX RMA | 27.5 | 84.6 | −126.17 |
| 47 | 171.6 | −140.60 | |
| 66.50 | 258.5 | −149.04 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, W.; Tian, Y.; Sun, S.; Liu, R. Safety Assessment for Full Flight between Beidou Radio Determination Satellite Service Airborne Equipment and 5G System. Electronics 2023, 12, 3350. https://doi.org/10.3390/electronics12153350
Chen W, Tian Y, Sun S, Liu R. Safety Assessment for Full Flight between Beidou Radio Determination Satellite Service Airborne Equipment and 5G System. Electronics. 2023; 12(15):3350. https://doi.org/10.3390/electronics12153350
Chicago/Turabian StyleChen, Wantong, Yuyin Tian, Shuguang Sun, and Ruihua Liu. 2023. "Safety Assessment for Full Flight between Beidou Radio Determination Satellite Service Airborne Equipment and 5G System" Electronics 12, no. 15: 3350. https://doi.org/10.3390/electronics12153350
APA StyleChen, W., Tian, Y., Sun, S., & Liu, R. (2023). Safety Assessment for Full Flight between Beidou Radio Determination Satellite Service Airborne Equipment and 5G System. Electronics, 12(15), 3350. https://doi.org/10.3390/electronics12153350






