Model-Based Estimation of Transmission Gear Ratio for Driving Energy Consumption of an EV
Abstract
:1. Introduction
2. Mathematical Models
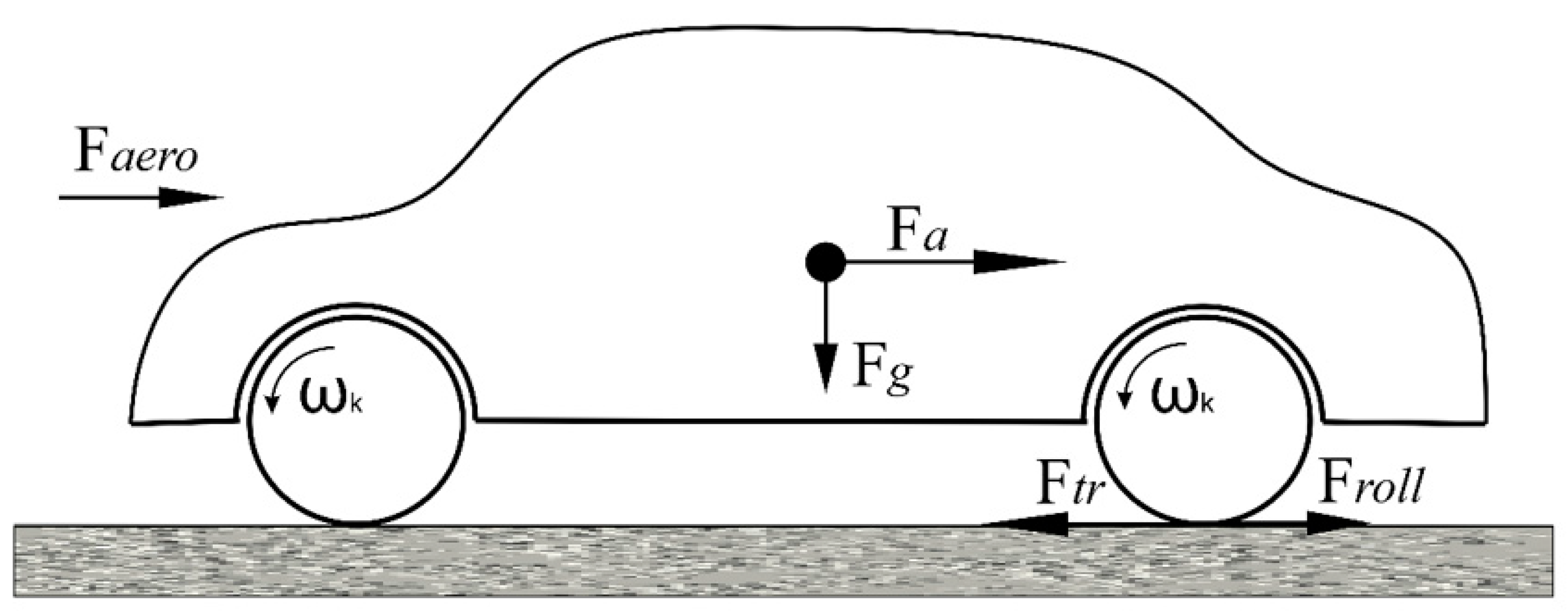
2.1. Vehicle Dynamics
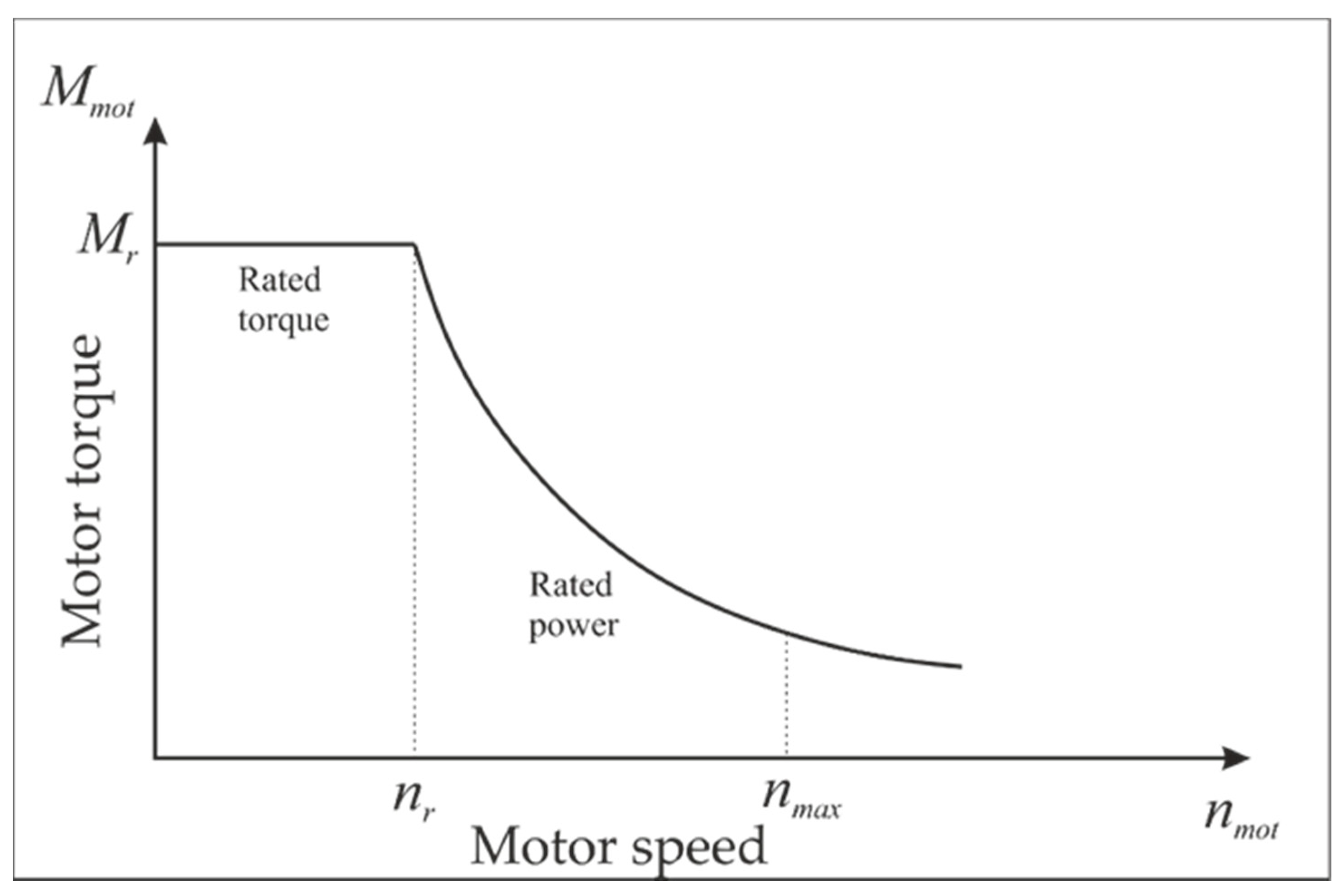
2.2. Traction Motor Model
2.3. Simulation Model
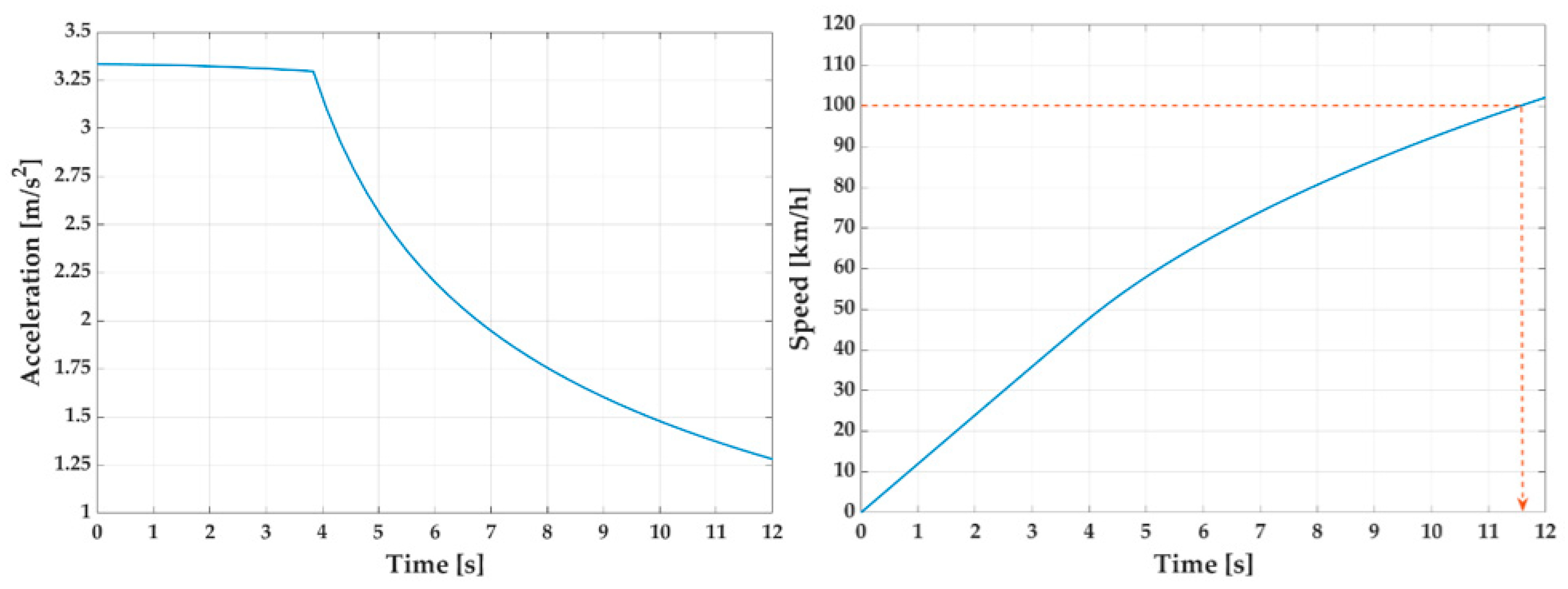
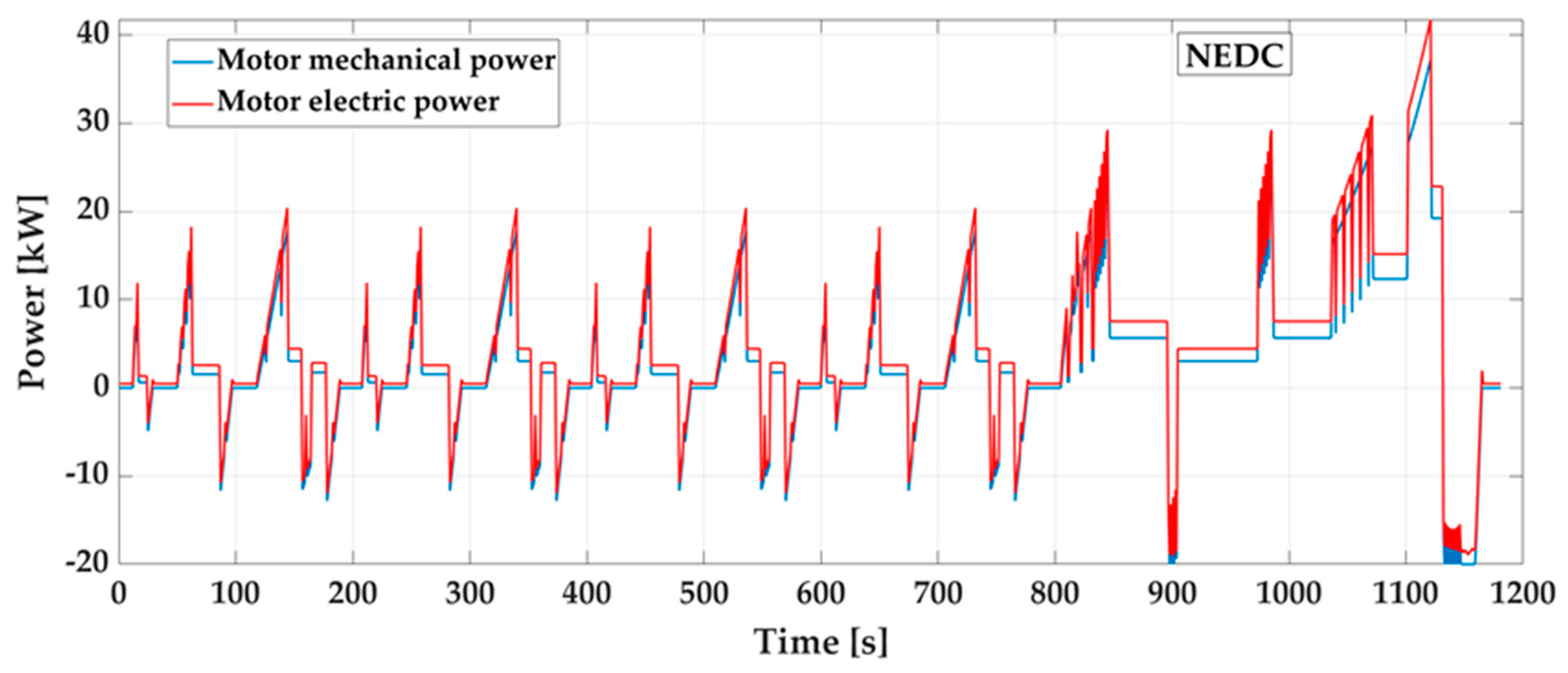
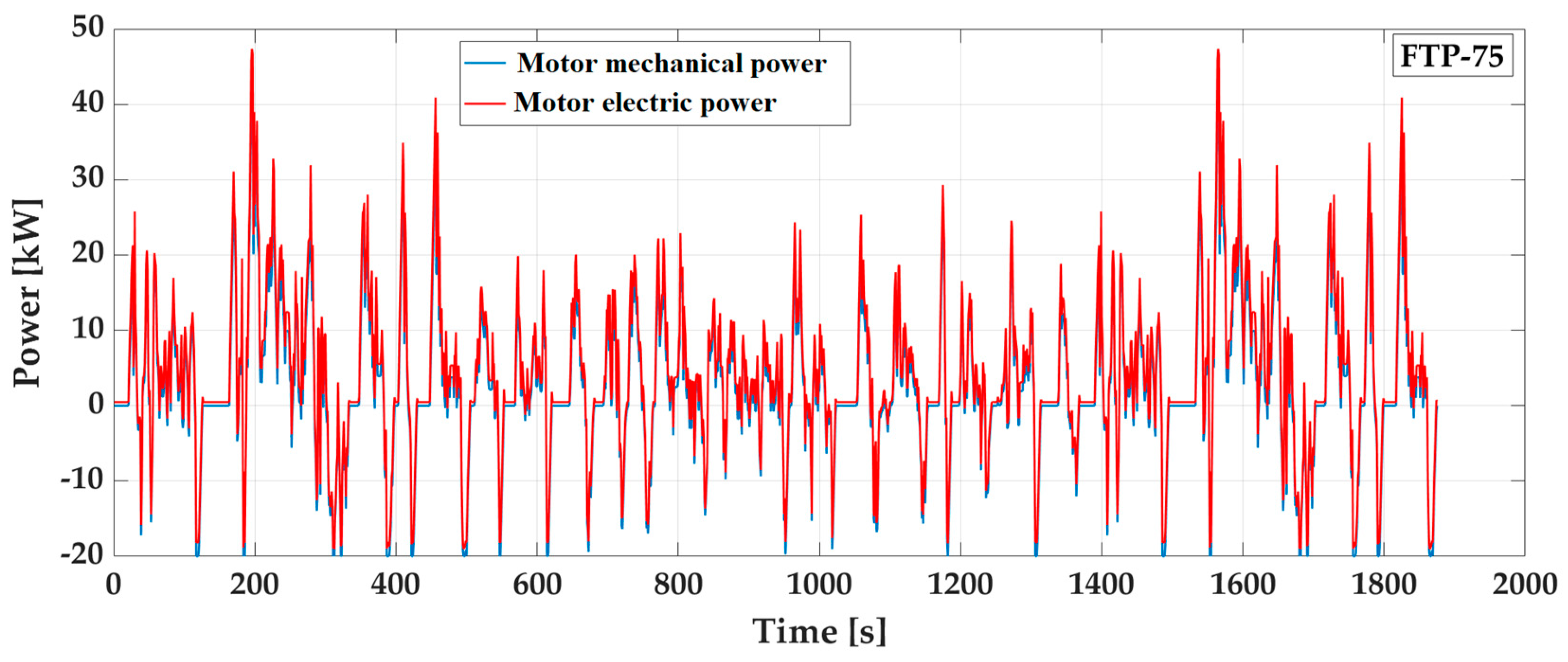
3. Model Validation
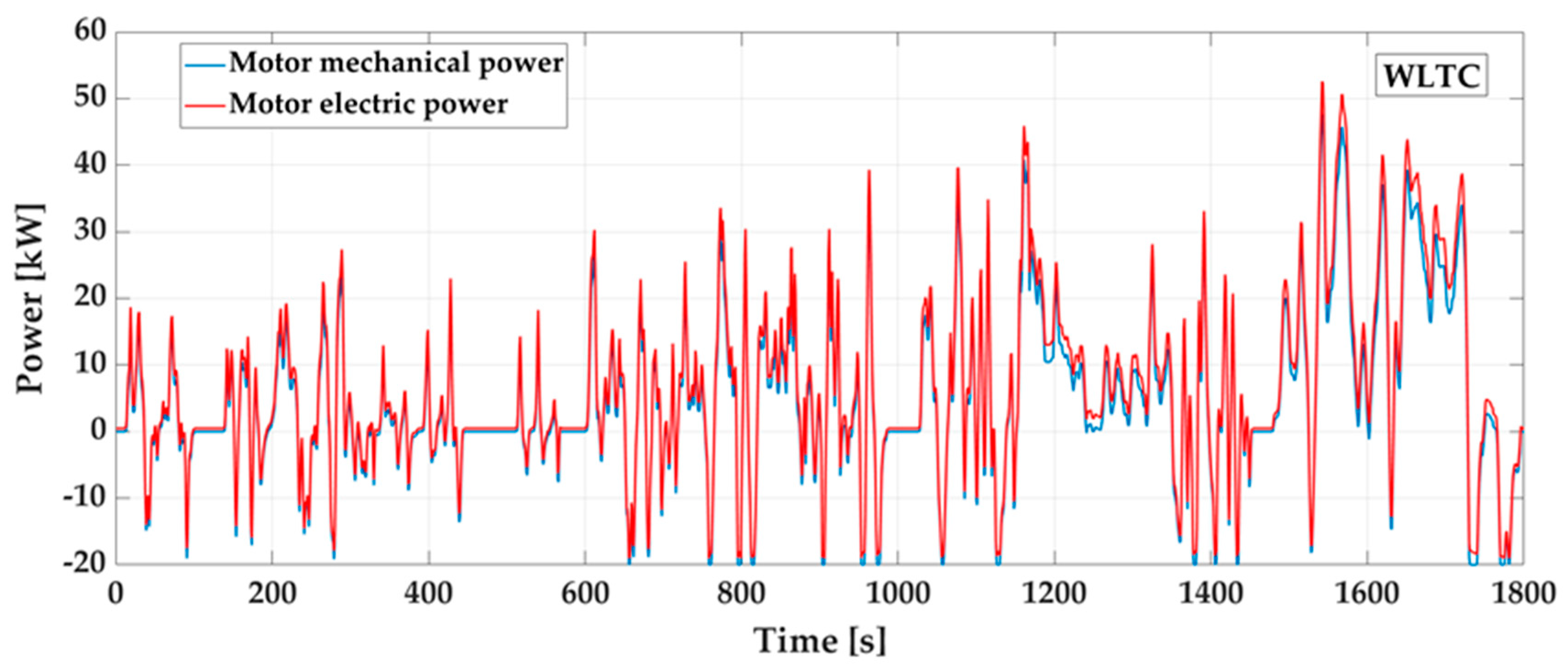
4. Simulation Results
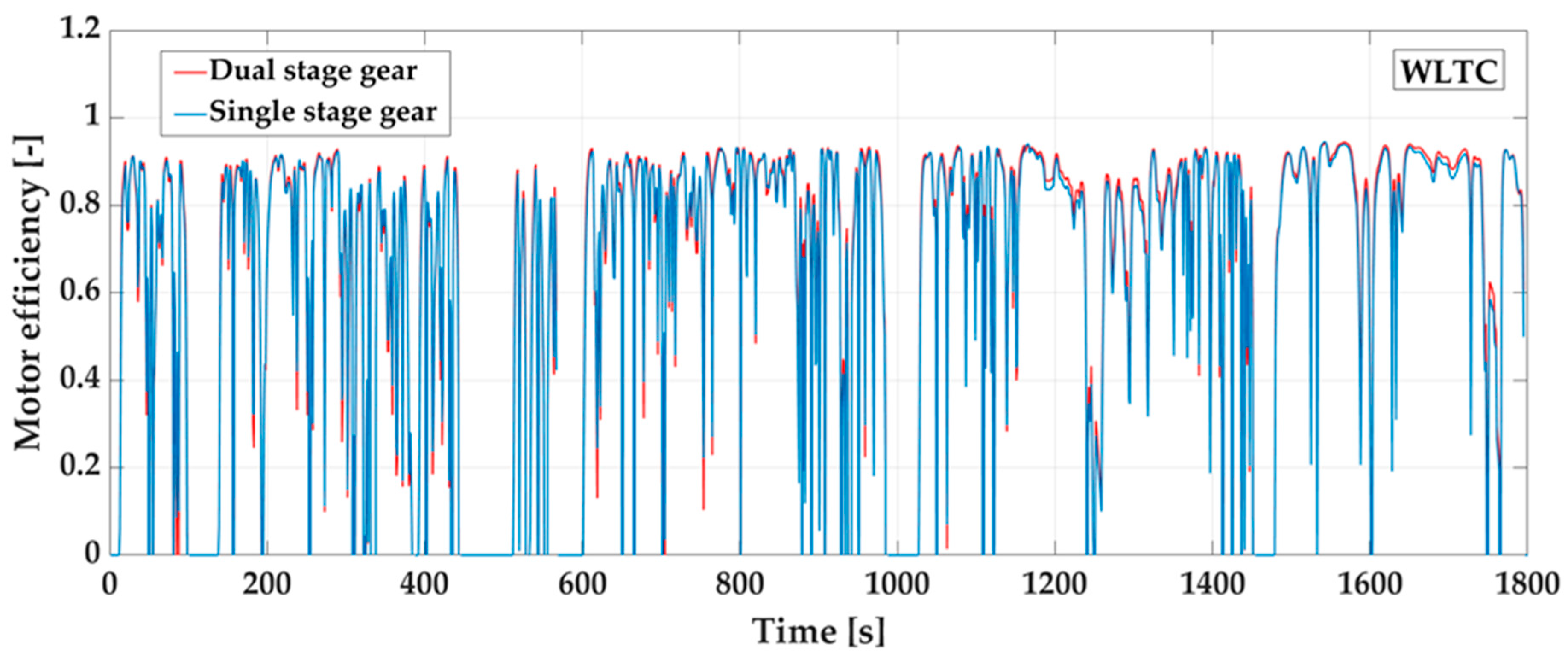
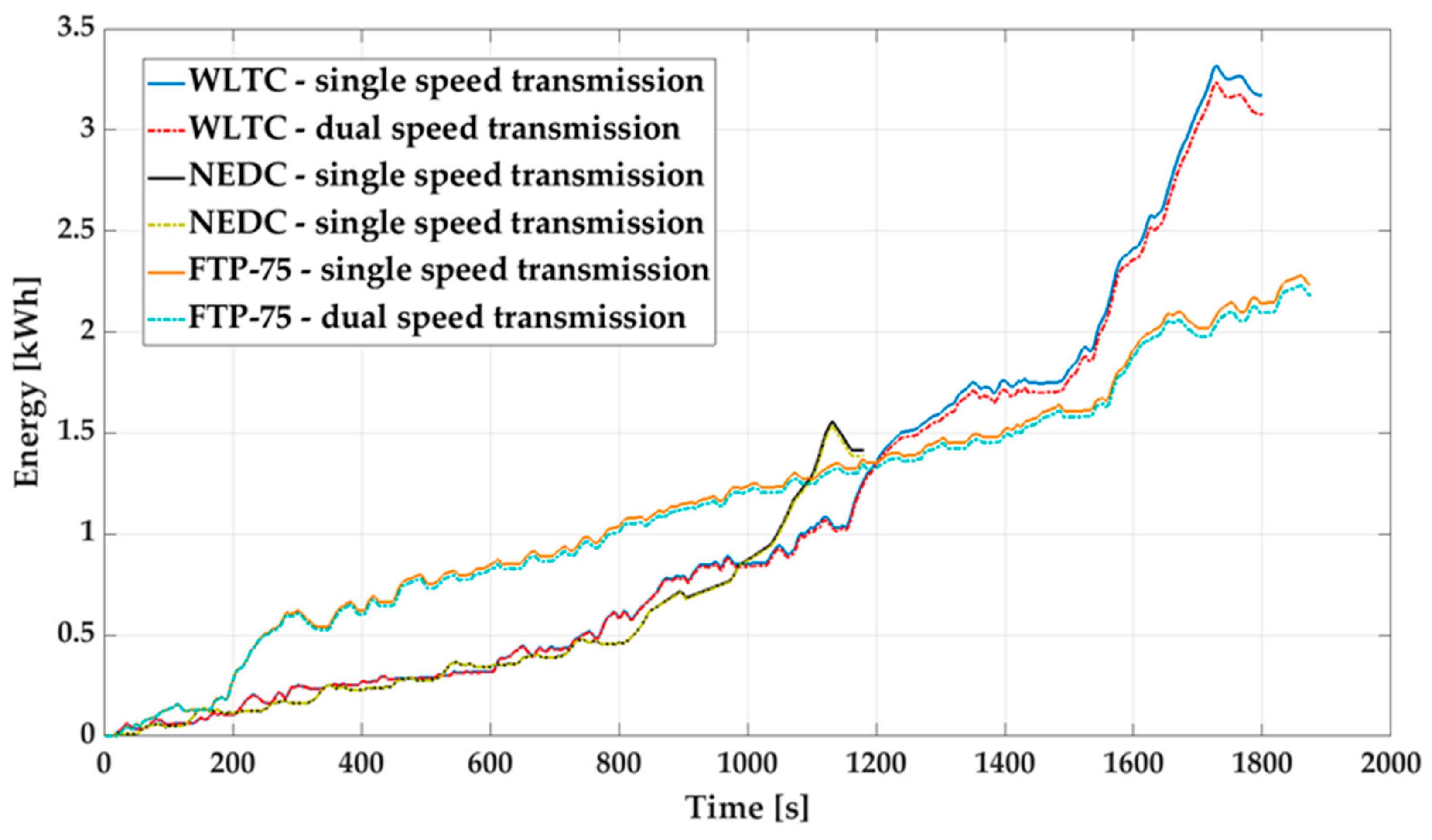
5. Numerical Study on Energy Consumption
5.1. Transmission Configuration and Gear Ratio
5.2. Vehicle Power Demand
5.3. Vehicle Energy Consumption
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Longitudinal vehicle acceleration | |
| ICE | internal combustion engine |
| HEV | hybrid electric vehicle |
| BEV | battery electric vehicle |
| EV | electric vehicle |
| traction force | |
| aerodynamic force | |
| rolling resistance | |
| equivalent vehicle mass | |
| traction motor output torque | |
| transmission ratio | |
| mechanical efficiency | |
| wheel dynamic radius | |
| maximum traction force | |
| tire friction coefficient | |
| vertical load on the driving axle | |
| drag coefficient | |
| vehicle frontal area | |
| air density | |
| V | vehicle speed |
| rolling friction coefficients | |
| rolling speed coefficient | |
| vehicle mass | |
| traction power | |
| driving energy | |
| power loss | |
| rated torque | |
| maximum speed of the motor | |
| electric power | |
| energy consumption | |
| motor speed | |
| mass correction coefficient |
References
- Zainuri, F.; Sumarsono, D.A.; Adhitya, M.; Siregar, R.; Prasetya, S.; Heryana, G.; Subarkah, R. Performance Analysis of Power Train Electric Vehicle Transmission Two Speed with Reverse Engineering Method. J. Mech. Eng. Res. Dev. 2020, 43, 417–427. [Google Scholar]
- Zainuri, F.; Sumarsono, D.A.; Adhitya, M.; Siregar, R. Design of synchromesh mechanism to optimization manual transmission’s electric vehicle. AIP Conf. Proc. 2017, 1823, 020031. [Google Scholar] [CrossRef] [Green Version]
- Chen, B.; Zhang, L.; Han, J.; Zhang, Q. A combination of electric supercharger and Miller Cycle in a gasoline engine to improve thermal efficiency without performance degradation. Case Stud. Therm. Eng. 2019, 14, 100429. [Google Scholar] [CrossRef]
- Paykani, A.; Kakaee, A.-H.; Rahnama, P.; Reitz, R.D. Progress and recent trends in reactivity-controlled compression ignition engines. Int. J. Engine Res. 2016, 17, 481–524. [Google Scholar] [CrossRef]
- Taylor, A.M.K.P. Science review of internal combustion engines. Energy Policy 2008, 36, 4657–4667. [Google Scholar] [CrossRef]
- Kaya, T.; Kutlar, O.; Taskiran, O. Evaluation of the Effects of Biodiesel on Emissions and Performance by Comparing the Results of the New European Drive Cycle and Worldwide Harmonized Light Vehicles Test Cycle. Energies 2018, 11, 2814. [Google Scholar] [CrossRef] [Green Version]
- Benajes, J.; García, A.; Monsalve-Serrano, J.; Martínez-Boggio, S. Emissions reduction from passenger cars with RCCI plug-in hybrid electric vehicle technology. Appl. Therm. Eng. 2020, 164, 114430. [Google Scholar] [CrossRef]
- Liu, Y.; Xie, J.; Qin, D.; Zhang, Y.; Chen, Z.; Li, G.; Zhang, Y. Design, Control and Validation of Two-Speed Clutch-less Automatic Transmission for Electric Vehicle. IEEE ASME Trans. Mechatron. 2021. [Google Scholar] [CrossRef]
- Ahssan, M.R.; Gorji, S.A. Electric Vehicle with Multi-Speed Transmission: A Review on Performances and Complexities. SAE Int. J. Altern. Powertrains 2018, 7, 169–182. [Google Scholar] [CrossRef]
- Ritari, A.; Vepsäläinen, J.; Kivekäs, K.; Tammi, K.; Laitinen, H. Energy Consumption and Lifecycle Cost Analysis of Electric City Buses with Multispeed Gearboxes. Energies 2020, 13, 2117. [Google Scholar] [CrossRef]
- Polinder, H.; Bandyopadhyay, S. Energy Consumption of Electric Powertrain Architectures: A Comparative Study. In Proceedings of the 2017 19th European Conference on Power Electronics and Applications (EPE’17 ECCE Europe), Warsaw, Poland, 11–14 September 2017. [Google Scholar] [CrossRef]
- Spanoudakis, P.; Tsourveloudis, N.; Koumartzakis, G. Evaluation of a 2-speed Transmission on Electric Vehicle’s Energy Consumption. In Proceedings of the 2014 IEEE International Electric Vehicle Conference (IEVC), Florence, Italy, 17–19 December 2014. [Google Scholar] [CrossRef]
- Pinto, S.; Camocardi, P.; Chatzikomis, C.; Sorniotti, A.; Bottiglione, F.; Mantriota, G.; Perlo, P. On the Comparison of 2- and 4-Wheel-Drive Electric Vehicle Layouts with Central Motors and Single- and 2-Speed Transmission Systems. Energies 2020, 13, 3328. [Google Scholar] [CrossRef]
- Ahssan, M.R.; Gorji, S.A. Gear Ratio Optimization along with a Novel Gearshift Scheduling Strategy for a Two-Speed Transmission System in Electric Vehicle. Energies 2020, 13, 5073. [Google Scholar] [CrossRef]
- Miri, I.; Fotouhi, A.; Ewin, N. Electric vehicle energy consumption modelling and estimation—A case study. Int. J. Energy Res. 2021, 45, 501–520. [Google Scholar] [CrossRef]
- Zhang, Y.; Shang, Y.; Cui, N.; Zhang, C. Parameters Identification and Sensitive Characteristics Analysis for Lithium-Ion Batteries of Electric Vehicles. Energies 2017, 11, 19. [Google Scholar] [CrossRef] [Green Version]
- Zhang, L.; Mu, Z.; Gao, X. Coupling Analysis and Performance Study of Commercial 18650 Lithium-Ion Batteries under Conditions of Temperature and Vibration. Energies 2018, 11, 2856. [Google Scholar] [CrossRef] [Green Version]
- Hayes, J.G.; Goodarzi, G.A. Electric Powertrain; John Wiley & Sons: Chichester, UK, 2018; ISBN 9781119063643. [Google Scholar]
- Iora, P.; Tribioli, L. Effect of Ambient Temperature on Electric Vehicles’ Energy Consumption and Range: Model Definition and Sensitivity Analysis Based on Nissan Leaf Data. World Electr. Veh. J. 2019, 10, 2. [Google Scholar] [CrossRef] [Green Version]
- Genikomsakis, K.N.; Mitrentsis, G. A computationally efficient simulation model for estimating energy consumption of electric vehicles in the context of route planning applications. Transp. Res. Part D Transp. Environ. 2017, 50, 98–118. [Google Scholar] [CrossRef]
- Davis, K.; Hayes, J.G. Analysis of electric vehicle powertrain simulators for fuel consumption calculations. In Proceedings of the 2016 International Conference on Electrical Systems for Aircraft, Railway, Ship Propulsion and Road Vehicles & International Transportation Electrification Conference (ESARS-ITEC), Toulouse, France, 2–4 November 2016; pp. 1–6. [Google Scholar]
- Hayes, J.G.; Davis, K. Simplified electric vehicle powertrain model for range and energy consumption based on EPA coast-down parameters and test validation by Argonne National Lab data on the Nissan Leaf. In Proceedings of the 2014 IEEE Transportation Electrification Conference and Expo (ITEC), Dearborn, MI, USA, 15–18 June 2014; pp. 1–6. [Google Scholar]
- Tamaro, C.A. Vehicle Powertrain Model to Predict Energy Consumption for Ecorouting Purposes. Ph.D. Thesis, Virginia Polytechnic Institute, Blacksburg, VA, USA, 2016. [Google Scholar]
- Spanoudakis, P.; Tsourveloudis, N.; Doitsidis, L.; Karapidakis, E. Experimental Research of Transmissions on Electric Vehicles’ Energy Consumption. Energies 2019, 12, 388. [Google Scholar] [CrossRef] [Green Version]
- Ruan, J.; Walker, P.; Zhang, N. A comparative study energy consumption and costs of battery electric vehicle transmissions. Appl. Energy 2016, 165, 119–134. [Google Scholar] [CrossRef] [Green Version]
- Wu, G.; Zhang, X.; Dong, Z. Impacts of Two-Speed Gearbox on Electric Vehicle’s Fuel Economy and Performance. SAE Int. 2013, 1. [Google Scholar] [CrossRef]
- Di Nicola, F.; Sorniotti, A.; Holdstock, T.; Viotto, F.; Bertolotto, S. Optimization of a Multiple-Speed Transmission for Downsizing the Motor of a Fully Electric Vehicle. SAE Int. J. Altern. Powertrains 2012, 1, 134–143. [Google Scholar] [CrossRef]
- White, E.H.; Nelson, D.J.; Manning, P.C. An Illustrative Look at Energy Flow through Hybrid Powertrains for Design and Analysis. SAE Int. 2015. [Google Scholar] [CrossRef]
- Autonomie. Available online: https://www.autonomie.net (accessed on 23 March 2021).
- MathWorks. Available online: https://www.mathworks.com/help/simulink/ug/select-and-run-model-advisor-checks.html#bufy0_x (accessed on 10 June 2019).
- Cruise. Available online: https://www.getcruise.com (accessed on 19 January 2021).





| Parameters | Designation | |
|---|---|---|
| Motor rated power (kW) | 80 | |
| Motor rated torque (Nm) | 280 | |
| Motor maximum speed (rpm) | 10,300 | |
| Cooper losses coefficient (−) | 0.14 | |
| Iron losses coefficient (−) | 2.3 | |
| Windage losses coefficient (−) | 6 × 10−7 | |
| Constant losses (−) | 500 |
| Parameters | WLTC | NEDC | FTP-75 |
|---|---|---|---|
| Duration (s) | 1800 | 1180 | 1874 |
| Stops duration (s) | 235 | 241 | 241 |
| Distance (km) | 23.26 | 11.02 | 17.77 |
| Maximum speed (km/h) | 131.3 | 120 | 91.1 |
| Average speed (km/h) | 46.5 | 33.6 | 34.2 |
| Maximum acceleration (m/s2) | 1.66 | 1.11 | 1.48 |
| Minimum acceleration (m/s2) | −1.5 | −1.39 | −1.48 |
| Parameters | Designation | |
|---|---|---|
| Type of EV | BEV | |
| Aerodynamic drag coefficient | 0.28 | |
| Frontal area (m2) | 2.19 | |
| Rolling friction coefficient | 0.0083 | |
| Rolling speed coefficient | 1 × 10−5 | |
| Vehicle mass (kg) | 1645 | |
| Mass correction coefficient | 1.1 | |
| Motor rated power (kW) | 80 | |
| Motor rated torque (Nm) | 254 | |
| Motor rated speed (rpm) | 3000 | |
| Motor maximum speed (rpm) | 10,000 | |
| Maximal speed (km/h) | 144 | |
| Acceleration time (s) | 0–100 km/h | 11.5 |
| Transmission ratio | 8.19 | |
| Wheel rolling radius (m) | 0.315 | |
| Transmission mechanical efficiency (-) | 0.95 |
| Parameters | WLTC | WLTC | NEDC | NEDC | FTP-75 | FTP-75 |
|---|---|---|---|---|---|---|
| Single Speed Gearbox | Dual Speed Gearbox | Single Speed Gearbox | Dual Speed Gearbox | Single Speed Gearbox | Dual Speed Gearbox | |
| Total energy per cycle (kWh) | 3.173 | 3.079 | 1.415 | 1.389 | 2.235 | 2.184 |
| Average power (kW) | 6.35 | 6.16 | 4.32 | 4.23 | 4.29 | 4.19 |
| Specific energy (kWh/km) | 0.136 | 0.132 | 0.129 | 0.126 | 0.126 | 0.123 |
| CPU time (ms) | 1.426 | 1.705 | 1.325 | 1.541 | 1.551 | 1.746 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hinov, N.; Punov, P.; Gilev, B.; Vacheva, G. Model-Based Estimation of Transmission Gear Ratio for Driving Energy Consumption of an EV. Electronics 2021, 10, 1530. https://doi.org/10.3390/electronics10131530
Hinov N, Punov P, Gilev B, Vacheva G. Model-Based Estimation of Transmission Gear Ratio for Driving Energy Consumption of an EV. Electronics. 2021; 10(13):1530. https://doi.org/10.3390/electronics10131530
Chicago/Turabian StyleHinov, Nikolay, Plamen Punov, Bogdan Gilev, and Gergana Vacheva. 2021. "Model-Based Estimation of Transmission Gear Ratio for Driving Energy Consumption of an EV" Electronics 10, no. 13: 1530. https://doi.org/10.3390/electronics10131530
APA StyleHinov, N., Punov, P., Gilev, B., & Vacheva, G. (2021). Model-Based Estimation of Transmission Gear Ratio for Driving Energy Consumption of an EV. Electronics, 10(13), 1530. https://doi.org/10.3390/electronics10131530









