Improving Decision Making about Natural Disaster Mitigation Funding in Australia—A Framework

Abstract

Economic losses from natural disasters pose significant challenges to communities and to the insurance industry. Natural disaster mitigation aims to reduce the threat to people and assets from natural perils. Good decisions relating to hazard risk mitigation require judgments both about the scientific and financial issues involved, i.e., the efficacy of some intervention, and the ethical or value principles to adopt in allocating resources. A framework for selecting a set of mitigation options within a limited budget is developed. Project selection about natural disaster mitigation options needs to trade off benefits offered by alternative investments (e.g., fatalities and injuries avoided, potential property and infrastructure losses prevented, safety concerns of citizens, etc.) against the costs of investment. Such costs include capital and on-going operational costs, as well as intangible costs, such as the impact of the project on the visual landscape or the loss of societal cohesion in the event of the relocation of part of a community. Furthermore, dollar costs of any potential project will need to be defined within some prescribed budget and time frame. Taking all of these factors into account, this paper develops a framework for good natural hazard mitigation decision making and selection.

1. Introduction

Economic and social losses from natural disasters pose significant challenges to communities and to the insurance industry globally. Whilst it is not possible to avoid these losses completely, developing resilience in communities will help them to recover more quickly from a disaster. This may include implementing mitigation measures, hard mitigation measures (e.g., a levee to protect a community from flooding) and soft mitigation measures (e.g., hazard awareness education campaigns) in at-risk communities.

In particular, Australia has experienced a number of disasters that have caused large economic losses and fatalities, injuries and disruption to people in recent years. A recent inquiry by the Productivity Commission, the Australian Government’s independent research and advisory body on economic, social and environmental issues affecting the welfare of Australians, into the funding of natural disaster mitigation and recovery in the country highlighted the value of investing in (pre-disaster) hazard mitigation, rather than spending on post-disaster recovery. The Commission made broad recommendations relating to, amongst other things, criteria and processes that should be applied in the prioritisation and selection of mitigation projects so as to qualify for increased mitigation funding from Federal Government funds. This paper develops these recommendations into a practical framework for mitigation decision making and selection.

2. Natural Hazard Losses in Australia

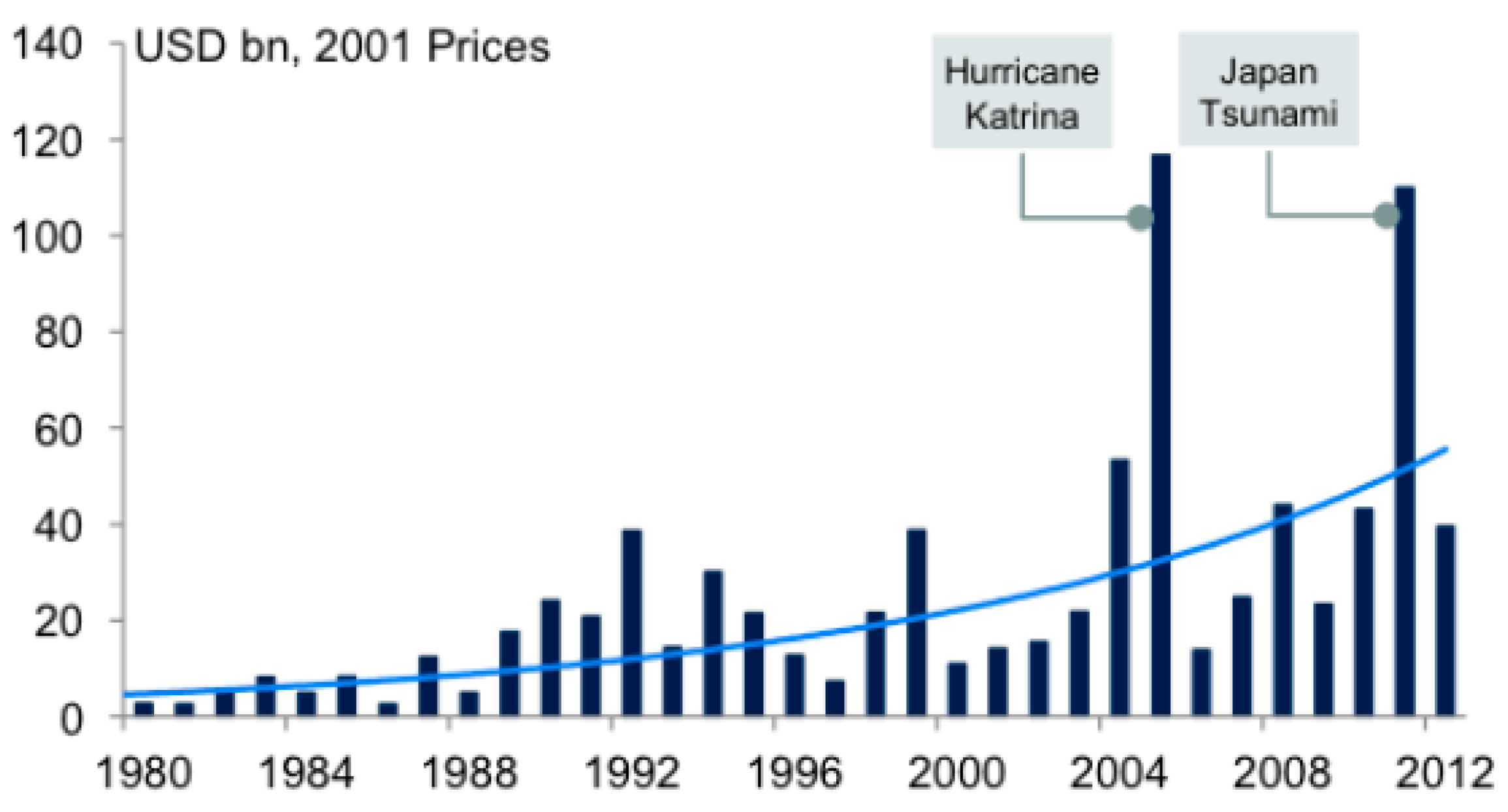
2.1. Economic Losses from Natural Disasters

Australia is at risk of large economic losses posed by a range of different natural perils. In fact, six different peril types are represented in the top ten most costly natural disaster events after normalization (Normalisation is a procedure commonly used to measure the impact of past events on present day society. In the case of insured building losses (as in

Table 1. Australia’s top ten most costly natural disaster events (insured losses, normalised to 2011 values) (Source: [1]).

Rank	Event	Peril Type	Year	Actual Insured Cost (A$bn)	Normalised Insured Cost (2011 A$bn)
1	Sydney hailstorm	Hail	1999	1.700	4.296
2	TC Tracy	Tropical cyclone	1974	0.200	4.090
3	Newcastle earthquake	Earthquake	1989	0.862	3.240
4	Brisbane flood	Flood	1974	0.068	2.645
5	Queensland floods	Flood	2011	2.400	2.500
6	Brisbane hailstorm	Hail	1985	0.180	2.063
7	Newcastle East Coast Low storm	Severe storm	2007	1.480	1.742
8	TC Madge	Tropical cyclone	1973	0.030	1.492
9	Ash Wednesday bushfires	Bushfire	1983	0.138	1.489
10	TC Yasi	Tropical cyclone	2011	1.300	1.352

), this involves taking account of changes in population size, personal wealth and inflation. In the case of fatalities, the changing population size should be accounted for.) to take account of changes in inflation, wealth and population [1,2] (Table 1).

Globally, insured losses caused by natural disaster events have increased rapidly in recent years. Figure 1 shows global insured disaster loss data from Swiss Re, Munich Re and Deutsche Bank Research.

Australian insured natural disaster losses caused by weather-related events have shown a similar increasing trend since about 1990 (Figure 2). Taken over the period of 1996–2013, a linear trend line has a statistically very significant slope (p < 0.0001).

The data in Figure 2 are insured losses only. Often, public infrastructure is uninsured, as are a proportion of household losses. It has been estimated that 1.8 million households in Australia (23% of the country’s total) do not have building or content insurance [4], and roads (31% of all state-owned assets by value in Australia) are only insured in two states [5]. Whilst this cannot be estimated accurately, for most purposes, the total economic cost of natural disasters is often estimated as of the order of double the insured losses.

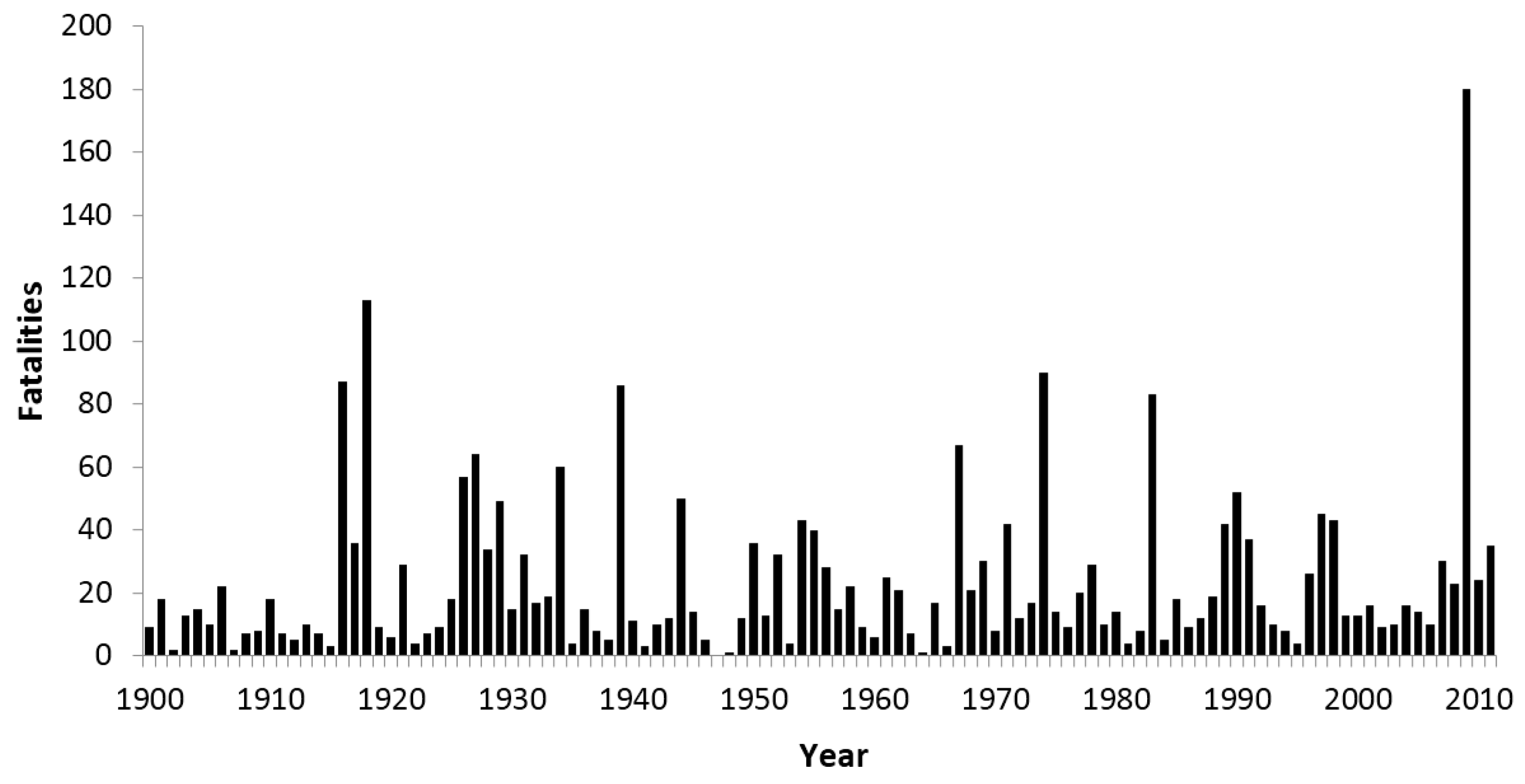
2.2. Natural Disaster Fatalities

Natural hazards have also taken their toll on human life. According to the International Federation of Red Cross and Red Crescent Societies [6], 1,059,072 lives were lost globally in the decade between 2004 and 2013 due to natural disasters. Figure 3 displays fatalities from bushfires, earthquakes, floods, wind gust, hail, landslides, lightning, rain, tornadoes and cyclones in Australia from 1900 to 2010.

A linear trend line through the fatality data in Figure 3 shows no significant trend, i.e., it can be inferred that the fatality rate has been constant across time. A total of 2596 deaths from natural hazards has been recorded in Australia since 1900.

The damage, fatalities, displacement and disruption to communities caused by natural disasters might justify that mitigation measures be put in place to reduce the impacts of unavoidable natural perils.

3. The Productivity Commission Inquiry: Impetus for a Decision Framework

Mindful of the economic and human costs of natural disasters, the Productivity Commission, the Australian Government’s independent research and advisory body on economic, social and environmental issues affecting the welfare of Australians [7], was charged with investigating the funding of natural disaster mitigation and recovery in Australia. The Commission’s final report from the Public Inquiry was published in May 2015 [8].

The report highlighted that effective planning and mitigation of risks is an essential task for governments, businesses and households. It furthermore concluded that:

“Governments overinvest in post‑disaster reconstruction, and underinvest in mitigation that would limit the impact of natural disasters in the first place. As such, natural disaster costs have become a growing, unfunded liability for governments”

[8]

The Commission recommended the Government move away from funding post-disaster recovery (which offers no incentive for individuals or communities to mitigate their own risk) towards funding pre-disaster mitigation of disaster risks. This could be via reducing the exposure of people and assets to natural perils and/or via reducing their vulnerability to the impacts of the perils concerned.

Recommendations were proposed relating to the criteria that should be applied by States and Territories to prioritise and select mitigation projects so as to qualify for increased mitigation funding from Federal Government coffers. These included the use of cost-benefit analysis, the assessment of non-quantifiable impacts and the inclusion of a measure of a project’s likely risk reduction. It was a condition that risk reduction should be conducted in accordance with Australia’s National Emergency Risk Assessment Guidelines (NERAG) [9], which provides a contextualised, emergency-related risk assessment method consistent with the Australian Standard AS/NZS ISO 31000:2009 Risk management—Principles and guidelines [10]. The outputs from NERAG risk assessments are intended to improve decision making when allocating scarce resources for risk treatment and emergency prevention and preparedness measures.

The Australia New Zealand Emergency Management Committee (ANZEMC) commissioned research to establish a framework for mitigation selection decision making, based on best-practice decision making modelling and in line with the recommendations of the Productivity Commission. The objective was to develop method(s) that could be applied to assess the risk-reduction benefits of mitigation investment options and to assist in making investment choices. The envisaged use of the framework was a recurrent allocation of a limited budget to a project set, with the understanding that the aggregated project costs in any round would likely exceed the available budget. The process should be repeatable (to be applied annually, say) and scalable (could be applied at any level of government, e.g., national, state or local levels). This called for a single model with common criteria to ensure temporal and spatial consistency.

4. Decision Making Regarding Disaster Risk Mitigation

Over the past 15 or so years, researchers have reported the modelling of cognitive decision making (both quantitative and qualitative) about reducing disaster risk. For example, reference [11] reviews a number of studies that describe decision making around actions that can be taken to increase the probability of the survival of people and structures (i.e., reduce risk) from wildfire (bushfire). These actions include preparation of the building and grounds by reducing fuel loads, developing a survival plan and the ensuring availability of appropriate equipment for survival and for defending the property. Penman et al. [11] determined that one of the key factors limiting adequate preparation is the time commitment and cost required to prepare adequately.

The literature on decision making modelling relating to mitigating disaster risks from an economic modelling perspective is fairly limited. Some early work was done on developing decision models for mitigating natural disaster risks. For example, reference [12] showed that a value function under risk was an appropriate approach to model and analyse a decision making process involving low probability, high consequence events, such as earthquakes. Reference [13] described a study to develop tools to analyse and measure the costs of disaster mitigation by exploring how cost-benefit analysis, environmental impact assessment and related methodologies can be expanded to consider the risks emanating from natural hazards. Reference [14] used social decision analysis to compare the costs and benefits of alternative policies for seismic safety faced by the city of Los Angeles with regard to its existing masonry structures from the viewpoints of all impacted constituents.

It was the economic and human losses caused by Hurricane Katrina in August 2005 that spawned significant research into decision making around funding allocation for natural disaster mitigation. Reference [15] described two paradoxes that can be used to explain the unprecedented losses caused by Hurricane Katrina. The “safe development paradox” is that in trying to make hazardous areas safer, the federal government in fact substantially increased the potential for catastrophic property damages and economic loss. This has come about from the thinking that land exposed to natural hazards can be profitably used if adequate steps are taken to make it safe for human occupancy. The federal government offers financial support for mitigation efforts and provides generous disaster relief to minimize the adverse financial consequences for individuals and businesses when steps to make development safe from hazards fail. The “local government paradox” is that while their citizens bear the brunt of human suffering and financial loss in disasters, local officials pay insufficient attention to policies to limit vulnerability. Reference [15] demonstrated that in spite of the two paradoxes, disaster losses can be blunted if local governments make development planning decisions that incorporate hazard risk mitigation. In a paper explaining the use of insurance as a means of mitigating natural disaster losses, reference [16] discussed the reasons why individuals fail to voluntarily mitigate against flooding and proposed a benefit-cost analysis to determine when a well-enforced building code would be appropriate as a financial mitigation measure from the perspective of both residents and the general taxpayer. In a similar vein, reference [17] discussed the use of benefit-cost analysis to assess the way public funds are spent on disaster mitigation projects.

Whilst the previous studies employed benefit-cost analysis, the economist’s tool of choice, to compare and select projects, it is unidimensional, considering the problem purely in an economic framework. On the other hand, multicriteria analysis allows a more comprehensive decision model if there are multiple decision variables (see [18]), such as risk, social benefits and costs/benefits. Reference [19] highlights that understanding risk as a multicriteria concept is a first step to facilitating its assessment and reduction through mitigation. Similarly, reference [20] explored a multicriteria decision making model for environmental decision making, including costs and benefits, environmental impacts for different populations, safety, ecological risk and human/social values as decision variables. Indeed, research output into risk management (including risk management around natural and other disasters) in a multicriteria framework has increased considerably in recent years. Some examples include [21], who used a multi-attribute utility model to select a value-focused strategy for reducing risk and protecting the population after a simulated nuclear accident; reference [22] described the role and use of multicriteria analysis in complex environmental impact assessment and decision making; reference [23] developed an electricity planning model dealing with uncertainty and its associated risk by minimizing environmental risk through a multiple-criteria model and performing a risk analysis, which applies decision rules for selecting the best planning strategy under uncertainty; reference [24] developed a methodology that combined the quantifiable conventional approach to risk (likelihood and consequence), the analysis of perceived risk based on stakeholders’ judgments and an analytical framework of a spatial multi-criteria analysis into a decision framework for risk assessment and applied this to a case of flood risk management; and reference [25] developed a multicriteria risk analysis (MCRA) model to compare the risk of ecological damage associated with various forest management systems, each with a different management intensity.

This paper continues to explore the multicriteria nature of the natural disaster mitigation decision and develops a framework for the selection and funding allocation based on a multicriteria decision making approach, including risk reduction and costs and benefits.

5. A Decision Model

5.1. The Project Objective and Key Decision Criteria

It is important to establish clearly and accurately at an early stage what the aim of the decision is. A stakeholder interest group, consisting of members of ANZEMC from several state jurisdictions, was convened to agree on the objective and to offer other input into the decision framework. Group members were located in different cities, and so, a Delphi-like process was employed: initially, one group member proposed a decision objective; the others then debated this via an exchange of emails, leading to an amended objective. This process was time-consuming, consisting of several iterations over a month. There was robust debate amongst stakeholders: those with an emergency management background favoured risk minimization at any cost, whilst others insisted on costs being the major factor to be considered. Using email as a medium of debate levelled the playing field; through the debate, some did not get their way; however, the end point was general acceptance amongst all stakeholders and, for most, a clearer understanding of what was being attempted.

The overall agreed project objective was:

“Produce a framework to consistently and reliably evaluate, compare and choose between potentially suitable alternative options for developing safer, healthier and more resilient communities, in the face of limited financial resources.”

5.2. A Hierarchy of Objectives

5.2.1. Decision Criteria

The project objective clearly includes three key decision criteria:

• Reduction of risk to the community (“safer”);
• Other social benefits (“healthier and more resilient”);
• Cost effectiveness (“in the face of limited financial resources”).

The objectives and criteria may be pictured as in the simple hierarchy in Figure 4.

Each of the three criteria (cost effectiveness, risk reduction and social benefits) can be represented by one or more measurable attributes, each of which assesses some property of the decision alternatives. The attributes used to capture the essence of the three criteria in the framework are described below.

Cost Effectiveness

Cost-benefit analysis (CBA) is the analytical tool most often advanced for evaluating alternative courses of action, projects or policy options by governments and business. Potential scenarios may be government-funded programs, such as infrastructure construction, resource allocation projects and policy or regulation changes. CBA calculates the present value (PV) of all benefits of a project, less the present value of all costs, subject to specified constraints, i.e., it calculates the net present value (NPV) of an investment decision. The costs and benefits of a project may occur at different points in time: major costs or investments generally occur up front, whilst benefits accrue over the project’s lifetime. CBA adjusts for both the time value of money and project risk though a discount rate that is used to reduce future costs and benefits to present values that can be simply added or subtracted. In principle, CBA can be applied across the entire range of government spending priorities, as long as all costs and benefits can be expressed in monetary terms.

Economic benefits from a mitigation project are reductions in asset and economic activity losses. Thus, the present value of the reduction in losses of economic activity (disrupted supply chains, disruption to services that support economic activity (e.g., transport, electricity) or a loss of markets) and reduction in the loss or damage to buildings, contents and infrastructure due to a mitigation option can be estimated by the CBA. An appropriate metric arising from the CBA is the benefit-to-cost ratio (BCR) (Transportation Economics Committee, n.d.), which can be computed as:

$$\begin{gathered} BCR=\frac{PV\left(reductioninassetlosses\right)+PV\left(reductionineconomicactivitylosses\right)}{PV\left(costs\right)} \\ =\frac{{{\displaystyle \sum }}_{t=1}^n\frac{c_t}{{\left(1+r\right)}^t}+{{\displaystyle \sum }}_{t=1}^n\frac{b_t}{{\left(1+r\right)}^t}}{{{\displaystyle \sum }}_{t=1}^n\frac{cost{s}_t}{{\left(1+r\right)}^t}} \end{gathered}$$

where:

• t = year
• n = time horizon (50 years, say; typically the expected lifetime of the mitigation project)
• r = discount rate
• b_t = reduction in economic activity losses in year t
• c_t = reduction in asset losses in year t
• costs_t = capital and operational costs of the project in year t

Recent examples of the use of CBA in natural hazard disaster mitigation investment decisions in Australia include the raising of the Warragamba Dam wall to prevent flooding losses [26] and an analysis of flood mitigation options for the suburb of Invermay, Launceston [27]. Walker et al. [28] proposes a probabilistic approach for undertaking a cost-benefit analysis for natural disaster mitigation, taking into account potential changes in the hazard through time, as well as changes in the vulnerability of the built asset environment and the aggregate value of assets exposed due to the growth of the community through time (and hence, the associated increased concentrations of wealth).

Whilst CBA is widely used in practice, it has several limitations (for a discussion see, for example, [29]), and the economic purity embodied in CBA may mask important decision attributes that are difficult to monetise.

In any event, the BCR of the economic characteristics of a potential mitigation project is always positive, i.e., BCR > 0. Furthermore, if only economic characteristics were to be considered, any potential mitigation project with BCR > 1 would generate a net benefit for society in terms of reducing potential disaster losses.

Risk Reduction

Emergency management officials would tend to focus on the risk aspects of a mitigation investment decision. Under the NERAG, risk is defined in terms of the likelihood of a natural disaster event and the consequences of the event on the community and its assets, should it occur, i.e.,

Risk = Likelihood × Consequence

Given that emergency risk management in Australia is undertaken in line with the NERAG, this way of measuring risk is likely to be most acceptable to emergency management stakeholders.

The NERAG describes the consequences of a natural disaster event in terms of five consequence criteria [9]. Each criterion has a well-defined table, whereby the impacts of an event can be categorised.

• Human fatalities and injuries ([9] (Table 3, p. 56, people consequences));
• Property, infrastructure or other economic losses ([9] (Table 5, p. 59, economic consequences));
• Critical ecosystem or species loss ([9] (Table 6, pp. 62–63, environmental consequences));
• Impairment of public administration ([9] (Table 7, p. 67, public administration consequences));
• Loss or destruction of community well-being or culturally-important objects or activities ([9] (Table 8, p. 69, social setting consequences)).

Thus risk can be viewed as a multi-criteria concept. The objective of mitigation is to reduce the risk to the community, and the “value” of a potential mitigation project is partly based on the degree to which the project succeeds in reducing the impact of one or more of the NERAG risk reduction criteria. The risk reduction criteria (and sub-criteria) can be pictured as in the simple hierarchical tree in Figure 5.

Each of the risk reduction criteria can be described by the consequences to that criterion with and without the mitigation project.

The NERAG consequence criteria contain categorical measurements of both quantitative and qualitative variables. For example, people consequences can be measured as an integer (i.e., the number of fatalities or the number of people injured in an event relative to the population of interest), whilst the economy consequences (i.e., the loss of economic activity or assets (including buildings, contents and infrastructure) due to an event of a given size or likelihood) is best measured in monetary units (dollars), relative to the gross product of the area under consideration (i.e., a ratio). The categorization of the people and economy criteria is displayed in

Table 2. Categorization of people consequences in the NERAG (source: [9]).

Level	Death	Injury and Illness
Catastrophic	Deaths directly from emergency greater than 1 in 10,000 people for the population of interest	Critical injuries with long-term or permanent incapacitation greater than 1 in 10,000 people for the population of interest
Major	Deaths directly from emergency greater than 1 in 100,000 people for the population of interest	Critical injuries with long-term or permanent incapacitation greater than 1 in 100,000 people for the population of interest or Serious injuries greater than 1 in 10,000 people for the population of interest
Moderate	Deaths directly from emergency greater than 1 in 1,000,000 people for the population of interest	Critical injuries with long-term or permanent incapacitation greater than 1 in 1,000,000 people for the population of interest or Serious injuries greater than 1 in 100,000 people for the population of interest
Minor	Deaths directly from emergency greater than 1 in 10,000,000 people for the population of interest	Critical injuries with long-term or permanent incapacitation greater than 1 in 10,000,000 people for the population of interest or Serious injuries greater than 1 in 1,000,000 people for the population of interest
Insignificant	Not applicable	Serious injuries less than 1 in 1,000,000 people for the population of interest or Minor injuries to any number of people

and

Table 3. Categorization of the economy criterion in the NERAG (source: [9]).

Level	Loss in Economic Activity and/or Asset Value
Catastrophic	Decline of economic activity and/or Loss of asset value greater than 4% of gross product produced by the area of interest
Major	Decline of economic activity and/or Loss of asset value greater than 0.4% of gross product produced by the area of interest
Moderate	Decline of economic activity and/or Loss of asset value greater than 0.04% of gross product produced by the area of interest
Minor	Decline of economic activity and/or Loss of asset value greater than 0.004% of gross product produced by the area of interest
Insignificant	Decline of economic activity and/or Loss of asset value less than 0.004% of gross product produced by the area of interest

, respectively.

The remaining three criteria:

• Environment (critical ecosystem and/or species loss);
• Impairment of public administration;
• Social setting (loss or destruction of community well-being or culturally-important objects or activities).

are all defined in the NERAG on a semantic (qualitative) scale and require a subjective estimate of the level of consequences with and without a risk-reducing mitigation project. For example, the categorization of the impairment of public administration is displayed in

Table 4. Categorization of the impairment of public administration criterion in the NERAG (source: [9]).

Level	State or National Description
Catastrophic	Governing bodies and utility providers are unable to deliver their core functions
Major	Governing bodies and utility providers encounter severe reduction in the delivery of core functions Governing bodies and utility providers are required to divert a significant amount of available resources to deliver core functions or seek external assistance to deliver the majority of their core functions
Moderate	Governing bodies and utility providers encounter significant reduction in the delivery of core functions Governing bodies and utility providers are required to divert some available resources to deliver core functions or seek external assistance to deliver some of their core functions
Minor	Governing bodies and utility providers encounter limited reduction in the delivery of core functions
Insignificant	Governing bodies’ and utility providers’ delivery of core functions is unaffected or within normal parameters

.

It will be noted that economic losses are included in both the measurement of the cost effectiveness of a mitigation project (as part of the BCR) and in the assessment of risk reduction (economic consequences, in the NERAG). These two loss measures are deemed quite different from one another: in the BCR, the dollar losses are compared to the dollar benefits of a project to evaluate cost effectiveness, whilst in the risk reduction criterion, the categorised economic loss measures the impact of asset losses and/or the decline in economic activity on the community. This therefore does not constitute double counting; these two representations of economic loss are distinctly different.

Social Benefits

Social benefits create a healthier and more resilient community. Social benefits accruing to a community through some natural hazard mitigation measure are the welfare values received by the community due to the mitigation measure. Many social benefits, such as the reduction in the perception of fear and dread from some natural hazard, or the increase in the utility derived from added recreational facilities created by building a levee bank, or the potential loss of the social fabric of a community caused by the relocation of a parcel of homes, cannot easily be quantified or monetised, but may play a role in mitigation option selection.

The measurement of a given social benefit may be on a categorical scale (none, little, moderate, significant, extreme); an appropriate semantic scale with clear definitions is required for every defined social benefit. Constructing a qualitative value scale is discussed in reference [30] (pp. 128–129). For simplicity, the social benefits and their inclusion in the framework will not be discussed further here; they can be dealt with in a similar fashion to the risk reduction criteria.

Assessing mitigation proposals may be considered under two different scenarios:

• Allocating a set amount of mitigation resources (a “mitigation fund”), where a ranking of proposals is required due to proposal values exceeding the available funds;
• Determining options for treating one or more specified hazard risks, where the total funding pool is not specified and will lead to future proposals for funding.

In the first scenario, as the total funding is fixed, the principal metrics to be assessed are the mitigation benefit (in terms of risk reduction and social benefits) versus the cost of the proposal. In the second scenario, the financial effectiveness of the project in terms of a traditional cost-benefit analysis is relevant, and projects with larger BCR will in general be preferred over those with a smaller BCR. A project with BCR < 1 would generally not be considered by the Treasury; however, its risk reduction and social benefits might be such that they may assist in getting the project implemented.

5.2.2. Relative Weighting of Decision Criteria

Not all of the criteria will necessarily be deemed equally important in the assessment of the value of a mitigation project’s ability to reduce risk. Thus, the relative weighting of the BCR vis-à-vis the risk reduction and social benefits criteria needs to be established.

The weights associated with criterion i, denoted w_i, will have a specific interpretation: for two attributes r and s, the ratio of weights w_r/w_s measures the amount the decision maker is prepared to sacrifice (or “trade off”) on the score of attribute s in order to obtain a one unit gain on attribute r. The weight w_i thus represents the relative importance of attribute i, in the sense of it being a measure of the gain associated with replacing the worst outcome with the best outcome for this attribute. The weights should be normalised so that Σw_i = 1. Several ways in which the weights can be determined have been documented, e.g., [29]. The weighting process should be undertaken by a qualified group (experts in policy, community resilience and decision making) independently and well before the evaluation of potential mitigation projects begins. This may, for example, be done in a workshop setting advised by a broad range of stakeholders. The weights used to arrive at a decision are an important part of the decision outcome: different judgments about weights may lead to a different decision, but the decision may be justified if transparency is preserved throughout the process. Once agreed upon, the weights should remain fixed for the duration of the decision process.

Several mathematically-sound methods for determining relative weights have been documented in [29]. A number of easy-to-use commercially available software packages exist to assist in setting weights in multi-attribute decision problems.

In reality, a decision making group will be unlikely to agree absolutely on exact values for all weights, either due to uncertainty in estimation or disagreement between group members. Under these circumstances, interval estimates of the weights can be determined [31,32]. These interval estimates might serve as an absolute range of potential values for criteria weights; any changes in criteria weights for future decisions of this nature should only occur within these ranges. The decision making group may vary the weights within the specified range to observe the sensitivities of the overall decision to the (sub-)criteria weights.

Since weights are based on subjective judgments, there is no prescribed value for the relative weight attached to each of the criteria or each of the criteria attributes. The ranges of weights imply uncertainty about weighting; a group of stakeholders, policy experts and decision makers in the domain of natural disaster response or recovery planning or funding is unlikely to display unanimous agreement regarding weights.

The sub-criteria of the risk reduction and social benefits criteria can be weighted in the same way as the overall decision criteria. Note that the weights should sum to one at each branch of the tree, e.g.,

• Weights for fatalities and injuries should equal one;
• Weights for the five NERAG consequence criteria (five weights) should add up to one.

5.3. Value Functions for the Decision Criteria

The cost effectiveness, risk reduction and social benefits are all measured on different mathematical scales (

Table 5. Mathematical scales of cost effectiveness, risk reduction and social benefits. BCR, benefit-to-cost ratio.

Decision Criterion	Scale	Measure
Cost effectiveness	Continuous	BCR > 0
Risk reduction	Categorical	Difference between risk categories (after-before mitigation)
Social benefits	Categorical	Difference between social benefit categories (after-before mitigation)

).

The impact of a disaster event (with or without mitigation) of a given size (or likelihood) is assessed by a set of (sub-)criteria represented by measurable attributes. The attributes measured on each criterion should be converted into a value on the unit interval [0, 1] for use in the multi-criteria decision making framework. The mathematical value function describes the subjective performance (arbitrarily scaled to the range from 0 to 1), or value, to the decision maker of the mitigation project under a given criterion. A value of one implies the decision maker subjectively views the mitigation project as the best possible under that criterion, whilst a value of zero implies the decision maker’s subjective view of the mitigation project to be impossibly small. The decision values are unitless.

The value to a decision maker of a mitigation project under some criterion may not show constant returns to scale across the range of attribute values, i.e., an improvement from 1 to 2 on some attribute may not have the same value to the decision maker as a move from 4 to 5 on the same attribute. This implies that the value function may not be linear.

5.3.1. A Value Function for Cost Effectiveness

A value function for the BCR describes the subjective performance (in the range from 0 to 1) or value to the decision maker of the mitigation project to offer benefits to the community for a given cost.

From a financial perspective only (i.e., economic losses and costs), the subjective value function of the BCR of the financial characteristics (economic activity and asset losses) of a potential mitigation project may be of a shape similar to that depicted in Figure 6. This shows that for BCR < 1, the value function is close to zero (i.e., the potential mitigation project has very little value), but increases rapidly as BCR increases in the range from 1 to 2, with little extra value added for larger values of BCR. The value V lies in the range [0, 1].

5.3.2. Value Functions for Risk Reduction Criteria

A Value Function for Consequences to People and the Economy

The gradations and descriptions for the NERAG consequence criteria categories (people and economy) are displayed in Table 2 and Table 3. For these quantifiable criteria, the NERAG uses an exponential scale to describe successive consequence levels, reflecting the NERAG approach that consequences of emergency events can cover several orders of magnitude ([9], p. 54).

The impacts of the five consequence categories for these criteria can be scaled onto a value function defined on a continuous [0, 1] range in a similar fashion to the BCR value function. The value function describes the subjective performance or value to the decision maker of the potential for the mitigation project to reduce the risk consequences to the community. The impacts of the five consequence categories for these criteria can be scaled onto a value function, requiring that:

• The lower gradation of the lowest consequence level (“Insignificant”) be allocated a value of zero;
• Successive gradations increase in multiples of 10 due to the exponential scale used in the NERAG consequence tables for these criteria;
• The successive gradations are mapped onto values that are increasing multiples of 10, from 10⁻⁴, to cover the range from 0 to 1.

Thus, the value function on the unit interval for the people and economy criteria is scaled as in

Table 6. Scaled values on [0, 1] for the people and economy criteria.

Level	Scaled Values
Insignificant	0–0.0001
Minor	0.0001–0.001
Moderate	0.001–0.01
Major	0.01–0.1
Catastrophic	0.1–1

, and displayed in Figure 7, showing that each successive consequence level is set to a factor of 10 greater than the previous one.

The shape of this value function implicitly recognises that there is a range of consequence values within each consequence level; this value function turns the discrete consequence levels into a continuous function. Furthermore, reducing the consequences of a natural disaster from the higher consequence levels (e.g., catastrophic to major) is more valuable than reducing consequences a similar amount in lower levels (e.g., minor to insignificant). In practical terms, this means that the difference between a catastrophic consequence and a major consequence is an order of magnitude greater than the difference between a major consequence and a moderate consequence, and so on.

Recall that in the NERAG, risk is defined as a function of consequence and likelihood. In the NERAG, the likelihood of a hazard event has been categorised into six categories (

Table 7. NERAG likelihood levels (source: Commonwealth of Australia, 2014).

Likelihood	Indicative Frequency	Annual Exceedance Probability (AEP)	Average Recurrence Interval (ARI)
Almost certain	Once or more per year	63% per year, or more	1 year or less
Likely	Once per 10 years	10%–63% per year	1–10 years
Unlikely	Once per 100 years	1%–10% per year	11–100 years
Rare	Once per 1000 years	0.1%–1% per year	101–1000 years
Very rare	Once per 10,000 years	0.01%–0.1% per year	1001–10,000 years
Extremely rare	Once per 100,000 years	0.001%–0.01% per year	More than 10,000 years

).

The likelihood categories in the NERAG are also logarithmically scaled, because the likelihood of disaster events can cover several orders of magnitude.

5.3.3. Value Functions for Social Benefit Criteria

Assuming that social benefits are all defined on a categorical scale (none, little, moderate, significant, extreme), with an appropriate semantic scale with clear definitions for every defined social benefit, then a convex value function (i.e., similar in shape to Figure 7) would describe a decision maker’s preferential values for each benefit category (none, little, moderate, significant, extreme).

5.4. Quantifying Risk Reducing Mitigation Benefits

5.4.1. Quantitative Risk Reduction Criteria

Events that may occur and which have significant negative consequences (i.e., “risky” events) should be considered for mitigation. In keeping with the NERAG, the risk of an event is determined by the likelihood and consequence of the event. In this method:

• The likelihood is measured by the annual exceedance probability (AEP). This is the probability of the event occurring exactly once in a year and is expressed as a number in the range 0–1. Another way of expressing this is the average recurrence interval (ARI) or average return period, which measures the average time between successive events of that magnitude.
• The consequence is measured as the weighted total of the consequence “values” (a dimensionless number in the range from 0 to 1), described above.

Both likelihood and consequence can be altered by a mitigation initiative. For example a flood levee might change the relative frequency (i.e., likelihood) of an event, whilst a change to the building code or construction type may change the consequence of an event on a community. Given that the intent of mitigation is to reduce the risk of a particular event, the benefit value of a given mitigation option (i.e., its risk reduction value (RRV)) is simply:

• the value of the reduction in consequences (if any) × the likelihood of the event (if consequences are reduced)

or

• the value of the consequences (if any) × the reduction in the likelihood of the event (if likelihood is reduced).

That is,

RRV = [Consequence value × AEP] _{before mitigation} − [Consequence value × AEP] _{after mitigation}

5.4.2. Qualitative Risk Reduction Criteria

The remaining three risk reduction criteria (environment, public administration and social setting) do not lend themselves easily to quantification and are not quantitatively defined in the NERAG. However, a value function with a shape as in Figure 7 may be used for these three criteria, with the values on [0, 1] for each consequence level being represented by the midpoint of the value range for the quantitative criteria.

Table 8. Scaled values for the qualitative criteria.

Level	Scaled Value
Insignificant	0.00005
Minor	0.00055
Moderate	0.0055
Major	0.055
Catastrophic	0.55

shows these values.

It should be noted that the consequence levels are defined on a semantic scale, with no assurance about the exponential scaling of successive consequence levels. The decision making team should determine whether these criteria are weighted the same as people and economy or whether their weightings need to be adjusted to account for the greater subjectivity of the criteria.

5.5. Aggregation of Weights and Values

An overall value for each potential mitigation project may be obtained by synthesising the (sub-)criteria weights and the mitigation project’s risk value scores (pre- and post-mitigation) on the unit interval under each (sub-)criterion, using a weighted sum of the risk value scores across all of the (sub-)criteria, i.e.,

$$RV\left(a\right)={\displaystyle \sum }_{i=1}^n{w}_{i}R{V}_i\left(a\right)$$

where:

• RV(a) = overall risk value of mitigation option a (pre- or post-mitigation)
• RV_i(a) = value score reflecting potential mitigation option a’s risk level under (sub-)criterion i
• w_i = weight assigned to reflect the importance of (sub-)criterion i.

If Σw_i = 1, the overall risk value for each potential mitigation option will be a unitless number in the range [0, 1].

5.6. Calculating Risk Reduction over Natural Disaster Events of All Possible Magnitudes

The risk reduction value (RRV) is unique to a single AEP value. This is the reduction in risk for an event of a single given magnitude. However, when evaluating a mitigation option, it should not be for a single event, but rather for a wide range of possible events. Naturally, the risk reduction abilities of a mitigation option will depend on the magnitude (or alternatively the likelihood) of the event.

Figure 8 displays the typical relationship between risk values (and resulting risk reduction values) for a range of likelihoods (AEP) for the reduction in economic consequence from a disaster mitigation proposal, with levels of consequence reduction varying across the range of events. Smaller events with higher AEP are typically already largely mitigated, and very large events with small AEP are not mitigated by the proposal. Consequences in the mid-AEP range are reduced to varying degrees. The blue curve represents the pre-mitigation risk values, whilst the red curve represents the typical post-mitigation risk values.

The overall risk reduction value of a mitigation option is the sum of the risk reduction values measured at every possible AEP value within the range. This is simply the area between the red and blue curves, which can be calculated using simple geometry.

The overall risk reduction value of the mitigation option should be reported as the proportion of risk reduced (measured across the entire range of AEP values), i.e., the area between the red and blue curves as a percentage of the area below the blue curve, across all AEP values.

5.7. The Overall Value of a Mitigation Option: Considering BCR and Risk Reduction

A mitigation option’s value lies in its ability to cost effectively reduce risk. This means that the overall value of a mitigation option should be calculated as a (weighted) linear combination of the value of the BCR on a [0, 1] range and the overall risk reduction value measured as a proportion of the pre-mitigation curve.

6. Simulation of Design Events Based on Historical Data

A potential mitigation project should be valued over a period equivalent to the expected life of the project. Thus, a period of 50–100 years is likely to be appropriate.

Implementation of the framework will require an understanding of the likelihood of a given magnitude event and consequences arising from the event. The easiest available form of this sort of data is a historical record of past events. Historical data can be collected from formal sources, such as council and emergency management logs and records and coronial and hospital records, and from informal sources, such as newspaper reports and anecdotal accounts by residents. Plotting historical data (flood height, tropical cyclone wind speed, etc.) against year allows a rough estimate of the likelihood of an event of a given magnitude (i.e., how often a certain threshold is exceeded in, say, 50 years).

The examination of historical data allows a picture to be created of the likely impacts (consequences) of an event of a given likelihood. The combination of consequences (i.e., impacts on the exposed buildings, infrastructure and people) and likelihood results in a measure of the risk attributable to the event.

If a more thorough and robust approach is desired and good historical data are available, a Monte Carlo simulation for a 50- or 100-year time span can be repeated a large number (say 10,000) of times and the key measures (building losses, fatalities, economic losses, etc.) averaged across the iterations. This will give a clear estimate of the likely outcome of each of the key measures, as well as an idea of the potential variation around the average outcome.

7. Project Portfolio Selection through Cost-Effectiveness Analysis

7.1. A Portfolio Decision Analysis Model for Mitigation Project Selection

This analysis is set in the context of a finite sum of money available to allocate to a range of potential natural disaster mitigation projects. The decision is to choose the best subset of projects within a given budget, where the term “best” refers to the combination of projects offering the greatest cost-effectiveness. This in turn is based on the projects’ values (as determined by the multi-criteria framework), and their capital and operational costs. This is a portfolio selection problem.

A simple heuristic for selecting a portfolio of projects may be the following:

Step 0

Let the overall mitigation project budget be $B.

Step 1

Of the available projects, select the one with the highest cost-effectiveness ratio (cost effectiveness ratio = CER = $\frac{Projectvalue}{Projectcost}$, where the project value is the score determined from the multicriteria model, scaled onto [0, 1]; this ratio allows a comparison of projects; those that offer greater value per dollar spent are preferred) (CER).

Step 2

Check that the cost of this project is lower than $B.

If yes, call this project P_[i] (where i = 1, 2, … is the selection rank of the project in the portfolio) and include it in the portfolio. Adjust the available budget to $B = $B-c_[i] where c_[i] is the cost of project P_[i]. Go to Step 1.

If no, select the project with the next highest cost effectiveness ratio (CER). Go to Step 2.

When no further projects can be selected, stop.

The portfolio of mitigation projects is the set {P_[1], P_[2], P_[3], ….}.

This heuristic may be appropriate when:

• There are a limited number of alternative projects
• The projects are independent of each other
• There are no constraints on the selection process.

The last two points require some explanation. Projects can be considered independent of one another if the impact (or value) of each of the projects is the same whether or not the other project was undertaken. For example, two mitigation measures might be proposed to address a single hazard in some community, e.g., building a levee, and physically moving home and infrastructure to higher ground, say, to address local flooding in a town. If the levee is built, the likelihood of flooding is minimised, and so, the value of moving the community is reduced. Similarly, if the community is moved, the threat to people and homes is minimised, and so, the value of the levee is reduced. Thus, these two mitigation measures are not independent. At the local level, where the decision is most likely to be around alternative measures to address the threat posed by a single hazard, the possibility of the dependence of the alternative options will likely exist. However, the decision at this level is likely to be around the single best option to address the threat. On the other hand, at the national level (where funding decisions are most likely made), the decision maker(s) are likely to be faced with choices amongst a range of potential mitigation projects across multiple hazards, a range of scales and in multiple jurisdictions/communities. These projects are then unlikely to exhibit the kind of interactions illustrated in the example above, and projects can then be considered independent.

The decision framework calls for the selection of the subset of potential mitigation projects that were most cost-effective in reducing risks to the community and maximizing social benefits. This framework evaluates each member of a set of potential mitigation options under a number of criteria; what remains is to identify the subset of projects that contains the most cost-effective projects, given the overall budget available for mitigation projects.

Furthermore, the selection of a portfolio of natural disaster mitigation projects is likely to be constrained in several ways:

(1)

Budget considerations will make it unlikely that every potential project will be able to be funded.

(2)

A selection panel is likely to impose an upper bound on the funds available for any single project.

(3)

The selection panel is likely to want to fund multiple projects. This will have several advantages:

(i)

Multiple projects means diversification of project impacts, both geographical and across domains

(ii)

Diversification will necessarily reduce the overall portfolio risk.

Other constraints may also be placed on the selection of projects by a decision making group.

Determining the best subset of alternative projects to select is a portfolio decision analysis (PDA) problem [33]. PDA has its roots in the field of quantitative finance, through Harry Markowitz’s approach to modern portfolio theory [34], which focuses on assessing both the risk and the returns of an asset portfolio. This approach is now widely used in R&D project selection.

The optimal selection of projects to be included in the portfolio can be achieved using linear programming, which is designed to achieve the best outcome for some objective (for example, the maximum profit or the lowest cost), given constraints on how the allocation may be achieved. It is a modelling requirement that the objective and constraints can all be represented by linear relationships.

Assume that n mitigation projects are under consideration for selection to a portfolio. A typical set of constraints for project selection might comprise

• a total funding budget of B dollars is available
• the cost of project i is c_i dollars
• the value score of project i is v_i units on [0, 1]
• at least s projects must be selected (for diversification)
• at most t projects must be selected
• at most r dollars can be spent on any one project.

In practice, further constraints can be added to this or any of these constraints removed.

Define a variable Y_i to be a binary variable, describing whether or not a project i is selected into the portfolio or not. Thus, Y_i is one if the project is selected, and Y_i is zero if the project is not selected.

  Objective function:

(While costs are additive, the project values are, by their definition, not additive. By this, it is meant that a portfolio of multiple projects may result in an aggregate value of greater than one, even though individual m project value is defined to have an upper bound of one. Thus, the aggregated value of the portfolio cannot be interpreted in the same way as an individual project’s value. In any event, a greater aggregated portfolio value is better than a lesser one.) Maximise v₁Y₁ + v₂Y₂ + v₃Y₃ + … + v_nY_n;

  Subject to:

Linear problem constraints:

c1Y1 + c2Y2 + c3Y3 + … + cnYn ≤ B	(total cost of all projects in the portfolio less than $B)
ci Yi ≤ r for all projects i = 1, 2, … , n	(at most r dollars can be spent on any one project)
Y1 + Y2 + Y3 + … + Yn ≥ s	(at least s projects selected, for diversification)
Y1 + Y2 + Y3 + … + Yn ≤ t	(at most t projects selected)

Non-negativity constraints:

Yi = 0 or 1

for all projects i = 1, 2, …, n

Powerful software tools exist to rapidly produce a solution to a linear optimisation model, such as the one presented above. For models of relatively small dimension, the Solver add-in for an Excel spreadsheet may be appropriate.

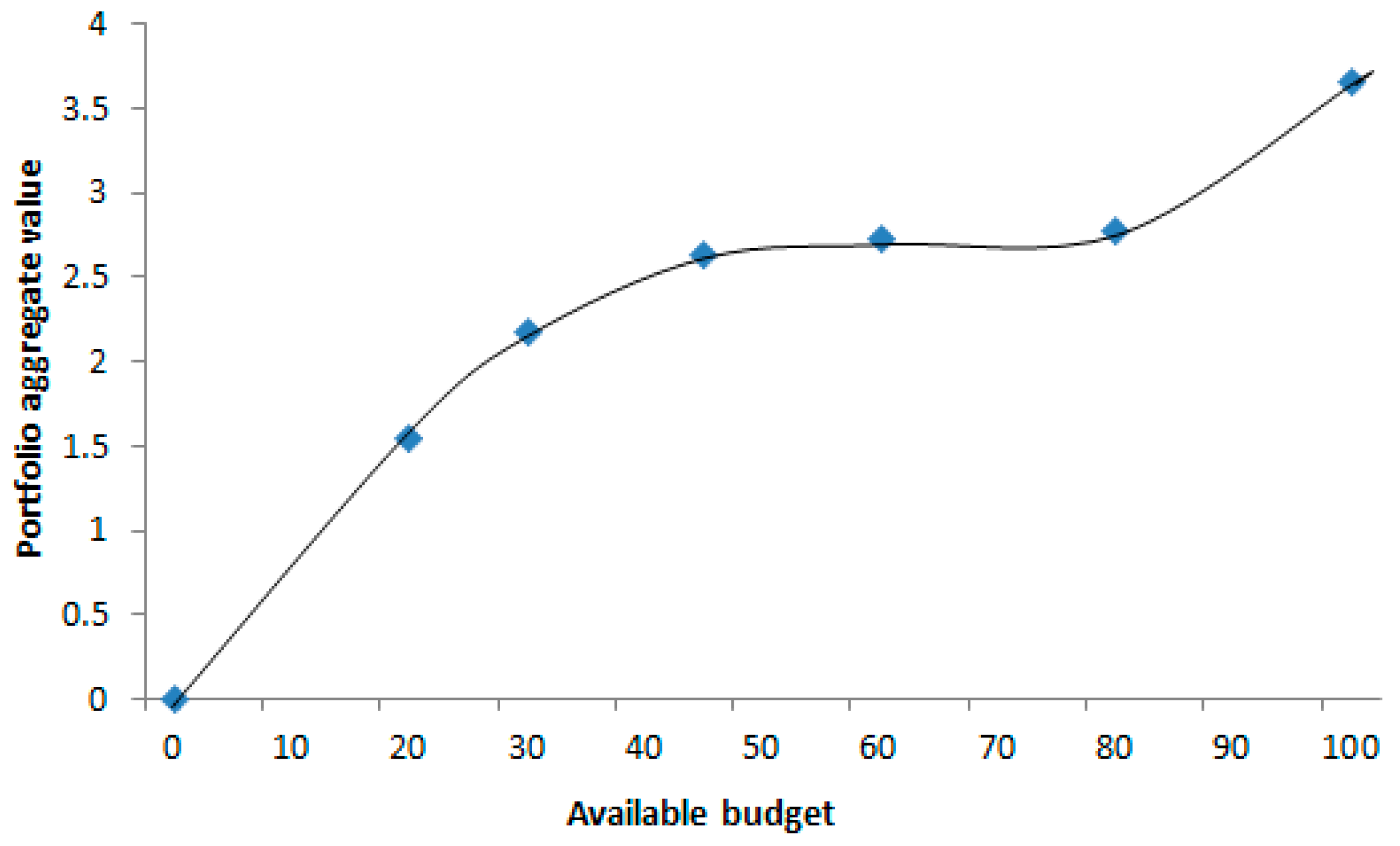
7.2. An Illustrative Example: Choosing a Portfolio of Mitigation Projects

Table 9. Assumed project costs and value scores.

Project	Cost ($m) (C_i)	MCDA "Benefit Score" (0–100) (V_i)	Cost-Effectiveness Ratio (×100)
1	22	0.66	3.000
2	4	0.49	12.250
3	15	0.45	3.000
4	47	0.82	1.745
5	2	0.4	20.000
6	6	0.34	5.667
7	20	0.37	1.850
8	7	0.31	4.429
9	10	0.64	6.400
	133

displays information for a set of nine potential mitigation projects. Assume that a budget of $45 million is available to allocate to projects in this funding round.

The project cost (Column 2) is essentially the present value of all costs related to the project (in $ million). The total cost of all projects together is $133 million, implying that not all projects can be funded given the budget. The multi-criteria “benefit score” (Column 3) is the overall value score associated with each project using a framework such as that developed in this paper. The cost-effectiveness ratio (Column 4) is the value score divided by the cost.

Project 4 clearly offers the greatest benefit (as defined by the multicriteria framework), but also has the greatest cost. By contrast, Project 5 has the lowest cost of all, but offers a relatively low benefit.

Applying the simple heuristic model would yield the following steps:

• Choose the most cost-effective project. This is Project 5 (CER is largest: 20.00). The cost of this project is $2 million, leaving $43 million available for other projects.
• Of the remaining projects, Project 2 has the largest cost effectiveness ratio (CER: 12.25). As the cost of this project is $4 million, it is added to the portfolio. The available budget is now $39 million.
• Projects 9 (CER = 6.40, cost = $10 million), 6 (CER = 5.67, cost = $6 million), 8 (CER = 4.43, cost = $7 million) and 3 (CER = 3.00, cost = $15 million) are then added iteratively, reducing the available budget to $29 million, $23 million, $16 million and $1 million at successive iterations. No further project can be added to the portfolio due to the budget constraint.

The final portfolio thus consists of Projects 2, 3, 5, 6, 8 and 9, with $1 million remaining.

Assume now that the selection of the portfolio of mitigation projects is constrained in the following ways (further constraints may also be placed on the selection of projects):

• A total funding budget of $45 million dollars is available.
• At least three projects must be selected (to ensure diversification).
• At most $30 million dollars can be spent on any one project.

The optimal selection of projects to be included in the portfolio using the Excel spreadsheet Solver routine is identical to that produced by the simple heuristic: Projects 2, 3, 5, 6, 8 and 9, costing $44 million.

Table 10. Project costs and value scores and the optimised solution: Excel Solver.

Project	Cost ($m) (C_i)	MCDA "Benefit Score" (0–100) (V_i)	Cost-Effectiveness Ratio (×100)	Y_i	C_i*Y_i
1	22	0.66	3.000	0	0.00
2	4	0.49	12.250	1	4.00
3	15	0.45	3.000	1	15.00
4	47	0.82	1.745	0	0.00
5	2	0.4	20.000	1	2.00
6	6	0.34	5.667	1	6.00
7	20	0.37	1.850	0	0.00
8	7	0.31	4.429	1	7.00
9	10	0.64	6.400	1	10.00
	133			6	44.00

displays the spreadsheet workings and optimised result for the linear programming model.

Further analysis may reveal changes to this optimal solution if constraints are altered. For example,

Table 11. Analysis of a change of the available budget.

	Budget ($ Million)
Project	20	30	45	60	80	100
1				x	x	x
2	x	x	x			x
3			x		x	x
4
5	x	x	x	x		x
6	x	x	x	x	x	x
7				x	x	x
8	x	x	x	x	x	x
9		x	x	x	x	x
Projects included	4	5	6	6	6	8
Portfolio aggregate value	1.54	2.18	2.63	2.72	2.77	3.66
Unused budget	1	1	1	0	0	14

displays the make-up of the optimal portfolio as the available budget changes from $20 million through to $100 million.

Table 11 shows that as the budget increases, more projects can be undertaken. However, the combination of projects changes:

• Projects 6 and 8 are selected in every portfolio
• Projects 5 and 9 are included in five of the six portfolios: Project 5 is included when the budget is lower, and Project 9 is included when the budget is higher
• Project 2 is included in four portfolios, mostly when the budget is low
• Projects 1, 3 and 7 are only included in half of the portfolios and only when the budget is high
• Project 4 is never included in any portfolio, as it is by far the costliest project and, thus, offers the lowest value for the money.

Figure 9 shows the increase in the aggregate value of the portfolio as the available budget increases. The slope of the curve is steepest for low budget amounts (less than $30 million, say) and for high budget amounts (greater than $80 million, say). These are the ranges in which an extra $1 million leads to the greatest increase in aggregate value. A careful study of Figure 9 should focus high level policy makers on the most cost effective budget for the mitigation decision.

8. Conclusions and Limitations

Whilst it is not possible to avoid incurring losses from natural disasters completely, there is evidence that implementing pre-disaster mitigation measures may help communities to recover more quickly from a disaster. This paper has outlined a decision framework for selecting a subset of pre-disaster mitigation options for cost effectively reducing the impact of natural hazard risks. The approach has employed a mix of concepts from economics, multicriteria decision analysis and portfolio decision analysis to arrive at an optimal selection of potential natural disaster mitigation projects within a given budget, where the term “optimal” refers to the combination of projects offering the greatest cost-effectiveness of achieving maximum risk reduction and social benefits.

8.1. Practical Utility of the Framework

The framework presented here puts a structure around the mitigation funding decision. Whilst the cost-benefit part of the model is simple and well understood, the multicriteria modelling concepts for risk reduction and social benefit evaluation may be difficult to understand for the non-quantitative user. It is envisaged, however, that in an operational form, the model would be developed into a software tool with a user-friendly graphical user interface, making usage potentially quite simple.

8.2. Limitations

In the proposed framework, risk reduction (the core of the mitigation decision evaluation) is based on the NERAG. Whilst this is accepted practice in the emergency management community in Australia, this may not be the case universally. Whilst this may be viewed as a limitation, it is feasible to consider alternative criteria (discrete or continuous in nature) in the definition of risk reduction.

Implementation of the framework relies on an understanding of the likelihood of a given magnitude event and consequences arising from the event, usually based on historical records of past events. A limitation of this framework is that historical data may be unavailable or sparse, forcing a user to resort to guesstimation to establish the values of these parameters. Inexperience may lead to large errors in the guesstimates, which might potentially lead to poor decisions.

8.3. Further Work

Several areas should be more closely investigated to operationalize the framework. The framework proposed here makes use of the NERAG-based definition of risk: one that is based on five distinct consequence criteria, which describe the way a natural disaster event may impact a community. This definition is specific to the Australian emergency management framework, and so, further research may be directed towards investigating alternative definitions of hazard risk to a community and how this risk reduction may be measured. Similarly, the social benefits arising out of mitigation should be investigated in some detail and the consequence criteria defined. Further behavioural research should be undertaken on the shape of the value functions (particularly the value function relating to asset and economic activity losses in Section 5.3.1). The portfolio selection model proposed in the framework is a first-pass representation of the constraints that may be placed on projects’ selection. Further investigation into what might comprise a realistic set of constraints (and under what conditions) may be undertaken.