An FSK and OOK Compatible RF Demodulator for Wake Up Receivers
Abstract
:1. Introduction
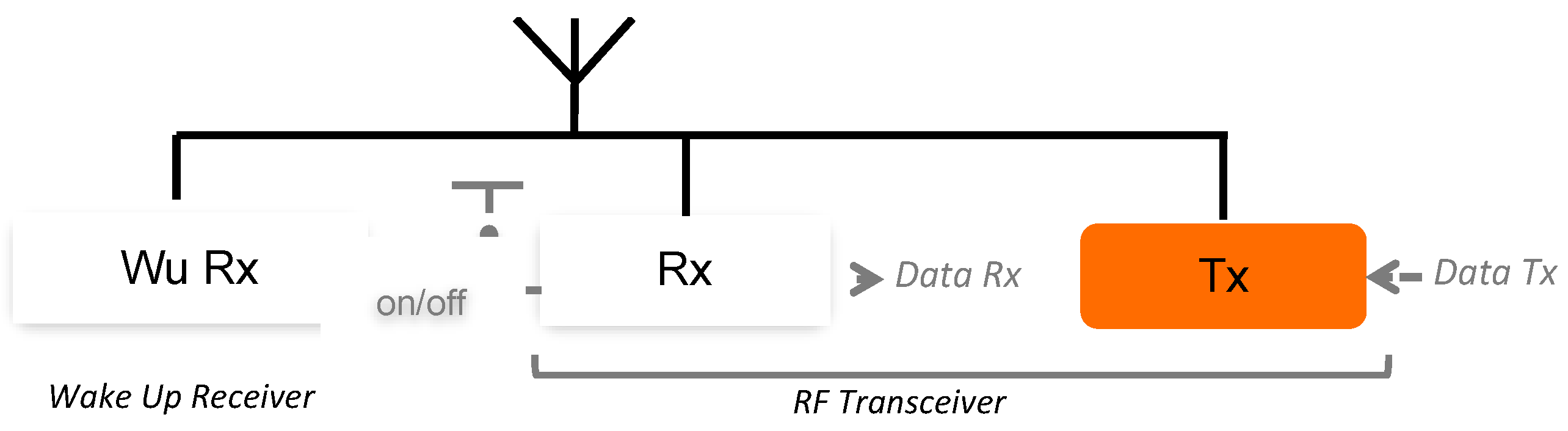
2. Principle of Frequency and Amplitude Demodulation with an LC Oscillator
2.1. Non-Coherent Demodulation of a Frequency Modulated Signal
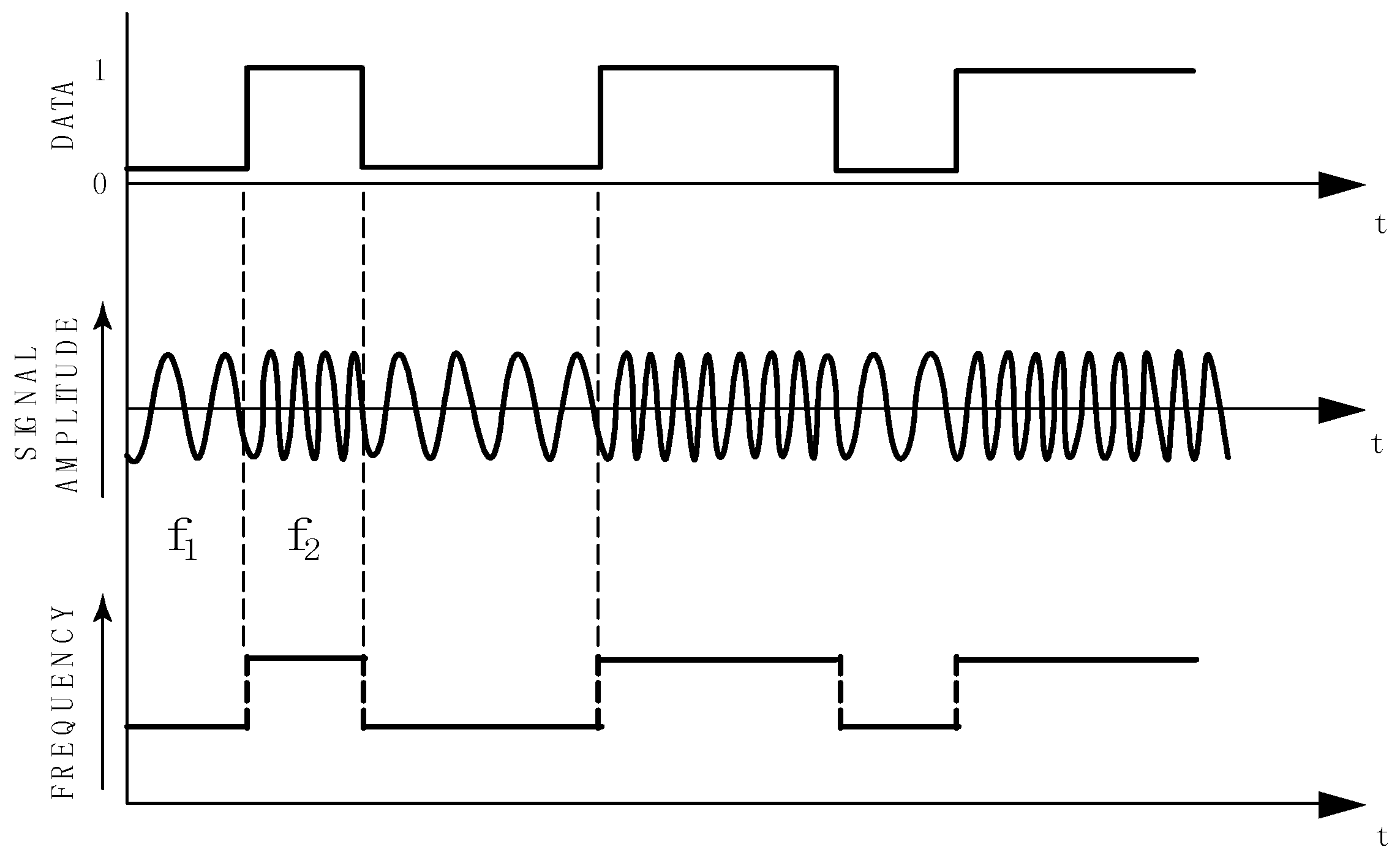
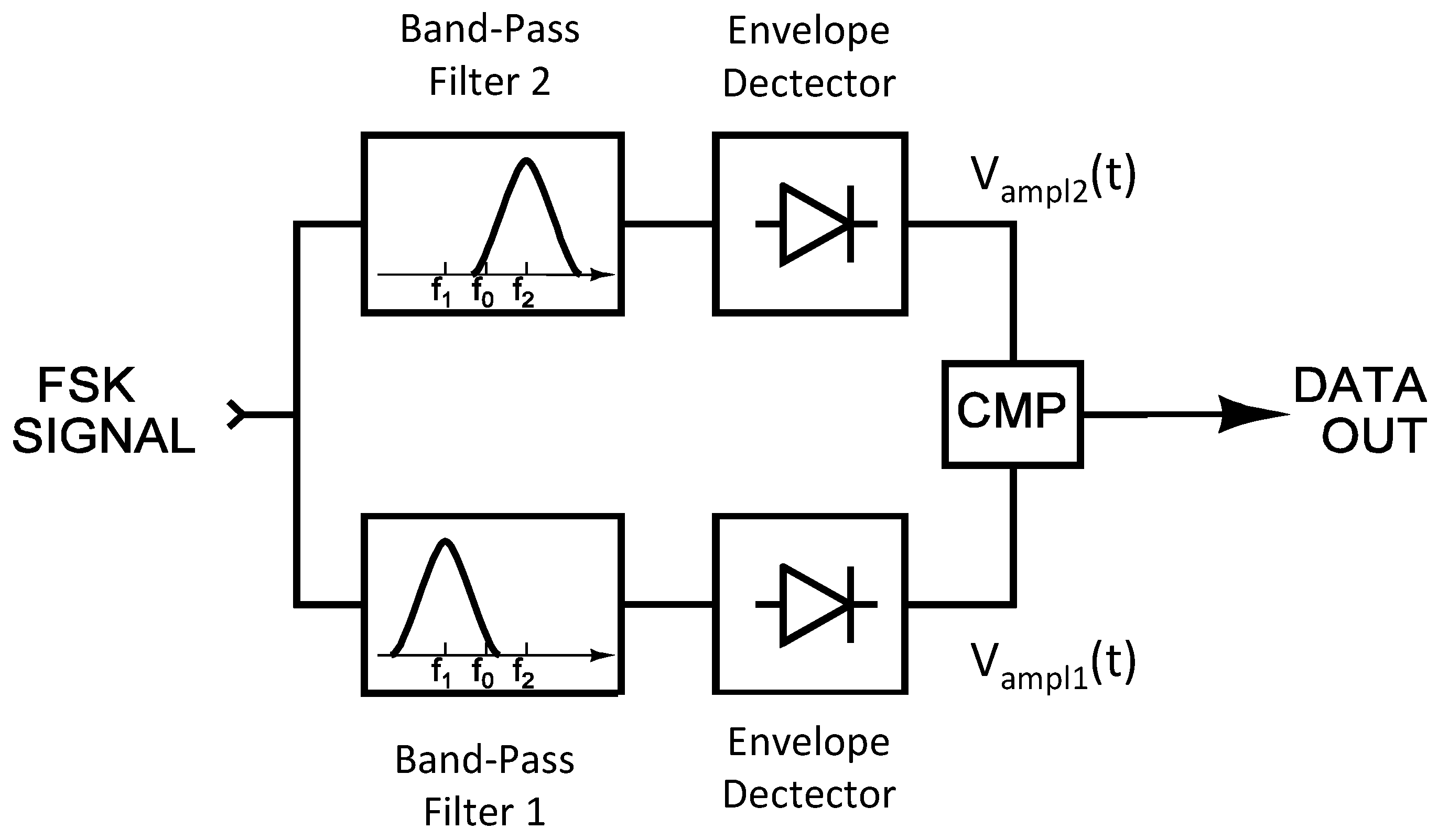

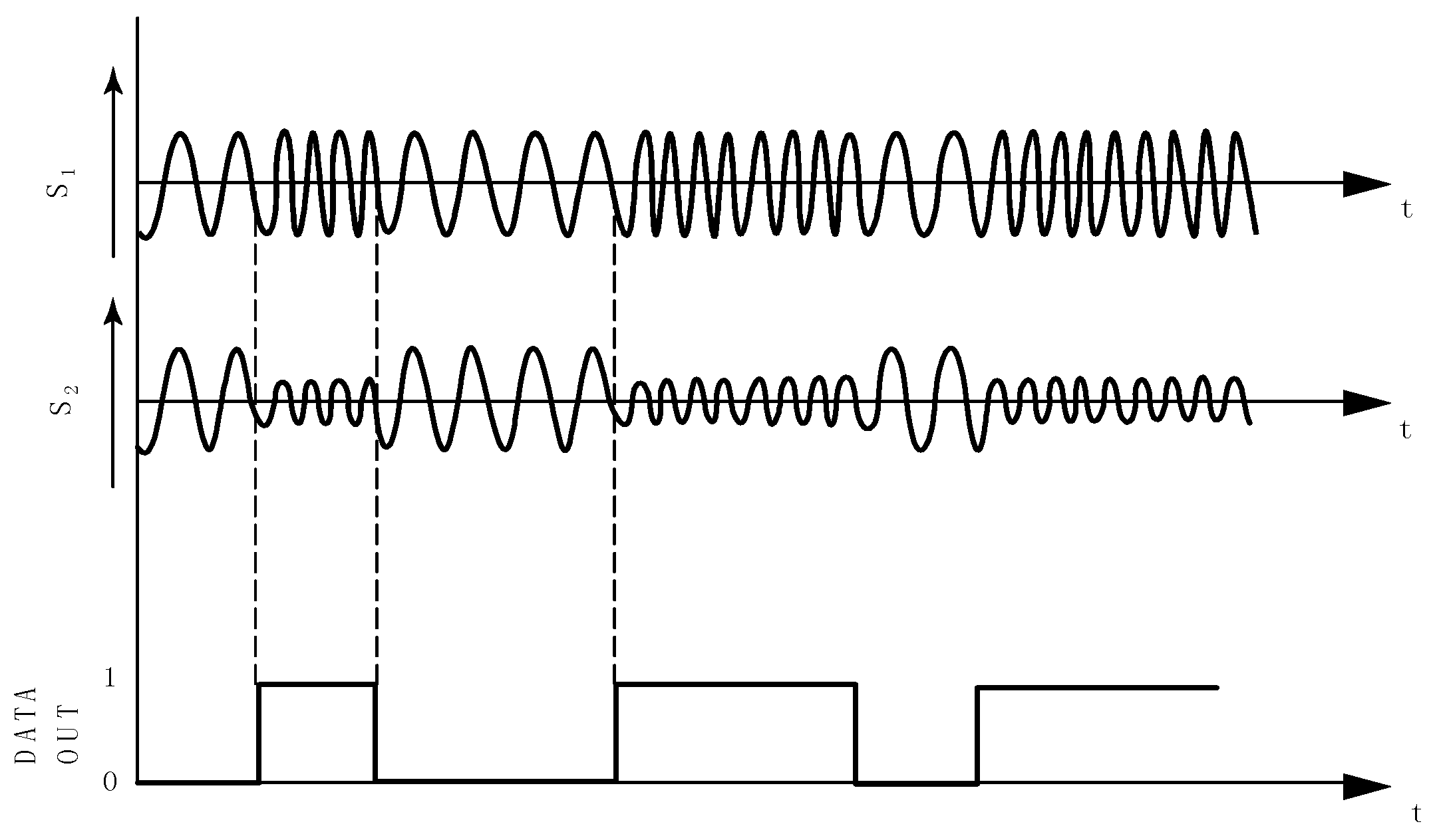
2.2. AM-to-FM Conversion and AM-AM Transfer with an LC Oscillator
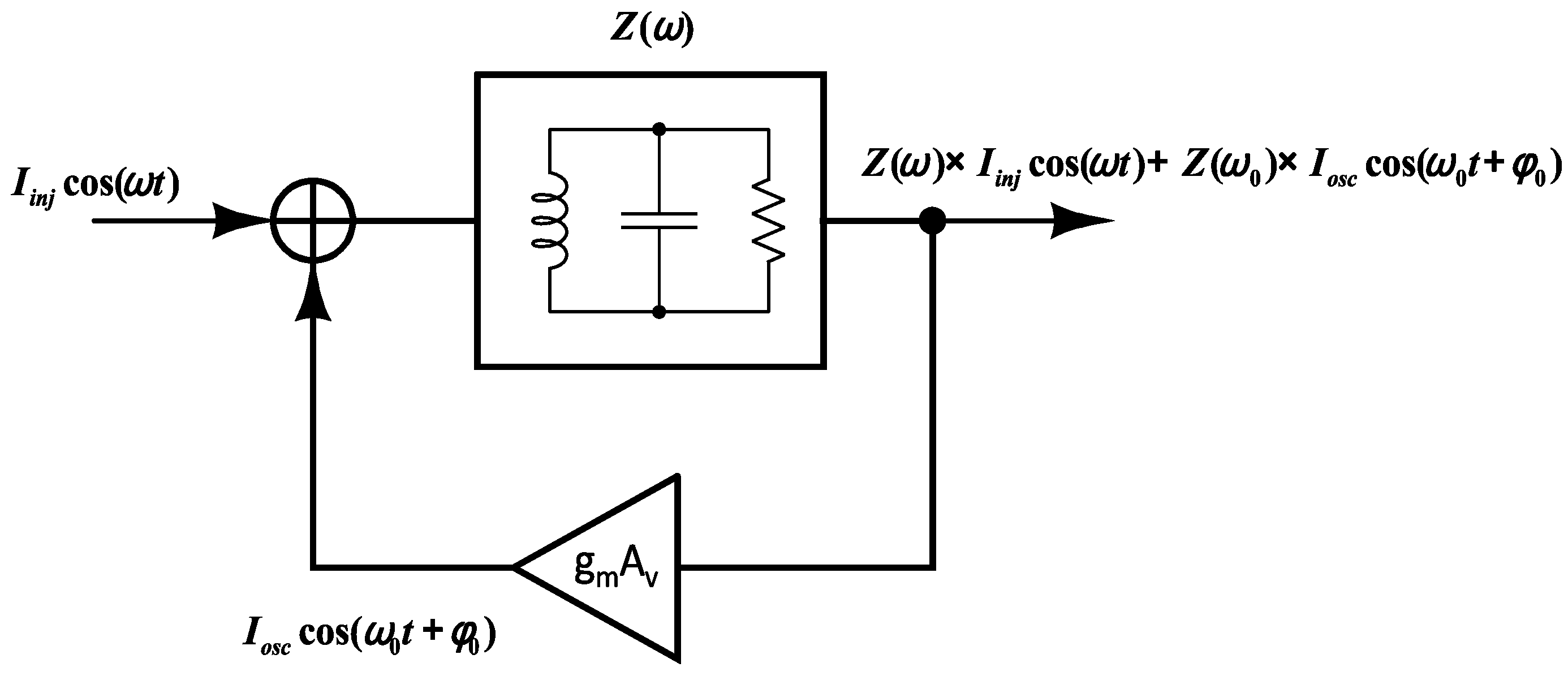
2.2.1. Injection of a FM Signal
2.2.2. Injection of an AM Signal
3. Implementation of a MOOD Demodulator
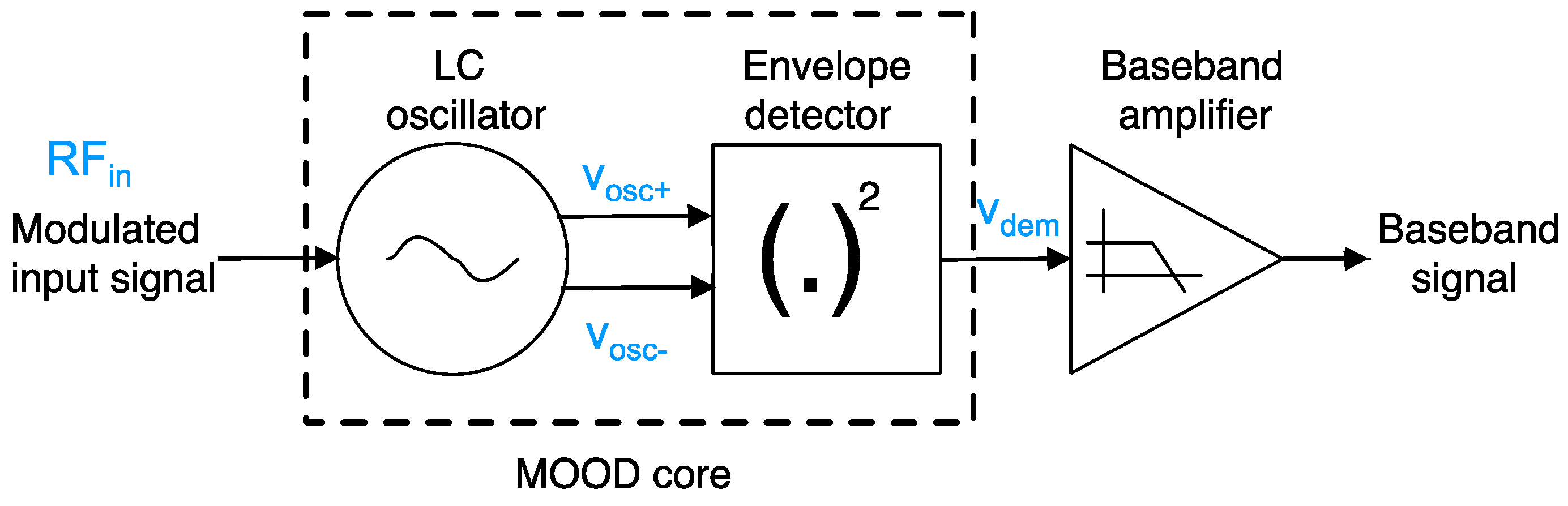
3.1. Low Power LC Oscillator
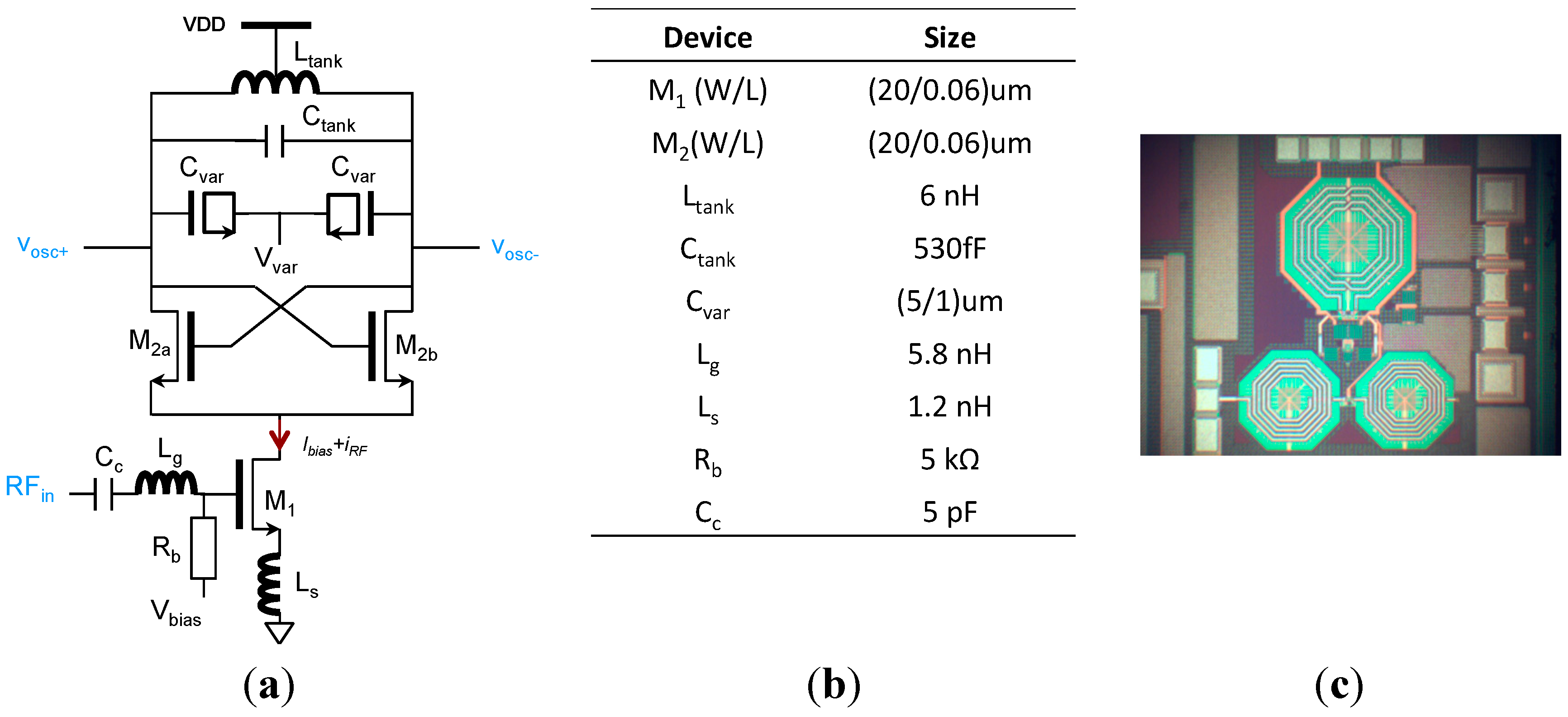

3.2. Envelope Detector Design
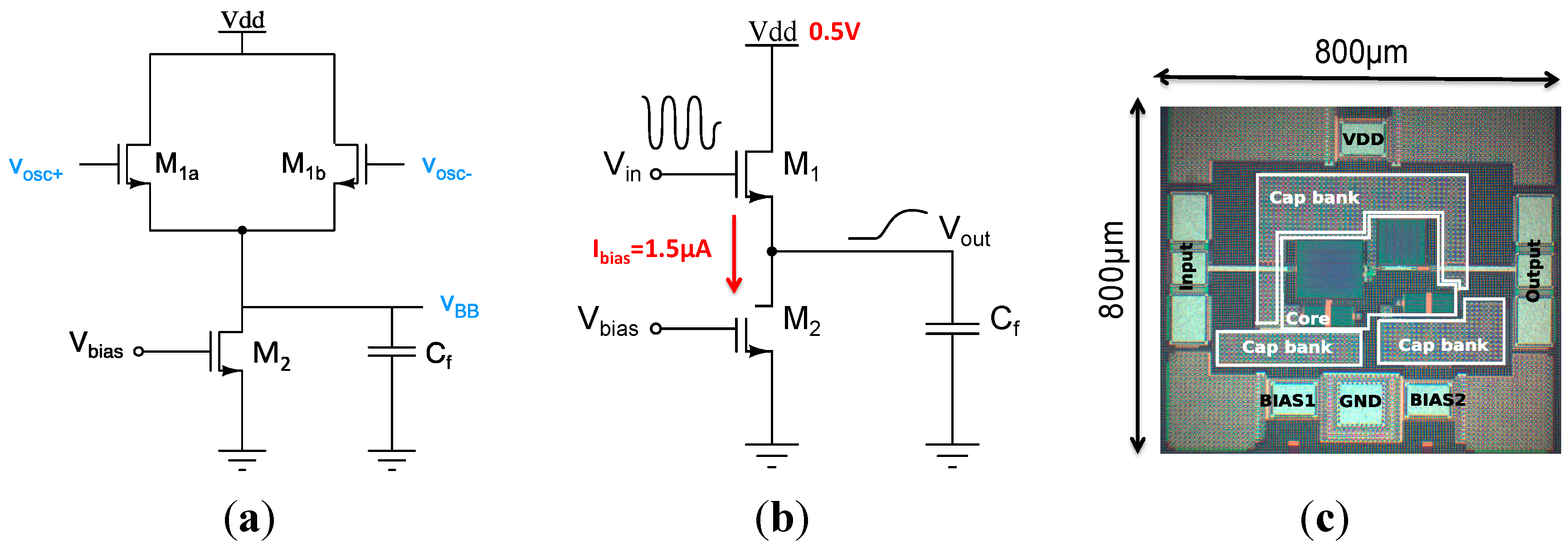
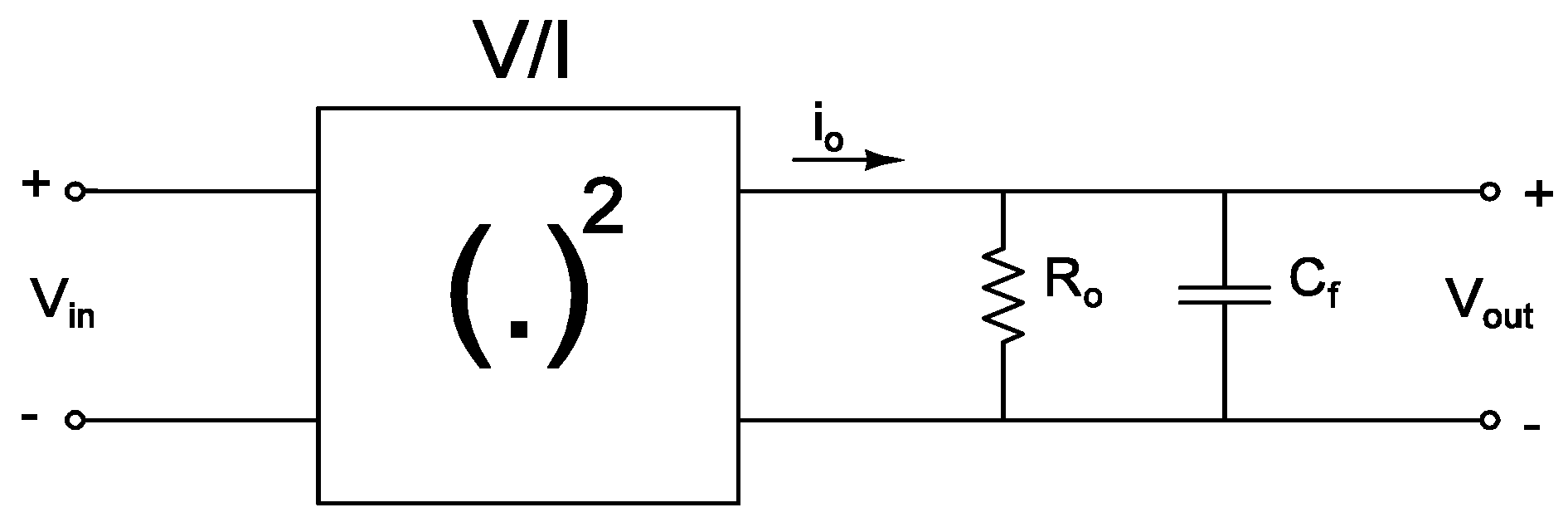
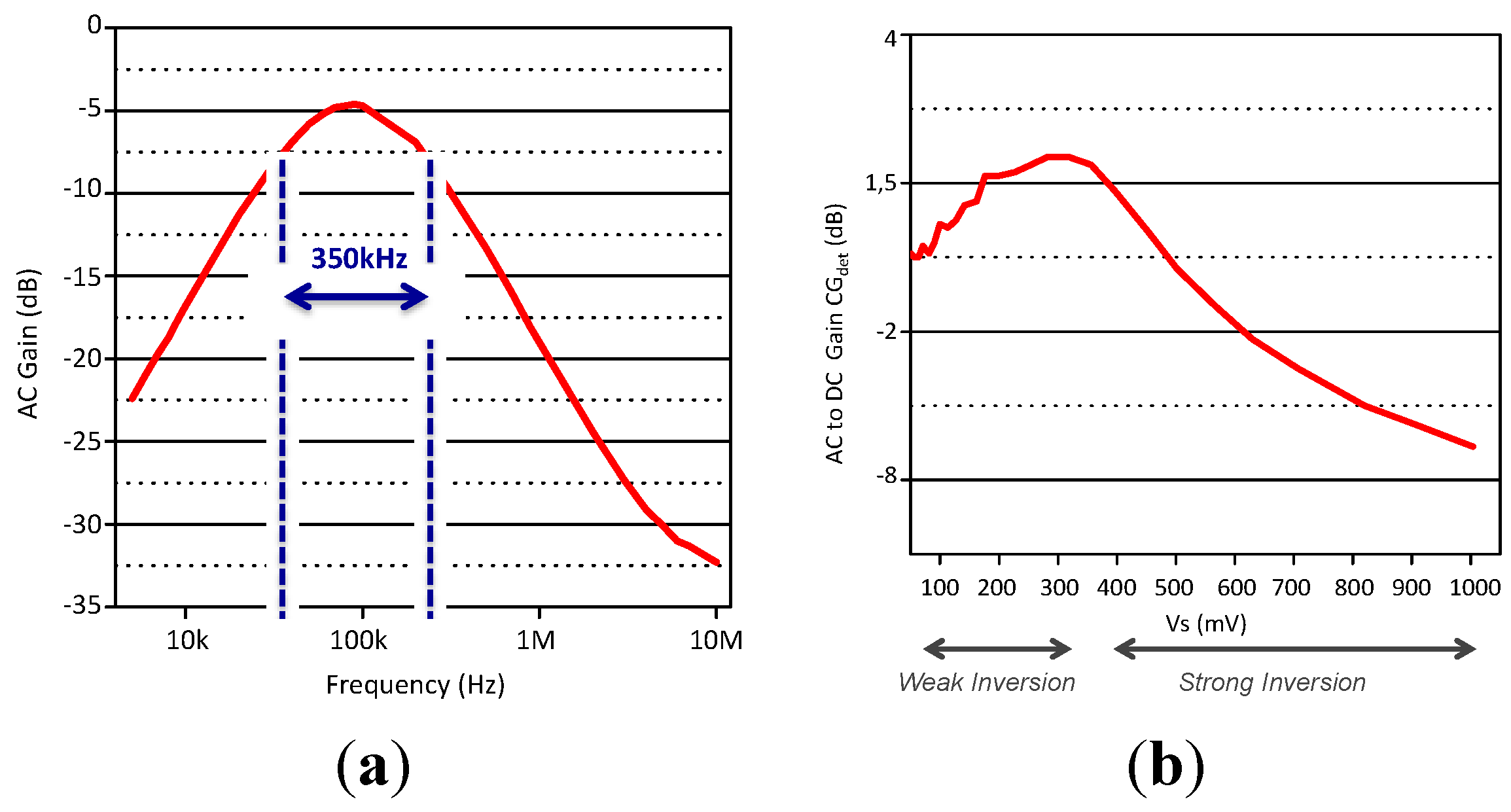
4. Characterization of MOOD Demodulator

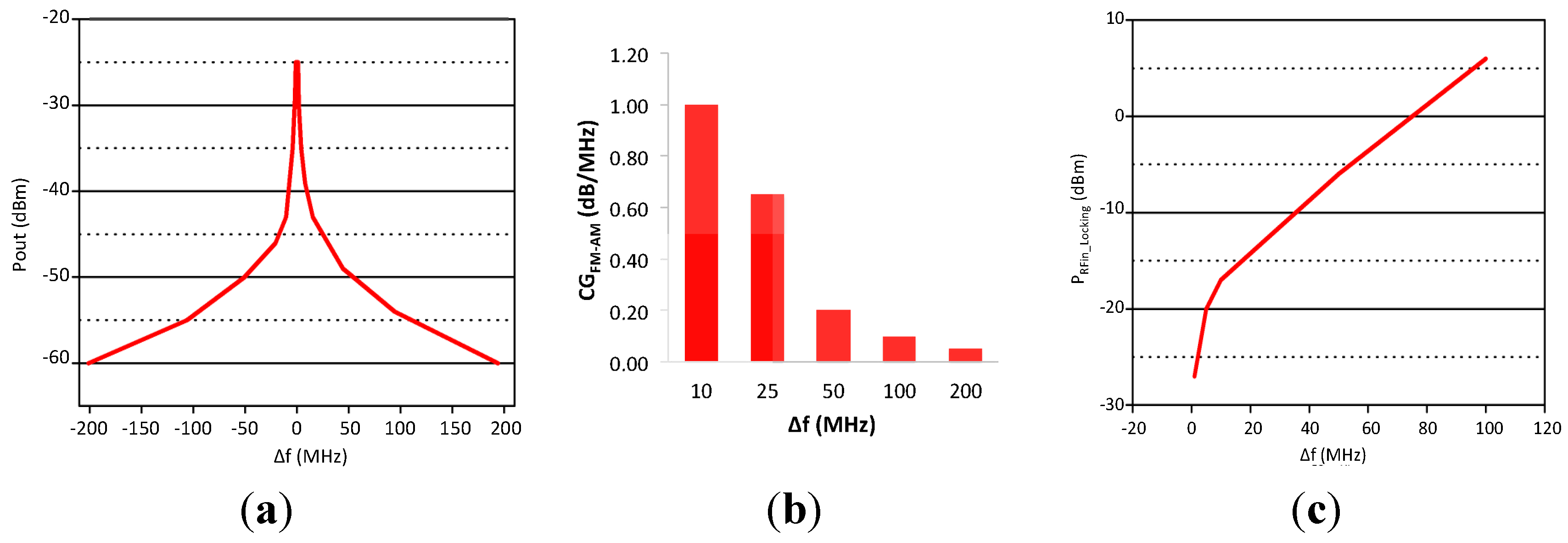
4.1. Demodulation of Amplitude and Frequency Modulated Signals
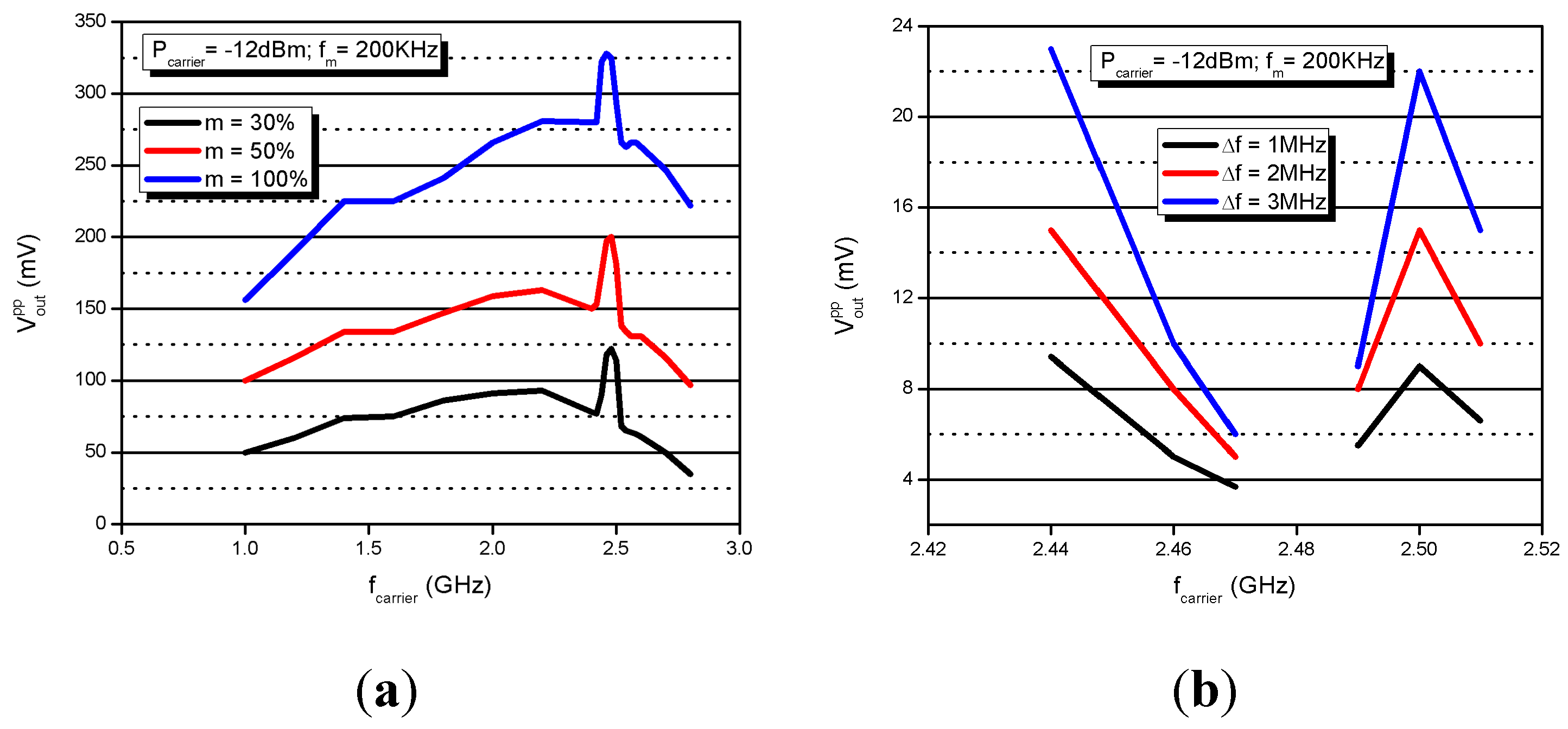
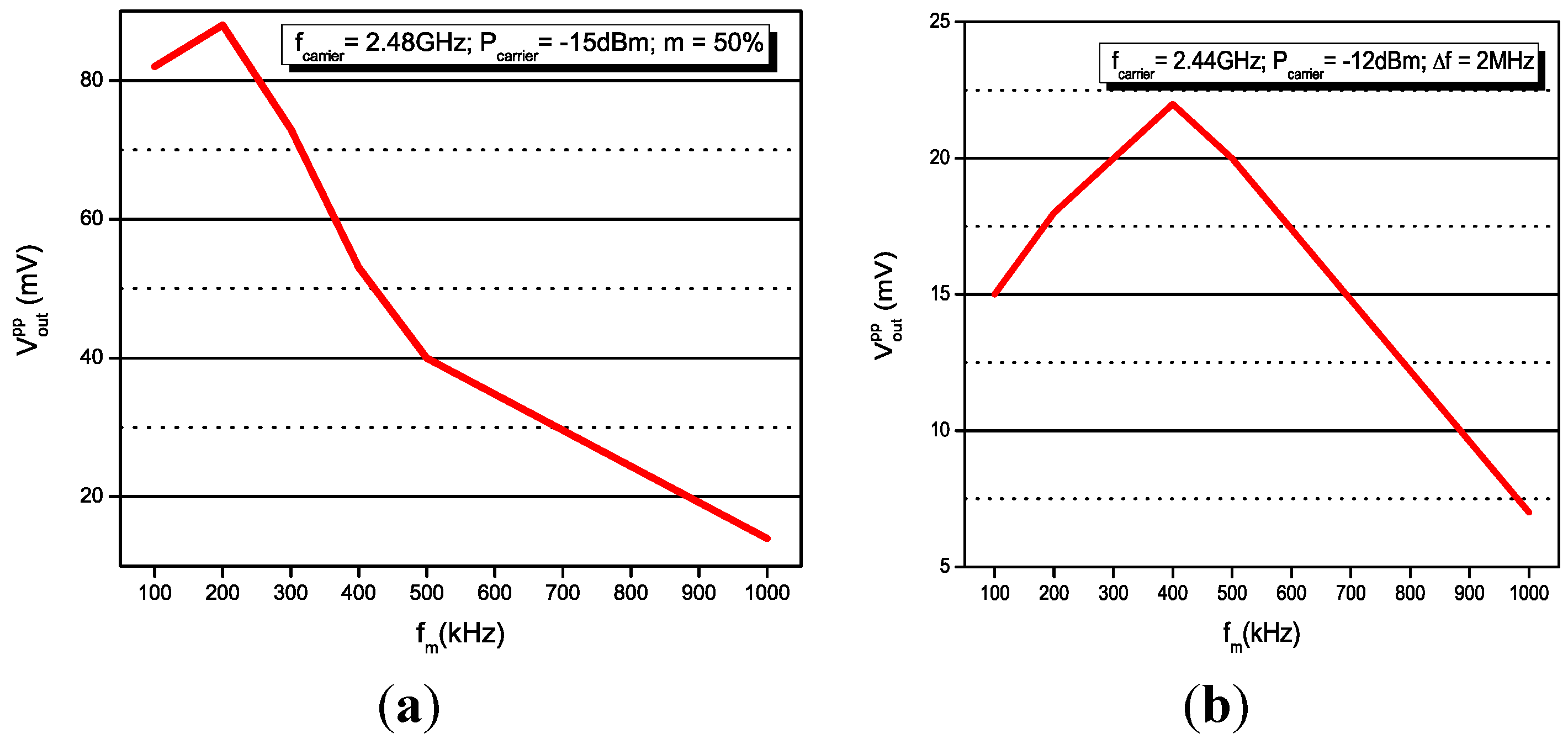
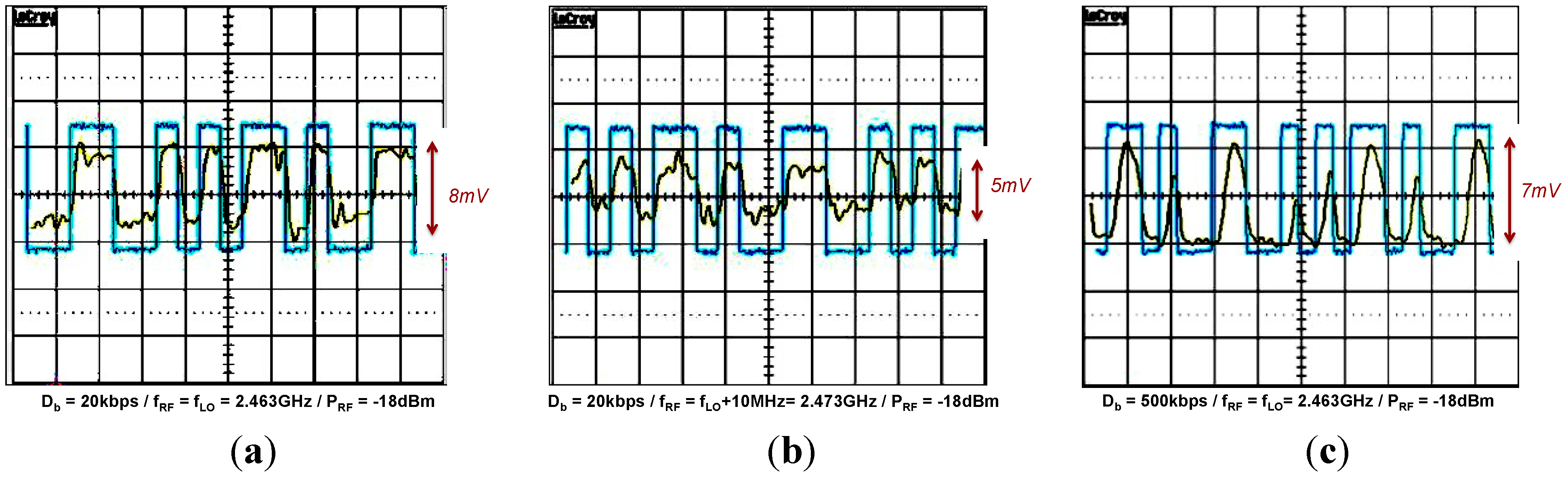
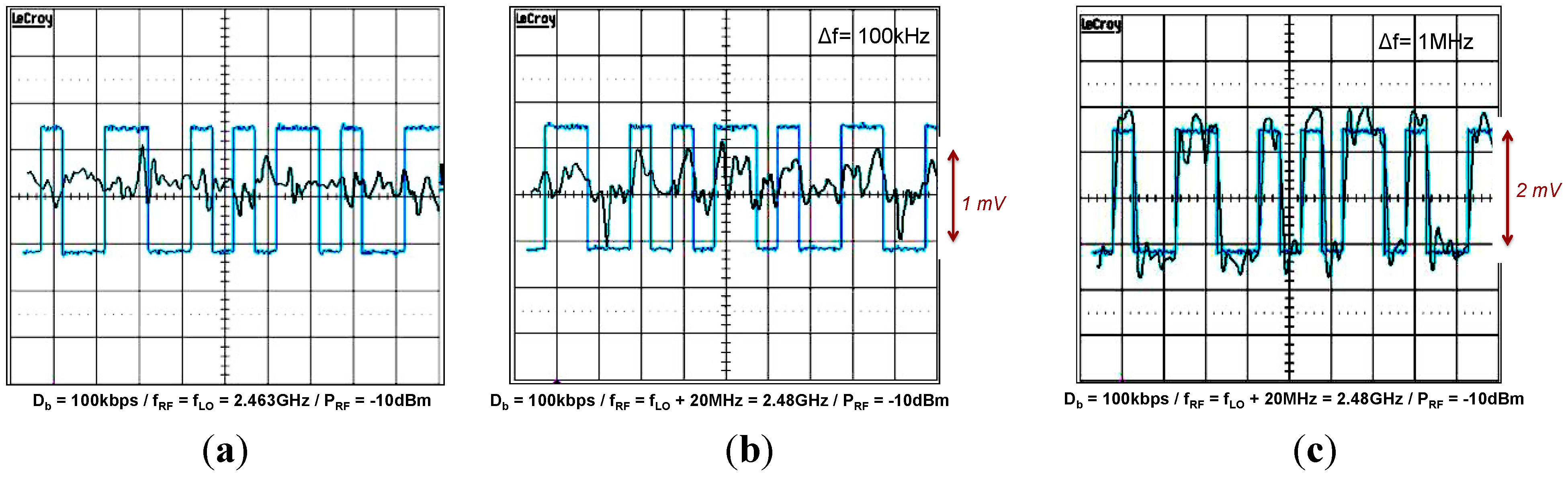
4.2. FSK and OOK Demodulation
| This Work | [15] | [4] | [16] | [17] | ||
|---|---|---|---|---|---|---|
| Process | 130 nm | 130 nm | 90 nm | 180 nm | 180 nm | |
| Frequency | 2.4 GHz | 915 MHz | 2 GHz | 2.4 GHz | 80 MHz | |
| Power Consumption | 120 μW | 0.1 μW | 52 μW | 10 μW | 45 μW | |
| VDD | 0.6 V | 1.2 V | 0.5 V | 0.5 V | 0.7 V | |
| Modulation | OOK | FSK | OOK | OOK | OOK | FSK |
| Data rate | 150 kbps | 300 kbps | 100 kbps | 100 kbps | 100 kbps | 300 kbps |
| Sensitivity | −36 dBm | −27 dBm | −41 dBm | −72 dBm | −65 dBm | −62 dBm |
5. Conclusions
Acknowledgments
Conflicts of Interest
References
- Lin, E.Y.; Rabaey, J.; Wolisz, A. Power-efficient rendezvous schemes for dense wireless sensor networks. Proc. IEEE Int. Conf. Commun. 2004, 7, 3769–3776. [Google Scholar]
- Vidojkovic, M.; Rampu, S.; Imamura, K.; Harpe, P.; Dolmans, G.; de Groot, H. A 500 μW 5 Mbps ULP super-regenerative RF Front-End. In Proceedings of the IEEE Solid-State Circuits Conference Digest of Technical Papers, San Francisco, CA, USA, 7–11 February 2010; pp. 222–223.
- Huang, X.; Rampu, S.; Wang, X.Y.; Dolmans, G.; de Groot, H. A 2.4 GHz/915 MHz 51 μW wake-up receiver with offset and noise suppression. In Proceedings of the European Solid State Circuits Conference, Seville, Spain, 13–17 September 2010; pp. 462–475.
- Pletcher, N.; Gambini, S.; Rabaey, J.M. A 2 GHz 52 μW Wake-Up Receiver with −72 dBm Sensitivity Using Uncertain-IF Architecture. IEEE J. Solid State Circuits 2009, 44, 269–280. [Google Scholar] [CrossRef]
- Lont, M.; Milosevic, D.; van Roermund, A.H.M.; Dolmans, G. Ultra-Low Power FSK Wake-up Receiver Front-End for Body Area Networks. In Proceedings of the IEEE Radio-Frequency Integrated Circuit Symposium, Baltimore, MD, USA, 5–7 June 2011; pp. 1–4.
- Razavi, B. A study of injection locking and pulling in oscillators. IEEE J. Solid State Circuits 2004, 39, 1415–1424. [Google Scholar] [CrossRef]
- Adler, R. A study of locking phenomena in oscillators. Proc. IEEE 1973, 61, 1380–1385. [Google Scholar] [CrossRef]
- Kurokawa, K. Injection locking of microwave solid-state oscillators. Proc. IEEE 1973, 61, 1386–1410. [Google Scholar] [CrossRef]
- Paciorek, L.J. Injection locking of oscillators. Proc. IEEE 1965, 53, 1723–1727. [Google Scholar] [CrossRef]
- Stover, H.L. Theoretical explanation for the output spectra of unlocked driven oscillators. Proc. IEEE 1966, 54, 310–311. [Google Scholar] [CrossRef]
- Armand, M. On the output spectrum of unlocked driven oscillators. Proc. IEEE 1969, 57, 798–799. [Google Scholar] [CrossRef]
- Pletcher, N.M. Ultra-low power wake-up receivers for Wireless Sensor Networks. Ph.D. Thesis, University of California, Berkeley, CA, USA, May 2008. [Google Scholar]
- Meyer, R.G. Low-power monolithic RF peak detector analysis. IEEE J. Solid State Circuits 1995, 30, 65–67. [Google Scholar] [CrossRef]
- Gray, P.R. Analysis and Design of Analog Integrated Circuits; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Roberts, N.E.; Wentzloff, D.D. A 98 nW Wake-Up Radio for Wireless Body Area Networks. In Proceedings of the IEEE Radio Frequency Integrated Circuits Symposium, Montreal, QC, Canada, 17–19 June 2012; pp. 373–376.
- Cheng, K.W.; Liu, X.; Je, M. A 2.4/5.8 GHz 10 μW Wake-Up Receiver woth −65/−50 dBm Sensitivity using direct active RF detection. In Proceeding of the IEEE Asian Solid-State Circuits Conference, Kobe, Japan, 12–14 November 2012; pp. 337–340.
- Bae, J.; Yoo, H.J. A 45 μW Injected Locked FSK Wake-Up Receiver with Frequency-to-Envelope Conversion for Crystal-less Wireless Body Area Network. IEEE J. Solid-State Circuits 2015, 50, 1351–1360. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taris, T.; Kraimia, H.; Belot, D.; Deval, Y. An FSK and OOK Compatible RF Demodulator for Wake Up Receivers. J. Low Power Electron. Appl. 2015, 5, 274-290. https://doi.org/10.3390/jlpea5040274
Taris T, Kraimia H, Belot D, Deval Y. An FSK and OOK Compatible RF Demodulator for Wake Up Receivers. Journal of Low Power Electronics and Applications. 2015; 5(4):274-290. https://doi.org/10.3390/jlpea5040274
Chicago/Turabian StyleTaris, Thierry, Hassène Kraimia, Didier Belot, and Yann Deval. 2015. "An FSK and OOK Compatible RF Demodulator for Wake Up Receivers" Journal of Low Power Electronics and Applications 5, no. 4: 274-290. https://doi.org/10.3390/jlpea5040274
APA StyleTaris, T., Kraimia, H., Belot, D., & Deval, Y. (2015). An FSK and OOK Compatible RF Demodulator for Wake Up Receivers. Journal of Low Power Electronics and Applications, 5(4), 274-290. https://doi.org/10.3390/jlpea5040274





