Analog Filters Design for Improving Precision in Proton Sound Detectors
Abstract
1. Introduction
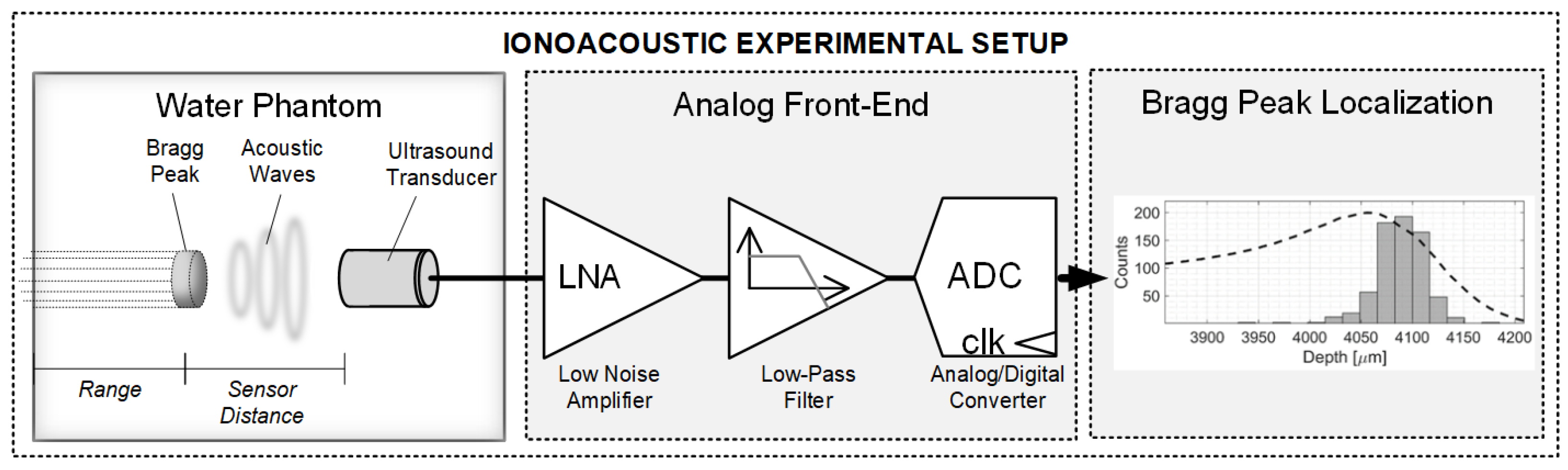
2. Ionoacoustic Experimental Setup and Scenarios
3. Factors Influencing Ionoacoustic Measurement Precision
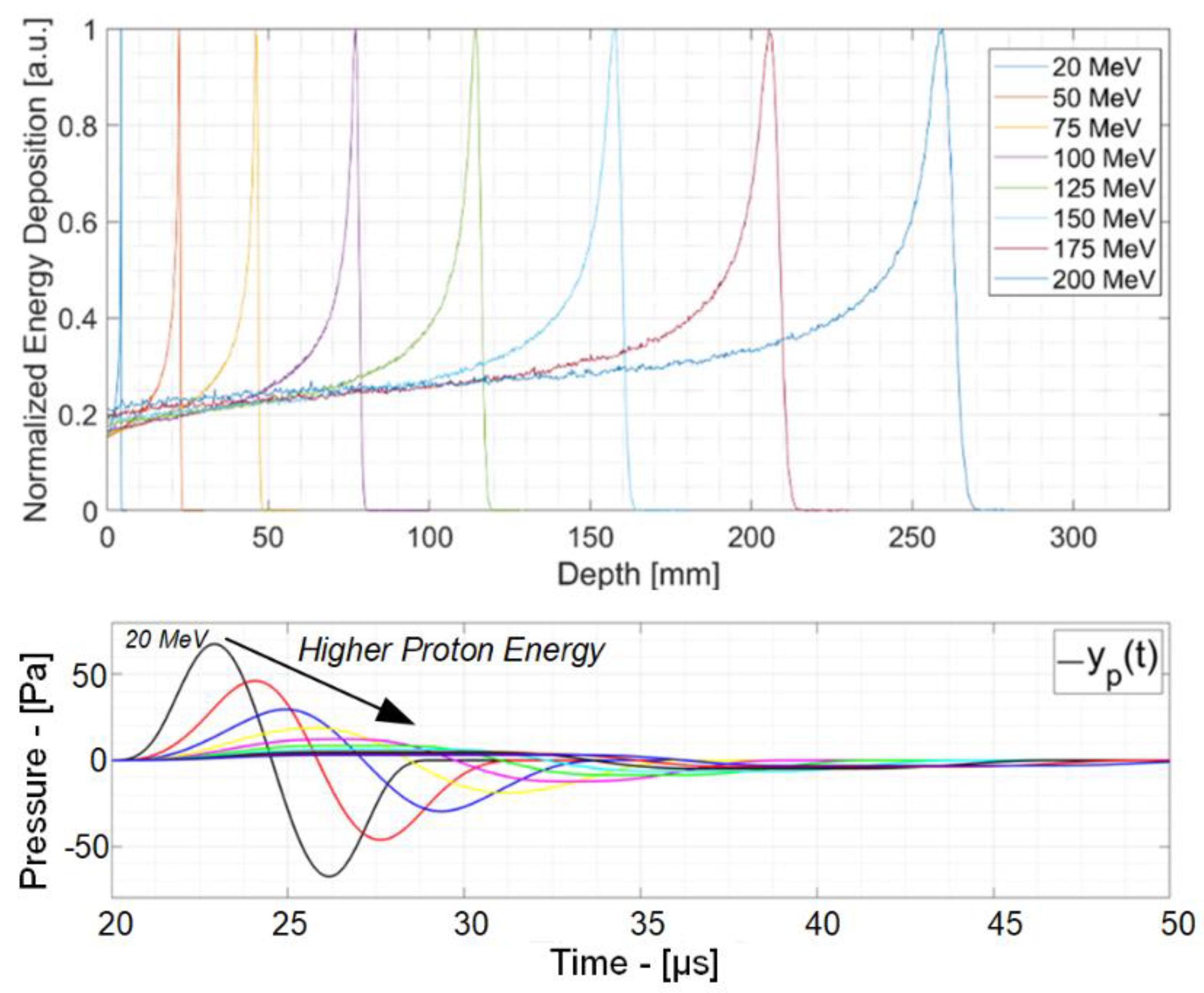
3.1. Signal Frequency
3.2. Signal-to-Noise Ratio
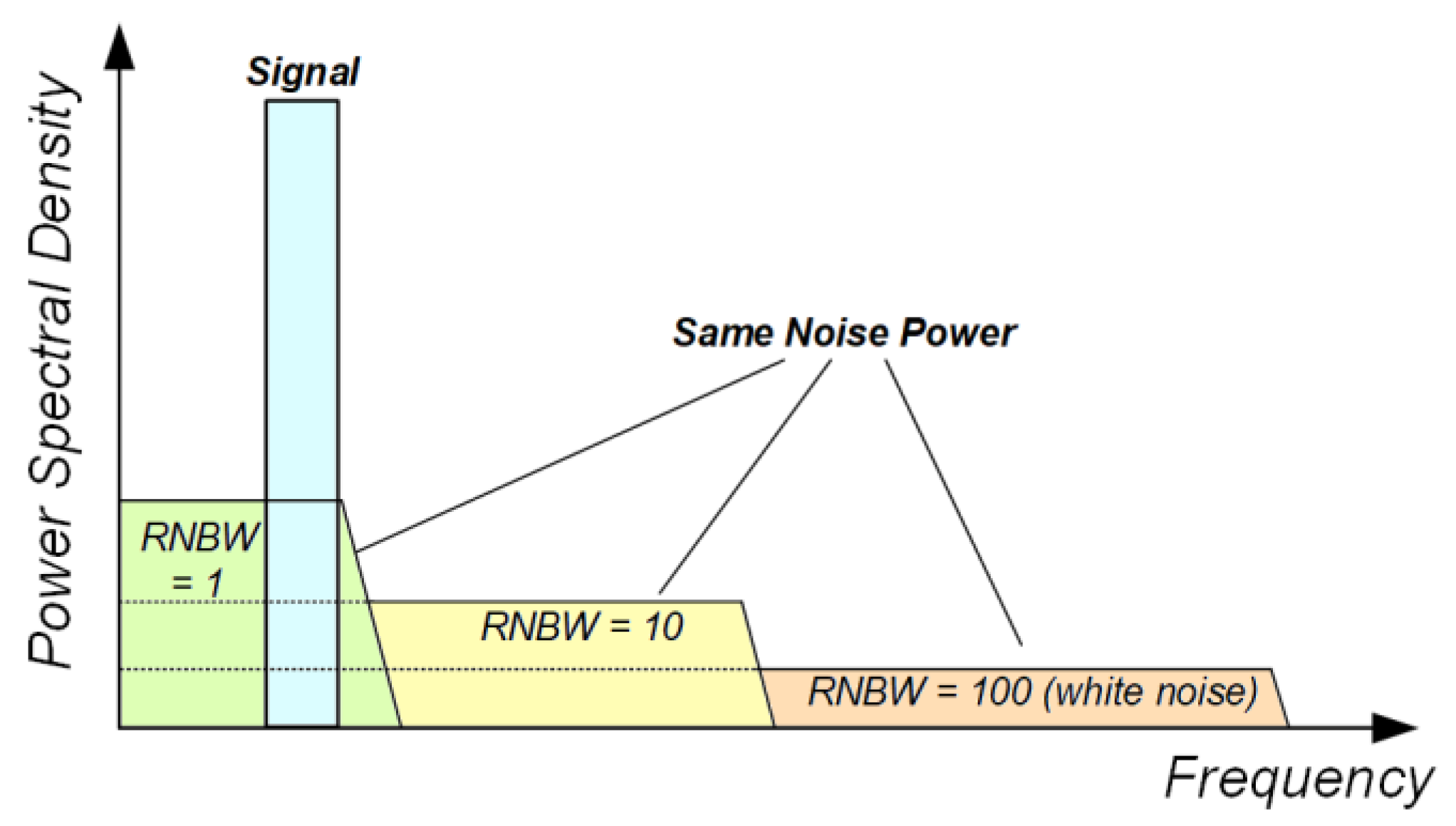
3.3. Relative Noise Bandwidth
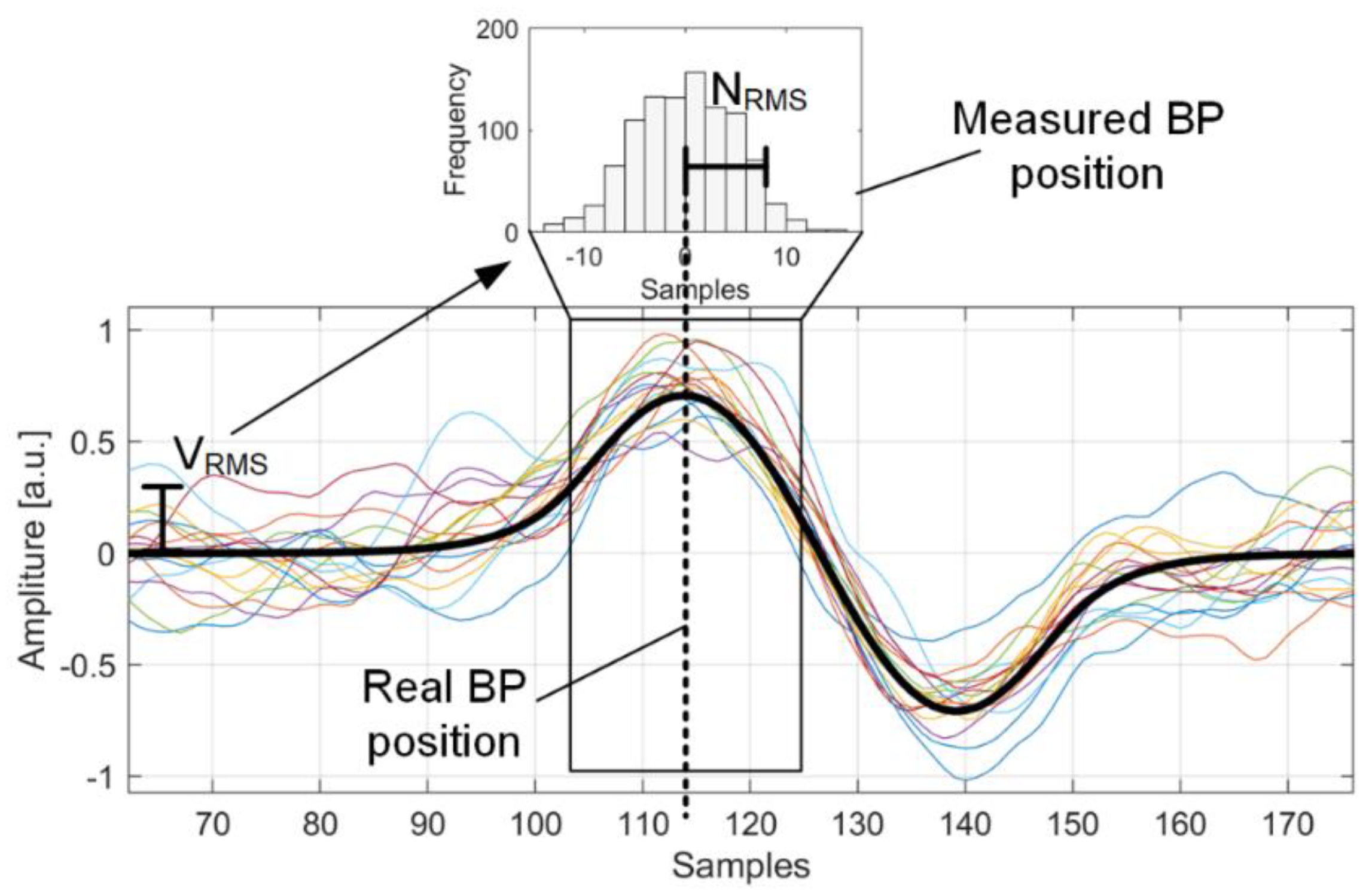
3.4. Ionoacoustic Relative Precision
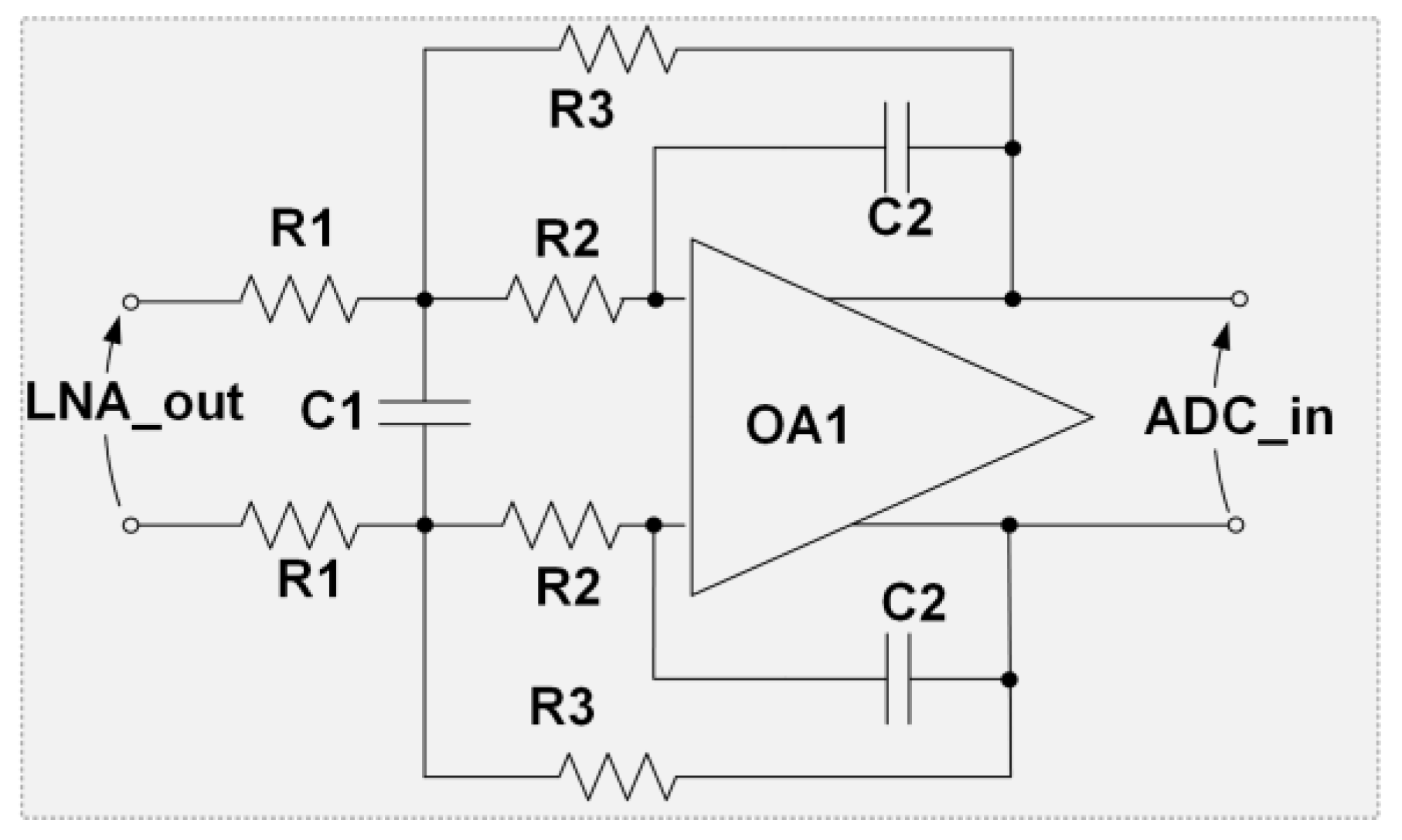
4. Analog Filter Design for 200 MeV Proton Sound Detector
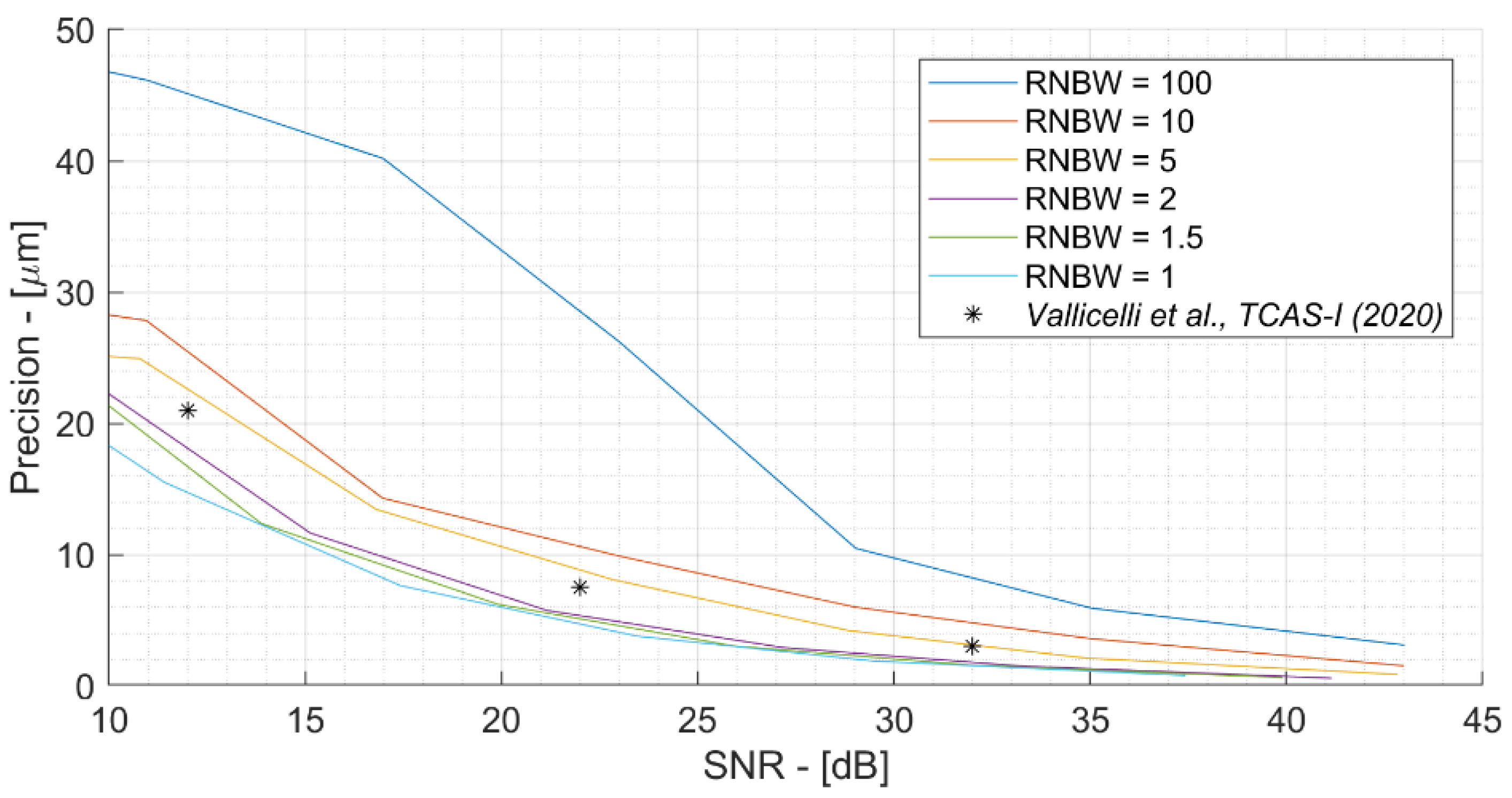
5. Results—Analog Filter Design Dose Reduction at 200 MeV
- ▪
- The band on which the noise is integrated is limited by a factor of 10, resulting in a 10 dB lower noise power and consequently an SNR increase by the same amount (top of Figure 11).
- ▪
- The reduction of the RNBW allows one to obtain the same precision (for example, of 0.5 mm in the bottom of Figure 11) with an 8 dB lower SNR.
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Knoll, G.F. Radiation Sources. In Radiation Detection and Measurement; John Wiley & Sons: Hoboken, NJ, USA, 2000; pp. 1–28. [Google Scholar]
- Parodi, K.; Polf, J.C. In vivo range verification in particle therapy. Med. Phys. 2018, 45, e1036–e1050. [Google Scholar] [CrossRef] [PubMed]
- Min, C.-H.; Kim, C.H.; Youn, M.-Y.; Kim, J.-W. Prompt gamma measurements for locating the dose falloff region in the proton therapy. Appl. Phys. Lett. 2006, 89, 183517. [Google Scholar] [CrossRef]
- Mirandola, A.; Molinelli, S.; Freixas, G.V.; Mairani, A.; Gallio, E.; Panizza, D.; Russo, S.; Ciocca, M.; Donetti, M.; Magro, G.; et al. Dosimetric commissioning and quality assurance of scanned ion beams at the Italian National Center for Oncological Hadrontherapy. Radiat. Meas. Phys. 2015, 42, 5287–5300. [Google Scholar] [CrossRef] [PubMed]
- Sulak, L.; Armstrong, T.; Baranger, H.; Bregman, M.; Levi, M.; Mael, D.; Strait, J.; Bowen, T.; Pifer, A.; Polakos, P.; et al. Experimental studies of the acoustic signature of proton beams traversing fluid media. Nucl. Instrum. Methods 1979, 161, 203–217. [Google Scholar] [CrossRef]
- Hayakawa, Y.; Tada, J.; Arai, N.; Hosono, K.; Sato, M.; Wagai, T.; Tsuji, H.; Tsujii, H. Acoustic pulse generated in a patient during treatment by pulsed proton radiation beam. Radiat. Oncol. Investig. 1995, 3, 42–45. [Google Scholar] [CrossRef]
- Assmann, W.; Kellnberger, S.; Reinhardt, S.; Lehrack, S.; Edlich, A.; Thirolf, P.G.; Moser, M.; Dollinger, G.; Omar, M.; Ntziachristos, V.; et al. Ionoacoustic characterization of the proton Bragg peak with sub-millimeter precision. Med. Phys. 2015, 42, 567–574. [Google Scholar] [CrossRef] [PubMed]
- Lehrack, S.; Assmann, W.; Bender, M.; Severin, D.; Trautmann, C.; Schreiber, J.; Parodi, K. Ionoacoustic detection of swift heavy ions. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2020, 950, 162935. [Google Scholar] [CrossRef]
- Patch, S.K.; Covo, M.K.; Jackson, A.; Qadadha, Y.M.; Campbell, K.S.; Albright, R.A.; Bloemhard, P.; Donoghue, A.P.; Siero, C.R.; Gimpel, T.L.; et al. Thermoacoustic range verification using a clinical ultrasound array provides perfectly co-registered overlay of the Bragg peak onto an ultrasound image. Phys. Med. Biol. 2016, 61, 5621–5638. [Google Scholar] [CrossRef] [PubMed]
- Jones, K.C.; Stappen, F.V.; Sehgal, C.M.; Avery, S. Acoustic time-of-flight for proton range verification in water. Med. Phys. 2016, 43, 5213–5224. [Google Scholar] [CrossRef] [PubMed]
- Riva, M.; Vallicelli, E.A.; Baschirotto, A.; De Matteis, M. Acoustic Analog Front End for Proton Range Detection in Hadron Therapy. IEEE Trans. Biomed. Circuits Syst. 2018, 12, 954–962. [Google Scholar] [CrossRef] [PubMed]
- Vallicelli, E.A.; Turossi, D.; Gelmi, L.; Baù, A.; Bertoni, R.; Fulgione, W.; Quintino, A.; Corcione, M.; Baschirotto, A.; De Matteis, M. A 0.3 nV/√ Hz Input-Referred-Noise Analog Front-End for Radiation-Induced Thermo-Acoustic Pulses. Integration 2020, 74, 11–18. [Google Scholar] [CrossRef]
- Vallicelli, E.A.; Baschirotto, A.; Lehrack, S.; Assmann, W.; Parodi, K.; Viola, S.; Riccobene, G.; De Matteis, M. 22 dB Signal-to-Noise Ratio Real-Time Proton Sound Detector for Experimental Beam Range Verification. IEEE Trans. Circuits Syst. I Regul. Pap. 2021, 68, 3–13. [Google Scholar] [CrossRef]
- Baschirotto, A.; D’Amico, S.; De Matteis, M. Advances on analog filters for telecommunications. In Proceedings of the 2006 Advanced Signal Processing, Circuit and System Design Techniques for Communications, Kos, Greece, 21–24 May 2006; pp. 131–168. [Google Scholar]
- De Matteis, M.; D’Amico, S.; Baschirotto, A. Power-minimization design procedure for Rauch biquadratic cells. In Proceedings of the 2006 Ph. D. Research in Microelectronics and Electronics, Otranto, Italy, 12–15 June 2006; pp. 141–144. [Google Scholar]





| Parameter | Value |
|---|---|
| Particle beam energy | 200 MeV |
| Dose per shot | 2 mGy |
| Signal frequency | 13 kHz |
| Signal amplitude at Bragg peak | 200 mPa |
| Signal amplitude at sensor | 2 mPa |
| Acoustic sensor distance | 7.5 cm |
| Acoustic sensor sensitivity | 4 mV/Pa |
| Acoustic sensor bandwidth | 130 kHz |
| Sensor noise power | 4.5 μVRMS |
| Sensor noise power spectral density | 12 nV/√Hz |
| LNA in-band gain | 60 dB |
| Parameter | Value |
|---|---|
| −3 dB cut frequency | 13 kHz |
| Quality factor | 0.707 |
| Input Referred Noise Power (0–13 kHz) | 13 μVRMS |
| Input Referred Noise Power Spectral Density @13 kHz | 100 nV/√Hz |
| Relative Noise Bandwidth (RNBW) | 1 |
| R1, R2, R3 | 44.2 kΩ |
| C1 | 294 pF |
| C2 | 130 pF |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vallicelli, E.A.; De Matteis, M. Analog Filters Design for Improving Precision in Proton Sound Detectors. J. Low Power Electron. Appl. 2021, 11, 12. https://doi.org/10.3390/jlpea11010012
Vallicelli EA, De Matteis M. Analog Filters Design for Improving Precision in Proton Sound Detectors. Journal of Low Power Electronics and Applications. 2021; 11(1):12. https://doi.org/10.3390/jlpea11010012
Chicago/Turabian StyleVallicelli, Elia Arturo, and Marcello De Matteis. 2021. "Analog Filters Design for Improving Precision in Proton Sound Detectors" Journal of Low Power Electronics and Applications 11, no. 1: 12. https://doi.org/10.3390/jlpea11010012
APA StyleVallicelli, E. A., & De Matteis, M. (2021). Analog Filters Design for Improving Precision in Proton Sound Detectors. Journal of Low Power Electronics and Applications, 11(1), 12. https://doi.org/10.3390/jlpea11010012







