Micromechanical Modeling of Anisotropy and Strain Rate Dependence of Short-Fiber-Reinforced Thermoplastics
Abstract
:1. Introduction
2. Micromechanical Modeling Approach
2.1. Representative Volume Elements
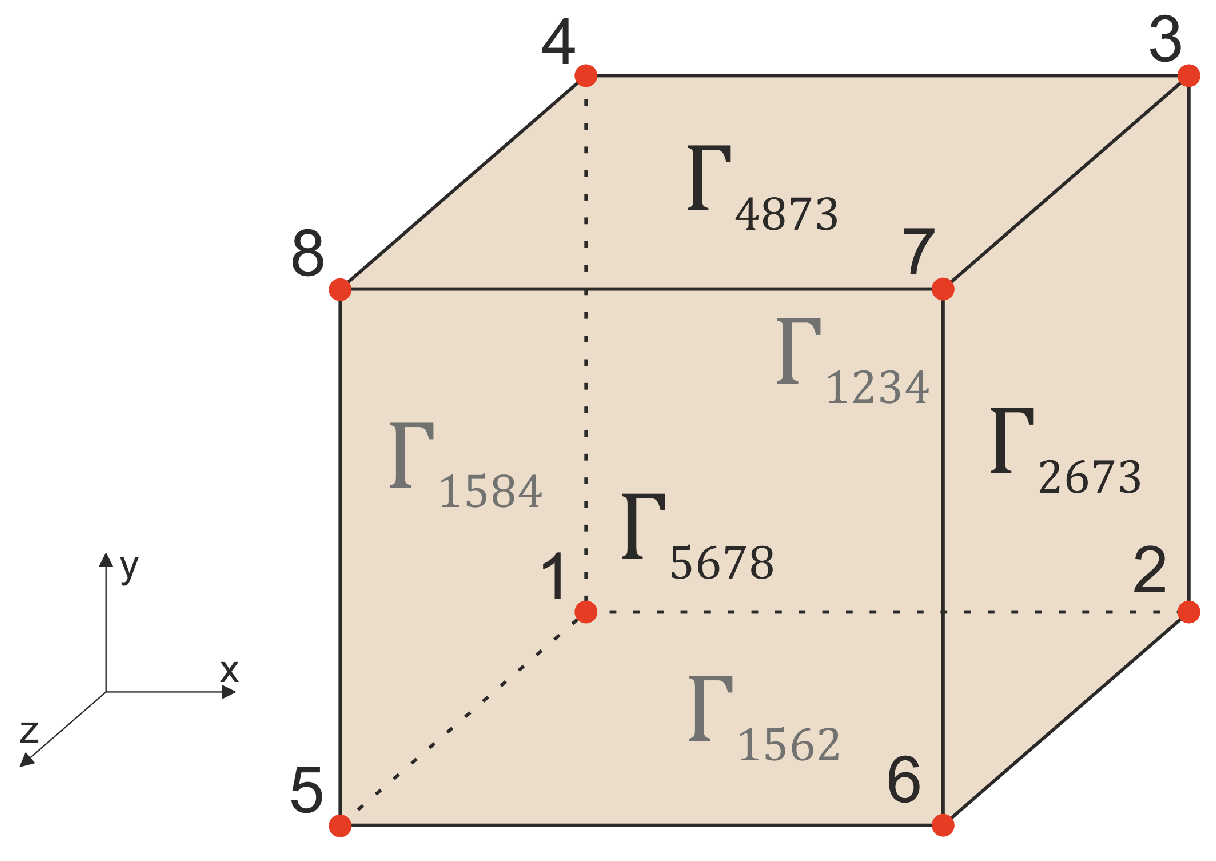
2.2. Periodic Boundary Condition
2.3. The Strain Rate-Dependent EGP Model
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| (MPa) | (MPa) | (MPa) | (-) | (-) | (-) | (-) | (-) |
|---|---|---|---|---|---|---|---|
| 26 | 3750 | 28 | 50 |
| Modes | (MPa) | (MPa · s) |
|---|---|---|
| 1 | ||
| 2 | ||
| 3 | ||
| 4 | ||
| 5 | ||
| 6 | ||
| 7 | ||
| 8 | ||
| 9 | ||
| 10 | ||
| 11 | ||
| 12 | ||
| 13 | ||
| 14 | ||
| 15 | ||
| 16 | ||
| 17 |
References
- Ishikawa, T.; Amaoka, K.; Masubuchi, Y.; Yamamoto, T.; Yamanaka, A.; Arai, M.; Takahashi, J. Overview of automotive structural composites technology developments in Japan. Compos. Sci. Technol. 2018, 155, 221–246. [Google Scholar] [CrossRef]
- Bernasconi, A.; Cosmi, F.; Hine, P.J. Analysis of fibre orientation distribution in short fibre reinforced polymers: A comparison between optical and tomographic methods. Compos. Sci. Technol. 2012, 72, 2002–2008. [Google Scholar] [CrossRef]
- Kim, E.G.; Park, J.K.; Jo, S.H. A study on fiber orientation during the injection molding of fiber-reinforced polymeric composites: (Comparison between image processing results and numerical simulation). J. Mater. Process. Technol. 2001, 111, 225–232. [Google Scholar] [CrossRef]
- Sedighiamiri, A.; van Erp, T.; Cathelin, J.; Brands, D. Validation methodology for accurate prediction of anisotropic mechanics in fiber reinforced thermoplastic (FRTP) materials. In Proceedings of the 20th International Conference on Composite Materials, Copenhagen, Denmark, 19–24 July 2015. [Google Scholar]
- Davies, R.G.; Magee, C.L. The effect of strain-rate upon the tensile deformation of materials. J. Eng. Mater. Technol. 1975, 97, 151–155. [Google Scholar] [CrossRef]
- Okoli, O.I. The effects of strain rate and failure modes on the failure energy of fibre reinforced composites. Compos. Struct. 2001, 54, 299–303. [Google Scholar] [CrossRef]
- Vashchenko, A.; Spiridonova, I.; Sukhovaya, E. Deformation and fracture of structural materials under high-rate strain. Metalurgija 2000, 39, 89–92. [Google Scholar]
- Li, R.K.Y.; Lu, S.N.; Choy, C.L. Tensile and compressive deformation of a short-glass-fiber-reinforced liquid crystalline polymer. J. Thermoplast. Compos. Mater. 1995, 8, 304–322. [Google Scholar] [CrossRef] [Green Version]
- Goldberg, R.K.; Stouffer, D.C. Strain rate dependent analysis of a polymer matrix composite utilizing a micromechanics approach. J. Compos. Mater. 2002, 36, 773–793. [Google Scholar] [CrossRef]
- Govaert, L.E.; van der Vegt, A.K.; van Drongelen, M. Polymers: From Structure to Properties; Delft Academic Press: Delft, The Netherlands, 2020; Chapter 7. [Google Scholar]
- Loo, L.S.; Cohen, R.E.; Gleason, K.K. Chain mobility in the amorphous region of nylon 6 observed under active uniaxial deformation. Science 2000, 288, 116–119. [Google Scholar] [CrossRef]
- Pastukhov, L.V.; and Govaert, L.E. Plasticity-controlled failure of fibre-reinforced thermoplastics. Compos. Part B Eng. 2021, 209, 108635. [Google Scholar] [CrossRef]
- Pastukhov, L.V.; Kanters, M.J.; Engels, T.A.; Govaert, L.E. Influence of fiber orientation, temperature and relative humidity on the long-term performance of short glass fiber reinforced polyamide 6. J. Appl. Polym. Sci. 2021, 138, 50382. [Google Scholar] [CrossRef]
- Amiri-Rad, A.; Wismans, M.; Pastukhov, L.V.; Govaert, L.E.; van Dommelen, J.A.W. Constitutive modeling of injection-molded short-fiber composites: Characterization and model application. J. Appl. Polym. Sci. 2020, 137, 49248. [Google Scholar] [CrossRef]
- Benveniste, Y. A new approach to the application of Mori-Tanaka’s theory in composite materials. Mech. Mater. 1987, 6, 147–157. [Google Scholar] [CrossRef]
- Mori, T.; Tanaka, K. Average stress in matrix and average elastic energy of materials with misfitting inclusions. Acta Metall. 1973, 21, 571–574. [Google Scholar] [CrossRef]
- Camacho, C.W.; Tucker, C.L., III; Yalvaç, S.; McGee, R.L. Stiffness and thermal expansion predictions for hybrid short fiber composites. Polym. Compos. 1990, 11, 229–239. [Google Scholar] [CrossRef]
- Lusti, H.R.; Gusev, A.A. Finite element predictions for the thermoelastic properties of nanotube reinforced polymers. Model. Simul. Mater. Sci. Eng. 2004, 12, S107. [Google Scholar] [CrossRef]
- Pierard, O.; Friebel, C.; Doghri, I. Mean-field homogenization of multi-phase thermo-elastic composites: A general framework and its validation. Compos. Sci. Technol. 2004, 64, 1587–1603. [Google Scholar] [CrossRef]
- Krairi, A.; Doghri, I.; Robert, G. Multiscale high cycle fatigue models for neat and short fiber reinforced thermoplastic polymers. Int. J. Fatigue 2016, 92, 179–192. [Google Scholar] [CrossRef]
- Yim, S.O.; Lee, W.J.; Cho, D.H.; Park, I.M. Finite element analysis of compressive behavior of hybrid short fiber/particle/mg metal matrix composites using RVE model. Met. Mater. Int. 2015, 21, 408–414. [Google Scholar] [CrossRef]
- Mortazavi, B.; Baniassadi, M.; Bardon, J.; Ahzi, S. Modeling of two-phase random composite materials by finite element, Mori–Tanaka and strong contrast methods. Compos. Part B Eng. 2013, 45, 1117–1125. [Google Scholar] [CrossRef]
- Ali, D.; Sen, S. Finite element analysis of the effect of boron nitride nanotubes in beta tricalcium phosphate and hydroxyapatite elastic modulus using the RVE model. Compos. Part B Eng. 2016, 90, 336–340. [Google Scholar] [CrossRef]
- Goldberg, R.K.; Roberts, G.D.; Gilat, A. Implementation of an associative flow rule including hydrostatic stress effects into the high strain rate deformation analysis of polymer matrix composites. J. Aerosp. Eng. 2005, 18, 18–27. [Google Scholar] [CrossRef]
- Tabiei, A.; Aminjikarai, S.B. A strain-rate dependent micro-mechanical model with progressive post-failure behavior for predicting impact response of unidirectional composite laminates. Compos. Struct. 2009, 88, 65–82. [Google Scholar] [CrossRef]
- Haward, R.N.; Thackray, G. The use of a mathematical model to describe isothermal stress–strain curves in glassy thermoplastics. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1968, 302, 453–472. [Google Scholar]
- Boyce, M.C.; Parks, D.M.; Argon, A.S. Large inelastic deformation of glassy polymers. Part I: rate dependent constitutive model. Mech. Mater. 1988, 7, 15–33. [Google Scholar] [CrossRef]
- Arruda, E.M.; Boyce, M.C. Evolution of plastic anisotropy in amorphous polymers during finite straining. Int. J. Plast. 1993, 9, 697–720. [Google Scholar] [CrossRef]
- Hasan, O.A.; Boyce, M.C.; Li, X.S.; Berko, S. An investigation of the yield and postyield behavior and corresponding structure of poly (methyl methacrylate). J. Polym. Sci. Part B Polym. Phys. 1993, 31, 185–197. [Google Scholar] [CrossRef]
- Buckley, C.P.; Jones, D.C. Glass-rubber constitutive model for amorphous polymers near the glass transition. Polymer 1995, 36, 3301–3312. [Google Scholar] [CrossRef]
- Buckley, C.P.; Dooling, P.J.; Harding, J.; Ruiz, C. Deformation of thermosetting resins at impact rates of strain. Part 2: Constitutive model with rejuvenation. J. Mech. Phys. Solids 2004, 52, 2355–2377. [Google Scholar] [CrossRef]
- Klompen, E.T.J.; Engels, T.A.P.; Govaert, L.E.; Meijer, H.E.H. Modeling of the postyield response of glassy polymers: Influence of thermomechanical history. Macromolecules 2005, 38, 6997–7008. [Google Scholar] [CrossRef] [Green Version]
- van Breemen, L.C.A.; Klompen, E.T.J.; Govaert, L.E.; Meijer, H.E.H. Extending the EGP constitutive model for polymer glasses to multiple relaxation times. J. Mech. Phys. Solids 2011, 59, 2191–2207. [Google Scholar] [CrossRef]
- Advani, S.G.; Tucker, C.L., III. The use of tensors to describe and predict fiber orientation in short fiber composites. J. Rheol. 1987, 31, 751–784. [Google Scholar] [CrossRef]
- Digimat, The Material Modeling Platform. Available online: https://www.e-xstream.com/ (accessed on 25 May 2021).
- Kouznetsova, V.G.; Geers, M.G.D.; Brekelmans, W.A.M. Multi-scale second-order computational homogenization of multi-phase materials: A nested finite element solution strategy. Comput. Methods Appl. Mech. Eng. 2004, 193, 5525–5550. [Google Scholar] [CrossRef]
- Bauwens-Crowet, C.; Bauwens, J.C.; Homes, G. The temperature dependence of yield of polycarbonate in uniaxial compression and tensile tests. J. Mater. Sci. 1972, 7, 176–183. [Google Scholar] [CrossRef]
- Hill, R. A theory of the yielding and plastic flow of anisotropic metals. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1948, 193, 281–297. [Google Scholar]
- Bay, R.S.; Tucker, C.L., III. Fiber orientation in simple injection moldings. Part I: Theory and numerical methods. Polym. Compos. 1992, 13, 317–331. [Google Scholar] [CrossRef]
- Bay, R.S.; Tucker, C.L., III. Fiber orientation in simple injection moldings. Part II: Experimental results. Polym. Compos. 1992, 13, 332–341. [Google Scholar] [CrossRef]
- Van Breemen, L.C.A.; Engels, T.A.P.; Klompen, E.T.J.; Senden, D.J.A.; Govaert, L.E. Rate-and temperature-dependent strain softening in solid polymers. J. Polym. Sci. Part B Polym. Phys. 2012, 50, 1757–1771. [Google Scholar] [CrossRef]

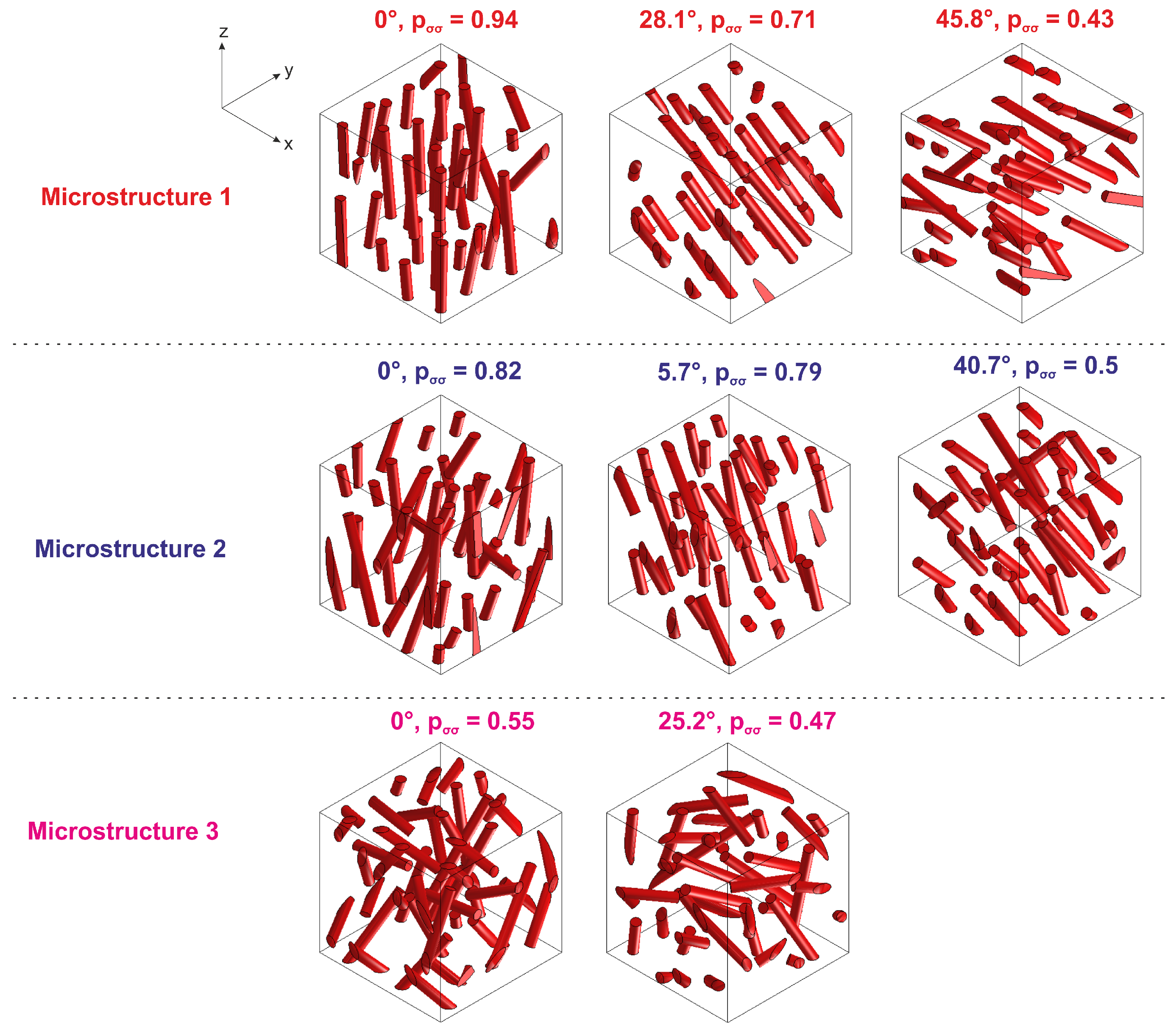
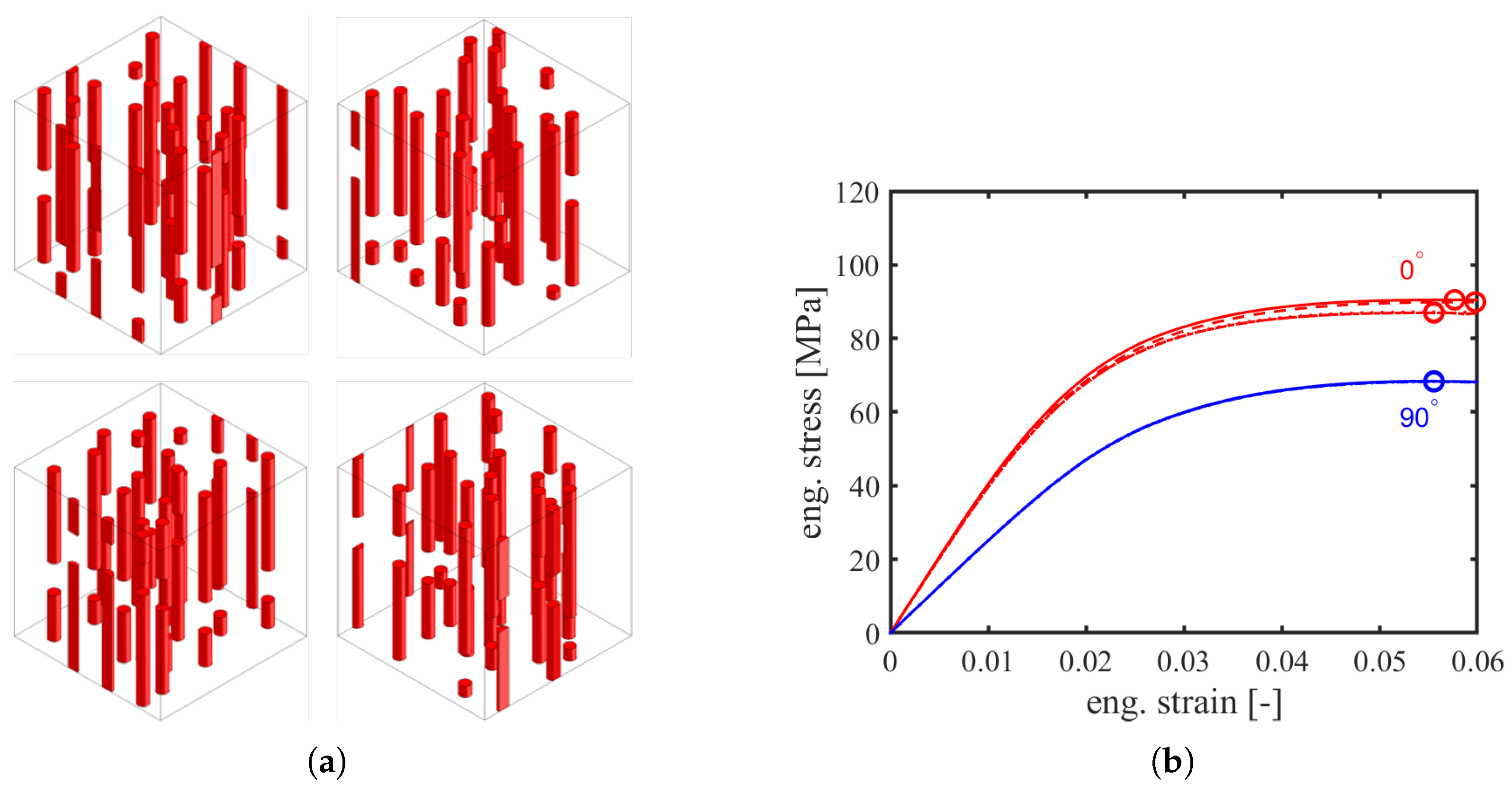
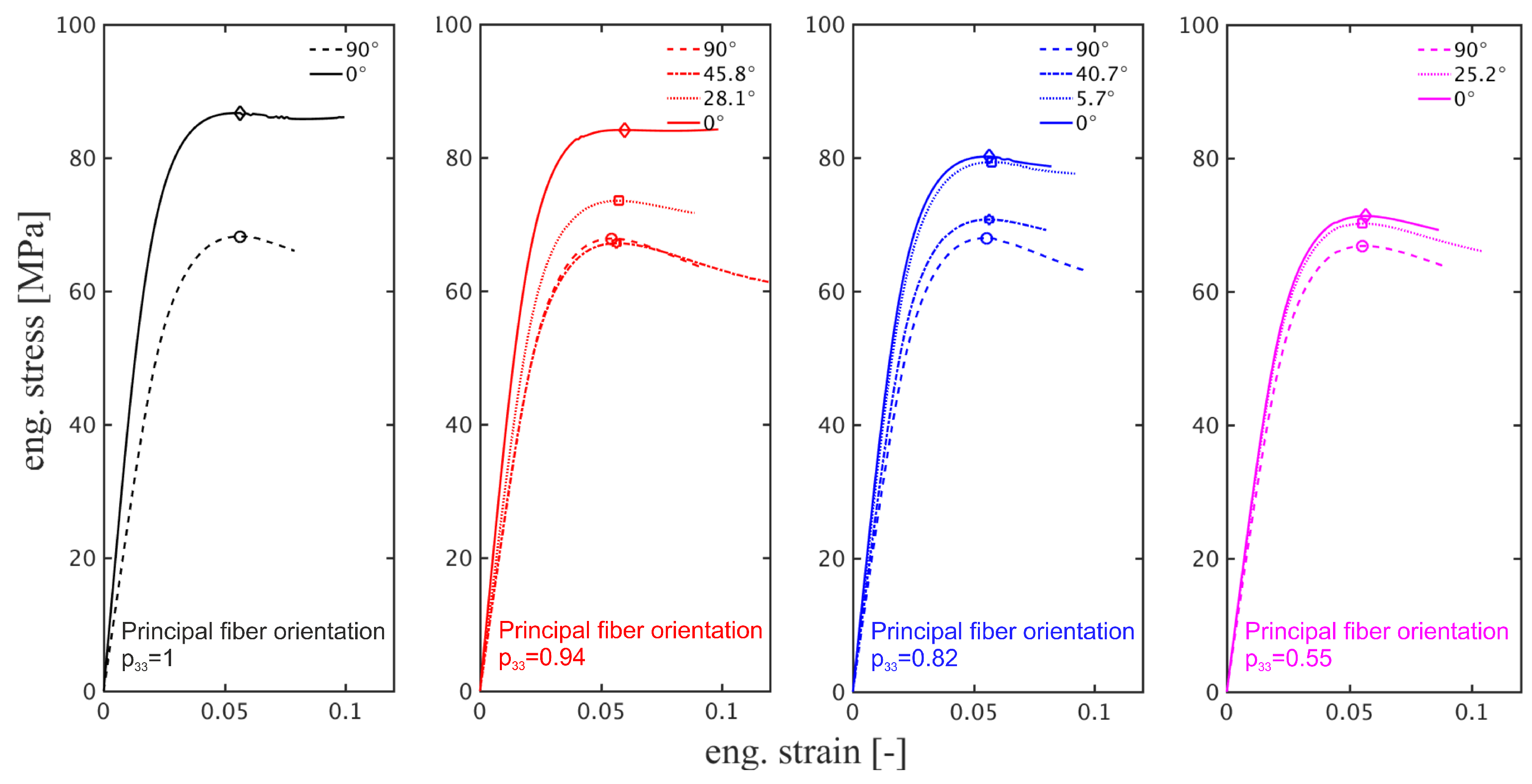
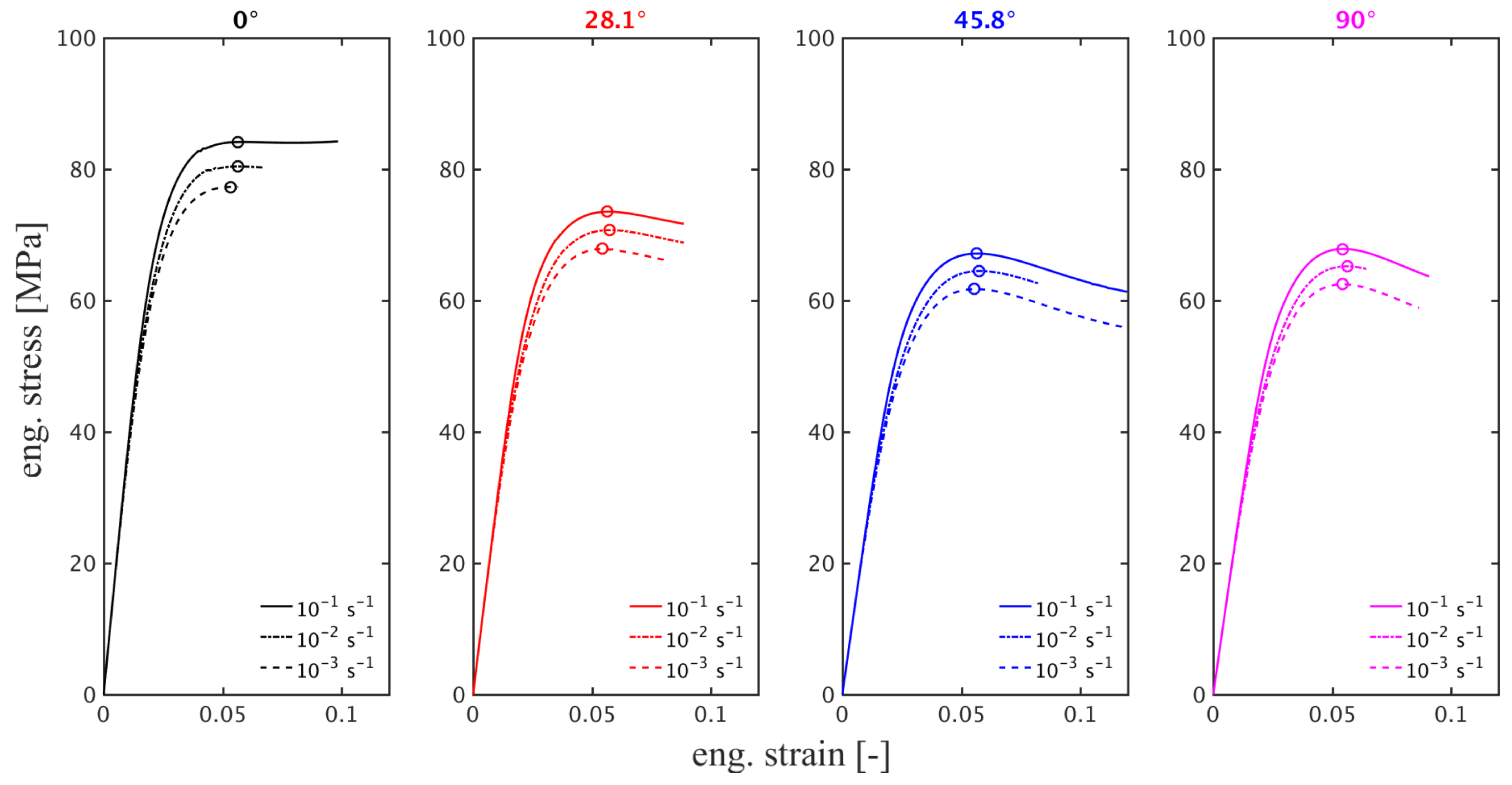

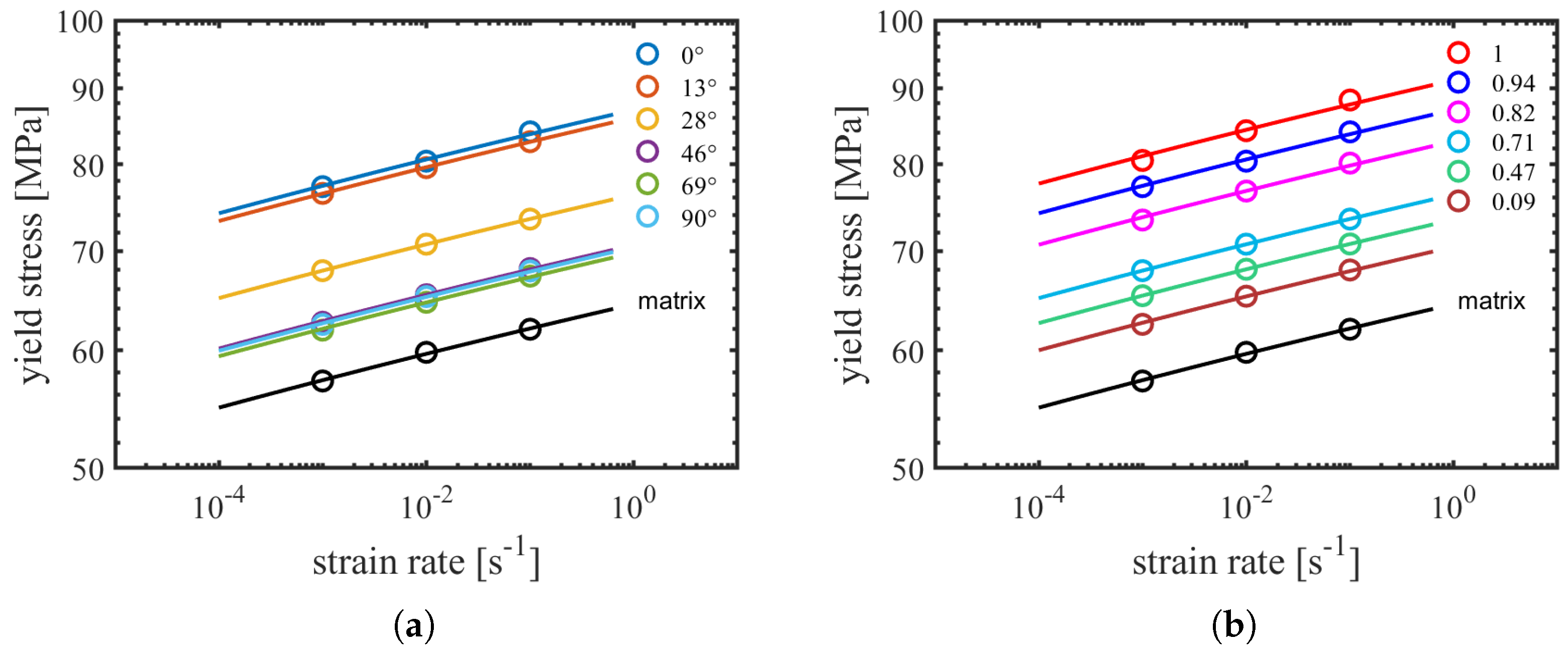
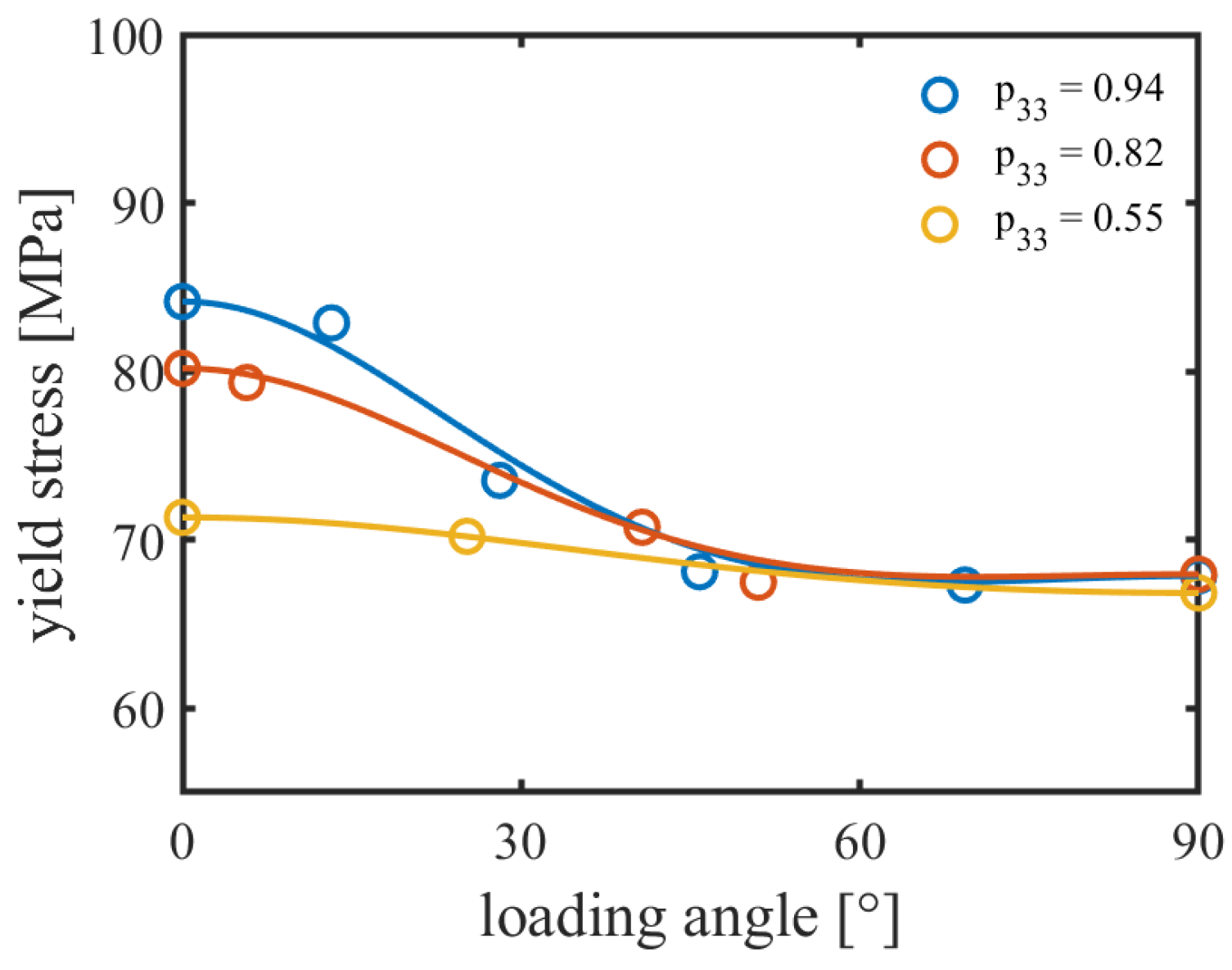
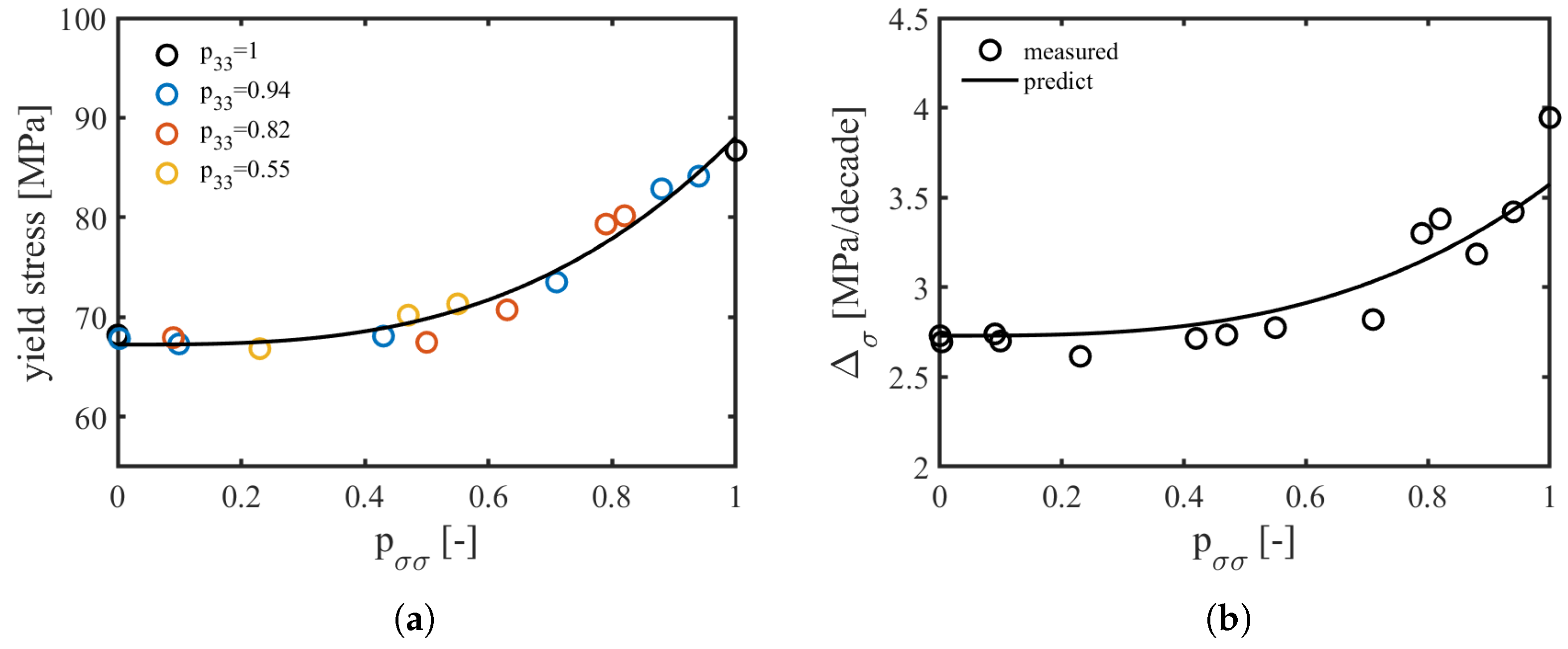
| Principal Orientation (-) | Loading Angle () | Orientation in Loading Direction (-) | Rate Dependence of Yield Stress (MPa/Decade) | Yield Stress@ (MPa) |
|---|---|---|---|---|
| matrix | isotropic | - | ||
| 1 | 90 | 0 | ||
| 90 | ||||
| 90 | ||||
| 69 | ||||
| 90 | ||||
| 46 | ||||
| 25 | ||||
| 0 | ||||
| 41 | ||||
| 28 | ||||
| 6 | ||||
| 0 | ||||
| 0 | ||||
| 1 | 0 | 1 |
| (-) | (MPa) | (-) | (-) | (-) |
|---|---|---|---|---|
| 1 | ||||
| 1 | ||||
| 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, S.; van Dommelen, J.A.W.; Govaert, L.E. Micromechanical Modeling of Anisotropy and Strain Rate Dependence of Short-Fiber-Reinforced Thermoplastics. Fibers 2021, 9, 44. https://doi.org/10.3390/fib9070044
Zhang S, van Dommelen JAW, Govaert LE. Micromechanical Modeling of Anisotropy and Strain Rate Dependence of Short-Fiber-Reinforced Thermoplastics. Fibers. 2021; 9(7):44. https://doi.org/10.3390/fib9070044
Chicago/Turabian StyleZhang, Shaokang, Johannes A. W. van Dommelen, and Leon E. Govaert. 2021. "Micromechanical Modeling of Anisotropy and Strain Rate Dependence of Short-Fiber-Reinforced Thermoplastics" Fibers 9, no. 7: 44. https://doi.org/10.3390/fib9070044
APA StyleZhang, S., van Dommelen, J. A. W., & Govaert, L. E. (2021). Micromechanical Modeling of Anisotropy and Strain Rate Dependence of Short-Fiber-Reinforced Thermoplastics. Fibers, 9(7), 44. https://doi.org/10.3390/fib9070044






