Closed-Form Solution Procedure for Simulating Debonding in FRP Strips Glued to a Generic Substrate Material
Abstract
1. Introduction
2. Model Formulation
2.1. Mechanical Assumptions
- -
- Debonding develops in pure mode II, and hence only relative slips are relevant to describing the displacement field of the FRP strip;
- -
- The FRP strip responds elastically with a Young modulus Ef;
- -
- Interface slips are uniform throughout the generic transversal chord of the FRP strip;
- -
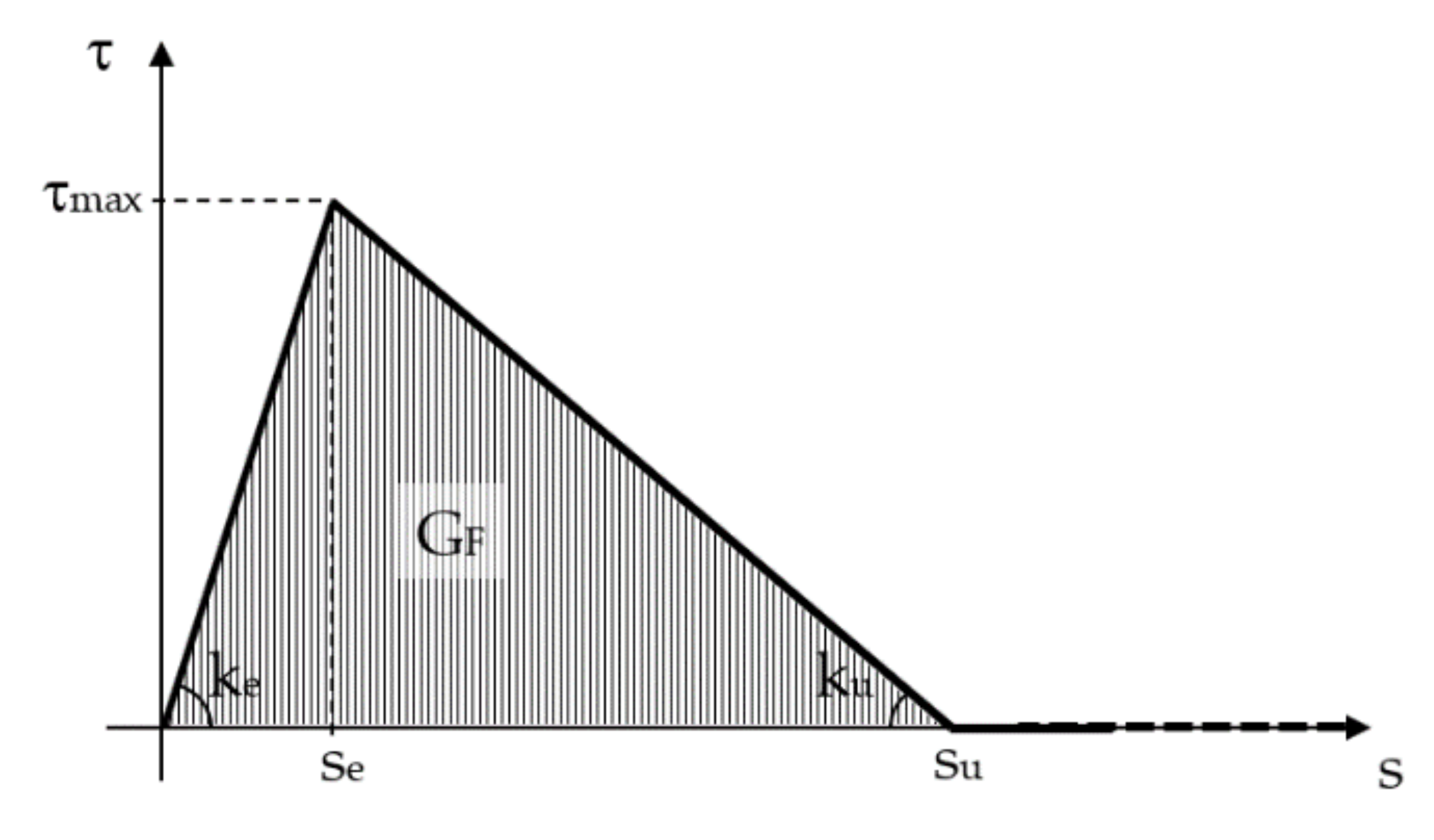
- The interface is described by a bilinear elastic-softening bond-slip law;
- -
- The substrate material is supposed to be stiff.
2.2. Main Equations and Close-Form Solutions
- -
- Elastic (“El”) branch (s ≤ se):
- -
- Softening (“So”) branch (se < s ≤su):
- -
- Debonded (“De”) branch (s > su):
2.3. Piecewise Formulation for Simulating the Debonding Process
2.3.1. Elastic (“El”) Stage
2.3.2. Elastic-Softening (“El-So”) Stage
2.3.3. Definition of “Short” and “Long” Anchorage
- -
- for (“short” anchorage), the condition s0 = se at the free end is attained, while sL < su: therefore, the debonding process proceeds towards a situation in which se < s(z) < su, meaning that the whole interface is stressed in the softening branch (“So” stage) of the bond-slip relationship (1);
- -
- for (“long” anchorage), the condition sL = su is attained, while s0 < se; therefore, the debonding process proceeds towards a situation in which the interface can be subdivided into three regions (“El-So-De”): the first one [0,] reacts elastically, the second one (,] mobilizes bond stresses in the softening branch, and the third one (,L] is debonded.
2.3.4. Short Anchorage: Debonding Evolution to Failure (“So” Stage)
2.3.5. Long Anchorage: Debonding Evolution to Failure (“El-So-De” Stage)
2.3.6. Final Considerations about the Simulation of the Debonding Process
3. Experimental Comparisons
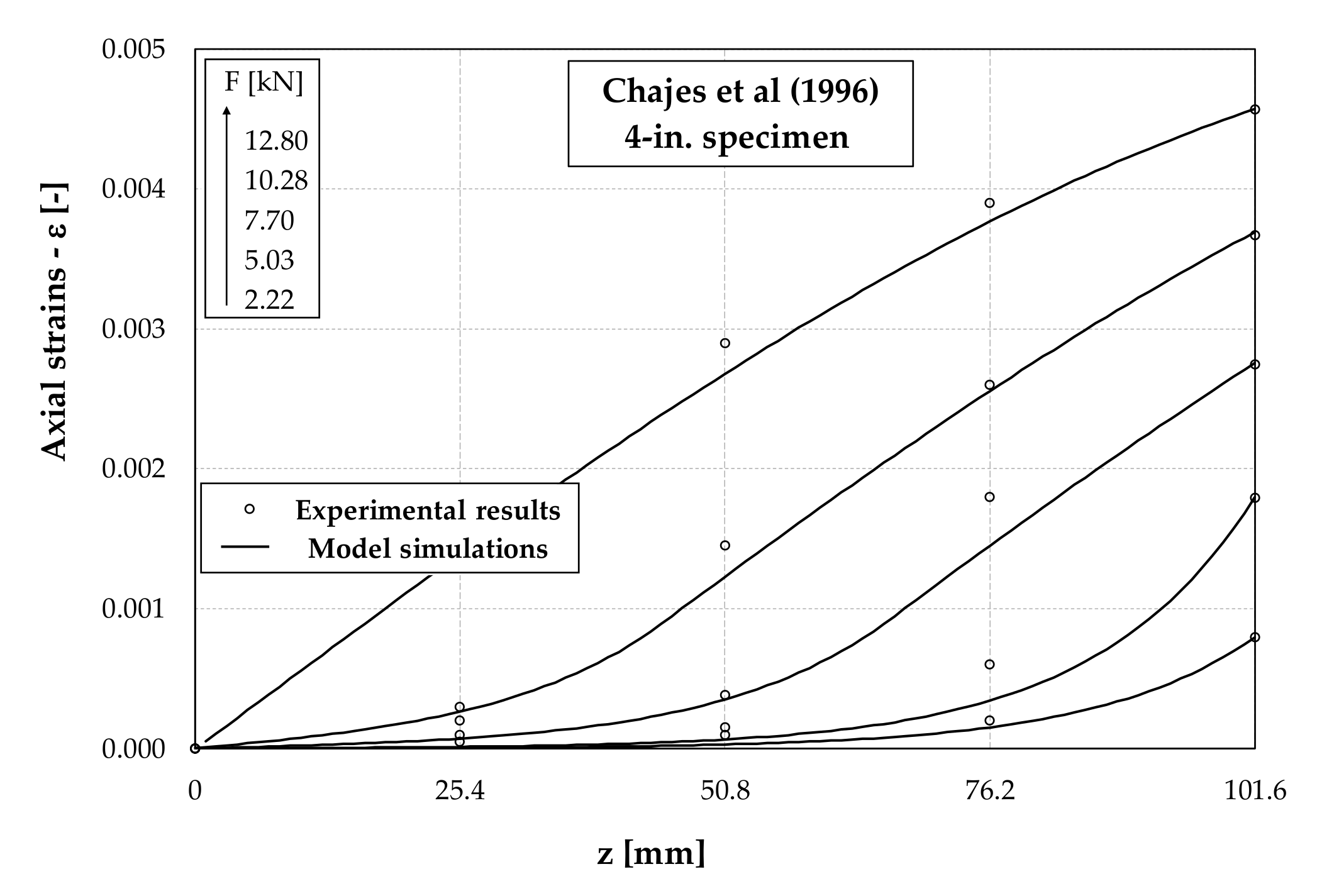
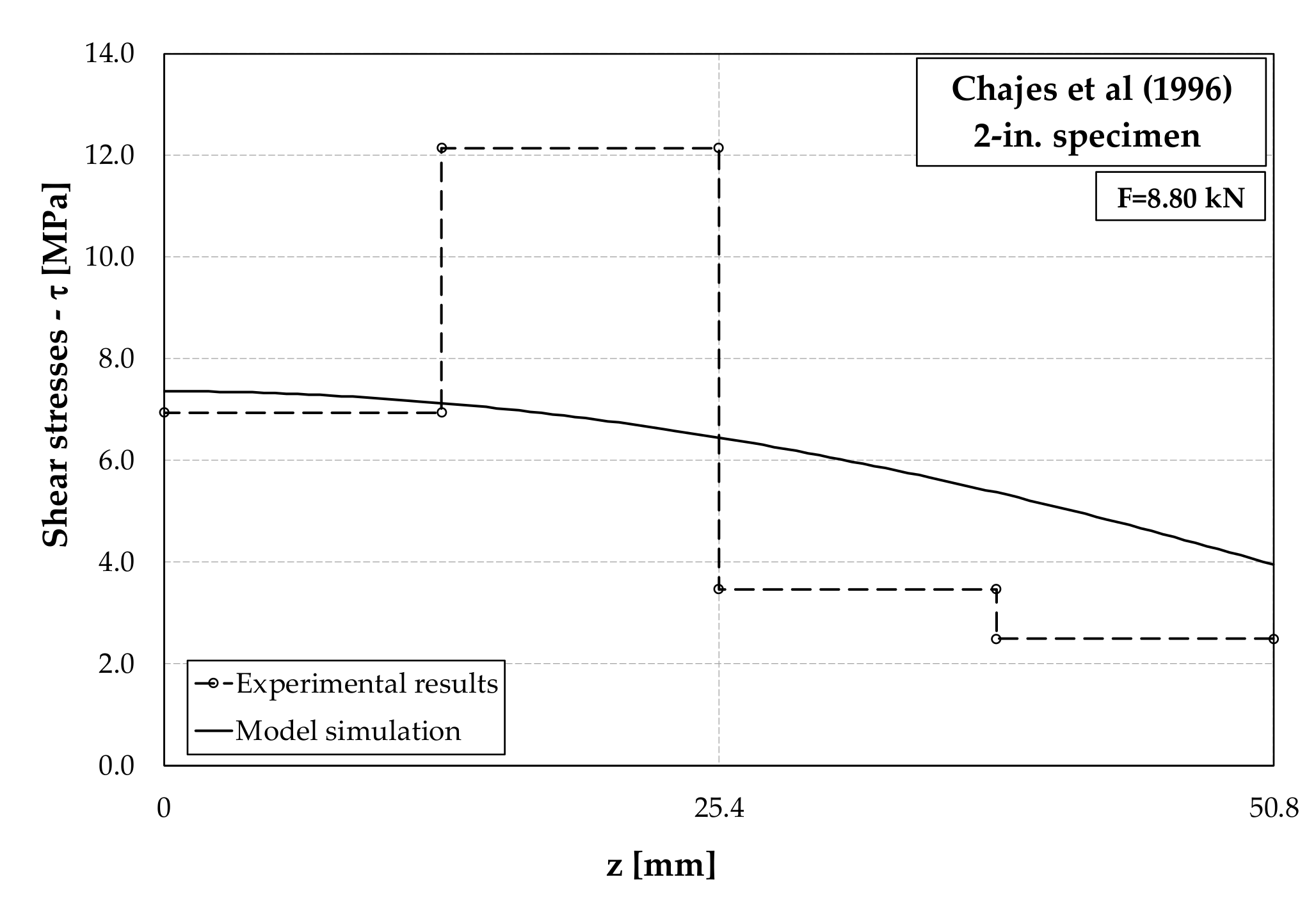
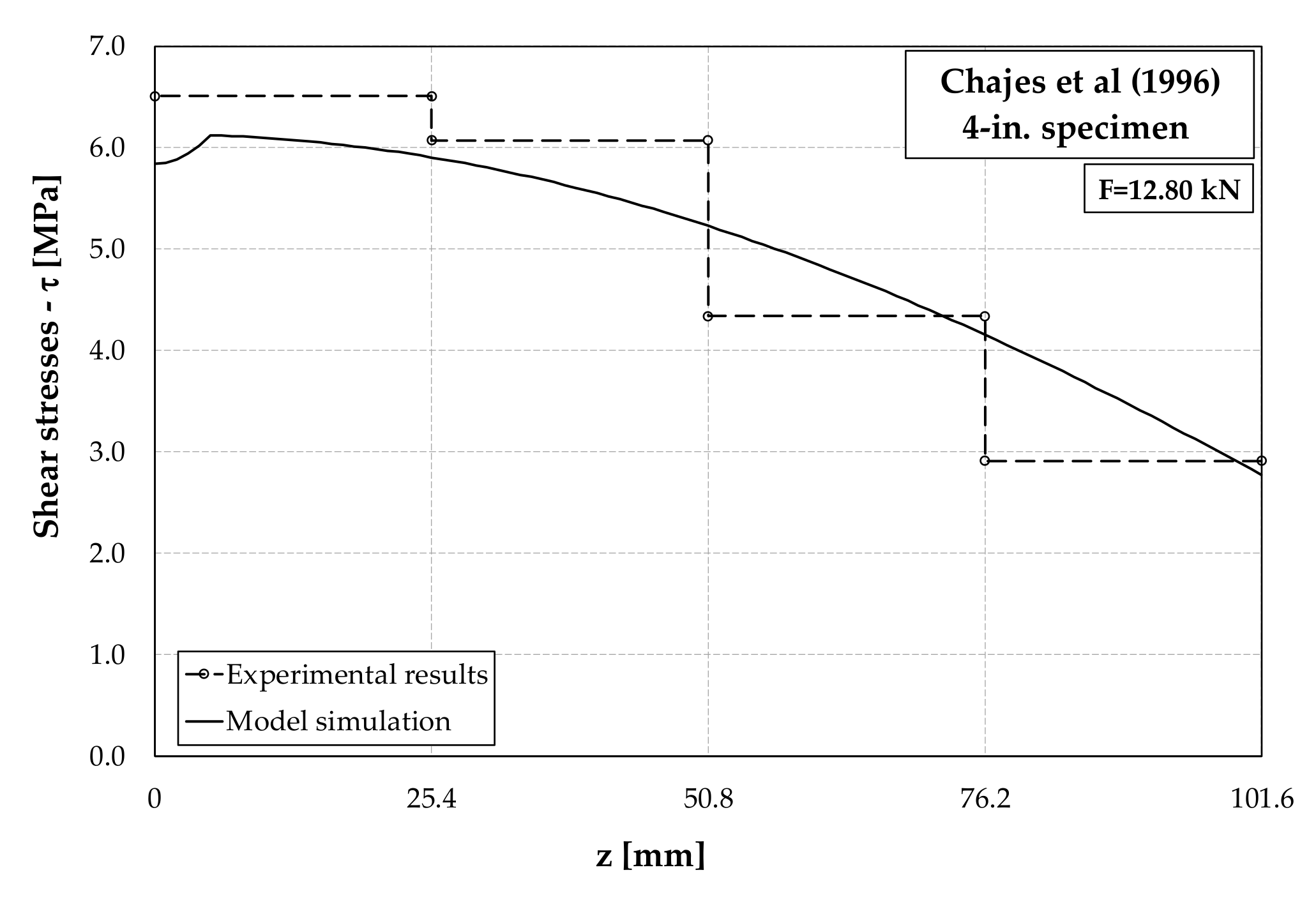
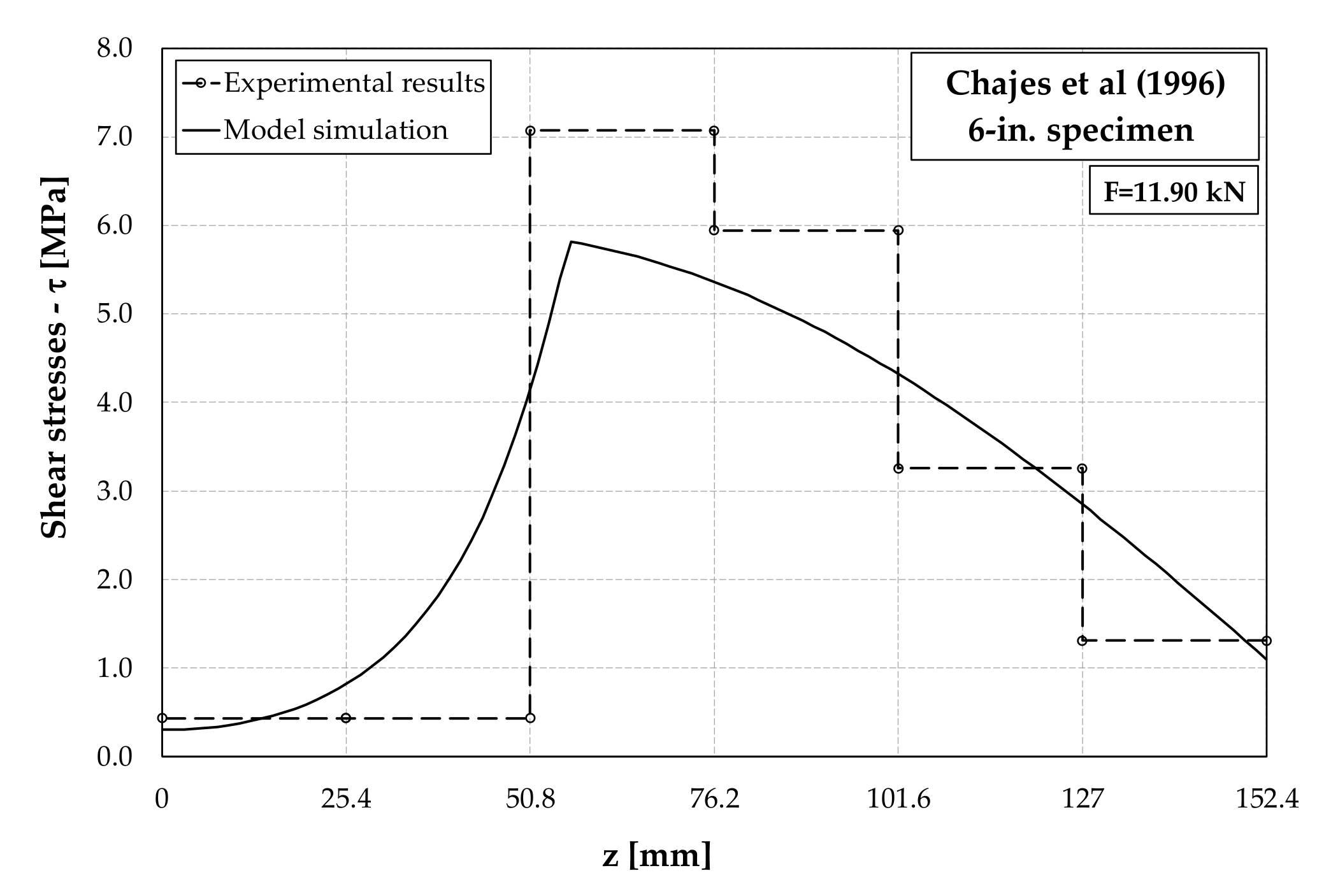
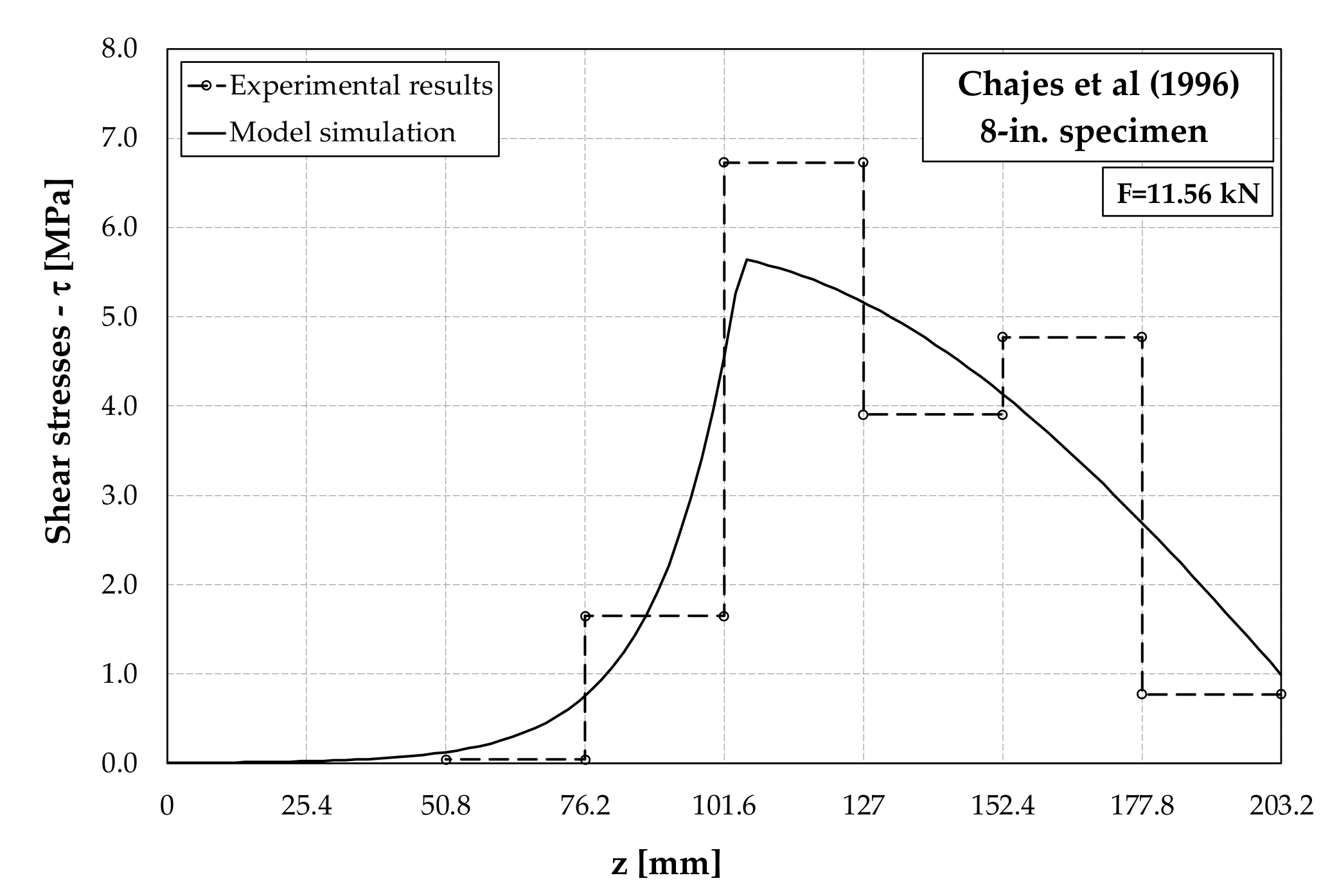
3.1. Pull-Out Tests by Chajes et al. (1996)
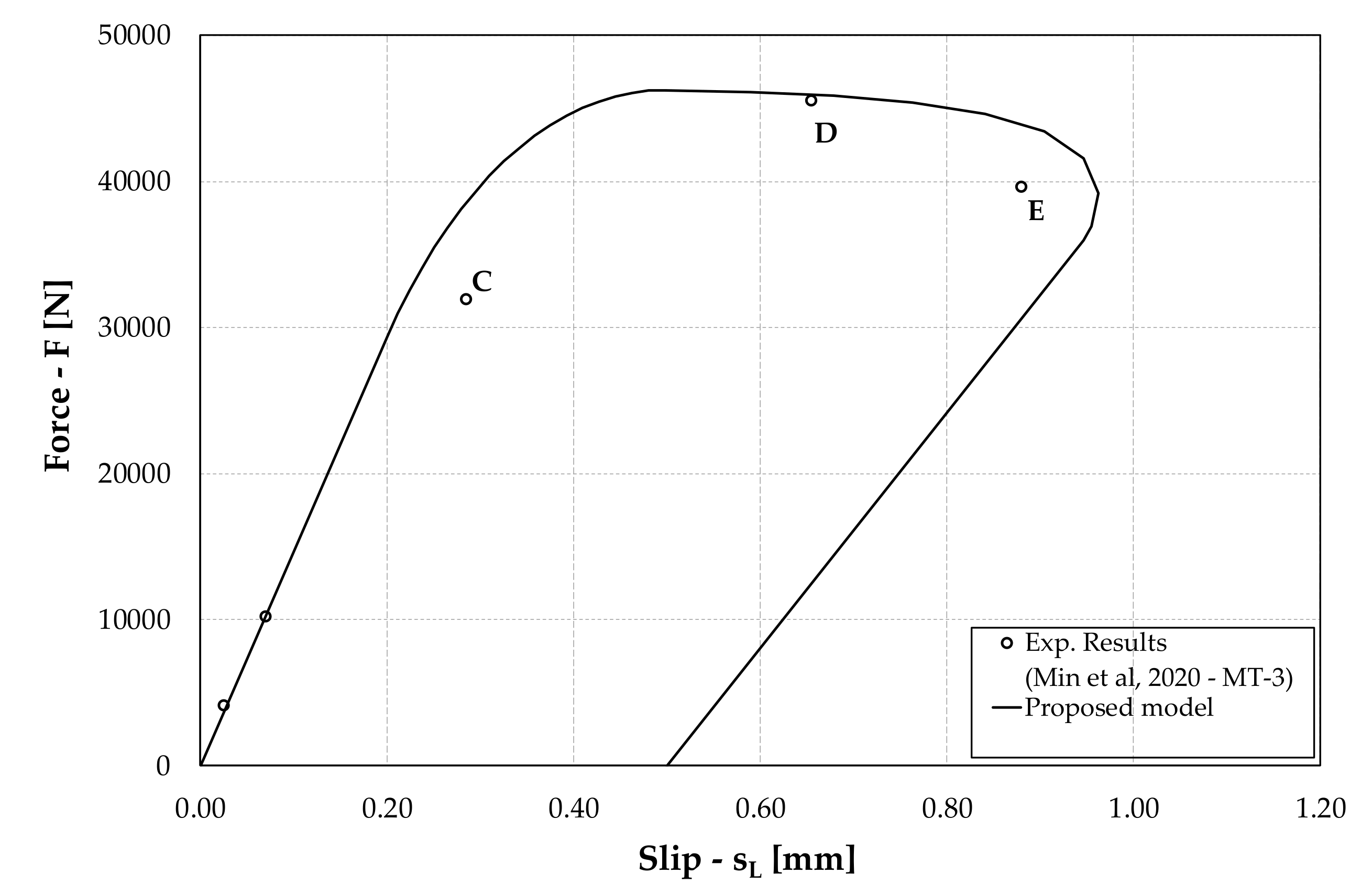
3.2. Pull-Out Tests by Min et al. (2020)
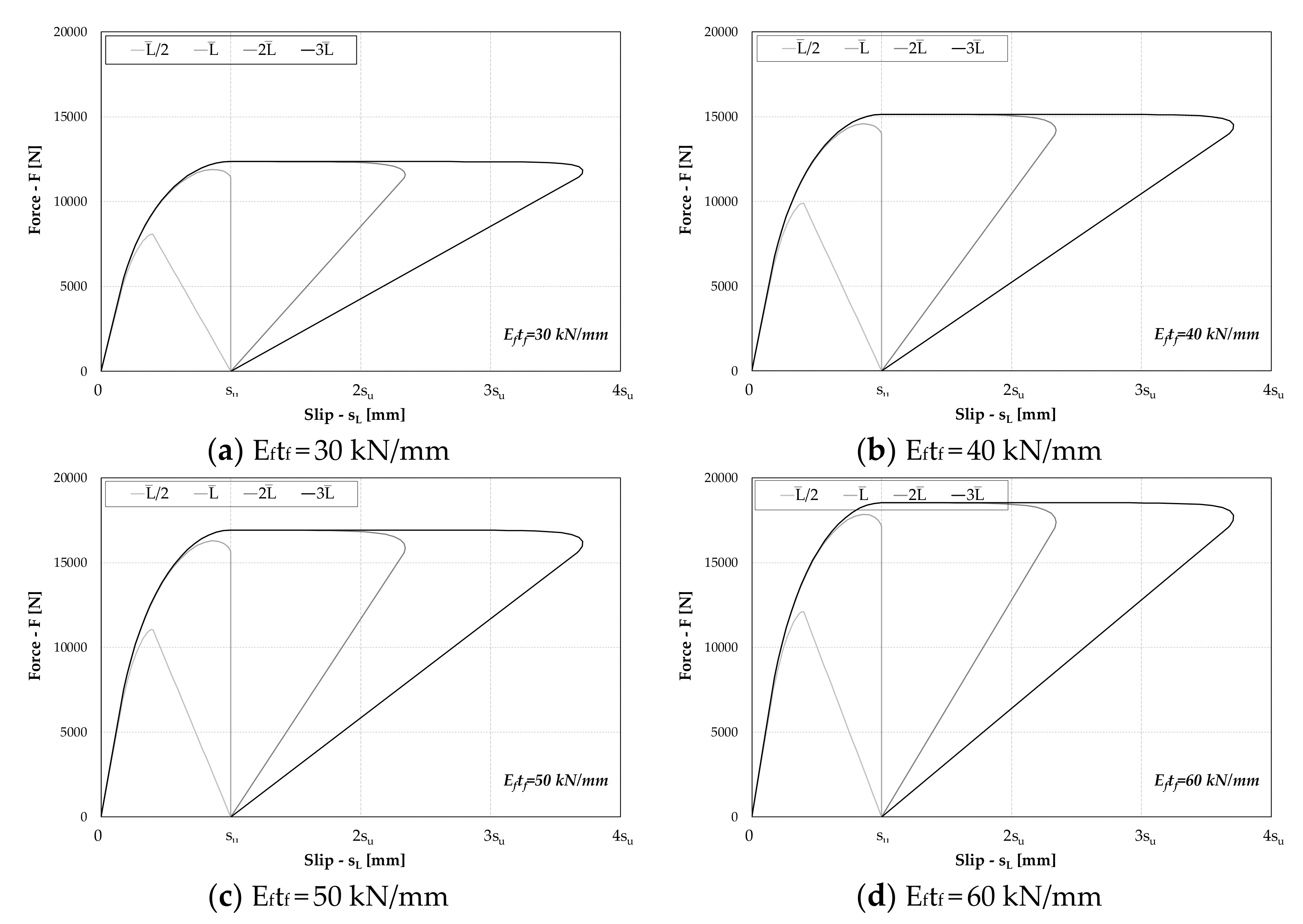
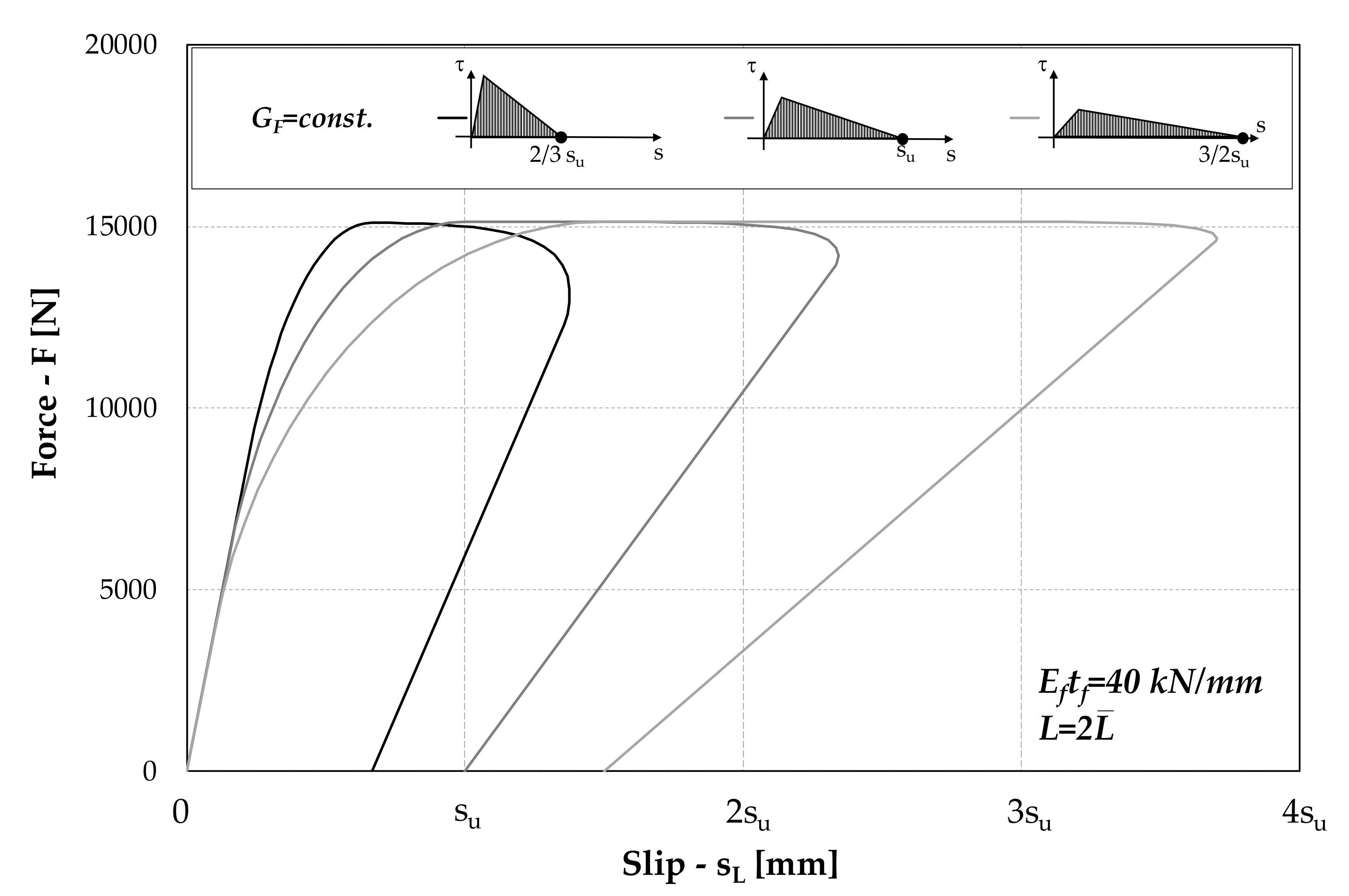
4. Parametric Analysis
5. Conclusions
- -
- The closed-form expressions obtained by assuming a cascading piecewise solution scheme are much simpler that the one presented in a previously co-authored paper [22];
- -
- The assumption of the free-end slip as the main controlling displacement parameters made it much easier to handle both the softening and snap-back response deriving, in principle, from the two cases of short and long anchorage, respectively;
- -
- The proposed results of parametric analyses pointed out some noteworthy relationships between the relevant geometric and mechanical parameters of the bond-slip law and the resulting force-slip response of the FRP-to-concrete joint;
- -
- Particularly, it pointed out the key importance of the critical the value of the of the bond length L depending on both the bond-slip parameters and the specific membrane stiffness of the composite strip.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zoghi, M. The International Handbook of FRP Composites in Civil Engineering; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]
- Teng, J.; Cheng, J.; Smith, S.; Lam, L. FRP-Strengthened RC Structures; Wiley: Chichester, UK, 2002. [Google Scholar]
- Seracino, R.; Griffith, M.C. FRP-Strengthened Masonry Structures, 1st ed.; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Zhao, X.-L. FRP-Strengthened Metallic Structures; Apple Academic Press: Palm Bay, FL, USA, 2013. [Google Scholar]
- Schober, K.-U.; Harte, A.M.; Kliger, R.; Jockwer, R.; Xu, Q.; Chen, J.-F. FRP reinforcement of timber structures. Constr. Build. Mater. 2015, 97, 106–118. [Google Scholar] [CrossRef]
- CNR. DT 200/R1—Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Existing Structures: Materials, RC and PC Structures, Masonry Structures, R1 Version, Italian National Research Council. 2005. Available online: https://www.cnr.it/en/node/2636 (accessed on 1 November 2020).
- CNR. DT 201—Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Existing Struc-tures: Timber Structures, Preliminary Study, Italian National Research Council. 2013. Available online: https://www.cnr.it/en/node/2637 (accessed on 1 November 2020).
- CNR. DT 202—Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Existing Struc-tures: Timber Structures, Preliminary Study, Italian National Research Council. 2013. Available online: https://www.cnr.it/en/node/2638 (accessed on 1 November 2020).
- Cauich, P.J.P.; Martínez-Molina, R.; Marrufo, J.L.G.; Franco, P.J.H. Adhesion, strengthening and durability issues in the retrofitting of Reinforced Concrete (RC) beams using Carbon Fiber Reinforced Polymer (CFRP)—A Review. Rev. ALCONPAT 2019, 9, 130–151. [Google Scholar] [CrossRef]
- Funari, M.F.; Lonetti, P. Initiation and evolution of debonding phenomena in layered structures. Theor. Appl. Fract. Mech. 2017, 92, 133–145. [Google Scholar] [CrossRef]
- Funari, M.F.; Spadea, S.; Fabbrocino, F.; Luciano, R. A Moving Interface Finite Element Formulation to Predict Dynamic Edge Debonding in FRP-Strengthened Concrete Beams in Service Conditions. Fibers 2020, 8, 42. [Google Scholar] [CrossRef]
- Kang, T.H.-K.; Howell, J.; Kim, S.; Lee, D.J. A State-of-the-Art Review on Debonding Failures of FRP Laminates Externally Adhered to Concrete. Int. J. Concr. Struct. Mater. 2012, 6, 123–134. [Google Scholar] [CrossRef]
- Faella, C.; Martinelli, E.; Nigro, E. Formulation and validation of a theoretical model for intermediate debonding in FRP-strengthened RC beams. Compos. Part B Eng. 2008, 39, 645–655. [Google Scholar] [CrossRef]
- Bennati, S.; Colonna, D.; Valvo, P.S. A cohesive-zone model for steel beams strengthened with pre-stressed laminates. Procedia Struct. Integr. 2016, 2, 2682–2689. [Google Scholar] [CrossRef]
- Lu, X.; Teng, J.; Ye, L.; Jiang, J. Bond-slip models for FRP sheets/plates externally bonded to concrete. Eng. Struct. 2006, 27, 920–937. [Google Scholar] [CrossRef]
- Chajes, M.; Finch, W.; Januska, T.; Thomson, T. Bond and force transfer of composite material plates bonded to concrete. ACI Struct. J. 1996, 93, 208–217. [Google Scholar]
- Min, X.; Zhang, J.; Wang, C.; Song, S.; Yang, D. Experimental Investigation of Fatigue Debonding Growth in FRP–Concrete Interface. Materials 2020, 13, 1459. [Google Scholar] [CrossRef] [PubMed]
- Faella, C.; Martinelli, E.; Nigro, E. Direct versus Indirect Method for Identifying FRP-to-Concrete Interface Relationships. J. Compos. Constr. 2009, 13, 226–233. [Google Scholar] [CrossRef]
- Faella, C.; Martinelli, E.; Nigro, E. Interface Behaviour in FRP Plates Bonded to Concrete: Experimental Tests and Theoretical Analyses. In Advanced Materials for Construction of Bridges, Buildings, and Other Structures III; Mistry, V., Azizinamini, A., Hooks, J.M., Eds.; ECI Symposium Series: New York, NY, USA, 2003; Volume P05, Available online: https://dc.engconfintl.org/advanced_materials/3 (accessed on 1 November 2020).
- Yuan, H.; Teng, J.; Seracino, R.; Wu, Z.; Yao, J. Full-range behaviour of FRP-to-concrete bonded joints. Eng. Struct. 2004, 26, 553–565. [Google Scholar] [CrossRef]
- Chen, F.; Qiao, P. Debonding analysis of FRP–concrete interface between two balanced adjacent flexural cracks in plated beams. Int. J. Solids Struct. 2009, 46, 2618–2628. [Google Scholar] [CrossRef]
- Caggiano, A.; Martinelli, E.; Faella, C. A fully-analytical approach for modelling the response of FRP plates bonded to a brit-tle substrate. Int. J. Solids Struct. 2012, 49, 2291–2300. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martinelli, E. Closed-Form Solution Procedure for Simulating Debonding in FRP Strips Glued to a Generic Substrate Material. Fibers 2021, 9, 22. https://doi.org/10.3390/fib9040022
Martinelli E. Closed-Form Solution Procedure for Simulating Debonding in FRP Strips Glued to a Generic Substrate Material. Fibers. 2021; 9(4):22. https://doi.org/10.3390/fib9040022
Chicago/Turabian StyleMartinelli, Enzo. 2021. "Closed-Form Solution Procedure for Simulating Debonding in FRP Strips Glued to a Generic Substrate Material" Fibers 9, no. 4: 22. https://doi.org/10.3390/fib9040022
APA StyleMartinelli, E. (2021). Closed-Form Solution Procedure for Simulating Debonding in FRP Strips Glued to a Generic Substrate Material. Fibers, 9(4), 22. https://doi.org/10.3390/fib9040022





