Plasmonic Biosensor on the End-Facet of a Dual-Core Single-Mode Optical Fiber
Abstract
1. Introduction
2. Sensor Structure and Proposed Interrogation Setup
3. Theoretical Design
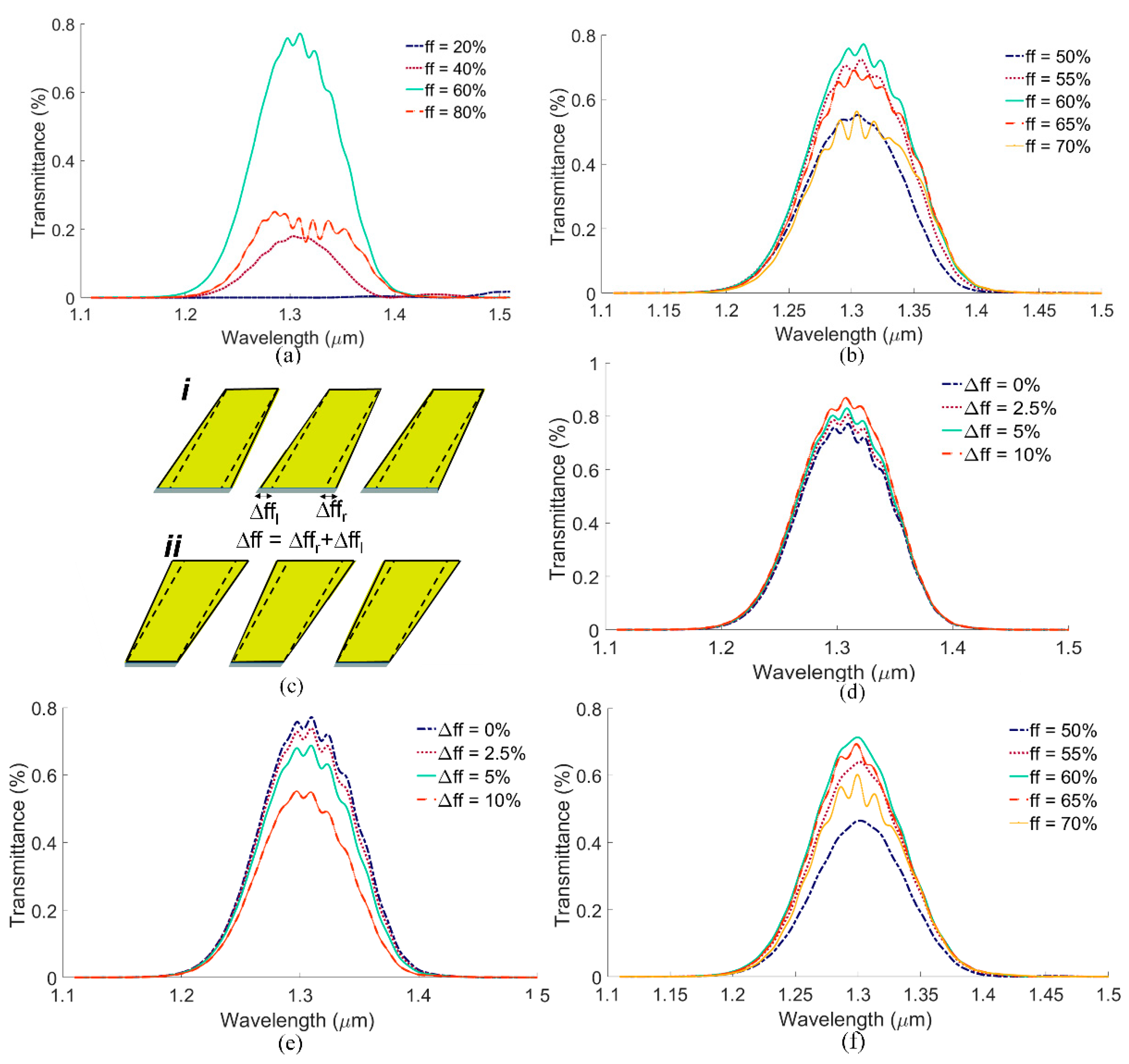
4. Proposed Fabrication Steps, Design Robustness, and Fabrication Tolerance
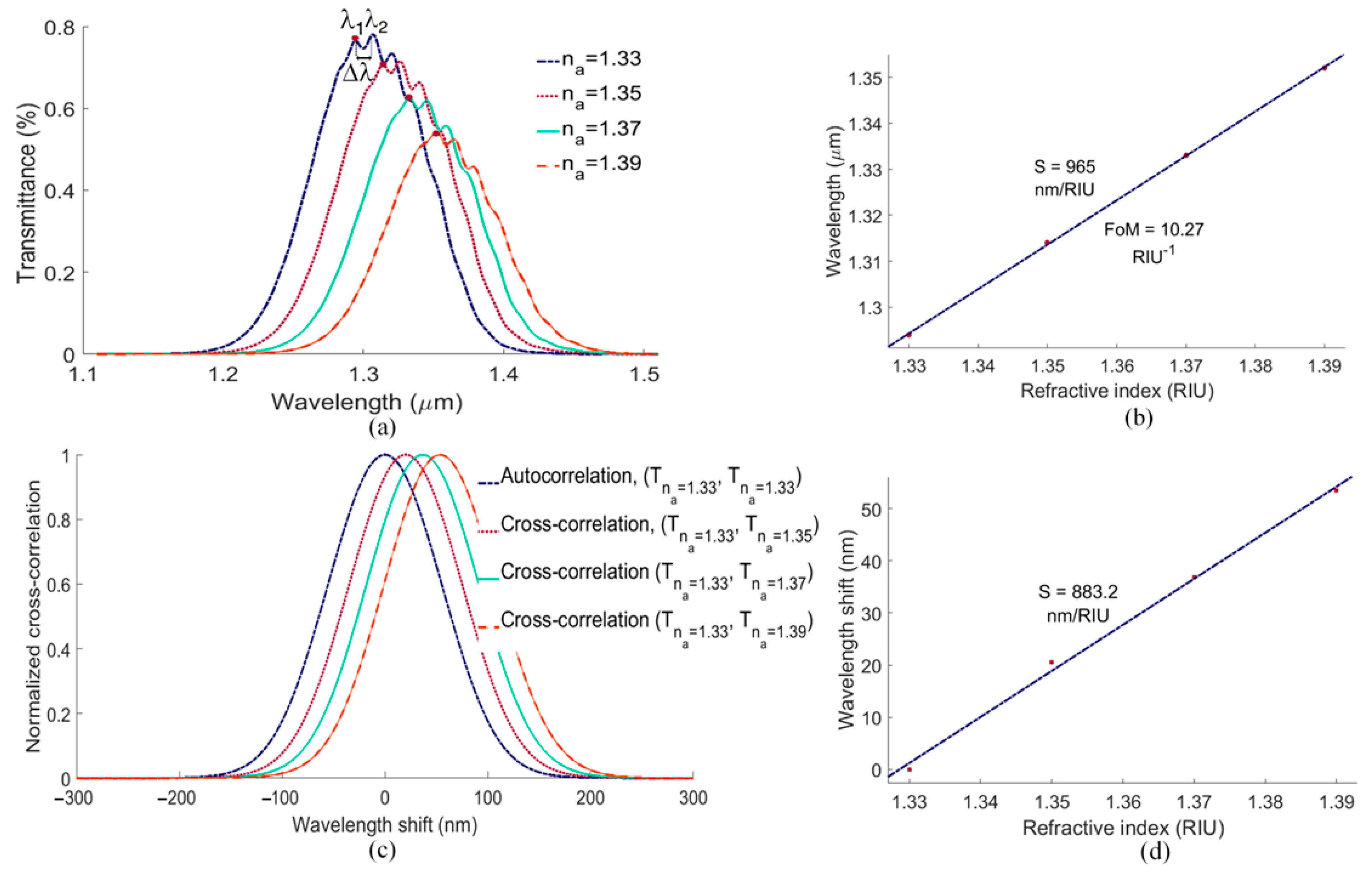
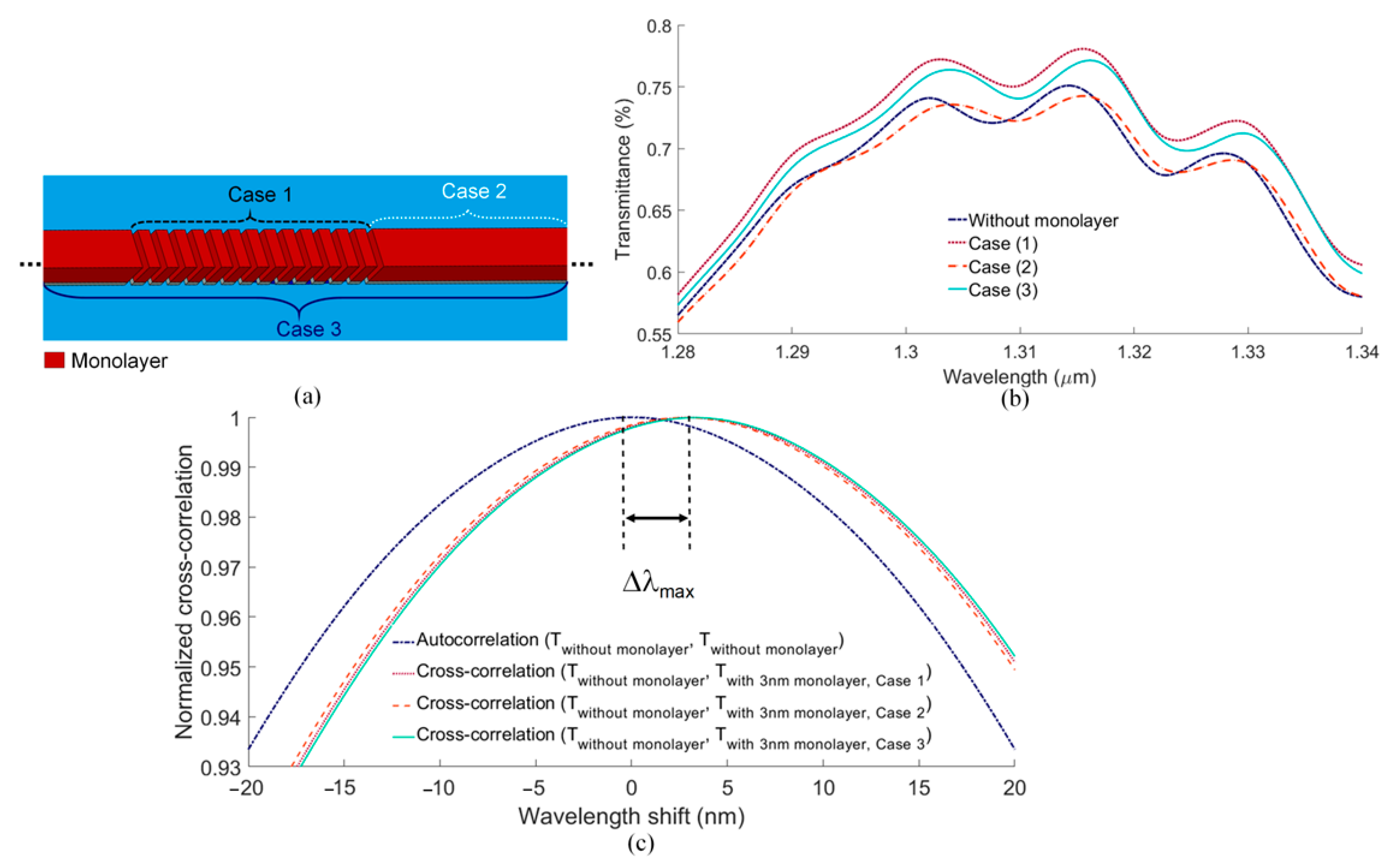
5. Sensitivity Computations
6. Discussion and Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| BMA | Boundary Mode Analysis |
| DCSMF | Dual-Core Single-Mode Optical Fiber |
| FEM | Finite Element Method |
| FIB | Focused Ion Beam Milling |
| FoM | Figure of Merit |
| FR | Fano-Resonance |
| FPIs | Fabry–Pérot Interferometers |
| FSR | Free Spectral Range |
| LSPR | Localized Surface Plasmon Resonance |
| LOF | Lab-on-a-Fiber |
| MMFs | Multi-Mode Fibers |
| PCFs | Photonic Crystal Fibers |
| PMLs | Perfectly Matched Layers |
| RI | Refractive Index |
| SMFs | Single-Mode Fibers |
| SPPs | Surface Plasmon Polaritons |
| SPR | Surface Plasmon Resonance |
| PSGCs | Plasmonic Slanted Grating Couplers |
| TM | Transverse Magnetic |
References
- Polley, N.; Basak, S.; Hass, R.; Pacholski, C. Fiber optic plasmonic sensors: Providing sensitive biosensor platforms with minimal lab equipment. Biosens. Bioelectron. 2019, 132, 368–374. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.-T.; Lee, Y.-C.; Lai, Y.-H.; Lim, J.-C.; Huang, N.-T.; Lin, C.-T.; Huang, J.-J.J.B. Review of integrated optical biosensors for point-of-care applications. Biosensors 2020, 10, 209. [Google Scholar] [CrossRef]
- Caucheteur, C.; Loyez, M.; González-Vila, Á.; Wattiez, R.J.O.E. Evaluation of gold layer configuration for plasmonic fiber grating biosensors. Opt. Express 2018, 26, 24154–24163. [Google Scholar] [CrossRef] [PubMed]
- Briliant, A.P.; Purwidyantri, A.; Kou-Chen, L.J.B. Surface plasmon resonance optical sensor: A review on light source technology. Biosensors 2018, 8, 80. [Google Scholar]
- Esfahani Monfared, Y. Overview of Recent Advances in the Design of Plasmonic Fiber-Optic Biosensors. Biosensors 2020, 10, 77. [Google Scholar] [CrossRef]
- Han, H.; Hou, D.; Luan, N.; Bai, Z.; Song, L.; Liu, J.; Hu, Y.J.S. Surface plasmon resonance sensor based on dual-side polished microstructured optical fiber with dual-core. Sensors 2020, 20, 3911. [Google Scholar] [CrossRef]
- Corres, J.M.; Matias, I.; Bravo, J.; Arregui, F.J.S.; Chemical, A.B. Tapered optical fiber biosensor for the detection of anti-gliadin antibodies. Sens. Actuators B Chem. 2008, 135, 166–171. [Google Scholar] [CrossRef]
- Viegas, D.; Goicoechea, J.; Santos, J.L.; Araújo, F.M.; Ferreira, L.A.; Arregui, F.J.; Matias, I.R.J.S. Sensitivity improvement of a humidity sensor based on silica nanospheres on a long-period fiber grating. Sensors 2009, 9, 519–527. [Google Scholar] [CrossRef]
- Xiong, Y.; Xu, F.J.A.P. Multifunctional integration on optical fiber tips: Challenges and opportunities. Adv. Photonics 2020, 2, 064001. [Google Scholar] [CrossRef]
- Gandhi, M.A.; Chu, S.; Senthilnathan, K.; Babu, P.R.; Nakkeeran, K.; Li, Q.J.A.S. Recent advances in plasmonic sensor-based fiber optic probes for biological applications. Appl. Sci. 2019, 9, 949. [Google Scholar] [CrossRef]
- Aliberti, A.; Ricciardi, A.; Giaquinto, M.; Micco, A.; Bobeico, E.; La Ferrara, V.; Ruvo, M.; Cutolo, A.; Cusano, A. Microgel assisted lab-on-fiber optrode. Sci. Rep. 2017, 7, 14459. [Google Scholar] [CrossRef]
- Ricciardi, A.; Crescitelli, A.; Vaiano, P.; Quero, G.; Consales, M.; Pisco, M.; Esposito, E.; Cusano, A. Lab-on-fiber technology: A new vision for chemical and biological sensing. Analyst 2015, 140, 8068–8079. [Google Scholar] [CrossRef] [PubMed]
- Mahani, F.F.; Maleki, M.; Mokhtari, A.; Berini, P. Design of an efficient Fabry-Perot biosensor using high-contrast slanted grating couplers on a dual-core single-mode optical fiber tip. IEEE Sens. J. 2021, 21, 19705–19713. [Google Scholar] [CrossRef]
- Alam, M.Z.; Yang, Z.; Sheik-Bahae, M.; Aitchison, J.S.; Mojahedi, M. Directional excitation of surface plasmon using multi-mode interference in an aperture. Sci. Rep. 2021, 11, 3170. [Google Scholar] [CrossRef]
- Liao, H.; Li, Z.; Chen, J.; Zhang, X.; Yue, S.; Gong, Q. A submicron broadband surface-plasmon-polariton unidirectional coupler. Sci. Rep. 2013, 3, 1–7. [Google Scholar] [CrossRef]
- Eftekharinia, B.; Moshaii, A.; Dabirian, A. Design of a slit-groove coupler for unidirectional excitation of the guided surface plasmon polaritons through a plasmonic slot waveguide. Plasmonics 2017, 12, 131–138. [Google Scholar] [CrossRef]
- Ayata, M.; Fedoryshyn, Y.; Koch, U.; Leuthold, J. Compact, ultra-broadband plasmonic grating couplers. Opt. Express 2019, 27, 29719–29729. [Google Scholar] [CrossRef]
- López-Tejeira, F.; Rodrigo, S.G.; Martin-Moreno, L.; García-Vidal, F.J.; Devaux, E.; Ebbesen, T.W.; Krenn, J.R.; Radko, I.; Bozhevolnyi, S.I.; González, M.U. Efficient unidirectional nanoslit couplers for surface plasmons. Nat. Phys. 2007, 3, 324–328. [Google Scholar] [CrossRef]
- Liu, Y.; Palomba, S.; Park, Y.; Zentgraf, T.; Yin, X.; Zhang, X. Compact magnetic antennas for directional excitation of surface plasmons. Nano Lett. 2012, 12, 4853–4858. [Google Scholar] [CrossRef]
- Liu, W.; Wang, G.; Wen, K.; Hu, X.; Qin, Y. Efficient unidirectional SPP launcher: Coupling the SPP to a smooth surface for propagation. Opt. Lett. 2022, 47, 621–624. [Google Scholar] [CrossRef] [PubMed]
- Bonod, N.; Popov, E.; Li, L.; Chernov, B. Unidirectional excitation of surface plasmons by slanted gratings. Opt. Express 2007, 15, 11427–11432. [Google Scholar] [CrossRef]
- McPolin, C.; Bouillard, J.-S.; Vilain, S.; Krasavin, A.V.; Dickson, W.; O’Connor, D.; Wurtz, G.A.; Justice, J.; Corbett, B.; Zayats, A.V. Integrated plasmonic circuitry on a vertical-cavity surface-emitting semiconductor laser platform. Nat. Commun. 2016, 7, 12409. [Google Scholar] [CrossRef]
- Suzuki, H.; Sugimoto, M.; Matsui, Y.; Kondoh, J. Effects of gold film thickness on spectrum profile and sensitivity of a multimode-optical-fiber SPR sensor. Sens. Actuators B Chem. 2008, 132, 26–33. [Google Scholar] [CrossRef]
- Geng, Y.; Li, S.; Li, M.-J.; Sutton, C.G.; McCollum, R.L.; McClure, R.L.; Koklyushkin, A.V.; Matthews, K.I.; Luther, J.P.; Butler, D.L. High-speed, bi-directional dual-core fiber transmission system for high-density, short-reach optical interconnects. In Proceedings of the Next-Generation Optical Networks for Data Centers and Short-Reach Links II, San Francisco, CA, USA, 9 May 2015; p. 939009. [Google Scholar]
- Bertie, J.E.; Lan, Z. Infrared intensities of liquids XX: The intensity of the OH stretching band of liquid water revisited, and the best current values of the optical constants of H2O (l) at 25 °C between 15,000 and 1 cm−1. Appl. Spectrosc. 1996, 50, 1047–1057. [Google Scholar] [CrossRef]
- Comsol Multiphysics, Finite Element Mode Solver. Available online: www.comsol.com (accessed on 20 August 2021).
- Taillaert, D.; Bogaerts, W.; Bienstman, P.; Krauss, T.F.; Van Daele, P.; Moerman, I.; Verstuyft, S.; De Mesel, K.; Baets, R. An out-of-plane grating coupler for efficient butt-coupling between compact planar waveguides and single-mode fibers. IEEE J. Quantum Electron. 2002, 38, 949–955. [Google Scholar] [CrossRef]
- Johnson, P.B.; Christy, R.-W. Optical constants of the noble metals. Phys. Rev. B 1972, 6, 4370. [Google Scholar] [CrossRef]
- Johnson, P.; Christy, R. Optical constants of transition metals: Ti, v, cr, mn, fe, co, ni, and pd. Phys. Rev. B 1974, 9, 5056. [Google Scholar] [CrossRef]
- Ditlbacher, H.; Galler, N.; Koller, D.; Hohenau, A.; Leitner, A.; Aussenegg, F.; Krenn, J.J.O.e. Coupling dielectric waveguide modes to surface plasmon polaritons. Opt. Express 2008, 16, 10455–10464. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.; Jiang, J.; Nordin, G.P. Systematic design process for slanted grating couplers. Appl. Opt. 2006, 45, 6223–6226. [Google Scholar] [CrossRef] [PubMed]
- Ayata, M.; Fedoryshyn, Y.; Heni, W.; Baeuerle, B.; Josten, A.; Zahner, M.; Koch, U.; Salamin, Y.; Hoessbacher, C.; Haffner, C. High-speed plasmonic modulator in a single metal layer. Science 2017, 358, 630–632. [Google Scholar] [CrossRef]
- Suleman, H.; Hajebifard, A.; Hahn, C.; Olivieri, A.; Berini, P. Plasmonic heptamer-arranged nanoholes in a gold film on the end-facet of a photonic crystal fiber. Opt. Lett. 2021, 46, 4482–4485. [Google Scholar] [CrossRef] [PubMed]
- Nagal, V.; Li, T.; Khurgin, J.B.; Gracias, D.H.J.A.A.N.M. Large-area arrays of quasi-3D Au nanostructures for polarization-selective mid-infrared metasurfaces. ACS Appl. Nano Mater. 2020, 3, 7029–7039. [Google Scholar] [CrossRef]
- Wang, L.; Li, T.; Li, L.; Xia, W.; Xu, X.; Zhu, S. Electrically generated unidirectional surface plasmon source. Opt. Express 2012, 20, 8710–8717. [Google Scholar] [CrossRef]
- Schrauwen, J.; Van Laere, F.; Van Thourhout, D.; Baets, R. Focused-ion-beam fabrication of slanted grating couplers in silicon-on-insulator waveguides. IEEE Photonics Technol. Lett. 2007, 19, 816–818. [Google Scholar] [CrossRef]
- Dhawan, A.; Muth, J.F. Engineering surface plasmon based fiber-optic sensors. Mater. Sci. Eng. B 2008, 149, 237–241. [Google Scholar] [CrossRef]
- Hahn, C.; Hajebifard, A.; Berini, P. Helium focused ion beam direct milling of plasmonic heptamer-arranged nanohole arrays. Nanophotonics 2020, 9, 393–399. [Google Scholar] [CrossRef]
- Miller, J.M.; de Beaucoudrey, N.; Chavel, P.; Turunen, J.; Cambril, E.J.A.o. Design and fabrication of binary slanted surface-relief gratings for a planar optical interconnection. Appl. Opt. 1997, 36, 5717–5727. [Google Scholar] [CrossRef] [PubMed]
- Farhadi, S.; Farmani, A.; Hamidi, A.J.O.; Electronics, Q. Figure of merit enhancement of surface plasmon resonance biosensor based on Talbot effect. Opt. Quantum Electron. 2021, 53, 518. [Google Scholar] [CrossRef]
- Antoniou, M.; Tsounidi, D.; Petrou, P.S.; Beltsios, K.G.; Kakabakos, S.E. Functionalization of silicon dioxide and silicon nitride surfaces with aminosilanes for optical biosensing applications. Med. Devices Sens. 2020, 3, e10072. [Google Scholar] [CrossRef]
- Jia, P.; Yang, J. A plasmonic optical fiber patterned by template transfer as a high-performance flexible nanoprobe for real-time biosensing. Nanoscale 2014, 6, 8836–8843. [Google Scholar] [CrossRef]
- Pisco, M.; Galeotti, F.; Quero, G.; Iadicicco, A.; Giordano, M.; Cusano, A. Miniaturized sensing probes based on metallic dielectric crystals self-assembled on optical fiber tips. ACS Photonics 2014, 1, 917–927. [Google Scholar] [CrossRef]
- Nguyen, H.; Sidiroglou, F.; Collins, S.F.; Baxter, G.W.; Roberts, A.; Davis, T.J. Periodic array of nanoholes on gold-coated optical fiber end-faces for surface plasmon resonance liquid refractive index sensing. In Proceedings of the Third Asia Pacific Optical Sensors Conference, Sydney, Australia, 21 January–3 February 2012; pp. 505–510. [Google Scholar]
- Consales, M.; Ricciardi, A.; Crescitelli, A.; Esposito, E.; Cutolo, A.; Cusano, A. Lab-on-fiber technology: Toward multifunctional optical nanoprobes. ACS Nano 2012, 6, 3163–3170. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, H.; Sidiroglou, F.; Collins, S.; Davis, T.; Roberts, A.; Baxter, G.J.A.P.L. A localized surface plasmon resonance-based optical fiber sensor with sub-wavelength apertures. Appl. Phys. Lett. 2013, 103, 193116. [Google Scholar] [CrossRef]
- Savinov, V.; Zheludev, N.J.A.P.L. High-quality metamaterial dispersive grating on the facet of an optical fiber. Appl. Phys. Lett. 2017, 111, 091106. [Google Scholar] [CrossRef]
- Novais, S.; Ferreira, M.S.; Pinto, J.L. Optical fiber Fabry–Perot tip sensor for detection of water–glycerin mixtures. J. Light. Technol. 2018, 36, 1576–1582. [Google Scholar] [CrossRef]
- Huang, C.; Yang, M.; Xie, W.; Dai, J. Optical fiber Fabry-Perot refractive index sensor based on porous Al2O3 film. In Proceedings of the 24th International Conference on Optical Fibre Sensors, Curitiba, Brazil, 28 September–2 October 2015; p. 963484. [Google Scholar]
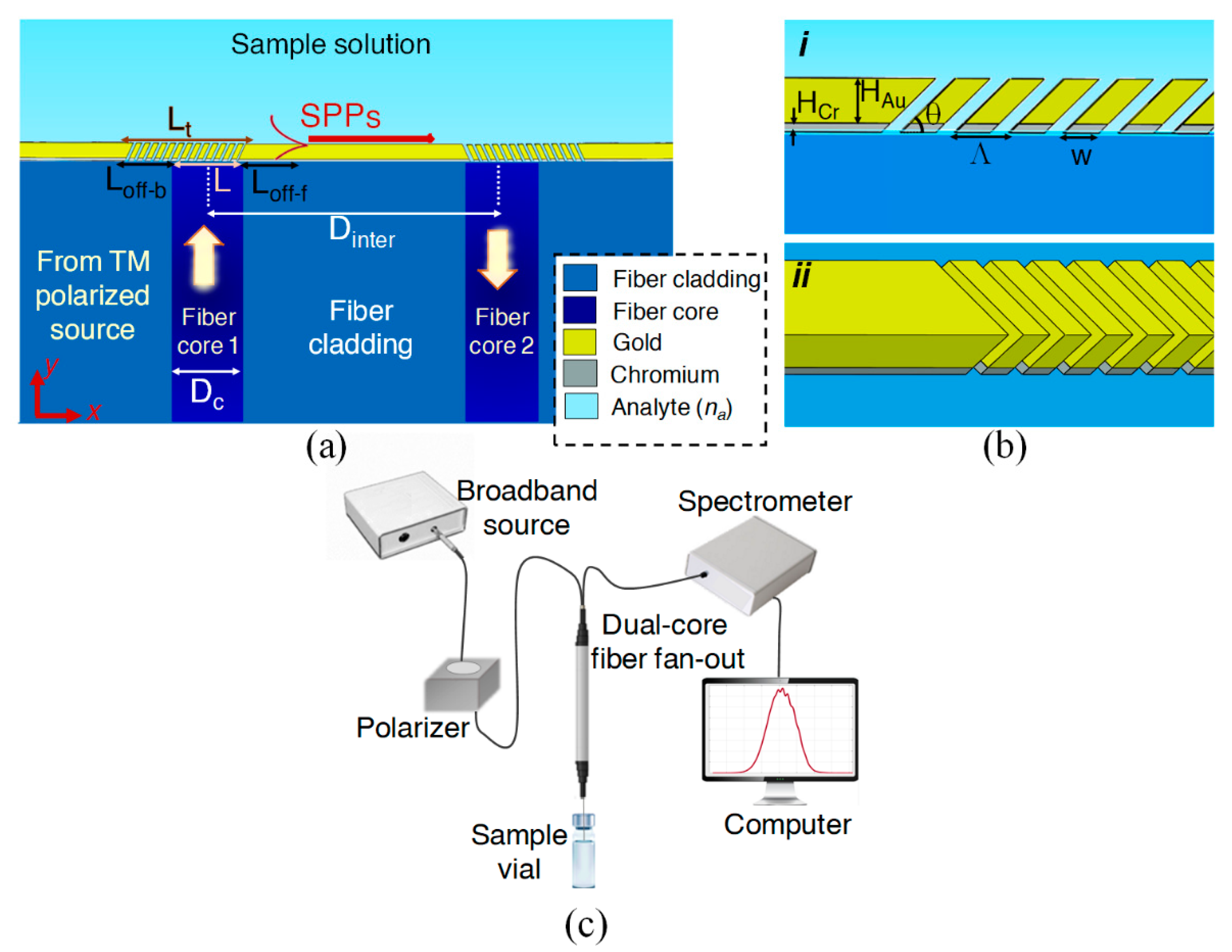
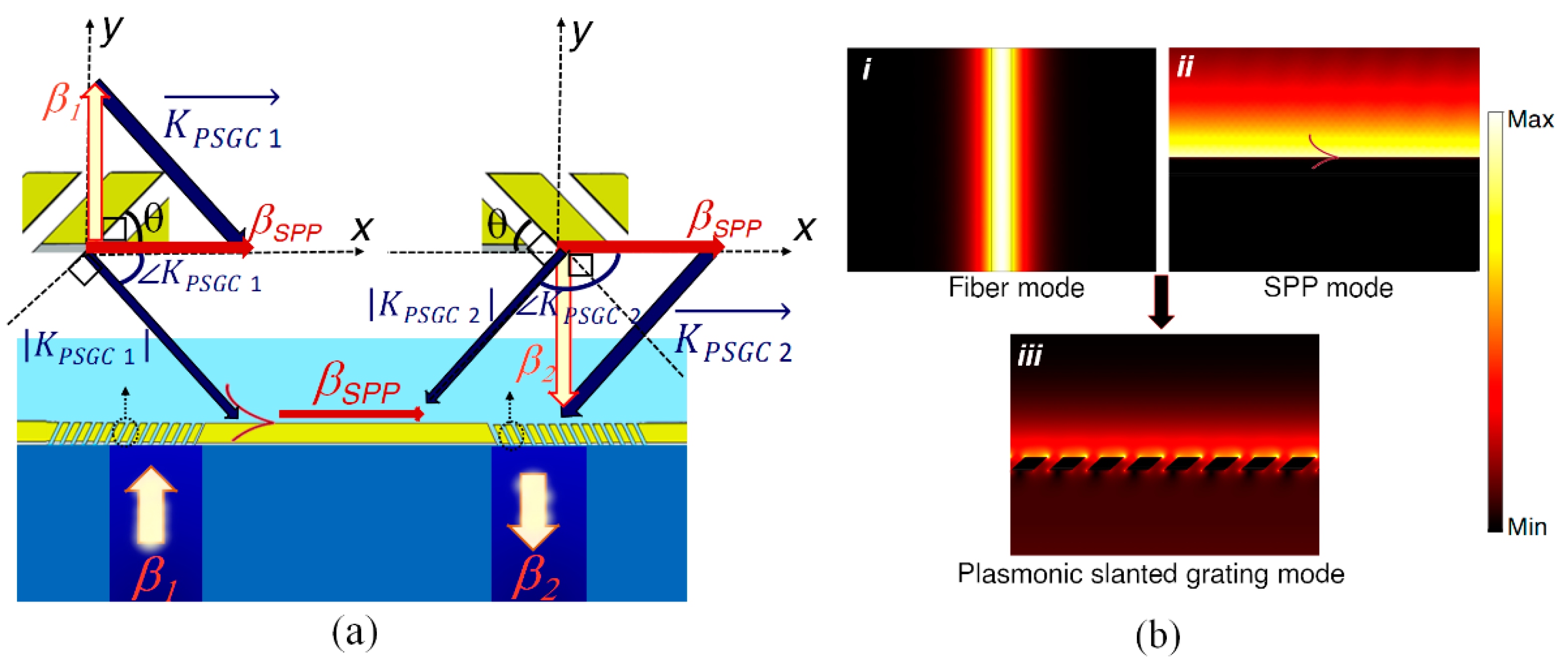
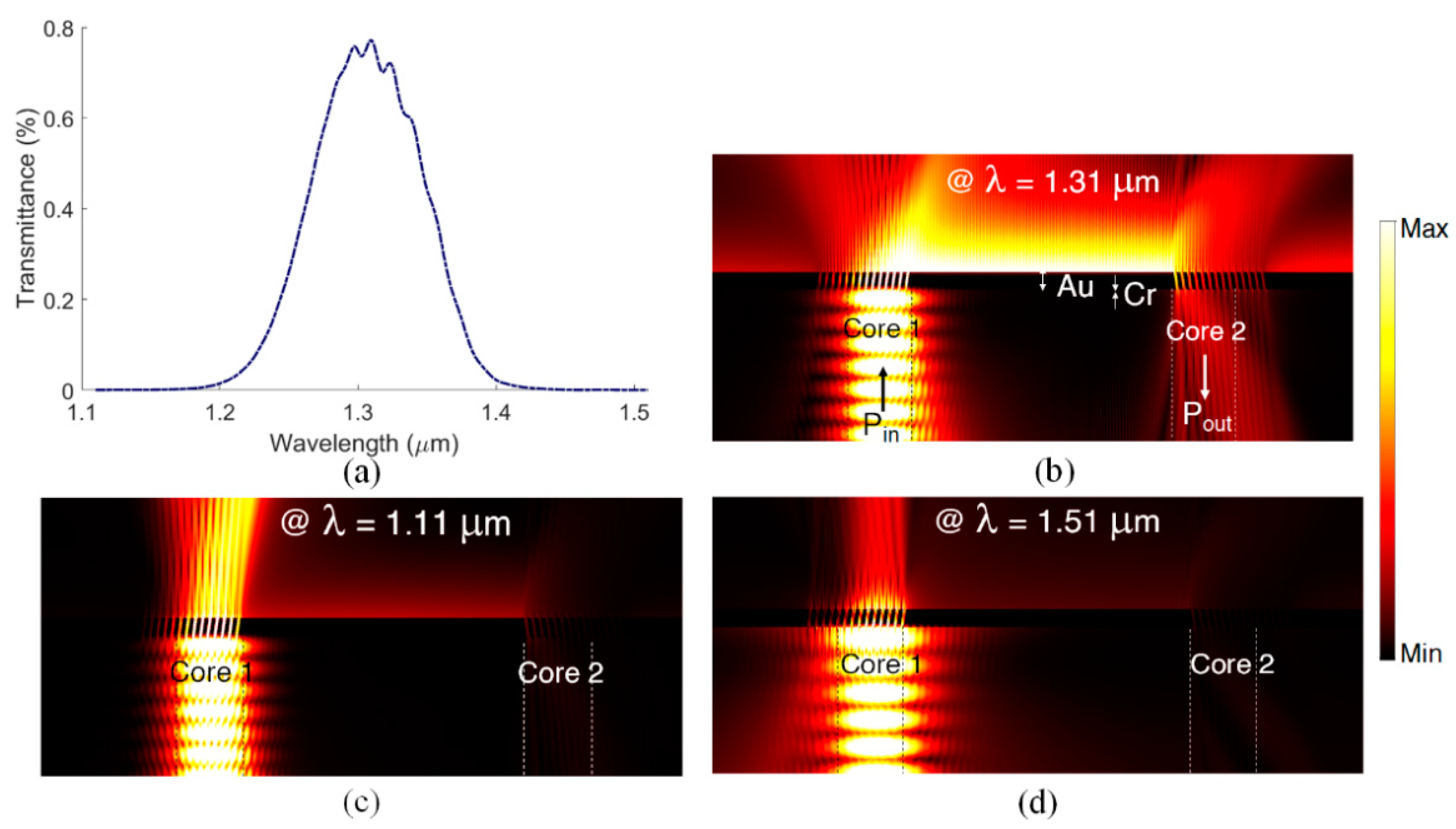
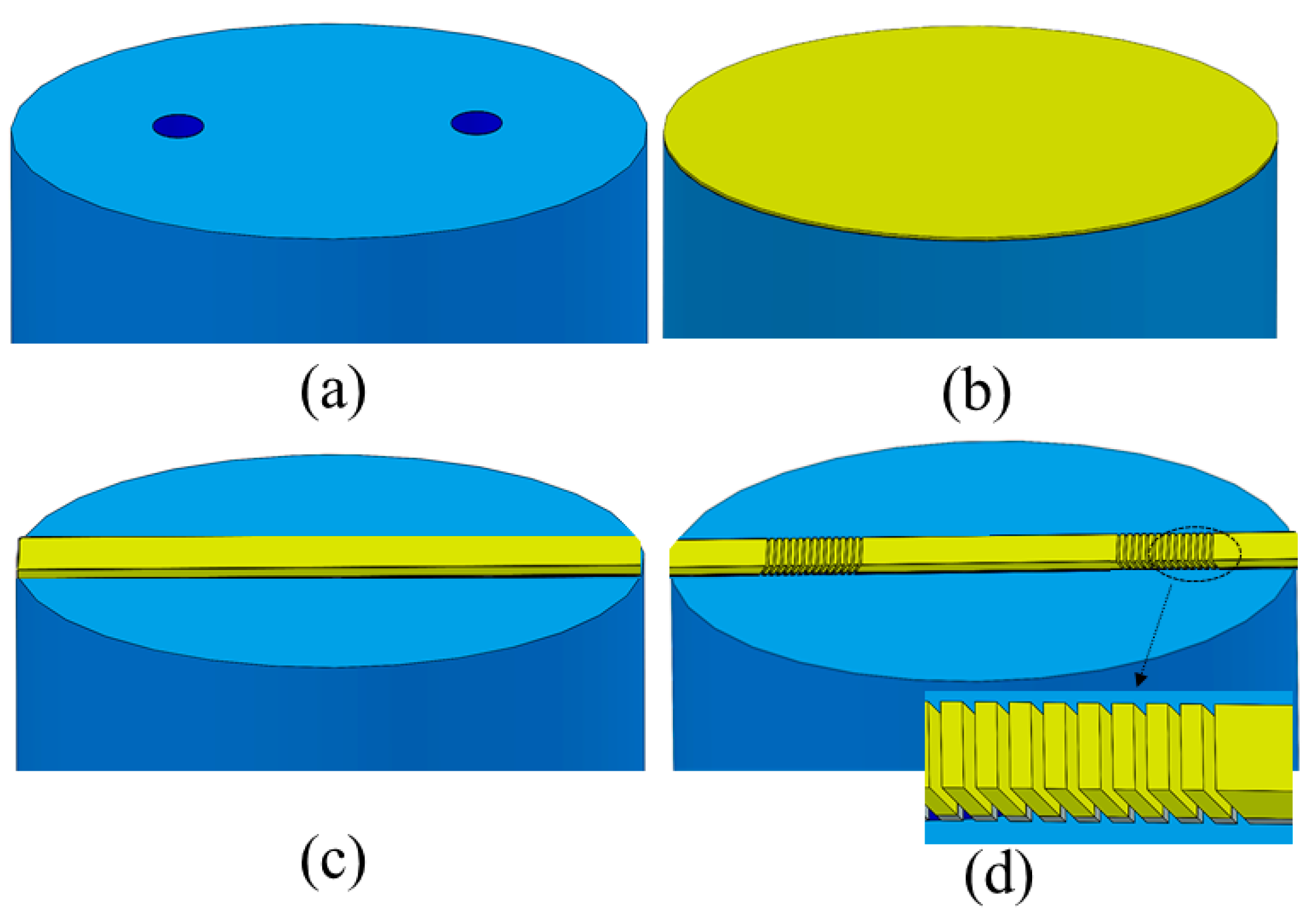

| Parameter | Definition | Parameter Value |
|---|---|---|
| θ | slant angle | 42.8° |
| Λ | grating pitch | 971.67 nm (initial) 980.72 nm (scaled) |
| ff | fill factor | 60% |
| w | grating width | 583 nm (initial) 588.43 nm (scaled) |
| HCr | chromium height | 50 nm |
| HAu | gold height | 300 nm |
| Dc | core diameter | 8 μm |
| L | grating length on the core | 8 μm |
| Loff-b | backward offset length | 6 μm |
| Loff-f | forward offset length | 0 |
| Lt | total grating length (L + Loff-b) | 16 μm |
| Dinter | intermediate distance between the cores | 46.6 μm |
| Structure | S (nm/RIU) | RIU Range | Wavelength Range (nm) | Wavelength Interrogation Mode * | Data Type | Optical Fiber |
|---|---|---|---|---|---|---|
| SPR [1] | 420 | 1.330–1.365 | 650–950 | R | Experimental | MMF |
| SPR [42] | 595 | 1.341–1.368 | 810–930 | T/R | Experimental/Numerical | MMF |
| SPR [43] | 2300 | 1–1.38 | 1250–1650 | R | Experimental/Numerical | SMF |
| SPR [44] | 294 | 1.32–1.38 | 700–1100 | R | Experimental/Numerical | SMF |
| SPR/LSPR [33] | 166.67–233.33 | 1–1.3 | 600–1100 | T | Experimental/Numerical | PCF |
| LSPR [45] | 125 | 1–1.4 | 1200–1550 | R | Experimental/Numerical | SMF |
| LSPR [46] | 755 | 1–1.5 | 800–1800 | R | Experimental/Numerical | SMF |
| FR [47] | 400 | 1.32–1.35 | 1220–1420 | R | Experimental/Numerical | SMF |
| FPI [48] | 5 | 1.31–1.47 | 1530–1570 | R | Experimental/Numerical | SMF |
| FPI [49] | 154 | 1.333–1.443 | 1260–1300 | R | Experimental | SMF |
| Gratings/FPI [13] | 50–100 | 1.33–1.52 | 1100–1500 | T | Numerical | DCSMF |
| This work | 883.2 | 1.33–1.39 | 1100–1500 | T | Numerical | DCSMF |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahani, F.F.; Mokhtari, A.; Berini, P. Plasmonic Biosensor on the End-Facet of a Dual-Core Single-Mode Optical Fiber. Biosensors 2023, 13, 558. https://doi.org/10.3390/bios13050558
Mahani FF, Mokhtari A, Berini P. Plasmonic Biosensor on the End-Facet of a Dual-Core Single-Mode Optical Fiber. Biosensors. 2023; 13(5):558. https://doi.org/10.3390/bios13050558
Chicago/Turabian StyleMahani, Fatemeh Fouladi, Arash Mokhtari, and Pierre Berini. 2023. "Plasmonic Biosensor on the End-Facet of a Dual-Core Single-Mode Optical Fiber" Biosensors 13, no. 5: 558. https://doi.org/10.3390/bios13050558
APA StyleMahani, F. F., Mokhtari, A., & Berini, P. (2023). Plasmonic Biosensor on the End-Facet of a Dual-Core Single-Mode Optical Fiber. Biosensors, 13(5), 558. https://doi.org/10.3390/bios13050558





