Thermo-Visco-Elastometry of RF-Wave-Heated and Ablated Flesh Tissues Containing Au Nanoparticles
Abstract
1. Introduction
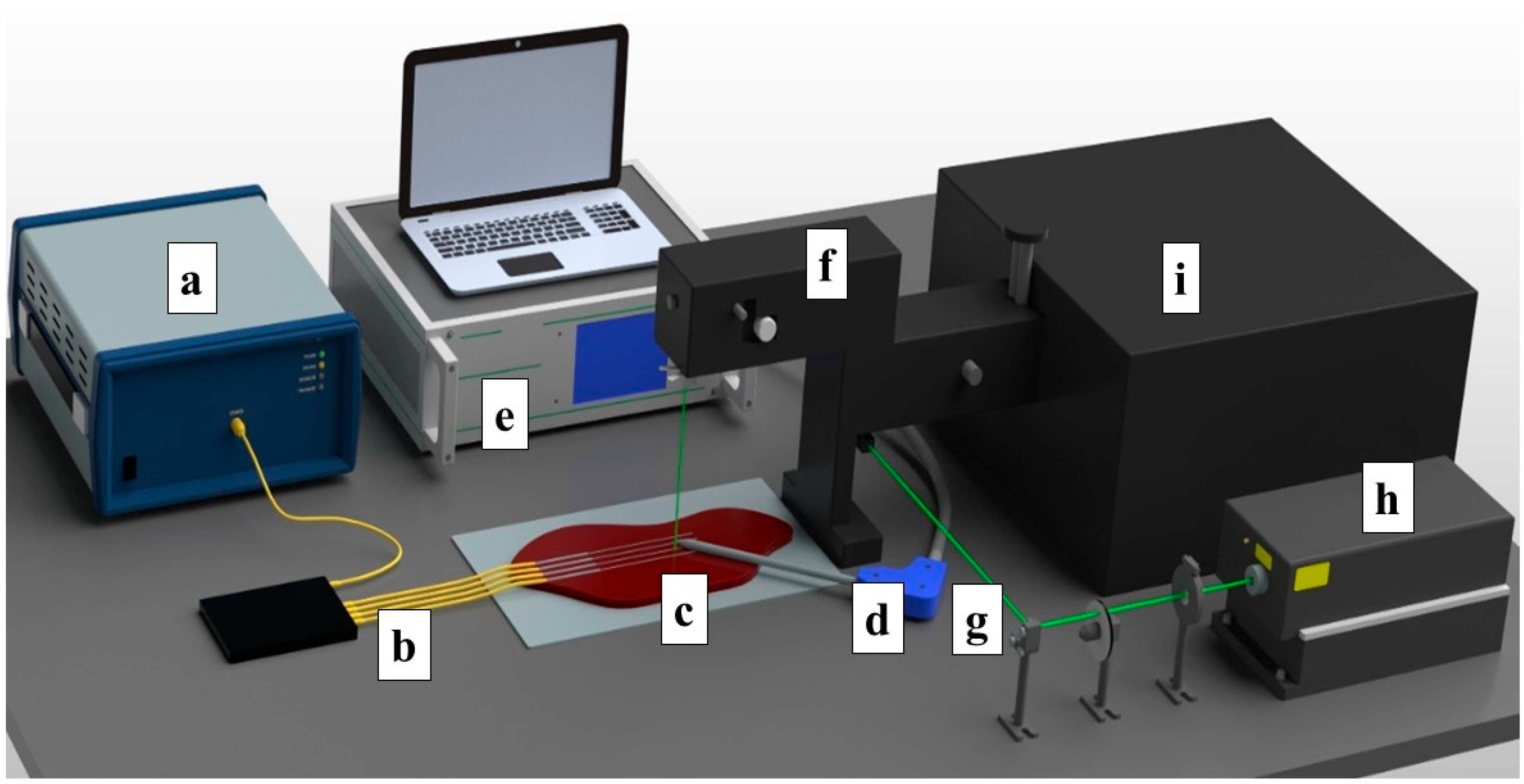
2. Materials and Methods
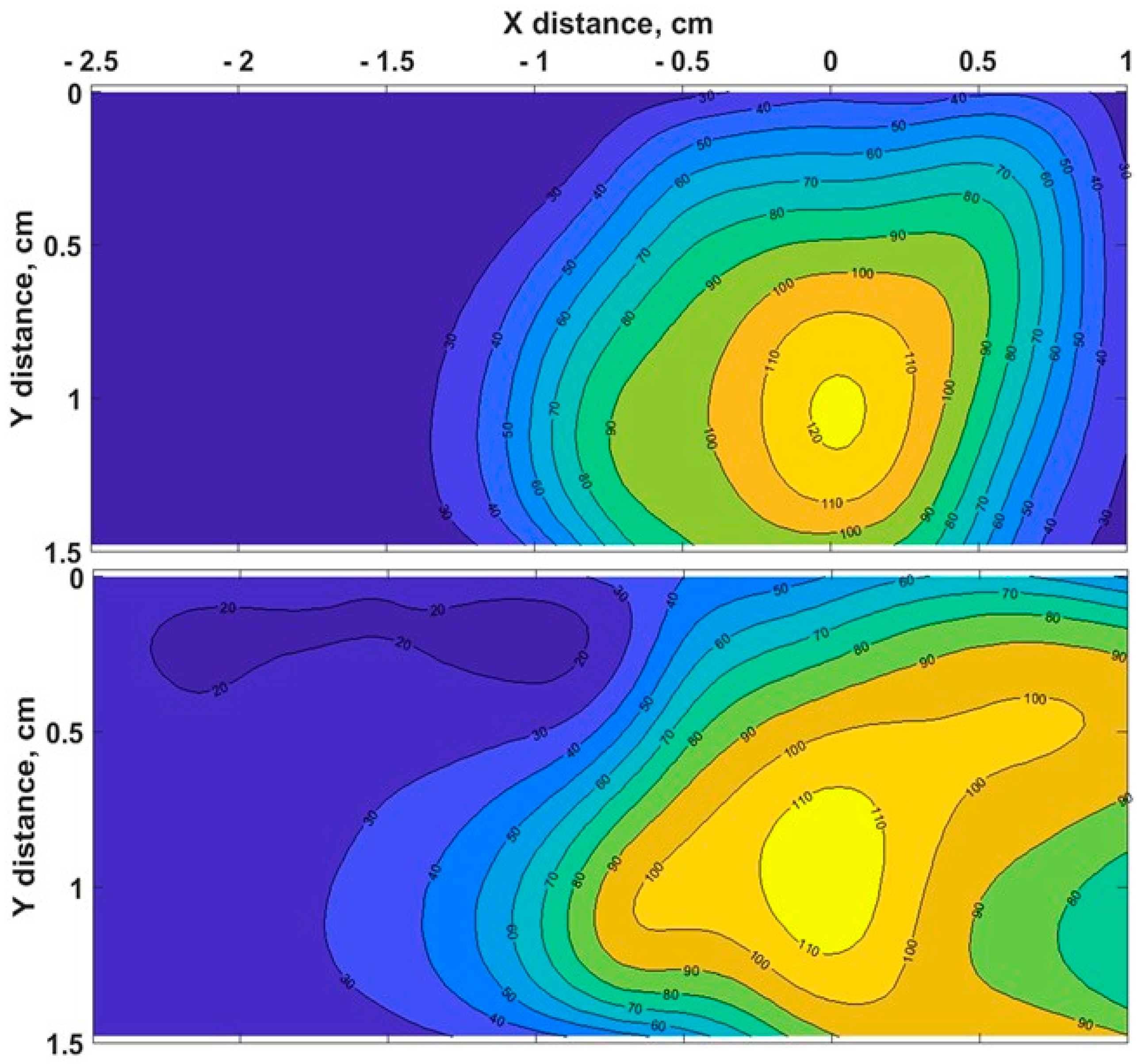
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Stauffer, P.R.; Goldberg, S.N. Introduction: Thermal ablation therapy. Int. J. Hyperth. 2004, 20, 671–677. [Google Scholar] [CrossRef] [PubMed]
- Knavel, E.M.; Brace, C.L. Tumor ablation: Common modalities and general practices. Tech. Vasc. Interv. Radiol. 2013, 16, 192–200. [Google Scholar] [CrossRef] [PubMed]
- Almeida, J.I.; Raines, J.K. Radiofrequency ablation and laser ablation in the treatment of varicose veins. Ann. Vasc. Surg. 2006, 20, 547–552. [Google Scholar] [CrossRef] [PubMed]
- Shaheen, N.J.; Sharma, P.; Overholt, B.F.; Wolfsen, H.C.; Sampliner, R.E. Radiofrequency ablation in Barrett’s esophagus with dysplasia. N. Engl. J. Med. 2009, 360, 2277–2288. [Google Scholar] [CrossRef] [PubMed]
- D’Arsonval, M.A. Action physiologique des courants alternatifs. CR Soc. Biol. 1891, 43, 283–286. [Google Scholar]
- Rossi, S.; Fornari, F.; Pathies, C.; Buscarini, L. Thermal lesions induced by 480 kHz localized current field in guinea pig and pig liver. Tumori J. 1990, 76, 54–57. [Google Scholar] [CrossRef]
- Obara, K.; Matsumoto, N.; Okamoto, M.; Kobayashi, M.; Ikeda, H.; Takahashi, H.; Katakura, Y.; Matsunaga, K.; Ishii, T.; Okuse, C. Insufficient radiofrequency ablation therapy may induce further malignant transformation of hepatocellular carcinoma. Hepatol. Int. 2008, 2, 116–123. [Google Scholar] [CrossRef]
- Bernardi, P.; Pisa, S. Specific Absorption Rate and Temperature Elevation in a Subject Exposed in the Far-Field of Radio-Frequency Sources Operating in the 10–900-MHz Range. IEEE Trans. Biomed. Eng. 2003, 50, 295–304. [Google Scholar] [CrossRef]
- Shao, Y.L.; Arjun, B.; Leo, H.L.; Chua, K.J. Nano-assisted radiofrequency ablation of clinically extracted irregularly shaped liver tumors. J. Therm. Biol. 2017, 66, 101–113. [Google Scholar] [CrossRef]
- Kennedy, L.C.; Bickford, L.R.; Lewinski, N.A.; Coughlin, A.J.; Hu, Y.; Day, E.S.; West, J.L.; Drezek, R.A. A New Era for Cancer Treatment: Gold-Nanoparticle Mediated Thermal Therapies. Phototherm. Ther. 2011, 7, 169–183. [Google Scholar] [CrossRef]
- Guo, M.; Sun, Y.; Zhang, X. Enhanced radiation therapy of gold nanoparticles in liver cancer. Appl. Sci. 2017, 7, 232. [Google Scholar] [CrossRef]
- Abadeer, N.S.; Murphy, C.J. Recent Progress in Cancer Thermal Therapy Using Gold Nanoparticles. J. Phys. Chem. C 2016, 120, 4691–4716. [Google Scholar] [CrossRef]
- Huang, P.; Marjanovic, M.; Spillman, D.R.; Odintsov, B.M.; Boppart, S.A. Magnetomotive optical coherence elastography (MM-OCE) for thermal therapy dosimetry. In Proceedings of the 3rd Conference on Optical Elastography and Tissue Biomechanics III, San Francisco, CA, USA, 13–15 February 2016. [Google Scholar]
- Attar, M.M.; Haghpanahi, M.; Shahverdi, H.; Imam, A. Thermo-mechanical analysis of soft tissue in local hyperthermia treatment. J. Mech. Sci. Technol. 2016, 30, 1459–1469. [Google Scholar] [CrossRef]
- Ezzat, M.A. The effects of thermal and mechanical material properties on tumorous tissue during hyperthermia treatment. J. Therm. Biol. 2020, 92, 102649. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Lay, R.J.; Roberts, S.K.; Chauhan, S. Towards real-time finite-strain anisotropic thermo-visco-elastodynamic analysis of soft tissues for thermal ablative therapy. Comput. Methods Programs Biomed. 2021, 198, 105789. [Google Scholar] [CrossRef]
- Grant, S.A.; Zhu, J.; Gootee, J.; Snider, C.L.; Bellrichard, M.; Grant, D.A. Gold Nanoparticle-Collagen Gels for Soft Tissue Augmentation. Tissue Eng. Part A 2018, 24, 1091–1098. [Google Scholar] [CrossRef]
- Palombo, F.; Winlove, C.; Edginton, R.S.; Green, E.; Stone, N.; Caponi, S.; Madami, M.; Fioretto, D. Biomechanics of fibrous proteins of the extracellular matrix studied by Brillouin scattering. J. R. Soc. Interface 2014, 11, 20140739. [Google Scholar] [CrossRef]
- Hammad, H.; Ma, M.; Hydamaka, A.; Elkhedir, A.; Jin, G. Effect of Freeze and Re-freeze on Chemical Composition of Beef and Poultry Meat at Storage Period 4.5 Months (SP4.5). J. Food Process. Technol. 2019, 10, 791. [Google Scholar]
- DeWall, R.; Varghese, T.; Brace, C. Quantifying Local Stiffness Variations in Radiofrequency Ablations With Dynamic Indentation. IEEE Trans. Biomed. Eng. 2012, 59, 728–735. [Google Scholar] [CrossRef]
- Chan, J.; Omana, D.; Betti, M. Functional and rheological properties of proteins in frozen turkey breast meat with different ultimate pH. Poult. Sci. 2011, 90, 1112–1123. [Google Scholar] [CrossRef]
- Dileep, A.O.; Shamasundar, B.A.; Binsi, P.K.; Badii, F.; Howell, N.K. Effect of Ice Storage on the Physicochemical and Dynamic Viscoelastic Properties of Ribbonfish (Trichiurus spp.) Meat. J. Food Sci. 2005, 70, E537–E545. [Google Scholar] [CrossRef]
- Brunton, N.; Lyng, J.; Zhang, L.; Jacquier, J. The use of dielectric properties and other physical analyses for assessing protein denaturation in beef biceps femoris muscle during cooking from 5 to 85 °C. Meat Sci. 2006, 72, 236–244. [Google Scholar] [CrossRef] [PubMed]
- Sapareto, S.; Dewey, W. Thermal dose determination in cancer therapy. Int. J. Radiat. Oncol. Biol. Phys. 1984, 10, 787–800. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Z.; Wu, S.; Wang, C.Y.; Ma, H.Y.; Lin, C.C.; Tsui, P.H. Monitoring Radiofrequency Ablation Using Real-Time Ultrasound Nakagami Imaging Combined with Frequency and Temporal Compounding Techniques. PLoS ONE 2015, 10, e0118030. [Google Scholar] [CrossRef]
- Ryu, S.; Martino, N.; Kwok, S.; Bernstein, L.; Yun, S. Label-free histological imaging of tissues using Brillouin light scattering contrast. Biomed. Opt. Express 2021, 12, 1437. [Google Scholar] [CrossRef]
- Fioretto, D.; Scarponi, F. Dynamics of a Glassy Polymer Studied by Brillouin Light Scattering. Mater. Sci. Eng. A 2009, 521, 243–246. [Google Scholar] [CrossRef]
- Almas, N.; Kurbanova, B.; Zhakiyev, N.; Rakhadilov, B.; Sagdoldina, Z.; Andybayeva, G.; Serik, N.; Alsar, Z.; Utegulov, Z.; Insepov, Z. Mechano-chemical properties of electron beam irradiated polyetheretherketone. Polymers 2022, 14, 3067. [Google Scholar] [CrossRef]
- Speziale, S.; Marquardt, H.; Duffy, T.S. Brillouin Scattering and its Application in Geosciences. Rev. Mineral. Geochem. 2014, 78, 543–603. [Google Scholar] [CrossRef]
- Akilbekova, D.; Ogay, V.; Yakupov, T.; Sarsenova, M.; Umbayev, B.; Nurakhmetov, A.; Tazhin, K.; Yakovlev, V.V.; Utegulov, Z.N. Brillouin spectroscopy and radiography for assessment of viscoelastic and regenerative properties of mammalian bones. J. Biomed. Opt. 2018, 23, 097004. [Google Scholar] [CrossRef]
- Rakymzhan, A.; Yakupov, T.; Yelemessova, Z.; Bukasov, R.; Yakovlev, V.V.; Utegulov, Z.N. Time-resolved assessment of drying plants by Brillouin and Raman spectroscopies. J. Raman Spectrosc. 2019, 50, 1881–1889. [Google Scholar] [CrossRef]
- Gaipov, A.; Utegulov, Z.; Bukasov, R.; Turebekov, D.; Tarlykov, P.; Markhametova, Z.; Nurekeyev, Z.; Kunushpayeva, Z.; Sultangaziyev, A. Development and validation of hybrid Brillouin-Raman spectroscopy for non-contact assessment of mechano-chemical properties of urine proteins as biomarkers of kidney diseases. BMC Nephrol. 2020, 21, 229. [Google Scholar] [CrossRef] [PubMed]
- Aitekenov, S.; Abdirova, P.; Yussupova, L.; Sultangaziyev, A.; Gaipov, A.; Utegulov, Z.; Bukasov, R. Raman, Infrared and Brillouin spectroscopies of biofluids for medical diagnostics and for detection of biomarkers. Crit. Rev. Anal. Chem. 2022, 1–30. [Google Scholar]
- Antonacci, G.; Braakman, S. Biomechanics of subcellular structures by non-invasive Brillouin microscopy. Sci. Rep. 2016, 6, 37217. [Google Scholar] [CrossRef] [PubMed]
- Elsayad, K.; Werner, S.; Gallemi, M.; Kong, J.; Guajardo, E.; Zhang, L.; Jaillais, Y.; Greb, T.; Belkhadir, Y. Mapping the subcellular mechanical properties of live cells in tissues with fluorescence emission–Brillouin imaging. Sci. Signal. 2016, 9, rs5. [Google Scholar] [CrossRef]
- Troyanova-Wood, M.; Meng, Z.; Yakovlev, V.V. Differentiating melanoma and healthy tissues based on elasticity-specific Brillouin microspectroscopy. Biomed. Opt. Express 2019, 10, 1774–1781. [Google Scholar] [CrossRef]
- Harley, R.; James, D.; Miller, A.; White, J.W. Phonons and the elastic moduli of collagen and muscle. Nature 1977, 267, 285–287. [Google Scholar] [CrossRef]
- Cusack, S.; Miller, A. Determination of the Elastic Constants of Collagen by Brillouin Light Scattering. J. Mol. Biol. 1979, 135, 39–51. [Google Scholar] [CrossRef]
- Berovic, N.; Thomas, N.; Thornhill, R.A.; Vaughan, J.M. Observation of Brillouin scattering from single muscle fibres. Eur. Biophys. J. 1989, 17, 69–74. [Google Scholar] [CrossRef]
- Yoshihara, A.; Miyazaki, A.; Maeda, T.; Fukushima, M.; Abe, T. Brillouin Light Scattering from Thin Albumen of Chicken Egg. J. Phys. Soc. Jpn. 2010, 79, 125001. [Google Scholar] [CrossRef]
- Beisenova, A.; Issatayeva, A.; Sovetov, S.; Korganbayev, S.; Jebuldina, M.; Ashikbayeva, Z.; Blanc, W.; Schena, E.; Sales, S.; Moraldi, C.; et al. Multi-fiber distributed thermal profiling of minimally invasive thermal ablation with scattering-level multiplexing in MgO-doped fibers. Biomed. Opt. Express 2019, 10, 1282–1296. [Google Scholar] [CrossRef]
- Martines-Arano, H.; Palacios-Barreto, S.; Castillo-Cruz, J.; Meda-Campana, J.A.; García-Pérez, B.E.; Torres-Torres, C. Fractional photodamage triggered by chaotic attractors in human lung epithelial cancer cells. Int. J. Therm. Sci. 2022, 181, 107734. [Google Scholar] [CrossRef]
- Coker, Z.; Troyanova-Wood, M.; Traverso, A.J.; Yakupov, T.; Utegulov, Z.; Yakovlev, V.V. Assessing performance of modern Brillouin spectrometers. Opt. Express 2018, 26, 2400–2409. [Google Scholar] [CrossRef] [PubMed]
- Ashikbayeva, Z.; Aitkulov, A.; Jelbuldina, M.; Issatayeva, A.; Beisenova, A.; Molardi, C.; Saccomandi, P.; Blanc, W.; Inglezakis, V.; Tosi, D. Distributed 2D temperature sensing during nanoparticles assisted laser ablation by means of high-scattering fiber sensors. Sci. Rep. 2020, 10, 12593. [Google Scholar] [CrossRef] [PubMed]
- Turkevich, J. Colloidal Gold: Part II Colour, Coagulation, Adhesion, Alloying and Catalytic Properties. Gold Bull. 1985, 18, 125–131. [Google Scholar] [CrossRef]
- Han, S.; Bouchard, R.; Sokolov, K. Molecular photoacoustic imaging with ultra-small gold nanoparticles. Biomed. Opt. Express 2019, 10, 3472–3483. [Google Scholar] [CrossRef] [PubMed]
- Sametova, A.; Kurmashev, S.; Ashikbayeva, Z.; Amantayeva, A.; Blanc, W.; Atabaev, T.S.; Tosi, D. Fiber-Optic Distributed Sensing Network for Thermal Mapping of Gold Nanoparticles-Mediated Radiofrequency Ablation. Biosensors 2022, 12, 352. [Google Scholar] [CrossRef] [PubMed]
- Sapin-De Brosses, E.; Gennisson, J.; Pernot, M.; Fink, M.; Tanter, M. Temperature dependence of the shear modulus of soft tissues assessed by ultrasound. Phys. Med. Biol. 2010, 55, 1701–1718. [Google Scholar] [CrossRef]
- Pervin, F.; Chena, W.; Weerasooriya, T. Dynamic compressive response of bovine liver tissues. J. Mech. Behav. Biomed. Mater. 2011, 4, 76–84. [Google Scholar] [CrossRef]
- Liu, Z.; Bilston, L. On the Viscoelastic Character of Liver Tissue: Experiments and Modelling of the Linear Behaviour. Biorheology 2000, 37, 191–201. [Google Scholar]
- Bianchi, L.; Cavarzan, F.; Ciampitti, L.; Cremonesi, M.; Grilli, F.; Saccomandi, P. Thermophysical and mechanical properties of biological tissues as a function of temperature: A systematic literature review. Int. J. Hyperth. 2022, 39, 297–340. [Google Scholar] [CrossRef]
- Tornberg, E. Effects of heat on meat proteins–Implications on structure and quality of meat products. Meat Sci. 2005, 70, 493–508. [Google Scholar] [CrossRef] [PubMed]
- Tseng, H.; Lin, S.; Chang, Y.; Chen, M.; Hung, S. Determining the critical effective temperature and heat dispersal pattern in monopolar radiofrequency ablation using temperature-time integration. Exp. Ther. Med. 2016, 11, 763–768. [Google Scholar] [CrossRef] [PubMed]
- Kiss, M.Z.; Daniels, M.J.; Varghese, T. Investigation of temperature-dependent viscoelastic properties of thermal lesions in ex vivo animal liver tissue. J. Biomech. 2009, 42, 959–966. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Wex, C.; Arndt, S.; Brandstadter, K.; Hermann, L.; Bruns, C. Biomechanical Characterization of Material Properties of Porcine Liver After Thermal Treatment. Soft Mater. 2014, 12, 411–419. [Google Scholar] [CrossRef]
- Li, H.; Flé, G.; Bhatt, M.; Qu, Z.; Ghazavi, S.; Yazdani, L.; Bosio, G.; Rafati, I.; Cloutier, G. Viscoelasticity Imaging of Biological Tissues and Single Cells Using Shear Wave Propagation. Front. Phys. 2021, 9, 666192. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kurbanova, B.; Ashikbayeva, Z.; Amantayeva, A.; Sametova, A.; Blanc, W.; Gaipov, A.; Tosi, D.; Utegulov, Z. Thermo-Visco-Elastometry of RF-Wave-Heated and Ablated Flesh Tissues Containing Au Nanoparticles. Biosensors 2023, 13, 8. https://doi.org/10.3390/bios13010008
Kurbanova B, Ashikbayeva Z, Amantayeva A, Sametova A, Blanc W, Gaipov A, Tosi D, Utegulov Z. Thermo-Visco-Elastometry of RF-Wave-Heated and Ablated Flesh Tissues Containing Au Nanoparticles. Biosensors. 2023; 13(1):8. https://doi.org/10.3390/bios13010008
Chicago/Turabian StyleKurbanova, Bayan, Zhannat Ashikbayeva, Aida Amantayeva, Akbota Sametova, Wilfried Blanc, Abduzhappar Gaipov, Daniele Tosi, and Zhandos Utegulov. 2023. "Thermo-Visco-Elastometry of RF-Wave-Heated and Ablated Flesh Tissues Containing Au Nanoparticles" Biosensors 13, no. 1: 8. https://doi.org/10.3390/bios13010008
APA StyleKurbanova, B., Ashikbayeva, Z., Amantayeva, A., Sametova, A., Blanc, W., Gaipov, A., Tosi, D., & Utegulov, Z. (2023). Thermo-Visco-Elastometry of RF-Wave-Heated and Ablated Flesh Tissues Containing Au Nanoparticles. Biosensors, 13(1), 8. https://doi.org/10.3390/bios13010008







