Study on the Effect of Non-Symmetrical Current Distribution Controlled by Capacitor Placement in Radio-Frequency Coils for 7T MRI
Abstract
1. Introduction
2. Materials and Methods
2.1. FDTD Simulation
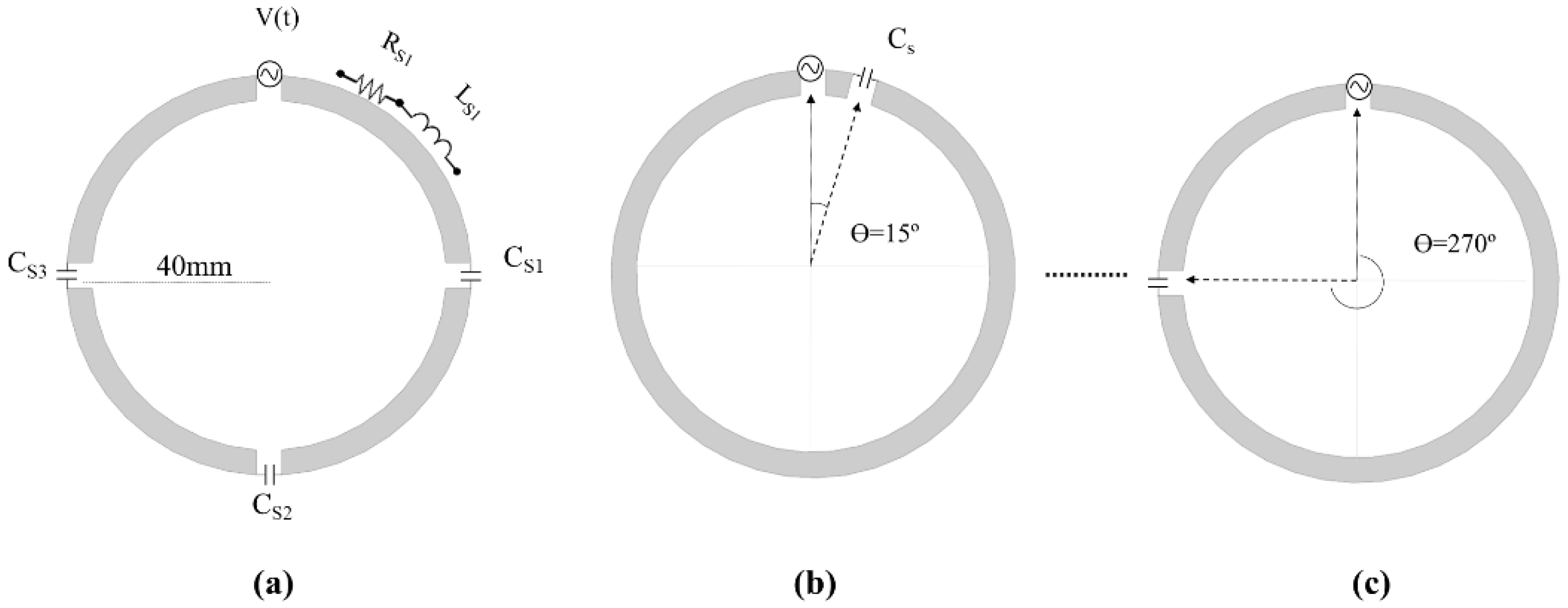
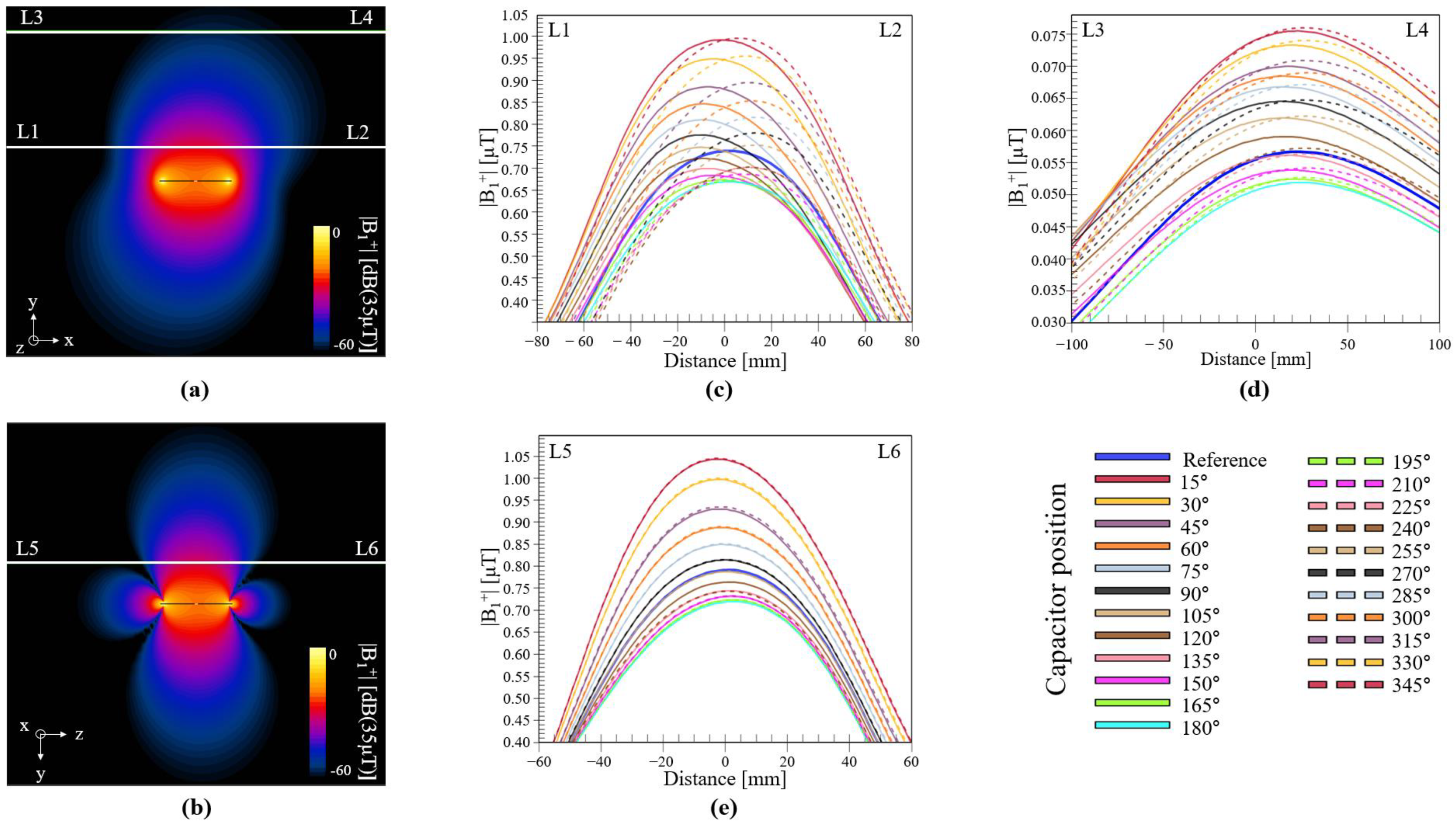
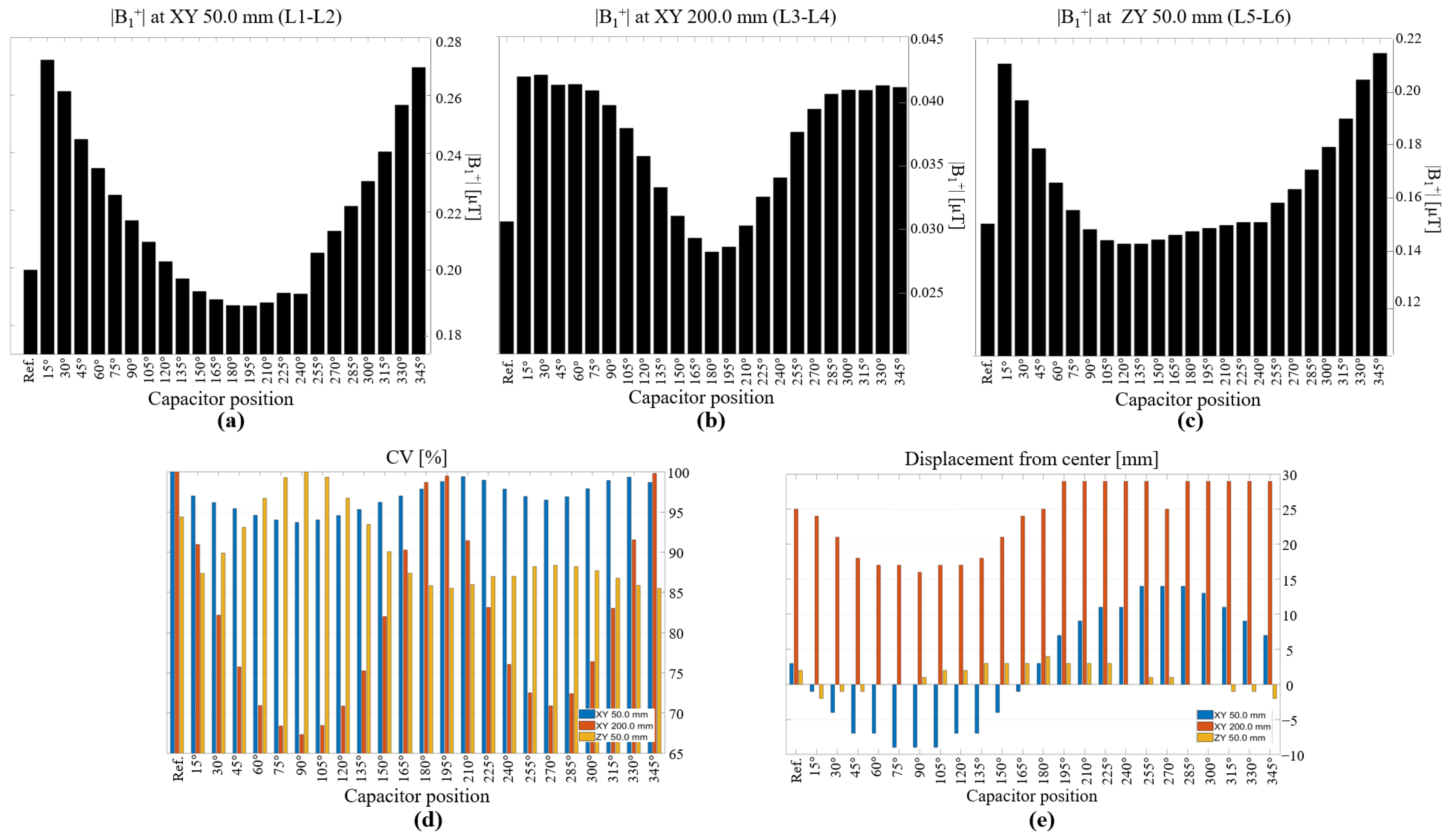
2.1.1. FDTD Simulation in Empty Space (Single Coil)
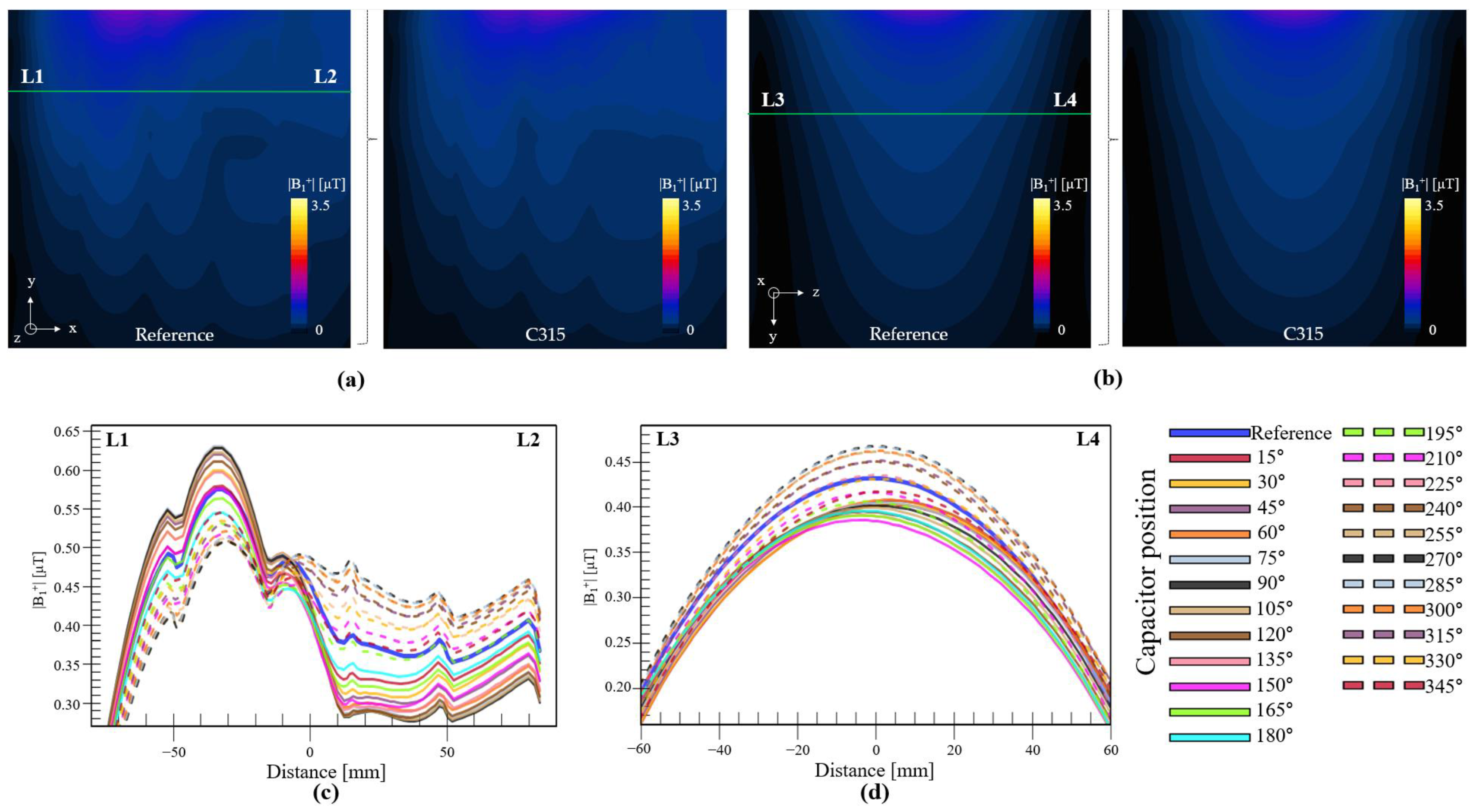
2.1.2. FDTD Simulations Phantom (Single Coil)
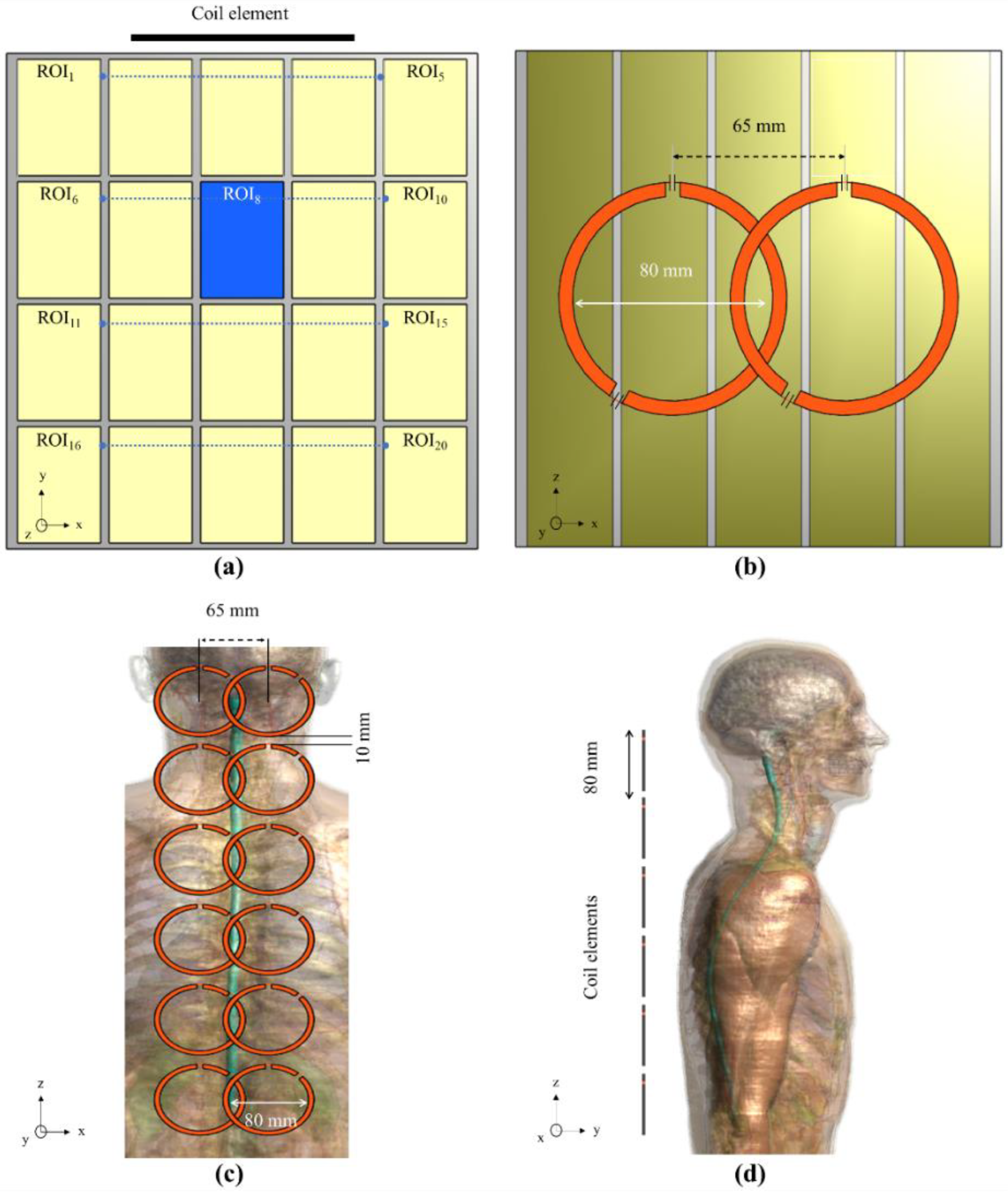
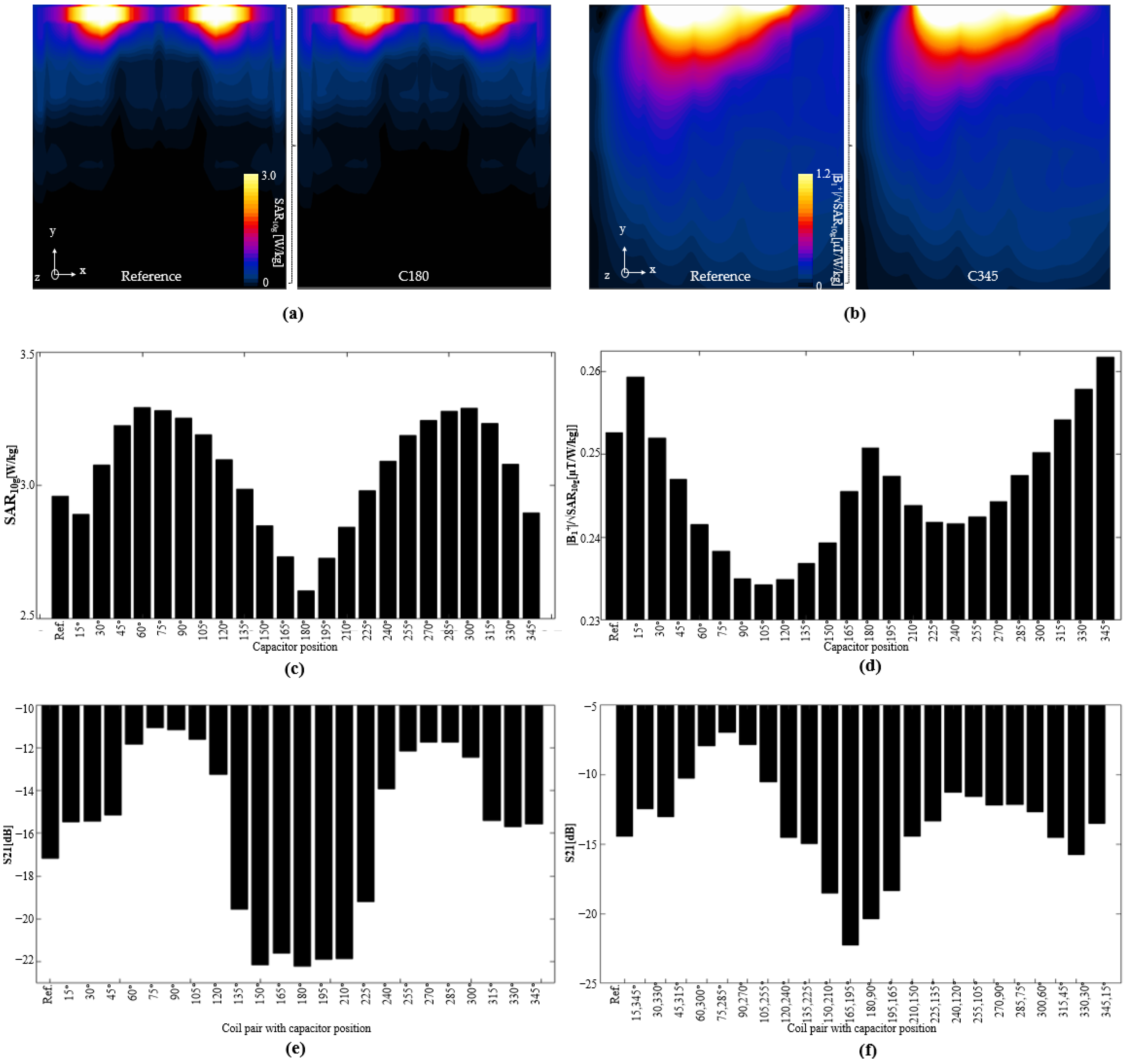
2.1.3. FDTD Simulations Phantom Coil Pairs
2.1.4. FDTD Simulation Human Spine Array Coil
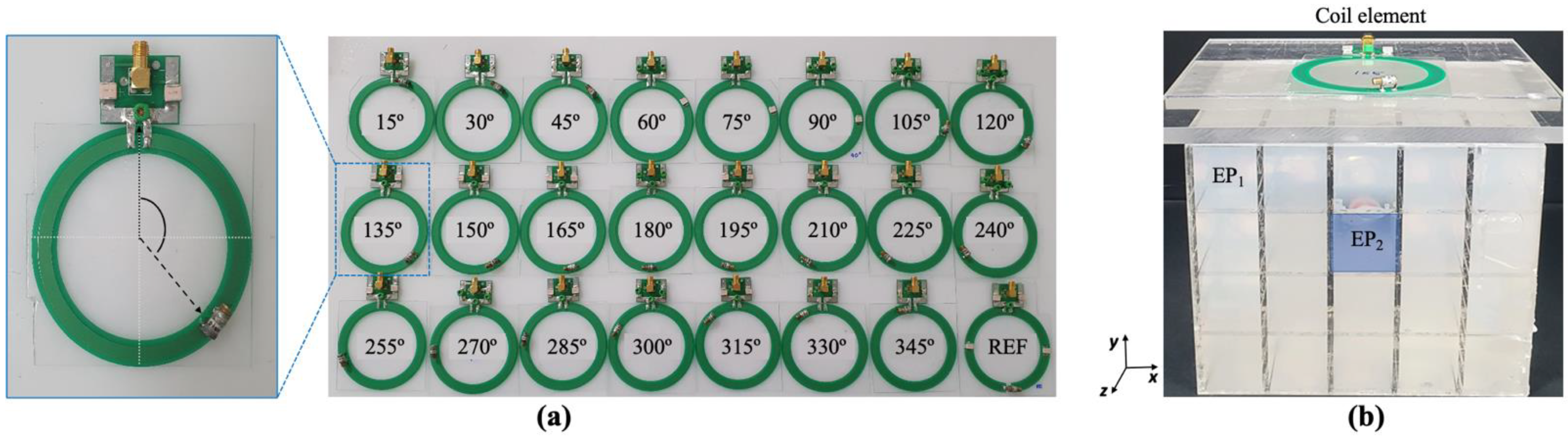
2.2. Coil Benchwork
2.3. MRI Experiment and Phantom Development
3. Results
3.1. FDTD Simulation
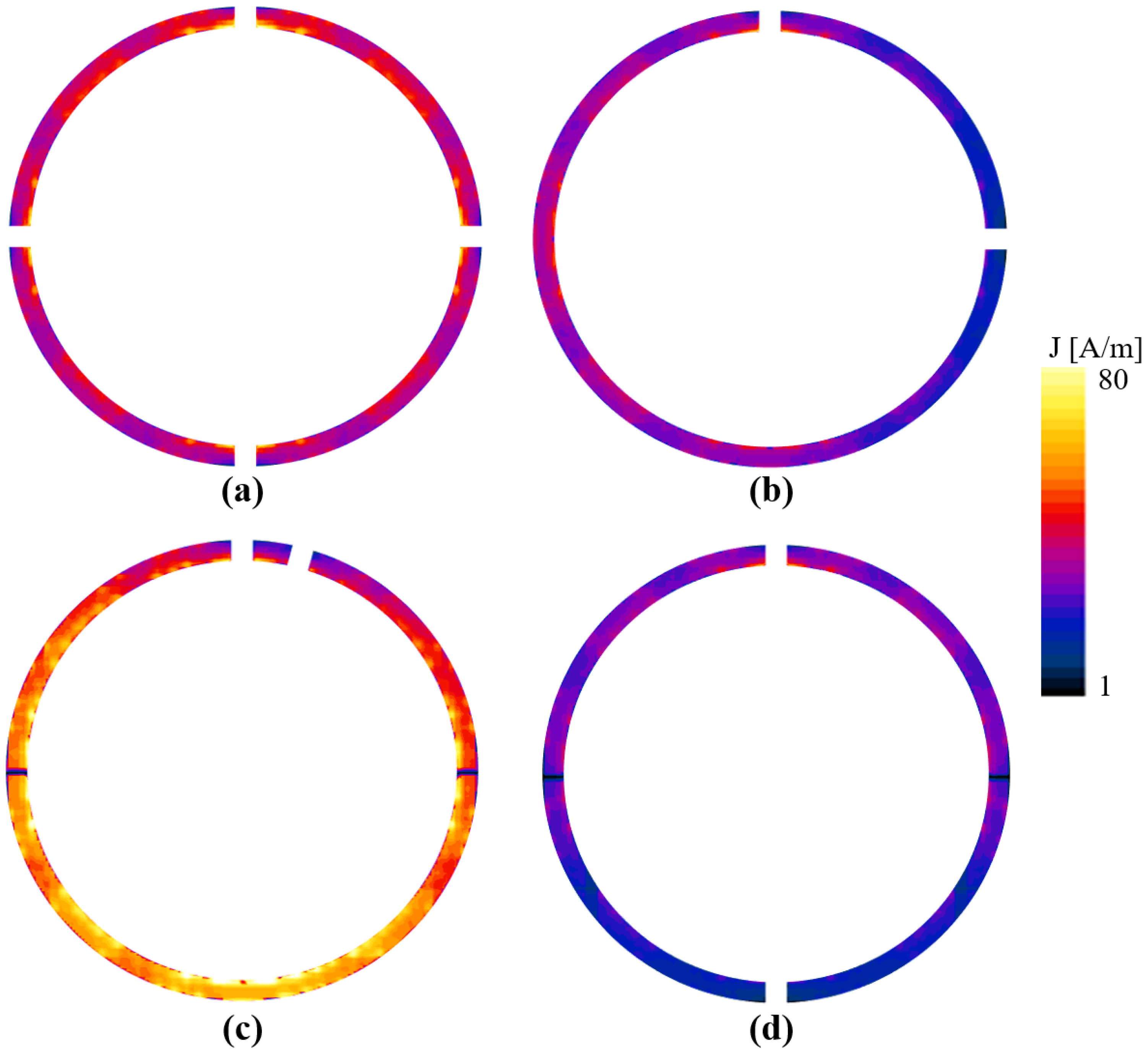
3.1.1. FDTD Simulation in Empty Space (Single Coil)
3.1.2. FDTD Simulations Phantom (Single Coil)
3.1.3. FDTD Simulations Phantom Coil Pairs
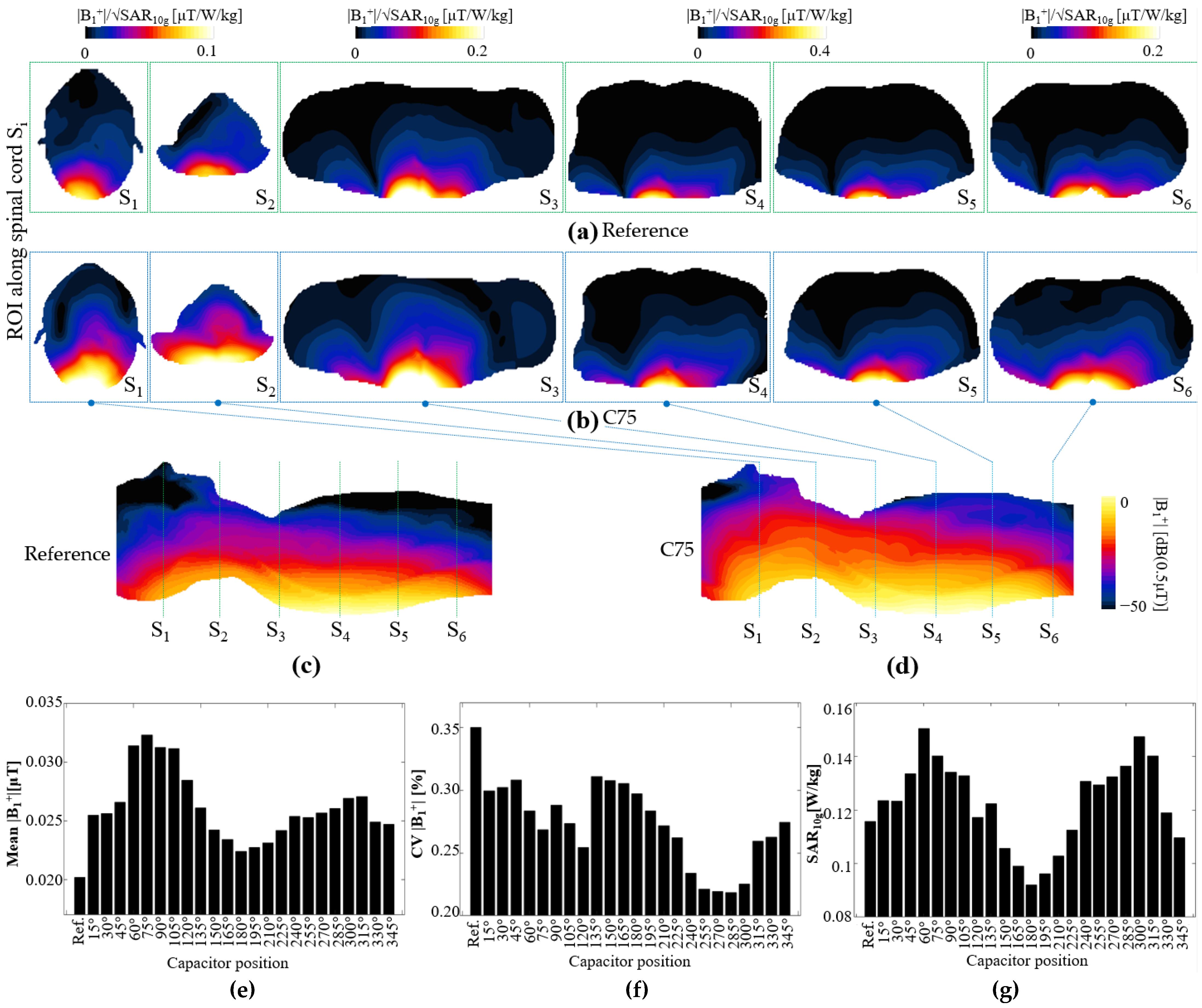
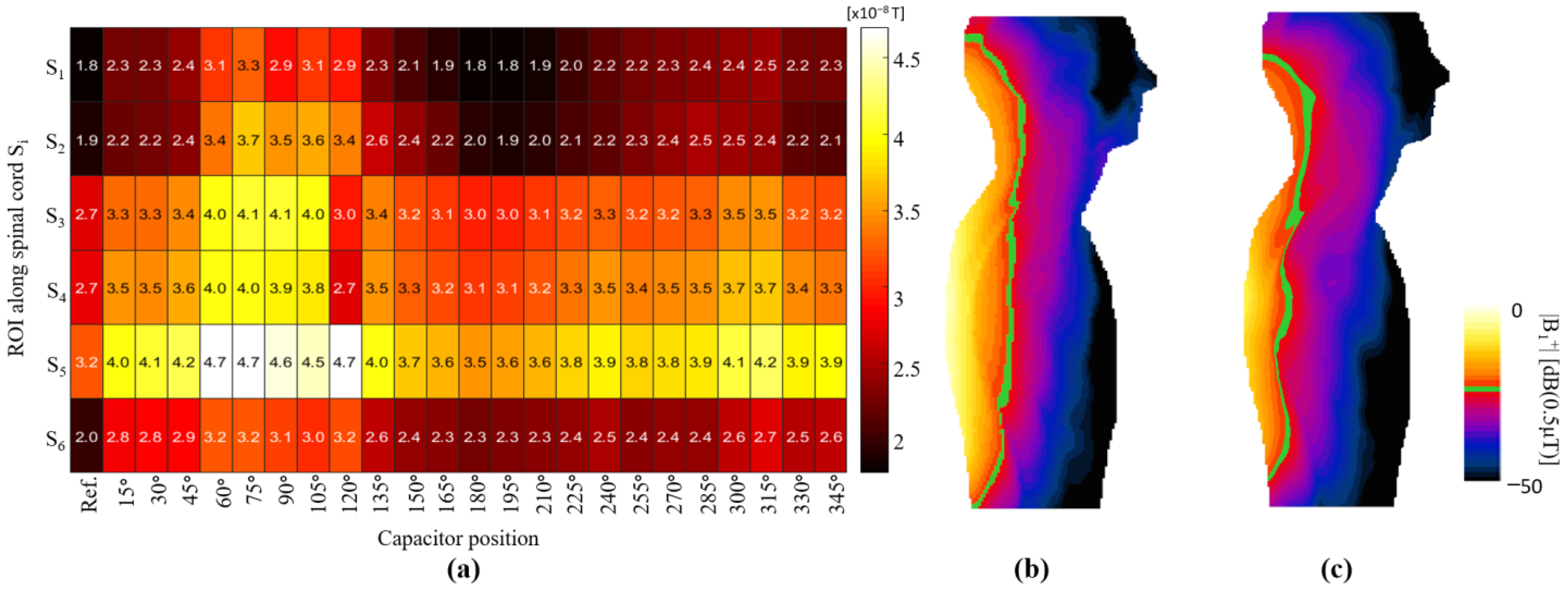
3.1.4. FDTD Simulation Human Spine Array Coil
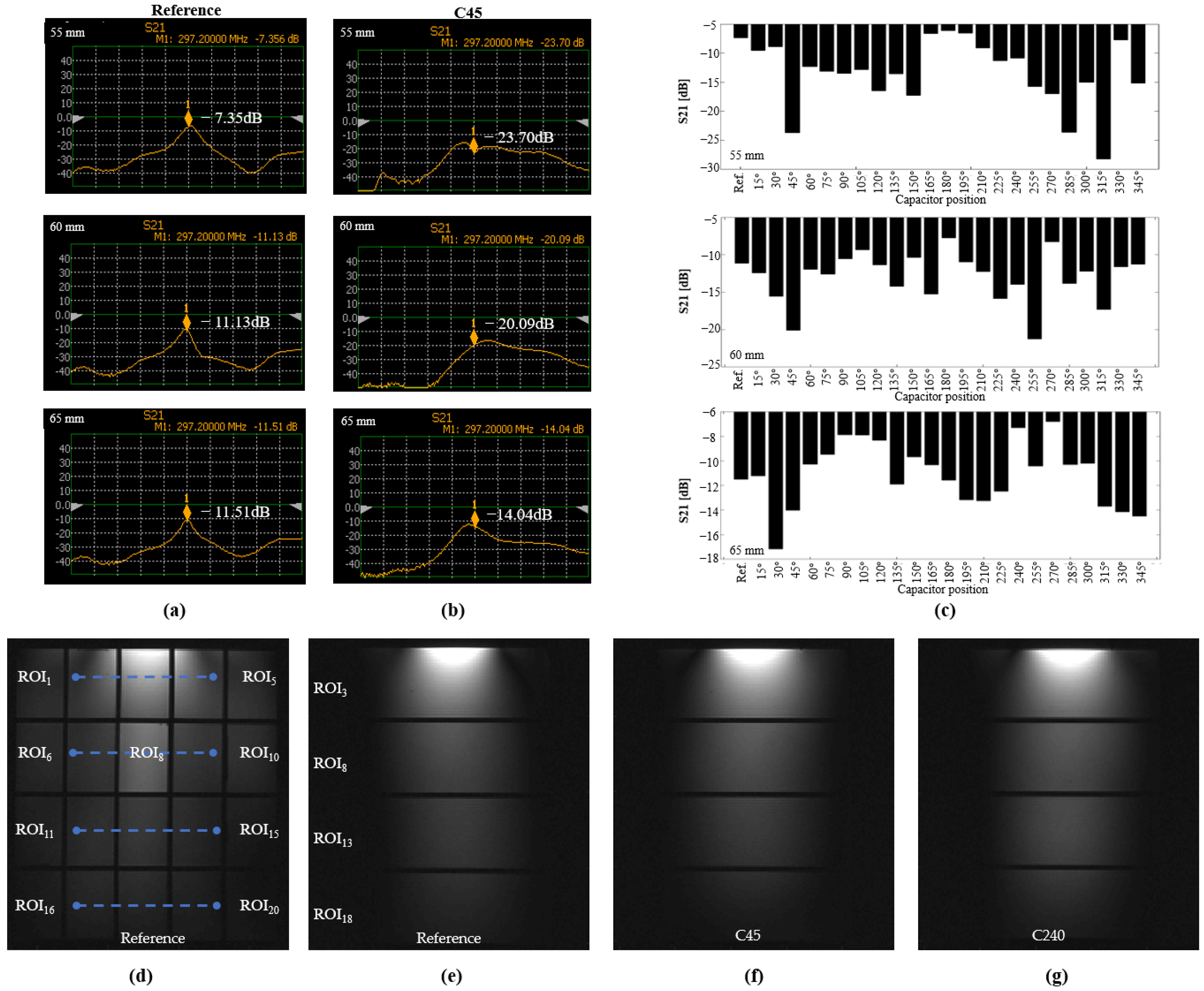
3.2. Coil Benchwork
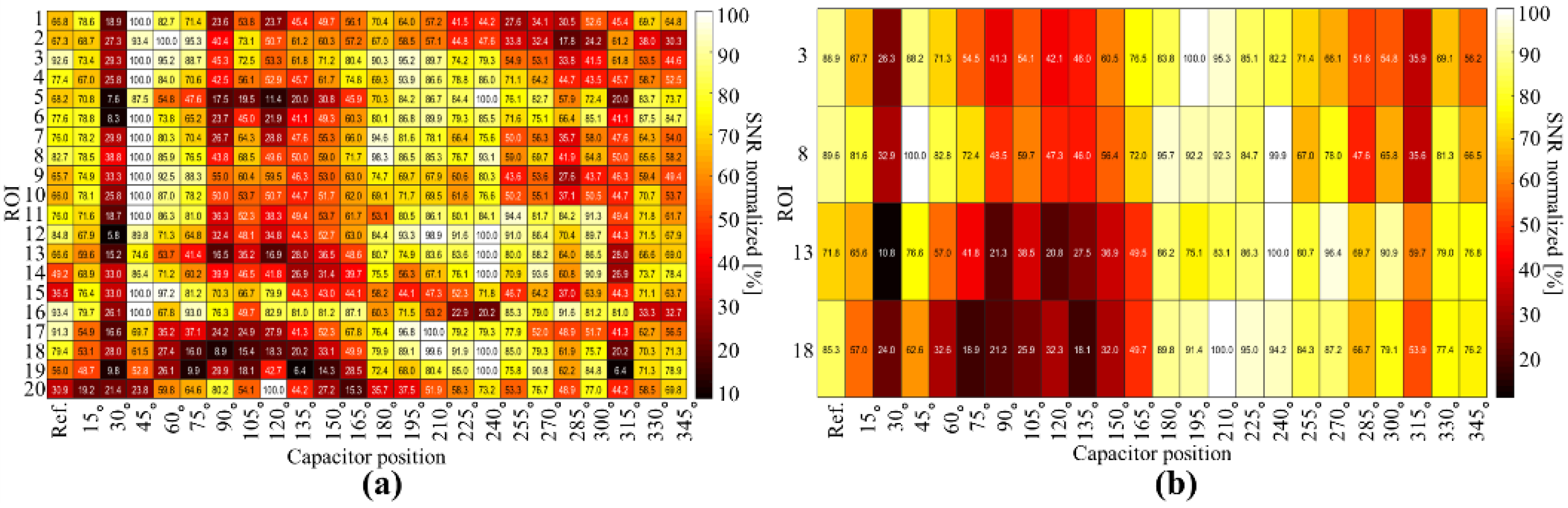
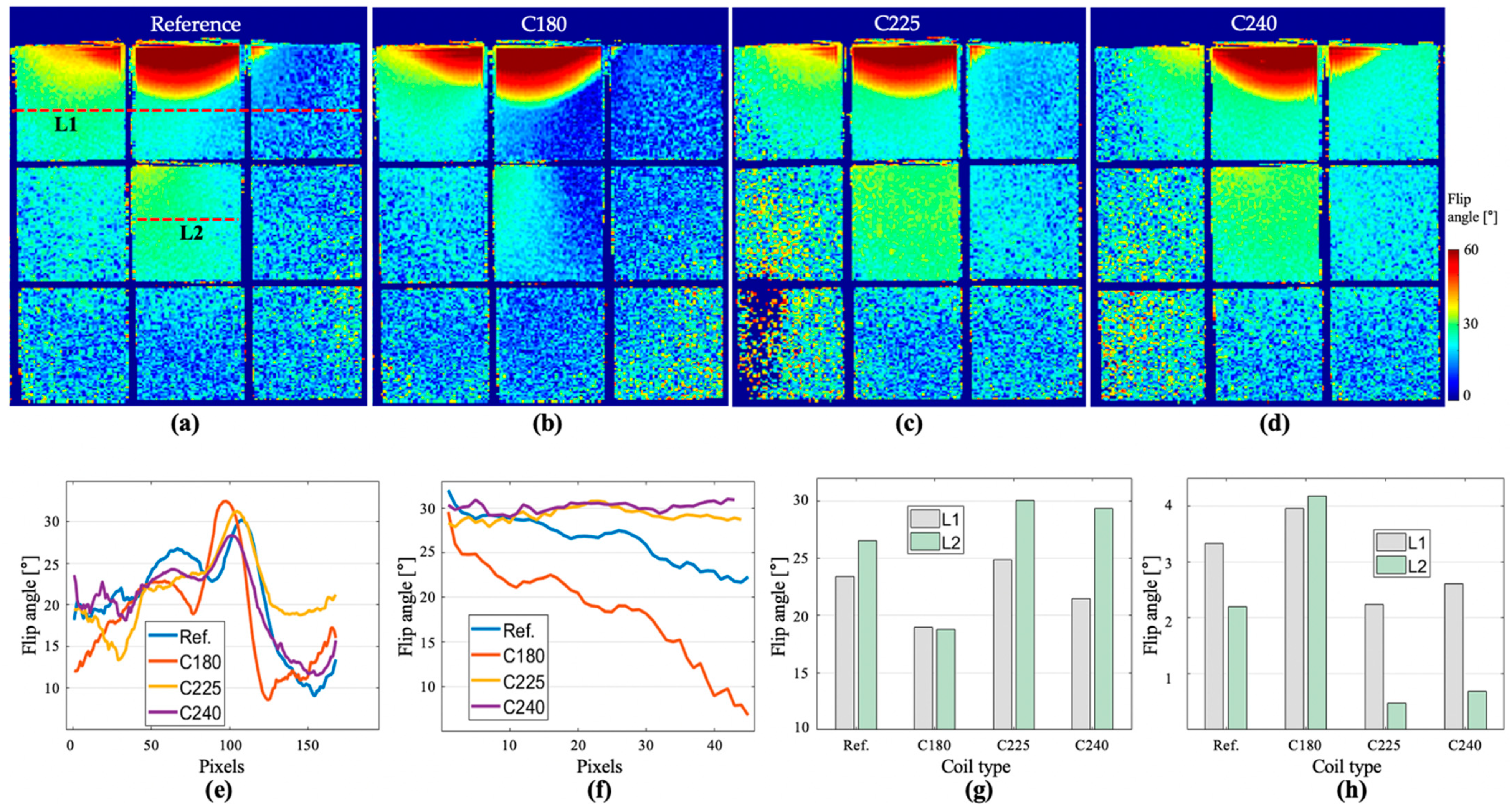
3.3. MRI Experiment
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Vaughan, J.T.; Garwood, M.; Collins, C.M.; Liu, W.; DelaBarre, L.; Adriany, G.; Andersen, P.; Merkle, H.; Goebel, R.; Smith, M.B.; et al. 7T vs. 4T: RF Power, Homogeneity, and Signal-To-Noise Comparison in Head Images. Magn. Reson. Med. 2001, 46, 24–30. [Google Scholar] [CrossRef] [PubMed]
- Uğurbil, K. Imaging at Ultrahigh Magnetic Fields: History, Challenges, and Solutions. NeuroImage 2018, 168, 7–32. [Google Scholar] [CrossRef] [PubMed]
- Slichter, C.P. Elements of Resonance. Princ. Magn. Reson. 1990, 1, 1–9. [Google Scholar]
- Ibrahim, T.S.; Hue, Y.-K.; Tang, L. Understanding and Manipulating the RF Fields at High Field MRI. NMR Biomed. 2009, 22, 927–936. [Google Scholar] [CrossRef] [PubMed]
- Seo, J.K.; Kim, D.-H.; Lee, J.; Kwon, O.I.; Sajib, S.Z.K.; Woo, E.J. Electrical Tissue Property Imaging Using MRI at Dc and Larmor Frequency. Inverse Probl. 2012, 28, 084002. [Google Scholar] [CrossRef]
- Zhang, X.; Zhu, S.; He, B. Imaging Electric Properties of Biological Tissues by RF Field Mapping in MRI. IEEE Trans. Med. Imaging 2010, 29, 474–481. [Google Scholar] [CrossRef]
- Vaughan, T.; DelaBarre, L.; Snyder, C.; Tian, J.; Akgun, C.; Shrivastava, D.; Liu, W.; Olson, C.; Adriany, G.; Strupp, J.; et al. 9.4T Human MRI: Preliminary Results. Magn. Reson. Med. 2006, 56, 1274–1282. [Google Scholar] [CrossRef]
- Park, M.; Noh, H.; Park, N. Mitigation of B1+ Inhomogeneity for Ultra-High-Field Magnetic Resonance Imaging: Hybrid Mode Shaping with Auxiliary EM Potential. Sci. Rep. 2020, 10, 11752. [Google Scholar] [CrossRef]
- Hwang, Y.; Noh, H.; Park, M.; Lee, J.; Park, N. B1+ Homogenization in 7T MRI Using Mode-Shaping with High Permittivity Materials. arXiv 2019, arXiv:1911.05313. [Google Scholar]
- Schmidt, R.; Webb, A. Improvements in RF Shimming in High Field MRI Using High Permittivity Materials with Low Order Pre-Fractal Geometries. IEEE Trans. Med. Imaging 2016, 35, 1837–1844. [Google Scholar] [CrossRef]
- Van de Moortele, P.; Akgun, C.; Adriany, G.; Moeller, S.; Ritter, J.; Collins, C.M.; Smith, M.B.; Vaughan, J.T.; Uğurbil, K. B1 Destructive Interferences and Spatial Phase Patterns at 7 T with a Head Transceiver Array Coil. Magn. Reson. Med. 2005, 54, 1503–1518. [Google Scholar] [CrossRef] [PubMed]
- Seginer, A.; Furman-Haran, E.; Goldberg, I.; Schmidt, R. Reducing SAR in 7T Brain FMRI by Circumventing Fat Suppression While Removing the Lipid Signal through a Parallel Acquisition Approach. Sci. Rep. 2021, 11, 1–13. [Google Scholar] [CrossRef] [PubMed]
- de Greef, M.; Ipek, O.; Raaijmakers, A.J.E.; Crezee, J.; van den Berg, C.A.T. Specific Absorption Rate Intersubject Variability in 7T Parallel Transmit MRI of the Head. Magn. Reson. Med. 2012, 69, 1476–1485. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; Alon, L.; Deniz, C.M.; Brown, R.; Sodickson, D.K. System and SAR Characterization in Parallel RF Transmission. Magn. Reson. Med. 2011, 67, 1367–1378. [Google Scholar] [CrossRef]
- Hernandez, D.; Kim, K.-N. A Review on the RF Coil Designs and Trends for Ultra High Field Magnetic Resonance Imaging. Investig. Magn. Reson. Imaging 2020, 24, 95. [Google Scholar] [CrossRef]
- Elabyad, I.A.; Herrmann, T.; Bernarding, J.; Omar, A. Combination of Travelling Wave Approach and Microstrip Transceiver Coil Arrays for MRI at 7T. In Proceedings of the 2011 IEEE MTT-S International Microwave Symposium, Baltimore, MD, USA, 5–10 June 2011. [Google Scholar]
- Connell, I.R.O.; Gilbert, K.M.; Abou-Khousa, M.A.; Menon, R.S. MRI RF Array Decoupling Method with Magnetic Wall Distributed Filters. IEEE Trans. Med. Imaging 2015, 34, 825–835. [Google Scholar] [CrossRef] [PubMed]
- Jasiński, K.; Młynarczyk, A.; Latta, P.; Volotovskyy, V.; Węglarz, W.P.; Tomanek, B. A Volume Microstrip RF Coil for MRI Microscopy. Magn. Reson. Imaging 2012, 30, 70–77. [Google Scholar] [CrossRef] [PubMed]
- Bogdanov, G.; Ludwig, R. Coupled Microstrip Line Transverse Electromagnetic Resonator Model for High-Field Magnetic Resonance Imaging. Magn. Reson. Med. 2002, 47, 579–593. [Google Scholar] [CrossRef] [PubMed]
- Connell, I.R.O.; Menon, R.S. Shape Optimization of an Electric Dipole Array for 7 Tesla Neuroimaging. IEEE Trans. Med. Imaging 2019, 38, 2177–2187. [Google Scholar] [CrossRef] [PubMed]
- Raaijmakers, A.J.E.; Luijten, P.R.; van den Berg, C.A.T. Dipole Antennas for Ultrahigh-Field Body Imaging: A Comparison with Loop Coils. NMR Biomed. 2015, 29, 1122–1130. [Google Scholar] [CrossRef]
- Kausar, A.S.M.Z.; Reutens, D.C.; Weber, E.; Vegh, V. Monopole Antenna Array Design for 3 T and 7 T Magnetic Resonance Imaging. PLoS ONE 2019, 14, e0214637. [Google Scholar] [CrossRef] [PubMed]
- Lakshmanan, K.; Cloos, M.; Brown, R.; Lattanzi, R.; Sodickson, D.K.; Wiggins, G.C. The “Loopole” Antenna: A Hybrid Coil Combining Loop and Electric Dipole Properties for Ultra-High-Field MRI. Concepts Magn. Reson. Part B Magn. Reson. Eng. 2020, 2020, 8886543. [Google Scholar] [CrossRef]
- Fujita, H.; Zheng, T.; Yang, X.; Finnerty, M.J.; Handa, S. RF Surface Receive Array Coils: The Art of an LC Circuit. J. Magn. Reson. Imaging 2013, 38, 12–25. [Google Scholar] [CrossRef] [PubMed]
- Giovannetti, G.; Hartwig, V.; Positano, V.; Vanello, N. Radiofrequency coils for magnetic resonance applications: Theory, design, and evaluation. Crit. Rev. Biomed. Eng. 2014, 42, 109–135. [Google Scholar] [CrossRef] [PubMed]
- Yan, X.; Gore, J.C.; Grissom, W.A. Self-Decoupled Radiofrequency Coils for Magnetic Resonance Imaging. Nat. Commun. 2018, 9, 3481. [Google Scholar] [CrossRef] [PubMed]
- Bitton, R.R.; Sheingaouz, E.; Assif, B.; Kelm, N.; Dayan, M.; Butts Pauly, K.; Ghanouni, P. Evaluation of an MRI Receive Head Coil for Use in Transcranial MR Guided Focused Ultrasound for Functional Neurosurgery. Int. J. Hyperth. 2021, 38, 22–29. [Google Scholar] [CrossRef]
- Fujita, H. New Horizons in MR Technology: RF Coil Designs and Trends. Magn. Reson. Med. Sci. 2007, 6, 29–42. [Google Scholar] [CrossRef]
- Hardy, C.J.; Cline, H.E.; Giaquinto, R.O.; Niendorf, T.; Grant, A.K.; Sodickson, D.K. 32-Element Receiver-Coil Array for Cardiac Imaging. Magn. Reson. Med. 2006, 55, 1142. [Google Scholar] [CrossRef]
- Chen, J.; Feng, Z.; Jin, J.-M. Numerical Simulation of SAR and B/Sub 1/-Field Inhomogeneity of Shielded RF Coils Loaded with the Human Head. IEEE Trans. Biomed. Eng. 1998, 45, 650–659. [Google Scholar] [CrossRef]
- Perez, M.; Hernandez, D.; Michel, E.; Cho, M.H.; Lee, S.Y. A Tool Box to Evaluate the Phased Array Coil Performance Using Retrospective 3D Coil Modeling. J. Korean Soc. Magn. Reson. Med. 2014, 18, 107. [Google Scholar] [CrossRef]
- Ibrahim, T.S.; Lee, R.; Baertlein, B.A.; Yu, Y.; Robitaille, P.-M.L. Computational Analysis of the High Pass Birdcage Resonator: Finite Difference Time Domain Simulations for High-Field MRI. Magn. Reson. Imaging 2000, 18, 835–843. [Google Scholar] [CrossRef]
- Jin, J.M.; Chen, J.; Chew, W.C.; Gan, H.; Magin, R.L.; Dimbylow, P.J. Computation of Electromagnetic Fields for High-Frequency Magnetic Resonance Imaging Applications. Phys. Med. Biol. 1996, 41, 2719–2738. [Google Scholar] [CrossRef] [PubMed]
- Gabriel, C.; Gabriel, S.; Corthout, E. The Dielectric Properties of Biological Tissues: I. Literature Survey. Phys. Med. Biol. 1996, 41, 2231–2249. [Google Scholar] [CrossRef] [PubMed]
- Christ, A.; Kainz, W.; Hahn, E.G.; Honegger, K.; Zefferer, M.; Neufeld, E.; Rascher, W.; Janka, R.; Bautz, W.; Chen, J.; et al. The Virtual Family—Development of Surface-Based Anatomical Models of Two Adults and Two Children for Dosimetric Simulations. Phys. Med. Biol. 2009, 55, N23–N38. [Google Scholar] [CrossRef] [PubMed]
- Lu, M.; Gore, J.C.; Yan, X. Over-Overlapped Loop Arrays: A Numerical Study. Magn. Reson. Imaging 2020, 72, 135–142. [Google Scholar] [CrossRef]
- Kumar, A.; Edelstein, W.A.; Bottomley, P.A. Noise Figure Limits for Circular Loop MR Coils. Magn. Reson. Med. 2009, 61, 1201–1209. [Google Scholar] [CrossRef]
- Duan, Q.; Duyn, J.H.; Gudino, N.; de Zwart, J.A.; van Gelderen, P.; Sodickson, D.K.; Brown, R. Characterization of a Dielectric Phantom for High-Field Magnetic Resonance Imaging Applications. Med. Phys. 2014, 41, 102303. [Google Scholar] [CrossRef]
- Stollberger, R.; Wach, P. Imaging of the ActiveB1 Field in Vivo. Magn. Reson. Med. 1996, 35, 246–251. [Google Scholar] [CrossRef]
- Hartwig, V.; Vanello, N.; Giovannetti, G.; De Marchi, D.; Lombardi, M.; Landini, L.; Santarelli, M.F. B1+/actual flip angle and reception sensitivity mapping methods: Simulation and comparison. Magn. Reson. Imaging 2011, 29, 717–722. [Google Scholar] [CrossRef]

| Test Scenario | Reference | Coil Type | Coil Type Value | Difference |
|---|---|---|---|---|
| Empty space |B1+| field single coil | 0.19 μT | C15 | 0.27 μT | 42% |
| Phantom |B1+| field single coil | 0.36 μT | C285 | 0.43 μT | 19.4% |
| Phantom SAR10g single coil | 2.9 W/kg | C180 | 2.6 W/Kg | −10.3% |
| Phantom transmit efficiency B1/SAR single coil | 0.251 μT/W/kg | C345 | 0.263 μT/W/kg | 4.8% |
| Phantom coupling S21 pair coils | −17 dB | C180 | −22.2 dB | 30.5% |
| Human model |B1+| field array coil | 0.020 μT | C75 | 0.032 μT | 60% |
| Human model SAR10g array coil | 0.118 W/kg | C180 | 0.09 W/kg | −23.7% |
| Benchwork measured coupling S21 pair coil | −11.8dB @65mm | C315 | −27dB @ 55mm | 128.8% |
| MR image SNR | 82.7% | C45 | 100% | 20.91% |
| MR image flip angle | 26° | C225 | 30° | 15.4% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hernandez, D.; Nam, T.; Jeong, Y.; Kim, D.; Kim, K.-N. Study on the Effect of Non-Symmetrical Current Distribution Controlled by Capacitor Placement in Radio-Frequency Coils for 7T MRI. Biosensors 2022, 12, 867. https://doi.org/10.3390/bios12100867
Hernandez D, Nam T, Jeong Y, Kim D, Kim K-N. Study on the Effect of Non-Symmetrical Current Distribution Controlled by Capacitor Placement in Radio-Frequency Coils for 7T MRI. Biosensors. 2022; 12(10):867. https://doi.org/10.3390/bios12100867
Chicago/Turabian StyleHernandez, Daniel, Taewoo Nam, Yonghwa Jeong, Donghyuk Kim, and Kyoung-Nam Kim. 2022. "Study on the Effect of Non-Symmetrical Current Distribution Controlled by Capacitor Placement in Radio-Frequency Coils for 7T MRI" Biosensors 12, no. 10: 867. https://doi.org/10.3390/bios12100867
APA StyleHernandez, D., Nam, T., Jeong, Y., Kim, D., & Kim, K.-N. (2022). Study on the Effect of Non-Symmetrical Current Distribution Controlled by Capacitor Placement in Radio-Frequency Coils for 7T MRI. Biosensors, 12(10), 867. https://doi.org/10.3390/bios12100867





