Coherent Thermal Conduction in Silicon Nanowires with Periodic Wings
Abstract
1. Introduction
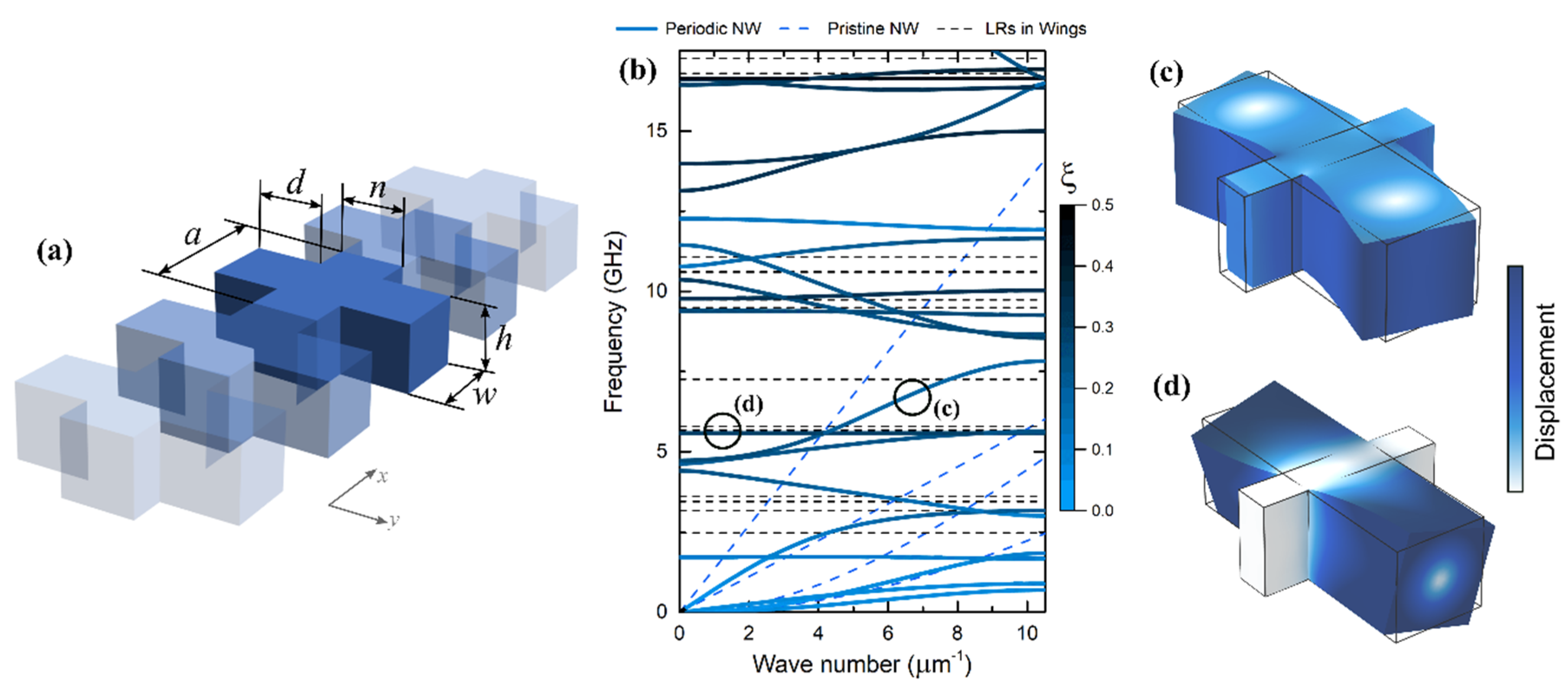
2. Materials and Methods
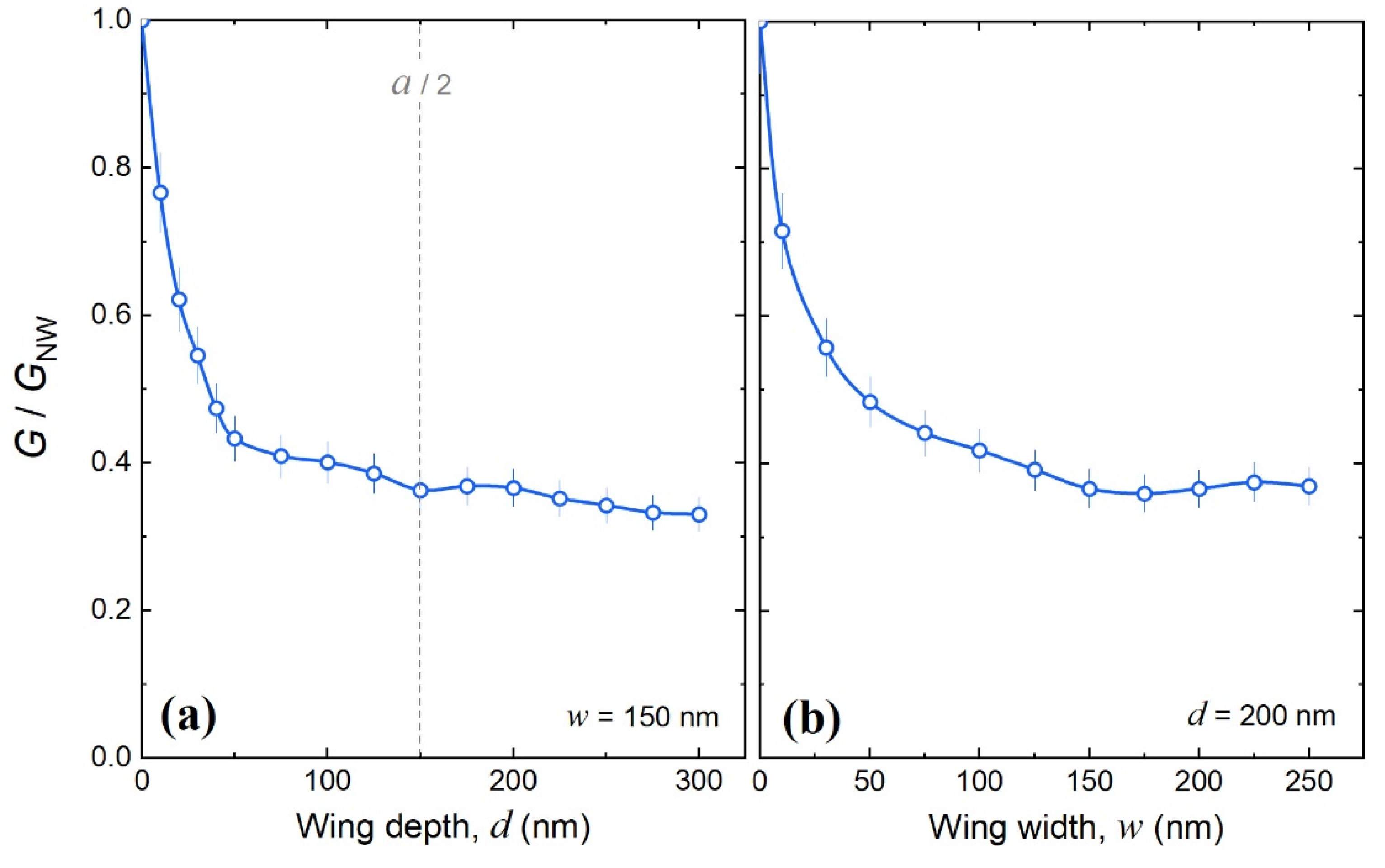
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Maldovan, M. Phonon wave interference and thermal bandgap materials. Nat. Mater. 2015, 14, 667–674. [Google Scholar] [CrossRef] [PubMed]
- Maldovan, M. Sound and heat revolutions in phononics. Nature 2013, 503, 209–217. [Google Scholar] [CrossRef] [PubMed]
- Volz, S.; Ordonez-Miranda, J.; Shchepetov, A.; Prunnila, M.; Ahopelto, J.; Pezeril, T.; Vaudel, G.; Gusev, V.; Ruello, P.; Weig, E.M.; et al. Nanophononics: State of the art and perspectives. Eur. Phys. J. B 2016, 89, 15. [Google Scholar] [CrossRef]
- Zen, N.; Puurtinen, T.A.; Isotalo, T.J.; Chaudhuri, S.; Maasilta, I.J. Engineering thermal conductance using a two-dimensional phononic crystal. Nat. Commun. 2014, 5, 3435. [Google Scholar] [CrossRef] [PubMed]
- Maasilta, I.J.; Puurtinen, T.A.; Tian, Y.; Geng, Z. Phononic thermal conduction engineering for bolometers: From phononic crystals to radial casimir limit. J. Low Temp. Phys. 2015, 184, 211–216. [Google Scholar] [CrossRef]
- Anufriev, R.; Nomura, M. Reduction of thermal conductance by coherent phonon scattering in two-dimensional phononic crystals of different lattice types. Phys. Rev. B 2016, 93, 045410. [Google Scholar] [CrossRef]
- Puurtinen, T.A.; Maasilta, I.J. Low temperature heat capacity of phononic crystal membranes. AIP Adv. 2016, 6, 121902. [Google Scholar] [CrossRef]
- Puurtinen, T.A.; Maasilta, I.J. Low-temperature coherent thermal conduction in thin phononic crystal membranes. Crystals 2016, 6, 72. [Google Scholar] [CrossRef]
- Anufriev, R.; Nomura, M. Heat conduction engineering in pillar-based phononic crystals. Phys. Rev. B 2017, 95, 155432. [Google Scholar] [CrossRef]
- Anufriev, R.; Yanagisawa, R.; Nomura, M. Aluminium nanopillars reduce thermal conductivity of silicon nanobeams. Nanoscale 2017, 9, 15083–15088. [Google Scholar] [CrossRef]
- Sledzinska, M.; Graczykowski, B.; Alzina, F.; Santiso Lopez, J.; Sotomayor Torres, C.M. Fabrication of phononic crystals on free-standing silicon membranes. Microelectron. Eng. 2016, 149, 41–45. [Google Scholar] [CrossRef]
- Anufriev, R.; Gluchko, S.; Volz, S.; Nomura, M. Quasi-Ballistic Heat Conduction due to Lévy Phonon Flights in Silicon Nanowires. ACS Nano 2018, 12, 11928–11935. [Google Scholar] [CrossRef] [PubMed]
- Maire, J.; Anufriev, R.; Hori, T.; Shiomi, J.; Volz, S.; Nomura, M. Thermal conductivity reduction in silicon fishbone nanowires. Sci. Rep. 2018, 8, 4452. [Google Scholar] [CrossRef] [PubMed]
- Blanc, C.; Rajabpour, A.; Volz, S.; Fournier, T.; Bourgeois, O. Phonon heat conduction in corrugated silicon nanowires below the Casimir limit. Appl. Phys. Lett. 2013, 103, 043109. [Google Scholar] [CrossRef]
- Gomis-Bresco, J.; Navarro-Urrios, D.; Oudich, M.; El-Jallal, S.; Griol, A.; Puerto, D.; Chavez, E.; Pennec, Y.; Djafari-Rouhani, B.; Alzina, F.; et al. A one-dimensional optomechanical crystal with a complete phononic band gap. Nat. Commun. 2014, 5, 4452. [Google Scholar] [CrossRef]
- Poborchii, V.; Morita, Y.; Hattori, J.; Tada, T.; Geshev, P.I. Corrugated Si nanowires with reduced thermal conductivity for wide-temperature-range thermoelectricity. J. Appl. Phys. 2016, 120, 154304. [Google Scholar] [CrossRef]
- Srivastava, A.; Lu, Y. Avoided crossings and band sorting in two-dimensional phononic crystals. Health Monit. Struct. Biol. Syst. XII 2018, 10600, 106001H. [Google Scholar]
- Pourabolghasem, R.; Khelif, A.; Mohammadi, S.; Eftekhar, A.A.; Adibi, A. Physics of band-gap formation and its evolution in the pillar-based phononic crystal structures. J. Appl. Phys. 2014, 116, 013514. [Google Scholar] [CrossRef]
- Xiong, S.; Sääskilahti, K.; Kosevich, Y.A.; Han, H.; Donadio, D.; Volz, S. Blocking phonon transport by structural resonances in alloy-based nanophononic metamaterials leads to ultralow thermal tonductivity. Phys. Rev. Lett. 2016, 117, 025503. [Google Scholar] [CrossRef]
- Graczykowski, B.; Sledzinska, M.; Kehagias, N.; Alzina, F.; Reparaz, J.S.; Sotomayor Torres, C.M. Hypersonic phonon propagation in one-dimensional surface phononic crystal. Appl. Phys. Lett. 2014, 104, 123108. [Google Scholar] [CrossRef]
- Graczykowski, B.; Sledzinska, M.; Alzina, F.; Gomis-Bresco, J.; Reparaz, J.S.; Wagner, M.R.; Sotomayor Torres, C.M. Phonon dispersion in hypersonic two-dimensional phononic crystal membranes. Phys. Rev. B 2015, 91, 075414. [Google Scholar] [CrossRef]
- Anufriev, R.; Nomura, M. Phonon and heat transport control using pillar-based phononic crystals. Sci. Technol. Adv. Mater. 2018, 19, 863–870. [Google Scholar] [CrossRef] [PubMed]
- Ma, D.; Wan, X.; Yang, N. Unexpected thermal conductivity enhancement in pillared graphene nanoribbon with isotopic resonance. Phys. Rev. B 2018, 98, 245420. [Google Scholar] [CrossRef]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anufriev, R.; Nomura, M. Coherent Thermal Conduction in Silicon Nanowires with Periodic Wings. Nanomaterials 2019, 9, 142. https://doi.org/10.3390/nano9020142
Anufriev R, Nomura M. Coherent Thermal Conduction in Silicon Nanowires with Periodic Wings. Nanomaterials. 2019; 9(2):142. https://doi.org/10.3390/nano9020142
Chicago/Turabian StyleAnufriev, Roman, and Masahiro Nomura. 2019. "Coherent Thermal Conduction in Silicon Nanowires with Periodic Wings" Nanomaterials 9, no. 2: 142. https://doi.org/10.3390/nano9020142
APA StyleAnufriev, R., & Nomura, M. (2019). Coherent Thermal Conduction in Silicon Nanowires with Periodic Wings. Nanomaterials, 9(2), 142. https://doi.org/10.3390/nano9020142





