Influence of Tartrate Ligand Coordination over Luminescence Properties of Chiral Lanthanide-Based Metal–Organic Frameworks
Abstract
1. Introduction
2. Materials and Methods
2.1. Synthesis of {[Ln2(μ4-tar)2(μ-tar)(H2O)2]·xH2O}n [Where Ln(III) = Sm, Eu and Gd]
2.2. Synthesis of [Ln(μ-Htart)2(OH)(H2O)2]n [Where Ln = Y(III), Sm(III), Eu(III), Gd(III), Tb(III), Dy(III), Ho(III), Er(III), Tm(III), or Yb(III)]
2.3. Physical Measurements
2.4. X-ray Diffraction Data Collection and Structure Determination
2.5. Photophysical and Chiroptical Properties
2.6. Computational Details
3. Results and Discussion
3.1. Comments on the Synthesis of Compounds
3.2. Structural Description of {[Ln2(μ4-tar)2(μ-tar)(H2O)2]·3H2O}n [where Ln(III) = Sm, Eu and Gd]
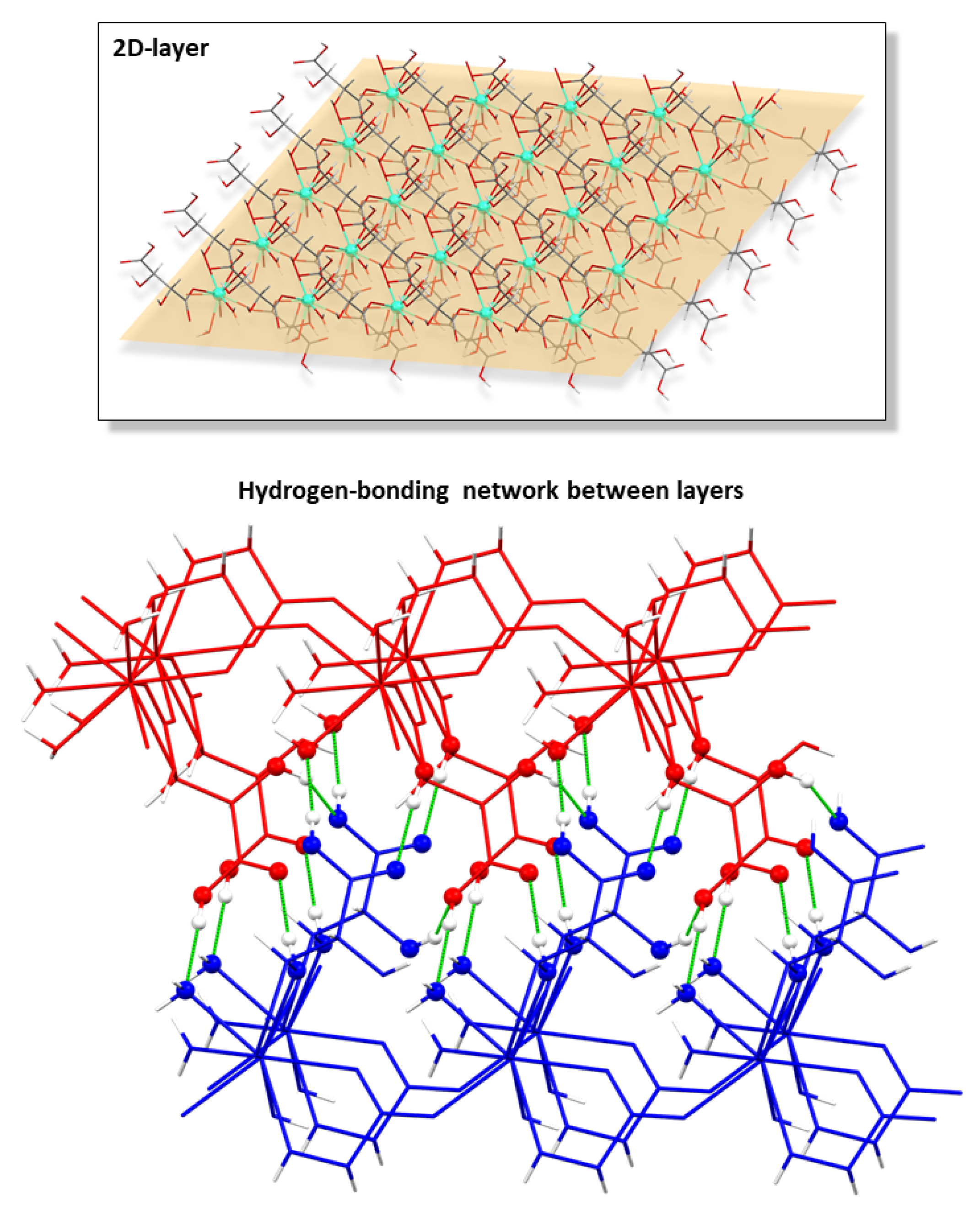
3.3. Structural Description of [Ln(μ-Htart)2(OH)(H2O)2]n [where Ln = Y(III), Sm(III), Eu(III), Gd(III), Tb(III), Dy(III), Ho(III), Er(III), Tm(III), or Yb(III)]
3.4. Thermal Evolution of the 3D and 2D Compounds
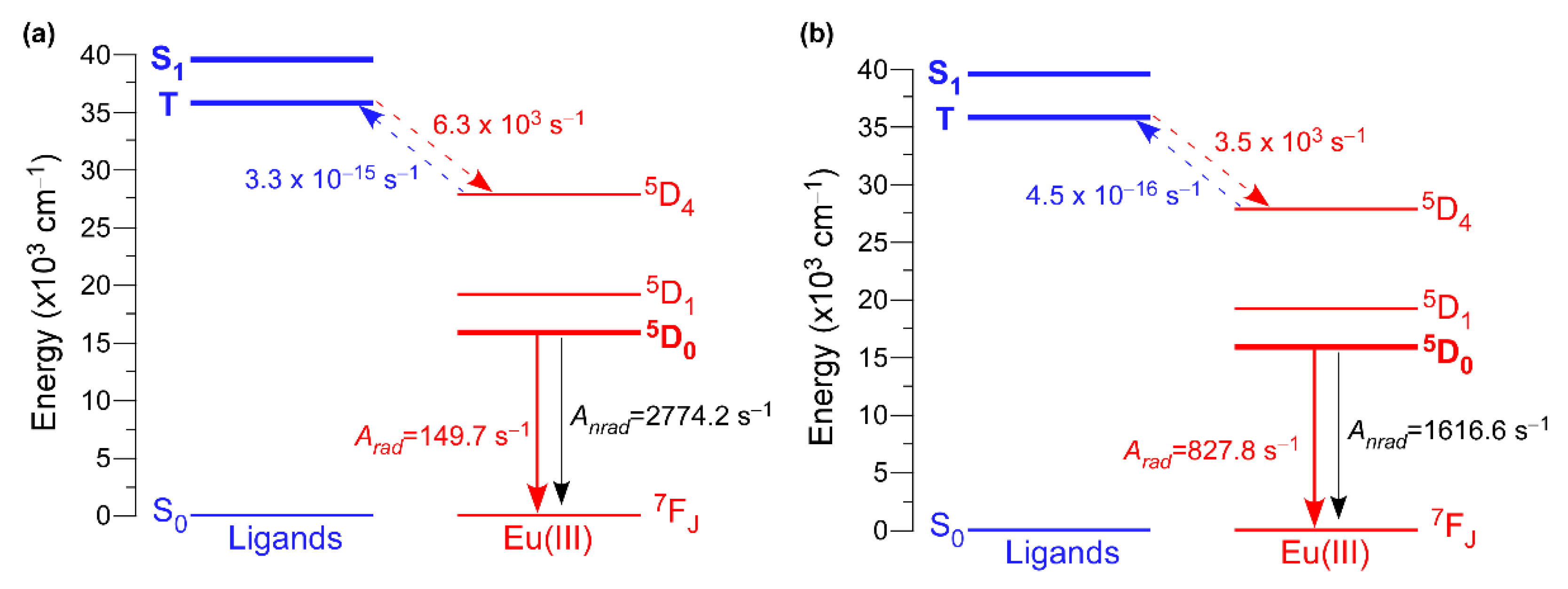
3.5. Luminescence Properties
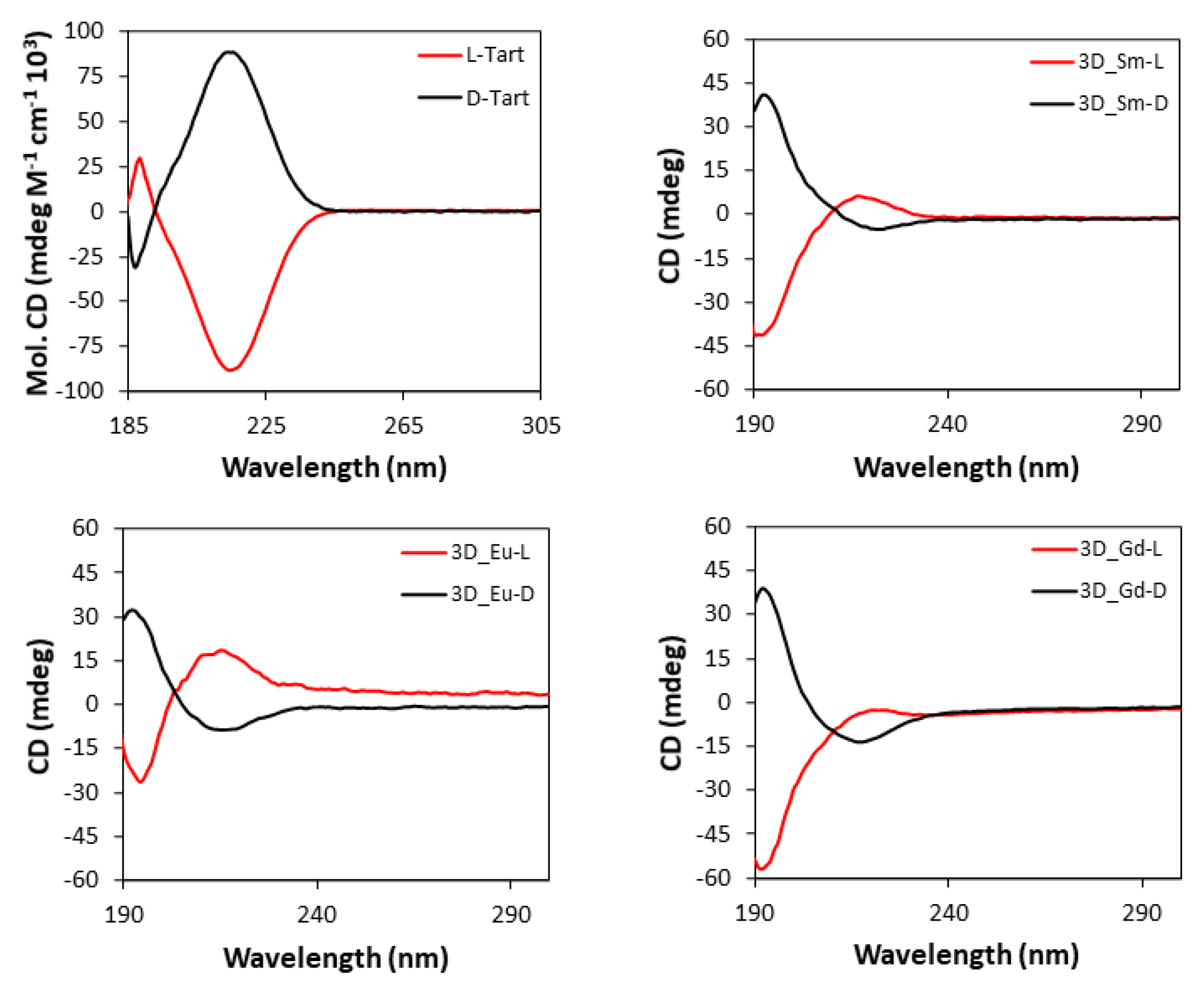
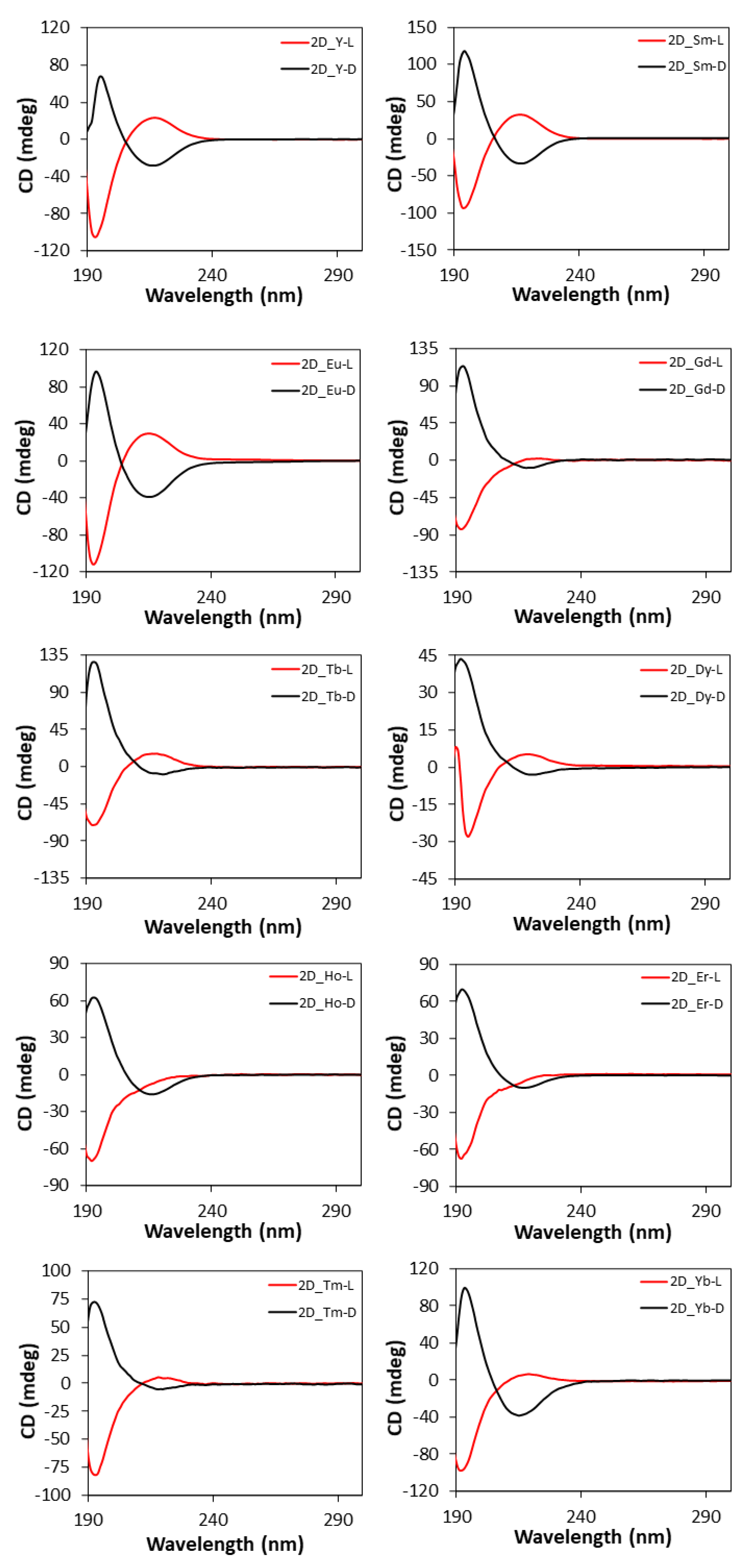
3.6. Circular Dichroism (CD) Experiments
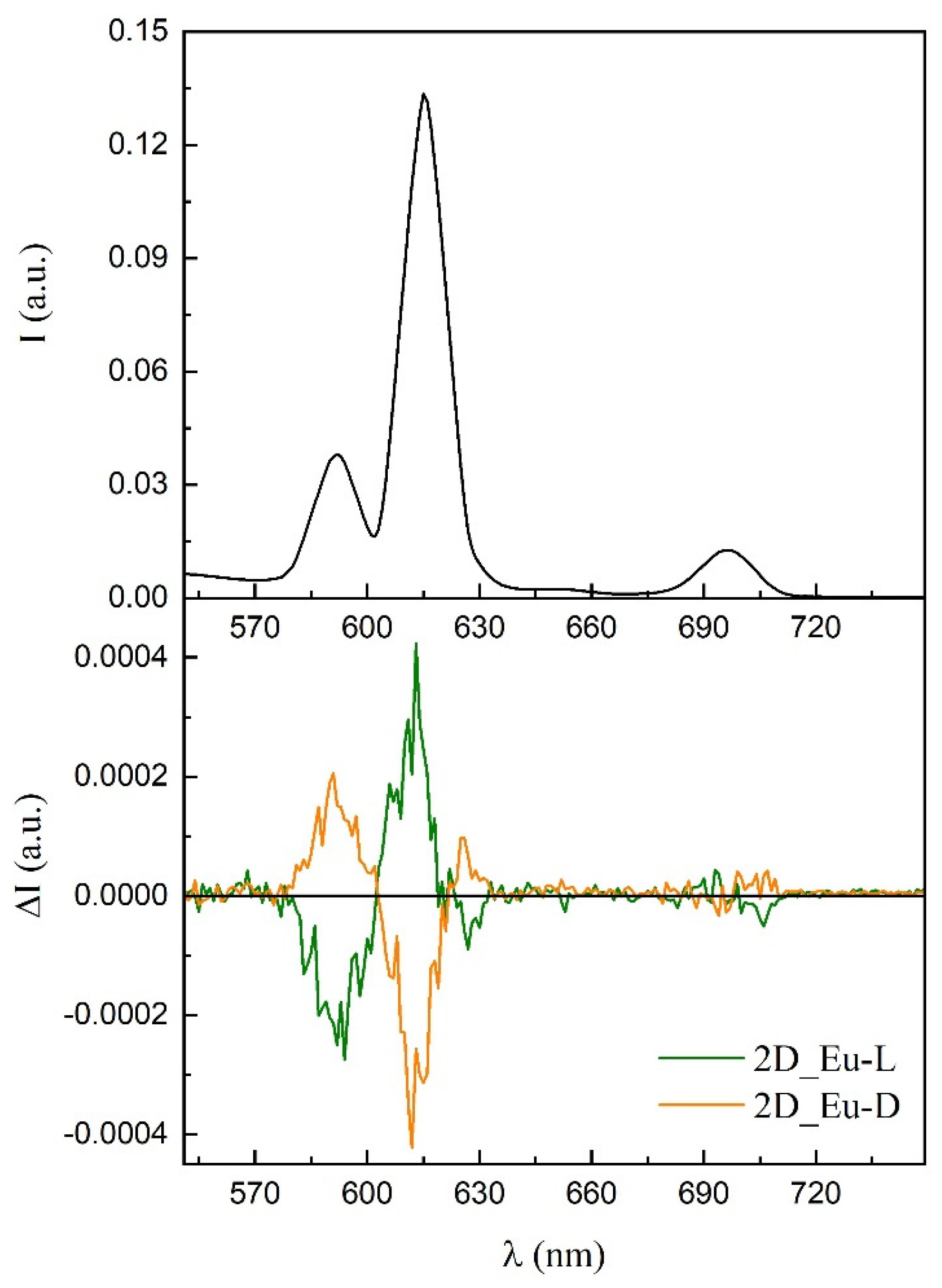
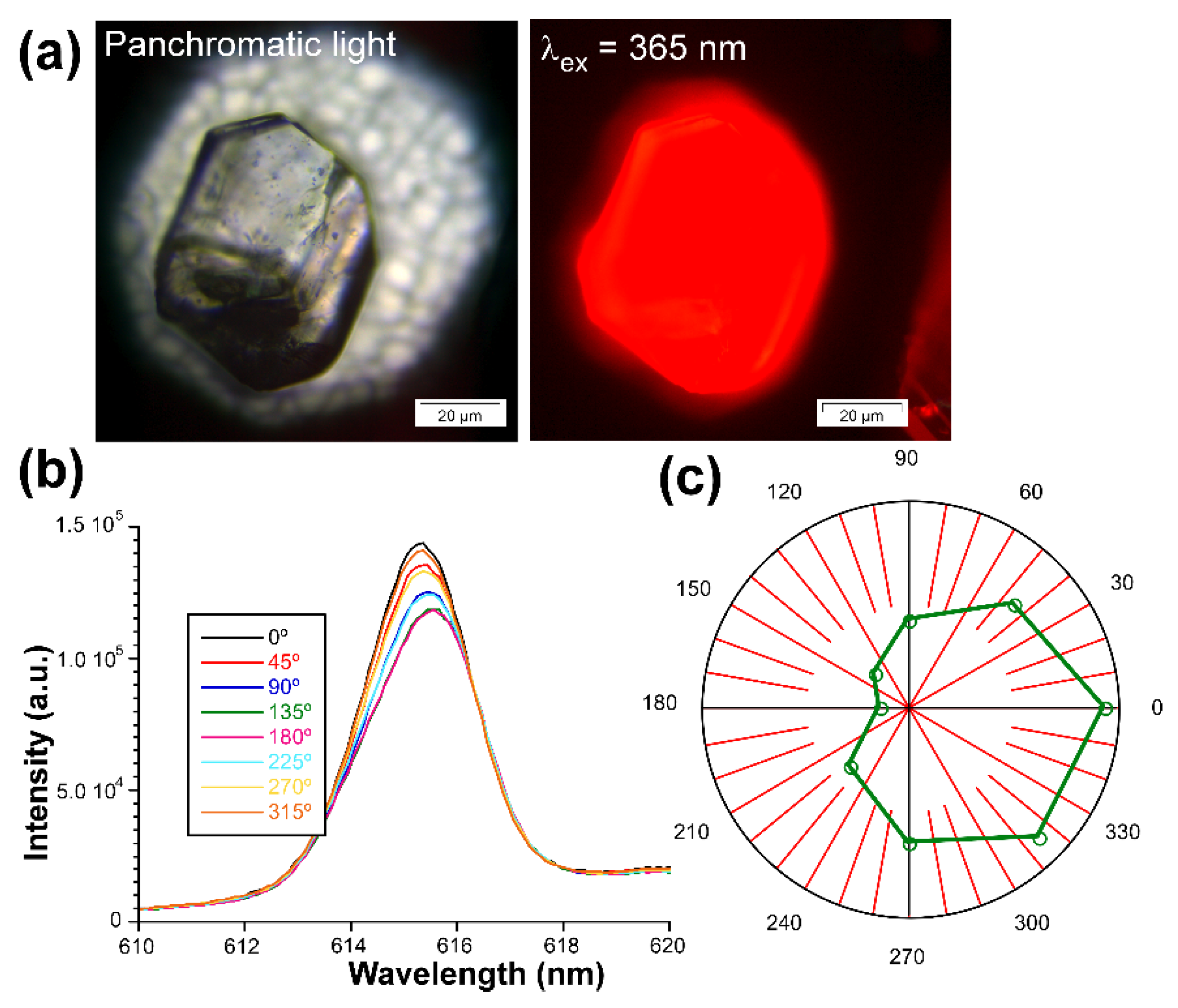
3.7. Polarized Luminescence Experiments
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guillerm, V.; Kim, D.; Eubank, J.F.; Luebke, R.; Liu, X.; Adil, K.; Lah, M.S.; Eddaoudi, M. A supermolecular building approach for the design and construction of metal-organic frameworks. Chem. Soc. Rev. 2014, 43, 6141–6172. [Google Scholar] [CrossRef]
- Eddaoudi, M.; Sava, D.F.; Eubank, J.F.; Adil, K.; Guillerm, V. Zeolite-like metal–organic frameworks (ZMOFs): Design{,} synthesis{,} and properties. Chem. Soc. Rev. 2015, 44, 228–249. [Google Scholar] [CrossRef]
- O’Keeffe, M.; Yaghi, O.M. Deconstructing the crystal structures of metal-organic frameworks and related materials into their underlying nets. Chem. Rev. 2012, 112, 675–702. [Google Scholar] [CrossRef] [PubMed]
- Batten, S.R.; Champness, N.R.; Chen, X.M.; Garcia-Martinez, J.; Kitagawa, S.; Öhrström, L.; O’Keeffe, M.; Suh, M.P.; Reedijk, J. Terminology of metal-organic frameworks and coordination polymers (IUPAC recommendations 2013). Pure Appl. Chem. 2013, 85, 1715–1724. [Google Scholar] [CrossRef]
- Allendorf, M.D.; Stavila, V. Crystal engineering, structure–function relationships, and the future of metal–organic frameworks. CrystEngComm 2014, 17, 229–246. [Google Scholar] [CrossRef]
- Zhang, Y.B.; Zhou, H.L.; Lin, R.B.; Zhang, C.; Lin, J.B.; Zhang, J.P.; Chen, X.M. Geometry analysis and systematic synthesis of highly porous isoreticular frameworks with a unique topology. Nat. Commun. 2012, 3, 642. [Google Scholar] [CrossRef]
- Cepeda, J.; Beobide, G.; Castillo, O.; Luque, A.; Pérez-Yáñez, S. Structural diversity of coordination compounds derived from double-chelating and planar diazinedicarboxylate ligands. Coord. Chem. Rev. 2017, 352, 83–107. [Google Scholar] [CrossRef]
- Gangu, K.K.; Maddila, S.; Mukkamala, S.B.; Jonnalagadda, S.B. A review on contemporary Metal–Organic Framework materials. Inorg. Chim. Acta 2016, 446, 61–74. [Google Scholar] [CrossRef]
- Farha, O.K.; Eryazici, I.; Jeong, N.C.; Hauser, B.G.; Wilmer, C.E.; Sarjeant, A.A.; Snurr, R.Q.; Nguyen, S.T.; Yazaydın, A.Ö.; Hupp, J.T. Metal–Organic Framework Materials with Ultrahigh Surface Areas: Is the Sky the Limit? J. Am. Chem. Soc. 2012, 134, 15016–15021. [Google Scholar] [CrossRef] [PubMed]
- Zhou, H.-C.J.; Kitagawa, S. Metal–Organic Frameworks (MOFs). Chem. Soc. Rev. 2014, 43, 5415–5418. [Google Scholar] [CrossRef] [PubMed]
- Tan, J.C.; Civalleri, B. Metal-organic frameworks and hybrid materials: From fundamentals to applications. CrystEngComm 2015, 17, 197–198. [Google Scholar] [CrossRef]
- Horcajada, P.; Gref, R.; Baati, T.; Allan, P.K.; Maurin, G.; Couvreur, P.; Férey, G.; Morris, R.E.; Serre, C. Metal-organic frameworks in biomedicine. Chem. Rev. 2012, 112, 1232–1268. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Chen, L.; Cui, H.; Zhang, J.; Zhang, L.; Su, C.Y. Applications of metal-organic frameworks in heterogeneous supramolecular catalysis. Chem. Soc. Rev. 2014, 43, 6011–6061. [Google Scholar] [CrossRef] [PubMed]
- Cepeda, J.; Pérez-Yáñez, S.; Beobide, G.; Castillo, O.; Goikolea, E.; Aguesse, F.; Garrido, L.; Luque, A.; Wright, P.A. Scandium/Alkaline Metal-Organic Frameworks: Adsorptive Properties and Ionic Conductivity. Chem. Mater. 2016, 28, 2519–2528. [Google Scholar] [CrossRef]
- Fujie, K.; Ikeda, R.; Otsubo, K.; Yamada, T.; Kitagawa, H. Lithium Ion Diffusion in a Metal-Organic Framework Mediated by an Ionic Liquid. Chem. Mater. 2015, 27, 7355–7361. [Google Scholar] [CrossRef]
- Inokuma, Y.; Yoshioka, S.; Ariyoshi, J.; Arai, T.; Hitora, Y.; Takada, K.; Matsunaga, S.; Rissanen, K.; Fujita, M. X-ray analysis on the nanogram to microgram scale using porous complexes. Nature 2013, 495, 461–466. [Google Scholar] [CrossRef]
- Wang, C.; Liu, D.; Lin, W. Metal-organic frameworks as a tunable platform for designing functional molecular materials. J. Am. Chem. Soc. 2013, 135, 13222–13234. [Google Scholar] [CrossRef]
- Zhao, D.; Timmons, D.J.; Yuan, D.; Zhou, H.C. Tuning the topology and functionality of metal-organic frameworks by ligand design. Acc. Chem. Res. 2011, 44, 123–133. [Google Scholar] [CrossRef]
- Yaghi, O.M.; Li, G.; Li, H. Selective binding and removal of guests in a microporous metal–organic framework. Nature 1995, 378, 703–706. [Google Scholar] [CrossRef]
- San Sebastian, E.; Rodríguez-Diéguez, A.; Seco, J.M.; Cepeda, J. Coordination Polymers with Intriguing Photoluminescence Behavior: The Promising Avenue for Greatest Long-Lasting Phosphors. Eur. J. Inorg. Chem. 2018, 2018, 2155–2174. [Google Scholar] [CrossRef]
- Lustig, W.P.; Mukherjee, S.; Rudd, N.D.; Desai, A.V.; Li, J.; Ghosh, S.K. Metal-organic frameworks: Functional luminescent and photonic materials for sensing applications. Chem. Soc. Rev. 2017, 46, 3242–3285. [Google Scholar] [CrossRef]
- Murawski, C.; Leo, K.; Gather, M.C. Efficiency roll-off in organic light-emitting diodes. Adv. Mater. 2013, 25, 6801–6827. [Google Scholar] [CrossRef]
- Gutiérrez, M.; Martin, C.; Kennes, K.; Hofkens, J.; Van der Auweraer, M.; Sánchez, F.; Douhal, A. OLEDs Based on Metal-Organic Framework: New OLEDs Based on Zirconium Metal-Organic Framework (Advanced Optical Materials 6/2018). Adv. Opt. Mater. 2018, 6, 1870022. [Google Scholar] [CrossRef]
- Yi, F.Y.; Chen, D.; Wu, M.K.; Han, L.; Jiang, H.L. Chemical Sensors Based on Metal–Organic Frameworks. Chempluschem 2016, 81, 675–690. [Google Scholar] [CrossRef] [PubMed]
- Hu, Z.; Deibert, B.J.; Li, J. Luminescent metal–organic frameworks for chemical sensing and explosive detection. Chem. Soc. Rev. 2014, 43, 5815–5840. [Google Scholar] [CrossRef]
- Rocha, J.; Brites, C.D.S.; Carlos, L.D. Lanthanide Organic Framework Luminescent Thermometers. Chem. A Eur. J. 2016, 22, 14782–14795. [Google Scholar] [CrossRef] [PubMed]
- Leo, P.; Briones, D.; García, J.A.; Cepeda, J.; Orcajo, G.; Calleja, G.; Rodríguez-Diéguez, A.; Martínez, F. Strontium-Based MOFs Showing Dual Emission: Luminescence Thermometers and Toluene Sensors. Inorg. Chem. 2020, 59, 18432–18443. [Google Scholar] [CrossRef]
- Errulat, D.; Marin, R.; Gálico, D.A.; Harriman, K.L.M.; Pialat, A.; Gabidullin, B.; Iikawa, F.; Couto, O.D.D.; Moilanen, J.O.; Hemmer, E.; et al. A Luminescent Thermometer Exhibiting Slow Relaxation of the Magnetization: Toward Self-Monitored Building Blocks for Next-Generation Optomagnetic Devices. ACS Cent. Sci. 2019, 5, 1187–1198. [Google Scholar] [CrossRef]
- Greenwood, N.N.; Earnshaw, A. Chemistry of the Elements, 2nd ed.; Butterworth-Heinemann: Oxford, UK; Elsevier: Amsterdam, The Netherlands, 1997. [Google Scholar]
- Layfield, R.; Murugesu, M. Lanthanides and Actinides in Molecular Magnetism; Wiley: Hoboken, NJ, USA, 2015; ISBN 978-3-527-33526-8. [Google Scholar]
- Bünzli, J.-C.G.; Piguet, C. Taking advantage of luminescent lanthanide ions. Chem. Soc. Rev. 2005, 34, 1048–1077. [Google Scholar] [CrossRef]
- Parker, D. Excitement in f block: Structure, dynamics and function of nine-coordinate chiral lanthanide complexes in aqueous media. Chem. Soc. Rev. 2004, 33, 156–165. [Google Scholar] [CrossRef] [PubMed]
- Heine, J.; Müller-Buschbaum, K. Engineering metal-based luminescence in coordination polymers and metal-organic frameworks. Chem. Soc. Rev. 2013, 42, 9232–9242. [Google Scholar] [CrossRef] [PubMed]
- Yip, Y.W.; Wen, H.; Wong, W.T.; Tanner, P.A.; Wong, K.L. Increased antenna effect of the lanthanide complexes by control of a number of terdentate n-donor pyridine ligands. Inorg. Chem. 2012, 51, 7013–7015. [Google Scholar] [CrossRef] [PubMed]
- Barry, D.E.; Caffrey, D.F.; Gunnlaugsson, T. Lanthanide-directed synthesis of luminescent self-assembly supramolecular structures and mechanically bonded systems from acyclic coordinating organic ligands. Chem. Soc. Rev. 2016, 45, 3244–3274. [Google Scholar] [CrossRef] [PubMed]
- Jiang, F.L.; Gai, Y.L.; Xiong, K.C.; Chen, L.; Bu, Y.; Li, X.J.; Hong, M.C. Visible and NIR photoluminescence properties of a series of novel lanthanide-organic coordination polymers based on hydroxyquinoline-carboxylate ligands. Inorg. Chem. 2012, 51, 13128–13137. [Google Scholar] [CrossRef]
- Gu, Z.G.; Zhan, C.; Zhang, J.; Bu, X. Chiral chemistry of metal–camphorate frameworks. Chem. Soc. Rev. 2016, 45, 3122–3144. [Google Scholar] [CrossRef]
- Liu, W.; Tang, X. Chiral lanthanide metal-organic frameworks. In Lanthanide Metal-Organic Frameworks; Springer: Berlin/Heidelberg, Germany, 2014; pp. 29–74. [Google Scholar]
- Kesanli, B.; Lin, W. Chiral porous coordination networks: Rational design and applications in enantioselective processes. Coord. Chem. Rev. 2003, 246, 305–326. [Google Scholar] [CrossRef]
- Verbiest, T.; Van Elshocht, S.; Kauranen, M.; Hellemans, L.; Snauwaert, J.; Nuckolls, C.; Katz, T.J.; Persoons, A. Strong enhancement of nonlinear optical properties through supramolecular chirality. Science 1998, 282, 913–915. [Google Scholar] [CrossRef]
- Férey, G. Hybrid porous solids: Past, present, future. Chem. Soc. Rev. 2007, 37, 191–214. [Google Scholar] [CrossRef] [PubMed]
- Wagenknecht, C.; Li, C.M.; Reingruber, A.; Bao, X.H.; Goebel, A.; Chen, Y.A.; Zhang, Q.; Chen, K.; Pan, J.W. Experimental demonstration of a heralded entanglement source. Nat. Photonics 2010, 4, 549–552. [Google Scholar] [CrossRef]
- Sherson, J.F.; Krauter, H.; Olsson, R.K.; Julsgaard, B.; Hammerer, K.; Cirac, I.; Polzik, E.S. Quantum teleportation between light and matter. Nature 2006, 443, 557–560. [Google Scholar] [CrossRef]
- Wang, C.; Fei, H.; Qiu, Y.; Yang, Y.; Wei, Z.; Tian, Y.; Chen, Y.; Zhao, Y. Photoinduced birefringence and reversible optical storage in liquid-crystalline azobenzene side-chain polymers. Appl. Phys. Lett. 1998, 74, 19. [Google Scholar] [CrossRef]
- Oka, T.; Aoki, H. Photovoltaic Hall effect in graphene. Phys. Rev. B Condens. Matter Mater. Phys. 2009, 79, 081406. [Google Scholar] [CrossRef]
- Wang, Y.H.; Steinberg, H.; Jarillo-Herrero, P.; Gedik, N. Observation of floquet-bloch states on the surface of a topological insulator. Science 2013, 342, 453–457. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Da Costa, R.C.; Fuchter, M.J.; Campbell, A.J. Circularly polarized light detection by a chiral organic semiconductor transistor. Nat. Photonics 2013, 7, 634–638. [Google Scholar] [CrossRef]
- Pierangelo, A.; De Martino, A.; Benali, A.; Novikova, T.; Validire, P. Polarimetric Imaging for Cancer Diagnosis and Staging. Opt. Photonics News 2012, 23, 26–33. [Google Scholar] [CrossRef]
- Mori, T.; Grimme, S.; Inoue, Y. A combined experimental and theoretical study on the conformation of multiarmed chiral aryl ethers. J. Org. Chem. 2007, 72, 6998–7010. [Google Scholar] [CrossRef]
- Abbate, S.; Lebon, F.; Longhi, G.; Passarello, M.; Liveri, V.T. Triggering dissymmetry in achiral dye molecules by chiral solvents: Circular dichroism experiments and DFT calculations. Chirality 2011, 23, 910–915. [Google Scholar] [CrossRef]
- Wakabayashi, M.; Yokojima, S.; Fukaminato, T.; Shiino, K.I.; Irie, M.; Nakamura, S. Anisotropic dissymmetry factor, g: Theoretical investigation on single molecule chiroptical spectroscopy. J. Phys. Chem. A 2014, 118, 5046–5057. [Google Scholar] [CrossRef]
- Chen, S.M.; Chang, L.M.; Yang, X.K.; Luo, T.; Xu, H.; Gu, Z.G.; Zhang, J. Liquid-Phase Epitaxial Growth of Azapyrene-Based Chiral Metal-Organic Framework Thin Films for Circularly Polarized Luminescence. ACS Appl. Mater. Interfaces 2019, 11, 31421–31426. [Google Scholar] [CrossRef]
- Wang, Y.; Li, X.; Yang, L.; Sun, W.Y.; Zhu, C.; Cheng, Y. Amplification effect of circularly polarized luminescence induced from binaphthyl-based zinc(II) chiral coordination polymers. Mater. Chem. Front. 2018, 2, 554–558. [Google Scholar] [CrossRef]
- Carr, R.; Evans, N.H.; Parker, D. Lanthanide complexes as chiral probes exploiting circularly polarized luminescence. Chem. Soc. Rev. 2012, 41, 7673–7686. [Google Scholar] [CrossRef]
- Bozoklu, G.; Gateau, C.; Imbert, D.; Pécaut, J.; Robeyns, K.; Filinchuk, Y.; Memon, F.; Muller, G.; Mazzanti, M. Metal-controlled diastereoselective self-assembly and circularly polarized luminescence of a chiral heptanuclear europium wheel. J. Am. Chem. Soc. 2012, 134, 8372–8375. [Google Scholar] [CrossRef] [PubMed]
- Huizi-Rayo, U.; Zabala-Lekuona, A.; Terenzi, A.; Cruz, C.M.; Cuerva, J.M.; Rodríguez-Diéguez, A.; García, J.A.; Seco, J.M.; San Sebastian, E.; Cepeda, J. Influence of thermally induced structural transformations on the magnetic and luminescence properties of tartrate-based chiral lanthanide organic-frameworks. J. Mater. Chem. C 2020, 8, 8243–8256. [Google Scholar] [CrossRef]
- Yan, P.; Xing, J.; Li, G.; Sun, W.; Zhang, J.; Hou, G. Two- and three-dimensional coordination polymers of lanthanide tartrate: Synthesis, crystal structures and luminescence. J. Coord. Chem. 2009, 62, 2095–2107. [Google Scholar] [CrossRef]
- Sheldrick, G.M. Crystal structure refinement with SHELXL. Acta Crystallogr. Sect. C 2015, 71, 3–8. [Google Scholar] [CrossRef]
- Farrugia, L.J. WinGX and ORTEP for Windows: An update. J. Appl. Crystallogr. 2012, 45, 849–854. [Google Scholar] [CrossRef]
- James J., P. Stewart MOPAC2016. Available online: http://OpenMOPAC.net (accessed on 15 October 2022).
- Dutra, J.D.L.; Bispo, T.D.; Freire, R.O. LUMPAC lanthanide luminescence software: Efficient and user friendly. J. Comput. Chem. 2014, 35, 772–775. [Google Scholar] [CrossRef] [PubMed]
- Coronado, E.; Galán-Mascarós, J.R.; Gómez-García, C.J.; Martínez-Agudo, J.M. Molecule-based magnets formed by bimetallic three-dimensional oxalate networks and chiral tris(bipyridyl) complex cations. The series [ZII(bpy)3][ClO4][MII CrIII(ox)3] (ZII = Ru, Fe, Co, and Ni; MII = Mn, Fe, Co, Ni, Cu, and Zn; ox = oxalate dianion). Inorg. Chem. 2001, 40, 113–120. [Google Scholar] [CrossRef]
- Seetharaj, R.; Vandana, P.V.; Arya, P.; Mathew, S. Dependence of solvents, pH, molar ratio and temperature in tuning metal organic framework architecture. Arab. J. Chem. 2019, 12, 295–315. [Google Scholar] [CrossRef]
- Sun, Y.X.; Sun, W.Y. Influence of temperature on metal-organic frameworks. Chinese Chem. Lett. 2014, 25, 823–828. [Google Scholar] [CrossRef]
- Nagarkar, S.S.; Chaudhari, A.K.; Ghosh, S.K. Role of temperature on framework dimensionality: Supramolecular isomers of Zn 3(RCOO) 8 based metal organic frameworks. Cryst. Growth Des. 2012, 12, 572–576. [Google Scholar] [CrossRef]
- Cheetham, A.K.; Kieslich, G.; Yeung, H.H.M. Thermodynamic and Kinetic Effects in the Crystallization of Metal-Organic Frameworks. Acc. Chem. Res. 2018, 51, 659–667. [Google Scholar] [CrossRef]
- Stock, N.; Biswas, S. Synthesis of metal-organic frameworks (MOFs): Routes to various MOF topologies, morphologies, and composites. Chem. Rev. 2012, 112, 933–969. [Google Scholar] [CrossRef] [PubMed]
- Llunell, M.; Casanova, D.; Cirera, J.; Bofill, J.M.; Alemany, P.; Alvarez, S.; Pinsky, M.; Avnir, D. Program for the Stereochemical Analysis of Molecular Fragments by Means of Continuous Shape Measures and Associated Tools; University of Barcelona: Barcelona, Spain, 2005; pp. 1–35. [Google Scholar]
- Blatov, V.A.; Shevchenko, A.P.; Proserpio, D.M. Applied topological analysis of crystal structures with the program package topospro. Cryst. Growth Des. 2014, 14, 3576–3586. [Google Scholar] [CrossRef]
- Hawthorne, F.C.; Borys, I.; Ferguson, R.B.; IUCr. Structure of erbium ditartrate trihydrate, Er4+.2C4H4O62−.3H2O. Acta Crystallogr. Sect. C 1983, 39, 540–542. [Google Scholar] [CrossRef]
- Xu, W.; Chang, H.S.; Liu, W.; Zheng, Y.Q. Synthesis, crystal structure, and properties of a new lanthanide tartrate coordination polymer. Russ. J. Coord. Chem. 2014, 40, 251–256. [Google Scholar] [CrossRef]
- Wu, C.-D.; Zhan, X.-P.; Lu, C.-Z.; Zhuang, H.-H.; Huang, J.-S. Poly[triaqua(μ-hydrogen tartrato)(μ-tartrato)samarium(III)]. Acta Crystallogr. Sect. E Struct. Rep. Online 2002, 58, m228–m230. [Google Scholar] [CrossRef]
- Ahmad, B.Z.; Want, B. Structure, ferroelectric ordering, and semiempirical quantum calculations of lanthanide based metal-organic framework: [Nd(C4H5O6)(C4H4O6)][3H2O]. J. Appl. Phys. 2016, 119, 144104. [Google Scholar] [CrossRef]
- Almond, M.J.; Drew, M.G.B.; Morris, S.; Rice, D.A. A single crystal x-ray diffraction study of yttrium tartrate hydrate [Y(C4H4O6)(C6H5O6)·2.5H2O]. Polyhedron 1996, 15, 3377–3383. [Google Scholar] [CrossRef]
- Kobayashi, S.; Molander, G.; Anwander, R.; Dowdy, E.C.; Groger, H.; Hou, Z.; Kagan, H. Lanthanides: Chemistry and Use in Organic Synthesis; Springer Science and Business Media: Berlin/Heidelberg, Germany, 1999; Volume 2. [Google Scholar]
- Groom, C.R.; Bruno, I.J.; Lightfoot, M.P.; Ward, S.C. The Cambridge structural database. Acta Crystallogr. Sect. B Struct. Sci. Cryst. Eng. Mater. 2016, 72, 171–179. [Google Scholar] [CrossRef]
- Evans, R.C.; Douglas, P.; Winscom, C.J. Coordination complexes exhibiting room-temperature phosphorescence: Evaluation of their suitability as triplet emitters in organic light emitting diodes. Coord. Chem. Rev. 2006, 250, 2093–2126. [Google Scholar] [CrossRef]
- Liu, Q.; Wang, R.; Wang, S. Blue phosphorescent Zn(II) and orange phosphorescent Pt(II) complexes of 4,4′-diphenyl-6,6′-dimethyl-2,2′-bipyrimidine. Dalt. Trans. 2004, 35, 2073–2079. [Google Scholar] [CrossRef]
- Binnemans, K. Lanthanide-Based Luminescent Hybrid Materials. Chem. Rev. 2009, 109, 4283–4374. [Google Scholar] [CrossRef] [PubMed]
- Bünzli, J.-C.G. On the design of highly luminescent lanthanide complexes. Coord. Chem. Rev. 2015, 293–294, 19–47. [Google Scholar] [CrossRef]
- Yamamoto, H.; Shionoya, S.; Yen, W.M. (Eds.) Phosphor Handbook, 2nd. ed.; CRC Press: Boca Raton, FL, USA; Taylor & Francis Group: Abingdon, UK, 2007; ISBN 9780849335648. [Google Scholar]
- Lustig, W.P.; Wang, F.; Teat, S.J.; Hu, Z.; Gong, Q.; Li, J. Chromophore-Based Luminescent Metal-Organic Frameworks as Lighting Phosphors. Inorg. Chem. 2016, 55, 7250–7256. [Google Scholar] [CrossRef]
- Cepeda, J.; Beobide, G.; Castillo, O.; Luque, A.; Pérez-Yáñez, S.; Román, P. Structure-directing effect of organic cations in the assembly of anionic In(III)/diazinedicarboxylate architectures. Cryst. Growth Des. 2012, 12, 1501–1512. [Google Scholar] [CrossRef]
- Pajuelo-Corral, O.; García, J.A.; Castillo, O.; Luque, A.; Rodríguez-Diéguez, A.; Cepeda, J. Single-ion magnet and photoluminescence properties of lanthanide(Iii) coordination polymers based on pyrimidine-4,6-dicarboxylate. Magnetochemistry 2021, 7, 8. [Google Scholar] [CrossRef]
- Beeby, A.; Clarkson, I.M.; Dickins, R.S.; Faulkner, S.; Parker, D.; Royle, L.; De Sousa, A.S.; Williams, J.G.; Woods, M. Non-radiative deactivation of the excited states of europium, terbium and ytterbium complexes by proximate energy-matched OH, NH and CH oscillators: An improved luminescence method for establishing solution hydration states. J. Chem. Soc. Perkin Trans. 2 1999, 493–504. [Google Scholar] [CrossRef]
- Zaitoun, M.A.; Al-Tarawneh, S. Effect of varying lanthanide local coordination sphere on luminescence properties illustrated by selected inorganic and organic rare earth complexes synthesized in sol–gel host glasses. J. Lumin. 2011, 131, 1795–1801. [Google Scholar] [CrossRef]
- Omary, M.A.; Patterson, H.H. Luminescence, Theory. In Encyclopedia of Spectroscopy and Spectrometry, 3rd ed.; Lindon, J.C., Tranter, G.E., Koppenaal, D.W., Eds.; Academic Press: Oxford, UK, 2017; pp. 636–653. ISBN 978-0-12-803224-4. [Google Scholar]
- Leo, P.; Orcajo, G.; García, J.A.; Ortuño, A.M.; Cuerva, J.M.; Briones, D.; Calleja, G.; Rodríguez-Diéguez, A.; Sanz, R.; Cepeda, J.; et al. An enantiomeric pair of alkaline-earth metal based coordination polymers showing room temperature phosphorescence and circularly polarized luminescence. J. Mater. Chem. C 2021, 9, 5544–5553. [Google Scholar] [CrossRef]
- Borges, A.S.; Caliman, E.V.; Dutra, J.D.L.; Da Silva, J.G.; Araujo, M.H. Structure and luminescent investigation of new Ln(III)-TTA complexes containing N-methyl-ε-caprolactam as ligand. J. Lumin. 2016, 170, 654–662. [Google Scholar] [CrossRef]
- Malta, O.L.; Gonçalves e Silva, F.R. A theoretical approach to intramolecular energy transfer and emission quantum yields in coordination compounds of rare earth ions. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 1998, 54, 1593–1599. [Google Scholar] [CrossRef]
- Malta, O.L. Ligand—Rare-earth ion energy transfer in coordination compounds. A theoretical approach. J. Lumin. 1997, 71, 229–236. [Google Scholar] [CrossRef]
- de Sá, G.F.; Malta, O.L.; de Mello Donegá, C.; Simas, A.M.; Longo, R.L.; Santa-Cruz, P.A.; da Silva, E.F. Spectroscopic properties and design of highly luminescent lanthanide coordination complexes. Coord. Chem. Rev. 2000, 196, 165–195. [Google Scholar] [CrossRef]
- da Silva Galaço, A.R.B.; Freire, R.O.; Jesus, L.T.; Serra, O.A. Experimental and theoretical study of isoreticular lanthanoid organic framework (LOF): Structure and luminescence. J. Lumin. 2020, 223, 117179. [Google Scholar] [CrossRef]
- Neese, F. The ORCA program system. WIREs Comput. Mol. Sci. 2012, 2, 73–78. [Google Scholar] [CrossRef]
- Neese, F.; Wennmohs, F.; Becker, U.; Riplinger, C. The ORCA quantum chemistry program package. J. Chem. Phys. 2020, 152, 224108. [Google Scholar] [CrossRef] [PubMed]
- Zenkevich, E.I.; Knyukshto, V.N.; Shulga, A.M.; Kuzmitsky, V.A.; Gael, V.I.; Levinson, E.G.; Mironov, A.F. Spectroscopic and photophysical properties of covalent ether-bonded porphyrin-chlorin heterodimers. J. Lumin. 1997, 75, 229–244. [Google Scholar] [CrossRef]
- Dobretsov, G.E.; Syrejschikova, T.I.; Smolina, N.V. On mechanisms of fluorescence quenching by water. Biophysics 2014, 59, 183–188. [Google Scholar] [CrossRef]
- Zhang, G.; Hu, H.; Li, H.; Zhao, F.; Liu, Y.; He, X.; Huang, H.; Xu, Y.; Wei, Y.; Kang, Z. Homochiral metal–organic porous materials for enantioselective recognition and electrocatalysis. CrystEngComm 2013, 15, 3288–3291. [Google Scholar] [CrossRef]
- Castriciano, M.A.; Romeo, A.; Zagami, R.; Micali, N.; Scolaro, L.M. Kinetic effects of tartaric acid on the growth of chiral J-aggregates of tetrakis(4-sulfonatophenyl)porphyrin. Chem. Commun. 2012, 48, 4872–4874. [Google Scholar] [CrossRef] [PubMed]
- Saha, R.; Biswas, S.; Mostafa, G. pH-Triggered construction of NLO active CMOFs: Change in supramolecular assembly, water clusters, helical architectures and their properties. CrystEngComm 2011, 13, 1018–1028. [Google Scholar] [CrossRef]
- Deng, L.; Zhou, Z.H. Chiral Supramolecular Microporous Thio-Oxomolybdenum(V) Tartrates for the Selective Adsorptions of Gases. Inorg. Chem. 2022, 61, 14787–14799. [Google Scholar] [CrossRef] [PubMed]
- Caputo, M.C.; Pelloni, S.; Lazzeretti, P. Theoretical prediction of the optical rotation of chiral molecules in ordered media: A computational study of (Ra)-1,3-dimethylallene, (2R)-2-methyloxirane, and (2R)-N-methyloxaziridine. Int. J. Quantum Chem. 2015, 115, 900–906. [Google Scholar] [CrossRef]
- Yang, X.; Lin, X.; Zhao, Y.; Zhao, Y.S.; Yan, D. Lanthanide Metal–Organic Framework Microrods: Colored Optical Waveguides and Chiral Polarized Emission. Angew. Chemie Int. Ed. 2017, 56, 7853–7857. [Google Scholar] [CrossRef] [PubMed]
- CrysAlisPro Software System; Agilent Technologies UK Ltd.: Oxford, UK, 2019.
- Bruker Apex2; Bruker AXS Inc.: Madison, WI, USA, 2004.
- Sheldrick, G.M. SADABS, Program for Empirical Adsorption Correction; Institute for Inorganic Chemistry, University of Göttingen: Göttingen, Germany, 1996. [Google Scholar]
- Altomare, A.; Burla, M.C.; Camalli, M.; Cascarano, G.L.; Giacovazzo, C.; Guagliardi, A.; Moliterni, A.G.; Polidori, G.; Spagna, R. SIR97: A new tool for crystal structure determination and refinement. J. Appl. Crystallogr. 1999, 32, 115–119. [Google Scholar] [CrossRef]
- Farrugia, L.J. WinGX suite for small-molecule single-crystal crystallography. J. Appl. Cryst. 1999, 32, 837–838. [Google Scholar] [CrossRef]
- Rodriguez-Carvajal, J. Recent advances in magnetic structure determination by neutron powder diffraction. Phys. B. Condens. Matter 1993, 192, 55–69. [Google Scholar] [CrossRef]





| Parameters | 3D_Sm-L | 3D_Sm-D | 3D_Eu-L | 3D_Eu-D | 3D_Gd-L | 3D_Gd-D |
|---|---|---|---|---|---|---|
| Crystal System | Triclinic | Triclinic | Triclinic | Triclinic | Triclinic | Triclinic |
| Space group | P1 | P1 | P1 | P1 | P1 | P1 |
| a (Å) | 6.03715 | 6.03242 | 6.09457 | 6.02532 | 6.01264 | 6.01300 |
| b (Å) | 7.48102 | 7.47384 | 7.46433 | 7.45733 | 7.44523 | 7.44541 |
| C (Å) | 13.27383 | 13.25745 | 13.28264 | 13.25675 | 13.23188 | 13.23119 |
| α (°) | 102.69998 | 102.65131 | 102.86695 | 102.73313 | 102.75539 | 102.78816 |
| β (°) | 101.36651 | 101.38302 | 101.99059 | 101.43393 | 101.42113 | 101.42812 |
| γ (°) | 90.77908 | 90.84119 | 89.85748 | 90.81119 | 90.84535 | 90.84964 |
| V (Å3) | 572.345 | 570.685 | 575.620 | 568.439 | 565.214 | 565.136 |
| D-H⋯A 2 | D-H | H⋯A | D⋯A | D–H⋯A |
|---|---|---|---|---|
| O1W-H11W⋯O12A | 0.87 | 1.87 | 2.694 (4) | 156.7 |
| O1W-H12W⋯O31A (i) | 0.86 | 1.91 | 2.751 | 166.1 |
| O2-H2⋯O31A (ii) | 0.88 | 2.08 | 2.913 (6) | 158.5 |
| D-H⋯A 2 | D-H | H⋯A | D⋯A | D–H⋯A |
|---|---|---|---|---|
| O21A-H21A⋯O42A (i) | 0.85 | 1.81 | 2.652(4) | 175.1 |
| O31A-H31A⋯O41A (ii) | 0.90 | 2.01 | 2.828(5) | 149.9 |
| O41A-H41A⋯O1W (iii) | 0.85 | 1.80 | 2.627(5) | 163.5 |
| Compound | System | Rate Constants | τ (µs) | Φ (%) | |
|---|---|---|---|---|---|
| kr (s−1) | knr (s−1) | ||||
| 2D_Eu-L | Experimental | 140 | 2784 | 342.0 | 4.8 |
| Calculated | 150 | 2774 | – | 5.1 | |
| 3D_Eu-L | Experimental | 785 | 1660 | 409.1 | 32.1 |
| Calculated | 828 | 1617 | – | 33.9 | |
| Compound/Atoms | Spherical Coordinates | g | α (Å3) | ||
|---|---|---|---|---|---|
| R (Å) | Θ (°) | Φ (°) | |||
| 2D_Eu-L | |||||
| O12A (i) | 2.3972 | 92.64 | 234.63 | 0.6097 | 2.4255 |
| O12A (ii) | 2.3911 | 44.67 | 160.49 | 0.6122 | 2.4149 |
| O11A | 2.4089 | 116.68 | 121.66 | 0.5934 | 2.4341 |
| O11A (iii) | 2.3386 | 53.78 | 344.68 | 0.6083 | 2.4241 |
| O1w (iii) | 2.4901 | 65.92 | 75.39 | 0.6149 | 2.4089 |
| O1w | 2.5427 | 113.97 | 306.89 | 0.5977 | 2.4304 |
| O21A (i) | 2.6929 | 149.85 | 220.23 | 0.6024 | 2.4220 |
| O21A (ii) | 2.6926 | 41.70 | 255.66 | 0.6031 | 2.4171 |
| O2 | 2.2698 | 133.80 | 21.33 | 0.3425 | 2.4327 |
| 3D_Eu-L | |||||
| O11A | 2.5109 | 50.05 | 14.63 | 0.5857 | 2.4355 |
| O12A | 2.4973 | 5.55 | 121.17 | 0.7211 | 2.4229 |
| O12B | 2.3260 | 82.08 | 278.45 | 0.5334 | 2.4581 |
| O11C | 2.5598 | 91.54 | 70.52 | 0.5849 | 2.4321 |
| O21C | 2.3645 | 123.36 | 15.53 | 0.6825 | 2.4199 |
| O1w | 2.3876 | 82.55 | 135.81 | 0.4388 | 2.4504 |
| O31B (i) | 2.4962 | 147.78 | 131.28 | 0.6025 | 2.4014 |
| O41B (i) | 2.5185 | 145.03 | 254.77 | 0.3587 | 2.4264 |
| O42B (ii) | 2.4264 | 75.41 | 204.54 | 0.6267 | 2.4189 |
| Compound 2D_Eu-L | Compound 3D_Eu-L | ||||||
|---|---|---|---|---|---|---|---|
| Transition | WET (s−1) | Transition | WBET (s−1) | Transition | WET (s−1) | Transition | WBET (s−1) |
| S0 ← S1 | 10−6 | S0 → S1 | - | S0 ← S1 | 10−6 | S0 → S1 | - |
| T ← S1 | 10−5 | T → S1 | - | T ← S1 | 10−5 | T → S1 | - |
| S0 ← T | 10−5 | S0 → T | - | S0 ← T | 10−5 | S0 → T | - |
| 5D4 ← T | 6.3 × 103 | 5D4 → T | 3.3 × 10−15 | 5D4 ← T | 3.5 × 103 | 5D4 → T | 4.5 × 10−16 |
| 5D4 ← S1 | 1.3 × 101 | 5D4 → S1 | 5.1 × 10−24 | 5D4 ← S1 | 4.4 × 102 | 5D4 → S1 | 7.6 × 10−22 |
| 5D1 ← T | 1.3 | 5D1 → T | 3.4 × 10−35 | 5D1 ← T | 3.1 | 5D1 → T | 6.0 × 10−37 |
| 5D0 ← T | 4.3 × 101 | 5D0 → T | 2.6 × 10−50 | 5D0 ← T | 8.9 × 102 | 5D0 → T | 4.2 × 10−42 |
| 5D1 ← 5D4 | 10−6 | 5D1 → 5D4 | - | 5D1 ← 5D4 | 10−6 | 5D1 → 5D4 | - |
| 5D0 ← 5D1 | 10−6 | 5D0 → 5D1 | - | 5D0 ← 5D1 | 10−6 | 5D0 → 5D1 | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huizi-Rayo, U.; Gastearena, X.; Ortuño, A.M.; Cuerva, J.M.; Rodríguez-Diéguez, A.; García, J.A.; Ugalde, J.; Seco, J.M.; Sebastian, E.S.; Cepeda, J. Influence of Tartrate Ligand Coordination over Luminescence Properties of Chiral Lanthanide-Based Metal–Organic Frameworks. Nanomaterials 2022, 12, 3999. https://doi.org/10.3390/nano12223999
Huizi-Rayo U, Gastearena X, Ortuño AM, Cuerva JM, Rodríguez-Diéguez A, García JA, Ugalde J, Seco JM, Sebastian ES, Cepeda J. Influence of Tartrate Ligand Coordination over Luminescence Properties of Chiral Lanthanide-Based Metal–Organic Frameworks. Nanomaterials. 2022; 12(22):3999. https://doi.org/10.3390/nano12223999
Chicago/Turabian StyleHuizi-Rayo, Uxua, Xuban Gastearena, Ana M. Ortuño, Juan M. Cuerva, Antonio Rodríguez-Diéguez, Jose Angel García, Jesus Ugalde, Jose Manuel Seco, Eider San Sebastian, and Javier Cepeda. 2022. "Influence of Tartrate Ligand Coordination over Luminescence Properties of Chiral Lanthanide-Based Metal–Organic Frameworks" Nanomaterials 12, no. 22: 3999. https://doi.org/10.3390/nano12223999
APA StyleHuizi-Rayo, U., Gastearena, X., Ortuño, A. M., Cuerva, J. M., Rodríguez-Diéguez, A., García, J. A., Ugalde, J., Seco, J. M., Sebastian, E. S., & Cepeda, J. (2022). Influence of Tartrate Ligand Coordination over Luminescence Properties of Chiral Lanthanide-Based Metal–Organic Frameworks. Nanomaterials, 12(22), 3999. https://doi.org/10.3390/nano12223999










