Topological Insulator Films for Terahertz Photonics
Abstract
1. Introduction
2. Theory
2.1. Kinetic Theory of THz Third Harmonic Generation
2.2. Generation of the THz Radiation in Photoconductive Antennas
3. Materials and Methods
3.1. Growth and Characterization
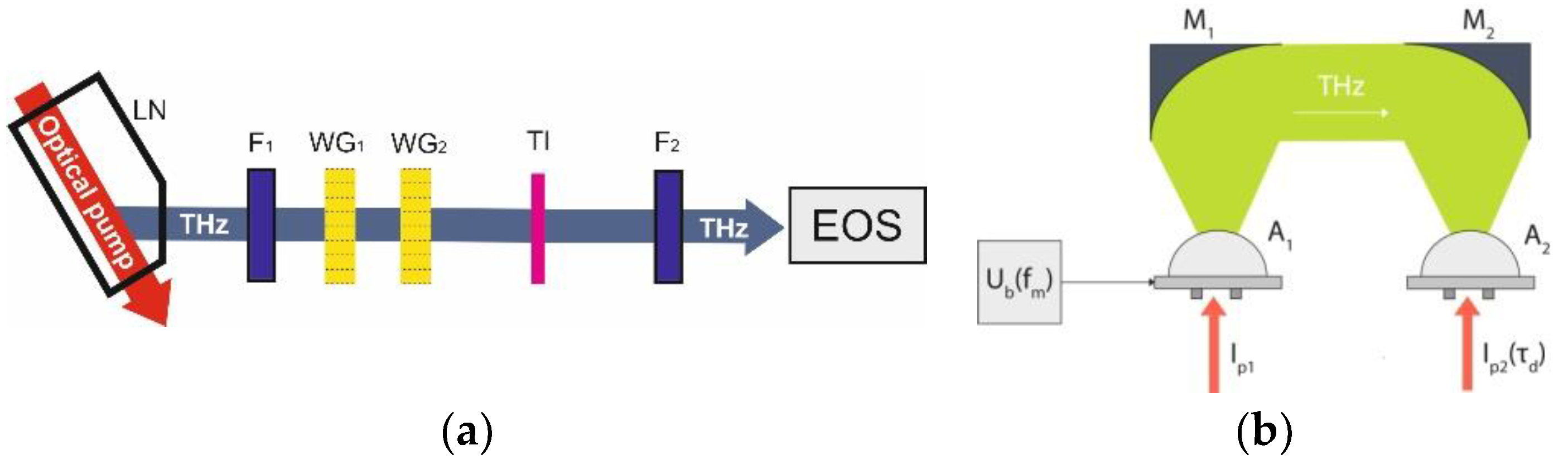
3.2. Experimental Techniques
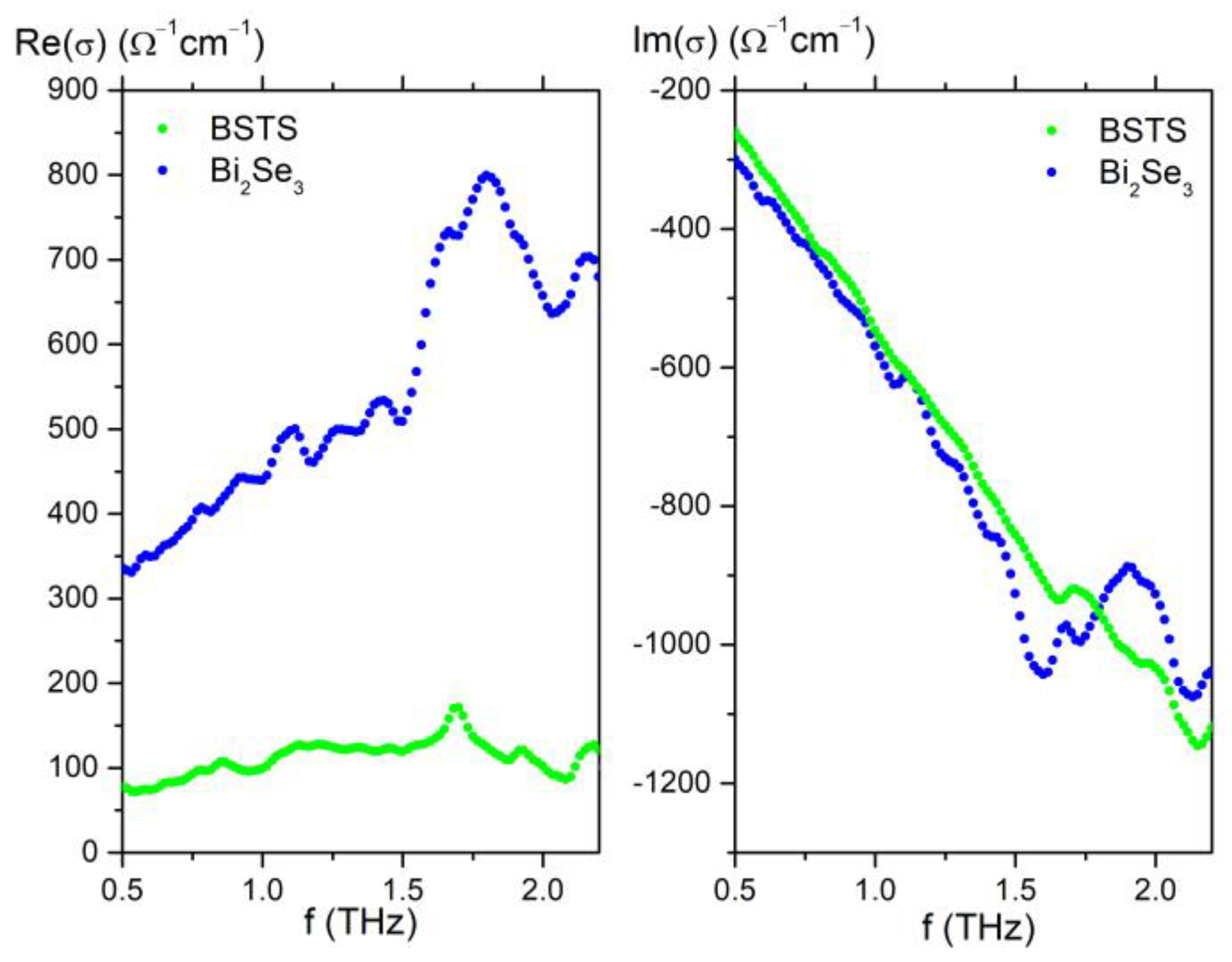
4. Results and Discussion
4.1. Generation of the Third THz Harmonic
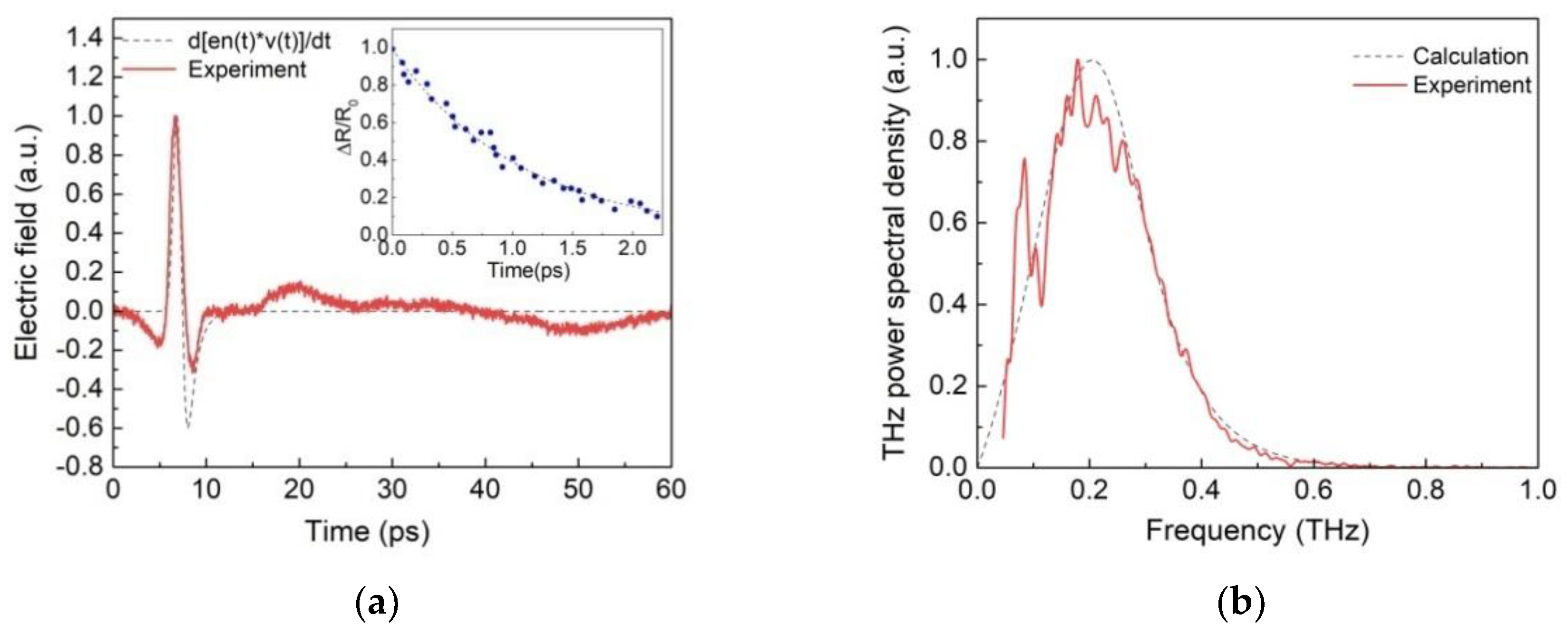
4.2. Photoconductive THz Antenna
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Volkov, B.A.; Pankratov, O.A. Two-dimensional massless electrons in an inverted contact. JETP 1985, 42, 178–181. [Google Scholar]
- Hasan, M.Z.; Kane, C.L. Colloquium. Topological insulators. Rev. Mod. Phys. 2010, 82, 3045. [Google Scholar] [CrossRef]
- Ando, Y. Topological insulator materials. J. Phys. Soc. Jpn. 2013, 82, 102001. [Google Scholar] [CrossRef]
- Dziom, V.; Shuvaev, A.; Pimenov, A.; Astakhov, G.V.; Ames, C.; Bendias, K.; Böttcher, J.; Tkachov, G.; Hankiewicz, E.M.; Brüne, C.; et al. Observation of the universal magnetoelectric effect in a 3D topological insulator. Nat. Commun. 2017, 8, 15197. [Google Scholar] [CrossRef]
- Kozlov, D.A.; Kvon, Z.D.; Olshanetsky, E.B.; Mikhailov, N.N.; Dvoretsky, S.A.; Weiss, D. Transport Properties of a 3D Topological Insulator based on a Strained High-Mobility HgTe FilmD. Phys. Rev. Lett. 2014, 112, 196801. [Google Scholar] [CrossRef]
- He, M.; Sun, H.; He, Q.L. Topological insulator: Spintronics and quantum computations. Front. Phys. 2019, 14, 43401. [Google Scholar] [CrossRef]
- Fan, Y.; Wang, K.L. Spintronics Based on Topological Insulators. SPIN 2016, 6, 1640001. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, J.; Zhang, S.-C. Topological insulators for high-performance terahertz to infrared applications. Phys. Rev. B 2010, 82, 245107. [Google Scholar] [CrossRef]
- Egorova, S.G.; Ryabova, L.I.; Skipetrov, E.P.; Yashina, L.V.; Danilov, S.N.; Ganichev, S.D.; Khokhlov, D.R. Detection of highly conductive surface electron states in topological crystalline insulators Pb1−xSnxSe using laser terahertz radiation. Sci. Rep. 2015, 5, 11540. [Google Scholar] [CrossRef] [PubMed]
- Durnev, M.V.; Tarasenko, S.A. High-Frequency Nonlinear Transport and Photogalvanic Effects in 2D Topological Insulators. Ann. Phys. 2019, 531, 1800418. [Google Scholar] [CrossRef]
- McIver, J.W.; Hsieh, D.; Steinberg, H.; Jarillo-Herrero, P.; Gedik, N. Control over topological insulator photocurrents with light polarization. Nat. Nanotechnol. 2012, 7, 96–100. [Google Scholar] [CrossRef] [PubMed]
- Olbrich, P.; Golub, L.E.; Herrmann, T.; Danilov, S.N.; Plank, H.; Bel’kov, V.V.; Mussler, G.; Weyrich, C.; Schneider, C.M.; Kampmeier, J.; et al. Room-temperature high-frequency transport of Dirac fermions in epitaxially grown Sb2Te3− and Bi2Te3− based topological insulators. Phys. Rev. Lett. 2014, 113, 096601. [Google Scholar] [CrossRef]
- Dantscher, K.M.; Kozlov, D.A.; Olbrich, P.; Zoth, C.; Faltermeier, P.; Lindner, M.; Budkin, G.V.; Tarasenko, S.A.; Bel’kov, V.V.; Kvon, Z.D.; et al. Cyclotron-resonance-assisted photocurrents in surface states of a three-dimensional topological insulator based on a strained high-mobility HgTe film. Phys. Rev. 2015, 92, 165314. [Google Scholar] [CrossRef]
- Dantscher, K.M.; Kozlov, D.A.; Scherr, M.T.; Gebert, S.; Bärenfänger, J.; Durnev, M.V.; Tarasenko, S.A.; Bel’kov, V.V.; Mikhailov, N.N.; Dvoretsky, S.A.; et al. Photogalvanic probing of helical edge channels in two-dimensional HgTe topological insulators. Phys. Rev. 2017, 95, 201103. [Google Scholar] [CrossRef]
- Giorgianni, F.; Chiadroni, E.; Rovere, A.; Cestelli-Guidi, M.; Perucchi, A.; Bellaveglia, M.; Castellano, M.; di Giovenale, D.; di Pirro, G.; Ferrario, M.; et al. Strong nonlinear terahertz response induced by Dirac surface states in Bi2Se3 topological insulator. Nat. Commun. 2016, 7, 11421. [Google Scholar] [CrossRef]
- Kovalev, S.; Tielrooij, K.J.; Deinert, J.C.; Ilyakov, I.; Awari, N.; Chen, M.; Ponomaryov, A.; Bawatna, M.; de Oliveira, T.V.A.G.; Eng, L.M.; et al. Terahertz signatures of ultrafast Dirac fermion relaxation at the surface of topological insulators. Npj Quantum Mater. 2021, 6, 84. [Google Scholar] [CrossRef]
- Burford, N.M.; El-Shenawee, M.O. Review of terahertz photoconductive antenna technology. Opt. Eng. 2017, 56, 10901. [Google Scholar] [CrossRef]
- Ponomarev, D.S.; Lavrukhin, D.V.; Zenchenko, N.V.; Frolov, T.V.; Glinskiy, I.A.; Khabibullin, R.A.; Katyba, G.M.; Kurlov, V.N.; Otsuji, T.; Zaytsev, K.I. Boosting THz photoconductive antenna-emitter using optical light confinement behind a high refractive sapphire fiber-lens. Opt. Lett. 2022, 47, 1899–1902. [Google Scholar] [CrossRef]
- Hamh, S.Y.; Park, S.-H.; Jerng, S.-K.; Jeon, J.H.; Chun, S.-H.; Lee, J.S. Helicity-dependent photocurrent in a Bi2Se3 thin film probed by terahertz emission spectroscopy. Phys. Rev. B 2016, 94, 161405. [Google Scholar] [CrossRef]
- Fang, Z.; Wang, H.; Wu, X.; Shan, S.; Wang, C.; Zhao, H.; Xia, C.; Nie, T.; Miao, J.; Zhang, C.; et al. Nonlinear terahertz emission in the three-dimensional topological insulator Bi2Te3 by terahertz emission spectroscopy. Appl. Phys. Lett. 2019, 115, 191102. [Google Scholar] [CrossRef]
- Zhu, L.-G.; Kubera, B.; Mak, K.F.; Shan, J. Effect of Surface States on Terahertz Emission from the Bi2Se3 Surface. Sci. Rep. 2015, 5, 10308. [Google Scholar] [CrossRef]
- Tu, C.-M.; Chen, Y.-C.; Huang, P.; Chuang, P.-Y.; Lin, M.-Y.; Cheng, C.-M.; Lin, J.-Y.; Juang, J.-Y.; Wu, K.-H.; Huang, J.-C.A.; et al. Helicity-dependent terahertz emission spectroscopy of topological insulator Sb2Te3 thin films. Phys. Rev. B 2017, 96, 195407. [Google Scholar] [CrossRef]
- Onishi, Y.; Ren, Z.; Novak, M.; Segawa, K.; Ando, Y.; Tanaka, K. Instantaneous Photon Drag Currents in Topological Insulators. arXiv 2014, arXiv:1403.2492. Available online: https://arxiv.org/abs/1403.2492 (accessed on 1 September 2022).
- Luo, C.W.; Chen, H.J.; Tu, C.M.; Lee, C.C.; Ku, S.A.; Tzeng, W.Y.; Yeh, T.T.; Chiang, M.C.; Wang, H.J.; Chu, W.C.; et al. THz Generation and Detection on Dirac Fermions in Topological Insulators. Adv. Opt. Mater. 2013, 1, 804–808. [Google Scholar] [CrossRef]
- Kuznetsov, K.A.; Safronenkov, D.A.; Kuznetsov, P.I.; Kitaeva, G.K. Terahertz Photoconductive Antenna Based on a Topological Insulator Nanofilm. Appl. Sci. 2021, 11, 5580. [Google Scholar] [CrossRef]
- Mikhailov, S.A. Non-linear electromagnetic response of graphene. EPL 2007, 79, 27002. [Google Scholar] [CrossRef]
- Mikhailov, S.A.; Ziegler, K. Nonlinear electromagnetic response of graphene: Frequency multiplication and the self-consistent-field effects. J. Phys. Condens. Matter 2008, 20, 384204. [Google Scholar] [CrossRef]
- Karch, J.; Drexler, C.; Olbrich, P.; Fehrenbacher, M.; Hirmer, M.; Glazov, M.M.; Tarasenko, S.A.; Ivchenko, E.L.; Birkner, B.; Eroms, J.; et al. Terahertz Radiation Driven Chiral Edge Currents in Graphene. Phys. Rev. Lett. 2011, 107, 276601. [Google Scholar] [CrossRef]
- Glazov, M.M.; Ganichev, S.D. High frequency electric field induced nonlinear effects in graphene. Phys. Rep. 2014, 535, 101–138. [Google Scholar] [CrossRef]
- Durnev, M.V.; Tarasenko, S.A. Second harmonic generation at the edge of a two-dimensional electron gas. Condens. Matter arXiv 2022, arXiv:2204.04069. [Google Scholar] [CrossRef]
- Costache, M.V.; Neumann, I.; Sierra, J.F.; Marinova, V.; Gospodinov, M.M.; Roche, S.; Valenzuela, S.O. Fingerprints of Inelastic Transport at the Surface of the Topological Insulator Bi2Se3: Role of Electron-Phonon Coupling. Phys. Rev. Lett. 2014, 112, 086601. [Google Scholar] [CrossRef]
- Weng, M.Q.; Wu, M.W. High-field charge transport on the surface of Bi2Se3. Phys. Rev. 2014, 90, 125306. [Google Scholar] [CrossRef]
- Heid, R.; Sklyadneva, I.Y.; Chulkov, E.V. Electron-phonon coupling in topological surface states: The role of polar optical modes. Sci. Rep. 2017, 7, 1095. [Google Scholar] [CrossRef] [PubMed]
- Principi, A.; Tielrooij, K.-J. Ultrafast electronic heat dissipation through surface-to-bulk Coulomb coupling in quantum materials. Condens. Matter arXiv 2022, arXiv:2206.09119. [Google Scholar] [CrossRef]
- Jepsen, P.U.; Jacobsen, R.H.; Keiding, S.R. Generation and detection of terahertz pulses from biased semiconductor antennas. J. Opt. Soc. Am. 1996, 13, 2424–2436. [Google Scholar] [CrossRef]
- Lavrukhin, D.V.; Yachmenev, A.E.; Pavlov, A.Y.; Khabibullin, R.A.; Goncharov, Y.G.; Spektor, I.E.; Komandin, G.A.; Yurchenko, S.O.; Chernomyrdin, N.V.; Zaytsev, K.I.; et al. Shaping the spectrum of terahertz photoconductive antenna by frequency-dependent impedance modulation. Semicond. Sci. Technol. 2019, 34, 34005. [Google Scholar] [CrossRef]
- Lepeshov, S.; Gorodetsky, A.; Krasnok, A.; Rafailov, E.; Belov, P. Enhancement of terahertz photoconductive antenna operation by optical nanoantennas. Laser Photonics Rev. 2017, 11, 1770001. [Google Scholar] [CrossRef]
- Tani, M.; Matsuura, S.; Sakai, K.; Nakashima, S.-I. Emission characteristics of photoconductive antennas based on low-temperature-grown GaAs and semi-insulating GaAs. App. Opt. 1997, 36, 7853–7859. [Google Scholar] [CrossRef]
- Kuznetsov, P.I.; Luzanov, V.A.; Yakusheva, G.G.; Temiryazev, A.G.; Shchamkhalova, B.S.; Zhitov, V.A.; Zakharov, L.Y. Deposition of heteroepitaxial layers of topological insulator Bi2Se3 in the trimethylbismuth–isopropylselenide–hydrogen system on the (0001) Al2O3 and (100) GaAs substrates. J. Commun. Technol. Electron. 2016, 61, 183–189. [Google Scholar] [CrossRef]
- Kuznetsov, P.I.; Yapaskurt, V.O.; Shchamkhalova, B.S.; Shcherbakov, V.D.; Yakushcheva, G.G.; Luzanova, V.A.; Jitov, V.A. Growth of Bi2Te3 films and other phases of Bi-Te system by MOVPE. J. Cryst. Growth 2016, 455, 122–128. [Google Scholar] [CrossRef]
- Kuznetsova, P.I.; Shchamkhalova, B.S.; Yapaskurt, V.O.; Shcherbakov, V.D.; Luzanova, V.A.; Yakushcheva, G.G.; Jitov, V.A.; Sizov, V.E. MOVPE deposition of Sb2Te3 and other phases of Sb-Te system on sapphire substrate. J. Cryst. Growth 2017, 471, 1–7. [Google Scholar] [CrossRef]
- Park, C.B.; Kim, T.-H.; Sim, K.I.; Kang, B.; Kim, J.W.; Cho, B.; Jeong, K.-H.; Cho, M.-H.; Kim, J.H. Terahertz single conductance quantum and topological phase transitions in topological insulator Bi2Se3 ultrathin films. Nat. Commun. 2015, 6, 6552. [Google Scholar] [CrossRef]
- Tinkham, M. Energy Gap Interpretation of Experiments on Infrared Transmission through Superconducting Films. Phys. Rev. 1956, 104, 845. [Google Scholar] [CrossRef]
- Bilbro, L.S.; Valdés Aguilar, R.; Logvenov, G.; Pelleg, O.; Bozovic, I.; Armitage, N.P. Temporal correlations of superconductivity above the transition temperature in La2−xSrxCuO4 probed by terahertz spectroscopy. Nat. Phys. 2011, 7, 298–302. [Google Scholar] [CrossRef]
- Kuznetsov, K.; Kuznetsov, P.; Frolov, A.; Kovalev, S.; Ilyakov, I.; Ezhov, A.; Kitaeva, G. Bulk and surface terahertz conductivity of Bi2−xSbxTe3−ySey topological insulators. Opt. Eng. 2021, 60, 82012. [Google Scholar] [CrossRef]
- Ren, Z.; Taskin, A.A.; Sasali, S.; Segawa, K.; Ando, Y. Optimizing Bi2−xSbxTe3−ySey solid solutions to approach the intrinsic topological insulator regime. Phys. Rev. 2011, 84, 165311. [Google Scholar] [CrossRef]
- Deinert, J.-C.; Iranzo, D.A.; Pérez, R.; Jia, X.; Hafez, H.A.; Ilyakov, I.; Awari, N.; Chen, M.; Bawatna, M.; Ponomaryov, .N.; et al. Grating-Graphene Metamaterial as a Platform for Terahertz Nonlinear Photonics. ACS Nano 2021, 15, 1145–1154. [Google Scholar] [CrossRef]
- Hafez, H.A.; Kovalev, S.; Deinert, J.-C.; Mics, Z.; Green, B.; Awari, N.; Chen, M.; Germanskiy, S.; Lehnert, U.; Teichert, J.; et al. Extremely efficient terahertz high-harmonic generation in graphene by hot Dirac fermions. Nature 2018, 561, 507–511. [Google Scholar] [CrossRef]
- Theodosi, A.; Tsilipakos, O.; Soukoulis, C.M.; Economou, E.N.; Kafesaki, M. 2D-patterned graphene metasurfaces for efficient third harmonic generation at THz frequencies. Opt. Express 2022, 30, 460–472. [Google Scholar] [CrossRef]
- Song, J.C.W.; Reizer, M.Y.; Levitov, L.S. Disorder-Assisted Electron-Phonon Scattering and Cooling Pathways in Graphene. Phys. Rev. Lett. 2012, 109, 106602. [Google Scholar] [CrossRef] [PubMed]
- Pogna, E.A.A.; Jia, X.; Principi, A.; Block, A.; Banszerus, L.; Zhang, J.; Liu, X.; Sohier, T.; Forti, S.; Soundarapandian, K.; et al. Hot-Carrier Cooling in High-Quality Graphene Is Intrinsically Limited by Optical Phonons. ACS Nano 2021, 15, 11285–11295. [Google Scholar] [CrossRef] [PubMed]
- Tielrooij, K.J.; Principi, A.; Saleta Reig, D.; Block, A.; Varghese, S.; Kiessling, T.; Ilyakov, I.; Ponomaryov, A.; Oliveira, T.; Chen, M.; et al. Light: Science & Applications, 2022; accepted for publication.
- Sobota, J.A.; Yang, S.; Analytis, J.G.; Chen, Y.L.; Fisher, I.R.; Kirchmann, P.S.; Shen, Z.-X. Ultrafast optical excitation of a persistent surface-state population in the topological insulator Bi2Se2. Phys. Rev. Lett. 2012, 108, 117403. [Google Scholar] [CrossRef] [PubMed]
- Gao, Y.-B.; He, B.; Parker, D.; Androulakis, I.; Heremans, J.P. Experimental study of the valence band of Bi2Se3. Phys. Rev. B. 2014, 90, 125204. [Google Scholar] [CrossRef]
- Bieńkowski, Z.; Lipiński, E. Amatorskieanteny KF i UKF; Komunikacji i Łączności: Warsaw, Poland, 1978. [Google Scholar]
- Onishi, Y.; Ren, Z.; Segawa, K.; Kaszub, W.; Lorenc, M.; Ando, Y.; Tanaka, K. Ultrafast carrier relaxation through Auger recombination in the topological insulator Bi1.5Sb0.5Te1.7Se1.3. Phys. Rev. 2015, 91, 85306. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuznetsov, K.A.; Tarasenko, S.A.; Kovaleva, P.M.; Kuznetsov, P.I.; Lavrukhin, D.V.; Goncharov, Y.G.; Ezhov, A.A.; Ponomarev, D.S.; Kitaeva, G.K. Topological Insulator Films for Terahertz Photonics. Nanomaterials 2022, 12, 3779. https://doi.org/10.3390/nano12213779
Kuznetsov KA, Tarasenko SA, Kovaleva PM, Kuznetsov PI, Lavrukhin DV, Goncharov YG, Ezhov AA, Ponomarev DS, Kitaeva GK. Topological Insulator Films for Terahertz Photonics. Nanomaterials. 2022; 12(21):3779. https://doi.org/10.3390/nano12213779
Chicago/Turabian StyleKuznetsov, Kirill A., Sergey A. Tarasenko, Polina M. Kovaleva, Petr I. Kuznetsov, Denis V. Lavrukhin, Yury G. Goncharov, Alexander A. Ezhov, Dmitry S. Ponomarev, and Galiya Kh. Kitaeva. 2022. "Topological Insulator Films for Terahertz Photonics" Nanomaterials 12, no. 21: 3779. https://doi.org/10.3390/nano12213779
APA StyleKuznetsov, K. A., Tarasenko, S. A., Kovaleva, P. M., Kuznetsov, P. I., Lavrukhin, D. V., Goncharov, Y. G., Ezhov, A. A., Ponomarev, D. S., & Kitaeva, G. K. (2022). Topological Insulator Films for Terahertz Photonics. Nanomaterials, 12(21), 3779. https://doi.org/10.3390/nano12213779





