Dynamics of Rotating Micropolar Fluid over a Stretch Surface: The Case of Linear and Quadratic Convection Significance in Thermal Management †
Abstract
1. Introduction
- (i)
- incorporate the micropolar fluid as host fluid,
- (ii)
- analyze with and without quadratic convection on the dynamic of micropolar fluid, and
- (iii)
- consider the significant effects of magnetohydrodynamics subject to a rotating frame of reference.
Research Questions
- (1)
- To explore the significance of material, magnetic, and rotating parameters on the dynamic of microplar fluid: the case of linear and quadratic convection.
- (2)
- To observe the influence of Lorentz and Coriolis forces on micro-rotation profile: the case of linear and quadratic convection.
- (3)
- To observe the variations in skin friction coefficients and Nusselt number against growing values of rotating, magnetic, thermal buoyancy, and material parameters in the case of linear and quadratic convection.
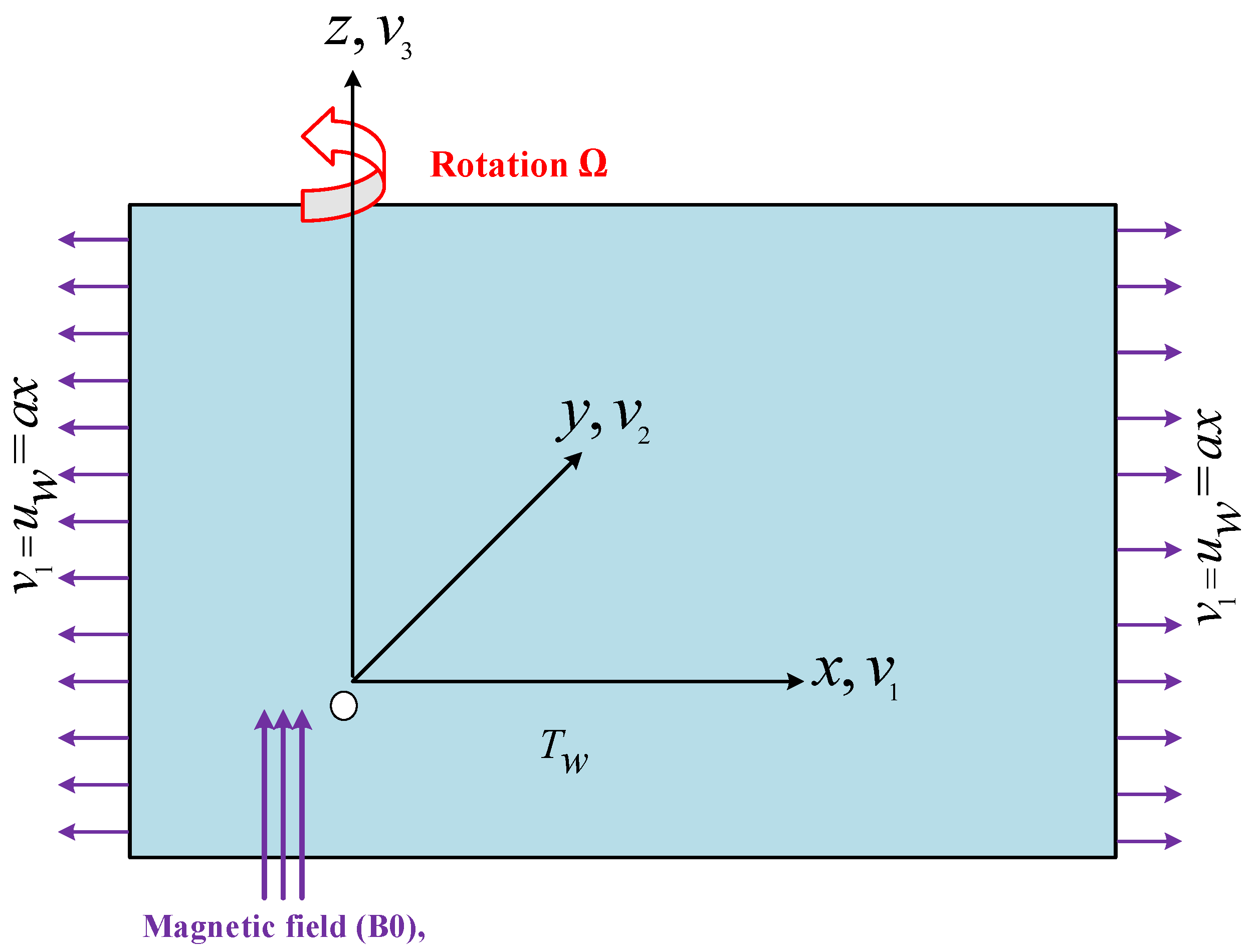
2. Mathematical Formulation
3. Solution Procedure
4. Results and Discussion
- Case 1: with linear convection (, ).
- Case 2: with quadratic convection (, ).
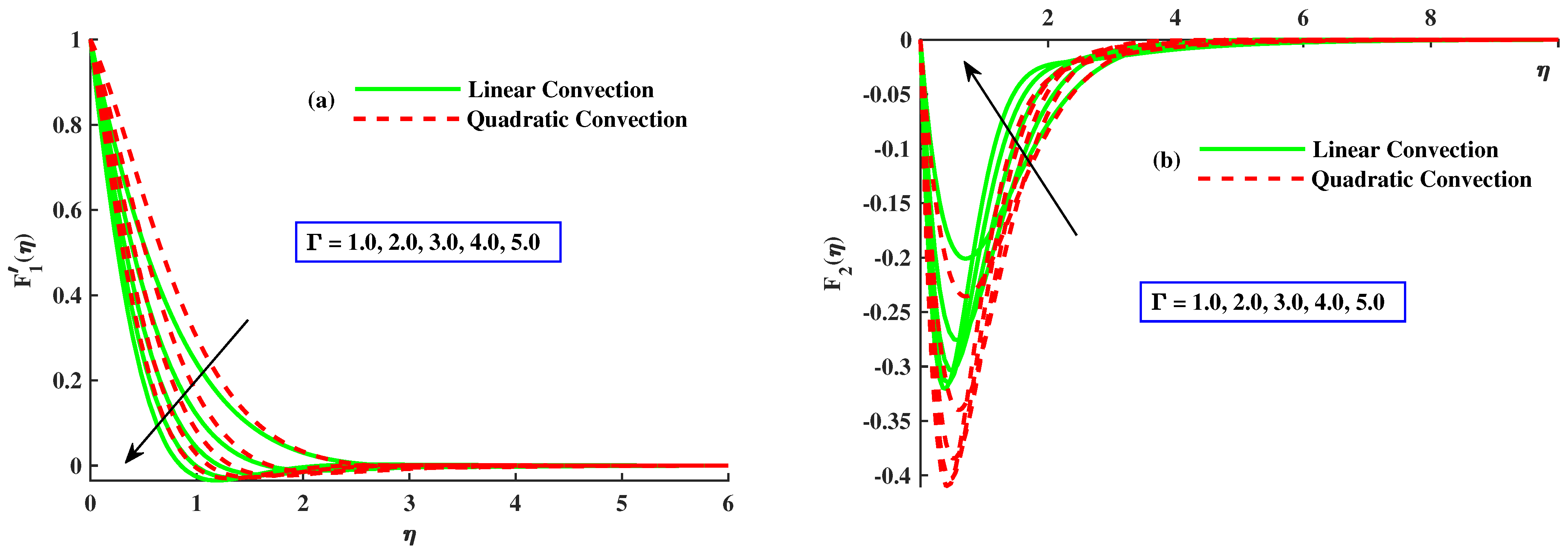
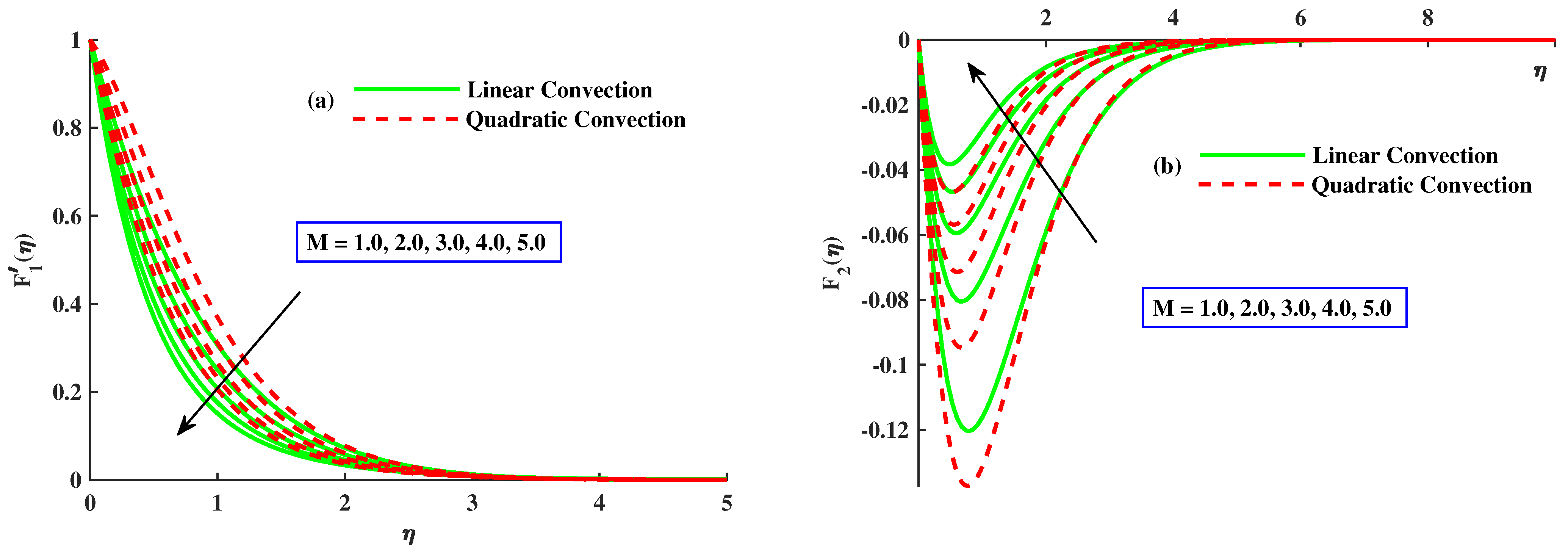

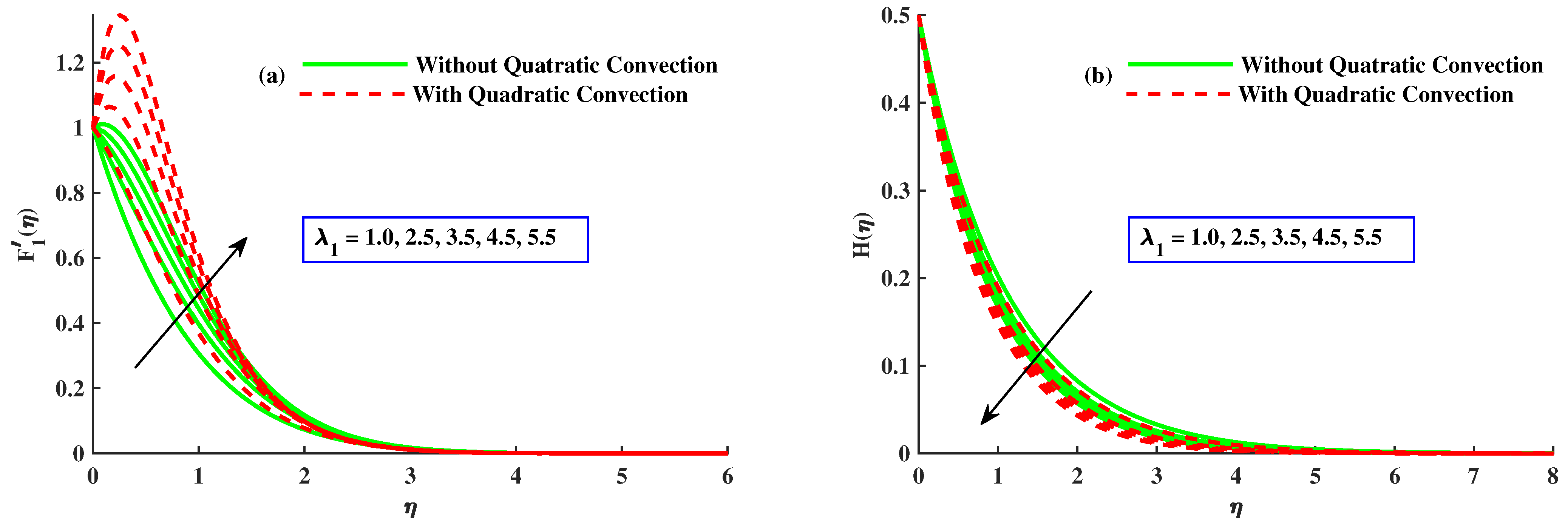
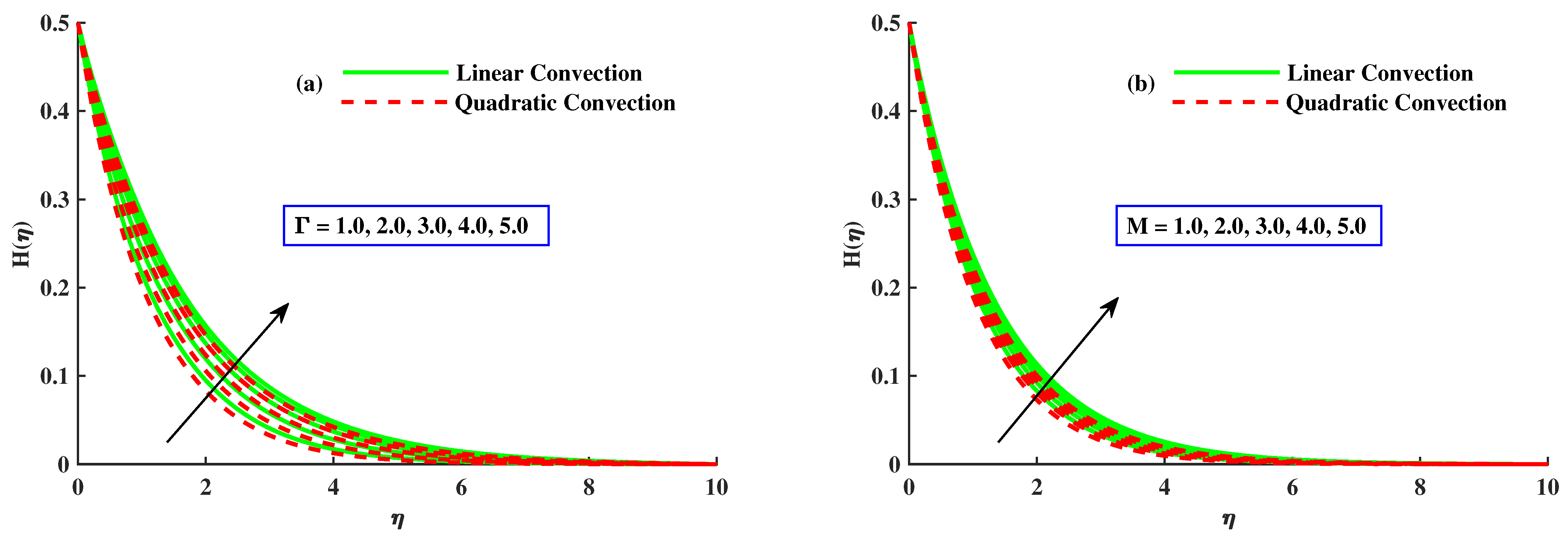
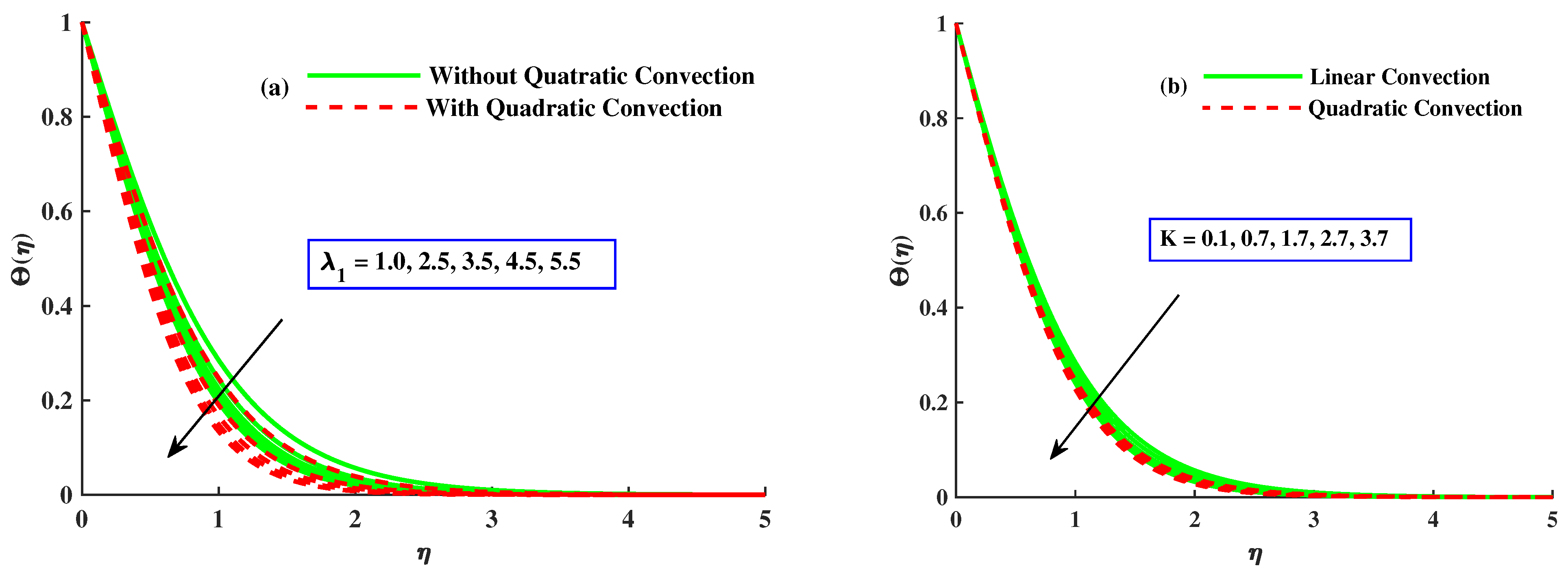
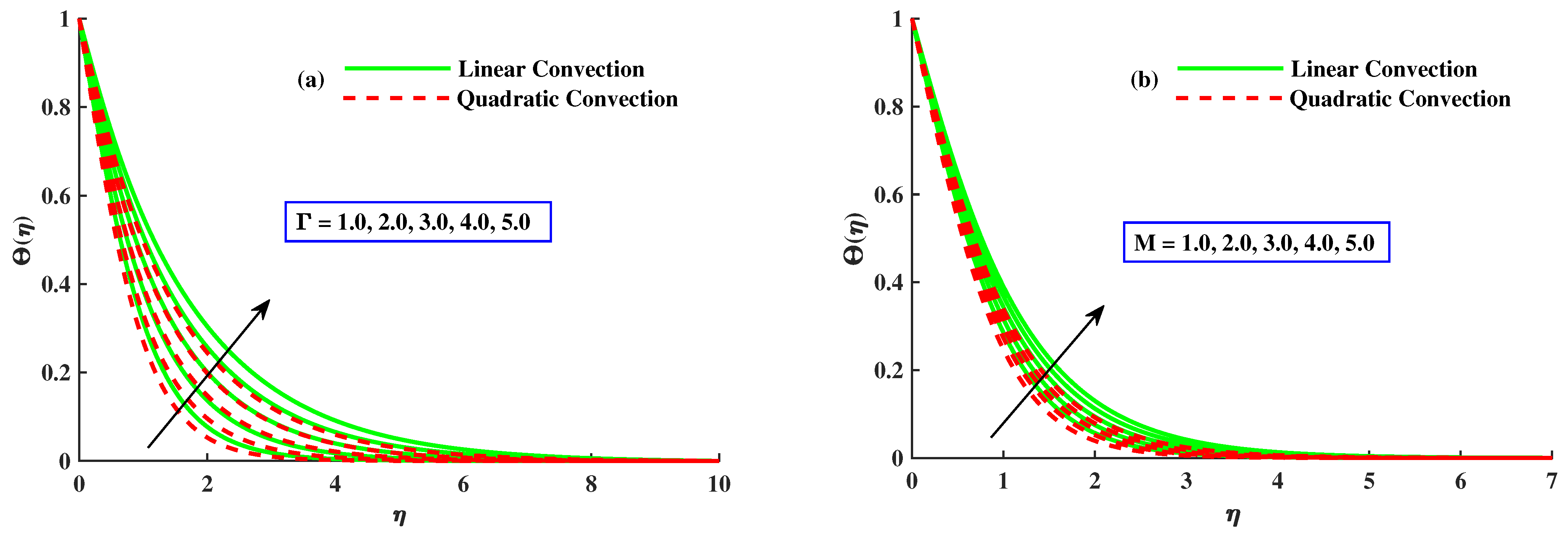
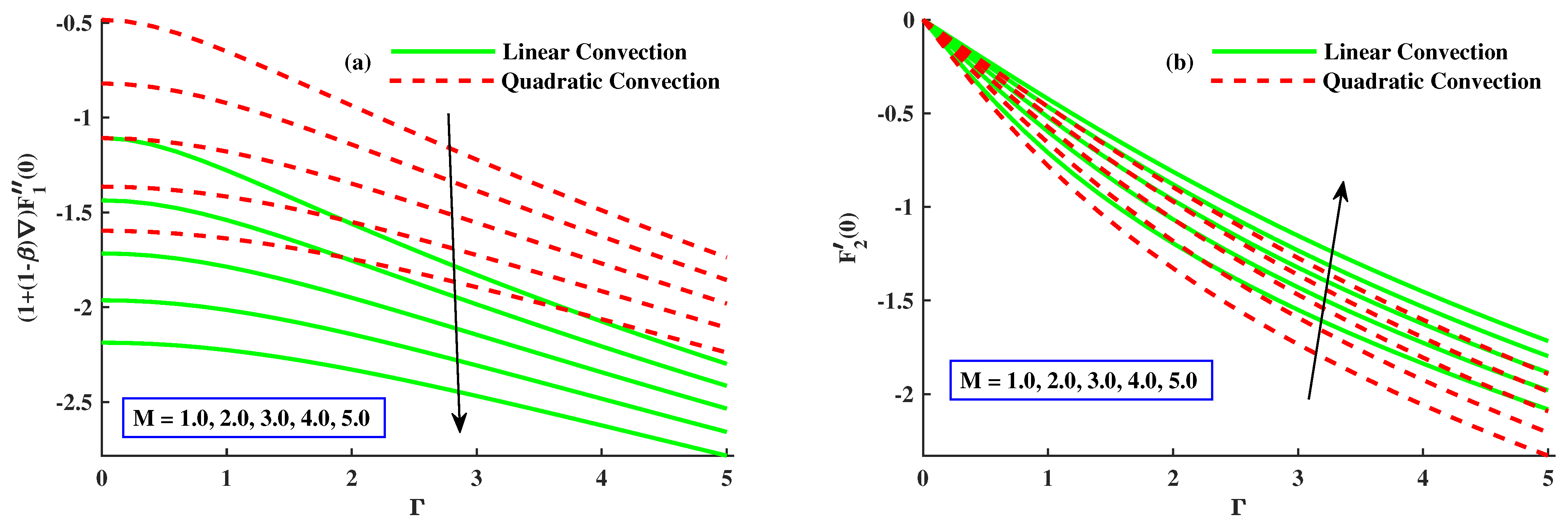
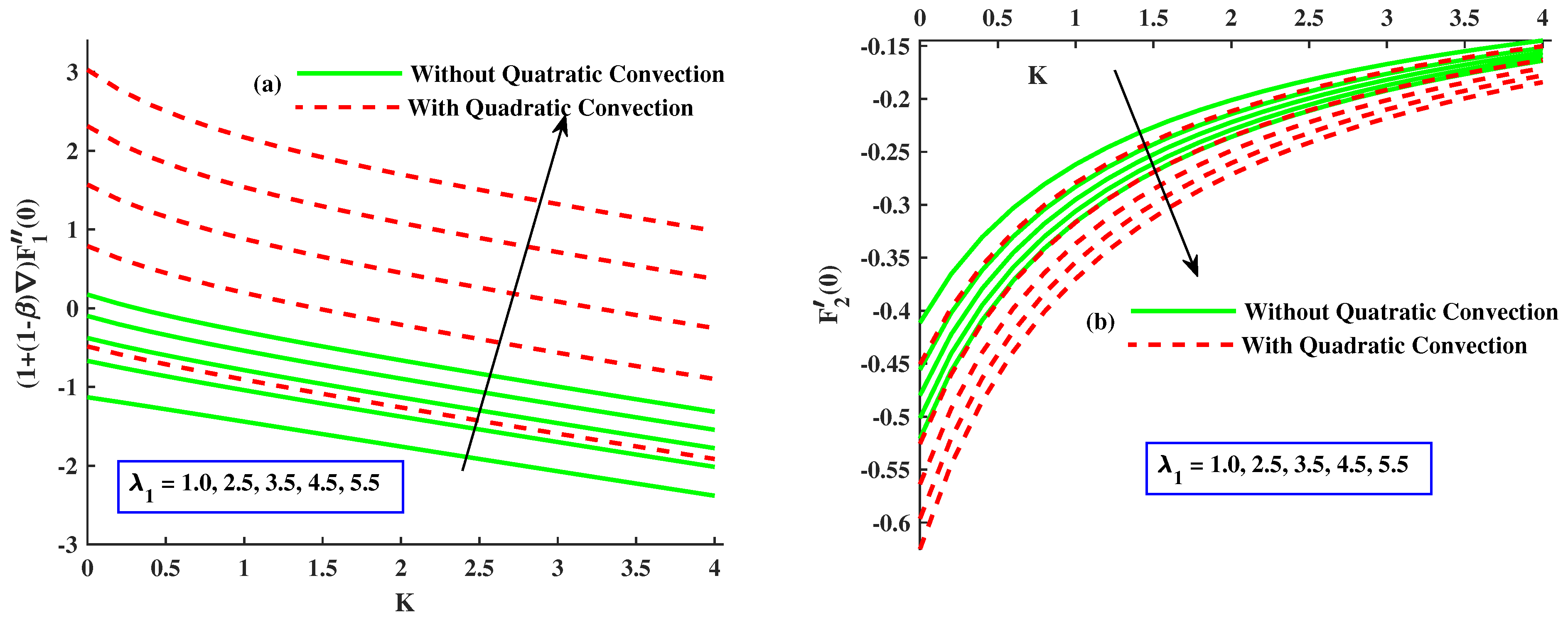
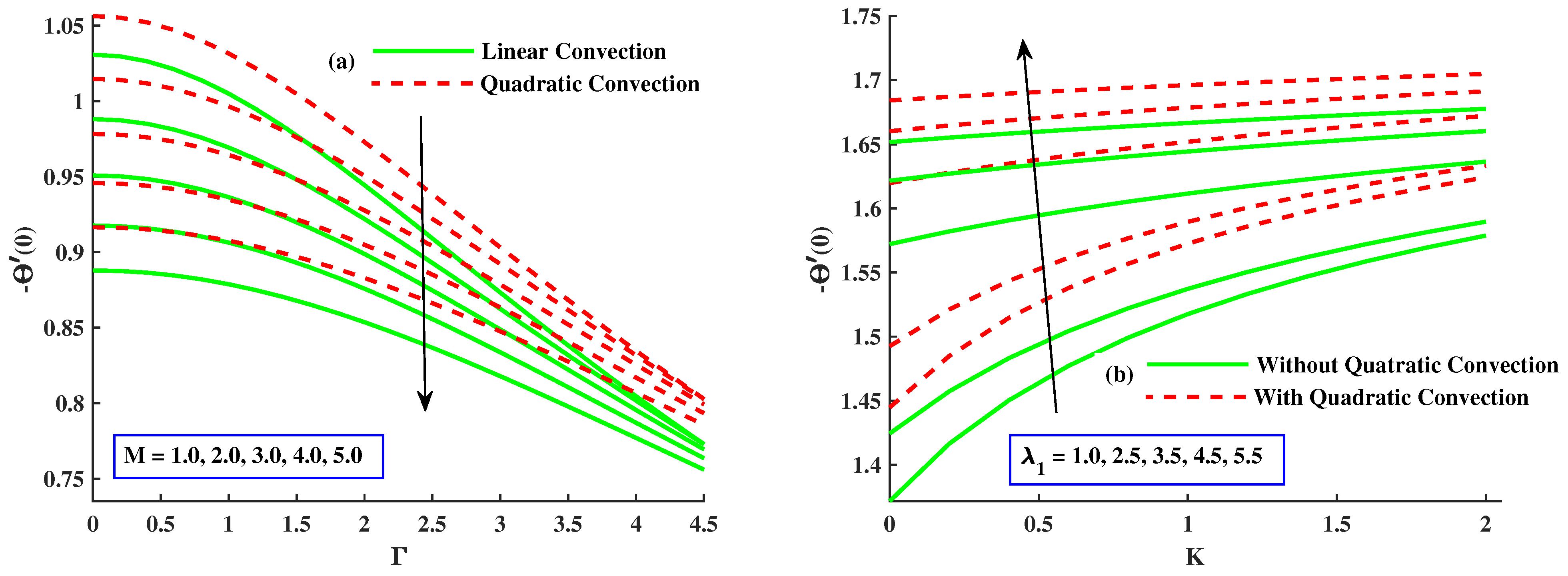
5. Conclusions
- The primary () and secondary () velocities significantly decrease along with rising values of magnetic (M) parameter, rotating () parameter, and in case of linear convection. However, has a growing tendency for the material parameter (K), thermal buoyancy (), and quadratic convection case. The magnitude of has a decreasing behavior for nonlinear convection and material parameter.
- The incremented inputs of rotating, magnetic, and the case of without quadratic convection are augmented the micro-rotation distribution. However, for thermal buoyancy and nonlinear convection case, the micro-rotation profile () decreases.
- The fluid particles’ temperature increases with higher inputs of rotating and magnetic parameters in linear convection case but is reduced against growing strength of rotating () and material (K) parameters in quadratic convection case.
- Along the x direction, the skin friction coefficient decreases with the amplified value of magnetic, rotating, and material parameters in the linear convection case. Along the y direction, the skin friction coefficient is raised by enlargement in magnetic and material parameters without quadratic convection case. However, it decreases for thermal buoyancy and rotating parameters in the quadratic convection case.
- The magnitude of the Nusselt number is reduced with a higher contribution of rotating and magnetic parameters in the linear convection case. However, it increases against material and thermal buoyancy in the nonlinear convection case.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| T | non-dimensional temperature, K |
| temperature away from the surface, K | |
| Nusselt number | |
| skin friction at x-direction | |
| skin friction at y-direction | |
| kinematic viscosity of nanofluid, ms | |
| specific heat at constant pressure, JKgK | |
| electrical conductivity of fluid, KgmA | |
| Prandtl number | |
| dynamic viscosity of fluid, Kgms | |
| surface heat flux, Wm | |
| thermal conductivity of the fluid, WmK | |
| temperature at surface, K | |
| Uniform magnetic field | |
| angular velocity, s | |
| Velocity components | |
| velocity of stretching sheet, ms | |
| Density of nanofluid, Kgm | |
| Uniform magnetic field | |
| K | Material parameter |
| Cartesian co-ordinates | |
| rotation parameter | |
| dimensionless variable | |
| M | magnetic parameter |
References
- Awan, A.U.; Shah, N.A.; Ahmed, N.; Ali, Q.; Riaz, S. Analysis of free convection flow of viscous fluid with damped thermal and mass fluxes. Chin. J. Phys. 2019, 60, 98–106. [Google Scholar] [CrossRef]
- Zikanov, O.; Belyaev, I.; Listratov, Y.; Frick, P.; Razuvanov, N.; Sviridov, V. Mixed convection in pipe and duct flows with strong magnetic fields. Appl. Mech. Rev. 2021, 73, 010801. [Google Scholar] [CrossRef]
- Ali, L.; Wang, Y.; Ali, B.; Liu, X.; Din, A.; Al Mdallal, Q. The function of nanoparticle’s diameter and Darcy-Forchheimer flow over a cylinder with effect of magnetic field and thermal radiation. Case Stud. Therm. Eng. 2021, 28, 101392. [Google Scholar] [CrossRef]
- Armaghani, T.; Sadeghi, M.; Rashad, A.; Mansour, M.; Chamkha, A.J.; Dogonchi, A.; Nabwey, H.A. MHD mixed convection of localized heat source/sink in an Al2O3-Cu/water hybrid nanofluid in L-shaped cavity. Alex. Eng. J. 2021, 60, 2947–2962. [Google Scholar] [CrossRef]
- Eringen, A.C. Theory of micropolar fluids. J. Math. Mech. 1966, 16, 1–18. [Google Scholar] [CrossRef]
- Galdi, G.P.; Rionero, S. A note on the existence and uniqueness of solutions of the micropolar fluid equations. Int. J. Eng. Sci. 1977, 15, 105–108. [Google Scholar] [CrossRef]
- Nazar, R.; Amin, N.; Filip, D.; Pop, I. Stagnation point flow of a micropolar fluid towards a stretching sheet. Int. J. Non-Linear Mech. 2004, 39, 1227–1235. [Google Scholar] [CrossRef]
- Yusuf, T.; Kumar, R.N.; Prasannakumara, B.; Adesanya, S. Irreversibility analysis in micropolar fluid film along an incline porous substrate with slip effects. Int. Commun. Heat Mass Transf. 2021, 126, 105357. [Google Scholar] [CrossRef]
- Bilal, M.; Saeed, A.; Gul, T.; Kumam, W.; Mukhtar, S.; Kumam, P. Parametric simulation of micropolar fluid with thermal radiation across a porous stretching surface. Sci. Rep. 2022, 12, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Ali, L.; Ali, B.; Liu, X.; Iqbal, T.; Zulqarnain, R.M.; Javid, M. A comparative study of unsteady MHD Falkner-Skan wedge flow for non-Newtonian nanofluids considering thermal radiation and activation energy. Chin. J. Phys. 2022, 77, 1625–1638. [Google Scholar] [CrossRef]
- Ali, L.; Ali, B.; Liu, X.; Ahmed, S.; Shah, M.A. Analysis of bio-convective MHD Blasius and Sakiadis flow with Cattaneo-Christov heat flux model and chemical reaction. Chin. J. Phys. 2021, 77, 1963–1975. [Google Scholar] [CrossRef]
- Ali, L.; Ali, B.; Ghori, M.B. Melting effect on Cattaneo–Christov and thermal radiation features for aligned MHD nanofluid flow comprising microorganisms to leading edge: FEM approach. Comput. Math. Appl. 2022, 109, 260–269. [Google Scholar] [CrossRef]
- Jang, J.; Lee, S.S. Theoretical and experimental study of MHD (magnetohydrodynamic) micropump. Sens. Actuators A Phys. 2000, 80, 84–89. [Google Scholar] [CrossRef]
- Ali, B.; Siddique, I.; Shafiq, A.; Abdal, S.; Khan, I.; Khan, A. Magnetohydrodynamic mass and heat transport over a stretching sheet in a rotating nanofluid with binary chemical reaction, non-fourier heat flux, and swimming microorganisms. Case Stud. Therm. Eng. 2021, 28, 101367. [Google Scholar] [CrossRef]
- Chabani, I.; Mebarek-Oudina, F.; Ismail, A.A.I. MHD Flow of a Hybrid Nano-fluid in a Triangular Enclosure with Zigzags and an Elliptic Obstacle. Micromachines 2022, 13, 224. [Google Scholar] [CrossRef] [PubMed]
- Abbas, N.; Nadeem, S.; Saleem, A.; Malik, M.; Issakhov, A.; Alharbi, F.M. Models base study of inclined MHD of hybrid nanofluid flow over nonlinear stretching cylinder. Chin. J. Phys. 2021, 69, 109–117. [Google Scholar] [CrossRef]
- Sajid, M.; Javed, T.; Hayat, T. MHD rotating flow of a viscous fluid over a shrinking surface. Nonlinear Dyn. 2008, 51, 259–265. [Google Scholar] [CrossRef]
- Wang, C. Stretching a surface in a rotating fluid. Zeitschrift für Angew. Math. Physik ZAMP 1988, 39, 177–185. [Google Scholar] [CrossRef]
- Dessie, H. Effects of Chemical Reaction, Activation Energy and Thermal Energy on Magnetohydrodynamics Maxwell Fluid Flow in Rotating Frame. J. Nanofluids 2021, 10, 67–74. [Google Scholar] [CrossRef]
- Nadeem, S.; Abbas, N.; Elmasry, Y.; Malik, M. Numerical analysis of water based CNTs flow of micropolar fluid through rotating frame. Comput. Methods Prog. Biomed. 2020, 186, 105194. [Google Scholar] [CrossRef]
- Spear, J.T.; Gore, J.C. New insights into rotating frame relaxation at high field. NMR Biomed. 2016, 29, 1258–1273. [Google Scholar] [CrossRef] [PubMed]
- Shahzad, F.; Jamshed, W.; Sajid, T.; Nisar, K.S.; Eid, M.R. Heat transfer analysis of MHD rotating flow of Fe3O4 nanoparticles through a stretchable surface. Commun. Theor. Phys. 2021, 73, 075004. [Google Scholar] [CrossRef]
- Rana, P.; Bhargava, R.; Bég, O.A. Finite element simulation of unsteady magneto-hydrodynamic transport phenomena on a stretching sheet in a rotating nanofluid. Proc. Inst. Mech. Eng. Part N 2013, 227, 77–99. [Google Scholar] [CrossRef]
- Ali, B.; Nie, Y.; Hussain, S.; Habib, D.; Abdal, S. Insight into the dynamics of fluid conveying tiny particles over a rotating surface subject to Cattaneo–Christov heat transfer, Coriolis force, and Arrhenius activation energy. Comput. Math. Appl. 2021, 93, 130–143. [Google Scholar] [CrossRef]
- Oke, A.S. Heat and mass transfer in 3D MHD flow of EG-based ternary hybrid nanofluid over a rotating surface. Arab. J. Sci. Eng. 2022, 1–17. [Google Scholar] [CrossRef]
- Afridi, M.I.; Alkanhal, T.A.; Qasim, M.; Tlili, I. Entropy generation in Cu-Al2O3-H2O hybrid nanofluid flow over a curved surface with thermal dissipation. Entropy 2019, 21, 941. [Google Scholar] [CrossRef]
- Abdul Latiff, N.A.; Uddin, M.J.; Bég, O.A.; Ismail, A.I. Unsteady forced bioconvection slip flow of a micropolar nanofluid from a stretching/shrinking sheet. Proc. Inst. Mech. Eng. Part N 2016, 230, 177–187. [Google Scholar] [CrossRef]
- Rees, D.A.S.; Bassom, A.P. The Blasius boundary-layer flow of a micropolar fluid. Int. J. Eng. Sci. 1996, 34, 113–124. [Google Scholar] [CrossRef]
- Ali, B.; Pattnaik, P.; Naqvi, R.A.; Waqas, H.; Hussain, S. Brownian motion and thermophoresis effects on bioconvection of rotating Maxwell nanofluid over a Riga plate with Arrhenius activation energy and Cattaneo-Christov heat flux theory. Therm. Sci. Eng. Prog. 2021, 23, 100863. [Google Scholar] [CrossRef]
- Javed, T.; Ahmad, I.; Abbas, Z.; Hayat, T. Rotating flow of a micropolar fluid induced by a stretching surface. Zeitschrift für Naturforschung A 2010, 65, 829–843. [Google Scholar] [CrossRef][Green Version]
- Jusoh, R.; Nazar, R.; Pop, I. Magnetohydrodynamic rotating flow and heat transfer of ferrofluid due to an exponentially permeable stretching/shrinking sheet. J. Magn. Magn. Mater. 2018, 465, 365–374. [Google Scholar] [CrossRef]
- Ali, B.; Hussain, S.; Nie, Y.; Ali, L.; Hassan, S.U. Finite element simulation of bioconvection and cattaneo-Christov effects on micropolar based nanofluid flow over a vertically stretching sheet. Chin. J. Phys. 2020, 68, 654–670. [Google Scholar] [CrossRef]
- Ali, B.; Khan, S.A.; Hussein, A.K.; Thumma, T.; Hussain, S. Hybrid nanofluids: Significance of gravity modulation, heat source/sink, and magnetohydrodynamic on dynamics of micropolar fluid over an inclined surface via finite element simulation. Appl. Math. Comput. 2022, 419, 126878. [Google Scholar] [CrossRef]
- Hayat, T.; Muhammad, T.; Mustafa, M.; Alsaedi, A. An optimal study for three-dimensional flow of Maxwell nanofluid subject to rotating frame. J. Mol. Liq. 2017, 229, 541–547. [Google Scholar] [CrossRef]
- Rehman, S.U.; Mariam, A.; Ullah, A.; Asjad, M.I.; Bajuri, M.Y.; Pansera, B.A.; Ahmadian, A. Numerical computation of buoyancy and radiation effects on MHD micropolar nanofluid flow over a stretching/shrinking sheet with heat source. Case Stud. Therm. Eng. 2021, 25, 100867. [Google Scholar] [CrossRef]
- Wei, Y.; Rehman, S.U.; Fatima, N.; Ali, B.; Ali, L.; Chung, J.D.; Shah, N.A. Significance of Dust Particles, Nanoparticles Radius, Coriolis and Lorentz Forces: The Case of Maxwell Dusty Fluid. Nanomaterials 2022, 12, 1512. [Google Scholar] [CrossRef]
- Ali, B.; Naqvi, R.A.; Ali, L.; Abdal, S.; Hussain, S. A Comparative Description on Time-Dependent Rotating Magnetic Transport of a Water Base Liquid H2O With Hybrid Nano-materials Al2O3-Cu and Al2O3-TiO2 Over an Extending Sheet Using Buongiorno Model: Finite Element Approach. Chin. J. Phys. 2021, 70, 125–139. [Google Scholar] [CrossRef]
- Awan, A.U.; Ahammad, N.A.; Majeed, S.; Gamaoun, F.; Ali, B. Significance of hybrid nanoparticles, Lorentz and Coriolis forces on the dynamics of water based flow. Int. Commun. Heat Mass Transf. 2022, 135, 106084. [Google Scholar] [CrossRef]
- Ali, B.; Nie, Y.; Hussain, S.; Manan, A.; Sadiq, M.T. Unsteady magneto-hydrodynamic transport of rotating Maxwell nanofluid flow on a stretching sheet with Cattaneo–Christov double diffusion and activation energy. Therm. Sci. Eng. Prog. 2020, 20, 100720. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, B.; Ahammad, N.A.; Awan, A.U.; Guedri, K.; Tag-ElDin, E.M.; Majeed, S. Dynamics of Rotating Micropolar Fluid over a Stretch Surface: The Case of Linear and Quadratic Convection Significance in Thermal Management. Nanomaterials 2022, 12, 3100. https://doi.org/10.3390/nano12183100
Ali B, Ahammad NA, Awan AU, Guedri K, Tag-ElDin EM, Majeed S. Dynamics of Rotating Micropolar Fluid over a Stretch Surface: The Case of Linear and Quadratic Convection Significance in Thermal Management. Nanomaterials. 2022; 12(18):3100. https://doi.org/10.3390/nano12183100
Chicago/Turabian StyleAli, Bagh, N. Ameer Ahammad, Aziz Ullah Awan, Kamel Guedri, ElSayed M. Tag-ElDin, and Sonia Majeed. 2022. "Dynamics of Rotating Micropolar Fluid over a Stretch Surface: The Case of Linear and Quadratic Convection Significance in Thermal Management" Nanomaterials 12, no. 18: 3100. https://doi.org/10.3390/nano12183100
APA StyleAli, B., Ahammad, N. A., Awan, A. U., Guedri, K., Tag-ElDin, E. M., & Majeed, S. (2022). Dynamics of Rotating Micropolar Fluid over a Stretch Surface: The Case of Linear and Quadratic Convection Significance in Thermal Management. Nanomaterials, 12(18), 3100. https://doi.org/10.3390/nano12183100







