Topological Phase and Quantum Anomalous Hall Effect in Ferromagnetic Transition-Metal Dichalcogenides Monolayer
Abstract
:1. Introduction
2. Method
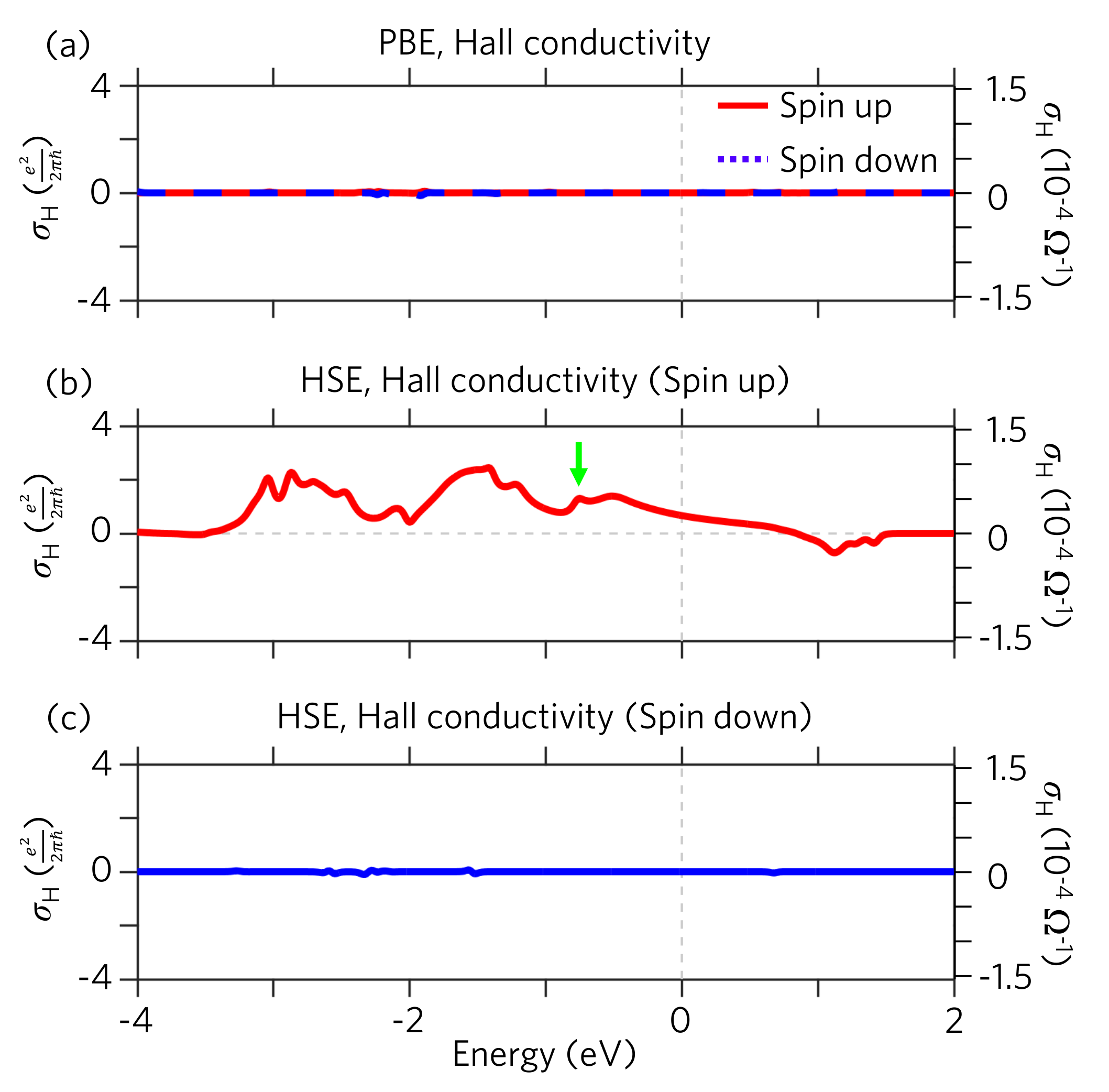
3. Result and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Haldane, F.D.M. Model for a Quantum Hall Effect without Landau Levels: Condensed-Matter Realization of the “Parity Anomaly”. Phys. Rev. Lett. 1988, 61, 2015–2018. [Google Scholar] [CrossRef]
- Kane, C.L.; Mele, E.J. Z2 Topological Order and the Quantum Spin Hall Effect. Phys. Rev. Lett. 2005, 95, 146802. [Google Scholar] [CrossRef] [Green Version]
- Bernevig, B.A.; Hughes, T.L.; Zhang, S.C. Quantum Spin Hall Effect and Topological Phase Transition in HgTe Quantum Wells. Science 2006, 314, 1757–1761. [Google Scholar] [CrossRef] [Green Version]
- Chen, Y.L.; Analytis, J.G.; Chu, J.H.; Liu, Z.K.; Mo, S.K.; Qi, X.L.; Zhang, H.J.; Lu, D.H.; Dai, X.; Fang, Z.; et al. Experimental Realization of a Three-Dimensional Topological Insulator, Bi2Te3. Science 2009, 325, 178–181. [Google Scholar] [CrossRef] [Green Version]
- Brousseau-Couture, V.; Antonius, G.; Côté, M. Temperature dependence of the topological phase transition of BiTeI from first principles. Phys. Rev. Res. 2020, 2, 023185. [Google Scholar] [CrossRef]
- Chang, T.R.; Xu, S.Y.; Chang, G.; Lee, C.C.; Huang, S.M.; Wang, B.; Bian, G.; Zheng, H.; Sanchez, D.S.; Belopolski, I.; et al. Prediction of an arc-tunable Weyl Fermion metallic state in MoxW1-xTe2. Nat. Commun. 2016, 7, 1–9. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, Y.; Li, Y.Y.; Rajput, S.; Gilks, D.; Lari, L.; Galindo, P.L.; Weinert, M.; Lazarov, V.K.; Li, L. Tuning Dirac states by strain in the topological insulator Bi2Se3. Nat. Phys. 2014, 10, 294–299. [Google Scholar] [CrossRef]
- Li, S.S.; Ji, W.X.; Zhang, C.W.; Li, P.; Wang, P.J. Robust room-temperature inversion-asymmetry topological transitions in functionalized HgSe monolayer. J. Mater. Chem. C 2016, 4, 2243–2251. [Google Scholar] [CrossRef]
- Chang, C.Z.; Zhang, J.; Feng, X.; Shen, J.; Zhang, Z.; Guo, M.; Li, K.; Ou, Y.; Wei, P.; Wang, L.L.; et al. Experimental Observation of the Quantum Anomalous Hall Effect in a Magnetic Topological Insulator. Science 2013, 340, 167–170. [Google Scholar] [CrossRef] [Green Version]
- Deng, Y.; Yu, Y.; Shi, M.Z.; Guo, Z.; Xu, Z.; Wang, J.; Chen, X.H.; Zhang, Y. Quantum anomalous Hall effect in intrinsic magnetic topological insulator MnBi2Te4. Science 2020, 367, 895–900. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Li, Y.; Du, S.; Wang, Z.; Gu, B.L.; Zhang, S.C.; He, K.; Duan, W.; Xu, Y. Intrinsic magnetic topological insulators in van der Waals layered MnBi2Te4-family materials. Sci. Adv. 2019, 5, eaaw5685. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yin, J.X.; Ma, W.; Cochran, T.A.; Xu, X.; Zhang, S.S.; Tien, H.J.; Shumiya, N.; Cheng, G.; Jiang, K.; Lian, B.; et al. Quantum-limit Chern topological magnetism in TbMn6Sn6. Nature 2020, 583, 533–536. [Google Scholar] [CrossRef] [PubMed]
- He, Q.L.; Pan, L.; Stern, A.L.; Burks, E.C.; Che, X.; Yin, G.; Wang, J.; Lian, B.; Zhou, Q.; Choi, E.S.; et al. Chiral Majorana fermion modes in a quantum anomalous Hall insulator–superconductor structure. Science 2017, 357, 294–299. [Google Scholar] [CrossRef] [Green Version]
- Yu, W.; Li, J.; Herng, T.S.; Wang, Z.; Zhao, X.; Chi, X.; Fu, W.; Abdelwahab, I.; Zhou, J.; Dan, J.; et al. Chemically Exfoliated VSe2 Monolayers with Room-Temperature Ferromagnetism. Adv. Mater. 2019, 31, 1903779. [Google Scholar] [CrossRef]
- Bonilla, M.; Kolekar, S.; Ma, Y.; Diaz, H.C.; Kalappattil, V.; Das, R.; Eggers, T.; Gutierrez, H.R.; Phan, M.H.; Batzill, M. Strong room-temperature ferromagnetism in VSe2 monolayers on van der Waals substrates. Nat. Nanotechnol. 2018, 13, 289–293. [Google Scholar] [CrossRef]
- Huang, B.; Clark, G.; Navarro-Moratalla, E.; Klein, D.R.; Cheng, R.; Seyler, K.L.; Zhong, D.; Schmidgall, E.; McGuire, M.A.; Cobden, D.H.; et al. Layer-dependent ferromagnetism in a van der Waals crystal down to the monolayer limit. Nature 2017, 546, 270–273. [Google Scholar] [CrossRef] [Green Version]
- Gong, C.; Li, L.; Li, Z.; Ji, H.; Stern, A.; Xia, Y.; Cao, T.; Bao, W.; Wang, C.; Wang, Y.; et al. Discovery of intrinsic ferromagnetism in two-dimensional van der Waals crystals. Nature 2017, 546, 265–269. [Google Scholar] [CrossRef] [Green Version]
- Deng, Y.; Yu, Y.; Song, Y.; Zhang, J.; Wang, N.Z.; Sun, Z.; Yi, Y.; Wu, Y.Z.; Wu, S.; Zhu, J.; et al. Gate-tunable room-temperature ferromagnetism in two-dimensional Fe3GeTe2. Nature 2018, 563, 94–99. [Google Scholar] [CrossRef] [PubMed]
- Chen, G.; Howard, S.T.; Maghirang, A.B.; Nguyen Cong, K.; Villaos, R.A.B.; Feng, L.Y.; Cai, K.; Ganguli, S.C.; Swiech, W.; Morosan, E.; et al. Correlating structural, electronic, and magnetic properties of epitaxial VSe2 thin films. Phys. Rev. B 2020, 102, 115149. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [Green Version]
- Krukau, A.V.; Vydrov, O.A.; Izmaylov, A.F.; Scuseria, G.E. Influence of the exchange screening parameter on the performance of screened hybrid functionals. J. Chem. Phys. 2006, 125, 224106. [Google Scholar] [CrossRef]
- Franchini, C.; Kováčik, R.; Marsman, M.; Murthy, S.S.; He, J.; Ederer, C.; Kresse, G. Maximally localized Wannier functions in LaMnO3 within PBE + U, hybrid functionals and partially self-consistent GW: An efficient route to construct ab initio tight-binding parameters for eg perovskites. J. Phys. Condens. Matter 2012, 24, 235602. [Google Scholar] [CrossRef] [Green Version]
- Sancho, M.P.L.; Sancho, J.M.L.; Rubio, J. Highly convergent schemes for the calculation of bulk and surface Green functions. J. Phys. F Met. Phys. 1985, 15, 851–858. [Google Scholar] [CrossRef]
- Yu, R.; Qi, X.L.; Bernevig, A.; Fang, Z.; Dai, X. Equivalent expression of Z2 topological invariant for band insulators using the non-Abelian Berry connection. Phys. Rev. B 2011, 84, 075119. [Google Scholar] [CrossRef] [Green Version]
- Soluyanov, A.A.; Vanderbilt, D. Wannier representation of Z2 topological insulators. Phys. Rev. B 2011, 83, 035108. [Google Scholar] [CrossRef] [Green Version]
- Vanderbilt, D. Berry Phases and Curvatures. In Berry Phases in Electronic Structure Theory: Electric Polarization, Orbital Magnetization and Topological Insulators; Cambridge University Press: Cambridge, UK, 2018; pp. 75–96. [Google Scholar] [CrossRef]
- Xiao, D.; Chang, M.C.; Niu, Q. Berry phase effects on electronic properties. Rev. Mod. Phys. 2010, 82, 1959–2007. [Google Scholar] [CrossRef] [Green Version]
- Feroze, A.; Na, H.R.; Park, Y.C.; Jun, J.H.; Jung, M.H.; Lee, J.H.; Kim, J.H.; Seong, M.J.; Hong, S.; Chun, S.H.; et al. In-Depth Structural Characterization of 1T-VSe2 Single Crystals Grown by Chemical Vapor Transport. Cryst. Growth Des. 2020, 20, 2860–2865. [Google Scholar] [CrossRef]
- Perdew, J.P.; Levy, M. Physical Content of the Exact Kohn-Sham Orbital Energies: Band Gaps and Derivative Discontinuities. Phys. Rev. Lett. 1983, 51, 1884–1887. [Google Scholar] [CrossRef]
- Ma, Q.; Xu, S.Y.; Shen, H.; MacNeill, D.; Fatemi, V.; Chang, T.R.; Mier Valdivia, A.M.; Wu, S.; Du, Z.; Hsu, C.H.; et al. Observation of the nonlinear Hall effect under time-reversal-symmetric conditions. Nature 2019, 565, 337–342. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xu, S.Y.; Ma, Q.; Shen, H.; Fatemi, V.; Wu, S.; Chang, T.R.; Chang, G.; Valdivia, A.M.M.; Chan, C.K.; Gibson, Q.D.; et al. Electrically switchable Berry curvature dipole in the monolayer topological insulator WTe2. Nat. Phys. 2018, 14, 900–906. [Google Scholar] [CrossRef]
- Zhang, B.Y.; Xu, K.; Yao, Q.; Jannat, A.; Ren, G.; Field, M.R.; Wen, X.; Zhou, C.; Zavabeti, A.; Ou, J.Z. Hexagonal metal oxide monolayers derived from the metal–gas interface. Nat. Mater. 2021, 20, 1073–1078. [Google Scholar] [CrossRef]
- Zhu, Z.; Cai, X.; Yi, S.; Chen, J.; Dai, Y.; Niu, C.; Guo, Z.; Xie, M.; Liu, F.; Cho, J.H.; et al. Multivalency-Driven Formation of Te-Based Monolayer Materials: A Combined First-Principles and Experimental study. Phys. Rev. Lett. 2017, 119, 106101. [Google Scholar] [CrossRef] [Green Version]
- Guo, Y.; Lin, Z.; Zhao, J.Q.; Lou, J.; Chen, Y. Two-dimensional Tunable Dirac/Weyl Semimetal in Non-Abelian Gauge Field. Sci. Rep. 2019, 9, 18516. [Google Scholar] [CrossRef] [PubMed]
- You, J.Y.; Chen, C.; Zhang, Z.; Sheng, X.L.; Yang, S.A.; Su, G. Two-dimensional Weyl half-semimetal and tunable quantum anomalous Hall effect. Phys. Rev. B 2019, 100, 064408. [Google Scholar] [CrossRef] [Green Version]
- Jia, T.; Meng, W.; Zhang, H.; Liu, C.; Dai, X.; Zhang, X.; Liu, G. Weyl Fermions in VI3 Monolayer. Front. Chem. 2020, 8, 722. [Google Scholar] [CrossRef]
- Hasan, M.Z.; Chang, G.; Belopolski, I.; Bian, G.; Xu, S.Y.; Yin, J.X. Weyl, Dirac and high-fold chiral fermions in topological quantum matter. Nat. Rev. Mater. 2021. [Google Scholar] [CrossRef]
- Balendhran, S.; Walia, S.; Nili, H.; Sriram, S.; Bhaskaran, M. Elemental Analogues of Graphene: Silicene, Germanene, Stanene, and Phosphorene. Small 2015, 11, 640–652. [Google Scholar] [CrossRef]
- Grauer, S.; Fijalkowski, K.M.; Schreyeck, S.; Winnerlein, M.; Brunner, K.; Thomale, R.; Gould, C.; Molenkamp, L.W. Scaling of the Quantum Anomalous Hall Effect as an Indicator of Axion Electrodynamics. Phys. Rev. Lett. 2017, 118, 246801. [Google Scholar] [CrossRef] [Green Version]
- Xiao, D.; Jiang, J.; Shin, J.H.; Wang, W.; Wang, F.; Zhao, Y.F.; Liu, C.; Wu, W.; Chan, M.H.W.; Samarth, N.; et al. Realization of the Axion Insulator State in Quantum Anomalous Hall Sandwich Heterostructures. Phys. Rev. Lett. 2018, 120, 056801. [Google Scholar] [CrossRef] [Green Version]
- Zhou, J.; Liang, Q.F.; Weng, H.; Chen, Y.B.; Yao, S.H.; Chen, Y.F.; Dong, J.; Guo, G.Y. Predicted Quantum Topological Hall Effect and Noncoplanar Antiferromagnetism in K0.5RhO2. Phys. Rev. Lett. 2016, 116, 256601. [Google Scholar] [CrossRef] [Green Version]
- Liu, C.X.; Qi, X.L.; Dai, X.; Fang, Z.; Zhang, S.C. Quantum Anomalous Hall Effect in Hg1-yMnyTe Quantum Wells. Phys. Rev. Lett. 2008, 101, 146802. [Google Scholar] [CrossRef] [Green Version]
- Si, L.; Janson, O.; Li, G.; Zhong, Z.; Liao, Z.; Koster, G.; Held, K. Quantum Anomalous Hall State in Ferromagnetic SrRuO3 (111) Bilayers. Phys. Rev. Lett. 2017, 119, 026402. [Google Scholar] [CrossRef] [Green Version]
- Wu, S.C.; Shan, G.; Yan, B. Prediction of Near-Room-Temperature Quantum Anomalous Hall Effect on Honeycomb Materials. Phys. Rev. Lett. 2014, 113, 256401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Huang, C.; Zhou, J.; Wu, H.; Deng, K.; Jena, P.; Kan, E. Quantum anomalous Hall effect in ferromagnetic transition metal halides. Phys. Rev. B 2017, 95, 045113. [Google Scholar] [CrossRef] [Green Version]
- Pushkarev, G.V.; Mazurenko, V.G.; Mazurenko, V.V.; Boukhvalov, D.W. Structural phase transitions in VSe2: Energetics, electronic structure and magnetism. Phys. Chem. Chem. Phys. 2019, 21, 22647–22653. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Esters, M.; Hennig, R.G.; Johnson, D.C. Dynamic instabilities in strongly correlated VSe2 monolayers and bilayers. Phys. Rev. B 2017, 96, 235147. [Google Scholar] [CrossRef] [Green Version]
- Fuh, H.R.; Chang, C.R.; Wang, Y.K.; Evans, R.F.L.; Chantrell, R.W.; Jeng, H.T. Newtype single-layer magnetic semiconductor in transition-metal dichalcogenides VX2 (X=S, Se and Te). Sci. Rep. 2016, 6, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Li, F.; Tu, K.; Chen, Z. Versatile Electronic Properties of VSe2 Bulk, Few-Layers, Monolayer, Nanoribbons, and Nanotubes: A Computational Exploration. J. Phys. Chem. C 2014, 118, 21264–21274. [Google Scholar] [CrossRef]
- Fuh, H.R.; Yan, B.; Wu, S.C.; Felser, C.; Chang, C.R. Metal-insulator transition and the anomalous Hall effect in the layered magnetic materials VS2 and VSe2. New J. Phys. 2016, 18, 113038. [Google Scholar] [CrossRef]
- Qi, X.L.; Hughes, T.L.; Zhang, S.C. Topological field theory of time-reversal invariant insulators. Phys. Rev. B 2008, 78, 195424. [Google Scholar] [CrossRef] [Green Version]
- Qi, X.L.; Li, R.; Zang, J.; Zhang, S.C. Inducing a Magnetic Monopole with Topological Surface States. Science 2009, 323, 1184–1187. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, C.X.; Zhang, S.C.; Qi, X.L. The Quantum Anomalous Hall Effect: Theory and Experiment. Annu. Rev. Condens. Matter Phys. 2016, 7, 301–321. [Google Scholar] [CrossRef]
- Serlin, M.; Tschirhart, C.L.; Polshyn, H.; Zhang, Y.; Zhu, J.; Watanabe, K.; Taniguchi, T.; Balents, L.; Young, A.F. Intrinsic quantized anomalous Hall effect in a moiré heterostructure. Science 2020, 367, 900–903. [Google Scholar] [CrossRef] [Green Version]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, A.; Chen, C.-H.; Chang, C.-H.; Jeng, H.-T.
Topological Phase and Quantum Anomalous Hall Effect in Ferromagnetic Transition-Metal Dichalcogenides Monolayer
Huang A, Chen C-H, Chang C-H, Jeng H-T.
Topological Phase and Quantum Anomalous Hall Effect in Ferromagnetic Transition-Metal Dichalcogenides Monolayer
Huang, Angus, Chin-Hsuan Chen, Ching-Hao Chang, and Horng-Tay Jeng.
2021. "Topological Phase and Quantum Anomalous Hall Effect in Ferromagnetic Transition-Metal Dichalcogenides Monolayer
Huang, A., Chen, C.-H., Chang, C.-H., & Jeng, H.-T.
(2021). Topological Phase and Quantum Anomalous Hall Effect in Ferromagnetic Transition-Metal Dichalcogenides Monolayer






