Electric Transport in Gold-Covered Sodium–Alginate Free-Standing Foils
Abstract
1. Introduction
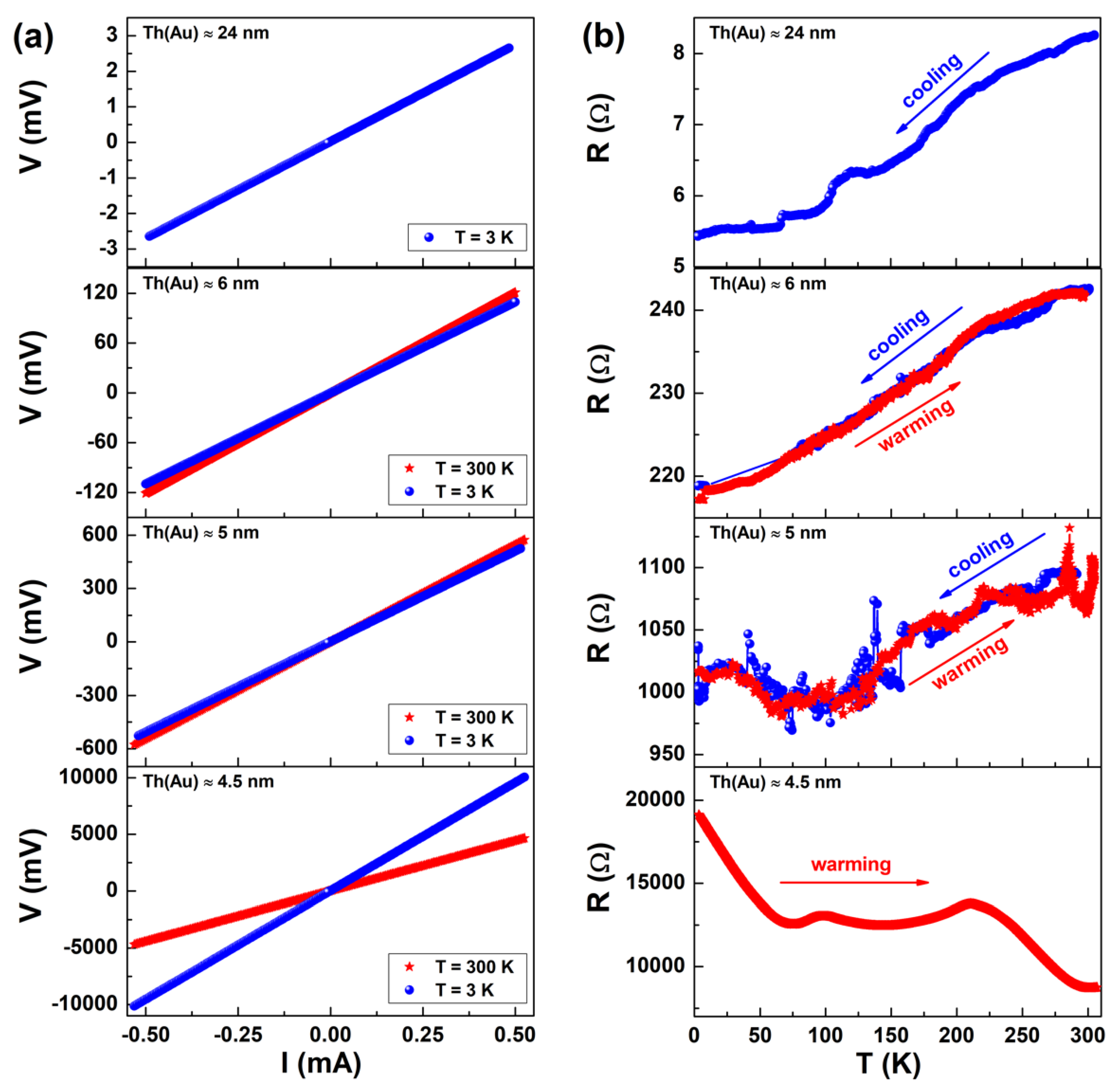
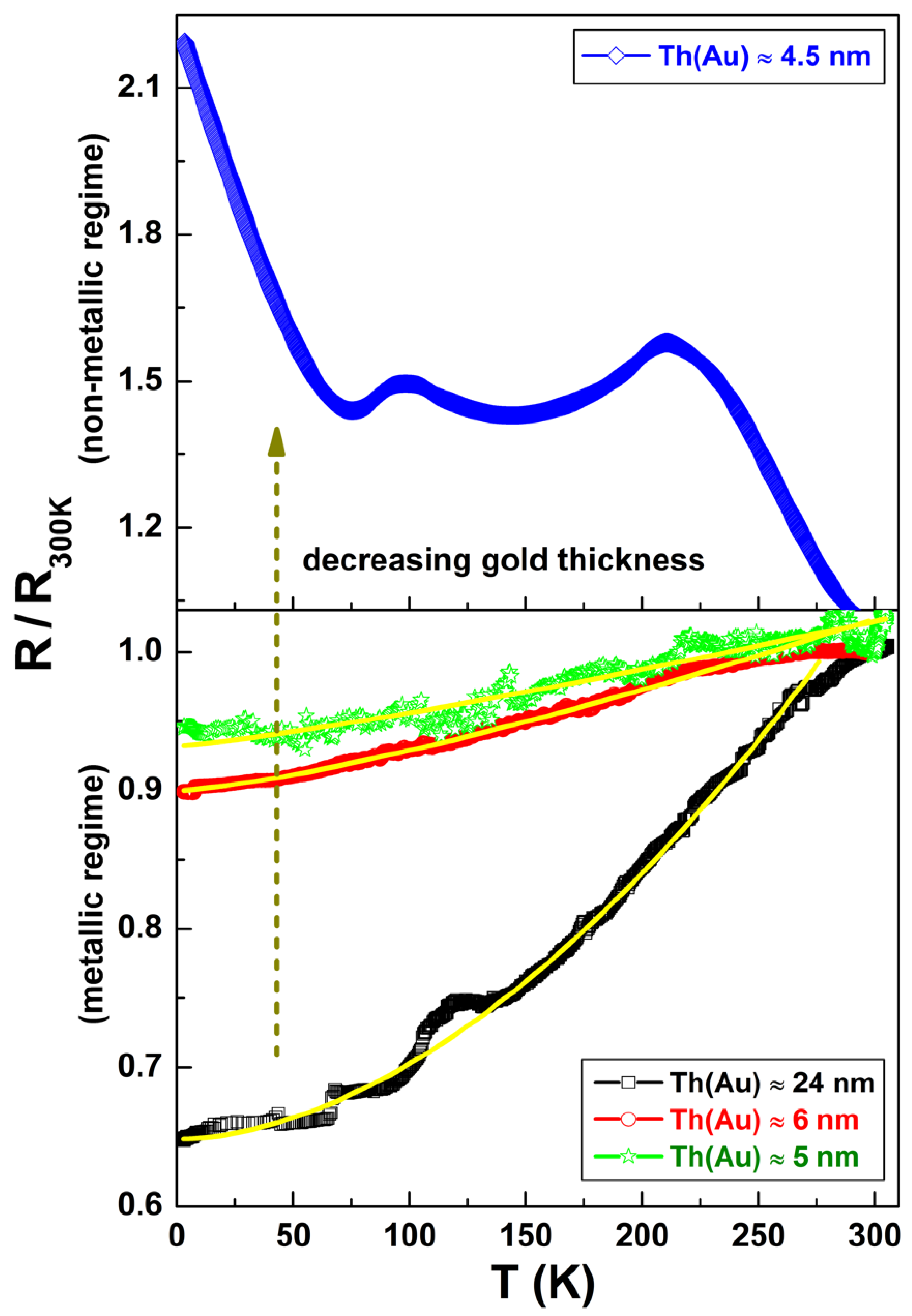
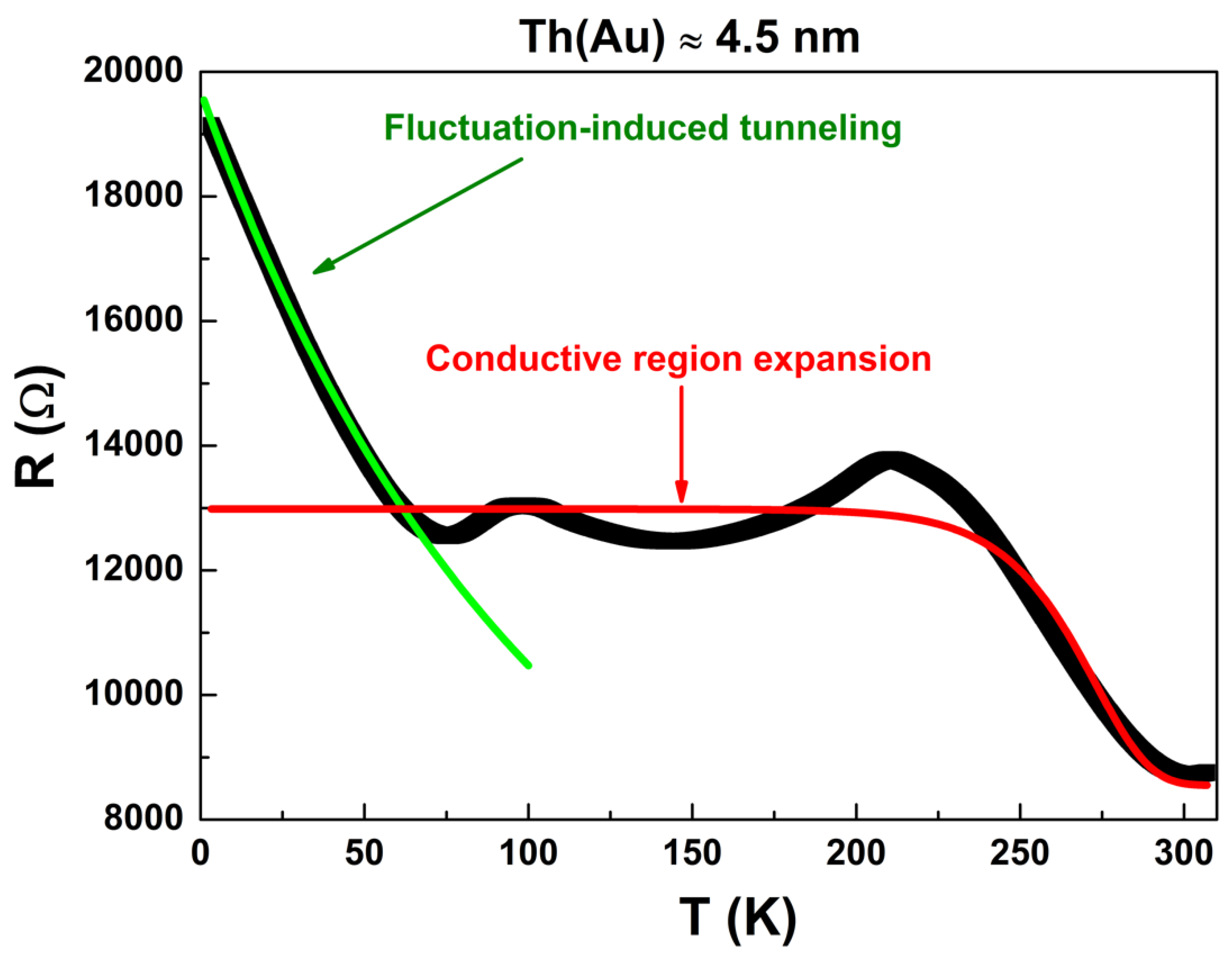
2. Experimental Results and Discussion
3. Materials and Methods
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Irimia-Vladu, M.; Troshin, P.A.; Reisinger, M.; Shmygleva, L.; Kanbur, Y.; Schwabegger, G.; Bodea, M.; Schwödiauer, R.; Mumyatov, A.; Fergus, J.W.; et al. Biocompatible and Biodegradable Materials for Organic Field-Effect Transistors. Adv. Funct. Mater. 2010, 20, 4069–4076. [Google Scholar] [CrossRef]
- Irimia-Vladu, M.; Głowacki, E.D.; Voss, G.; Bauer, S.; Sariciftci, N.S. Green and biodegradable electronics. Mater. Today 2012, 15, 340–346. [Google Scholar] [CrossRef]
- Maccagnani, P.; Bertoldo, M.; Dinelli, F.; Murgia, M.; Summonte, C.; Ortolani, L.; Pizzochero, G.; Verucchi, R.; Collini, C.; Capelli, R. Flexible Conductors from Brown Algae for Green Electronics. Adv. Sustain. Syst. 2019, 3, 1900001. [Google Scholar] [CrossRef]
- Redlinger, M.; Eggert, R.; Woodhouse, M. Evaluating the availability of gallium, indium, and tellurium from recycled photovoltaic modules. Sol. Energy Mater. Sol. Cells 2015, 138, 58–71. [Google Scholar] [CrossRef]
- Frenzel, M.; Mikolajczak, C.; Reuter, M.A.; Gutzmer, J. Quantifying the relative availability of high-tech by-product metals—The cases of gallium, germanium and indium. Resour. Policy 2017, 52, 327–335. [Google Scholar] [CrossRef]
- Crone, B.; Dodabalapur, A.; Lin, Y.-Y.; Filas, R.W.; Bao, Z.; LaDuca, A.; Sarpeshkar, R.; Katz, H.E.; Li, W. Large-scale complementary integrated circuits based on organic transistors. Nature 2000, 403, 521–523. [Google Scholar] [CrossRef] [PubMed]
- Capelli, R.; Amsden, J.J.; Generali, G.; Toffanin, S.; Benfenati, V.; Muccini, M.; Kaplan, D.L.; Omenetto, F.G.; Zamboni, R. Integration of silk protein in organic and light-emitting transistors. Org. Electron. 2011, 12, 1146–1151. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.; Fuentes-Hernandez, C.; Khan, T.M.; Liu, J.-C.; Hsu, J.; Shim, J.W.; Dindar, A.; Youngblood, J.P.; Moon, R.J.; Kippelen, B. Recyclable organic solar cells on cellulose nanocrystal substrates. Sci. Rep. 2013, 3, 1536. [Google Scholar] [CrossRef] [PubMed]
- Fujisaki, Y.; Koga, H.; Nakajima, Y.; Nakata, M.; Tsuji, H.; Yamamoto, T.; Kurita, T.; Nogi, M.; Shimidzu, N. Transparent Nanopaper-Based Flexible Organic Thin-Film Transistor Array. Adv. Funct. Mater. 2014, 24, 1657–1663. [Google Scholar] [CrossRef]
- Rehm, B.H.A.; Moradali, M.F. Alginates and Their Biomedical Applications; Springer: Singapore, 2018; ISBN 978-981-10-6909-3. [Google Scholar]
- Zhu, H.; Fang, Z.; Preston, C.; Li, Y.; Hu, L. Transparent paper: Fabrications, properties, and device applications. Energy Environ. Sci. 2014, 7, 269–287. [Google Scholar] [CrossRef]
- Li, S.; Lee, P.S. Development and applications of transparent conductive nanocellulose paper. Sci. Technol. Adv. Mater. 2017, 18, 620–633. [Google Scholar] [CrossRef]
- Hora, J.; Hall, C.; Evans, D.; Charrault, E. Inorganic Thin Film Deposition and Application on Organic Polymer Substrates. Adv. Eng. Mater. 2018, 20, 1700868. [Google Scholar] [CrossRef]
- Capelli, R.; Maccagnani, P.; Dinelli, F.; Murgia, M.; Bertoldo, M.; Montecchi, M.; Doyle, B.P.; Carleschi, E.; Pasquali, L. Understanding adhesion of gold conductive films on sodium-alginate by photoelectron spectroscopy. Thin Solid Films 2019, 690, 137535. [Google Scholar] [CrossRef]
- Gabrielli, L.; Altoè, G.; Glaeske, M.; Juergensen, S.; Reich, S.; Setaro, A.; Menna, E.; Mancin, F.; Gatti, T. Controlling the Decoration of the Reduced Graphene Oxide Surface with Pyrene-Functionalized Gold Nanoparticles. Phys. Status Solidi 2017, 254, 1700281. [Google Scholar] [CrossRef]
- Mittal, K.L. Metallized Plastic: Fundamentals and Applications; Marcel Dekker: New York, NY, USA, 1998; ISBN 0-8247-9925-9. [Google Scholar]
- Tam, S.K.; Dusseault, J.; Polizu, S.; Ménard, M.; Hallé, J.-P.; Yahia, L. Physicochemical model of alginate–poly-l-lysine microcapsules defined at the micrometric/nanometric scale using ATR-FTIR, XPS, and ToF-SIMS. Biomaterials 2005, 26, 6950–6961. [Google Scholar] [CrossRef]
- Summonte, C.; Maccagnani, P.; Desalvo, A.; Bolognini, G.; Ortolani, L.; Sanmartin, M.; Capelli, R.; Bertoldo, M.; Dinelli, F. Gold nanoparticles on sodium alginate: Simulation of optical properties. In Proceedings of the 20th Italian National Conference on Photonic Technologies (Fotonica 2018), Lecce, Italy, 23–25 May 2018; pp. 1–4. [Google Scholar]
- Barone, C.; Pagano, S.; Pallecchi, I.; Bellingeri, E.; Putti, M.; Ferdeghini, C. Thermal and voltage activated excess 1/f noise in FeTe0.5Se0.5 epitaxial thin films. Phys. Rev. B 2011, 83, 134523. [Google Scholar] [CrossRef]
- Asa, M.; Autieri, C.; Barone, C.; Mauro, C.; Picozzi, S.; Pagano, S.; Cantoni, M. Detecting antiferromagnetism in tetragonal Cr2O3 by electrical measurements. Phys. Rev. B 2019, 100, 174423. [Google Scholar] [CrossRef]
- Cirillo, C.; Barone, C.; Bradshaw, H.; Urban, F.; Di Bernardo, A.; Mauro, C.; Robinson, J.W.A.; Pagano, S.; Attanasio, C. Magnetotransport and magnetic properties of amorphous NdNi5 thin films. Sci. Rep. 2020, 10, 13693. [Google Scholar] [CrossRef] [PubMed]
- Di Trolio, A.; Amore Bonapasta, A.; Barone, C.; Leo, A.; Carapella, G.; Pagano, S.; Polimeni, A.; Testa, A.M. Transport mechanisms in Co-doped ZnO (ZCO) and H-irradiated ZCO polycrystalline thin films. Phys. Chem. Chem. Phys. 2021, 23, 2368–2376. [Google Scholar] [CrossRef] [PubMed]
- Barone, C.; Rotzinger, H.; Mauro, C.; Dorer, D.; Münzberg, J.; Ustinov, A.V.; Pagano, S. Kondo-like transport and magnetic field effect of charge carrier fluctuations in granular aluminum oxide thin films. Sci. Rep. 2018, 8, 13892. [Google Scholar] [CrossRef] [PubMed]
- Barone, C.; Rotzinger, H.; Mauro, C.; Dorer, D.; Ustinov, A.V.; Pagano, S. Unconventional magnetic field effect on noise properties of AlOx thin films in Kondo-like transport regime. Eur. Phys. J. Spec. Top. 2019, 228, 697–702. [Google Scholar] [CrossRef]
- Barone, C.; Rotzinger, H.; Voss, N.J.; Mauro, C.; Schön, Y.; Ustinov, V.A.; Pagano, S. Current-Resistance Effects Inducing Nonlinear Fluctuation Mechanisms in Granular Aluminum Oxide Nanowires. Nanomaterials 2020, 10, 524. [Google Scholar] [CrossRef] [PubMed]
- Barone, C.; Romeo, F.; Pagano, S.; Di Gennaro, E.; Miletto Granozio, F.; Pallecchi, I.; Marrè, D.; Scotti di Uccio, U. Carrier-number fluctuations in the 2-dimensional electron gas at the LaAlO3/SrTiO3 interface. Appl. Phys. Lett. 2013, 103, 231601. [Google Scholar] [CrossRef]
- Mauro, C.; Barone, C.; Di Gennaro, E.; Sambri, A.; Guarino, A.; Granozio, F.M.; Pagano, S. Photoconductivity in 2D electron gases at the amorphous-LGO/STO oxide interface: A dynamical analysis. Eur. Phys. J. Spec. Top. 2019, 228, 675–681. [Google Scholar] [CrossRef]
- Barone, C.; Mauro, C.; Sambri, A.; Scotti di Uccio, U.; Pagano, S. Conductivity response of amorphous oxide interfaces to pulsed light illumination. Nanotechnology 2019, 30, 254005. [Google Scholar] [CrossRef] [PubMed]
- Ziman, J.M. Electrons and Phonons: The Theory of Transport. Phenomena in Solids; Oxford University Press: Oxford, UK, 2001; ISBN 9780198507796. [Google Scholar]
- Poker, D.B.; Klabunde, C.E. Temperature dependence of electrical resistivity of vanadium, platinum, and copper. Phys. Rev. B 1982, 26, 7012–7014. [Google Scholar] [CrossRef]
- Bid, A.; Bora, A.; Raychaudhuri, A.K. Temperature dependence of the resistance of metallic nanowires of diameter ≥ 15 nm: Applicability of Bloch-Grüneisen theorem. Phys. Rev. B 2006, 74, 035426. [Google Scholar] [CrossRef]
- Hor, P.H.; Gao, L.; Meng, R.L.; Huang, Z.J.; Wang, Y.Q.; Forster, K.; Vassilious, J.; Chu, C.W.; Wu, M.K.; Ashburn, J.R.; et al. High-pressure study of the new Y-Ba-Cu-O superconducting compound system. Phys. Rev. Lett. 1987, 58, 911–912. [Google Scholar] [CrossRef] [PubMed]
- Cava, R.J.; Batlogg, B.; van Dover, R.B.; Murphy, D.W.; Sunshine, S.; Siegrist, T.; Remeika, J.P.; Rietman, E.A.; Zahurak, S.; Espinosa, G.P. Bulk superconductivity at 91 K in single-phase oxygen-deficient perovskite Ba2YCu3O9-δ. Phys. Rev. Lett. 1987, 58, 1676–1679. [Google Scholar] [CrossRef] [PubMed]
- Kästle, G.; Boyen, H.-G.; Schröder, A.; Plettl, A.; Ziemann, P. Size effect of the resistivity of thin epitaxial gold films. Phys. Rev. B 2004, 70, 165414. [Google Scholar] [CrossRef]
- Leosson, K.; Ingason, A.S.; Agnarsson, B.; Kossoy, A.; Olafsson, S.; Gather, M.C. Ultra-thin gold films on transparent polymers. Nanophotonics 2013, 2, 3–11. [Google Scholar] [CrossRef]
- Siegel, J.; Lyutakov, O.; Rybka, V.; Kolská, Z.; Švorčík, V. Properties of gold nanostructures sputtered on glass. Nanoscale Res. Lett. 2011, 6, 96. [Google Scholar] [CrossRef]
- Fischer, W.; Geiger, H.; Rudolf, P.; Wissmann, P. Structure investigations on single-crystal gold films. Appl. Phys. 1977, 13, 245–253. [Google Scholar] [CrossRef]
- Qin, W.; Chen, Z.H.; Huang, P.Y.; Zhuang, Y.H. Crystal lattice expansion of nanocrystalline materials. J. Alloys Compd. 1999, 292, 230–232. [Google Scholar] [CrossRef]
- Hazra, D.; Datta, S.; Mondal, M.; Ghatak, J.; Satyam, P.V.; Gupta, A.K. Thickness dependent lattice expansion in nanogranular Nb thin films. J. Appl. Phys. 2008, 103, 103535. [Google Scholar] [CrossRef]
- Zhu, Y.F.; Zheng, W.T.; Jiang, Q. Modeling lattice expansion and cohesive energy of nanostructured materials. Appl. Phys. Lett. 2009, 95, 083110. [Google Scholar] [CrossRef]
- Sheng, P. Fluctuation-induced tunneling conduction in disordered materials. Phys. Rev. B 1980, 21, 2180–2195. [Google Scholar] [CrossRef]
- Lee, P.A.; Ramakrishnan, T. V Disordered electronic systems. Rev. Mod. Phys. 1985, 57, 287–337. [Google Scholar] [CrossRef]
- Savo, B.; Barone, C.; Galdi, A.; Di Trolio, A. dc transport properties and resistance fluctuation processes in Sr2FeMoO6 polycrystalline thin films. Phys. Rev. B 2006, 73, 094447. [Google Scholar] [CrossRef]
- Westerburg, W.; Martin, F.; Jakob, G. Hall effect of epitaxial double-perovskite Sr2FeMoO6 thin films. J. Appl. Phys. 2000, 87, 5040–5042. [Google Scholar] [CrossRef]
- Fisher, B.; Chashka, K.B.; Patlagan, L.; Reisner, G.M. Intergrain tunneling in granular Sr2FeMoO6 studied by pulsed high currents. Phys. Rev. B 2003, 68, 134420. [Google Scholar] [CrossRef]
- Barone, C.; Pagano, S. What Can Electric Noise Spectroscopy Tell Us on the Physics of Perovskites? Coatings 2021, 11, 96. [Google Scholar] [CrossRef]
- Barone, C.; Pagano, S.; Neitzert, H.C. Effect of concentration on low-frequency noise of multiwall carbon nanotubes in high-density polyethylene matrix. Appl. Phys. Lett. 2010, 97, 152107. [Google Scholar] [CrossRef]
- Barone, C.; Landi, G.; Mauro, C.; Neitzert, H.C.; Pagano, S. Universal crossover of the charge carrier fluctuation mechanism in different polymer/carbon nanotubes composites. Appl. Phys. Lett. 2015, 107, 143106. [Google Scholar] [CrossRef]
- Barone, C.; Landi, G.; Mauro, C.; Pagano, S.; Neitzert, H.C. Low-frequency electric noise spectroscopy in different polymer/carbon nanotubes composites. Diam. Relat. Mater. 2016, 65, 32–36. [Google Scholar] [CrossRef]
- Pagano, S.; Barone, C.; Martucciello, N.; Enrico, E.; Croin, L.; Monticone, E.; Iida, K.; Kurth, F. Co-Doped BaFe2As2 Superconducting Nanowires for Detector Applications. IEEE Trans. Appl. Supercond. 2018, 28, 2200204. [Google Scholar] [CrossRef]
- Routoure, J.M.; Wu, S.; Barone, C.; Méchin, L.; Guillet, B. A Low-Noise and Quasi-Ideal DC Current Source Dedicated to Four-Probe Low-Frequency Noise Measurements. IEEE Trans. Instrum. Meas. 2020, 69, 194–200. [Google Scholar] [CrossRef]
- Pagano, S.; Martucciello, N.; Enrico, E.; Monticone, E.; Iida, K.; Barone, C. Iron-Based Superconducting Nanowires: Electric Transport and Voltage-Noise Properties. Nanomaterials 2020, 10, 862. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barone, C.; Bertoldo, M.; Capelli, R.; Dinelli, F.; Maccagnani, P.; Martucciello, N.; Mauro, C.; Pagano, S. Electric Transport in Gold-Covered Sodium–Alginate Free-Standing Foils. Nanomaterials 2021, 11, 565. https://doi.org/10.3390/nano11030565
Barone C, Bertoldo M, Capelli R, Dinelli F, Maccagnani P, Martucciello N, Mauro C, Pagano S. Electric Transport in Gold-Covered Sodium–Alginate Free-Standing Foils. Nanomaterials. 2021; 11(3):565. https://doi.org/10.3390/nano11030565
Chicago/Turabian StyleBarone, Carlo, Monica Bertoldo, Raffaella Capelli, Franco Dinelli, Piera Maccagnani, Nadia Martucciello, Costantino Mauro, and Sergio Pagano. 2021. "Electric Transport in Gold-Covered Sodium–Alginate Free-Standing Foils" Nanomaterials 11, no. 3: 565. https://doi.org/10.3390/nano11030565
APA StyleBarone, C., Bertoldo, M., Capelli, R., Dinelli, F., Maccagnani, P., Martucciello, N., Mauro, C., & Pagano, S. (2021). Electric Transport in Gold-Covered Sodium–Alginate Free-Standing Foils. Nanomaterials, 11(3), 565. https://doi.org/10.3390/nano11030565








