Numerical Analysis of a Novel Twin-Impeller Centrifugal Compressor
Abstract
:1. Introduction
2. Experiment Study
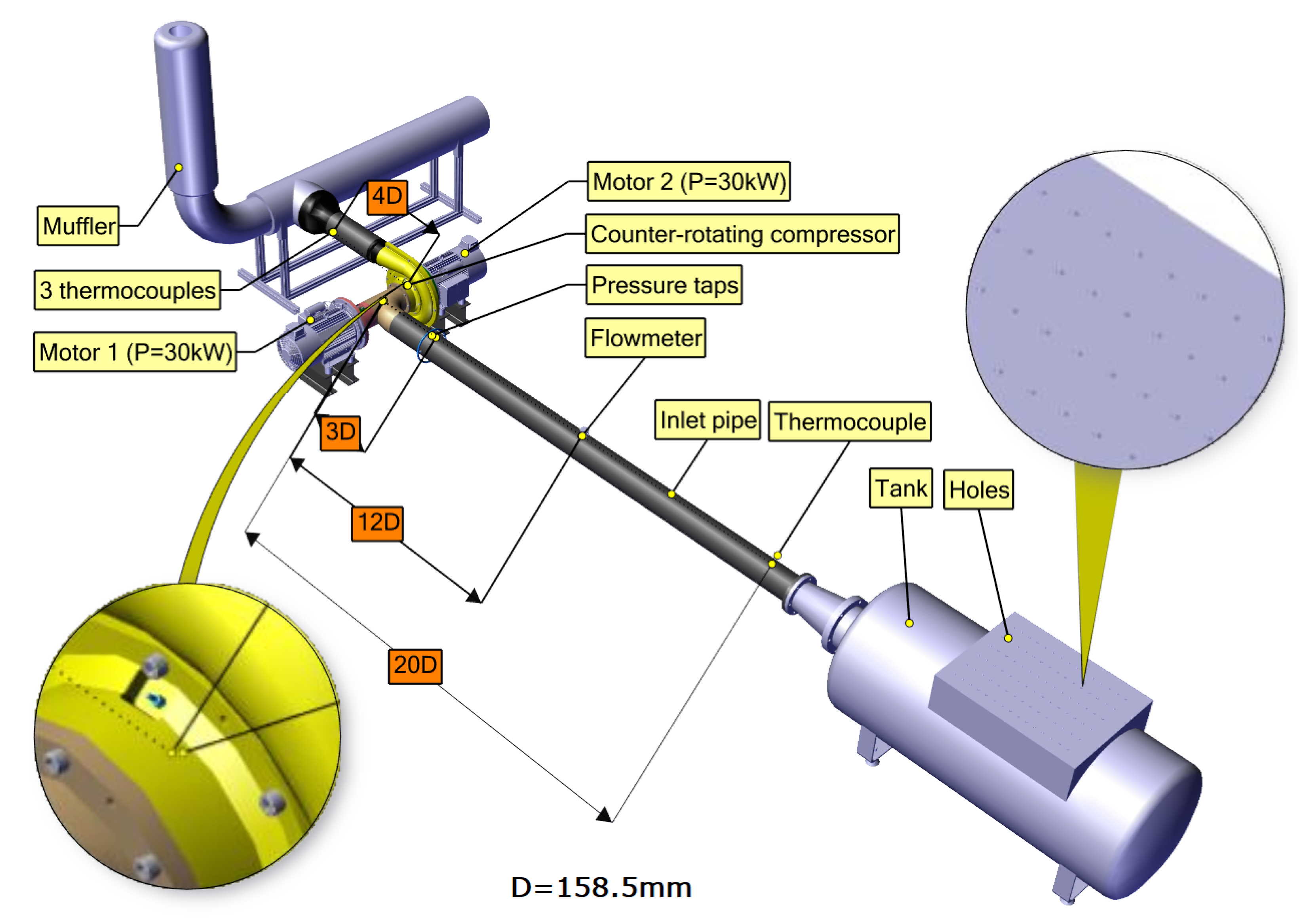
2.1. Experiment Facilities
2.2. Active Control Method to Extend the Operating Range
3. Numerical Study
4. Results and Discussion
4.1. Steady-State Simulation
4.1.1. Flow Analysis
4.1.2. Asymmetry Effect
4.2. Unsteady State Simulation
5. Conclusions
- The steady simulation shows the compressor works with the highest performance because the flow pattern, the pressure distribution within the impellers as well as in the volute are homogeneous at BEP. Nevertheless, the vortex regions can be observed near the volute tongue of the downstream blade passages at NSP. It decreases in size in the adjacent blade passage towards the opposite rotational direction of the impeller. The blade passages are partly or completely blocked by these vortexes, and the local high-pressure regions are generated on the blade surface. The same phenomenon can be seen at ESP, but the number of vortex regions increased significantly in the blade passages. The blade passages are almost blocked by these vortexes except for some passages where the flow pattern is quite uniform. This allows the compressor avoiding the surge state. The increase of the local entropy causing an increase in blade passage losses due to these vortex decrease significantly the compressor efficiency.
- The unsteady simulation illustrates that the blade row position does not affect the flow pattern at BEP but it greatly influences at NSP and ESP. The decrease in size of the vortexes depends on the relative position of the blade row can be observed at NSP. In addition, these vortexes always exist in the blade passages at ESP. This point is the extended surge point whereas the compressor stably operates thanks to the co-rotating effect.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| TICC | Twin Impeller Centrifugal Compressor |
| SICC | Single Impeller Centrifugal Compressor |
| BEP | Best Efficiency Point |
| NSP | Near Surge Point |
| ESP | Extended Surge Point |
| BPF | Blade Passing Frequency |
References
- Botros, K.K.; Henderson, J.F. Developments in centrifugal compressor surge Control-A technology assess-ment. J. Turbomach. 1994, 116, 240–249. [Google Scholar] [CrossRef]
- Dukle, N.; Narayanan, K. Validating anti-surge control systems. PTQ Mag. 2003, 8, 87, 89–92, 94. [Google Scholar]
- Willems, F.P.T. Modelling and Bounded Feedback Stabilization of Centrifugal Compressor Surge. Ph.D. Thesis, Technische Universiteit Eindhoven, Eindhoven, The Netherlands, 2000. [Google Scholar] [CrossRef]
- Day, I.J. Active suppression of rotating stall and surge in axial compressors. J. Turbomach. 1993, 115, 40–47. [Google Scholar] [CrossRef]
- Stein, A.; Niazi, S.; Sankar, L.N. Computational Analysis of Stall and Separation Control in Centrifugal Compres-sors. J. Propuls. Power 2000, 16, 65–71. [Google Scholar] [CrossRef]
- Koyyalamudi, V.V.N.K.; Nagpurwala, Q.H. Stall margin improvement in a centrifugal compressor through inducer casing treatment. Int. J. Rotating Mach. 2016. [Google Scholar] [CrossRef] [Green Version]
- Eynon, P.A.; Whitfield, J.L.; Firth, M.R.; Perkes, A.J.; Sexton, R. A study of the flow characteristics in the inducer bleed slot of a centrifugal compressor. In Proceedings of the ASME 1996 International Gas Turbine and Aeroengine Congress and Exhibition, Birmingham, UK, 10–13 June 1996. [Google Scholar] [CrossRef] [Green Version]
- Nikpour, B. Turbocharger compressor flow range improvement for future heavy duty diesel engines. In Proceedings of the Conference on Thermo and Fluid Dynamics Processes in Diesel Engines, Valencia, Spain, 7–10 September 2004; pp. 125–143. [Google Scholar]
- Cravero, C.; Marsano, D. Criteria for the stability limit prediction of high-speed centrifugal compressors with vaneless diffuser. Part I: Flow structure analysis. In Proceedings of the ASME Turbo Expo 2020: Turbomachinery Technical Conference and Exposition, Virtual Conference. 21–25 September 2020. ASME Paper GT2020-14579. [Google Scholar]
- Cravero, C.; Marsano, D. Criteria for the stability limit prediction of high-speed centrifugal compressors with vaneless diffuser. Part II: The development of prediction criteria. In Proceedings of the ASME Turbo Expo 2020: Turbomachinery Technical Conference and Exposition, Virtual Conference. 21–25 September 2020. ASME Paper GT2020-14589. [Google Scholar]
- Nguyen, V.T.; Danlos, A.; Paridaens, R.; Ravelet, F.; Deligant, M.; Khelladi, S.; Bakir, F. Experimental study of a centrifugal compressor with two successive and counter-rotating impellers. J. Phys. Conf. Ser. 2021, 1909, 012023. [Google Scholar] [CrossRef]
- Nguyen, V.; Abed, C.B.; Danlos, A.; Ravelet, F.; Paridaens, R.; Deligant, M.; Khelladi, S.; Bakir, F. Experimental Study of a Novel Centrifugal Compressor with Two Successive and Independent Rotors. ASME J. Eng. Gas Turbines Power 2021, 144, 011005. [Google Scholar] [CrossRef]
- Nguyen, V.T.; Danlos, A.; Ravelet, F.; Deligant, M.; Solis, M.; Khelladi, S.; Bakir, F. CFD Analysis to explain the Operating range extension observed during Operation in Co-rotating Mode of a Twin-impeller Centrifugal Compressor. In Proceedings of the XIII International Conference on Computational Heat, Mass and Momentum Transfer (ICCHMT 2021), Virtual Conference. 18–19 May 2021; Volume 321. [Google Scholar] [CrossRef]
- Broatch, A.; Galindo, J.; Navarro, R.; García-Tíscar, J.; Daglish, A.; Sharma, R.K. Simulations and measurements of automotive turbocharger compressor whoosh noise. Eng. Appl. Comput. Fluid Mech. 2015, 9, 12–20. [Google Scholar] [CrossRef] [Green Version]
- Galindo, J.; Hoyas, S.; Fajardo, P.; Navarro, R. Set-up analysis and optimization of CFD simulation for radial tur-bines. Eng. Appl. Comput. Fluid Mech. 2013, 7, 441–460. [Google Scholar]
- Bardina, J.E.; Huang, P.G.; Coakley, T.J. Turbulence Modeling Validation, Testing, and Development; NASA Technical Memorandum 110446; Ames Research Center: Moffett Field, CA, USA, 1997; pp. 8–20. [Google Scholar] [CrossRef]
- Eckardt, D. Instantaneous Measurements in the Jet-Wake Discharge Flow of a Centrifugal Compressor Impeller. J. Eng. Power 1975, 97, 337–345. [Google Scholar] [CrossRef]
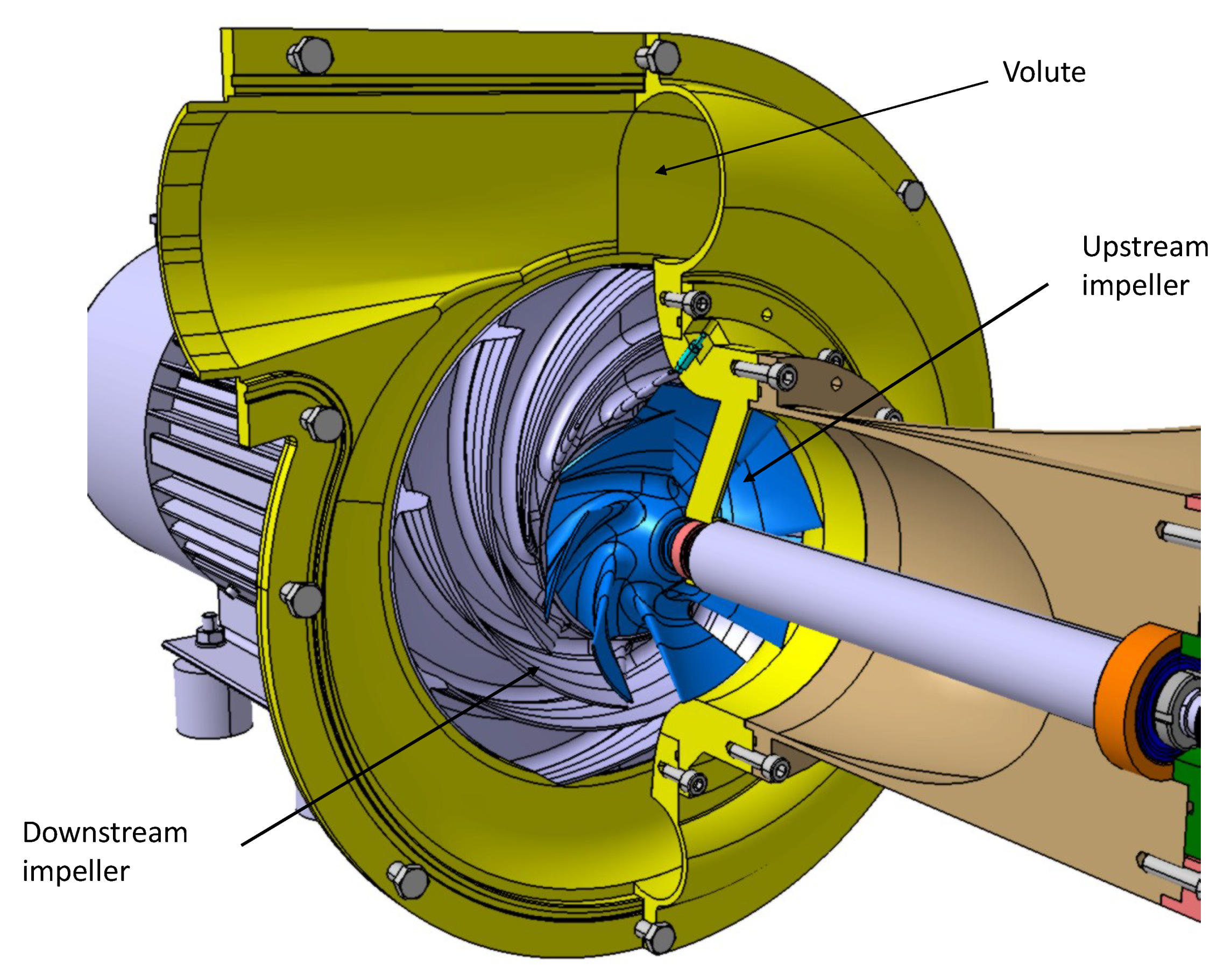













| Parameter | Value | Parameter | Value |
|---|---|---|---|
| 59.5 mm | |||
| 161 mm | |||
| 118 mm | |||
| 176 mm | |||
| 7 |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| 128.5 mm | |||
| 183 mm | |||
| 286 mm | |||
| 9 |
| Mesh model (number of cells) | 2,983,792 |
| −11k rpm | |
| 11k rpm | |
| Inlet temperature | 300 °K |
| Outlet pressure | 101,325 Pa |
| Inlet flow rate | Experimental data |
| Turbulence model | k- SST |
| Interface | Direct (frozen rotor) |
| Time step (for unsteady simulation) | 2.143 × s |
| Mesh 1 | Mesh 2 | Mesh 3 | |
|---|---|---|---|
| Inlet pipe | 74,880 | 185,760 | 308,160 |
| Inlet elbow | 97,737 | 227,858 | 366,104 |
| Impeller 1 | 309,151 | 697,922 | 1,373,753 |
| Impeller 2 | 635,511 | 1,273,090 | 2,139,742 |
| Cavity | 188,911 | 342,755 | 518,865 |
| Volute | 70,711 | 155,067 | 311,061 |
| Outlet pipe | 74,880 | 101,340 | 212,220 |
| Total | 1,430,541 | 2,983,792 | 5,229,905 |
| BSP | NSP | ESP | |
|---|---|---|---|
| Mass flow rate (kg/s) | 0.4568 | 0.15 | 0.066 |
| N1 (rpm) | −10,000 | −6240 | 6240 |
| N2 (rpm) | 10,000 | 10,920 | 12,000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, V.T.; Danlos, A.; Ravelet, F.; Deligant, M.; Solis, M.; Khelladi, S.; Bakir, F. Numerical Analysis of a Novel Twin-Impeller Centrifugal Compressor. Computation 2021, 9, 143. https://doi.org/10.3390/computation9120143
Nguyen VT, Danlos A, Ravelet F, Deligant M, Solis M, Khelladi S, Bakir F. Numerical Analysis of a Novel Twin-Impeller Centrifugal Compressor. Computation. 2021; 9(12):143. https://doi.org/10.3390/computation9120143
Chicago/Turabian StyleNguyen, Van Thang, Amélie Danlos, Florent Ravelet, Michael Deligant, Moises Solis, Sofiane Khelladi, and Farid Bakir. 2021. "Numerical Analysis of a Novel Twin-Impeller Centrifugal Compressor" Computation 9, no. 12: 143. https://doi.org/10.3390/computation9120143
APA StyleNguyen, V. T., Danlos, A., Ravelet, F., Deligant, M., Solis, M., Khelladi, S., & Bakir, F. (2021). Numerical Analysis of a Novel Twin-Impeller Centrifugal Compressor. Computation, 9(12), 143. https://doi.org/10.3390/computation9120143










